A Comparative Study of VLF Transmitter Signal Measurements and Simulations during Two Solar Eclipse Events
Abstract
1. Introduction
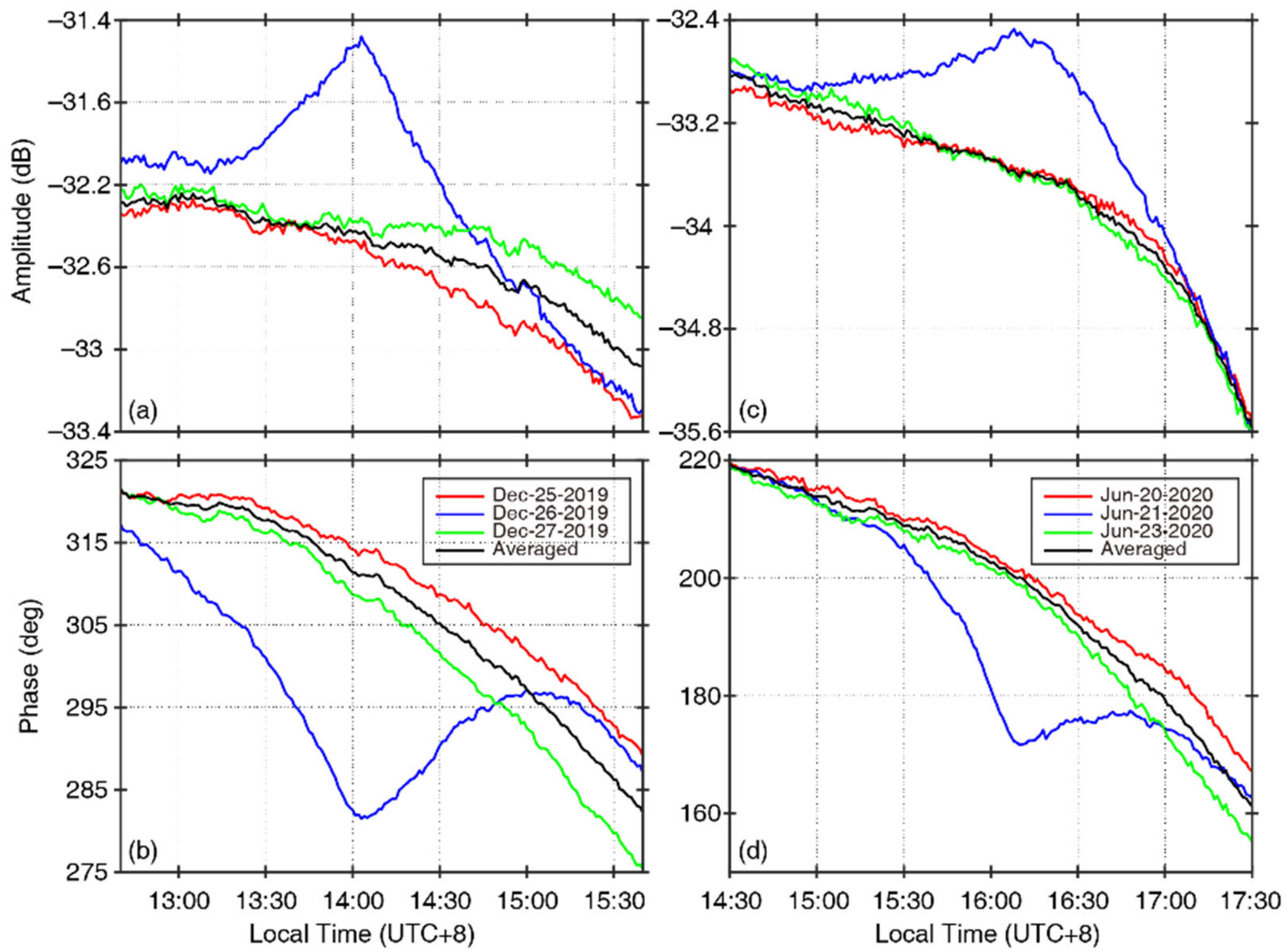
2. VLF Instrument and Eclipse Measurement
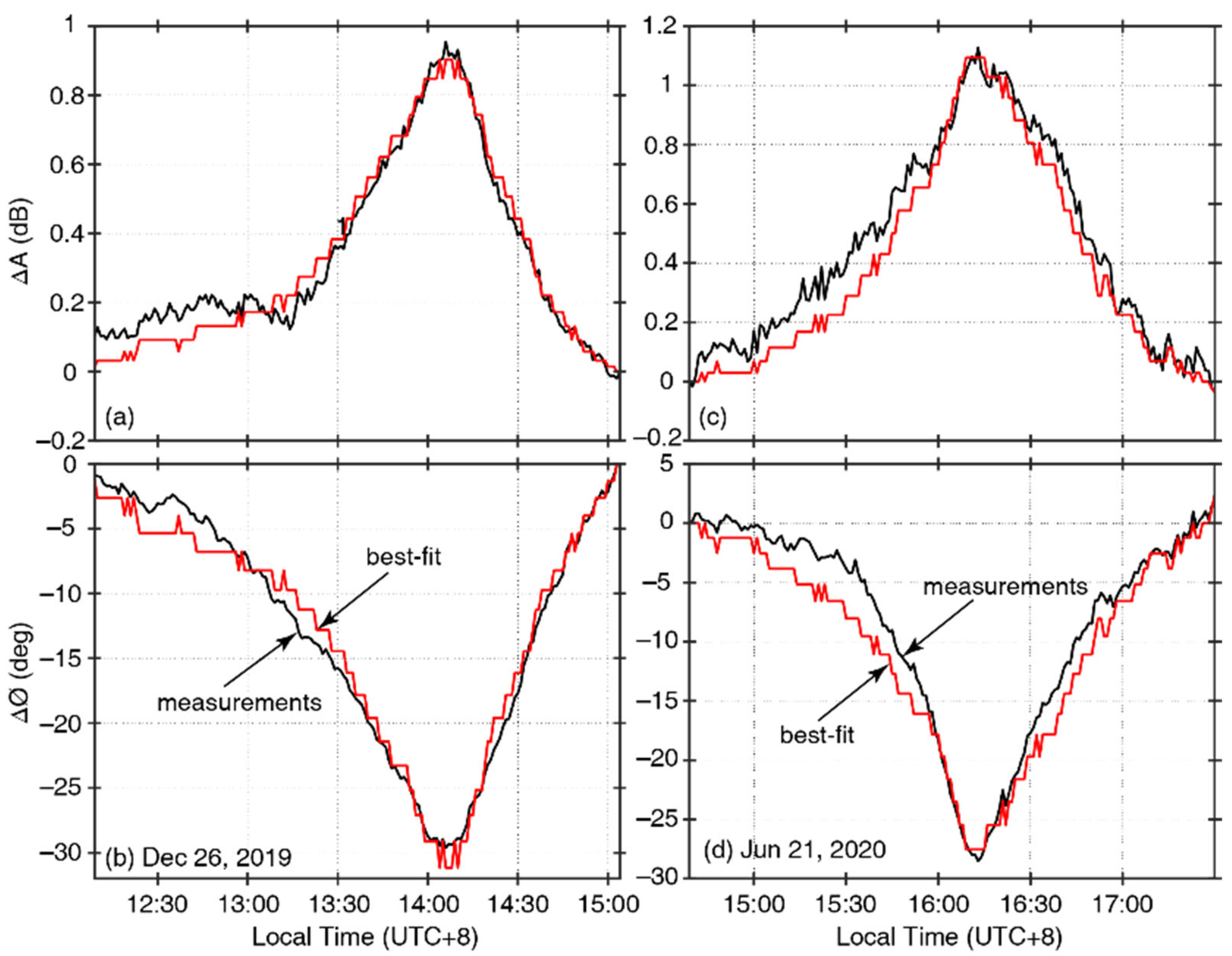
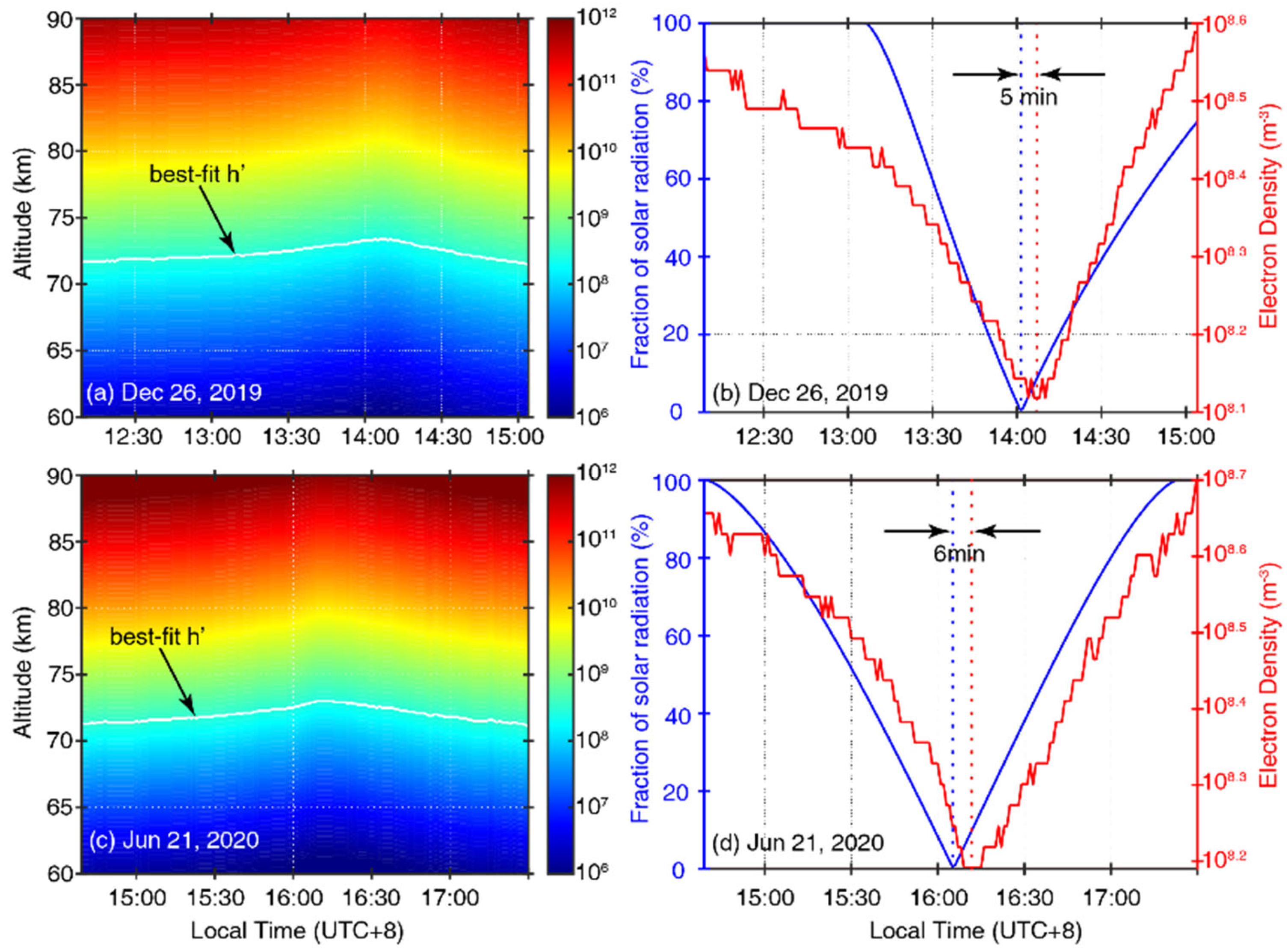
3. Ionospheric Inversion
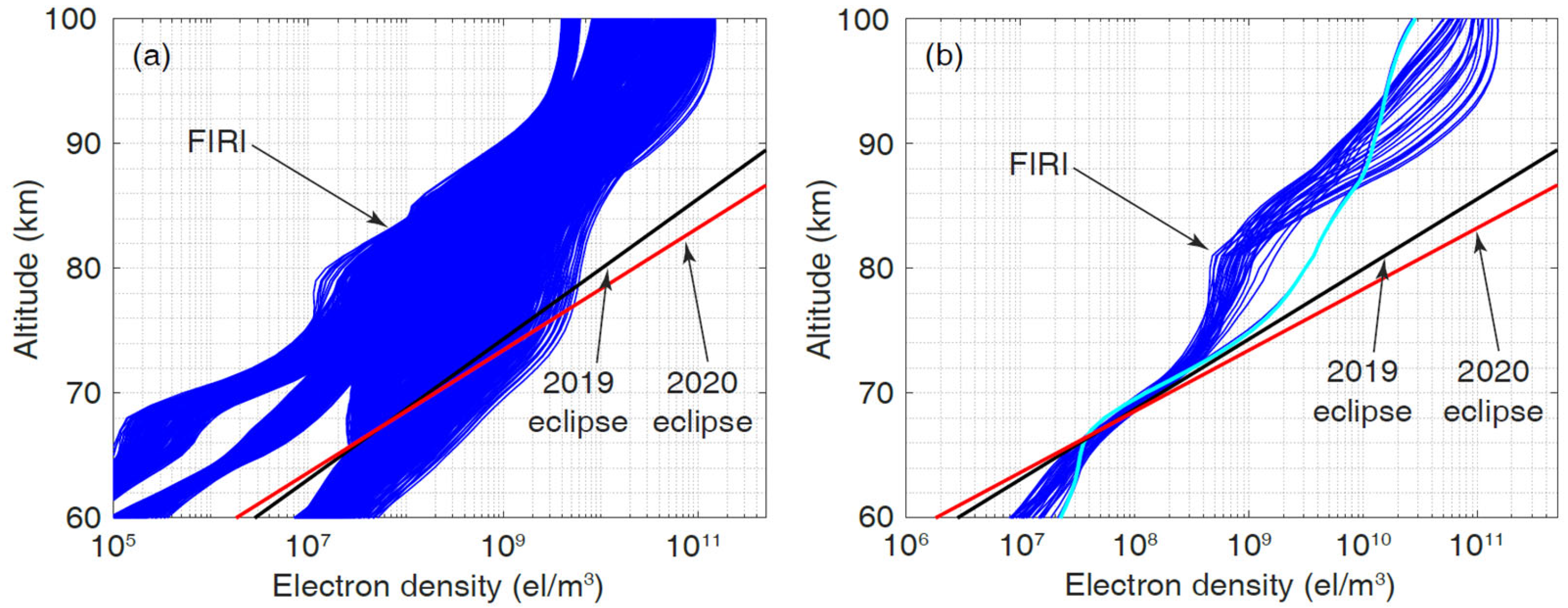
4. Validation of the Inversion Results
5. Conclusions and Discussion
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Brasseur, G.P.; Solomon, S. Aeronomy of the Middle Atmosphere: Chemistry and Physics of the Stratosphere and Mesosphere, 3rd ed.; Springer: Dordrecht, The Netherlands, 2006; pp. 443–531. [Google Scholar]
- Budden, K.G. Radio Waves in the Ionosphere; Cambridge University Press: Cambridge, UK, 2009; pp. 474–781. [Google Scholar]
- Codrescu, M.V.; Fuller-Rowell, T.J.; Roble, R.G.; Evans, D.S. Medium Energy Particle Precipitation Influences on the Mesosphere and Lower Thermosphere. J. Geophys. Res. Space Phys. 1997, 102, 19977–19987. [Google Scholar] [CrossRef]
- Inan, U.S.; Cummer, S.A.; Marshall, R.A. A Survey of ELF and VLF Research on Lightning-Ionosphere Interactions and Causative Discharges. J. Geophys. Res. Space Phys. 2010, 115, A00E36. [Google Scholar] [CrossRef]
- Clilverd, M.A.; Nunn, D.; Lev-Tov, S.J.; Inan, U.S.; Dowden, R.L.; Rodger, C.J.; Smith, A.J. Determining the Size of Lightning-Induced Electron Precipitation Patches. J. Geophys. Res. Space Phys. 2002, 107, SIA 10-1–SIA 10-11. [Google Scholar] [CrossRef]
- Xu, W.; Marshall, R.A.; TyssØy, H.N.; Fang, X. A Generalized Method for Calculating Atmospheric Ionization by Energetic Electron Precipitation. J. Geophys. Res. Space Phys. 2020, 125, e2020JA028482. [Google Scholar] [CrossRef]
- Fishman, G.J.; Inan, U.S. Observation of an Ionospheric Disturbance Caused by a Gamma-Ray Burst. Nature 1988, 331, 418–420. [Google Scholar] [CrossRef]
- Thomson, N.R.; Clilverd, M.A. Solar Flare Induced Ionospheric D-Region Enhancements from VLF Amplitude Observations. J. Atmos. Sol.-Terr. Phys. 2001, 63, 1729–1737. [Google Scholar] [CrossRef]
- Inan, U.S.; Bell, T.F.; Rodriguez, J.V. Heating and Ionization of the Lower Ionosphere by Lightning. Geophys. Res. Lett. 1991, 18, 705–708. [Google Scholar] [CrossRef]
- Rodger, C.J.; Cho, M.; Clilverd, M.A.; Rycroft, M.J. Lower Ionospheric Modification by Lightning-EMP: Simulation of the Night Ionosphere over the United States. Geophys. Res. Lett. 2001, 28, 199–202. [Google Scholar] [CrossRef]
- Hosseini, P.; Gołkowski, M.; Hared, V. Remote Sensing of Radiation Belt Energetic Electrons Using Lightning Triggered Upper Band Chorus. Geophys. Res. Lett. 2019, 46, 37–47. [Google Scholar] [CrossRef]
- Xu, W.; Marshall, R.A.; Kero, A.; Sousa, A. Chemical Response of the Upper Atmosphere Due to Lightning-Induced Electron Precipitation. J. Geophys. Res. Atmos. 2021, 126, e2021JD034914. [Google Scholar] [CrossRef]
- Fritts, D.; Alexander, M. Gravity Wave Dynamics and Effects in the Middle Atmosphere. Rev. Geophys. 2003, 41, 1003. [Google Scholar] [CrossRef]
- Bortnick, J.; Inan, U.S.; Bell, T.F. Temporal Signatures of Radiation Belt Electron Precipitation Induced by Lightning-Generated MR Whistler Waves: 2. Global Signatures. J. Geophys. Res. Space Phys. 2006, 111, A02204. [Google Scholar] [CrossRef]
- Xu, W.; Marshall, R.A.; Fang, X.; Turunen, E.; Kero, A. On the Effects of Bremsstrahlung Radiation During Energetic Electron Precipitation. Geophys. Res. Lett. 2018, 45, 1167–1176. [Google Scholar] [CrossRef]
- Ni, B.; Hua, M.; Gu, X.; Fu, S.; Xiang, Z.; Cao, X.; Ma, X. Artificial Modification of Earth’s Radiation Belts by Ground-Based Very-Low-Frequency (VLF) Transmitters. Sci. China Earth Sci. 2022, 65, 391–413. [Google Scholar] [CrossRef]
- Hua, M.; Li, W.; Ni, B.; Ma, Q.; Green, A.; Shen, X.; Claudepierre, S.G.; Bortnik, J.; Gu, X.; Fu, S.; et al. Very-Low-Frequency Transmitters Bifurcate Energetic Electron Belt in near-Earth Space. Nat. Common. 2020, 11, 4847. [Google Scholar] [CrossRef]
- Xu, W.; Marshall, R.A.; Kero, A.; Turunen, E.; Drob, D.; Sojka, J.; Rice, D. VLF Measurements and Modeling of the D-Region Response to the 2017 Total Solar Eclipse. IEEE Trans. Geosci. Remote Sens. 2019, 57, 7613–7622. [Google Scholar] [CrossRef]
- Han, F.; Cummer, S.A. Midlatitude Daytime D Region Ionosphere Variations Measured from Radio Atmospherics. J. Geophys. Res. Space Phys. 2010, 115, A10314. [Google Scholar] [CrossRef]
- Barr, R.; Jones, D.L.; Rodger, C.J. ELF and VLF Radio Waves. J. Atmos. Sol.-Terr. Phys. 2000, 62, 1689–1718. [Google Scholar] [CrossRef]
- Hardman, S.F.; Rodger, C.J.; Dowden, R.L.; Brundell, J.B. Measurements of the VLF Scattering Pattern of the Structured Plasma of Red Sprites. IEEE Antennas Propag. Mag. 1998, 40, 29–38. [Google Scholar] [CrossRef]
- Cummer, S.A.; Inan, U.S.; Bell, T.F. Ionospheric D Region Remote Sensing Using VLF Radio Atmospherics. Radio Sci. 1998, 33, 1781–1792. [Google Scholar] [CrossRef]
- Cohen, M.B.; Inan, U.S.; Fishman, G. Terrestrial Gamma Ray Flashes Observed Aboard the Compton Gamma Ray Observatory/Burst and Transient Source Experiment and ELF/VLF Radio Atmospherics. J. Geophys. Res. Atmos. 2006, 111, D24109. [Google Scholar] [CrossRef]
- Cohen, M.B.; Inan, U.S.; Paschal, E.W. Sensitive Broadband ELF/VLF Radio Reception with the AWESOME Instrument. IEEE Trans. Geosci. Remote Sens. 2010, 48, 3–17. [Google Scholar] [CrossRef]
- Belcher, S.R.G.; Clilverd, M.A.; Rodger, C.J.; Cook, S.; Thomson, N.R.; Brundell, J.B.; Raita, T. Solar Flare X-Ray Impacts on Long Sub ionospheric VLF Paths. Space Weather 2021, 19, e2021SW002820. [Google Scholar] [CrossRef]
- Schaal, R.E.; Mendes, A.M.; Ananthakrishnan, S.; Kaufmann, P. VLF Propagation Effects Produced by the Eclipse. Nature 1970, 226, 1127–1129. [Google Scholar] [CrossRef]
- Bracewell, R.N. Theory of Formation of an Ionospheric Layer below E Layer Based on Eclipse and Solar Flare Effects at 16 Kc/Sec. J. Atmos. Terr. Phys. 1952, 2, 226–235. [Google Scholar] [CrossRef]
- Lynn, K.J.W. The Total Solar Eclipse of 23 October 1976 Observed at VLF. J. Atmos. Terr. Phys. 1981, 43, 1309–1316. [Google Scholar] [CrossRef]
- Sears, R.D.; Heaps, M.G.; Niles, F.E. Modeling the Ion Chemistry of the D Region: A Case Study Based upon the 1966 Total Solar Eclipse. J. Geophys. Res. Space Phys. 1981, 86, 10073–10086. [Google Scholar] [CrossRef]
- Clilverd, M.A.; Rodger, C.J.; Thomson, N.R.; Lichtenberger, J.; Steinbach, P.; Cannon, P.; Angling, M.J. Total Solar Eclipse Effects on VLF Signals: Observations and Modeling. Radio Sci. 2001, 36, 773–788. [Google Scholar] [CrossRef]
- Kumar, S.; Kumar, A.; Maurya, A.K.; Singh, R. Changes in the D Region Associated with Three Recent Solar Eclipses in the South Pacific Region. J. Geophys. Res. Space Phys. 2016, 121, 5930–5943. [Google Scholar] [CrossRef]
- Singh, R.; Veenadhari, B.; Maurya, A.K.; Cohen, M.B.; Kumar, S.; Selvakumaran, R.; Pant, P.; Singh, A.K.; Inan, U.S. D-Region Ionosphere Response to the Total Solar Eclipse of 22 July 2009 Deduced from ELF-VLF Tweek Observations in the Indian Sector. J. Geophys. Res. Space Phys. 2011, 116, A10301. [Google Scholar] [CrossRef]
- Pal, S.; Chakrabarti, S.K.; Mondal, S.K. Modeling of Sub-Ionospheric VLF Signal Perturbations Associated with Total Solar Eclipse, 2009 in Indian Subcontinent. Adv. Space Res. 2012, 50, 196–204. [Google Scholar] [CrossRef]
- Cohen, M.B.; Gross, N.C.; Higginson-Rollins, M.A.; Marshall, R.A.; Gołkowski, M.; Liles, W.; Rodriguez, D.; Rockway, J. The Lower Ionospheric VLF/LF Response to the 2017 Great American Solar Eclipse Observed Across the Continent. Geophys. Res. Lett. 2018, 45, 3348–3355. [Google Scholar] [CrossRef]
- Tripathi, G.; Singh, S.B.; Kumar, S.; Singh, A.K.; Singh, R.; Singh, A.K. Effect of 21 June 2020 Solar Eclipse on the Ionosphere Using VLF and GPS Observations and Modeling. Adv. Space Res. 2022, 69, 254–265. [Google Scholar] [CrossRef]
- Chen, Y.; Yang, G.; Ni, B.; Zhao, Z.; Gu, X.; Zhou, C.; Wang, F. Development of Ground-Based ELF/VLF Receiver System in Wuhan and Its First Results. Adv. Space Res. 2016, 57, 1871–1880. [Google Scholar] [CrossRef]
- Chen, Y.; Ni, B.; Gu, X.; Zhao, Z.; Yang, G.; Zhou, C.; Zhang, Y. First Observations of Low Latitude Whistlers Using WHU ELF/VLF Receiver System. Sci. China Technol. Sci. 2017, 60, 166–174. [Google Scholar] [CrossRef]
- Wang, S.W.; Gu, X.D.; Luo, F.; Peng, R.; Chen, H.; Li, G.; Ni, B.; Zhao, Z.Y.; Yuan, D. Observations and analyses of the sunrise effect for NWC VLF transmitter signals. Chin. J. Geophys. 2020, 63, 4300–4311. [Google Scholar] [CrossRef]
- Gu, X.; Li, G.; Pang, H.; Wang, S.; Ni, B.; Luo, F.; Peng, R.; Chen, L. Statistical Analysis of Very Low Frequency Atmospheric Noise Caused by the Global Lightning Using Ground-Based Observations in China. J. Geophys. Res. Space Phys. 2021, 126, e2020JA029101. [Google Scholar] [CrossRef]
- Yi, J.; Gu, X.; Cheng, W.; Tang, X.; Chen, L.; Ni, B.; Zhou, R.; Zhao, Z.; Wang, Q.; Zhou, L. A Detailed Investigation of Low Latitude Tweek Atmospherics Observed by the WHU ELF/VLF Receiver: 2. Occurrence Features and Associated Ionospheric Parameters. Earth Planet. Phys. 2020, 4, 238–245. [Google Scholar] [CrossRef]
- Zhou, R.; Gu, X.; Yang, K.; Li, G.; Ni, B.; Yi, J.; Chen, L.; Zhao, F.; Zhao, Z.; Wang, Q.; et al. A Detailed Investigation of Low Latitude Tweek Atmospherics Observed by the WHU ELF/VLF Receiver: I. Automatic Detection and Analysis Method. Earth Planet. Phys. 2020, 4, 120–130. [Google Scholar] [CrossRef]
- Gu, X.; Wang, Q.; Ni, B.; Xu, W.; Wang, S.; Yi, J.; Hu, Z.; Li, B.; He, F.; Chen, X.; et al. First Results of the Wave Measurements by the WHU VLF Wave Detection System at the Chinese Great Wall Station in Antarctica. J. Geophys. Res. Space Phys. 2022, 127, e2022JA030784. [Google Scholar] [CrossRef]
- Pasupathy, S. Minimum Shift Keying: A Spectrally Efficient Modulation. IEEE Commun. Mag. 1979, 17, 14–22. [Google Scholar] [CrossRef]
- Paschal, E.W. Phase Measurements of Very-Low-Frequency Signals from the Magnetosphere; Stanford University: Stanford, CA, USA, 1988. [Google Scholar]
- Gross, N.C.; Cohen, M.B.; Said, R.K.; Gołkowski, M. Polarization of Narrowband VLF Transmitter Signals as an Ionospheric Diagnostic. J. Geophys. Res. Space Phys. 2018, 123, 901–917. [Google Scholar] [CrossRef]
- Chakrabarti, S.K.; Sasmal, S.; Basak, T.; Chakraborty, S.; Tucker, R.L. Modeling D-Region Ionospheric Response of the Great American TSE of August 21, 2017 from VLF Signal Perturbation. Adv. Space Res. 2018, 62, 651–661. [Google Scholar] [CrossRef]
- Thomson, N.R. Experimental Daytime VLF Ionospheric Parameters. J. Atmos. Terr. Phys. 1993, 55, 173–184. [Google Scholar] [CrossRef]
- Thomson, N.R.; Clilverd, M.A.; McRae, W.M. Nighttime Ionospheric D Region Parameters from VLF Phase and Amplitude. J. Geophys. Res. Space Phys. 2007, 112, A07304. [Google Scholar] [CrossRef]
- McCrae, W.; Thomson, N. VLF Phase and Amplitude: Daytime Ionospheric Parameters. J. Atmos. Sol.-Terr. Phys. 2000, 62, 609–618. [Google Scholar] [CrossRef]
- Wait, J.R. Characteristics of the Earth-Ionosphere Waveguide for VLF Radio Waves; National Bureau of Standards: Gaithersburg, MD, USA, 1964; p. NBS TN 300.
- Xu, W.; Marshall, R.A.; Bortnik, J.; Bunnell, J.W. An Electron Density Model of the D- and E-Region Ionosphere for Trans ionospheric VLF Propagation. J. Geophys. Res. Space Phys. 2021, 126, e2021JA029288. [Google Scholar] [CrossRef]
- Gołkowski, M.; Renick, C.; Cohen, M.B. Quantification of Ionospheric Perturbations from Lightning Using Overlapping Paths of VLF Signal Propagation. J. Geophys. Res. Space Phys. 2021, 126, e2020JA028540. [Google Scholar] [CrossRef]
- Marshall, R.A. An Improved Model of the Lightning Electromagnetic Field Interaction with the D-Region Ionosphere. J. Geophys. Res. Space Phys. 2012, 117, A03316. [Google Scholar] [CrossRef]
- Marshall, R.A.; Wallace, T.; Turbe, M. Finite-Difference Modeling of Very-Low-Frequency Propagation in the Earth-Ionosphere Waveguide. IEEE Trans. Antennas Propag. 2017, 65, 7185–7197. [Google Scholar] [CrossRef]
- Marshall, R.A.; Snively, J.B. Very Low Frequency Sub Ionospheric Remote Sensing of Thunderstorm-Driven Acoustic Waves in the Lower Ionosphere. J. Geophys. Res. Atmos. 2014, 119, 5037–5045. [Google Scholar] [CrossRef]
- Marshall, R.A.; Xu, W.; Sousa, A.; McCarthy, M.; Millan, R. X-Ray Signatures of Lightning-Induced Electron Precipitation. J. Geophys. Res. Space Phys. 2019, 124, 10230–10245. [Google Scholar] [CrossRef]
- Ferguson, J.A. Computer Programs for Assessment of Long-Wavelength Radio Communications, Version 2.0: User’s Guide and Source Files; Space and Naval Warfare Systems Center: San Diego, CA, USA, 1998. [Google Scholar]
- Lehtinen, N.G.; Inan, U.S. Full-Wave Modeling of Trans ionospheric Propagation of VLF Waves. Geophys. Res. Lett. 2009, 36, L03104. [Google Scholar] [CrossRef]
- Gasdia, F.; Marshall, R.A. A New Longwave Mode Propagator for the Earth–Ionosphere Waveguide. IEEE Trans. Antennas Propag. 2021, 69, 8675–8688. [Google Scholar] [CrossRef]
- Friedrich, M.; Torkar, K.M. FIRI: A Semiempirical Model of the Lower Ionosphere. J. Geophys. Res. Space Phys. 2001, 106, 21409–21418. [Google Scholar] [CrossRef]
- McCormick, J.C.; Cohen, M.B. A New Four-Parameter D-Region Ionospheric Model: Inferences from Lightning-Emitted VLF Signals. J. Geophys. Res. Space Phys. 2021, 126, e2021JA029849. [Google Scholar] [CrossRef]
- Shao, X.M.; Lay, E.H.; Jacobson, A.R. Reduction of Electron Density in the Night-Time Lower Ionosphere in Response to a Thunderstorm. Nat. Geosocial. 2013, 6, 29–33. [Google Scholar] [CrossRef]
- Mechtly, E.A.; Seino, K.; Smith, L.G. Lower Ionosphere Electron Densities Measured During the Solar Eclipse of November 12, 1966. Radio Sci. 1969, 4, 371–375. [Google Scholar] [CrossRef]
- Guha, A.; De, B.K.; Roy, R.; Choudhury, A. Response of the Equatorial Lower Ionosphere to the Total Solar Eclipse of 22 July 2009 during Sunrise Transition Period Studied Using VLF Signal. J. Geophys. Res. Space Phys. 2010, 115, A11302. [Google Scholar] [CrossRef]
- Turunen, E.; Matveinen, H.; Tolvanen, J.; Ranta, H. D-region ion chemistry model. In STEP Handbook of Ionospheric Models; Schunk, R.W., Ed.; SCOSTEP Secretariat: Boulder, CO, USA, 1996; pp. 1–25. [Google Scholar]
- Verronen, P.T.; Andersson, M.E.; Marsh, D.R.; Kovács, T.; Plane, J.M.C. WACCM-D—Whole Atmosphere Community Climate Model with D-Region Ion Chemistry. J. Adv. Model. Earth Syst. 2016, 8, 954–975. [Google Scholar] [CrossRef]
- Phanikumar, D.V.; Kwak, Y.S.; Patra, A.K.; Maurya, A.K.; Singh, R.; Park, S.M. Response of the Mid-Latitude D-Region Ionosphere to the Total Solar Eclipse of 22 July 2009 Studied Using VLF Signals in South Korean Peninsula. Adv. Space Res. 2014, 54, 961–968. [Google Scholar] [CrossRef]
- Venkatesham, K.; Singh, R.; Maurya, A.K.; Dube, A.; Kumar, S.; Phanikumar, D.V. The 22 July 2009 Total Solar Eclipse: Modeling D Region Ionosphere Using Narrowband VLF Observations. J. Geophys. Res. Space Phys. 2019, 124, 616–627. [Google Scholar] [CrossRef]
- Pant, P.; Mahra, H. Effect of solar eclipse on VLF propagation. Indian J. Radio Space Phys. 1994, 23, 399–402. [Google Scholar]
- Clilverd, M.A.; Rodger, C.J.; Nunn, D. Radiation Belt Electron Precipitation Fluxes Associated with Lightning. J. Geophys. Res. Space Phys. 2004, 109, A12208. [Google Scholar] [CrossRef]

| Studies | h′ (km) | β (km−1) | ∆h′ (km) | ∆β (km−1) |
|---|---|---|---|---|
| Clilverd et al. [30] | 79 | 0.5 | 8 | 0.07 |
| Guha et al. [64] | 74.5 | 0.46 | 3.5 | 0.03 |
| Pal et al. [33] 1 | 78 | 0.34 | 4 | 0.04 |
| 75.8 | 0.32 | 1.8 | 0.02 | |
| 77.8 | 0.37 | 1.8 | 0.02 | |
| 77 | 0.32 | 3.0 | 0.02 | |
| Phanikumar et al. [67] | 77 | 0.485 | 7 | 0.055 |
| Kumar et al. [31] 2 | 71.7 | 0.402 | 0.6 | 0.012 |
| 71 | 0.405 | 0.5 | 0.015 | |
| 72 | 0.34 | 1.5 | −0.055 | |
| Venkatesham et al. [68] 3 | 80 | 0.4 | 3 | 0.02 |
| 80.7 | 0.36 | 2.3 | 0.02 | |
| 80 | 0.38 | 3 | 0.02 | |
| 72.4 | 0.44 | 2.4 | 0.01 | |
| Tripathi et al. [35] | 71.4 | 0.43 | 0.4 | 0.028 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cheng, W.; Xu, W.; Gu, X.; Wang, S.; Wang, Q.; Ni, B.; Lu, Z.; Xiao, B.; Meng, X. A Comparative Study of VLF Transmitter Signal Measurements and Simulations during Two Solar Eclipse Events. Remote Sens. 2023, 15, 3025. https://doi.org/10.3390/rs15123025
Cheng W, Xu W, Gu X, Wang S, Wang Q, Ni B, Lu Z, Xiao B, Meng X. A Comparative Study of VLF Transmitter Signal Measurements and Simulations during Two Solar Eclipse Events. Remote Sensing. 2023; 15(12):3025. https://doi.org/10.3390/rs15123025
Chicago/Turabian StyleCheng, Wen, Wei Xu, Xudong Gu, Shiwei Wang, Qingshan Wang, Binbin Ni, Zilong Lu, Binxiao Xiao, and Xiaoyu Meng. 2023. "A Comparative Study of VLF Transmitter Signal Measurements and Simulations during Two Solar Eclipse Events" Remote Sensing 15, no. 12: 3025. https://doi.org/10.3390/rs15123025
APA StyleCheng, W., Xu, W., Gu, X., Wang, S., Wang, Q., Ni, B., Lu, Z., Xiao, B., & Meng, X. (2023). A Comparative Study of VLF Transmitter Signal Measurements and Simulations during Two Solar Eclipse Events. Remote Sensing, 15(12), 3025. https://doi.org/10.3390/rs15123025







