Non-Least Square GNSS Positioning Algorithm for Densely Urbanized Areas
Abstract
:1. Introduction
2. Material and Methods
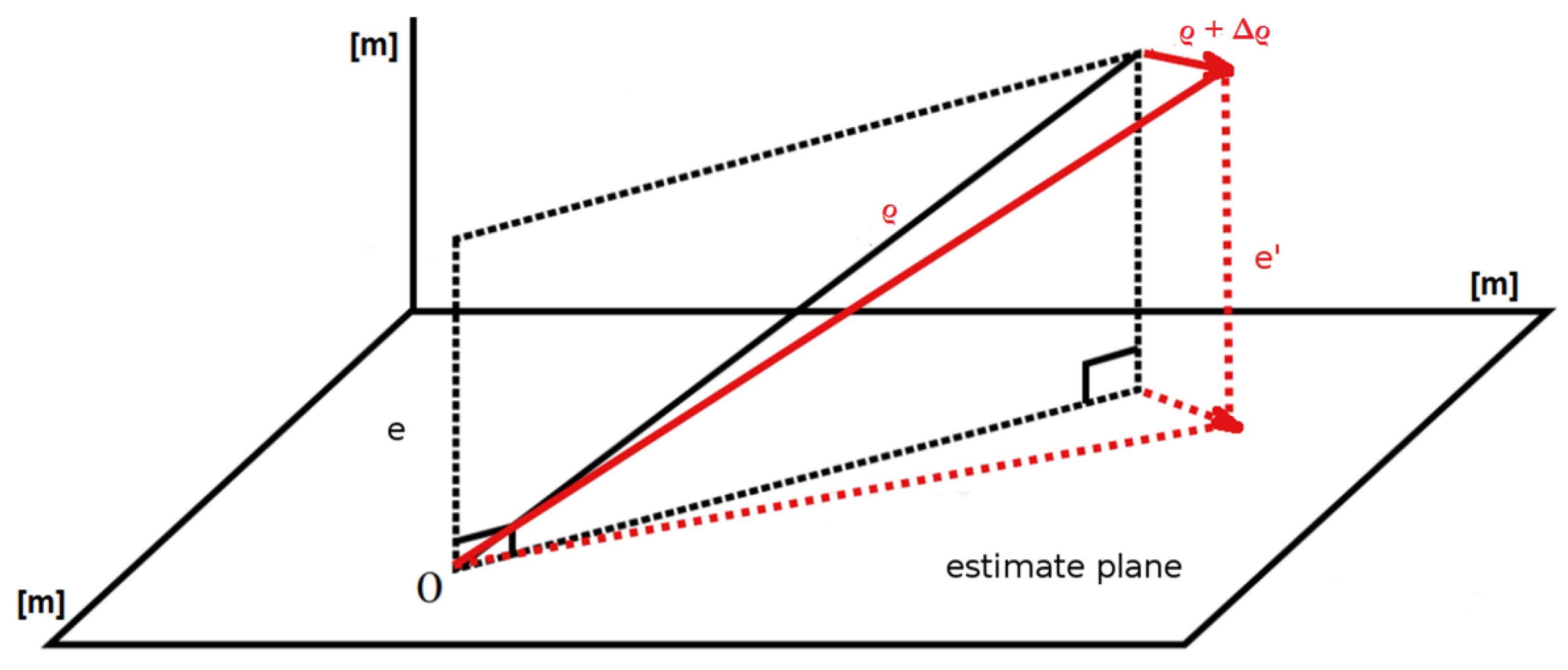
2.1. New GNSS Position Calculation Algorithm Details
| Algorithm 1: Simplified Pseudocode Representation of the Proposed Algorithm. |
| Initialization xyzK, xyzKs, xyzMed sets calculate receiver position xyzK using 4 satellites save calculated receiver position to memory, xyzKs = [xyzKs xyzK] EndFor find xyzMed = median of xyzKs; end |
2.2. Numerical Example
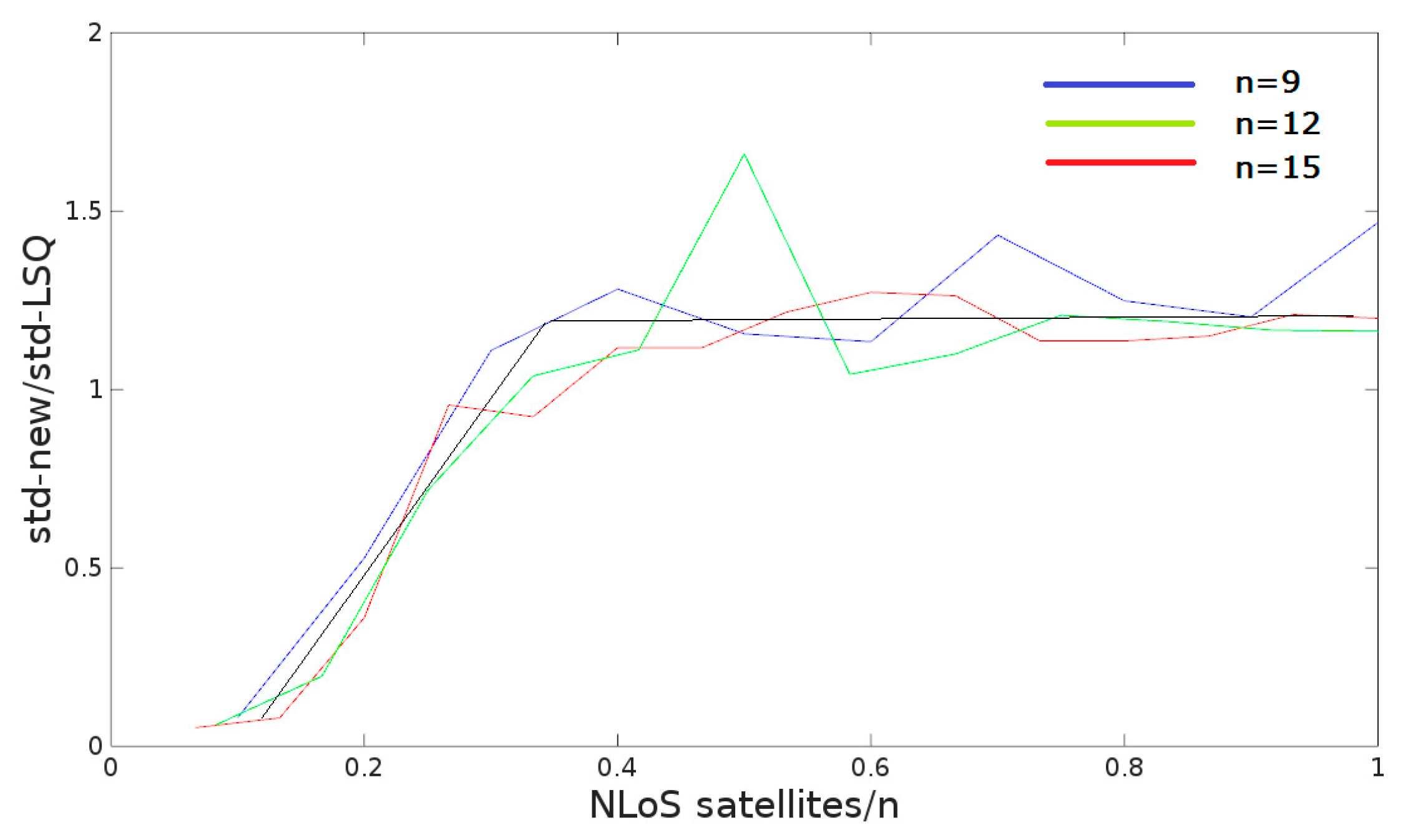
2.3. Algorithm Verification Using Simulation
| Algorithm 2: Algorithm Test Pseudocode Representation. |
| Initialization of xyzLsqs, xyzMeds For i = number of iterations Initialization xyzLsq, xyzMed, recPos k = 8; number of available satellites n = 2; number of multipath satellites generate or choose satellites position add randomly multipath distance for n randomly chosen pseudoranges calculate receiver position xyzLsq using analytic or iteration method xyzLsqs = [xyzLsqs xyzLsq]; save calculated xyzLsq receiver position calculate receiver position xyzMed using Algorithm 1 save xyzMed, xyzMeds = [xyzMeds xyzMed] EndFor calculate standard error of xyzLsqs, xyzMeds to be compared |
3. Results
3.1. Algorithm Testing
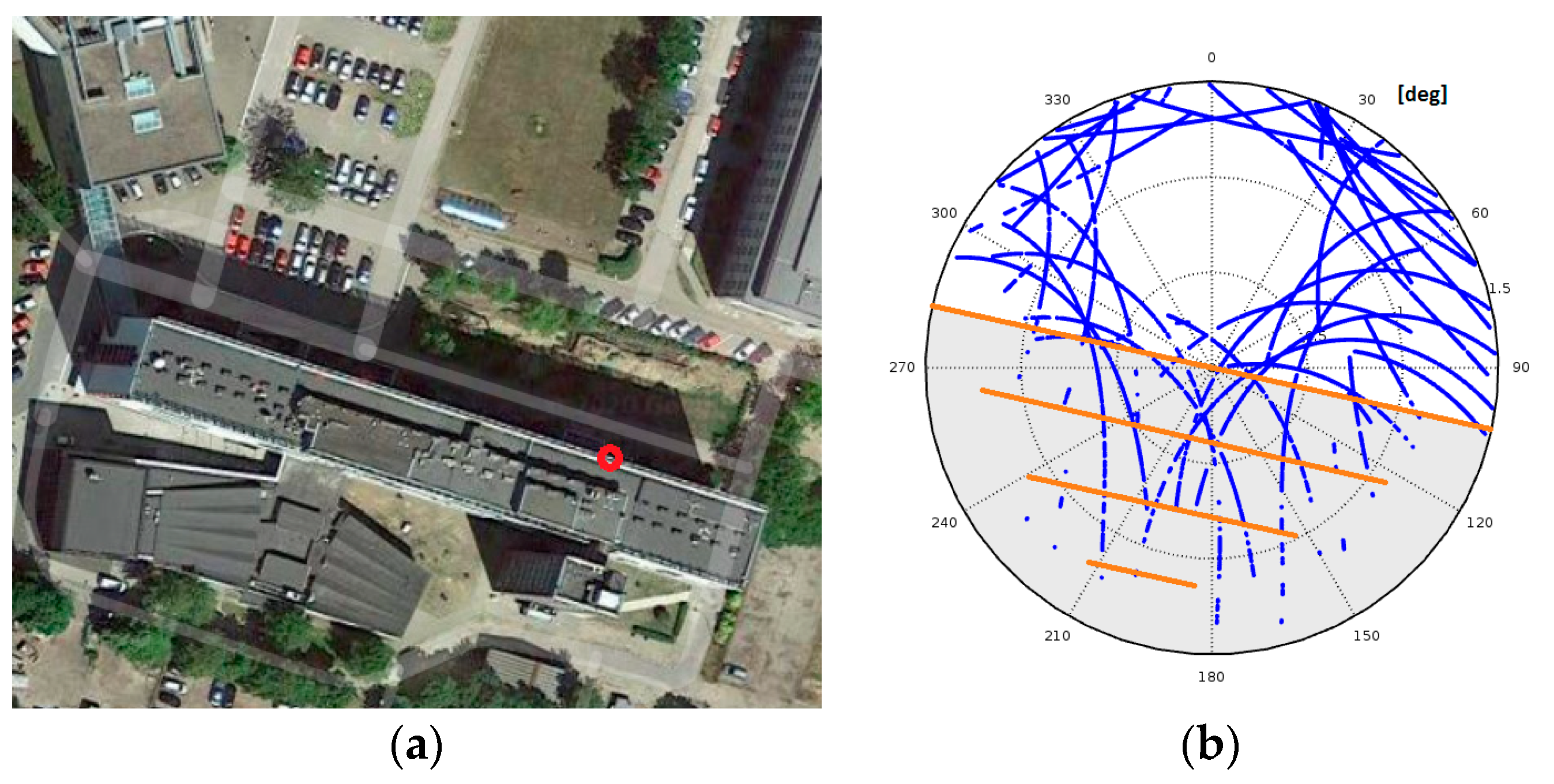
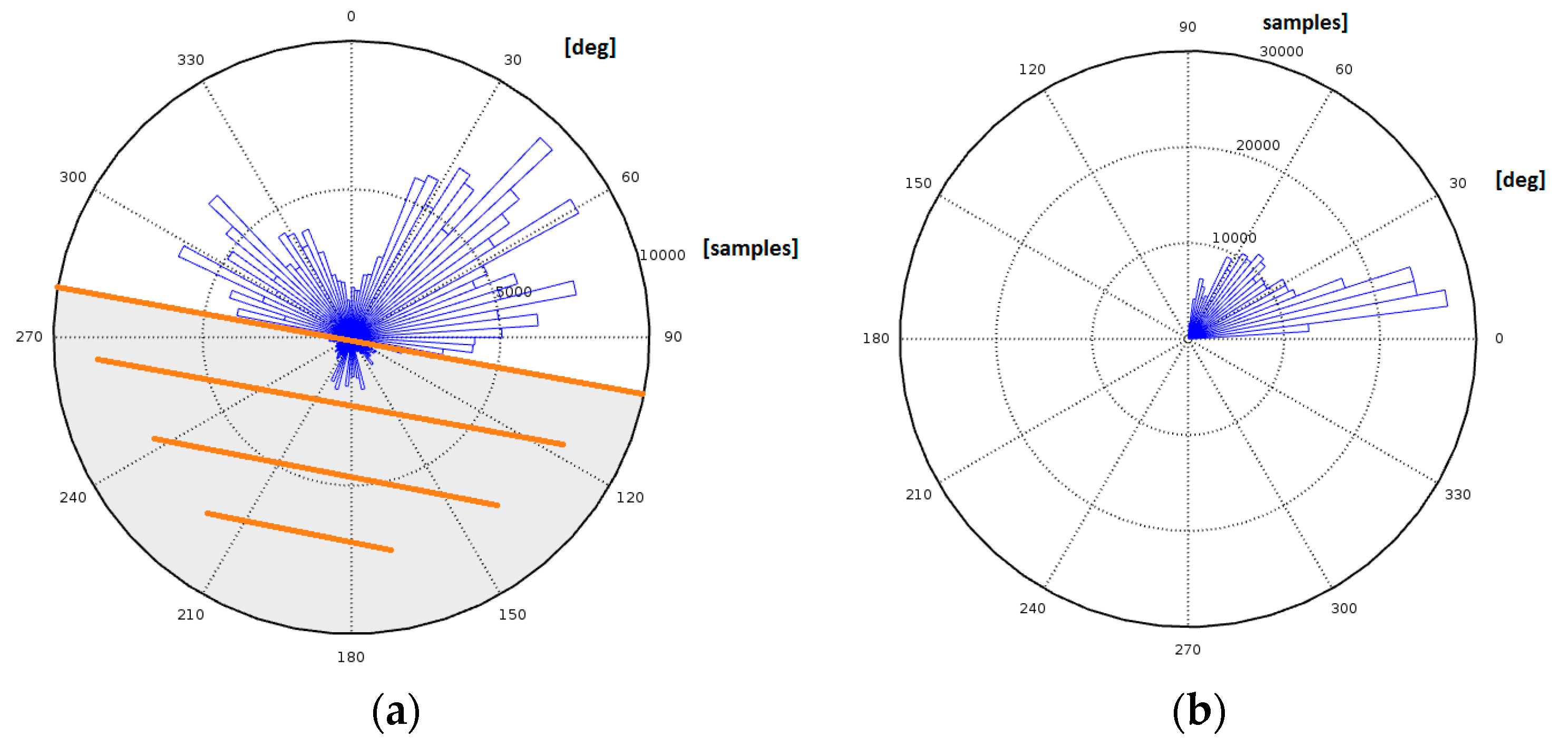
3.2. Environment Conditions
3.3. Algorithm Testing in Open Area in Static Conditions
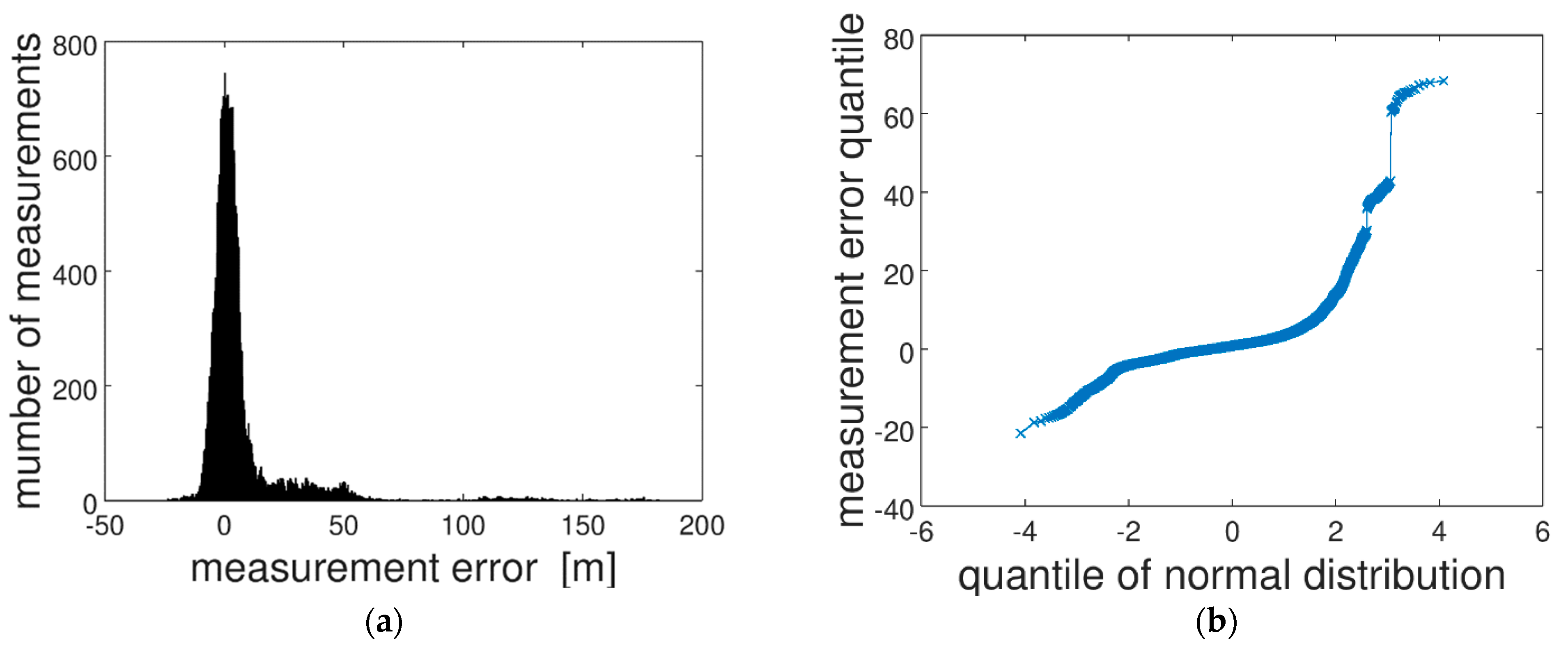
3.4. Static Testing Results of the Proposed Method in an Urban Area
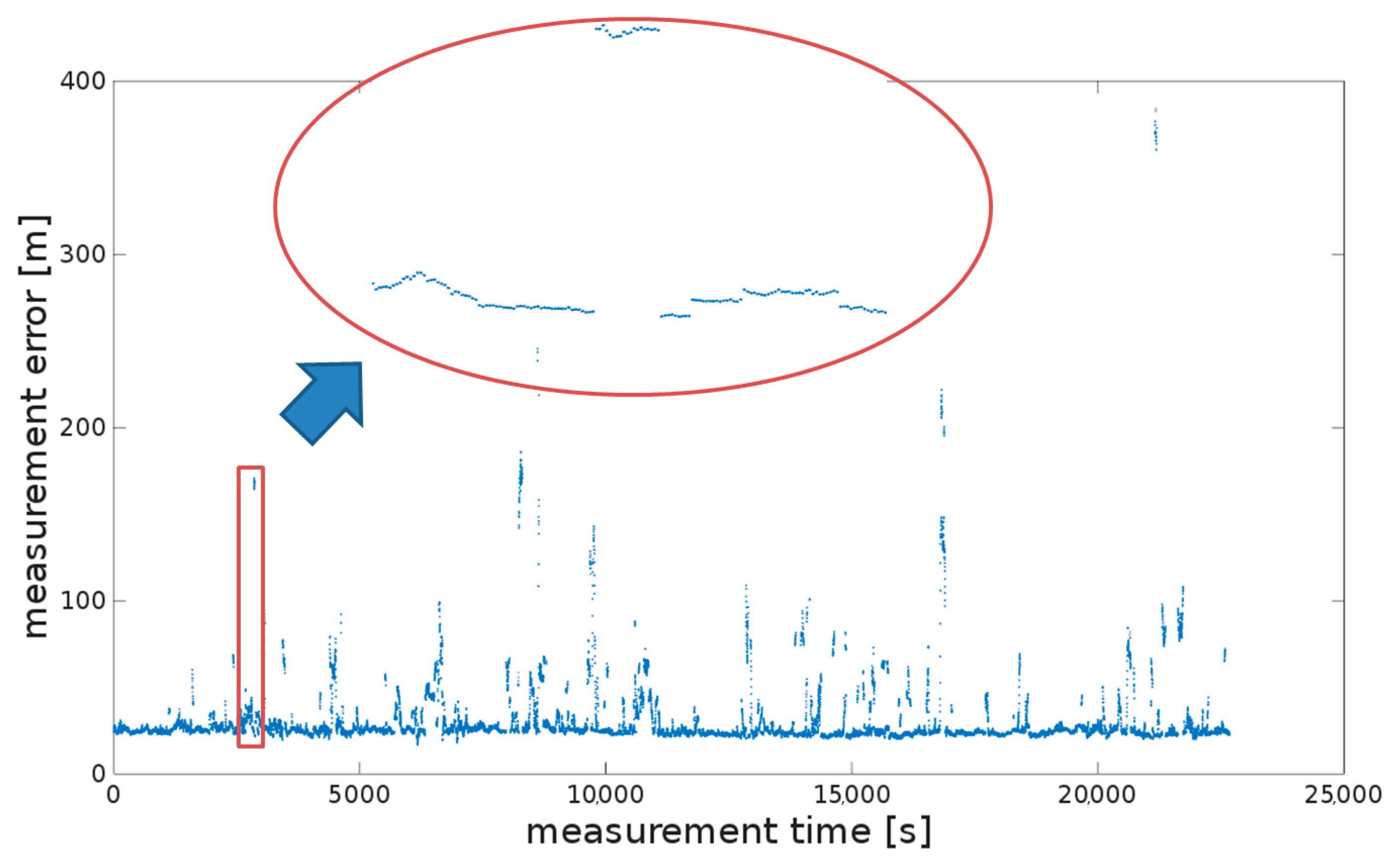
3.5. Long-Term Static Verification Using Low-Cost Receiver
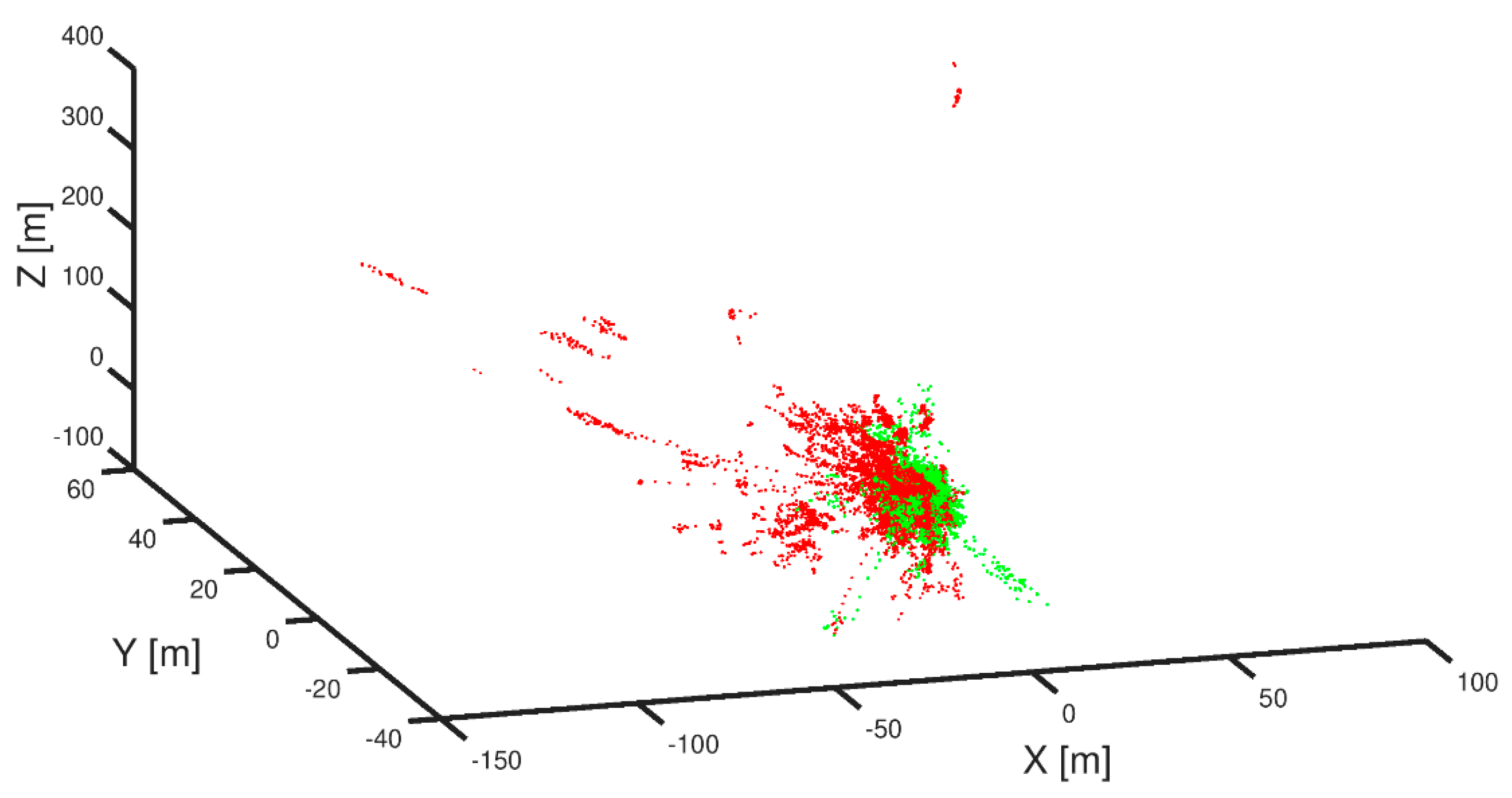
3.6. Application of New Method in Urbanized Area—Dynamic Measurements
4. Discussion
5. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lin, T.; Ma, M.; Broumandan, A.; Lachapelle, G. Demonstration of a High Sensitivity GNSS Software Receiver for Indoor Positioning. Adv. Space Res. 2013, 51, 1035–1045. [Google Scholar] [CrossRef]
- Dierendonck, A.J.V.; Fenton, P.; Ford, T. Theory and performance of narrow correlator spacing in a GPS receiver. J. Inst. Navig. 1992, 39, 265–283. [Google Scholar] [CrossRef]
- Garlin, L.; Van Digelen, F.; Rousseau, J.M. Strobe and edge correlator multipathmitigation for code. In Proceedings of the 9th International Technical Meeting of the Satellite Division of the Institute of Navigation, Kansas City, MO, USA, 17–20 September 1996. [Google Scholar]
- Van Nee, D.J.; Siereveld, J.; Fenton, P.C.; Townsend, B.R. The multipath estimating delay lock loop: Approaching theoretical accuracy limits. In Proceedings of the 1994 IEEE Position, Location and Navigation Symposium-PLANS’94, Las Vegas, NV, USA, 11–15 April 1994. [Google Scholar]
- Vagle, N.; Broumandan, A.; Jafarnia-Jahromi, A.; Lachapelle, G. Performance analysis of GNSS multipath mitigation using antenna arrays. J. Glob. Position. Syst. 2016, 14, 4. [Google Scholar] [CrossRef] [Green Version]
- Fernández-Prades, C.; Closas, P.; Arribas, J. Eigenbeamforming for interferencemitigation in GNSS receivers. In Proceedings of the 1st International Conference on Localization and GNSS (ICLGNSS’11), Tampere, Finland, 29–30 June 2011; pp. 93–97. [Google Scholar]
- Gupta, I.J.; Weiss, I.M.; Morrison, A.W. Desired features of adaptive antennaarrays for GNSS receivers. Proc. IEEE 2016, 99, 1–12. [Google Scholar]
- Sokhandan, N.; Ziedan, N.; Broumandan, A.; Lachapelle, G. Context-Aware Adaptive Multipath Compensation Based on Channel Pattern Recognition for GNSS Receivers. J. Navig. 2017, 70, 944–962. [Google Scholar] [CrossRef] [Green Version]
- Tao, Y.; Liu, C.; Chen, T.; Zhao, X.; Liu, C.; Hu, H.; Zhou, T.; Xin, H. Real-Time Multipath Mitigation in Multi-GNSS Short Baseline Positioning via CNN-LSTM Method. Math. Probl. Eng. 2021, 2021, 6573230. [Google Scholar] [CrossRef]
- Groves, P.D.; Jiang ZSkelton, B.; Cross, P.A. Novel multipath mitigation methods using a dual-polarization antenna. In Proceedings of the 23rd International Technical Meeting of the Satellite Division of The Institute of Navigation (ION GNSS 2010), Portland, OR, USA, 21–24 September 2010; pp. 140–151. [Google Scholar]
- Lu, R.; Chen, W.; Dong, D.; Wang, Z.; Zhang, C.; Peng, Y.; Yu, C. Multipath mitigation in GNSS precise point positioning based on trend-surface analysis and multipath hemispherical map. GPS Solut. 2021, 25, 119. [Google Scholar] [CrossRef]
- Anderson, B.D.O.; Moore, J.B. Optimal Filtering; Courier Corporation: Chelmsford, MA, USA, 2005. [Google Scholar]
- Wang, Z.; Chen, W.; Dong, D.; Wang, M.; Cai, M.; Yu, C.; Zheng, Z.; Liu, M. Multipath mitigation based on trend surface analysis applied to dual-antenna receiver with common clock. GPS Solut. 2019, 23, 104. [Google Scholar] [CrossRef]
- Spangenberg, M.; Tourneret, J.; Calmettes, V.; Duchateau, G. Detection of Variance Changes andMean Value Jumps in Measurement Noise for Multipath Mitigation in Urban Navigation. Navigation 2010, 57, 35–52. [Google Scholar] [CrossRef] [Green Version]
- Hsu, L.-T.; Jan, S.-S.; Groves, P.D.; Kubo, N. Multipath mitigation and NLOS detection using vector tracking in urban environments. GPS Solut. 2015, 19, 249–262. [Google Scholar] [CrossRef]
- Xu, B.; Hsu, L.-T. Open-source MATLAB code for GPS vector tracking on a software-defined receiver. GPS Solut. 2019, 23, 46. [Google Scholar] [CrossRef]
- Shultz Kenneth, S. Measurement Theory in Action; Taylor & Francis Ltd.: New York, NY, USA, 2020. [Google Scholar]
- Chikhi, S. Modelling and Implementation of Complex Systems; Springer: Berlin/Heidelberg, Germany, 2016. [Google Scholar]
- Branham, R.L., Jr. Scientific Data Analysis: An Introduction to Overdetermined Systems; Springer Science: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Leick, A.; Lapoport, L.; Tatarnikov, D. GPS Satellite Surveying, 4th ed.; John Wiley & Sons Inc.: Hoboken, NJ, USA, 2015; ISBN 978-1-118-67557-1. [Google Scholar]
- Kaplan, E.D.; Hegarty, C.J. Understanding GPS/GNSS Principles and Applications; Artech House: Norwood, MA, USA, 2017. [Google Scholar]
- David, H.A.; Nagaraja, H.N. Order Statistics, 3rd ed.; Wiley: Hoboken, NJ, USA, 2003; ISBN 978-0-471-38926-2. [Google Scholar]
- Gonzalez, R.; Woods, R. Digital Image Processing; Prentice Hall: Upper Saddle River, NJ, USA, 2008. [Google Scholar]
- Bancroft, S. An Algebraic Solution of the GPS Equations. IEEE Trans. Aerosp. Electron. Syst. 1985, 1, 56–59. [Google Scholar] [CrossRef]
- Kleusberg, A. Analytical GPS Navigation Solution. In Geodesy-The Challenge of the 3rd Millennium; Springer: Berlin/Heidelberg, Germany, 2003; pp. 93–96. [Google Scholar]
- Abdolkarimi, E.S.; Mosavi, M.R. A low-cost integrated MEMS-based INS/GPS vehicle navigation system with challenging conditions based on an optimized IT2FNN in occluded environments. GPS Solut. 2020, 24, 108. [Google Scholar] [CrossRef]
- Demkowicz, J. MEMS Gyro in the Context of Inertial Positioning. In Proceedings of the 2017 Baltic Geodetic Congress (BGC Geomatics), Gdansk, Poland, 22–25 June 2017; pp. 247–251. [Google Scholar]
- ASG-EUPOS. EUPOS, System Description. 2013. Available online: http://www.asgeupos.pl/ (accessed on 1 February 2022).
- CHC X91 Manual. Available online: https://chcnav.es/es/productos/x91-gnss/chc-Manual-Gps-Centimetrico-X91-en.pdf (accessed on 1 February 2022).
- UBlox EVK-5 Evaluation Kit. Available online: https://www.u-blox.com (accessed on 1 February 2022).
- RINEX Format, International GNSS Service. Available online: https://kb.igs.org/hc/en-us/articles/201096516-IGS-Formats (accessed on 1 February 2022).
- RTKLib ver 2.4.2 Manual. Available online: http://www.rtklib.com/prog/manual_2.4.2.pdf (accessed on 1 February 2022).
- RTKLib Source Code. Available online: https://github.com/tomojitakasu/RTKLIB (accessed on 1 October 2021).



| Satellite Number | Satellite xs [m] | Satellite ys [m] | Satellite zs [m] | Pseudorange ρ [m] |
|---|---|---|---|---|
| S1 | 17,345,523.118542 | −6,961,716.76442 | 18,824,282.012595 | 21,096,738.395152 |
| S2 | 12,466,634.722893 | −16,017,736.026726 | 17,000,530.544790 | 22,743,308.005079 |
| S3 | 17,777,510.053212 | 5,338,057.779070 | 19,076,768.926548 | 20,369,442.772950 |
| S4 | 13,772,185.231545 | 1,158,381.944537 | 21,460,334.042443 | 19,275,978.194772 |
| S5 | 1,475,851.838985 | 14,524,224.711896 | 20,929,766.556001 | 22,380,480.335172 |
| S6 | 21,460,226.02293 | 3,404,608.922848 | 13,354,551.79329 | 19,863,955.8471 |
| Combination | Receiver xr [m] | Receiver yr [m] | Receiver zr [m] |
|---|---|---|---|
| S1 S2 S3 S4 | 3,528,890.909046428 | 1,188,562.560529460 | 5,161,008.002971370 |
| S1 S2 S3 S5 | 3,528,894.3771535796 | 1,188,545.0001551111 | 5,161,001.9942599339 |
| S1 S2 S3 S6 | 3,528,891.6359943291 | 1,188,544.8388303395 | 5,161,008.1129308203 |
| S1 S2 S4 S5 | 3,528,892.3417388950 | 1,188,548.6533239735 | 5,160,995.5516880797 |
| S1S2 S4 S6 | 3,528,894.3178653666 | 1,188,543.1777995408 | 5,161,007.4226960409 |
| S1 S2 S5 S6 | 3,528,895.9587457380 | 1,188,542.1615108063 | 5,161,007.0003817966 |
| S1 S3 S4 S5 | 3,528,894.6291332841 | 1,188,544.8365306091 | 5,161,004.3937985701 |
| S1 S3 S4 S6 | 3,528,971.0776209440 | 1,188,535.0362633942 | 5,161,071.0962915756 |
| S1 S3 S5 S6 | 3,528,895.3269799771 | 1,188,544.3833856143 | 5,161,011.0392386625 |
| S1 S4 S5 S6 | 3,528,895.7118150592 | 1,188,543.0299482914 | 5,161,008.5790018179 |
| S2 S3 S4 S5 | 3,528,895.1983166863 | 1,188,544.3383257843 | 5,161,004.3531358577 |
| S2 S3 S4 S6 | 3,528,877.3334941966 | 1,188,551.6158001884 | 5,160,995.0672052046 |
| S2 S3 S5S6 | 3,528,898.7735123727 | 1,188,541.4568495557 | 5,161,014.6232669046 |
| S2 S4 S5 S6 | 3,528,896.7746096510 | 1,188,541.9572628422 | 5,161,009.2098844238 |
| S3 S4 S5 S6 | 3,528,889.3024272998 | 1,188,549.4989806332 | 5,161,004.7743413746 |
| 3,528,894.62913 | 1,188,544.38338561 | 5,161,007.42269604 |
| Method/Accuracy | Standard Deviation X [m] | Standard Deviation Y [m] | Standard Deviation Z [m] |
|---|---|---|---|
| LSQ method | 1.2281 | 0.68151 | 0.9560 |
| Proposed method | 0.94947 | 0.62384 | 1.6070 |
| Method/Accuracy | Standard Deviation X [m] | Standard Deviation Y [m] | Standard Deviation Z [m] |
|---|---|---|---|
| LSQ method | 9.9944 | 4.7845 | 24.035 |
| Proposed method | 3.1243 | 2.8342 | 9.8193 |
| Error Type | Algorithm Type | |||
|---|---|---|---|---|
| LSQ Method | LSQ [m] | Median Filter Method [m] | ||
| Latitude | Longitude | Latitude | Longitude | |
| Standard deviation | 6.39 | 4.89 | 5.32 | 1.96 |
| 75th percentile error | 5.70 | 4.19 | 4.61 | 3.93 |
| 95th percentile error | 35.88 | 9.79 | 9.11 | 6.06 |
| Outliers | 1292 | 2427 | 218 | 346 |
| Method/Accuracy | Standard Deviation X [m] | Standard Deviation Y [m] | Standard Deviation Z [m] |
|---|---|---|---|
| LSQ method | 1.2434 × 104 | 5960.8 | 2.6929 × 104 |
| Proposed method | 733.03 | 223.86 | 4700.6 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Demkowicz, J. Non-Least Square GNSS Positioning Algorithm for Densely Urbanized Areas. Remote Sens. 2022, 14, 2027. https://doi.org/10.3390/rs14092027
Demkowicz J. Non-Least Square GNSS Positioning Algorithm for Densely Urbanized Areas. Remote Sensing. 2022; 14(9):2027. https://doi.org/10.3390/rs14092027
Chicago/Turabian StyleDemkowicz, Jerzy. 2022. "Non-Least Square GNSS Positioning Algorithm for Densely Urbanized Areas" Remote Sensing 14, no. 9: 2027. https://doi.org/10.3390/rs14092027
APA StyleDemkowicz, J. (2022). Non-Least Square GNSS Positioning Algorithm for Densely Urbanized Areas. Remote Sensing, 14(9), 2027. https://doi.org/10.3390/rs14092027





