Advancements of Geodetic Activities in Nepal: A Review on Pre- and Post-2015 Gorkha Earthquake Eras with Future Directions

Abstract

From celestial objects to every feature on Earth, geodesy provides a reference frame and is the foundation for surveying, mapping, and other geoscience activities. In Nepal, geodesy was officially introduced after 1924 to prepare the topographic map series. Although the previous geodetic project occurred with foreign assistance, Nepal is using national resources to conduct milestone projects such as the re-measurement of Mount Everest height in 2020 and the ongoing LiDAR survey of western Terai. Taking the 2015 Gorkha earthquake as a reference, this paper reviews the past and present geodetic activities in Nepal. It presents the history of conventional Nepal datum as a horizontal datum and Indian mean sea level-based vertical datum, and modern satellite geodesy works on the Himalayas. Considering recent earthquakes, continuous crustal motion, international and global compliance, and increasing demand for precise positional accuracy from the users and stakeholders, this paper discusses future directions to build, establish, maintain, and operate modern terrestrial, height, and gravity reference systems and frames. This paper consolidates many reports and experiences from Nepal and will serve as useful documentation for newcomers whose interests align in geodesy and Nepal.

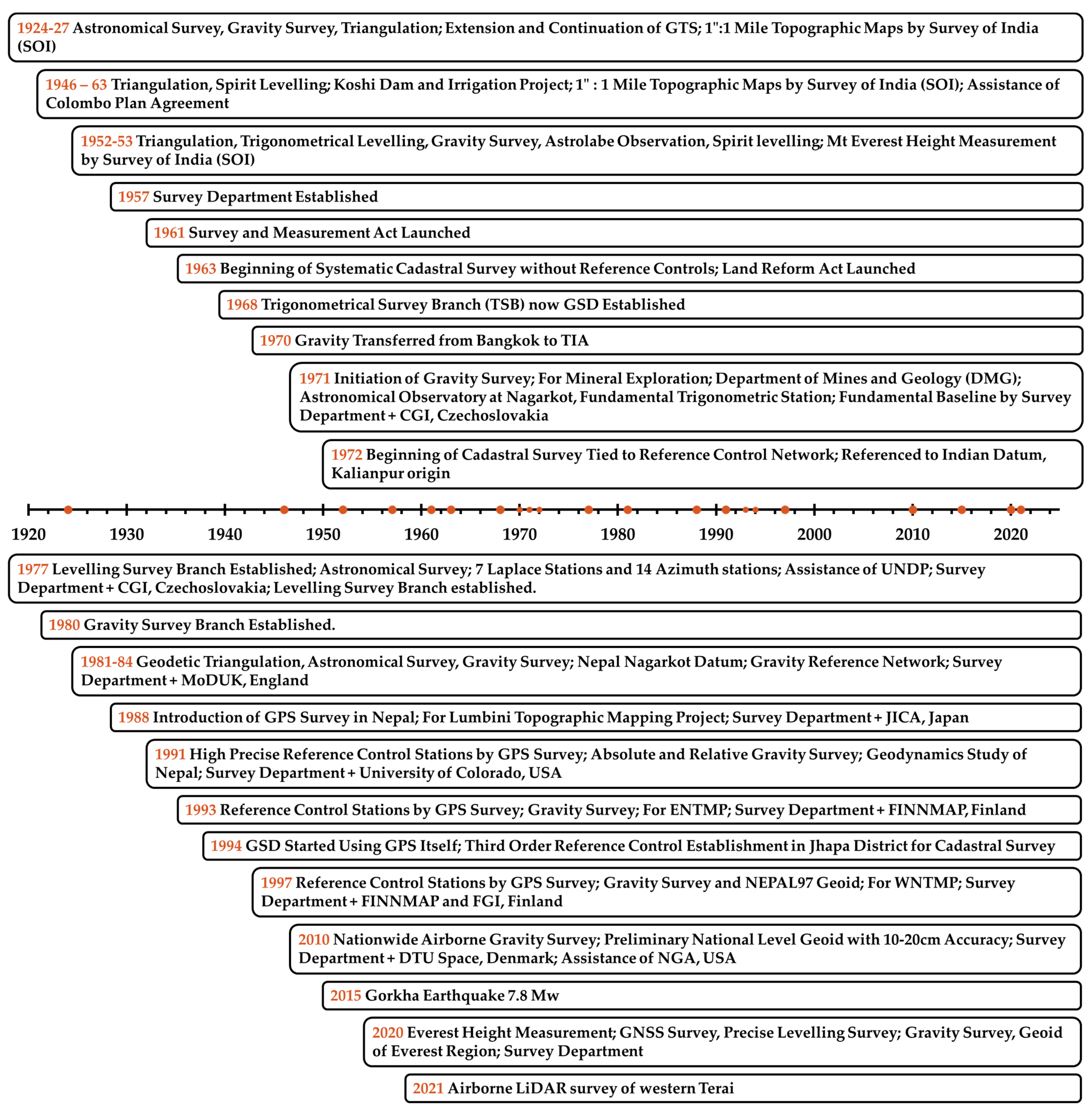
1. Background

A network of reference control stations is the groundwork of every surveying and mapping activity, such as topographical mapping, property boundary mapping, natural resources mapping, engineering mapping, utility mapping, etc., at local, state, national, regional-scale [1]. These reference control stations and the network, which later formed provides a coordinate system required for positioning, are built and established by a very precise and accurate measurement called a geodetic survey. Every country must define and establish a reference frame. The geodetic survey provides a reference frame, which is a fundamental and primary requirement for positioning of Earth’s surface, is the foundation of every surveying and mapping work [1]. The branch of science that deals with the Earth’s shape and size, its orientation in space, gravity field and provide the basis for geodetic survey and output reference system and the frame is called geodesy. It has come a long way from terrestrial-based classical geodesy, mainly a triangulation method, to modern satellite-based observations, precise time measurement with atomic clocks, computers, and many others [2,3,4].

Nepal being a developing country, started its surveying and mapping activities with foreign financial, technical, and human resources. A geodetic survey in Nepal appears first in 1924–1927 for 1”:4 Mile topographical mapping of Nepal [5]. The astronomical and triangulation survey was carried out to establish a network of triangulation stations, which is the basis for carrying out a topographical survey. After this, various other surveying and mapping activities occurred, and thus, various kinds of geodetic surveys were performed, providing the foundation for surveying and mapping works. Series of triangulation network was established in 1946–1963 for 1”:1 Mile topographic map preparation [5]. Triangulation network with an astronomical survey, trigonometrical leveling, spirit leveling, gravity survey was carried out in eastern Nepal in 1952–1954 for Mount (Mt) Everest height measurement [6]. All these above-mentioned projects were carried out by the Survey of India (SOI). The geodetic survey division (GSD) of the survey department (SD) carried out second, third, fourth-order control survey tied to the America (USA) introduced primary triangulation network established by SOI to carry out cadastral survey after 1972. Czechoslovakia Geodetic Institute (CGI) established Nagarkot Geodetic Observatory and seven Laplace stations in 1971−1977 with United Nations Development Program (UNDP) assistance [7]. Directorate of Military Survey (DoMS), Ministry of Defense, U.K. (MoDUK), established Nepal datum during 1981–1986 by astronomical, Doppler observations, and triangulation methods [8]. Up to this time, terrestrial-based classical geodetic surveys were most prevailing. After the United States of America (USA) introduced a global positioning system (GPS) in 1980, satellite positioning methods became easy, efficient, precise, and accurate, getting rid of the limitation posed by terrestrial-based classical geodetic survey methods [3]. Japan International Cooperation Agency (JICA) introduced GPS first time in Nepal in 1988 to establish a reference control station [9,10]. In 1991, the Cooperative Institute for Research in Environment Science (CIRES), University of Colorado (CU) at Boulder, and SD together built a GPS network popularly known as the CIRES network to study deformations and tectonics of the Himalayas [11,12,13]. In 1993–1997, Finland used a GPS survey to establish a network of reference control stations for Eastern Nepal Topographic Mapping Project (ENTMP), Western Nepal Topographic Mapping Project (WNTMP) [9,10]. Later GSD, on its own, bought GPS instruments and began higher-order geodetic observation for control stations densification. In 2010, a nationwide airborne gravity survey was carried out with the help of the National Space Institute at the Technical University of Denmark (DTU Space) and the United States National Geospatial-Intelligence Agency (NGA), and SD resulting in a preliminary national-level geoid [14]. Nepal, being the country resting above the collision between Indian and Eurasian plates, which results in continuous surface deformations and large earthquakes such as the 25 April 2015 Gorkha earthquake, has been studied by modern satellite-based geodetic methods and observations. Campaign observations at initial, after 1991, and continuous observations after 2000 by GPS techniques have been made. Satellite gravity mission Gravity Recovery and Climate Experiment (GRACE) observations have been used to quantify the change in mass because of monsoon precipitation, Himalaya ice loss, and change in groundwater storage. Surface displacements from Interferometric Synthetic Aperture Radar (InSAR) data available from Sentinel1-A, Advanced Land Observing System (ALOS-2), RADARSAT-2 due to the 25 April 2015 Gorkha earthquake have been prepared by numerous international institutes and researchers. However, all these geodetic survey works were performed with foreign financial, technical, human, instrumental, computing support; recently, in 2018–2020, SD re-measured the height of Mt Everest successfully with its resources. Though all these works have taken place during this period of more than 90 years period, beginning from 1924 to present times, the geodesy of Nepal still has a long way to go ahead.

Developed nations around the globe have modernized their national spatial reference system (NSRS) based on modern geodesy and technology and have adopted a practice of regular updates to meet the accuracy and precision demands of various kinds of users. Nepal lacks modernized NSRS. Nepal’s existing NSRS is built by classical geodetic methods based upon a classical geodetic theory. Nepal should opt for the definition of the modern terrestrial reference system (TRS) and its realization; the definition of the modern height reference system and its realization; and the definition of the modern gravity reference system (GRS) and its realization. Kinematic terrestrial reference frame (TRF) composed of a network of continuously operating reference stations (CORS), height reference frame (HRF) based on national geoid and GRS, global navigation satellite system (GNSS) (leveling in place of tedious precise leveling) are the major things that should be done in Nepal. Effects of crustal motion and the Gorkha earthquake 2015, along with the adoption of modern technology, have become the major reason to leave the existing NSRS and adopt modern NSRS.

Being a developing nation with growing geodetic developments, it is quite difficult to find proper documentation related to geodetic activities in Nepal. Given the literature gap and the author’s effort to combine all the information collected so far, this work aims to introduce geodesy, its advancements globally, and document all the past and present activities related to geodesy in Nepal. Focusing on Nepal, an extensive literature review of ancient documents, reports, and Nepalese journals were studied, a series of interviews with previous employees were performed, and the rest were based on the firsthand experience of the first author while in service. The organization of this work is as follow: Section 2 presents an introduction to geodesy, historic and modern geodesy, and advancement in the international community; Section 3 provides a detailed review of past and recent activities of geodesy in Nepal in two eras: pre and post 2015 Gorkha earthquake; Section 3 documents the modernization of geodesy in Nepal and its effort to build modern reference system; and final section discusses the lacking factors, needs and future directions to make progress in comparison to developed countries.

2. Geodesy in Nepal

Based on our study, Figure 1 shows the timeline of geodesy in Nepal. It has major events that are described in the following subsections in detail.

2.1. Pre 2015 Gorkha Earthquake Era

This section presents each significant and milestone project conducted and completed in Nepal, beginning from the ancient era to until 2015 Gorkha earthquake in terms of geodesy point of view. Details of each geodetic project in terms of date, technology adopted, products and services produced is presented, and each project is analyzed considering the significance of that project in Nepalese and global context, problems and challenges faced, and how Nepal overcame those obstacles.

2.1.1. Geodesy at Primitive Time

The Great Trigonometric Survey (GTS) in India began in 1802 [15]. British administration initiated this project through the SOI. GTS was a very large-scale geodetic science endeavor. India is a very large country. It took seven decades to complete the triangulation of all of India [15]. This GTS led to the discovery of the world’s tallest mountain, Mt. Everest. The height of the peak of Mt Everest was measured from trigonometrical stations situated northern part of India then in 1849–1850 for the first time [6]. It is a geodesy science that discovered Earth’s tallest peak and computed its height, which is taken as one of the significant scientific achievements after the scientific revolution began in mid-16th century 1543.

At the time GTS began in India, Nepal was not unified, and it was not in its present form. Prithvi Narayan Shah was fighting against the British to protect the sovereignty of Nepal. British administration brought modern science with them wherever they went or whichever part they colonized. Because Nepal was never colonized, modern science entered Nepal very late after the Rana regime was removed and democracy was established in 1951.

Extension and continuation of GTS came in Nepal in 1924–1927 to prepare 1” = 4 mile topographic map series. For this purpose, SOI carried out astronomical observations, gravity observations, and triangulation surveys during this period. The errors and uncertainties of astronomical surveys, gravity surveys, and triangulation surveys of this project could not be found as the documents of this work, which was performed more than 95 years ago, are not available. Figure 2 shows the snap of the 1” = 4 mile topographic map of Kathmandu.

This is the first scientific kind of survey. Both geodetic surveys (astronomical, gravity, and triangulation) and topographical surveys occurred in Nepal. To the best of our knowledge, there are no records of scientific survey works before 1924 in the history of Nepal [5].

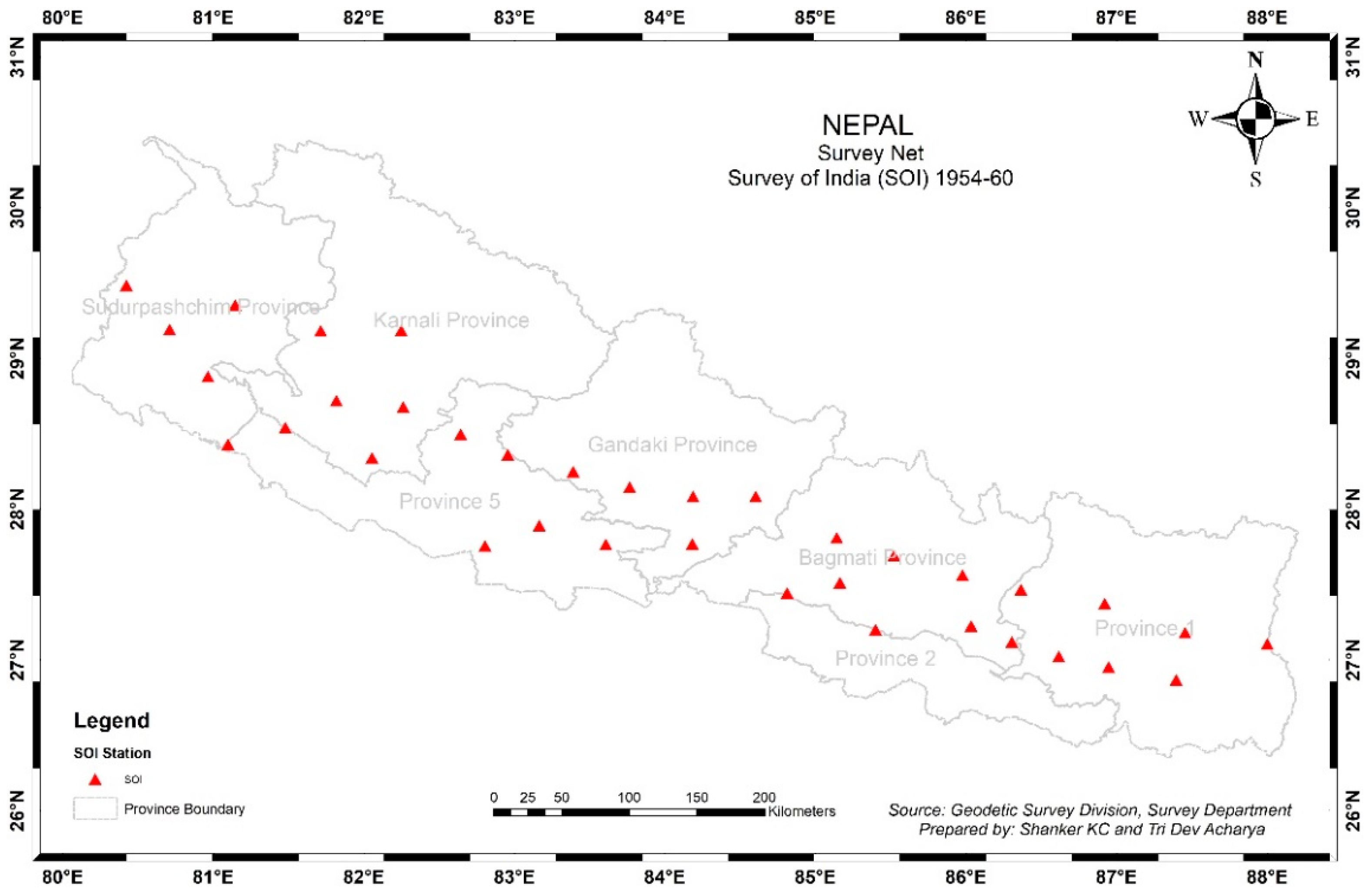
2.1.2. Topographic Map Series 1” = 1 Mile

Next, during the period 1946–1963, three significant achievements occurred in the history of surveying and mapping activities of Nepal. First: Nepal is covered by, excluding northern inaccessible mountainous and Himalayas part, nine different triangulation series [7,9,11]. Second: triangulation networks and spirit leveling network for Koshi Dam and irrigation project established in the eastern part of Nepal [6]. The triangulation network reached the northern district Solukhumbu with the purpose of measurement of the height of Everest led by Bihari L. Gulatee in 1952–1954. Trigonometrical leveling was performed for height computation works, astronomical observations, and gravity observations were made up to Namche Bazar for Everest height measurement purposes. It was 100 years after 1852 when the height of Everest peak was first determined. Details of Everest height measurement in 1952–1954 are explained in the following section separately. Third: 1”:1 mile topographic base map series of Nepal is produced. It was for this reason that the above-mentioned triangulation network was established. Figure 3 shows the distribution of triangulation control stations.

All these tasks were performed by SOI. SOI carried out this triangulation network establishment work and further topographic base map production work under the Colombo plan agreement [10]. SOI did fieldworks, computation, and adjustment of such nine different triangulation frameworks. Based on these control frameworks as primary frameworks, SOI established secondary control network.

The instrument used for angular observation was 1-arcsecond theodolite. Among those 9 triangulation networks, maximum and minimum triangular errors were 16 arcseconds and 8 arcseconds, and maximum and minimum average triangular misclosure errors were 5 arcseconds and 3 arcseconds. Similarly, the maximum and minimum relative error of closure (linear error) was 1/5000 and 1/42,000 [7]. Some of the networks among those nine networks were unadjusted either because the starting and closing stations of these networks were not tied to higher-order stations or the obtained misclosure exceeds the tolerance, or the accuracy obtained was sufficient for the mapping works. Other triangulation networks inside Nepal were formed based on these 9 networks, and the reported average triangular error was 5 arcseconds, and maximum triangular error was 30 arcseconds [7]. These networks were not rigorous and accurate but enough for topographical mapping purposes [11]. Coordinates of all these controls were given to geodetic survey branch (GSB) then, now GSD. All these triangulation works were based on the Indian datum of Kalianpur origin [11].

Before 1945, the importance of surveying and mapping works was poorly understood [11] in Nepal. Our first periodic five-year plan only began in 1956 [17]. At that time, there was no organization that we call National Mapping Agency (NMA) nowadays. Furthermore, there were no survey professionals. There were no survey science education institutes for manufacturing surveyors. Our oldest university Tribhuvan University established in 1959. Lacking all these infrastructures inside the nation, there was no possibility that Nepal on its own could have performed surveying and mapping works. Similarly, Nepal had no policies and acts regarding surveying and mapping. This shows that Nepal was at a very primitive stage of surveying and mapping before 1950, while the neighboring country India had begun the GTS in 1800, almost 150 years ago. This topographic map preparation work: both geodetic survey and topographical survey to prepare topographic map series of 1”:1 mile, complete with all foreign assistances, assistance in terms of finance, science and technology, human resources, and technical resources. However, after the successful completion of this project, we had our geodetic control by triangulation, astronomical and gravity survey method, and topographic base map of the country for the first time. A photograph showing the snap of the 1”:1 mile topographic map of Kathmandu can be found in the Flickr album by Mike Paskin [18].

2.1.3. Everest Height Measurement (1952–1954)

After 1800, British surveyors, based on some rough intersection observations to peaks of the Himalayas northwards, it was suspected that most peaks of the Himalayas north of Nepal could be the highest peaks around the world. It was during 1849–1850 that the peak of Mt Everest was established as the tallest peak of the world, and its height was computed for the first time, and it was 8839.81 m [6]. Northeast Longitudinal series of GTS built during 1846–1851 and was intended to run along mountains of Nepal, but Nepal government did not allow then [6]. So, during 1849–1850, trigonometrical leveling observations of the peak of Everest were made from six stations of the northeast longitudinal series, which are around 175 km away from the peak, and the height of Mt Everest mentioned above was determined [6]. Since then, the peak of Mt Everest became an interest of the world’s scientific community. Later, several heights of this peak were calculated after 1850 at various dates by various mathematicians and geodesists, based on a more advanced science of mountain height measurement and refined observations. More detailed historical accounts of various height determination of peak of Mt Everest and its mapping can be read in a recent magazine article by Khimlal Gautam [19].

The height of Mt Everest peak determined in 1850 prevailed century-long until the Bihari L. Gulatee, the director of the geodetic survey branch of SOI, began to point out that the height of peak should be measured and computed again using sufficient observational and geodetic data, which previous measurements and computation lack [6]. He proposed scientifically planned geodetic surveys, namely triangulation, trigonometrical leveling, spirit leveling, and gravity survey, which should be carried out in Nepal and from nearby topography of the peak of Mt Everest and observations to peak should be from mountains of Nepal [6]. Around 1950, geodesy and science of mountain height measurement were more improved compared to 1850. So, SOI found the necessity of re-measurement of the height of Mt Everest with improved methods and technology, works of new determination of the height of the peak is performed in 1952–1954.

The Everest height measurement in 1952–1954 used the geodetic control established during 1946–1963, which provided triangulation control for irrigation projects in connection with Koshi Dam [6]. This triangulation net contains four Laplace stations and three baselines. This net was closed with an error of 2 arcseconds in azimuth, −0.07 arcseconds in latitude, and −0.11 arcseconds in longitude. This triangulation was extended up to the mountains of Solukhumbu, Khotang, and Bhojpur districts to provide observation stations for the peak of Mt Everest. The height of these stations was determined from trigonometrical leveling. The obtained triangular misclosure in the height range is 0 to 4 feet. In addition, astronomical observations using astrolabe and gravity observation using pendulum were made up to Namche Bazar to obtain the geoid information of the region [6]. The accuracy of geoid thus computed has the uncertainty at the order of 2 feet. The weighted mean value of the height of the peak was 29,028 feet (8848 m).

The novelty of this work compared to the previous determination and significance of this work in the history of geodesy of Nepal is important because of various reasons. This time observations to peak were made from the mountains of Nepal, while past observations were from very far from the peak, north of India. Astrolabe observations and gravity observations provided more geodetic data. The concept of geoid and ellipsoid was established understanding then, so these observational data provided geoidal undulations referenced to a spheroid. Though SOI used its Indian datum with Everest spheroid for this height measurement work, international spheroid 1924 is used. Thus, height determined in 1952–1954 is way better than previous because of these three reasons: observations made from close to peak; two: more necessary geodetic data, astronomical and gravity data; and three: use of international spheroid and geoidal undulations of surrounding of the peak. Figure 4 shows the network of the northeast longitudinal series of GTS, extended triangulation network up to mountains of Nepal, and intersection network to the peak of Mt Everest.

In the history of geodesy of Nepal, this project implemented the international spheroid 1924, which was an advanced concept then, this spheroid way better than Everest 1830 spheroid for geoid and scientific studies, Nepal connected to global spheroid along with Everest spheroid. In addition, this project developed the geoid of Nepal around the surroundings of Mt Everest and prepared the geoidal undulation chart of such a remote area. This is a milestone for Nepal at that time. All the fieldworks, observations, computations, and scientific computing works were performed by SOI.

The height of peak determined during 1952–1954 is the official height of Mt Everest adopted by the Government of Nepal for 70 years until 2020. In 2020 Nepal measured the height of Mt Everest on its own. More about this in the following section.

Table 1. The elevation of Mount Everest peak with the geoid ellipsoid separation N (geoid ellipsoid separation in meters) from different authorities, modified from the work of [21].

Organization, Country	Year	Elevation of the Peak (Meter)	Method
Survey of India, India	1852	8840	Triangulation
Survey of India, India	1904	8882	Triangulation
Survey of India, India	1930	8854 ± 5	Triangulation
Survey of India, India	1954	8848	Triangulation
Ardito Desio, Italy	1987	8872	Triangulation
EV-K2-CNR, Italy and National Bureauof Surveying and Mapping, China	1992	8848.65 ± 0.35	GPS and Geoid
National Bureau of Surveying and Mapping, China	1999	8849.71	GPS and Geoid
Boston Museum of Science, USA	1999	8850 ± 2	GPS and Geoid
EV-K2-CNR, Italy	2004	8852.12 ± 0.12	GPS and Geoid
State Bureau of Surveying and Mapping, China	2005	8847.93 ± 0.14	GNSS and Geoid
Survey Department, Nepal	2020	8848.86	GNSS and Geoid

summarizes all the measurements of Mt Everest so far. Further details can be found in the post by Yury Molodtsov [20].

2.1.4. Nepal-India, Nepal-China Boundary Survey

Nepal shares international boundaries with India at East, West, and South, and with China on the north side. Nepal-China boundary agreement occurred in 1960, while the Nepal-China boundary treaty was signed in 1961. During 1961–1962 borderline demarcation was carried out based on the watershed principle of the Himalayan region. The joint survey team from both the countries erected permanent pillars and markers from west to east. The first Nepal-China Boundary Protocol was signed in 1963. In 2005, existing boundary markers were repaired, and disappeared markers were re-installed. At the same time, a team of surveyors from SD carried GNSS survey of those boundary markers [22].

Nepal-India borderline was demarcated based on the Sugauli Treaty of 1816 and Boundary Treaty of 1860 [23]. The boundary line between Nepal-India was surveyed from 1816 to 1940/41 at various dates. In 1981, the Joint Technical Level Boundary Committee (JTBC) was formed by both countries to resolve and manage the border issues [23]. This JTBC prepared boundary maps based on the fixed boundary principle and worked until 2008. Because of various disputes that occurred, SD again began the Nepal-India border survey after 2014. Boundary pillars are surveyed using the GNSS survey, and boundary maps are prepared in GRS80 reference ellipsoid with Lambert conformal conical (LCC) projection system. The error and uncertainty information regarding the control survey performed in the Nepal-India boundary and Nepal-China boundary are not available to the public as these documents with the surveying details of the border survey are considered very sensitive.

2.1.5. Initiation of Nationwide Cadaster Preparation

The necessity of a scientific, integrated, consistent nationwide cadaster was realized, which will provide the basis for the national land revenue collection system after 1950. Before, there was a rudimentary, inconsistent, inefficient cadaster during monarchy and Rana period. Thus, the necessary policy and act formation occurred to create a nationwide cadaster. Survey and Measurement Act in 1961 and Land Reform Act in 1963 formed, and systematic cadastral survey commenced from 1963. This cadastral surveying necessitates the establishment of the network of accurate and precise reference control points, higher-order to the lower order, which is the works of geodesy.

During the first periodic plan, 1956–1961, the Government of Nepal (GoN) then realized the need for a source of basic information, data, and statistics regarding land records of the whole nation [17]. The government realized that it must provide a reliable system of securing the ownership rights of landowners, justifiable taxation for land, and a scientific, systematic, organized land revenue collection [17]. GoN decided to create surveying and mapping policies, perform the cadastral survey, produce surveyors, establish dedicated organizations to carry out cadastral survey works. SD was thus established in 1957.

In 1961, GoN published Survey and Measurement Act to govern, regulate, and drive forward surveying and mapping work inside the nation. This act is meant to cover cadastral survey, topographical survey, engineering survey for engineering projects and developmental projects, and envision to adopt newer methods of survey and instruments to keep pace with modern science and technology of the time. This policy is a milestone achievement in the history of surveying and mapping Nepal.

Intending to replace rudimentary, primitive, inefficient land records keeping systems spread in many small territories, GoN envisioned carrying out scientific, systematic, and organized surveying of various kinds of the whole country. This necessitated the establishment of a public organization such as NMA now, establishing education and training organizations to produce survey technicians, allocating budget/finance, etc.

Now since GoN planned to carry out nationwide cadaster preparation beginning from 1964 by publishing and implementing Land Reform Act of 1963 and carrying out other large national development and engineering construction projects. This necessitated high precise and accurate nationwide geodetic control network considering the shape and size of the Earth over Nepal extent [9,11,24].

SD began to conduct cadastral surveys without any geodetic control since 1963. It prepared cadastral maps of 38 districts as island maps. In 1968, the trigonometrical survey branch (TSB), now GSD, was established. It began performing astronomical surveys, preparing for the establishment of the network of reference control points for the cadastral survey and other kinds of geodetic surveys. More about TSB and its works are presented in the following section.

SD used the triangulation network based on the Indian datum, formed during 1946–1963 by SOI to prepare a 1”:1 mile topographic map series for a cadastral survey of the remaining 37 districts to prepare cadastral maps based on reference control network since 1972. Coordinates of these controls were given to TSB by SOI [11]. Then TSB established second, third, and fourth-order networks of reference control points on those districts. The accuracy and precision standards of second, third, and fourth-order control stations are shown in

Table 2. The accuracy and precision standards of second-, third-, and fourth-order control stations.

Order of Control Survey	Triangular Misclosure	No of Sets (Angular Measurement)	Reference Object to Reference Object Closing	Distance between Stations
Second	10 cc	9	10 cc	9–15 km
Third	10 cc	6	15 cc	3–7 km
Fourth	75 cc	3	30 cc	0.2–3 km

. Those controls and coordinates are only used for cadastral purposes. Thus, the necessity of cadastral maps preparation based on reference control network resulted in the establishment of TSB.

2.1.6. Establishment of Triangulation Survey Branch (TSB)

At present, TSB is GSD. The necessity of an even precise and accurate geodetic reference control network of its own (national geodetic control framework) grew even stronger after cadastral maps of 38 districts were mapped without a reference control framework. SOI established a triangulation framework during 1946–1963 was there, but that was not used in cadastral mapping purposes yet. Based on SOI’s established triangulation control framework, other secondary and tertiary reference control networks could be built to provide reference control points for the cadastral survey. Similarly, SOI-prepared topographic base maps were not that useful because of their outdated nature. We needed to create large-scale topographical base maps compared to small-scale 1”:1 mile to meet the needs of planning of large engineering and developmental projects and many more. However, the fundamental requirement of the cadastral and topographical survey is the network of reference control points. Thus, a separate unit called TSB was established in 1968 [7] to build a nationwide geodetic control framework necessary for various kinds of surveying and mapping activities. Initially, TSB was led by a foreigner, the Czech Republic national, ZM Wiedner. He ran the TSB as assistant director for 7 years, 1970–1977.

Cadastral mapping was already being carried out without any reference control points. TSB, after its establishment, developed second, third, and fourth-order reference control points based on the triangulation framework built by SOI during 1946–1963 [11]. So, for the first time, a cadastral survey based on reference control points began in 1972. These networks were only used for cadastral mapping purposes. It served the immediate need [11].

TSB then began to carry out systematic astronomical, gravity survey, besides the above-mentioned work. During 1970–1971, TSB established the Astronomical Observatory at Nagarkot, Fundamental Trigonometric Station, Fundamental Baseline [5,7]. TSB, in cooperation with CGI, established seven Laplace stations with the standard error ±0.10 to ±0.20 arcseconds in both latitude and longitude and measured astronomical azimuths in both directions at those seven stations having standard error ±0.2 to ±0.4 arcseconds producing astronomic azimuths in 1971–1972 and 1976–1977 with the assistance of UNDP [5,7] as shown in Figure 5.

In 1975−1976, TSB measured length on 10 lines using a geodimeter. Geodimeter length measurements lines are shown in Figure 6.

In 1970, absolute gravity transferred to Tribhuvan International Airport (TIA) from Bangkok [5]. Beginning in 1977, the leveling survey branch was established to carry out first-order leveling in a country [7]. Later in 1980, the gravity survey branch was established to carry out gravity observation required for the geodetic purpose [7]. More about the history of the gravity survey in the upcoming section.

The establishment of TSB, realizing the importance and need of a nationwide geodetic reference control framework, is another steppingstone. It built geodetic infrastructure such as Astronomical Observatory in Nagarkot planned and carried out astronomical observations. Though the primary triangulation framework based on Indian datum was established by SOI, Nepalese surveyor professionals carried out second, third, and fourth-order control surveys and established a reference control network for cadastral mapping. This is another achievement in terms of human technical resources. After CGI established 7 astronomical observation stations and 14 astronomical azimuths, competent Nepalese survey professionals carried out further astronomical observations at the astronomical observatory. This is another achievement in terms of capacity building. Though it started from UNDP assistance and SOI assistance in terms of both financial and technical resources, it is suitable that afterward, Nepal can conduct an astronomical survey and carry out the extension of the control network on its human technical capacity. As the scope of the geodetic survey widened, TSB evolved as GSD.

2.1.7. Nepal Datum

SOI datum had problems with the foot to-meter conversion of the length of the semi-major axis and longitude correction [11]. The reference ellipsoid is characterized by its semi-major axis and flatting (a, f). The primary triangulation framework established by SOI was not rigorous and accurate enough for high precise survey [11]. Second, third, and fourth-order reference control points were established based on the above primary network were only fit for cadastral purposes. Those coordinates are thus called cadastral coordinates [11]. As time went by, the need for a higher-order and accurate nationwide geodetic control network became obvious suited for the whole of Nepal.

GoN and DoMS, MoDUK together began to work on establishing a rigorous SRS for Nepal in 1980 [11]. All the fieldworks, such as the Doppler survey, gravity survey, triangulation, length measurements, etc., were carried out in 1981–1984, and final adjustment results were released in 1986 [8]. Network of 68 higher-order geodetic control points established referenced to Everest1830 ellipsoid, which is termed as Nepal datum with origin in Nagarkot. A total of 16/68 were Doppler stations with an uncertainty of ±1 m in position, 7/68 astronomical observation stations by CGI with uncertainty ±0.10 to ±0.20 arcseconds in latitude and longitude, 32/68 astronomical observation stations having the same uncertainty as maintained by CGI, and remaining stations were included in this network. Existing stations from the SOI triangulation framework from 1946 to 1963 were used, and other new stations were monumented to build this Nepal datum [8]. A total of 39/68 stations were astronomical azimuth and deflection of vertical observed [5]. Gravity observations were also carried out at some of these stations and leveling BMs [5]. T3 and T2 theodolite were used for angular measurements, and astronomical observations, electronic distance measurement (EDM) instruments were used for distance measurements. The standard error (SE) uncertainty of angular measurement was ±1 arcsecond, that of astronomic azimuth was in the range of ±0.2 to ±0.4 arcseconds, and that of distance measurements was ±5 ppm [8]. It used the existing infrastructure and past geodetic works of TSB as much as possible. This project used astronomical observations, deflection of verticals, gravity observations, Doppler observations, triangulations, EDM distance measurements, trigonometric leveling, first-order leveling benchmarks (BM), and existing stations from SOI framework and a rigorous reference system and frame was realized having datum origin at Nagarkot and 68 first-order geodetic control points distributed throughout the country. The distribution of 68 stations is as shown in Figure 7.

Trigonometric leveling was used to obtain the height of these control stations. Initial reference heights for trigonometric leveling were the unadjusted heights of level BMs surveyed by SD, as shown in Figure 8, available in 1981–1984.

Northern parts of Nepal lack the higher-order geodetic reference control points based on this datum. All technical human resources were from the DoMS, MoDUK, all the managerial and technical works from the planning phase, fieldworks, to computation and adjustment were all performed by technical resources from the DoMS, MoDUK.

Nepal datum is one of the greatest achievements in the history of geodesy of Nepal. Now all other surveying and mapping activities are referenced to this framework. This datum is a rigorous reference system with high precise geodetic control stations. The network is oriented to conventional international origin (CIO), suited for any kind of higher-order geodetic works [11].

2.1.8. Global Positioning System (GPS) in Nepal

With progressing science and technology, geodesy, surveying, and mapping lead to more precise, more accurate, more efficient, timely, and more automated kinds of technology that appear with successive improvements. After the launch of the first satellite in space by Russia in 1957 and the USA in 1958 [25], the use of space, space technology, and a spaceborne platform was exploited for geodetic purposes. Gradually terrestrial-based geodetic survey methods such as triangulation and trilateration begin to lose their strength and begin to be obsolete in front of space geodesy. Space-based technology, platform, and systems such as the Doppler Orbitography and Radiopositioning Integrated by Satelliteand integrated by satellite (DORIS): a French-built system to derive satellite orbits and ground position based on Doppler frequency shift, navigation system timing and ranging (NAVSTAR) GPS: a USA built satellite positioning system where a ground receiver anywhere on the Earth receives the signals to derive its position, satellite laser ranging (SLR): a range measurement system between the ground station and satellite by round trip travel time, Lunar laser ranging (LLR): range measurement between Earth and the moon by the round trip travel time of laser reflected by retroreflector installed in moon surface, very-long-baseline interferometry (VLBI): a technique to measure the distance between radiotelescopes by determining the time difference between the arrivals of radio signals from Quasars, etc. are being favored, used, and explored in geodetic measurement work in surveying and mapping. USA began to use GPS in 1970 [3]. GPS is the satellite-based positioning system and is used for 3D position determination of any corner of the Earth.

In the case of Nepal, JICA used GPS for high precise reference control establishment required for photogrammetric triangulation purposes for the Lumbini Topographic Mapping Project in 1988 for the first time in Nepal. The control network of 20 stations was surveyed by the GPS method achieving the mean square error of position at the range 0.000 to 0.293 m. Again, it was new technology and foreign JICA assistance in both financial and technical resources. It was impossible at that time that Nepalese surveyors were competent in using GPS for the geodetic survey. However, advanced spaceborne technology was brought and used in Nepal, not much after (18 years) the USA and other developed nations exploited GPS for geodetic purposes. This work is another milestone in the history of geodesy of Nepal. This was the beginning point. Later GSD, SD began to use GPS regularly, and it became the only method of establishing high precise reference controls. The establishment of higher-order reference control using GPS survey became the routine work in SD.

However, various GPS networks have been established in Nepal since 1991 without the direct involvement of SD to study the geodynamics of the Himalayas by various international institutes. Besides the CIRES network mentioned above, established in 1991 and extended later gradually, was the effort of CIRES, CU at Boulder and SD while Laboratoire de Detection Geophysique (LDG) network was the effort of the French institution LDG established during 1995–2000, targeted to study surface/crustal deformations along the longitude of Kathmandu [13] and another GPS network established by Imagerie et Dynamique de la Lithosphere (IDYL) project called IDYLHIM network was established in the same period 1995–2000 to study the central/western Nepal segmentation [26] where SD was not involved.

2.1.9. Geodynamics Study of Nepal

Tools and techniques of geodesy are also useful for other disciplines of science. Traditional and terrestrial-based geodetic survey works and observations such as astronomical observations, gravity observations, high precise triangulation and trilateration, high precise EDM distance measurement, high precise leveling could be used for geodynamics. Geodynamics is the study of internal and external dynamical Earth’s processes such as tectonic plate movement, subsidence, and uplift of a large mass of the portion of the Earth, earthquake, and others. Since geodesy studies the shape and size of the Earth, geodynamics gets great assistance in these aspects from geodesy. Geodesy also exploits information and knowledge from geodynamics to determine the true, accurate, and precise shape and size of the Earth.

The collision between the Indian plate and Eurasian plate is the only continent-continent plate convergence process in the Earth that began 50 million years ago. The 2400 km long Himalayan arc and Tibetan plateau are the best manifestations of this collision/convergence process [27]. Nepal became the best ground to study this collision process/convergence process/geology, seismotectonic settings for the researcher of geology/geodynamics/seismology, etc. The international community of Earth science begins to put their research/investigations interest by collecting geologic, seismic, geophysical, geodetic data by ground-based and space-based platform to infer geometry, structure, geology, collision process, convergence rate, strain energy, fault lines, etc. Geodetic observations provided the information that verifies results from geological, seismic, geophysical studies, and the space-based geodetic observations covered those large spatial coverages from Ganges plain at the south to Nepal Himalaya to Tibetan plateau at the north, which assisted in gaining even further knowledge of this collision process/convergence rate/seismotectonic settings of Himalaya region/uplift and subsidence of the Earth’s surface, etc.

After the introduction of GPS in 1980, GPS provided space geodetic observations/measurements for geodynamics. In 1991 campaign GPS observations were made to study the crustal motion of the Himalayas [27]. Those stations were established over the main tectonic structures between the Ganges plain and Tibetan plateau [27]. The authors suggested repeated GPS observations to enhance the knowledge of current deformation and the nature of geodynamics in the Himalayas [27]. In the period 1991–1992, repeated GPS measurements were taken to that extent from Bihar at the south to Nepal and southern and eastern Tibet [12], confirming the geologically determined convergence rate of 20 mm/year using geodetic observations of these GPS stations. Jackson et al. [12] used absolute gravity data, spirit leveling along with these GPS observations for the assessment of convergence of two continental plates and resulting deformation. Another study carried out by the authors of [28] used additional GPS measurements beginning from 1991 to 1995 GPS observations and estimated the northward motion of the Indian plate and the convergence rate of the southern Himalayas and the Indian subcontinent. Biham et al. [29] used the same GPS observations collected in 1991–1995 and derived the rate of convergence to be 17.52 ± 2 mm/year and anticipated the earthquake that will be equivalent to 1934 Bihar/Nepal 8.1 moment magnitude (Mw) in western Nepal.

Blume F. [30] carried out the study that determined the location and the source parameters of the 1934 Bihar/Nepal earthquake using both historic (gravity data, leveling data, triangulation data created in Mt Everest height measurement) and modern GPS observations. Ader et al. [26] used the previous GPS network (CIRES network, LDG net, IDYLHIM net) and Garhwal-Kumaon Himalaya (India-west of Nepal) to study the pattern of interseismic coupling on the main Himalayan thrust (MHT) fault.

2.1.10. Seasonal Deformation Studies over Nepal and Himalaya

It has been observed that crustal deformation occurs not only because of underlying tectonic plate movement. The elastic response of the lithosphere to the seasonal change in hydrological loading has been observed, too [31]. Satellite-based geodetic observations, mainly CORS and the GRACE, can measure seasonal variations of hydrological load [32]. GRACE is a satellite gravity mission that is intended to measure periodically the change in the Earth’s gravity field and mass variations from the surface water and ice. These variations can be quantitatively measured [33]. Several studies have been carried out, which have confirmed the seasonal variations in horizontal and vertical components of GPS time series over the Nepal Himalaya region because of the heavy rainfall during the monsoon. These studies have derived vertical displacement from GRACE observations, which measured changes in the gravity field because of changes in surface load (hydrologic) because of heavy rainfall in the monsoon. Studies have shown that there is a long-term mass loss in the Himalayas derived from GRACE observations also.

Flouzat et al. [31] used time series derived from CORS stations, including IGS station and DORIS stations across the Himalayas of central Nepal, and observed the significant seasonal fluctuations in both horizontal and vertical components of the time series. Fu et al. [32,33] used CORS and GRACE geodetic observations and studied seasonal mass change and resulting vertical displacements over Nepal and southern Tibet. Fu et al. [32,33] observed long-term mass loss in the Himalayas revealed from GRACE observations and assessed its impact on vertical displacement caused by tectonics. Fu et al. [34] studied the horizontal seasonal deformations in two of the world’s largest seasonal mass variations Amazon basin and Southeast Asia. Fu et al. [34] used CORS observations and found seasonal crustal deformations as elastic responses caused by heavy rainfall in the Amazon basin and monsoon in Southeast Asia to be consistent/correlated with GRACE-derived change gravity observations of surface water loading. Chanard et al. [35] pointed out that seasonal deformation of the Himalayas because of continental water storage driven by monsoon should be modeled and accounted to obtain realistic Earth structure over the Himalayas region. The work used GRACE observations that provided variations in continental water storage, and CORS provided geodetic displacements over southern Tibet, Nepal, and India [35].

Liu et al. [36] and Zhang et al. [37] used the CORS observations over Nepal and GRACE observations. They came up with the same finding that vertical displacements derived from both geodetic methods are consistent with each other. Like Chanard et al. [35], Zhang et al. [37] removed the GRACE-derived vertical displacements from GPS/CORS-derived displacements to estimate the vertical crustal deformation. Similarly, the other two studies by Saji et al. [38] and Ray et al. [39] came up with the same findings that the North of Himalaya experiences a seasonal deformation due to change in surface mass loads caused by the annual monsoon.

2.1.11. Groundwater Resource Monitoring Using Space Geodetic Observations from CORS and GRACE

GRACE observations have been used to monitor groundwater resources over Nepal Himalaya and northern India [40,41]. Authors of [40] found continuous large-scale mass loss in Northen India measured from GRACE 2002–2008 observations. This mass loss is attributed to excessive extraction of groundwater for irrigation purposes. Samsudduha et al. [41] used the GRACE observations to study the changes in GWS (ground water storage) of a basin of the large Himalayan river system of Brahmaputra, Ganges, Indus, Irrawady, and Meghna. Same as findings in Tiwari et al. [40], Samsuddha et al. [41] found that there is alarming decreasing groundwater storage in northern India due to excessive extraction of groundwater for dry season irrigation.

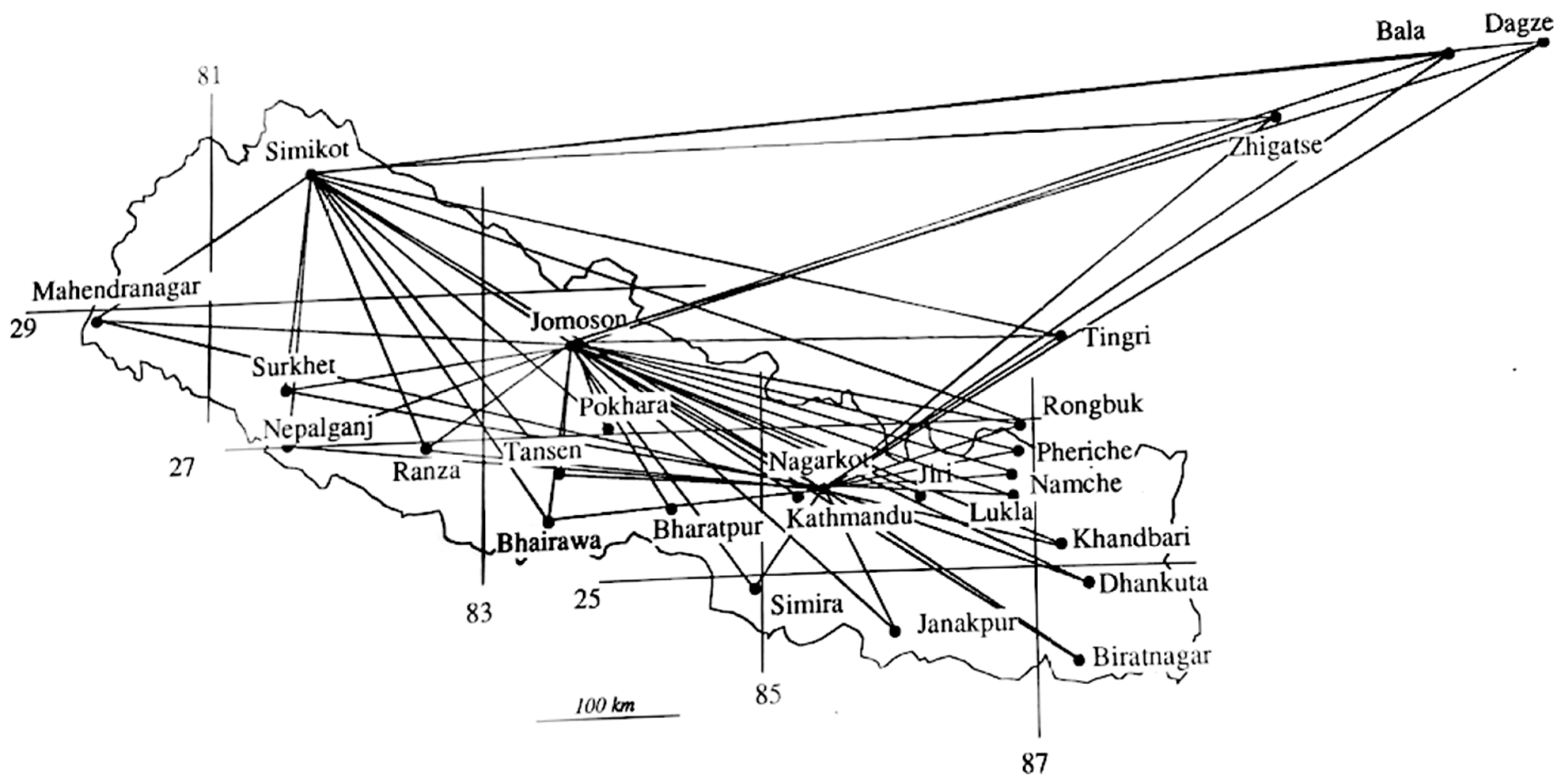
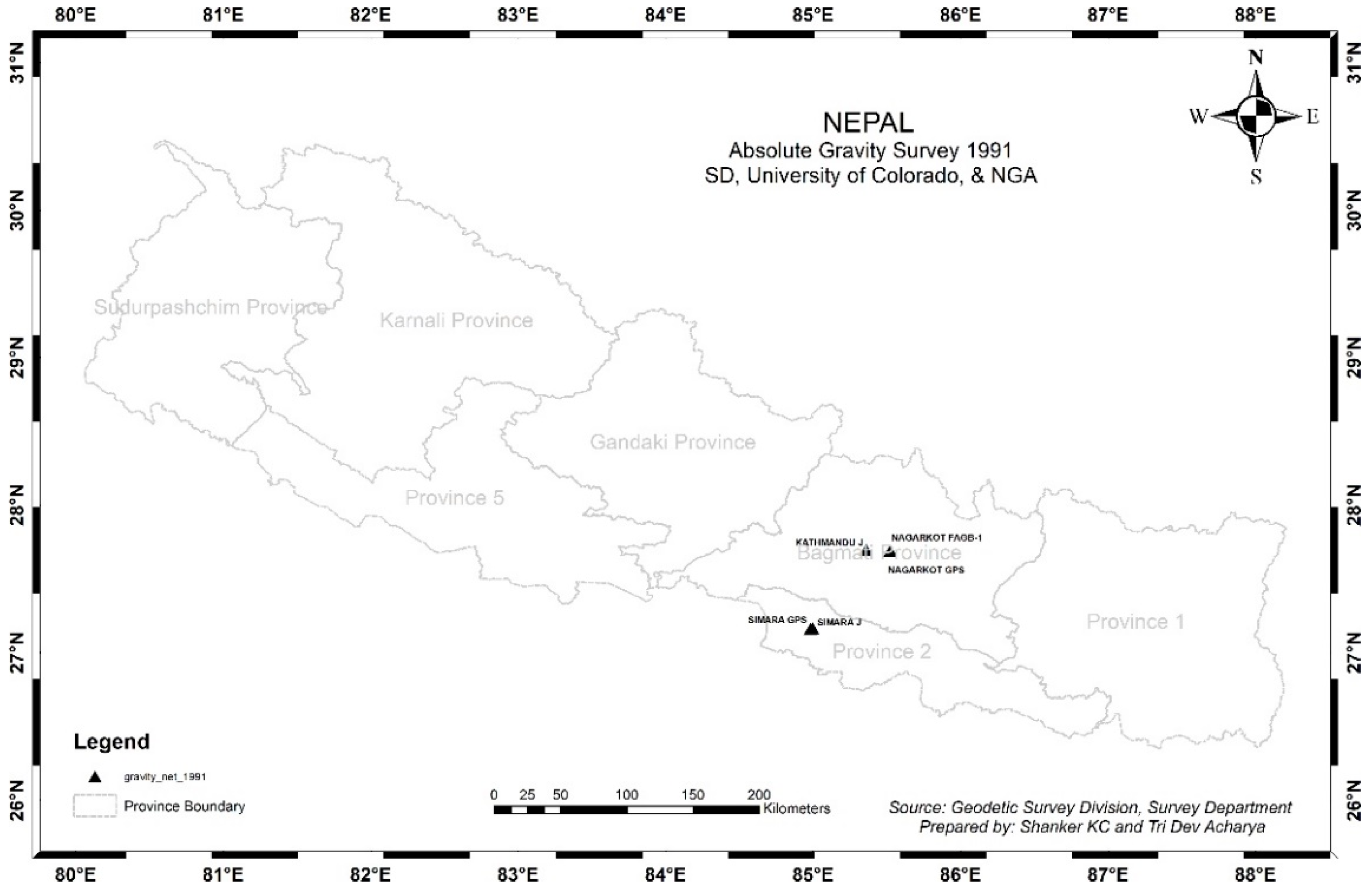
2.1.12. Details of 1991 GPS Survey by SD and CU at Boulder

In the year 1991, March–April, SD, CU at Boulder, and NGA, USA established 28 high precise reference control points by GPS survey method distributed throughout the country [11], as shown in Figure 9. The project aims both: to provide high precise GPS control for geodetic survey and study and measure the geodynamics of crustal motion phenomenon and the uplift of terrain across Himalayan collision zone [11].

WGS84 datum with Nagarkot tracking station as origin used for high precision GPS reference control. Absolute gravity observations were also made for geodynamics study purposes. More about gravity observation later in the gravity section.

It was the first geodynamics study of Nepal, and the involvement of SD was appreciable. SD got another set of high precise reference control established by GPS survey after just 6 years from 1981 to 1984 MoDUK established higher-order geodetic control stations established by triangulation technology. MoDUK established a geodetic reference control network that was terrestrial-based and used traditional techniques and instruments. The established datum was local and topocentric. However, 28 highly precise reference controls established in association with CU were based on space and satellite-based geodetic techniques and modern instrument GPS receivers, and the established datum was of global and geocentric nature.

It was suitable initiative work by SD to use GPS technology for both geodetic and geodynamic purposes. Though both financial and technical resources were foreign, Nepalese survey professionals got the exposure and training ground to use GPS surveys for geodetic control purposes. This work provided SD more confidence in using GPS for high precise control establishment in future days. Even more important is that we obtained knowledge of the geodynamics of our country through these geodetic observations.

2.1.13. Eastern Nepal Topographic Mapping Project (ENTMP)

The need for an updated and accurate and large-scale topographic base map (TBM) of the country led to the topographic mapping project of a country. In 1993, ENTMP began to prepare TBMs of eastern and central Nepal with the help of Finland by aerial photogrammetric survey method. This project established the 29 primaries and 72 secondary height-precise GPS controls [43]. The baseline accuracy of the primary network was better than 2 ppm, and that of the secondary network was better than 12 ppm. WGS84-based coordinates of primary and secondary stations were transformed to Nepal datum [43]. The accuracy of transformation parameters was better than 30 cm. The distribution of these 101 stations is as shown in Figure 10. The height of TBMs was based on Indian MSL.

ENTMP was performed with the assistance of Finland. Instrumental, technical, and human resources were from Finland, but Nepalese survey professionals were also involved as a counterpart to work and learn both.

2.1.14. Western Nepal Topographic Mapping Project (WNTMP)

In 1997, SD and Finland prepared TBMs of the western, mid-western, and far-western regions of Nepal. This project established 51 high precise GPS control of primary order, one station from ENTMP used, and 76 control of secondary order stations. The baseline accuracy of the primary network was better than 3 ppm, and that of the secondary network was better than 7 ppm. The distribution of these controls is as shown in Figure 10. WGS84-based coordinates of these 127 stations were converted to Nepal datum. The accuracy of the transformation parameter was around 30 cm. Orthometric heights were from the geoid model [44]. More about geoid in the upcoming gravity section.

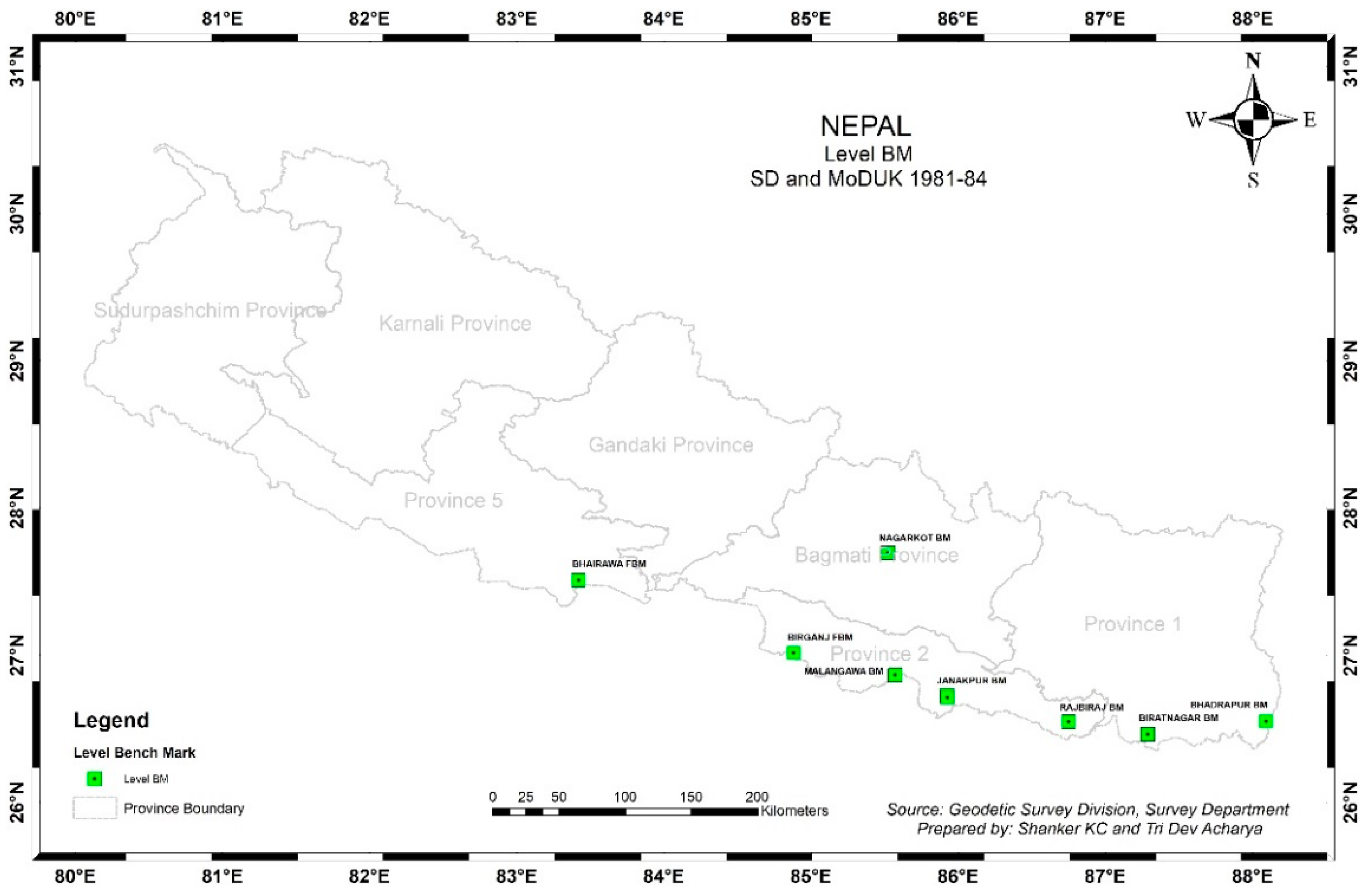
2.1.15. Strengthening of Geodetic Control Network

After 1994/1995, Nepal had well established geodetic reference control network, which includes a reference control network established by triangulation and trigonometrical survey, high precise leveling networks, Laplace stations, gravity stations, Doppler survey stations, high precision GPS reference controls [7]. Nepal has its organization, SD and GSD, its competent survey professionals, and instrumental resources carrying out these various kinds of geodetic surveys. Nepal is capable of carrying out the geodetic survey without any foreign assistance in any aspect.

GSD launched the program, strengthening the geodetic control network. This program intends to extend the geodetic control network, carry out gravity surveys, and re-survey the existing higher-order control stations to obtain even more precise positional accuracy. SD started to use the control points for cadastral mapping in 1972, and methods of control point establishment were triangulation and traversing using theodolite and EDMs. In 1994, after 22 years, SD started to use the GPS on its own for the extension of control networks. Third-order control points were established by the GPS method in the Jhapa district at first and carried out to other districts by GSD’s GPS instruments, survey professionals, technical and processing professionals. In addition, GSD regularly carried out GPS surveys of primary order control stations, gravity surveys, precise leveling surveys, etc.

2.1.16. Gravity Survey

The first account of gravity survey performed in Nepal was conducted by SOI at the eastern part of Nepal, carried up to Namche Bazar, to obtain geoid undulation of this region required for Everest height measurement, led by Bihari L. Gulatee in 1952–1954 AD [5,6].

In 1970, the absolute gravity value transferred from Bangkok to TIA, Kathmandu [5]. Department of Mines and Geology (DMG) began a gravity survey in 1971 to explore mineral resources [5]. A separate branch called the gravity survey branch was established in 1980 at GSD [5]. Historical account of gravity survey in Nepal to serve the geodetic purpose by SD is explained hereafter.

• Nepal Gravity Survey 1981–1984

This survey was carried out by a British military survey unit under MoDUK. The aim was to establish a gravity base station net and detail gravity stations. The gravity reference system adopted was International Gravity Standardization Net (IGSN71) Modified 1979 [45].

During the 1981–1984 gravity survey of Nepal covered most of the more accessible parts of the country with a fundamental gravity base station (KATHMANDU J) at the accuracy level of 0.05 mgal. A total of 45 gravity base stations and 375 gravity detail stations were established at the accuracy level of ±0.03 and ±0.3 mgal, respectively [45]. These stations formed a national gravity network of Nepal. Figure 11 shows the network of gravity base stations.

2.

Absolute Gravity Measurement for Geodynamics

In 1991, SD, National geodetic survey, and CU did high precise GPS survey and absolute gravity survey for the geodynamics study of Nepal. Joint Institute for Laboratory Astrophysics (JILA) absolute gravimeter, based on the free fall principle, was used to measure the absolute gravity value of the Fundamental Absolute Gravity Station (FAGS-1) [46]. The accuracy of measurement of absolute gravity was up to 6 micro-gal. Model D Lacoste & Romberg relative gravimeter was used to transfer the gravity value from FAGS-1 to other 4 stations: Nagarkot GPS, Kathmandu, Simara airports, and Simara GPS with the accuracy range 10 to 20 micro-gal [47], as shown in Figure 12.

3.

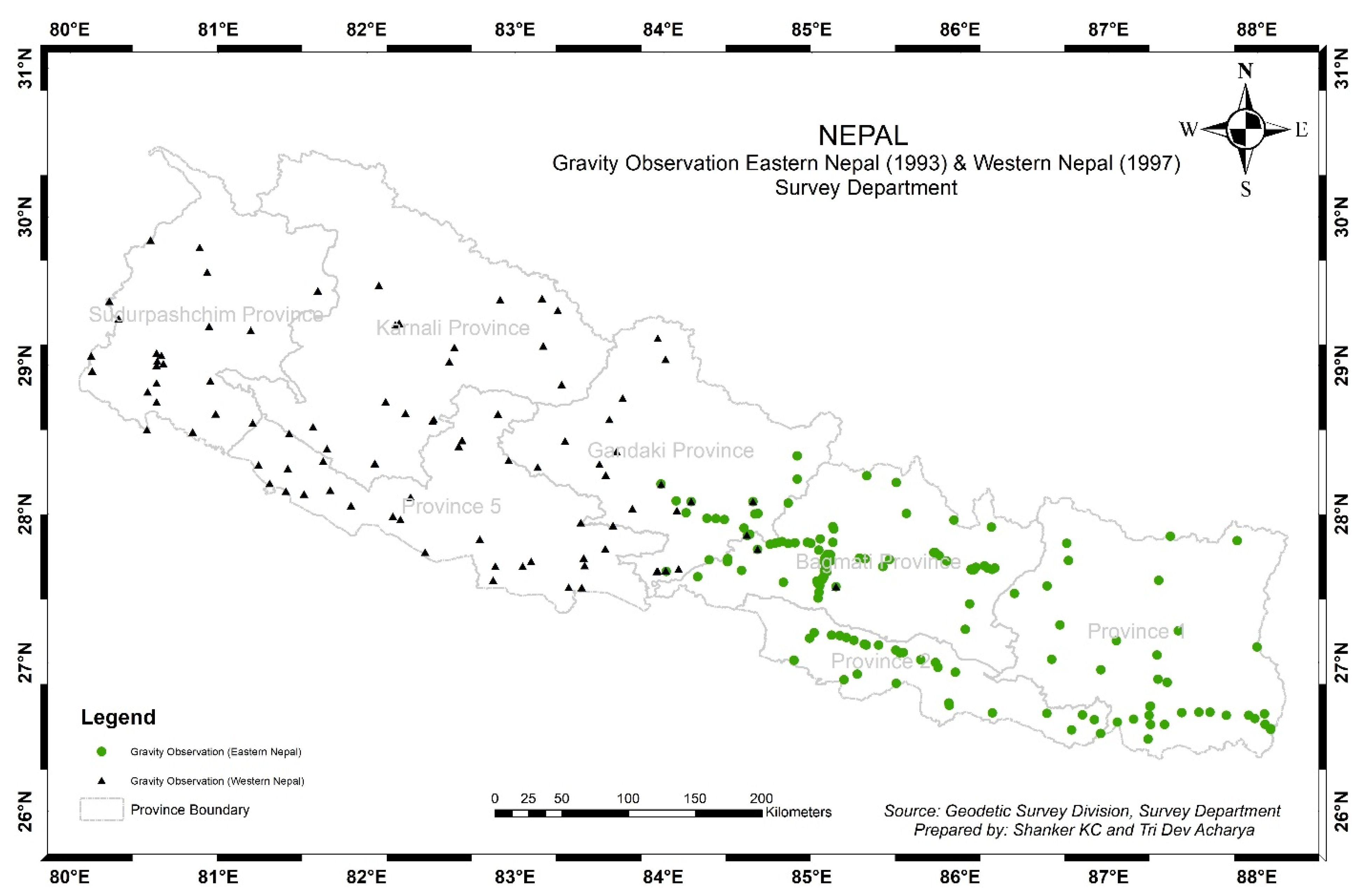
Gravity Survey in 1993 by ENTMP

During the ENTMP project, gravity was measured at ENTMP GPS stations, and new gravity stations were established as well using a Worden gravimeter. FAGS-1 of Nagarkot, gravity station of TIA, Kathmandu, and gravity station of Simara airport were used as the reference gravity station to carry out further relative gravity survey. The distribution of the gravity stations is as shown in Figure 13. GPS survey carried out over the precise leveling stations, which provided separation between MSL and ellipsoid called geoid undulations. These geoid separation data, along with gravity data from both existing and newly added gravity stations, were used to develop gravimetric geoids. This geoid is then used to provide heights above MSL published in topographic maps by ENTMP [48].

4.

Gravity Survey in 1997 by WNTMP and NEPAL97 Geoid

Within the project WNTMP, gravity observations were performed in several GPS stations using a Worden gravimeter. The distribution of gravity stations is as shown in Figure 13. The Finnish Geodetic Institute (FGI) developed the gravimetric geoid of Nepal using a combination of new gravity observations, gravity data from ENTMP, GPS-leveling differences, and EGM96. Geoid thus developed called NEPAL97 and has an accuracy of ±1 m [48]. This geoid is then used to provide heights above MSL published in topographic maps by WNTMP.

Figure 14 shows the geoidal contour of Geoid NEPAL97 computed during WNTMP by Finnish Geodetic Institute.

5.

Airborne Gravity Survey 2010

The countrywide airborne gravity survey was conducted by SD in 2010 AD in cooperation with the DTU Space, and NGA, USA. The reported root mean square is 3.3 mgal. These airborne gravity data were collected to build a new national geoid model of Nepal and to provide gravity data for global gravity models such as Earth Gravity Model 2020 (EGM2020). Geoid then computed has the accuracy of 10–20 cm [49]. Figure 15 shows the geoid of Nepal prepared after the airborne gravity survey 2010.

Gravity surveys mentioned above were carried out to help in surveying and mapping works. However, various international institutes and researchers have used a different kind of gravity measurement to study gravity increment before the 2015 Gorkha earthquake, to analyze gravity in the context of crustal structure, etc. Use of spaceborne gravity mission, the GRACE observations to study long-term ice mass loss over Himalaya, to study changes in groundwater storage, changes in gravity because of monsoon have been mentioned in Section 2.1.10 and Section 2.1.11. Chen et al. [51] recorded changes in gravity over four absolute gravity stations over southern Tibet, suggesting that these changes may be related to a mass change in the broad source region of the earthquake. Phuyal [52] used the surface gravity data and airborne gravity data of Nepal provided to the author by NGA to derive the various anomaly maps from analyzing the crustal structure of the Himalayas. The dissertation work found that gradual change in gravity around the source of the earthquake may be related to strain accumulation and mass relocation [52].

2.2. Post 2015 Gorkha Earthquake Era

2.2.1. Gorkha Earthquake 2015 and Its Impact on Horizontal and Vertical Datum

On 25 of April 2015, a 7.8 Mw earthquake with the epicenter at Barpak of Gorkha district hit Nepal seriously. Kathmandu basin and surrounding the lesser Himalayas were found to shift about 2 m southwest, and about 1 m uplift due to this earthquake analyzed from interferometry synthetic aperture radar (InSAR) method using European Space Agency (ESA) Sentinel-1 satellite imageries [53]. This abrupt shift and uplift of the landmass have distorted the integrity of both the Nepal datum and the vertical datum. Horizontal coordinates and height values are no longer consistent with the real ground.

Right after the Gorkha earthquake 2015, SD conducted a GNSS survey at five stations around Kathmandu valley. Coordinates obtained post-earthquake GNSS surveys were compared to pre-earthquake coordinates of the same stations. Nagarkot station was found to shift 1.82 m southwest and uplift 1.58 m, Lakhe Danda station was found to shift 0.99 m south and uplift 0.77 m. Similarly, Swayambhu, Kumari, and Phulchowki stations were found to shift 1.64 m southwest, 1.71 m southwest, and 0.92 m southwest and uplift 0.98, 1.093, and 0.63 m [54]. This result showed the average shift of Kathmandu valley is 1.416 m, and the average uplift is 0.92 m. These shifts of the ground because of the earthquake should be incorporated into coordinates of high-order geodetic controls to make it consistent.

2.2.2. CORS Data to Study Earthquake

CORS observations have been essential to study the nature of the 2015 Gorkha earthquake and associated deformations. A total of 33 CORS stations observations that spanned before and after earthquake time point and confirmed no transient pre-seismic displacements but found clear postseismic displacements [55] Huang et al. [56] used CORS stations from the Nepal region and Crustal Movement Observation Network of China (CMONOC) observations to study rupture propagation of the Gorkha earthquake and coseismic displacements. This earthquake is the largest continental thrust earthquake that has been recorded by 5 Hz GPS observations [56].

2.2.3. InSAR Measurements from International Communities

The 2015 Gorkha earthquake is the largest earthquake after the 1934 Bihar-Nepal earthquake [53]. The KKN4 CORS stations north of Kathmandu experience the largest displacements 1.8 m southward, 0.44 m westward, and uplift of 1.27 m [57].

Several research groups have studied the 2015 Gorkha earthquake using two major spaceborne geodetic observations: CORS and InSAR techniques to assess the surface displacements associated with the earthquake and other aspects of this earthquake. Previous large earthquakes before 2015 that occurred in the collision zone between the Indian and Eurasian plates do not have geodetic data available, but the 2015 Gorkha earthquake is studied in a more detailed manner with GPS and InSAR geodetic data [58].

A study by Grandin et al. [59] used Sentinel 1 A and ALOS-2 InSAR data, high-rate and static GPS data, and other relevant data to unfold the rupture process of the earthquake. Joint inversion of InSAR and GPS data to derive coseismic and postseismic slip on the MHT was performed by Luo et al. [60]. Three-dimensional surface displacements associated with the Gorkha earthquake by integrated InSAR and GPS observations with Extended Simultaneous and Integrated Strain Tensor Estimation From Geodetic and Satellite Deformation Measurement (ESISTEM) method have been derived by Luo et al. [60]. InSAR has been a powerful technique to study the surface displacements created by the earthquake with large spatial coverage and higher temporal resolution [57]. Feng et al. [57] analyzed coseismic interferograms from Sentinel 1A, ALOS-2, and RADARSAT-2 and three-dimensional displacements from GPS to derive the best-fit slip model for both 7.8 Mw mainshock and 7.3 Mw aftershock.

Wang et al. [61] also used InSAR and GPS data, investigated coseismic and postseismic deformations, and developed the finite element model (FEM) of the 2015 Gorkha earthquake that accounts for both topography variations and material properties of the Himalayan region. [62] used two decades of CORS data, InSAR data, and spirit leveling data to determine interseismic coupling along the MHT and spatial variations of strain rate. Hong et al. [63] used 3 years of InSAR data from Sentinel 1A and GPS observations to study the postseismic displacements after the Gorkha earthquake and attributed the observed postseismic displacements to afterslip.

United States Geological Survey (USGS) National Earthquake Information Center (NEIC) monitors and reports the globally occurring earthquakes [64]. NEIC PAGER reports about the 2015 Gorkha earthquake coseismic slip distributions derived from InSAR and GPS observations. NEIC got the coseismic displacements of the 2015 Gorkha earthquake at first from GPS observations provided by the National Aeronautics and Space Administration (NASA) advanced rapid imaging and analysis (ARIA) group. After NEIC got coseismic displacements obtained from Sentinel 1A interferograms provided by the Center for Observations and Modeling of Earthquakes, Volcanos, and Tectonics (COMET) group, later NEIC got coseismic displacements derived by UC San Diego provided ALOS-2 ScanSAR interferograms [64].

2.2.4. Everest Height Measurement 2020

Though height measurement of the peak of Mt Everest has been carried out in the past by several foreign countries and organizations, Nepal has not performed such scientific task of measurement of the height of the world’s tallest peak yet. Nepal has adopted the height measured by SOI from 1952 to 1954 until 2020. In addition, the continuous subduction of the Indian plate to the Eurasian plate is uplifting the Himalayas, and it is suspected that both horizontal position and elevation of peak might have changed by the 2015 Gorkha earthquake. Because various international organizations have measured and published several heights of the peak of Mt Everest, this necessitates the immediate need to maintain the consistency of height. Realizing this, SD organized the task of Everest height measurement using GNSS survey, precise leveling survey, gravity survey, and ground-penetrating radar. The accuracy of the geodetic surveys of this project will be published in the detailed document published by SD later. This project established the high precise GNSS control stations, gravity stations, leveling alignments around the east-west span of Mt Everest peak, beginning from southernmost Terai to the accessible northern region. An improved geoid model of this region is developed. All resources required for this project, such as human resources, technical resources, instruments, computing platforms, and software, were of SD. This new height of the peak referenced to IHRS 8848.86 m was jointly announced by Nepal and China in 8 December 2020. Some glimpses of activities during the project are available on the website of SD [65].

2.2.5. LiDAR Survey of Western Terai

SD began LiDAR survey of western Terai, covering Terai and Chure region from Chitwan to westernmost Kanchanpur district from May of 2021. The project is expected to deliver a high-resolution digital elevation model (DEM) and high-resolution orthophotos. The planimetric and altimetric accuracies of this LiDAR survey are expected to be better than 20 and 50 cm.

3. Modernization of Geodesy in Nepal

3.1. Conventional Geodesy

Until 2015, Nepal prepared TRS/TRF, HRS/HRF, GRS/GRF based on the concept of conventional geodesy and using conventional geodetic observation techniques. Conventional geodesy takes Earth as static while modern geodesy views Earth as Earth system approach, models its internal and external dynamic processes, and applies the changes thus occurred into reference system and frame [4]. Nepal had SOI established horizontal datum with Kalianpur origin from 1924 to 1986. This datum served as a conventional TRS/TRF until 1986. During 1981–1986, Nepal built a national datum called Nepal datum with Nagarkot origin realized by 68 first-order triangulation control stations. Nepal datum is our current TRF. Every surveying and mapping work after its release in 1986 is based on this TRS. Later, geodynamics study 1991, ENTMP 1993, WNTMP 1997 established high precise GPS reference control stations based on global TRS WGS84 and transformed coordinates of those reference control stations into Nepal datum. The current practice is GNSS survey is performed for control survey works geodetic coordinates are derived in GRS80 reference ellipsoid. Such coordinates are transformed into local TRS Nepal datum using localized transformation models and made available to the public. From history to the present, Nepal used and has been using three different TRSs; SOI datum with Kalianpur origin, Nepal datum with Nagarkot origin, and WGS84 datum. Though WGS84 datum is global, geocentric, and derived from space and satellite-based geodetic techniques, modern TRS, coordinates obtained from this system are transformed to national Nepal datum.

Nepal built a conventional height reference system and frame for elevation measurement purposes, and the reference surface is MSL of the Bay of Bengal defined around 1930, precise leveling was carried up to Indo–Nepal border at Datum Stations (DS), DSs were established with high precision. These DSs served as the height reference frame for Nepal. All other leveling alignments and benchmarks established throughout the country are relative to these DSs. Topographic mapping project of eastern Nepal, ENTMP 1993 used leveling heights, ellipsoidal heights from GPS survey, gravity data and developed the contour of equal geoidal undulations tied to MSL to provide heights in topographic maps. Similarly, WNTMP 1997 prepared NEPAL97 geoid, which is also tied to MSL to provide heights in topographic maps. Until present, GSD is carrying out precise leveling for vertical control. In 2010, SD carried out a nationwide airborne gravity survey, developed a preliminary national geoid model. and can be used as the reference surface for elevation measurement purposes. Using geoid as the reference surface for elevation measurement purposes is part of the modern height reference system, and it offers lots of advantages compared to conventional height reference systems. Nepal is working further toward the improvement of national geoid by incorporating more surface gravity data.

In 1981–1984, at the time of building Nepal datum, a gravity reference network tied to IGSN1971 was formed [45]. In 1991, high precision gravity stations at Nagarkot, Kathmandu airport, and Simara airport were established tied to FAGS at Nagarkot. FAGS at Nagarkot was established by absolute gravity survey while gravity station at Kathmandu and Simara airport was performed by relative gravity survey [46]. Later ENTMP 1993, WNTMP 1997, Airborne Gravity Survey 2010, and GSD did a gravity survey tied to this network.

3.2. Modern Geodesy

Modern geodesy is characterized by study, observation, and modeling of the three pillars of geodesy: geometry of the Earth, the orientation of the Earth in space, and gravity field of the Earth along with the interaction between each other, and changes in these pillars over time [4]. Observation of these three pillars and their changes over time is enabled by modern terrestrial, airborne, and spaceborne geodetic techniques. Determination of all three pillars and changes is directed to form high precise and accurate, higher spatial resolution, and temporal stability SRS.

Geodesy and Earth system is global by nature. For example, Earth rotation, crustal motion, sea-level change, etc., are not only the concern of a single nation. Because of this, international collaboration and cooperation have been made for global geodesy for global observation, global products, and services. Several global geodetic services are: IERS, International GNSS Service (IGS), International Laser Ranging Service (ILRS), Internation-al VLBI Service (IVS), International Doris Service (IDS), and Permanent Service for Mean Sea Level (PSMSL). Global geodetic services related to the gravity field are International Gravity Field Service (IGFS), International Service for Global Earth Models (ICGEM), International Digital Elevation Models Service (IDEMS), International Geodynamics and Earth Tide Service (IGETS), International Service for Geoid (ISG), International Gravimetric Bureau (BGI), and Combination of Service for Time-Variable Gravity Fields (COST-G) [63]. These services can be used while building an integrated national/regional-level modern SRS.

In the context of Nepal, the landmass of Nepal is constantly under the collision process of Indian and Eurasian plates, causing crustal motion and experiencing seismic deformation too. The 2015 Gorkha earthquake has caused significant surface deformation in eastern Nepal. Since these phenomena alter both the surface and gravity field, changes thus occurred must be observed, determined, and monitored through modern geodetic techniques and incorporated into SRS. Thus, unlike conventional SRSs, modern SRSs are dynamic, reflecting the time-dependent position of features and phenomena.

A geodynamics study made in 1991 revealed that the landmass of Nepal is moving at the rate of 20 mm/year [12]. In addition, Nepal datum has been hit seriously by the 7.8 Mw 2015 Gorkha earthquake. Coordinates assigned to reference control stations of this Nepal datum got distorted. The deformation caused by crustal motion and earthquake to Nepal datum has exceeded its precision and accuracy. This forced Nepal to build modernized NSRS. Similarly, modern geospatial data acquisition techniques such as LiDAR, RTK, etc., are compatible with modernized NSRS. Thus, building modernized NSRS is the necessity of the present time.

3.3. Conventional NSRS to Modern NSRS

Geodesy appears in service by providing the basis for defining and building an SRS. As the Earth is dynamic, the reference system should be time-dependent, which must reflect the continuous changes in the Earth’s surface reasonably. Nepal, too, must define and build the most fundamental NSRS, which is precise and accurate, compatible with the global reference system, based on modern-day geodetic techniques, modern technology friendlier, meet the needs of present-day stakeholders and users.

NSRS is a composite of terrestrial, height, and gravity reference systems. Each of these systems is realized through terrestrial reference frame, height reference frame, and gravity reference frame successively [66], as shown in Figure 16b.

Conventional NSRS of Nepal, Nepal datum as a TRS, MSL-based vertical datum, and gravity reference system have become fundamentally erroneous, have become obsolete in terms of accuracy, precision, and modern geospatial data acquisition technology, fulfill no modern-day requirements and necessities. Nepal must modernize its conventional NSRS and build, operate, maintain modernized NSRS.

3.3.1. Modern Terrestrial Reference System and Frame

TRS provides the foundation for positioning every Earth’s feature and phenomenon. TRS is made up of two major components: the definition of the coordinate system and the TRF [67]. Defining the coordinate system includes: specifying the origin, axes, and scale [67]. The coordinate system is an abstract notion. Such a coordinate system is brought to physical realization through a network of reference control stations. High precise and accurate coordinates and velocities of such stations are determined tied to the same coordinate system [67]. The network of such stations is called TRF.

CS reference control stations of conventional TRS do not have velocities determined and assigned. Modern TRS can provide time-dependent coordinates with the help of velocity models, plate motion models, and deformation models. Other examples of conventional TRS are North America Datum 1983 (NAD83) datum, Tokyo datum, etc.

Space- and satellite-based geodetic techniques enabled us to define a geocentric 3D Cartesian coordinate system, coordinates system of modern TRS. The origin of such systems is geo-center, the z-axis is aligned to the rotation axis of the Earth at some defined epoch, the x-axis is made coincident to the intersection line between the Greenwich meridian plane and equatorial plane, and the Y-axis is such that coordinate system is a right-handed coordinate system. This coordinate system is also called the Earth Centered Earth Fixed (ECEF) coordinates system, moves as the Earth rotates on its axis and revolves around the sun. Realization of such coordinate system is made through reference control stations distributed on Earth’s surface, whose high precise and accurate 3D coordinates and velocities are determined referenced to coordinate system and assigned to them forming reference frame. Continuous satellite-based geodetic observation is made at such reference control stations; thus, precise coordinates and velocities are determined at some epoch. Some suitable ellipsoid is chosen to obtain the curvilinear coordinates, i.e., latitudes and longitudes. For example, the Geodetic Reference System 1980 (GRS80) ellipsoid is taken as a reference surface for latitudes and longitudes by major TRS around the world. Modern geodesy has been able to observe, measure, and quantify change caused by such Earth’s processes and incorporate such changes into TRS. Big earthquake brings a change in the gravity field and orientation of the Earth. That is why coordinates of reference control stations of TRF are defined at some fixed epoch. If we want the coordinates of such stations at different epochs, then the velocity model, deformation model, or plate motion model is used, which transforms coordinates from one epoch to different epochs using movement rate of station and time interval between epochs. Such reference control stations are occupied with continuously operating GNSS receivers. Few numbers of such control stations might have VLBI, SLR, DORIS collocated together around the world. Most of the nation has the network of CORS as their TRF.

One of the known modern TRS is ITRS, defined by IERS and its realization ITRF. Until now, different ITRFs have been developed at regular time intervals reflecting the dynamic nature of the reference station and its coordinates. Recent ITRF being ITRF2020 after ITRF2014. Similarly, Japan has been using TRS called Japan Geodetic Datum 2000 (JGD2000) since 2002, but in 2011, off the Pacific coast of the Tohoku earthquake caused the large-scale crustal deformation, which necessitated the revision of coordinates of affected reference stations. With the revision, Japan released the updated TRS called JGD2011 [68].

The modern TRF for Nepal is the network of a set of reference control stations on which precise coordinates and velocities are assigned. These reference control stations are CORS stations. The number of such stations and distribution must be scientific, serving the geodetic purpose, economic, accessible, safeguard. A primary network with the bare minimum number of CORSs should be established first. Then secondary and tertiary networks: whose main purpose is the densification of control stations, should be based on a need basis. For example, an urban area might have denser secondary and tertiary CORSs than a remote village.

Continuously recorded geodetic measurements are processed, and coordinates of such stations are defined at a certain epoch called a reference epoch. Velocities are computed from coordinates of such stations from different epochs. If one wants the coordinates of a station other than a defined epoch, one can obtain that by velocities and time interval to reference the epoch. Such terrestrial frame is geocentric, compliant with ITRF, defined at reference epoch, and have a provision of change in coordinates after regular interval of years. Coordinates are 3D relative to geo-center. A reference ellipsoid must be introduced to obtain the coordinates in terms of latitude and longitude. This reference ellipsoid is not only part of the national TRS but also part of the height reference system and gravity reference system. GRS80 [69] ellipsoid should be adopted for Nepal NSRS.

3.3.2. Modern Height Reference System and Frame

Nepal must shift from the existing height system to a modern height system whose reference surface is the national geoid. A reliable national-level geoid must be developed using airborne gravity data from 2010 and surface gravity data collected afterward. Using geoid undulations from national geoid, ellipsoidal heights obtained from the GNSS survey then could be readily converted to orthometric height. This is called GNSS leveling, an alternative and modern-day solution of precise leveling. Geoid-based national height system could be connected to International Height Reference System (IHRS) using/computing offset between two surfaces: surface of IHRS and surface of the national geoid. Connection to IHRS enables to be part of the Global Geodetic Reference System (GGRS).

3.3.3. Modern Gravity Reference System and Frame

Gravity reference system and frame serve for national gravity anomaly, geoid, height system, vertical uplift and subsidence, the study of geodynamical processes and crustal deformation, changes in mass distribution due to earthquake, and many more. The gravity reference frame network consists of absolute gravity stations and relative gravity stations. Gravity at these stations is subject to temporal changes; thus, the periodic measurement of gravity value at those stations reveals crustal deformation, mass changes, uplift, and subsidence, which is required by geodynamics, seismology, ecology, and natural hazard. So, establishing a gravity reference system and the frame is not only the interest of SD but other national/regional/international organizations focusing on geophysics, geodynamics, cryosphere, environmental studies, climate change, and other scientific studies. SD must establish a gravity reference frame in cooperation with other organizations and institutes then operate, maintain, and build products and services.

4. Discussion and Future Directions

4.1. Nepalese Geodesy

Beginning from 1924 to the present, in almost 100 years, Nepal achieved several significant milestones in the geodesy of the country. In earlier times, it was SOI that carried out astronomical, triangulation, gravity survey, precise leveling survey for 1”:4 mile topographic maps in 1924–1927, 1”:1 mile topographic maps in 1946–1963, and Everest height measurement in 1952–1954. The need for systematic and fair land revenue collection led to the preparation of the systematic cadaster nationwide, which resulted in the establishment of SD in 1957, the establishment of education and training organization to produce survey professionals, the launch of the Survey and Measurement Act 1961, the launch of Land Reform Act 1963, beginning of free sheet-based cadastral mapping in 1963, and beginning of reference control points-based cadastral mapping since 1972. TSB was established in 1968. TSB used a triangulation network established by SOI and established lower-order reference control points, which were used for cadastral mapping purposes. Establishing lower-order control points and carrying out a cadastral survey, Nepal did this on its own financial, technical, instrumental, and human resources. TSB gradually started to conduct astronomical survey and gravity survey with UNDP assistance, transferred absolute gravity from Bangkok to TIA, established Nagarkot Geodetic Observatory, established 7 Laplace stations, and measured astronomic azimuth at those stations during the 1971–1977 period. Later 1981–1984 period, DoMS, MoDUK conducted a Doppler, astronomical, triangulation, gravity survey and developed the Nepal datum and gravity network completed in 1986. Afterward, high precision GPS reference controls were established, gravity survey and gravity network densification carried out, geoid developed, etc., by projects such as geodynamics study 1991 in cooperation with CU at Boulder, ENTMP 1993, WNTMP 1997. In addition, because of the unique continent-continent collision process over the Himalayas, several terrestrial-based and space-geodetic-based research projects to study the nature of the convergence process and the past earthquakes have been carried out. These were the major scientific and geodetic projects performed with foreign financial and technical assistance. TSB evolved to GSD, and GSD began GNSS surveying for reference control point densification, re-survey of higher-order reference control points of Nepal datum, spread precise leveling alignments. The airborne gravity survey was performed in 2010 with the help of DTU Space and NGA. A preliminary national-level geoid was developed.

The concept of modern geodesy gradually being prevailed. Advantages offered by modern geodetic techniques are gradually being realized. The necessity of modernization of NSRS: TRS, HRS, GRS, and others being realized. Geodynamics studies made in 1991 and other regional and international geodynamics studies helped to recognize crustal motion and its impact on the reference system. The 2015 Gorkha earthquake became the main trigger to step from static conventional NSRS to time-dependent modernized NSRS. The use of modern surveying techniques (terrestrial, airborne, spaceborne) by various stakeholders, surveying and mapping communities, geoscience communities’ force droves Nepal to establish modernized NSRS.

With the introduction of Geomatics Engineering in Nepal, GoN started to recruit geomatics engineers in 2012. These geomatics professionals began to put constructive and progressive momentum on surveying and mapping activities within SD and other public and private organizations. Moreover, few of these engineers are getting an advanced international degree in geodesy annually and bring new techniques and knowledge to the department. Recently a team of professionals took training in GNSS data processing software BERNESE and geoid computation. One immediate and significant output of such training was the computation of the height of Mt Everest in 2018−2020. These professionals would be key human resources for the modernization of NSRS. GSD has established two CORS stations at Nagarkot in 2005 and 2011 and just established another CORS station at SD premises in 2020, taking a step toward adopting modern geodetic techniques and others.

During the 2018–2020 period, Nepal re-measured the height of Mt Everest. GNSS-geoid approach was adopted. GNSS observation at the peak along with a network of temporary CORSs operated at numerous sites around the Everest region on the Nepal side. That network is tied to ITRF by using coordinates and velocities of nearby IGS stations and other CORSs within Nepal. Gravity stations were established at the regular grid from South Terai to North. Relative gravimetry was conducted to measure gravity at these stations. Precise leveling alignments were taken to stations as farthest north as possible. GNSS survey and gravity survey were also performed at the benchmarks along precise leveling alignments. These surface gravity data, GNSS, and leveling data, along with 2010 airborne gravity data and global gravity model used to develop the geoid around the Everest region. This geoid is tied to IHRS. Ellipsoidal height from the GNSS survey and geoid undulation from geoid in connection with IHRS provided the updated height of Mt Everest. Measurement of height of Mt Everest was performed using the science of modern geodesy, using modern space-based geodetic techniques. What Nepal did for Everest height measurement should be performed nationwide. This would be defining a geocentric, ITRS compliant TRS, establishing a network of CORSs, computing coordinates and velocities of these stations at reference epoch, conducting surface gravimetry nationwide, developing accurate enough national-level geoid.

The 2015 Gorkha earthquake hit serious our horizontal and vertical data and distorted the coordinates assigned to them. Damage to our conventional reference frame caused by this earthquake, the motion of our landmass because of crustal phenomena, proliferation, and wide use of GNSS and other modern surveying techniques, meeting accuracy and precision demand of various kinds of stakeholders and users direct us to define, establish, operate, and maintain modern NSRS.

4.2. Modern Terrestrial Reference System and Its Advantages

Reflecting on the past, Nepal made quite suitable progress in geodesy. However, this is not enough. Observation, products, and services of traditional geodesy begin to become obsolete. Traditional geodesy could not meet the required accuracy and precision of the modern-day geodetic community, of surveying and mapping community, of other geoscience communities, and many other stakeholders and users. The use of GNSS has completely changed the surveying scenario. Modern geodesy armed with space and satellite-based techniques provided the basis to define and develop geocentric, 3D, ITRS/ITRF compliant, dynamic national and regional TRS. China, Japan, Australia, and various other countries have modernized their NSRS, replacing their older datums. China replaced its older Xi’an geodetic coordinate system in 1980 using a modern reference system called Chinese Geodetic Coordinate System 2000 (CGCS2000) since 2008. Japan replaced its older Tokyo datum with JGD2000 in 2002. Australia has been using GDA2020 since 2017.

GNSS, a satellite-based positioning technique has become the major. In the case of Nepal, in the past, lower-order reference control stations such as secondary- and tertiary-level networks, such as reference control stations required for cadastral, topographical, engineering and construction surveys, and many more, were established through GNSS. All three: terrestrial, airborne, and spaceborne platform-based modern geospatial data acquisition methods use GNSS, so the acquired geospatial data aligned to ITRF, ITRF referenced data then converted to National Terrestrial Reference Frame (NTRF) referenced data through transformation models. Terrestrial surveys such as detail survey and stakeout with real-time kinematic (RTK), terrestrial laser scanning (TLS), mobile LiDAR survey, absolute and relative surface gravimetry, hydrographic surveys; airborne surveys such as airborne photogrammetric survey, airborne LiDAR, airborne gravimetry, unmanned aerial vehicle (UAV) surveys; spaceborne surveys such as remote sensing satellites, satellite gravity mission, etc. All these platforms and data from these are positioned with GNSS. So GNSS has become the de facto method in positioning. In the context of Nepal, RTK has been used for topographical surveying and stakeout purpose for large engineering and construction projects. TLS has been used for geology and geoscience research purposes. A nationwide airborne gravity survey was performed in 2010. The topographical survey for the Budhigandaki Hydropower site and Pokhara-Muktinath cable car site was performed by LiDAR survey. SD is conducting a LiDAR survey of western Terai and planning to conduct a LiDAR survey of eastern Terai soon in the coming years. UAV survey has become popular for acquiring geospatial data for planning, conservation of public and open areas, disaster risk reduction assessment, and reduction. All these works indicate that the fundamental reference system and frame of the nation should be such that our reference system and the frame is compliant with all above-mentioned modern surveying and mapping methods in terms of technology, accuracy, and precision, time.

4.3. Modern Height Reference System and Its Advantages

SD is using a height system composed of networks of benchmarks and leveling alignments referenced to MSL, whose elevations are determined through a precise leveling survey. The modern approach to establishing a height system that we call a modern height system, is through defining and developing geoids. Adopting geoid as the reference surface for height systems has several advantages over conventional MSL-based height systems. Our leveling networks have their origin at DSs established at the India–Nepal border, southernmost Terai. Elevation of these DSs is carried by precise leveling survey from leveling benchmarks in India, such as leveling alignment might not have directly traversed from MSL defined at the Bay of Bengal to our DSs; such alignment could have traveled a longer route before reaching our DS. Distortions and inconsistency might have crept in because of various factors while traversing such a long length from MSL to DS. Leveling alignment inside Nepal travels through plainland Terai, undulating hills, up to mountains of north. Because of this geography of Nepal having plainland, high hills, mountains, and the Himalayas, mass distribution is not uniform, causing variation in gravity while moving from south to north. To obtain proper orthometric height from a precise leveling survey, precise leveling height should be subjected to gravity correction. In mountainous terrain such as ours with the northern Himalayas. Such gravity correction is more pronounced than in plain and benign land. This requires measuring gravity value at those benchmarks at every 2 km interval [70]. Our leveling benchmarks and alignments do not have gravity measured; thus, no gravity correction has been applied. Gorkha earthquake 2015 has also distorted these leveling alignments and networks; thus, our existing height system is not consistent and does not maintain integrity. Our leveling alignments run along the road having hilly regions, very few leveling alignments, and almost no leveling alignments in a mountainous region. There are no precise leveling alignments above 3000 m elevation. It is almost impossible to carry out precise leveling surveys at high hills and mountainous regions. In addition, those monuments of benchmarks are prone to damage and being lost because of widening of the road, other construction activities, landslides, etc. Some monuments and survey markers are vandalized by unaware locals and in the absence of proper safeguard.

Adopting the height system based on geoid overcomes all the above-mentioned difficulties and offers other advantages. The geoid is referenced to the surface of the reference ellipsoid, thus connected to the TRS. GNSS provides 3D coordinates: ECEF cartesian coordinates, which are transformed to latitude, longitude, ellipsoidal height (φ, λ, h) coordinates. The third component is the ellipsoidal height (h) could be transformed to orthometric height with geoid information. In this way, the GNSS survey could provide the orthometric height of any point on Earth’s surface easily by a method called GNSS leveling. So, all the advantages and convenience offered by GNSS could be harnessed against a tedious precise leveling survey. The data source of geoid development is gravity data collected by surface, airborne, and spaceborne gravimetry. The airborne gravity survey and spaceborne gravity survey both have large spatial coverage. The terrain of the whole nation can be gravity surveyed in a very small period. Similarly, spaceborne gravity mission covers a whole globe. Just imagine covering the whole of Nepal with a precise leveling survey. It would be impossible, would take infinite time, would take an infinite amount of budget, and would take the huge crowd of surveyors. The modern height system is compatible with modern surveying methods. For example, DEM from either airborne photogrammetry, or airborne LiDAR or UAV surveys or spaceborne photogrammetries such as advanced spaceborne thermal emission and reflection radiometer (ASTER), ALOS, CartoSat or spaceborne synthetic aperture radar (SAR) such as shuttle radar topographic mission (SRTM) are initially referenced to the surface of the reference ellipsoid. Elevation of such DEM can be readily converted to orthometric height. The modern height system can easily be connected to IHRS. It takes computation of offset between national height system and IHRS and applies this offset while transforming heights from one system to another. Connection to IHRS provides avenues for scientific studies finding the height of Mt Everest, ice/glacier melting and climate change, environmental and ecology studies, sea-level changes, transnational engineering projects such as railways (China–Nepal railways), and many more.

4.4. Modern Gravity Reference System and Its Advantages

The gravity field of the Earth is determined through a mix of terrestrial, airborne, and spaceborne techniques. Spaceborne gravity observation techniques such as CHAMP, GRACE, GOCE provides global gravity field, airborne gravity survey provides national-scale gravity field, and surface gravimetry provides local scale gravity data. Gravity data from airborne and terrestrial gravity surveys need to be referenced to reference the gravity network. Reference gravity network consists of absolute gravity stations and base gravity stations whose gravity values are determined in a precise manner. In the context of Nepal, absolute gravity surveys were carried out between 1980 and 2000. The gravity field is subject to temporal changes necessitating the update of these absolute gravity stations and the establishment of new absolute gravity stations as not all absolute gravity stations from the past remained intact.

The gravity reference system is the basis for the development of geoid, which in turn is the basis for the height reference system. Adoption of a geoid-based height system in upcoming days necessitates the updated gravity reference system and frame as the new surface gravity survey is tied to this reference system. Similarly, heights from precise leveling should be subjected to gravity correction to convert these geometric heights to orthometric (physical heights). Gravity should be measured at every benchmark. The upcoming national leveling network of Nepal should be subjected to gravity correction to make it more meaningful physical heights.

Similarly, a periodic gravity survey could be used to determine subsidence or uplift of the ground caused by either crustal motion, earthquake, groundwater extraction, subsurface mineral extraction. Since Nepal’s landmass is under continuous crustal motion, a periodic absolute gravity survey could provide a valuable measure of vertical displacement. All these necessitate the establishment of a gravity reference system and gravity survey. Gravity is not only the interest of surveying and mapping but also equally important for other geosciences such as geodynamics, geophysics, environment and ecology, and others. So, the establishment of a gravity reference system and frame should be a collaborative effort of various organizations such as SD, organizations of geology, geophysics, geodynamics, universities, national and international-level research organizations, etc.

4.5. Modern NSRS and Its Advantages

Modern NSRS is the collection of TRSs/TRFs, HRSs/HRFs, GRSs/GRFs, geoid, velocity models, deformation models, etc. A spatial reference system (SRS) is the principal output of the geodesy, which is required for positioning and navigation. This SRS has characteristics of being highly precise and accurate, long-term stability, high spatial and temporal resolution. In addition, despite being long-term stable, such SRS provides a time-varying position of features too. Modern NSRS adopt two approaches: static and time-dependent. In a static approach, coordinates of the reference frame are defined at the reference epoch, and such coordinates are only updated at the regular interval after the amount of deformation over time gets greater than the accuracy and precision offered by the reference system. Surveying and mapping communities use such a static reference system at reference epoch. Other geoscience communities need a time-dependent reference frame; their research and studies need instantaneous coordinates. Velocity models in combination with both static and time-dependent reference frames provide the ability to transform coordinates between different epochs as per requirements.

Nepal’s land surface is under constant movement because of the collision process between the Indian and Eurasian plates. In addition, there have been large earthquakes, such as the 1934 Bihar-Nepal earthquake and the 2015 Gorkha earthquake. The modern NSRS is capable of measuring the slow creep due to crustal motion and sudden shift due to earthquakes and incorporating these changes into NSRS. Conventional SRS is neither able to quantify such minor movement nor able to incorporate to make itself dynamic nature.

Adopting a modern height reference system in combination with a modern TRS offers GNSS leveling capability. With GNSS leveling, ellipsoidal height can easily be converted to orthometric height using geoid. Although the precise leveling provides mm level height difference over a short distance, the error in precise leveling gets accumulated over a long distance. Thus GNSS leveling could be an alternative to traditional precise leveling when the geoid accuracy is at 1–2 cm level. Nepal’s topography, except for the Terai region, which covers only 17% of the total area of the country, is mountainous and the most northern part is the Himalayan region. Both mountainous regions and the Himalayas portion are geographically challenging terrain. Taking precise leveling surveys in these regions is very difficult. GNSS leveling is the most suited approach that can make the height system available in such terrain. Therefore, developing the cm-level geoid and adopting geoid to develop the modern height reference system is the best approach for Nepal.

Modernized NSRS is the basis that modern surveying methods such as RTK survey, airborne gravimetry, airborne LiDAR, UAV survey, remote sensing, etc., are compatible. Data from these methods are positioned to the modern terrestrial reference frame and modern height reference frame. Modernized NSRS provides the ability for integration of diverse geospatial data from various producers and various techniques and various periods into one system resulting in consistency in geospatial data. Several open global geospatial products and services need to be combined and analyzed with national geospatial products for various geoscience research and studies. Modernized NSRS makes this possible. For example, developing national-level geoid needs a global gravity model such as the Earth geopotential model 2008 (EGM08).

Modern NSRS is the way; there are various bilateral/regional/global issues related to Nepal, which could be solved using the modern NSRS. A recent example is Mt Everest height measurement. The position and height of Mt Everest are both bilateral and of global interest. China and Nepal jointly announced the updated height of the peak in 2020 referenced to IHRS. Nepal is bordered by China and India. Indo–Nepal border, China–Nepal border, and tri-junction between Nepal-India-China can be positioned to one common reference system, which is possible only when all three countries adopt modernized NSRS. Modernized NSRS is the fundamental infrastructure for transnational transportation systems such as Trans-Himalayas railways that connect China, Nepal, and India, India–Nepal railways. The northern topography of Nepal is the part of the Himalayas; 30% of the Himalayan range that extends from Kazakhstan at west to Bhutan at east, lies inside Nepal. Because of climate change, ice melting and glacier variations in the Himalayas are threats and challenging issues. Scientific research and studies of ice melting and glacier variations demand the modernized NSRS, which is consistent with the regional and global reference system.

4.6. Future Perspectives

Surveying and mapping activities in Nepal are governed by the Survey and Measurement Act 1961. This act does not explicitly mention NSRS, such as which TRS and frame to follow, which height reference system and frame to follow, which gravity reference system and frame to follow, accuracy and precision to be guaranteed, and others. Provision of establishment, operation, maintenance of NSRS and its other aspect must be mentioned in such act, which would then compel to work accordingly. The establishment, operation, maintenance of modernized NSRS and making products and services available is a challenging task. Such a task must be dealt with a strategic plan for a definite period to make it happen. Detailed step-by-step planning including every key aspect (what, when, where, how of every element of modernized NSRS) must be made so a clear picture of implementation can be seen. This approach motivates implementation, keeps track of work progress, and maintains consistency in work.

The use of already existing geodetic infrastructure and resources would be very beneficial for SD. There are networks of GPS that have run since 1991, and there are more than 30 GPS stations established throughout Nepal for various purposes by various international institutions in collaboration with national organizations and universities. Major is CIRES, LDG, and IDYLHIM networks. After 2002, the California Institute of Technology and UNAVCO, in cooperation with DMG, have run GPS networks for collision processes and earthquakes over the Himalayas. Tribhuvan University, Kathmandu University, Institutes from China, and others have established CORS stations. SD should take a step further and bring all these scattered CORS resources under its jurisdiction and make them part of the national TRF. In addition, SD should act as an authoritative organization that every party who builds geodetic infrastructure must do so under SD’s policy and directives.

It would be very difficult for only SD to work toward the modernization of NSRS. Collaboration, cooperation, and partnering with international geodetic organizations would be very helpful. We could obtain advantages from their strength, experiences, and resources so that the modernization of NSRS in our country could be performed successfully. There are various other geoscience-related public organizations such as the Department of Mines and Geology (DMG) and others, academic institutions and universities, regional and international organizations, and various stakeholders participating in this endeavor would be suitable. Organizations and researchers of astronomy, geodynamics, geophysics, cryosphere, seismology, environment and ecology, and many others should be encouraged to work together on this endeavor.

Human resources within SD are the key aspect for building modernized NSRS, especially geodesy professionals to plan, design, establishment, implement the modernization of NSRS and operate/maintenance and make available products and services. At present, there are not enough such geodesy professionals in SD. So competent geodesy professionals must be built, via various ways, either through training or capacity building or involving them into graduate and postgraduate geodesy studies or through the combination of these approaches. Similarly, computing facility and data center is another key component of modernization, high processing capacity computers, large storage devices and non-stop running servers, database and computing software plus competent geodesy professionals forms the well-run computing, processing, delivery platform.

Nepal should aim to be the excellence of geodesy and geodetic works at national, regional, and global levels gradually. Modernization of NSRS could be the starting point toward the journey of excellence organization of geodesy that can only be achieved by a combination of modern space-based geodetic observations for geodynamics measurements with high precision at different spatial and temporal resolutions. Reading this review would provide detailed knowledge about the geodesy of Nepal and can encourage Nepal and interested stakeholders to build modernized NSRS and help understand the dynamic Earth phenomena.