Improving the Inversion Accuracy of Terrestrial Water Storage Anomaly by Combining GNSS and LSTM Algorithm and Its Application in Mainland China
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
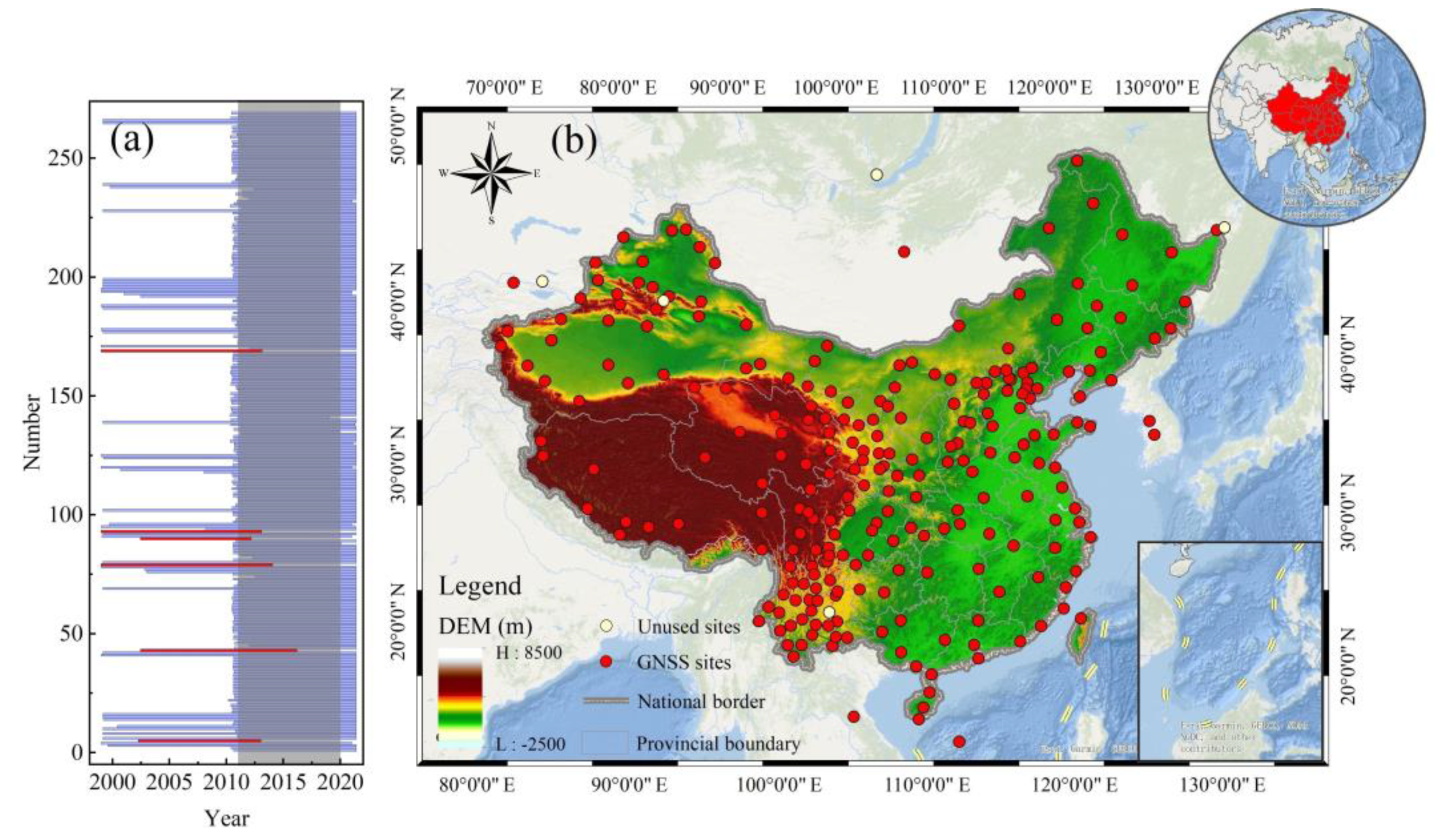
2.1.1. GNSS Datasets
2.1.2. GRACE Datasets
2.1.3. Auxiliary Datasets
2.2. Methods
2.2.1. LSTM Algorithm
2.2.2. The Crustal Load-Deformation Model
2.2.3. Construction of DWLIM
- (1)
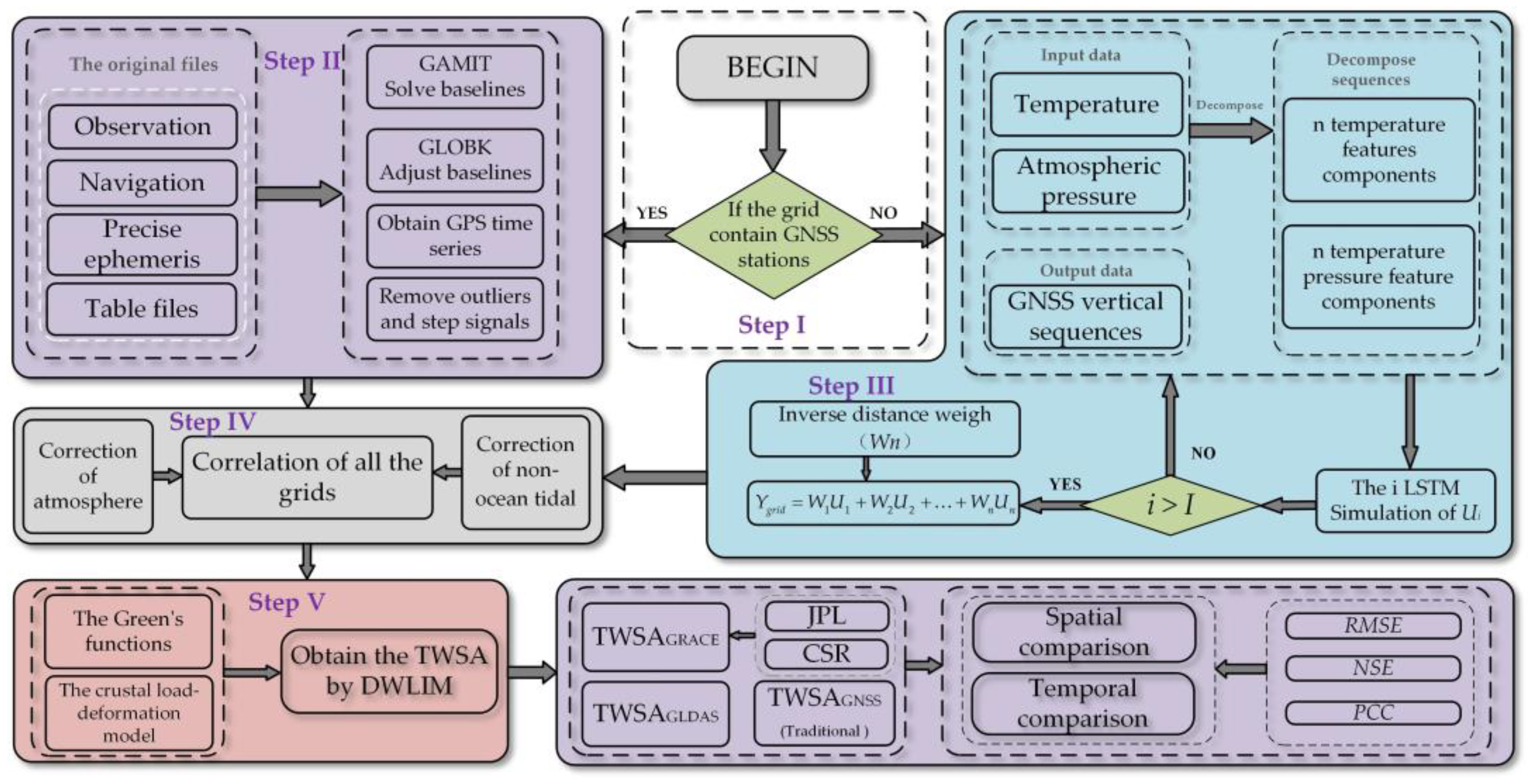
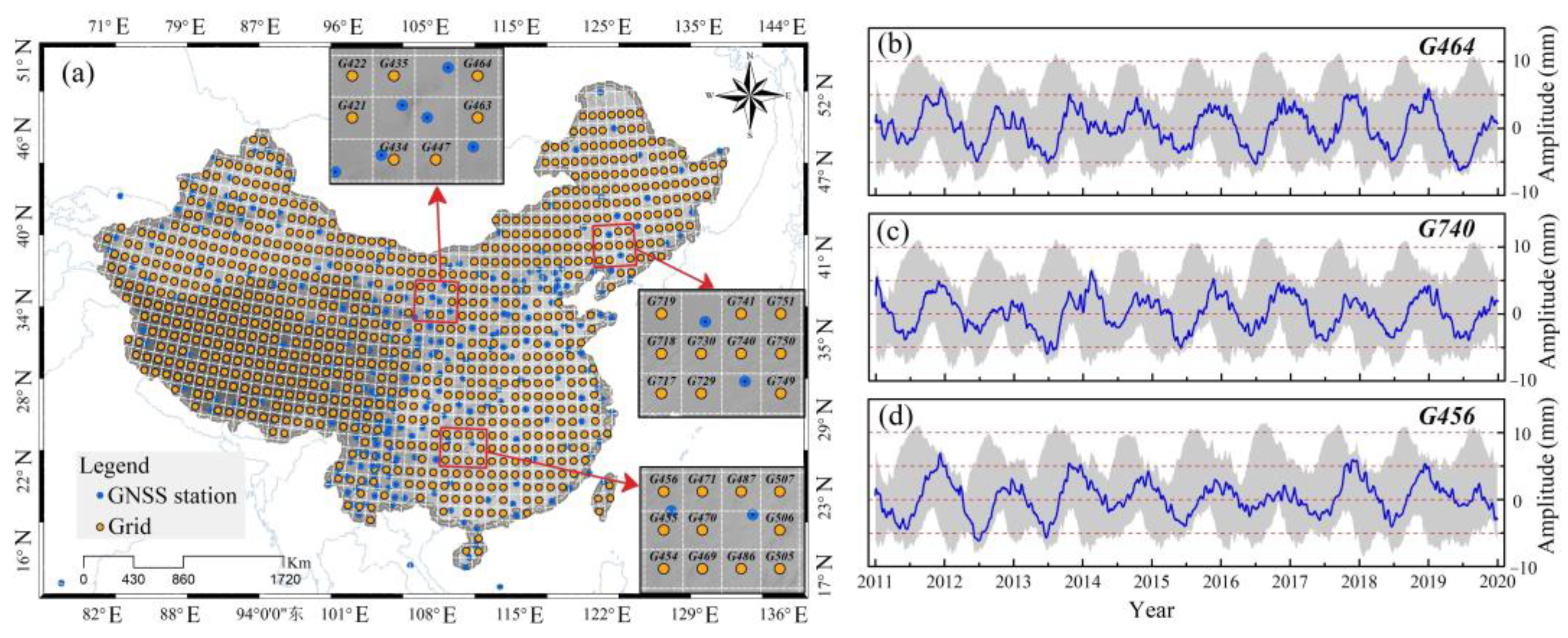
- Step I: The study region is divided into 1° × 1° grids, and the grids are divided into two situations; specifically, the grids contain or do not contain GNSS stations. This algorithm will proceed to step II if the grid has GNSS stations. Moreover, the grid will be defined as an unobserved grid if it does not contain GNSS stations, and the vertical deformation will be simulated in step III.
- (2)
- Step II: The GNSS coordinate solution will be calculated by using observation, precision ephemeris, navigation, and table files based on GAMIT software [42]. The daily coordinates are calculated by the GLOBK software based on baseline data files (h-files), and series outliers and step terms that are three times larger than the standard deviation are removed.
- (3)
- Step III: The surface temperature sequence (ST) and atmosphere pressure sequence (SAP) are normalized on the grid scale. Furthermore, the normalized results are decomposed using the modified ensemble empirical mode decomposition (MEEMD) method to obtain 2 n feature sequences, including n ST and n SAP feature sequences. In the unobserved grid, the GNSS vertical deformation sequences are employed as the target sequences, and the 2 n feature sequences are utilized as the input sequences. Then, the LSTM regression method and the inverse distance weight method are employed to simulate the vertical displacement.
- (4)
- Step IV: The corrected sequences of atmospheric (NTAL) and non-tidal ocean loading (NTOL) are employed to obtain the hydrologic deformation in all the grids, including the GNSS grids and unobserved grids [56].
- (5)
- Step V: The TWSA results are obtained by combining the Green function and the inversion of the crustal load model with all hydrologic deformation. The flow chart of this study is shown in Figure 2.
2.3. Evaluation Index
3. Results
3.1. Inversion of TWSA Using DWLIM
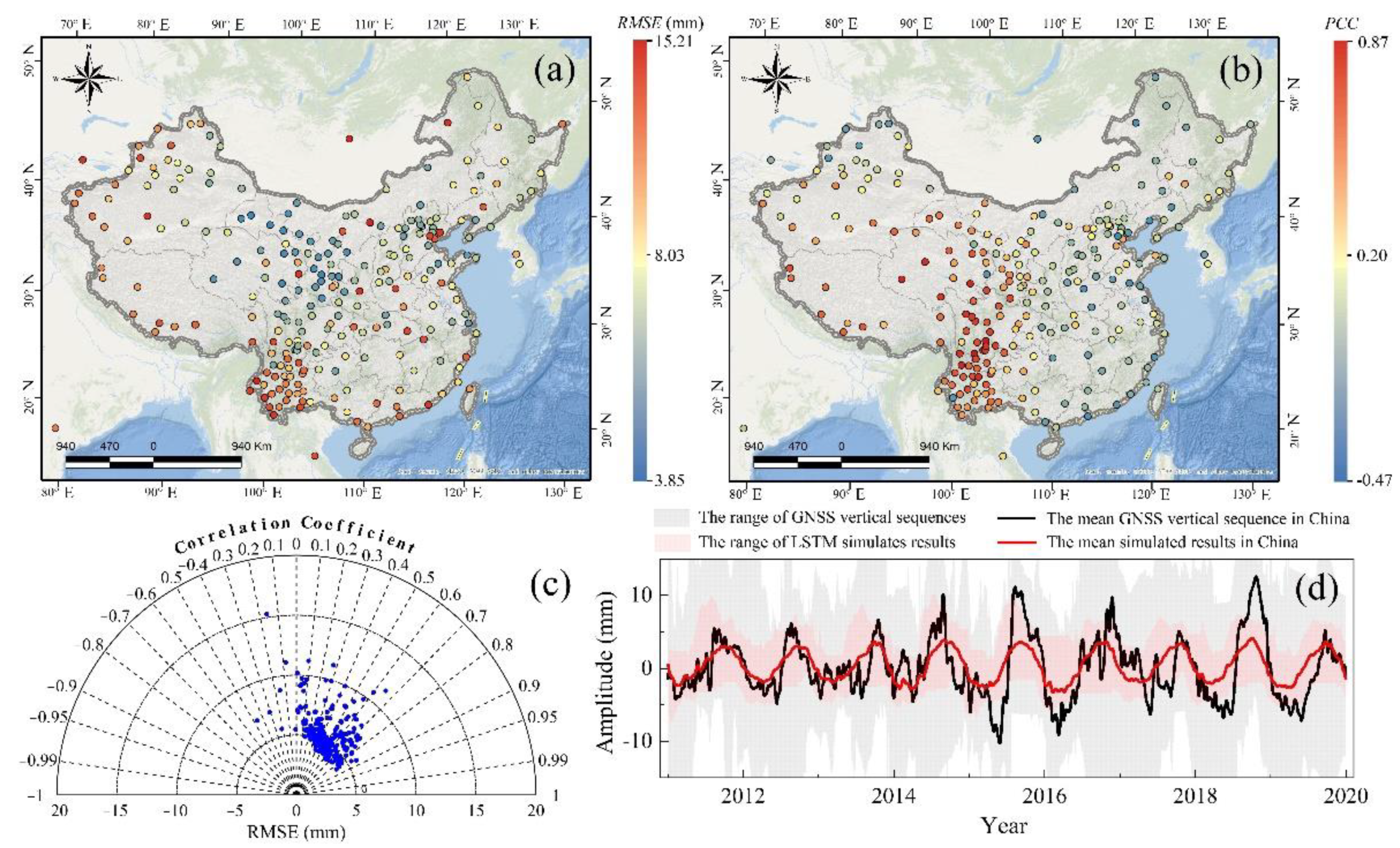
3.1.1. Validation of Simulated Crustal Deformation
3.1.2. Simulation of Hydrological Load-Deformation
- (1)
- Simulation of crustal deformation
- (2)
- Correction of all deformation sequences
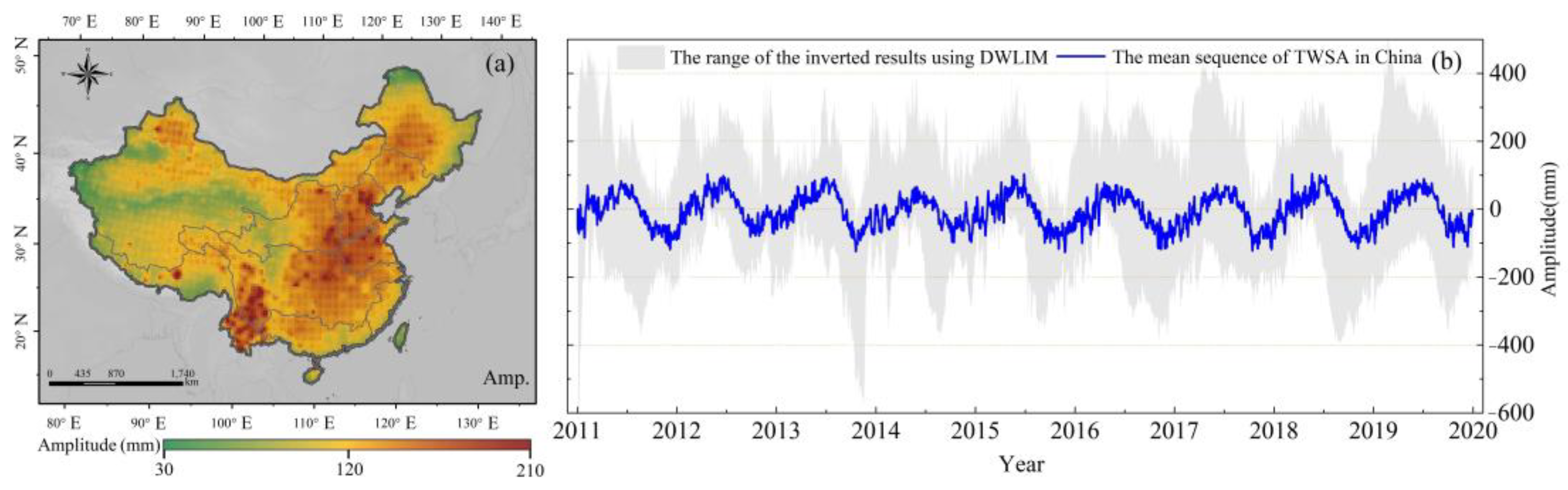
3.1.3. Inversion of TWSA Based on DWLIM
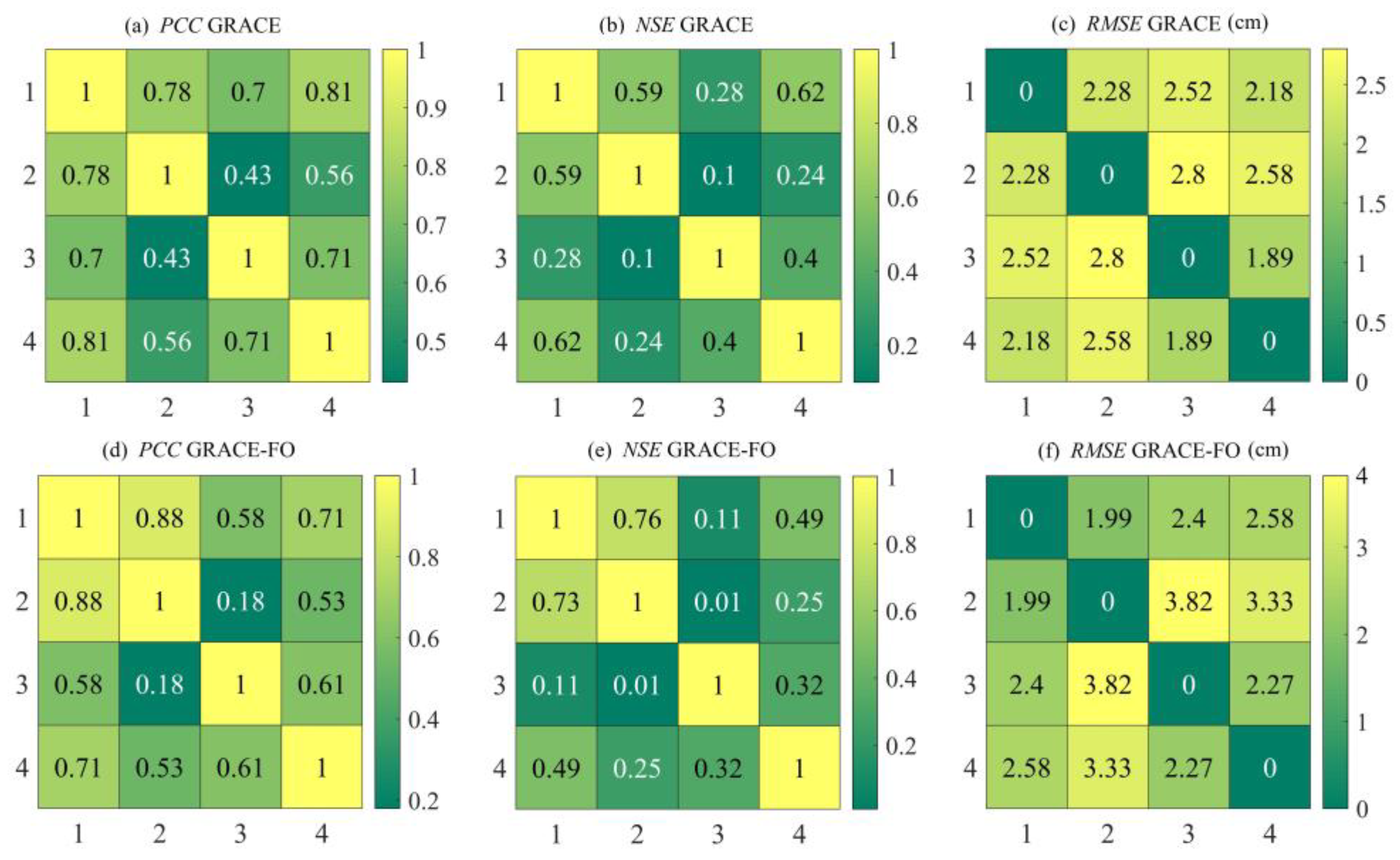
3.2. Validation of DWLIM
3.2.1. Spatial Verification of TWSA Results
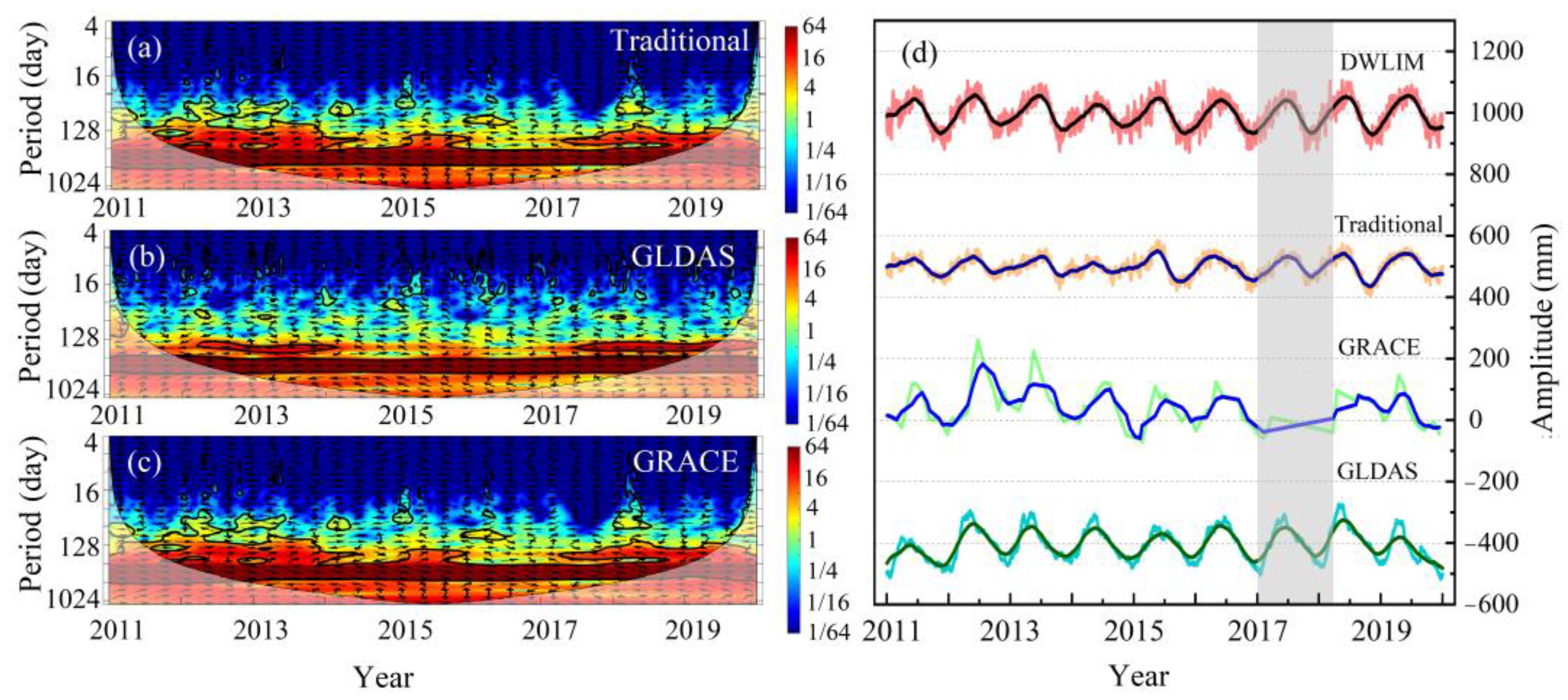
3.2.2. Temporal Verification of the TWSA Results
4. Discussion
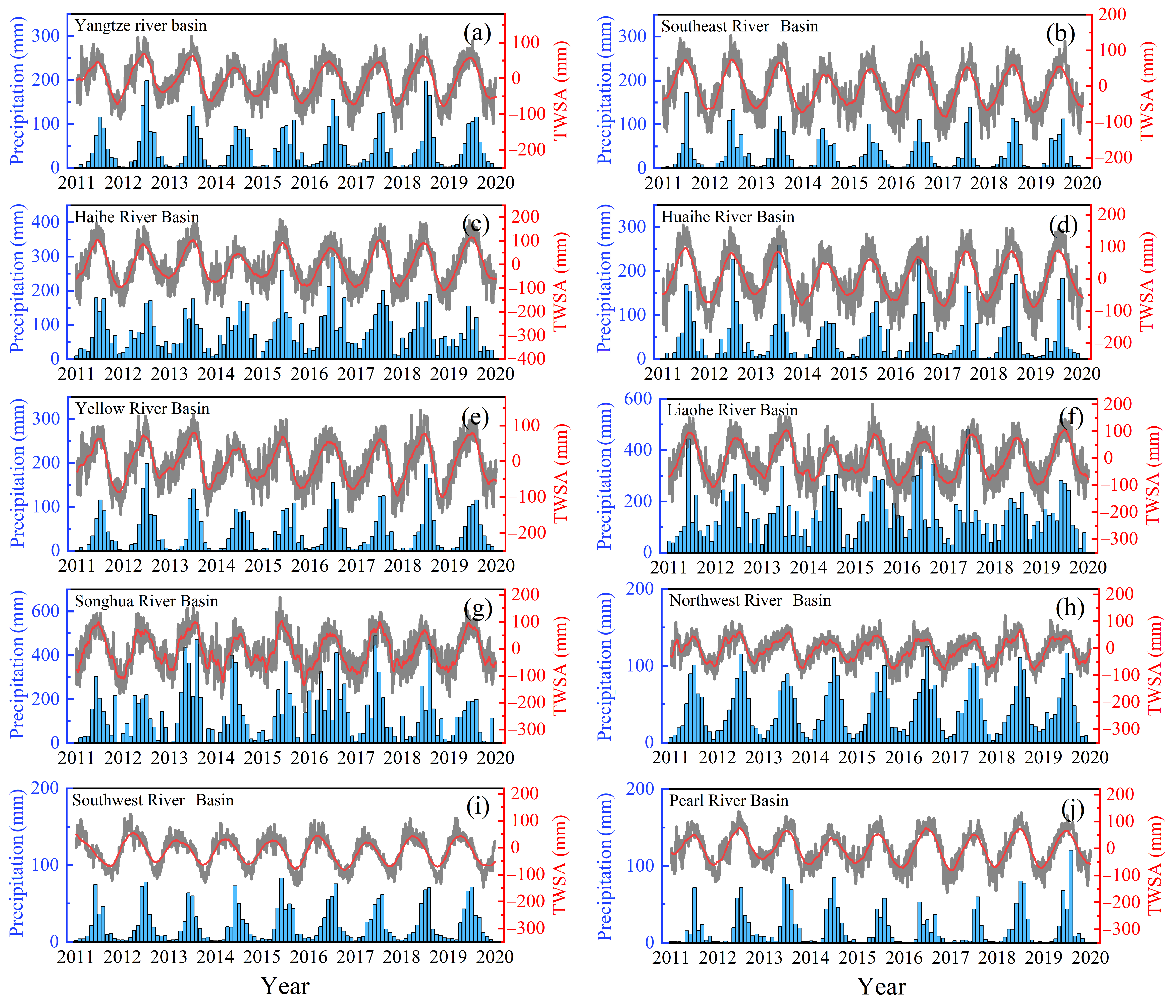
4.1. Comparison with Precipitation over 10 River Basins
4.2. Discussion of the Difference between Products
5. Conclusions
- (1)
- To increase the derived accuracy for TWSA, DWLIM was constructed by combining LSTM, inverse distance weight, and the crustal load-deformation model. First, the study region was divided into 1° × 1° grids, and then we determined whether the grid contained GNSS stations. Second, this study selected the surface temperature and atmospheric pressure as input data, and the GNSS vertical sequences were utilized as the output data. Each unobserved grid was simulated 263 times, and the inverse distance weight was used to calculate the weighted sequence. Third, the NTAL and NTOL models were employed to correct vertical deformation over all of the grids to obtain the hydrologic distribution. Finally, all of the corrected sequences were used as the input data for the crustal load model to derive TWSA in mainland China.
- (2)
- To verify the accuracy of DWLIM, the TWSA results of DWLIM were compared with the traditional GNSS TWSA inversion, GRACE, and GLDAS results. The results indicate that the annual amplitude distribution of DWLIM is smoother than the traditional GNSS inversion results. The strategy of DWLIM greatly suppresses the effect of a small disk expansion radius. The maximum PCC, NSE, and RMSE of DWLIM results compared with GRACE and GLDAS are equal to 0.81, 0.62, and 2.18 cm, respectively, which are improved by 67.11, 128.15, and 22.75% compared with the traditional GNSS-derived TWSA method, respectively. Overall, the DWLIM can effectively invert the TWSA in regions with an uneven distribution of GNSS stations.
- (3)
- This study employed precipitation data to analyze the relationship between TWSA and rainfall. We inverted TWSA based on DWLIM in 10 river basins of mainland China. The results indicate that TWSA is positively correlated with precipitation. The annual amplitudes of precipitation and TWSA in the Songhua River basin and the Liaohe River basin are significantly higher than those in other basins. Furthermore, the wave peaks of precipitation are in good agreement with the peaks of TWSA, which are located in June or July. This result further verifies the reliability of the DWLIM inversion results in terms of phase.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Long, D.; Shen, Y.; Sun, A.; Hong, Y.; Longuevergne, L.; Yang, Y.; Li, B.; Chen, L. Drought and flood monitoring for a large karst plateau in southwest China using extended GRACE data. Remote Sens. Environ. 2014, 155, 145–160. [Google Scholar] [CrossRef]
- Ahi, G.O.; Cekim, H.O. Long-term temporal prediction of terrestrial water storage changes over global basins using GRACE and limited GRACE-FO data. Acta Geod. Geophys. 2021, 56, 321–344. [Google Scholar] [CrossRef]
- Shen, Y.; Zheng, W.; Yin, W.; Xu, A.; Zhu, H.; Yang, S.; Su, K. Inverted algorithm of terrestrial water-storage anomalies based on machine learning combined with load model and its application in southwest China. Remote Sens. 2021, 13, 3358. [Google Scholar] [CrossRef]
- Yin, W.; Hu, L.; Han, S.C.; Zhang, M.; Teng, Y. Reconstructing terrestrial water storage variations from 1980 to 2015 in the Beishan area of China. Geofluids 2019, 2019, 3874742. [Google Scholar] [CrossRef]
- Li, W.; Wang, D.; Liu, S.; Zhu, Y.; Yan, Z. Reclamation of Cultivated Land Reserves in Northeast China: Indigenous Ecological Insecurity Underlying National Food Security. Int. J. Environ. Res. Public Health 2020, 17, 1211. [Google Scholar] [CrossRef] [Green Version]
- Davis, J.L.; Elosegui, P.; Mitrovica, J.X.; Tamisiea, M.E. Climate-driven deformation of the solid Earth from GRACE and GPS. Geophys. Res. Lett. 2004, 31, L24605. [Google Scholar] [CrossRef] [Green Version]
- Li, W.; Wang, W.; Zhang, C.; Wen, H.; Zhong, Y.; Zhu, Y.; Li, Z. Bridging terrestrial water storage anomaly during GRACE/GRACE-FO gap using SSA method: A case study in China. Sensors 2019, 19, 4144. [Google Scholar] [CrossRef] [Green Version]
- Jiang, Z.; Hsu, Y.-J.; Yuan, L.; Cheng, S.; Li, Q.; Li, M. Estimation of daily hydrological mass changes using continuous GNSS measurements in mainland China. J. Hydrol. 2021, 598, 126349. [Google Scholar] [CrossRef]
- Jin, S.; Zhang, T. Terrestrial water storage anomalies associated with drought in southwestern USA from GPS observations. Surv. Geophys. 2016, 37, 1139–1156. [Google Scholar] [CrossRef]
- Yin, W.; Hu, L.; Zhang, M.; Wang, J.; Han, S. Statistical downscaling of GRACE-derived groundwater storage using ET data in the North China Plain. J. Geophys. Res. Atmos. 2018, 123, 5973–5987. [Google Scholar] [CrossRef]
- Tangdamrongsub, N.; Šprlák, M. The assessment of hydrologic- and flood-induced land deformation in data-sparse regions using GRACE/GRACE-FO data assimilation. Remote Sens. 2021, 13, 235. [Google Scholar] [CrossRef]
- Jiang, Z.; Hsu, Y.-J.; Yuan, L.; Huang, D. Monitoring time-varying terrestrial water storage changes using daily GNSS measurements in Yunnan, southwest China. Remote Sens. Environ. 2021, 254, 112249. [Google Scholar] [CrossRef]
- Schmidt, R.; Flechtner, F.; Meyer, U.; Neumayer, K.-H.; Dahle, C.; Knig, R.; Kusche, J. Hydrological signals observed by the GRACE satellites. Surv. Geophys. 2008, 29, 319–334. [Google Scholar] [CrossRef]
- Xiang, Y.; Yue, J.; Cong, K.; Xing, Y.; Cai, D. Characterizing the seasonal hydrological loading over the asian continent using GPS, GRACE, and hydrological model. Pure Appl. Geophys. 2019, 176, 5051–5068. [Google Scholar] [CrossRef]
- Syed, T.H.; Famiglietti, J.S.; Rodell, M.; Chen, J.; Wilson, C.R. Analysis of terrestrial water storage changes from GRACE and GLDAS. Water Resour. Res. 2008, 44, 2433–2448. [Google Scholar] [CrossRef]
- Moghim, S. Assessment of water storage changes using GRACE and GLDAS. Water Resour. Manag. 2020, 34, 685–697. [Google Scholar] [CrossRef]
- Zheng, W.; Xu, Z.; Zhong, M.; Yun, M. Efficient accuracy improvement of GRACE global gravitational field recovery using a new inter-satellite range interpolation method. J. Geodyn. 2012, 53, 1–7. [Google Scholar] [CrossRef]
- Feng, W.; Zhong, M.; Lemoine, J.M.; Biancale, R.; Hsu, H.T.; Xia, J. Evaluation of groundwater depletion in North China using the Gravity Recovery and Climate Experiment (GRACE) data and ground-based measurements. Water Resour. Res. 2013, 49, 2110–2118. [Google Scholar] [CrossRef]
- Fok, H.S.; Liu, Y. An improved GPS-inferred seasonal terrestrial water storage using terrain-corrected vertical crustal displacements constrained by GRACE. Remote Sens. 2019, 11, 1433. [Google Scholar] [CrossRef] [Green Version]
- Fu, Y.; Argus, D.F.; Freymueller, J.T.; Heflin, M.B. Horizontal motion in elastic response to seasonal loading of rain water in the Amazon Basin and monsoon water in Southeast Asia observed by GPS and inferred from GRACE. Geophys. Res. Lett. 2013, 40, 6048–6053. [Google Scholar] [CrossRef]
- Velicogna, I. Increasing rates of ice mass loss from the Greenland and Antarctic ice sheets revealed by GRACE. Geophys. Res. Lett. 2009, 36, L19503. [Google Scholar] [CrossRef] [Green Version]
- Liu, R.; Zou, R.; Li, J.; Zhang, C.; Zhao, B.; Zhang, Y. Vertical displacements driven by groundwater storage changes in the North China Plain detected by GPS observations. Remote Sens. 2018, 10, 259–272. [Google Scholar] [CrossRef] [Green Version]
- Zhong, B.; Li, X.; Chen, J.; Li, Q.; Liu, T. Surface mass variations from GPS and GRACE/GFO: A case study in southwest China. Remote Sens. 2020, 12, 1835–1846. [Google Scholar] [CrossRef]
- Pan, Y.; Zhang, C.; Gong, H.L.; Yeh, P.J.F.; Shen, Y.; Guo, Y.; Huang, Z.; Li, X. Detection of human-induced evapotranspiration using GRACE satellite observations in the Haihe River Basin of China. In Proceedings of the Egu General Assembly Conference, Vienna, Austria, 23–28 April 2017; pp. 190–199. [Google Scholar]
- Vishwakarma, B.D.; Zhang, J.; Sneeuw, N. Downscaling GRACE total water storage change using partial least squares regression. Sci. Data 2021, 8, 95. [Google Scholar] [CrossRef] [PubMed]
- Hussain, D.; Khan, A.A.; Hassan, S.N.U.; Naqvi, S.A.A.; Jamil, A. A time series assessment of terrestrial water storage and its relationship with hydro-meteorological factors in Gilgit-Baltistan region using GRACE observation and GLDAS-Noah model. SN Appl. Sci. 2021, 3, 533. [Google Scholar] [CrossRef]
- Chen, C.; Zou, R.; Liu, R.L. Vertical deformation of seasonal hydrological loading in southern tibet detected by joint analysis of GPS and GRACE. Geomat. Inf. Sci. Wuhan Univ. 2018, 43, 669–675. [Google Scholar]
- Dagan, G. Solute transport in heterogeneous porous formations. Water Resour. Res. 2004, 55, 671–682. [Google Scholar] [CrossRef]
- Fu, Y.; Freymueller, J.T. Seasonal and long-term vertical deformation in the Nepal Himalaya constrained by GPS and GRACE measurements. J. Geophys. Res. Solid Earth 2012, 117, B03407. [Google Scholar] [CrossRef]
- Wahr, J.; Khan, S.A.; Dam, T.V.; Liu, L.; Angelen, J.V.; Van, D.; Meertens, C.M. The use of GPS horizontals for loading studies, with applications to northern California and southeast Greenland. J. Geophys. Res. Solid Earth 2013, 118, 1795–1806. [Google Scholar] [CrossRef] [Green Version]
- Gautam, P.K.; Gahalaut, V.K.; Prajapati, S.K.; Kumar, N.; Yadav, R.K.; Rana, N.; Dabral, C.P. Continuous GPS measurements of crustal deformation in Garhwal-Kumaun Himalaya. Quat. Int. 2017, 462, 124–129. [Google Scholar] [CrossRef]
- Argus, D.F.; Fu, Y.; Landerer, F.W. Seasonal variation in total water storage in California inferred from GPS observations of vertical land motion. Geophys. Res. Lett. 2014, 41, 1971–1980. [Google Scholar] [CrossRef]
- Argus, D. Sustained water changes in California during drought and heavy precipitation inferred from GPS, InSAR, and GRACE. In Proceedings of the Agu Fall Meeting, San Francisco, CA, USA, 14–18 December 2015. [Google Scholar]
- Borsa, A.A.; Agnew, D.C.; Cayan, D.R. Ongoing drought-induced uplift in the western United States. Science 2014, 345, 1587–1590. [Google Scholar] [CrossRef] [PubMed]
- Enzminger, T.L.; Small, E.E.; Borsa, A.A. Accuracy of snow water equivalent estimated from GPS vertical displacements: A synthetic loading case study for western U.S. mountains. Water Resour. Res. 2018, 54, 581–599. [Google Scholar] [CrossRef]
- Heki, K. Dense gps array as a new sensor of seasonal changes of surface loads. In The State of the Planet: Frontiers and Challenges in Geophysics; American Geophysical Union: Washington, DC, USA, 2004; Volume 150, pp. 177–196. [Google Scholar]
- Adusumilli, S.; Borsa, A.A.; Fish, M.A.; McMillan, H.K.; Silverii, F. A decade of water storage changes across the contiguous united states from GPS and satellite gravity. Geophys. Res. Lett. 2019, 46, 13006–13015. [Google Scholar] [CrossRef]
- Liu, R.; Li, J.; Fok, H.; Shum, C.K.; Li, Z. Earth surface deformation in the North China Plain detected by joint analysis of GRACE and GPS data. Sensors 2014, 14, 19861–19876. [Google Scholar] [CrossRef] [Green Version]
- Pan, Y.; Shen, W.B.; Ding, H.; Hwang, C.; Li, J.; Zhang, T. The quasi-biennial vertical oscillations at global GPS stations: Identification by ensemble empirical mode decomposition. Sensors 2015, 15, 26096–26114. [Google Scholar] [CrossRef]
- Zheng, G.; Wang, H.; Wright, T.J.; Lou, Y.D.; Zhang, R.; Zhang, W.X.; Shi, C.; Huang, J.F.; Wei, N. Crustal deformation in the India-Eurasia collision zone from 25 years of GPS measurements: Crustal deformation in Asia from GPS. J. Geophys. Res. Solid Earth 2017, 122, 9290–9312. [Google Scholar] [CrossRef]
- Shen, Y.; Zheng, W.; Yin, W.; Xu, A.; Zhu, H. Feature extraction algorithm using a correlation coefficient combined with the VMD and its application to the GPS and GRACE. IEEE Access 2021, 9, 17507–17519. [Google Scholar] [CrossRef]
- Herring, T.A.; King, R.W.; Mcclusky, S.C. GAMIT Reference Manual. 2010. [Google Scholar]
- Xue, K. Combined GRACE and GPS to Study Terrestrial Water Storage; Chang’an University: Xi’an, China, 2017. [Google Scholar]
- Ji, L.; Senay, G.B.; Verdin, J.P. Evaluation of the global land data assimilation system (GLDAS) air temperature data products. J. Hydrometeorol. 2015, 16, 150731131106004. [Google Scholar] [CrossRef]
- Yu, X.; Zhang, L.; Zhou, T.; Liu, J. The Asian subtropical westerly jet stream in CRA-40, ERA5, and CFSR reanalysis data: Comparative assessment. J. Meteorol. Res. 2021, 35, 46–63. [Google Scholar] [CrossRef]
- Hochreiter, S.; Schmidhuber, J. Long Short-Term Memory. Neural Comput. 1997, 9, 1735–1780. [Google Scholar] [CrossRef] [PubMed]
- Yao, Y.; Sfarra, S.; Ibarra-Castanedo, C.; You, R.; Maldague, X.P.V. The multi-dimensional ensemble empirical mode decomposition (MEEMD). J. Therm. Anal. Calorim. 2017, 128, 1841–1858. [Google Scholar] [CrossRef]
- Wang, H.; Xiang, L.; Jia, L.; Jiang, L.; Wang, Z.; Hu, B.; Gao, P. Load love numbers and Green’s functions for elastic earth models PREM, iasp91, ak135, and modified models with refined crustal structure from Crust 2.0. Comput. Geosci. 2012, 49, 190–199. [Google Scholar] [CrossRef]
- Wang, Z.; Ou, J. Determining the ridge parameter in a ridge estimation using L-curve method. Geomat. Inf. Sci. Wuhan Univ. 2004, 29, 235–238. [Google Scholar]
- Abdrakhmatov, K.Y.; Aldazhanov, S.A.; Hager, B.H.; Hamburger, M.W.; Herring, T.A.; Kalabaev, K.B.; Makarov, V.I.; Molnar, P.; Panasyuk, S.V.; Prilepin, M.T.; et al. Relatively recent construction of the Tien Shan inferred from GPS measurements of present-day crustal deformation rates. Nature 1996, 384, 450–453. [Google Scholar] [CrossRef]
- Dam, T.v.; Wahr, J.; Lavallée, D. A comparison of annual vertical crustal displacements from GPS and Gravity Recovery and Climate Experiment (GRACE) over Europe. J. Geophys. Res. Solid Earth 2007, 112, 404–415. [Google Scholar]
- Gülal, E.; Erdo An, H.; Tiryakio Lu, B. Research on the stability analysis of GNSS reference stations network by time series analysis. Digit. Signal. Process. 2013, 23, 1945–1957. [Google Scholar] [CrossRef]
- Ding, Y.H.; Huang, D.F.; Shi, Y.L.; Jiang, Z.S.; Chen, T. Determination of vertical surface displacements in Sichuan using GPS and GRACE measurements. Chin. J. Geophys. 2018, 061, 4777–4788. [Google Scholar]
- He, M.; Shen, W.; Pan, Y.; Chen, R.; Guo, G. Temporal–Spatial surface seasonal mass changes and vertical crustal deformation in south china block from GPS and GRACE measurements. Sensors 2017, 18, 99. [Google Scholar] [CrossRef] [Green Version]
- Fu, Y.; Argus, D.F.; Landerer, F.W. GPS as an independent measurement to estimate terrestrial water storage variations in Washington and Oregon. J. Geophys. Res. Solid Earth 2015, 120, 552–566. [Google Scholar] [CrossRef]
- Dill, R.; Dobslaw, H. Numerical simulations of global-scale high-resolution hydrological crustal deformations. J. Geophys. Res. Solid Earth 2013, 118, 5008–5017. [Google Scholar] [CrossRef]
- Chai, T.; Draxler, R.R. Root mean square error (RMSE) or mean absolute error (MAE) aguments against avoiding RMSE in the literature. Geosci. Model. Dev. 2014, 7, 1247–1250. [Google Scholar] [CrossRef] [Green Version]
- Heinzl, H.; Mittlböck, M. Pseudo R-squared measures for poisson regression models with over- or underdispersion. Comput. Stat. Data Anal. 2003, 44, 253–271. [Google Scholar] [CrossRef]
- Gupta, H.V.; Kling, H.; Yilmaz, K.K.; Martinez, G.F. Decomposition of the mean squared error and NSE performance criteria: Implications for improving hydrological modelling. J. Hydrol. 2009, 377, 80–91. [Google Scholar] [CrossRef] [Green Version]
- Zimmerman, D.; Pavlik, C.; Ruggles, A.; Armstrong, M.P. An Experimental Comparison of Ordinary and Universal Kriging and Inverse Distance Weighting. Math. Geol. 1999, 31, 375–390. [Google Scholar] [CrossRef]
- Klos, A.; Karegar, M.A.; Kusche, J.; Springer, A. Quantifying noise in daily GPS height time series: Harmonic function versus GRACE-assimilating modeling approaches. IEEE Geosci. Remote Sens. Lett. 2020, 18, 627–631. [Google Scholar] [CrossRef]



| Parameters | Value | Parameters | Value |
|---|---|---|---|
| Reference frame | ITRF 2008 | Flat difference | Weighted least-squares estimation + Kalman filtering |
| Height cut-off angle | 10° | Ionosphere | LC portfolio observations |
| A priori troposphere | 0.5 m | Earth rotation parameters | Polar shift, UT1 |
| Mapping functions | HGMF, DGMF | Inertial coordinate system | J2000.0 |
| Tidal correction | IERS 2003 Model; Polar Tide Correction; FES 2004 Sea Tide Model | Precession of the equinoxes | IAU 1976 |
| Satellite phase center | IGS ANTEX Model | Chapter movement | IAU 1980 |
| Method | Period Time | Time Resolution | Spatial Resolution |
|---|---|---|---|
| DWLIM | 2011–2020 | 1 day | 0.25° × 0.25° |
| TRAGNSS | 2011–2020 | 1 day | 0.25° × 0.25° |
| GRACE | 2011–2017 2018–2020 | 1 month | 0.5° × 0.5° |
| GLDAS | 2011–2020 | 1 day | 0.25° × 0.25° |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shen, Y.; Zheng, W.; Yin, W.; Xu, A.; Zhu, H.; Wang, Q.; Chen, Z. Improving the Inversion Accuracy of Terrestrial Water Storage Anomaly by Combining GNSS and LSTM Algorithm and Its Application in Mainland China. Remote Sens. 2022, 14, 535. https://doi.org/10.3390/rs14030535
Shen Y, Zheng W, Yin W, Xu A, Zhu H, Wang Q, Chen Z. Improving the Inversion Accuracy of Terrestrial Water Storage Anomaly by Combining GNSS and LSTM Algorithm and Its Application in Mainland China. Remote Sensing. 2022; 14(3):535. https://doi.org/10.3390/rs14030535
Chicago/Turabian StyleShen, Yifan, Wei Zheng, Wenjie Yin, Aigong Xu, Huizhong Zhu, Qingqing Wang, and Zhiwei Chen. 2022. "Improving the Inversion Accuracy of Terrestrial Water Storage Anomaly by Combining GNSS and LSTM Algorithm and Its Application in Mainland China" Remote Sensing 14, no. 3: 535. https://doi.org/10.3390/rs14030535
APA StyleShen, Y., Zheng, W., Yin, W., Xu, A., Zhu, H., Wang, Q., & Chen, Z. (2022). Improving the Inversion Accuracy of Terrestrial Water Storage Anomaly by Combining GNSS and LSTM Algorithm and Its Application in Mainland China. Remote Sensing, 14(3), 535. https://doi.org/10.3390/rs14030535






