Analysis of the Optical Turbulence Model Using Meteorological Data
Abstract
:1. Introduction
2. Experiment and Methodology
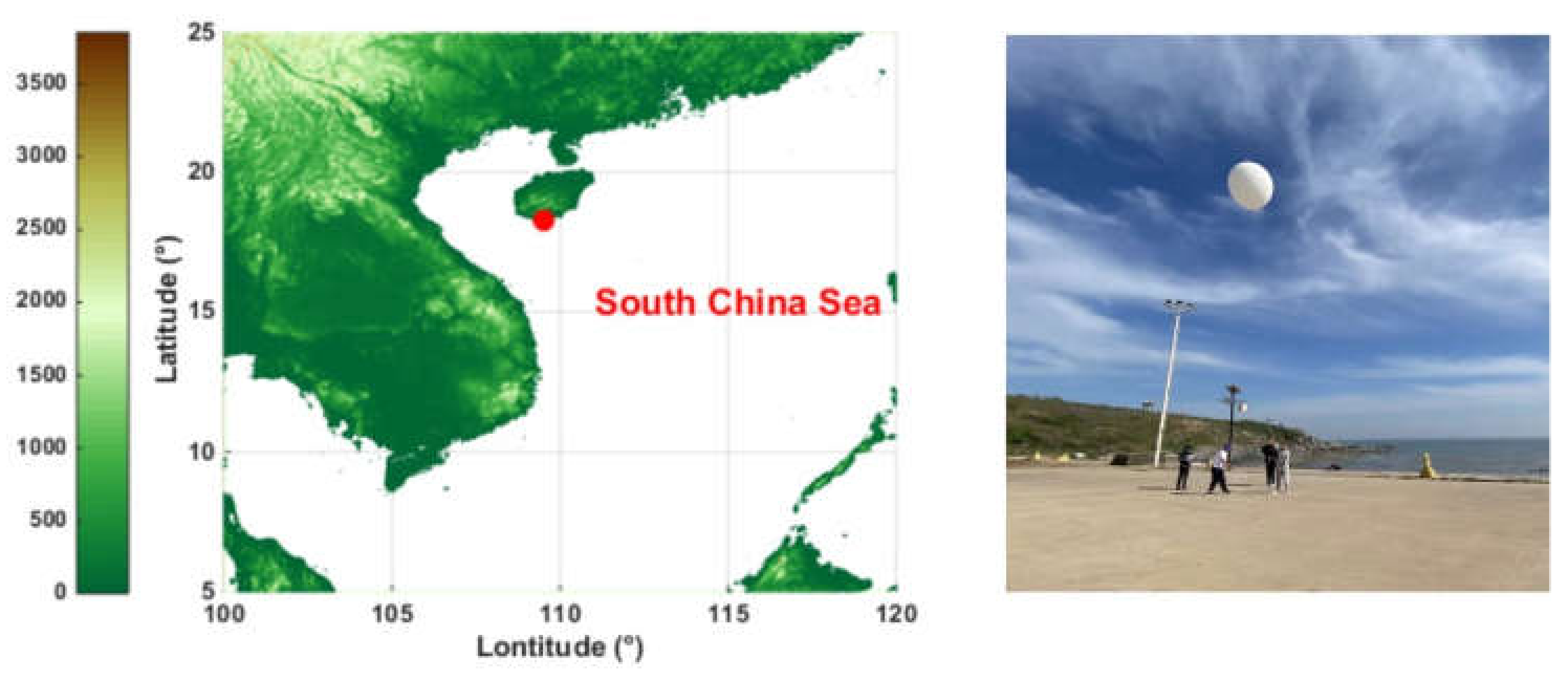
2.1. Experiment Detail
2.2. Estimation Model
2.3. Statistical Operators
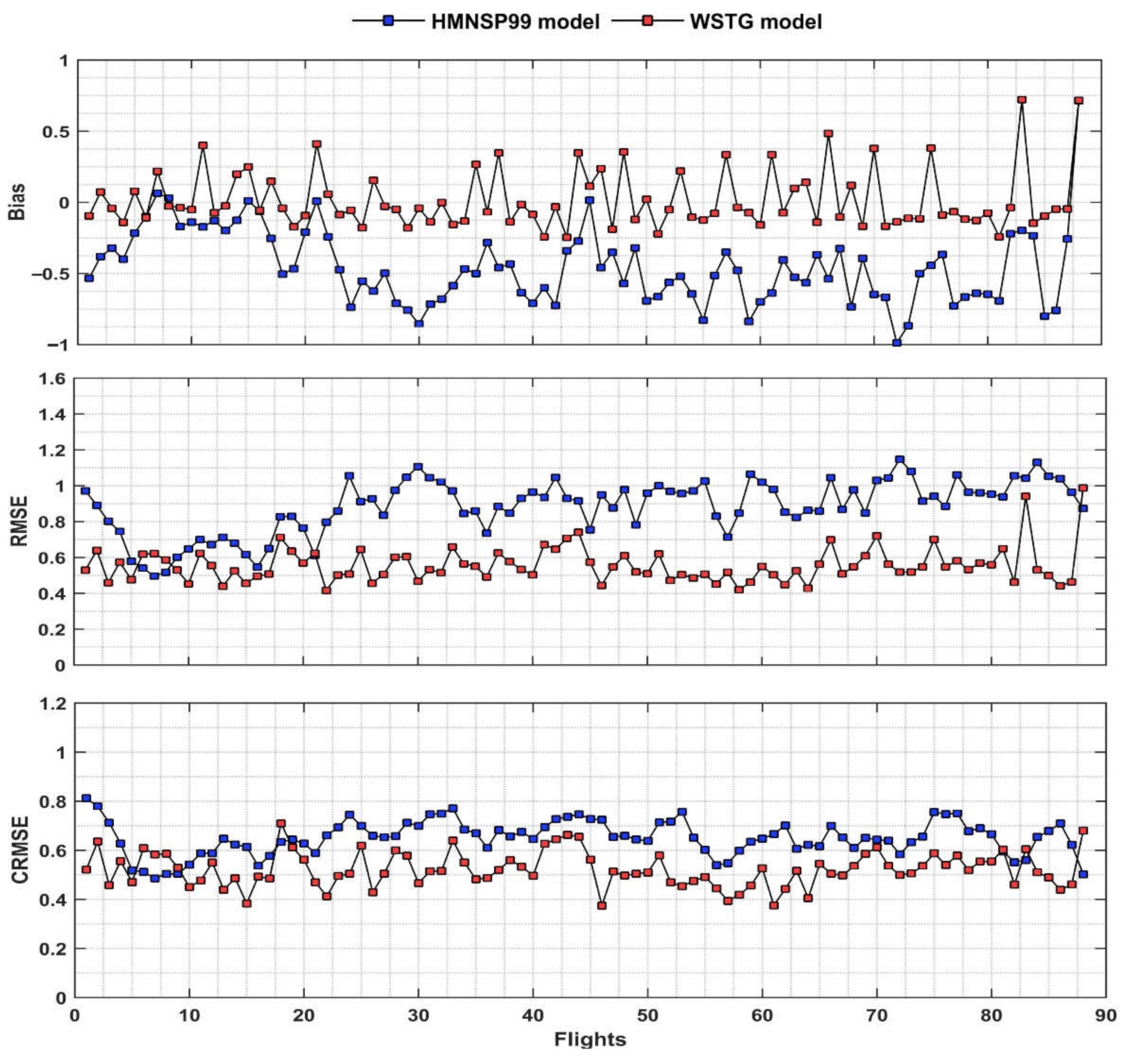
3. Results and Discussion
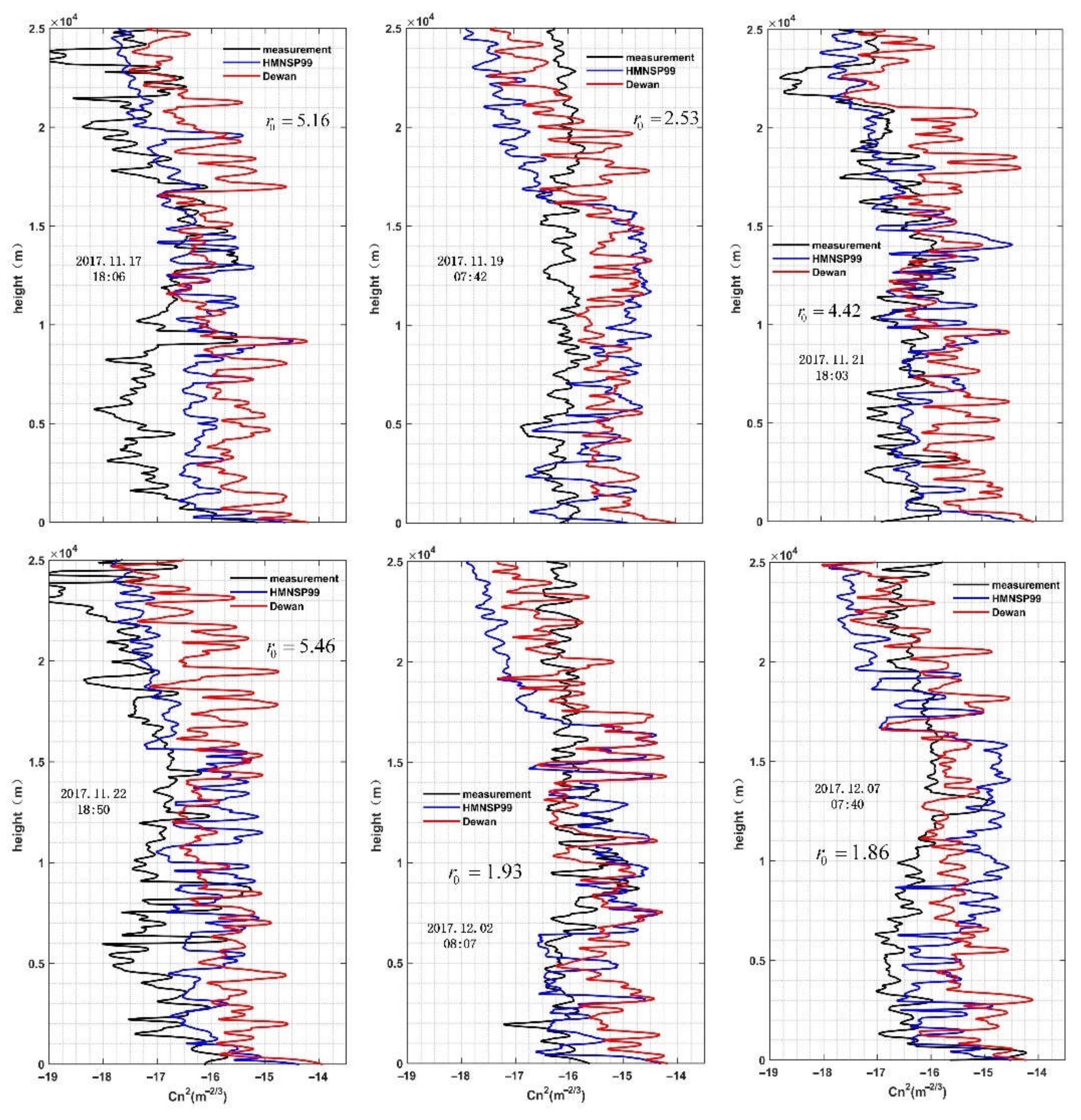
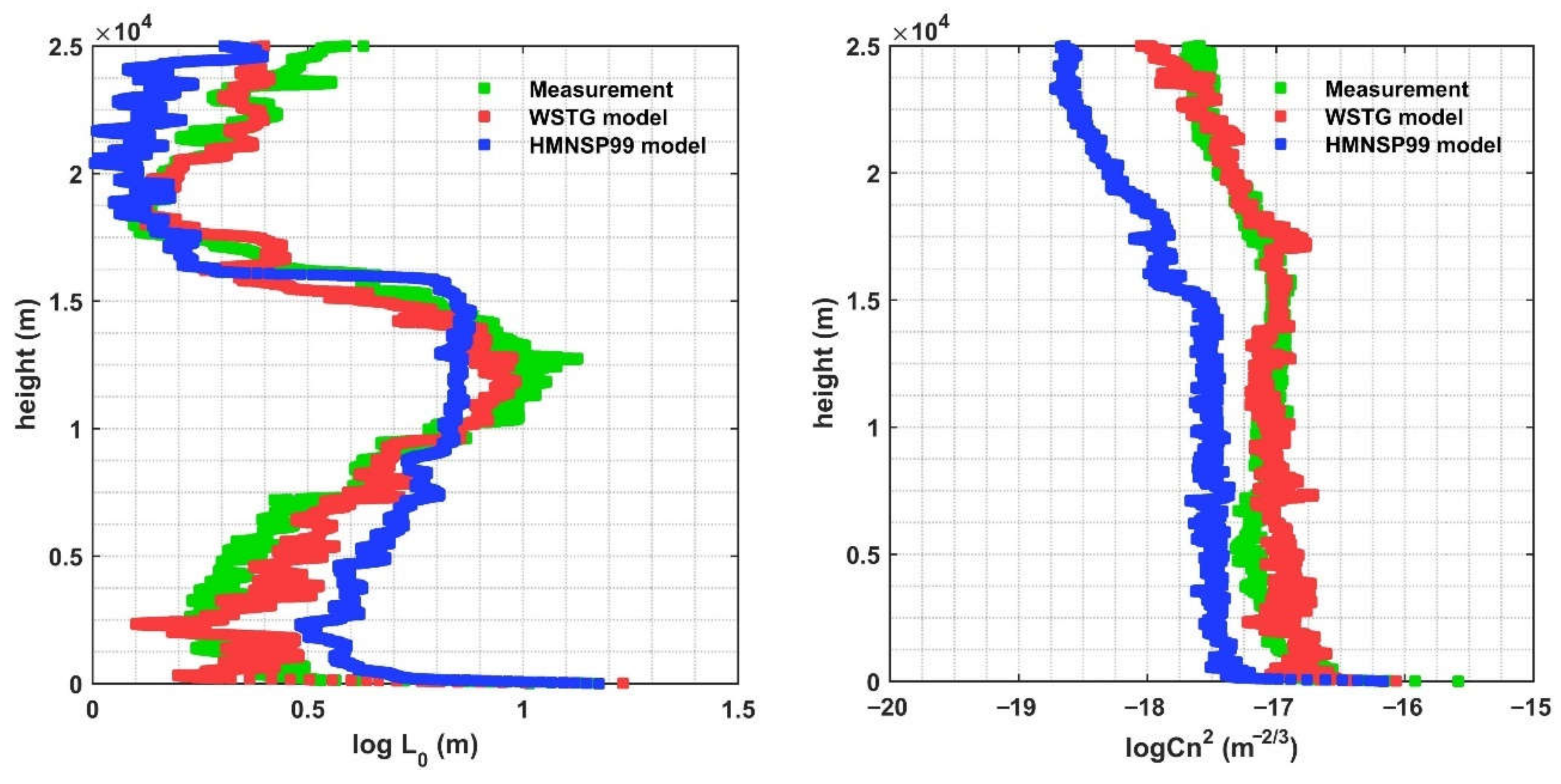
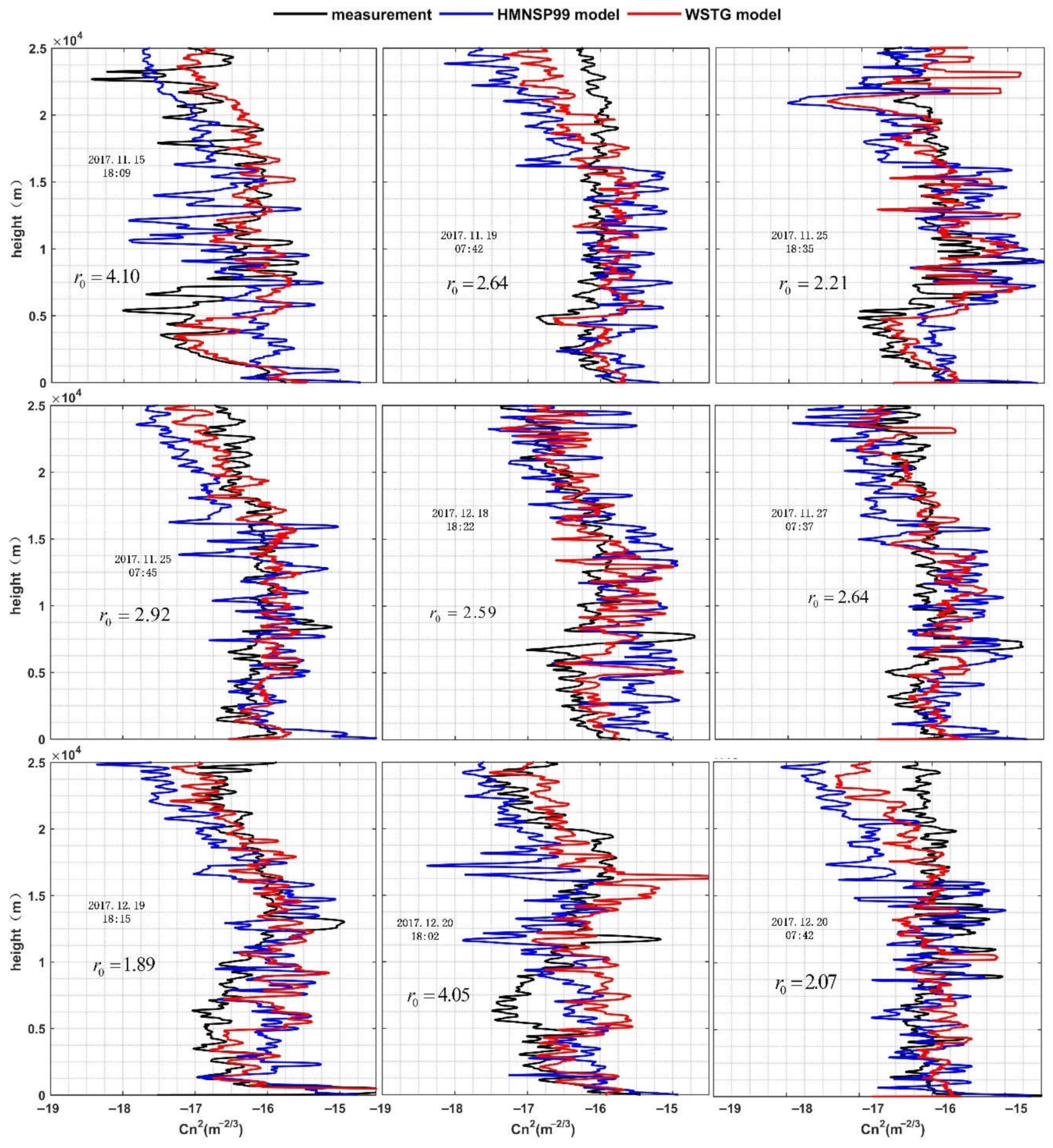
3.1. Profiles from Models and Radiosonde Measurement
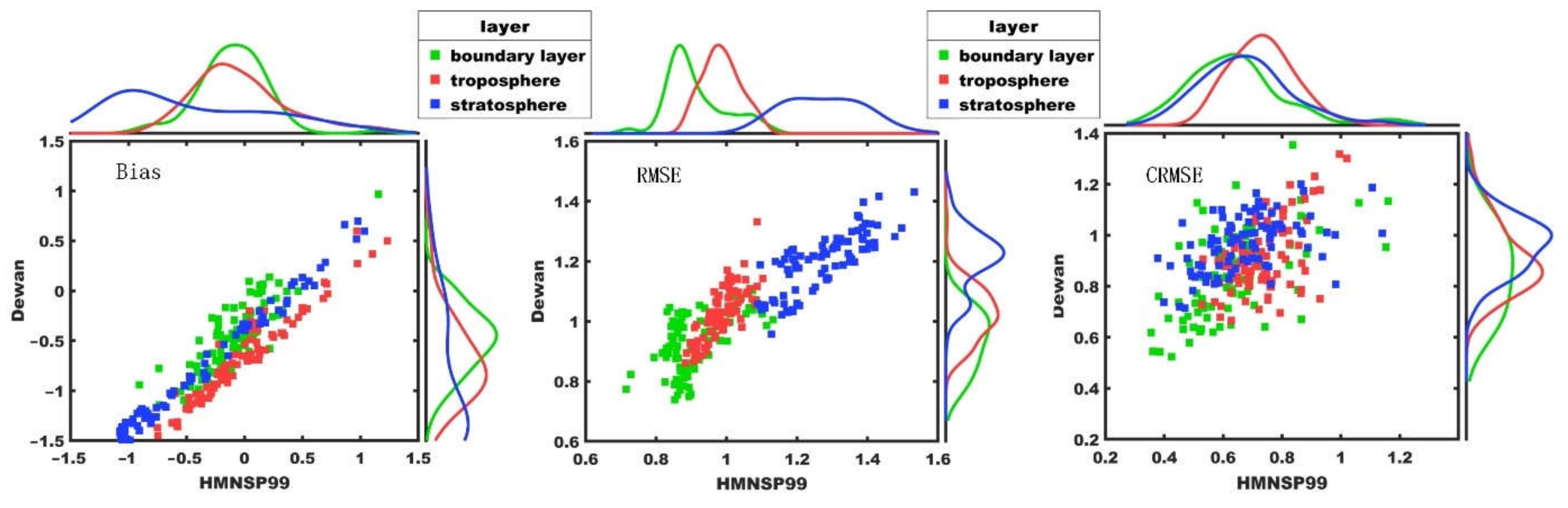
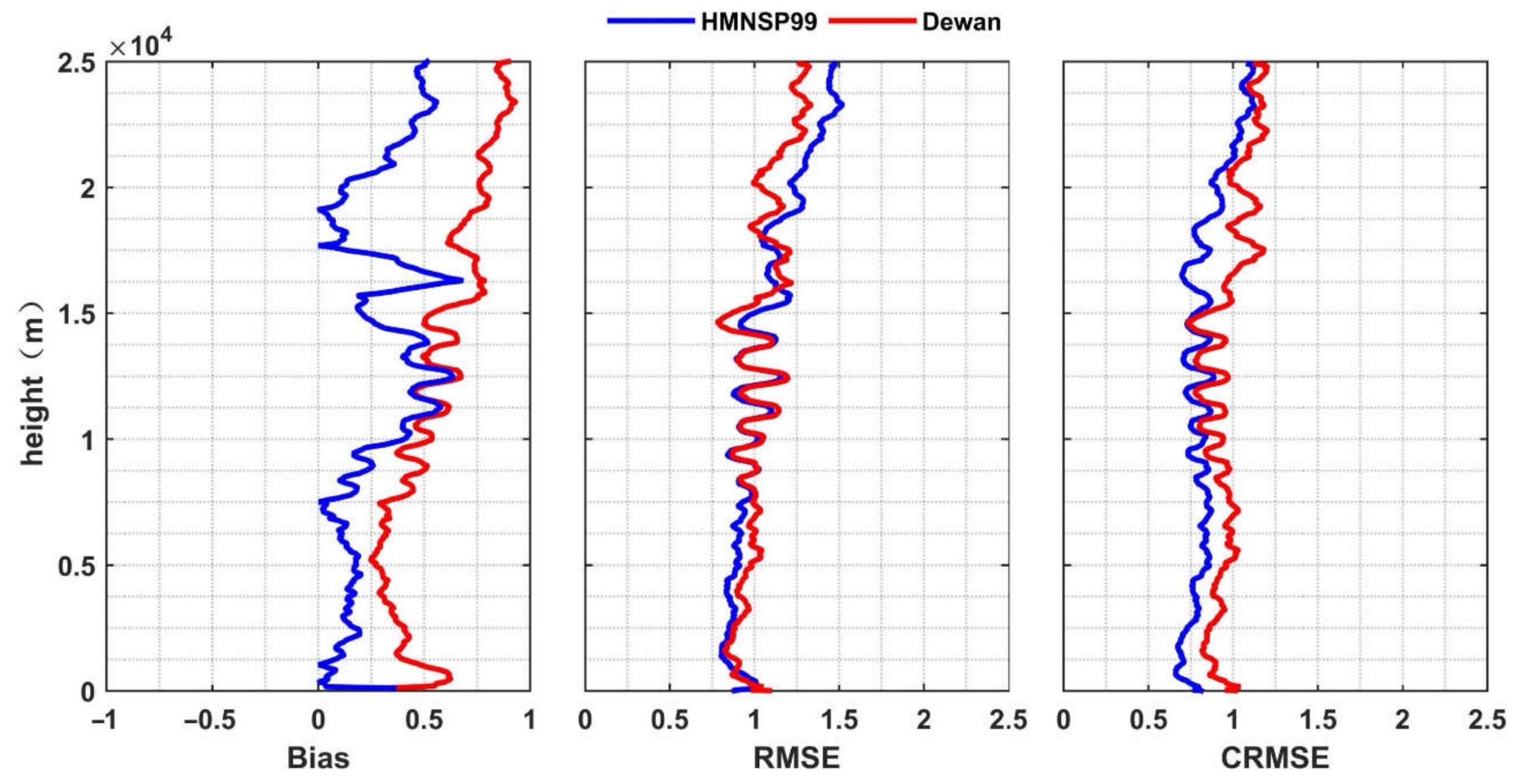
3.2. Statistical Model Performances
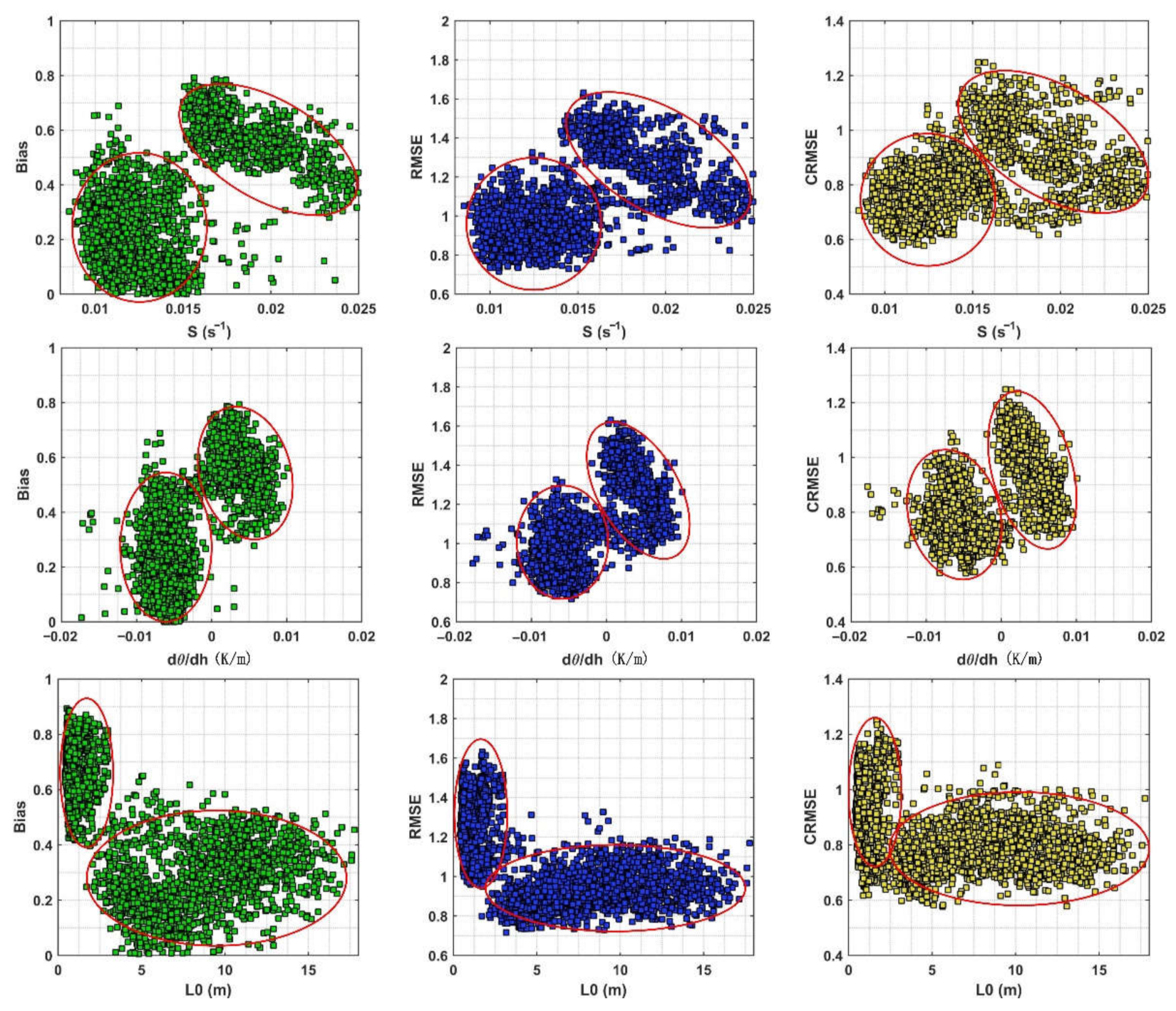
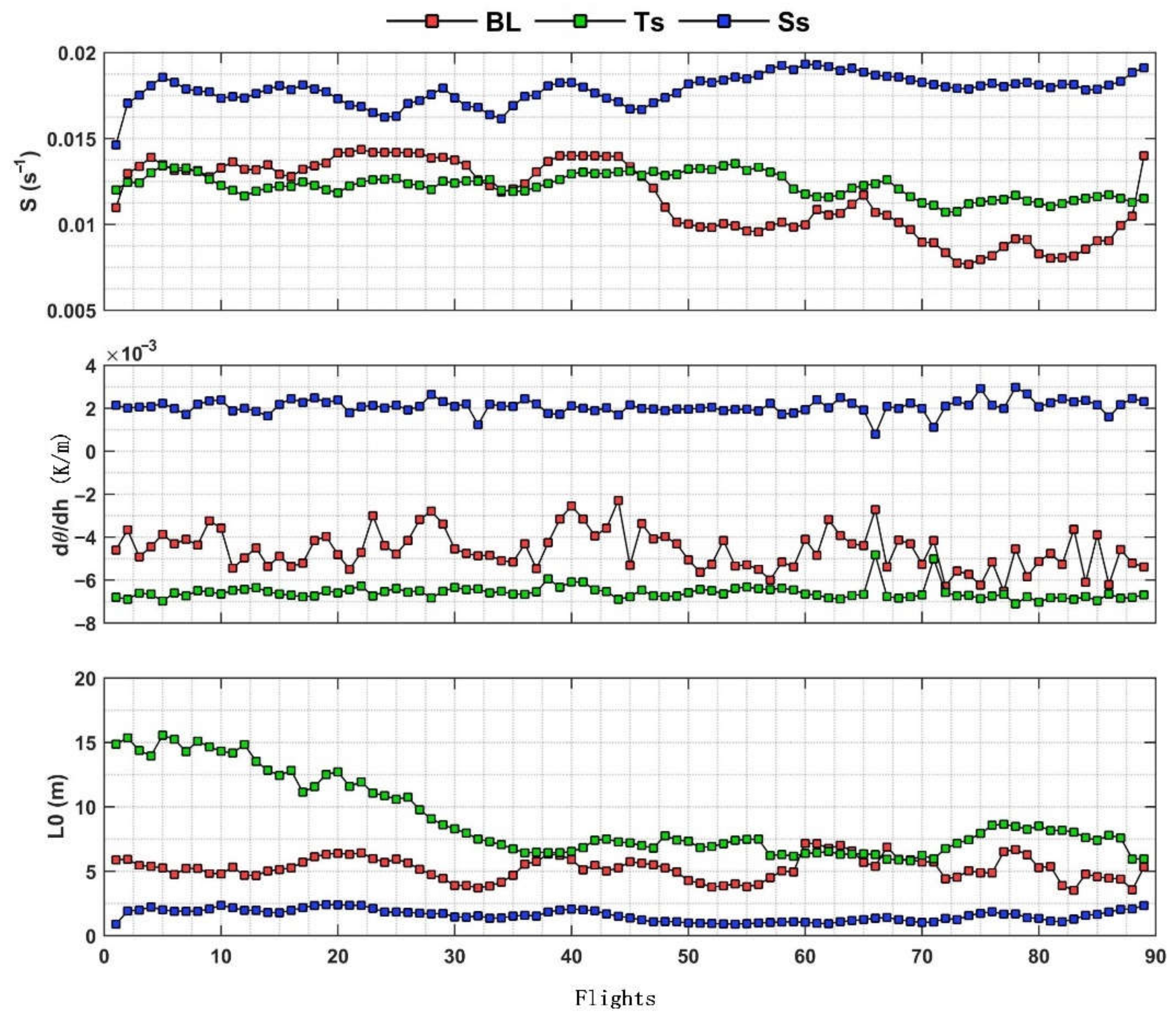
3.3. Statistical Analysis of the Effects of Turbulence Parameters
3.4. New Statistical Outer Scale Model
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ata, Y.; Baykal, Y.; Gökçe, M.C. Average channel capacity in anisotropic atmospheric non-Kolmogorov turbulent medium. Opt. Commun. 2019, 451, 129–135. [Google Scholar] [CrossRef]
- Baykal, Y.; Gerçekcioğlu, H. Multimode laser beam scintillations in strong atmospheric turbulence. Appl. Phys. B 2019, 125, 152. [Google Scholar] [CrossRef]
- Mahalov, A.; McDaniel, A. Long-range propagation through inhomogeneous turbulent atmosphere: Analysis beyond phase screens. Phys. Scr. 2019, 94, 034003. [Google Scholar] [CrossRef]
- Tatarski, V.I.; Silverman, R.A.; Chako, N. Wave Propagation in a Turbulent Medium. Phys. Today 1961, 14, 46. [Google Scholar] [CrossRef]
- Hutt, D.L. Modeling and measurement of atmospheric optical turbulence over land. Opt. Eng. 1999, 38, 1288. [Google Scholar] [CrossRef]
- Roddier, F. V The Effects of Atmospheric Turbulence in Optical Astronomy. In Progress in Optics; Wolf, E., Ed.; Elsevier: Amsterdam, The Netherlands, 1981; Volume 19, pp. 281–376. [Google Scholar]
- Azouit, M.; Vernin, J. Optical Turbulence Profiling with Balloons Relevant to Astronomy and Atmospheric Physics. Publ. Astron. Soc. Pac. 2005, 117, 536–543. [Google Scholar] [CrossRef]
- Avila, R.; Vernin, J.; Masciadri, E. Whole atmospheric-turbulence profiling with generalized scidar. Appl. Opt. 1997, 36, 7898–7905. [Google Scholar] [CrossRef]
- Kornilov, V.; Tokovinin, A.; Shatsky, N.; Voziakova, O.; Potanin, S.; Safonov, B. Combined MASS-DIMM instrument for atmospheric turbulence studies. Mon. Not. R. Astron. Soc. 2007, 382, 1268–1278. [Google Scholar] [CrossRef] [Green Version]
- McHugh, J.; Jumper, G.; Chun, M. Balloon Thermosonde Measurements over Mauna Kea and Comparison with Seeing Measurements. Publ. Astron. Soc. Pac. 2008, 120, 1318–1324. [Google Scholar] [CrossRef]
- Wang, Z.; Zhang, L.; Kong, L.; Bao, H.; Guo, Y.; Rao, X.; Zhong, L.; Zhu, L.; Rao, C. A modified S-DIMM+: Applying additional height grids for characterizing daytime seeing profiles. Mon. Not. R. Astron. Soc. 2018, 478, 1459–1467. [Google Scholar] [CrossRef]
- Scharmer, G.B.; van Werkhoven, T.I.M.J.A. S-DIMM+ height characterization of day-time seeing using solar granulation. Astron. Astrophys. 2010, 513, A25. [Google Scholar] [CrossRef]
- Miller, M.; Zieske, P. Turbulence Environment Characterization; Interim Report; Avco-Everett Research Lab: Wilmington, MA, USA, 1979; p. 135. [Google Scholar]
- Good, R.E.; Beland, R.R.; Murphy, E.A.; Brown, J.H.; Dewan, E.M. Atmospheric models of optical turbulence. Proc. SPIE 1988, 928, 165–186. [Google Scholar] [CrossRef]
- Jumper, G.; Beland, R. Progress in the understanding and modeling of atmospheric optical turbulence. In Proceedings of the 31st Plasmadynamics and Lasers Conference, Denver, CO, USA, 19–22 June 2000. [Google Scholar]
- Beland, R.R.; Brown, J.H. A deterministic temperature model for stratospheric optical turbulence. Phys. Scr. 1988, 37, 419–423. [Google Scholar] [CrossRef]
- Warnock, J.M.; Vanzandt, T.E. A statistical model to estimate refractivity turbulence structure constant Cn2 in the free atmosphere. Int. Counc. Sci. Unions Middle Atmos. Program. Handb. MAP 1986, 20, 166. [Google Scholar]
- Coulman, C.E.; Vernin, J.; Coqueugniot, Y.; Caccia, J.L. Outer Scale of Turbulence Appropriate to Modeling Refractive-Index Structure Profiles. Appl. Opt. 1988, 27, 155–160. [Google Scholar] [CrossRef]
- Dewan, E.; Good, R.; Beland, R.; Brown, J. A Model for Cn(2) (Optical Turbulence) Profiles Using Radiosonde Data; Phillips Laboratory, Directorate of Geophysics, Air Force Materiel Command: Hanscom AFB, MA, USA, 1993; p. 50. [Google Scholar]
- Trinquet, H.; Vernin, J. A Model to Forecast Seeing and Estimate C2N Profiles from Meteorological Data. Publ. Astron. Soc. Pac. 2006, 118, 756–764. [Google Scholar] [CrossRef] [Green Version]
- Basu, S. A simple approach for estimating the refractive index structure parameter (Cn2) profile in the atmosphere. Opt. Lett. 2015, 40, 4130–4133. [Google Scholar] [CrossRef]
- Ruggiero, F.H.; Debenedictis, F.H. Forecasting optical turbulence from mesoscale numerical weather prediction models. In Proceedings of the In Proceedings of the DoD High Performance Modernization Program Users Group Conference, Austin, TX, USA, 10–14 June 2002. [Google Scholar]
- Bi, C.; Qian, X.; Liu, Q.; Zhu, W.; Li, X.; Luo, T.; Wu, X.; Qing, C. Estimating and measurement of atmospheric optical turbulence accordingto balloon-borne radiosonde for three sites in China. J. Opt. Soc. Am. A 2020, 37, 1785–1794. [Google Scholar] [CrossRef]
- Qing, C.; Wu, X.; Li, X.; Luo, T.; Su, C.; Zhu, W. Mesoscale optical turbulence simulations above Tibetan Plateau: First attempt. Opt. Express 2020, 28, 4571–4586. [Google Scholar] [CrossRef]
- Han, Y.; Wu, X.; Luo, T.; Qing, C.; Yang, Q.; Jin, X.; Liu, N.; Wu, S.; Su, C. New Cn2 statistical model based on first radiosonde turbulence observation over Lhasa. J. Opt. Soc. Am. A 2020, 37, 995–1001. [Google Scholar] [CrossRef] [PubMed]
- Abahamid, A.; Jabiri, A.; Vernin, J.; Zouhair, B.; Azouit, M.; Agabi, A. Optical turbulence modeling in the boundary layer and free atmosphere using instrumented meteorological balloons. Astron. Astrophys. 2004, 416, 1193–1200. [Google Scholar] [CrossRef] [Green Version]
- Masciadri, E.; Lascaux, F.; Fini, L. MOSE: Operational forecast of the optical turbulence and atmospheric parameters at European Southern Observatory ground-based sites—I. Overview and vertical stratification of atmospheric parameters at 0–20 km. Mon. Not. R. Astron. Soc. 2013, 436, 1968–1985. [Google Scholar] [CrossRef] [Green Version]
- Kovadlo, P.G.; Shikhovtsev, A.Y.; Kopylov, E.A.; Kiselev, A.V.; Russkikh, I.V. Study of the Optical Atmospheric Distortions using Wavefront Sensor Data. Russ. Phys. J. 2021, 63, 1952–1958. [Google Scholar] [CrossRef]
- Wu, S.; Su, C.; Wu, X.; Luo, T.; Li, X. A Simple Method to Estimate the Refractive Index Structure Parameter (${C}_{n}^{2}$) in the Atmosphere. Publ. Astron. Soc. Pac. 2020, 132, 084501. [Google Scholar] [CrossRef]
- Wu, S.; Wu, X.; Su, C.; Yang, Q.; Xu, J.; Luo, T.; Huang, C.; Qing, C. Reliable model to estimate the profile of the refractive index structure parameter (Cn2) and integrated astroclimatic parameters in the atmosphere. Opt. Express 2021, 29, 12454–12470. [Google Scholar] [CrossRef]
- Wu, S.; Hu, X.; Han, Y.; Wu, X.; Su, C.; Luo, T.; Li, X. Measurement and analysis of atmospheric optical turbulence in Lhasa based on thermosonde. J. Atmos. Sol. Terr. Phys. 2020, 201, 105241. [Google Scholar] [CrossRef]
- Shao, S.; Qin, F.; Xu, M.; Liu, Q.; Han, Y.; Xu, Z. Temporal and spatial variation of refractive index structure coefficient over South China sea. Results Eng. 2020, 9, 100191. [Google Scholar] [CrossRef]
- Han, Y.; Yang, Q.; Nana, L.; Zhang, K.; Qing, C.; Li, X.; Wu, X.; Luo, T. Analysis of wind-speed profiles and optical turbulence above Gaomeigu and the Tibetan Plateau using ERA5 data. Mon. Not. R. Astron. Soc. 2021, 501, 4692–4702. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, M.; Shao, S.; Weng, N.; Liu, Q. Analysis of the Optical Turbulence Model Using Meteorological Data. Remote Sens. 2022, 14, 3085. https://doi.org/10.3390/rs14133085
Xu M, Shao S, Weng N, Liu Q. Analysis of the Optical Turbulence Model Using Meteorological Data. Remote Sensing. 2022; 14(13):3085. https://doi.org/10.3390/rs14133085
Chicago/Turabian StyleXu, Manman, Shiyong Shao, Ningquan Weng, and Qing Liu. 2022. "Analysis of the Optical Turbulence Model Using Meteorological Data" Remote Sensing 14, no. 13: 3085. https://doi.org/10.3390/rs14133085
APA StyleXu, M., Shao, S., Weng, N., & Liu, Q. (2022). Analysis of the Optical Turbulence Model Using Meteorological Data. Remote Sensing, 14(13), 3085. https://doi.org/10.3390/rs14133085





