The Integration of Two Interferometric Radars for Measuring Dynamic Displacement of Bridges
Abstract
:1. Introduction
2. Method
2.1. Purpose of the Thesis
- Analysis of existing solutions for measuring displacements in two or three directions using interferometric radars.
- Development of a method of measuring displacements in two directions using the existing set of two interferometric radars.
- Conducting comparative research using the set of two radars and the reference method. Research is planned on bridge structures with different levels of horizontal displacement in relation to vertical displacement.
- Analysis of comparative research results.
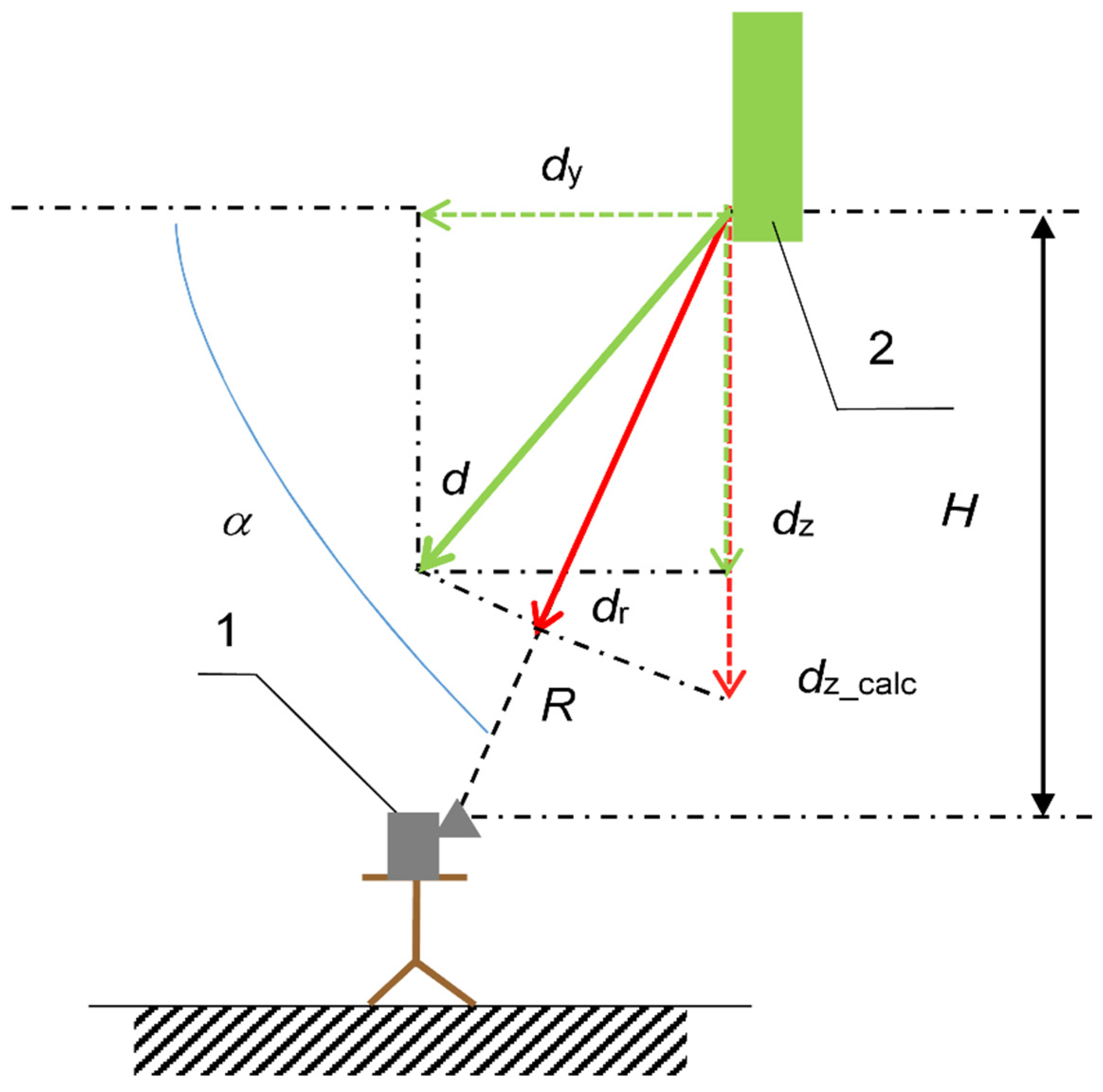
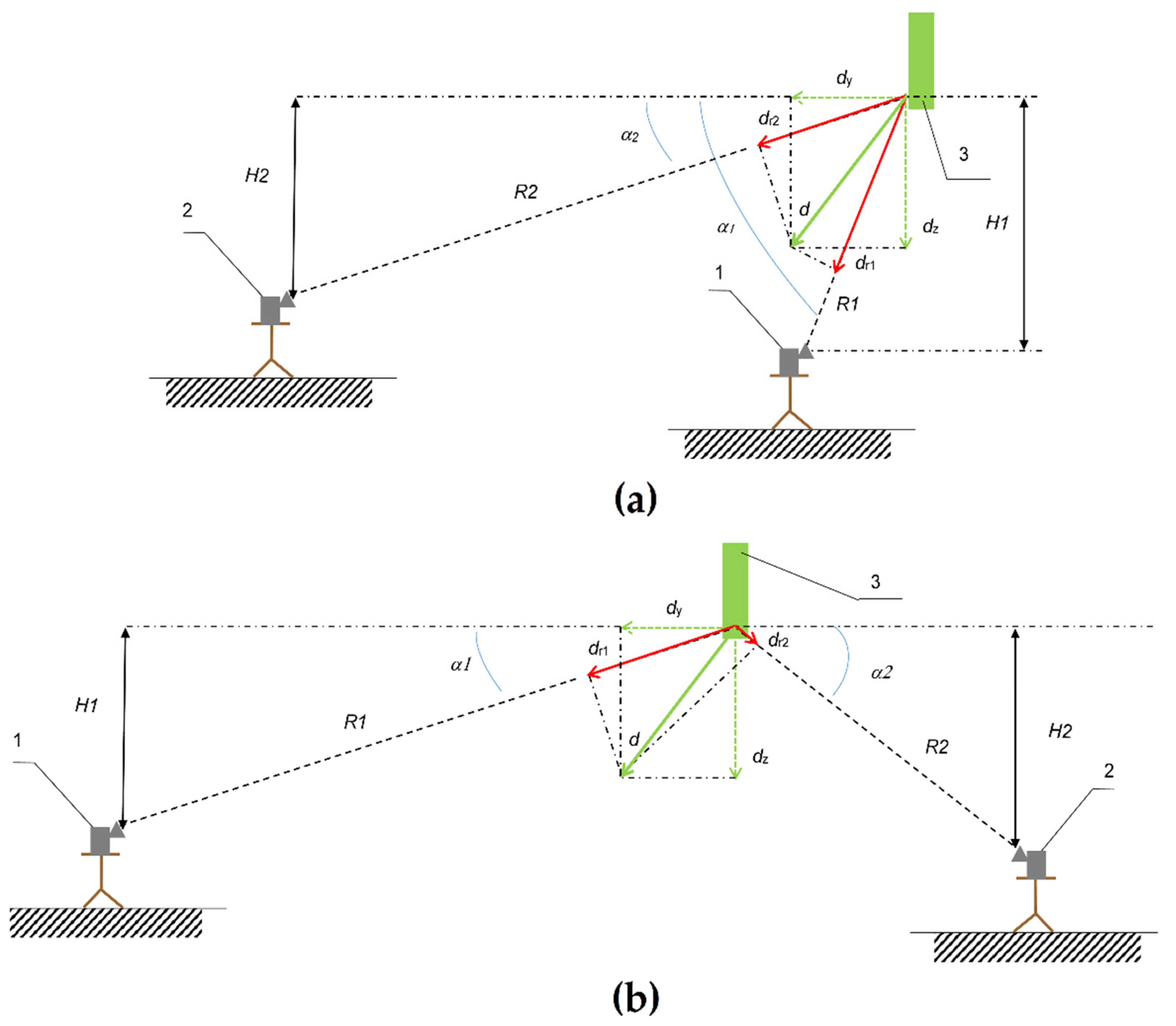
2.2. Proposed Methodology
- In the case of the location on one side of the tested element:
- In the case of the location of radars on both sides of the tested element:Vertical displacements dz and horizontal displacements dy are determined based on the solution of this system of two equations:
- In the case of the location of radars on one side of the tested element:
- In the case of the location of radars on both sides of the tested element:
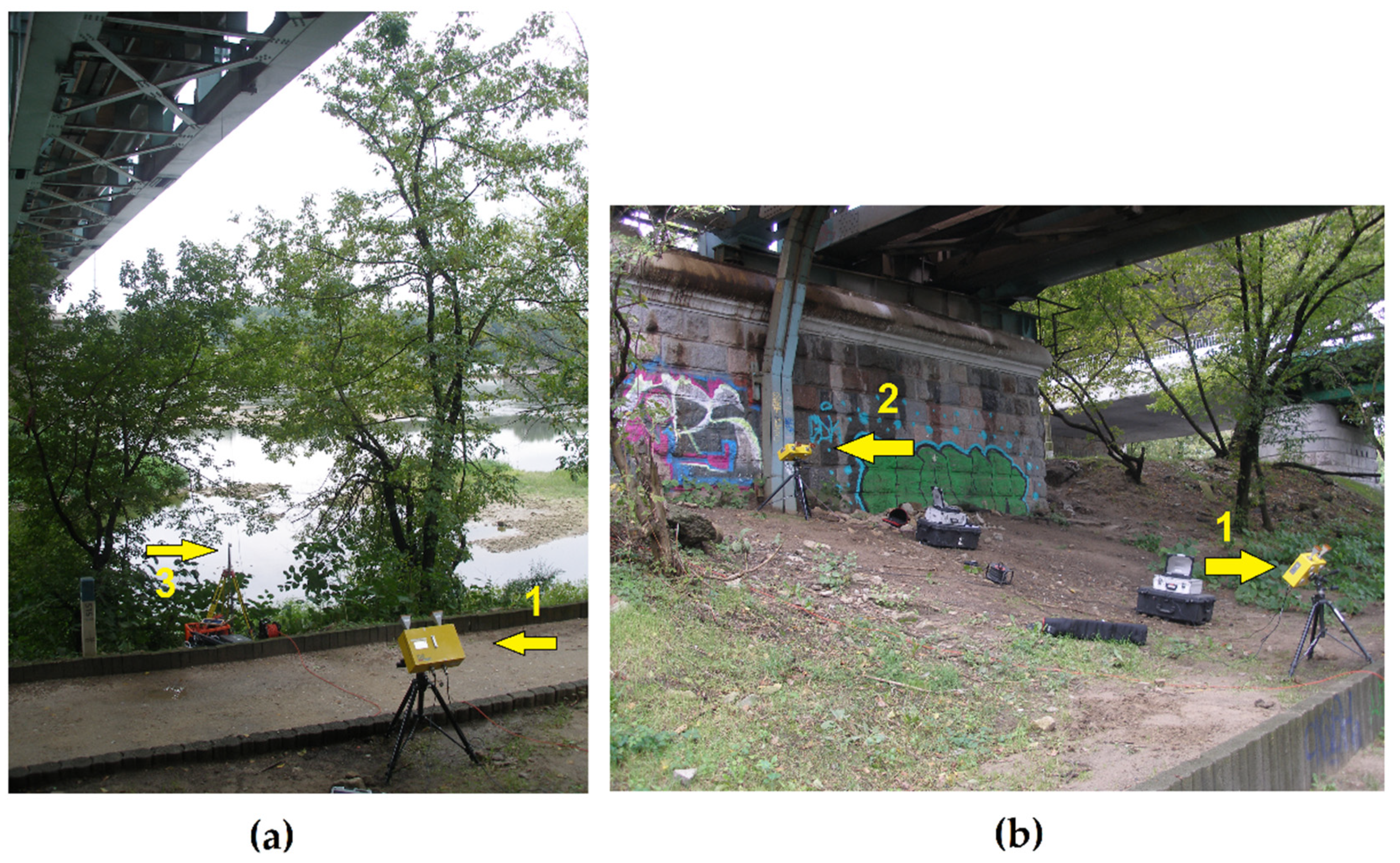
- Selecting the structure element to be observed using additional reflective elements or structure elements directly.
- R1 and R2 readout from both radars correlated with manual measurement of the radar—tested point distance using a laser rangefinder.
- Measurement of H1 and H2 using a laser rangefinder.
- Simultaneous measurement as a function of time of displacements dr1(t) by radar No. 1 and dr2(t) by radar No. 2.
- After the completion of the measurement, low pass filtration of dr1(t) and dr2(t).
- Alignment of the sampling frequency of displacements dr1(t) and dr2(t).
- Time synchronization of both displacements dr1(t) and dr2(t).
- Determination of dz(ti) and dy(ti) values for each pair of dr1(ti) and dr2(ti) readouts using Equations (5) or (6).
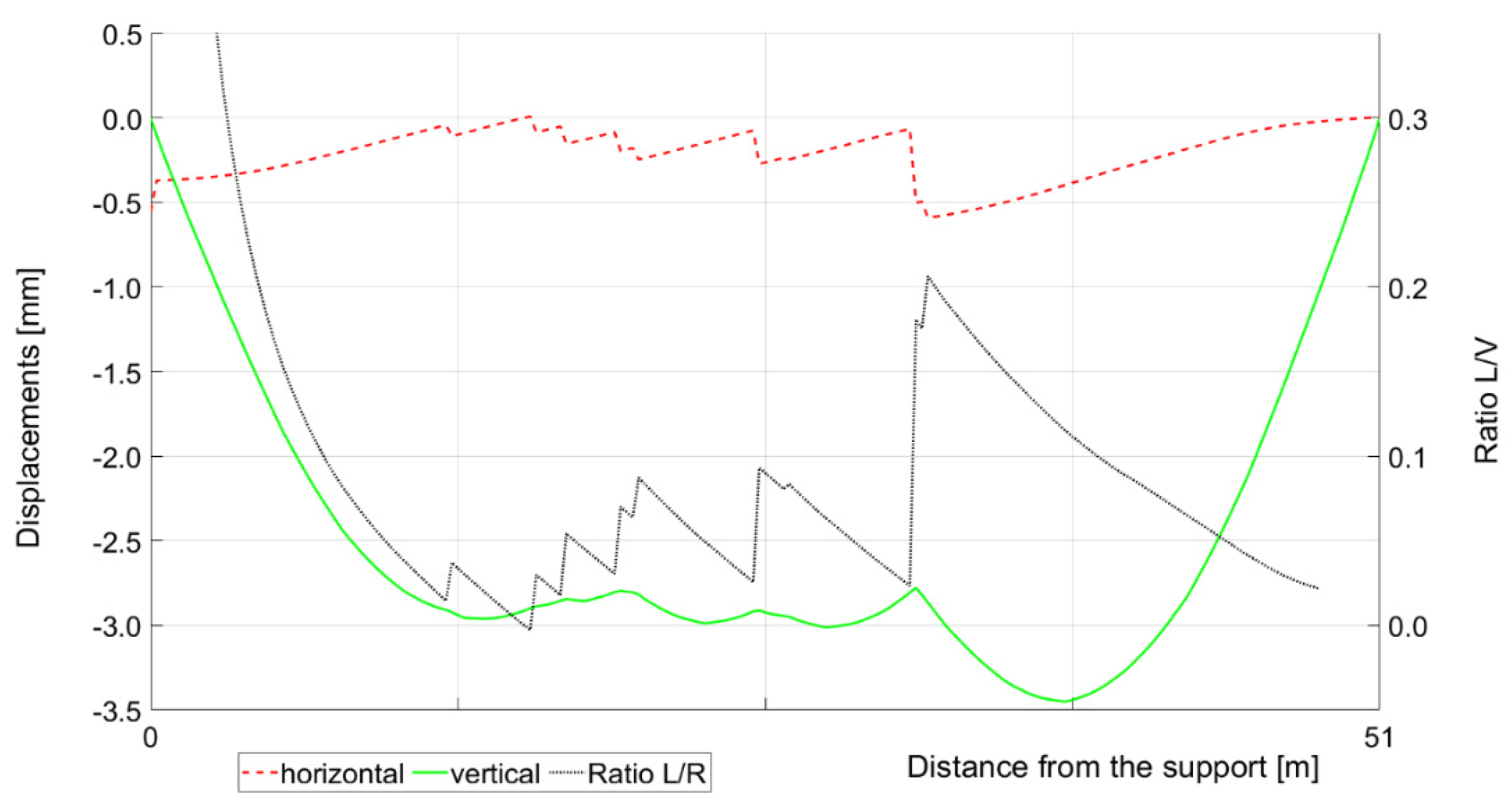
2.3. Bridges Selected for Verification Tests
2.3.1. Test Bridge No. 1
2.3.2. Test Bridge No. 2
3. Results
3.1. Tests on Bridge No. 1
3.2. Tests on Bridge No. 2
3.3. Summary of Results
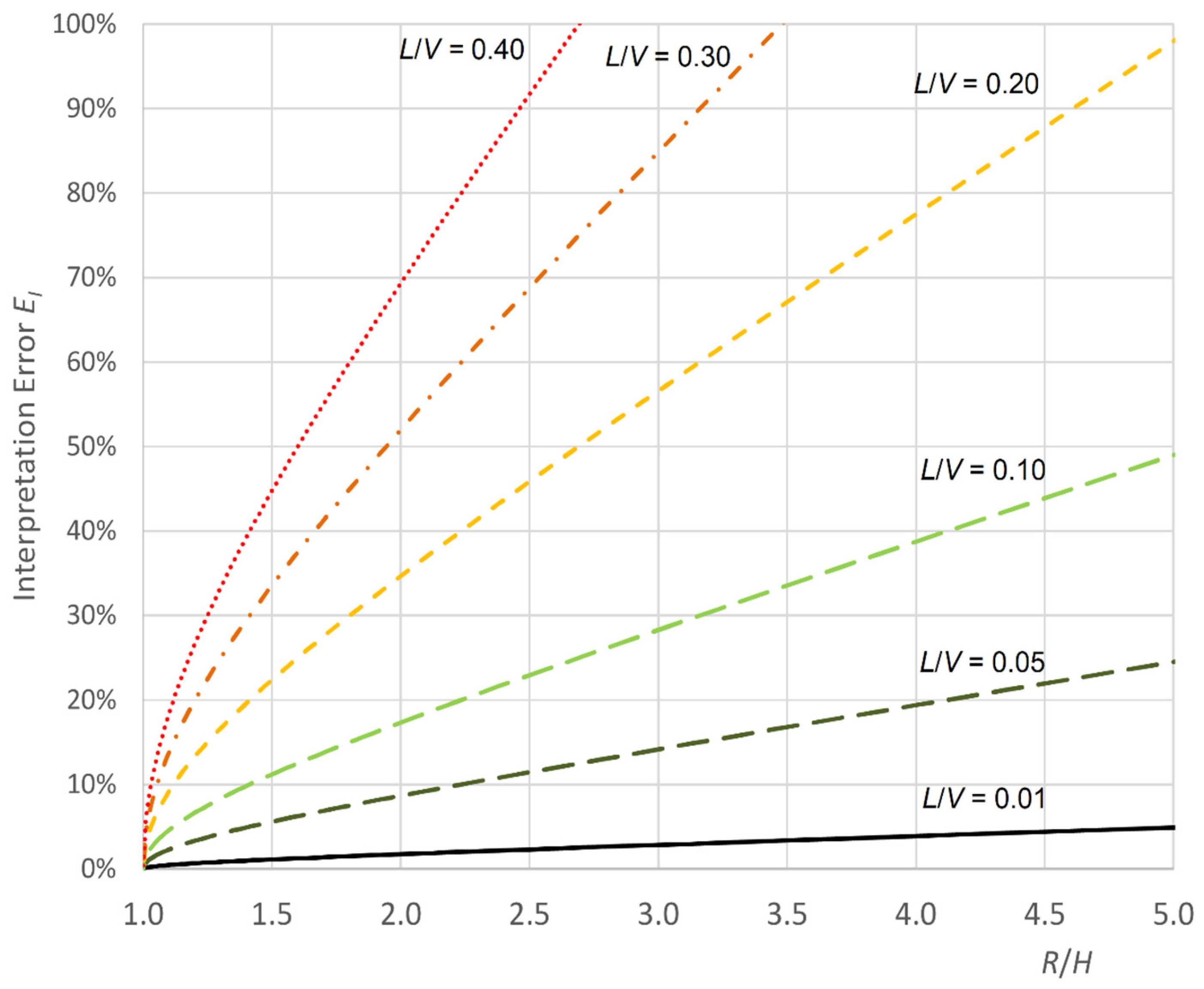
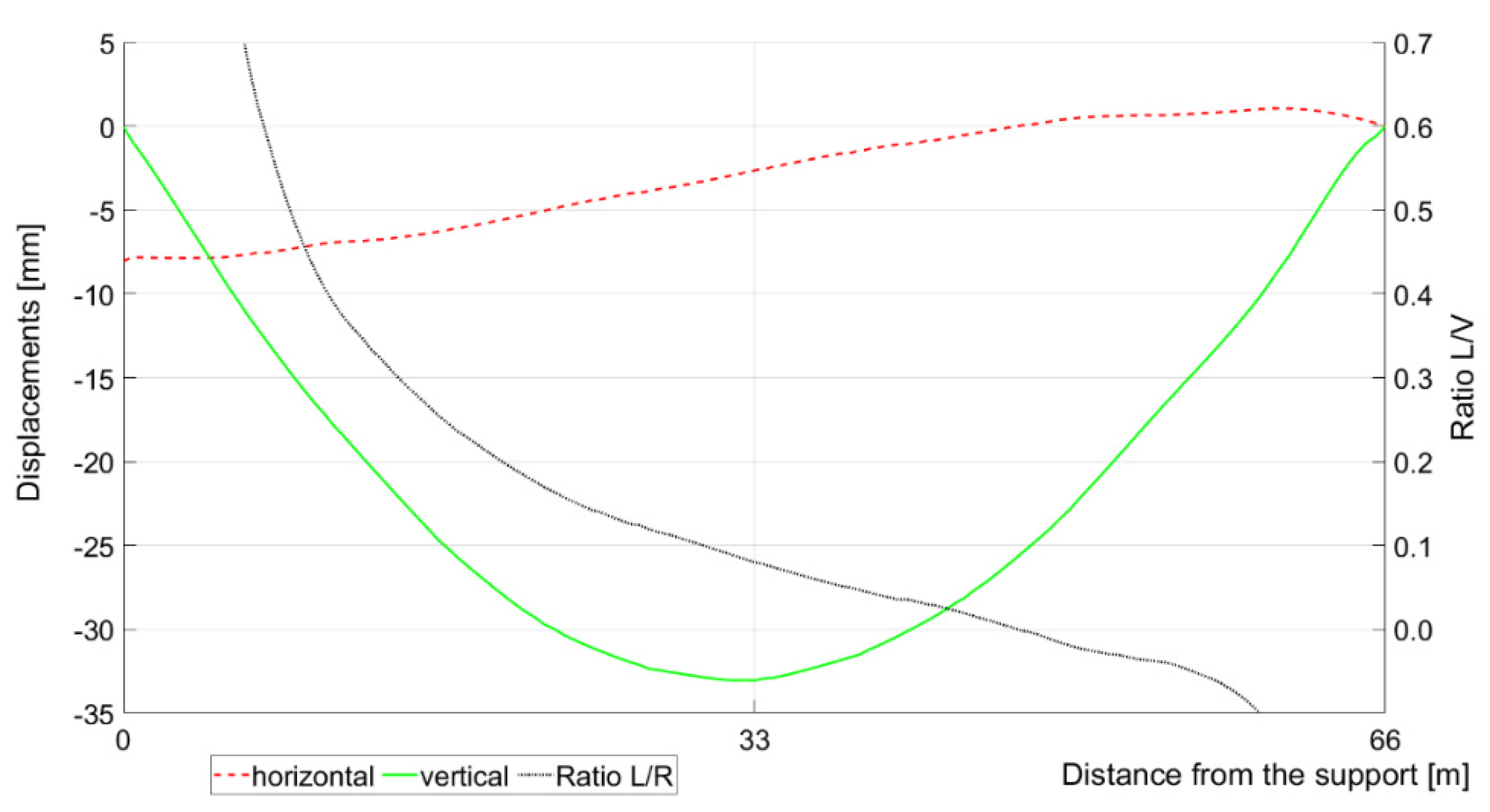
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Deng, Y.; Li, A.; Chen, S.; Feng, D. Serviceability Assessment for Long-Span Suspension Bridge Based on Deflection Measurements. Struct. Control. Health Monit. 2018, 25, e2254. [Google Scholar] [CrossRef]
- Feng, D.; Feng, M.Q. Model Updating of Railway Bridge Using In Situ Dynamic Displacement Measurement under Trainloads. J. Bridge Eng. 2015, 20, 04015019. [Google Scholar] [CrossRef]
- Olaszek, P.; Łagoda, M.; Casas, J.R. Diagnostic Load Testing and Assessment of Existing Bridges: Examples of Application. Struct. Infrastruct. Eng. 2014, 10, 834–842. [Google Scholar] [CrossRef]
- Matsuoka, K.; Collina, A.; Somaschini, C.; Sogabe, M. Influence of Local Deck Vibrations on the Evaluation of the Maximum Acceleration of a Steel-Concrete Composite Bridge for a High-Speed Railway. Eng. Struct. 2019, 200, 109736. [Google Scholar] [CrossRef]
- Bačinskas, D.; Kamaitis, Z.; Kilikevičius, A. A Sensor Instrumentation Method for Dynamic Monitoring of Railway Bridges. J. Vibroengineering 2013, 15.1, 176–184. [Google Scholar]
- Brownjohn, J.M.W.; De Stefano, A.; Xu, Y.-L.; Wenzel, H.; Aktan, A.E. Vibration-Based Monitoring of Civil Infrastructure: Challenges and Successes. J. Civ. Struct. Health Monit. 2011, 1, 79–95. [Google Scholar] [CrossRef]
- Nakamura, S.; Kawasaki, T. Lateral Vibration of Footbridges by Synchronous Walking. J. Constr. Steel Res. 2006, 62, 1148–1160. [Google Scholar] [CrossRef]
- Cremona, C. Dynamic investigations of the solferino footbridge. In Proceedings of the 3rd International Operational Modal Analysis Conference (IOMAC 2009), Portonovo, Italy, 4–6 May 2009; pp. 72–81. [Google Scholar]
- Dallard, P.; Fitzpatrick, A.J.; Flint, A.; Le Bourva, S.; Low, A.; Ridsdill Smith, R.M.; Willford, M. The London Millennium Footbridge. Struct. Eng. 2001, 79, 17–21. [Google Scholar]
- Olaszek, P. Investigation of the Dynamic Characteristic of Bridge Structures Using a Computer Vision Method. Measurement 1999, 25, 227–236. [Google Scholar] [CrossRef]
- Cigada, A.; Mazzoleni, P.; Zappa, E. Vibration Monitoring of Multiple Bridge Points by Means of a Unique Vision-Based Measuring System. Exp. Mech. 2014, 54, 255–271. [Google Scholar]
- Busca, G.; Cigada, A.; Mazzoleni, P.; Tarabini, M.; Zappa, E. Static and Dynamic Monitoring of Bridges by Means of Vision-Based Measuring System. In Topics in Dynamics of Bridges; Cunha, A., Ed.; Conference Proceedings of the Society for Experimental Mechanics Series; Springer: New York, NY, USA, 2013; Volume 3, pp. 83–92. ISBN 978-1-4614-6518-8. [Google Scholar]
- Lee, J.J.; Shinozuka, M. A Vision-Based System for Remote Sensing of Bridge Displacement. NDT E Int. 2006, 39, 425–431. [Google Scholar] [CrossRef]
- Malesa, M.; Szczepanek, D.; Kujawińska, M.; Świercz, A.; Kołakowski, P. Monitoring of Civil Engineering Structures Using Digital Image Correlation Technique. In Proceedings of the EPJ Web of Conferences, Poitiers, France, 4–9 July 2010; Volume 6, p. 31014. [Google Scholar] [CrossRef] [Green Version]
- Hou, X.; Yang, X.; Huang, Q. Using Inclinometers to Measure Bridge Deflection. J. Bridge Eng. 2005, 10, 564–569. [Google Scholar] [CrossRef]
- Hem, X.; Yang, X.; Zhao, L. Application of Inclinometer in Arch Bridge Dynamic Deflection Measurement. Telkomnika Indones. J. Electr. Eng. 2014, 12, 3331–3337. [Google Scholar]
- Hem, X.; Yang, X.; Zhao, L. New Method for High-Speed Railway Bridge Dynamic Deflection Measurement. J. Bridge Eng. 2014, 19, 05014004. [Google Scholar] [CrossRef]
- Olaszek, P.; Wyczałek, I.; Sala, D.; Kokot, M.; Świercz, A. Monitoring of the Static and Dynamic Displacements of Railway Bridges with the Use of Inertial Sensors. Sensors 2020, 20, 2767. [Google Scholar] [CrossRef]
- Gentile, C.; Bernardini, G. An Interferometric Radar for Non-Contact Measurement of Deflections on Civil Engineering Structures: Laboratory and Full-Scale Tests. Struct. Infrastruct. Eng. 2010, 6, 521–534. [Google Scholar] [CrossRef]
- Gentile, C.; Bernardini, G. Radar-Based Measurement of Deflections on Bridges and Large Structures. Eur. J. Environ. Civ. Eng. 2010, 14, 495–516. [Google Scholar] [CrossRef]
- Gentile, C. Deflection Measurement on Vibrating Stay Cables by Non-Contact Microwave Interferometer. NDT E Int. 2010, 43, 231–240. [Google Scholar] [CrossRef]
- Pieraccini, M.; Miccinesi, L. Ground-Based Radar Interferometry: A Bibliographic Review. Remote Sens. 2019, 11, 1029. [Google Scholar] [CrossRef] [Green Version]
- Pieraccini, M.; Parrini, F.; Fratini, M.; Atzeni, C.; Spinelli, P.; Micheloni, M. Static and Dynamic Testing of Bridges through Microwave Interferometry. NDT E Int. 2007, 40, 208–214. [Google Scholar] [CrossRef]
- Bęben, D. Application of the Interferometric Radar for Dynamic Tests of Corrugated Steel Plate (CSP) Culvert. NDT E Int. 2011, 44, 405–412. [Google Scholar] [CrossRef]
- Mayer, L.; Yanev, B.S.; Olson, L.D.; Smyth, A.W. Monitoring of Manhattan Bridge for Vertical and Torsional Performance with GPS and Interferometric Radar Systems. In Proceedings of the Transportation Research Board 89th Annual Meeting, Washington, DC, USA, 10–14 January 2010. [Google Scholar]
- Pieraccini, M.; Fratini, M.; Parrini, F.; Atzeni, C.; Bartoli, G. Interferometric Radar vs. Accelerometer for Dynamic Monitoring of Large Structures: An Experimental Comparison. NDT E Int. 2008, 41, 258–264. [Google Scholar] [CrossRef]
- Pieraccini, M.; Miccinesi, L.; Abdorazzagh Nejad, A.; Naderi Nejad Fard, A. Experimental Dynamic Impact Factor Assessment of Railway Bridges through a Radar Interferometer. Remote Sens. 2019, 11, 2207. [Google Scholar] [CrossRef] [Green Version]
- Xiang, J.; Zeng, Q.; Lou, P. Transverse Vibration of Train-Bridge and Train-Track Time Varying System and the Theory of Random Energy Analysis for Train Derailment. Veh. Syst. Dyn. 2004, 41, 129–155. [Google Scholar] [CrossRef]
- Jin, Z.; Pei, S.; Li, X.; Qiang, S. Vehicle-Induced Lateral Vibration of Railway Bridges: An Analytical-Solution Approach. J. Bridge Eng. 2016, 21, 04015038. [Google Scholar] [CrossRef]
- Fujino, Y.; Pacheco, B.M.; Nakamura, S.-I.; Warnitchai, P. Synchronization of Human Walking Observed during Lateral Vibration of a Congested Pedestrian Bridge. Earthq. Eng. Struct. Dyn. 1993, 22, 741–758. [Google Scholar] [CrossRef]
- Dallard, P.; Fitzpatrick, T.; Flint, A.; Low, A.; Smith, R.R.; Willford, M.; Roche, M. London Millennium Bridge: Pedestrian-Induced Lateral Vibration. J. Bridge Eng. 2001, 6, 412–417. [Google Scholar] [CrossRef]
- Miccinesi, L.; Beni, A.; Pieraccini, M. Multi-Monostatic Interferometric Radar for Bridge Monitoring. Electronics 2021, 10, 247. [Google Scholar] [CrossRef]
- Dei, D.; Mecatti, D.; Pieraccini, M. Static Testing of a Bridge Using an Interferometric Radar: The Case Study of “Ponte Degli Alpini,” Belluno, Italy. Sci. World J. 2013, 2013, e504958. [Google Scholar] [CrossRef]
- Gocał, J.; Ortyl, Ł.; Owerko, T.; Kuras, P.; Kocierz, R.; Ćwiąkała, P.; Puniach, E.; Sukta, O.; Bałut, A. Determination of Displacements and Vibrations of Engineering Structures Using Ground-Based Radar Interferometry; Wydawnictwa AGH: Kraków, Poland, 2013; ISBN 978-83-7464-645-1. [Google Scholar]
- Miccinesi, L.; Pieraccini, M. Bridge Monitoring by a Monostatic/Bistatic Interferometric Radar Able to Retrieve the Dynamic 3D Displacement Vector. IEEE Access 2020, 8, 210339–210346. [Google Scholar] [CrossRef]
- Monti-Guarnieri, A.; Falcone, P.; D’Aria, D.; Giunta, G. 3D Vibration Estimation from Ground-Based Radar. Remote Sens. 2018, 10, 1670. [Google Scholar] [CrossRef] [Green Version]
- Michel, C.; Keller, S. Advancing Ground-Based Radar Processing for Bridge Infrastructure Monitoring. Sensors 2021, 21, 2172. [Google Scholar] [CrossRef] [PubMed]
- Olaszek, P. The Application of Interferometric Radar for Measuring Lateral Vibration of Bridges; CRC Press: Boca Raton, FL, USA, 2021; pp. 2359–2366. ISBN 978-0-429-27911-9. [Google Scholar]



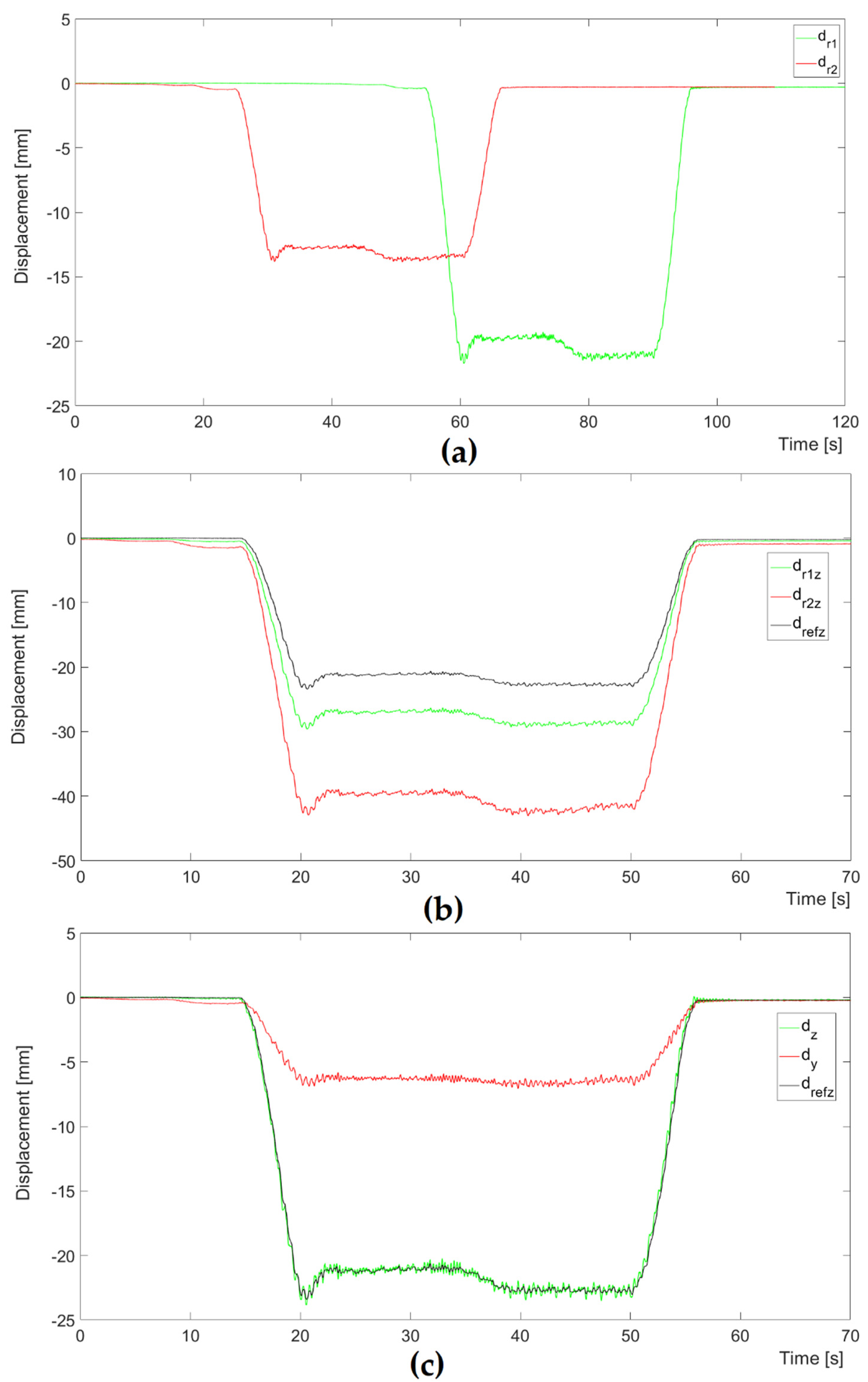


| Trains | Extremal Measurement Results (mm) | ||
| dr1 z | dr2 z | dz | |
| (1) Separate locomotive and cargo or passenger wagons | −29.65 | −43.07 | −23.87 |
| (2) Multiple-unit passenger train | −13.08 | −19.01 | −10.45 |
| (3) Separate locomotive and passenger wagons | −17.4 | −13.2 | −16.7 |
| (4) Multiple-unit passenger train | −11.60 | −9.64 | −12.89 |
| Trains | Extremal Deviation to Reference Measurements (mm) | ||
| | dr1z, r2z, z) − dref z | | |||
| (1) Separate locomotive and cargo or passenger wagons | 6.25 | 19.67 | 0.47 |
| (2) Multiple-unit passenger train | 2.67 | 8.60 | 0.14 |
| (3) Separate locomotive and passenger wagons | 0.94 | 3.30 | 0.20 |
| (4) Multiple-unit passenger train | 1.05 | 3.02 | 0.26 |
| Trains | Extremal Relative Deviation to Reference Measurements | ||
| | dr1z, r2z, z) − dref z |/|dref z | | |||
| (1) Separate locomotive and cargo or passenger wagons | 27% | 91% | 2% |
| (2) Multiple-unit passenger train | 26% | 83% | 0% |
| (3) Separate locomotive and passenger wagons | 6% | 20% | 1% |
| (4) Multiple-unit passenger train | 9% | 24% | 3% |
| Trains | Extremal Measurement Results (mm) | ||
| dr1 z | dr2 z | dz | |
| (1) Separate locomotive and passenger wagons | −6.39 | −6.40 | −6.40 |
| (2) Multiple-unit passenger train | −2.81 | −2.82 | −2.82 |
| Trains | Extremal Deviation to Reference Measurements (mm) | ||
| | dr1z, r2z, z) − dref z | | |||
| (1) Separate locomotive and passenger wagons | 0.19 | 0.15 | 0.14 |
| (2) Multiple-unit passenger train | 0.10 | 0.18 | 0.14 |
| Trains | Extremal Relative Deviation to Reference Measurements | ||
| |dr1z, r2z, z) − dref z |/|dref z | | |||
| (1) Separate locomotive and passenger wagons | 3% | 3% | 2% |
| (2) Multiple-unit passenger train | 4% | 7% | 5% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Olaszek, P.; Świercz, A.; Boscagli, F. The Integration of Two Interferometric Radars for Measuring Dynamic Displacement of Bridges. Remote Sens. 2021, 13, 3668. https://doi.org/10.3390/rs13183668
Olaszek P, Świercz A, Boscagli F. The Integration of Two Interferometric Radars for Measuring Dynamic Displacement of Bridges. Remote Sensing. 2021; 13(18):3668. https://doi.org/10.3390/rs13183668
Chicago/Turabian StyleOlaszek, Piotr, Andrzej Świercz, and Francesco Boscagli. 2021. "The Integration of Two Interferometric Radars for Measuring Dynamic Displacement of Bridges" Remote Sensing 13, no. 18: 3668. https://doi.org/10.3390/rs13183668
APA StyleOlaszek, P., Świercz, A., & Boscagli, F. (2021). The Integration of Two Interferometric Radars for Measuring Dynamic Displacement of Bridges. Remote Sensing, 13(18), 3668. https://doi.org/10.3390/rs13183668







