Entropy Generation Optimization in Couple Stress Fluid Flow with Variable Viscosity and Aligned Magnetic Field
Abstract
1. Introduction
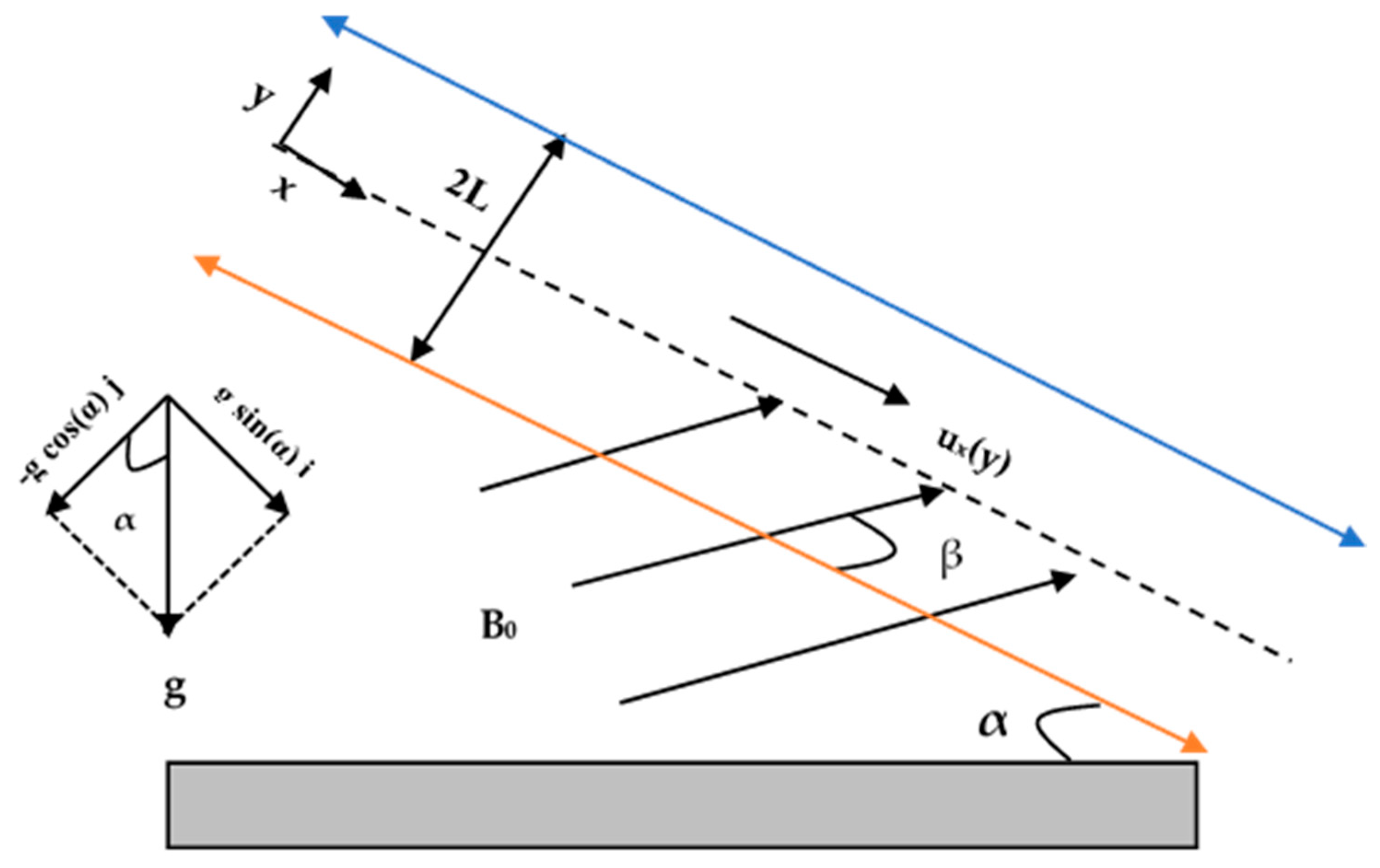
2. Outline of the Problem
3. Method of Solution
3.1. Derived Equations
3.1.1. Zeroth Order Equations
3.1.2. First Order Equations
3.1.3. Rate of Entropy Generation Analysis
3.2. Deductions
3.2.1. In the Absence of Couple Stress Fluid
3.2.2. In the absence of Magnetic field (M = 0)
3.2.3. In the Absence of Couple Stress Fluid and Magnetic Field
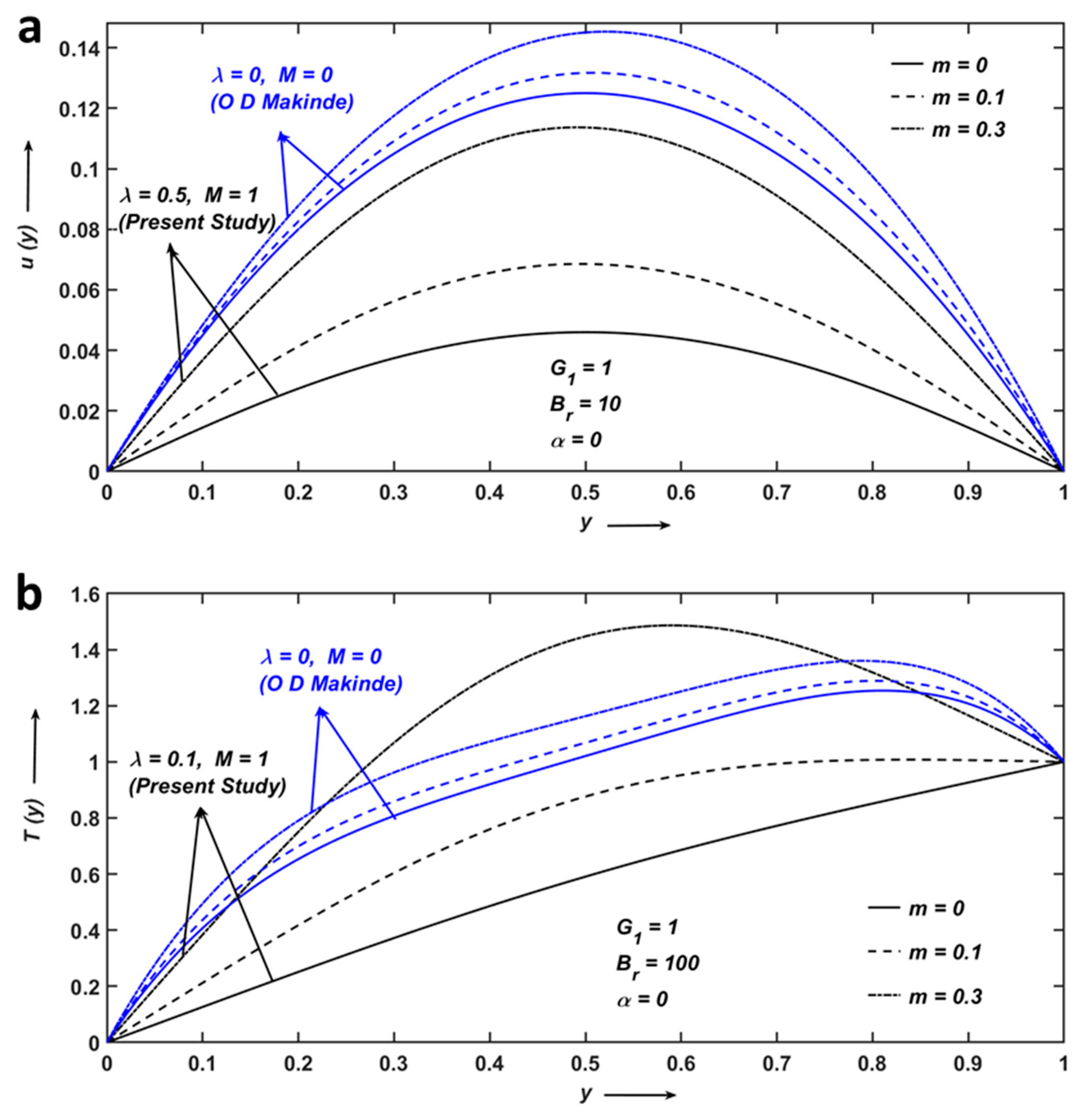
3.3. Validation
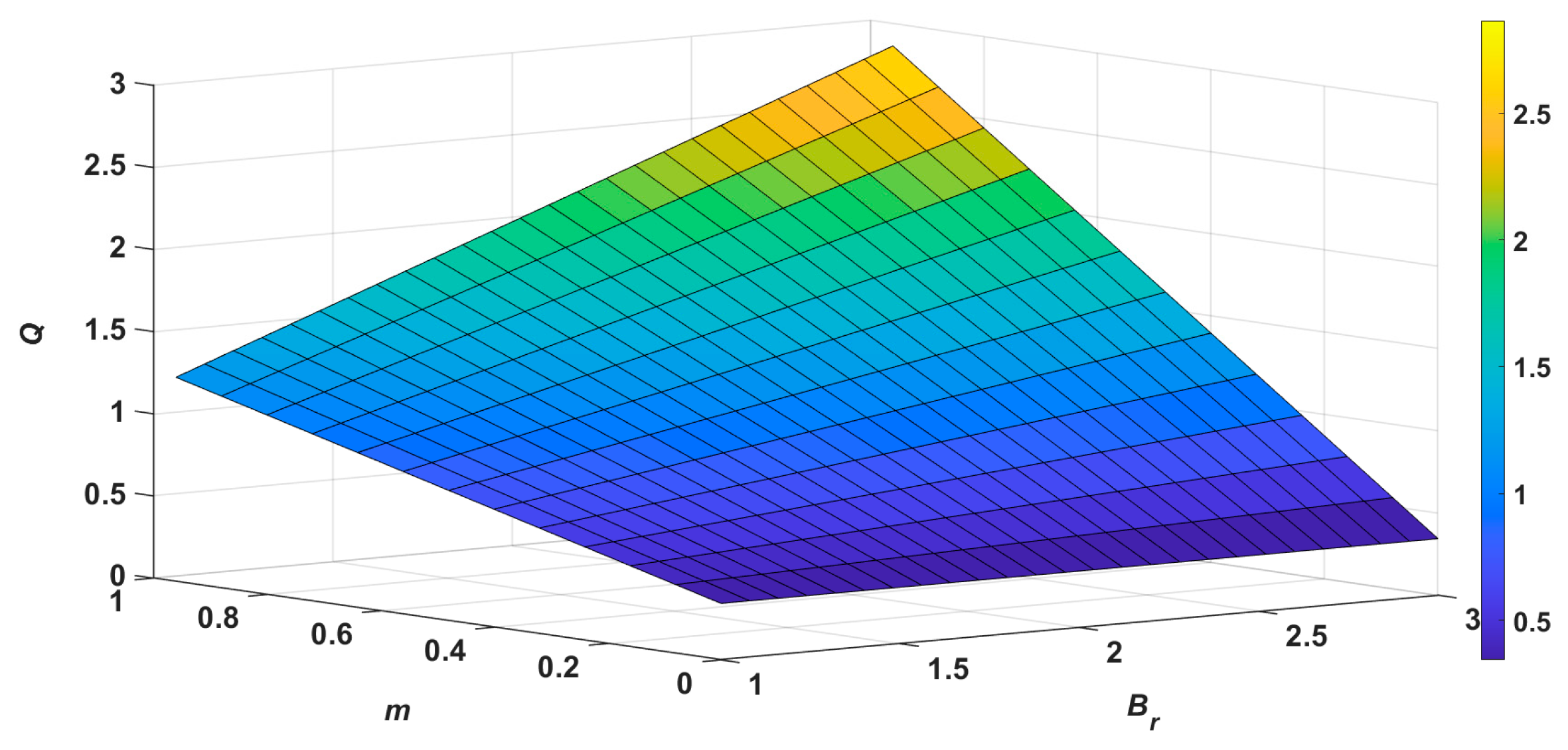
4. Results and Discussion
5. Conclusions
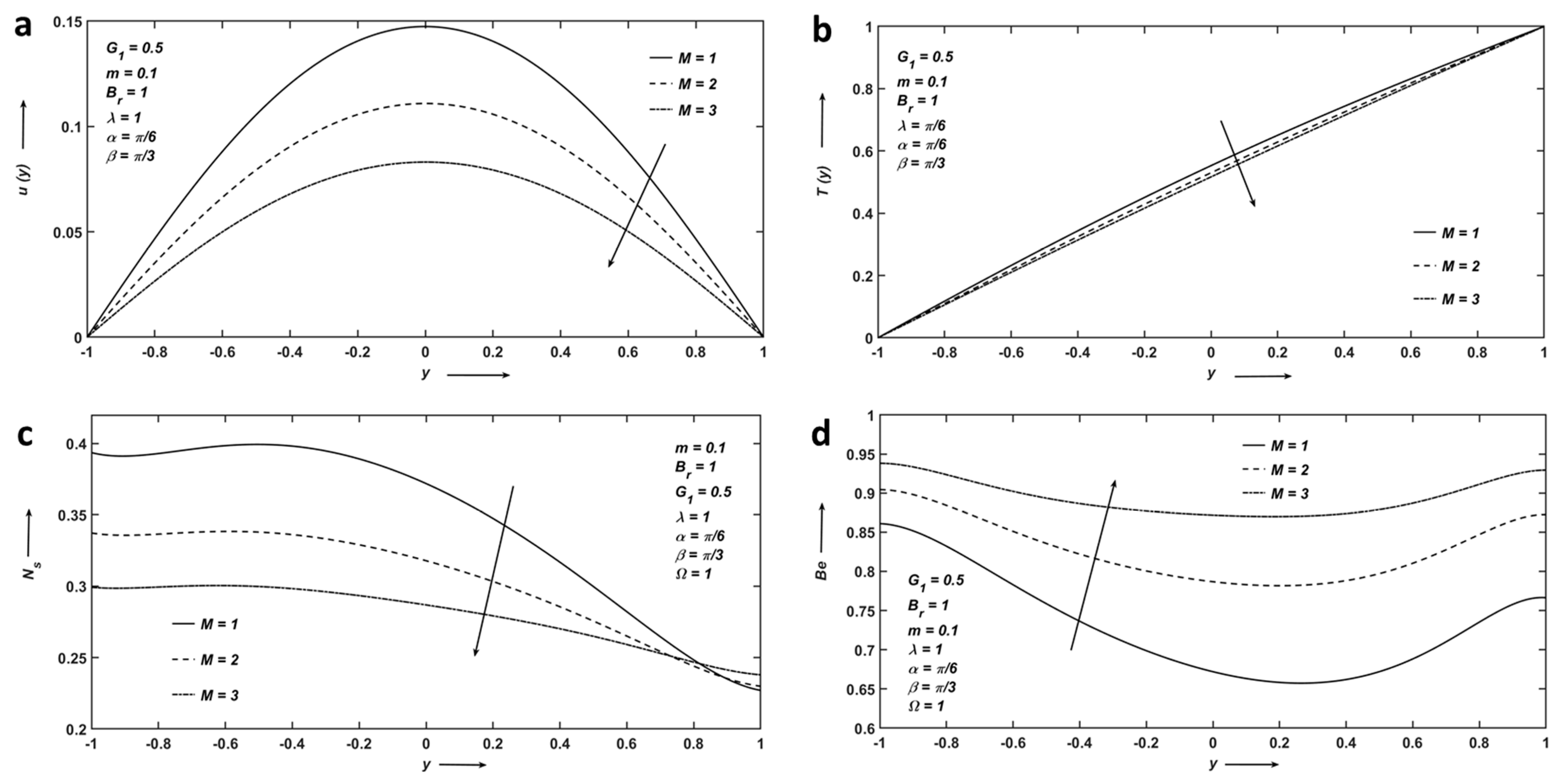
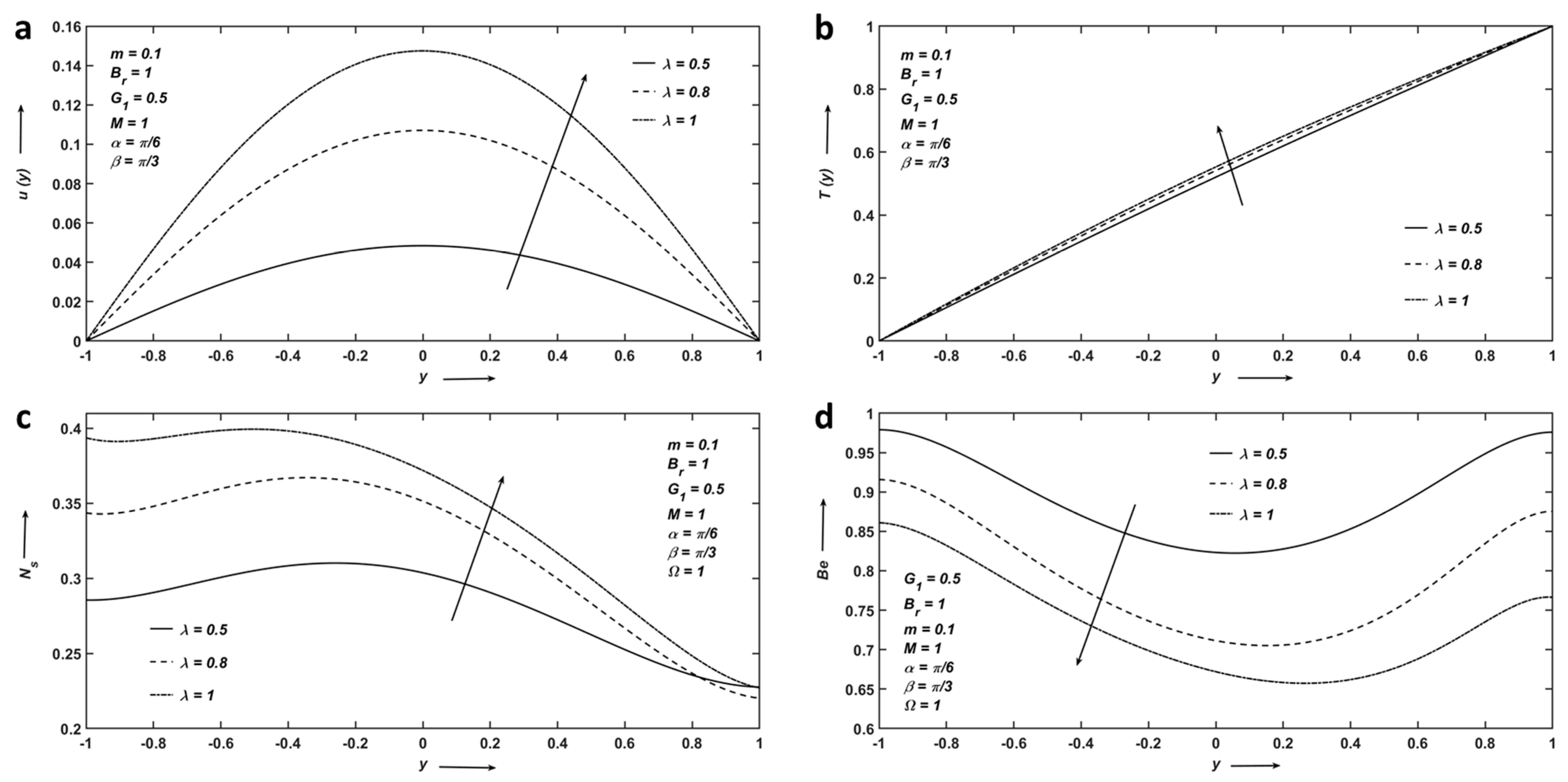
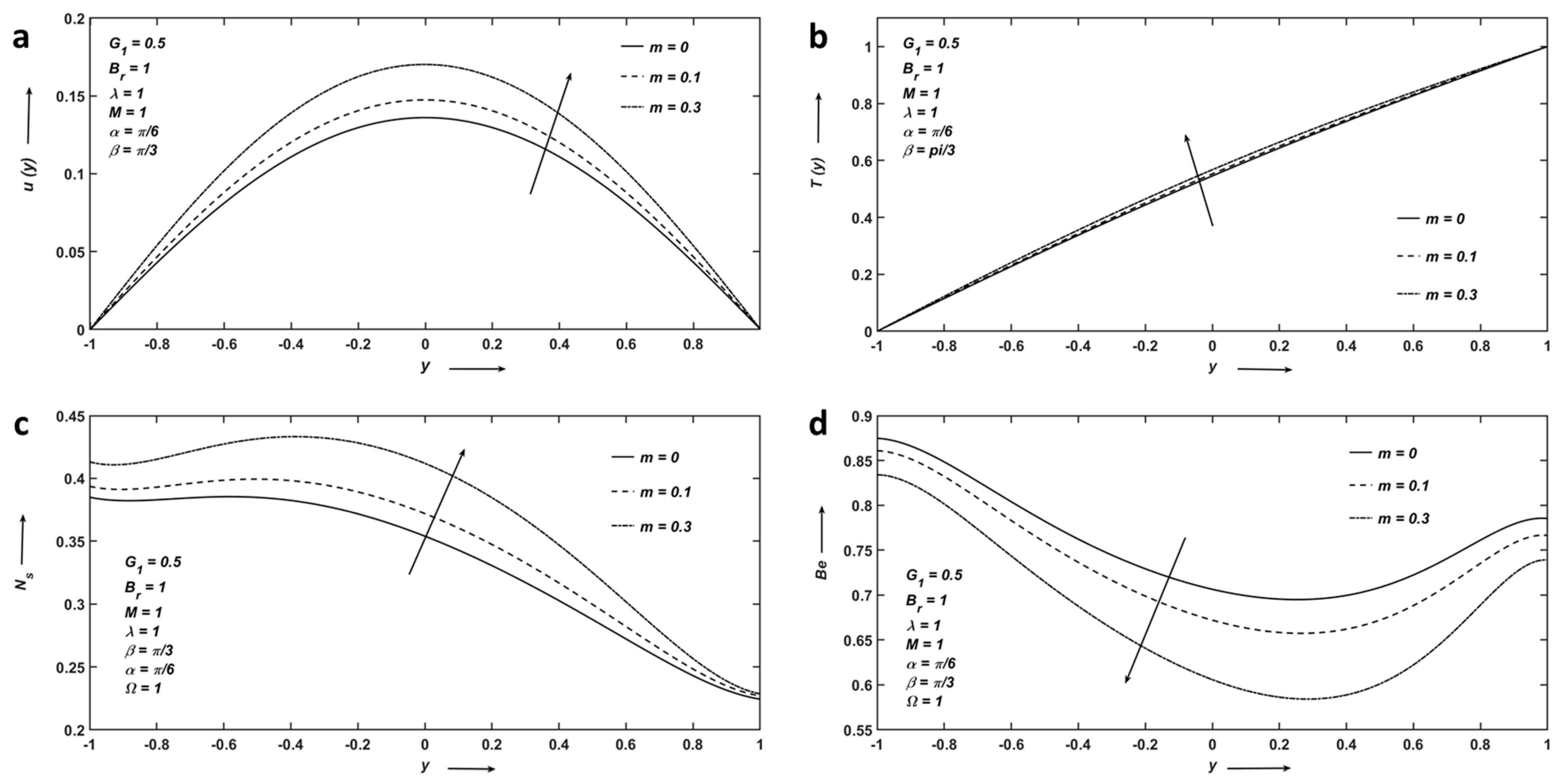
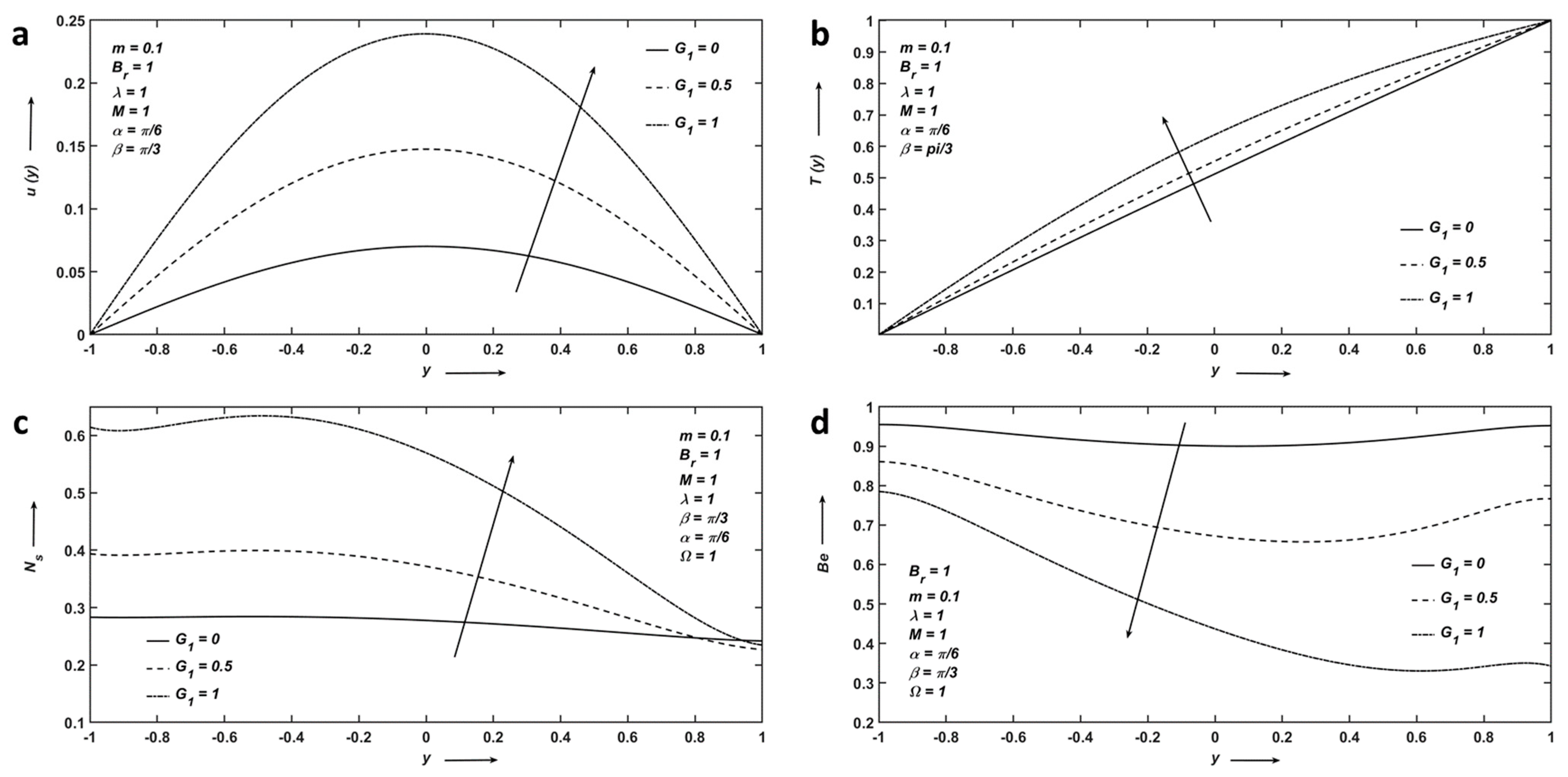
- Velocity and temperature distributions increase as the viscosity variation parameter, couple stress parameter, pressure gradient parameter, Brinkman number, and angle of inclined channel expand.
- By enhancing the Hartmann number and angle of the inclined magnetic field parameter, velocity, and temperature distributions decline.
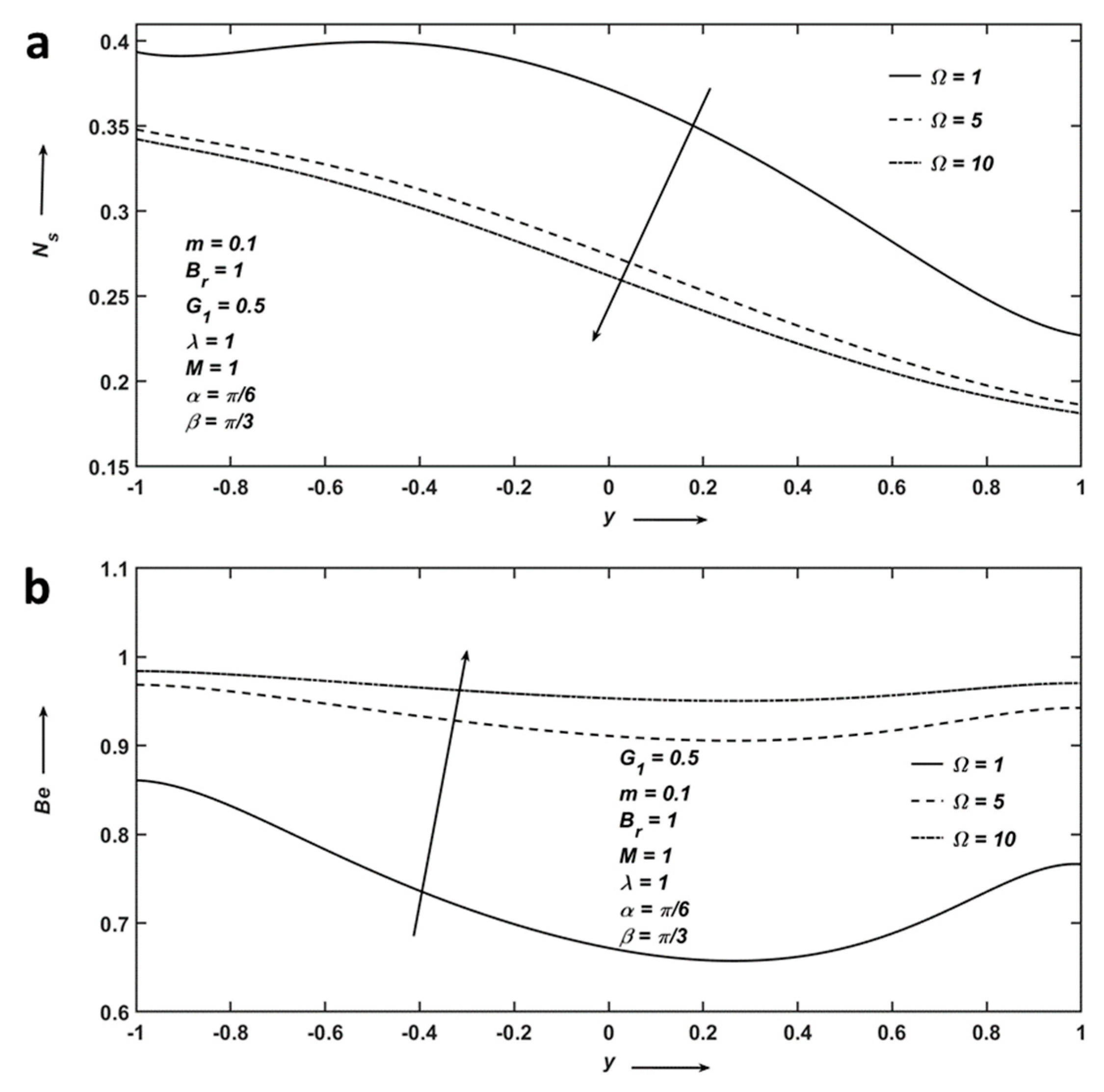
- The entropy production increases as the viscosity variation parameter, couple stress parameter, pressure gradient parameter, Brinkman number, and the angle of an inclined channel are enhanced.
- The entropy production decreases as the Hartmann number, temperature difference parameter, and angle of inclined magnetic field parameter increase.
- The Bejan number decreases as the viscosity variation parameter, couple stress parameter, pressure gradient parameter, Brinkman number, and angle of the inclined channel increase.
- The Hartmann number, angle of inclined magnetic field parameter, and temperature difference parameter increase the Bejan number.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| width of the channel | |
| Brinkman number | |
| dimensional fluid velocity | |
| gravitational force | |
| non-dimensional fluid velocity | |
| Reference velocity | |
| dynamic pressure | |
| non-dimensional entropy | |
| dimensional temperature | |
| Hartmann number | |
| Dimensional Entropy generation | |
| non-dimensional temperature | |
| non-dimensional viscosity parameter | |
| Bejan number | |
| dimensional couple stress parameter | |
| Thermal conductivity | |
| electrical conductivity | |
| dimensional viscosity | |
| constant density | |
| non-dimensional couple stress parameter | |
| Reference viscosity | |
| Temperature difference parameter | |
| angle of inclination of channel | |
| angle of inclined magnetic field |
Appendix A
References
- Seddeek, M.A. Heat and mass transfer on a stretching sheet with a magnetic field in a visco-elastic fluid flow through a porous medium with heat source or sink. Comput. Mater. Sci. 2007, 38, 781–787. [Google Scholar] [CrossRef]
- Mansour, M.; El-Hakiem, M.; El Kabeir, S. Heat and mass transfer in magnetohydrodynamic flow of micropolar fluid on a circular cylinder with uniform heat and mass flux. J. Magn. Magn. Mater. 2000, 220, 259–270. [Google Scholar] [CrossRef]
- Bég, O.A.; Bakier, A.; Prasad, V.; Zueco, J.; Ghosh, S. Nonsimilar, laminar, steady, electrically-conducting forced convection liquid metal boundary layer flow with induced magnetic field effects. Int. J. Therm. Sci. 2009, 48, 1596–1606. [Google Scholar] [CrossRef]
- Tashtoush, B. Magnetic and buoyancy effects on melting from a vertical plate embedded in saturated porous media. Energy Convers. Manag. 2005, 46, 2566–2577. [Google Scholar] [CrossRef]
- El-Amin, M. Magnetohydrodynamic free convection and mass transfer flow in micropolar fluid with constant suction. J. Magn. Magn. Mater. 2001, 234, 567–574. [Google Scholar] [CrossRef]
- Stokes, V.K. Couple Stresses in Fluids. Phys. Fluids 1966, 9, 1709. [Google Scholar] [CrossRef]
- Cheng, X.-T.; Liang, X.-G. Role of entropy generation minimization in thermal optimization. Chin. Phys. B 2017, 26, 120505. [Google Scholar] [CrossRef]
- Ahmed, S.; Bég, O.A.; Ghosh, S. A couple stress fluid modeling on free convection oscillatory hydromagnetic flow in an inclined rotating channel. Ain Shams Eng. J. 2014, 5, 1249–1265. [Google Scholar] [CrossRef]
- Jangili, S.; Adesanya, S.O.; Ogunseye, H.A.; Lebelo, R. Couple stress fluid flow with variable properties: A second law analysis. Math. Methods Appl. Sci. 2019, 42, 85–98. [Google Scholar] [CrossRef]
- Dar, A.A.; Elangovan, K. Influence of an inclined magnetic field on heat and mass transfer of the peristaltic flow of a couple stress fluid in an inclined channel. World J. Eng. 2017, 14, 7–18. [Google Scholar] [CrossRef]
- Xiong, P.-Y.; Nazeer, M.; Hussain, F.; Khan, M.I.; Saleem, A.; Qayyum, S.; Chu, Y.-M. Two-phase flow of couple stress fluid thermally effected slip boundary conditions: Numerical analysis with variable liquids properties. Alex. Eng. J. 2021, 61, 3821–3830. [Google Scholar] [CrossRef]
- Ajala, O.A.; Aselebe, L.O.; Abimbade, S.F.; Ogunsola, A.W. Effects of magnetic Fields on the Boundary Layer Flow of Heat Transfer with variable Viscosity in the presence of Thermal Radiation. Int. J. Sci. Res. Publ. 2019, 9, 13–19. [Google Scholar] [CrossRef]
- Falade, J.; Adesanya, S.; Ukaegbu, J.; Osinowo, M. Entropy generation analysis for variable viscous couple stress fluid flow through a channel with non-uniform wall temperature. Alex. Eng. J. 2016, 55, 69–75. [Google Scholar] [CrossRef]
- Ramesh, K. Influence of heat and mass transfer on peristaltic flow of a couple stress fluid through porous medium in the presence of inclined magnetic field in an inclined asymmetric channel. J. Mol. Liq. 2016, 219, 256–271. [Google Scholar] [CrossRef]
- Swarnalathamma, B.V.; Krishna, M.V. Peristaltic hemodynamic flow of couple stress fluid through a porous medium under the influence of magnetic field with slip effect. AIP Conf. Proc. 2016, 1728, 020603. [Google Scholar] [CrossRef]
- Divya, B.B.; Manjunatha, G.; Rajashekhar, C.; Vaidya, H.; Prasad, K.V. Effects of Inclined Magnetic Field and Porous Medium on Peristaltic Flow of a Bingham Fluid with Heat Transfer. J. Appl. Comput. Mech. 2021, 7, 1892–1906. [Google Scholar] [CrossRef]
- Hayat, T.; Bibi, S.; Rafiq, M.; Alsaedi, A.; Abbasi, F. Effect of an inclined magnetic field on peristaltic flow of Williamson fluid in an inclined channel with convective conditions. J. Magn. Magn. Mater. 2016, 401, 733–745. [Google Scholar] [CrossRef]
- Abbasi, F.M.; Hayat, T.; Ahmad, B. Hydromagnetic Peristaltic Transport of Variable Viscosity Fluid with Heat Transfer and Porous Medium. Appl. Math. Inf. Sci. 2016, 10, 2173–2181. [Google Scholar] [CrossRef]
- Abbasi, F.; Hayat, T.; Alsaedi, A. Effects of inclined magnetic field and Joule heating in mixed convective peristaltic transport of non-Newtonian fluids. Bull. Pol. Acad. Sci. Tech. Sci. 2015, 63, 501–514. [Google Scholar] [CrossRef]
- Effect of Aligned Magnetic Field on MHD Squeezing Flow of Casson Fluid between Parallel Plates | Scientific.Net. Available online: https://www.scientific.net/DDF.384.1 (accessed on 4 December 2022).
- Adesanya, S.O.; Onanaye, A.; Adeyemi, O.; Rahimi-Gorji, M.; Alarifi, I.M. Evaluation of heat irreversibility in couple stress falling liquid films along heated inclined substrate. J. Clean. Prod. 2019, 239, 117608. [Google Scholar] [CrossRef]
- Adesanya, S.O.; Dairo, O.; Yusuf, T.; Onanaye, A.; Arekete, S. Thermodynamics analysis for a heated gravity-driven hydromagnetic couple stress film with viscous dissipation effects. Phys. A Stat. Mech. Appl. 2020, 540, 123150. [Google Scholar] [CrossRef]
- Ganji, D.D.; Nezhad, H.R.A.; Hasanpour, A. Effect of variable viscosity and viscous dissipation on the Hagen-Poiseuille flow and entropy generation. Numer. Methods Partial. Differ. Equations 2011, 27, 529–540. [Google Scholar] [CrossRef]
- Ahmed, N. Heat and Mass Transfer in MHD Poiseuille Flow with Porous Walls. J. Eng. Phys. Thermophys. 2019, 92, 122–131. [Google Scholar] [CrossRef]
- Mopuri, O.; Kodi, R.; Ganteda, C.; Srikakulapu, R.; Lorenzini, G. MHD Heat and Mass Transfer Steady Flow of a Convective Fluid through a Porous Plate in The Presence of Diffusion Thermo and Aligned Magnetic Field. J. Adv. Res. Fluid Mech. Therm. Sci. 2022, 89, 62–76. [Google Scholar] [CrossRef]
- Krishna, M.V.; Anand, P.V.S.; Chamkha, A.J. Heat and mass transfer on free convective flow of amicropolar fluid through a porous surface with inclined magnetic field and hall effects. Spec. Top. Amp Rev. Porous Media Int. J. 2019, 10, 203–223. [Google Scholar] [CrossRef]
- Dada, M.; Salawu, S. Analysis of Heat and Mass Transfer of an Inclined Magnetic Field Pressure-driven Flow Past a Permeable Plate. Appl. Appl. Math. Int. J. AAM 2017, 12, 12. Available online: https://digitalcommons.pvamu.edu/aam/vol12/iss1/12 (accessed on 20 December 2022).
- Manjunatha, S.; Kuttan, B.A.; Jayanthi, S.; Chamkha, A.; Gireesha, B. Heat transfer enhancement in the boundary layer flow of hybrid nanofluids due to variable viscosity and natural convection. Heliyon 2019, 5, e01469. [Google Scholar] [CrossRef]
- Roja, A.; Gireesha, B.J. Impact of Hall and Ion effects on MHD couple stress nanofluid flow through an inclined channel subjected to convective, hydraulic slip, heat generation, and thermal radiation. Heat Transf. 2020, 49, 3314–3333. [Google Scholar] [CrossRef]
- Hayat, T.; Shafiq, A.; Alsaedi, A.; Asghar, S. Effect of inclined magnetic field in flow of third grade fluid with variable thermal conductivity. AIP Adv. 2015, 5, 087108. [Google Scholar] [CrossRef]
- Saif, R.S.; Muhammad, T.; Sadia, H. Significance of inclined magnetic field in Darcy—Forchheimer flow with variable porosity and thermal conductivity. Phys. A Stat. Mech. Appl. 2020, 551, 124067. [Google Scholar] [CrossRef]
- Makinde, O.D. Entropy-generation analysis for variable-viscosity channel flow with non-uniform wall temperature. Appl. Energy 2008, 85, 384–393. [Google Scholar] [CrossRef]
- Makinde, O.; Iskander, T.; Mabood, F.; Khan, W.; Tshehla, M. MHD Couette-Poiseuille flow of variable viscosity nanofluids in a rotating permeable channel with Hall effects. J. Mol. Liq. 2016, 221, 778–787. [Google Scholar] [CrossRef]
- Disu, A.; Dada, M. Reynold’s model viscosity on radiative MHD flow in a porous medium between two vertical wavy walls. J. Taibah Univ. Sci. 2017, 11, 548–565. [Google Scholar] [CrossRef]
- Makinde, O.; Khan, W.; Culham, J. MHD variable viscosity reacting flow over a convectively heated plate in a porous medium with thermophoresis and radiative heat transfer. Int. J. Heat Mass Transf. 2016, 93, 595–604. [Google Scholar] [CrossRef]
- Venkateswarlu, A.; Murshid, N.; Mulki, H.; Abu-samha, M.; Suneetha, S.; Babu, M.J.; Raju, C.S.K.; Homod, R.Z.; Al-Kouz, W. A Sig-nificant Role of Activation Energy and Fourier Flux on the Quadratically Radiated Sphere in Low and High Conductivity of Hybrid Nanoparticles. Symmetry 2022, 14, 2335. [Google Scholar] [CrossRef]
- Murshid, N.; Mulki, H.; Abu-Samha, M.; Owhaib, W.; Raju, S.S.K.; Raju, C.S.; JayachandraBabu, M.; Homod, R.Z.; Al-Kouz, W. Entropy Gen-eration and Statistical Analysis of MHD Hybrid Nanofluid Unsteady Squeezing Flow between Two Parallel Rotating Plates with Activation Energy. Nanomaterials 2022, 12, 2381. [Google Scholar] [CrossRef]
- Rashidi, M.M.; Nazari, M.A.; Mahariq, I.; Assad, M.E.H.; Ali, M.E.; Almuzaiqer, R.; Nuhait, A.; Murshid, N. Ther-mophysical properties of hybrid nanofluids and the proposed models: An updated comprehensive study. Nanomaterials 2021, 11, 3084. [Google Scholar] [CrossRef]
- Sohail, M.; Nazir, U.; Chu, Y.M.; Al-Kouz, W.; Thounthong, P. Bioconvection phenomenon for the boundary layer flow of magnetohydrodynamic Carreau liquid over a heated disk. Sci. Iran. 2021, 28, 1896–1907. [Google Scholar]
- Rana, P.; Al-Kouz, W.; Mahanthesh, B.; Mackolil, J. Heat transfer of TiO2−EG nanoliquid with active and pas-sive control of nanoparticles subject to nonlinear Boussinesq approximation. Int. Commun. Heat Mass Transf. 2021, 126, 105443. [Google Scholar] [CrossRef]
- Al-Kouz, W.; Abderrahmane, A.; Shamshuddin, M.D.; Younis, O.; Mohammed, S.; Bég, O.A.; Toghraie, D. Heat transfer and entropy generation analysis of water-Fe3O4/CNT hybrid magnetic nanofluid flow in a trapezoidal wavy enclosure containing porous media with the Galerkin finite element method. Eur. Phys. J. Plus 2021, 136, 1184. [Google Scholar] [CrossRef]
- Mahesh, A.; Varma, S.V.K.; Raju, C.S.K.; Babu, M.J.; Vajravelu, K.; Al-Kouz, W. Significance of non-Fourier heat flux and radiation on PEG–water based hybrid nanofluid flow among revolving disks with chemical reaction and en-tropy generation optimization. Int. Commun. Heat Mass Transf. 2021, 127, 105572. [Google Scholar] [CrossRef]
- Al-Kouz, W.; Al-Waked, R.; Sari, M.E.; Owhaib, W.; Atieh, A. Numerical study of heat transfer enhancement in the entrance region for low-pressure gaseous laminar pipe flows using Al2O3–air nanofluid. Adv. Mech. En Gineering 2018, 10, 1687814018784410. [Google Scholar] [CrossRef]
- Rana, P.; Mahanthesh, B.; Mackolil, J.; Al-Kouz, W. Nanofluid flow past a vertical plate with nanoparticle aggregation kinematics, thermal slip and significant buoyancy force effects using modified Buongiorno model. Waves Random Complex Media 2021, 1–25. [Google Scholar] [CrossRef]
- Ferhi, M.; Djebali, R.; Al-Kouz, W.; Abboudi, S.; Chamkha, A.J. MHD conjugate heat transfer and entropy generation analysis of MWCNT/water nanofluid in a partially heated divided medium. Heat Transf. 2021, 50, 126–144. [Google Scholar] [CrossRef]
- Algehyne, E.A.; Alrihieli, H.F.; Bilal, M.; Saeed, A.; Weera, W. Numerical Approach toward Ternary Hybrid Nanofluid Flow Using Variable Diffusion and Non-Fourier’s Concept. ACS Omega 2022, 7, 29380–29390. [Google Scholar] [CrossRef] [PubMed]
- Rehman, A.; Saeed, A.; Bilal, M. Analytical study of three-dimensional MHD hybrid nanofluid flow along with thermal characteristics and radiative solar energy. Waves Random Complex Media 2022, 1–15. [Google Scholar] [CrossRef]
- Bilal, M.; Alduais, F.S.; Alrabaiah, H.; Saeed, A. Numerical evaluation of Darcy Forchhemier hybrid nanofluid flow under the consequences of activation energy and second-order chemical reaction over a slender stretching sheet. Waves Random Complex Media 2022, 1–16. [Google Scholar] [CrossRef]
- Farooq, M.; Rahim, M.; Islam, S.; Siddiqui, A.M. Steady Poiseuille flow and heat transfer of couple stress fluids between two parallel inclined plates with variable viscosity. J. Assoc. Arab. Univ. Basic Appl. Sci. 2013, 14, 9–18. [Google Scholar] [CrossRef]
- Ellahi, R.; Zeeshan, A.; Hussain, F.; Abbas, T. Two-Phase Couette Flow of Couple Stress Fluid with Temperature Dependent Viscosity Thermally Affected by Magnetized Moving Surface. Symmetry 2019, 11, 647. [Google Scholar] [CrossRef]
- Dar, A.A. Effect of Thermal Radiation, Temperature Jump and Inclined Magnetic Field on the Peristaltic Transport of Blood Flow in an Asymmetric Channel with Variable Viscosity and Heat Absorption/Generation. Iran. J. Sci. Technol. Trans. Mech. Eng. 2021, 45, 487–501. [Google Scholar] [CrossRef]






| O D Makinde [32] | Present Study | |||
|---|---|---|---|---|
| 10 | 0.1317 | 0.5549 | 0.1317 | 0.5549 |
| 50 | 0.1334 | 0.7788 | 0.1334 | 0.7788 |
| 100 | 0.1356 | 1.0682 | 0.1356 | 1.0682 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Saini, G.; Hanumagowda, B.N.; Mulki, H.; Raju, S.S.K.; Varma, S.V.K.; Barghout, K.; Murshid, N.; Al-Kouz, W. Entropy Generation Optimization in Couple Stress Fluid Flow with Variable Viscosity and Aligned Magnetic Field. Sustainability 2023, 15, 2493. https://doi.org/10.3390/su15032493
Saini G, Hanumagowda BN, Mulki H, Raju SSK, Varma SVK, Barghout K, Murshid N, Al-Kouz W. Entropy Generation Optimization in Couple Stress Fluid Flow with Variable Viscosity and Aligned Magnetic Field. Sustainability. 2023; 15(3):2493. https://doi.org/10.3390/su15032493
Chicago/Turabian StyleSaini, Geetika, B. N. Hanumagowda, Hasan Mulki, S. Suresh Kumar Raju, S. V. K. Varma, Kamal Barghout, Nimer Murshid, and Wael Al-Kouz. 2023. "Entropy Generation Optimization in Couple Stress Fluid Flow with Variable Viscosity and Aligned Magnetic Field" Sustainability 15, no. 3: 2493. https://doi.org/10.3390/su15032493
APA StyleSaini, G., Hanumagowda, B. N., Mulki, H., Raju, S. S. K., Varma, S. V. K., Barghout, K., Murshid, N., & Al-Kouz, W. (2023). Entropy Generation Optimization in Couple Stress Fluid Flow with Variable Viscosity and Aligned Magnetic Field. Sustainability, 15(3), 2493. https://doi.org/10.3390/su15032493







