A Comparison of Internal and External Preheat Methods for NMC Batteries †
Abstract
:1. Introduction
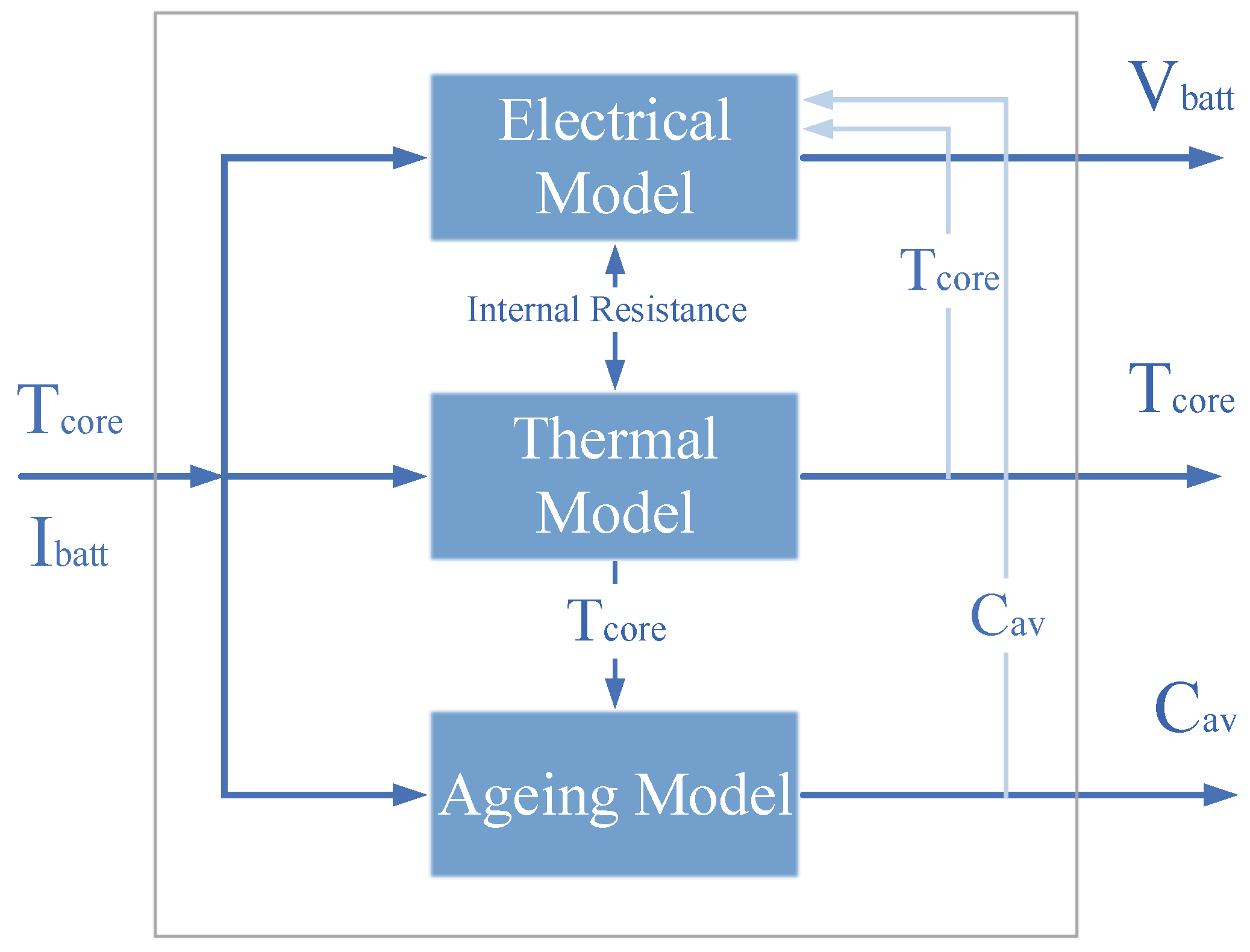
2. Model Development
2.1. General Model
2.2. Electrical Model
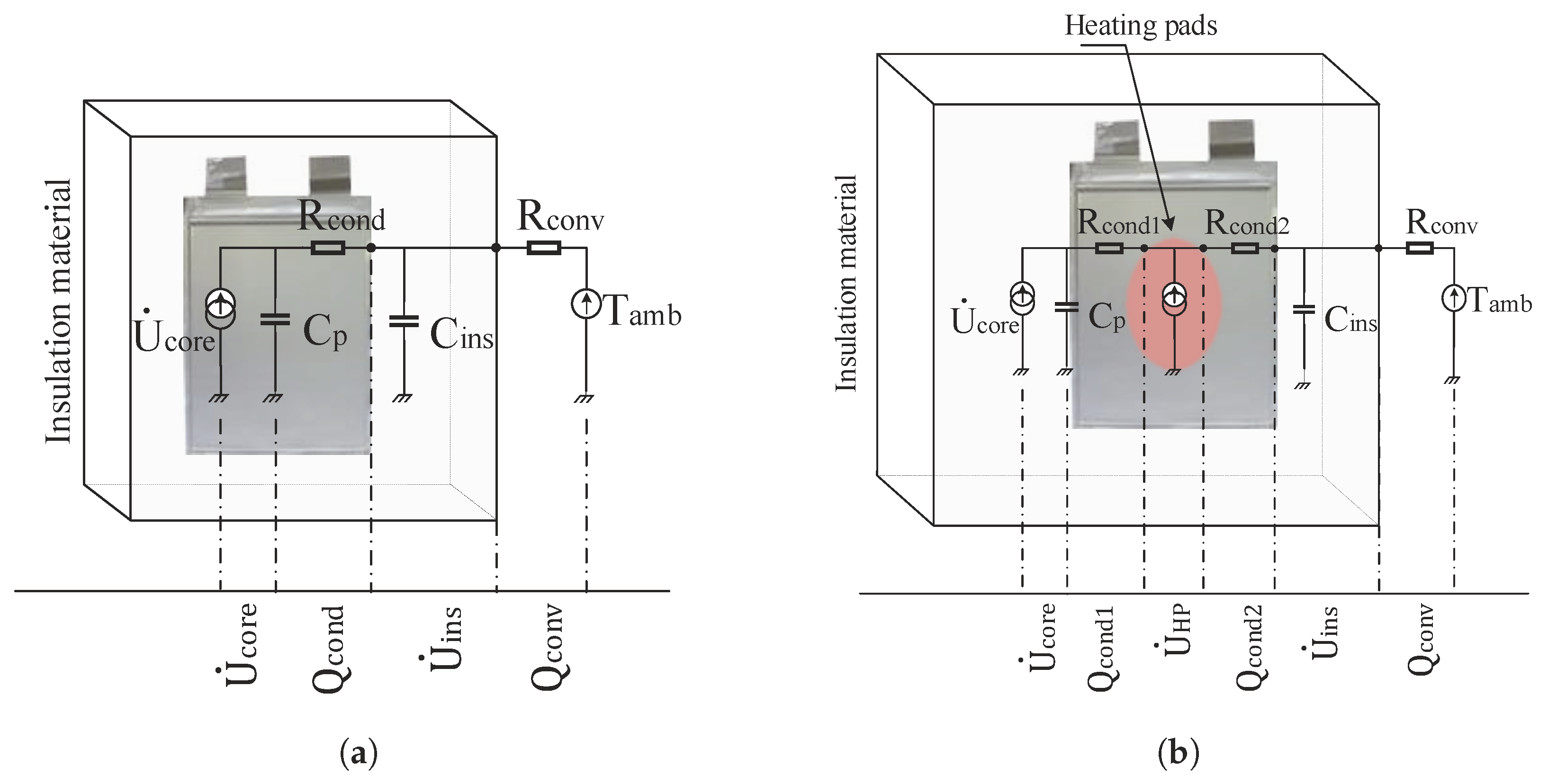
2.3. Thermal Model
2.3.1. APC Thermal Model Description
2.3.2. HP Thermal Model Description
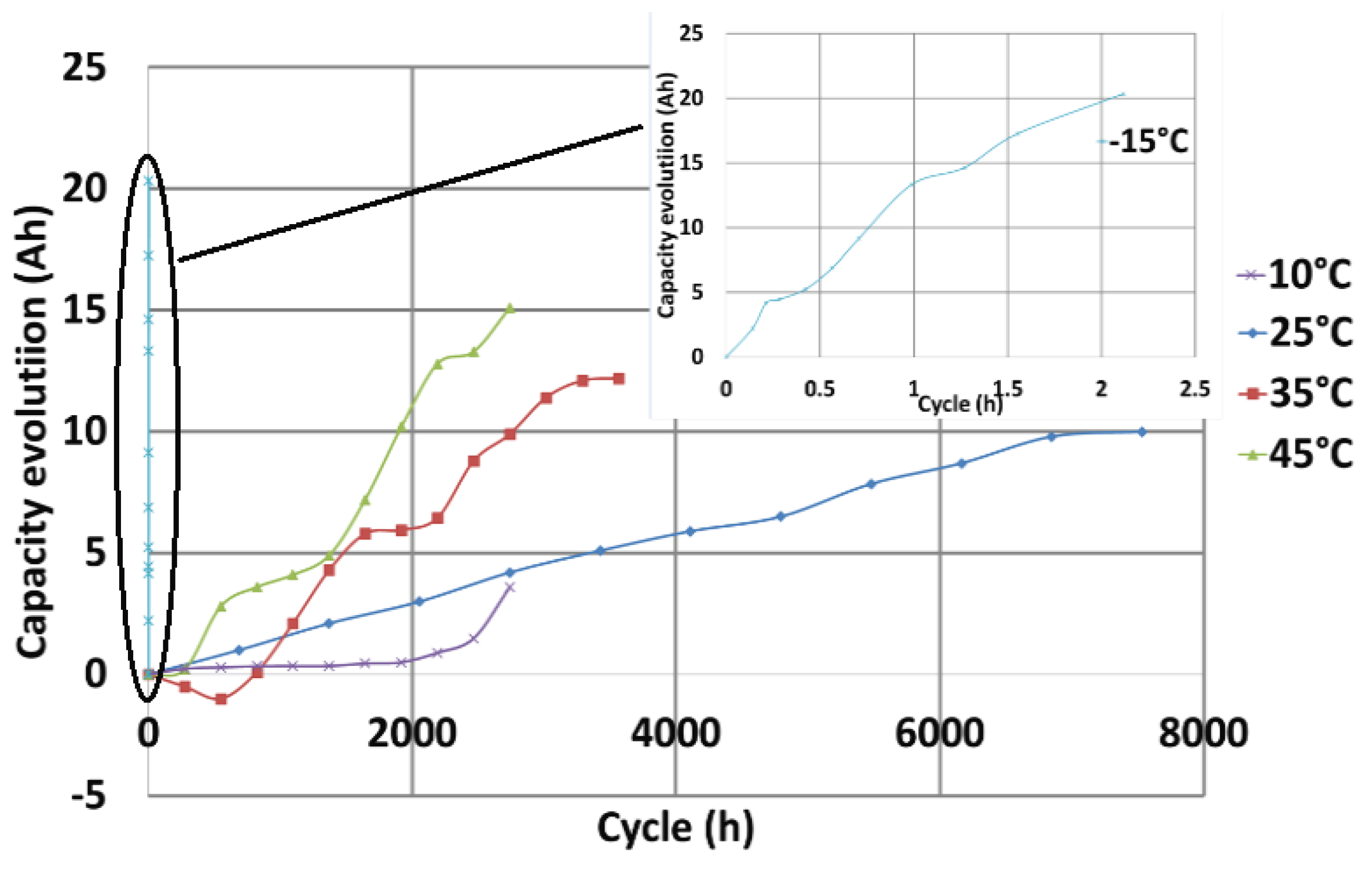
2.4. Aging Model
3. Experimental Profiles and Model Validation
3.1. Case Study
- The internal temperature of the battery cell is C due to the first cycle of the heating up phase.
- The ambient temperatures are set at C and C due to fast degraded performance at subzero temperatures of the pouch battery cell.
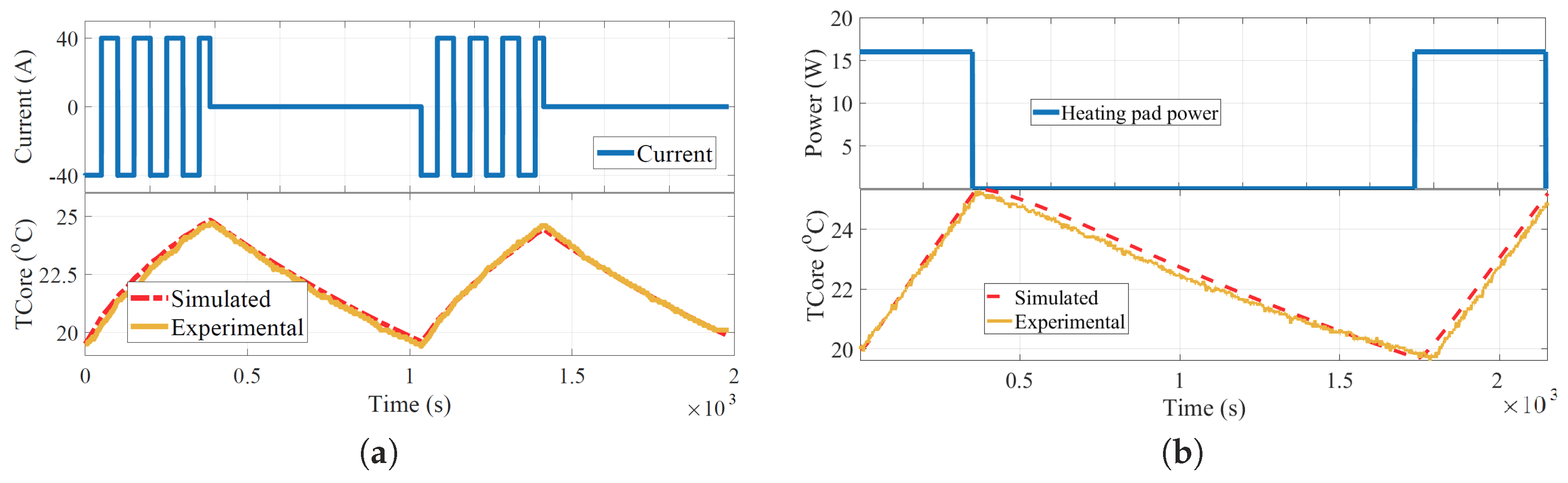
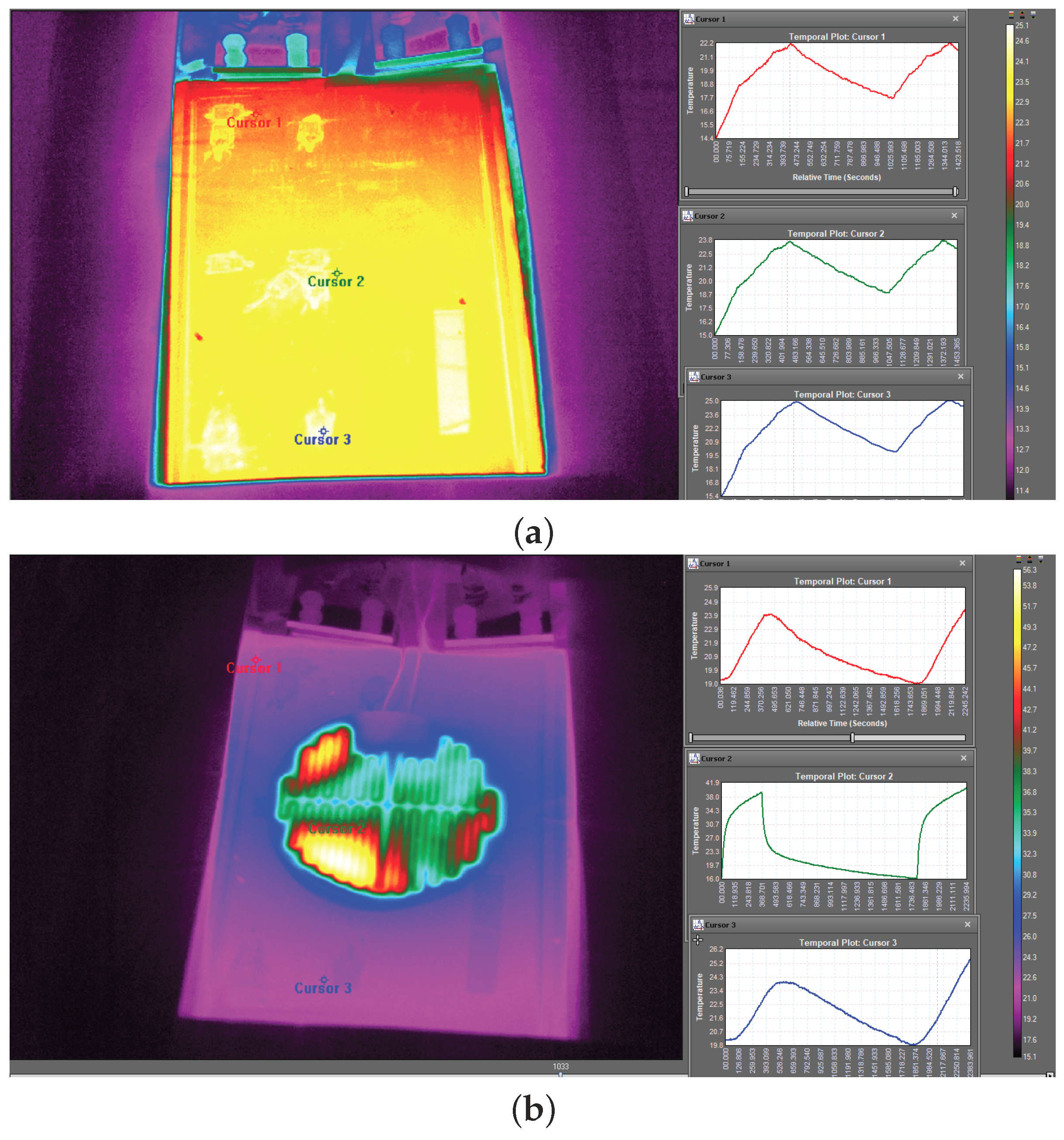
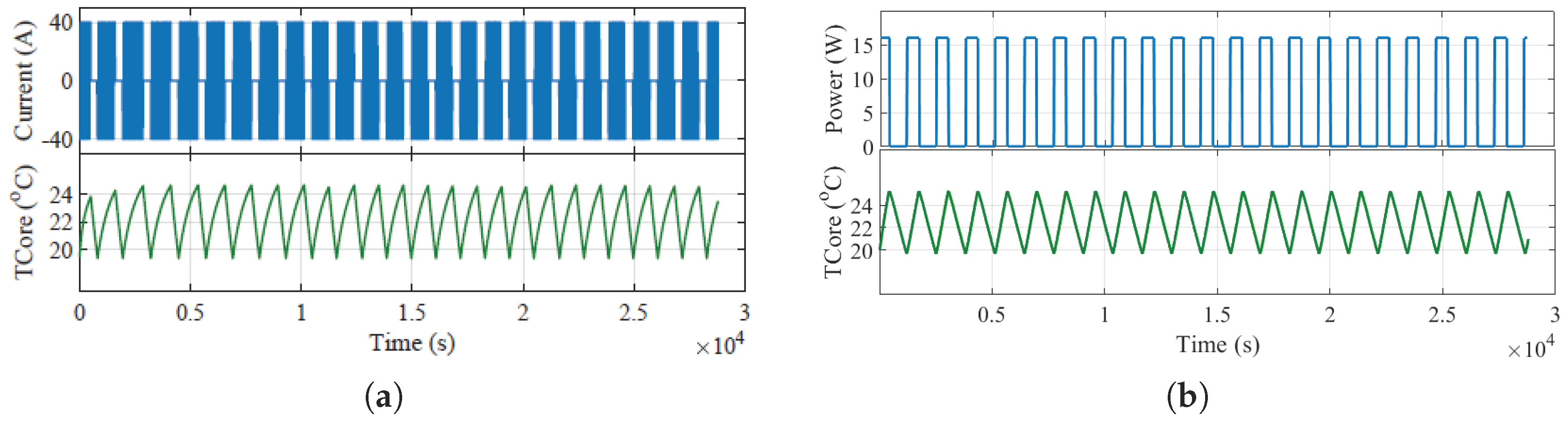
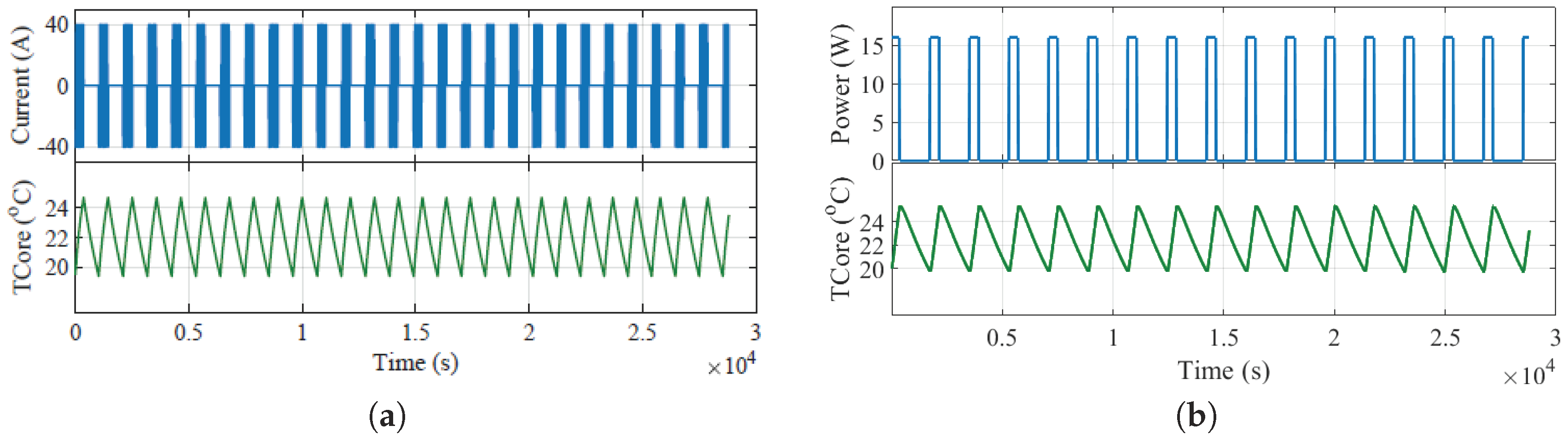
- The internal heating method (APC/bi-directional current pulses) applies a square-wave 40 A (2C of nominal current) amplitude, with a constant-low frequency 0.01 Hz and 50% duty cycle current, in order to maintain the SoC of the battery cell as close to a certain value as possible within a 100 s cycle. The external heating method heats up the cell with silicon-rubber heating sources rated at 16 W, which preserve its OCV constant throughout the whole experiment.
- The NMC/G battery cell is at the heating and temperature adjustment conditions for eight hours.
3.2. Results
3.2.1. Model Validation
3.2.2. IR Images
4. Cost Function and Comparison of Both Methods
4.1. Cost Functions
4.2. Results
4.2.1. Influence of the Ambient Temperature
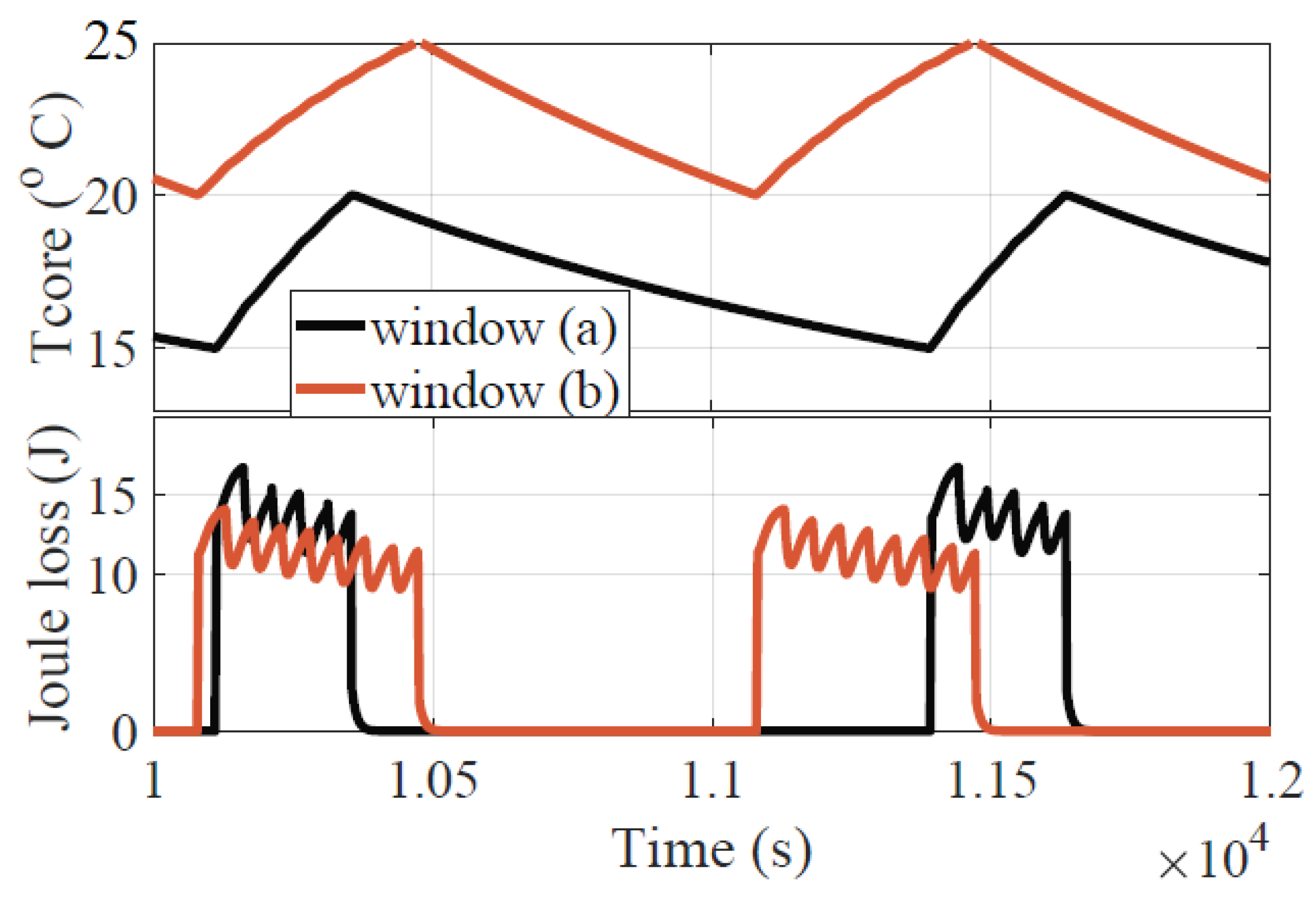
4.2.2. Influence of the Temperature Window
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Jaguemont, J.; Boulon, L.; Dubé, Y. A comprehensive review of lithium-ion batteries used in hybrid and electric vehicles at cold temperatures. Appl. Energy 2016, 164, 99–114. [Google Scholar] [CrossRef]
- Ji, Y.; Zhang, Y.; Wang, C.Y. Li-Ion Cell Operation at Low Temperatures. J. Electrochem. Soc. 2013, 160, A636–A649. [Google Scholar] [CrossRef]
- Park, M.; Zhang, X.; Chung, M.; Less, G.B.; Sastry, A.M. A review of conduction phenomena in Li-ion batteries. J. Power Sources 2010, 195, 7904–7929. [Google Scholar] [CrossRef]
- Jaguemont, J.; Boulon, L.; Dubé, Y.; Poudrier, D. Low Temperature Discharge Cycle Tests for a Lithium Ion Cell. In Proceedings of the 2014 IEEE Vehicle Power and Propulsion Conference (VPPC), Coimbra, Portugal, 27–30 October 2014; pp. 1–6. [Google Scholar]
- Shiao, H.C.; Chua, D.; Lin, H.P.; Slane, S.; Salomon, M. Low temperature electrolytes for Li-ion PVDF cells. J. Power Sources 2000, 87, 167–173. [Google Scholar] [CrossRef]
- Zhang, S.S.; Xu, K.; Allen, J.L.; Jow, T.R. Effect of propylene carbonate on the low temperature performance of Li-ion cells. J. Power Sources 2002, 110, 216–221. [Google Scholar] [CrossRef]
- Huang, C.K.; Sakamoto, J.S.; Wolfenstine, J.; Surampudi, S. The Limits of Low-Temperature Performance of Li-Ion Cells. J. Electrochem. Soc. 2002, 147, 2893. [Google Scholar] [CrossRef]
- Fan, J.; Tan, S. Studies on Charging Lithium-Ion Cells at Low Temperatures. J. Electrochem. Soc. 2007, 153, A1081. [Google Scholar] [CrossRef]
- Tippmann, S.; Walper, D.; Balboa, L.; Spier, B.; Bessler, W.G. Low-temperature charging of lithium-ion cells part I: Electrochemical modeling and experimental investigation of degradation behavior. J. Power Sources 2014, 252, 305–316. [Google Scholar] [CrossRef]
- Lei, Z.; Zhang, C.; Li, J.; Fan, G.; Lin, Z. Preheating method of lithium-ion batteries in an electric vehicle. J. Mod. Power Syst. Clean Energy 2015, 3, 289–296. [Google Scholar] [CrossRef]
- Samba, A.; Omar, N.; Gualous, H.; Capron, O.; Van den Bossche, P.; Van Mierlo, J. Impact of Tab Location on Large Format Lithium-Ion Pouch Cell Based on Fully Coupled Tree-Dimensional Electrochemical-Thermal Modeling. Electrochim. Acta 2014, 147, 319–329. [Google Scholar] [CrossRef]
- Vlahinos, A.; Pesaran, A.A. Energy Efficient Battery Heating in Cold Climates. SAE Tech. Pap. Ser. 2002, 1. [Google Scholar] [CrossRef]
- Jaguemont, J.; Nikolian, A.; Omar, N.; Goutam, S.; Van Mierlo, J.; Van Den Bossche, P. Development of a Two-Dimensional-Thermal Model of Three Battery Chemistries. IEEE Trans. Energy Convers. 2017, 32, 1447–1455. [Google Scholar] [CrossRef]
- de Hoog, J.; Jaguemont, J.; Abdel-Monem, M.; Van Den Bossche, P.; Van Mierlo, J.; Omar, N. Combining an Electrothermal and Impedance Aging Model to Investigate Thermal Degradation Caused by Fast Charging. Energies 2018, 11, 804. [Google Scholar] [CrossRef]
- International Electrotechnical Commission. Secondary Lithium-Ion Cells for the Propulsion of Electric Road Vehicles-Part 1: Performance Testing; Technical Report 62660-1; International Electrotechnical Commission: Geneva, Switzerland, 2011. [Google Scholar]
- Electrically Propelled Road Vehicles—Test Specification for Lithium-Ion Traction Battery Packs and Systems—Part 1: High-Power Applications; Technical Report ISO 12405-1:2011; International Organization for Standardization: Geneva, Switzerland, 2011.
- Nikolian, A.; Firouz, Y.; Gopalakrishnan, R.; Timmermans, J.M.; Omar, N.; van den Bossche, P.; van Mierlo, J. Lithium ion batteries-development of advanced electrical equivalent circuit models for nickel manganese cobalt lithium-ion. Energies 2016, 9, 360. [Google Scholar] [CrossRef]
- Farmann, A.; Sauer, D.U. Comparative study of reduced order equivalent circuit models for on-board state-of-available-power prediction of lithium-ion batteries in electric vehicles. Appl. Energy 2018, 225, 1102–1122. [Google Scholar] [CrossRef]
- Lai, X.; Zheng, Y.; Sun, T. A comparative study of different equivalent circuit models for estimating state-of-charge of lithium-ion batteries. Electrochim. Acta 2018, 259, 566–577. [Google Scholar] [CrossRef]
- Nikolian, A.; Jaguemont, J.; de Hoog, J.; Goutam, S.; Omar, N.; Van Den Bossche, P.; Van Mierlo, J. Complete cell-level lithium-ion electrical ECM model for different chemistries (NMC, LFP, LTO) and temperatures—Optimized modelling techniques. Int. J. Electr. Power Energy Syst. 2018, 98, 133–146. [Google Scholar] [CrossRef]
- Forgez, C.; Vinh Do, D.; Friedrich, G.; Morcrette, M.; Delacourt, C. Thermal modeling of a cylindrical LiFePO4/graphite lithium-ion battery. J. Power Sources 2010, 195, 2961–2968. [Google Scholar] [CrossRef]
- Bergman, T.L.; Lavigne, A.S.; Incropera, F.P.; Dewitt, D.P. Fundamentals of Heat and Mass Transfer; Wiley: Hoboken, NJ, USA, 2011; p. 1076. [Google Scholar]
- Jaguemont, J.; Boulon, L.; Dubé, Y. Characterization and modeling of a hybrid-electric-vehicle lithium-ion battery pack at low temperatures. IEEE Trans. Veh. Technol. 2016, 65, 1–14. [Google Scholar] [CrossRef]
- Ji, Y.; Wang, C.Y. Heating strategies for Li-ion batteries operated from subzero temperatures. Electrochim. Acta 2013, 107, 664–674. [Google Scholar] [CrossRef]
- Jaguemont, J.; Omar, N.; Martel, F.; Van den Bossche, P.; Van Mierlo, J. Streamline three-dimensional thermal model of a lithium titanate pouch cell battery in extreme temperature conditions with module simulation. J. Power Sources 2017, 367, 24–33. [Google Scholar] [CrossRef]
- de Hoog, J.; Jaguemont, J.; Nikolian, A.; Van Mierlo, J.; Van Den Bossche, P.; Omar, N. A combined thermo-electric resistance degradation model for nickel manganese cobalt oxide based lithium-ion cells. Appl. Therm. Eng. 2018, 135, 54–65. [Google Scholar] [CrossRef]
- de Hoog, J.; Timmermans, J.M.; Ioan-Stroe, D.; Swierczynski, M.; Jaguemont, J.; Goutam, S.; Omar, N.; Van Mierlo, J.; Van Den Bossche, P. Combined cycling and calendar capacity fade modeling of a Nickel-Manganese-Cobalt Oxide Cell with real-life profile validation. Appl. Energy 2017, 200, 47–61. [Google Scholar] [CrossRef]
- Zhang, G.; Ge, S.; Yang, X.G.; Leng, Y.; Marple, D.; Wang, C.Y. Rapid restoration of electric vehicle battery performance while driving at cold temperatures. J. Power Sources 2017, 371, 35–40. [Google Scholar] [CrossRef]
- Song, W.; Chen, M.; Bai, F.; Lin, S.; Chen, Y.; Feng, Z. Non-uniform effect on the thermal/aging performance of Lithium-ion pouch battery. Appl. Therm. Eng. 2018, 128, 1165–1174. [Google Scholar] [CrossRef]
- Lei, Z.; Zhang, Y.; Lei, X. Temperature uniformity of a heated lithium-ion battery cell in cold climate. Appl. Therm. Eng. 2018, 129, 148–154. [Google Scholar] [CrossRef]


| Main Characteristics | Value | Unit |
|---|---|---|
| Type | NMC/G | [-] |
| Nominal voltage | 3.65 | [V] |
| Nominal capacity | 20 | [Ah] |
| Specific energy | 174 | [Wh/kg] |
| Volumetric energy density | 370 | [Wh/L] |
| End-of-charge maximum voltage | 4.2 | [V] |
| End-of-discharge cut-off voltage | 3 | [V] |
| Volumetric energy density | 370 | [Wh/L] |
| AC impedance (1 KHz) | 3 | [mOhms] |
| Recommended charge current rate | C/2 | [-] |
| Weight | 0.428 | [kg] |
| Dimensions (Length × Width × Thickness) | 217 × 130 × 7 | [mm] |
| Main Characteristics | Value | Unit |
|---|---|---|
| Specific heat () | 785 | [J/kg·K] |
| Thermal conductivity () | 30 | [W/m·K] |
| Heat transfer coefficient () | 5 | [W/m·K] |
| Density () | 2247 | [kg/m] |
| Insulation material thermal properties | ||
| Main Characteristics | Value | Unit |
| Type | Polystyrene foam | [-] |
| Specific heat () | 1100 | [J/kg·K] |
| Thermal conductivity () | 0.033 | [W/m·K] |
| Mass () | 0.145 | [kg] |
| Area () | 0.0825 | [m] |
| Thickness () | 0.005 | [m] |
| Heat transfer coefficient () | 7.7 | [W/m·K] |
| Dimensions () | 0.0825 | [m] |
| Heating pad thermal properties | ||
| Main Characteristics | Value | Unit |
| Watt densities | 0.78 | [W/cm)] |
| Specific heat () | 900 | [J/kg·K] |
| Thermal Conductivity () | 0.03 | [W/m·K] |
| Area () | 0.031 | [m] |
| Thickness () | 0.0015 | [m] |
| Wire-wound element thickness | 0.0014 | [m] |
| Dimensions () | 0.105 | [m] |
| Temperature (C) | Degradation rate, (% per Hours of Cycling) |
|---|---|
| −15 | 10.2 |
| 10 | 0.004 |
| 25 | 0.0014 |
| 35 | 0.0034 |
| 45 | 0.0052 |
| Bi-directional current—Internal heating approach | |||
| Ambient Temperature (C) | Energy Cost (euro) | Degradation Cost (euro) | Total Cost (euro) |
| −15 | 0.018 | EoL conditions | EoL conditions |
| 0 | 0.012 | 0.025 | 0.037 |
| 10 | 0.006 | 0.024 | 0.030 |
| Heating pads—External heating approach | |||
| Ambient Temperature (C) | Energy Cost (euro) | Degradation Cost (euro) | Total Cost (euro) |
| −15 | 0.019 | 0.023 | 0.042 |
| 0 | 0.011 | 0.023 | 0.035 |
| 10 | 0.005 | 0.023 | 0.028 |
| Bi-directional Current Approach | ||||
|---|---|---|---|---|
| Ambient Temp. (C) | Temp. Window | Energy Cost (euro) | Degradation Cost (euro) | Total Cost (euro) |
| 0 | window (a) | 0.009 | 0.018 | 0.027 |
| window (b) | 0.012 | 0.025 | 0.037 | |
| 10 | window (a) | 0.005 | 0.017 | 0.022 |
| window (b) | 0.006 | 0.024 | 0.030 | |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kalogiannis, T.; Jaguemont, J.; Omar, N.; Van Mierlo, J.; Van den Bossche, P. A Comparison of Internal and External Preheat Methods for NMC Batteries. World Electr. Veh. J. 2019, 10, 18. https://doi.org/10.3390/wevj10020018
Kalogiannis T, Jaguemont J, Omar N, Van Mierlo J, Van den Bossche P. A Comparison of Internal and External Preheat Methods for NMC Batteries. World Electric Vehicle Journal. 2019; 10(2):18. https://doi.org/10.3390/wevj10020018
Chicago/Turabian StyleKalogiannis, Theodoros, Joris Jaguemont, Noshin Omar, Joeri Van Mierlo, and Peter Van den Bossche. 2019. "A Comparison of Internal and External Preheat Methods for NMC Batteries" World Electric Vehicle Journal 10, no. 2: 18. https://doi.org/10.3390/wevj10020018
APA StyleKalogiannis, T., Jaguemont, J., Omar, N., Van Mierlo, J., & Van den Bossche, P. (2019). A Comparison of Internal and External Preheat Methods for NMC Batteries. World Electric Vehicle Journal, 10(2), 18. https://doi.org/10.3390/wevj10020018








