Ab Initio Molecular Dynamics Insights into Stress Corrosion Cracking and Dissolution of Metal Oxides
Abstract
1. Introduction
2. Materials and Methods
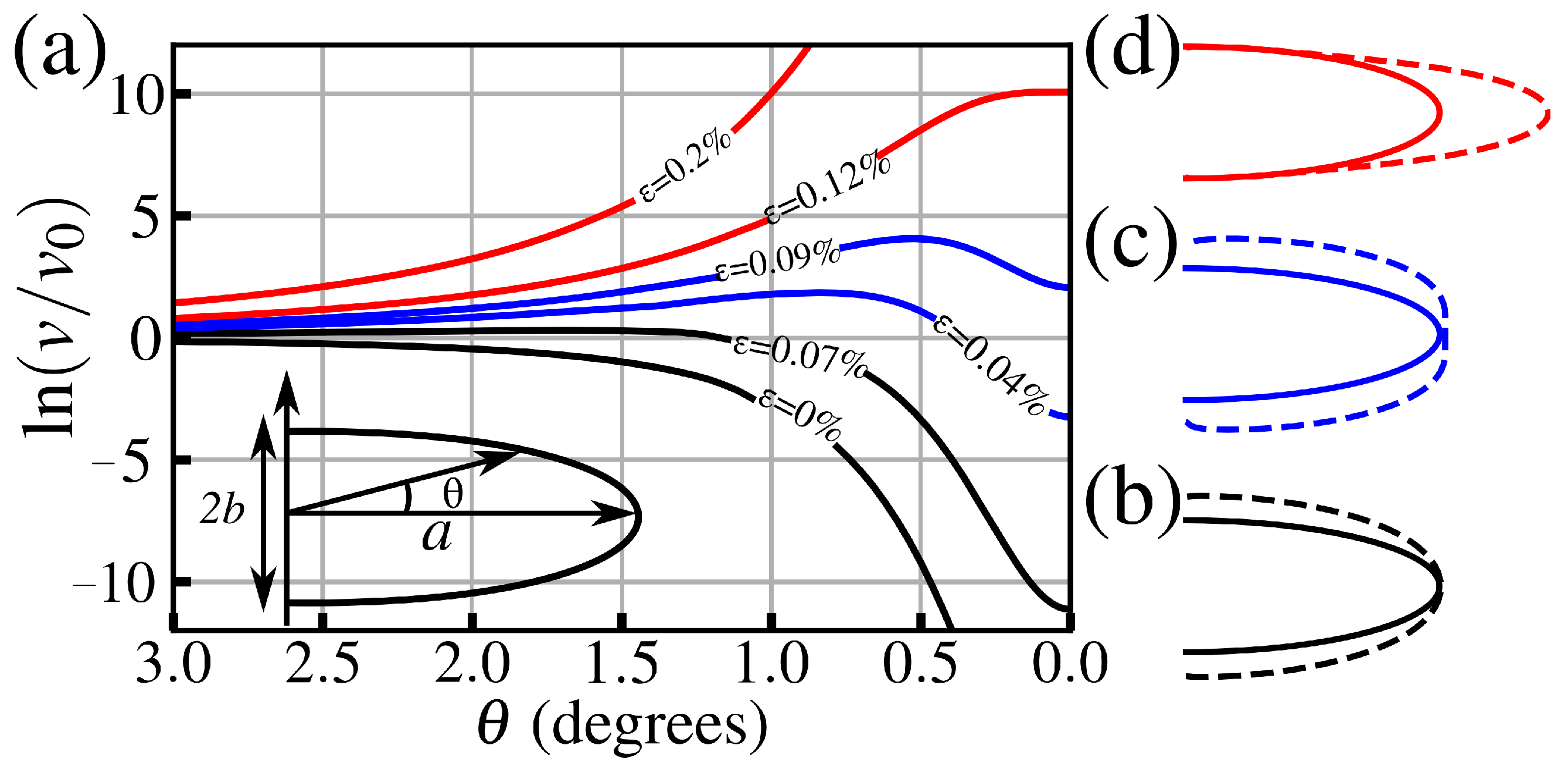
2.1. Fracture Energies and Crack Tip Analysis
2.2. J-Integral from Atomistic Simulations
2.3. Free-Energy Sampling with Ab Initio Metadynamics
3. Results and Discussion
3.1. Fracture Energy and Lattice Trapping of Cracks in -Al2O3
3.2. Stress-Enhanced Dissolution
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cui, G.; Yang, Z.; Liu, J.; Li, Z. A comprehensive review of metal corrosion in a supercritical CO2 environment. Int. J. Greenh. Gas Control 2019, 90, 102814. [Google Scholar] [CrossRef]
- McGrail, B.P.; Schaef, H.T.; Glezakou, V.A.; Dang, L.X.; Owen, A.T. Water reactivity in the liquid and supercritical CO2 phase: Has half the story been neglected? Energy Procedia 2009, 1, 3415–3419. [Google Scholar] [CrossRef]
- Li, Q.K.; Kutana, A.; Penev, E.S.; Yakobson, B.I. Iron corrosion in the “inert” supercritical CO2, ab initio dynamics insights: How impurities matter. Matter 2022, 5, 751–762. [Google Scholar] [CrossRef]
- Yamamoto, Y.; Brady, M.P.; Lu, Z.P.; Maziasz, P.J.; Liu, C.T.; Pint, B.A.; More, K.L.; Meyer, H.M.; Payzant, E.A. Creep-Resistant, Al2O3-Forming Austenitic Stainless Steels. Science 2009, 316, 433–436. [Google Scholar] [CrossRef]
- Hyer, H.C.; Dryepondt, S.; Su, Y.F.; Yamamoto, Y.; Pint, B.A.; Massey, C.P. Strength stability at high temperatures for additively manufactured alumina forming austenitic alloy. Scr. Mater. 2024, 253, 116286. [Google Scholar] [CrossRef]
- Guo, X.; Chen, K.; Gao, W.; Shen, Z.; Zhang, L. Corrosion behavior of alumina-forming and oxide dispersion strengthened austenitic 316 stainless steel in supercritical water. Corros. Sci. 2018, 138, 297–306. [Google Scholar] [CrossRef]
- Gwalani, B.; Escobar, J.; Song, M.; Thomas, J.; Silverstein, J.; Chuang, A.C.; Singh, D.; Brady, M.P.; Yamamoto, Y.; Watkins, T.R.; et al. Mechanisms for high creep resistance in alumina forming austenitic (AFA) alloys. Acta Mater. 2023, 263, 119494. [Google Scholar] [CrossRef]
- Liu, Z.; Zhou, Q.; Cong, S.; Duan, Z.; Ma, Z.; Shu, M.; Zhou, Z.; Zhang, L.; Guo, X. Corrosion behavior of a new alumina-forming duplex stainless steel with different surface treatment in supercritical carbon dioxide. Surf. Coat. Technol. 2023, 466, 129619. [Google Scholar] [CrossRef]
- Yakobson, B.I. Morphology and rate of fracture in chemical decomposition of solids. Phys. Rev. Lett. 1991, 67, 1590–1593. [Google Scholar] [CrossRef]
- Xu, Z. Environmentally-Assisted Fatigue at Small Scales. In Comprehensive Structural Integrity, 2nd ed.; Aliabadi, M.H.F., Soboyejo, W.O., Eds.; Elsevier: Amsterdam, The Netherlands, 2023; pp. 131–162. [Google Scholar] [CrossRef]
- Jones, D.E.H. Rusty Armor. In The Inventions of Daedalus: A Compendium of Plausible Schemes; W.H. Freeman: New York, NY, USA, 1982; p. 56. [Google Scholar]
- Wiederhorn, S.M.; Fuller, E.R.; Thomson, R. Micromechanisms of crack growth in ceramics and glasses in corrosive environments. Met. Sci. 1980, 14, 450–458. [Google Scholar] [CrossRef]
- Michalske, T.A.; Freiman, S.W. A molecular interpretation of stress corrosion in silica. Nature 1982, 295, 511–512. [Google Scholar] [CrossRef]
- Michalske, T.A.; Bunker, B.C.; Freiman, S.W. Stress Corrosion of Ionic and Mixed Ionic/Covalent Solids. J. Am. Ceram. Soc. 1986, 69, 721–724. [Google Scholar] [CrossRef]
- Michalske, T.A.; Bunker, B.C. Steric Effects in Stress Corrosion Fracture of Glass. J. Am. Ceram. Soc. 1987, 70, 780–784. [Google Scholar] [CrossRef]
- Hass, K.C.; Schneider, W.F.; Curioni, A.; Andreoni, W. First-Principles Molecular Dynamics Simulations of H2O on α-Al2O3 (0001). J. Phys. Chem. B 2000, 104, 5527–5540. [Google Scholar] [CrossRef]
- Wiederhorn, S.M. Moisture assisted crack growth in ceramics. Int. J. Fract. Mech. 1968, 4, 171–177. [Google Scholar] [CrossRef]
- Cook, R.F. Environmentally-controlled non-equilibrium crack propagation in ceramics. Mater. Sci. Eng. A 1999, 260, 29–40. [Google Scholar] [CrossRef]
- Brett, C.M.A.; Gomes, I.A.R.; Martins, J.P.S. The electrochemical behaviour and corrosion of aluminium in chloride media. The effect of inhibitor anions. Corros. Sci. 1994, 36, 915–923. [Google Scholar] [CrossRef]
- Yakobson, B.I.; Shchukin, E.D. Environmentally enhanced instability of stressed solids. J. Mater. Sci. 1993, 28, 4816–4820. [Google Scholar] [CrossRef]
- Yakobson, B.I. Stress-promoted interface diffusion as a precursor of fracture. J. Chem. Phys. 1993, 99, 6923–6934. [Google Scholar] [CrossRef]
- Scher, J.A.; Weitzner, S.E.; Hao, Y.; Heo, T.W.; Castonguay, S.T.; Aubry, S.; Carroll, S.A.; Kroonblawd, M.P. Model for Humidity-Mediated Diffusion on Aluminum Surfaces and Its Role in Accelerating Atmospheric Aluminum Corrosion. ACS Appl. Mater. Interfaces 2023, 15, 28716–28730. [Google Scholar] [CrossRef]
- Spatschek, R.; Brener, E.A. Grinfeld instability on crack surfaces. Physical Review E 2001, 64, 046120. [Google Scholar] [CrossRef]
- Kahn, H.; Ballarini, R.; Bellante, J.J.; Heuer, A.H. Fatigue Failure in Polysilicon Not Due to Simple Stress Corrosion Cracking. Science 2002, 298, 1215–1218. [Google Scholar] [CrossRef]
- Moshtaghi, M.; Eškinja, M.; Mori, G.; Griesser, T.; Safyari, M.; Cole, I. The effect of HPAM polymer for enhanced oil recovery on corrosion behaviour of a carbon steel and interaction with the inhibitor under simulated brine conditions. Corros. Sci. 2023, 217, 111118. [Google Scholar] [CrossRef]
- Mukhametzianova, G.; Wagner, S.; Eskinja, M.; Moshtaghi, M.; Mori, G.; Prohaska, T. Mapping elemental solutes at sub-picogram levels during aqueous corrosion of Al alloys using diffusive gradients in thin films (DGT) with LA-ICP-MS. Anal. Bioanal. Chem. 2024, 416, 3373–3388. [Google Scholar] [CrossRef] [PubMed]
- Zhang, H.; Sun, W.; Xie, X.; He, J.; Zhang, C. Insights into the Fracture Nature of Hematite from First Principles DFT Calculations. ACS Omega 2023, 8, 8248–8255. [Google Scholar] [CrossRef]
- Kühne, T.D.; Iannuzzi, M.; Del Ben, M.; Rybkin, V.V.; Seewald, P.; Stein, F.; Laino, T.; Khaliullin, R.Z.; Schütt, O.; Schiffmann, F.; et al. CP2K: An electronic structure and molecular dynamics software package—Quickstep: Efficient and accurate electronic structure calculations. J. Chem. Phys. 2020, 152, 194103. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Liu, X.; van Duin, A.C.T.; Lu, X.; Meijer, E.J. Development and validation of a general-purpose ReaxFF reactive force field for earth material modeling. J. Chem. Phys. 2024, 160, 094103. [Google Scholar] [CrossRef] [PubMed]
- Thompson, A.P.; Aktulga, H.M.; Berger, R.; Bolintineanu, D.S.; Brown, W.M.; Crozier, P.S.; in ’t Veld, P.J.; Kohlmeyer, A.; Moore, S.G.; Nguyen, T.D.; et al. LAMMPS—A flexible simulation tool for particle-based materials modeling at the atomic, meso, and continuum scales. Comput. Phys. Commun. 2022, 271, 108171. [Google Scholar] [CrossRef]
- Larsen, A.H.; Mortensen, J.J.; Blomqvist, J.; Castelli, I.E.; Christensen, R.; Dułak, M.; Friis, J.; Groves, M.N.; Hammer, B.; Hargus, C.; et al. The atomic simulation environment—A Python library for working with atoms. J. Physics Condens. Matter 2017, 29, 273002. [Google Scholar] [CrossRef]
- Zhang, S.; Zhu, T.; Belytschko, T. Atomistic and multiscale analyses of brittle fracture in crystal lattices. Phys. Rev. B 2007, 76, 094114. [Google Scholar] [CrossRef]
- Zhang, Z.; Kutana, A.; Yakobson, B.I. Edge reconstruction-mediated graphene fracture. Nanoscale 2015, 7, 2716–2722. [Google Scholar] [CrossRef] [PubMed]
- Rice, J.R. A Path Independent Integral and the Approximate Analysis of Strain Concentration by Notches and Cracks. J. Appl. Mech. 1968, 35, 379–386. [Google Scholar] [CrossRef]
- Zimmerman, J.A.; Bammann, D.J.; Gao, H. Deformation gradients for continuum mechanical analysis of atomistic simulations. Int. J. Solids Struct. 2009, 46, 238–253. [Google Scholar] [CrossRef]
- Jin, Y.; Yuan, F.G. Atomistic Simulations of J-Integral in 2D Graphene Nanosystems. J. Nanosci. Nanotechnol. 2005, 5, 2099–2107. [Google Scholar] [CrossRef] [PubMed]
- Meng, F.; Chen, C.; Song, J. Lattice trapping and crack decohesion in graphene. Carbon 2017, 116, 33–39. [Google Scholar] [CrossRef]
- Kim, H.; Kim, S.; Kim, S.Y. Lattice-based J integral for a steadily moving dislocation. Int. J. Plast. 2021, 138, 102949. [Google Scholar] [CrossRef]
- Khare, R.; Mielke, S.L.; Paci, J.T.; Zhang, S.; Ballarini, R.; Schatz, G.C.; Belytschko, T. Coupled quantum mechanical/molecular mechanical modeling of the fracture of defective carbon nanotubes and graphene sheets. Phys. Rev. B 2007, 75, 075412. [Google Scholar] [CrossRef]
- Jones, R.E.; Zimmerman, J.A.; Oswald, J.; Belytschko, T. An atomistic J-integral at finite temperature based on Hardy estimates of continuum fields. J. Physics Condens. Matter 2010, 23, 015002. [Google Scholar] [CrossRef]
- Rimsza, J.M.; Jones, R.E.; Criscenti, L.J. Crack propagation in silica from reactive classical molecular dynamics simulations. J. Am. Ceram. Soc. 2018, 101, 1488–1499. [Google Scholar] [CrossRef]
- Rimsza, J.M.; Jones, R.E.; Criscenti, L.J. Chemical Effects on Subcritical Fracture in Silica From Molecular Dynamics Simulations. J. Geophys. Res. Solid Earth 2018, 123, 9341–9354. [Google Scholar] [CrossRef]
- Shen, Z.; Kerisit, S.N.; Stack, A.G.; Rosso, K.M. Free-Energy Landscape of the Dissolution of Gibbsite at High pH. J. Phys. Chem. Lett. 2018, 9, 1809–1814. [Google Scholar] [CrossRef] [PubMed]
- Martínez, L.; Andrade, R.; Birgin, E.G.; Martínez, J.M. PACKMOL: A package for building initial configurations for molecular dynamics simulations. J. Comput. Chem. 2009, 30, 2157–2164. [Google Scholar] [CrossRef]
- Cygan, R.T.; Greathouse, J.A.; Kalinichev, A.G. Advances in Clayff Molecular Simulation of Layered and Nanoporous Materials and Their Aqueous Interfaces. J. Phys. Chem. C 2021, 125, 17573–17589. [Google Scholar] [CrossRef]
- Réocreux, R.E.; Girel, E.; Clabaut, P.; Tuel, A.; Besson, M.; Chaumonnot, A.; Cabiac, A.; Sautet, P.; Michel, C. Reactivity of shape-controlled crystals and metadynamics simulations locate the weak spots of alumina in water. Nat. Commun. 2019, 10, 3139. [Google Scholar] [CrossRef] [PubMed]
- Wiederhorn, S.M.; Hockey, B.J.; Roberts, D.E. Effect of temperature on the fracture of sapphire. Philos. Mag. J. Theor. Exp. Appl. Phys. 1973, 28, 783–796. [Google Scholar] [CrossRef]
- Liao, P.; Carter, E.A. Ab initio DFT + U predictions of tensile properties of iron oxides. J. Mater. Chem. 2010, 20, 6703–6719. [Google Scholar] [CrossRef]
- Connolly, M.L. Solvent-Accessible Surfaces of Proteins and Nucleic Acids. Science 1983, 221, 709–713. [Google Scholar] [CrossRef] [PubMed]
- Zhu, T.; Li, J.; Lin, X.; Yip, S. Stress-dependent molecular pathways of silica–water reaction. J. Mech. Phys. Solids 2005, 53, 1597–1623. [Google Scholar] [CrossRef]
- Peskleway, C.D.; Henderson, G.S.; Wicks, F.J. Dissolution of gibbsite: Direct observations using fluid cell atomic force microscopy. Am. Mineral. 2003, 88, 18–26. [Google Scholar] [CrossRef]
- Bénézeth, P.; Palmer, D.A.; Wesolowski, D.J. Dissolution/precipitation kinetics of boehmite and gibbsite: Application of a pH-relaxation technique to study near-equilibrium rates. Geochim. Cosmochim. Acta 2008, 72, 2429–2453. [Google Scholar] [CrossRef]
- Zou, S.; Burke, M.S.; Kast, M.G.; Fan, J.; Danilovic, N.; Boettcher, S.W. Fe (Oxy)hydroxide Oxygen Evolution Reaction Electrocatalysis: Intrinsic Activity and the Roles of Electrical Conductivity, Substrate, and Dissolution. Chem. Mater. 2015, 27, 8011–8020. [Google Scholar] [CrossRef]
- Klyukin, K.; Rosso, K.M.; Alexandrov, V. Iron Dissolution from Goethite (α-FeOOH) Surfaces in Water by Ab Initio Enhanced Free-Energy Simulations. J. Phys. Chem. C 2018, 122, 16086–16091. [Google Scholar] [CrossRef]
- Dumitrică, T.; Belytschko, T.; Yakobson, B.I. Bond-breaking bifurcation states in carbon nanotube fracture. J. Chem. Phys. 2003, 118, 9485–9488. [Google Scholar] [CrossRef]
- Chuang, T.j.; Fuller Jr., E. R. Extended Charles–Hillig Theory for Stress Corrosion Cracking of Glass. J. Am. Ceram. Soc. 1992, 75, 540–545. [Google Scholar] [CrossRef]
- Liu, X.; Hwang, W.; Park, J.; Van, D.; Chang, Y.; Lee, S.H.; Kim, S.Y.; Han, S.; Lee, B. Toward the multiscale nature of stress corrosion cracking. Nucl. Eng. Technol. 2018, 50, 1–17. [Google Scholar] [CrossRef]
- Yang, Y.; Zhou, W.; Yin, S.; Wang, S.Y.; Yu, Q.; Olszta, M.J.; Zhang, Y.Q.; Zeltmann, S.E.; Li, M.; Jin, M.; et al. One dimensional wormhole corrosion in metals. Nat. Commun. 2023, 14, 988. [Google Scholar] [CrossRef]
- Pelmenschikov, A.; Strandh, H.; Pettersson, L.G.M.; Leszczynski, J. Lattice Resistance to Hydrolysis of Si-O-Si Bonds of Silicate Minerals: Ab Initio Calculations of a Single Water Attack onto the (001) and (111) β-Cristobalite Surfaces. J. Phys. Chem. B 2000, 104, 5779–5783. [Google Scholar] [CrossRef]
- Huang, S.; Zhang, S.; Belytschko, T.; Terdalkar, S.S.; Zhu, T. Mechanics of nanocrack: Fracture, dislocation emission, and amorphization. J. Mech. Phys. Solids 2009, 57, 840–850. [Google Scholar] [CrossRef]
- Lawn, B. Fracture of Brittle Solids, 2nd ed.; Cambridge Solid State Science Series; Cambridge University Press: Cambridge, UK, 1993. [Google Scholar]
- Aryanpour, M.; van Duin, A.C.T.; Kubicki, J.D. Development of a Reactive Force Field for Iron-Oxyhydroxide Systems. J. Phys. Chem. A 2010, 114, 6298–6307. [Google Scholar] [CrossRef] [PubMed]
- Shin, Y.K.; Kwak, H.; Vasenkov, A.V.; Sengupta, D.; van Duin, A.C.T. Development of a ReaxFF Reactive Force Field for Fe/Cr/O/S and Application to Oxidation of Butane over a Pyrite-Covered Cr2O3 Catalyst. ACS Catal. 2015, 5, 7226–7236. [Google Scholar] [CrossRef]
- Huang, Y.; Hu, C.; Xiao, Z.; Gao, N.; Wang, Q.; Liu, Z.; Hu, W.; Deng, H. Atomic insight into iron corrosion exposed to supercritical water environment with an improved Fe-H2O reactive force field. Appl. Surf. Sci. 2022, 580, 152300. [Google Scholar] [CrossRef]
- Sen, F.G.; Qi, Y.; van Duin, A.C.T.; Alpas, A.T. Oxidation induced softening in Al nanowires. Appl. Phys. Lett. 2013, 102, 051912. [Google Scholar] [CrossRef]
- Hong, S.; van Duin, A.C.T. Atomistic-Scale Analysis of Carbon Coating and Its Effect on the Oxidation of Aluminum Nanoparticles by ReaxFF-Molecular Dynamics Simulations. J. Phys. Chem. C 2016, 120, 9464–9474. [Google Scholar] [CrossRef]
- Raman, A.S.; Vojvodic, A. Providing Atomistic Insights into the Dissolution of Rutile Oxides in Electrocatalytic Water Splitting. J. Phys. Chem. C 2022, 126, 922–932. [Google Scholar] [CrossRef]
- Schliemann, R.; Churakov, S.V. Atomic scale mechanism of clay minerals dissolution revealed by ab initio simulations. Geochim. Cosmochim. Acta 2021, 293, 438–460. [Google Scholar] [CrossRef]
- Liu, M.; Ruiz Pestana, L. Mechanisms and energetics of calcium aluminosilicate glass dissolution through ab initio molecular dynamics-metadynamics simulations. NPJ Mater. Degrad. 2024, 8, 1–9. [Google Scholar] [CrossRef]
- Guo, Q.; Pouvreau, M.; Rosso, K.M.; Clark, A.E. Mechanisms of dissolution from gibbsite step edges elucidated by ab initio molecular dynamics with enhanced sampling. Geochim. Cosmochim. Acta 2024, 366, 201–209. [Google Scholar] [CrossRef]
- Tribello, G.A.; Bonomi, M.; Branduardi, D.; Camilloni, C.; Bussi, G. PLUMED 2: New feathers for an old bird. Comput. Phys. Commun. 2014, 185, 604–613. [Google Scholar] [CrossRef]
- Grossfield, A. WHAM—Grossfield Lab. Available online: http://membrane.urmc.rochester.edu/?page_id=126 (accessed on 19 December 2024).
- Lubin, M.I.; Bylaska, E.J.; Weare, J.H. Ab initio molecular dynamics simulations of aluminum ion solvation in water clusters. Chem. Phys. Lett. 2000, 322, 447–453. [Google Scholar] [CrossRef]
- Henkelman, G.; Jónsson, H. Improved tangent estimate in the nudged elastic band method for finding minimum energy paths and saddle points. J. Chem. Phys. 2000, 113, 9978–9985. [Google Scholar] [CrossRef]
- Feng, S.Q.; Zang, H.P.; Wang, Y.Q.; Cheng, X.L.; Yue, J.S. Ab initio investigation of photoinduced non-thermal phase transition in β-cristobalite. Chin. Phys. B 2015, 25, 016701. [Google Scholar] [CrossRef]
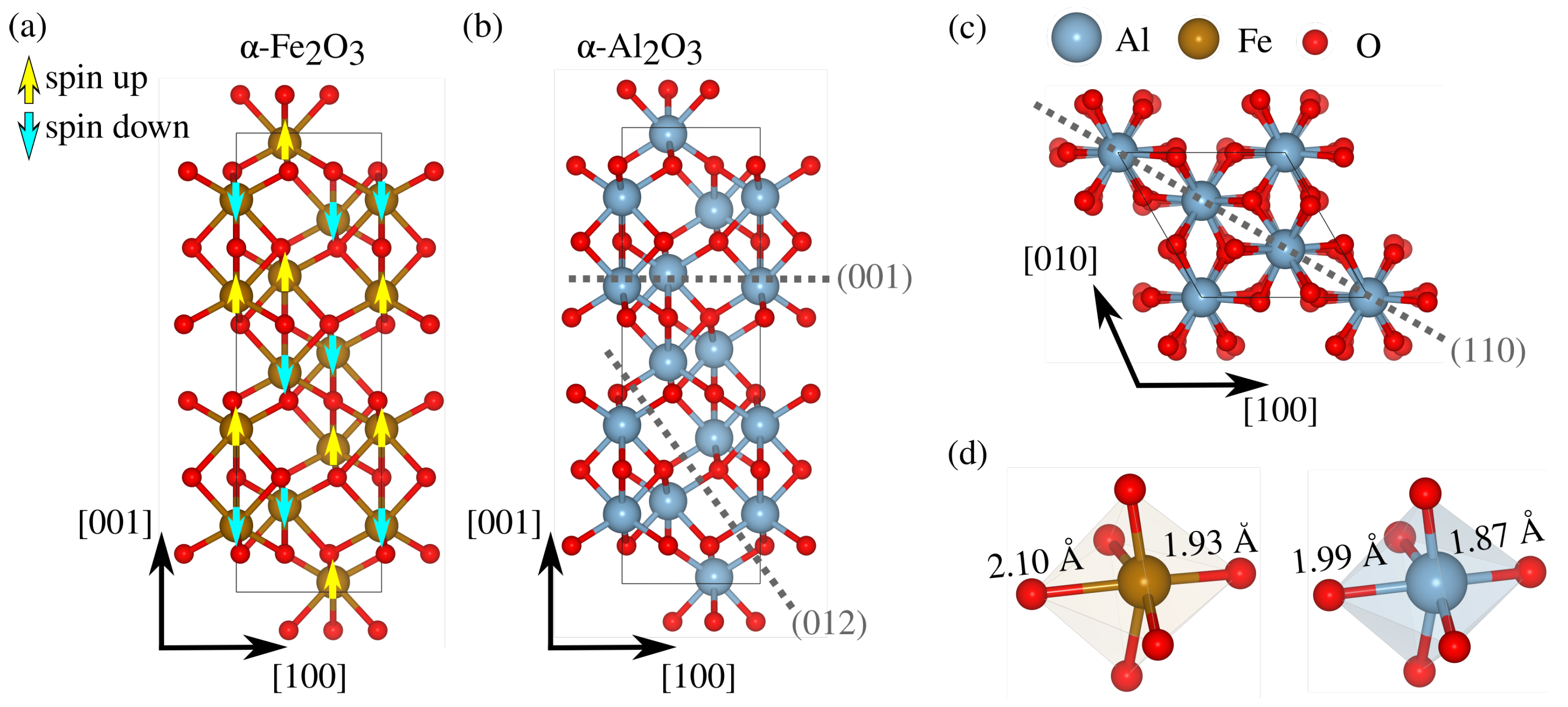
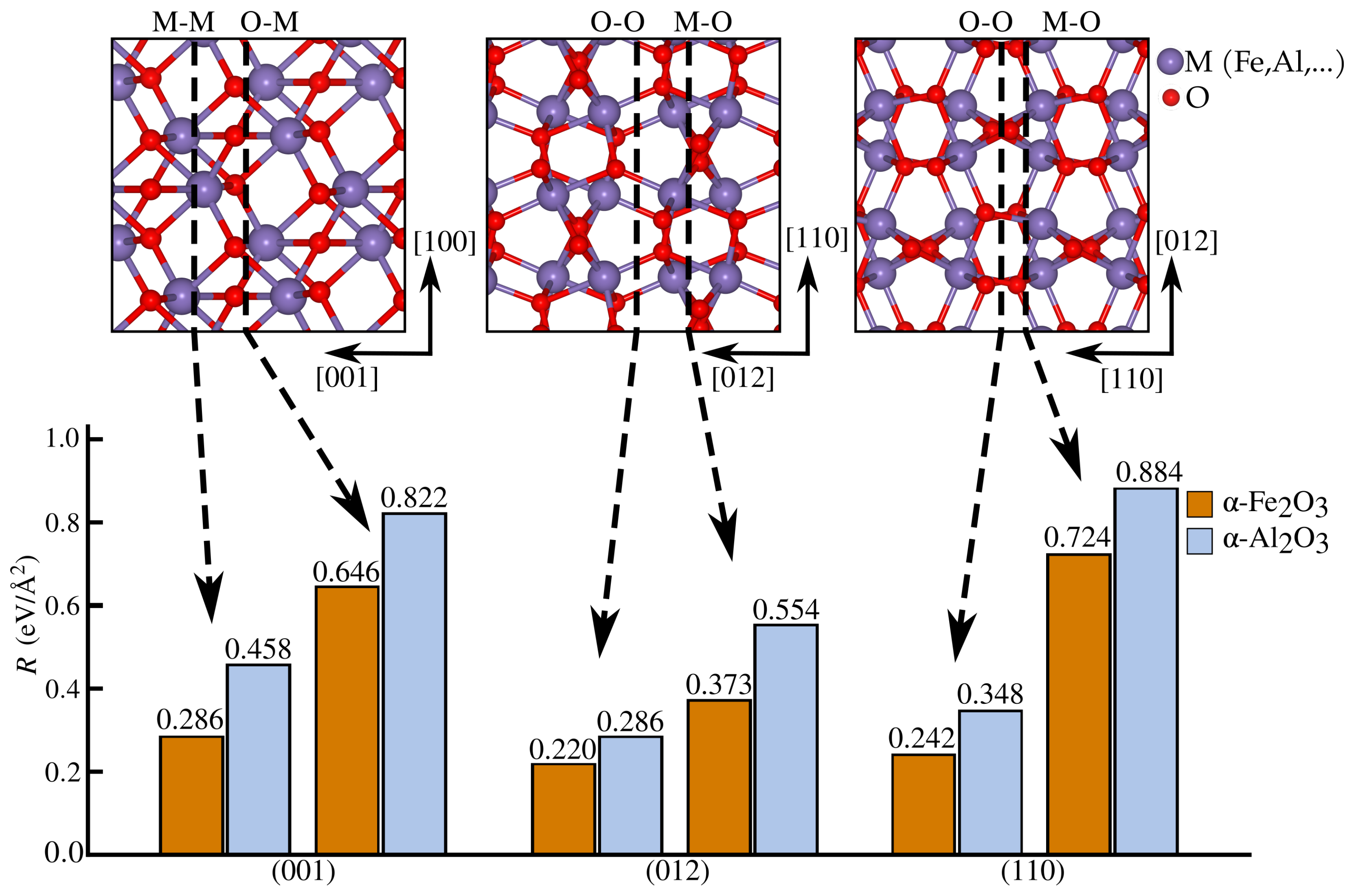
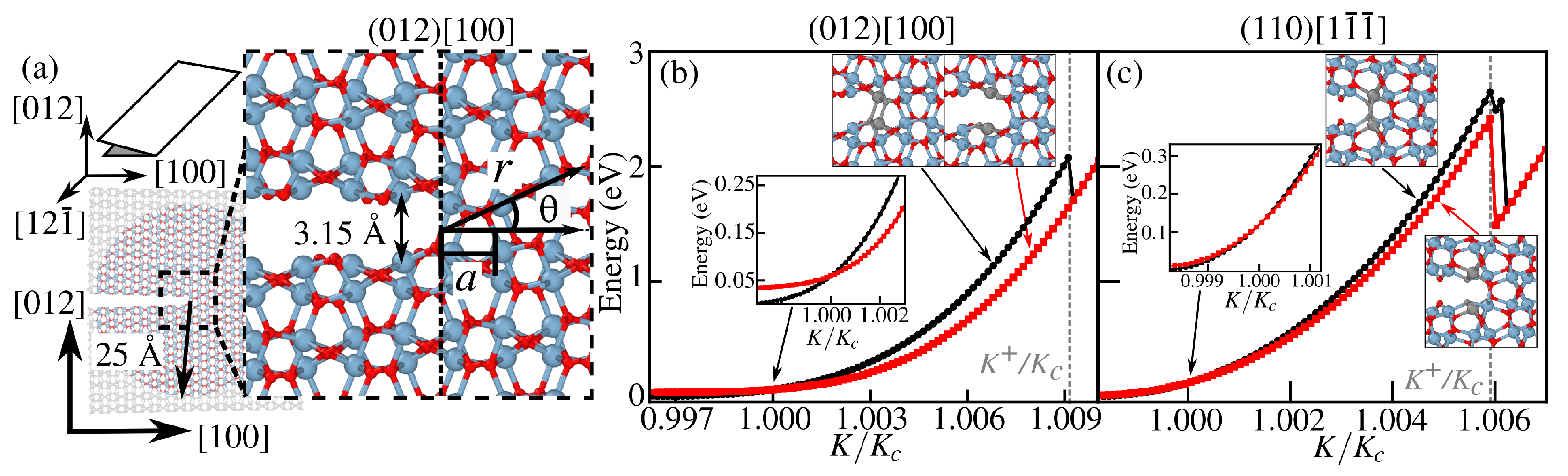
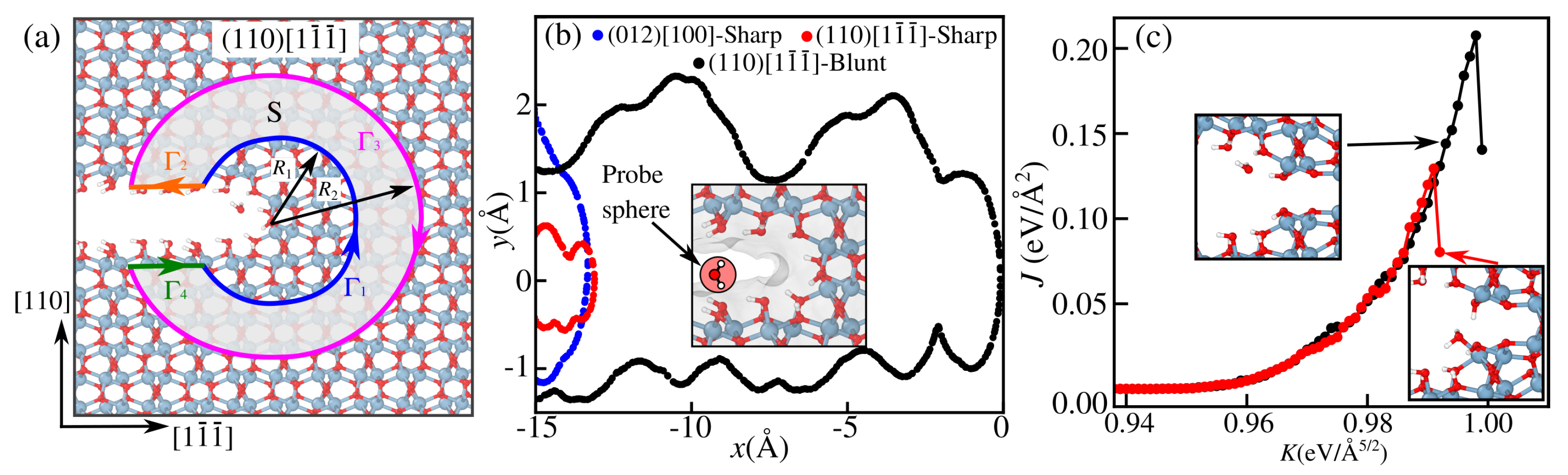

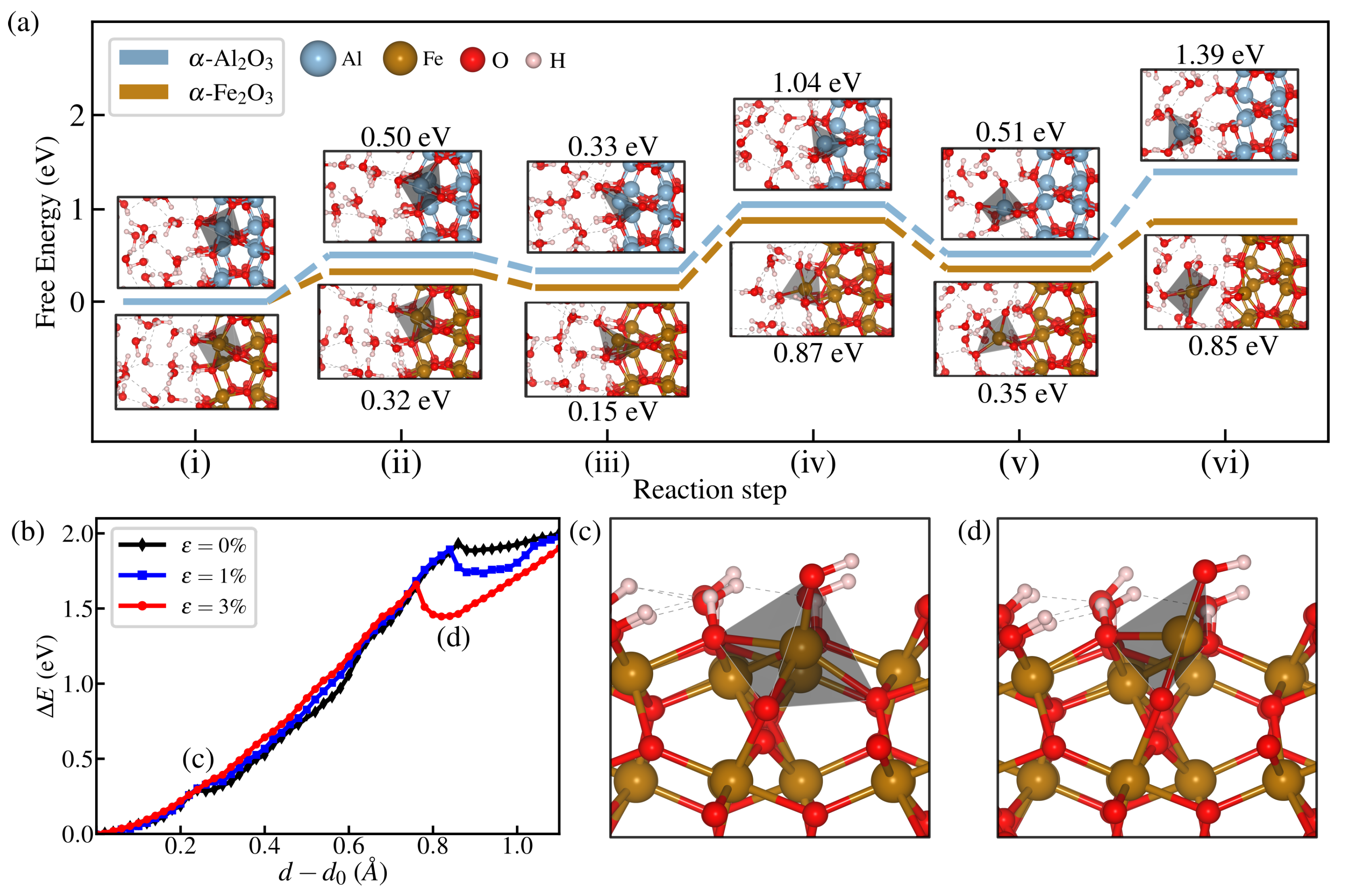
| Fe2O3 | 1.89 | 1.45 | 0.87 | 32.72 |
| Al2O3 | 2.51 | 2.23 | 1.39 | 16.75 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Felix, L.C.; Li, Q.-K.; Penev, E.S.; Yakobson, B.I. Ab Initio Molecular Dynamics Insights into Stress Corrosion Cracking and Dissolution of Metal Oxides. Materials 2025, 18, 538. https://doi.org/10.3390/ma18030538
Felix LC, Li Q-K, Penev ES, Yakobson BI. Ab Initio Molecular Dynamics Insights into Stress Corrosion Cracking and Dissolution of Metal Oxides. Materials. 2025; 18(3):538. https://doi.org/10.3390/ma18030538
Chicago/Turabian StyleFelix, Levi C., Qin-Kun Li, Evgeni S. Penev, and Boris I. Yakobson. 2025. "Ab Initio Molecular Dynamics Insights into Stress Corrosion Cracking and Dissolution of Metal Oxides" Materials 18, no. 3: 538. https://doi.org/10.3390/ma18030538
APA StyleFelix, L. C., Li, Q.-K., Penev, E. S., & Yakobson, B. I. (2025). Ab Initio Molecular Dynamics Insights into Stress Corrosion Cracking and Dissolution of Metal Oxides. Materials, 18(3), 538. https://doi.org/10.3390/ma18030538







