Gas-Thermal Spraying Synthesis of β-Ga2O3 Luminescent Ceramics
Abstract
1. Introduction
2GaO(g) → Ga2O(g) + ½O2(g);
Ga2O(g) → 2Ga(g) + ½O2(g).
2. Materials and Methods
3. Results and Discussion
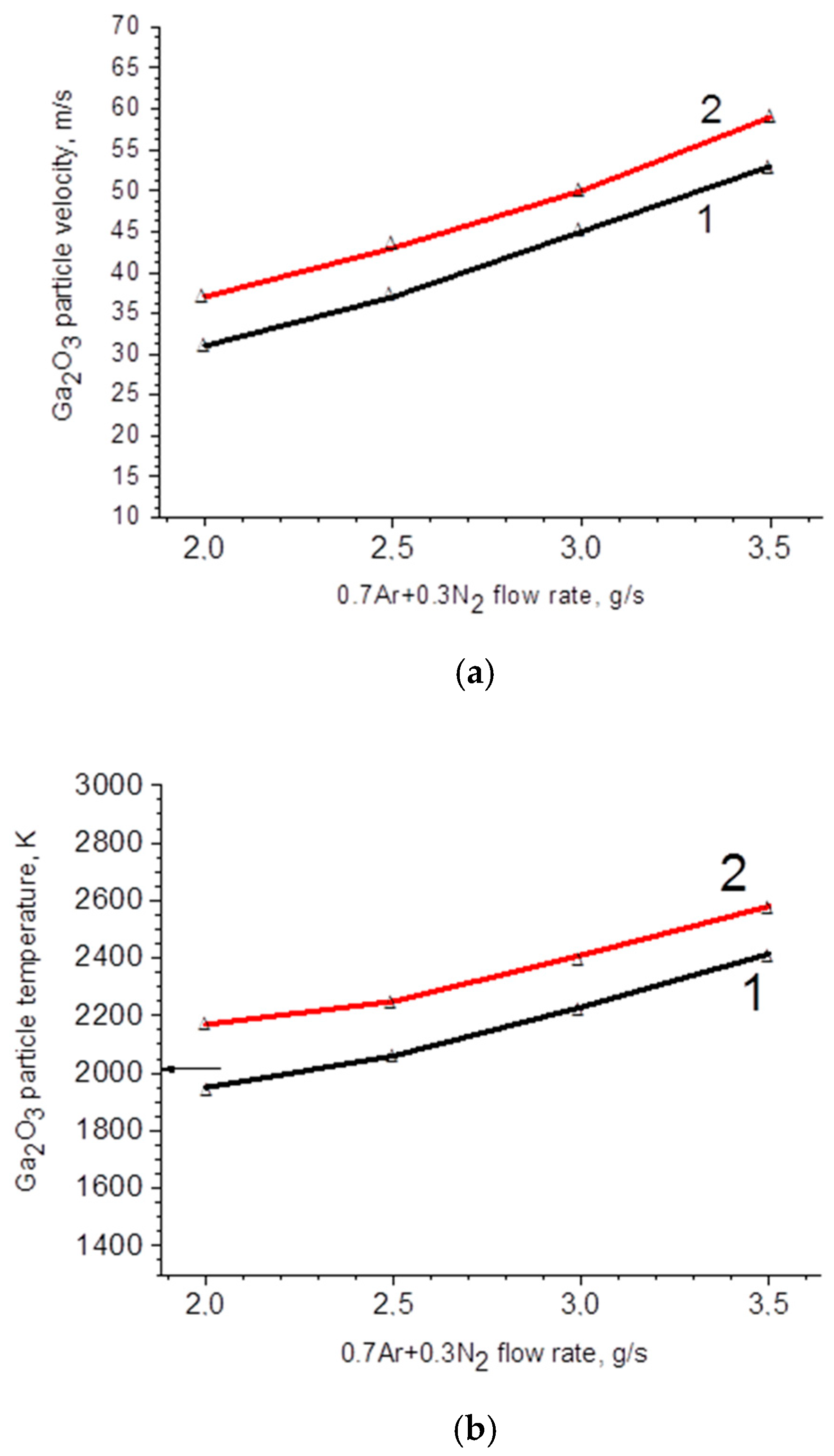
3.1. Theoretical Calculations of Velocity and Heating Temperature of Particles in Plasma
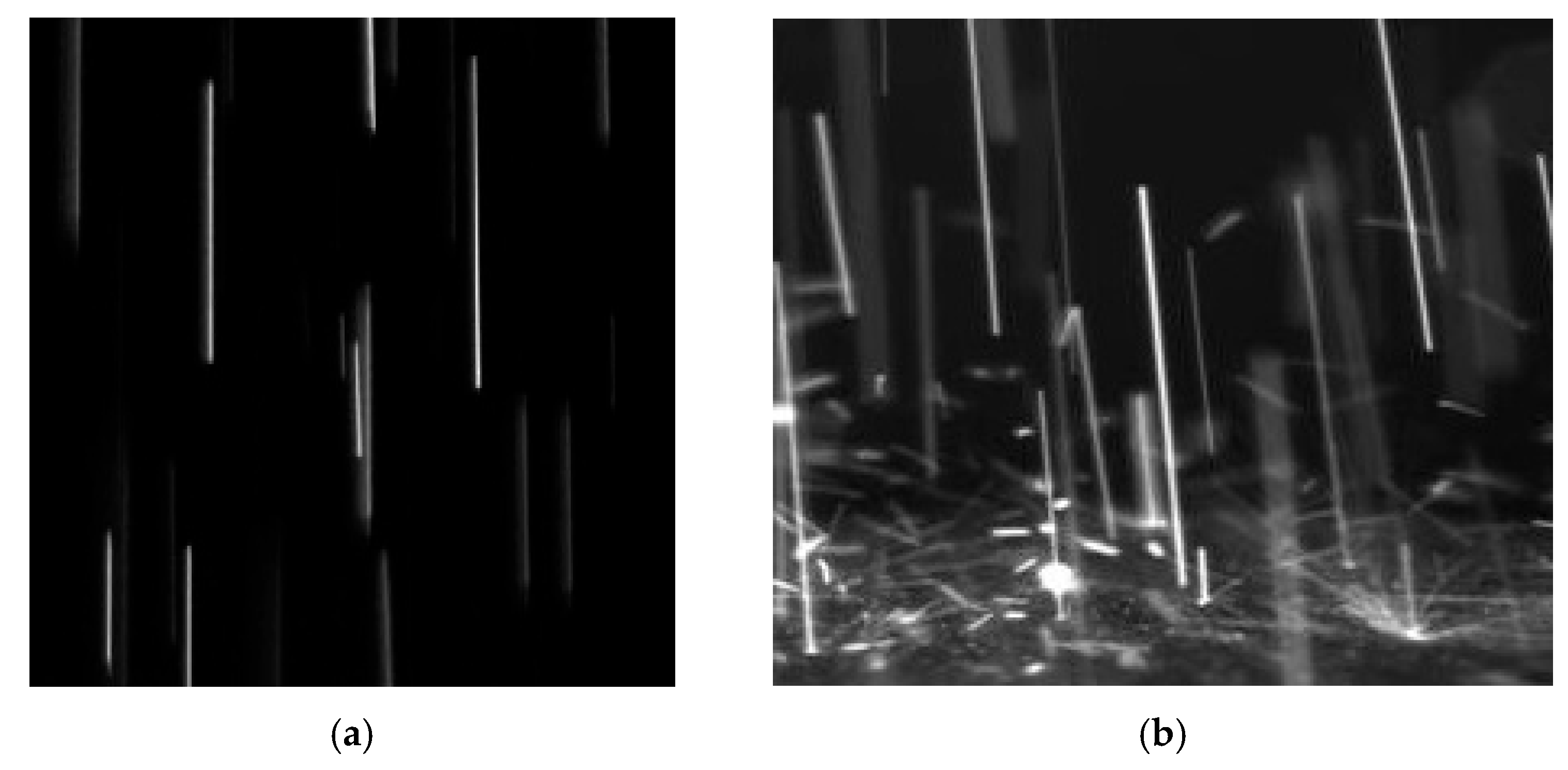
3.2. Experimental Study of Velocity and Temperature of Particles in Plasma
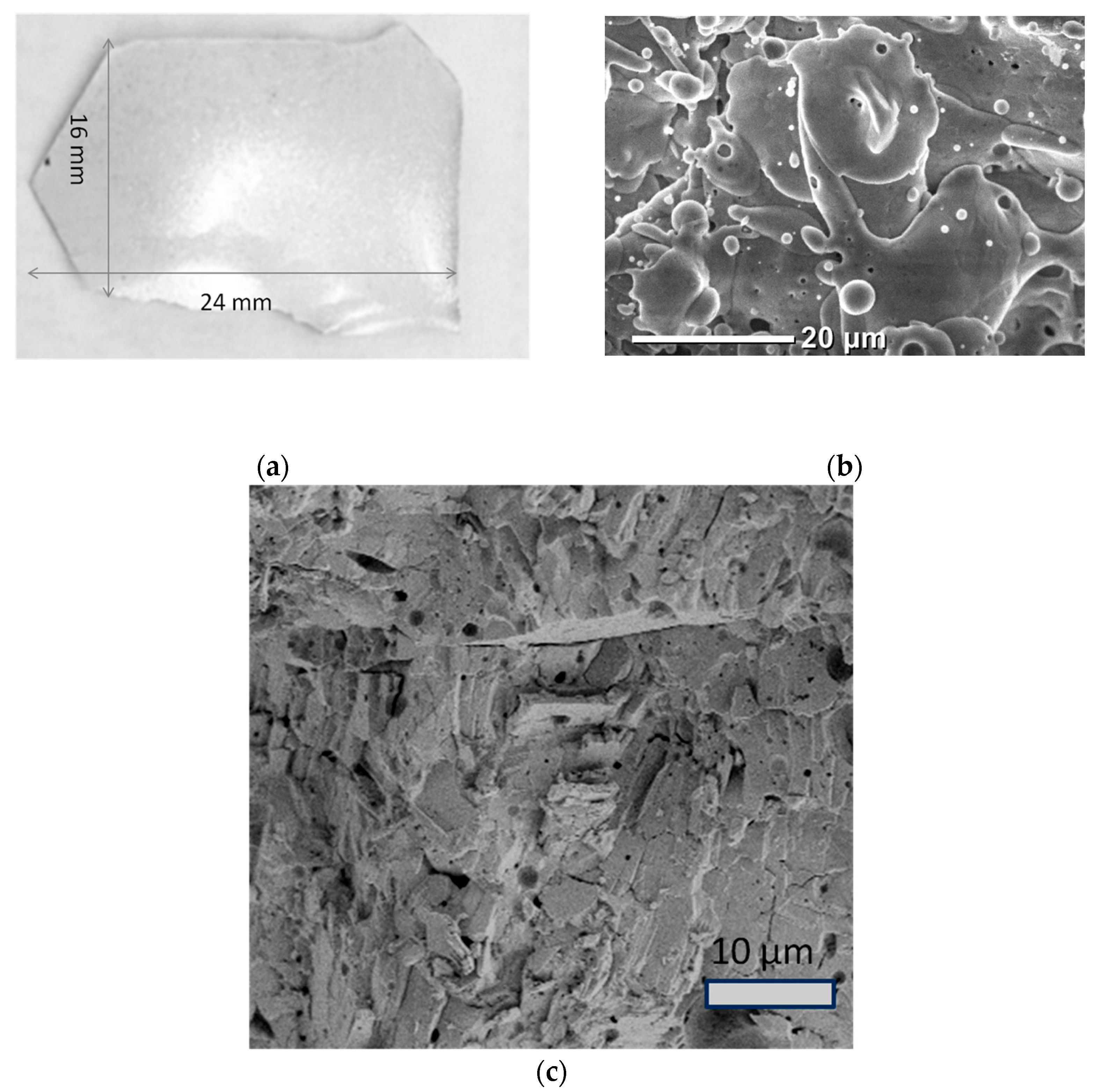
3.3. The Gas-Thermal Synthesis of β-Ga2O3 Ceramics
3.4. Modelling of Gas-Thermal Synthesis Process of β-Ga2O3 Ceramics
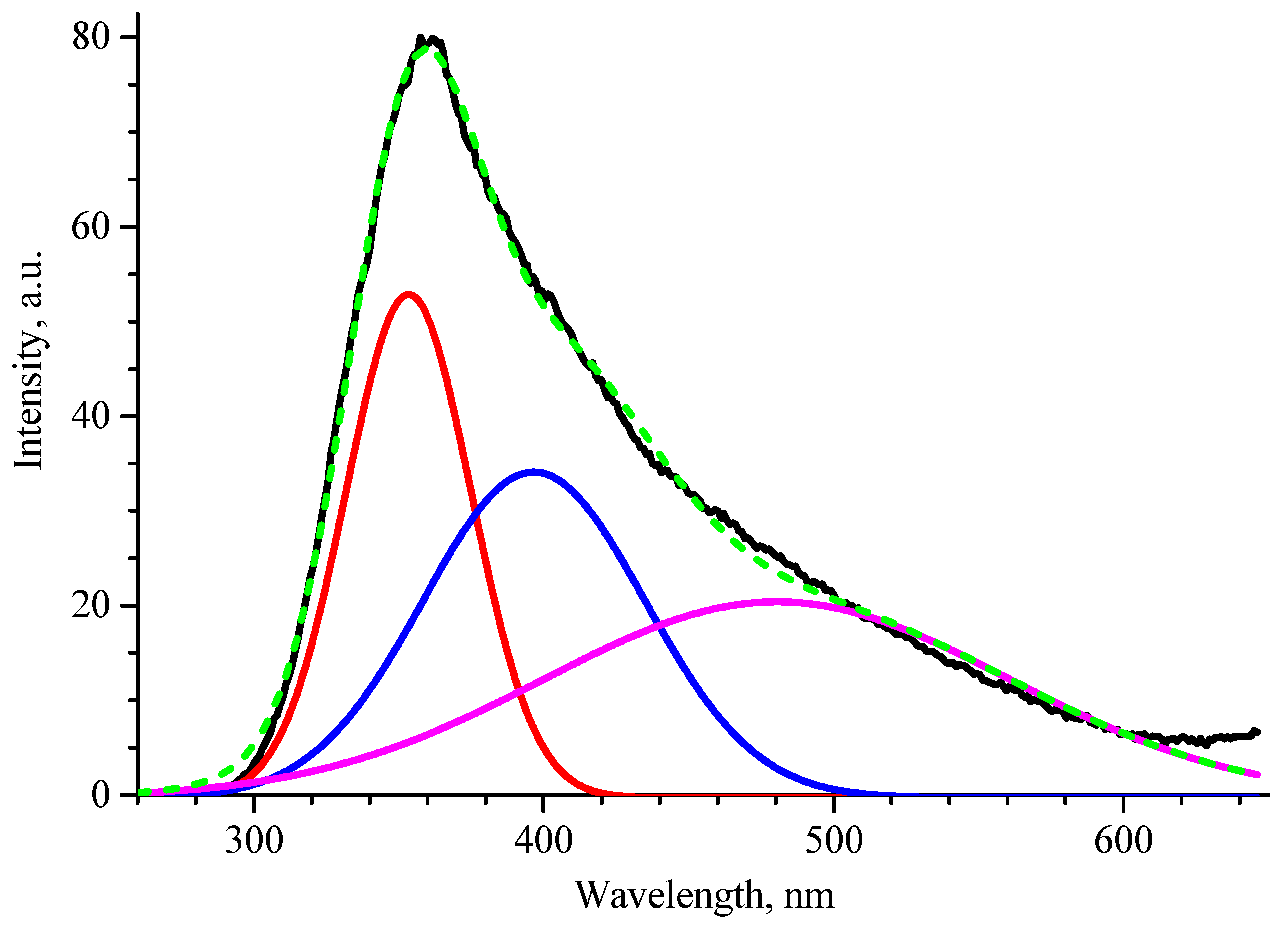
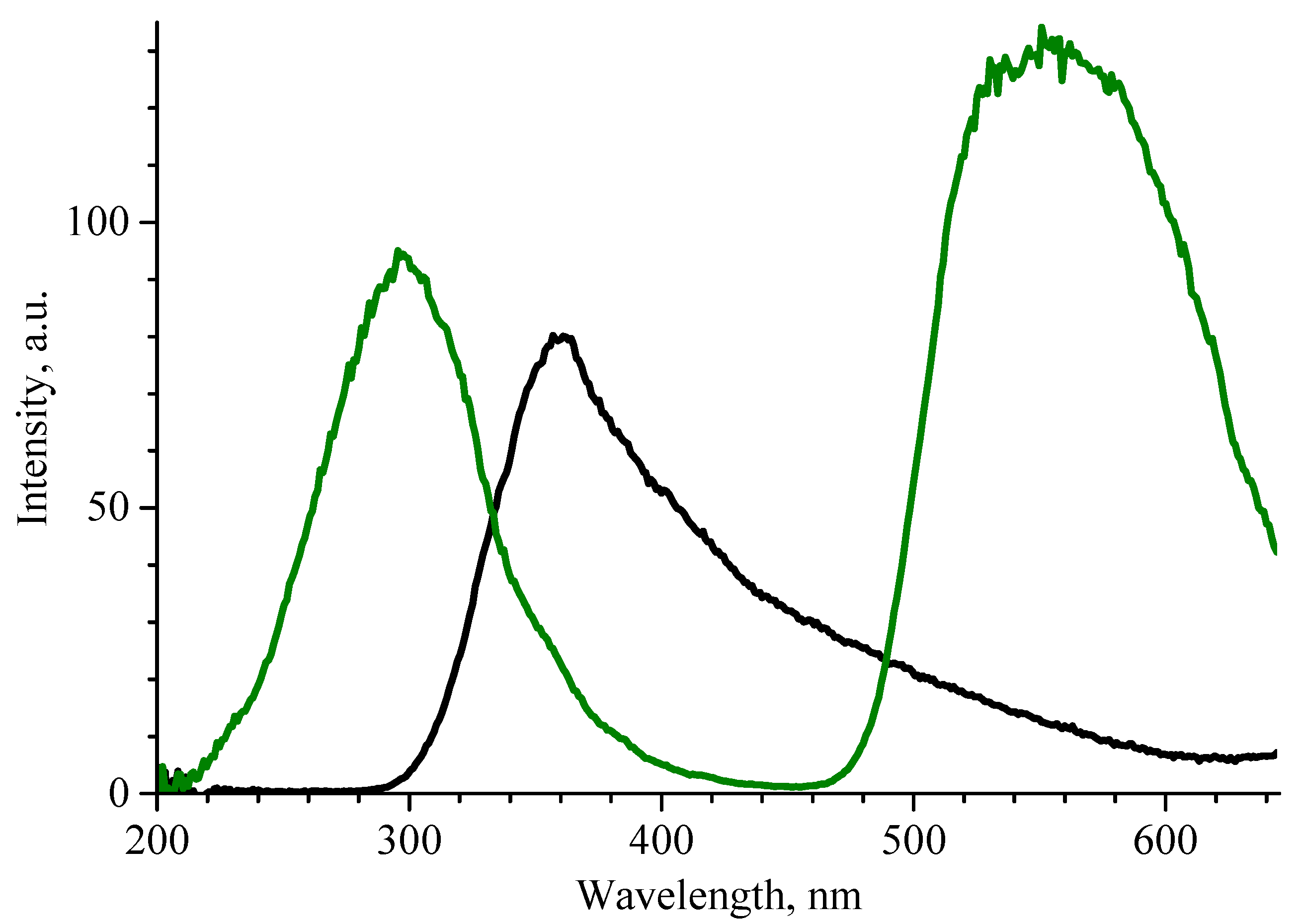
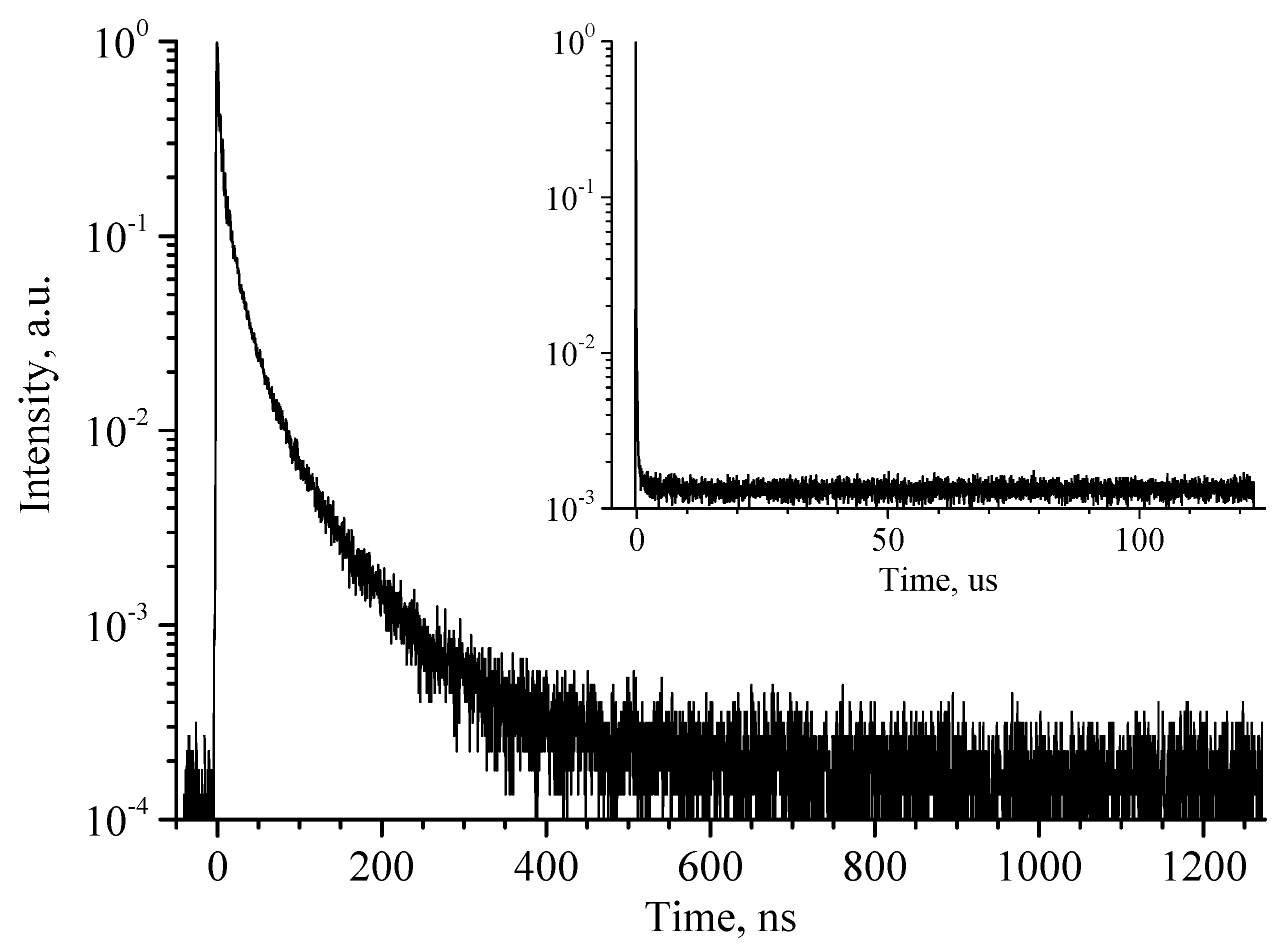
3.5. Radioluminescence Characteristics of β-Ga2O3 Ceramics
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zhang, M.; Liu, Z.; Yang, L.; Yao, J.; Chen, J.; Zhang, J.; Wei, W.; Guo, Y.; Tang, W. β-Ga2O3-Based Power Devices: A Concise Review. Crystals 2022, 12, 406. [Google Scholar] [CrossRef]
- Manikanthababu, N.; Sheoran, H.; Siddham, P.; Singh, R. Review of Radiation-Induced Effects on β-Ga2O3 Materials and Devices. Crystals 2022, 12, 1009. [Google Scholar] [CrossRef]
- Sprincean, V.; Leontie, L.; Caraman, I.; Lupan, O.; Adeling, R.; Gurlui, S.; Carlescu, A.; Doroftei, C.; Caraman, M. Preparation, Chemical Composition, and Optical Properties of (β-Ga2O3 Composite Thin Films)/(GaSxSe1−x Lamellar Solid Solutions) Nanostructures. Nanomaterials 2023, 13, 2052. [Google Scholar] [CrossRef] [PubMed]
- Lee, J.-H.; Doan, T.A.; Park, Y.J.; Hoa, H.T.M.; Phuong, P.H.; Le, D.T.; Hung, N.H.; Tran, Q.T.; Lee, H.-S.; Ryu, J.H.; et al. Synthesis and Photocatalytic Activity of β-Ga2O3 Nanostructures for Decomposition of Formaldehyde under Deep Ultraviolet Irradiation. Catalysts 2020, 10, 1105. [Google Scholar] [CrossRef]
- He, Y.; Zhao, F.; Huang, B.; Zhang, T.; Zhu, H. A Review of β-Ga2O3 Power Diodes. Materials 2024, 17, 1870. [Google Scholar] [CrossRef]
- Yan, Z.; Li, S.; Liu, Z.; Yue, J.; Ji, X.; Wang, J.; Hou, S.; Wu, G.; Lei, J.; Sun, G.; et al. Investigation and Comparison of the Performance for β-Ga2O3 Solar-Blind Photodetectors Grown on Patterned and Flat Sapphire Substrate. Crystals 2024, 14, 625. [Google Scholar] [CrossRef]
- Zhang, C.; Dou, W.; Yang, X.; Zang, H.; Chen, Y.; Fan, W.; Wang, S.; Zhou, W.; Chen, X.; Shan, C. X-ray Detectors Based on Ga2O3 Microwires. Materials 2023, 16, 4742. [Google Scholar] [CrossRef]
- Zhu, J.; Xu, Z.; Ha, S.; Li, D.; Zhang, K.; Zhang, H.; Feng, J. Gallium Oxide for Gas Sensor Applications: A Comprehensive Review. Materials 2022, 15, 7339. [Google Scholar] [CrossRef]
- Hossain, E.; Kulkarni, R.; Mondal, R.; Guddolian, S.; Rahman, A.A.; Thamizhavel, A.; Bhattacharya, A. Optimization of Gas Ambient for High Quality β-Ga2O3 Single Crystals Grown by the Optical Floating Zone Technique. ECS J. Solid State Sci. Technol. 2019, 8, Q3144–Q3148. [Google Scholar] [CrossRef]
- Galazka, Z.; Uecker, R.; Irmscher, K.; Albrecht, M.; Klimm, D.; Pietsch, M.; Fornari, R. Czochralski growth and characterization of β-Ga2O3 single crystals. Cryst. Res. Technol. 2010, 45, 1229–1236. [Google Scholar] [CrossRef]
- Wang, W.; Du, C.; Wang, X.; Xu, D. β-Ga2O3: Ultralow loss and low permittivity dielectric ceramics for high-frequency packaging substrate. Inorg. Chem. Front. 2023, 10, 3723–3729. [Google Scholar] [CrossRef]
- Usseinov, A.B.; Karipbayev, Z.T.; Purans, J.; Kakimov, A.B.; Bakytkyzy, A.; Zhunusbekov, A.M.; Koketai, T.A.; Kozlovskyi, A.L.; Suchikova, Y.; Popov, A.I. Study of β-Ga2O3 Ceramics Synthesized under Powerful Electron Beam. Materials 2023, 16, 6997. [Google Scholar] [CrossRef]
- Prokopov, V.G.; Fialko, N.M.; Sherenkovskaya, G.P. Effect of coating porosity on the process of heat transfer with gas-thermal deposition. Powder Met. Met. Ceram. 1993, 32, 118–121. [Google Scholar] [CrossRef]
- Bogdanovich, V.I.; Giorbelidze, M.G. Metallographic Study of Mesostructure-Ordered Plasma Ceramic Coatings. Key Eng. Mater. 2017, 743, 118–123. [Google Scholar] [CrossRef]
- Huang, Y.; Yu, Q.; Li, M.; Sun, S.; Zhao, H.; Jin, S.; Wang, J. An overview of low-temperature plasma surface modification of carbon materials for removal of pollutants from liquid and gas phases. Plasma Process. Polym. 2021, 18, 3. [Google Scholar] [CrossRef]
- Moszczyńska, J.; Roszek, K.; Wiśniewski, M. Non-Thermal Plasma Application in Medicine—Focus on Reactive Species Involvement. Int. J. Mol. Sci. 2023, 24, 12667. [Google Scholar] [CrossRef]
- Holubová, Ľ.; Kyzek, S.; Ďurovcová, I.; Fabová, J.; Horváthová, E.; Ševčovičová, A.; Gálová, E. Non-Thermal Plasma—A New Green Priming Agent for Plants? Int. J. Mol. Sci. 2020, 21, 9466. [Google Scholar] [CrossRef]
- Muslimov, A.E.; Gadzhiev, M.K.; Kanevsky, V.M. Influence of Plasma Treatment Parameters on the Structural-Phase Composition, Hardness, Moisture-Resistance, and Raman-Enhancement Properties of Nitrogen-Containing Titanium Dioxide. Materials 2022, 15, 8514. [Google Scholar] [CrossRef]
- Muslimov, A.E.; Gadzhiev, M.K.; Kanevsky, V.M. New Approaches to Increasing the Superhydrophobicity of Coatings Based on ZnO and TiO2. Coatings 2021, 11, 1369. [Google Scholar] [CrossRef]
- Kalinichenko, A.S.; Krivosheyev, Y.K.; Meshkova, V.V.; Devoino, O.G. Calculation of Particles Flow Temperature during Plasma Spraying of Mixture Consisting of Self-Fluxing Powder and Ceramics. Sci. Tech. 2018, 17, 177–182. [Google Scholar] [CrossRef]
- Mariaux, G.; Vardelle, A. 3-D time-dependent modelling of the plasma spray process. Part 1: Flow modelling. Int. J. Therm. Sci. 2005, 44, 357–366. [Google Scholar] [CrossRef]
- Son, E.E.; Vetchinin, S.P.; Gadzhiev, M.K.; Yusupov, D.I.; Tyuftyaev, A.S.; Chinnov, V.F.; Sargsyan, M.A. On plasma thermal spraying by the torch with divergent output nozzle. J. Phys. Conf. Ser. 2021, 1787, 012061. [Google Scholar] [CrossRef]
- Butashin, A.V.; Venevtsev, I.D.; Fedorov, V.A.; Muslimov, A.E.; Antonov, E.V.; Deryabin, A.N.; Kvartalov, V.B.; Kanevsky, V.M. Optical and X-ray Spectroscopy of Color Centers in Cerium-Doped Yttrium–Aluminum Garnet. Crystallogr. Rep. 2023, 68, 594–599. [Google Scholar] [CrossRef]
- Rodnyi, P.A.; Mikhrin, S.B.; Mishin, A.N.; Sidorenko, A.V. Small-size pulsed X-ray source for measurements of scintillator decay time constants. IEEE Trans. Nucl. Sci. 2001, 48, 2340–2343. [Google Scholar] [CrossRef]
- Solonenko, O.P.; Gulyaev, P.Y.; Smirnov, A.V. Hydrodynamic features of the impact of a hollow spherical drop on a flat surface. Tech. Phys. Lett. 2009, 35, 885–888. [Google Scholar] [CrossRef]
- Murphy, A.B. Transport coefficients of plasmas in mixtures of nitrogen and hydrogen. Chem. Phys. 2012, 398, 64–72. [Google Scholar] [CrossRef]
- Ahman, J.; Svenssonm, G.; Albertsson, J. A Reinvestigation of β-Gallium Oxide. Acta Crystallogr. Sect. C Cryst. Struct. Commun. 1996, 52, 1336. [Google Scholar] [CrossRef]
- Jamwal, N.S.; Kiani, A. Gallium Oxide Nanostructures: A Review of Synthesis, Properties and Applications. Nanomaterials 2022, 12, 2061. [Google Scholar] [CrossRef] [PubMed]
- Wu, D.; Li, C.; Ma, K.; Wang, J.; Xia, N.; Yan, Y.; Jin, Z.; Zhang, H.; Yang, D. The surface tension of Ga2O3 melt measured by a drop-weight method in an optical floating-zone furnace. Semicond. Sci. Technol. 2023, 38, 8. [Google Scholar] [CrossRef]
- Wang, Y.; Dickens, P.T.; Varley, J.B.; Ni, X.; Lotubai, E.; Sprawls, S.; Liu, F.; Lordi, V.; Krishnamoorthy, S.; Blair, S.; et al. Incident wavelength and polarization dependence of spectral shifts in β-Ga2O3 UV photoluminescence. Sci. Rep. 2018, 8, 18075. [Google Scholar] [CrossRef]
- Cui, H.; Sai, Q.; Qi, H.; Zhao, J.; Si, J.; Pan, M. Analysis on the electronic trap of β-Ga2O3 single crystal. J. Mater. Sci. 2019, 54, 12643–12649. [Google Scholar] [CrossRef]
- Binet, L.; Gourier, D. Origin of the blue luminescence of β-Ga2O3. J. Phys. Chem. Solids 1998, 59, 1241–1249. [Google Scholar] [CrossRef]




| Element | Atom, % | |
|---|---|---|
| Initial powder | O | 60.52 |
| Ga | 39.48 | |
| Ceramics β-Ga2O3 | O | 55.71 |
| Ga | 44.29 |
| Direction | D of Initial Powder, nm | D of Ceramics β-Ga2O3, nm |
|---|---|---|
| [200] | 34.6 | 62.0 |
| [111] | 24.4 | 60.0 |
| [202] | 22.5 | 53.6 |
| [113] | 22.6 | 51.8 |
| Lattice Parameters | Initial Powder | Ceramic β-Ga2O3 | Ga2O3 [27] |
|---|---|---|---|
| a, A | 12.145 | 12.177 | 12.214 |
| b, A | 5.774 | 5.794 | 5.798 |
| c, A | 3.034 | 3.033 | 3.037 |
| β, degree | 103.521 | 103.829 | 103.830 |
| V, A3 | 2.068 | 2.078 | 2.088 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gadzhiev, M.K.; Muslimov, A.E.; Yusupov, D.I.; Il’ichev, M.V.; Kulikov, Y.M.; Chistolinov, A.V.; Venevtsev, I.D.; Volchkov, I.S.; Kanevsky, V.M.; Tyuftyaev, A.S. Gas-Thermal Spraying Synthesis of β-Ga2O3 Luminescent Ceramics. Materials 2024, 17, 6078. https://doi.org/10.3390/ma17246078
Gadzhiev MK, Muslimov AE, Yusupov DI, Il’ichev MV, Kulikov YM, Chistolinov AV, Venevtsev ID, Volchkov IS, Kanevsky VM, Tyuftyaev AS. Gas-Thermal Spraying Synthesis of β-Ga2O3 Luminescent Ceramics. Materials. 2024; 17(24):6078. https://doi.org/10.3390/ma17246078
Chicago/Turabian StyleGadzhiev, Makhach Kh., Arsen E. Muslimov, Damir I. Yusupov, Maksim V. Il’ichev, Yury M. Kulikov, Andrey V. Chistolinov, Ivan D. Venevtsev, Ivan S. Volchkov, Vladimir M. Kanevsky, and Alexander S. Tyuftyaev. 2024. "Gas-Thermal Spraying Synthesis of β-Ga2O3 Luminescent Ceramics" Materials 17, no. 24: 6078. https://doi.org/10.3390/ma17246078
APA StyleGadzhiev, M. K., Muslimov, A. E., Yusupov, D. I., Il’ichev, M. V., Kulikov, Y. M., Chistolinov, A. V., Venevtsev, I. D., Volchkov, I. S., Kanevsky, V. M., & Tyuftyaev, A. S. (2024). Gas-Thermal Spraying Synthesis of β-Ga2O3 Luminescent Ceramics. Materials, 17(24), 6078. https://doi.org/10.3390/ma17246078






