A Study on the Effect of Piezoelectric Nonlinearity on the Bending Behaviour of Smart Laminated Composite Beam
Abstract
1. Introduction
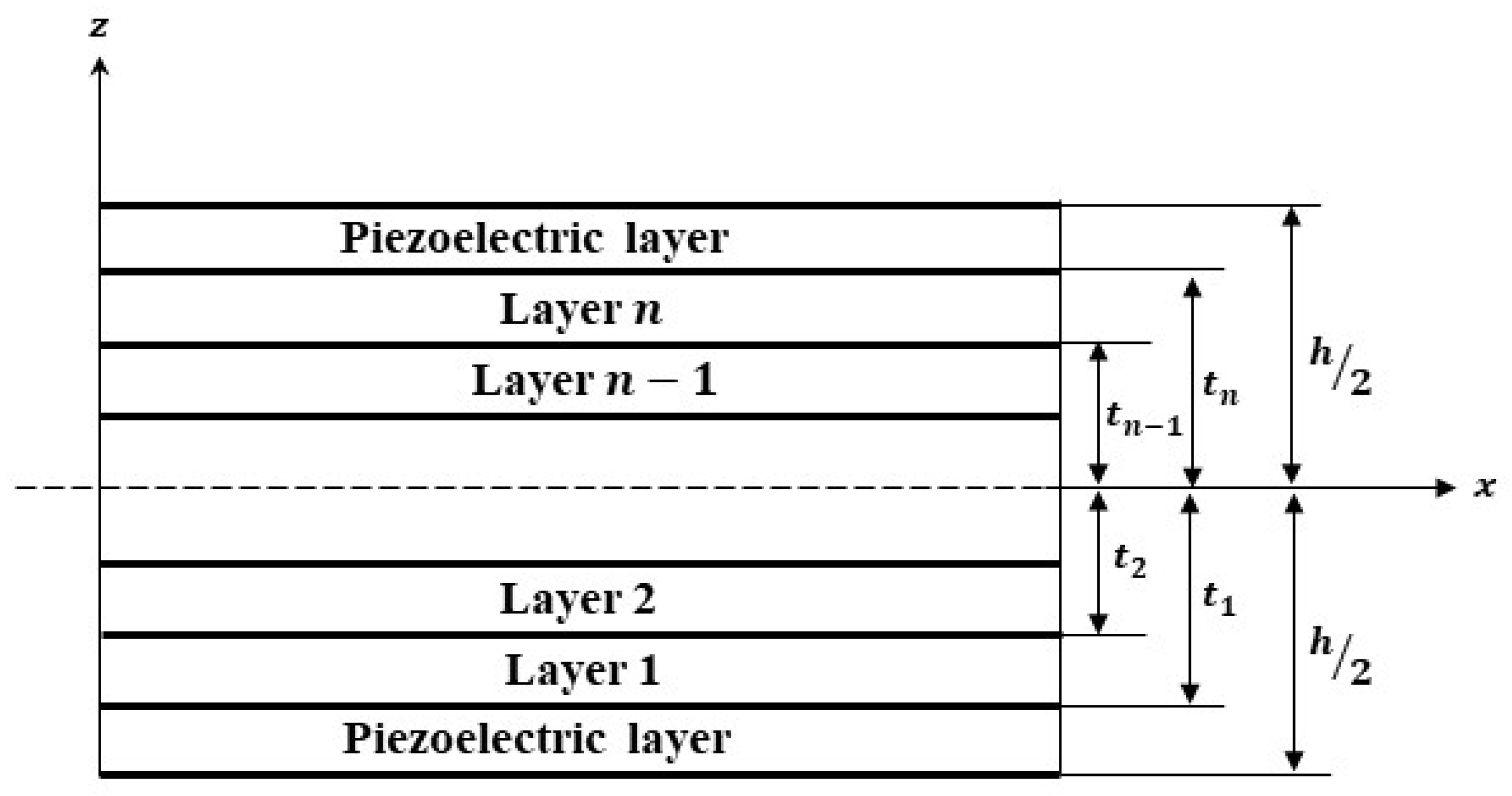
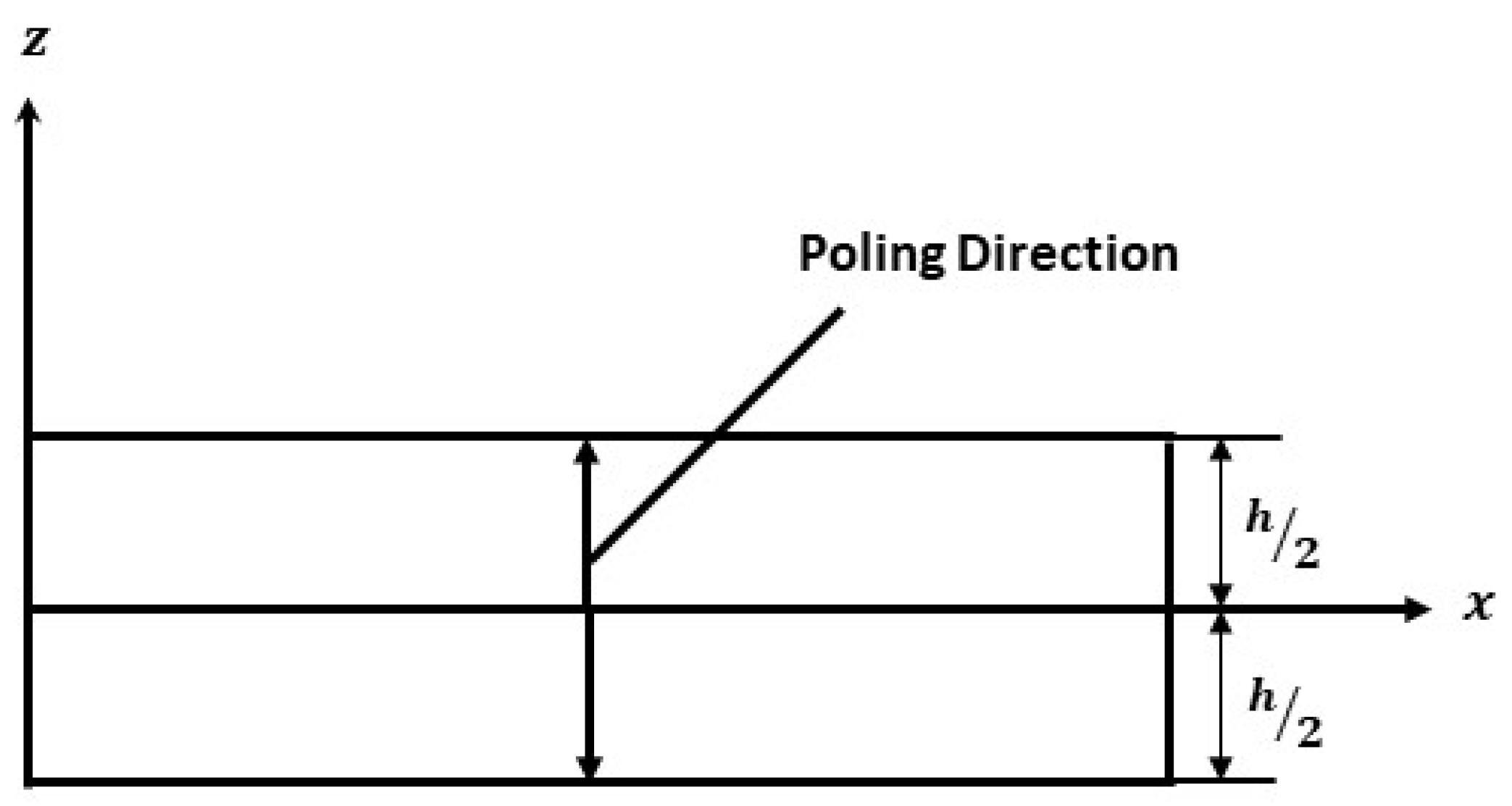
2. Mathematical Formulation
2.1. Theoretical Formulation Using HSDT
2.2. Nonlinear Constitutive Relation
2.3. Energy Formulation
2.4. Finite Element Formulation
2.5. Principle of Virtual Work
3. Results and Discussion
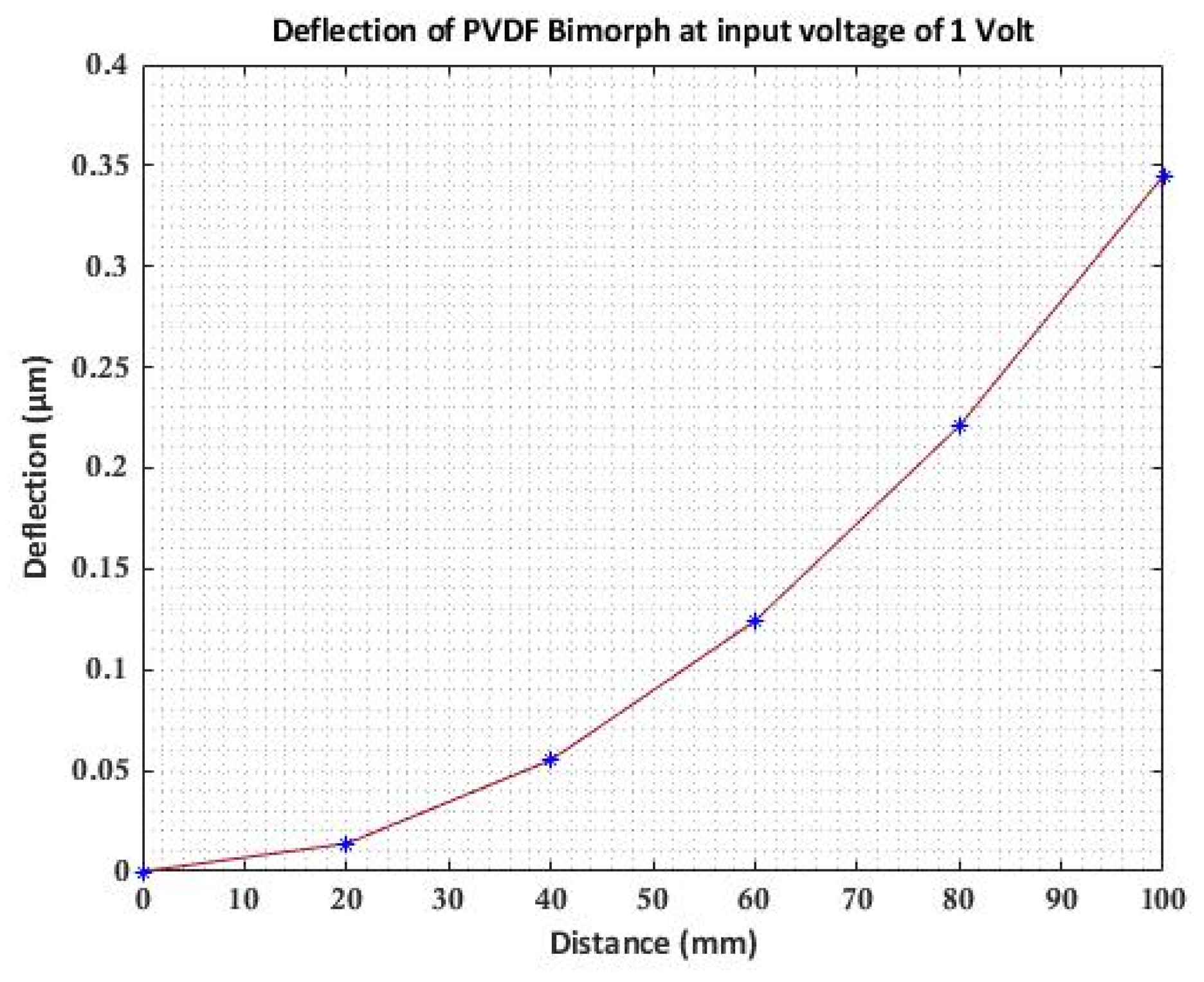
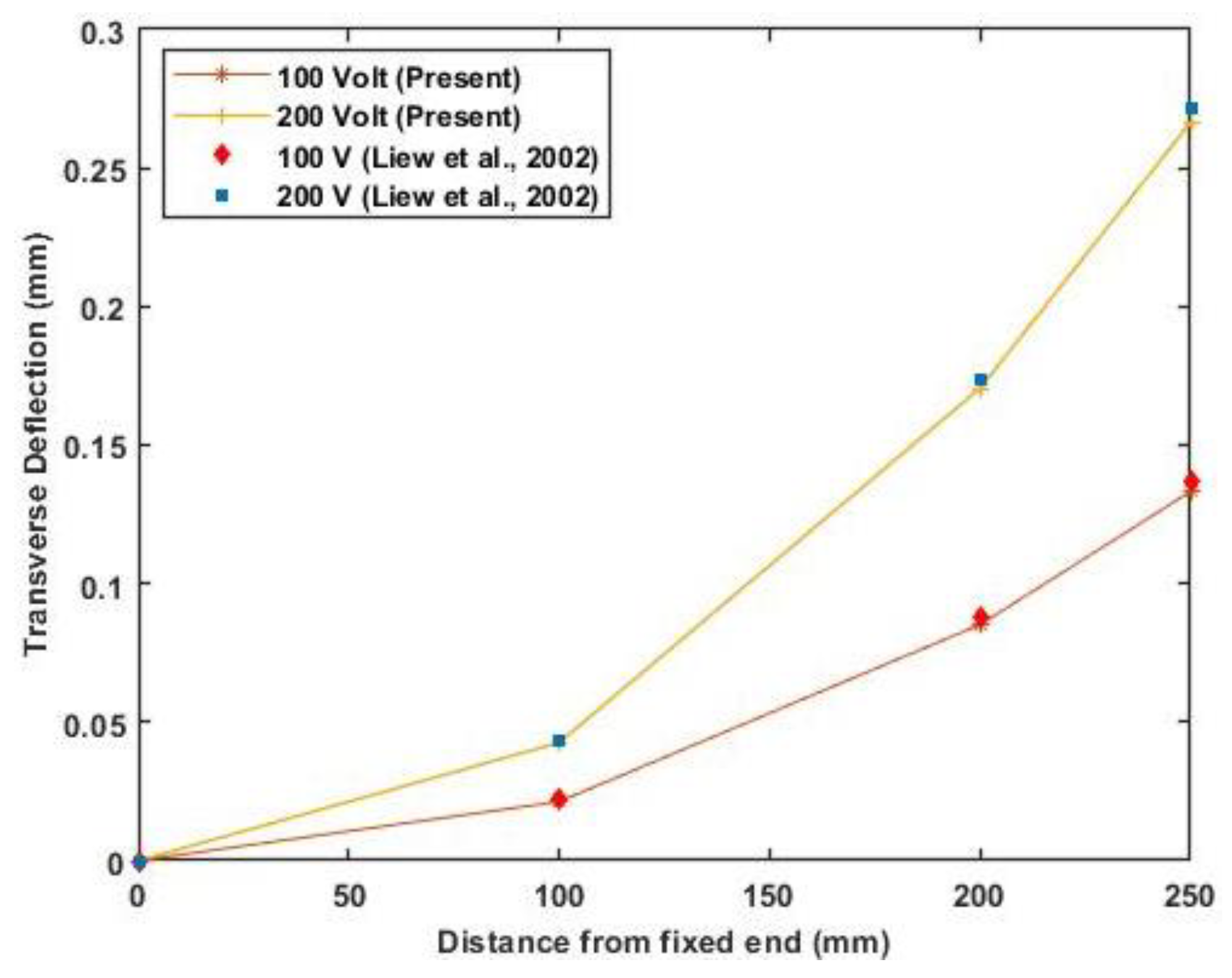
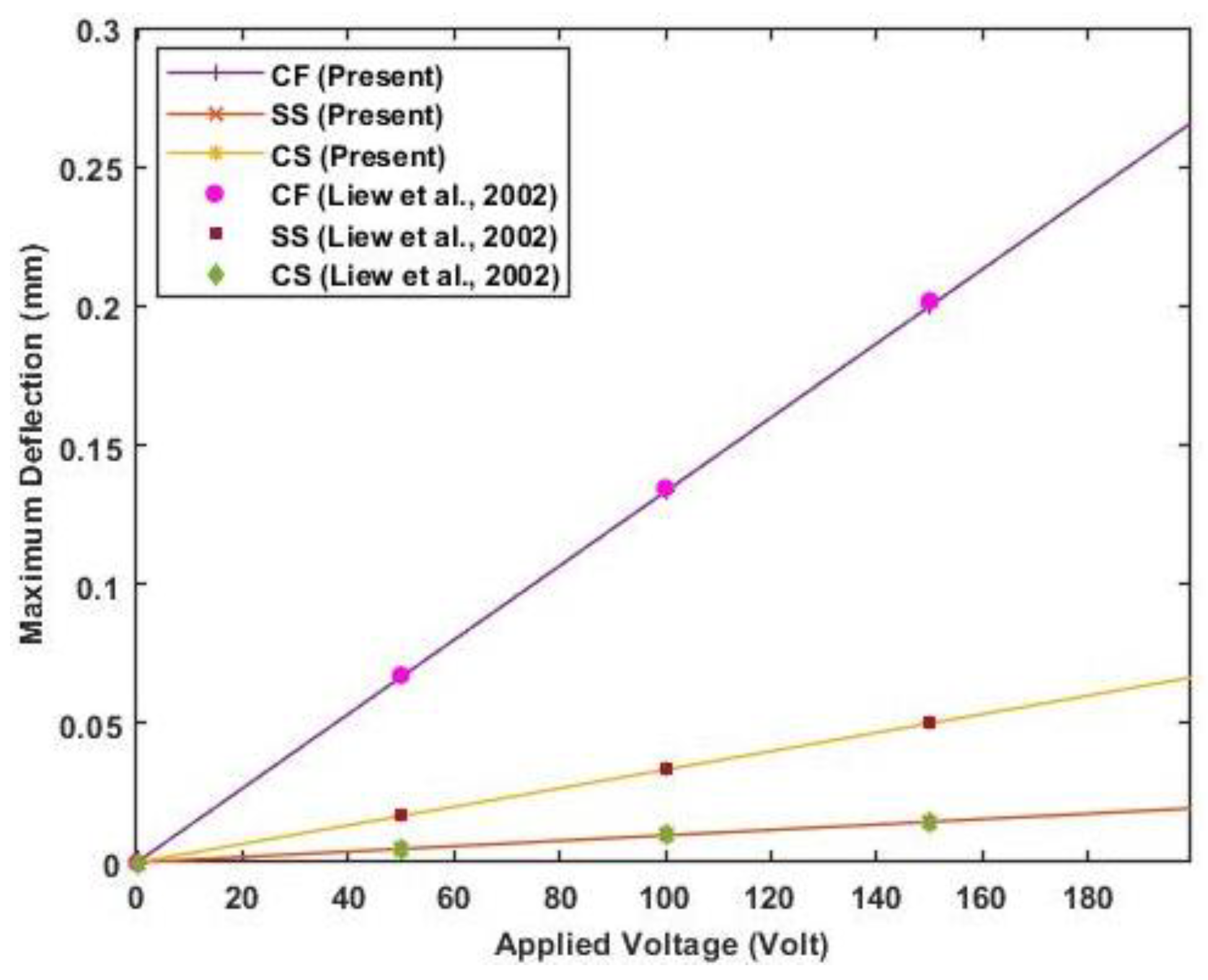
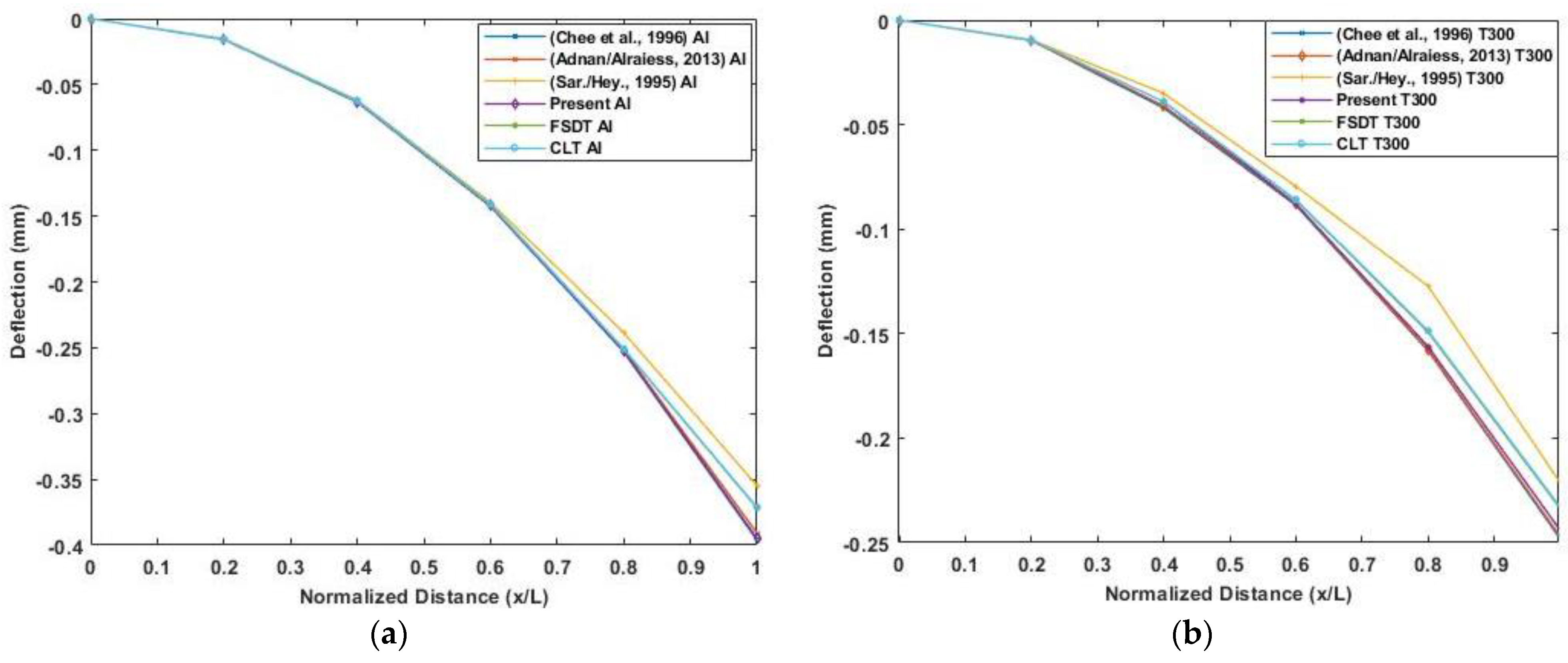
3.1. Validation of Results with Unimorph and Bimorph with Linear Piezoelectric Coefficients
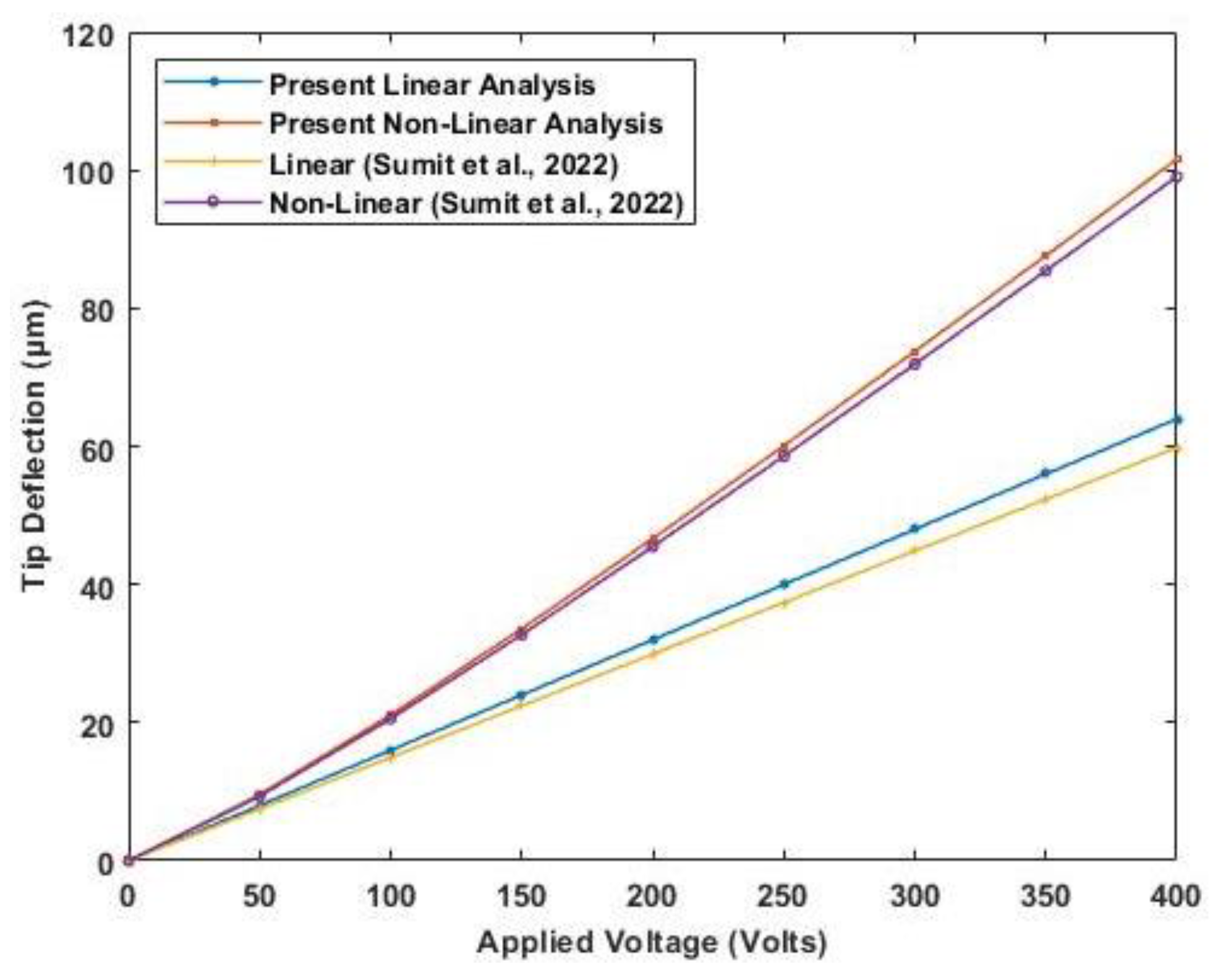
3.2. Validation of Nonlinear Analysis of Piezoelectric Cantilever Bimorph and Unimorph
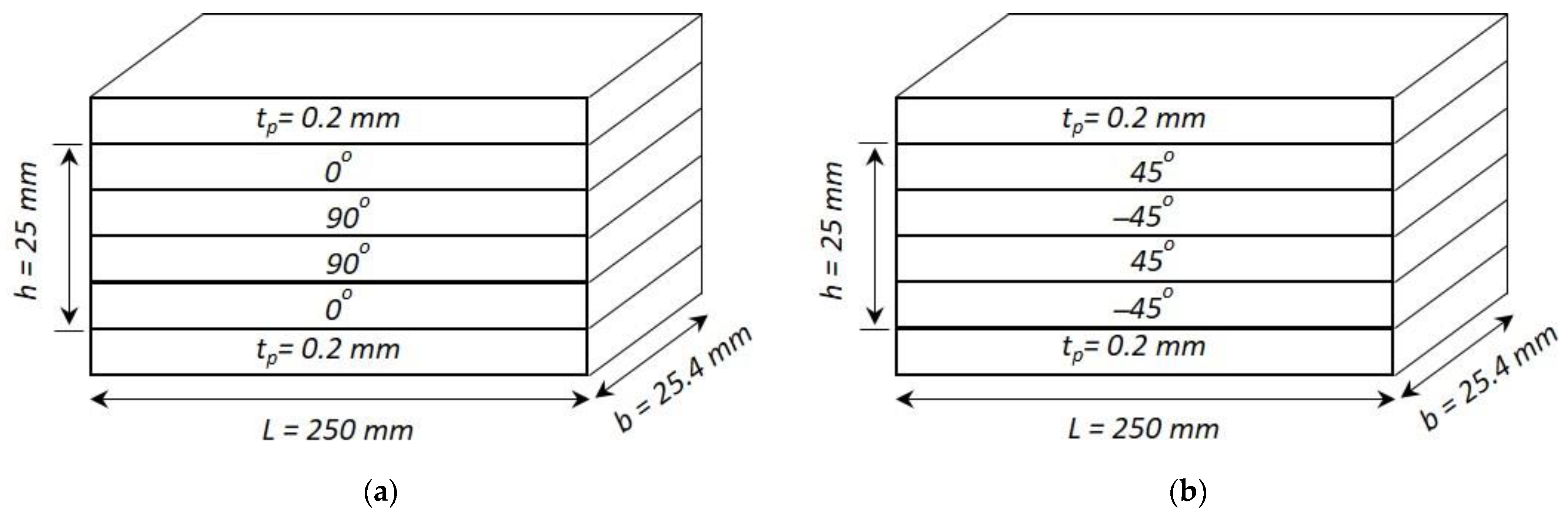
3.3. Nonlinear Analysis of Piezo-Actuated Laminated Composite Beam
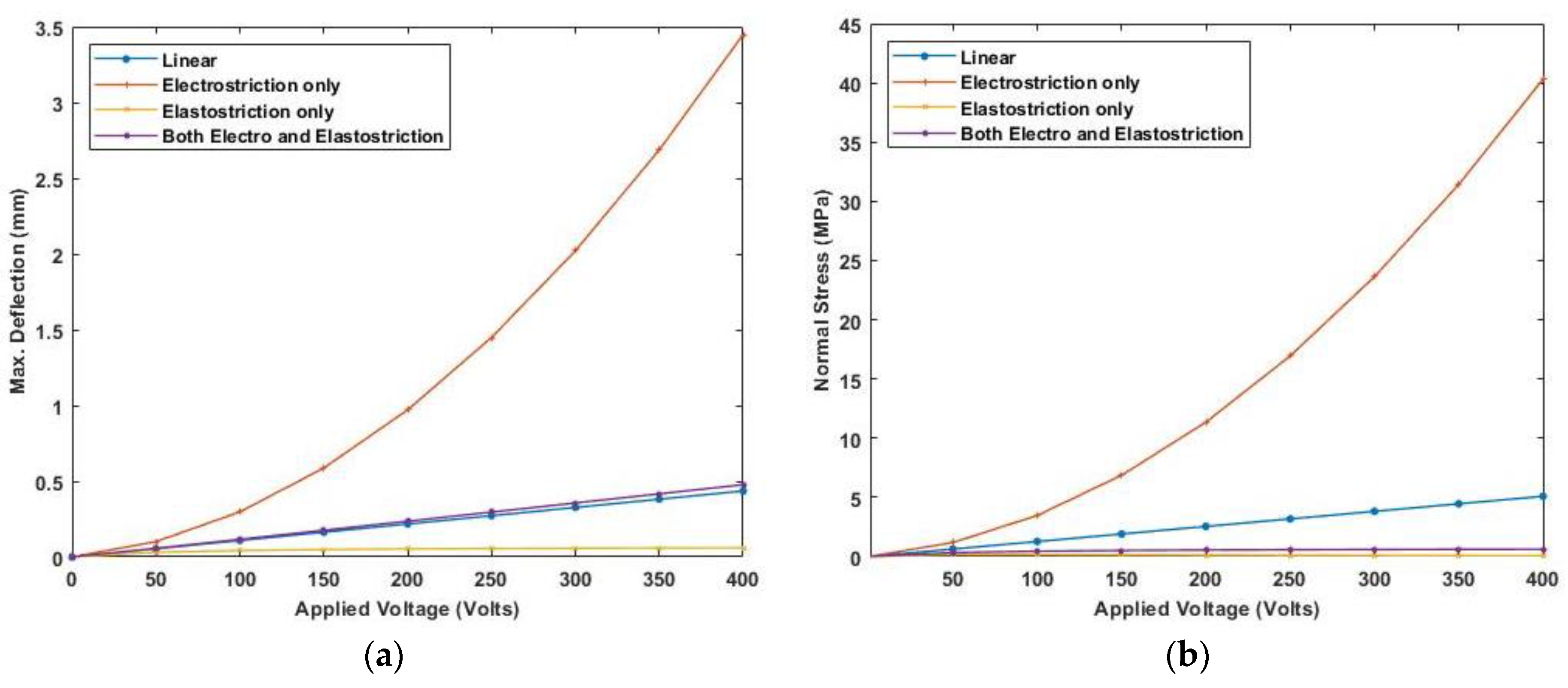
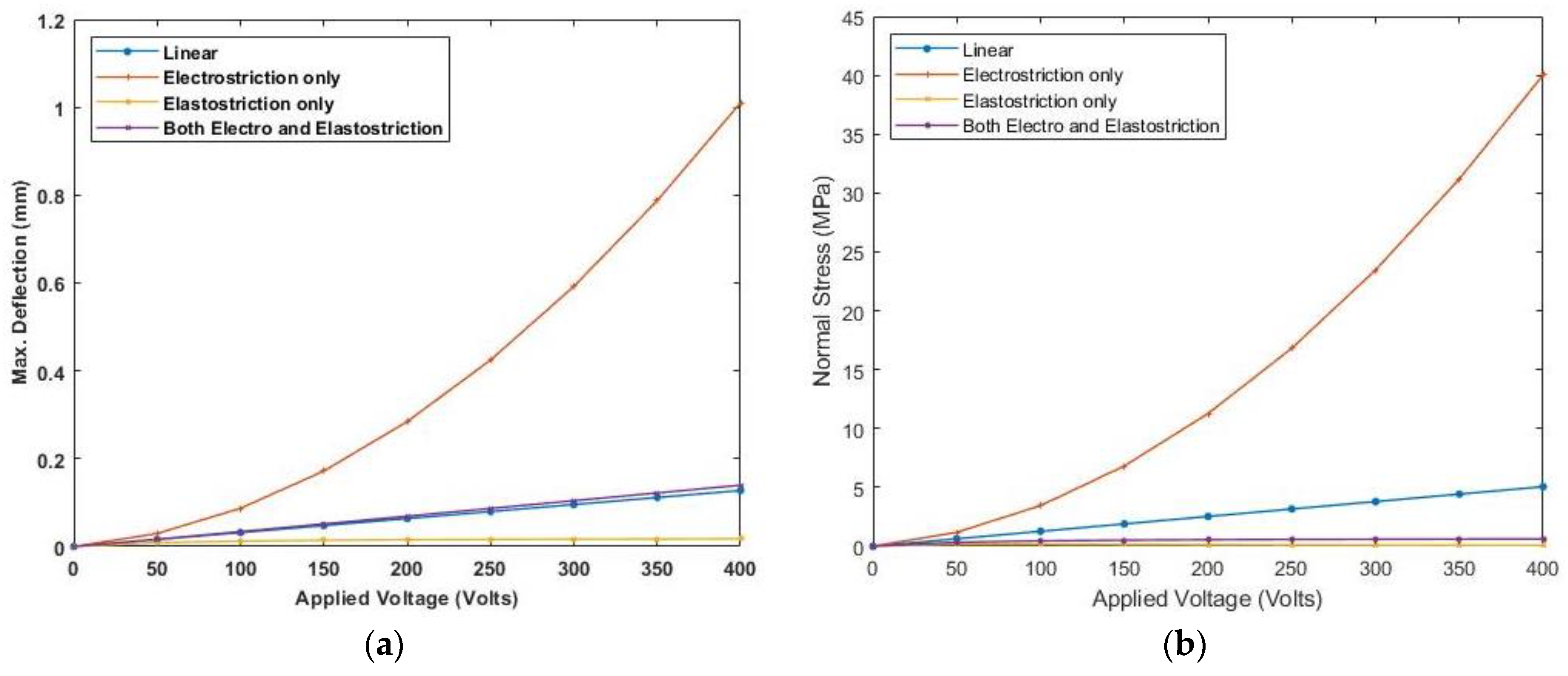
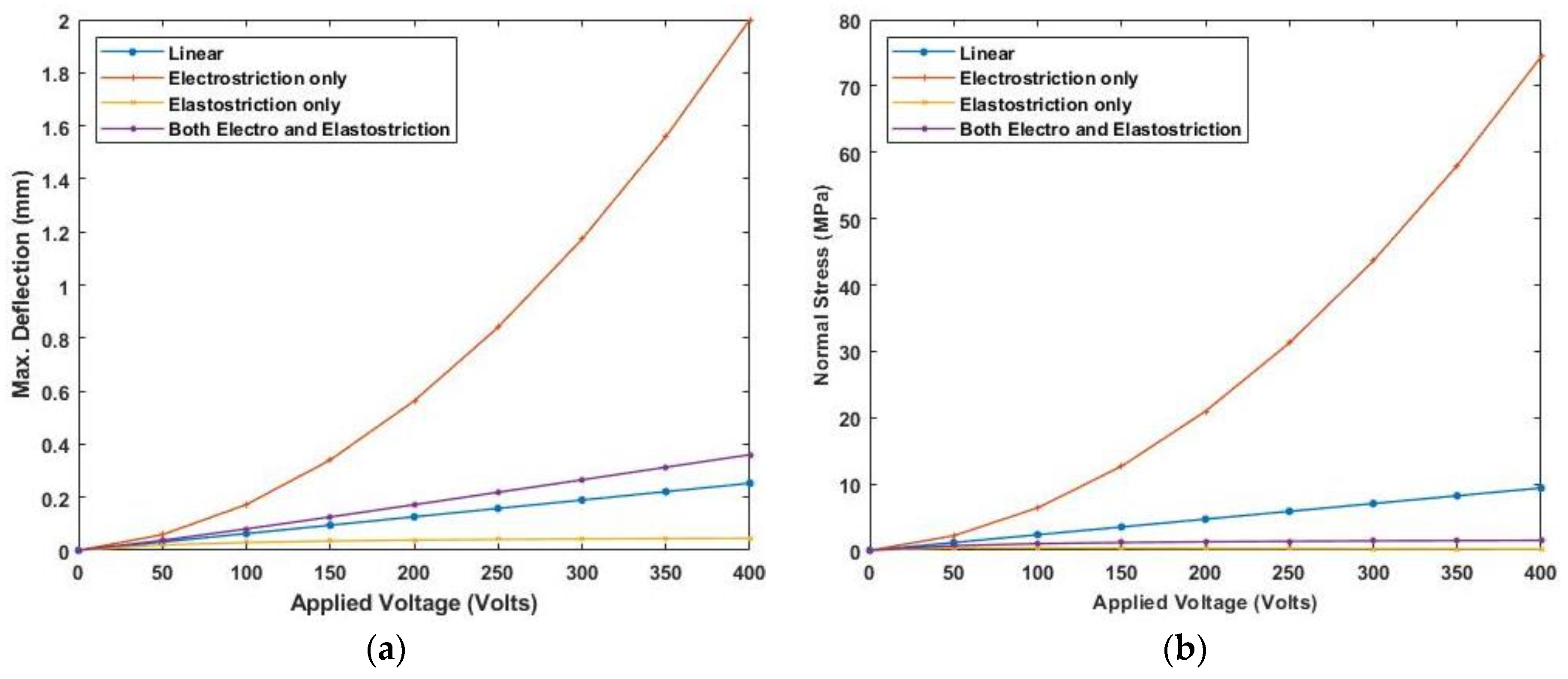
3.4. Analysis of Piezo-Actuated Laminated Composite Beam with Different End Conditions
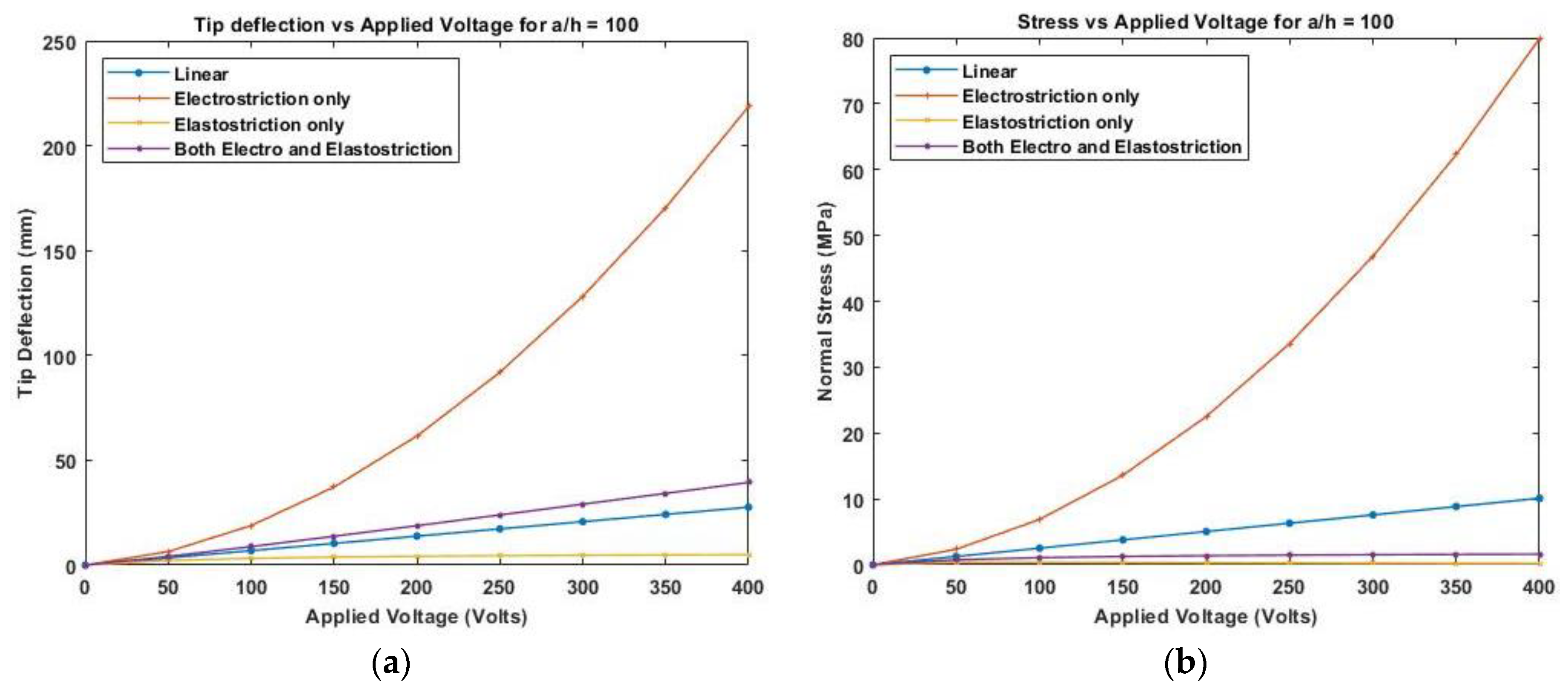
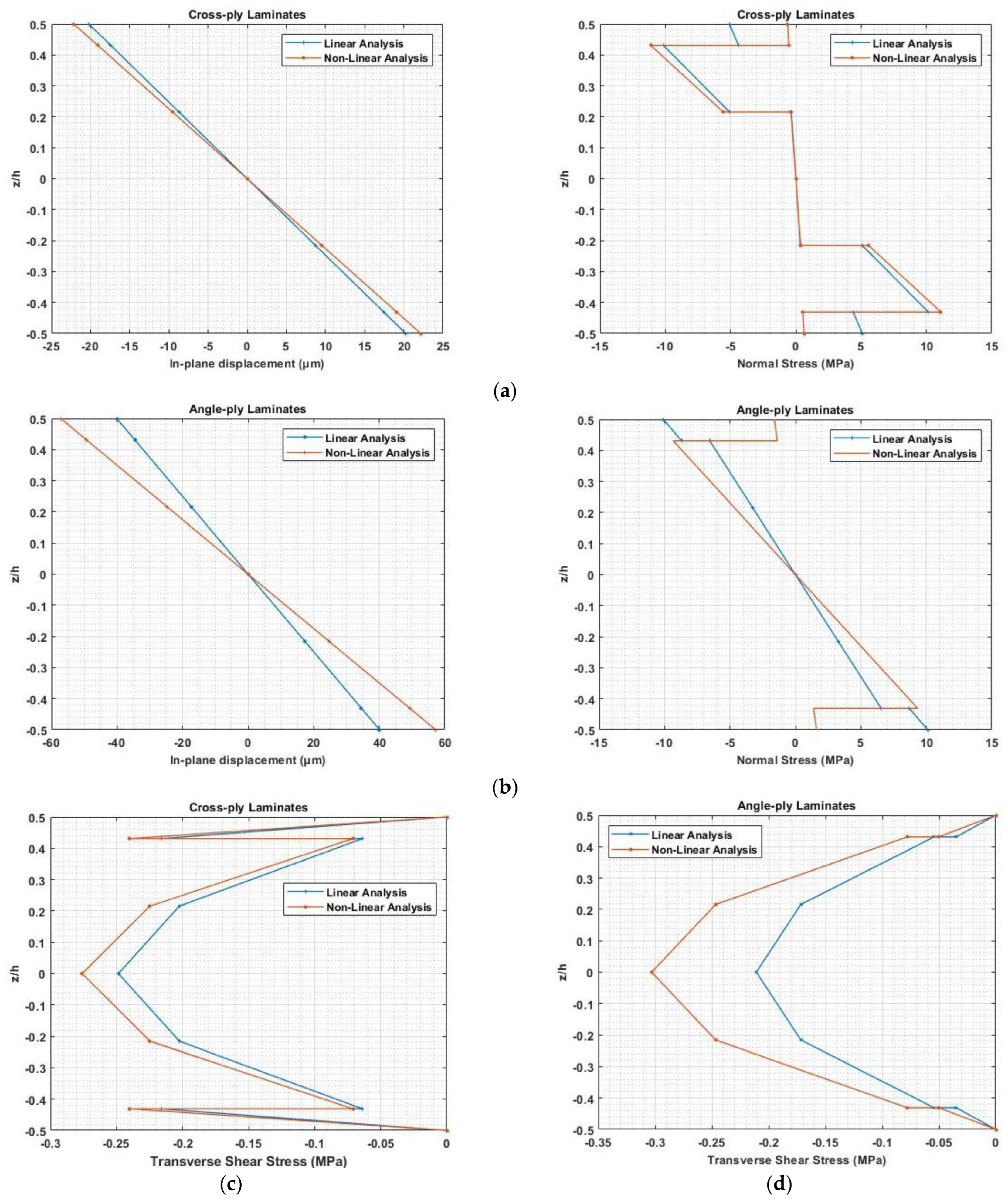
3.5. Nonlinear Analysis for Deflection and Stress Distribution of Composite Laminates
4. Conclusions
Author Contributions
Funding
Informed Consent Statement
Conflicts of Interest
References
- Rao, M.N.; Tarun, S.; Schmidt, R.; Schröder, K.U. Finite Element Modeling and Analysis of Piezo-Integrated Composite Structures under Large Applied Electric Fields. Smart Mater. Struct. 2016, 25, 055044. [Google Scholar] [CrossRef]
- Mallek, H.; Jrad, H.; Wali, M.; Dammak, F. Nonlinear Dynamic Analysis of Piezoelectric-Bonded FG-CNTR Composite Structures Using an Improved FSDT Theory. Eng. Comput. 2021, 37, 1389–1407. [Google Scholar] [CrossRef]
- Carrera, E.; Brischetto, S.; Nali, P. Plates and Shells for Smart Structures; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar] [CrossRef]
- Jiang, J.P.; Li, D.X. A New Finite Element Model for Piezothermoelastic Composite Beam. J. Sound Vib. 2007, 306, 849–864. [Google Scholar] [CrossRef]
- Wischke, M.; Haller, D.; Goldschmidtboeing, F.; Woias, P. Assessing the Elastostriction and the Electrostriction Parameter of Bulk PZT Ceramics. Smart Mater. Struct. 2010, 19, 085003. [Google Scholar] [CrossRef]
- Joshi, S.P. Non-Linear Constitutive Relations for p Iezocera m Ic Materia Is. World 1992, 1, 80. [Google Scholar]
- Tiersten, H.F. Electroelastic Equations for Electroded Thin Plates Subject to Large Driving Voltages. J. Appl. Phys. 1993, 74, 3389–3393. [Google Scholar] [CrossRef]
- Wang, Q.M.; Zhang, Q.; Xu, B.; Liu, R.; Cross, L.E. Nonlinear Piezoelectric Behavior of Ceramic Bending Mode Actuators under Strong Electric Fields. J. Appl. Phys. 1999, 86, 3352–3360. [Google Scholar] [CrossRef]
- Yao, L.Q.; Zhang, J.G.; Lu, L.; Lai, M.O. Nonlinear Static Characteristics of Piezoelectric Bending Actuators under Strong Applied Electric Field. Sens. Actuators A Phys. 2004, 115, 168–175. [Google Scholar] [CrossRef]
- Thornburgh, R.P.; Chattopadhyay, A. Nonlinear Actuation of Smart Composites Using a Coupled Piezoelectric—Mechanical Model. Smart Mater. Struct. 2001, 10, 743–749. [Google Scholar] [CrossRef]
- Kapuria, S.; Yasin, M.Y. A Nonlinear Efficient Layerwise Finite Element Model for Smart Piezolaminated Composites under Strong Applied Electric Field. Smart Mater. Struct. 2013, 22. [Google Scholar] [CrossRef]
- Yaqoob Yasin, M.; Kapuria, S. Influence of Piezoelectric Nonlinearity on Active Vibration Suppression of Smart Laminated Shells Using Strong Field Actuation. J. Vib. Control 2016, 24, 505–526. [Google Scholar] [CrossRef]
- Kapuria, S.; Yasin, M.Y.; Hagedorn, P. Active Vibration Control of Piezolaminated Composite Plates Considering Strong Electric Field Nonlinearity. AIAA J. 2015, 53, 603–616. [Google Scholar] [CrossRef]
- Chattaraj, N.; Ganguli, R. Electromechanical Analysis of Piezoelectric Bimorph Actuator in Static State Considering the Nonlinearity at High Electric Field. Mech. Adv. Mater. Struct. 2016, 23, 802–810. [Google Scholar] [CrossRef]
- Sumit; Kane, S.R.; Sinha, A.K.; Shukla, R. Electric Field-Induced Nonlinear Behavior of Lead Zirconate Titanate Piezoceramic Actuators in Bending Mode. Mech. Adv. Mater. Struct. 2022, 1–10. [Google Scholar] [CrossRef]
- Chattaraj, N.; Ganguli, R. Performance Improvement of a Piezoelectric Bimorph Actuator by Tailoring Geometry. Mech. Adv. Mater. Struct. 2018, 25, 829–835. [Google Scholar] [CrossRef]
- Ahoor, Z.H.; Ghafarirad, H.; Zareinejad, M. Nonlinear Dynamic Modeling of Bimorph Piezoelectric Actuators Using Modified Constitutive Equations Caused by Material Nonlinearity. Mech. Adv. Mater. Struct. 2019, 28, 763–773. [Google Scholar] [CrossRef]
- Zhang, S.Q.; Zhao, G.Z.; Zhang, S.Y.; Schmidt, R.; Qin, X.S. Geometrically Nonlinear FE Analysis of Piezoelectric Laminated Composite Structures under Strong Driving Electric Field. Compos. Struct. 2017, 181, 112–120. [Google Scholar] [CrossRef]
- Koutsawa, Y.; Giunta, G.; Belouettar, S. Hierarchical FEM Modelling of Piezo-Electric Beam Structures. Compos. Struct. 2013, 95, 705–718. [Google Scholar] [CrossRef]
- Koutsawa, Y.; Giunta, G.; Belouettar, S. A Free Vibration Analysis of Piezo-Electric Beams via Hierarchical One-Dimensional Finite Elements. J. Intell. Mater. Syst. Struct. 2014, 25, 1009–1023. [Google Scholar] [CrossRef]
- Reddy, J.N. A Simple Higher-Order Theory for Laminated Composite Plates. J. Appl. Mech. Trans. ASME 1984, 51, 745–752. [Google Scholar] [CrossRef]
- Adnan Elshafei, M.; Alraiess, F. Modeling and Analysis of Smart Piezoelectric Beams Using Simple Higher Order Shear Deformation Theory. Smart Mater. Struct. 2013, 22, 035006. [Google Scholar] [CrossRef]
- Hwang, W.; Park, H.C. Finite Element Modeling of Piezoelectric Sensors and Actuators. AIAA J. 1993, 31, 930–937. [Google Scholar] [CrossRef]
- Tzou, H.S.; Ye, R. Analysis of Piezoelastic Structures with Laminated Piezoelectric Triangle Shell Elements. AIAA J. 1996, 34, 110–115. [Google Scholar] [CrossRef]
- Liew, K.M.; Lim, H.K.; Tan, M.J.; He, X.Q. Analysis of Laminated Composite Beams and Plates with Piezoelectric Patches Using the Element-Free Galerkin Method. Comput. Mech. 2002, 29, 486–497. [Google Scholar] [CrossRef]
- Chee, C.Y.K.; Tong, L.; Steven, G.P. Mixed Model for Composite Beams with Piezoelectric Actuators and Sensors. Smart Mater. Struct. 1999, 8, 417–432. [Google Scholar] [CrossRef]
- Saravanos, D.A.; Heyliger, P.R. Coupled Layerwise Analysis of Composite Beams with Embedded Piezoelectric Sensors and Actuators. J. Intell. Mater. Syst. Struct. 1995, 6, 350–363. [Google Scholar] [CrossRef]



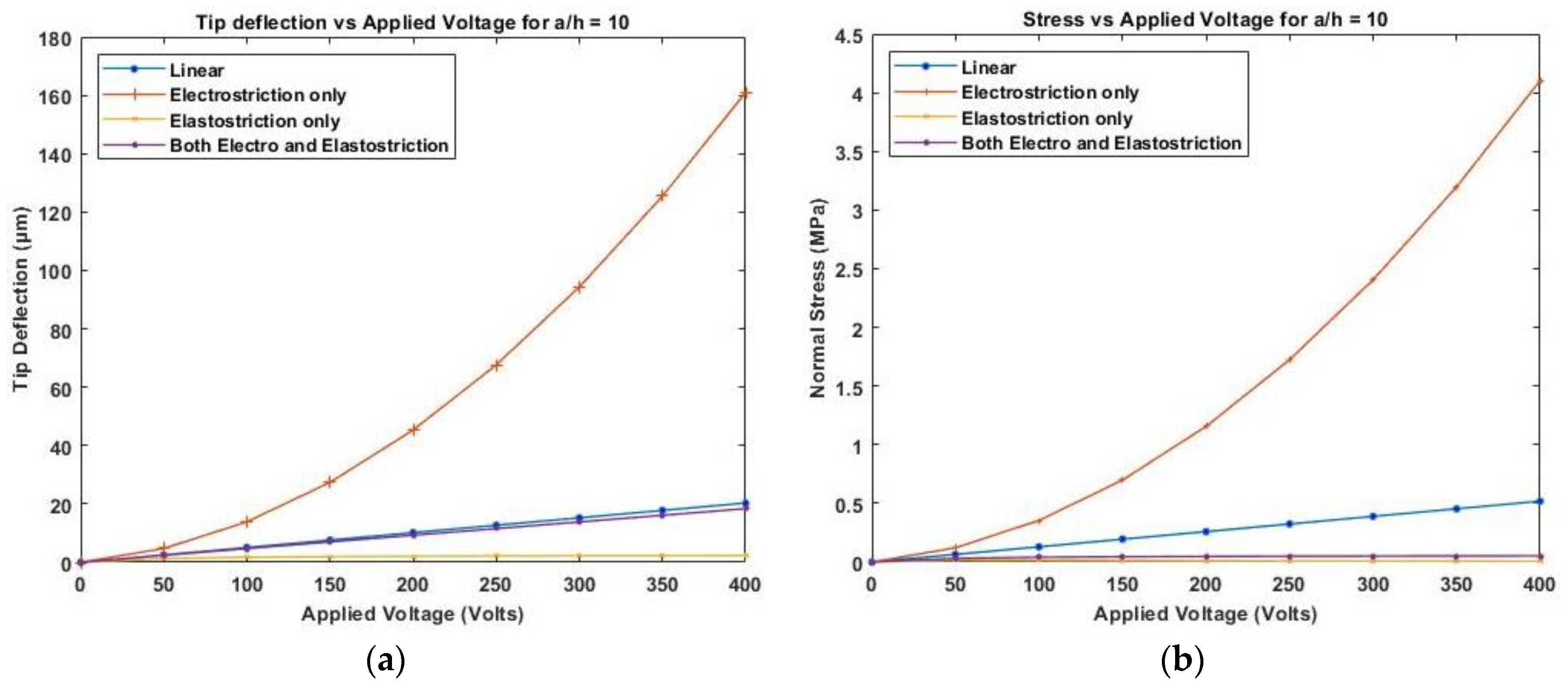

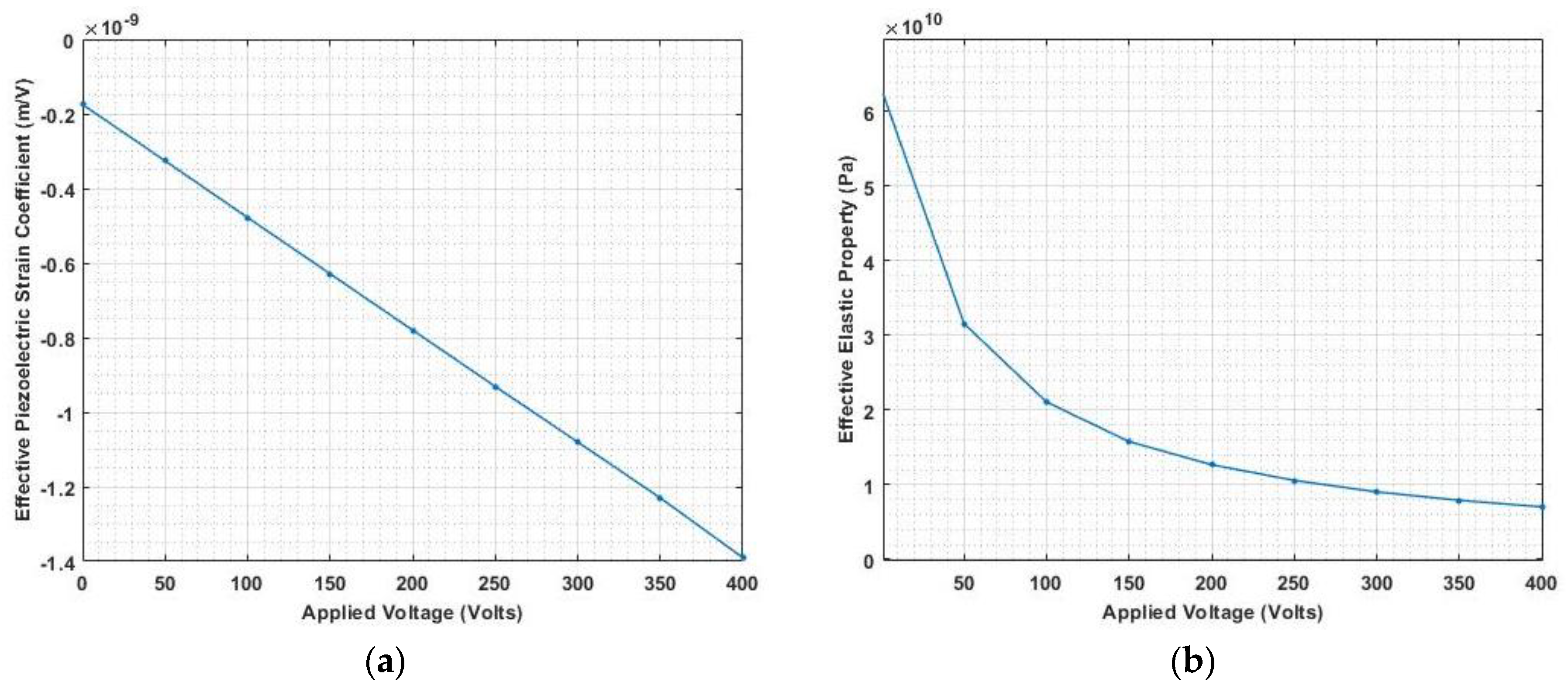
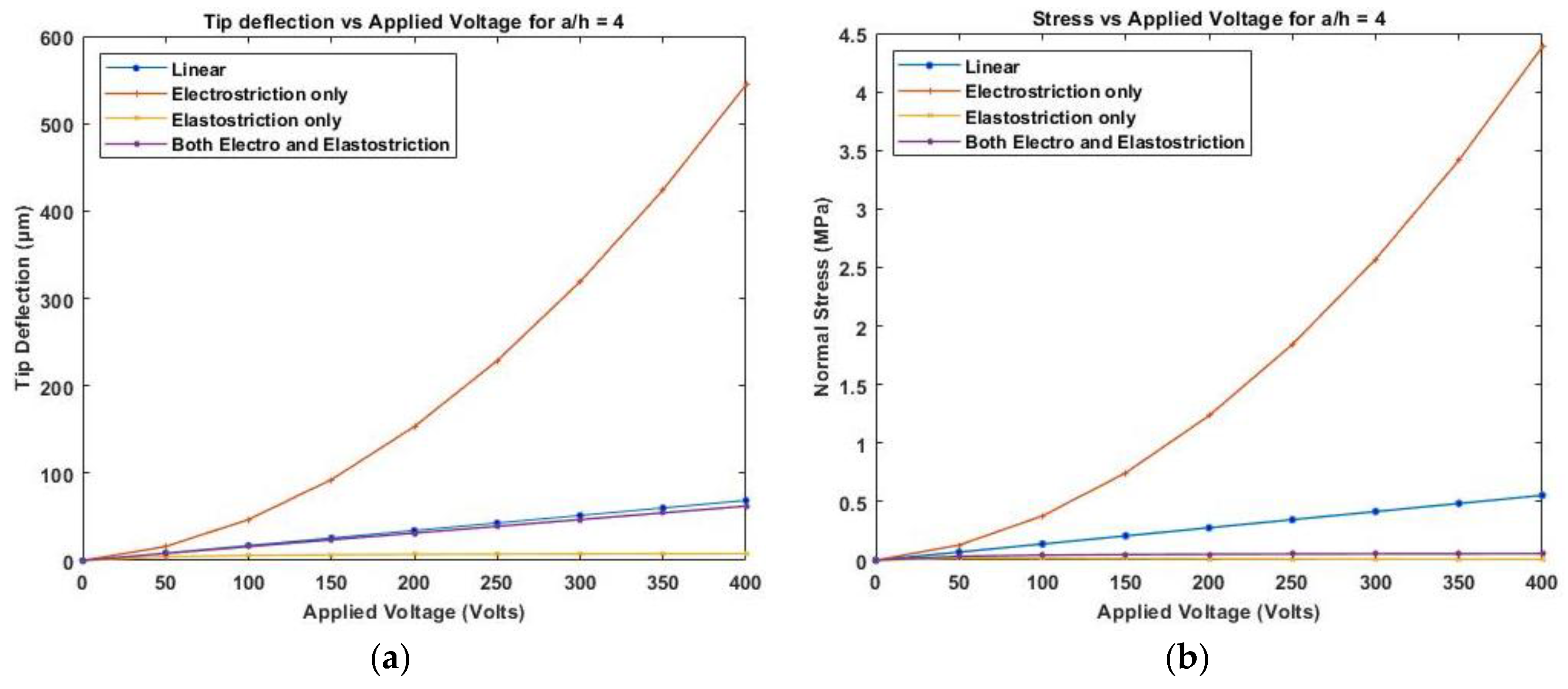
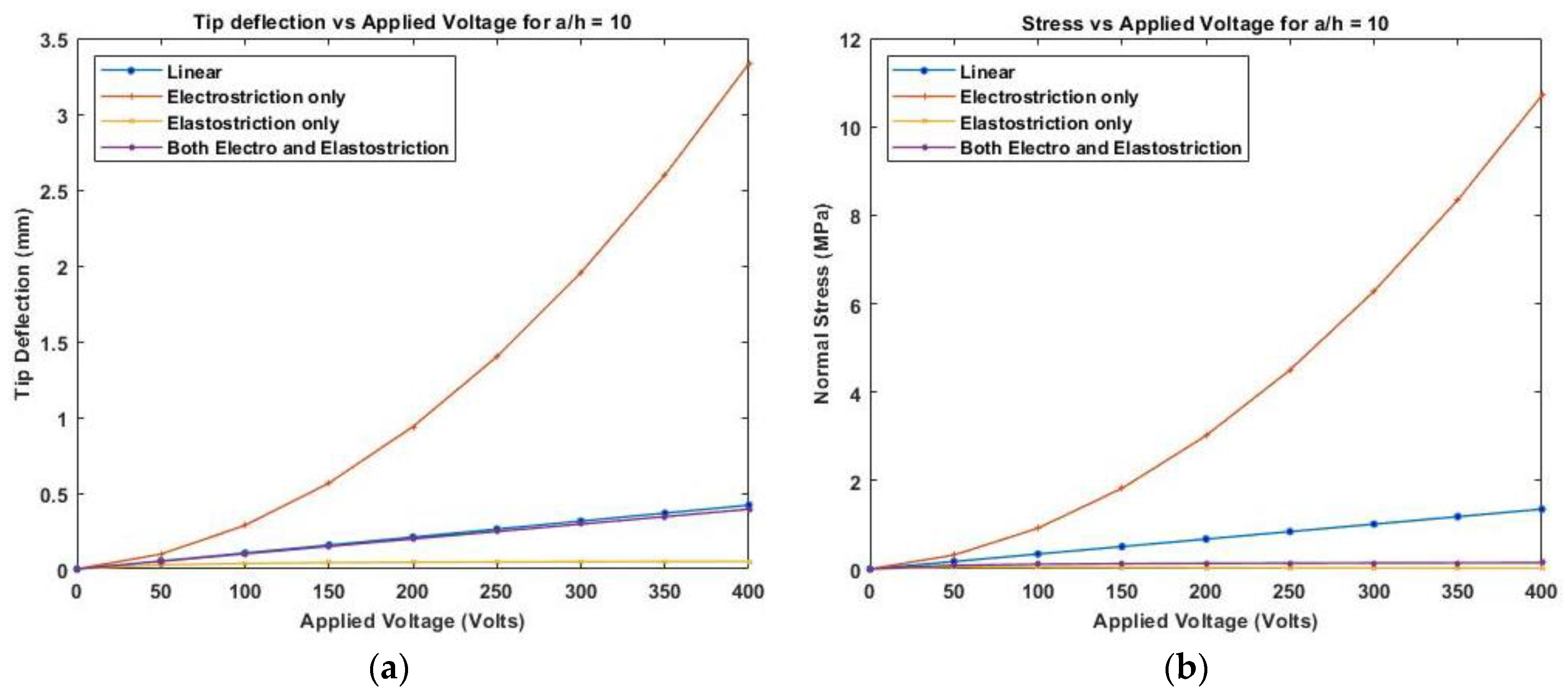

| 775 | 0.29 | 0.046 cm−2 | 0.046 cm−2 |
| FE Tzou/Ye [24] | FE Jiang & Li [4] | FE Present | Theoretical Jiang & Li [4] | |
|---|---|---|---|---|
| 0.02 | 0.132 | 0.136 | 0.138 | 0.138 |
| 0.04 | 0.528 | 0.545 | 0.552 | 0.552 |
| 0.06 | 1.19 | 1.226 | 1.242 | 1.242 |
| 0.08 | 2.11 | 2.18 | 2.208 | 2.208 |
| 0.1 | 3.30 | 3.41 | 3.45 | 3.45 |
| Material | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| PZT [25] | 63 | 63 | 63 | 24.8 | 24.8 | 24.8 | 0.28 | 0.28 | 0.28 | −166 | −166 |
| PZT-4 [26] | 81.3 | - | 64.5 | - | 25.6 | 25.6 | - | 0.43 | 0.43 | −122 | −122 |
| Al [26] | 68.9 | 68.9 | 68.9 | 27.6 | 27.6 | 27.6 | 0.25 | 0.25 | 0.25 | - | - |
| Adhesive [26] | 6.9 | 6.9 | 6.9 | 2.46 | 2.46 | 2.46 | 0.4 | 0.4 | 0.4 | - | - |
| T300/934 [26] | 132.38 | - | 107.6 | - | 56.5 | 56.5 | - | 0.24 | 0.43 | - | - |
| Material | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| PZT 3203 HD [11] | 60.24 | 60.24 | 47.62 | 19.084 | 19.084 | 24.04 | 0.494 | 0.494 | 0.253 |
| AS/3501 Gr/Ep [25] | 144.8 | 9.65 | - | 5.92 | 7.1 | 7.1 | - | - | 0.3 |
| PZT APC 850 [15] | 63 | 63 | 63 | 24.05 | 24.05 | 24.05 | 0.31 | 0.31 | 0.31 |
| Silicon [15] | 166 | 166 | 166 | 65.9 | 65.9 | 65.9 | 0.26 | 0.26 | 0.26 |
| PZT 3203 HD [11] | −320 | −320 | 650 | −520 | - | ||||
| PZT APC 850 [15] | −175 | −175 | - | −1210 | −6.3 | ||||
| Elastostriction (%) | Electrostriction (%) | Both (%) | |
|---|---|---|---|
| Deflection | −86.16 | 691.41 | 9.49 |
| Normal Stress | −98.45 | 691.42 | −87.74 |
| Shear Stress | −85.81 | 691.32 | 12.23 |
| Elastostriction (%) | Electrostriction (%) | Both (%) | |
|---|---|---|---|
| Deflection | −81.97 | 691.42 | 42.65 |
| Normal Stress | −97.98 | 691.42 | −84.038 |
| Shear Stress | −82.22 | 691.36 | 43.73 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Akhlaq, A.; Shaik Dawood, M.S.I.; Jaffar Syed, M.A.; Sulaeman, E. A Study on the Effect of Piezoelectric Nonlinearity on the Bending Behaviour of Smart Laminated Composite Beam. Materials 2023, 16, 2839. https://doi.org/10.3390/ma16072839
Akhlaq A, Shaik Dawood MSI, Jaffar Syed MA, Sulaeman E. A Study on the Effect of Piezoelectric Nonlinearity on the Bending Behaviour of Smart Laminated Composite Beam. Materials. 2023; 16(7):2839. https://doi.org/10.3390/ma16072839
Chicago/Turabian StyleAkhlaq, Adnan, Mohd Sultan Ibrahim Shaik Dawood, Mohamed Ali Jaffar Syed, and Erwin Sulaeman. 2023. "A Study on the Effect of Piezoelectric Nonlinearity on the Bending Behaviour of Smart Laminated Composite Beam" Materials 16, no. 7: 2839. https://doi.org/10.3390/ma16072839
APA StyleAkhlaq, A., Shaik Dawood, M. S. I., Jaffar Syed, M. A., & Sulaeman, E. (2023). A Study on the Effect of Piezoelectric Nonlinearity on the Bending Behaviour of Smart Laminated Composite Beam. Materials, 16(7), 2839. https://doi.org/10.3390/ma16072839






