Estimation of Residual Stress in Selective Laser Melting of a Zr-Based Amorphous Alloy
Abstract
1. Introduction
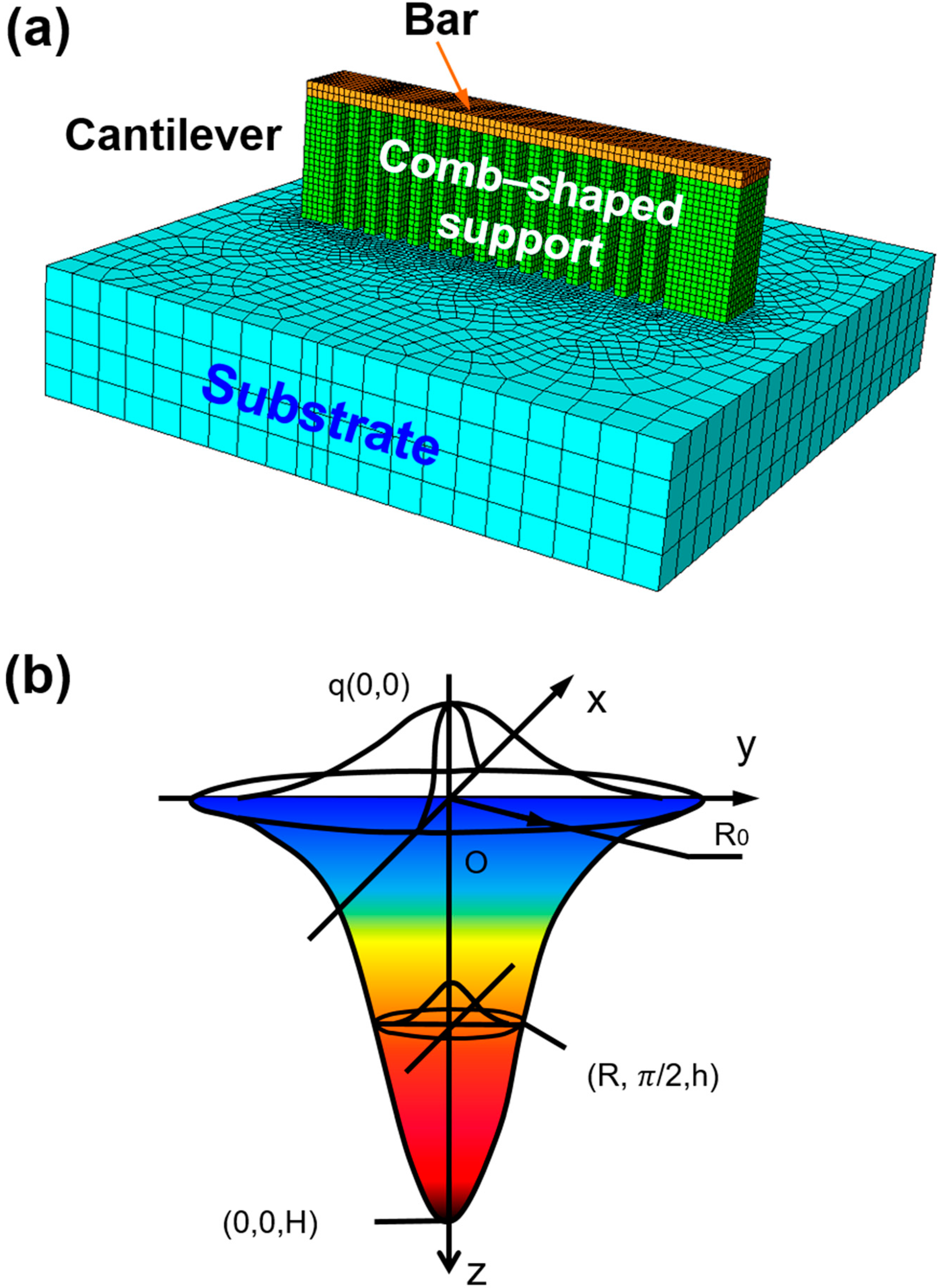
2. Constitutive Model and Finite Element Simulation
2.1. Heat Input Modeling
2.2. Heat Transfer Modeling
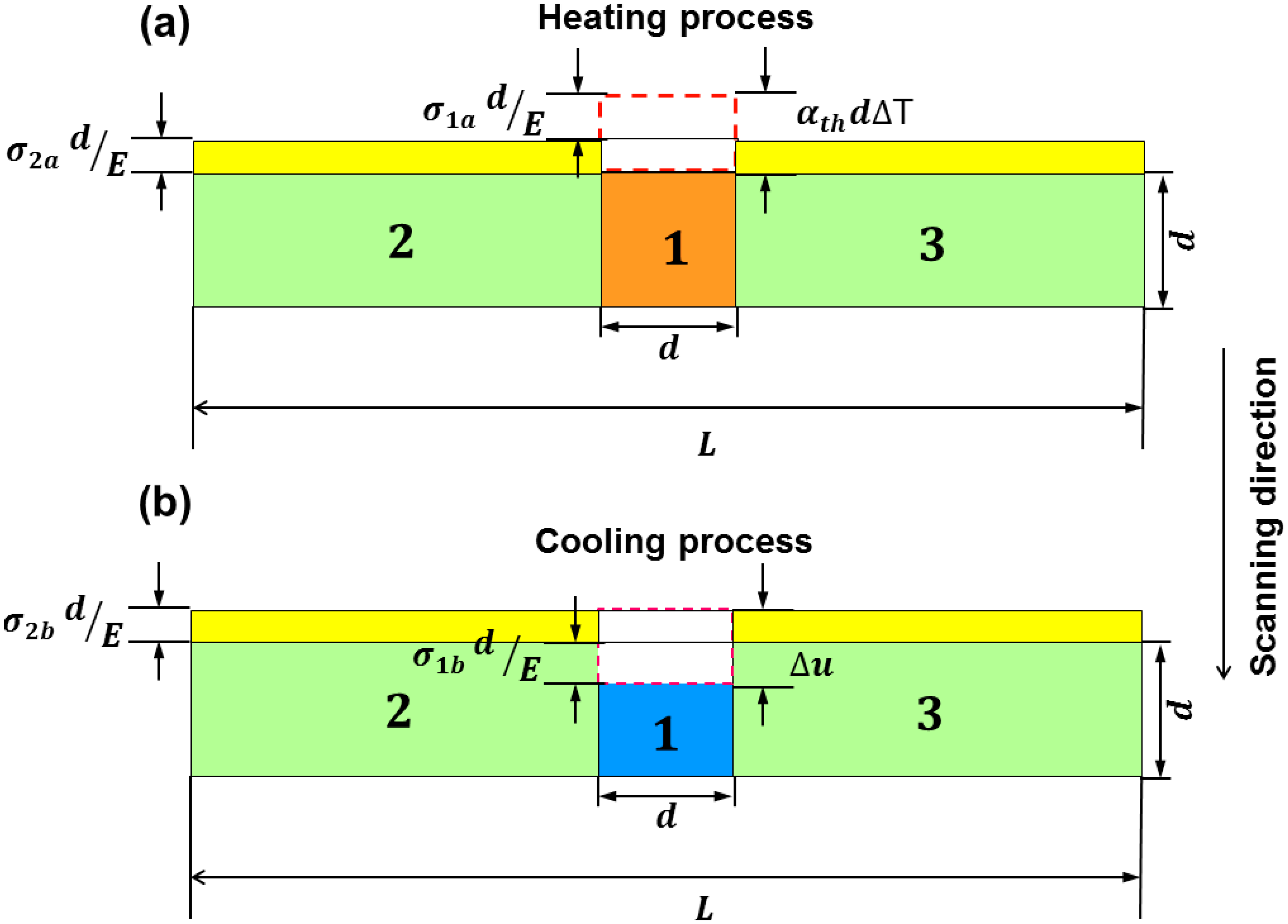
2.3. Residual Stress Model
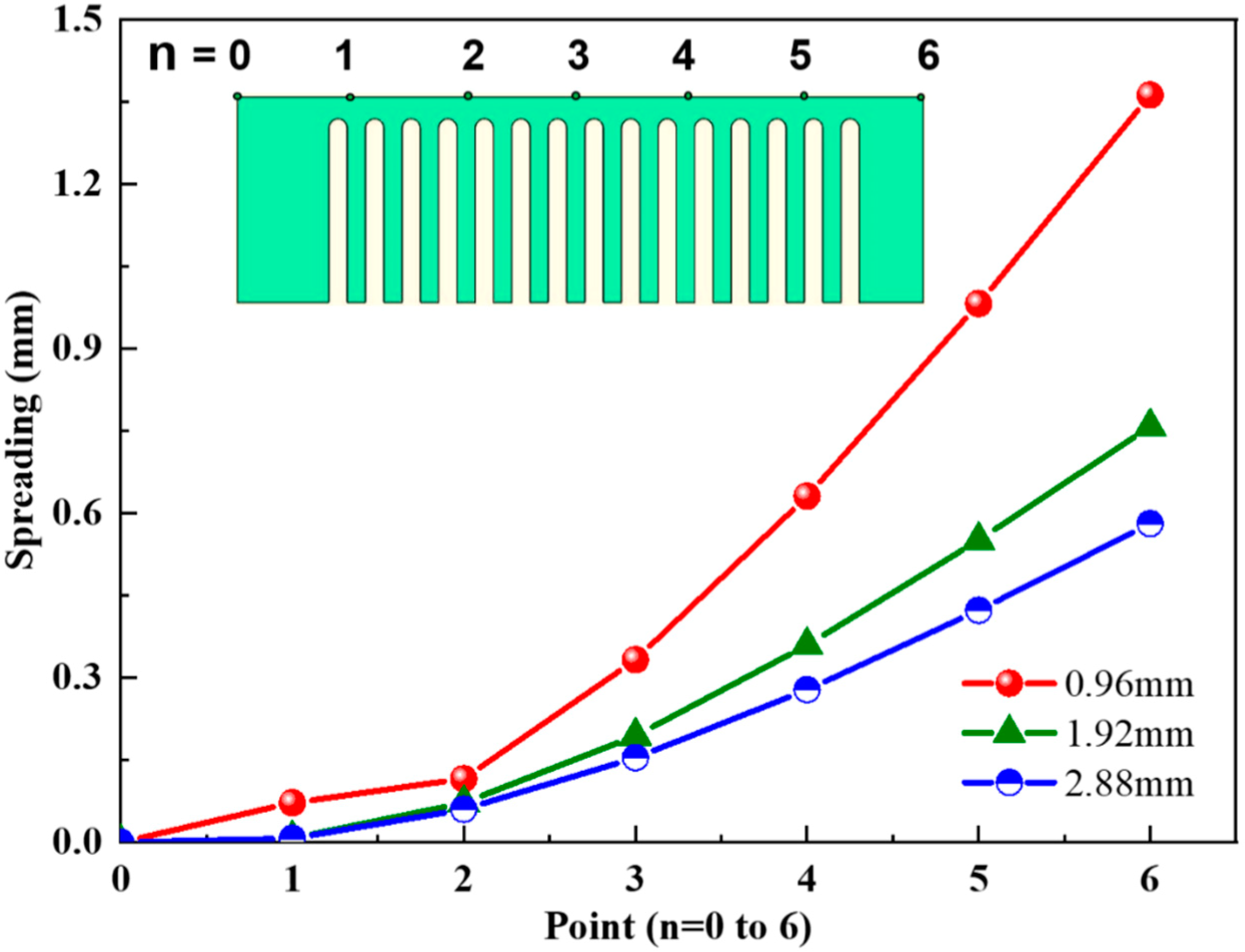
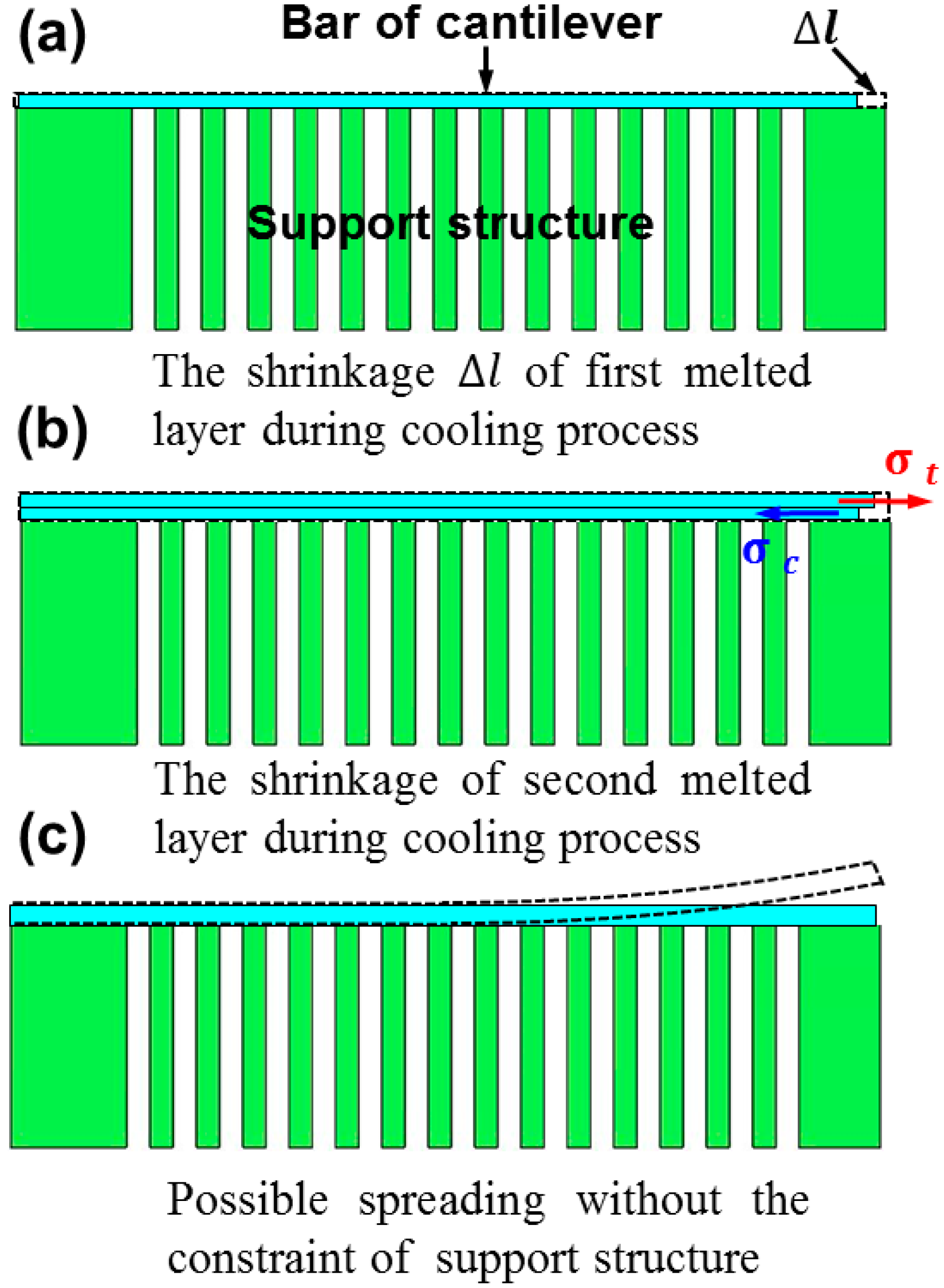
2.4. Inherent Shrinkage Model
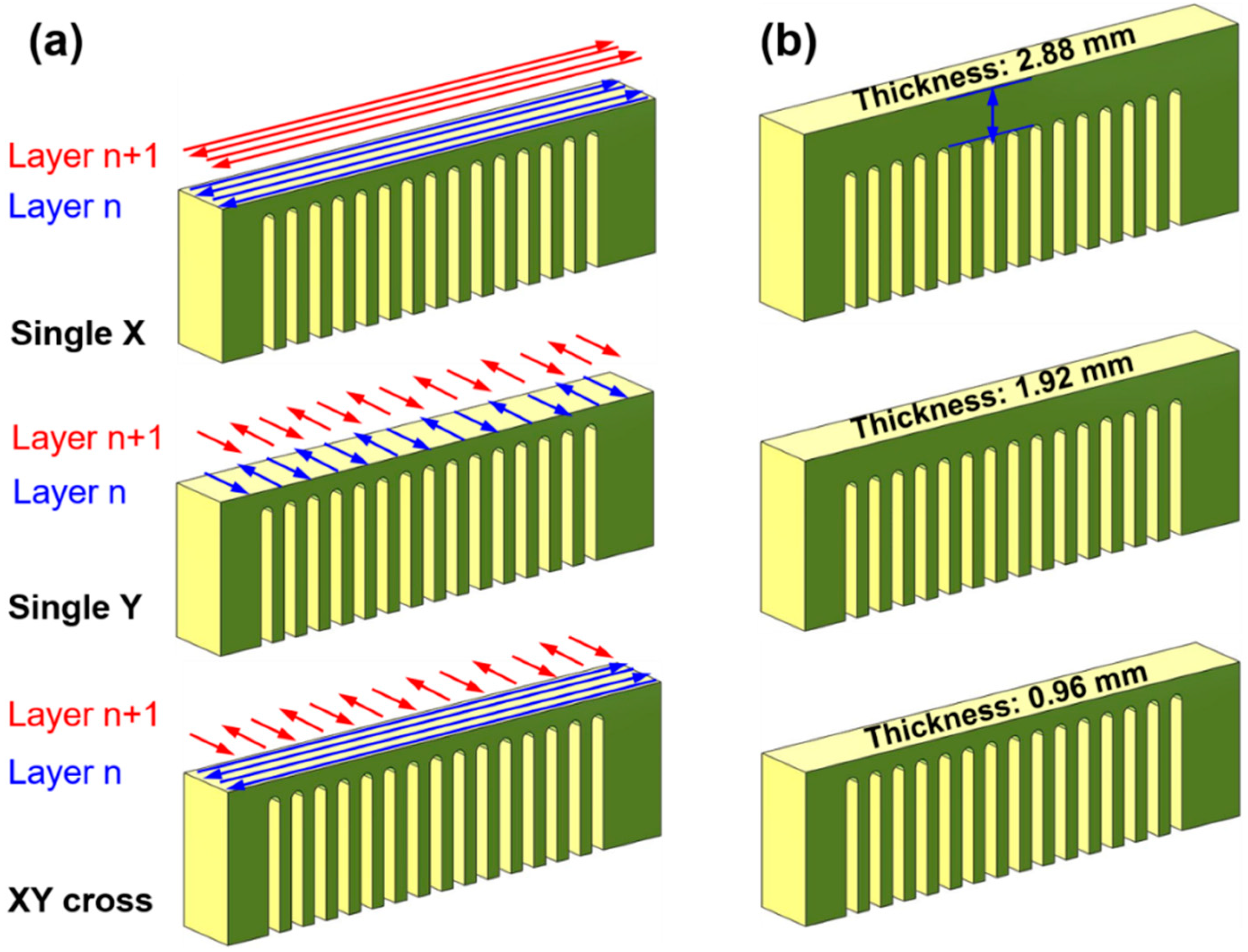
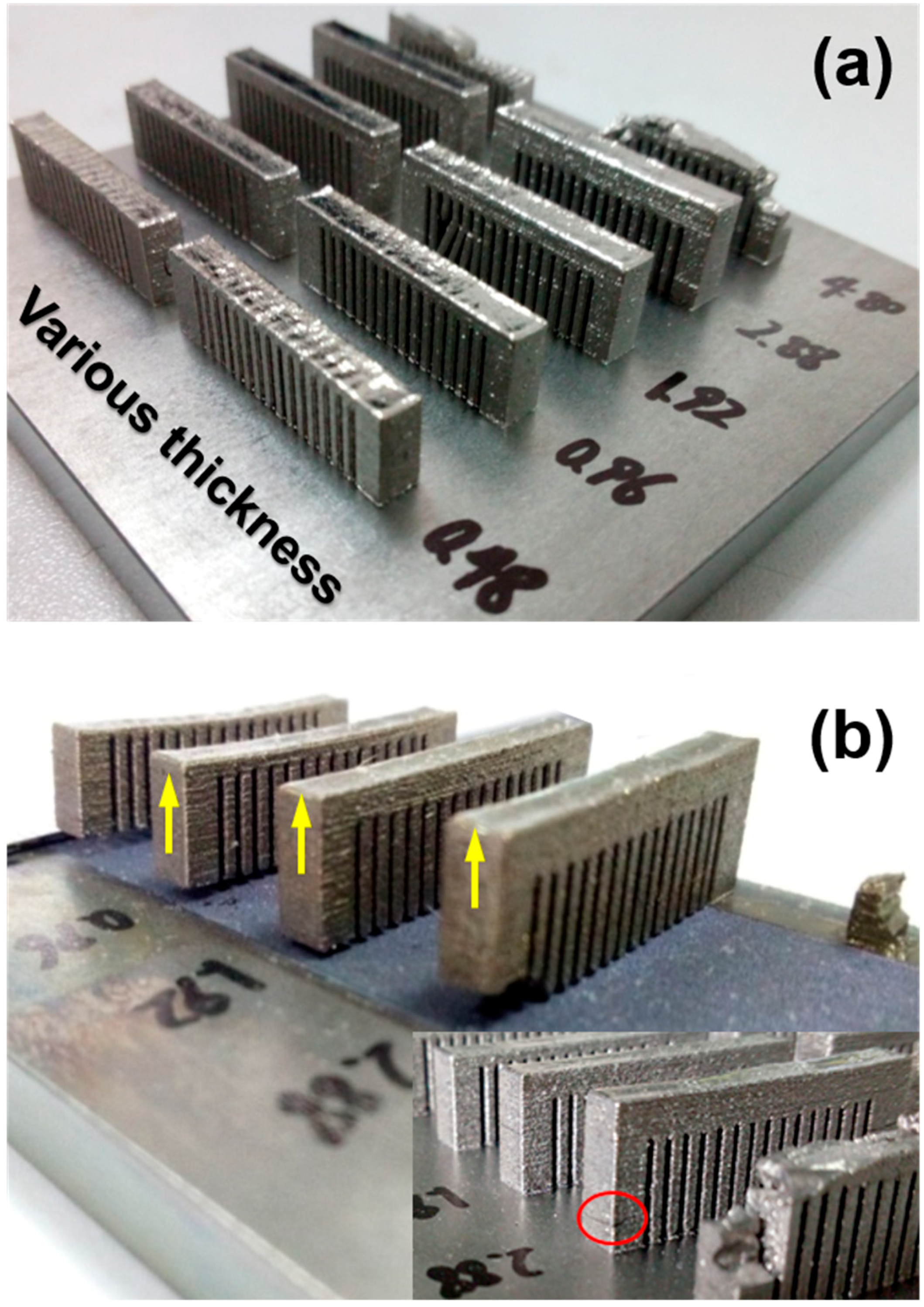
3. Experimental Procedures
4. Results
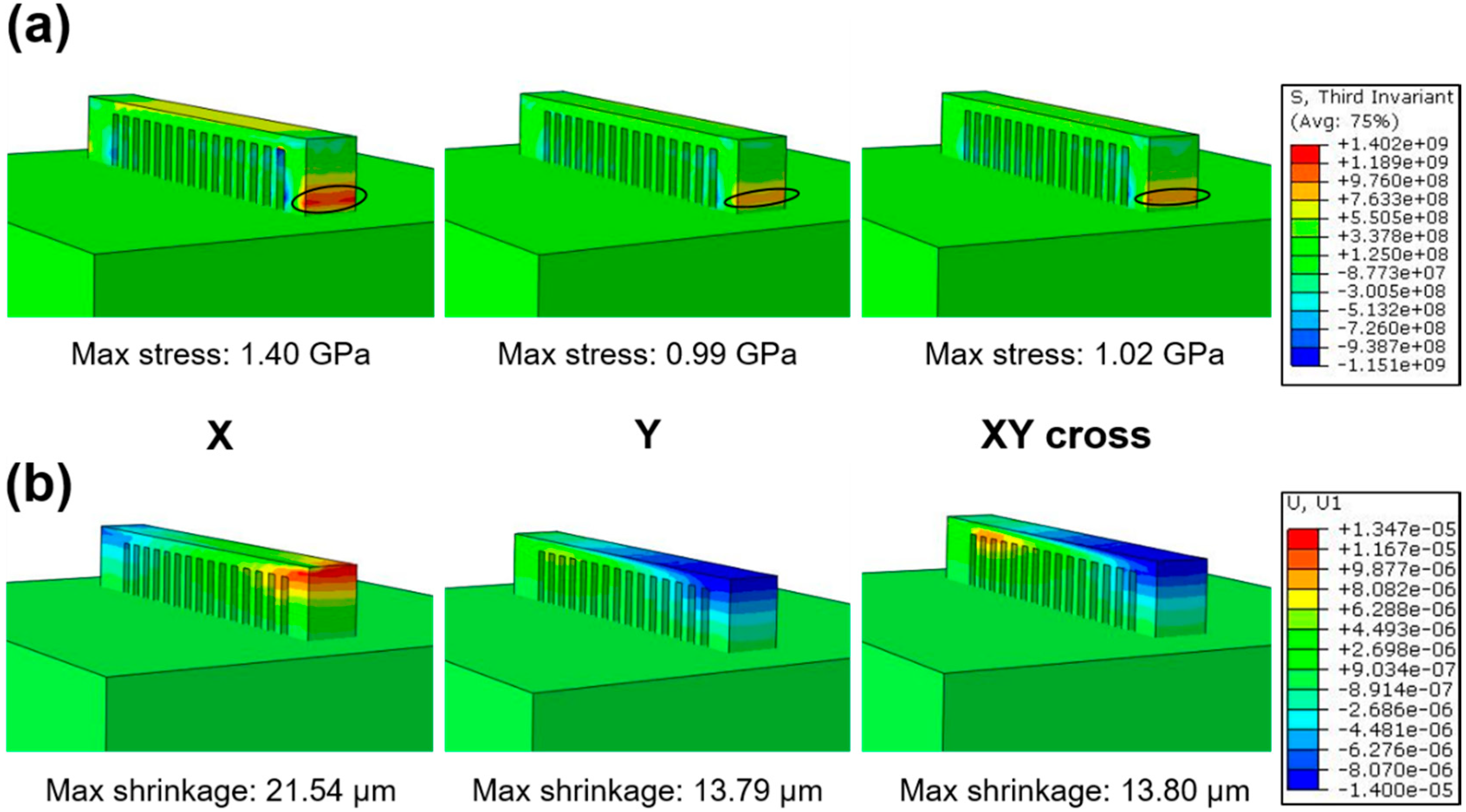
4.1. FEM-Simulated Residual Stress Field
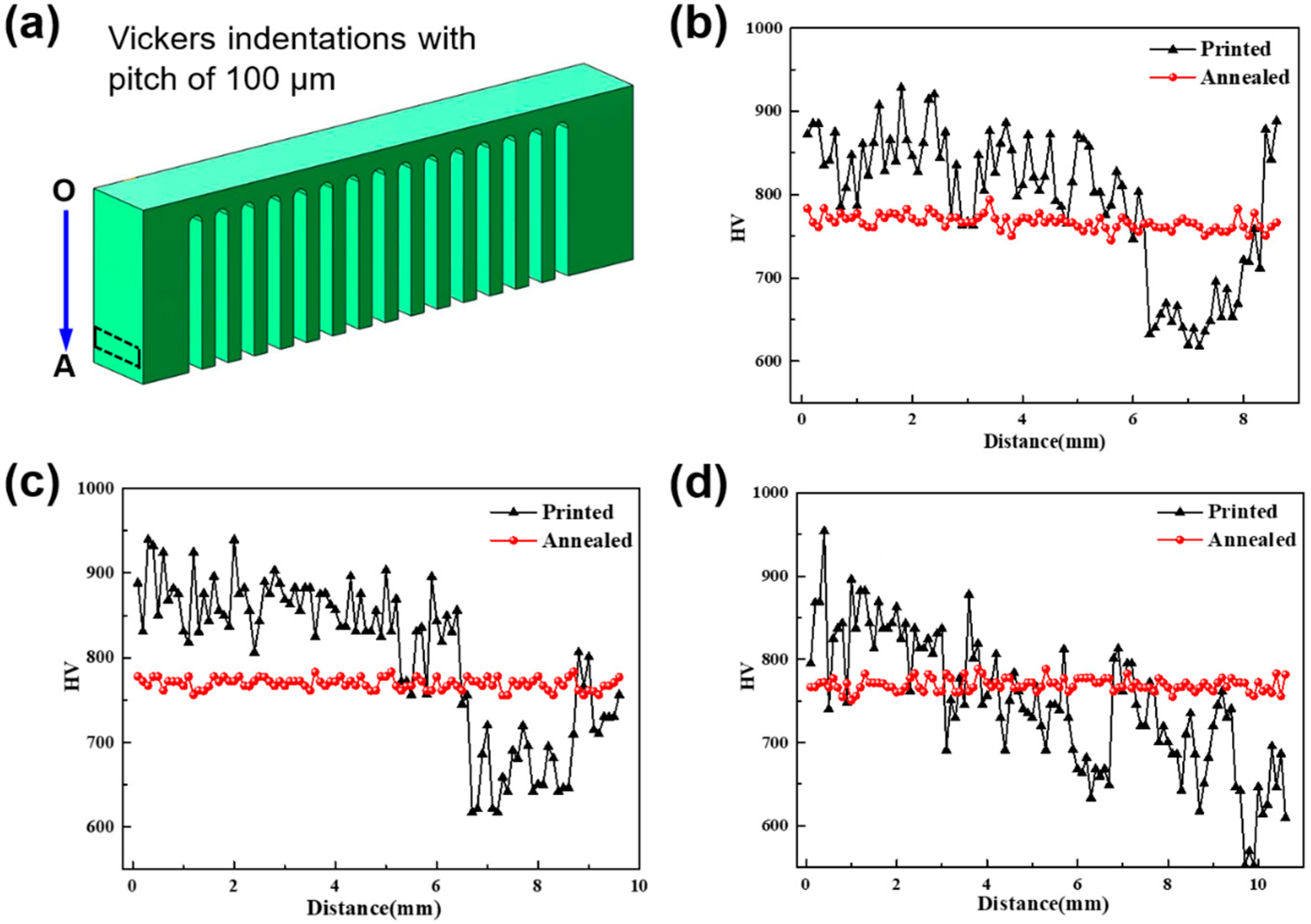
4.2. Measured Residual Stress Distribution
4.3. Residual Stress Released after Annealing and Preheating
5. Discussion
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Ghidelli, M.; Idrissi, H.; Gravier, S.; Blandin, J.J.; Raskin, J.P.; Schryvers, D.; Pardoen, T. Homogeneous flow and size dependent mechanical behavior in highly ductile Zr65Ni35metallic glass films. Acta Mater. 2017, 131, 246–259. [Google Scholar] [CrossRef]
- Li, N.; Xu, X.; Zheng, Z.; Liu, L. Enhanced formability of a Zr-based bulk metallic glass in a supercooled liquid state by vibrational loading. Acta Mater. 2014, 65, 400–411. [Google Scholar] [CrossRef]
- Li, N.; Chen, Y.; Jiang, M.Q.; Li, D.J.; He, J.J.; Wu, Y.; Liu, L. A thermoplastic forming map of a Zr-based bulk metallic glass. Acta Mater. 2013, 61, 1921–1931. [Google Scholar] [CrossRef]
- Wang, X.; Dai, W.; Zhang, M.; Gong, P.; Li, N. Thermoplastic micro-formability of TiZrHfNiCuBe high entropy metallic glass. J. Mater. Sci. Technol. 2018, 25, 4–11. [Google Scholar] [CrossRef]
- Li, N.; Liu, Z.; Wang, X.; Zhang, M. Vibration-accelerated activation of flow units in a Pd-based bulk metallic glass. Mater. Sci. Eng. A 2017, 692, 62–66. [Google Scholar] [CrossRef]
- Li, N.; Chen, W.; Liu, L. Thermoplastic Micro-Forming of Bulk Metallic Glasses: A Review. JOM 2016, 68, 1246–1261. [Google Scholar] [CrossRef]
- Li, N.; Chen, Q.; Liu, L. Size dependent plasticity of a Zr-based bulk metallic glass during room temperature compression. J. Alloys Compd. 2010, 493, 142–147. [Google Scholar] [CrossRef]
- Li, N.; Xu, E.; Liu, Z.; Wang, X.; Liu, L. Tuning apparent friction coefficient by controlled patterning bulk metallic glasses surfaces. Sci. Rep. 2016, 6, 1–9. [Google Scholar] [CrossRef] [PubMed]
- Shi, X.; Ma, S.; Liu, C.; Chen, C.; Wu, Q.; Chen, X.; Lu, J. Performance of High Layer Thickness in Selective Laser Melting of Ti6Al4V. Materials 2016, 9, 975. [Google Scholar] [CrossRef] [PubMed]
- Wang, S. Research on High Layer Thickness Fabricated of 316L by Selective Laser Melting. Materials 2017, 10, 1. [Google Scholar] [CrossRef] [PubMed]
- Cai, C.; Gao, X.; Teng, Q.; Li, M.; Pan, K.; Song, B.; Yan, C.; Wei, Q.; Shi, Y. A novel hybrid selective laser melting/hot isostatic pressing of near-net shaped Ti-6Al-4V alloy using an in-situ tooling: Interfacial microstructure evolution and enhanced mechanical properties. Mater. Sci. Eng. A 2018, 717, 95–104. [Google Scholar] [CrossRef]
- Ouyang, D.; Li, N.; Liu, L. Structural heterogeneity in 3D printed Zr-based bulk metallic glass by selective laser melting. J. Alloys Compd. 2018, 740, 603–609. [Google Scholar] [CrossRef]
- Li, N.; Zhang, J.; Xing, W.; Ouyang, D.; Liu, L. 3D printing of Fe-based bulk metallic glass composites with combined high strength and fracture toughness. Mater. Des. 2018, 143, 285–296. [Google Scholar] [CrossRef]
- Jung, H.Y.; Choi, S.J.; Prashanth, K.G.; Stoica, M.; Scudino, S.; Yi, S.; Kühn, U.; Kim, D.H.; Kim, K.B.; Eckert, J. Fabrication of Fe-based bulk metallic glass by selective laser melting: A parameter study. Mater. Des. 2015, 86, 703–708. [Google Scholar] [CrossRef]
- Li, X.P.; Roberts, M.P.; O’Keeffe, S.; Sercombe, T.B. Selective laser melting of Zr-based bulk metallic glasses: Processing, microstructure and mechanical properties. Mater. Des. 2016, 112, 217–226. [Google Scholar] [CrossRef]
- Pauly, S.; Schricker, C.; Scudino, S.; Deng, L.; Kühn, U. Processing a glass-forming Zr-based alloy by selective laser melting. Mater. Des. 2017, 135, 133–141. [Google Scholar] [CrossRef]
- Mishurova, T.; Cabeza, S.; Artzt, K.; Haubrich, J.; Klaus, M.; Genzel, C.; Requena, G.; Bruno, G. An Assessment of Subsurface Residual Stress Analysis in SLM Ti-6Al-4V. Materials 2017, 10, 348. [Google Scholar] [CrossRef] [PubMed]
- Rickhey, F.; Marimuthu, K.P.; Lee, H. Investigation on indentation cracking-based approaches for residual stress evaluation. Materials 2017, 10, 404. [Google Scholar] [CrossRef] [PubMed]
- Le, S.; Salem, M.; Hor, A. Improvement of the bridge curvature method to assess residual stresses in selective laser melting. Addit. Manuf. 2018, 22, 320–329. [Google Scholar] [CrossRef]
- Simulation, N.; Analysis, N.D. Investigation on the residual stress state of drawn tubes by numerical simulation and neutron diffraction analysis. Materials 2013, 6, 5118–5130. [Google Scholar] [CrossRef]
- Method, M.C.; Liu, C. Experimental investigation on the residual stresses in a thick joint with a partial repair weld using. Materials 2018, 11, 633. [Google Scholar] [CrossRef]
- Kruth, J.P.; Froyen, L.; Van Vaerenbergh, J.; Mercelis, P.; Rombouts, M.; Lauwers, B. Selective laser melting of iron-based powder. J. Mater. Process. Technol. 2004, 149, 616–622. [Google Scholar] [CrossRef]
- Buchbinder, D.; Meiners, W.; Pirch, N.; Wissenbach, K.; Schrage, J. Investigation on reducing distortion by preheating during manufacture of aluminum components using selective laser melting. J. Laser Appl. 2014, 26, 012004. [Google Scholar] [CrossRef]
- Kolossov, S.; Boillat, E.; Glardon, R.; Fischer, P.; Locher, M. 3D FE simulation for temperature evolution in the selective laser sintering process. Int. J. Mach. Tool. Manuf. 2004, 44, 117–123. [Google Scholar] [CrossRef]
- Dai, K.; Shaw, L. Thermal and mechanical finite element modeling of laser forming from metal and ceramic powders. Acta Mater. 2004, 52, 69–80. [Google Scholar] [CrossRef]
- Matsumoto, M.; Shiomi, M.; Osakada, K.; Abe, F. Finite element analysis of single layer forming on metallic powder bed in rapid prototyping by selective laser processing. Int. J. Mach. Tool. Manuf. 2002, 42, 61–67. [Google Scholar] [CrossRef]
- Alvarez, P.; Ecenarro, J.; Setien, I.; Sebastian, M.S.; Echeverria, A.; Eciolaza, L. Computationally efficient distortion prediction in Powder Bed Fusion Additive Manufacturing. Int. J. Eng. Res. Sci. 2016, 2, 2395–6992. [Google Scholar]
- Li, C.; Liu, J.F.; Fang, X.Y.; Guo, Y.B. Efficient predictive model of part distortion and residual stress in selective laser melting. Addit. Manuf. 2017, 17, 157–168. [Google Scholar] [CrossRef]
- Parry, L.; Ashcroft, I.A.; Wildman, R.D. Understanding the effect of laser scan strategy on residual stress in selective laser melting through thermo-mechanical simulation. Addit. Manuf. 2016, 12, 1–15. [Google Scholar] [CrossRef]
- Shen, Y.; Li, Y.; Chen, C.; Tsai, H. 3D printing of large, complex metallic glass structures. Mater. Des. 2017, 117, 213–222. [Google Scholar] [CrossRef]
- Pauly, S.; Löber, L.; Petters, R.; Stoica, M.; Scudino, S.; Kühn, U.; Eckert, J. Processing metallic glasses by selective laser melting. Mater. Today 2013, 16, 37–41. [Google Scholar] [CrossRef]
- Hofmann, D.C.; Bordeenithikasem, P.; Pate, A.; Roberts, S.N.; Vogli, E. Developing processing parameters and characterizing microstructure and properties of an additively manufactured FeCrMoBC metallic glass forming alloy. Adv. Eng. Mater. 2018. [Google Scholar] [CrossRef]
- Shen, Y.; Li, Y.; Tsai, H. Evolution of crystalline phase during laser processing of Zr-based metallic glass. J. Non-Cryst. Solids 2018, 481, 299–305. [Google Scholar] [CrossRef]
- Li, X.P.; Kang, C.W.; Huang, H.; Sercombe, T.B. The role of a low-energy-density re-scan in fabricating crack-free Al85Ni5Y6Co2Fe2 bulk metallic glass composites via selective laser melting. Mater. Des. 2014, 63, 407–411. [Google Scholar] [CrossRef]
- Ouyang, D.; Li, N.; Xing, W.; Zhang, J.; Liu, L. 3D printing of crack-free high strength Zr-based bulk metallic glass composite by selective laser melting. Intermetallics 2017, 90, 128–134. [Google Scholar] [CrossRef]
- Chand, R.; Kim, I.-S.; Lee, J.-H.; Kim, J.-S. Numerical and Experiment Study of Residual Stress and Strain in Multi-Pass GMA Welding. Adv. Mater. Res. 2013, 717, 403–409. [Google Scholar] [CrossRef]
- Yu, G.; Gu, D.; Dai, D.; Xia, M. Influence of processing parameters on laser penetration depth and melting/re-melting densification during selective laser melting of aluminum alloy. Appl. Phys. A 2016, 122, 1–12. [Google Scholar] [CrossRef]
- Li, Y.; Gu, D. Thermal behavior during selective laser melting of commercially pure titanium powder: Numerical simulation and experimental study. Addit. Manuf. 2014, 1, 99–109. [Google Scholar] [CrossRef]
- Li, Z.; Li, B.; Bai, P.; Liu, B.; Wang, Y. Research on the Thermal Behaviour of a Selectively Laser Melted Aluminium Alloy: Simulation. Materials 2018, 11, 1172. [Google Scholar] [CrossRef] [PubMed]
- Tolochko, N.K.; Khlopkov, Y.V.; Mozzharov, S.E.; Michail, B.; Laoui, T.; Titov, V.I.; Ignatiev, M.B. Emerald Article: Absorptance of powder materials suitable for laser sintering Absorptance of powder materials suitable for laser sintering. Rapid Prototyp. J. 2005, 6, 155–161. [Google Scholar] [CrossRef]
- Gu, D.; He, B. Finite element simulation and experimental investigation of residual stresses in selective laser melted Ti-Ni shape memory alloy. Comput. Mater. Sci. 2016, 117, 221–232. [Google Scholar] [CrossRef]
- Akbari, D. International Journal of Pressure Vessels and Piping Effect of the welding heat input on residual stresses in butt-welds of dissimilar pipe joints. Int. J. Press. Vessels Pip. 2009, 86, 769–776. [Google Scholar] [CrossRef]
- Ghidelli, M.; Sebastiani, M.; Collet, C.; Guillemet, R. Determination of the elastic moduli and residual stresses of freestanding Au-TiW bilayer thin films by nanoindentation. Mater. Des. 2016, 106, 436–445. [Google Scholar] [CrossRef]
- Huang, Y.; Ning, Z.; Shen, Z.; Liang, W.; Sun, H. Bending behavior of as-cast and annealed ZrCuNiAl bulk metallic glass. J. Mater. Sci. Technol. 2017, 33, 1153–1158. [Google Scholar] [CrossRef]
- Shi, Y.; Shen, H.; Yao, Z.; Hu, J. Temperature gradient mechanism in laser forming of thin plates. Opt. Laser Technol. 2007, 39, 858–863. [Google Scholar] [CrossRef]
- Wan, H.Y.; Zhou, Z.J.; Li, C.P.; Chen, G.F.; Zhang, G.P. Effect of scanning strategy on grain structure and crystallographic texture of Inconel 718 processed by selective laser melting. J. Mater. Sci. Technol. 2018, 34, 1799–1804. [Google Scholar] [CrossRef]
- Ali, H.; Ma, L.; Ghadbeigi, H.; Mumtaz, K. In-situ residual stress reduction, martensitic decomposition and mechanical properties enhancement through high temperature powder bed pre-heating of Selective Laser Melted Ti6Al4V. Mater. Sci. Eng. A 2017, 695, 211–220. [Google Scholar] [CrossRef]
- Xiong, J.; Lei, Y.; Li, R. Finite element analysis and experimental validation of thermal behavior for thin-walled parts in GMAW-based additive manufacturing with various substrate preheating temperatures. Appl. Therm. Eng. 2017, 126, 43–52. [Google Scholar] [CrossRef]
- Pan, J.; Wang, Y.X.; Guo, Q.; Zhang, D.; Greer, A.L.; Li, Y. Extreme rejuvenation and softening in a bulk metallic glass. Nat. Commun. 2018. [Google Scholar] [CrossRef] [PubMed]
- Li, N.; Liu, L.; Chen, Q.; Pan, J.; Chan, K.C. The effect of free volume on the deformation behaviour of a Zr-based metallic glass under nanoindentation. J. Phys. D Appl. Phys. 2007, 40, 6055–6059. [Google Scholar] [CrossRef]
- Li, N.; Chan, K.C.; Liu, L. The indentation size effect in Pd40Cu30Ni10P20 bulk metallic glass. J. Phys. D Appl. Phys. 2008, 41, 5. [Google Scholar] [CrossRef]
- Flores, K.M.; Dauskardt, R.H. Mean stress effects on flow localization and failure in a bulk metallic glass. Acta Mater. 2001, 49, 2527–2537. [Google Scholar] [CrossRef]
- Wang, L.; Bei, H.; Gao, Y.F.; Lu, Z.P.; Nieh, T.G. Effect of residual stresses on the hardness of bulk metallic glasses. Acta Mater. 2011, 59, 2858–2864. [Google Scholar] [CrossRef]


| Parameter | Value |
|---|---|
| Laser power, P (W) | 240 |
| Scanning speed, V (mm/s) | 1200 |
| Density of Zr-based MG powder, ρ (kg m−3) | 4129 |
| Latent heat of fusion (J Kg−1) | 2.638 × 105 |
| Melting point of Zr-based BMG (K) | 1165 |
| Coefficient for the heat convection, h (W m−2 K−1) | 20 |
| The Stefan-Boltzmann constant, (W m−2 K−4) | 5.67 × 10−8 |
| Radiation emissivity, | 0.77 |
| Temperature T (°C) | Specific Heat Capacity (J Kg−1 °C−1) | Thermal Conductivity k (W m−1 °C−1) |
|---|---|---|
| 20 | 326 | 4.9 |
| 100 | 346 | 6.2 |
| 200 | 342 | 7.4 |
| 300 | 271 | 6.8 |
| 400 | 233 | 6.6 |
| 500 | −323 | 7.6 |
| 600 | 112 | 7.7 |
| 700 | 362 | 10.1 |
| 800 | 375 | 9.6 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xing, W.; Ouyang, D.; Li, N.; Liu, L. Estimation of Residual Stress in Selective Laser Melting of a Zr-Based Amorphous Alloy. Materials 2018, 11, 1480. https://doi.org/10.3390/ma11081480
Xing W, Ouyang D, Li N, Liu L. Estimation of Residual Stress in Selective Laser Melting of a Zr-Based Amorphous Alloy. Materials. 2018; 11(8):1480. https://doi.org/10.3390/ma11081480
Chicago/Turabian StyleXing, Wei, Di Ouyang, Ning Li, and Lin Liu. 2018. "Estimation of Residual Stress in Selective Laser Melting of a Zr-Based Amorphous Alloy" Materials 11, no. 8: 1480. https://doi.org/10.3390/ma11081480
APA StyleXing, W., Ouyang, D., Li, N., & Liu, L. (2018). Estimation of Residual Stress in Selective Laser Melting of a Zr-Based Amorphous Alloy. Materials, 11(8), 1480. https://doi.org/10.3390/ma11081480




