Reliability of X7R Multilayer Ceramic Capacitors During High Accelerated Life Testing (HALT)
Abstract
1. Introduction
- t: time to failure (TTF) (s);
- Ea: activation energy for the thermally activated process (eV);
- k: Boltzmann’s constant (8.62 × 10−5 eV/K);
- T: absolute temperature (K);
- A: pre-exponential factor (s−1).
- t1 = TTF, at T1 and V1, (s);
- t2 = TTF, at T2 and V2, (s);
- V1 and V2 = test voltages under conditions (V);
- n = voltage stress exponent;
- Ea = activation energy for dielectric wear out (eV);
- k = Boltzmann’s constant (8.62 × 10−5 eV/K);
- T1 and T2 = absolute test temperature (K).
2. Materials and Methods
2.1. Multilayer Ceramic Capacitors Preparation
2.2. Electrical Characterization
3. Results and discussion
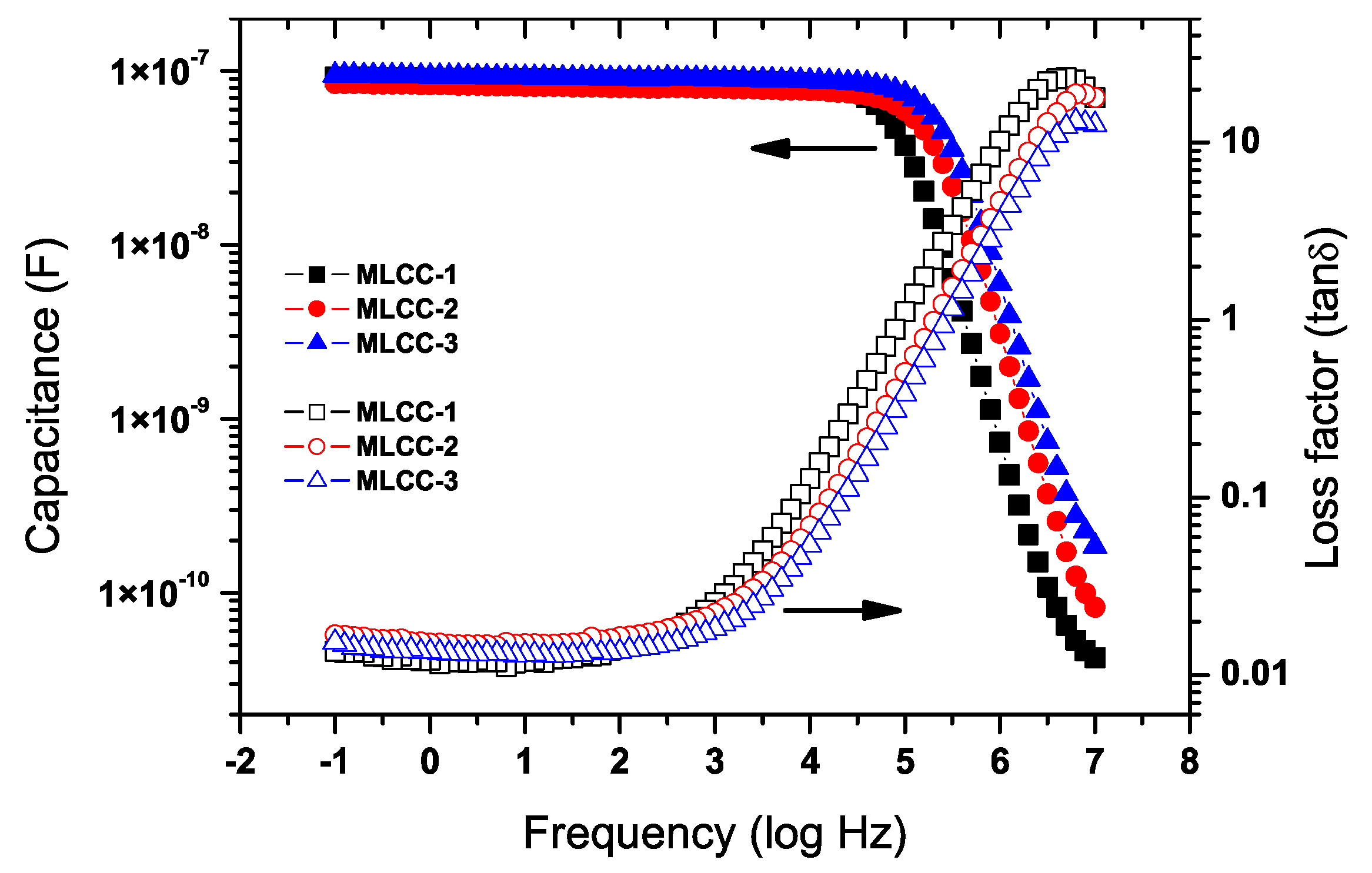
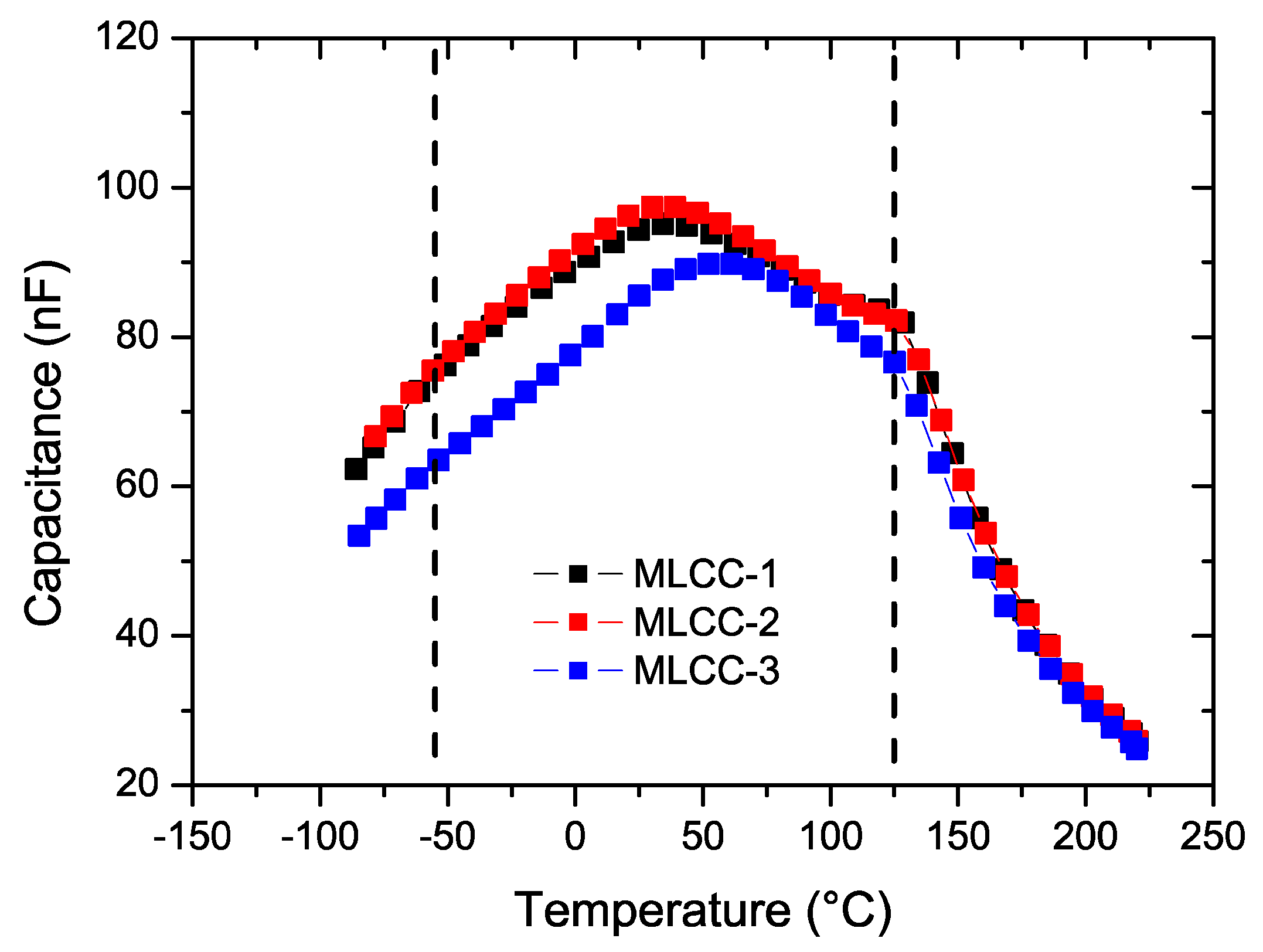
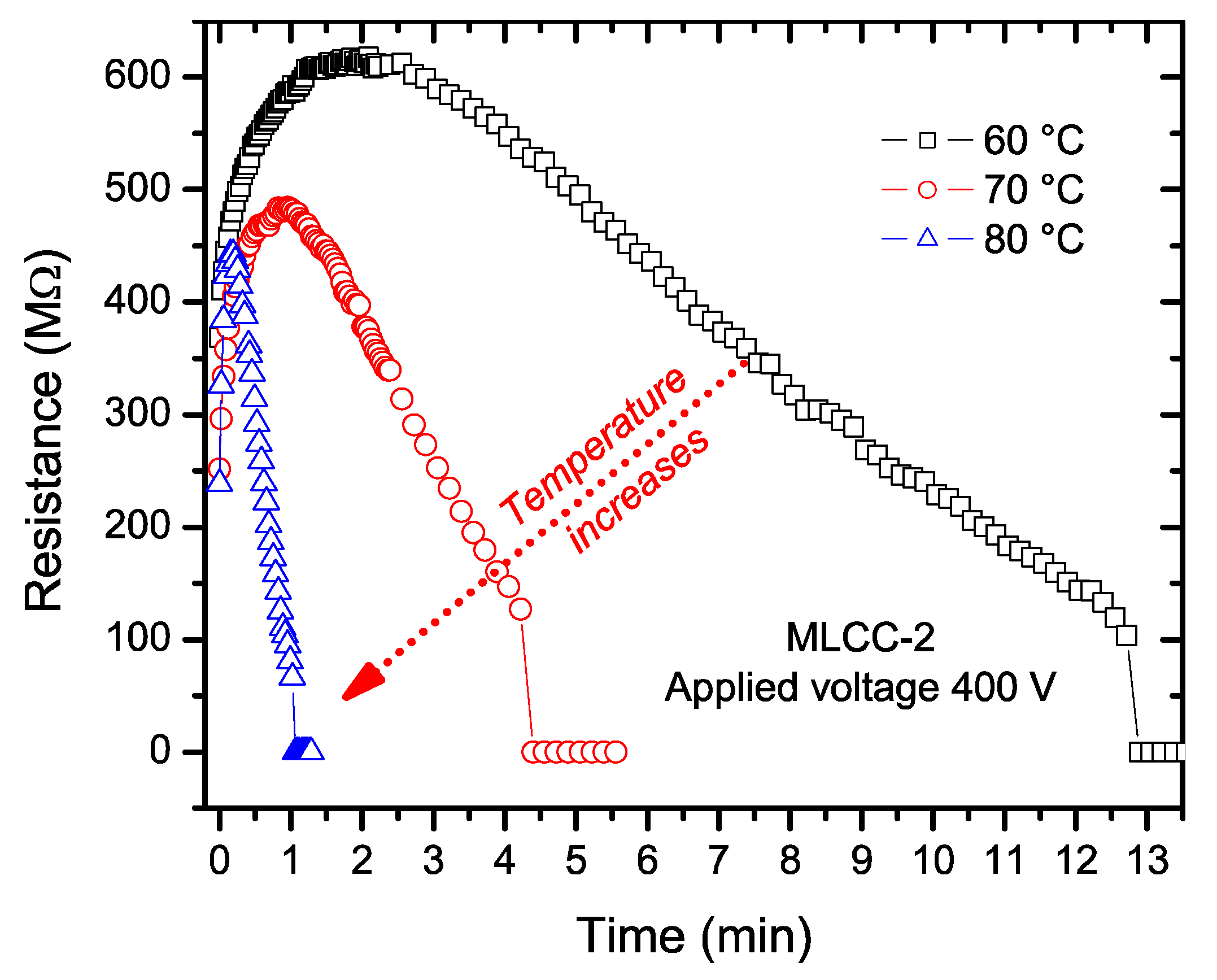
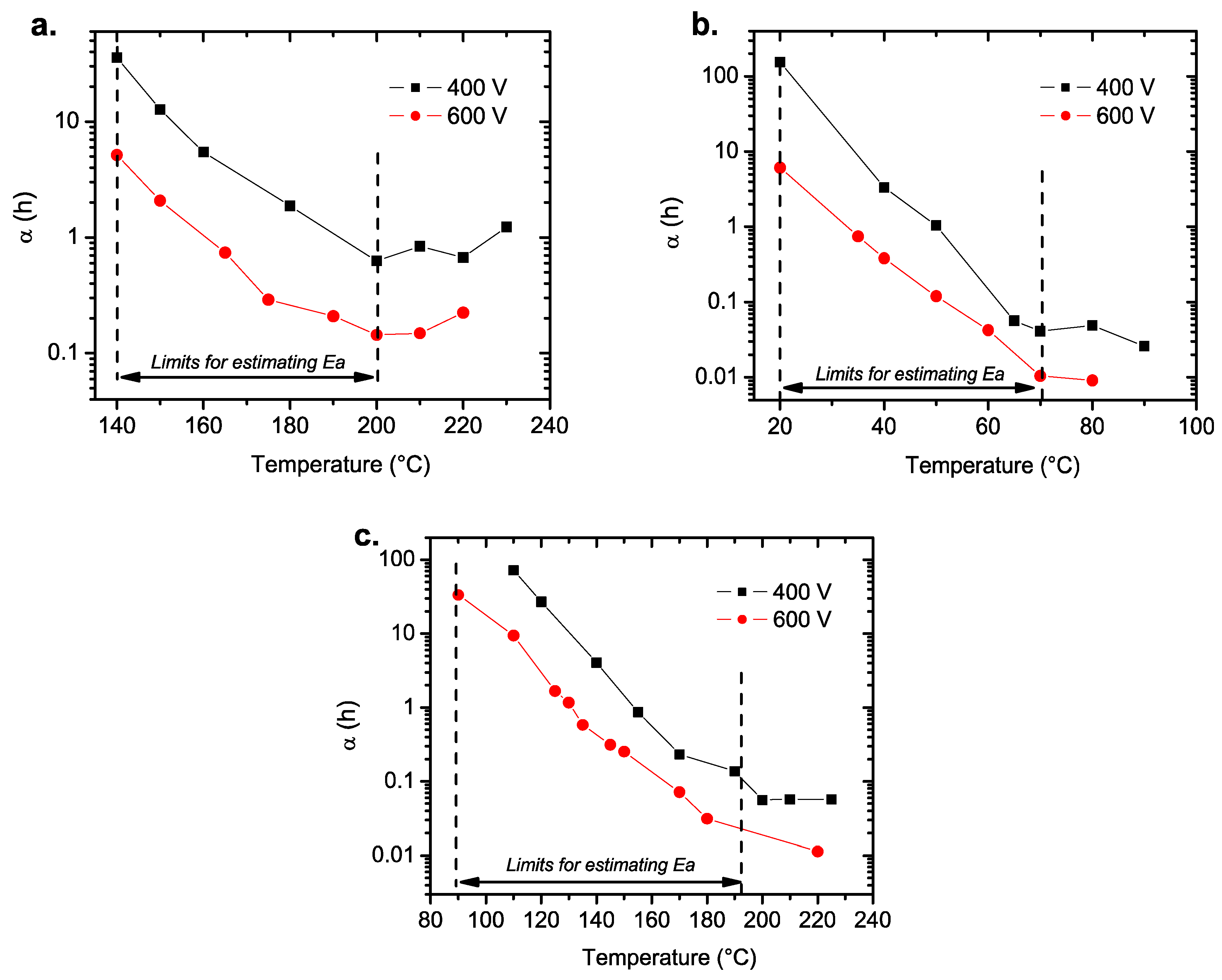
3.1. Initial HALT Test Results
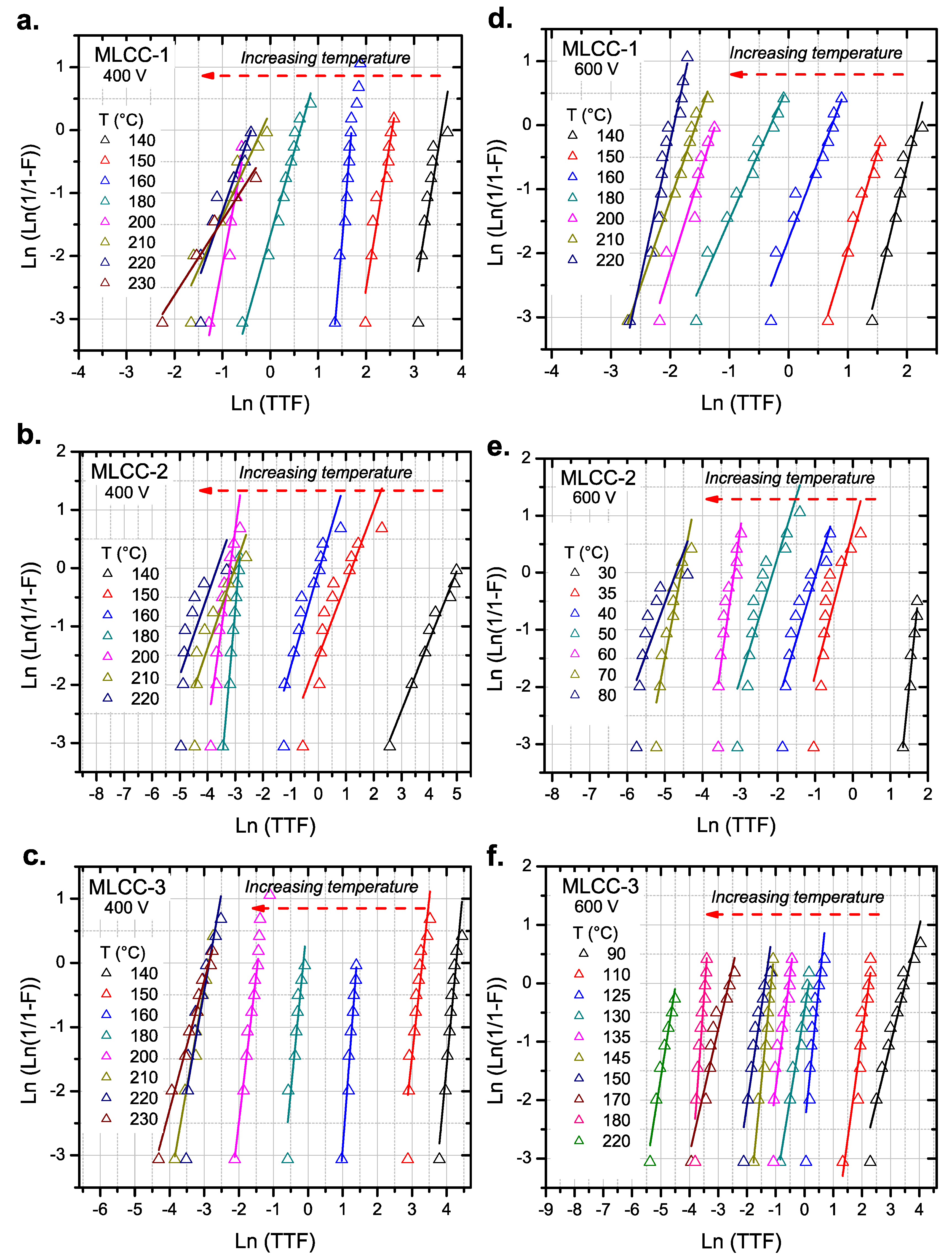
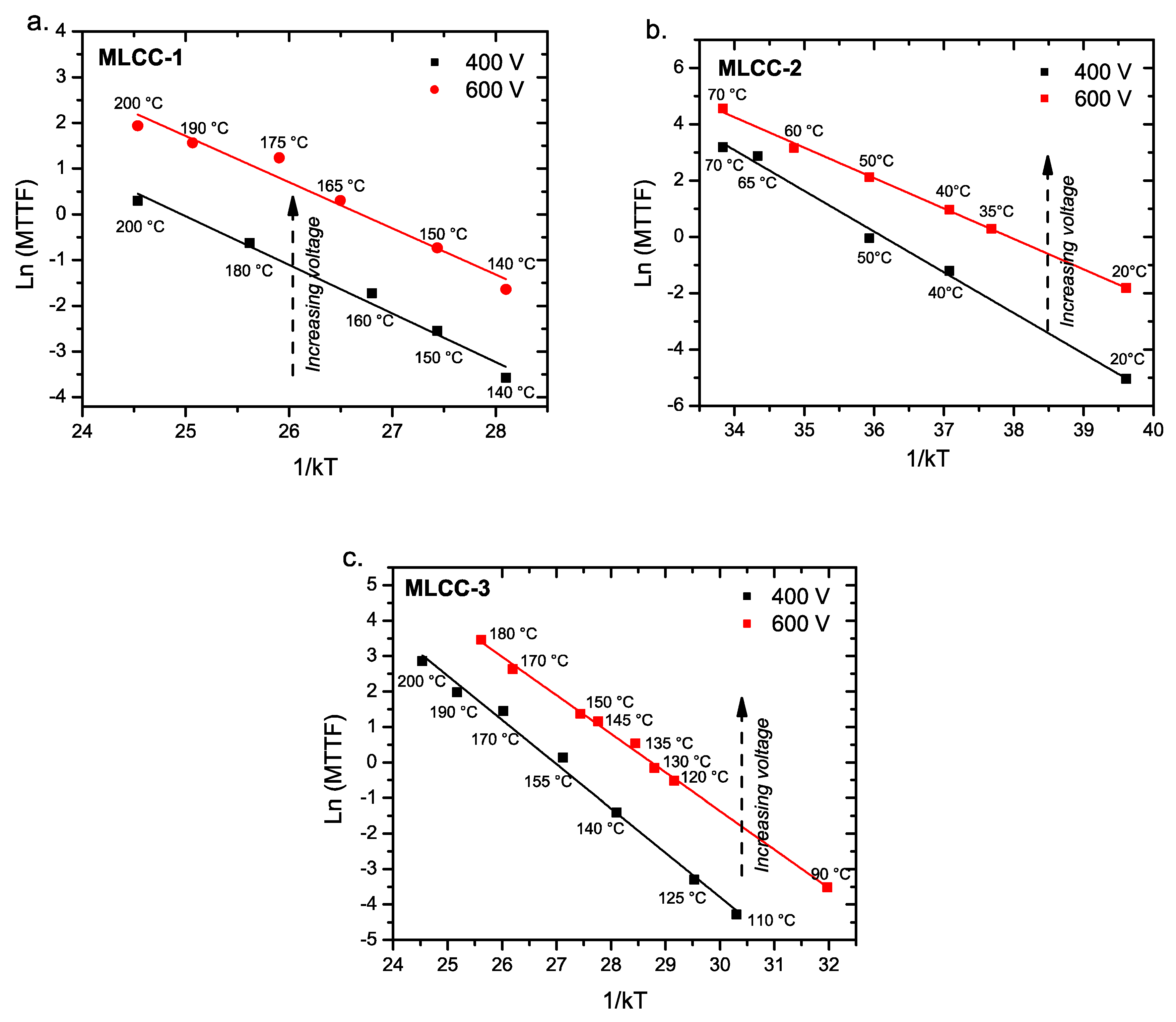
3.2. Activation Energies
3.3. Discussion on Activation Energies
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Foeller, P.Y.; Dean, J.S.; Reaney, I.M.; Sinclair, D.C. Design of a bilayer ceramic capacitor with low temperature coefficient of capacitance. Appl. Phys. Lett. 2016, 109, 082904. [Google Scholar] [CrossRef]
- Gurav, A.; Scruggs, C.; Turner, R.; Ashburn, T. Considerations for base-metal electrode (BME) ceramic capacitors for high reliability applications. In Proceedings of the CARTS International-ECA, Houston, Texas, USA, 25–28 March 2013. [Google Scholar]
- Tsur, Y.; Dunbar, T.D.; Randall, C.A. Crystal and defect chemistry of rare earth cations in BaTiO3. J. Electroceram. 2001, 7, 25–34. [Google Scholar] [CrossRef]
- Wang, M.-J.; Yang, H.; Zhang, Q.-L.; Hu, L.; Yu, D.; Lin, Z.-S.; Zhang, Z.-S. Doping behaviors of yttrium, zinc and gallium in BaTiO3 ceramics for AC capacitor application. J. Mater. Sci.: Mater. Electron. 2014, 25, 2905–2912. [Google Scholar] [CrossRef]
- Yoon, S.-H.; Park, Y.-S.; Hong, J.-O.; Sinn, D.-S. Effect of the pyrochlore (Y2Ti2O7) phase on the resistance degradation in yttrium-doped BaTiO3 ceramic capacitors. J. Mater. Res. 2011, 22, 2539–2543. [Google Scholar] [CrossRef]
- Ashburn, T.; Skamser, D. Highly Accelerated Testing of Capacitors for Medical Applications. In Proceedings of the 5th SMTA Medical Electronics Symposium, Anaheim, CA, USA, 29–30 January 2008. [Google Scholar]
- Liu, D. Highly accelerated life stress testing (HALST) of base-metal electrode multilayer ceramic capacitors. Proc. CARTS 2013, 235–248. [Google Scholar]
- Groebel, D.J.; Mettas, A.; Sun, F.-B. Determination and interpretation of activation energy using accelerated-test data. In Proceedings of the 2001 International Symposium on Product Quality and Integrity, Annual Reliability and Maintainability Symposium(RAMS-01), Philadelphia, PA, USA, 22–25 January 2001; pp. 58–63. [Google Scholar]
- Liu, D.D. Insulation Resistance Degradation in Ni-BaTiO3 Multilayer Ceramic Capacitors. IEEE Trans. Compon. Packag. Manuf. Technol. 2015, 5, 40–48. [Google Scholar] [CrossRef]
- Hartler, G. Parameter Estimation for the Arrhenius Model. Reliab. IEEE Trans. Reliab. 1986, 35, 414–418. [Google Scholar] [CrossRef]
- Maher, G. Highly Accelerated Life Testing (HALT) of K-4500 Low Fired X7R Dielectric. In Proceedings of the Passive Components for Power Electronics Workshop, Pittsburgh, PA, USA, 26–27 April 2000; pp. 26–27. [Google Scholar]
- Maher, G.H.; Wilson, J.M.; Maher, S.G. Electric Field Effects on the Insulation Resistance and Reliability of Various Types of BaTiO3 based X7R MLCC’s at Elevated Temperatures. In Proceedings of the 11th US-Japan Seminar, Sapporo, Japan, 9 September 2003. [Google Scholar]
- Randall, M.; Gurav, A.; Skamser, D.; Beeson, J. Lifetime modeling of sub 2 micron dielectric thickness BME MLCC. In Proceedings of the CARTS’2003, Scottsdale, AZ, USA, 31 March–3 April 2003; pp. 134–140. [Google Scholar]
- Yoon, S.-H.; Kang, S.-H.; Kwon, S.-H.; Hur, K.-H. Resistance degradation behavior of Ca-doped BaTiO3. J. Mater. Res. 2010, 25, 2135–2142. [Google Scholar] [CrossRef]
- Liu, G.; Roseman, R.D. Effect of BaO and SiO2 addition on PTCR BaTiO3 ceramics. J. Mater. Sci. 1999, 34, 4439–4445. [Google Scholar] [CrossRef]
- Öksüz, K.E.; Torman, M.; Şen, Ş.; Şen, U. Effect of sintering temperature on dielectric properties of SiO2 doped BaTiO3 ceramics. Mater. Methods Technol. 2016, 10, 361–366. [Google Scholar]
- Zhang, J.; Hou, Y.; Zheng, M.; Jia, W.; Zhu, M.; Yan, H. The Occupation Behavior of Y2O3 and Its Effect on the Microstructure and Electric Properties in X7R Dielectrics. J. Am. Ceram. Soc. 2016, 99, 1375–1382. [Google Scholar] [CrossRef]
- Wang, M.-J.; Yang, H.; Zhang, Q.-L.; Lin, Z.-S.; Zhang, Z.-S.; Yu, D.; Hu, L. Microstructure and dielectric properties of BaTiO3 ceramic doped with yttrium, magnesium, gallium and silicon for AC capacitor application. Mater. Res. Bull. 2014, 60, 485–491. [Google Scholar] [CrossRef]
- Yang, G.Y.; Lian, G.D.; Dickey, E.C.; Randall, C.A.; Barber, D.E.; Pinceloup, P.; Henderson, M.A.; Hill, R.A.; Beeson, J.J.; Skamser, D.J. Oxygen nonstoichiometry and dielectric evolution of BaTiO3. Part II—insulation resistance degradation under applied dc bias. J. Appl. Phys. 2004, 96, 7500–7508. [Google Scholar] [CrossRef]
- Liu, D.D.; Sampson, M.J. Some Aspects of the Failure Mechanisms in BaTiO3-Based Multilayer Ceramic Capacitors. In Proceedings of the CARTS International, Las Vegas, NV, USA, 26–29 March 2012. [Google Scholar]
- Nomura, T.; Kawano, N.; Yamatsu, J.; Aashi, T.; Nakano, Y.; Sato, A. Aging Behavior of Ni-Electrode Multilayer Ceramic Capacitors with X7R Characteristics. Jpn. J. Appl. Phys., Part 1. 1995, 34, 5389–5395. [Google Scholar] [CrossRef]
- Rawal, B.S.; Chan, N.H. Conduction and failure mechanisms in barium titanate based ceramics under highly accelerated conditions. In Proceedings of the 34th Electronic Components Conference, New Orleans, LA, USA, 16 May 1984; pp. 184–188. [Google Scholar]
- Yoon, J.-R.; Lee, K.-M.; Lee, S.-W. Analysis the Reliability of Multilayer Ceramic Capacitor with inner Ni Electrode under highly Accelerated Life Test Conditions. Trans. Electr. Electr. Mater. 2009, 10, 5–8. [Google Scholar] [CrossRef]
- Chazono, H.; Kishi, H. DC-Electrical Degradation of the BT-Based Material for Multilayer Ceramic Capacitor with Ni internal Electrode: Impedance Analysis and Microstructure. Jpn. J. Appl. Phys. 2001, 40, 5624–5629. [Google Scholar] [CrossRef]
- Chan, N.H.; Sharma, R.K.; Smyth, D.M. Nonstoichiometry in Undoped BaTiO3. J. Am. Ceram. Soc. 1981, 64, 556–562. [Google Scholar] [CrossRef]
- Han, Y.H.; APPLEBY, J.B.; Smyth, D.M. Calcium as an Acceptor Impurity in BaTiO3. J. Am. Ceram. Soc. 1987, 70, 96–100. [Google Scholar] [CrossRef]
- Sakabe, Y.; Wada, N.; Hiramatsu, T.; Tonogaki, T. Dielectric Properties of Fine-Grained BaTiO3 Ceramics Doped with CaO. Jpn. J. Appl. Phys. 2002, 41, 6922–6925. [Google Scholar] [CrossRef]
- Nakamura, T.; Yao, T.; Ikeda, J.; Kubodera, N.; Takagi, H. Improvement of the Reliability of Dielectrics for MLCC. IOP Conf. Ser.: Mater. Sci. Eng. 2011, 18, 092007. [Google Scholar] [CrossRef]
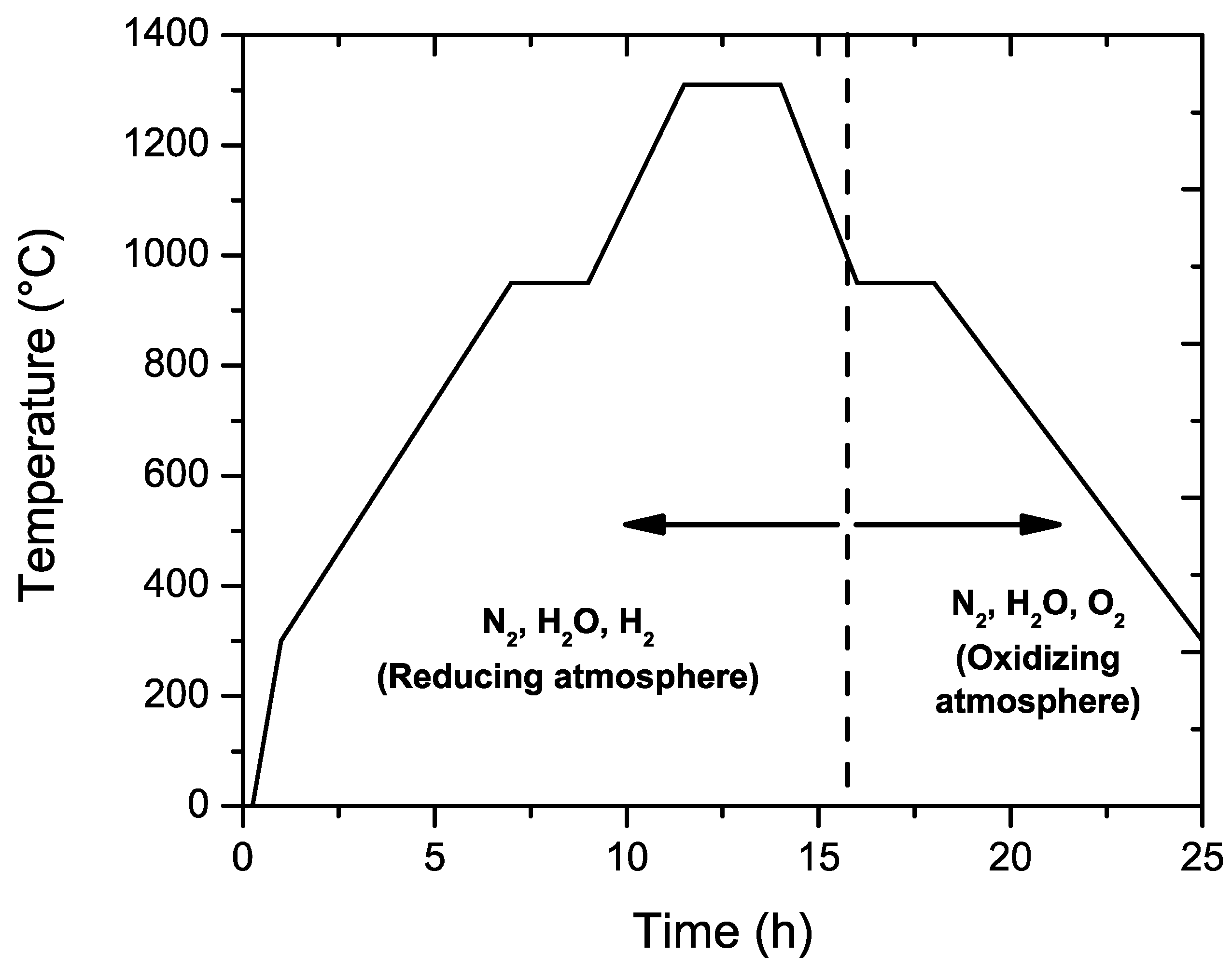
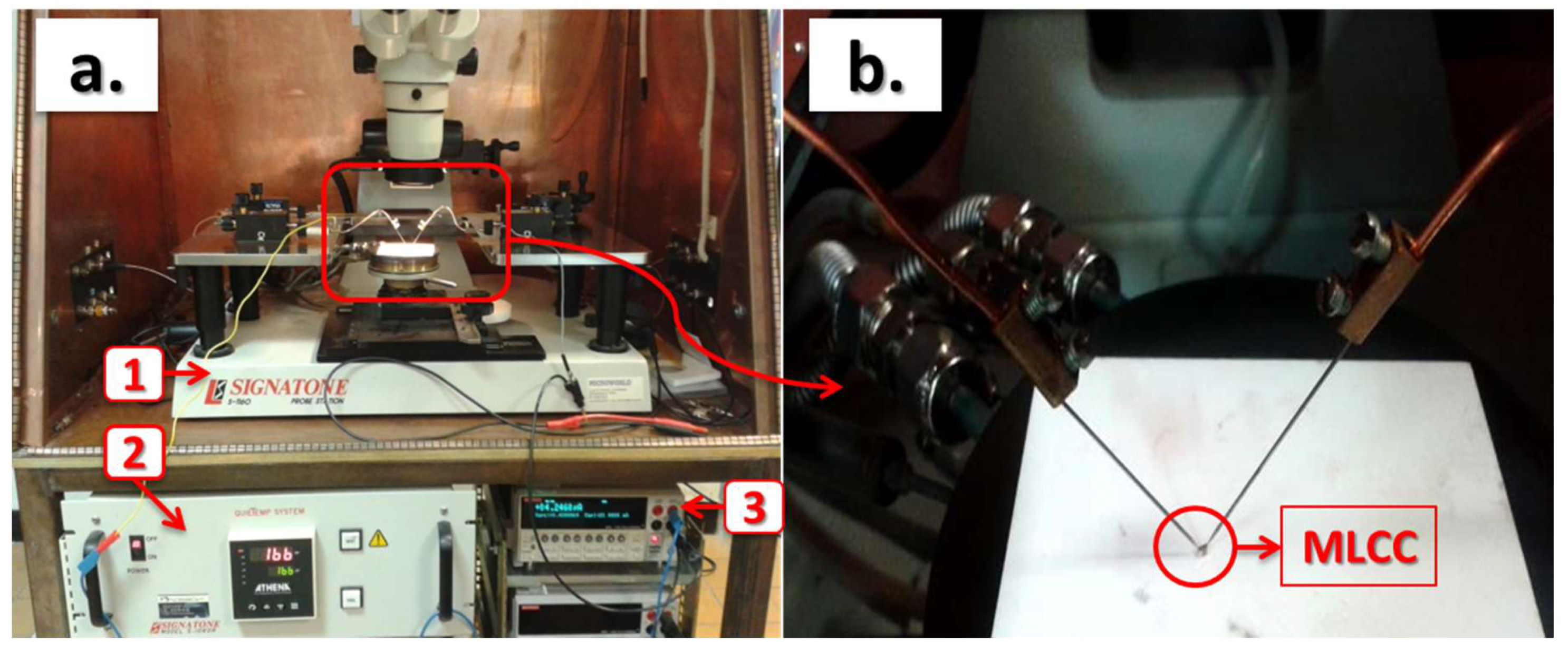
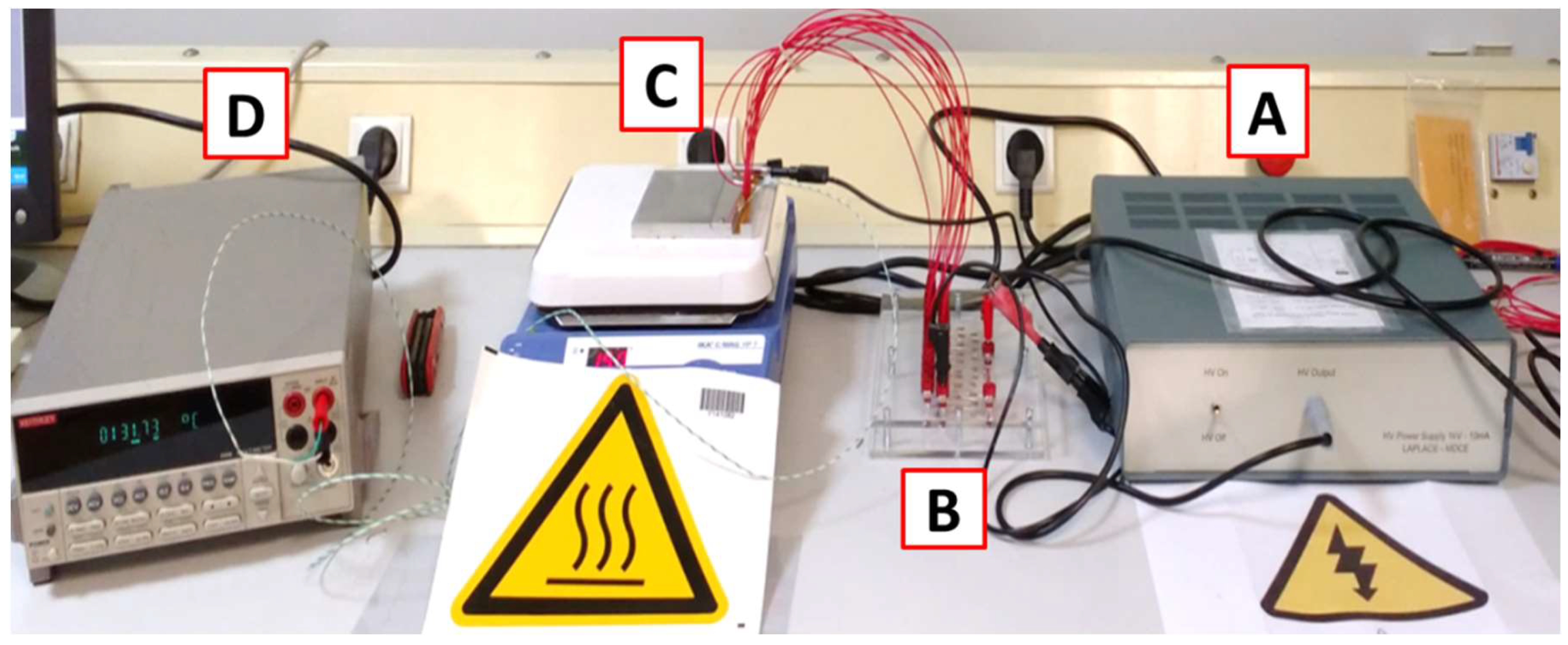
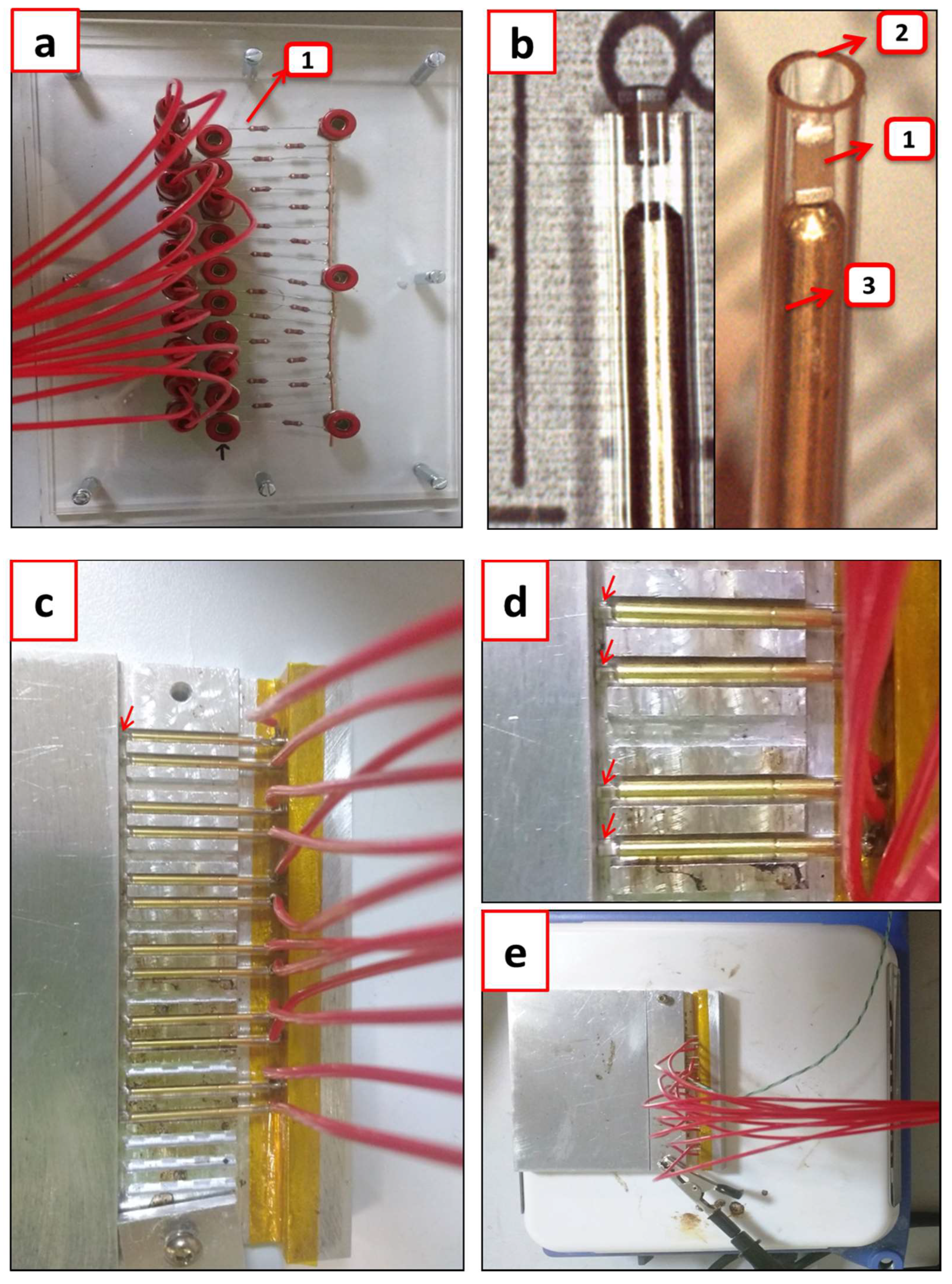

| Element | MLCC-1 | MLCC-2 | MLCC-3 |
|---|---|---|---|
| Ba/Ti ratio | 2.88 | 2.86 | 2.85 |
| Y wt% | 1.05 | 1.05 | 1.05 |
| Ca wt% | < 0.01 (≈ 0.01 mol%) | 0.5–0.6 (≈ 2.8 mol%) | 1.3–1.5 (≈ 8.1 mol%) |
| Sample Type | Time to Breakdown at 140 °C and 400 V |
|---|---|
| MLCC-1 | 37 h |
| MLCC-2 | <1 s |
| MLCC-3 | 3.5 h |
| MLCCs | Ea (eV) at 400 V | Ea (eV) at 600 V | ln(A) at 400 V | ln(A) at 600 V | Temperature Range at 400 V | Temperature Range at 600 V |
|---|---|---|---|---|---|---|
| MLCC-1 | 1.06 ± 0.07 | 1.01 ± 0.09 | 26.96 ± 2.5 | 26.52 ± 2.1 | 140–200 °C | 120–200 °C |
| MLCC-2 | 1.45 ± 0.05 | 1.08 ± 0.02 | 39.90 ± 2.9 | 38.30 ± 1.8 | 20–70 °C | 20–70 °C |
| MLCC-3 | 1.25 ± 0.06 | 1.09 ± 0.02 | 33.62 ± 1.3 | 31.23 ± 0.7 | 110–200 °C | 90–180 °C |
| MLCCs | n (T1 = T2) | n (All Conditions) |
|---|---|---|
| MLCC-1 | 5.51 | 4.46 |
| MLCC-2 | 4.95 | 3.26 |
| MLCC-3 | 1.93 | 5.02 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hernández-López, A.M.; Aguilar-Garib, J.A.; Guillemet-Fritsch, S.; Nava-Quintero, R.; Dufour, P.; Tenailleau, C.; Durand, B.; Valdez-Nava, Z. Reliability of X7R Multilayer Ceramic Capacitors During High Accelerated Life Testing (HALT). Materials 2018, 11, 1900. https://doi.org/10.3390/ma11101900
Hernández-López AM, Aguilar-Garib JA, Guillemet-Fritsch S, Nava-Quintero R, Dufour P, Tenailleau C, Durand B, Valdez-Nava Z. Reliability of X7R Multilayer Ceramic Capacitors During High Accelerated Life Testing (HALT). Materials. 2018; 11(10):1900. https://doi.org/10.3390/ma11101900
Chicago/Turabian StyleHernández-López, Ana María, Juan Antonio Aguilar-Garib, Sophie Guillemet-Fritsch, Roman Nava-Quintero, Pascal Dufour, Christophe Tenailleau, Bernard Durand, and Zarel Valdez-Nava. 2018. "Reliability of X7R Multilayer Ceramic Capacitors During High Accelerated Life Testing (HALT)" Materials 11, no. 10: 1900. https://doi.org/10.3390/ma11101900
APA StyleHernández-López, A. M., Aguilar-Garib, J. A., Guillemet-Fritsch, S., Nava-Quintero, R., Dufour, P., Tenailleau, C., Durand, B., & Valdez-Nava, Z. (2018). Reliability of X7R Multilayer Ceramic Capacitors During High Accelerated Life Testing (HALT). Materials, 11(10), 1900. https://doi.org/10.3390/ma11101900





