Efficiency of True-Green Light Emitting Diodes: Non-Uniformity and Temperature Effects
Abstract
:1. Introduction
2. Experimental
2.1. Samples and Characterization Techniques
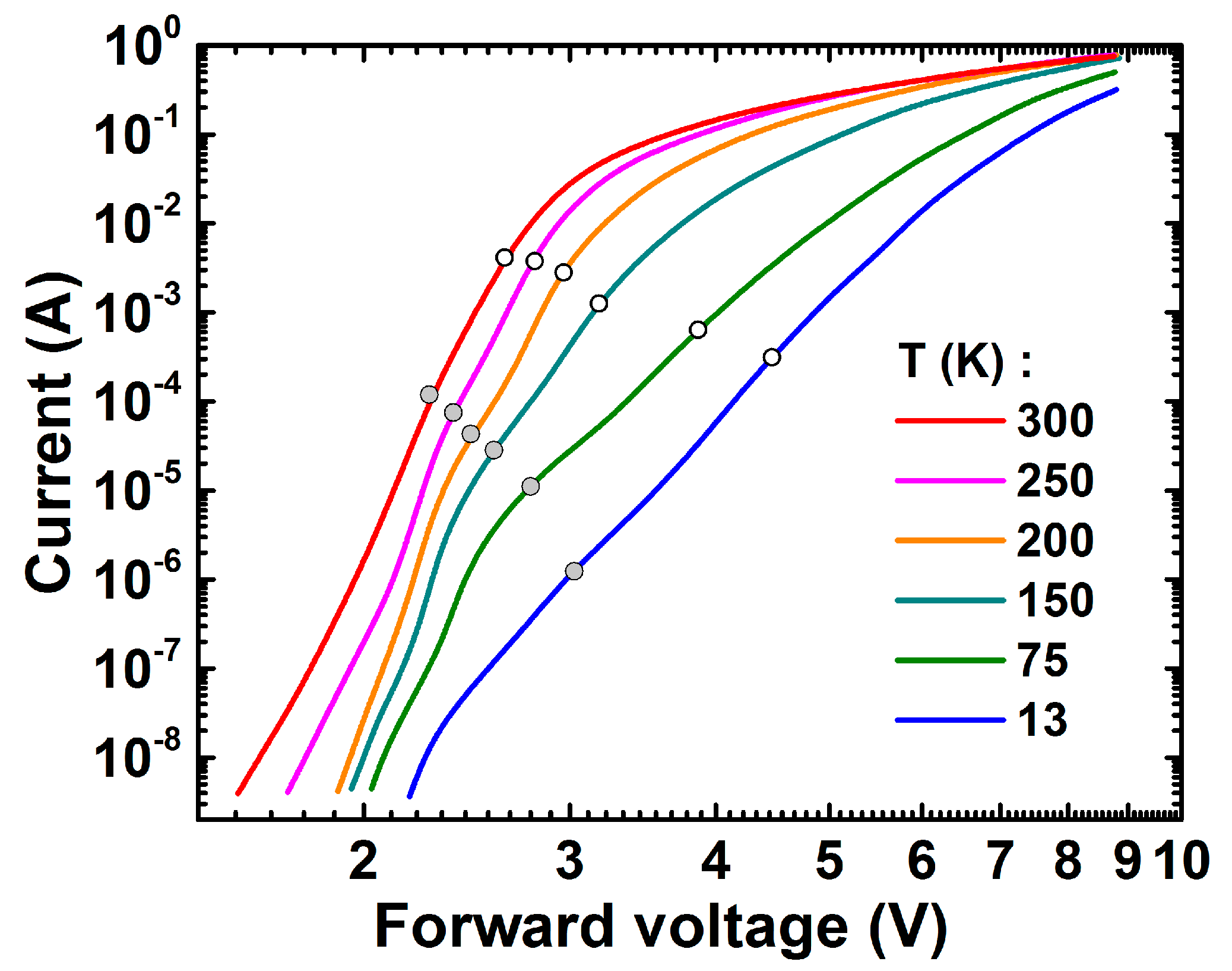
2.2. Current-Voltage Characteristics
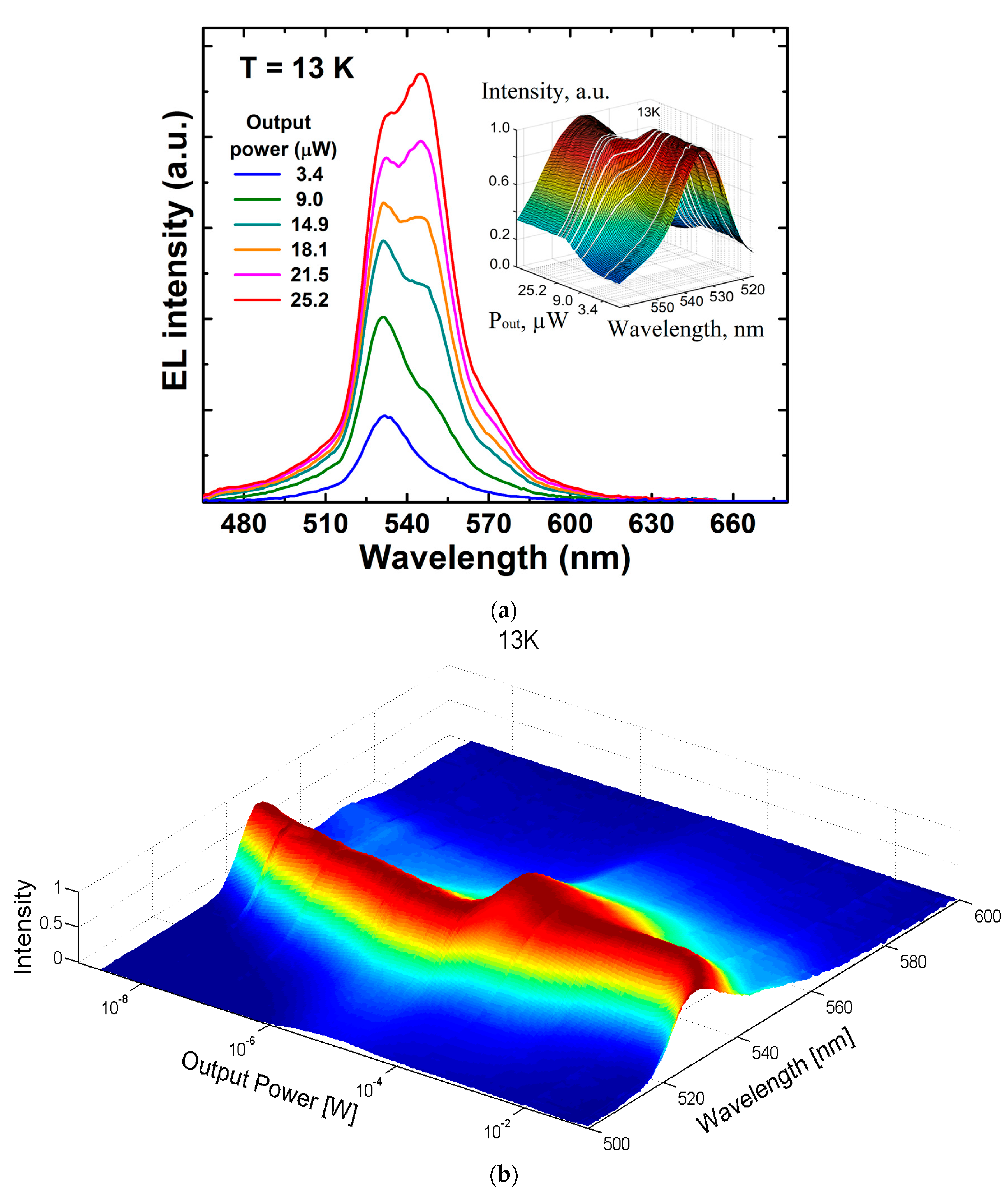
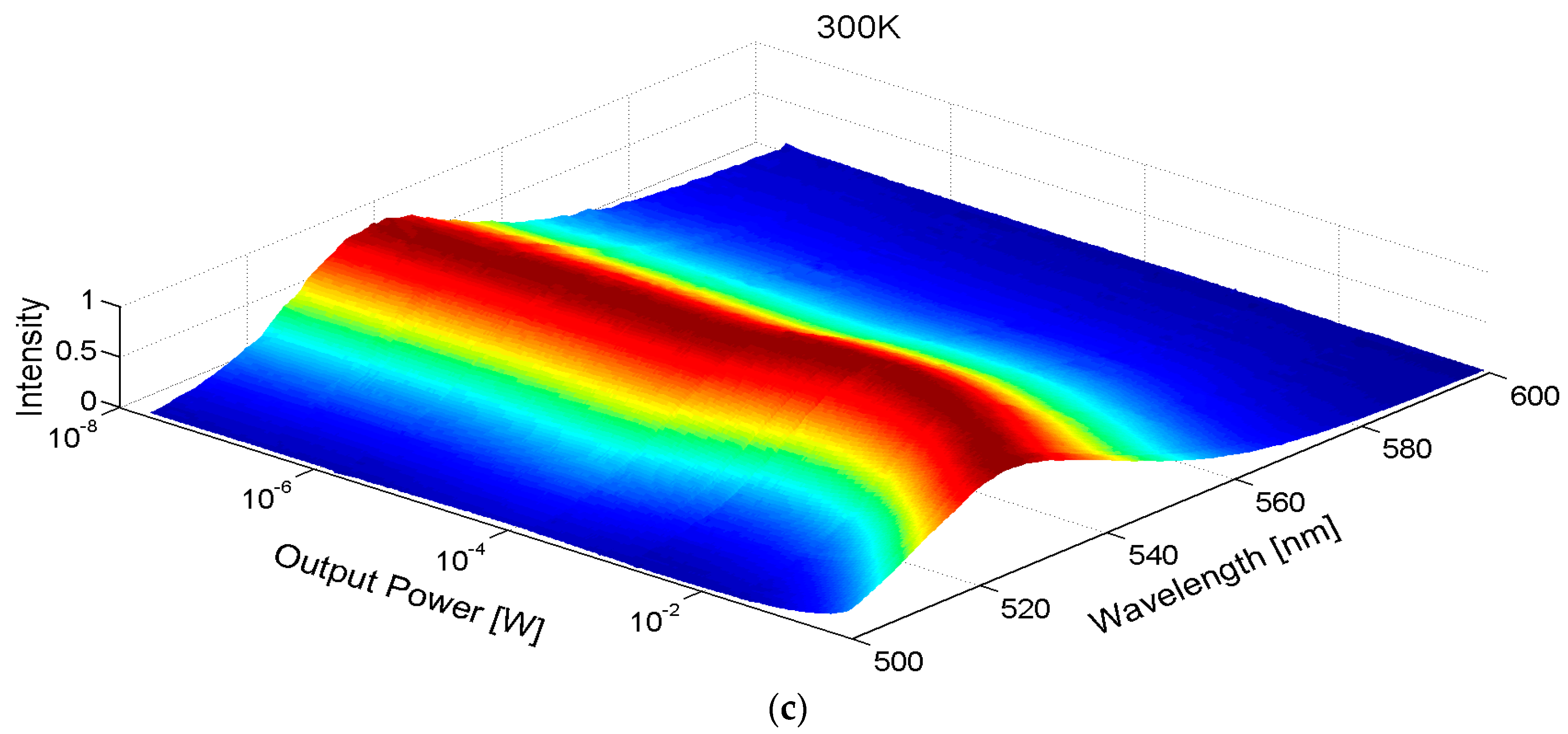
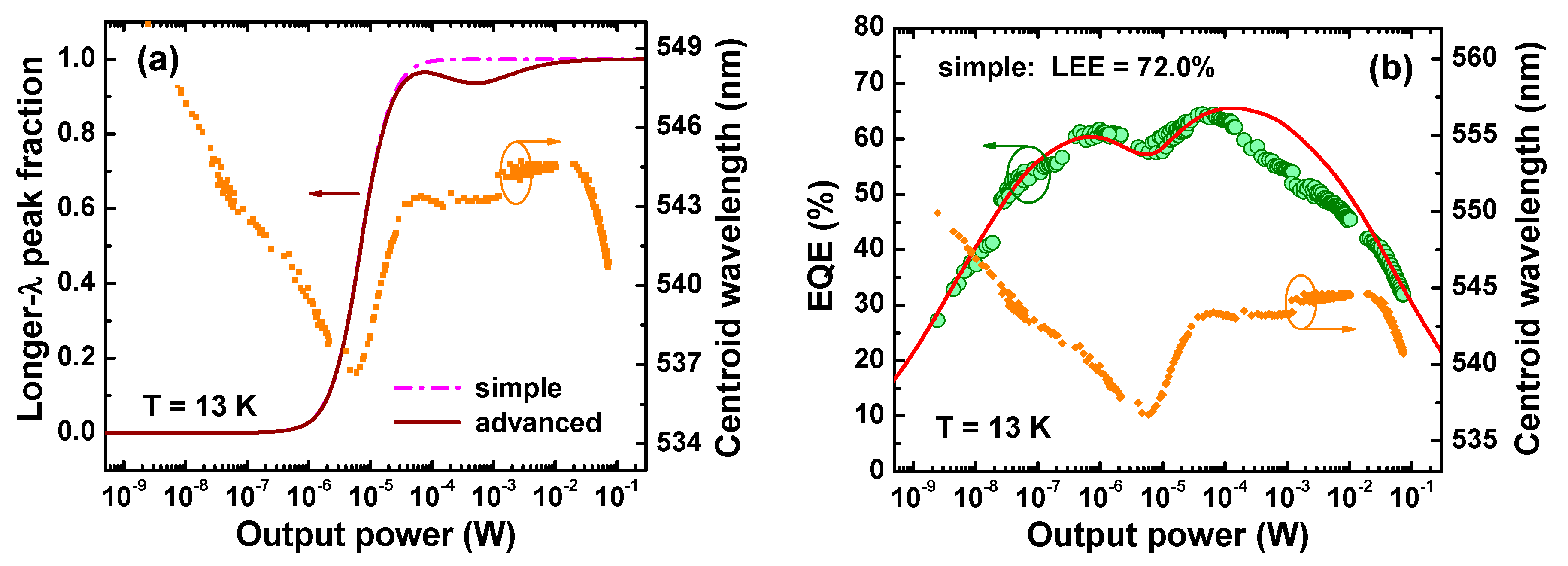
2.3. Emission Spectra
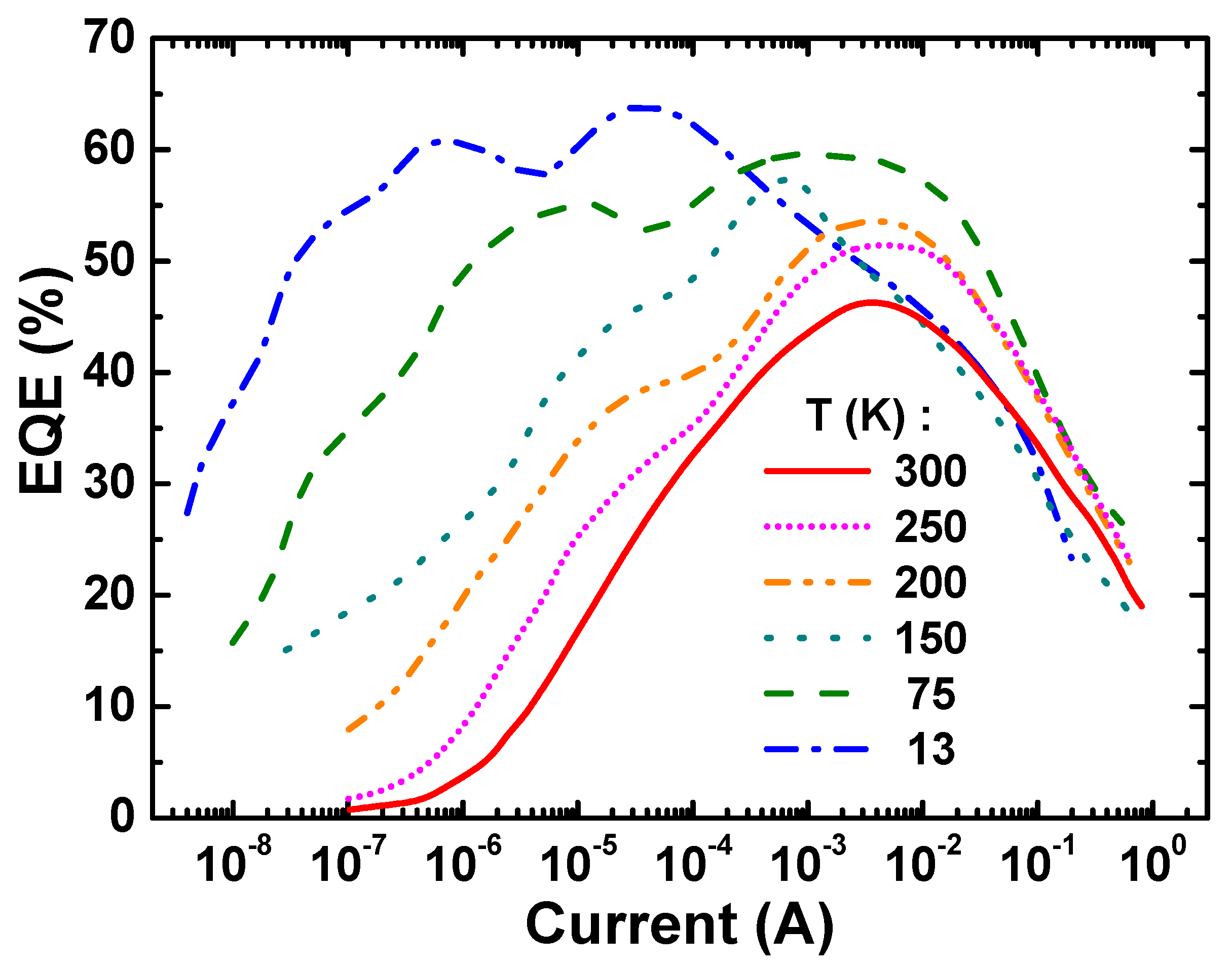
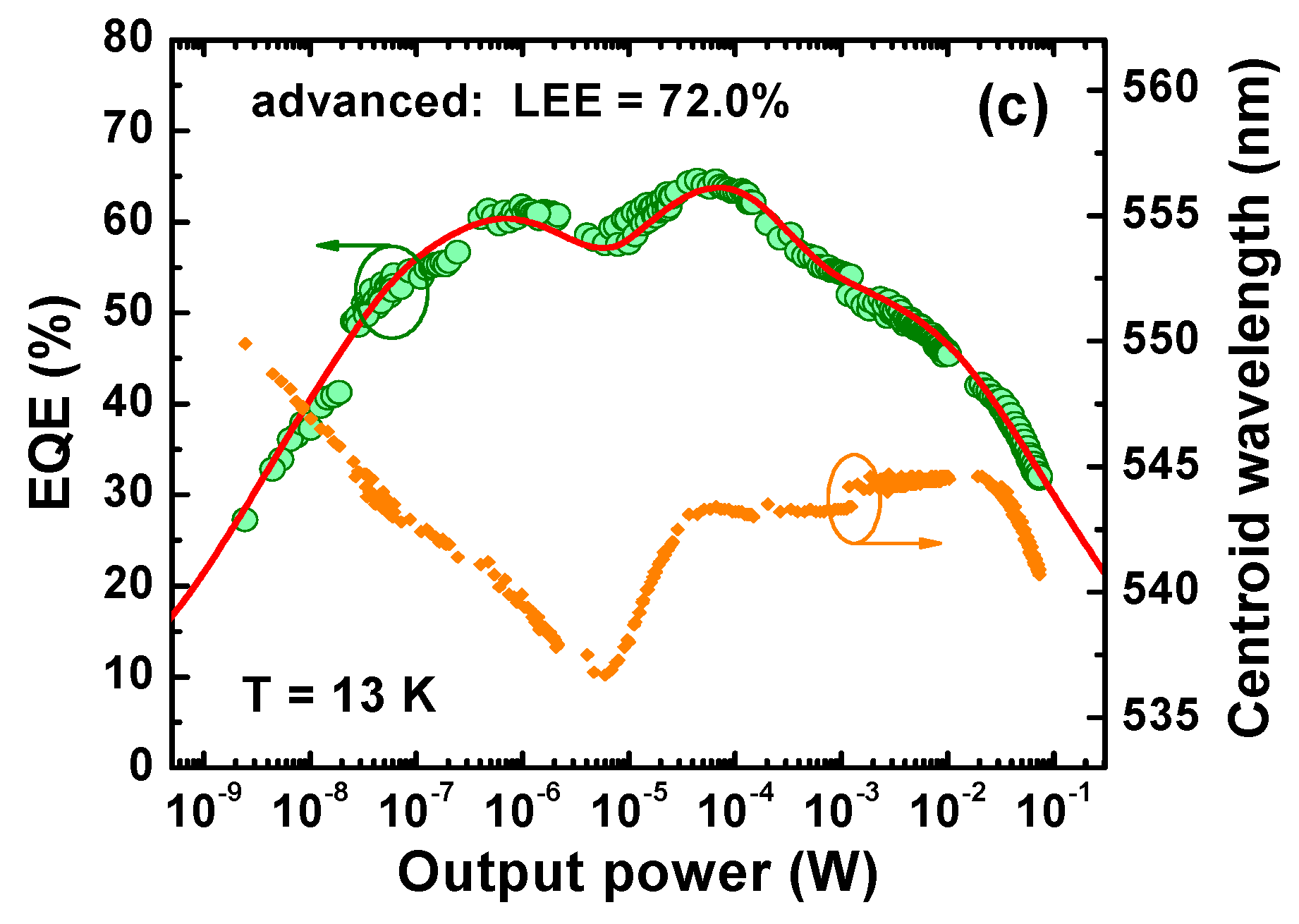
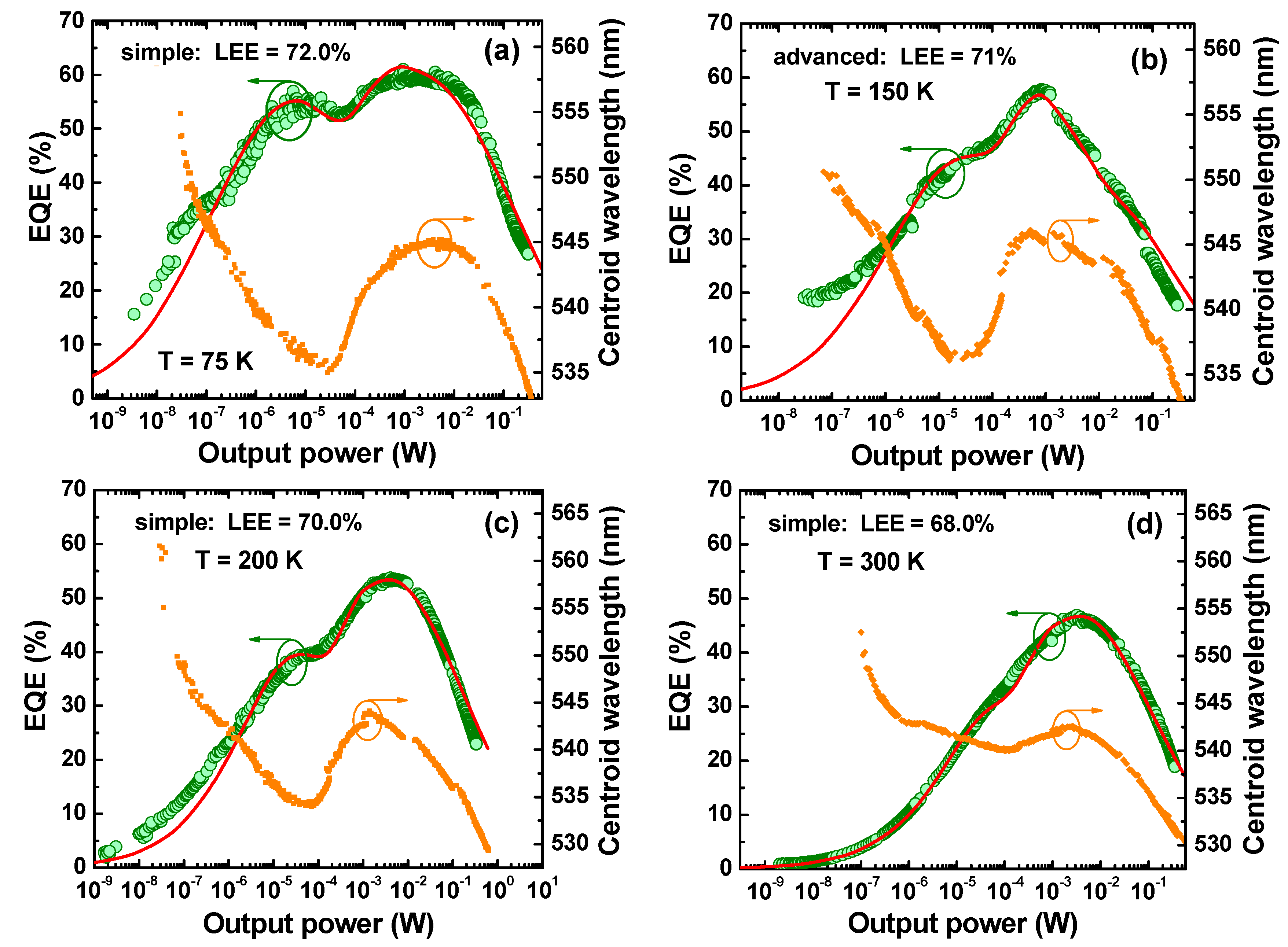
2.4. Emission Efficiency
3. Modeling
3.1. Model
3.2. Model Application
4. Discussion
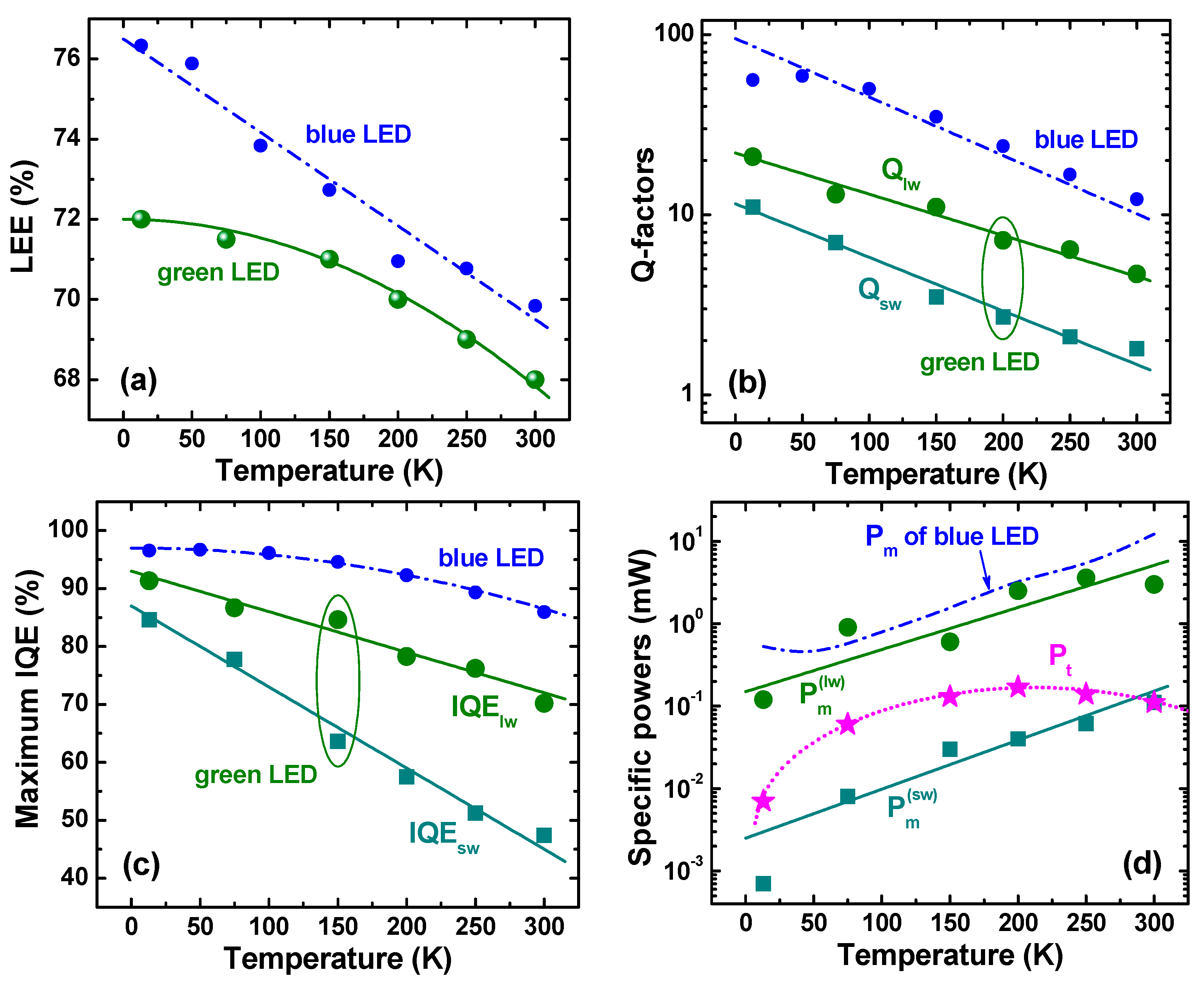
4.1. Light Extraction Efficiency
4.2. Quality Factors and Internal Quantum Efficiency
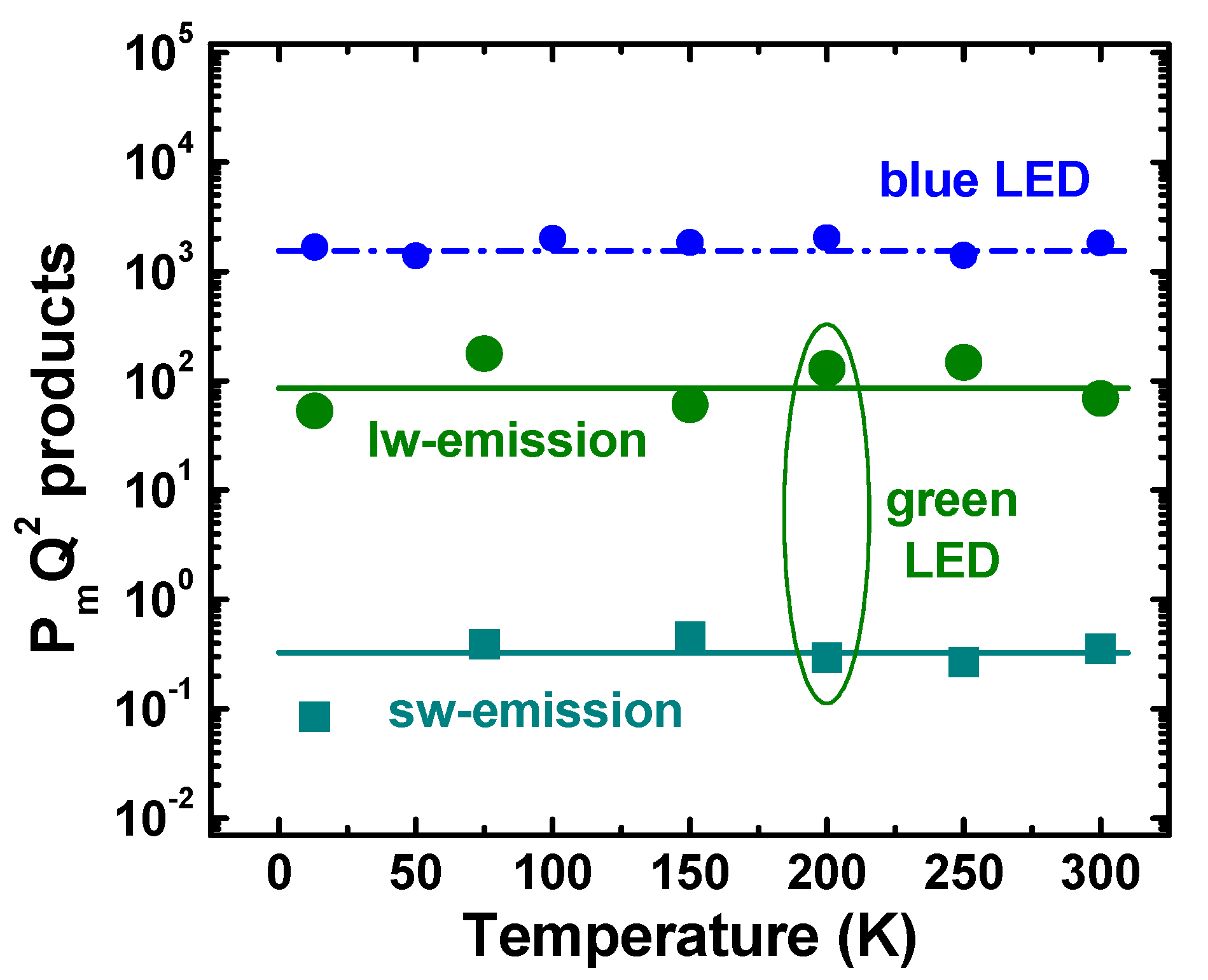
4.3. Specific Powers and Recombination Volumes
4.4. Possible Origins of Active Region Non-Uniformity
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Abbreviations
| AR | Active region |
| EQE | External quantum efficiency |
| EL | Electroluminescence |
| IQE | Internal quantum efficiency |
| LED | Light-emitting diode |
| LEE | Light extraction efficiency |
| LO | Longitudinal optical |
| MQW | Multiple quantum well |
| ND | Neutral density |
| QW | Quantum well |
| RT | Room temperature |
| SQW | Single quantum well |
| WPE | Wall plug efficiency |
References
- Zheludev, N. The life and times of the LED a 100-year history. Nat. Photonics 2007, 1, 189–192. [Google Scholar] [CrossRef]
- Crawford, M.H. LEDs for Solid-State Lighting: Performance Challenges and Recent Advances. IEEE J. Sel. Top. Quantum Electron. 2009, 15, 1028–1040. [Google Scholar] [CrossRef]
- Weisbuch, C.; Piccardo, M.; Martinelli, L.; Iveland, J.; Peretti, J.; Speck, J.S. The efficiency challenge of nitride light-emitting diodes for lighting. Phys. Status Solidi A 2015, 212, 899–913. [Google Scholar] [CrossRef]
- Broell, M.; Sundgren, P.; Rudolph, A.; Schmidt, W.; Vogl, A.; Behringer, M. New developments on high efficiency infrared and InGaAlP light emitting diodes at OSRAM Opto-Semiconductors. Proc. SPIE 2014. [Google Scholar] [CrossRef]
- Titkov, I.E.; Karpov, S.Y.; Yadav, A.; Zerova, V.L.; Zulonas, M.; Galler, B.; Strassburg, M.; Pietzonka, I.; Lugauer, H.-J.; Rafailov, E.U. Temperature-Dependent Internal Quantum Efficiency of Blue High-Brightness Light-Emitting Diodes. IEEE J. Quantum Electron. 2014, 50, 911–920. [Google Scholar] [CrossRef]
- Karpov, S.Y.; Binder, M.; Galler, B.; Schiavon, D. Spectral dependence of light extraction efficiency of high-power III-nitride light-emitting diodes. Phys. Status Solidi RRL 2015, 1, 321–326. [Google Scholar] [CrossRef]
- Peter, M.; Laubsch, A.; Bergbauer, W.; Meyer, T.; Sabathil, M.; Baur, J.; Hahn, B. New developments in green LEDs. Phys. Status Solidi A 2007, 1, 1125–1129. [Google Scholar] [CrossRef]
- Shin, D.-S.; Han, D.-P.; Oh, J.-Y.; Shim, J.-I. Study of droop phenomena in InGaN-based blue and green light-emitting diodes by temperature-dependent electroluminescence. Appl. Phys. Lett. 2012, 100, 153506. [Google Scholar] [CrossRef]
- Dai, Q.; Shan, Q.; Cho, J.; Schubert, E.F.; Crawford, M.H.; Koleske, D.D.; Kim, M.-H.; Park, Y. On the symmetry of efficiency-versus-carrier-concentration curves in GaInN/GaN light-emitting diodes and relation to droop-causing mechanisms. Appl. Phys. Lett. 2011, 98, 033506. [Google Scholar] [CrossRef]
- Lin, G.-B.; Meyaard, D.; Cho, J.; Schubert, E.F.; Shim, H.; Sone, C. Analytic model for the efficiency droop in semiconductors with asymmetric carrier-transport properties based on drift-induced reduction of in-jection efficiency. Appl. Phys. Lett. 2012, 100, 161106. [Google Scholar] [CrossRef]
- Wang, J.; Wang, L.; Wang, L.; Hao, Z.; Luo, Y.; Dempewolf, A.; Müller, M.; Bertram, F.; Christen, J. An improved carrier rate model to evaluate internal quantum efficiency and analyze efficiency droop origin of InGaN based light-emitting diodes. Appl. Phys. Lett. 2012, 102, 023107. [Google Scholar] [CrossRef]
- Kisin, M.V.; El-Ghoroury, H.S. Inhomogeneous injection in III-nitride light emitters with deep multiple quantum wells. J. Comput. Electron. 2015, 14, 432–443. [Google Scholar] [CrossRef]
- Karpov, S.Y. Effect of localized states on internal quantum efficiency of III-nitride LEDs. Phys. Status Solidi RRL 2010, 4, 320–322. [Google Scholar] [CrossRef]
- Laubsch, A.; Sabathil, M.; Baur, J.; Peter, M.; Hahn, B. High-Power and High-Efficiency InGaN-Based Light Emitters. IEEE Trans. Electron. Devices 2010, 57, 79–87. [Google Scholar] [CrossRef]
- Shah, J.M.; Li, Y.-L.; Gessmann, T.; Schubert, E.F. Experimental analysis and theoretical model for anomalously high ideality factors (n_2.0) in Al-GaN/GaN p-n junction diodes. J. Appl. Phys. 2003, 94, 2627–2630. [Google Scholar] [CrossRef]
- Masui, H. Diode ideality factor in modern light-emitting diodes. Semicond. Sci. Technol. 2011, 26, 075011. [Google Scholar] [CrossRef]
- Cao, X.A.; LeBoeuf, S.F.; Rowland, L.B.; Liu, H. Temperature-Dependent Electroluminescence in InGaN/GaN Multiple-Quantum-Well Light-Emitting Diodes. J. Electron. Mater. 2003, 32, 316–321. [Google Scholar] [CrossRef]
- Karpov, S.Y. ABC-model for interpretation of internal quantum efficiency and its droop in III-nitride LEDs: A review. Opt. Quant. Electron. 2015, 47, 1293–1303. [Google Scholar] [CrossRef]
- Karpov, S.Y.; Cherkashin, N.A.; Lundin, W.V.; Nikolaev, A.E.; Sakharov, A.V.; Sinitsin, M.A.; Usov, S.O.; Zavarin, E.E.; Tsatsulnikov, A.F. Multi-color monolithic III-nitride light-emitting diodes: Factors controlling emission spectra and efficiency. Phys. Status Solidi A 2016, 213, 19–29. [Google Scholar] [CrossRef]
- Langer, T.; Jönen, H.; Kruse, A.; Bremers, H.; Rossow, U.; Hangleiter, A. Strain-induced defects as nonradiative recombination centers in green-emitting GaInN/GaN quantum well structures. Appl. Phys. Lett. 2013, 103, 022108. [Google Scholar] [CrossRef]
- Hammersley, S.; Kappers, M.J.; Massabuau, F.C.-P.; Sahonta, S.-L.; Dawson, P.; Oliver, R.A.; Humphreys, C.J. Effects of quantum well growth temperature on the recombination efficiency of InGaN/GaN multiple quantum wells that emit in the green and blue spectral regions. Appl. Phys. Lett. 2015, 107, 132106. [Google Scholar] [CrossRef]
- Der Maur, M.A.; Pecchia, A.; Penazzi, G.; Rodrigues, W.; Di Carlo, A. Efficiency Drop in Green InGaN/GaN Light Emitting Diodes: The Role of Random Alloy Fluctuations. Phys. Rev. Lett. 2016, 116, 027401. [Google Scholar] [CrossRef] [PubMed]
- Nippert, F.; Karpov, S.Y.; Callsen, G.; Galler, B.; Kure, T.; Nenstiel, C.; Wagner, M.R.; Straßburg, M.; Lugauer, H.-J.; Hoffmann, A. Temperature-dependent recombination coefficients in InGaN light-emitting diodes: Hole lo-calization, Auger processes, and the green gap. Appl. Phys. Lett. 2016, 109, 61103. [Google Scholar] [CrossRef]
- Karpov, S.Y. Carrier localization in InGaN by composition fluctuations: Implication to “green gap”. Photonics Res. 2017, 5, A7–A12. [Google Scholar] [CrossRef]
- Schiavon, D.; Binder, M.; Peter, M.; Galler, B.; Drechsel, P.; Scholz, F. Wave-length-dependent determination of the recombination rate coefficients in single-quantum-well GaInN/GaN light emitting diodes. Phys. Status Solidi B 2013, 250, 283–290. [Google Scholar] [CrossRef]
- SiLENSe—Software Tool for Light Emitting Diode (LED) Bandgap Engineering. Available online: http://www.str-soft.com/products/SiLENSe/index.htm (accessed on 3 July 2017).
- Lobanova, A.V.; Kolesnikova, A.L.; Romanov, A.E.; Karpov, S.Y.; Rudinsky, M.E.; Yakovlev, E.V. Mechanism of stress relaxation in (0001) InGaN/GaN via formation of V-shaped dislocation half-loops. Appl. Phys. Lett. 2013, 103, 152106. [Google Scholar] [CrossRef]
- Sakharov, A.V.; Lundin, W.V.; Zavarin, E.E.; Sinitsyn, M.A.; Nikolaev, A.E.; Usov, S.O.; Sizov, V.S.; Mikhailovsky, G.A.; Cherkashin, N.A.; Hytch, M.; et al. Effect of strain relaxation on formation of active region of InGaN/(Al)GaN heterostructures for green range LEDs. Semiconductors 2009, 43, 812–817. [Google Scholar] [CrossRef]
- Galler, B.; Laubsch, A.; Wojcik, A.; Lugauer, H.; Gomez-Iglesias, A.; Sabathil, M.; Hahn, B. Investigation of the carrier distribution in InGaN-based multi-quantum-well structures. Phys. Status Solidi C 2011, 8, 2372–2374. [Google Scholar] [CrossRef]
- Hangleiter, A.; Hitzel, F.; Netzel, C.; Fuhrmann, D.; Rossow, U.; Ade, G.; Hinze, P. Suppression of Nonradiative Recombination by V-Shaped Pits in GaInN/GaN Quantum Wells Produces a Large Increase in the Light Emission Efficiency. Phys. Rev. Lett. 2005, 95, 127402. [Google Scholar] [CrossRef] [PubMed]
- Danhof, J.; Solowan, H.-M.; Schwarz, U.T.; Kaneta, A.; Kawakami, Y.; Schiavon, D.; Meyer, T.; Peter, M. Lateral charge carrier diffusion in InGaN quantum wells. Phys. Status Solidi B 2012, 249, 480–484. [Google Scholar] [CrossRef]
- Li, C.-K.; Wu, C.-K.; Hsu, C.-C.; Lu, L.-S.; Li, H.; Lu, T.-C.; Wu, Y.-R. 3D numerical modeling of the carrier transport and radiative efficiency for InGaN/GaN light emitting diodes with V-shaped pits. AIP Adv. 2016, 6, 055208. [Google Scholar] [CrossRef]
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Titkov, I.E.; Karpov, S.Y.; Yadav, A.; Mamedov, D.; Zerova, V.L.; Rafailov, E. Efficiency of True-Green Light Emitting Diodes: Non-Uniformity and Temperature Effects. Materials 2017, 10, 1323. https://doi.org/10.3390/ma10111323
Titkov IE, Karpov SY, Yadav A, Mamedov D, Zerova VL, Rafailov E. Efficiency of True-Green Light Emitting Diodes: Non-Uniformity and Temperature Effects. Materials. 2017; 10(11):1323. https://doi.org/10.3390/ma10111323
Chicago/Turabian StyleTitkov, Ilya E., Sergey Yu. Karpov, Amit Yadav, Denis Mamedov, Vera L. Zerova, and Edik Rafailov. 2017. "Efficiency of True-Green Light Emitting Diodes: Non-Uniformity and Temperature Effects" Materials 10, no. 11: 1323. https://doi.org/10.3390/ma10111323
APA StyleTitkov, I. E., Karpov, S. Y., Yadav, A., Mamedov, D., Zerova, V. L., & Rafailov, E. (2017). Efficiency of True-Green Light Emitting Diodes: Non-Uniformity and Temperature Effects. Materials, 10(11), 1323. https://doi.org/10.3390/ma10111323





