Numerical Simulation of CO2 Injection and Extraction Heat Transfer in Complex Fracture Networks
Abstract
1. Introduction
2. THM Theoretical Modeling
2.1. Basic Assumptions
2.2. THM Governing Equations
3. Numerical Computational Modeling
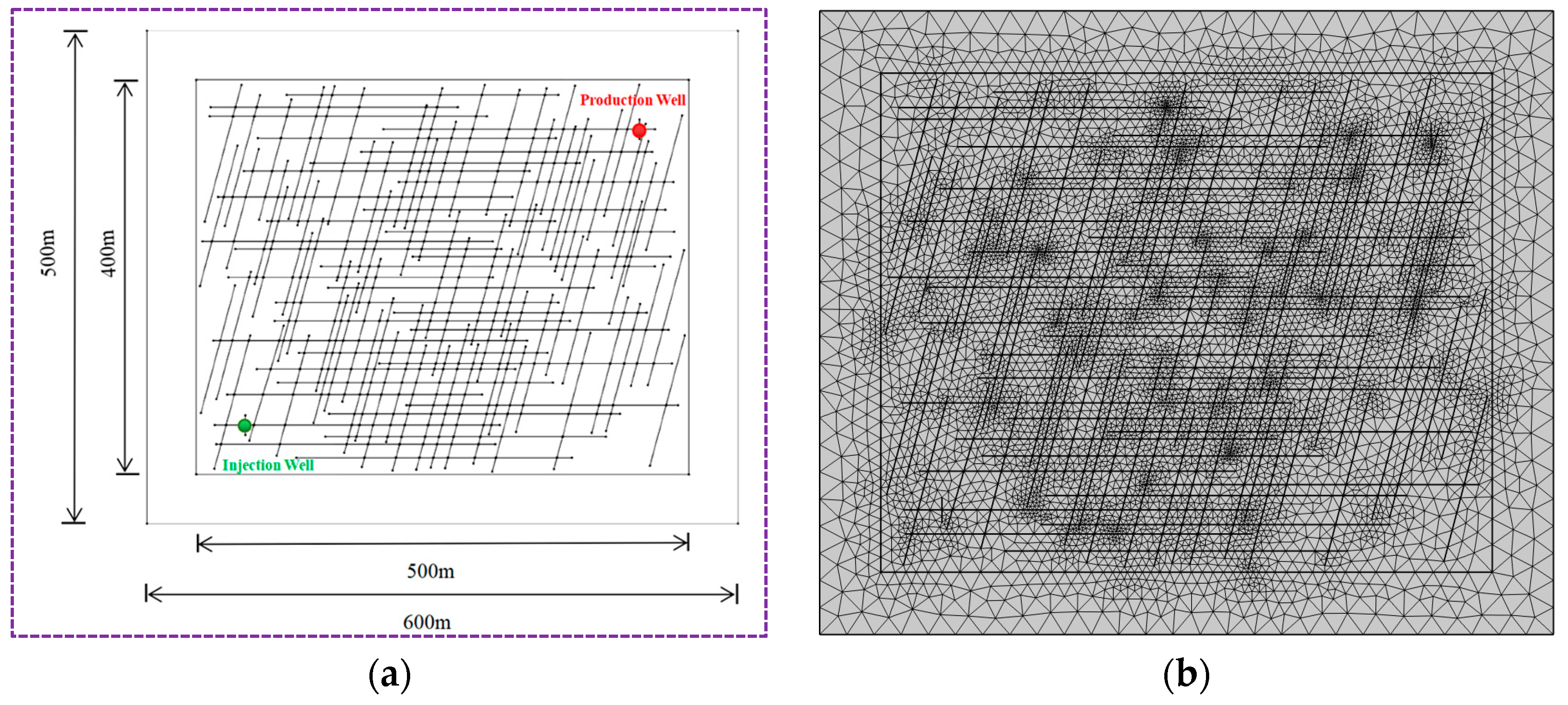
3.1. Introduction to the Numerical Calculation Model
3.2. Initial and Boundary Calculation Conditions
4. Results and Discussion
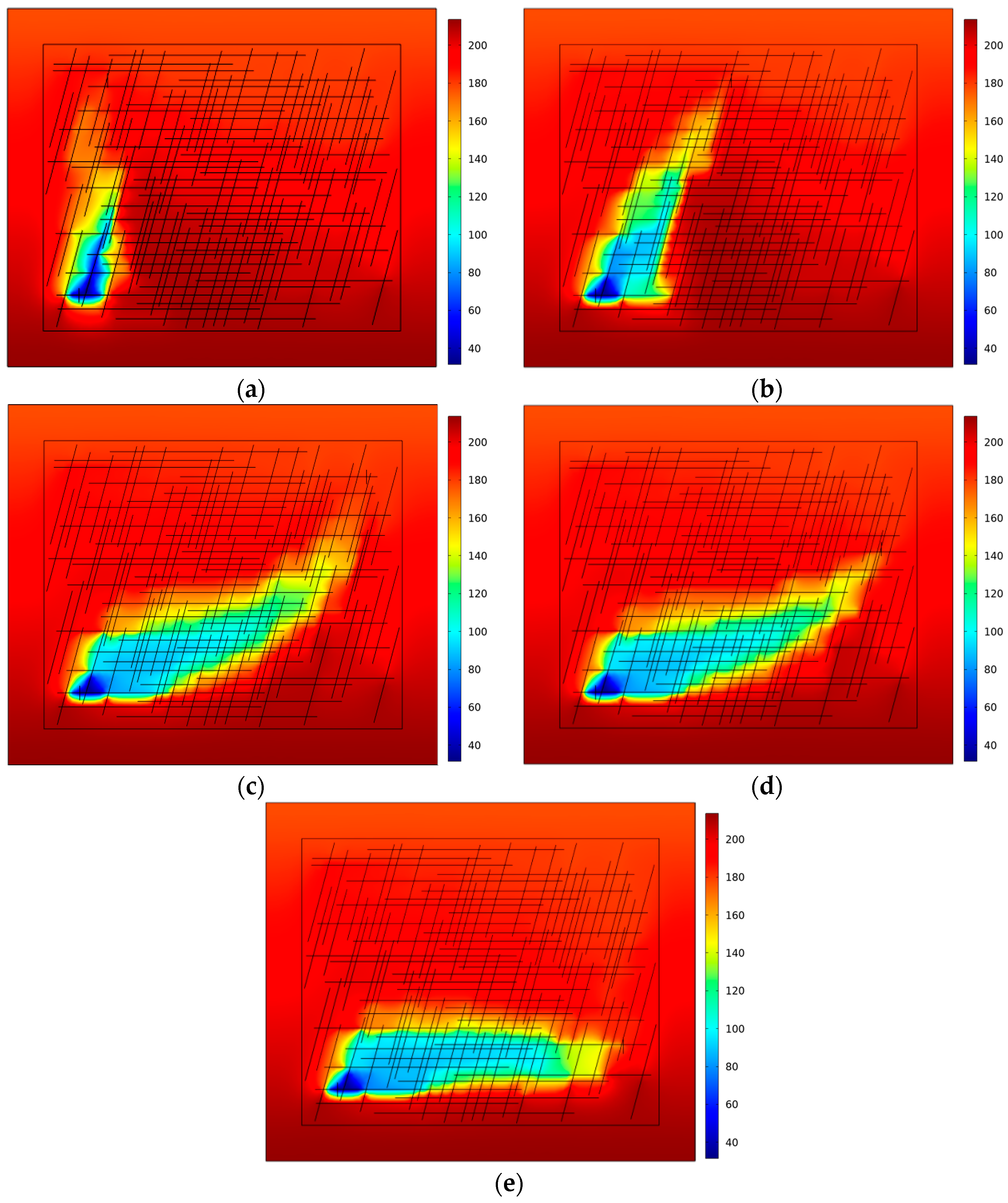
4.1. Temperature Characteristics During Injection and Production Process
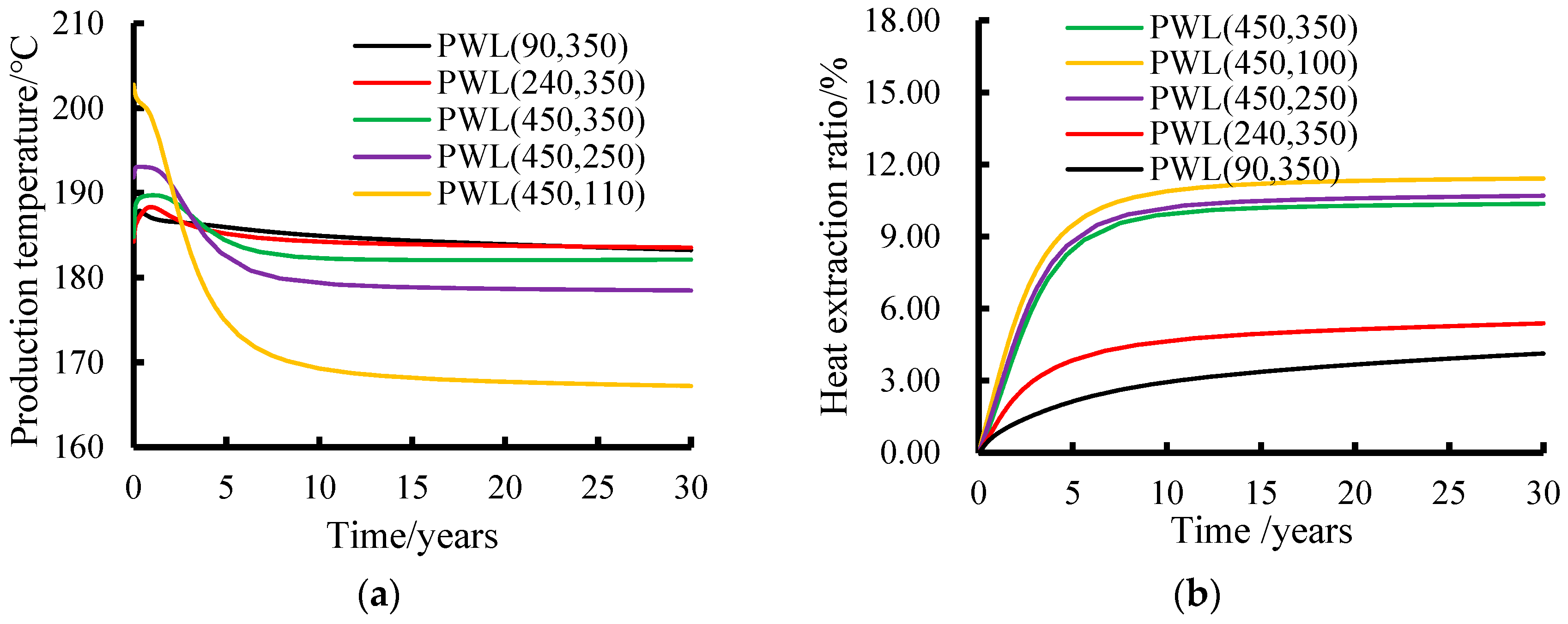
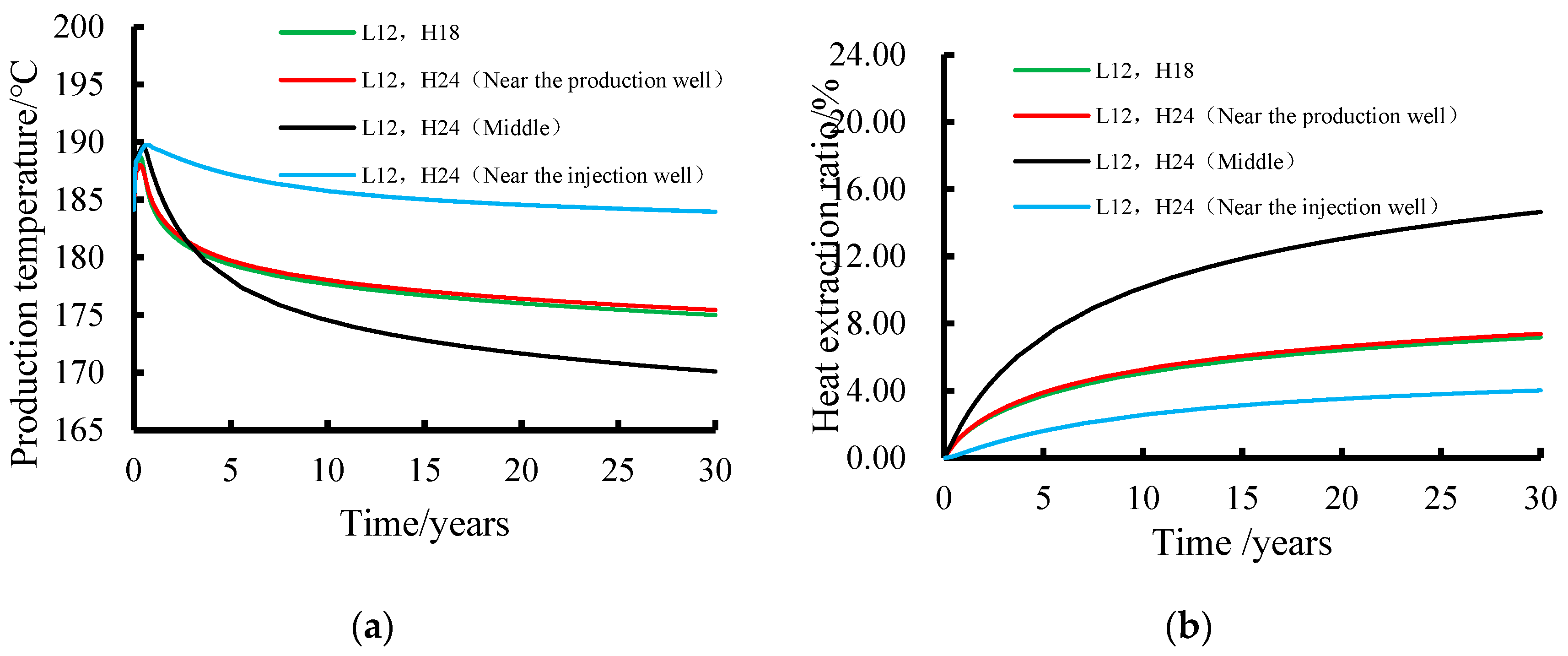
4.2. Influence of Production Well Location on Heat Recovery Performance
4.3. Effect of Different Parameters on Thermal Extraction Performance
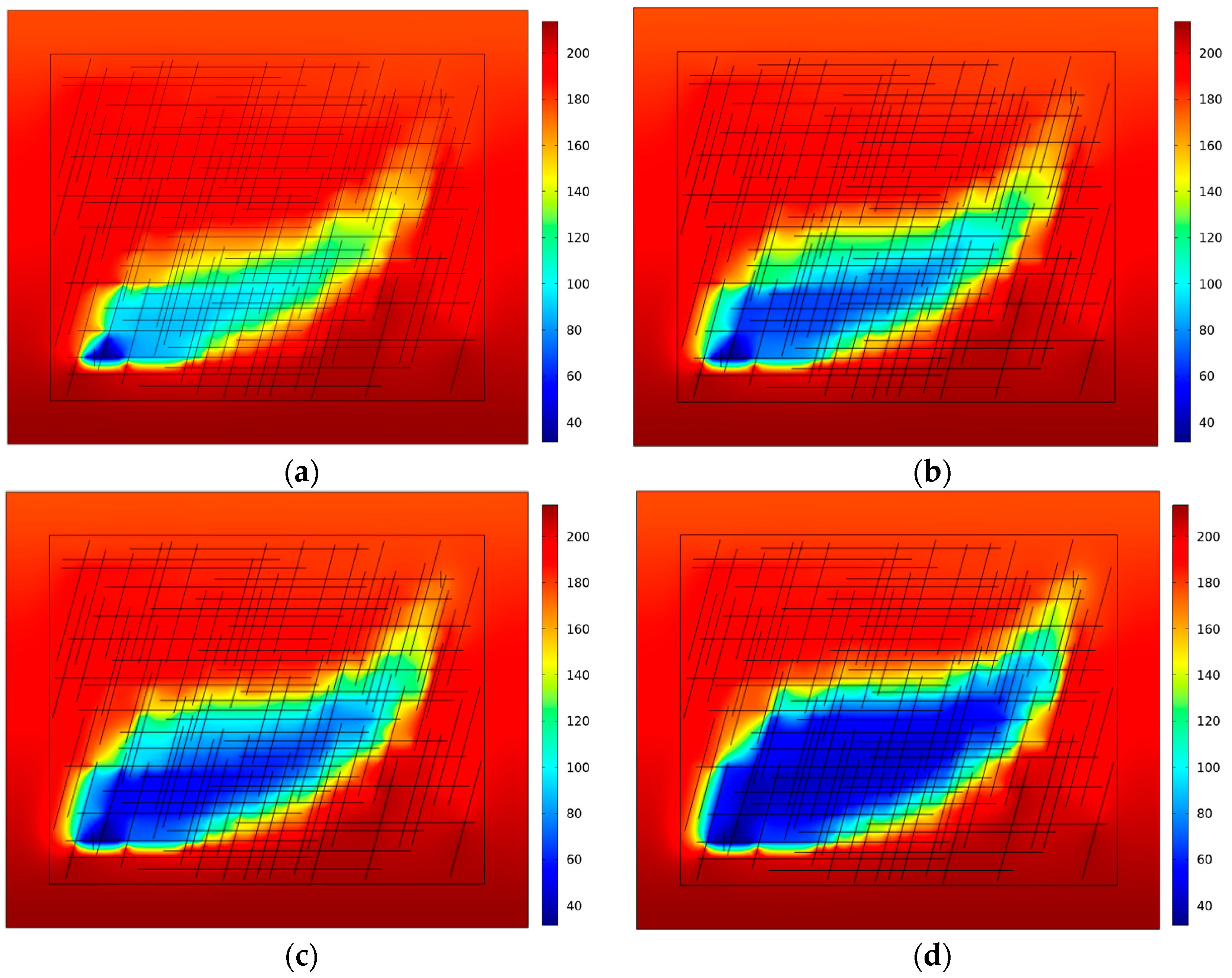
4.3.1. CO2 Injection Rate
4.3.2. Initial Fracture Width
4.3.3. Number of High-Angle Fractures
5. Summary and Conclusions
- (1)
- In the reservoir rock fracture network, the fracture heat exchange efficiency is higher than that of rock matrix, the temperature mainly expands along the fracture after low-temperature CO2 injection, a low-temperature zone is gradually formed in the reservoir fracture and its connecting zone, and the heat exchange of the reservoir in the later stages is dominated by the rock matrix. Under the same conditions of injection and extraction for 30 years, the influence range of the low-temperature zone formed by using CO2 as the injection and extraction fluid is much less than that formed by using water as the injection and extraction fluid. Therefore, CO2 injection heat exchange is more conducive to prolonging the life of geothermal reservoirs.
- (2)
- The horizontal position of the production wells has a significant effect on temperature field distribution. The farther the horizontal distance from the injection wells, the better the fracture connectivity, the stronger the heat exchange and the larger the range of the low-temperature zones. Changing of the vertical position of the production wells has a smaller effect on the temperature fields and the better the connectivity between the production wells and the injection wells at different vertical positions, the higher the heat extraction rate.
- (3)
- The faster the CO2 injection rate is, the larger the range of low-temperature area there is, the more obvious the change in distribution shape is and the easier it is to flow along the fracture, The rate of temperature drop in the production well and the growth rate of the heat extraction rate of the reservoir at the early stages of injection and extraction increase with the increase in the injection rate, and then the changes in the temperature of the production well and the heat extraction rate of the reservoir tend to be stable in the later stages.
- (4)
- The increase in high-angle fracture width facilitates vertical fluid flow and heat exchange, which promotes the vertical extension of low-temperature regions and the elongation of temperature fields along the vertical direction. The temperature of production wells decreases quickly and the heat extraction rate increases rapidly at the initial stage, while the increase in horizontal fracture width is opposite to this law. In addition, the increase in high-angle fractures at different locations will change the range, temperature gradient and shape of the low-temperature region, which in turn affects the heat exchange, with the fastest growth of the heat extraction rate occurring when it is located between the production wells and the injection wells, is less obvious near the production wells, and slower near the injection wells.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wang, J.; Li, P.-J.; Zhang, T.; Zhu, C.-Y.; Zhang, Y.-N. Simulation of CO2 and water convective heat transfer in single fracture of practical rock sample. Chem. Ind. Eng. Prog. 2024, 43, 5403–5414. [Google Scholar]
- Zhao, P.; Zhu, H.-Y.; Li, G.-S.; Chen, Z.; Chen, S.-J.; Shang Guan, S.-T.; Qi, X.-F. Large-scale physical simulation of injection and production of hot dry rock in Gonghe Basin, Qinghai Province, China. Petrol. Explor. Dev. 2024, 51, 646–654. [Google Scholar] [CrossRef]
- Hui, Z.; Feng, Z.-J.; Wu, Z.-S.; Shi, X.-D. Experimental Study on Seepage and Heat Transfer of Sandstone with Single Fracture Under Multi-Level Confining Pressure. Min. Res. Dev. 2020, 40, 105–110. [Google Scholar]
- He, Y.-Y.; Bai, B.; Hu, S.-B.; Li, X.-C. Effects of surface roughness on the heat transfer characteristics of water flow through a single granite fracture. Comput. Geotech. 2016, 80, 312–321. [Google Scholar]
- Luo, Y.-F.; Xu, W.-L.; Lei, Y.-D.; Wu, P.; Qin, G.-X.; Ba, R.-S. Experimental study of heat transfer by water flowing through smooth and rough rock fractures. Energy Rep. 2019, 5, 1025–1029. [Google Scholar]
- Yao, J.; Zhang, X.; Sun, Z.-X.; Huang, Z.-Q.; Liu, J.-R.; Li, Y.; Xin, Y.; Yan, X.; Liu, W.-Z. Numerical simulation of the heat extraction in 3D-EGS with thermal-hydraulic-mechanical coupling method based on discrete fractures model. Geothermics 2018, 74, 19–34. [Google Scholar]
- Song, G.-F.; Song, X.-Z.; Xu, F.-Q.; Li, G.-S.; Wang, G.-S.; Ji, J.-Y.; Shi, Y. Numerical parametric investigation of thermal extraction from the enhanced geothermal system based on the thermal-hydraulic-chemical coupling model. J. Clean. Prod. 2022, 352, 131609. [Google Scholar]
- Du, X.; Jiang, Y.-X.; Yang, F.; Lu, D.-T. Thermo-hydro-mechanical fully coupled model for enhanced geothermal system and numerical solution method based on finite volume method. Renew. Energy. 2024, 237, 121559. [Google Scholar]
- Wang, T.-Y.; Zhou, X.-X.; Li, G.-S.; Wang, Y.; Tian, S.-Z. Geothermal development model of multilateral radial well and its heat extraction effect analysis based on thermal-hydraulic-mechanical coupling. Nat. Gas Ind. 2023, 43, 133–144. [Google Scholar]
- Sun, Z.-X.; Zhang, X.; Xu, Y.; Yao, J.; Wang, H.-X.; Lv, S.-H.; Sun, Z.-L.; Huang, Y.; Cai, M.-Y.; Huang, X.-X. Numerical simulation of the heat extraction in EGS with thermal-hydraulic-mechanical coupling method based on discrete fractures model. Energy 2017, 120, 20–33. [Google Scholar]
- Tsang, C.-F.; Bernier, F.; Davies, C. Geohydromechanical processes in the excavation damaged zone in crystalline rock, rock salt, and indurated and plastic clays-in the context of radioactive waste disposal. Int. J. Rock Mech. Min. Sci. 2005, 42, 109–125. [Google Scholar]
- Gutierrez, M.; Makurat, A. Coupled HTM modellingof cold water injection in fractured hydrocarbon reservoirs. Int. J. Rock Mech. Min. Sci. 1997, 34, 113.e1–113.e15. [Google Scholar]
- Chen, J.-L.; Jiang, F.M. Designing multi-well layout for enhanced geothermal system to better exploit hot dry rock geothermal energy. Renew. Energ. 2015, 74, 37–48. [Google Scholar]
- Salimzadeh, S.; Nick, H.-M.; Zimmerman, R.-W. Thermoporoelastic effects during heat extraction from low-permeability reservoirs. Energy 2018, 142, 546–558. [Google Scholar]
- Duan, X.-Y.; Huang, D.; Lei, W.-X.; Chen, S.-C.; Huang, Z.-Q.; Zhu, C.-Y. Investigation of Heat Extraction in an Enhanced Geothermal System Embedded with Fracture Networks Using the Thermal-Hydraulic-Mechanical Coupling Model. Energies 2023, 16, 3758. [Google Scholar] [CrossRef]
- Cheng, Y.-X.; Zhang, Y.-J.; Yu, Z.-W.; Hu, Z.-J.; Yang, Y.-X. An investigation on hydraulic fracturing characteristics in granite geothermal reservoir. Eng. Fract. Mech. 2020, 237, 107252. [Google Scholar]
- Yoshioka, K.; Pasikki, R.; Stimac, J. A long term hydraulic stimulation study conducted at the Salak geothermal field. Geothermics 2019, 82, 168–181. [Google Scholar]
- Park, S.; Xie, L.-M.; Kim, K.; Kwon, S.; Min, K.; Choi, J.; Yoon, W.; Song, Y. First hydraulic stimulation in fractured geothermal reservoir in Pohang PX-2 Well. Procedia Eng. 2017, 191, 829–837. [Google Scholar]
- Pruess, K. Enhanced geothermal systems (EGS) using CO2 as working fluid: A novel approach for generating renewable energy with simultaneous sequestration of carbon. Geothermics 2006, 35, 351–367. [Google Scholar]
- Xu, R.-N.; Zhang, L.; Zhang, F.-Z.; Jiang, P.-X. A review on heat transfer and energy conversion in the enhanced geo thermal systems with water/CO2 as working fluid. Int. J. Energy Res. 2015, 39, 1722–1741. [Google Scholar]
- Na, J.; Xu, T.-F.; Yuan, Y.-L.; Feng, B.; Tian, H.-L.; Bao, X.-H. An integrated study of fluid-rock interaction in a CO2-based enhanced geothermal system: A case study of Songliao Basin, China. Appl. Geochem. 2015, 59, 166–177. [Google Scholar] [CrossRef]
- Qiao, Z.-L.; Cao, Y.; Tang, Y.-F.; Si, F.-Q. Numerical Analysis of Membrane-Absorption Separation for Supercritical Carbon Dioxide and Water Mixture of Plume Geothermal Power Generation Systems. Energy Convers. Manag. 2020, 208, 112609. [Google Scholar] [CrossRef]
- Xu, Z.-P.; Tan, Y.-F.; Zhang, T.-T.; Li, Z.-Y. Research Review on Supercritical Carbon Dioxide for Geothermal Extraction. Gas Heat 2024, 44, 16–27. [Google Scholar]
- Chen, S.-J.; Pan, Y.; Sun, L.; Si, Y.; Liang, F.; Gao, L. Mechanism of enhanced oil recovery by CO2 combination flooding in low permeability and high pour-point reservoir. Petrol. Reserv. Eval. Dev. 2021, 11, 823–830. [Google Scholar]
- Liu, Y.-L.; Xu, T.-F.; Yuan, Y.-L.; Feng, B.; Tang, X.-H.; Liu, X.; Cui, Z.-P. A laboratory study on fracture initiation and propagation of granite under cyclic-in jection hydraulic fracturing. J. Petrol. Sci. Eng. 2022, 212, 110278. [Google Scholar] [CrossRef]
- Wang, D.-B.; Bian, X.-B.; Qin, H.; Sun, D.-L.; Yu, B. Experimental investigation of mechanical properties and failure behavior of fluid-saturated hot dry rocks. Nat. Resour. Res. 2021, 30, 289–305. [Google Scholar] [CrossRef]
- Shi, Y.; Song, X.-Z.; Li, G.-S.; Xu, F.-Q.; Cui, Q.-L. Comparison of heat extraction performance between CO2 and water in a multilateral-well geothermal system. Nat. Gas Ind. 2021, 41, 179–190. [Google Scholar]
- Ren, S.-R.; Cui, G.-D.; Li, D.-X.; Zhuang, Y.; Li, X.; Zhang, L. Development of geothermal energy from depleted high temperature gas reservoir via supercritical CO2 injection. J. China Univ. Petrol. 2016, 40, 91–98. [Google Scholar]
- Chen, Y.; Ma, G.-W.; Wang, H.-D.; Li, T.; Wang, Y. Application of carbon dioxide as working fluid in geothermal development con sidering a complex fractured system. Energy Convers. Manag. 2019, 180, 1055–1067. [Google Scholar] [CrossRef]
- Wang, Y.; Li, T.; Ma, G.-W. Numerical analysis of heat mining and geological carbon sequestration in supercritical CO2 circulating enhanced geothermal systems inlayed with com plex discrete fracture networks. Energy 2019, 173, 92–108. [Google Scholar] [CrossRef]
- Li, Q.; Li, Q.-C.; Cao, H.-Q.; Wu, J.-J.; Wang, F.; Wang, Y.-L. The Crack Propagation Behaviour of CO2 Fracturing Fluid in Unconventional Low Permeability Reservoirs: Factor Analysis and Mechanism Revelation. Processes 2025, 13, 159. [Google Scholar] [CrossRef]
- Li, Q.-C.; Li, Q.; Wu, J.-J.; Li, X.-Z.; Li, H.-B.; Cheng, Y.-F. Wellhead Stability During Development Process of Hydrate Reservoir in the Northern South China Sea: Evolution and Mechanism. Processes 2025, 13, 40. [Google Scholar] [CrossRef]
- Xu, C.-S.; Dowd, P.A.; Tian, Z.-F. A simplified coupled hydro-thermal model for enhanced geothermal systems. Appl. Energy 2015, 140, 135e45. [Google Scholar]
- Yan, B.-C.; Xu, Z.; Manojkumar, G.; Tariq, Z.; Sun, S.-Y.; Finkbeiner, T. Physics-informed machine learning for reservoir management of enhanced geothermal systems. Geoenergy Sci. Eng. 2024, 234, 212663. [Google Scholar]
- Song, X.-Z.; Shi, Y.; Li, G.-S.; Yang, R.-Y.; Wang, G.-S.; Zheng, R.; Li, J.-C.; Lyu, Z.-H. Numerical simulation of heat extraction performance in enhanced geothermal system with multilateral wells. Appl. Energy 2018, 218, 325–337. [Google Scholar]
- Zhang, X.; Huang, Z.-W.; Li, G.-S.; Wu, X.-G.; Wang, T.-Y.; Zhou, X.-X. Enhancing reservoir stimulation and heat extraction performance for fractured geothermal reservoirs: Utilization of novel multilateral wells. Energy 2024, 291, 130410. [Google Scholar]
- Zhao, P.; Liu, J.; Elsworth, D. Numerical study on a multifracture enhanced geothermal system considering matrix permeability enhancement induced by thermal unloading. Renew. Energy 2023, 203, 33–44. [Google Scholar] [CrossRef]
- Liu, D.-D.; Wei, L.-X.; Xu, G.-Y.; Xiang, Y.-Y. Simulation of seepage and heat transfer in 3D fractured rock mass based on fracture continuum method. Rock Soil Mech. 2023, 44, 7. [Google Scholar]
- Guo, T.-K.; Zhang, Y.-L.; Zhang, W.; Niu, B.-L.; He, J.-Y.; Chen, M.; Yu, Y.; Xiao, B.; Xu, R.-L. Numerical simulation of geothermal energy productivity considering the evolution of permeability in various fractures. Appl. Therm. Eng. 2022, 201, 117756. [Google Scholar]
- Peng, J.; Zhao, P.; Zhu, H.-Y.; Chen, S.-J.; Xian, H.-Y.; Ni, T. Simulating the impact of complex fracture networks on the heat extraction performance of hot-dry rock masses. Nat. Gas Ind. B 2024, 11, 196–212. [Google Scholar]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Zhao, X.; Zhao, Y.; Zhao, P.; Zhu, Y.; Wu, Y.; He, X. Numerical Simulation of CO2 Injection and Extraction Heat Transfer in Complex Fracture Networks. Energies 2025, 18, 1606. https://doi.org/10.3390/en18071606
Liu Y, Zhao X, Zhao Y, Zhao P, Zhu Y, Wu Y, He X. Numerical Simulation of CO2 Injection and Extraction Heat Transfer in Complex Fracture Networks. Energies. 2025; 18(7):1606. https://doi.org/10.3390/en18071606
Chicago/Turabian StyleLiu, Yuguo, Xiaolong Zhao, Yizhong Zhao, Peng Zhao, Yinghui Zhu, Yi Wu, and Xinru He. 2025. "Numerical Simulation of CO2 Injection and Extraction Heat Transfer in Complex Fracture Networks" Energies 18, no. 7: 1606. https://doi.org/10.3390/en18071606
APA StyleLiu, Y., Zhao, X., Zhao, Y., Zhao, P., Zhu, Y., Wu, Y., & He, X. (2025). Numerical Simulation of CO2 Injection and Extraction Heat Transfer in Complex Fracture Networks. Energies, 18(7), 1606. https://doi.org/10.3390/en18071606






