Mitigating Skin and Proximity Effect in High-Voltage Underground Segmented Cables Through Individually Insulating Conductor Strings
Abstract
1. Introduction
Contributions and Novelty
2. Models and Methods
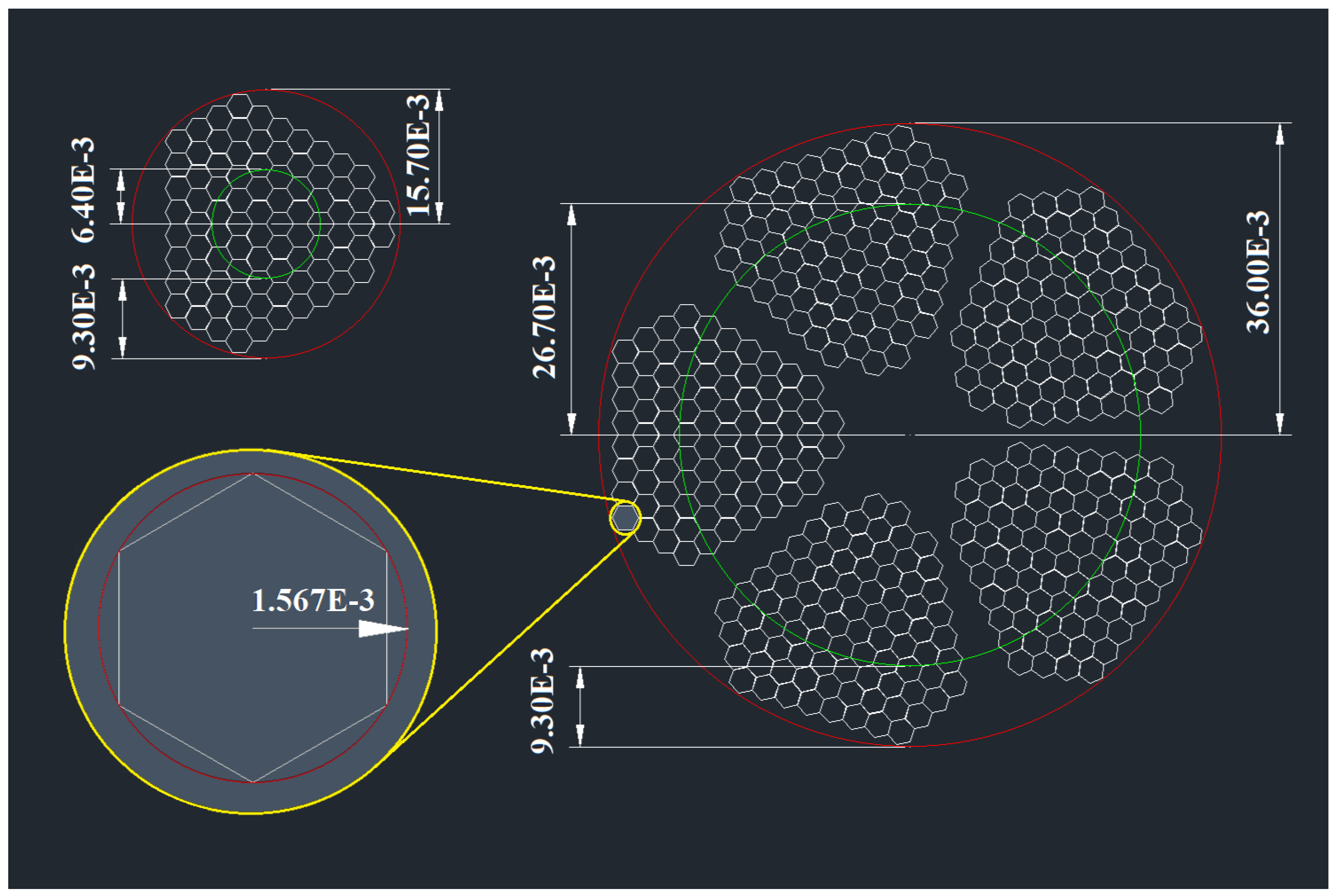
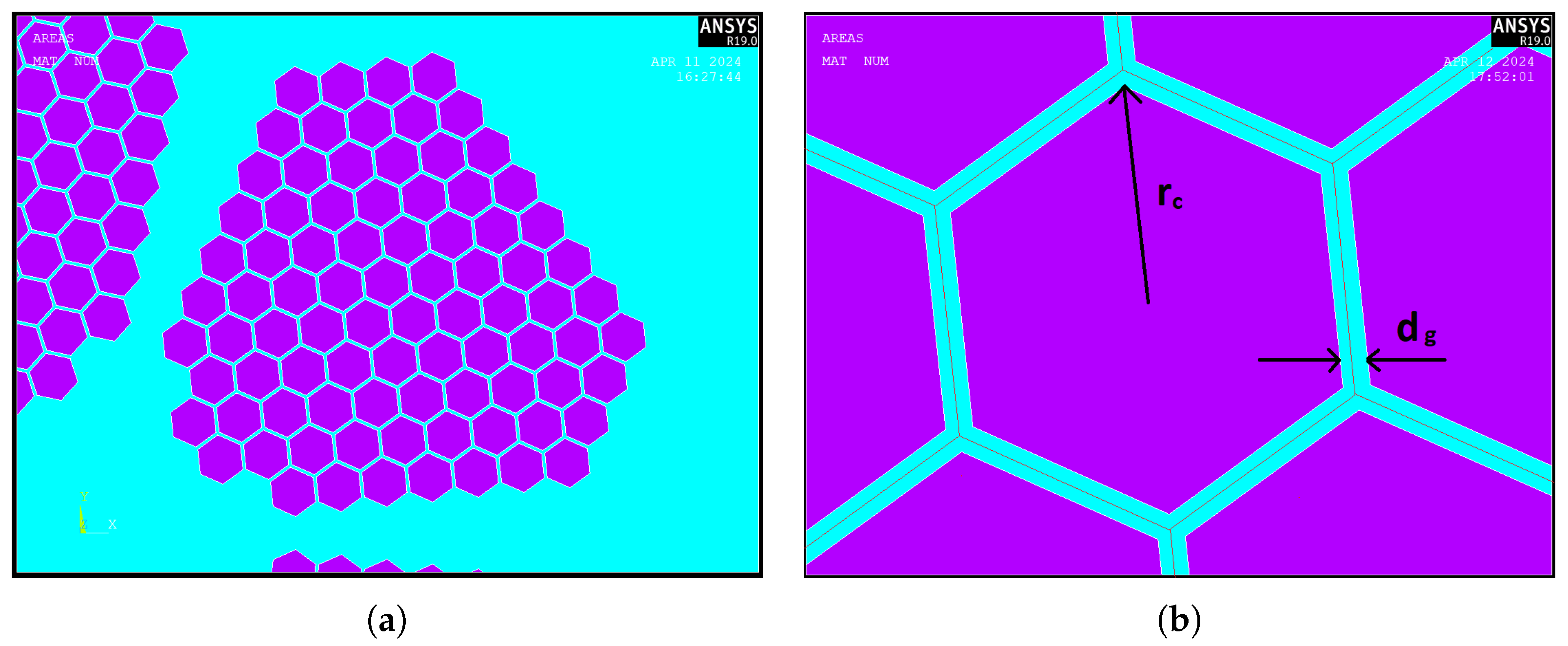
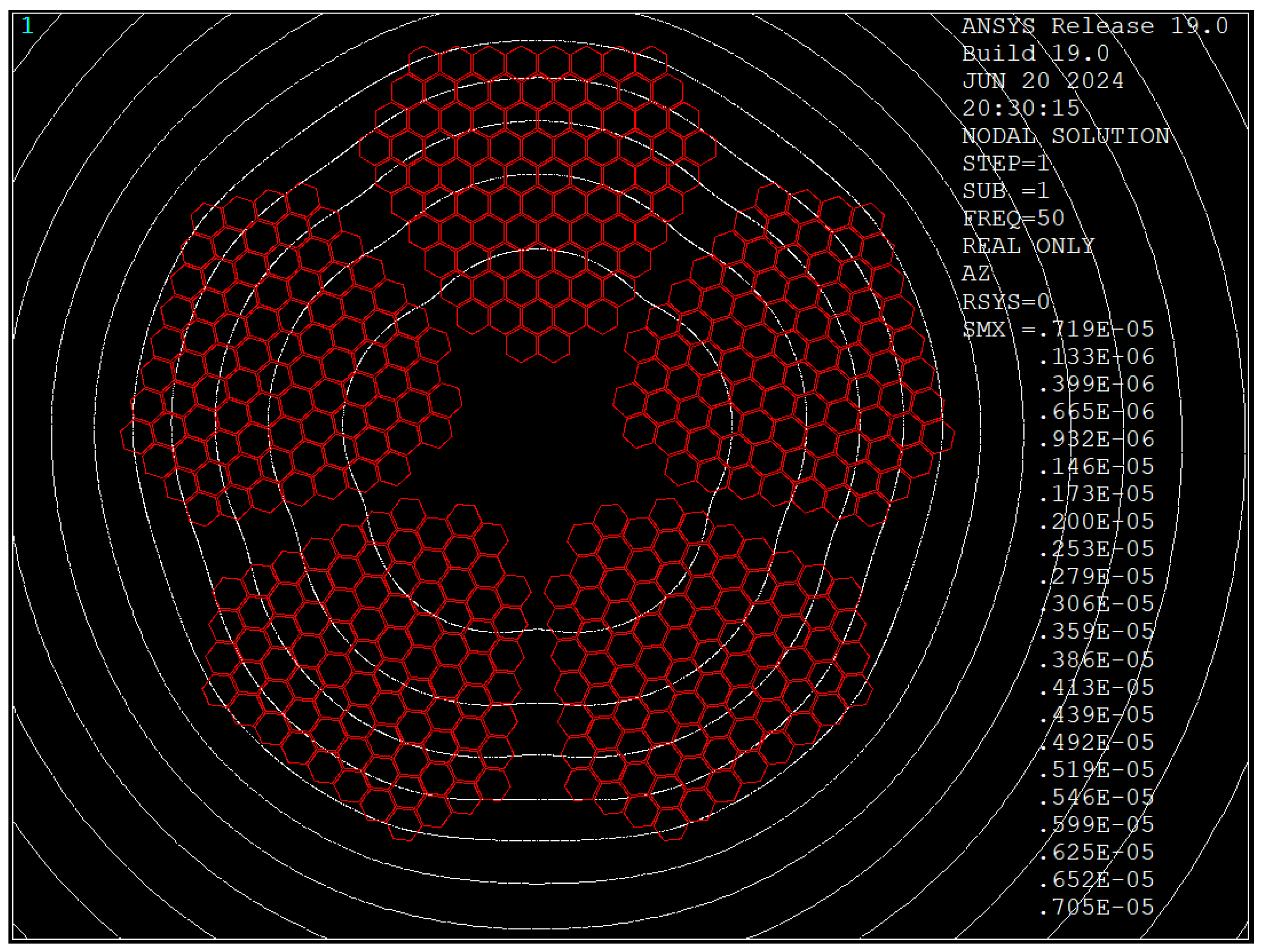
2.1. Five-Segment Cable Geometry
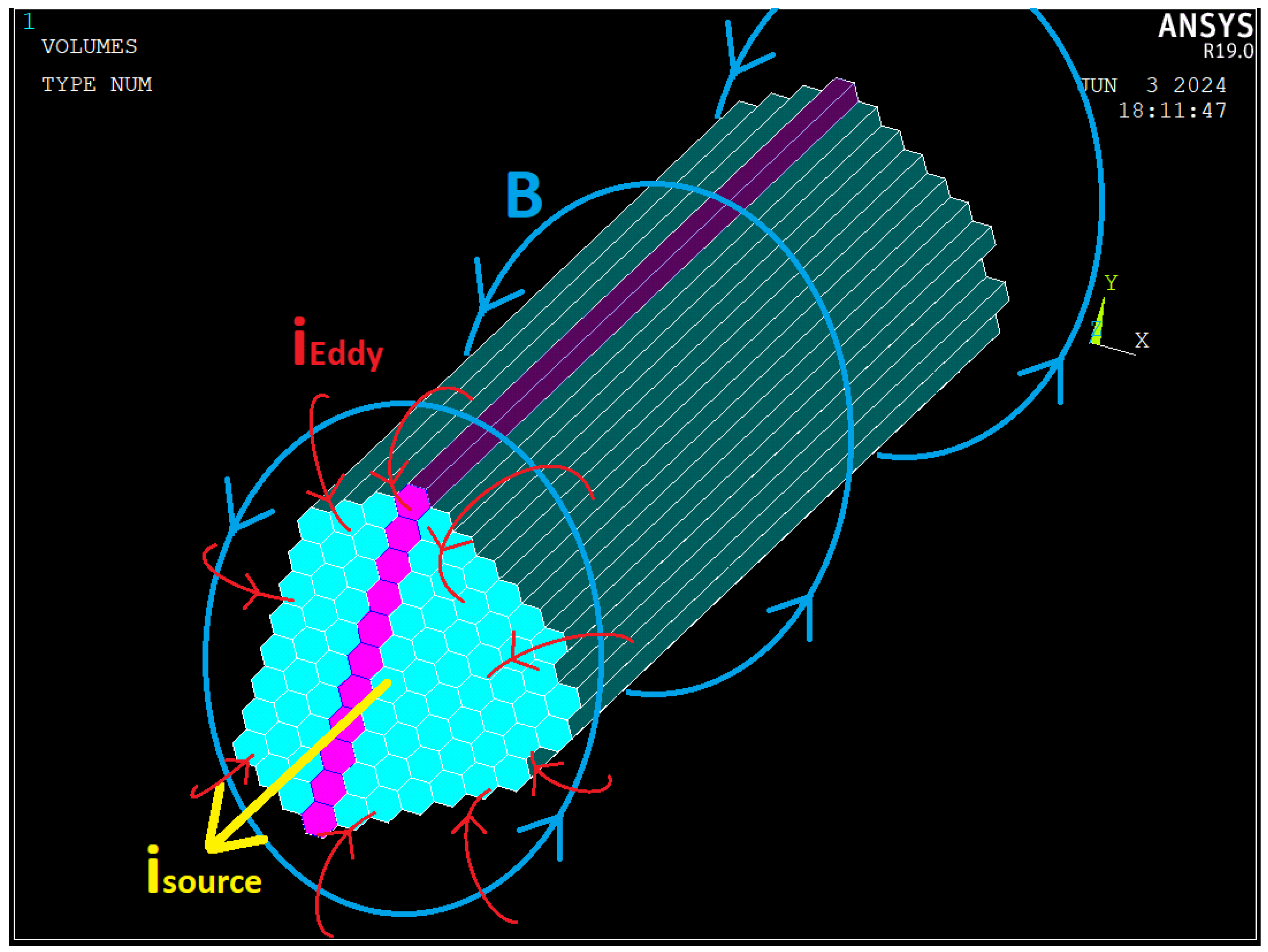
2.2. Rationale for Insulating Individual Conductor Strings
2.3. Insulation Layer and Gap Definition
2.4. Material Properties
2.5. Boundary Conditions and Source Current
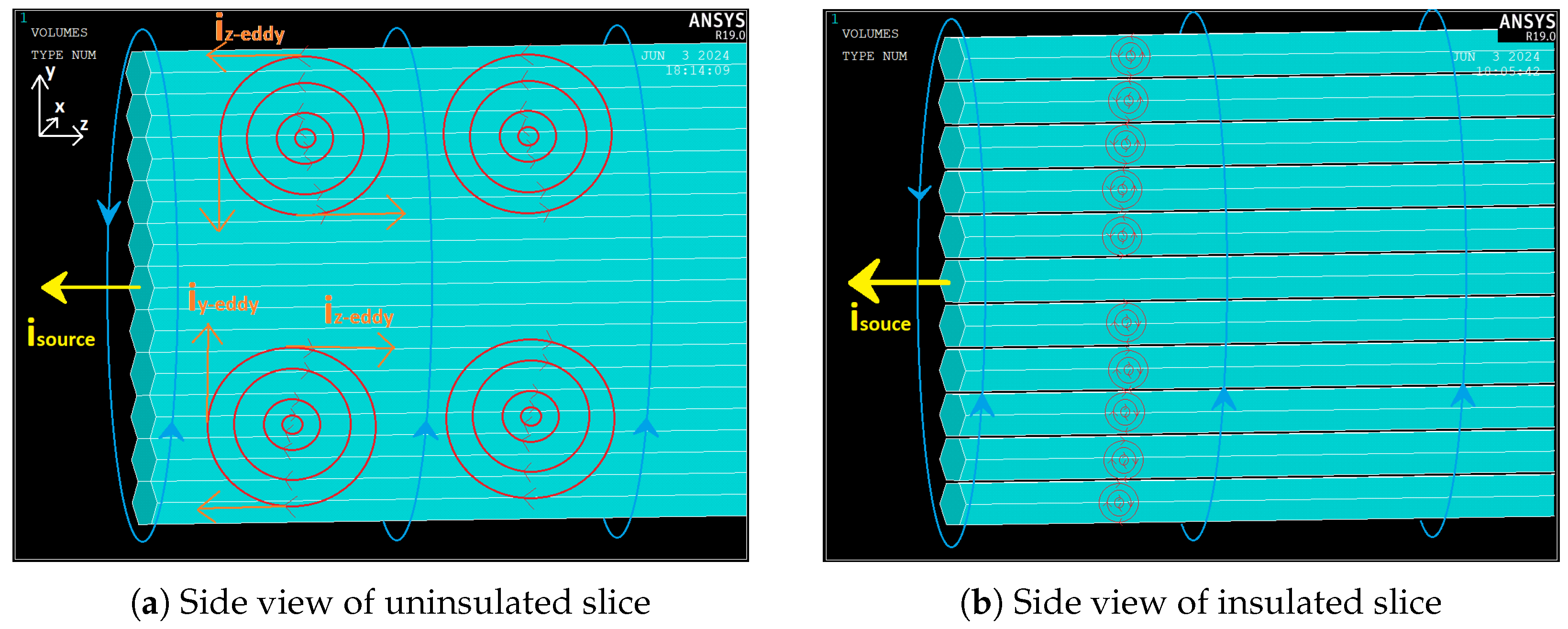
- No-Insulation Model: Voltage degrees of freedom (DOFs) within each segment are coupled, ensuring the current can freely distribute among the 85 conductor strings in that segment. Across different segments, the cable is assumed electrically isolated;
- Insulated Model: Each conductor string has its own set of coupled nodes, ensuring that current does not jump between adjacent strings in the x-y plane. Instead, is divided equally among all 425 conductors (85 in each of 5 segments).
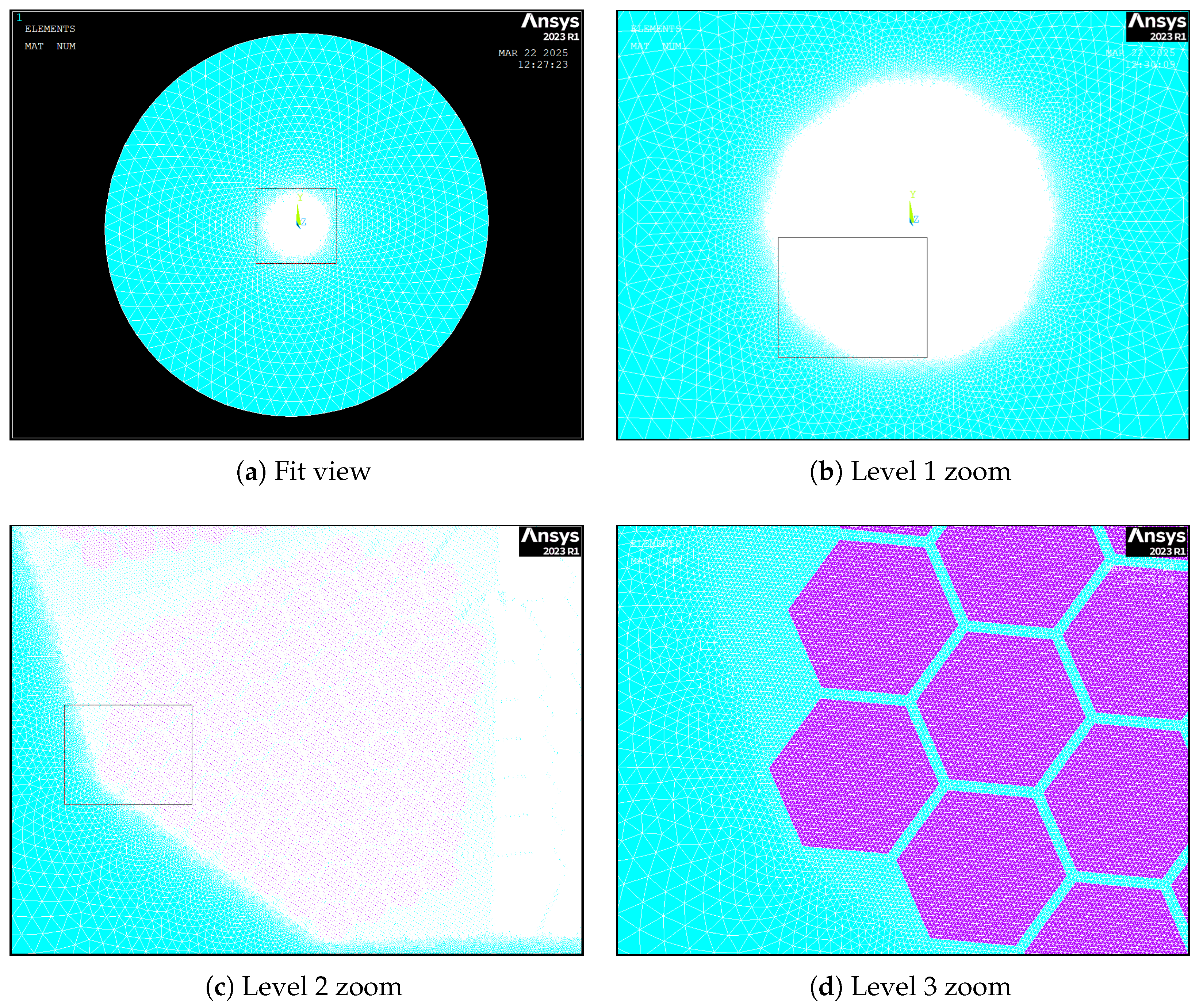
2.6. Finite Element Meshing
- Mapped Mesh for Conductor Core: Hexagonal copper areas are meshed with a mapped or uniform density to accurately capture current distribution;
- Finer Mesh for Insulating Gaps: The thin air gaps between conductor strings and around segment boundaries are meshed more densely to resolve steep field gradients.
2.7. Solver Setup and Post-Processing
- Current Density (J) within each conductor;
- Magnetic Flux Density (B) in and around the conductors;
- Power Loss (), from which is deduced via (3).
2.8. Definition of Key Parameters
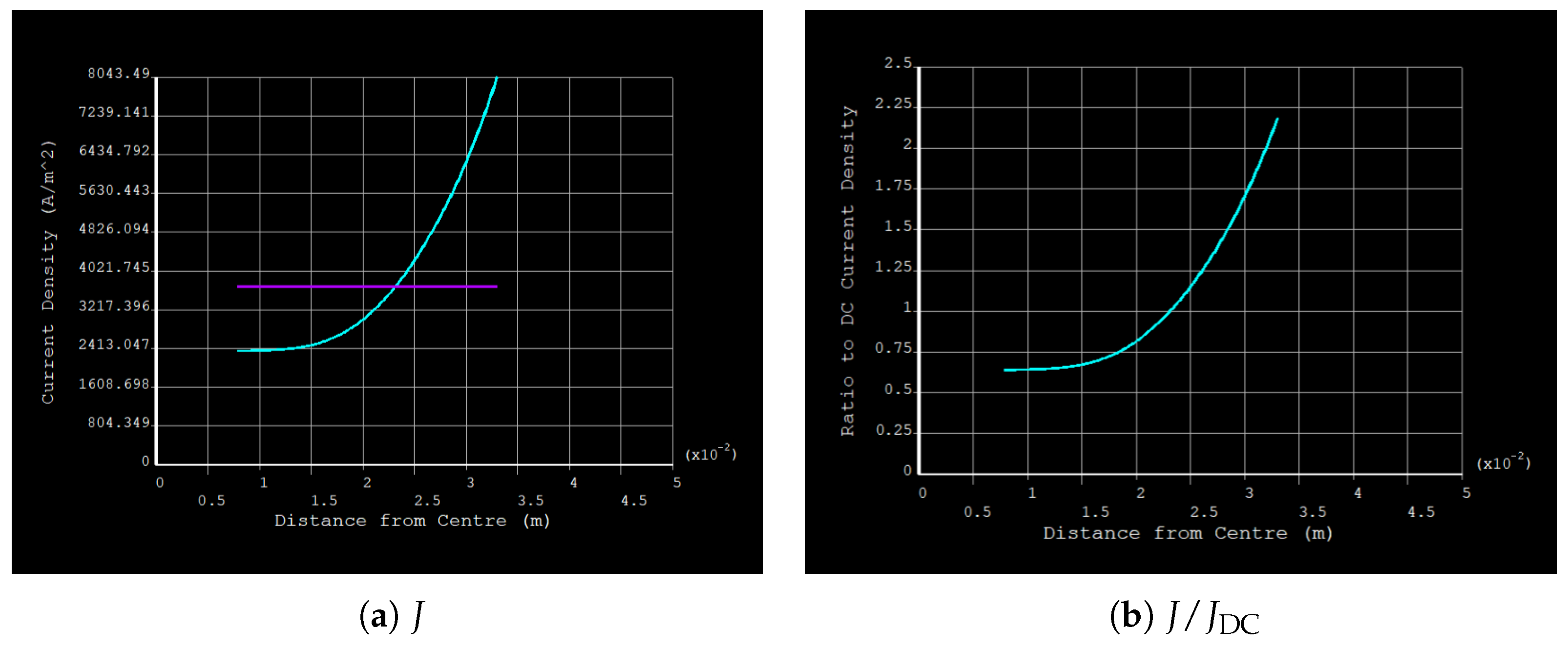
2.8.1. Current Density J (A/m2)
2.8.2. Power Loss (W/m)
2.8.3. AC Resistance (/m)
2.8.4. DC Resistance (/m)
3. Results
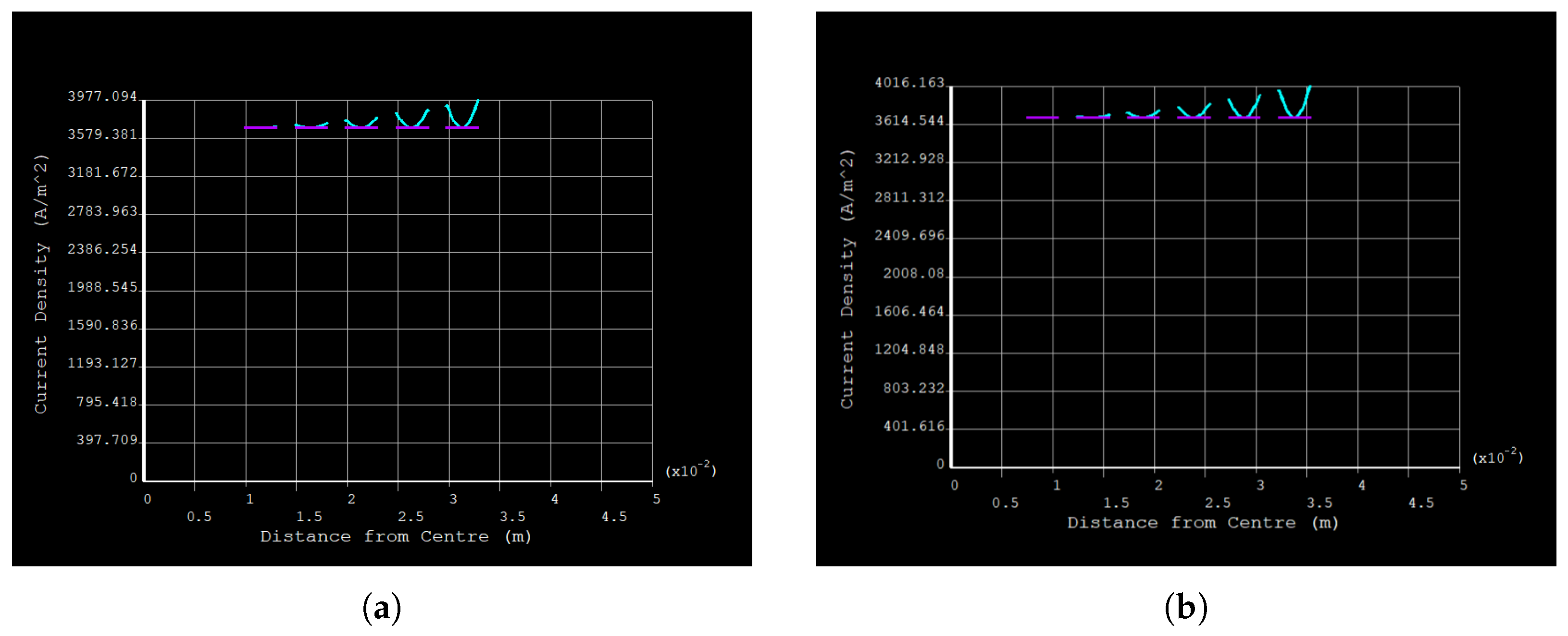
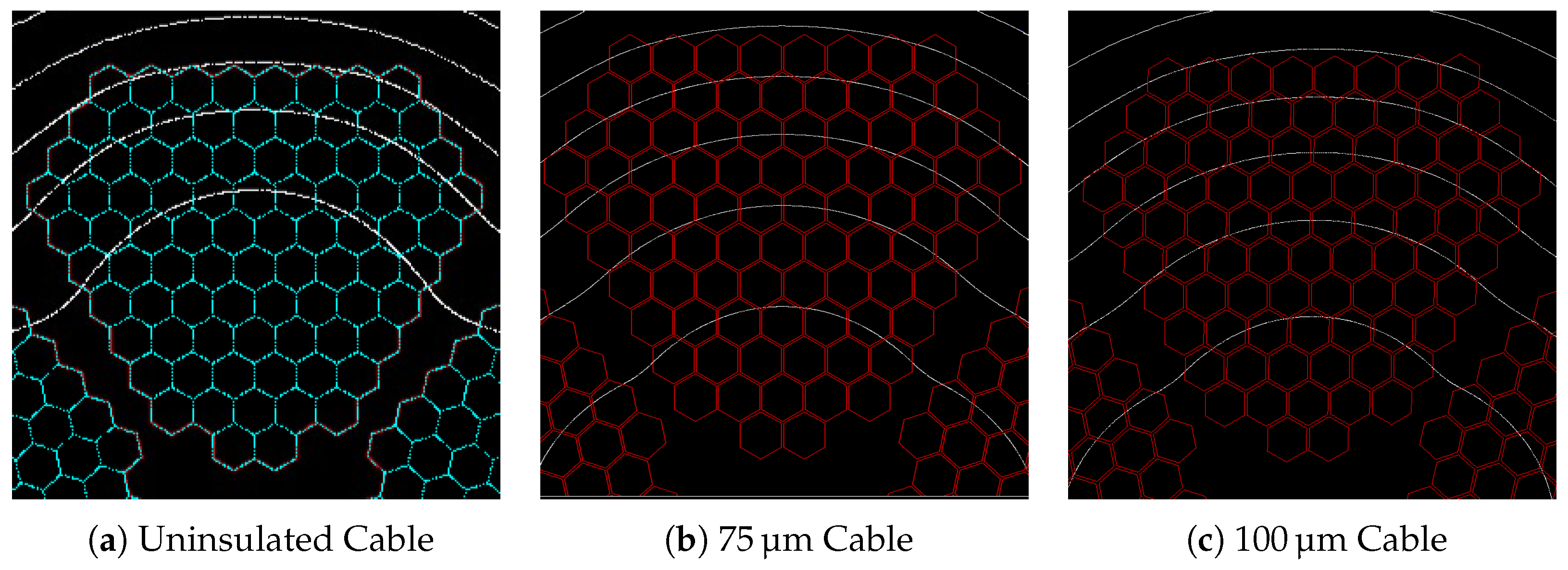
3.1. Uninsulated 5-Segment Cable
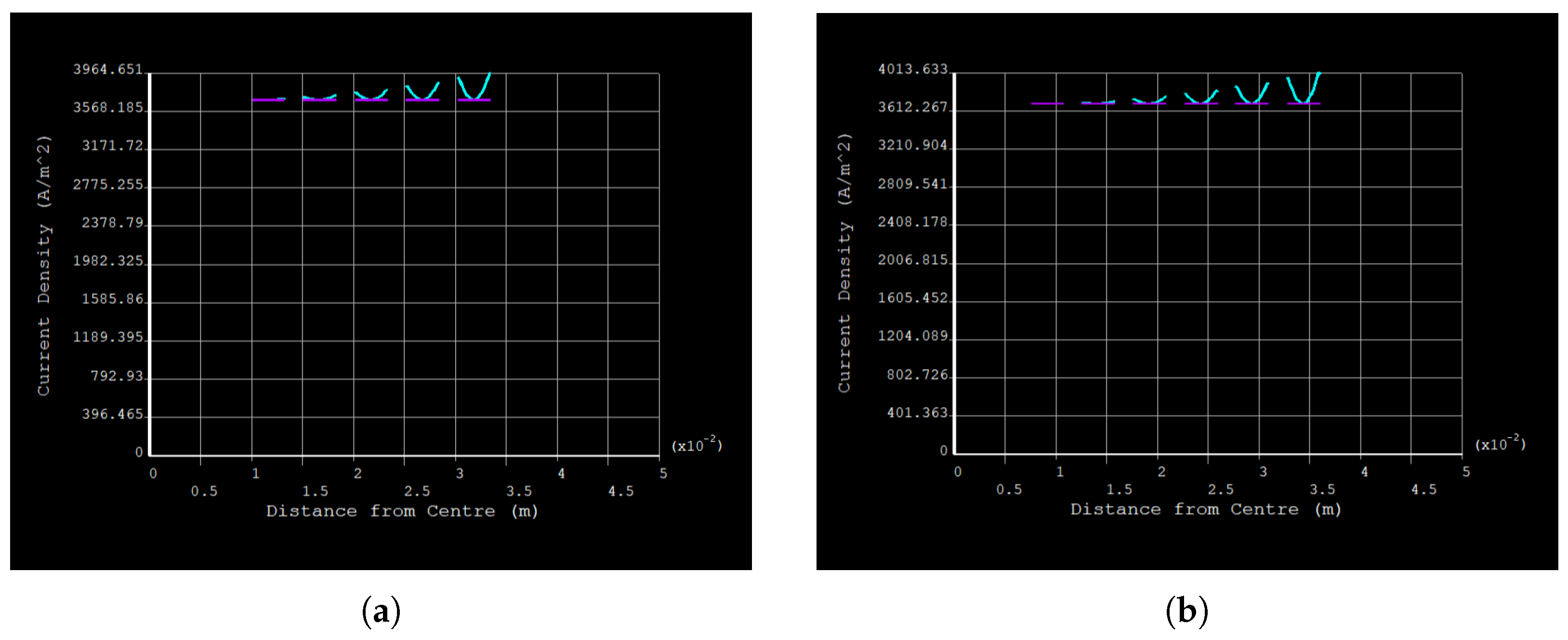
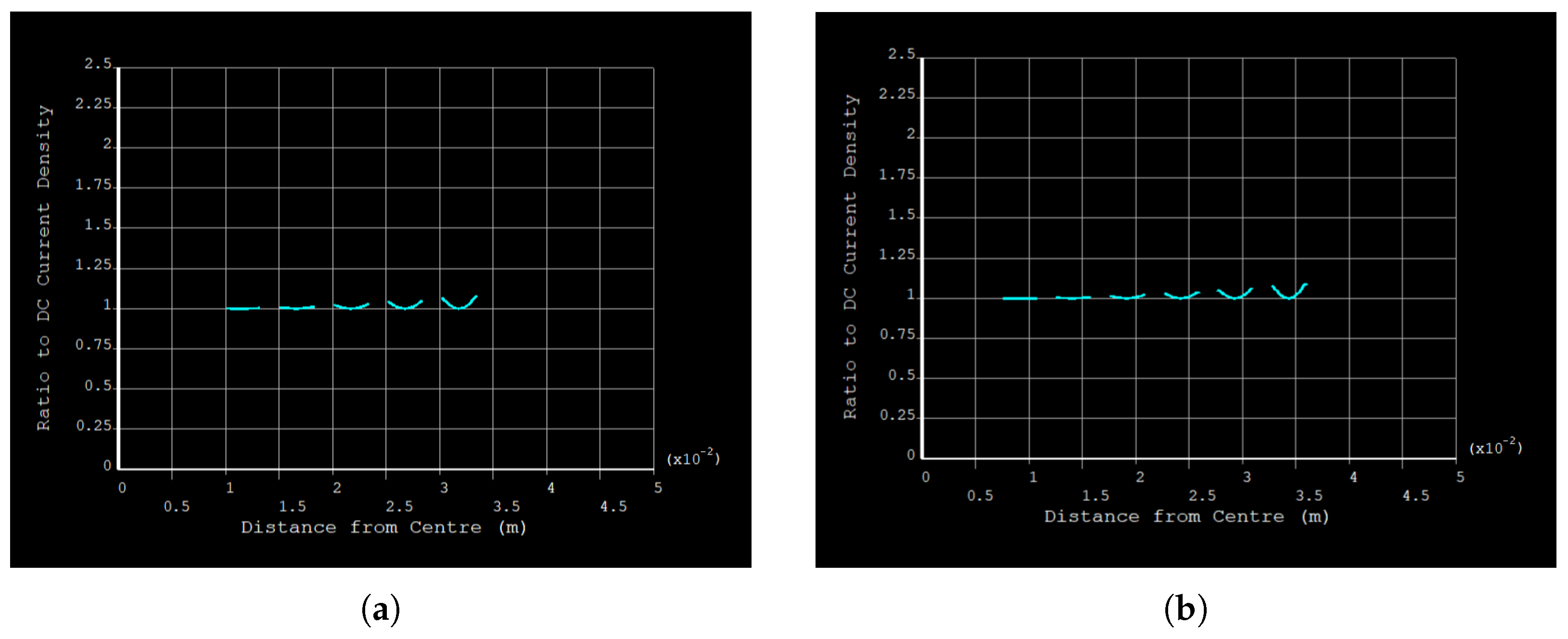
3.2. Insulated 5-Segment Cable (75 m)
3.3. Insulated 5-Segment Cable (100 m)
4. Discussion
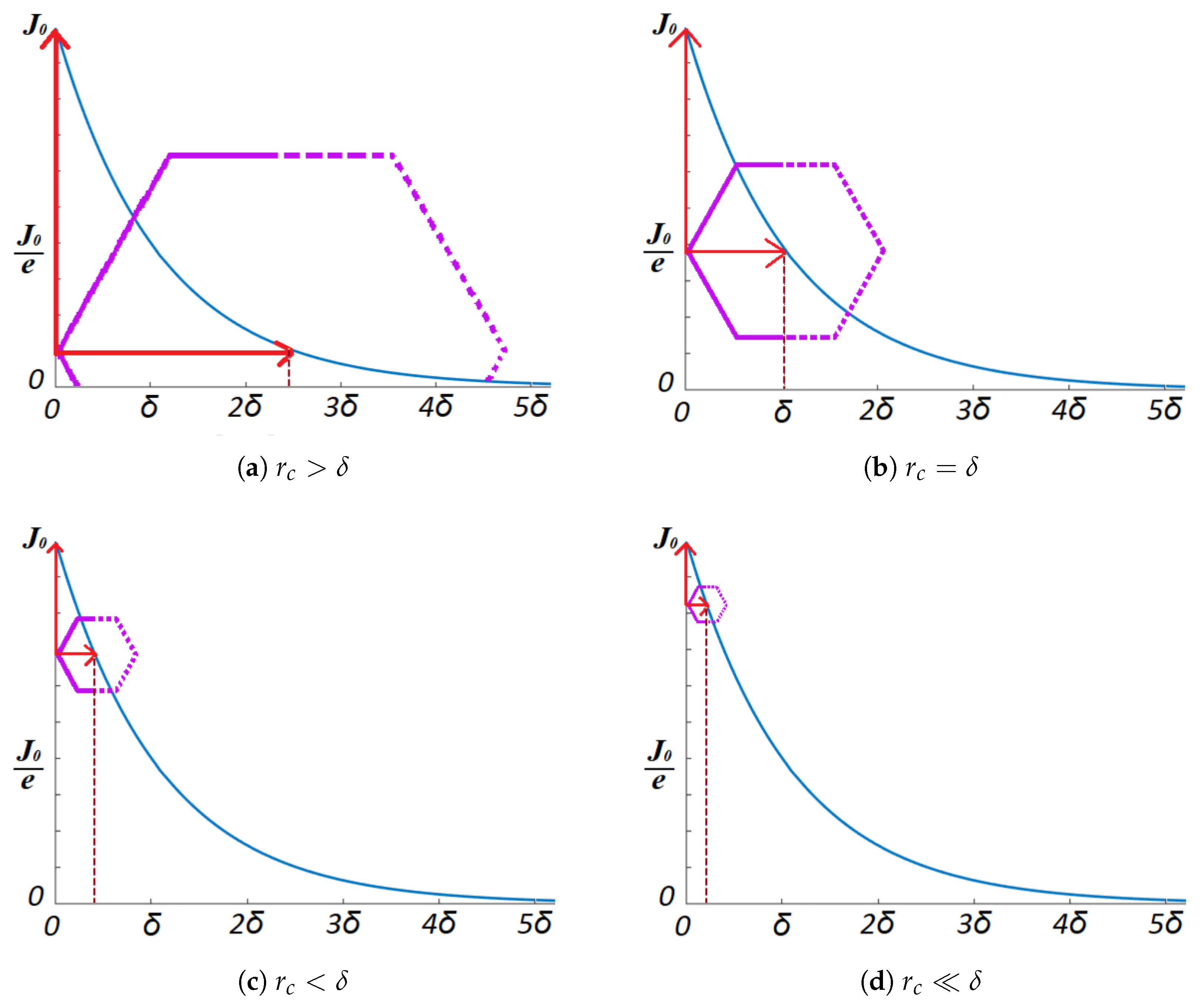
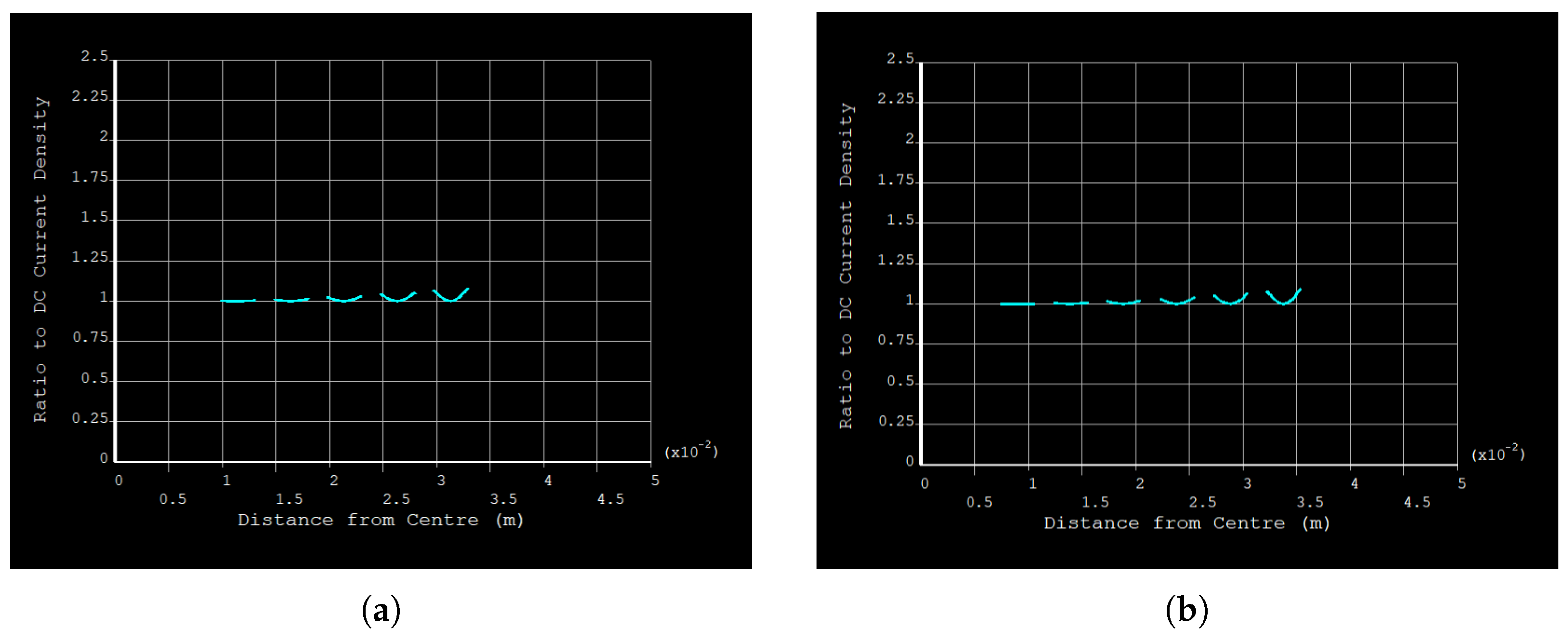
4.1. Skin and Proximity Loss Reduction
4.2. Insulation Thickness and Diminishing Returns
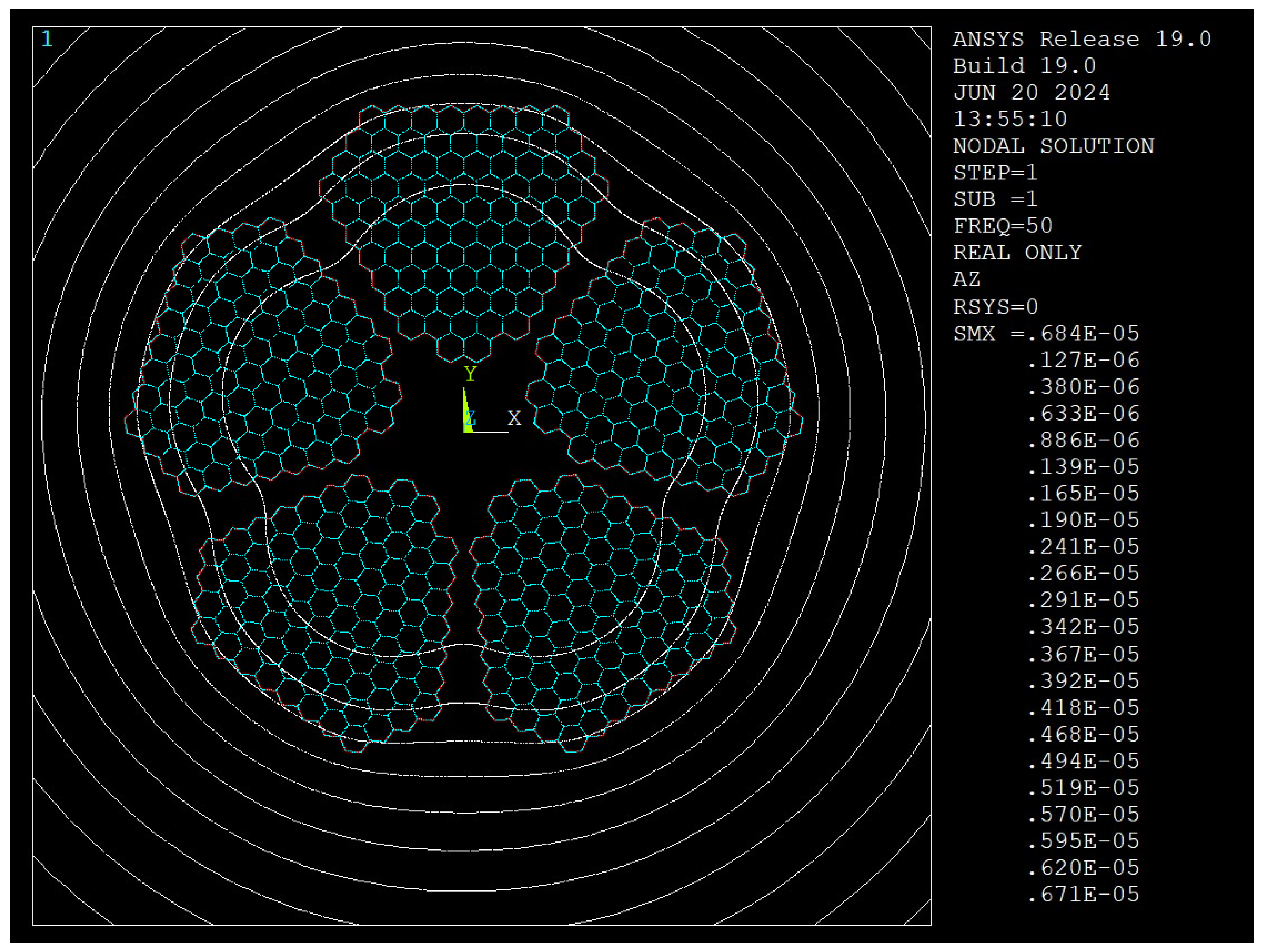
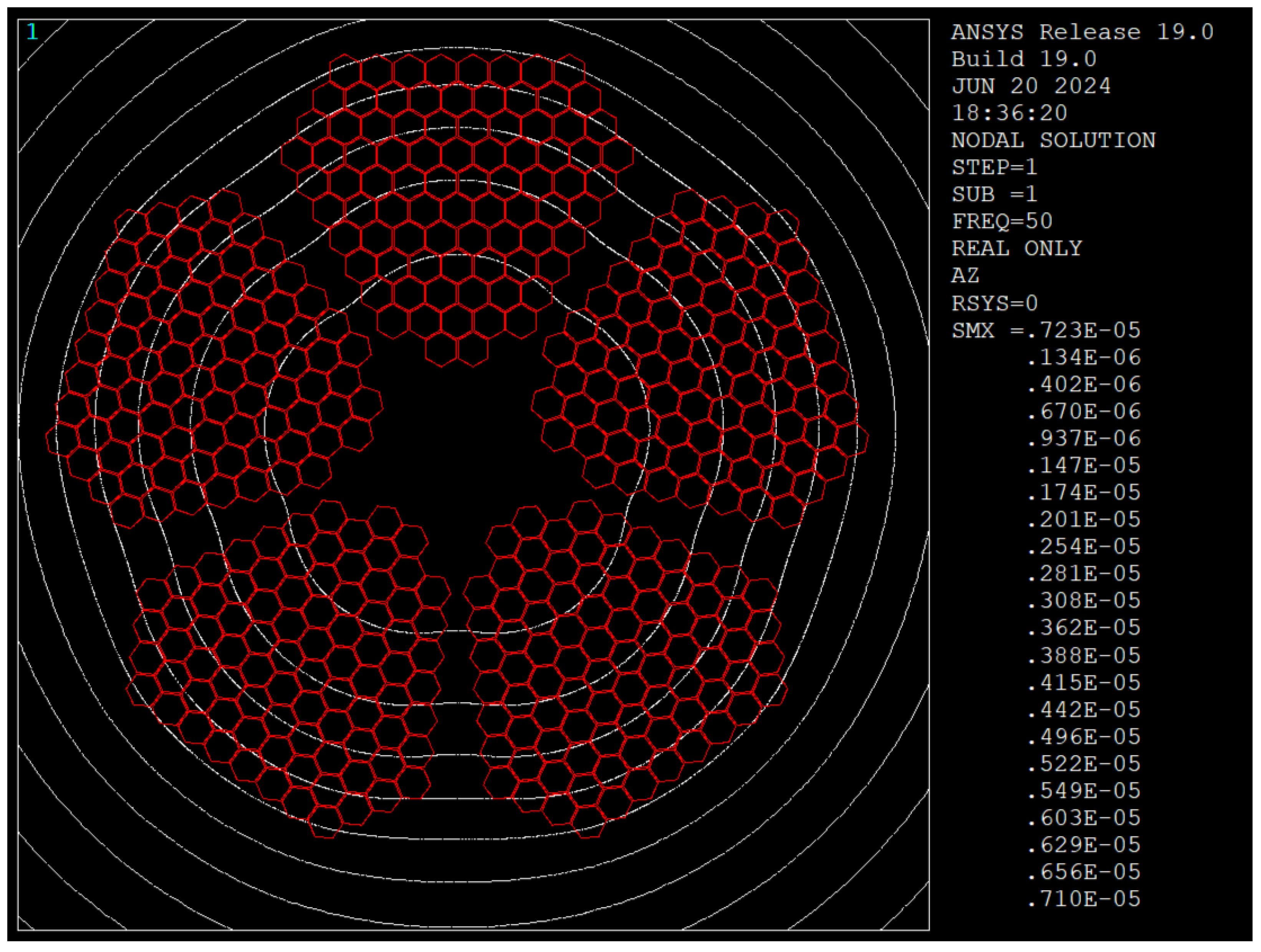
4.3. Comparison of Magnetic Flux Distributions
4.4. Model Validation at 50 Hz
4.5. Potential Energy and Cost Savings
5. Conclusions
- Conductor Insulation Efficacy: Adding a thin insulating layer between conductor strings effectively disrupts large eddy current loops, leading to more uniform current distribution and significantly lower AC resistance. A 75 µm layer alone substantially reduces both skin and proximity effects.
- High-Frequency Performance: At harmonic frequencies (150–250 Hz), the uninsulated cable’s ratio can climb to 3.66, whereas the insulated versions remain below 1.50, underscoring the insulation’s resilience to higher-order harmonics.
- Diminishing Returns: While increasing the layer thickness from 75 µm to 100 µm yields a small additional improvement, the marginal gains may not justify the added cost and manufacturing complexity.
- Magnetic Flux Dynamics: Simulations confirm that uninsulated segments concentrate flux lines at conductor boundaries, whereas insulating each conductor diffuses these fields, reducing proximity losses.
- Practical Value: Despite the potential challenges of integrating multiple thin insulation layers, the improved efficiency and reduced thermal stress make this approach well-suited to modern networks with a high harmonic content.
- Thicker Insulation and Capacitance: Further study is needed to see whether thicker insulation can yield additional loss reductions, balanced against increased capacitance and potential mechanical complexities that may affect high-frequency performance.
- Conductor Twisting and 3D Deformation: Considering conductor twisting may yield insights into how transposition interacts with electromagnetic phenomena. Three-dimensional modeling could also help capture mechanical stresses and deformations in greater detail.
- Geometric Dependencies: Additional research on the relationship between total cable cross-section area, the cross-sectional area of individual conductor strings, and their arrangement could clarify how these geometric factors influence .
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Morgan, V.T. The Effects of Frequency on the Proximity Losses in Cable Conductors. IEEE Trans. Power Deliv. 1985, 4, 1282–1289. [Google Scholar]
- Tang, X.; Qiu, Y.; Zhao, F. Finite Element Modeling of Proximity Effect in Segmented High-Voltage Cables. IEEE Trans. Magn. 2018, 54, 1–8. [Google Scholar]
- Okamoto, R.; Matsui, H. Experimental Validation of Segmented Cable Designs for High-Frequency Loss Reduction. Appl. Sci. 2022, 12, 2330. [Google Scholar]
- Lee, K.; Dominguez, B. Assessment of Eddy Currents in Multi-Strand Cables under Compressive Contact. In Proceedings of the 2019 IEEE International Conference on Electrical Systems, Kremenchuk, Ukraine, 23–25 September 2019; pp. 102–107. [Google Scholar]
- Okonkwo, O.; Li, X. A Review of Modern Harmonic Distortion Sources in HV Systems. IEEE Access 2023, 11, 54839–54850. [Google Scholar]
- Sunde, E.D. Earth Conduction Effects in Transmission Systems; Dover Publications: New York, NY, USA, 1968. [Google Scholar]
- Gao, Y.; Huang, D.X.; Ren, W. Impacts of Strand Insulation Thickness on Skin Effect Mitigation in HV Cables: A 3D FEM Study. IEEE Trans. Magn. 2017, 53, 1–6. [Google Scholar]
- Zhang, L.; Funaki, T. Skin and Proximity Effects in High-Frequency High-Power Cables for Inverter Applications. IEEE Trans. Ind. Appl. 2011, 47, 2165–2174. [Google Scholar]
- Brandt, D.; Brown, K.L.; Kim, Y.S. Effect of Air-Gap Insulation on Eddy Current Suppression in Medium-Voltage Conductors. IEEE Trans. Power Deliv. 2021, 36, 2779–2788. [Google Scholar]
- Chang, Y.; Wang, K.; Klein, M. Analytical and Numerical Study of High-Frequency Conductor Losses in Segmented Power Cables. IEEE Trans. Ind. Electron. 2021, 68, 10974–10983. [Google Scholar]
- IEEE 519-2014; IEEE Recommended Practice and Requirements for Harmonic Control in Electric Power Systems. Institute of Electrical and Electronics Engineers: New York, NY, USA, 2014.
- EN 50160; Voltage Characteristics of Electricity Supplied by Public Distribution Systems. European Committee for Electrotechnical Standardization: Brussels, Belgium, 2010.
- Carter, M.; Li, G.; Sun, Z. Reducing Skin-Effect Losses in High-Voltage Cables Using Insulated Segments: A 2D FEM Analysis. Energies 2020, 13, 1641. [Google Scholar]
- Peterson, J.; Garcia, N. Proximity Effects in Bundled Cables: A Theoretical and Experimental Comparison. Energies 2020, 13, 2369. [Google Scholar]
- Song, H.; Liu, J.; Zhu, P. Analysis of Segmented Conductor Designs with Partial Insulation Layers for Reduced AC Resistance. Appl. Sci. 2022, 12, 5150. [Google Scholar]
- Shin, H.; Kim, S.; Choi, T.Y. Reduction of Skin Effect in Thick Conductors through Layered Insulation: Experimental Results. In Proceedings of the 2016 IEEE Energy Conversion Congress and Exposition (ECCE), Milwaukee, WI, USA, 18–22 September 2016; pp. 4663–4669. [Google Scholar]
- Kumar, A.; Said, M.P.; Goh, T.Y. Finite Element Investigation of Layered Insulation in MV Cables under Harmonic Loads. IEEE Trans. Ind. Electron. 2023, 70, 7512–7523. [Google Scholar]
- Roth, R.; Yat, P.S. High Voltage and Electrical Insulation Engineering; Wiley: Hoboken, NJ, USA, 2010. [Google Scholar]
- Garcia, M.; Rebollo, J.; del Rio, L.J. High-Frequency Modeling of Shielded Power Cables for Harmonic Current Analysis. IEEE Trans. Magn. 2015, 51, 1–9. [Google Scholar]
- Johansson, B.; Borsje, P.M. Influence of Layered Insulation on Current Density Distribution in Compact Power Conductors. IET Electr. Power Appl. 2019, 13, 1162–1170. [Google Scholar]
- Quercio, M.; Del Pino Lopez, J.C.; Grasso, S.; Canova, A. Numerical and Experimental Analysis of Thermal Behaviour of High Voltage Power Cable in Unfilled Ducts. Sci. Rep. 2024, 14, 1–16. [Google Scholar] [CrossRef] [PubMed]
- Ahmadi, S.; Khan, S.H.; Grattan, K.T.V. Impact of Twisting on Skin and Proximity Losses in Segmented Underground Cables: A 3D Finite-Element Study. Appl. Sci. 2025, 15, 2814. [Google Scholar] [CrossRef]
- Khan, S.; Grattan, K.T.V.; Attwood, J.R. Finite Element Modelling of Skin and Proximity Effects in High Voltage Power Cables. In Proceedings of the COMPUMAG, Evian, France, 2–5 July 2001; pp. 2–5. [Google Scholar]
- Ahmadi, S.; Khan, S.H.; Grattan, K.T.V. Two-Dimensional vs. Three-Dimensional FE Modeling of Skin and Proximity Effects in Segmented Cables with Parallel Conductors: A Comparative Study. Appl. Sci. 2025, 15, 2981. [Google Scholar] [CrossRef]
- Harrington, R.F. Time-Harmonic Electromagnetic Fields; McGraw-Hill Series in Electrical Engineering: New York, NY, USA, 2001. [Google Scholar]
- Balanis, C.A. Advanced Engineering Electromagnetics; John Wiley & Sons: Hoboken, NJ, USA, 2012. [Google Scholar]
- Zhao, H.; Li, W. Assessment of Low-Frequency Harmonic Distortion in Large Urban Power Grids. IET Gener. Transm. Distrib. 2022, 16, 2005–2013. [Google Scholar]
- Abdel Aleem, S.H.E.; Ibrahim, A.M.; Zobaa, A.F. Harmonic assessment-based adjusted current total harmonic distortion. J. Eng. 2016, 2016, 64–72. [Google Scholar] [CrossRef]
- Kraus, J.D. Electromagnetics, 4th ed.; McGraw-Hill: New York, NY, USA, 1991. [Google Scholar]
- CIGRÉ Study Committee B1. Technical Brochure 880: High Voltage Cables; CIGRÉ: Paris, France, 2021. [Google Scholar]
- European Network of Transmission System Operators for Electricity (ENTSO-E): Ten-Year Network Development Plan. 2021. Available online: https://annualreport2021.entsoe.eu/ (accessed on 1 March 2025).
- Wholesale Market Indicators. 2023. Available online: https://www.ofgem.gov.uk/ (accessed on 1 March 2025).
- Electricity Transmission Network Data. 2023. Available online: https://www.nationalgrid.com/uk/electricity-transmission (accessed on 1 March 2025).
- International Energy Agency. World Energy Outlook. 2023. Available online: https://www.iea.org/reports/world-energy-outlook-2023 (accessed on 1 March 2025).



| Material | |||
|---|---|---|---|
| Copper | 1 | ||
| Air | 1 |
| Frequency (Hz) | Min J | Max J | (/m) | (/m) | ||
|---|---|---|---|---|---|---|
| 0 | 3688.68 | 3688.68 | 0.6359 | 6.36 | 6.36 | 1.00 |
| 50 | 2352.70 | 9860.40 | 1.1074 | 11.07 | 6.36 | 1.74 |
| 150 | 623.21 | 18,433.40 | 1.8317 | 18.32 | 6.36 | 2.88 |
| 250 | 174.83 | 24,904.80 | 2.3281 | 23.28 | 6.36 | 3.66 |
| Frequency (Hz) | Min J | Max J | (/m) | (/m) | ||
|---|---|---|---|---|---|---|
| 0 | 3686.43 | 3686.43 | 0.6359 | 6.36 | 6.36 | 1.00 |
| 50 | 3686.43 | 4032.74 | 0.6485 | 6.48 | 6.36 | 1.02 |
| 150 | 3668.50 | 6122.90 | 0.7485 | 7.49 | 6.36 | 1.18 |
| 250 | 3632.70 | 8933.35 | 0.9479 | 9.48 | 6.36 | 1.49 |
| Frequency (Hz) | Min J | Max J | (/m) | (/m) | ||
|---|---|---|---|---|---|---|
| 0 | 3686.57 | 3686.57 | 0.6359 | 6.36 | 6.36 | 1.00 |
| 50 | 3686.57 | 4022.14 | 0.6481 | 6.48 | 6.36 | 1.02 |
| 150 | 3669.73 | 6059.95 | 0.7449 | 7.45 | 6.36 | 1.17 |
| 250 | 3636.13 | 8813.77 | 0.9378 | 9.38 | 6.36 | 1.47 |
| Frequency (Hz) | Uninsulated | Insulation | Insulation |
|---|---|---|---|
| 0 | 1.00 | 1.00 | 1.00 |
| 50 | 1.74 | 1.02 | 1.02 |
| 150 | 2.88 | 1.18 | 1.17 |
| 250 | 3.66 | 1.49 | 1.47 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ahmadi, S.; Khan, S.H.; Grattan, K.T.V. Mitigating Skin and Proximity Effect in High-Voltage Underground Segmented Cables Through Individually Insulating Conductor Strings. Energies 2025, 18, 1605. https://doi.org/10.3390/en18071605
Ahmadi S, Khan SH, Grattan KTV. Mitigating Skin and Proximity Effect in High-Voltage Underground Segmented Cables Through Individually Insulating Conductor Strings. Energies. 2025; 18(7):1605. https://doi.org/10.3390/en18071605
Chicago/Turabian StyleAhmadi, Soheil, S. H. Khan, and K. T. V. Grattan. 2025. "Mitigating Skin and Proximity Effect in High-Voltage Underground Segmented Cables Through Individually Insulating Conductor Strings" Energies 18, no. 7: 1605. https://doi.org/10.3390/en18071605
APA StyleAhmadi, S., Khan, S. H., & Grattan, K. T. V. (2025). Mitigating Skin and Proximity Effect in High-Voltage Underground Segmented Cables Through Individually Insulating Conductor Strings. Energies, 18(7), 1605. https://doi.org/10.3390/en18071605






