The Effect of Nozzle Configuration on Adsorption-Chiller Performance
Abstract
1. Introduction
2. Materials and Methods
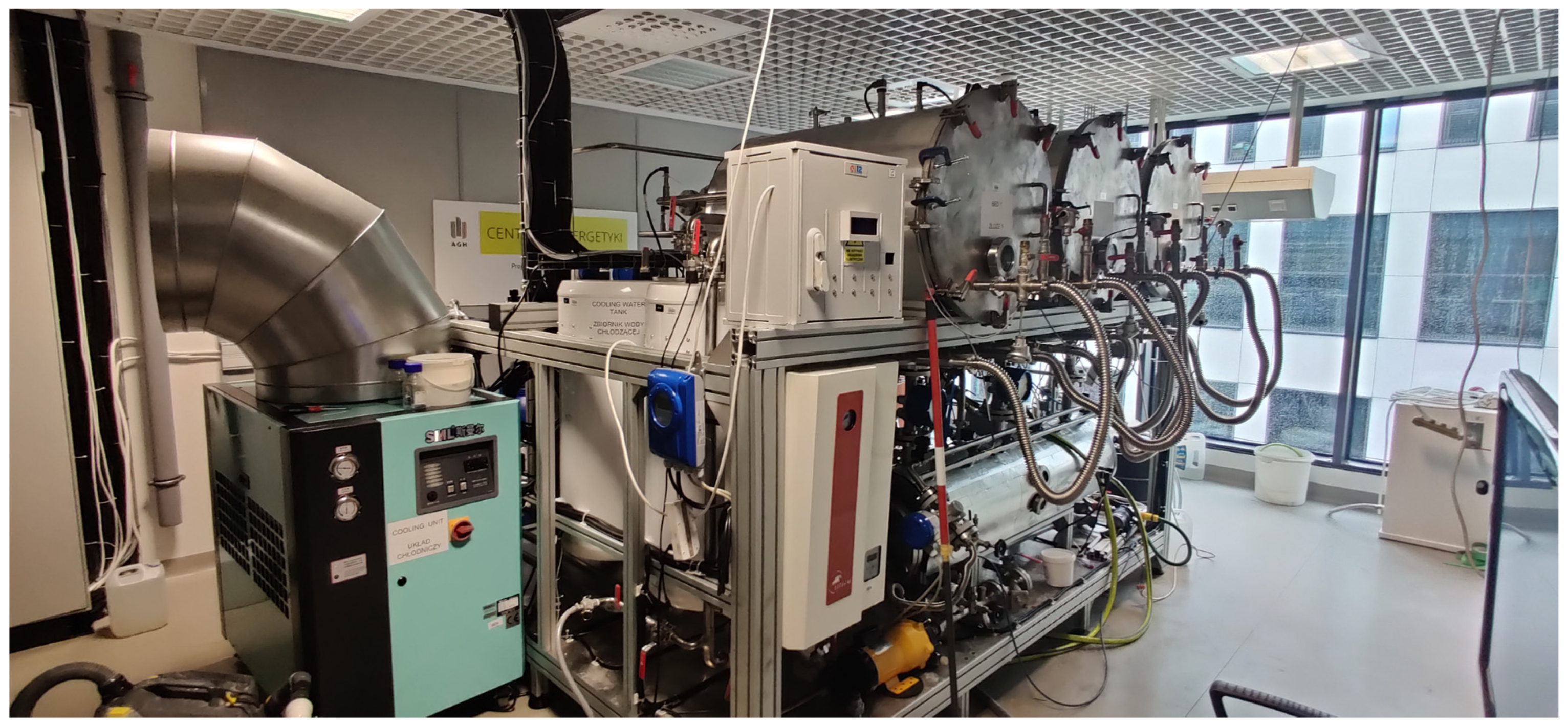
2.1. Experimental Setup
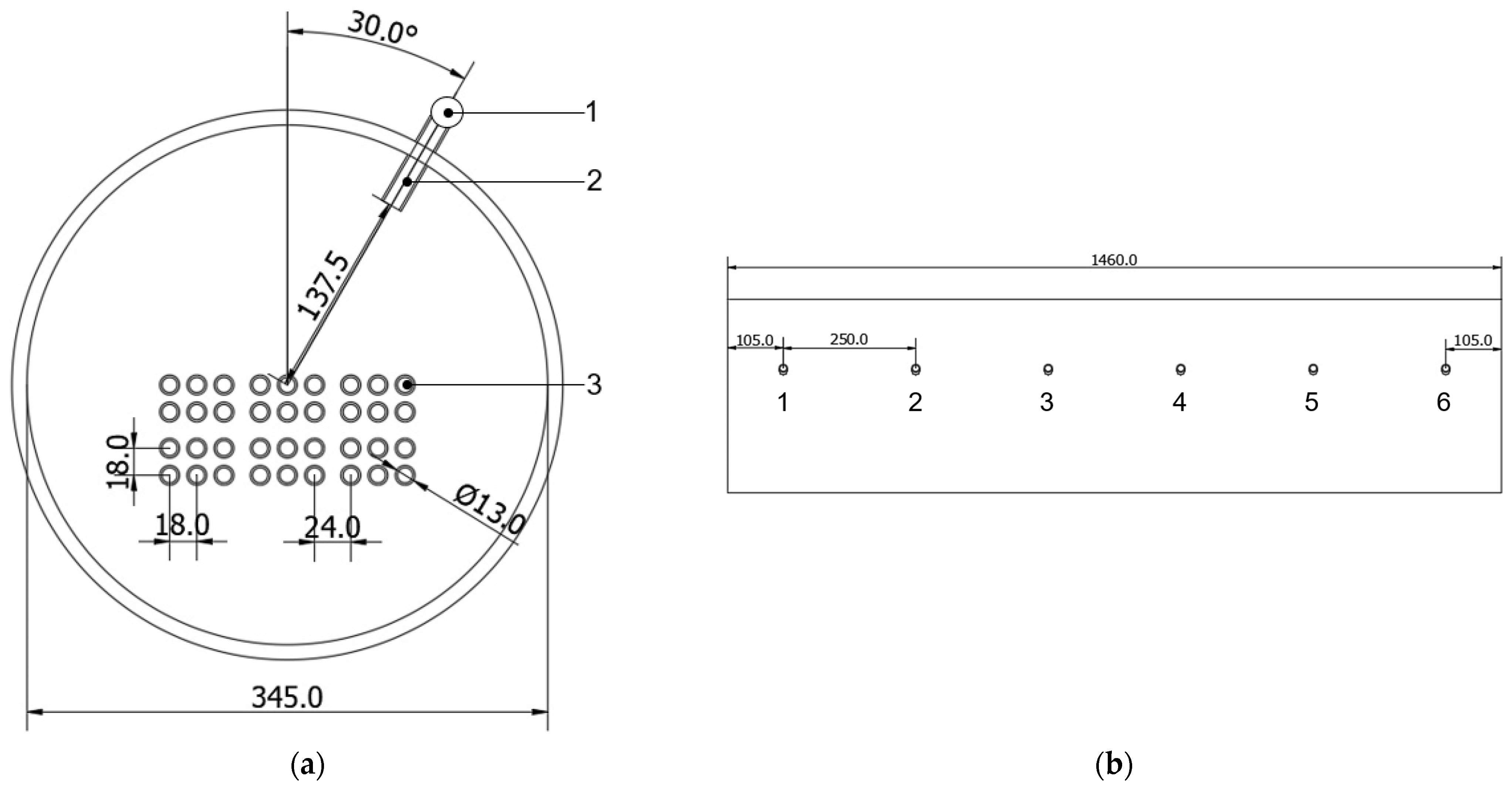
2.2. Nozzles in the Evaporator
2.3. Experimental Procedure and Measuring Devices
- 300 s—adsorption/desorption;
- 30 s—heat recovery;
- 60 s—bed regeneration.
3. Results
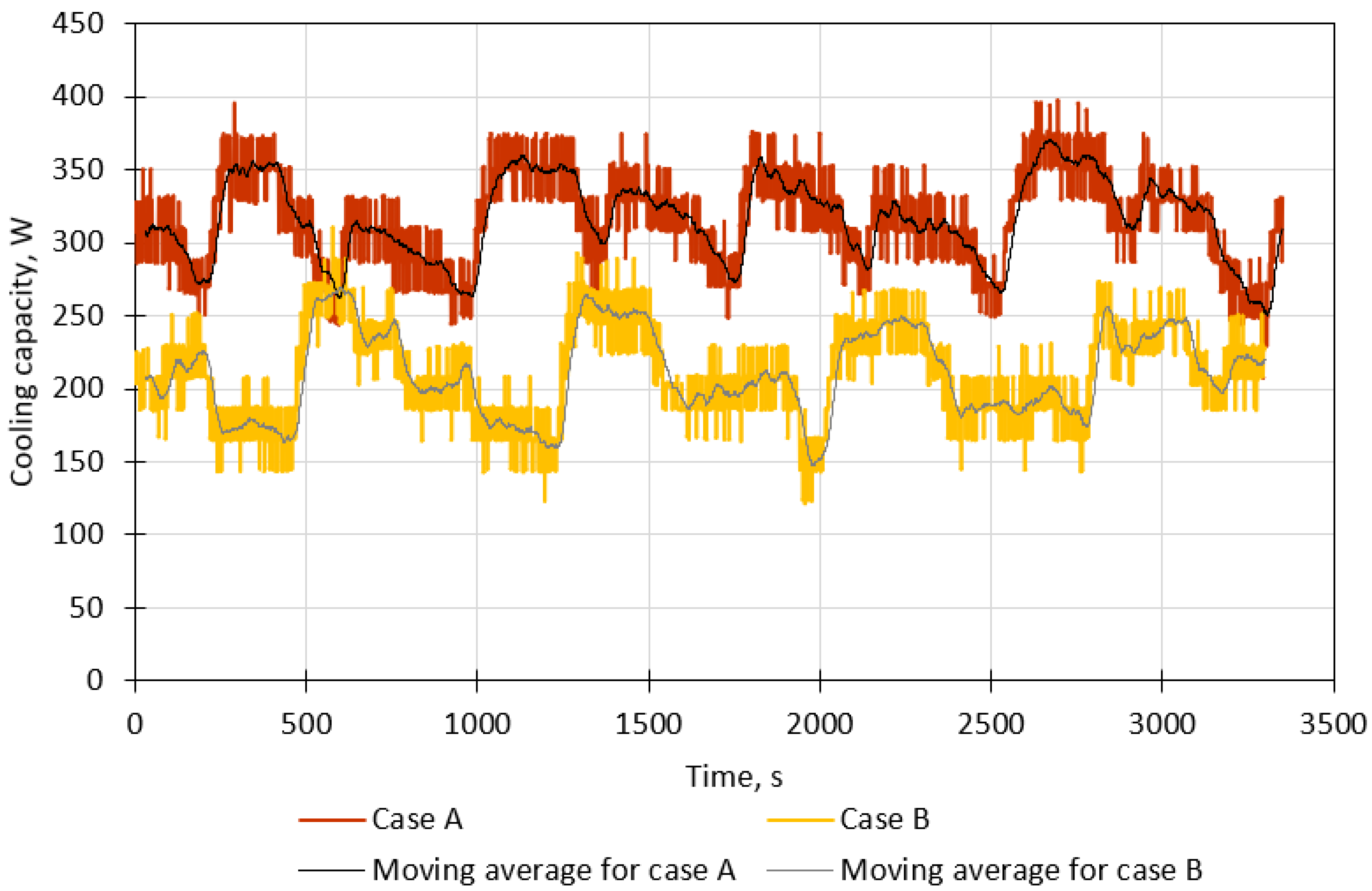
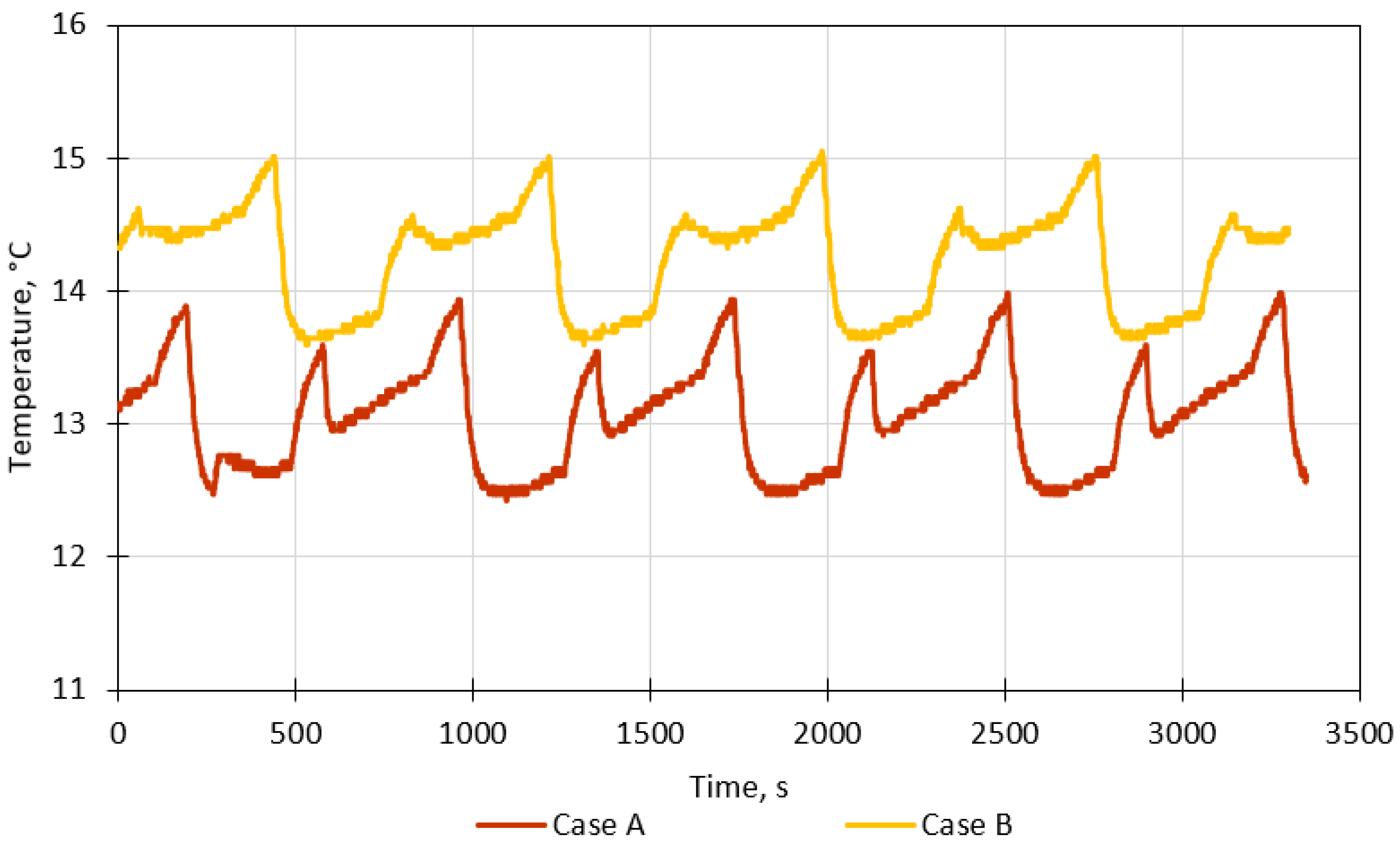
3.1. The Effect of Spray Angle on Adsorption-Chiller Performance
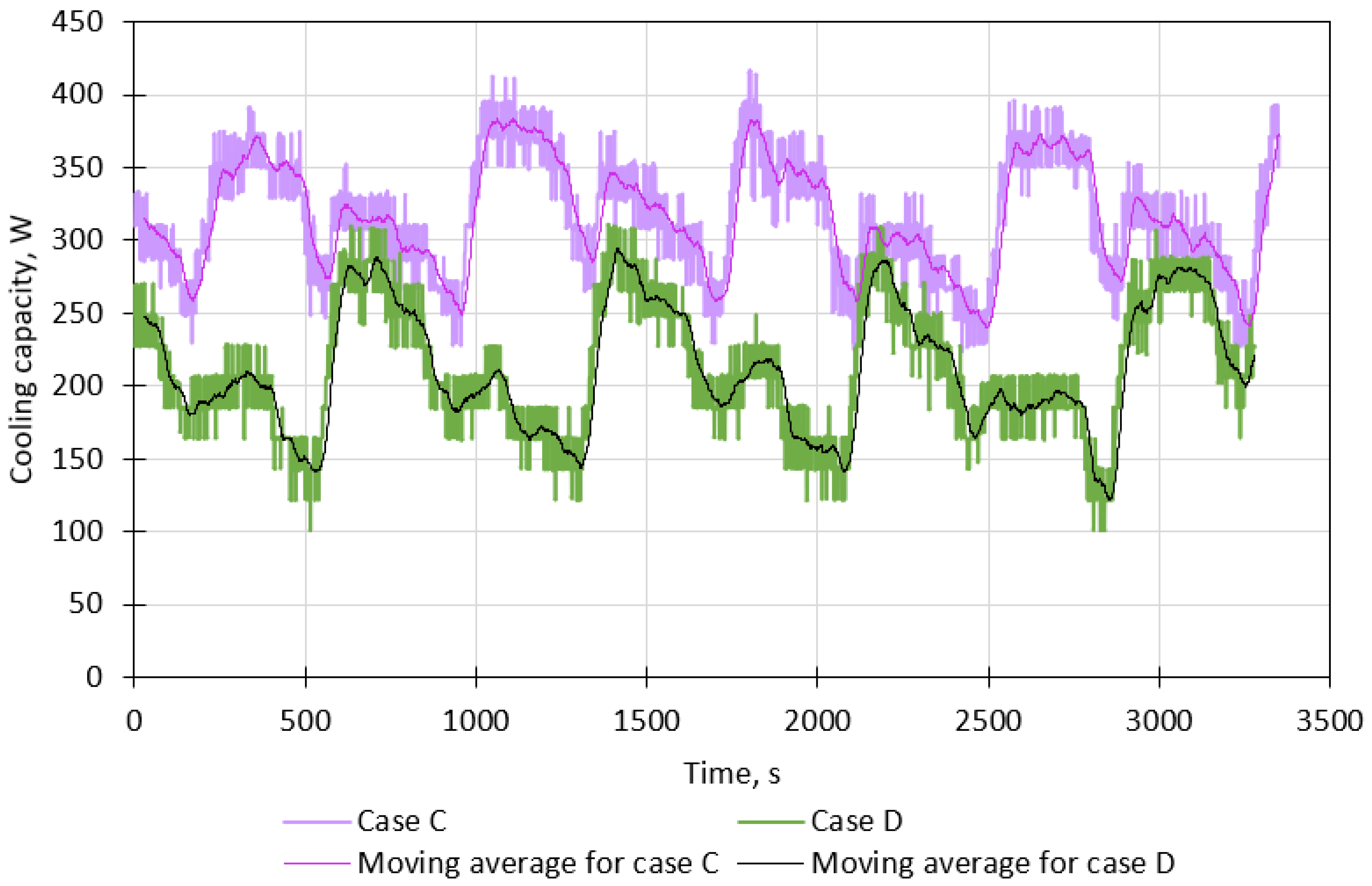
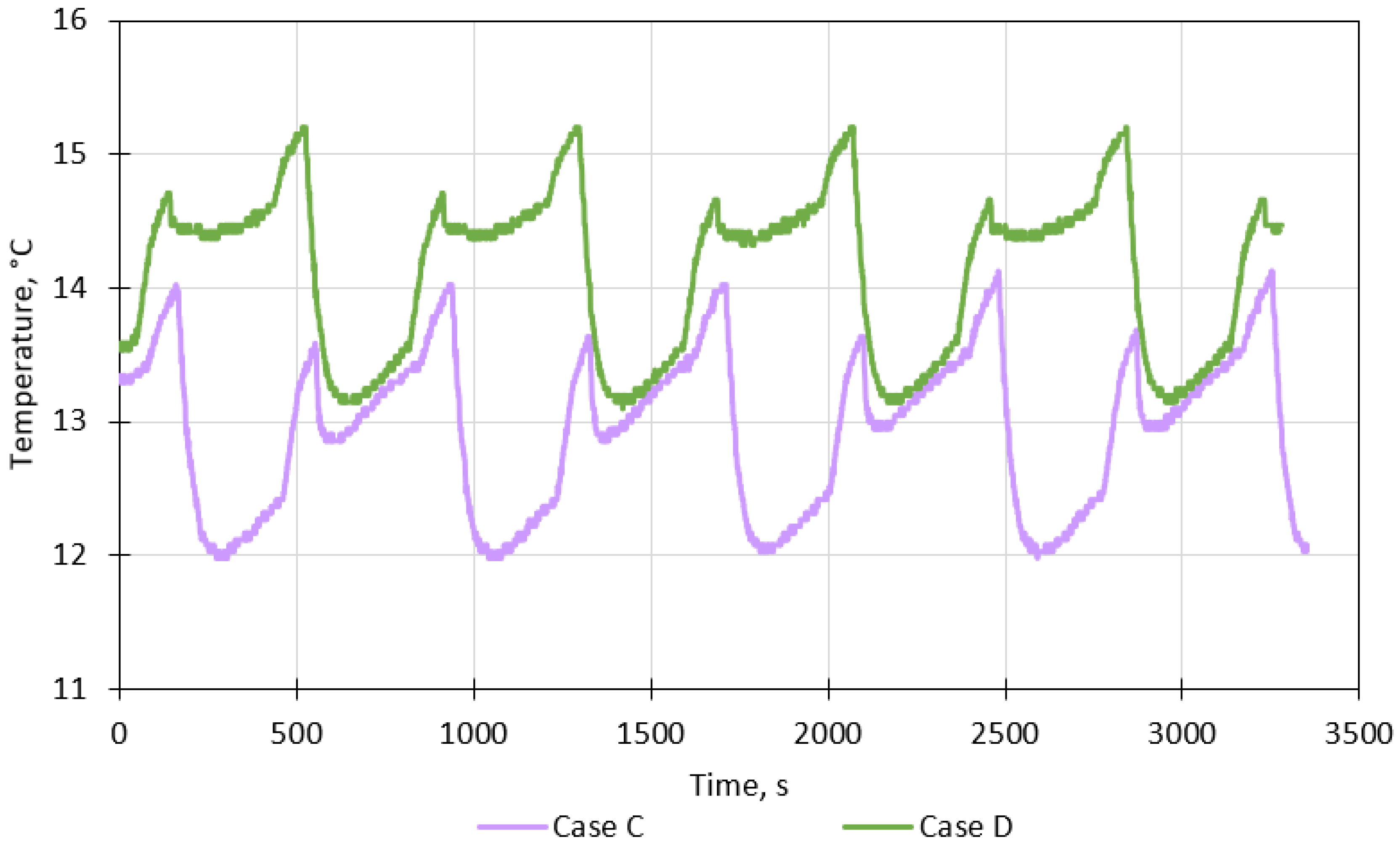
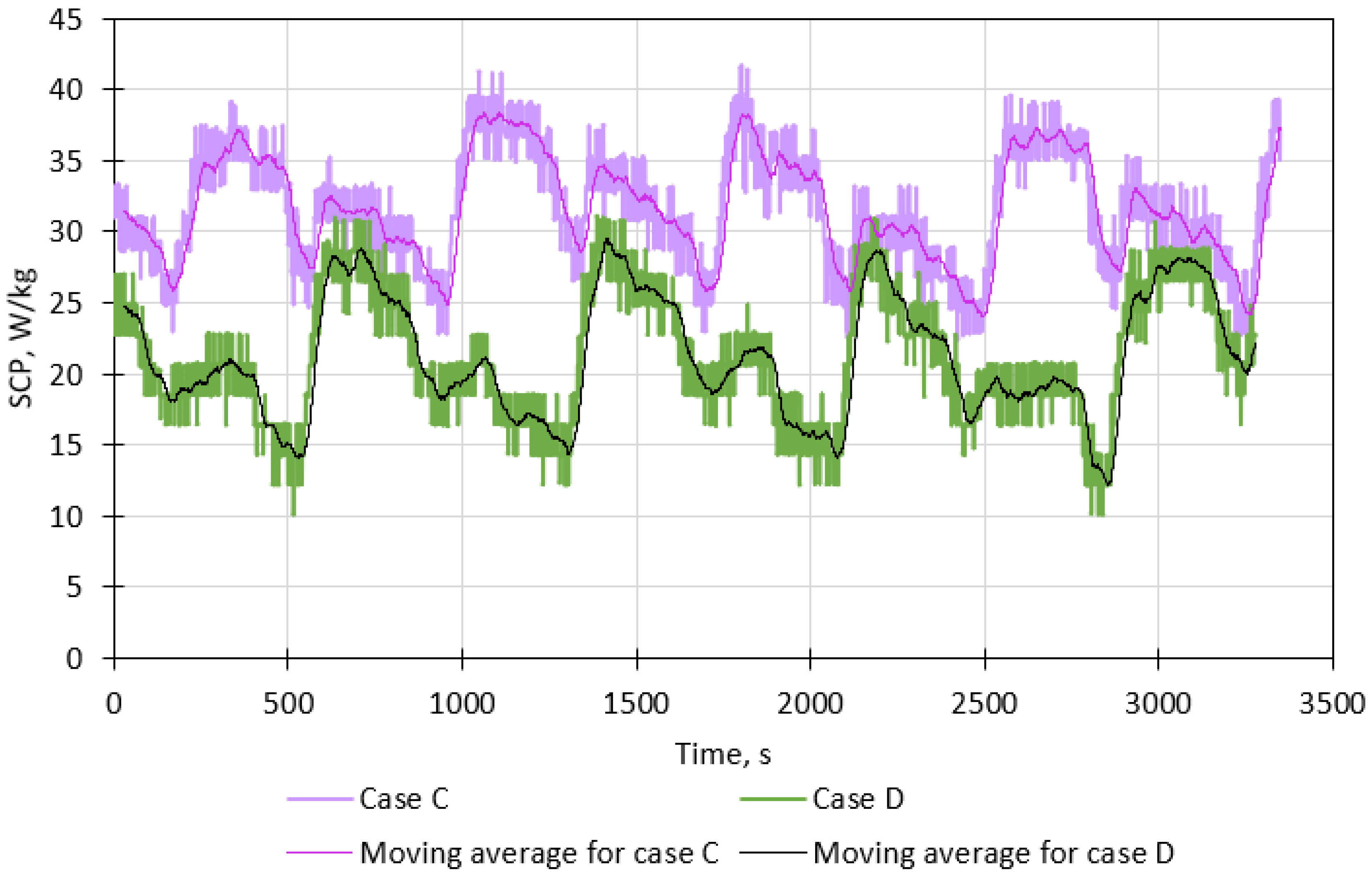
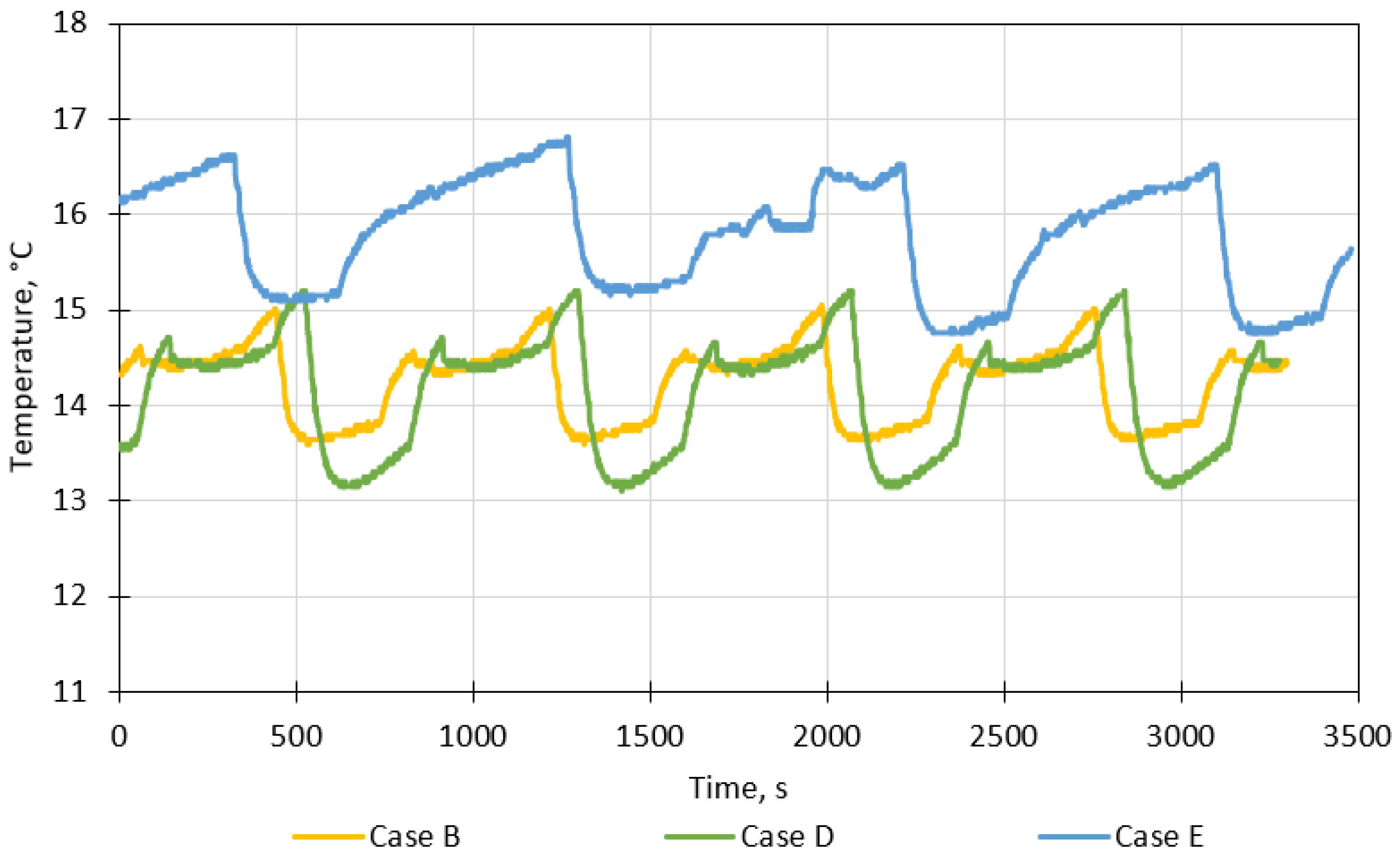
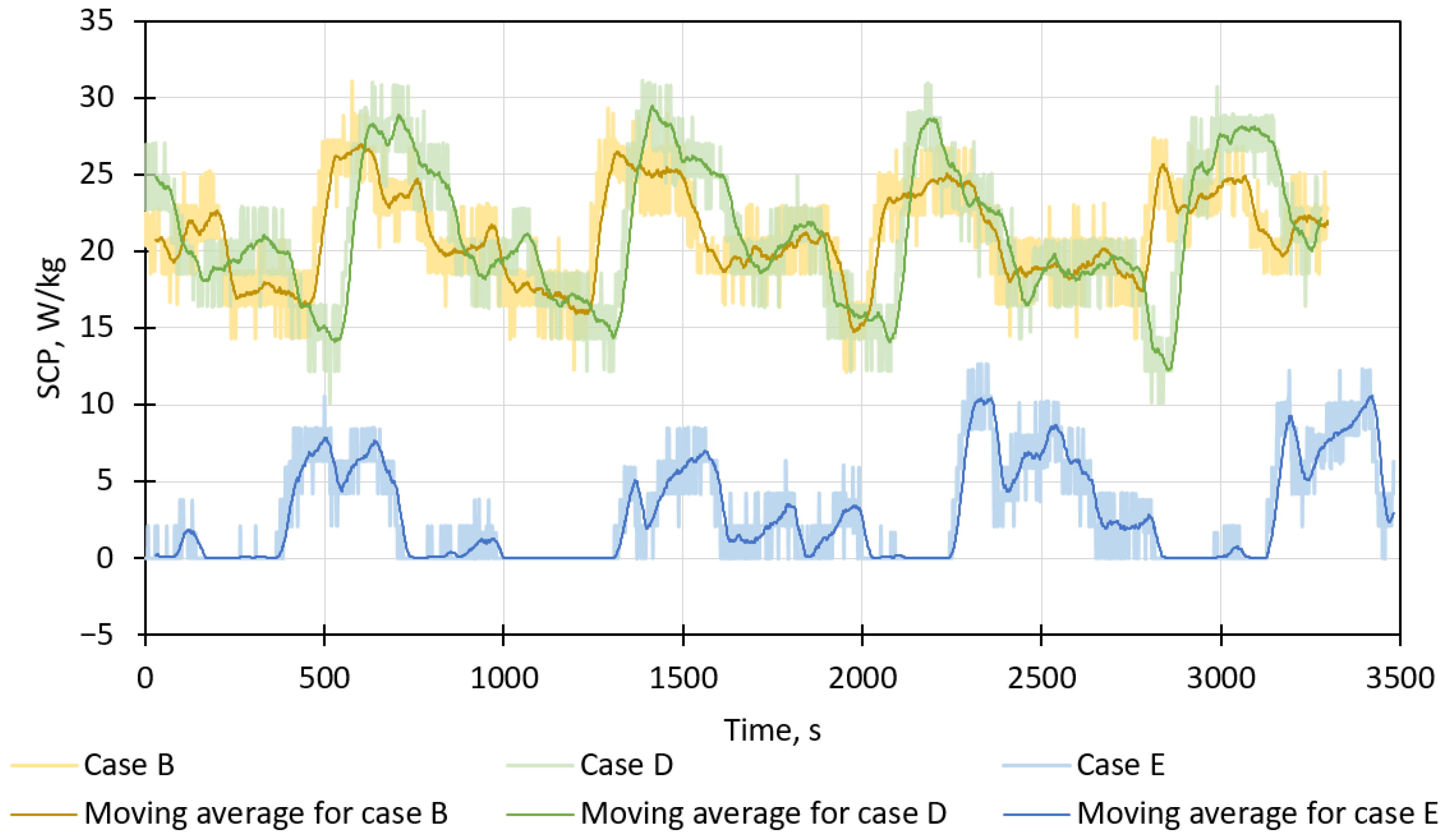
3.2. The Effect of the Number of Nozzles on Adsorption-Chiller Performance
3.3. Summary of Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- International Energy Agency. The Future of Cooling. Available online: https://www.iea.org/reports/the-future-of-cooling (accessed on 10 November 2023).
- Bolaji, B.O.; Huan, Z. Ozone depletion and global warming: Case for the use of natural refrigerant—A review. Renew. Sustain. Energy Rev. 2013, 18, 49–54. [Google Scholar] [CrossRef]
- Chauhan, P.R.; Kaushik, S.C.; Tyagi, S.K. Current status and technological advancements in adsorption refrigeration systems: A review. Renew. Sustain. Energy Rev. 2022, 154, 111808. [Google Scholar] [CrossRef]
- Alsaman, A.S.; Askalany, A.A.; Harby, K.; Ahmed, M.S. A state of the art of hybrid adsorption desalination–cooling systems. Renew. Sustain. Energy Rev. 2016, 58, 692–703. [Google Scholar] [CrossRef]
- Kuczyńska, A.; Szaflik, W. Absorption and adsorption chillers applied to air conditioning systems. Arch. Thermodyn. 2010, 31, 77–94. [Google Scholar] [CrossRef]
- Akhtar, S.; Khan, T.S.; Ilyas, S.; Alshehhi, M.S. Feasibility and Basic Design of Solar Integrated Absorption Refrigeration for an Industry. Energy Procedia 2015, 75, 508–513. [Google Scholar] [CrossRef]
- Sztekler, K.; Kalawa, W.; Nowak, W.; Mika, L.; Gradziel, S.; Krzywanski, J.; Radomska, E. Experimental study of three-bed adsorption chiller with desalination function. Energies 2020, 13, 5827. [Google Scholar] [CrossRef]
- Sztekler, K.; Kalawa, W.; Mika, L.; Lis, L.; Radomska, E.; Nowak, W. The effects of using steam to preheat the beds of an adsorption chiller with desalination function. Energies 2021, 14, 6454. [Google Scholar] [CrossRef]
- Iguchi, K.; Nakayama, M.; Akisawa, A. Method for estimating optimum cycle time based on adsorption chiller parameters. Int. J. Refrig. 2019, 105, 66–71. [Google Scholar] [CrossRef]
- Shmroukh, A.N.; Ali, A.H.H.; Ookawara, S. Adsorption working pairs for adsorption cooling chillers: A review based on adsorption capacity and environmental impact. Renew. Sustain. Energy Rev. 2015, 50, 445–456. [Google Scholar] [CrossRef]
- Thimmaiah, P.C.; Sharafian, A.; Rouhani, M.; Huttema, W.; Bahrami, M. Evaluation of low-pressure flooded evaporator performance for adsorption chillers. Energy 2017, 122, 144–158. [Google Scholar] [CrossRef]
- Kalawa, W.; Grabowska, K.; Sztekler, K.; Krzywański, J.; Sosnowski, M.; Stefański, S.; Siwek, T.; Nowak, W. Progress in design of adsorption refrigeration systems. Evaporators. EPJ Web Conf. 2019, 213, 02035. [Google Scholar] [CrossRef]
- Toppi, T.; Villa, T.; Vasta, S.; Mittelbach, W.; Freni, A. Testing of a Falling-Film Evaporator for Adsorption Chillers. Energies 2022, 15, 1709. [Google Scholar] [CrossRef]
- Woo, S.Y.; Lee, H.S.; Ji, H.; Moon, D.S.; Kim, Y.D. Silica gel-based adsorption cooling cum desalination system: Focus on brine salinity, operating pressure, and its effect on performance. Desalination 2019, 467, 136–146. [Google Scholar] [CrossRef]
- Spray Nozzles. Available online: https://www.spray-nozzle.co.uk/spray-nozzles (accessed on 12 November 2023).
- Cheng, W.L.; Zhang, W.W.; Chen, H.; Hu, L. Spray cooling and flash evaporation cooling: The current development and application. Renew. Sustain. Energy Rev. 2016, 55, 614–628. [Google Scholar] [CrossRef]
- Balaji, D. Experimental study on the effect of feed water nozzles on non-equilibrium temperature difference and flash evaporation in a single-stage evaporator and an investigation of effect of process parameters on the liquid flashing in a LTTD desalination process. Desalin. Water Treat. 2016, 57, 27152–27168. [Google Scholar] [CrossRef]
- Chien, L.H.; Xu, J.J.; Yang, T.F.; Yan, W.M. Experimental study on water spray uniformity in an evaporative condenser of a water chiller. Case Stud. Therm. Eng. 2019, 15, 100512. [Google Scholar] [CrossRef]
- Fathinia, F.; Khiadani, M.; Al-Abdeli, Y.M.; Shafieian, A. Performance improvement of spray flash evaporation desalination systems using multiple nozzle arrangement. Appl. Therm. Eng. 2019, 163, 114385. [Google Scholar] [CrossRef]
- Zhang, T.; Mo, Z.; Xu, X.; Liu, X.; Chen, H.; Han, Z.; Yan, Y.; Jin, Y. Advanced Study of Spray Cooling: From Theories to Applications. Energies 2022, 15, 9219. [Google Scholar] [CrossRef]
- Fathinia, F.; Khiadani, M.; Al-Abdeli, Y.M. Experimental and mathematical investigations of spray angle and droplet sizes of a flash evaporation desalination system. Powder Technol. 2019, 355, 542–551. [Google Scholar] [CrossRef]
- Chen, Q.; Thu, K.; Bui, T.D.; Li, Y.; Ng, K.C.; Chua, K.J. Development of a model for spray evaporation based on droplet analysis. Desalination 2016, 399, 69–77. [Google Scholar] [CrossRef]
- Chang, T.B.; Yu, L.Y. Optimal nozzle spray cone angle for triangular-pitch shell-and-tube interior spray evaporator. Int. J. Heat Mass Transf. 2015, 85, 463–472. [Google Scholar] [CrossRef]
- Sztekler, K.; Kalawa, W.; Nowak, W.; Mika, Ł.; Krzywański, J.; Grabowska, K.; Sosnowski, M.; Alharbi, A.A. Performance Evaluation of a Single-Stage Two-Bed Adsorption Chiller With Desalination Function. J. Energy Resour. Technol. 2021, 143, 082101. [Google Scholar] [CrossRef]
- Taylor, J.R. An Introduction to Error Analysis. In The Study of Uncertainties in Physical Measurements, 2nd ed.; University Science Books: Sausalito, CA, USA, 1997. [Google Scholar]
- Liu, X.; Yu, L.; Zhao, L.; Hua, Z.; Xue, H. A comprehensive simulation analysis to optimize the performance of spray evaporator on treating concentrated brine. Desalination 2023, 548, 116292. [Google Scholar] [CrossRef]
- Dai, Z.; Zhang, Y.; Wang, S.; Nawaz, K.; Jacobi, A. Falling-film heat exchangers used in desalination systems: A review. Int. J. Heat Mass Transf. 2022, 185, 122407. [Google Scholar] [CrossRef]





| Parameter | Value | Unit |
|---|---|---|
| Heating-water temperature | 80 | °C |
| Cooling-water temperature at the inlet to the beds | 20 | °C |
| Cooling-water temperature at the inlet to the condenser | 18 | °C |
| Supply-water temperature at the inlet to the evaporator | 15 | °C |
| Parameter | Measuring Device | Range of the Measuring Device | Accuracy of the Measuring Device |
|---|---|---|---|
| Condenser pressure (PT-10) | Pressure transducer Simex DMK-457, Simex, Gdańsk, Poland | From 0 to 99 kPa | ±0.5% |
| Cooling-water temperature—inlet to the condenser (TT-03) | Pt-1000 sensor JUMO 902020/15, JUMO Polska, Wrocław, Poland | From −80 to 150 °C | ±0.1 °C |
| Cooling-water temperature—outlet from the condenser (TT-02) | Pt-1000 sensor | From −80 to 150 °C | ±0.1 °C |
| Condenser cooling-water flow rate (FT-02) | Electromagnetic flow meter KHRONE Optiflux 4050C, Khrone Polska, Gdańsk, Poland | From 1 to 100 L/min | ±0.5% |
| Evaporator pressure (PT-04) | Pressure transducer | From 0 to 99 kPa | ±0.5% |
| Evaporator temperature (TT-01) | |||
| Pressure inside bed 1 (PT-05) | Pressure transducer | From 0 to 99 kPa | ±0.5% |
| Pressure inside bed 2 (PT-06) | Pressure transducer | From 0 to 99 kPa | ±0.5% |
| Chilled-water temperature—inlet to the evaporator (TT-06) | Pt-1000 sensor | From −80 to 150 °C | ±0.1 °C |
| Chilled-water temperature—outlet from the evaporator (TT-07) | Pt-1000 sensor | From −80 to 150 °C | ±0.1 °C |
| Chilled-water flow rate (FT-03) | Electromagnetic flow meter | From 1 to 100 L/min | ±0.5% |
| Heating-water flow rate | Electromagnetic flow meter | From 1 to 100 L/min | ±0.5% |
| Heating-water temperature—inlet to the bed (B2-01 and B3-01) | Pt-1000 sensor | From −80 to 150 °C | ±0.1 °C |
| Heating-water temperature—outlet from the bed (TT-04) | Pt-1000 sensor | From −80 to 150 °C | ±0.1 °C |
| Pressure, Bar | Water Flow Rate—Type 1 Nozzles, L/h | Water Flow Rate—Type 2 Nozzles, L/h |
|---|---|---|
| 0.5 | 54.0 | 34.2 |
| 1.0 | 64.2 | 45.6 |
| 2.0 | 108.0 | 60.0 |
| 3.0 | 132.6 | 70.8 |
| 5.0 | 153.0 | 86.4 |
| Experiment | Number of Nozzles | Type of Nozzle | Spray Angle, ° | Total Water Flow Rate, L/h | Water Flow Rate through One Nozzle, L/h |
|---|---|---|---|---|---|
| A | 6 | 1 | 120 | 400 | 66.7 |
| B | 6 | 2 | 90 | 400 | 66.7 |
| C | 6 | 1 | 120 | 200 | 33.3 |
| D | 6 | 2 | 90 | 200 | 33.3 |
| E | 3 | 2 | 90 | 200 | 66.7 |
| Parameter | Unit | A * | B * | C * | D * | E * |
|---|---|---|---|---|---|---|
| EER | - | 0.167 | 0.110 | 0.167 | 0.115 | 0.022 |
| Cooling capacity | W | 316 | 210 | 319 | 211 | 29 |
| SCP | W/kg | 31.6 | 21.0 | 31.9 | 21.1 | 2.9 |
| Condenser pressure | kPa | 5.71 | 6.09 | 5.80 | 6.27 | 6.39 |
| Evaporator pressure | kPa | 1.57 | 1.70 | 1.55 | 1.68 | 1.98 |
| Bed pressure during adsorption | kPa | 1.66 | 1.81 | 1.65 | 1.82 | 2.35 |
| Bed pressure during desorption | kPa | 5.80 | 6.17 | 5.90 | 6.35 | 6.47 |
| Evaporator temperature | °C | 13.06 | 14.24 | 12.91 | 14.12 | 15.78 |
| Chilled-water temperature at the inlet to the evaporator | °C | 15.22 | 15.60 | 15.17 | 15.59 | 15.81 |
| Chilled-water temperature at the outlet from the evaporator | °C | 14.47 | 15.10 | 14.42 | 15.09 | 15.76 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kalawa, W.; Sztekler, K.; Kozaczuk, J.; Mika, Ł.; Radomska, E.; Nowak, W.; Gołdasz, A. The Effect of Nozzle Configuration on Adsorption-Chiller Performance. Energies 2024, 17, 1181. https://doi.org/10.3390/en17051181
Kalawa W, Sztekler K, Kozaczuk J, Mika Ł, Radomska E, Nowak W, Gołdasz A. The Effect of Nozzle Configuration on Adsorption-Chiller Performance. Energies. 2024; 17(5):1181. https://doi.org/10.3390/en17051181
Chicago/Turabian StyleKalawa, Wojciech, Karol Sztekler, Jakub Kozaczuk, Łukasz Mika, Ewelina Radomska, Wojciech Nowak, and Andrzej Gołdasz. 2024. "The Effect of Nozzle Configuration on Adsorption-Chiller Performance" Energies 17, no. 5: 1181. https://doi.org/10.3390/en17051181
APA StyleKalawa, W., Sztekler, K., Kozaczuk, J., Mika, Ł., Radomska, E., Nowak, W., & Gołdasz, A. (2024). The Effect of Nozzle Configuration on Adsorption-Chiller Performance. Energies, 17(5), 1181. https://doi.org/10.3390/en17051181






