Research Developments and Applications of Ice Slurry
Abstract
1. Introduction
2. Ice Slurry Production Methods
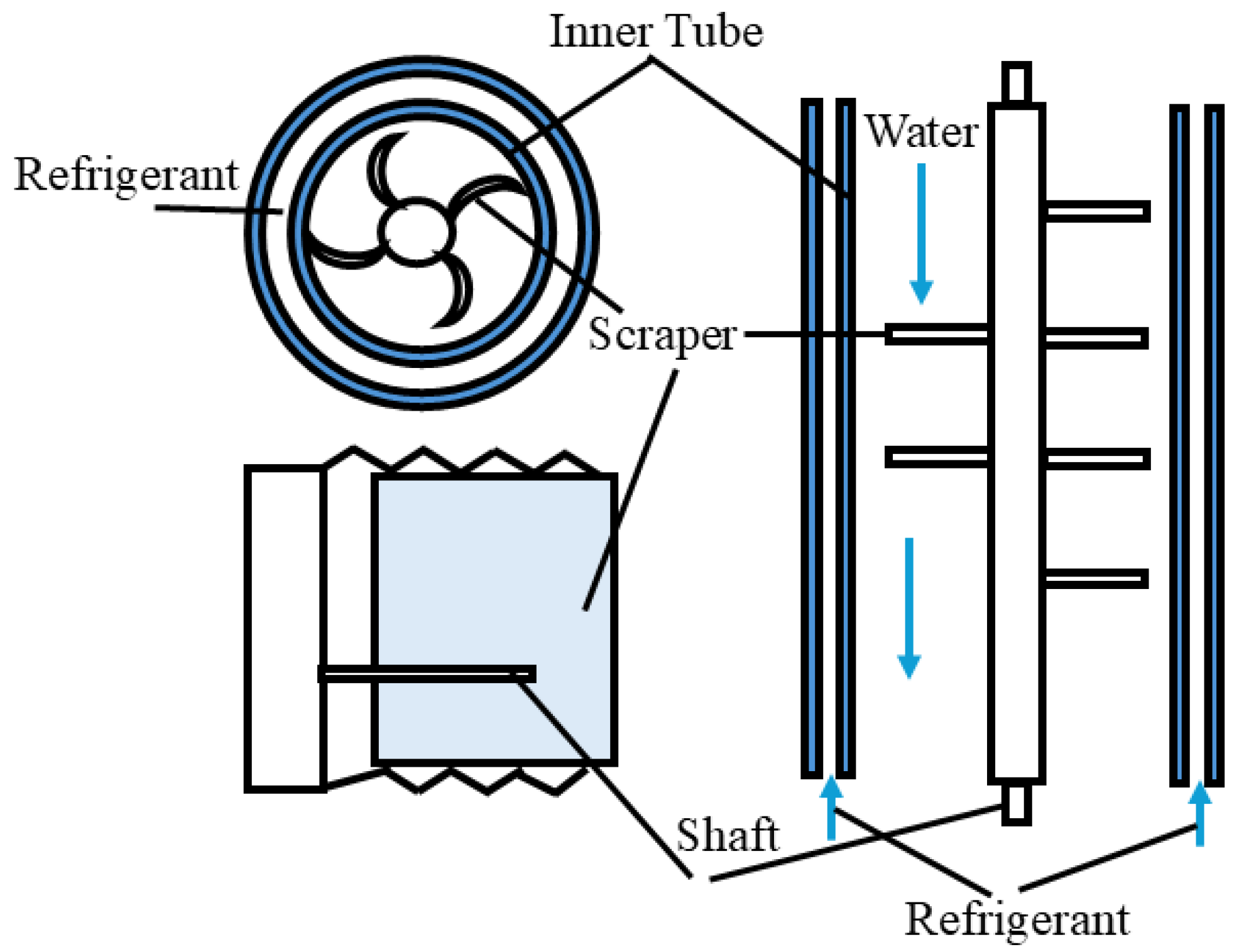
2.1. Surface-Scraping Method
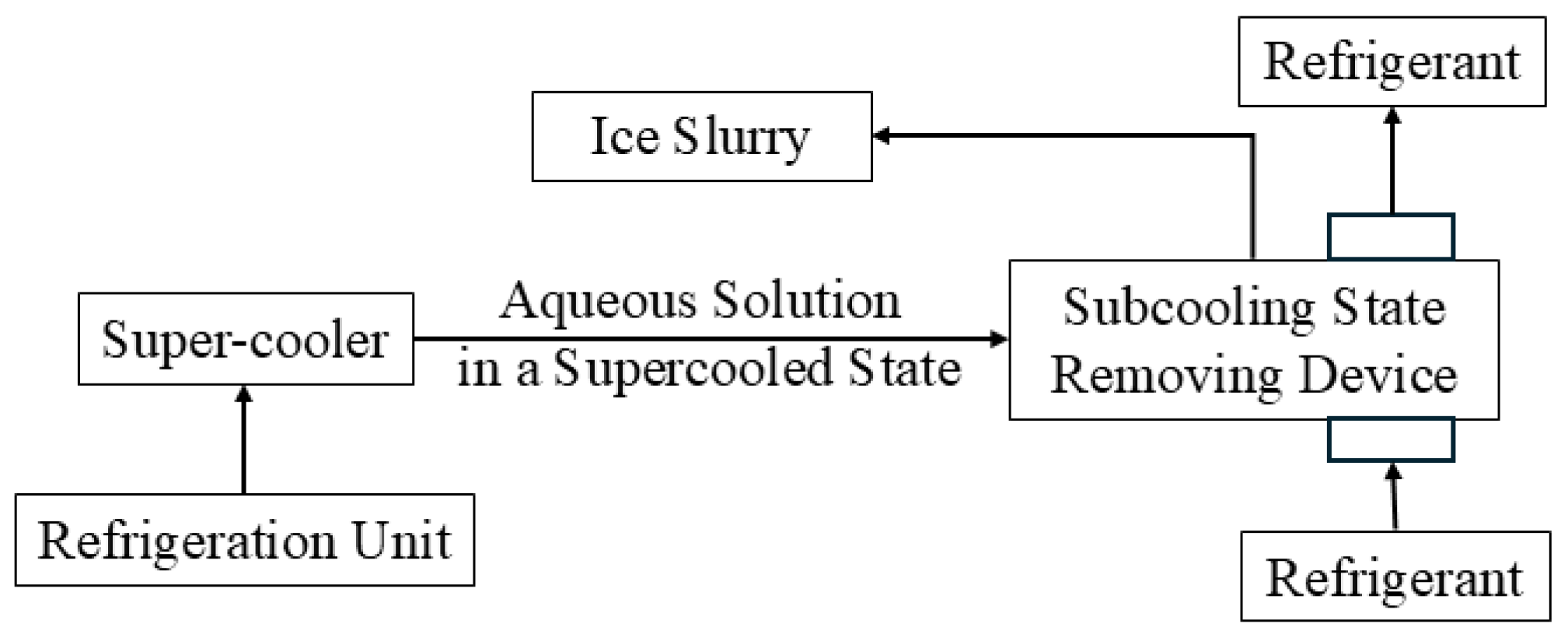
2.2. Supercooling Method
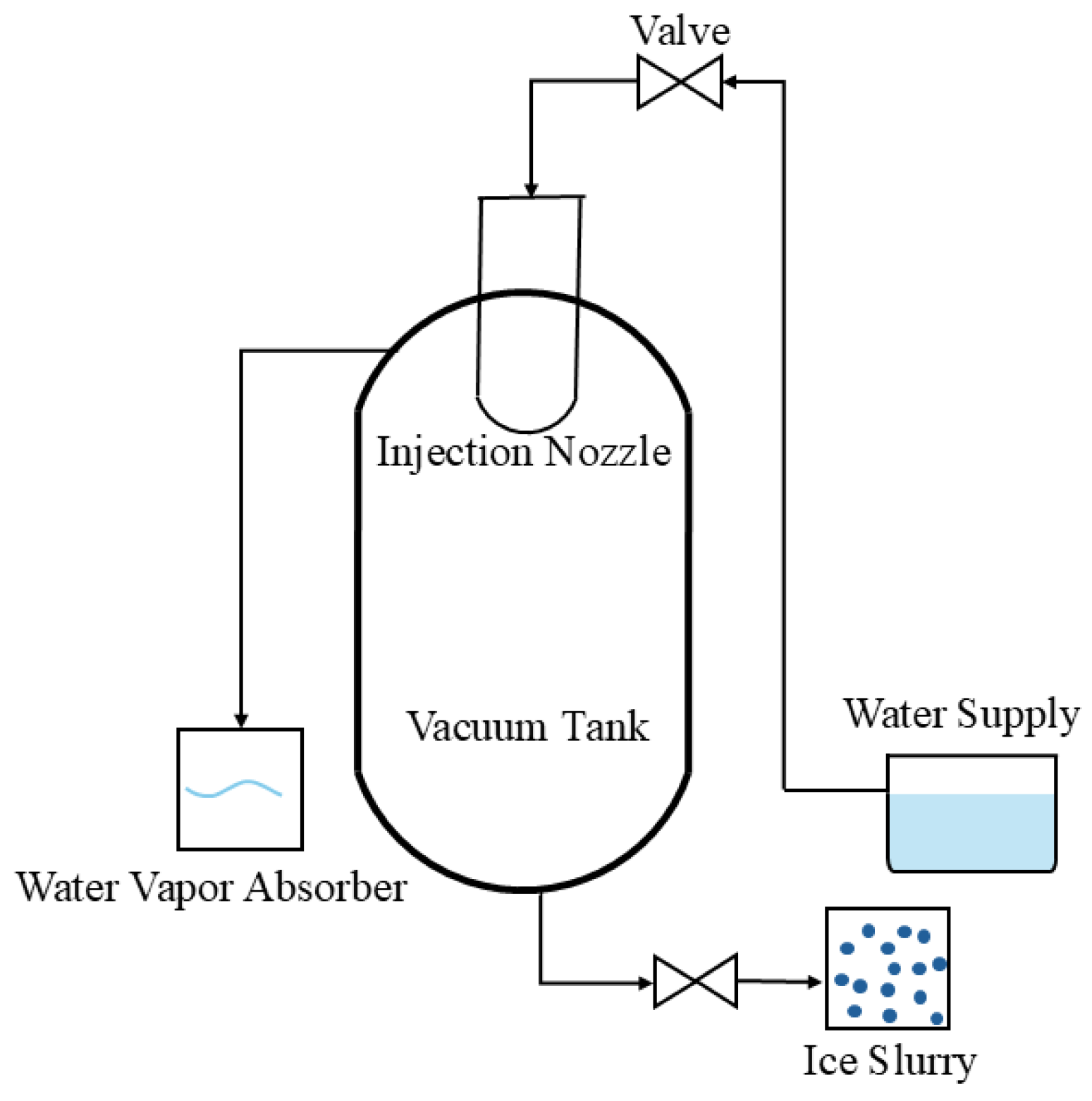
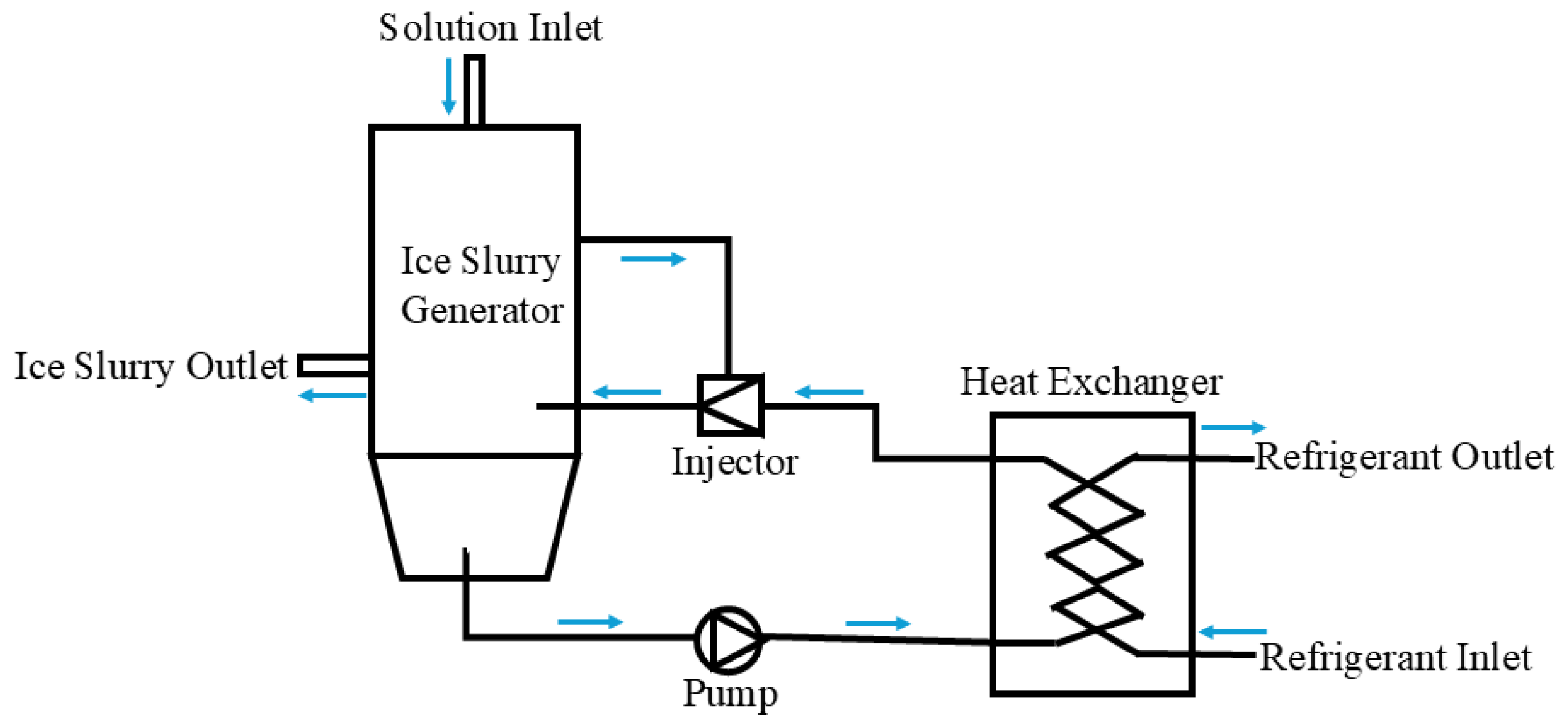
2.3. Vacuum Method
2.4. Fluidized Bed Method
2.5. Direct Contact Method
2.6. Deicing Method
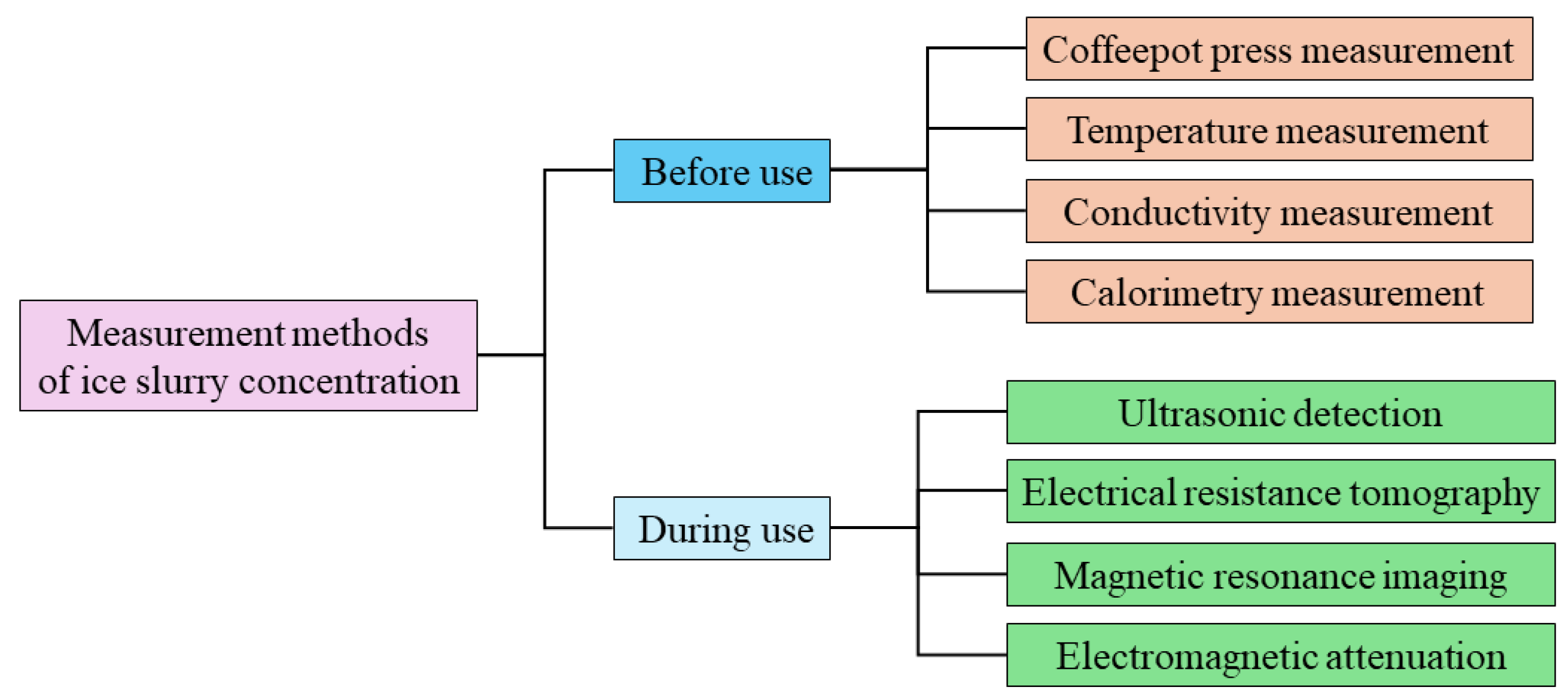
3. Measurement Methods of Ice Slurry Concentration
3.1. Measurement Prior Use
3.1.1. Coffeepot Press Measurement
3.1.2. Temperature Measurement
3.1.3. Conductivity Measurement
3.1.4. Calorimetry Measurement
3.2. Measurement During Use
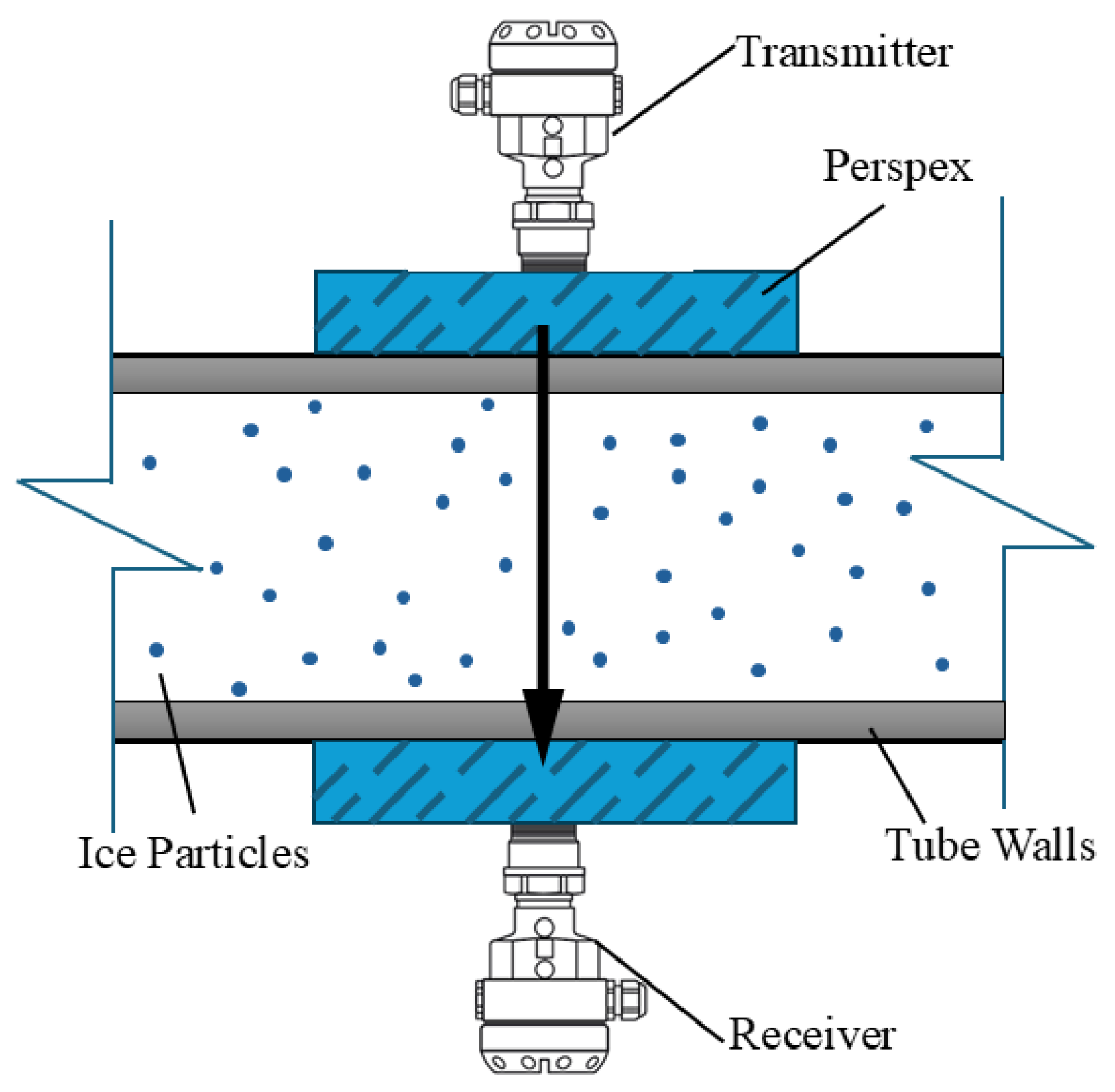
3.2.1. Ultrasonic Detection
3.2.2. Electrical Resistance Tomography
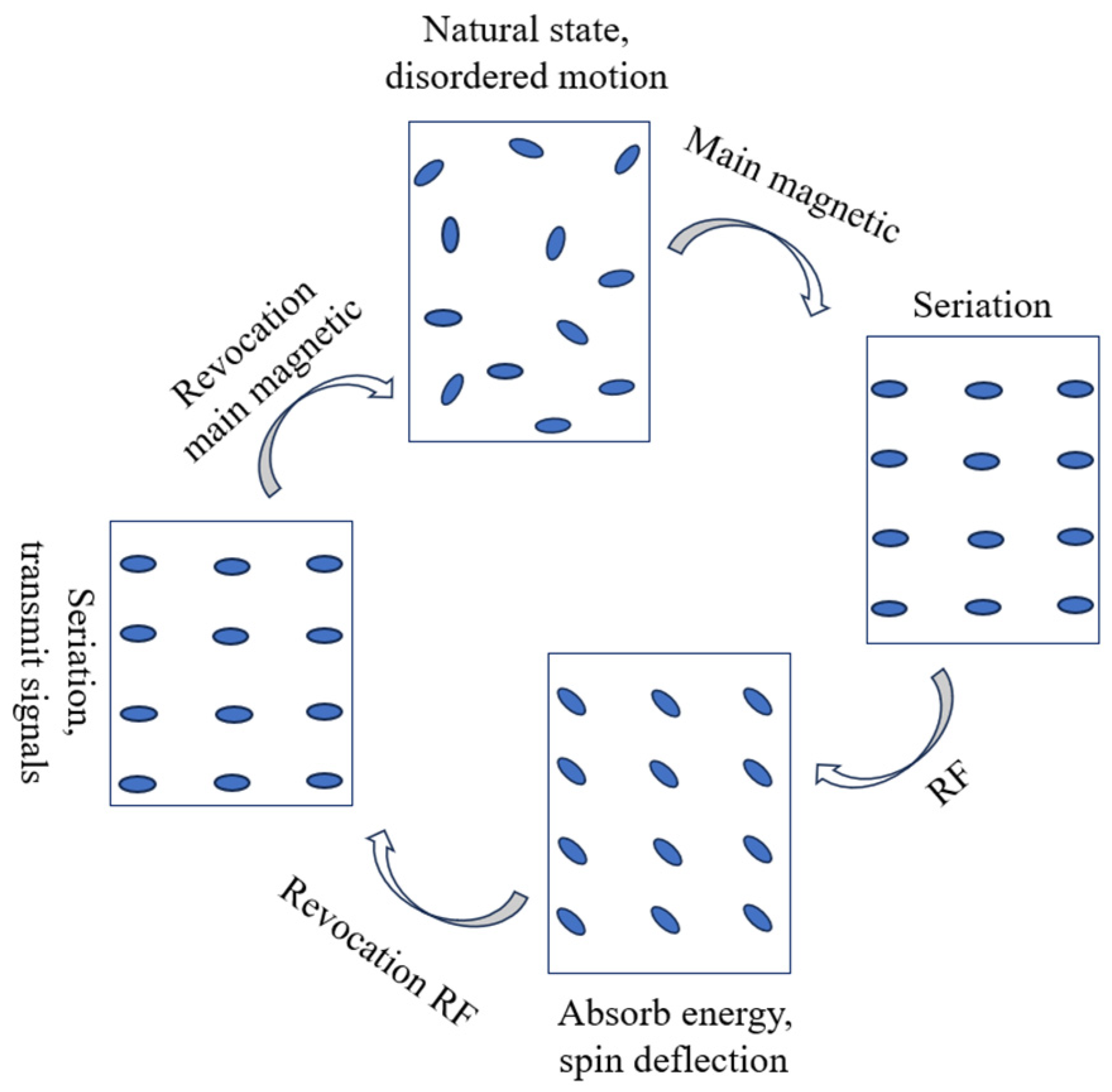
3.2.3. Magnetic Resonance Imaging
3.2.4. Electromagnetic Attenuation
4. Flow Characteristics of Ice Slurry
4.1. Flow Patterns
4.2. Rheological Behavior
| Viscosity Formula | Solid Fraction | Fluid Type | Description | References |
|---|---|---|---|---|
| <15% | Newtonian | No consideration of particle diameter and interaction between particles | [60] | |
| <62.5% | Newtonian/ non-Newtonian | Particle diameters: 0.099–435 μm; Interaction between particles considered | [61] | |
| <58.97% | Newtonian/ non-Newtonian | Shear viscosity increases with particle concentration | [63] | |
| >15% | non-Newtonian | Power law model | [65,66] | |
| >15% | non-Newtonian | Bingham model | [67,68] | |
| >15% | non-Newtonian | Herschel-Bulkley model | [69,70] | |
| >15% | non-Newtonian | Casson model | [71] |
4.3. Pressure Drop
5. Heat Transfer Characteristics of Ice Slurry
5.1. Heat Transfer Coefficient
5.2. Variation Rules of Heat Transfer Coefficient
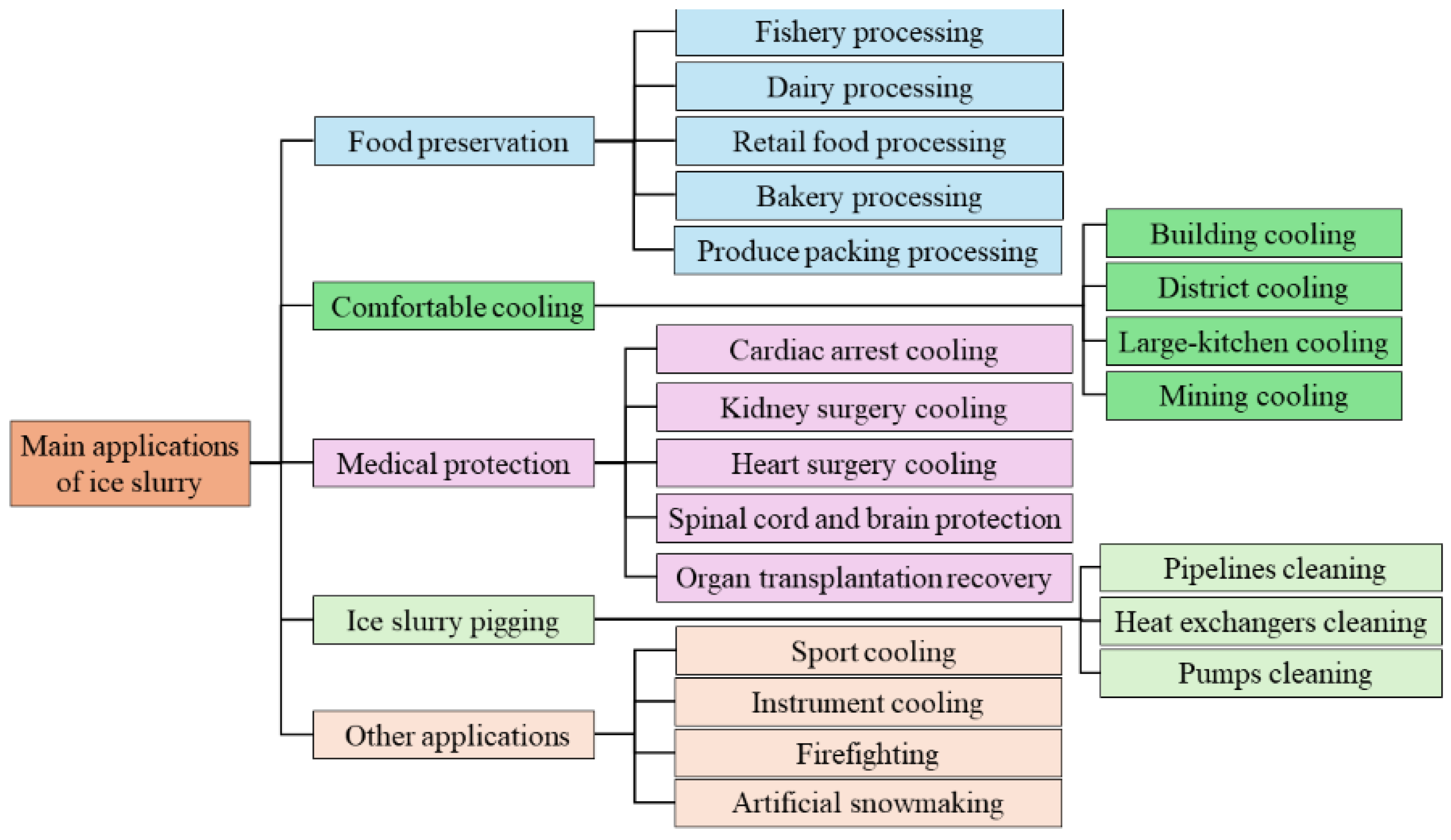
6. Applications
6.1. Food Preservation
6.1.1. Fishery Processing
6.1.2. Dairy and Retail Food Processing
6.1.3. Bakery Processing
6.1.4. Packing Processing
6.2. Comfortable Cooling
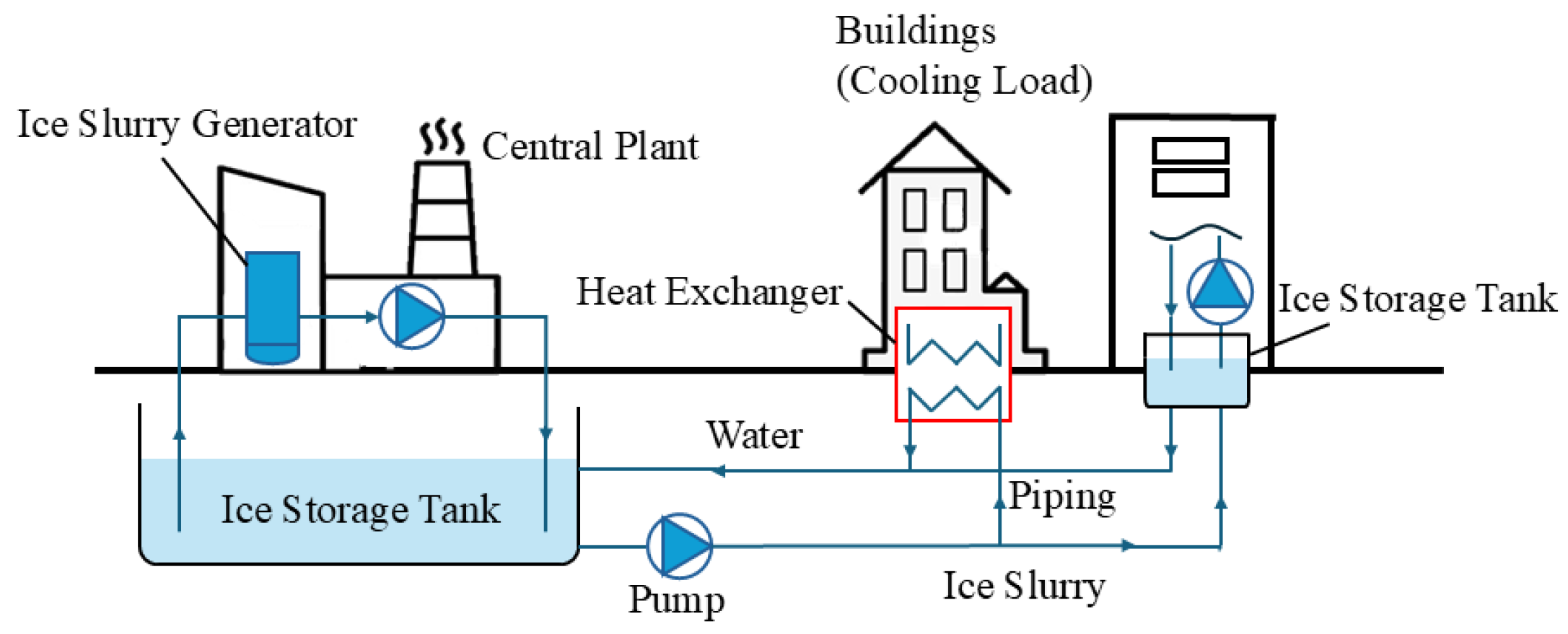
6.2.1. Building Cooling
6.2.2. District and Large Kitchen Cooling
6.2.3. Mining Cooling
6.3. Medical Protective Cooling
6.3.1. Cardiac Arrest Cooling
6.3.2. Kidney Surgery Cooling
6.3.3. Other Medical Protective Cooling
6.4. Ice Slurry Pigging
6.5. Other Applications
7. Conclusions
- The production methods of ice slurry mainly include surface-scraping, supercooling, vacuum, fluidized bed, and direct contact methods. Among them, the surface-scraping method is still the most widely used approach due to its mature technology. Other methods are still under development and are not ready for full commercialization yet.
- The concentration of ice crystals in ice slurry is changing due to the heat exchange. The measurement of ice crystal concentration is divided into two steps: prior and current use. For the prior use, the common methods are the coffeepot press measurement, temperature measurement, conductivity measurement, and calorimetry measurement. For ice slurry flowing in pipelines, non-invasive approaches are adopted to measure its concentration, including ultrasonic detection, electrical resistance tomography, magnetic resonance imaging, and electromagnetic attenuation, which do not affect the ice crystal movements and carrier fluid flows and ensure the synchronization of the concentration and velocity measurement compared to intrusive detection.
- The flow characteristics of ice slurry are more complex than those of normal Newtonian fluids. There are four rheological models suitable for ice slurry as a non-Newtonian fluid: the Power law model, the Bingham model, the Herschel-Bulkley model, and the Casson model. Based on numerous studies on the rheological behavior and the pressure drop change of ice slurry, a generally accepted result is that the pressure drop of ice slurry increases with flow rate and ice concentration.
- Likewise, the heat transfer characteristics of ice slurry are also more complex than those of normal Newtonian fluids. Based on previous studies on the heat transfer property of ice slurry, the generally accepted conclusion is that the heat transfer coefficient of ice slurry increases with ice concentration, which is due to the phase change with a large latent heat.
- To date, ice slurry has been used in many industries, such as food preservation, comfortable cooling, medical protective cooling, pipeline cleaning, sport cooling, instrument cooling, firefighting, artificial snowmaking, etc. Most of these applications utilize its cooling. Pipeline pigging applications use the friction between the ice slurry with high concentration and the inner wall of the pipe, where the friction from the ice slurry is several times greater than that of water at the same flow rate.
- A better production method that is efficient, reliable, energy-saving, mass-production, and fully commercial.
- A better storage method that can control the growth of ice crystals in the ice slurry, prevent ice slurry from clumping, and ensure its smoothness and uniformity.
- A better concentration measurement method that is nonintrusive, accurate, easily operated, and cheap.
- A clearer relationship between rheological and heat transfer characteristics, including flow rate, ice concentration, ice volume, diameter, length, material, and shape of the equipment, through experiments and numerical simulations.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Bellas, I.; Tassou, S.A. Present and future applications of ice slurries. Int. J. Refrig. Rev. Int. Du Froid 2005, 28, 115–121. [Google Scholar] [CrossRef]
- Kuriyama, T.; Sawahata, Y. Slurry ice transportation and cold distribution system. In Proceedings of the Information Booklet for the Technical Tour of the Fourth Workshop of IIR Ice Slurry, Working Party, Kyoto, Japan, 13 November 2001; pp. 1–6. [Google Scholar]
- Evans, T.S. Technical Aspects of Pipeline Pigging with Flowing Ice Slurries; University of Bristol: Bristol, UK, 2007. [Google Scholar]
- Tabuchi, S.; Horie, S.; Kawanami, S.; Inoue, D.; Morizane, S.; Inoue, J.; Nagano, C.; Sakurai, M.; Serizawa, R.; Hamada, K. Efficacy of ice slurry and carbohydrate–electrolyte solutions for firefighters. J. Occup. Health 2021, 63, e12263. [Google Scholar] [CrossRef] [PubMed]
- Tuchayi, S.M.; Wang, Y.; Khodorova, A.; Pence, I.J.; Evans, C.L.; Anderson, R.R.; Lerner, E.A.; Woolf, C.J.; Garibyan, L. Cryoneurolysis with injectable ice slurry modulates mechanical skin pain. J. Investig. Dermatol. 2023, 143, 134–141. [Google Scholar] [CrossRef] [PubMed]
- Honke, M.; Safarik, M.; Herzog, R. R718 turbo chillers and vacuum ice generation—Two applications of a new generation of high speed, high capacity R718 centrifugal compressors. In Proceedings of the 24th IIR International Congress of Refrigeration, Yokohama, Japan, 16–22 August 2015; pp. 16–22. [Google Scholar]
- Kauffeld, M. Heat transfer and pressure drop with ice slurry. In Proceedings of the Fourth IIR Gustav Lorentzen Conference on Natural Working Fluids, West Lafayette, IN, USA, 25–28 July 2000. [Google Scholar]
- Kauffeld, M.; Ernst, G. Influence of the wall surface roughness on the supercooling degree of water flowing inside a heat exchanger. In Proceedings of the 11th IIR Conference on Phase Change Materials and Slurries for Refrigeration and Air Conditioning, Karlsruhe, Germany, 18–20 May 2016. [Google Scholar]
- Langlois, V.; Gautherin, W.; Laurent, J.; Royon, L.; Fournaison, L.; Delahaye, A.; Jia, X. Ultrasonic determination of the particle concentration in model suspensions and ice slurry. Int. J. Refrig. 2011, 34, 1972–1979. [Google Scholar] [CrossRef]
- Norgaard, E.; Albrecht, S. Ice concentrators. In Proceedings of the Fourth IIR Ice slurry Workshop, Osaka, Japan, 12–13 November 2001. [Google Scholar]
- Nørgaard, E.; Sørensen, T.; Hansen, T.; Kauffeld, M. Performance of components of ice slurry systems: Pumps, plate heat exchangers, and fittings. Int. J. Refrig. 2005, 28, 83–91. [Google Scholar] [CrossRef]
- Liu, X.; Li, Y.; Zhuang, K.; Fu, R.; Lin, S.; Li, X. Performance study and efficiency improvement of ice slurry production by scraped-surface method. Appl. Sci. 2018, 9, 74. [Google Scholar] [CrossRef]
- Rayhan, F.A.; Pamitran, A.S. Performance of Ice Slurry Generator with Mechanical Scraper Using R-22 and R-290. Int. J. Technol. 2017, 8, 1191–1196. [Google Scholar] [CrossRef]
- Yamada, M.; Fukusako, S.; Kawabe, H. A quantitative evaluation of the production performance of ice slurry by the oscillatory moving cooled wall method. Int. J. Refrig. 2002, 25, 199–207. [Google Scholar] [CrossRef]
- Bédécarrats, J.-P.; David, T.; Castaing-Lasvignottes, J. Ice slurry production using supercooling phenomenon. Int. J. Refrig. 2010, 33, 196–204. [Google Scholar] [CrossRef]
- Brooks, S. Supercooling Brine in a Helical Coiled Heat Exchanger for Ice Slurry Production: Experiments Supercooling Brine in Heat Exchangers to Generate Ice Slurry Hygienically and Efficiently; University of Bristol: Bristol, UK, 2019. [Google Scholar]
- Shi, X.J.; Zhang, P. A comparative study of different methods for the generation of tetra-n-butyl ammonium bromide clathrate hydrate slurry in a cold storage air-conditioning system. Appl. Energy 2013, 112, 1393–1402. [Google Scholar] [CrossRef]
- Kauffeld, M.; Gund, S. Ice slurry–History, current technologies and future developments. Int. J. Refrig. 2019, 99, 264–271. [Google Scholar] [CrossRef]
- Liu, X.; Zhuang, K.Y.; Lin, S.; Zhang, Z.; Li, X.L. Determination of Supercooling Degree, Nucleation and Growth Rates, and Particle Size for Ice Slurry Crystallization in Vacuum. Crystals 2017, 7, 128. [Google Scholar] [CrossRef]
- Shin, H.T.; Lee, Y.P.; Jurng, J. Spherical-shaped ice particle production by spraying water in a vacuum chamber. Appl. Therm. Eng. 2000, 20, 439–454. [Google Scholar] [CrossRef]
- Hongfen, C.; Wenzhuang, Z.; Weisan, H.; Xuelai, Z. Vacuum ice-making technology and characteristic analysis. J. Mol. Liq. 2022, 360, 119360. [Google Scholar] [CrossRef]
- Meewisse, J.W. Fluidized Bed Ice Slurry Generator for Enhanced Secondary Cooling Systems; Technische Universiteit Delft: Delft, The Netherlands, 2002. [Google Scholar]
- Zhengbiao, P.; Zhulin, Y.; Liang, K.; Jie, C. Ice slurry formation in a cocurrent liquid-liquid flow. Chin. J. Chem. Eng. 2008, 16, 552–557. [Google Scholar]
- Thongwik, S.; Vorayos, N.; Kiatsiriroat, T.; Nuntaphan, A. Thermal analysis of slurry ice production system using direct contact heat transfer of carbon dioxide and water mixture. Int. Commun. Heat Mass Transf. 2008, 35, 756–761. [Google Scholar] [CrossRef]
- Zhang, Y.K.; Su, L.; Dong, K.J.; Liu, T.Q. Experimental study of ice slurry production system using direct contact heat transfer of RC318 and water in a horizontal pipe. Energy Procedia 2019, 158, 4495–4501. [Google Scholar] [CrossRef]
- Amer, M.; Wang, C. Review of defrosting methods. Renew. Sustain. Energy Rev. 2017, 73, 53–74. [Google Scholar] [CrossRef]
- Evans, T.S.; Quarini, G.L.; Shire, G.S.F. Investigation into the transportation and melting of thick ice slurries in pipes. Int. J. Refrig. 2008, 31, 145–151. [Google Scholar] [CrossRef]
- Melinder, Å. Thermophysical Properties of Aqueous Solutions Used as Secondary Working Fluids. Ph.D. Thesis, Kungliga Tekniska Högskolan, Stockholm, Sweden, 2007. [Google Scholar]
- Wang, J.H.; Wang, S.G.; Zhang, T.F.; Battaglia, F. Mathematical and experimental investigation on pressure drop of heterogeneous ice slurry flow in horizontal pipes. Int. J. Heat Mass Transf. 2017, 108, 2381–2392. [Google Scholar] [CrossRef]
- Hägg, C. Ice Slurry as Secondary Fluid in Refrigeration Systems: Fundamentals and Applications in Supermarkets. Ph.D. Thesis, Kungliga Tekniska Högskolan, Stockholm, Sweden, 2005. [Google Scholar]
- Rui, W. Experimental Study on Pigging Effect of Ice Slurry; China University of Petroleum: Beijing, China, 2018. (In Chinese) [Google Scholar]
- Ferguson, H.F.; Frurip, D.J.; Pastor, A.J.; Peerey, L.M.; Whiting, L.F. A review of analytical applications of calorimetry. Thermochim. Acta 2000, 363, 1–21. [Google Scholar] [CrossRef]
- Shire, S.; Quarini, I.; Ayala, R.S. Ultrasonic detection of slurry ice flows. Proc. Inst. Mech. Eng. Part E J. Process Mech. Eng. 2005, 219, 217–225. [Google Scholar] [CrossRef]
- Quarini, G.L. Pigging with pumpable ice-update. Food Link News 2003, 45, 10–11. [Google Scholar]
- Albion, K.J.; Briens, L.; Briens, C.; Berruti, F. Multiphase Flow Measurement Techniques for Slurry Transport. Int. J. Chem. React. Eng. 2011, 9, 1–50. [Google Scholar] [CrossRef]
- Dickin, F.; Wang, M. Electrical resistance tomography for process applications. Meas. Sci. Technol. 1996, 7, 247–260. [Google Scholar] [CrossRef]
- Fangary, Y.S.; Williams, R.A.; Neil, W.A.; Bond, J.; Faulks, I. Application of electrical resistance tomography to detect deposition in hydraulic conveying systems. Powder Technol. 1998, 95, 61–66. [Google Scholar] [CrossRef]
- Giguère, R.; Fradette, L.; Mignon, D.; Tanguy, P.A. Characterization of slurry flow regime transitions by ERT. Chem. Eng. Res. Des. 2008, 86, 989–996. [Google Scholar] [CrossRef]
- Lucas, G.; Cory, J.; Waterfall, R.; Loh, W.; Dickin, F. Measurement of the solids volume fraction and velocity distributions in solids–liquid flows using dual-plane electrical resistance tomography. Flow Meas. Instrum. 1999, 10, 249–258. [Google Scholar] [CrossRef]
- Norman, J.T.; Bonnecaze, R.T. Measurement of solids distribution in suspension flows using electrical resistance tomography. Can. J. Chem. Eng. 2005, 83, 24–36. [Google Scholar] [CrossRef]
- Wang, M.; Jones, T.F.; Williams, R.A. Visualization of asymmetric solids distribution in horizontal swirling flows using electrical resistance tomography. Chem. Eng. Res. Des. 2003, 81, 854–861. [Google Scholar] [CrossRef]
- Zhang, J.G.; Mab, F.C. Application of Electrical Resistance Tomography to Ice-water Two-phase Flow Parameters Measurement. Key Eng. Mater. 2013, 562–565, 686–690. [Google Scholar] [CrossRef]
- Aw, S.R.; Rahim, R.A.; Rahiman, M.H.F.; Yunus, F.R.M.; Goh, C.L. Electrical resistance tomography: A review of the application of conducting vessel walls. Powder Technol. 2014, 254, 256–264. [Google Scholar] [CrossRef]
- Edelhoff, D.; Walczak, L.; Henning, S.; Weichert, F.; Suter, D. High-resolution MRI velocimetry compared with numerical simulations. J. Magn. Reson. 2013, 235, 42–49. [Google Scholar] [CrossRef] [PubMed]
- Graham, L.J.W.; Pullum, L. An investigation of complex hybrid suspension flows by magnetic resonance imaging. Can. J. Chem. Eng. 2002, 80, 200–207. [Google Scholar] [CrossRef]
- Powell, R.L. Experimental techniques for multiphase flows. Phys. Fluids 2008, 20, 040605. [Google Scholar] [CrossRef]
- Pullum, L.; Graham, L.; Rudman, M.; Hamilton, R. High concentration suspension pumping. Miner. Eng. 2006, 19, 471–477. [Google Scholar] [CrossRef]
- Sederman, A.J.; Johns, M.L.; Bramley, A.S.; Alexander, P.; Gladden, L.F. Magnetic resonance imaging of liquid flow and pore structure within packed beds. Chem. Eng. Sci. 1997, 52, 2239–2250. [Google Scholar] [CrossRef]
- Ray, P.S. Broadband complex refractive indices of ice and water. Appl. Opt. 1972, 11, 1836–1844. [Google Scholar] [CrossRef]
- Hales, A.; Quarini, G.; Hilton, G.; Ash, D.; Lucas, E.; McBryde, D.; Yun, X. Ice fraction measurement of ice slurries through electromagnetic attenuation. Int. J. Refrig. 2014, 47, 98–104. [Google Scholar] [CrossRef]
- Hales, A.; Quarini, G.; Hilton, G.; Jones, L.; Lucas, E.; McBryde, D.; Yun, X. The effect of salinity and temperature on electromagnetic wave attenuation in brine. Int. J. Refrig. 2015, 51, 161–168. [Google Scholar] [CrossRef]
- Tian, Q.Q.; He, G.G.; Wang, H.; Cai, D.H. Simulation on transportation safety of ice slurry in ice cooling system of buildings. Energy Build. 2014, 72, 262–270. [Google Scholar] [CrossRef]
- Bel, O. Contribution à l’étude du Comportement Thermohydraulique d’un Mélange Diphasique: Lecoulis de Glace. Etude Théorique et Expérimentale. Ph.D. Thesis, INSA Lyon, Villeurbanne, France, 1996. [Google Scholar]
- Lakhdar, M.A.B.; Lallemand, A. Comportement Thermohydraulique d’un Fluide Frigoporteur Diphasique: Le Coulis de Glace. Etude Théorique et Expérimentale. Ph.D. Thesis, INSA Lyon, Villeurbanne, France, 1998. [Google Scholar]
- Christensen, K.G.; Kauffeld, M. Heat transfer measurements with ice slurry. In Proceedings of the Heat Transfer Issues in Natural Refrigerants, IIR/IIF, College Park, MD, USA, 31 December 1997; pp. 127–141. [Google Scholar]
- Doetsch, C. Pressure drop and flow pattern of ice slurries. In Proceedings of the 3rd Workshop on Ice Slurries of the IIR, Lucerne, Switzerland, 1 January 2001; pp. 53–54. [Google Scholar]
- Jensen, E.N.; Christensen, K.G.; Hansen, T.M.; Schneider, P.; Kauffeld, M. Pressure drop and heat transfer with ice slurry. In Proceedings of the Conférence IIR-Gustav Lorentzen sur les fluides actifs naturels à Purdue, West Lafayette, IN, USA, 25 July 2000; pp. 572–580. [Google Scholar]
- Royon, L. Qu’est-ce que le coulis de glace stabilisée: Fluides et lubrifiants. Rev. Générale Du Froid 1998, 88, 57–60. [Google Scholar]
- Ayel, V.; Lottin, O.; Peerhossaini, H. Rheology, flow behaviour and heat transfer of ice slurries: A review of the state of the art. Int. J. Refrig. 2003, 26, 95–107. [Google Scholar] [CrossRef]
- Einstein, A. A new determination of molecular dimensions. Annln. Phys. 1906, 19, 289–306. [Google Scholar] [CrossRef]
- Thomas, D.G. Transport characteristics of suspension: VIII. A note on the viscosity of Newtonian suspensions of uniform spherical particles. J. Colloid Sci. 1965, 20, 267–277. [Google Scholar] [CrossRef]
- Hansen, T.M.; Kauffeld, M.; Grosser, K.; Zimmermann, R. Viscosity of ice slurry. In Proceedings of the Second Workshop on Ice Slurries of the International Institute of Refrigeration, Paris, France, 25–26 May 2000; p. 8. [Google Scholar]
- Ismail, K.A.R.; Radwan, M.M. Modeling of ice crystal growth in laminar falling films for the production of pumpable ice slurries. Energy Convers. Manag. 2003, 44, 65–84. [Google Scholar] [CrossRef]
- Chhabra, R.P.; Richardson, J.F. Non-Newtonian Flow and Applied Rheology: Engineering Applications; Butterworth-Heinemann: Oxford, UK, 2011. [Google Scholar]
- Kumano, H.; Hirata, T.; Shirakawa, M.; Shouji, R.; Hagiwara, Y. Flow characteristics of ice slurry in narrow tubes. Int. J. Refrig. 2010, 33, 1513–1522. [Google Scholar] [CrossRef]
- Kumano, H.; Yamanada, Y.; Makino, Y.; Asaoka, T. Effect of initial aqueous solution concentration on rheological behavior of ice slurry. Int. J. Refrig. 2016, 68, 218–225. [Google Scholar] [CrossRef]
- Niezgoda-Żelasko, B.; Zalewski, W. Momentum transfer of ice slurry flows in tubes, experimental investigations. Int. J. Refrig. 2006, 29, 418–428. [Google Scholar] [CrossRef]
- Niezgoda-Żelasko, B.; Żelasko, J. Generalized non-Newtonian flow of ice-slurry. Chem. Eng. Process. Process Intensif. 2007, 46, 895–904. [Google Scholar] [CrossRef]
- Illán, F.; Viedma, A. Experimental study on pressure drop and heat transfer in pipelines for brine based ice slurry. Part I: Operational parameters correlations. Int. J. Refrig. 2009, 32, 1015–1023. [Google Scholar] [CrossRef]
- Illán, F.; Viedma, A. Experimental study on pressure drop and heat transfer in pipelines for brine based ice slurry Part II: Dimensional analysis and rheological model. Int. J. Refrig. 2009, 32, 1024–1031. [Google Scholar] [CrossRef]
- Doetsch, C. Pressure drop calculation of ice slurries using the Casson model. In Proceedings of the 5th Workshop on Ice Slurries, IIF/IIR, Stockholm, Sweden, 30–31 May 2002; pp. 15–21. [Google Scholar]
- Monteiro, A.C.S.; Bansal, P.K. Pressure drop characteristics and rheological modeling of ice slurry flow in pipes. Int. J. Refrig. 2010, 33, 1523–1532. [Google Scholar] [CrossRef]
- Brown, G.O. The history of the Darcy-Weisbach equation for pipe flow resistance. In Environmental and Water Resources History; ASCE: Reston, VA, USA, 2002; pp. 34–43. [Google Scholar]
- Grozdek, M.; Khodabandeh, R.; Lundqvist, P. Experimental investigation of ice slurry flow pressure drop in horizontal tubes. Exp. Therm. Fluid Sci. 2009, 33, 357–370. [Google Scholar] [CrossRef]
- Kumano, H.; Mizui, A.; Higashi, N. Flow characteristics of ice slurry in a horizontal tube during solidification. Int. J. Refrig. 2018, 85, 184–190. [Google Scholar] [CrossRef]
- Dodge, D.; Metzner, A. Turbulent flow of non—Newtonian systems. AIChE J. 1959, 5, 189–204. [Google Scholar] [CrossRef]
- Hanks, R.W.; Ricks, B.L. Transitional and turbulent pipeflow of pseudoplastic fluids. J. Hydronautics 1975, 9, 39–44. [Google Scholar] [CrossRef]
- Shire, G.S.F.; Quarini, G.L.; Rhys, T.D.L.; Evans, T.S. The anomalous pressure drop behaviour of ice slurries flowing through constrictions. Int. J. Multiph. Flow 2008, 34, 510–515. [Google Scholar] [CrossRef][Green Version]
- Shire, G.S.F.; Quarini, G.L.; Evans, T.S. Pressure drop of flowing ice slurries in industrial heat exchangers. Appl. Therm. Eng. 2009, 29, 1500–1506. [Google Scholar] [CrossRef][Green Version]
- Bellas, J.; Chaer, I.; Tassou, S.A. Heat transfer and pressure drop of ice slurries in plate heat exchangers. Appl. Therm. Eng. 2002, 22, 721–732. [Google Scholar] [CrossRef]
- Gupta, R.P. Effect of a New Friction-Reducing Additive on Sunwell Ice Slurry Characteristics; National Research Council of Canada: Ottawa, ON, Canada, 1990. [Google Scholar]
- Kalaiselvam, S.; Karthik, P.; Prakash, S.R. Numerical investigation of heat transfer and pressure drop characteristics of tube–fin heat exchangers in ice slurry HVAC system. Appl. Therm. Eng. 2009, 29, 1831–1839. [Google Scholar] [CrossRef]
- Fernández-Seara, J.; Diz, R.; Uhía, F.J.; Dopazo, J.A. Experimental analysis on pressure drop and heat transfer of a terminal fan-coil unit with ice slurry as cooling medium. Int. J. Refrig. 2010, 33, 1095–1104. [Google Scholar] [CrossRef]
- Wang, J.H.; Zhang, T.F.; Wang, S.G. Heterogeneous ice slurry flow and concentration distribution in horizontal pipes. Int. J. Heat Fluid Flow 2013, 44, 425–434. [Google Scholar] [CrossRef]
- Singh, R.; Kachhwaha, S.S. Heat Transfer and Pressure Drop Analysis of Chilled Water and Ice Slurry in a Plate Heat Exchanger. J. Therm. Sci. Eng. Appl. 2016, 8, 011020. [Google Scholar] [CrossRef]
- Mi, S.; Cai, L.L.; Ma, K.B.; Liu, Z.Q. Investigation on flow and heat transfer characteristics of ice slurry without additives in a plate heat exchanger. Int. J. Heat Mass Transf. 2018, 127, 11–20. [Google Scholar] [CrossRef]
- Farajollahi, B.; Etemad, S.G.; Hojjat, M. Heat transfer of nanofluids in a shell and tube heat exchanger. Int. J. Heat Mass Transf. 2010, 53, 12–17. [Google Scholar] [CrossRef]
- Wang, J.H.; Battaglia, F.; Wang, S.G.; Zhang, T.F.; Ma, Z.J. Flow and heat transfer characteristics of ice slurry in typical components of cooling systems: A review. Int. J. Heat Mass Transf. 2019, 141, 922–939. [Google Scholar] [CrossRef]
- Vicente, P.G.; García, A.; Viedma, A. Experimental investigation on heat transfer and frictional characteristics of spirally corrugated tubes in turbulent flow at different Prandtl numbers. Int. J. Heat Mass Transf. 2004, 47, 671–681. [Google Scholar] [CrossRef]
- Meewisse, J.; Infante-Ferreira, C. Optimal properties of ice slurries in secondary cooling systems. In Proceedings of the 4 th–IIR-Gustaw Lorentzen Conference on Natural Working Fluids, Purdue University, West Lafayette, IN, USA; 2000; pp. 513–520. [Google Scholar]
- Harada, E.; Toda, M.; Kuriyama, M.; Konno, H. Heat-Transfer between Wall and Solid-Water Suspension Flow in Horizontal Pipes. J. Chem. Eng. Jpn. 1985, 18, 33–38. [Google Scholar] [CrossRef]
- Kawanami, T.; Yamada, M.; Fukusako, S. Melting characteristics of fine particle ice slurry at the return bend of flow path. In Proceedings of the Third Workshop on Ice Slurries of the International Institute of Refrigeration, Lucerne, Switzerland; 2001; pp. 16–18. [Google Scholar]
- Knodel, B.D.; France, D.M.; Choi, U.S.; Wambsganss, M.W. Heat transfer and pressure drop in ice-water slurries. Appl. Therm. Eng. 2000, 20, 671–685. [Google Scholar] [CrossRef]
- Stamatiou, E.; Kawaji, M.; Lee, B.; Goldstein, V. Experimental investigation of ice-slurry flow and heat transfer in a plate-type heat exchanger. In Proceedings of the 3rd IIR Workshop on Ice Slurries, Lucerne, Switzerland, 16–18 May 2001; pp. 16–18. [Google Scholar]
- Zhao, J.; Liu, Y.; Zhang, E.; Chen, P.J.S.e.t.d.f. Experiment study on cooling coil performance with ice slurry as working fluid. In Proceedings of the International Symposium on Air Conditioning in High Rise Buildings, Shanghai, China, 9–12 September 1997; pp. 495–499. [Google Scholar]
- Mokrani, A.; Castelain, C.; Peerhossaini, H. The effects of chaotic advection on heat transfer. Int. J. Heat Mass Transf. 1997, 40, 3089–3104. [Google Scholar] [CrossRef]
- Egolf, P.W.; Kitanovski, A.; Ata-Caesar, D.; Stamatiou, E.; Kawaji, M.; Bedecarrats, J.P.; Strub, F. Thermodynamics and heat transfer of ice slurries. Int. J. Refrig. 2005, 28, 51–59. [Google Scholar] [CrossRef]
- Bédécarrats, J.-P.; Strub, F.; Peuvrel, C. Thermal and hydrodynamic considerations of ice slurry in heat exchangers. Int. J. Refrig. 2009, 32, 1791–1800. [Google Scholar] [CrossRef]
- Haruki, N.; Horibe, A. Flow and heat transfer characteristics of ice slurries in a helically-coiled pipe. Int. J. Refrig. 2013, 36, 1285–1293. [Google Scholar] [CrossRef]
- Fernández-Seara, J.; Diz, R. Thermo-hydraulic behavior of ice slurry in an offset strip-fin plate heat exchanger. Int. J. Refrig. 2014, 41, 171–180. [Google Scholar] [CrossRef]
- Leiper, A.N.; Ash, D.G.; McBryde, D.J.; Quarini, G.L. Improving the thermal efficiency of ice slurry production through comminution. Int. J. Refrig. 2012, 35, 1931–1939. [Google Scholar] [CrossRef]
- Leiper, A.N.; Hammond, E.C.; Ash, D.G.; McBryde, D.J.; Quarini, G.L. Energy conservation in ice slurry applications. Appl. Therm. Eng. 2013, 51, 1255–1262. [Google Scholar] [CrossRef]
- Quarini, G.; Aislie, E.; Ash, D.; Leiper, A.; McBryde, D.; Herbert, M.; Deans, T. Transient thermal performance of ice slurries pumped through pipes. Appl. Therm. Eng. 2013, 50, 743–748. [Google Scholar] [CrossRef]
- Brooks, S.; Tierney, M.; Quarini, G. Experimental investigation of different materials for use in ice slurry generation. Int. J. Refrig. 2021, 129, 97–108. [Google Scholar] [CrossRef]
- Yang, S.W.; Liang, K.F.; Wang, L.; Liu, J.; Li, Y.C.; Chen, C.S.; Yuan, Y. Experimental study on effects of particle melting and micro-convection on measurement of thermal conductivity of ice slurry. Int. Commun. Heat Mass Transf. 2022, 136, 106178. [Google Scholar] [CrossRef]
- Gao, Y.G.; Ning, Y.L.; Xu, M.H.; Wu, C.Z.; Mujumdar, A.S.; Sasmito, A.P. Numerical investigation of aqueous graphene nanofluid ice slurry passing through a horizontal circular pipe: Heat transfer and fluid flow characteristics. Int. Commun. Heat Mass Transf. 2022, 134, 106022. [Google Scholar] [CrossRef]
- Cai, L.; Mi, S.; Luo, C.; Liu, Z. Numerical investigation of hydraulic and heat transfer characteristics of two-phase ice slurry in helically coiled tubes. Energy Build. 2022, 256, 111773. [Google Scholar] [CrossRef]
- Cai, L.; Mi, S.; Luo, C.; Liu, Z. Numerical investigation on heat and mass transfer characteristics of ice slurry in pulsating flow. Int. J. Heat Mass Transf. 2022, 189, 122722. [Google Scholar] [CrossRef]
- Suzuki, K.; Kuroiwa, T.; Asaoka, T.; Yoshino, M. Particle-resolved simulations of ice slurry flows in a square duct by the thermal immersed boundary–lattice Boltzmann method. Comput. Fluids 2021, 228, 105064. [Google Scholar] [CrossRef]
- Kishore, A.; Aravind, S.M.; Kumar, P.; Singh, A.; Kumari, K.; Kumar, N. Innovative Packaging Strategies for Freshness and Safety of Food Products: A Review. Packag. Technol. Sci. 2024, 37, 399–427. [Google Scholar] [CrossRef]
- Ssubi, J.A.; Mukisa, I.M.; Muyanja, C.K. Knowledge, attitudes and practices of fresh Nile perch value chain handlers towards food safety requirements in Uganda. Heliyon 2024, 10, e31432. [Google Scholar] [CrossRef]
- Lydon, K.A.; Farrell-Evans, M.; Jones, J.L. Evaluation of Ice Slurries as a Control for Postharvest Growth of Vibrio spp. in Oysters and Potential for Filth Contamination. J. Food Prot. 2015, 78, 1375–1379. [Google Scholar] [CrossRef]
- Rey, M.S.; Miranda, J.M.; Aubourg, S.; Barros-Velázquez, J. Improved microbial and sensory quality of clams (Venerupis rhomboideus), oysters (Ostrea edulis) and mussels (Mytilus galloprovincialis) by refrigeration in a slurry ice packaging system. Int. J. Food Sci. Technol. 2012, 47, 861–869. [Google Scholar] [CrossRef]
- Rodríguez, Ó.; Barros-Velázquez, J.; Piñeiro, C.; Gallardo, J.M.; Aubourg, S.P. Effects of storage in slurry ice on the microbial, chemical and sensory quality and on the shelf life of farmed turbot (Psetta maxima). Food Chem. 2006, 95, 270–278. [Google Scholar] [CrossRef]
- Wang, M.; Hansen, T.; Kauffeld, M.; Christensen, K.; Goldstein, V. Application of ice slurry technology in fishery. In Proceedings of the 20th International Congress of Refrigeration, IIF/IIR, Sydney, Australia, 19–24 September 1999; pp. 569–573. [Google Scholar]
- Paul, J. Innovative Applications of Pumpable Ice Slurry; Institute of Refrigeration: London, UK, 2002. [Google Scholar]
- Annamalai, J.; Lakshmi, N.M.; Sivam, V.; Rao, R.C.N. A Comparative Study on the Quality Changes of Croaker (Johnius dussumieri) Fish Stored in Slurry Ice and Flake Ice. J. Aquat. Food Prod. Technol. 2018, 27, 508–517. [Google Scholar] [CrossRef]
- Piñeiro, C.; Barros-Velázquez, J.; Aubourg, S.P. Effects of newer slurry ice systems on the quality of aquatic food products: A comparative review versus flake-ice chilling methods. Trends Food Sci. Technol. 2004, 15, 575–582. [Google Scholar] [CrossRef]
- Wang, M.J.; Goldstein, V. Ice slurry: Advanced fish chilling and preservation technology. In Proceedings of the American Fisheries Society Symposium, Quebec, QC, Canada, 11–12 August 2003; pp. 379–386. [Google Scholar]
- Gladis, S. Ice slurry thermal energy storage for cheese process cooling. ASHRAE Trans. 1997, 103, 725–729. [Google Scholar]
- Hansen, T.M.; Kauffeld, M.; Sari, O.; Egolf, P.W.; Pasche, F. Research, development and applications of ice slurry in Europe. In Proceedings of the Fourth Workshop on Ice Slurries of the International Institute of Refrigeration, Osaka, Japan, 1 January 2001; pp. 1–12. [Google Scholar]
- Tassou, S.; Chaer, I.; Bellas, J.; Terzis, G. Comparison of the performance of ice slurry and traditional primary and secondary refrigerants in refrigerated food display cabinet cooling coil. In Proceedings of the Fourth Workshop on Ice Slurries, Osaka, Japan, 12–13 November 2001; pp. 87–95. [Google Scholar]
- Lueders, D. Application of ice slurry/TES technology to supermarkets using ammonia refrigeration. In Proceedings of the 21st Annual Meeting of the International Meeting of Ammonia Refrigeration, Dallas, TX, USA, 21–24 March 1999; pp. 301–326. [Google Scholar]
- Davies, T.W.; Lowes, A.L. A high efficiency refrigeration system. In Proceedings of the IIR conference New Technologies in Commercial Refrigeration, Paris, France, Urbana, IL, USA, 22–23 July 2002; pp. 93–100. [Google Scholar]
- Kauffeld, M.; Wang, M.J.; Goldstein, V.; Kasza, K.E. Ice Slurry Applications. Int. J. Refrig. 2010, 33, 1491–1505. [Google Scholar] [CrossRef] [PubMed]
- Wang, M.J.; Kusumoto, N. Ice slurry based thermal storage in multifunctional buildings. Heat Mass Transf. 2001, 37, 597–604. [Google Scholar] [CrossRef]
- Rivet, P. Overview of PCM applications around the worls—Regional overview Europe. In Proceedings of the 8 th IIR Conference on Phase Change Materials and Slurries for Refrigeration and Air Conditioning, Karlsruhe, Germany, 3–5 June 2009; pp. 3–5. [Google Scholar]
- Yoo, H.; Lee, S.H.; Lee, Y.P. A Field Application Case of the Direct Ice Slurry Transporting System for District Cooling. Int. J. Air Cond. Refrig. 2012, 18, 43–53. [Google Scholar] [CrossRef]
- Chung, J.D.; Lee, Y.P.; Yoo, H.; Lee, J.H.; Lee, C.J.; Moon, S.J. Feasibility Study for Ice-Slurry to District Cooling System in Korea. In Proceedings of the Volume 1: Heat Transfer in Energy Systems; Thermophysical Properties; Theory and Fundamental Research in Heat Transfer, Minneapolis, MN, USA, 14–19 July 2013; p. 001. [Google Scholar]
- Compingt, A.; Blanc, P.; Quidort, A. Slurry for refrigeration industrial kitchen application. In Proceedings of the 8th IIR Conference on Phase Change Material and Slurries for Refrigeration and Air Conditioning, Karlsruhe, Germany, 3–5 June 2009. [Google Scholar]
- Hager, M.; Kaemper, T. The hydraulic transport of ice for mine-cooling. Powder Handl. Process. 1991, 3, 317–325. [Google Scholar]
- Sheer, T.; Correia, R.; Chaplain, E.; Hemp, R. Research into the use of ice for cooling deep mines. In Proceedings of the Third International Mine Ventilation Congress, Harrogate, UK, 13–19 June 1984; pp. 277–282. [Google Scholar]
- Sheer, T.J. Pneumatic Conveying of Ice Particles through Mine-Shaft Pipelines. Powder Technol. 1995, 85, 203–219. [Google Scholar] [CrossRef]
- Ophir, A.; Koren, A. Vacuum freezing vapor compression process (VFVC) for mine cooling. In Proceedings of the 20th International Congress of Refrigeration, IIF/IIR, Sydey, Australia, 19–24 September 1999; p. 508. [Google Scholar]
- Chen, W.; Liang, S.; Liu, J. Proposed split-type vapor compression refrigerator for heat hazard control in deep mines. Appl. Therm. Eng. 2016, 105, 425–435. [Google Scholar] [CrossRef]
- Kasza, K.; Chang, F.; Oras, J.; Fisher, B.; Shalhav, A.; Oriveto, M.; Mills, R. Modelling of kidney cooling using ice slurry during laparoscopic surgery. In Proceedings of the Bioengineering Conference, Amelia Island, FL, USA, 21–25 June 2006. [Google Scholar]
- Laven, B.A.; Kasza, K.E.; Rapp, D.E.; Orvieto, M.A.; Lyon, M.B.; Oras, J.J.; Beiser, D.G.; Vanden Hoek, T.L.; Son, H.; Shalhav, A.L. A pilot study of ice-slurry application for inducing laparoscopic renal hypothermia. BJU Int. 2007, 99, 166–170. [Google Scholar] [CrossRef]
- Colli, J.L.; Dorsey, P.; Grossman, L.; Lee, B.R. Retrograde renal cooling to minimize ischemia. Int. Braz. J. Urol. 2013, 39, 37–45. [Google Scholar] [CrossRef][Green Version]
- Summers, E.; Cervantes, T.; Batzer, R.; Simpson, C.; Lewis, R.; Stark, J. Renal Cooling Device for Use in Minimally Invasive Surgery. J. Med. Devices 2012, 6, 021007. [Google Scholar] [CrossRef]
- Tyritzis, S.I.; Kyroudi, A.; Liatsikos, E.; Manousakas, T.; Karayannacos, P.; Kostomitsopoulos, N.; Zervas, A.; Pavlakis, K.; Stolzenburg, J.U.; Constantinides, C. Comparison of prolonged warm and cold ischemia on the solitary kidney during partial nephrectomy in a rabbit model. World J. Urol. 2007, 25, 635–640. [Google Scholar] [CrossRef] [PubMed]
- Otake, H.; Shite, J.; Paredes, O.L.; Shinke, T.; Yoshikawa, R.; Tanino, Y.; Watanabe, S.; Ozawa, T.; Matsumoto, D.; Ogasawara, D.; et al. Catheter-based transcoronary myocardial hypothermia attenuates arrhythmia and myocardial necrosis in pigs with acute myocardial infarction. J. Am. Coll. Cardiol. 2007, 49, 250–260. [Google Scholar] [CrossRef]
- Quarini, G.; Ainslie, E.; Herbert, M.; Deans, T.; Ash, D.; Rhys, D.; Haskins, N.; Norton, G.; Andrews, S.; Smith, M. Investigation and development of an innovative pigging technique for the water-supply industry. Proc. Inst. Mech. Eng. Part E J. Process Mech. Eng. 2010, 224, 79–89. [Google Scholar] [CrossRef]
- Vreeburg, J.H.; Boxall, J.B. Discolouration in potable water distribution systems: A review. Water Res. 2007, 41, 519–529. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, L.; Lv, J.; Zhang, Y.; Ye, B. Public Awareness of Drinking Water Safety and Contamination Accidents: A Case Study in Hainan Province, China. Water 2018, 10, 446. [Google Scholar] [CrossRef]
- Meroufel, A.A. Corrosion Control during Acid Cleaning of Heat Exchangers. In Corrosion and Fouling Control in Desalination Industry; Saji, V.S., Meroufel, A.A., Sorour, A.A., Eds.; Springer International Publishing: Cham, Switzerland, 2020; pp. 209–224. [Google Scholar]
- Tait, W.S. Corrosion prevention and control of chemical processing equipment. In Handbook of Environmental Degradation of Materials; Kutz, M., Ed.; William Andrew Publishing: Norwich, NY, USA, 2005; pp. 565–581. [Google Scholar]
- Burfoot, D.; Middleton, K. Effects of operating conditions of high pressure washing on the removal of biofilms from stainless steel surfaces. J. Food Eng. 2009, 90, 350–357. [Google Scholar] [CrossRef]
- Mucchetti, G.; Bonvini, B.; Francolino, S.; Neviani, E.; Carminati, D. Effect of washing with a high pressure water spray on removal of Listeria innocua from Gorgonzola cheese rind. Food Control. 2008, 19, 521–525. [Google Scholar] [CrossRef]
- Quarini, J.; Shire, S. A review of fluid-driven pipeline pigs and their applications. Proc. Inst. Mech. Eng. Part E J. Process Mech. Eng. 2007, 221, 1–10. [Google Scholar] [CrossRef]
- Yang, K.; Wu, C.; Yuan, Y.; Yu, J. Application of Virtual Instrument Technique in Data Acquisition of Gas-Water Pulse Pipe Cleaning Experiment. In Proceedings of the 2nd International Congress on Computer Applications and Computational Science, Bali, Indonesia, 15–17 November 2011; Gaol, F.L., Nguyen, Q.V., Eds.; Springer: Berlin/Heidelberg, Germany, 2012; pp. 465–470. [Google Scholar]
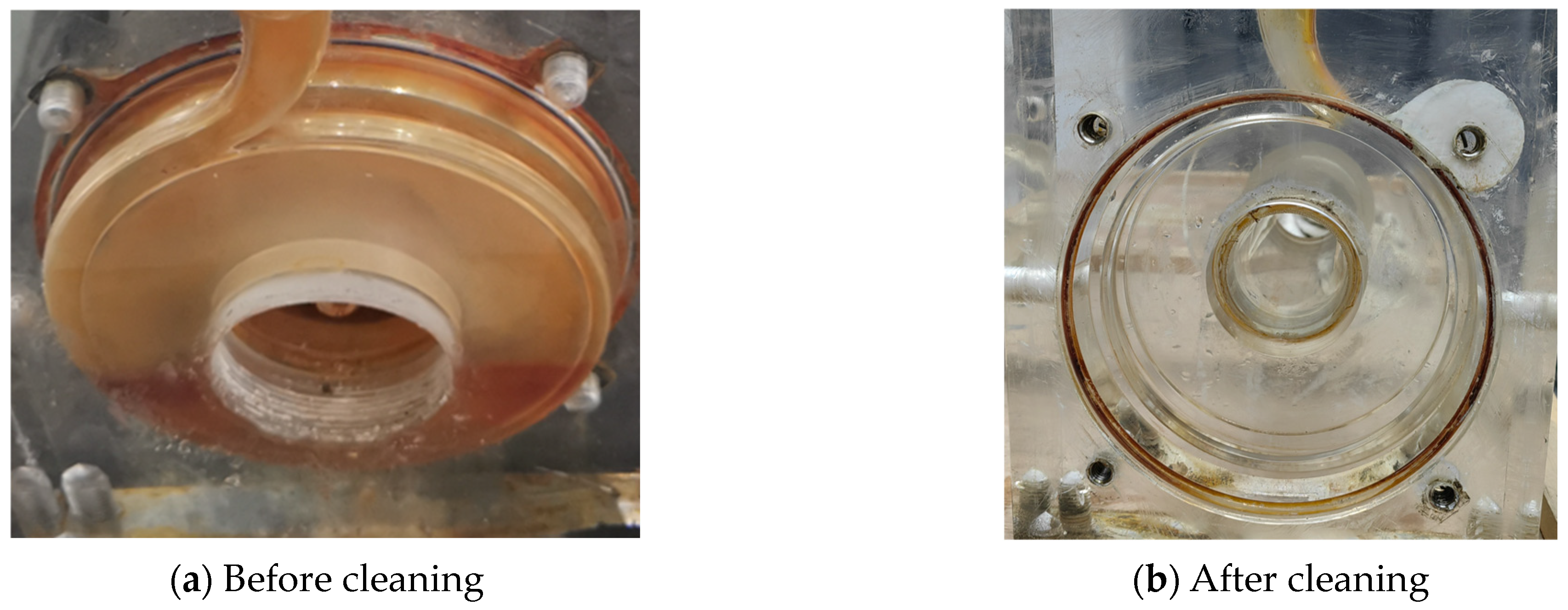
- Quarini, J. Ice-pigging to reduce and remove fouling and to achieve clean-in-place. Appl. Therm. Eng. 2002, 22, 747–753. [Google Scholar] [CrossRef]
- Hales, A.J.; Quarini, G.; Ash, D. The use of ice pigging technology to clean shell and tube exchangers. In Proceedings of the 13th UK Heat Transfer Conference (UKHTC2013), London, UK, 2–3 September 2013. [Google Scholar]
- Shire, G.S.F. The Behaviour of Ice Pigging Slurries; University of Bristol: Bristol, UK, 2006. [Google Scholar]
- Davies, T.W. Slurry ice as a heat transfer fluid with a large number of application domains. Int. J. Refrig. 2005, 28, 108–114. [Google Scholar] [CrossRef]
- Morris, N.B.; Coombs, G.; Jay, O. Ice Slurry Ingestion Leads to a Lower Net Heat Loss during Exercise in the Heat. Med. Sci. Sports Exerc. 2016, 48, 114–122. [Google Scholar] [CrossRef] [PubMed]
- Stevens, C.J.; Dascombe, B.; Boyko, A.; Sculley, D.; Callister, R. Ice slurry ingestion during cycling improves Olympic distance triathlon performance in the heat. J. Sports Sci. 2013, 31, 1271–1279. [Google Scholar] [CrossRef] [PubMed]



| Types | Structural Formula | Advantages | Drawbacks |
|---|---|---|---|
| Sodium chloride | NaCl | Innoxious, common | Corroding metal equipment, large discharges causing the salinization of soil and water |
| Ethanol | C2H5OH | Innoxious, common, biodegradable | Volatile, large emissions affecting water quality |
| Ethylene glycol | C2H6O2 | Biodegradable, cheap, high heat capacity | Toxic, large spills contaminating water bodies and aquatic life, a certain corrosiveness |
| Propylene glycol | C3H8O2 | Innoxious, common, biodegradable, cheap, high heat capacity | Viscosity of the solution increasing rapidly at low temperature, a certain corrosiveness |
| Production Methods | Advantages | Drawbacks |
|---|---|---|
| Surface-scraping | Stable performance, fine ice crystals | Low efficiency, high energy consumption, scraper-prone to wear |
| Supercooling | Good heat transfer efficiency, technical maturity, mass production | Difficult production process, easy ice blockage |
| Vacuum | Good heat transfer, environmental | Difficult production process, complex system |
| Fluidized bed | Fine ice crystals, good heat exchange, simple tube shells | Large refrigerant consumption, hard control of process |
| Direct contact | Thermal resistance reduction, small equipment size | Large refrigerant consumption, easy ice blockage, hard separation |
| Rheological Model | Friction Factor Formula | Fluid Type | Model Application Description | ||
|---|---|---|---|---|---|
| Power law model | Laminar | non-Newtonian | Used widely; suitable for low ice fraction; accurately predict the flow resistance coefficient of mixed slurry at high flow velocity [74,76,77]. | ||
| Turbulent | |||||
| Bingham model | Laminar | non-Newtonian | Large error between predicted and experimental values of the flow resistance coefficient at low flow velocity; not suitable for low concentration slurry [64,67,68]. | ||
| Turbulent | |||||
| Herschel-Bulkley model | Laminar | non-Newtonian | Used widely; the model needs to be modified when slurry is in different shear rate regions; accurate due to the consideration of ice crystal particle size [69,70]. | ||
| Turbulent | |||||
| Casson model | Laminar | non-Newtonian | Used widely; prediction accuracy between the Power law model and the Bingham model [71]. | ||
| Turbulent | |||||
| Heat Transfer Coefficient | Fluid Type | Application Equipment | Description |
|---|---|---|---|
| non-Newtonian | Tubular heat exchangers | Uo is the overall heat transfer coefficient, hs and hl are the heat transfer coefficients between the pipe wall and the ice slurry and between the laboratory (ambient) air and the pipe wall, respectively [87]. | |
| non-Newtonian | Plate heat exchangers | ew is the wall thickness of the plate heat exchanger [88]. | |
| non-Newtonian | Smooth tubes | Pr and α are the Prandtl number and severity index of the corrugated pipe [89]. | |
| non-Newtonian | Corrugated tubes | ||
| non-Newtonian | Smooth pipes (modified) | Reref and Prref were calculated using reference fluid properties (corresponding to the carrier fluid at 0 °C) [69,70]. | |
| non-Newtonian | Corrugated pipes (modified) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, H.; Verdin, P.G.; Zhang, J. Research Developments and Applications of Ice Slurry. Energies 2024, 17, 5213. https://doi.org/10.3390/en17205213
Song H, Verdin PG, Zhang J. Research Developments and Applications of Ice Slurry. Energies. 2024; 17(20):5213. https://doi.org/10.3390/en17205213
Chicago/Turabian StyleSong, Haiqin, Patrick G. Verdin, and Jinfeng Zhang. 2024. "Research Developments and Applications of Ice Slurry" Energies 17, no. 20: 5213. https://doi.org/10.3390/en17205213
APA StyleSong, H., Verdin, P. G., & Zhang, J. (2024). Research Developments and Applications of Ice Slurry. Energies, 17(20), 5213. https://doi.org/10.3390/en17205213