Ejectors in Hydrogen Recirculation for PEMFC-Based Systems: A Comprehensive Review of Design, Operation, and Numerical Simulations
Abstract
1. Introduction
2. Search Strategy, Eligibility Criteria, and Study Selection
3. Results
3.1. Ejector
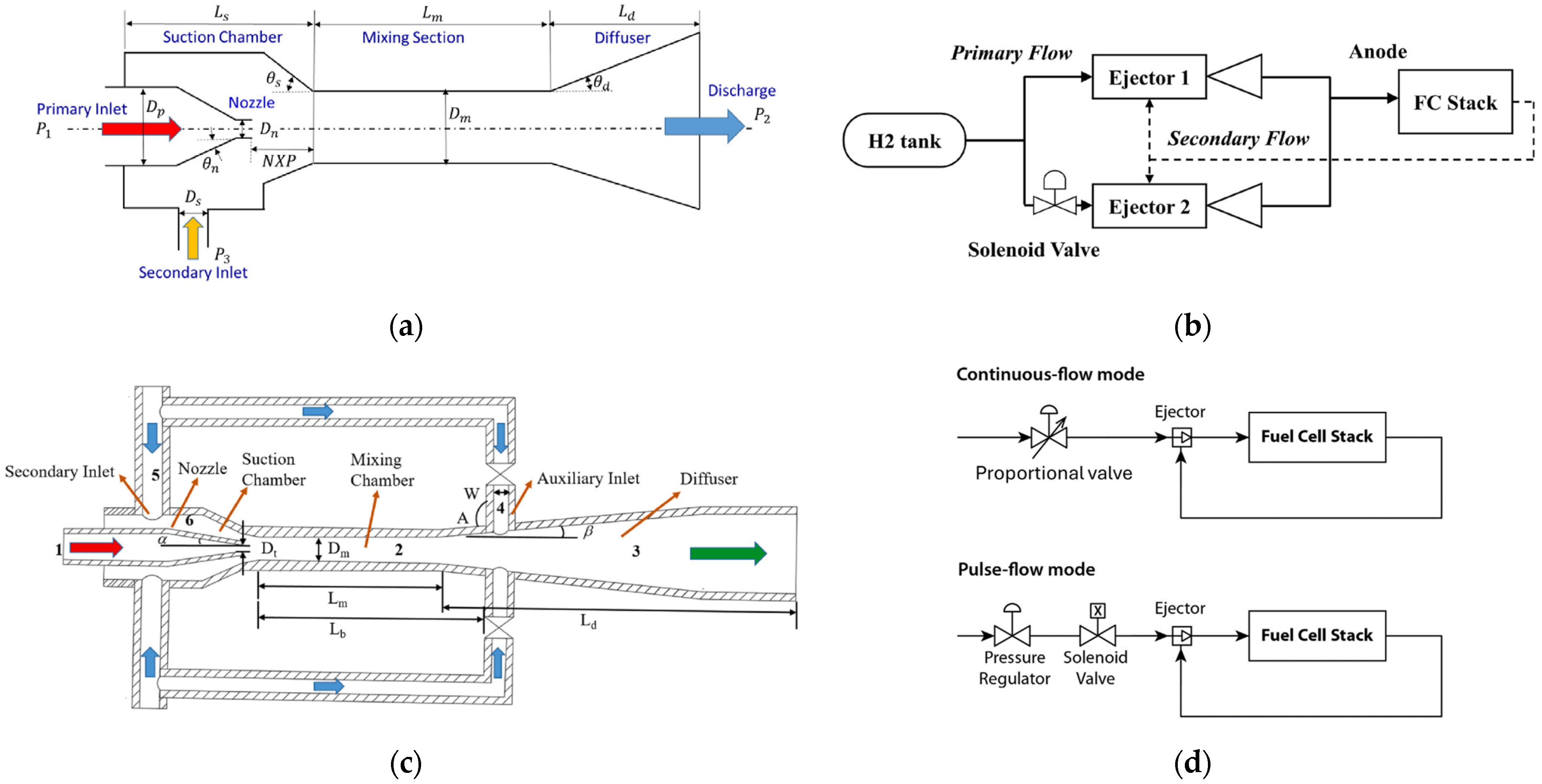
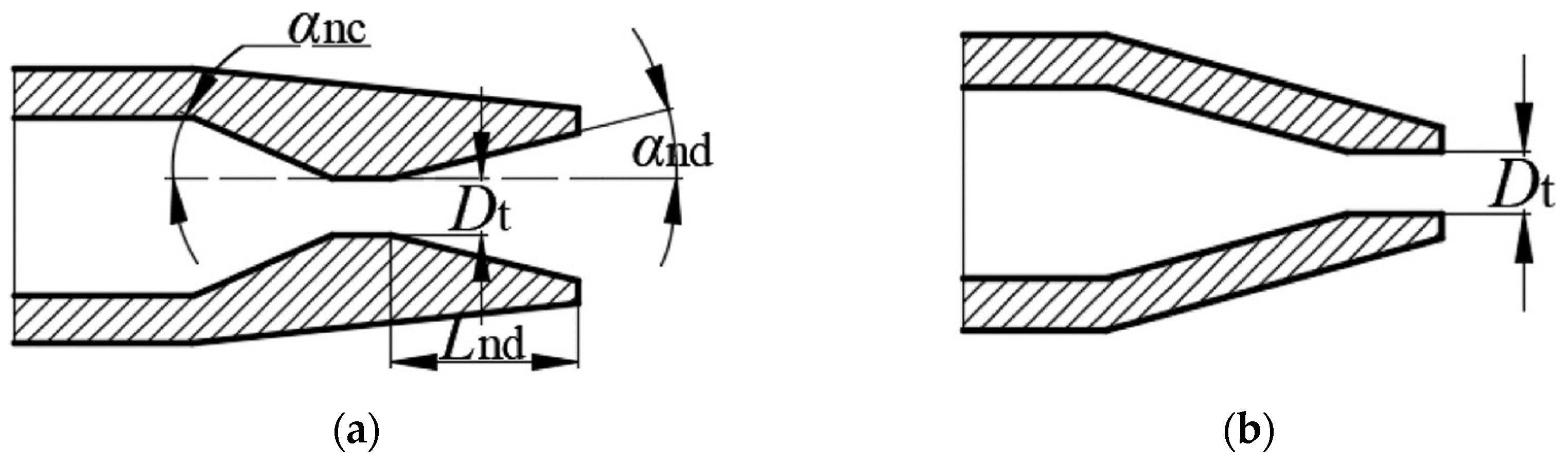
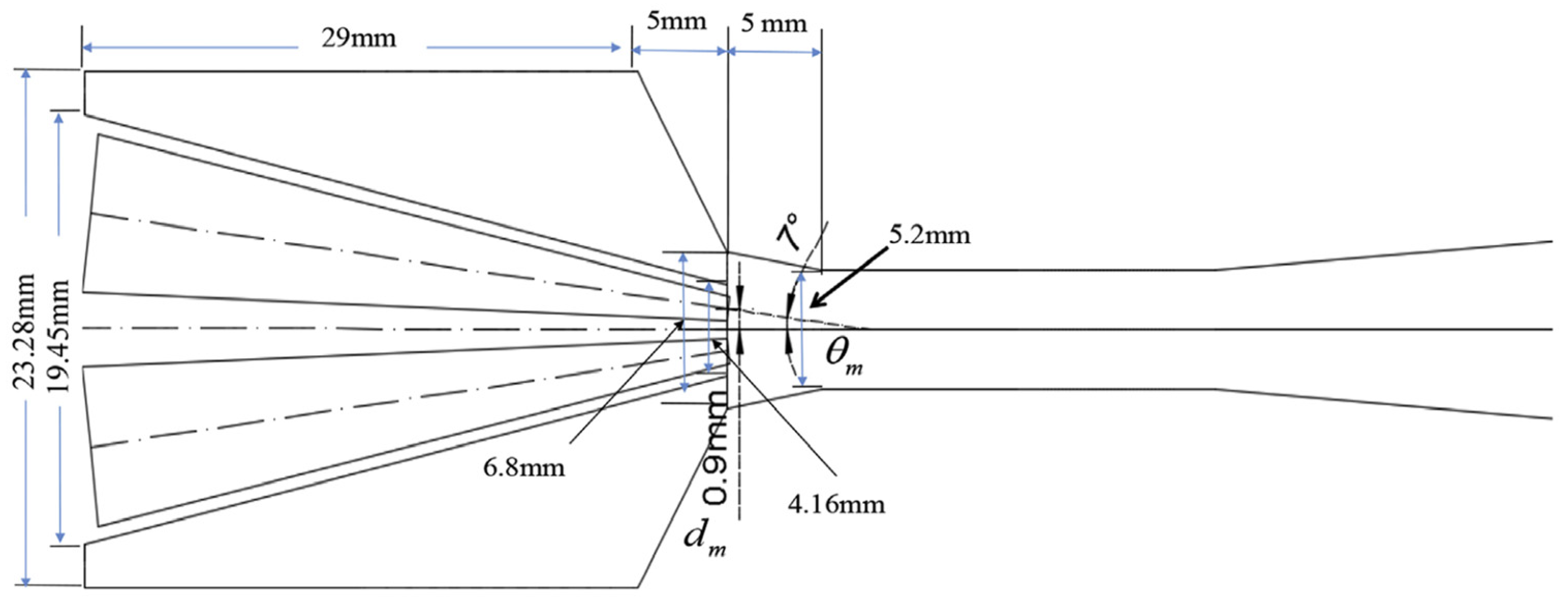
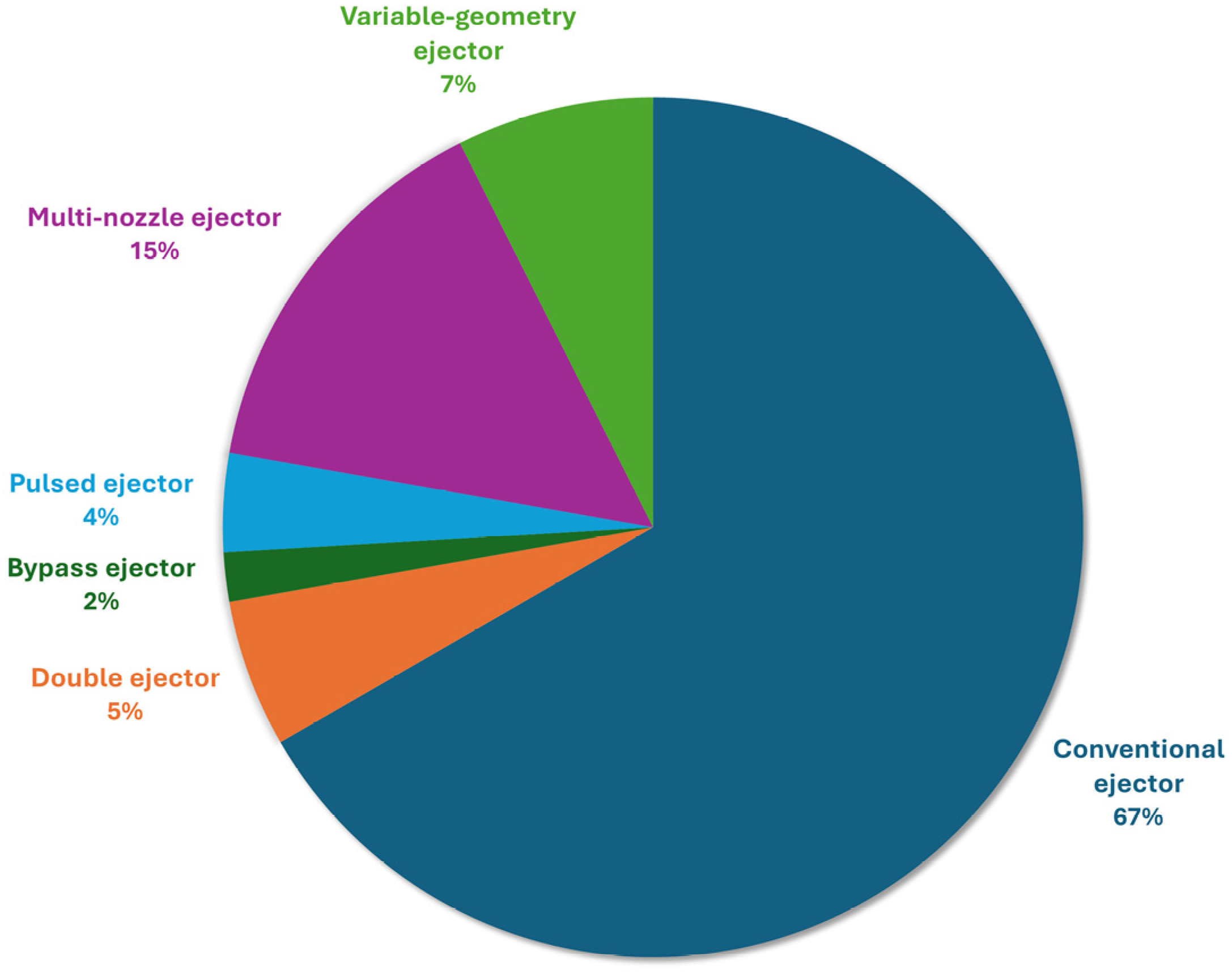
3.1.1. Structure
3.1.2. Operational States
- Critical or dual choking state: This mode is characterized by the discharge pressure being lower than the critical pressure. In this state, the entrainment ratio remains relatively stable, and the ejector operates at its highest efficiency;
- Subcritical or single choking state: In this mode, the discharge pressure (Pc,b < Pb < P0,b) exceeds the critical pressure. As a result, the entrainment ratio experiences a significant reduction as the discharge pressure increases;
- Backflow or malfunction mode: In this mode, Pb > P0,b, the entrainment is reversed, the ejector is not able to work, and no suction occurs.
3.1.3. Various Ejector-Based Hydrogen Recirculation Configuration in Fuel Cell Systems
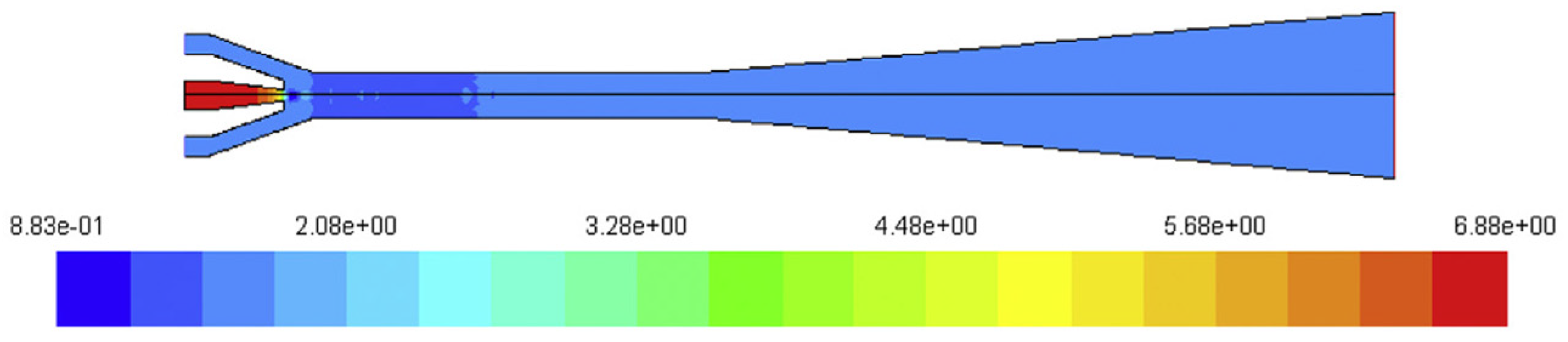
3.2. Numerical Modeling
4. Limitations and Future Directions
5. Conclusions
- More precise numerical simulations should be performed in order to state the significance of pressure drops across various ejector designs, highlighting the impact of shape and configuration on performance;
- Although some studies consider various ejector shapes and configurations to cover a wide operating range, including multi-nozzle and double ejector designs, further research is required to optimize these designs;
- More detailed and sophisticated numerical analysis should be considered to better mimic the ejector’s performance and decrease the limitations of simulation. This can be achieved by employing more advanced turbulence models together with 3D simulations.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Liu, Y.; Tu, Z.; Chan, S.H. Applications of ejectors in proton exchange membrane fuel cells: A review. Fuel Process. Technol. 2021, 214, 106683. [Google Scholar] [CrossRef]
- Wu, S.; Liu, Z.; Du, J.; Liu, Y. Change of global ocean temperature and decadal variability under 1.5 °C warming in FOAM. J. Mar. Sci. Eng. 2022, 10, 1231. [Google Scholar] [CrossRef]
- Gu, L.; Chen, J.; Yin, J.; Slater, L.J.; Wang, H.M.; Guo, Q.; Feng, M.; Qin, H.; Zhao, T. Global increases in compound flood-hot extreme hazards under climate warming. Geophys. Res. Lett. 2022, 49, e2022GL097726. [Google Scholar] [CrossRef]
- Lü, X.; Qu, Y.; Wang, Y.; Qin, C.; Liu, G. A comprehensive review on hybrid power system for PEMFC-HEV: Issues and strategies. Energy Convers. Manag. 2018, 171, 1273–1291. [Google Scholar] [CrossRef]
- Peighambardoust, S.J.; Rowshanzamir, S.; Amjadi, M. Review of the proton exchange membranes for fuel cell applications. Int. J. Hydrogen Energy 2010, 35, 9349–9384. [Google Scholar] [CrossRef]
- Başar, M.S.; Çağlayan, B.S.; Aksoylu, A.E. A study on catalytic hydrogen production: Thermodynamic and experimental analysis of serial OSR-PROX system. Fuel Process. Technol. 2018, 178, 301–311. [Google Scholar] [CrossRef]
- Wang, X.; Qin, Y.; Wu, S.; Shangguan, X.; Zhang, J.; Yin, Y. Numerical and experimental investigation of baffle plate arrangement on proton exchange membrane fuel cell performance. J. Power Sources 2020, 457, 228034. [Google Scholar] [CrossRef]
- Walters, M.; Wick, M.; Tinz, S.; Ogrzewalla, J.; Sehr, A.; Pischinger, S. Fuel Cell System Development. SAE Int. J. Altern. Powertrains 2018, 7, 335–350. [Google Scholar] [CrossRef]
- Hassan, Q.; Azzawi, I.D.; Sameen, A.Z.; Salman, H.M. Hydrogen fuel cell vehicles: Opportunities and challenges. Sustainability 2023, 15, 11501. [Google Scholar] [CrossRef]
- Fragiacomo, P.; Genovese, M.; Piraino, F.; Corigliano, O.; De Lorenzo, G. Hydrogen-fuel cell hybrid powertrain: Conceptual layouts and current applications. Machines 2022, 10, 1121. [Google Scholar] [CrossRef]
- Bethoux, O. Hydrogen fuel cell road vehicles and their infrastructure: An option towards an environmentally friendly energy transition. Energies 2020, 13, 6132. [Google Scholar] [CrossRef]
- Shen, J.; Liu, Z.; Liu, F.; Liu, W. Numerical simulation of water transport in a proton exchange membrane fuel cell flow channel. Energies 2018, 11, 1770. [Google Scholar] [CrossRef]
- Song, Y.; Zhang, C.; Jia, Q.; Birgersson, E.; Han, M.; Zhang, P. Novel closed anode pressure-swing system for proton exchange membrane fuel cells. Int. J. Hydrogen Energy 2020, 45, 17727–17735. [Google Scholar] [CrossRef]
- Liu, J.; Li, Q.; Chen, W.; Cao, T. A discrete hidden Markov model fault diagnosis strategy based on K-means clustering dedicated to PEM fuel cell systems of tramways. Int. J. Hydrogen Energy 2018, 43, 12428–12441. [Google Scholar] [CrossRef]
- Liu, Z.; Liu, Z.; Jiao, K.; Yang, Z.; Zhou, X.; Du, Q. Numerical investigation of ejector transient characteristics for a 130-kW PEMFC system. Int. J. Energy Res. 2020, 44, 3697–3710. [Google Scholar] [CrossRef]
- Maghsoodi, A.; Afshari, E.; Ahmadikia, H. Optimization of geometric parameters for design a high-performance ejector in the proton exchange membrane fuel cell system using artificial neural network and genetic algorithm. Appl. Therm. Eng. 2014, 71, 410–418. [Google Scholar] [CrossRef]
- Xue, H.; Wang, L.; Zhang, H.; Jia, L.; Ren, J. Design and investigation of multi-nozzle ejector for PEMFC hydrogen recirculation. Int. J. Hydrogen Energy 2020, 45, 14500–14516. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, L.; Zhang, H.; Jia, L. Optimization of ejector structure for the PEMFC hydrogen recirculation system. In Proceedings of the 2020 Chinese Automation Congress (CAC), Shanghai, China, 6–8 November 2020; pp. 2954–2959. [Google Scholar]
- Jenssen, D.; Berger, O.; Krewer, U. Improved PEM fuel cell system operation with cascaded stack and ejector-based recirculation. Appl. Energy 2017, 195, 324–333. [Google Scholar] [CrossRef]
- Liu, Y.; Tu, Z.; Chan, S.H. Performance analysis and dynamic characteristics of a proton exchange membrane fuel cell with dual recirculation pumps for air-free applications. J. Power Sources 2023, 566, 232926. [Google Scholar] [CrossRef]
- Singer, G.; Pinsker, R.; Stelzer, M.; Aggarwal, M.; Pertl, P.; Trattner, A. Ejector validation in proton exchange membrane fuel cells: A comparison of turbulence models in computational fluid dynamics (CFD) with experiment. Int. J. Hydrogen Energy 2024, 61, 1405–1416. [Google Scholar] [CrossRef]
- Little, A.B.; Garimella, S. A review of ejector technology for refrigeration applications. Int. J. Air-Cond. Refrig. 2011, 19, 1–15. [Google Scholar] [CrossRef]
- Scopus. Available online: https://www.scopus.com/ (accessed on 17 August 2024).
- Web of Science. Available online: https://www.webofscience.com/ (accessed on 17 August 2024).
- Gil, B.; Kasperski, J. Performance estimation of ejector cycles using ethers and fluorinated ethers as refrigerants. Appl. Therm. Eng. 2018, 133, 269–275. [Google Scholar] [CrossRef]
- Rusly, E.; Aye, L.; Charters, W.; Ooi, A. CFD analysis of ejector in a combined ejector cooling system. Int. J. Refrig. 2005, 28, 1092–1101. [Google Scholar] [CrossRef]
- Li, M.; Lin, M.; Wang, L.; Wang, Y.; Pan, F.; Zhao, X. Observation and Analysis of Ejector Hysteresis Phenomena in the Hydrogen Recirculation Subsystem of PEMFCs. Entropy 2023, 25, 426. [Google Scholar] [CrossRef]
- Li, K.; Wang, C.; Li, J.; Wang, L.; Li, Z.; Zhang, C. Experimental Investigation into the Performance of PEMFCs with Three Different Hydrogen Recirculation Schemes. Inventions 2024, 9, 33. [Google Scholar] [CrossRef]
- Tang, X.; Yang, M.; Shi, L.; Hou, Z.; Xu, S.; Sun, C. Adaptive state-of-health temperature sensitivity characteristics for durability improvement of PEM fuel cells. Chem. Eng. J. 2024, 491, 151951. [Google Scholar] [CrossRef]
- Tang, X.; Shi, L.; Zhang, Y.; Li, B.; Xu, S.; Song, Z. Degradation adaptive energy management strategy for FCHEV based on the Rule-DDPG method: Tailored to the current SOH of the powertrain. In IEEE Transactions on Transportation Electrification; IEEE: Piscataway, NJ, USA, 2024. [Google Scholar]
- Bai, S.; Wang, L.; Wang, X. Optimization of ejector geometric parameters with hybrid artificial fish swarm algorithm for PEM fuel cell. In Proceedings of the 2017 Chinese Automation Congress (CAC), Jinan, China, 20–22 October 2017; pp. 3319–3322. [Google Scholar]
- Ma, T.; Cong, M.; Meng, Y.; Wang, K.; Zhu, D.; Yang, Y. Numerical studies on ejector in proton exchange membrane fuel cell system with anodic gas state parameters as design boundary. Int. J. Hydrogen Energy 2021, 46, 38841–38853. [Google Scholar] [CrossRef]
- Le Tri, D.T.; Vu, H.N.; Woo, J.; Kim, Y.; Yu, S. Optimization of the ejector parameters for anodic recirculation systems in high-performance dual-stack proton-exchange membrane fuel cells. Energy Convers. Manag. 2023, 296, 117712. [Google Scholar] [CrossRef]
- Chen, L.; Xu, K.; Yang, Z.; Yan, Z.; Dong, Z. Optimal design and operation of dual-ejector PEMFC hydrogen supply and circulation system. Energies 2022, 15, 5427. [Google Scholar] [CrossRef]
- Yu, M.; Wang, C.; Wang, L.; Wang, X.; Li, D. Auxiliary-ejector-based hydrogen recirculation system to broaden PEMFC operating range. Int. J. Hydrogen Energy 2024, 57, 515–529. [Google Scholar] [CrossRef]
- Hwang, J.-J. Passive hydrogen recovery schemes using a vacuum ejector in a proton exchange membrane fuel cell system. J. Power Sources 2014, 247, 256–263. [Google Scholar] [CrossRef]
- Chen, L.; Xu, K.; Yang, Z.; Yan, Z.; Zhai, C.; Dong, Z. Optimal design of a novel nested-nozzle ejector for PEMFC’s hydrogen supply and recirculation system. Int. J. Hydrogen Energy 2023, 48, 27330–27343. [Google Scholar] [CrossRef]
- Baba, S.; Kobayashi, N.; Takahashi, S.; Hirano, S. Development of anode gas recycle system using ejector for 1 kW solid oxide fuel cell. J. Eng. Gas Turbine. Power 2015, 137, 021504. [Google Scholar] [CrossRef]
- Feng, J.; Han, J.; Hou, T.; Peng, X. Performance analysis and parametric studies on the primary nozzle of ejectors in proton exchange membrane fuel cell systems. In Energy Sources, Part A: Recovery, Utilization, and Environmental Effects; Taylor & Francis: Abingdon, UK, 2020; pp. 1–20. [Google Scholar]
- Han, J.; Zhao, B.; Pang, Z.; Feng, J.; Peng, X. Transient characteristics investigation of the integrated ejector-driven hydrogen recirculation by multi-component CFD simulation. Int. J. Hydrogen Energy 2022, 47, 29053–29068. [Google Scholar] [CrossRef]
- Hosseinzadeh, E.; Rokni, M.; Jabbari, M.; Mortensen, H. Numerical analysis of transport phenomena for designing of ejector in PEM forklift system. Int. J. Hydrogen Energy 2014, 39, 6664–6674. [Google Scholar] [CrossRef]
- Huang, P.-H.; Kuo, J.-K.; Wu, C.-B. Simulation and experimental measurements of 10-kW PEMFC passive hydrogen recovery system. Int. J. Hydrogen Energy 2023, 48, 16790–16801. [Google Scholar] [CrossRef]
- Huang, Y.; Jiang, P.; Zhu, Y. Shape optimization and flow irreversibility mechanism analysis of normal temperature, high temperature and wet stream ejectors. Appl. Therm. Eng. 2024, 242, 122468. [Google Scholar] [CrossRef]
- Kuo, J.-K.; Jiang, W.-Z.; Li, C.-H.; Hsu, T.-H. Numerical investigation into hydrogen supply stability and IV performance of PEM fuel cell system with passive Venturi ejector. Appl. Therm. Eng. 2020, 169, 114908. [Google Scholar] [CrossRef]
- He, J.; Ahn, J.; Choe, S.-Y. Analysis and control of a fuel delivery system considering a two-phase anode model of the polymer electrolyte membrane fuel cell stack. J. Power Sources 2011, 196, 4655–4670. [Google Scholar] [CrossRef]
- Bernardi, D.; Bozzolo, M.; Marsano, F.; Tarnowski, O.; Agnew, G. Ejectors Design in the Rolls-Royce 1MW Hybrid System. In Proceedings of the ASME Turbo Expo 2005: Power for Land, Sea, and Air, Reno, NV, USA, 6–9 June 2005. [Google Scholar]
- Ferrari, M.L.; Bernardi, D.; Massardo, A.F. Design and testing of ejectors for high temperature fuel cell hybrid systems. J. Fuel Cell Sci. Technol. 2006, 3, 284–291. [Google Scholar] [CrossRef]
- Zhu, Y.; Li, Y. New theoretical model for convergent nozzle ejector in the proton exchange membrane fuel cell system. J. Power Sources 2009, 191, 510–519. [Google Scholar] [CrossRef]
- Zhu, Y.; Li, Y.; Cai, W. Control oriented modeling of ejector in anode gas recirculation solid oxygen fuel cell systems. Energy Convers. Manag. 2011, 52, 1881–1889. [Google Scholar] [CrossRef]
- Brunner, D.A.; Marcks, S.; Bajpai, M.; Prasad, A.K.; Advani, S.G. Design and characterization of an electronically controlled variable flow rate ejector for fuel cell applications. Int. J. Hydrogen Energy 2012, 37, 4457–4466. [Google Scholar] [CrossRef]
- Hwang, J.-J.; Cho, C.-C.; Wu, W.; Chiu, C.-H.; Chiu, K.-C.; Lin, C.-H. Numerical and experimental investigation into passive hydrogen recovery scheme using vacuum ejector. J. Power Sources 2015, 275, 539–546. [Google Scholar] [CrossRef]
- Nikiforow, K.; Koski, P.; Karimäki, H.; Ihonen, J.; Alopaeus, V. Designing a hydrogen gas ejector for 5 kW stationary PEMFC system–CFD-modeling and experimental validation. Int. J. Hydrogen Energy 2016, 41, 14952–14970. [Google Scholar] [CrossRef]
- Yin, Y.; Fan, M.; Jiao, K.; Du, Q.; Qin, Y. Numerical investigation of an ejector for anode recirculation in proton exchange membrane fuel cell system. Energy Convers. Manag. 2016, 126, 1106–1117. [Google Scholar] [CrossRef]
- Besagni, G.; Mereu, R.; Inzoli, F.; Chiesa, P. Application of an integrated lumped parameter-CFD approach to evaluate the ejector-driven anode recirculation in a PEM fuel cell system. Appl. Therm. Eng. 2017, 121, 628–651. [Google Scholar] [CrossRef]
- Genc, O.; Toros, S.; Timurkutluk, B. Determination of optimum ejector operating pressures for anodic recirculation in SOFC systems. Int. J. Hydrogen Energy 2017, 42, 20249–20259. [Google Scholar] [CrossRef]
- Chen, J.; Chen, Y.; Zhang, H.; Weng, S. Effect of different operating strategies for a SOFC-GT hybrid system equipped with anode and cathode ejectors. Energy 2018, 163, 1–14. [Google Scholar] [CrossRef]
- Pei, P.; Ren, P.; Li, Y.; Wu, Z.; Chen, D.; Huang, S.; Jia, X. Numerical studies on wide-operating-range ejector based on anodic pressure drop characteristics in proton exchange membrane fuel cell system. Appl. Energy 2019, 235, 729–738. [Google Scholar] [CrossRef]
- Baba, S.; Ohguri, N.; Suzuki, Y.; Murakami, K. Evaluation of a variable flow ejector for anode gas circulation in a 50-kW class SOFC. Int. J. Hydrogen Energy 2020, 45, 11297–11308. [Google Scholar] [CrossRef]
- Wang, X.; Lu, Y.; Zhang, B.; Liu, J.; Xu, S. Experimental analysis of an ejector for anode recirculation in a 10 kW polymer electrolyte membrane fuel cell system. Int. J. Hydrogen Energy 2022, 47, 1925–1939. [Google Scholar] [CrossRef]
- Chen, F.; Hou, M.; Li, J.; Pei, Y.; Wang, Y. Proton exchange membrane fuel cell ejector test platform design and ejector test analysis. World Electr. Veh. J. 2021, 12, 103. [Google Scholar] [CrossRef]
- Du, Z.; Liu, Q.; Wang, X.; Wang, L. Performance investigation on a coaxial-nozzle ejector for PEMFC hydrogen recirculation system. Int. J. Hydrogen Energy 2021, 46, 38026–38039. [Google Scholar] [CrossRef]
- Han, J.; Feng, J.; Hou, T.; Peng, X. Performance investigation of a multi-nozzle ejector for proton exchange membrane fuel cell system. Int. J. Energy Res. 2021, 45, 3031–3048. [Google Scholar] [CrossRef]
- Kuo, J.-K.; Hsieh, C.-Y. Numerical investigation into effects of ejector geometry and operating conditions on hydrogen recirculation ratio in 80 kW PEM fuel cell system. Energy 2021, 233, 121100. [Google Scholar] [CrossRef]
- Song, Y.; Wang, X.; Wang, L.; Pan, F.; Chen, W.; Xi, F. A twin-nozzle ejector for hydrogen recirculation in wide power operation of polymer electrolyte membrane fuel cell system. Appl. Energy 2021, 300, 117442. [Google Scholar] [CrossRef]
- Singer, G.; Gappmayer, G.; Macherhammer, M.; Pertl, P.; Trattner, A. A development toolchain for a pulsed injector-ejector unit for PEM fuel cell applications. Int. J. Hydrogen Energy 2022, 47, 23818–23832. [Google Scholar] [CrossRef]
- Fan, A.M.; Fan, D.X.; Xiao, W.P.; Ye, C.L. Simulation and optimization design of hydrogen ejector in proton exchange membrane fuel cell system. In Proceedings of the 2nd International Conference on Energy, Power and Advanced Thermodynamic Systems (EPATS 2022), Nanjing, China, 21–24 July 2022. [Google Scholar]
- Han, J.; Feng, J.; Peng, X. Phase change characteristics and their effect on the performance of hydrogen recirculation ejectors for PEMFC systems. Int. J. Hydrogen Energy 2022, 47, 1144–1156. [Google Scholar] [CrossRef]
- Huang, Y.; Jiang, P.; Zhu, Y. Quasi-two-dimensional ejector model for anode gas recirculation fuel cell systems. Energy Convers. Manag. 2022, 262, 115674. [Google Scholar] [CrossRef]
- Li, C.; Sun, B.; Luo, Q. Effect of Structural Parameters and Operational Characteristic Analysis on Ejector Used in Proton Exchange Membrane Fuel Cell. Sustainability 2022, 14, 9205. [Google Scholar] [CrossRef]
- Liu, Y.; Tu, Z.; Chan, S.H. Performance enhancement in a H2/O2 PEMFC with dual-ejector recirculation. Int. J. Hydrogen Energy 2022, 47, 12698–12710. [Google Scholar] [CrossRef]
- Liu, Q.; Xu, Z.; Wang, A.; Ping, Z.; Wang, L. Weight analysis on geometric parameters of ejector under high back pressure condition of SOFC recirculation system. Int. J. Hydrogen Energy 2022, 47, 27150–27165. [Google Scholar] [CrossRef]
- Sun, W.; Zhang, H.; Jia, L.; Xue, H. Study on multicomponent and multiphase of the ejector for proton exchange membrane fuel cell hydrogen recirculation. J. Therm. Anal. Calorim. 2022, 147, 13681–13697. [Google Scholar] [CrossRef]
- Zhang, T.; Wang, L.; Dong, Z.; Zhou, X. Numerical analysis of key structural parameters of ejector for PEMFC system under low power conditions. Vibroengineering Procedia 2022, 44, 117–123. [Google Scholar] [CrossRef]
- Bian, J.; Zhang, Y.; Liu, Y.; Gong, L.; Cao, X. Structural optimization of hydrogen recirculation ejector for proton exchange membrane fuel cells considering the boundary layer separation effect. J. Clean. Prod. 2023, 397, 136535. [Google Scholar] [CrossRef]
- Chen, Y.; Maji, L.; Huang, Y. Design and Analysis on Separator-Ejector Integrated Device of Hydrogen Recirculation System in Proton Exchange Membrane Fuel Cell; SAE Technical Paper; 2023; Available online: https://www.researchgate.net/publication/356672552_Designing_and_performance_investigations_on_an_ejector_with_auxiliary_inlet_for_PEMFC_hydrogen_recirculation_system (accessed on 18 August 2024).
- Ding, H.; Dong, Y.; Zhang, Y.; Yang, Y.; Wen, C. Energy efficiency assessment of hydrogen recirculation ejectors for proton exchange membrane fuel cell (PEMFC) system. Appl. Energy 2023, 346, 121357. [Google Scholar] [CrossRef]
- Kuo, J.-K.; Wu, P.-R.; Yang, T.-F.; Yan, W.-M. Optimal technical analysis of vacuum ejector for passive hydrogen recovery. Int. J. Hydrogen Energy 2023, 48, 8260–8272. [Google Scholar] [CrossRef]
- Ping, Z.; Chen, B.; Wang, C.; Xu, Z.; Wang, L. High performance ejector enhanced by heat exchanger in solid oxide fuel cell anode recirculation system. Appl. Therm. Eng. 2023, 221, 119856. [Google Scholar] [CrossRef]
- Yin, B.; Li, Z.; Dong, F.; Xu, S.; Ni, H. A novel dual-nozzle ejector for enhancement of hydrogen recirculation applied to proton exchange membrane fuel cell system. J. Power Sources 2023, 580, 233444. [Google Scholar] [CrossRef]
- Zhang, L.; Li, C.; Liu, Q.; Wan, X.; Fu, J. A Novel Ejector with Water-Separator Function for Proton Exchange Membrane Fuel Cell System Based on a Transient 3D Model. J. Energy Eng. 2023, 149, 04023012. [Google Scholar] [CrossRef]
- Le Tri, D.T.; Vu, H.N.; Nguyen, H.L.; Kim, Y.; Yu, S. A comparative study of single and dual ejector concepts for anodic recirculation system in high-performance vehicular proton exchange membrane fuel cells. Int. J. Hydrogen Energy 2023, 48, 27344–27360. [Google Scholar] [CrossRef]
- Yu, M.; Wang, C.; Wang, L.; Wang, X. Flow characteristics of coaxial-nozzle ejector for PEMFC hydrogen recirculation system. Appl. Therm. Eng. 2024, 236, 121541. [Google Scholar] [CrossRef]








| Database | Search Syntax |
|---|---|
| Scopus | TITLE-ABS-KEY (ejector AND (CFD OR simulation) AND fuel cell) |
| Web of Science | (TS = (ejector) AND (TS = (CFD) OR TS = (simulation)) AND TS = (fuel cell)) |
| Author (Year) | Ejector Type | Nozzle Type | Power Range |
|---|---|---|---|
| Bernardi et al. [46] (2005) | Conventional | Subsonic | 1 MW |
| Supersonic | |||
| Ferrari et al. [47] (2006) | Conventional | Subsonic | 1 MW |
| Supersonic | |||
| Zhu et al. [48] (2009) | Conventional | Subsonic | N/A |
| Zhu et al. [49] (2011) | Conventional | Subsonic | N/A |
| Brunner et al. [50] (2012) | Variable geometry | Subsonic | 7–17 kW |
| Hosseinzadeh et al. [41] (2014) | Double ejector | Subsonic | 1.3–12.5 kW |
| Hwang et al. [36] (2014) | Pulse ejector | Supersonic | 1.45 kW |
| Hwang et al. [51] (2015) | Conventional | Supersonic | N/A |
| Baba et al. [38] (2015) | Variable geometry | Subsonic | 1 kW |
| Nikiforow et al. [52] (2016) | Conventional | Subsonic | 5 kW |
| Yin et al. [53] (2016) | Conventional | Subsonic | 80 kW |
| Besagni et al. [54] (2017) | Conventional | Subsonic | 25–200 kW |
| Genc et al. [55] (2017) | Conventional | Supersonic | 18 kW |
| Jenssen et al. [19] (2017) | Variable geometry | Subsonic | 100 kW |
| Chen et al. [56] (2018) | Conventional | Supersonic | 270 kW |
| Pei et al. [57] (2019) | Conventional | Subsonic | 10 kW |
| Baba et al. [58] (2020) | Variable geometry | Subsonic | 50 kW |
| Feng et al. [39] (2020) | Conventional | Supersonic | 40–160 kW |
| Kuo et al. [44] (2020) | Conventional | Subsonic | 3 kW |
| Liu et al. [15] (2020) | Conventional | Subsonic | 130 kW |
| Wang et al. [59] (2020) | Conventional | Subsonic | 40 kW |
| Xue et al. [17] (2020) | Multi-nozzle | Subsonic | 20–100 kW |
| Zhang et al. [18] (2020) | Conventional | Subsonic | N/A |
| Chen et al. [60] (2021) | Conventional | Supersonic | N/A |
| Du et al. [61] (2021) | Multi-nozzle | Subsonic | 17.9–84 kW |
| Han et al. [62] (2021) | Multi-nozzle | Subsonic | 22–100 kW |
| Kuo et al. [63] (2021) | Conventional | Supersonic | 80 kW |
| Ma et al. [32] (2021) | Conventional | Subsonic | N/A |
| Song et al. [64] (2021) | Multi-nozzle | Subsonic | 17–85 kW |
| Chen et al. [34] (2022) | Double ejector | Subsonic | 6–70 kW |
| Singer et al. [65] (2022) | Pulsed ejector | Subsonic | N/A |
| Fan et al. [66] (2022) | Conventional | Subsonic | 10.7–57.2 kW |
| Han et al. [67] (2022) | Conventional | Subsonic | 5 kW |
| Han et al. [40] (2022) | Conventional | Subsonic | 80 kW |
| Huang et al. [68] (2022) | Conventional | Subsonic | 4–6 kW |
| Li et al. [69] (2022) | Conventional | Subsonic | 3.8–23.5 kW |
| Liu et al. [70] (2022) | Conventional | Subsonic | 1 kW |
| Liu et al. [71] (2022) | Conventional | Subsonic | N/A |
| Sun et al. [72] (2022) | Conventional | Subsonic | 40–70 kW |
| Zhang et al. [73] (2022) | Conventional | Subsonic | N/A |
| Bian et al. [74] (2023) | Conventional | Subsonic | N/A |
| Chen et al. [75] (2023) | Conventional with water separator | Subsonic | 45–100 kW |
| Chen et al. [37] (2023) | Multi-nozzle | Subsonic | 13.5–100 kW |
| Ding et al. [76] (2023) | Conventional | Subsonic | N/A |
| Huang et al. [42] (2023) | Conventional | Supersonic | 10 kW |
| Kuo et al. [77] (2023) | Conventional | Subsonic | 1 kW |
| Ping et al. [78] (2023) | Conventional | Subsonic | 34.84–45.88 kW |
| Yin et al. [79] (2023) | Multi-nozzle | Subsonic | N/A |
| Zhang et al. [80] (2023) | Conventional with water separator | Subsonic | 30, 40, 50 kW |
| Le tri et al. [81] (2023) | Double ejector | Subsonic | 100 kW |
| Huang et al. [42] (2024) | Conventional | Supersonic | N/A |
| Singer et al. [21] (2024) | Variable geometry | Subsonic | 160 kW |
| Yu et al. [82] (2024) | Multi-nozzle | Subsonic | 17–110 kW |
| Yu et al. [35] (2024) | Bypass ejector | Subsonic | 17.9–84 kW |
| Li et al. [28] (2024) | Multi-nozzle | Supersonic | N/A |
| Author (Year) | Working Fluid | Dimensions | Turbulence Model | Grid Size (Type) | Experimental Validation | Remarks |
|---|---|---|---|---|---|---|
| Bernardi et al. [46] (2005) | Methane | 2D axisymmetric | k-ε (Standard) | N/A | Yes | CFD, despite its limitations, aids in optimizing ejector design. |
| Ferrari et al. [47] (2006) | Methane | 2D axisymmetric | k-ε (Standard) | N/A | Yes | CFD-validated models have enhanced ejector performance for hybrid systems by optimizing geometry. |
| Zhu et al. [48] (2009) | Hydrogen | 2D axisymmetric | k-ε (RNG) | N/A | N/A | CFD, regression analysis, and parameter recognition approaches are used to determine the exponent of the function of velocity. |
| Zhu et al. [49] (2011) | Methane | 2D axisymmetric | k-ε (RNG) | N/A | N/A | CFD method is used to achieve the required information to establish the model factors. |
| Brunner et al. [50] (2012) | Hydrogen and water vapor | 2D axisymmetric | k-ω (SST) | N/A | Yes | The CFD results match the measurements, except at 17 kW, where the actual pressure rise is lower. This discrepancy could be due to the model not considering condensation or freezing of water vapor at high flow rates. |
| Hosseinzadeh et al. [41] (2014) | Hydrogen and water vapor | 2D axisymmetric | k-ω (SST) | 37,640 (Triangular) | N/A | A CFD model is first developed and validated using established literature, then applied to various ejector geometries under diverse conditions. |
| Hwang et al. [50] (2015) | Hydrogen | 3D | k-ε | N/A | Yes | Simulations study how mass flow rate at the inlet together with nozzle throat diameter affect pressure, Mach number, temperature, and flow rates in suction and outlet channels. |
| Nikiforow et al. [52] (2016) | Hydrogen | 2D axisymmetric | k-ε (RNG, Realizable) k-ω (SST) | 159,000 | Yes | CFD results varied by 60–70% from experimental data, with less deviation in PEMFC-related conditions. |
| Yin et al. [53] (2016) | Dry hydrogen | 3D | k-ε (RNG) | 1–1.5 million | N/A | Ejector selectivity is influenced by the secondary flow’s humidity and temperature, with more water vapor and less hydrogen recirculated at higher levels of both. |
| Besagni et al. [54] (2017) | Air, Hydrogen | 2D axisymmetric | k-ω (SST) | 320,000 (Quadrilateral) | N/A | An integrated model combining lumped parameters and CFD is suggested to study the performance of convergent-nozzle ejectors in PEMFC anode recirculation. |
| Genc et al. [55] (2017) | Methane | 3D | k-ε (RNG) | 400,000 | Yes | The optimal ejector operating pressures, the impact of varying inlet and outlet pressures, and the fuel inlet temperature on ejector performance for a fixed geometry are numerically studied. |
| Jenssen et al. [19] (2017) | Hydrogen-air | 2D axisymmetric | k-ω (SST) | N/A | Yes | CFD is used to assess the performance of an ejector and an automotive fuel cell stack. |
| Pei et al. [57] (2019) | Hydrogen | 2D axisymmetric | k-ε (Standard) | N/A | No | A CFD model is developed that integrates the stationary characteristic equation of a hydrogen ejector. This equation is derived using the formula for anodic pressure drop. |
| Feng et al. [39] (2020) | Air | 3D | k- ω (SST) | 213,264 (Hexahedral) | Yes | A 3D numerical model was developed to study ejectors, and its consistency was confirmed through experimental validation. |
| Kuo et al. [44] (2020) | Hydrogen | 2D axisymmetric | N/A | 25,058 (Triangular) | Yes | The simulation structure is employed to analyze the temperature, pressure, and velocity distributions inside the ejector for different inlet hydrogen pressure magnitudes. |
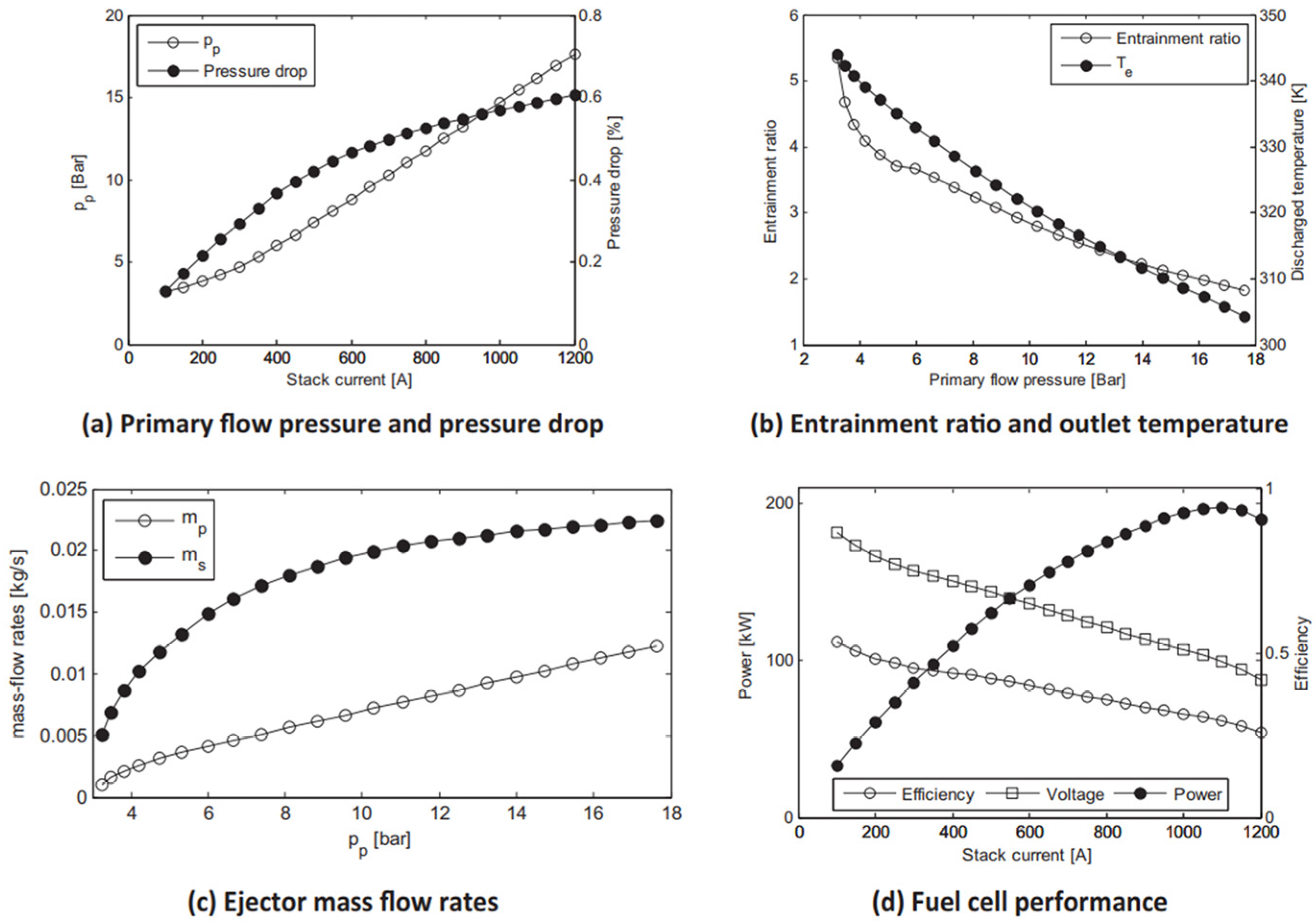
| Liu et al. [15] (2020) | Hydrogen | 3D | k-ε (RNG) | 2 million (Hexahedral) | No | A dynamic 3D ejector model for the PEMFC system has been developed and validated with results from experiments, including entrainment ratios and mass flow rates. This model is utilized to investigate the effects of different operational statuses, such as pressure, water vapor, and nitrogen mass fraction. |
| Wang et al. [59] (2020) | Hydrogen | 2D axisymmetric | k-ε (RNG) | N/A | N/A | The prediction model of the outlet humidity of the ejector is established and validated by simulation. |
| Xue et al. [17] (2020) | Hydrogen | 3D | k-ε (RNG) | 1 million (Hexahedral) | Yes | Numerical simulations use a validated 3D model to study the performance as well as the inner flow distributed inside a multi-nozzle ejector. |
| Zhang et al. [18] (2020) | Hydrogen | 2D axisymmetric | k-ε (RNG) | 107,782 (Triangular) | No | CFD calculations and experiments are employed to assess how changes in NXP and working conditions affect ejector performance, as well as to examine how NXP’s impact evolves under varying working conditions. |
| Chen et al. [60] (2021) | Hydrogen | N/A | k-ε (RNG, Realizable, standard) k-ω (SST) | N/A | Yes | Different turbulence models are utilized to analyze the performance of the ejector. |
| Du et al. [61] (2021) | Hydrogen | 2D axisymmetric | k-ε (RNG) | 162,020 (Triangular) | Yes | The proposed ejector undergoes numerical investigation using an experimentally validated simulation model to analyze flow distribution and anticipate its performance. |
| Han et al. [62] (2021) | Air | 3D | k-ω (SST) | 387,974 | Yes | A 3D CFD model was developed to simulate ejector performance, and an experimental apparatus was constructed to verify the simulation’s accuracy. |
| Ma et al. [32] (2021) | Dry hydrogen | 3D | k-ε (RNG) | 40,000 | No | A CFD model of an ejector is developed, and a sensitivity analysis is conducted to assess the entrainment performance with respect to four key geometrical factors of the ejector. |
| Song et al. [64] (2021) | Hydrogen | 3D | k-ε (RNG) | 1.5 million (Hexahedral) | Yes | A CFD model for the recommended twin-nozzle ejector was prepared, and then the verification of the results was obtained using experiments. |
| Chen et al. [34] (2022) | Hydrogen | 3D | k-ω (SST) | N/A | Yes | CFD modeling is utilized to optimize design and control processes, while experimental tests are conducted to verify the simulation outcomes. |
| Singer et al. [65] (2022) | Hydrogen | 1D, 3D | N/A | 1.68 million (Hexahedral, tetrahedral) | Yes | 1D and 3D CFD simulations correlated perfectly with experimental measurements. |
| Fan et al. [66] (2022) | Hydrogen | 3D | N/A | 800,000 (Hexahedral) | N/A | The parameters of the diffuser section and mixing section of an ejector were studied and analyzed using CFD. |
| Han et al. [67] (2022) | Hydrogen | 2D axisymmetric | k-ω (SST) | 55,179 (Quadrilateral) | N/A | CFD is done neglecting the phase change of vapor and also considering the single-phase flow |
| Han et al. [39] (2022) | Hydrogen | 3D | k-ω (SST) | 603,255 (Hexahedral) | N/A | A 3D CFD model is prepared to examine the behavior of hydrogen recirculation-based elements, including the hydrogen injector, ejector, and purge valve. |
| Huang et al. [68] (2022) | Hydrogen | 2D axisymmetric | k-ε (RNG) | 500,000 | Yes | The performance of an ejector, together with the parameters of flow, is gained by means of CFD and experimental studies. |
| Li et al. [69] (2022) | Hydrogen | 2D axisymmetric | k-ω (SST) | 153,144 | N/A | A CFD model is constructed to optimize geometrical-based parameters, taking into account performance across various operating conditions. |
| Liu et al. [70] (2022) | Hydrogen | 2D axisymmetric | N/A | 246,891 (Hexahedral) | N/A | A thorough examination is conducted to explore how ejector operational conditions affect the performance of PEMFC. |
| Liu et al. [71] (2022) | Methane | 2D axisymmetric | k-ε (RNG) | 115,937 | Yes | This paper conducts an assessment of the geometrical parameters of the ejector using the results derived from an experimentally validated simulation. |
| Sun et al. [72] (2022) | Hydrogen | 2D axisymmetric | k-ε (RNG) | 122,470 (Quadrilateral) | Yes | Mathematical and numerical models have been created for an ejector used in anode recirculation within PEMFC. These models take into account factors such as multi-component secondary flow, multiphase phenomena within the ejector, and the connected operational parameters of various fluid inlets. |
| Zhang et al. [73] (2022) | Pure hydrogen | 3D | k-ε (RNG) | 864,497 (Hexahedral) | N/A | CFD technique is employed to analyze the ejector under low power conditions. |
| Bian et al. [74] (2023) | Hydrogen and water vapor | 2D axisymmetric | k-ε (RNG) | 107,400 | N/A | To confirm the ejector performs efficiently across a wide range of conditions, its structure is optimized throughout the entire operational spectrum. |
| Chen et al. [75] (2023) | Hydrogen | 3D | k-ε (RNG) | 1.8 million | N/A | The findings of the simulation indicate that, in comparison to traditional hydrogen recirculation systems, the system incorporating a separator-ejector integrated device reduces condensation and pressure drop within the tube. This enhancement boosts hydrogen recirculation efficiency and allows the PEMFC stack to operate effectively over a broader power output range. |
| Chen et al. [37] (2023) | Hydrogen | 3D axisymmetric | k-ω (SST) | 510,000 | Yes | The outcomes of the CFD simulation of the optimum configuration of the ejector are compared with the experimental results. |
| Ding et al. [76] (2023) | Hydrogen and water vapor | 2D axisymmetric | k-ω (SST) | 74,495 | N/A | A model of two-phase flow that incorporates non-equilibrium phase change conservation equations and four types of entropy transport equations is assessed. |
| Huang et al. [42] (2023) | Hydrogen | 3D | k-ε | 1,300,791 | Yes | Simulations are conducted to assess the distributions of temperature, pressure, velocity, and Mach number inside the ejector for various boundary conditions at the inlet of primary flow. |
| Kuo et al. [77] (2023) | Hydrogen | 3D | k-ε (Standard) | 436 × 106 | Yes | The influence of Mach number, temperature, and pressure is assessed by means of CFD simulation for different ejectors. |
| Ping et al. [78] (2023) | Methane | 2D axisymmetric | k-ε (RNG) | 51,694 | Yes | The performance of the ejector is investigated by means of varying boundary conditions at the inlet. |
| Yin et al. [79] (2023) | Hydrogen | 3D | k-ω (SST) | 1.78 million | Yes | The results of CFD simulation are validated by means of experimental tests. |
| Zhang et al. [80] (2023) | Hydrogen | 3D | k-ε (RNG) | 2,469,995 | N/A | The water separator is integrated with a conventional ejector to perform both functionalities in a single component. |
| Huang et al. [43] (2024) | Nitrogen | 2D axisymmetric | k-ε (RNG) | N/A | N/A | The ejector is optimized with two various shape optimization processes, and then 2D modeling is conducted to assess the performance of the ejector. |
| Singer et al. [21] (2024) | Hydrogen | 2D axisymmetric | k-ε (RNG) k-ω (SST) RSM | 330,000 | Yes | An optimization algorithm is used to maximize the entrainment ratio. |
| Yu et al. [82] (2024) | Hydrogen | 3D | LES | N/A | Yes | The physical parameters of a coaxial-nozzle ejector are investigated by means of LES as the turbulence model. |
| Yu et al. [35] (2024) | Hydrogen | 2D axisymmetric | k-ε (RNG) | 100,208 | N/A | The effects of geometry-based factors of an ejector on its performance and output power are investigated. |
| Li et al. [28] (2024) | Hydrogen | 2D axisymmetric | k-ω (SST) | 374,000 | Yes | Both experimental tests, together with simulations, are conducted to compare the characteristics of various ejectors. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Arabbeiki, M.; Mansourkiaei, M.; Ferrero, D.; Santarelli, M. Ejectors in Hydrogen Recirculation for PEMFC-Based Systems: A Comprehensive Review of Design, Operation, and Numerical Simulations. Energies 2024, 17, 4815. https://doi.org/10.3390/en17194815
Arabbeiki M, Mansourkiaei M, Ferrero D, Santarelli M. Ejectors in Hydrogen Recirculation for PEMFC-Based Systems: A Comprehensive Review of Design, Operation, and Numerical Simulations. Energies. 2024; 17(19):4815. https://doi.org/10.3390/en17194815
Chicago/Turabian StyleArabbeiki, Masoud, Mohsen Mansourkiaei, Domenico Ferrero, and Massimo Santarelli. 2024. "Ejectors in Hydrogen Recirculation for PEMFC-Based Systems: A Comprehensive Review of Design, Operation, and Numerical Simulations" Energies 17, no. 19: 4815. https://doi.org/10.3390/en17194815
APA StyleArabbeiki, M., Mansourkiaei, M., Ferrero, D., & Santarelli, M. (2024). Ejectors in Hydrogen Recirculation for PEMFC-Based Systems: A Comprehensive Review of Design, Operation, and Numerical Simulations. Energies, 17(19), 4815. https://doi.org/10.3390/en17194815








