Hydrogen, E-Fuels, Biofuels: What Is the Most Viable Alternative to Diesel for Heavy-Duty Internal Combustion Engine Vehicles?
Abstract
1. Introduction
1.1. Regulatory Framework
1.2. State of the Art: Power Train Concept Comparison
1.3. Scope of the Paper
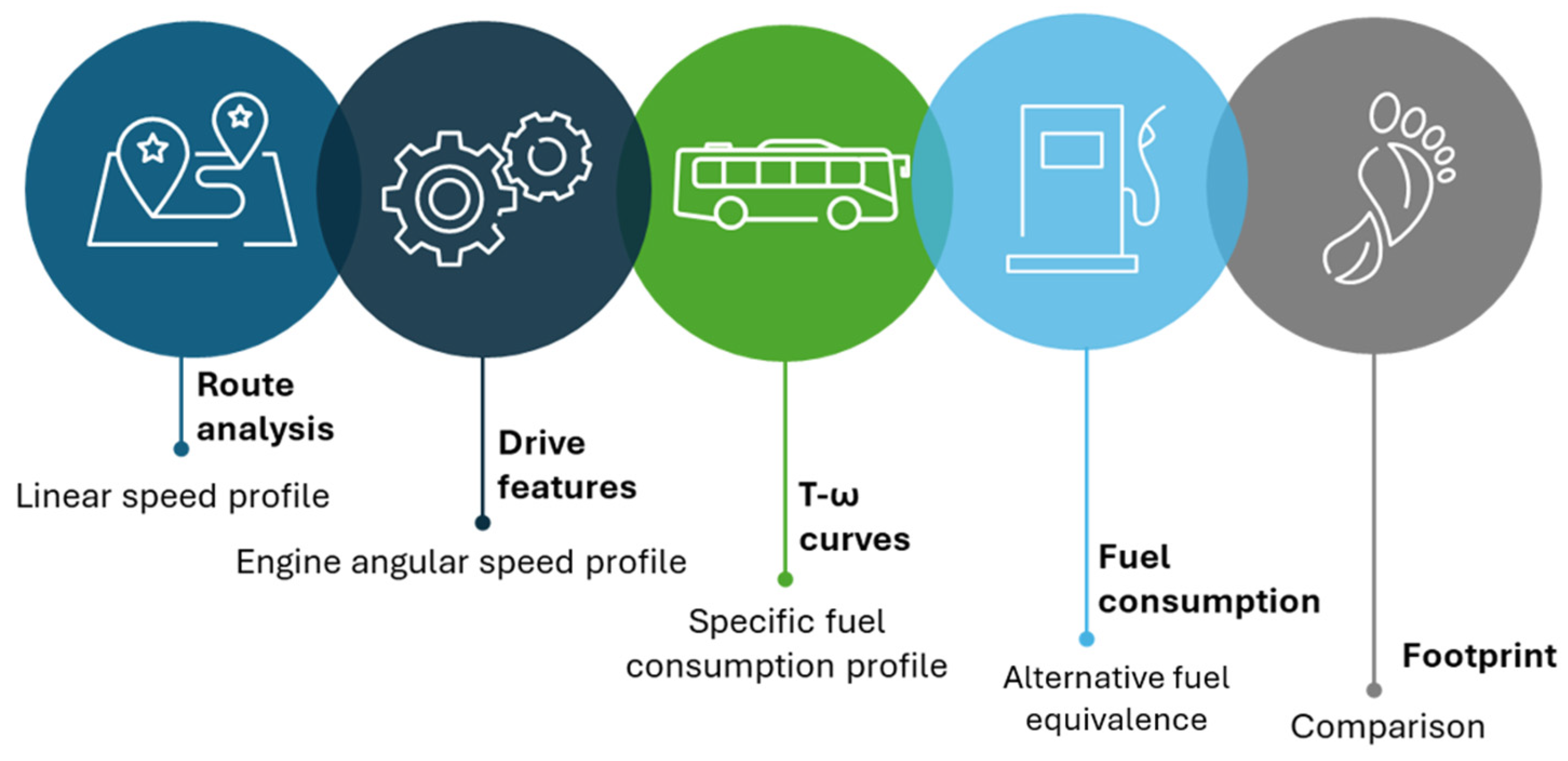
2. Model and Assessment Methodology
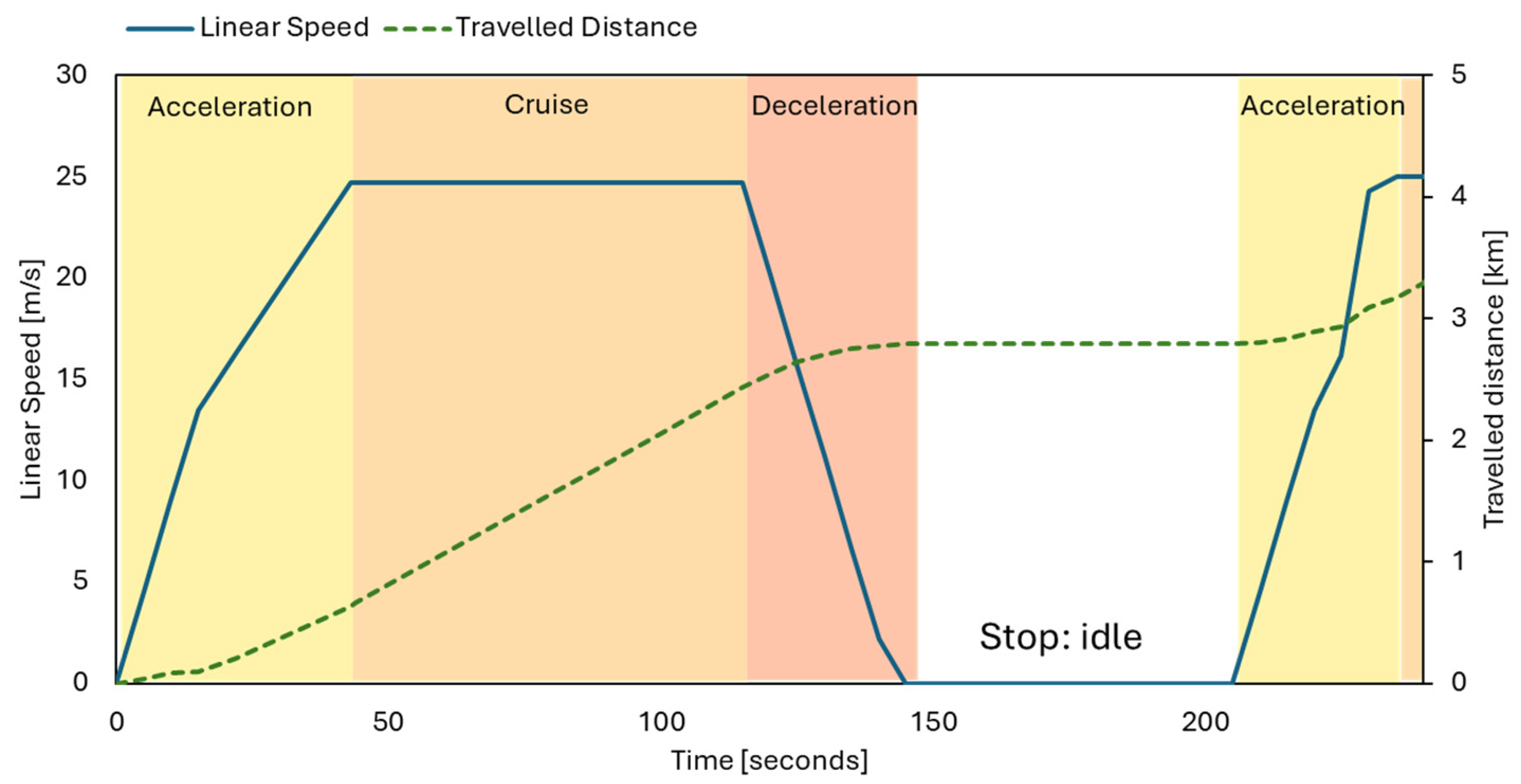
2.1. Mission Load Profile: Route Analysis
2.2. Mission Load Profile: Drive Features
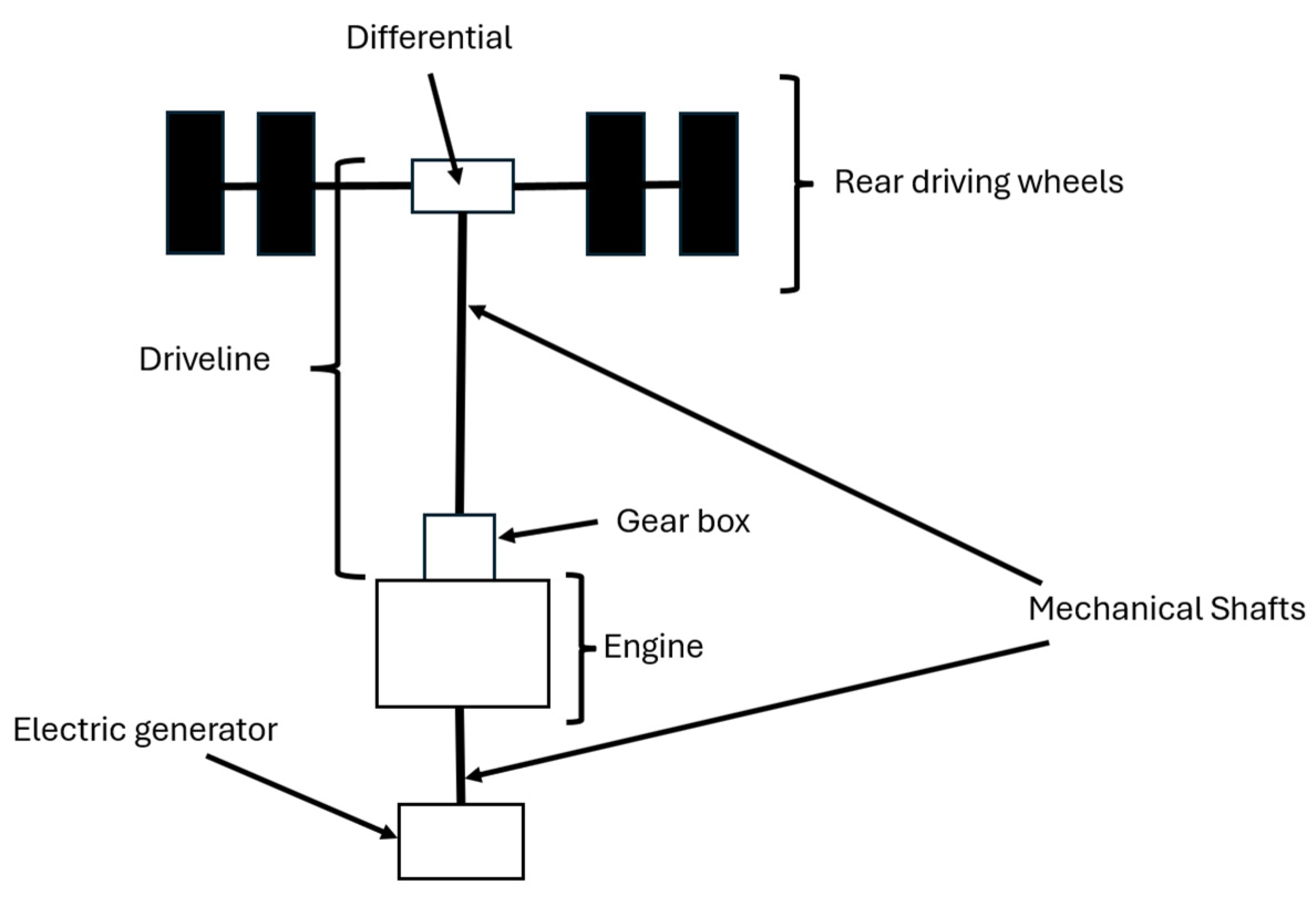
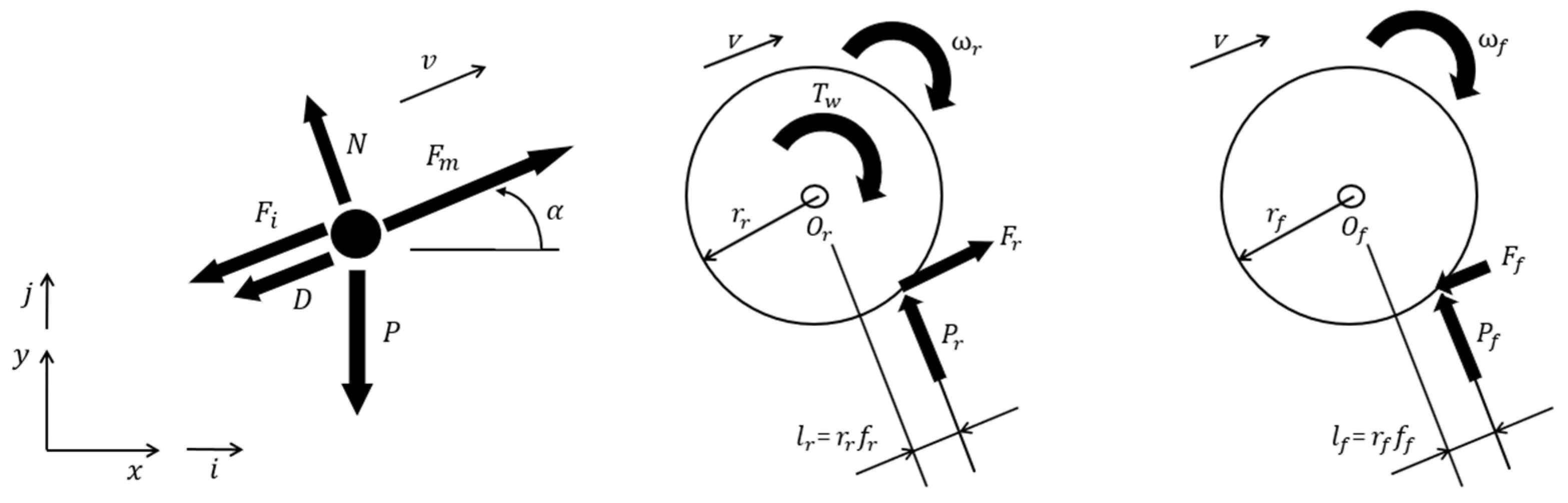
2.3. Dynamic Coach Model: Torque–Velocity Correlation
2.4. Primary Energy Consumption: Calculation and Comparison Metrics
2.5. Carbon Footprint: Calculation and Comparison Metrics
3. Case Study
3.1. Route
3.2. Coach Engines and Fuel Properties
3.3. Design of Simulation Cases
| Case | Fuels | LHV MJ/kg | gCO2eq/MJfuel | gCO2eq/MJfuel | MJWtT/MJTtW |
|---|---|---|---|---|---|
| 0 | Fossil Diesel B0 | 42.7 | 69.3 | 76.7 | 0.28 |
| 1 | Syn. Diesel (e-fuel) | 44.0 | −42.4 | 74.1 | 1.57 |
| 2 | Syn. Diesel (FT Wood gas+CCS) | 44.0 | −133.3 | 74.1 | 1.33 |
| 3 | FAME (Rapeseed) | 37.1 | −24.6 | 79.6 | 1.13 |
| 4 | HVO (Waste cooking oil) | 44.4 | −355.6 | 74.1 | 0.17 |
| 5 | Hydrogen (electrolysis, EU electricity mix) | 120.0 | 35.7 | 0 | 0.35 |
4. Results and Discussion
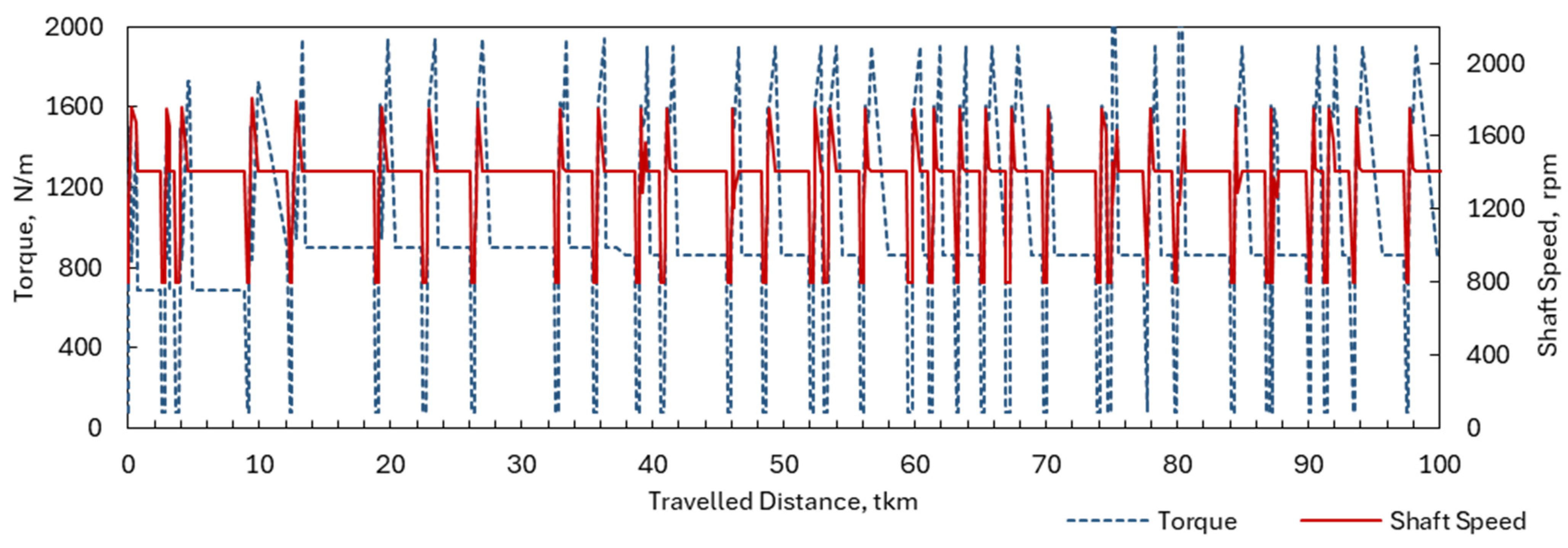
4.1. Travel Mission Simulation in the Base Case: Results and Validation
4.2. Direct Fuel Consumption
4.3. Well-to-Wheel Assessments
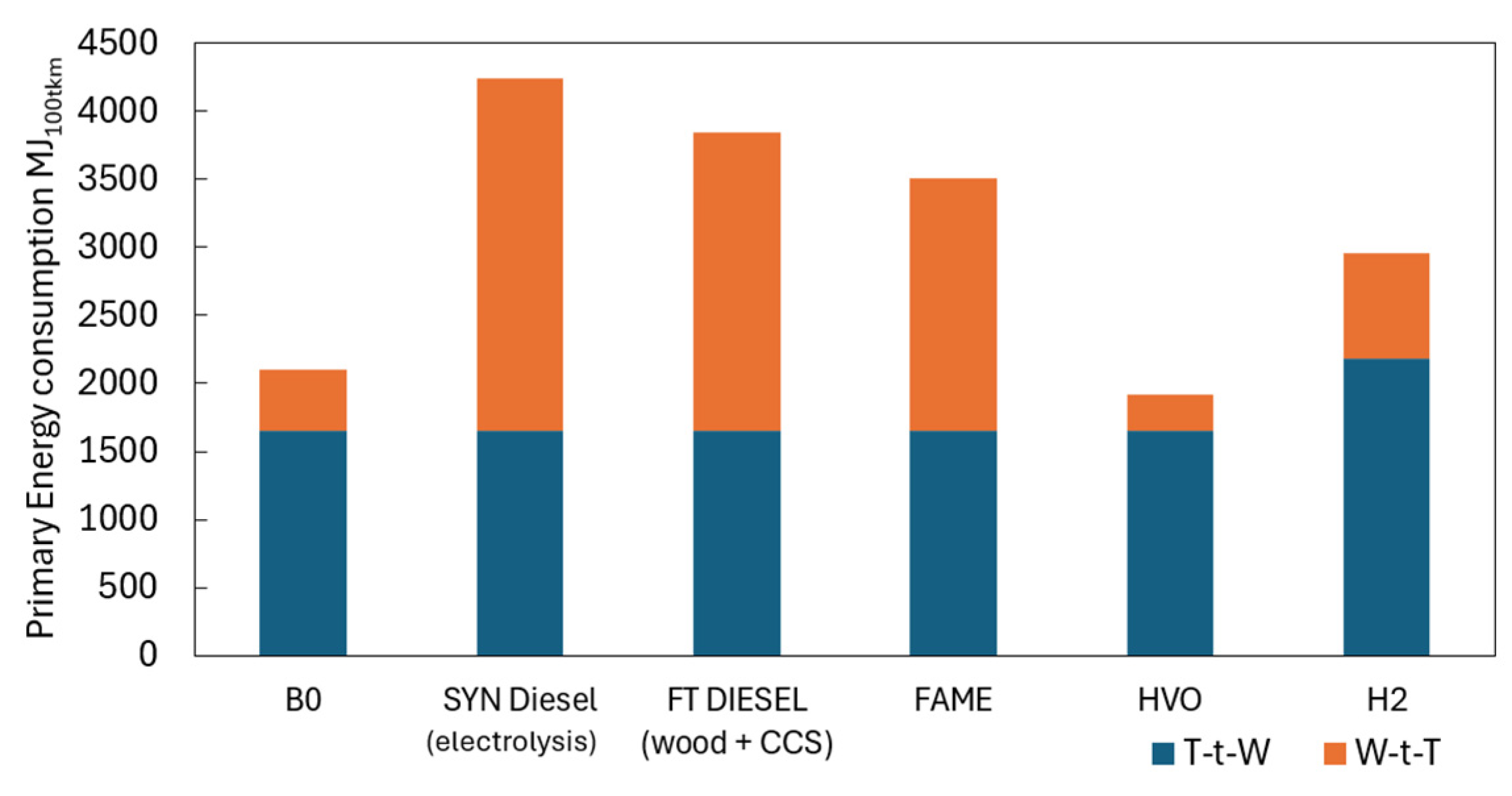
4.3.1. Primary Energy
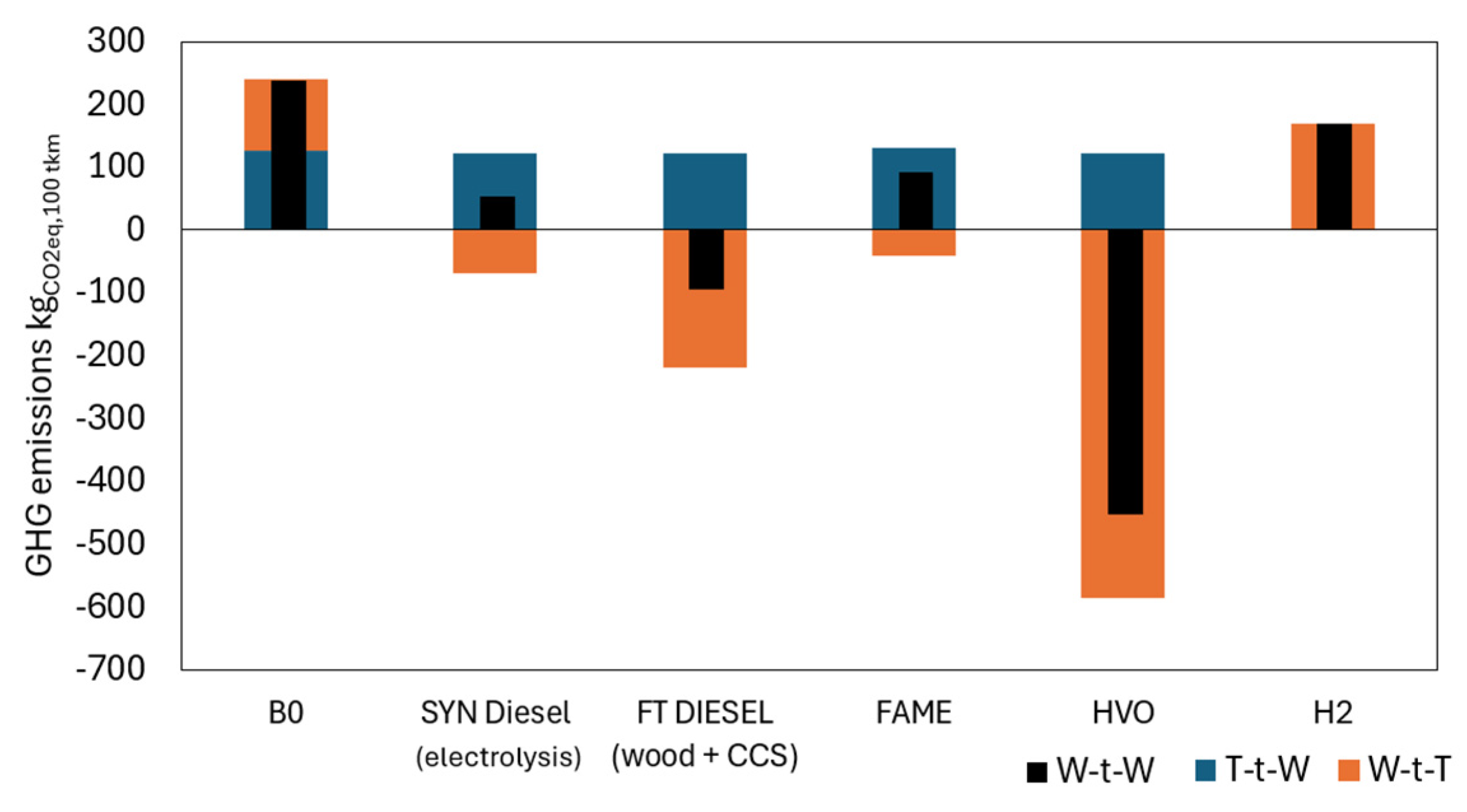
4.3.2. GHG Emissions Assessment
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| BEV | Battery electric vehicle |
| CCS | Carbon capture and storage |
| DI | Direct injection |
| EU | Europe |
| FAME | Fatty Acid Methyl Ester |
| FC | Fuel cell |
| FCEV | Fuel cell electric vehicle |
| FT | Fisher Tropsch |
| GHG | Green House Gas |
| HVO | Hydrotreated Vegetable Oil |
| ICE | Internal combustion engine |
| LHV | Low Heating Value |
| PEM | Polymer Electrolyte Membrane |
| RED | Renewable Energy Directive |
| RFNBO | Renewable Fuel of Non-Biologic Origin |
| TKM | traveled kilometer |
| T-t-W | Tank-to-Wheel |
| W-t-T | Well-to-Tank |
| W-t-W | Well-to-Wheel |
Appendix A
References
- Pyza, D.; Gołda, P.; Sendek-Matysiak, E. Use of hydrogen in public transport systems. J. Clean. Prod. 2022, 335. [Google Scholar] [CrossRef]
- Hren, R.; Vujanović, A.; Van Fan, Y.; Klemeš, J.J.; Krajnc, D.; Čuček, L. Hydrogen production, storage and transport for renewable energy and chemicals: An environmental footprint assessment. Renew. Sustain. Energy Rev. 2023, 173. [Google Scholar] [CrossRef]
- Andaloro, L.; Napoli, G.; Micari, S.; Dispenza, G.; Sergi, F.; Fragiacomo, P.; Antonucci, V. Experimental activities on a PEFC based powertrain for a hybrid electric minibus. Int. J. Hydrogen Energy 2020, 45, 34011–34023. [Google Scholar] [CrossRef]
- Rinscheid, A.; Rosenbloom, D.; Markard, J.; Turnheim, B. From terminating to transforming: The role of phase-out in sustainability transitions. Environ. Innov. Soc. Transitions 2021, 41, 27–31. [Google Scholar] [CrossRef]
- International Energy Agency. Global Hydrogen Review 2022; International Energy Agency: Paris, France, 2022. [Google Scholar]
- Genovese, M.; Cigolotti, V.; Jannelli, E.; Fragiacomo, P. Hydrogen Refueling Process: Theory, Modeling, and In-Force Applications. Energies 2023, 16, 2890. [Google Scholar] [CrossRef]
- Shinde, B.J.; Karunamurthy, K. Recent progress in hydrogen fuelled internal combustion engine (H2ICE)—A comprehensive outlook. Mater. Today Proc. 2022, 51, 1568–1579. [Google Scholar] [CrossRef]
- European Commission. Directive (EU) 2023/2413 of the European Parliament and of the Council; European Commission: Brussels, Belgium, 2023. [Google Scholar]
- International Energy Agency. Energy Statistics Data Browser; International Energy Agency: Paris, France, 2023. [Google Scholar]
- Georgescu, R.; Pana, C.; Negurescu, N.; Cernat, A.; Nutu, C.; Sandu, C. Theoretical and experimental research on the use of hydrogen in the automotive spark ignition engine. IOP Conf. Ser. Mater. Sci. Eng. 2023, 1290, 012010. [Google Scholar] [CrossRef]
- Ugurlu, A. An emission analysis study of hydrogen powered vehicles. Int. J. Hydrogen Energy 2020, 45, 26522–26535. [Google Scholar] [CrossRef]
- Yip, H.L.; Srna, A.; Yuen, A.C.Y.; Kook, S.; Taylor, R.A.; Yeoh, G.H.; Medwell, P.R.; Chan, Q.N. A Review of Hydrogen Direct Injection for Internal Combustion Engines: Towards Carbon-Free Combustion. Appl. Sci. 2019, 9, 4842. [Google Scholar] [CrossRef]
- Luo, Y.; Zhao, C. An Overview of Pre-ignition of Hydrogen Engine. J. Sci. Res. Rep. 2020, 26, 1–7. [Google Scholar] [CrossRef]
- Guo, H.; Zhou, S.; Zou, J.; Shreka, M. A Numerical Investigation on De-NOx Technology and Abnormal Combustion Control for a Hydrogen Engine with EGR System. Processes 2020, 8, 1178. [Google Scholar] [CrossRef]
- Wróbel, K.; Wróbel, J.; Tokarz, W.; Lach, J.; Podsadni, K.; Czerwiński, A. Hydrogen Internal Combustion Engine Vehicles: A Review. Energies 2022, 15, 8937. [Google Scholar] [CrossRef]
- Moriconi, N.; Laranci, P.; D’Amico, M.; Bartocci, P.; D’Alessandro, B.; Cinti, G.; Baldinelli, A.; Discepoli, G.; Bidini, G.; Desideri, U.; et al. Design and preliminary operation of a gasification plant for micro-CHP with internal combustion engine and SOFC. Energy Procedia 2015, 81, 298–308. [Google Scholar] [CrossRef]
- Haug, P.; Koj, M.; Turek, T. Influence of process conditions on gas purity in alkaline water electrolysis. Int. J. Hydrogen Energy 2017, 42, 9406–9418. [Google Scholar] [CrossRef]
- Taner, T. The novel and innovative design with using H2 fuel of PEM fuel cell: Efficiency of thermodynamic analyze. Fuel 2021, 302, 121109. [Google Scholar] [CrossRef]
- Du, Z.; Liu, C.; Zhai, J.; Guo, X.; Xiong, Y.; Su, W.; He, G. A Review of Hydrogen Purification Technologies for Fuel Cell Vehicles. Catalysts 2021, 11, 393. [Google Scholar] [CrossRef]
- Shayan, E.; Zare, V.; Mirzaee, I. Hydrogen production from biomass gasification; a theoretical comparison of using different gasification agents. Energy Convers. Manag. 2018, 159, 30–41. [Google Scholar] [CrossRef]
- Han, J.; Kim, H. The reduction and control technology of tar during biomass gasification/pyrolysis: An overview. Renew. Sustain. Energy Rev. 2006, 12, 397–416. [Google Scholar] [CrossRef]
- Persson, M.; Jonsson, O.; Wellinger, A. Biogas Upgrading To Vehicle Fuel Standards and Grid. IEA Bioenergy 2007, 37, 1–32. [Google Scholar]
- Chinatrucks.com. China’s First Hydrogen Internal Combustion Engine Powered Heavy Truck; Chinatrucks: Beijing, China, 2022. [Google Scholar]
- Vuchic, V.R. Urban Transit Systems and Technology; Wiley: Hoboken, NJ, USA, 2007. [Google Scholar] [CrossRef]
- Korn, T. The new highly efficient hydrogen internal combustion engine as ideal powertrain for the heavy-duty sector. In Internationaler Motorenkongress 2019; Springer: Berlin/Heidelberg, Germany, 2019; pp. 385–400. [Google Scholar] [CrossRef]
- Google Earth. Available online: https://www.google.it/intl/it/earth/index.html (accessed on 1 May 2024).
- Bayındırlı, C.; Çelik, M. The Experimentally and Numerically Determination Of The Drag Coefficient Of A Bus Model. Int. J. Automot. Eng. Technol. 2018, 7, 117–123. [Google Scholar] [CrossRef][Green Version]
- ETIP Bioenergy. Fatty Acid Methyl Esters (FAME) Fact Sheet; ETIP Bioenergy: Brussels, Belgium, 2023. [Google Scholar]
- ETIP Bioenergy. Hydrogenated Vegetable Oil (HVO); ETIP Bioenergy: Brussels, Belgium, 2020. [Google Scholar]
- Kulikov, I.; Kozlov, A.; Terenchenko, A.; Karpukhin, K. Comparative Study of Powertrain Hybridization for Heavy-Duty Vehicles Equipped with Diesel and Gas Engines. Energies 2020, 13, 2072. [Google Scholar] [CrossRef]
- Wojcieszyk, M.; Kroyan, Y.; Kaario, O.; Larmi, M. Prediction of heavy-duty engine performance for renewable fuels based on fuel property characteristics. Energy 2023, 285, 129494. [Google Scholar] [CrossRef]
- Gustafsson, M.; Svensson, N.; Eklund, M.; Öberg, J.D.; Vehabovic, A. Well-to-wheel greenhouse gas emissions of heavy-duty transports: Influence of electricity carbon intensity. Transp. Res. Part D Transp. Environ. 2021, 93, 102757. [Google Scholar] [CrossRef]
- Prussi, M.; Yugo, M.; De Prada, L.; Padella, M.; Edwards, R.; Lonza, L. “JEC Well-To-Wheels Report v5” EUR 30284 EN; Publications Office of the European Union: Luxembourg, 2020; ISBN 978-92-76-20109-0. [Google Scholar] [CrossRef]
- Staffolani, A.; Baldinelli, A.; Bidini, G.; Nobili, F.; Barelli, L. Operando Analysis of Losses in Commercial-Sized Solid Oxide Cells: Methodology Development and Validation. Energies 2022, 15, 4978. [Google Scholar] [CrossRef]
- Cinti, G.; Baldinelli, A.; Di Michele, A.; Desideri, U. Integration of Solid Oxide Electrolyzer and Fischer-Tropsch: A sustainable pathway for synthetic fuel. Appl. Energy 2016, 162, 308–320. [Google Scholar] [CrossRef]

| Vehicle Type | Tank-to-Wheel Efficiency | Long-Haul Range | Tank-to-Wheel Emissions | Fuel Purity | Refueling Time | TRL | Outlook |
|---|---|---|---|---|---|---|---|
| ICE (Diesel) | + | ++++ | CO2 NOx PM | Low | Minutes | 9 | Negative |
| ICE (Biofuels) | + | ++++ | CO2 * NOx PM | Low | Minutes | 8 | Positive |
| ICE (Synfuels) | + | ++++ | CO2 ** NOx PM | Low | Minutes | 7 | Positive |
| ICE (H2/Gasoline blend) | ++ | ++++ | CO2/less NOx | Low | Minutes | 9 | Neutral |
| ICE (Hydrogen) | ++ | +++ | Low NOx | Low | Minutes | 6–7 | Positive |
| FCEV (Hydrogen) | +++ | +++ | - | High | Minutes | 8 | Positive |
| BEV | ++++ | + | - | n.d. | Hours | 9 | Positive |
| Speed Range | Acceleration vs. Speed Range | Gear | Gear Maximum Speed |
|---|---|---|---|
| 0 → 16.6 m/s | +0.9 m/s2 | rc1 | 8.5 m/s |
| rc2 | 12.4 m/s | ||
| 16.6 → 27.8 m/s | +0.4 m/s2 | rc3 | 17.7 m/s |
| rc4 | 25.0 m/s | ||
| 27.8 → 0 m/s | −0.9 m/s2 | rc5 | 27.8 m/s |
| Modeling Parameter | Symbol | Case Study Value |
|---|---|---|
| Coach weight (vehicle + passengers) | m | 15,320 |
| Coach front section | A | 6 m2 |
| Drag coefficient | 0.5 | |
| Wheel radius | 521.5 mm | |
| Rolling friction coefficient | 0.015 | |
| Ancillary electrical power | Max: 10 kW, Cruise: 50%max, Stops: 60%max | |
| Ancillary system efficiency | 95% | |
| Driveline efficiency | 91% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Baldinelli, A.; Francesconi, M.; Antonelli, M. Hydrogen, E-Fuels, Biofuels: What Is the Most Viable Alternative to Diesel for Heavy-Duty Internal Combustion Engine Vehicles? Energies 2024, 17, 4728. https://doi.org/10.3390/en17184728
Baldinelli A, Francesconi M, Antonelli M. Hydrogen, E-Fuels, Biofuels: What Is the Most Viable Alternative to Diesel for Heavy-Duty Internal Combustion Engine Vehicles? Energies. 2024; 17(18):4728. https://doi.org/10.3390/en17184728
Chicago/Turabian StyleBaldinelli, Arianna, Marco Francesconi, and Marco Antonelli. 2024. "Hydrogen, E-Fuels, Biofuels: What Is the Most Viable Alternative to Diesel for Heavy-Duty Internal Combustion Engine Vehicles?" Energies 17, no. 18: 4728. https://doi.org/10.3390/en17184728
APA StyleBaldinelli, A., Francesconi, M., & Antonelli, M. (2024). Hydrogen, E-Fuels, Biofuels: What Is the Most Viable Alternative to Diesel for Heavy-Duty Internal Combustion Engine Vehicles? Energies, 17(18), 4728. https://doi.org/10.3390/en17184728








