Next Article in Journal
A Semi-Supervised Approach for Partial Discharge Recognition Combining Graph Convolutional Network and Virtual Adversarial Training
Next Article in Special Issue
Electrical Tree and Partial Discharge Characteristics of Silicone Rubber Under Mechanical Pressure
Previous Article in Journal
An Analysis of the Physicochemical and Energy Parameters of Briquettes Manufactured from Sewage Sludge Mixtures and Selected Organic Additives
Previous Article in Special Issue
Current Measurement of Three-Core Cables via Magnetic Sensors
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Energies
Volume 17
Issue 18
10.3390/en17184576
energies-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Effects of Water Absorption on the Insulating Properties of Polypropylene

by
Rui Xi
1,
Qiyang Jiang
1,
Liang Cao
1,*,
Chuping Li
2,
Jiaxun He
2,
Ya Zhang
2,
Gaohui He
1,
Yingang Gui
1 and
Chao Tang
1,*
1
College of Engineering and Technology, Southwest University, Chongqing 400715, China
2
Chongqing Taishan Cable Co., Ltd., Chongqing 401125, China
*
Authors to whom correspondence should be addressed.
Energies 2024, 17(18), 4576; https://doi.org/10.3390/en17184576
Submission received: 12 July 2024 / Revised: 14 August 2024 / Accepted: 6 September 2024 / Published: 12 September 2024
(This article belongs to the Special Issue Power Cables in Energy Systems)
Browse Figures
Versions Notes

Abstract

:
Moisture has been a crucial problem during the operation of cable systems. When we are faced with polypropylene (PP)-based insulation for the development of cable systems, there are few reports on the effects of water intrusion on the electrical performances of PP. In this study, the water absorption characteristics of isotactic PP (iPP) and atactic PP (aPP), as well as their effects on volume resistivity and relative permittivity, were investigated. The structure evolution during the water absorption process of the two PPs was also compared via infrared spectra and X-ray diffraction analyses. The results show that both of the two PPs show a saturation of water absorption at ~216 h, even though there are structural differences. And water intrusion into bulk could increase the interplanar spacing of iPP while decreasing the interplanar spacing of aPP. Moreover, with the increase in water absorption, the volume resistivity of the two PPs show a decreasing trend while the relative permittivity presents an increasing behavior, which shows an almost linear correlation.

1. Introduction

As a crucial component for transmitting and distributing electrical energy, the safe, reliable, and efficient operation of power cables is essential for maintaining a stable power supply [1,2,3,4]. The electrical properties and quality of insulation materials in cable systems are critical for ensuring the safe and stable operation of power systems [5]. With the advocacy of green, low-carbon, and environmentally friendly sustainable development strategies, the development of environmentally friendly cable insulation materials has become an urgent challenge for the power cable industry [6,7]. Polypropylene (PP) possesses excellent electrical properties, good thermal stability, ease of processing and manufacturing, low production costs, high production efficiency, and recyclability after decommissioning [8,9,10,11]. Therefore, PP represents a promising direction for the development of environmentally friendly cable insulation materials, addressing several environmental drawbacks associated with cross-linked polyethylene (XLPE). Firstly, manufacturing XLPE-insulated cables involves complex, time-consuming, and energy-intensive processes, including crosslinking and degassing. Secondly, XLPE is a thermosetting insulation material that cannot be directly recycled after decommissioning; thus, landfill, thermal, incineration, and catalytic pyrolysis are the current treatment methods for retired XLPE insulation, and these pose significant environmental hazards [12]. Therefore, due to the above factors, PP insulation materials have garnered significant attention from both academia and the power cable industry in recent years [13,14].
Currently, power cables in service are experiencing frequent accidents, with insulation moisture being the most susceptible defect that can trigger these occurrences [15]. Cable bodies and accessories are often installed in cable trenches, conduits, or tunnels, and due to weather conditions or other factors, these areas tend to accumulate significant amounts of water. As a result, cables and their intermediate accessories can become submerged, ultimately leading to insulation moisture defects [16]. It is reported that without considering external damage, most cable failures are attributed to insulation moisture [17]. Li and Liang et al. analyzed the variation in insulation parameters of the cable main insulation of XLPE and the cable accessory-reinforced insulation of silicone rubber under different moisture conditions were studied experimentally. Furthermore, the effects of typical moisture defect forms and moisture positions on electric field distributions have been studied [18]. Li and Zhao et al. analyzed the influence of the accelerated water treeing test on the properties of 10 kV XLPE cable insulating materials. The dielectric and physicochemical properties of both aged and unaged samples were tested [19]. When insulation becomes damp, it reduces the insulating properties and current-carrying capacity of the cable. This can lead to uneven electric field distribution, resulting in partial discharge or tree discharge, which can ultimately cause insulation failure [20]. With environmentally friendly PP-insulated power cables emerging as the new mainstream, the issue of water intrusion in PP cables remains a crucial research direction in the power industry. However, the majority of current studies are focused on optimizing and modifying PP materials and their engineering trials [21,22,23], leaving a gap in the research regarding the evolution mechanism of dielectric properties in PP-based insulated cable systems under the threat of water intrusion.
Therefore, this study focuses on two types of PPs, including isothermal polypropylene (iPP) and atactic polypropylene (aPP). Initially, a comparative analysis is conducted to examine the differences in molecular structure, free volume, and crystal structure between the two PPs before a water immersion test. Next, the weight variation of the PPs is measured at different immersion times to analyze their water absorption properties. Subsequently, the structural changes in the PPs under the water immersion are investigated by Fourier transform infrared (FTIR) spectroscopy and X-ray diffraction (XRD) analysis. Finally, the variations in volume resistivity and relative permittivity of PPs with different frequencies at different immersion time are acquired. And the relationship between the dielectric properties of PPs and water absorption process is further discussed.

2. Materials and Methods

2.1. Sample Preparation

Commercially available iPP and aPP were selected. The iPP shows an isotactic content of 98%, with a density of ~0.91 g/cm3 (23 °C) and a melt index of 3.0 g/10 min (230 °C/2.16 kg). And aPP has a density of ~0.90 g/cm3 (23 °C) and a melt index of 2.5 g/10 min (230 °C/2.16 kg). Details of the PPs are listed in Table 1.
Pellets of PPs were first dried in an oven at 70 °C for 24 h. And then plate samples with a dimension of 10 cm × 10 cm × 0.1 cm were prepared by a vulcanizer. The preparation process involved placing a mold containing the appropriate amount of raw pellets onto the vulcanizing machine, preheating it to 190 °C for 1 min, and conducting 3~5 exhaust cycles. The pressure was then increased to 15 MPa, and after 15 min of heating, the mold was removed. Once it was cooled to room temperature, the PP film specimen was extracted and its surface was wiped with anhydrous ethanol. Before tests, all the plate samples were pre-treated at 70 °C for 48 h to remove internal moisture and to relieve residual stress.

2.2. Water Immersion Test

The plate samples were totally immersed in a water tank containing deionized water. The upper edge of each specimen was 20 cm below the water surface, and each side of every specimen was kept in full contact with the water. During the whole immersion process, the temperature was controlled at 30 °C. The water soaking process of samples lasted for 216 h [24].

2.3. Water Absorption Rate Measurement

The mass variation of plate samples in the immersing process was evaluated by a precision balance with a resolution of 0.01 mg. Samples were taken out and weighted on a timed interval. Before weighing, residual water on the surface of samples was removed with bibulous paper. The water absorption rate is defined as follows:
χt = (Wt − W0)/W0 × 100%,
where χt is the water absorption rate at the immersion time of t, Wt is the weight of the sample at the immersion time of t, and W0 is the original weight of the sample in dry conditions.
The average value of three measurements was recorded. After weighing, samples were immersed in water again [25].

2.4. FTIR Spectroscopic Analysis

FTIR spectra (Spectrum Two, PerkinElmer, Waltham, MA, USA) were used to compare changes in chemical bonds before and after water absorption of PPs. After residual water on the sample surface was removed, the wavenumber of samples ranged from 525 cm−1 to 4000 cm−1 and a resolution of 4 cm−1 in the transmission mode was recorded. A total of 32 scans was conducted.

2.5. XRD Analysis

XRD analysis (D2 Phaser, Bruker AXS GmbH, Karlsruhe, Germany) of samples was employed to determine the effect of water absorption on the crystalline structure of PPs. A copper target was used, generating an X-ray at a wavelength of 0.15406 nm. When a voltage of 36 kV and a current of 20 mA were applied, the data were scanned from 10° to 60° at a rate of 5° per minute.

2.6. Differential Scanning Calorimerty (DSC) Analysis

The melting and crystallization behaviors of two PPs were analyzed by using a DSC (DSC1, Mettler, Zürich, Switzerland). The samples of 5 mg were heated in a nitrogen atmosphere from 30 °C to 200 °C at a heating rate of 10 °C/min and then maintained at 200 °C for 3 min. They were then cooled back to 30 °C at a cooling rate of 10 °C/min. Thermograms were recorded during the heating and cooling processes. The crystallinity (XC) of PP was calculated by the following equation:
XC = ΔHm/ΔH100 × 100%,
where ΔHm was the measured heat of fusion from the melting curve for two PPs, and ΔH100 = 209 J/g was adopted as the melting enthalpy of fully crystalline PP [26,27].

2.7. Positron Annihilation Lifetime Spectrum (PALS) Analysis

PLAS (PLS2-SYSTEMTK, ORTEC, Atlanta, GA, USA) was measured using a fast–fast coincidence device with a spectrometer resolution of ~290 ps. The radioactive activity of the positron source 22Na was approximately 30 μCi, and the counts for each spectrum were greater than 1.5 × 106. The spectral fitting was performed using a three-lifetime model. In the positron annihilation of polymers, based on the infinite deep spherical potential well model of free volume, the relationship between the third lifetime τ3 and the radius R of the free volume is as follows.
τ3−1 = 2{1 − R/(R + ΔR) + 1/2π sin [2πR/(R + ΔR)]},
where ΔR is 0.166 nm for polymers and free volume VF and corresponding fraction FV of the sample can be illustrated by the following equations [28,29,30].
VF = 4πR3/3,
FV = CI3VF,
where C is a constant, which is equal to 0.0018 nm−3, and I3 is the intensity of the third lifetime τ3 in %.

2.8. Volume Resistivity Measurement

A three-electrode system (ZC36, Shanghai Sixth Electric Meter Factory Co., Ltd., Shanghai, China) was used to investigate the volume resistivity of the two PPs. The environment temperature remained at 30 °C. Direct-current voltage at 1 kV was applied to samples with different water immersion times. The current values at 15 min were obtained. When the applied voltage and specimen thickness were acquired, the volume resistivity was calculated and the average value of three samples was taken.

2.9. Relative Permittivity Analysis

To reveal the influence of water content on the relative permittivity of PPs, an LCR (LCR 6365, SAIMR, Suzhou, China) analyzer was used to measure the variation of capacitance Cp for PPs with increasing immersion time under various frequencies, including 1 Hz, 50 Hz, 1 kHz, and 10 kHz. With the influence of edge effects being neglected, the relative permittivity εr of plate samples was then calculated based on the following equation:
εr = Cp·t/(ε0·S),
where Cp is the value of capacity in F; t is the thickness of the sample in m; ε0 is the permittivity of the vacuum, which is 8.85 × 10−12 F/m; and S is the area of electrodes in m2.

3. Results

3.1. Structure Differences between iPP and aPP

The differences in chemical groups, free volume and crystal structures between iPP and aPP when dry are shown in Figure 1. Figure 1a presents the FTIR spectra of the iPP and aPP, both revealing peaks at 2953 cm−1, 2917 cm−1, 2866 cm−1, 2839 cm−1, 1459 cm−1, and 1375 cm−1. The peaks at 2953 cm−1 and 2917 cm−1 correspond to asymmetric stretching of –CH3 and –CH2–, respectively. And the peaks at 2866 cm−1 and 2839 cm−1 represent symmetric stretching motions of –CH3 and –CH2–. The peaks at 1459 cm−1 and 1375 cm−1 are associated with bending vibrations and symmetric deformation vibrations of –CH3 and –CH2–. The peak locations are consistent with the results of Zheng et al. [31] and Shen et al. [32]. Obviously, there are no significant differences in the positions of functional groups between iPP and aPP samples.
As depicted in Figure 1b, the free volume characteristics of iPP and aPP are different. It can be observed that the τ3 of iPP is higher than that of aPP, and the free volume radius R for iPP and aPP is 0.614 and 0.598, respectively. This indicates that the average pore diameter of iPP is larger than that of aPP. Additionally, the free volume fraction of iPP is 0.042, which is slightly higher than that of aPP.
From the XRD patterns of iPP and aPP shown in Figure 1c, it can be seen that, for iPP, there are obvious peaks at 2θ = 14.3°, 17.2°, 18.9°, 21.3° and 22.1°, which correspond to the plane diffraction of the α-crystal form for PP at (110), (040), (130), (131) and (041), thus indicating that the main crystal type of the iPP is α-crystal. For aPP, there are peaks at 2θ = 14.0°, 16.8°, 18.6°, 20.1°, 21.2°, and 21.9°, where five peaks have the same location corresponding to the peaks of the iPP and one additional peak at 2θ = 20.1° corresponds to the plane diffraction of γ-crystal form for PP at (117). This phenomenon indicates that the crystal type of the aPP is a mixture of α-crystal and γ-crystal forms.
The melting curves of the two PPs are shown in Figure 1d. The melting peak of iPP is a single peak with a sharp shape, and the melting peak temperature Tm is 165.29 °C. The calculated crystallinity degree of iPP is about 55.19%. The melting peak range of aPP is wider than that of iPP. Except for a sharp melting peak located in the vicinity of 149.39 °C, there is also a broad peak partially overlapping with it near 137 °C, and its degree of crystallinity is 44.05%, which is less than that of iPP. The difference in crystallinity degree for the two PPs is due to the fact that iPP has a higher tendency to crystallize because of its more regular chain structure. The double peaks shown in the melting process of aPP are probably due to the fact that there are α and γ crystal forms in aPP, as illustrated by the XRD results. The existence of different crystal types in PP will lead to the generation of melting double peaks [33].

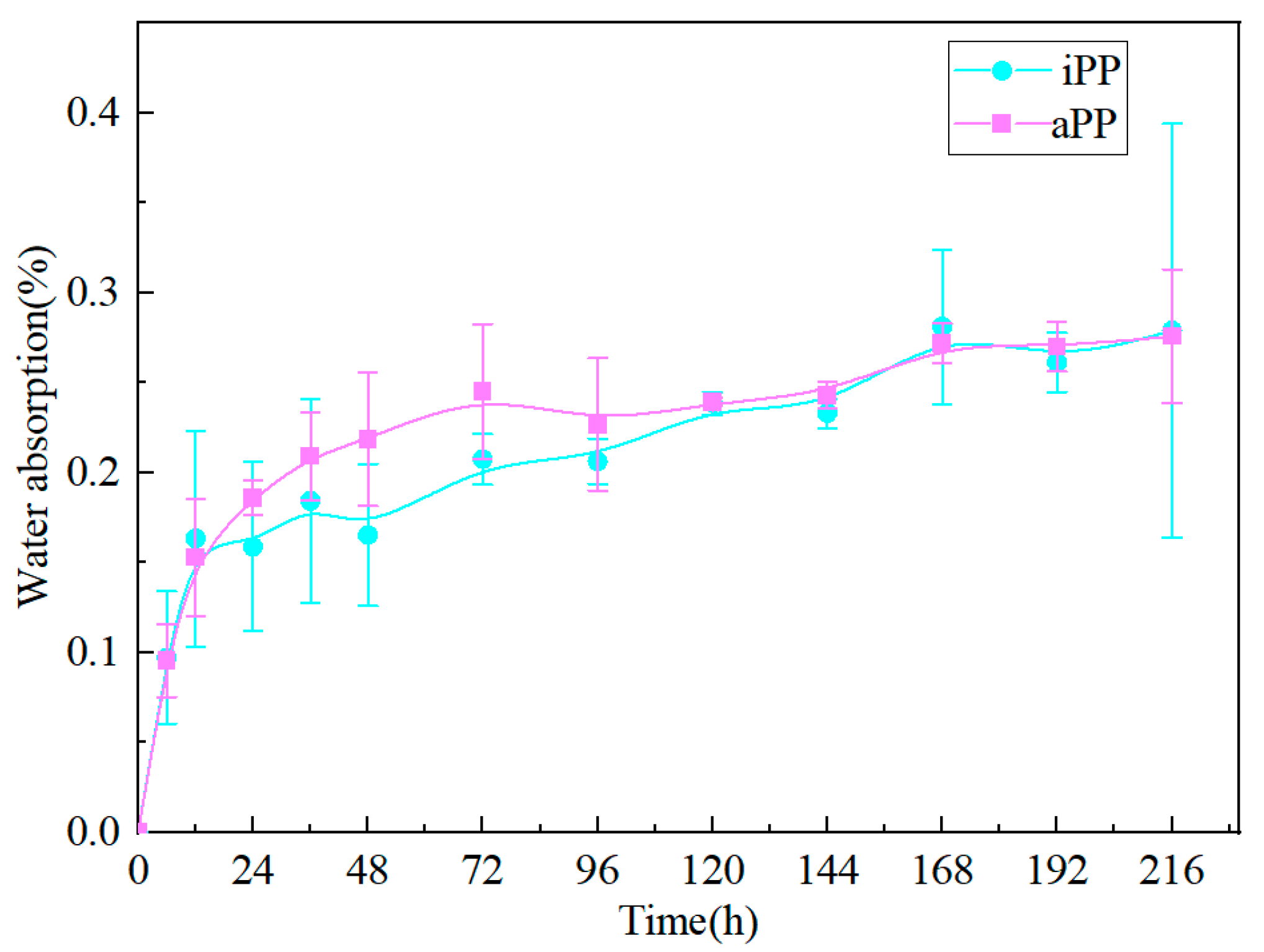
3.2. Variation of Water Absorpiton with Time for PPs

The relationship between the water absorption rates of PPs and the water immersion time is shown in Figure 2. It can be seen that the water absorption rates of PPs show an upward trend. Simultaneously, by comparing the water absorption rate of iPP and aPP, it is observed that during the early 12 h of water immersion, the water absorption rate of iPP is slightly higher than that of aPP. The reason for this phenomenon may be due to the larger free volume of iPP compared to aPP.
However, in the immersion time range of 12 h to 120 h, the water absorption rate of aPP was higher than that of iPP, and the gap between them first increased and then decreased. At the immersion time of 48 h, the difference in water absorption rates between the two samples reached its maximum, at a value of 0.04%. From 120 h to 168 h, the water absorption rates of both PPs showed a slow upward trend, with comparable increases. This growth trend may be attributed to both samples approaching their water absorption saturation states, resulting in slower growth rates for the two PPs. From 168 h to 216 h, the growth in water absorption rates of both samples was relatively flat, indicating that the two PPs were approaching a saturated state with a water absorption rate of approximately 0.28%. At the immersion time of 216 h, it can be observed that the water absorption rate of iPP is slightly higher than that of aPP, which may be attributed to its relatively larger free volume and higher free volume fraction.

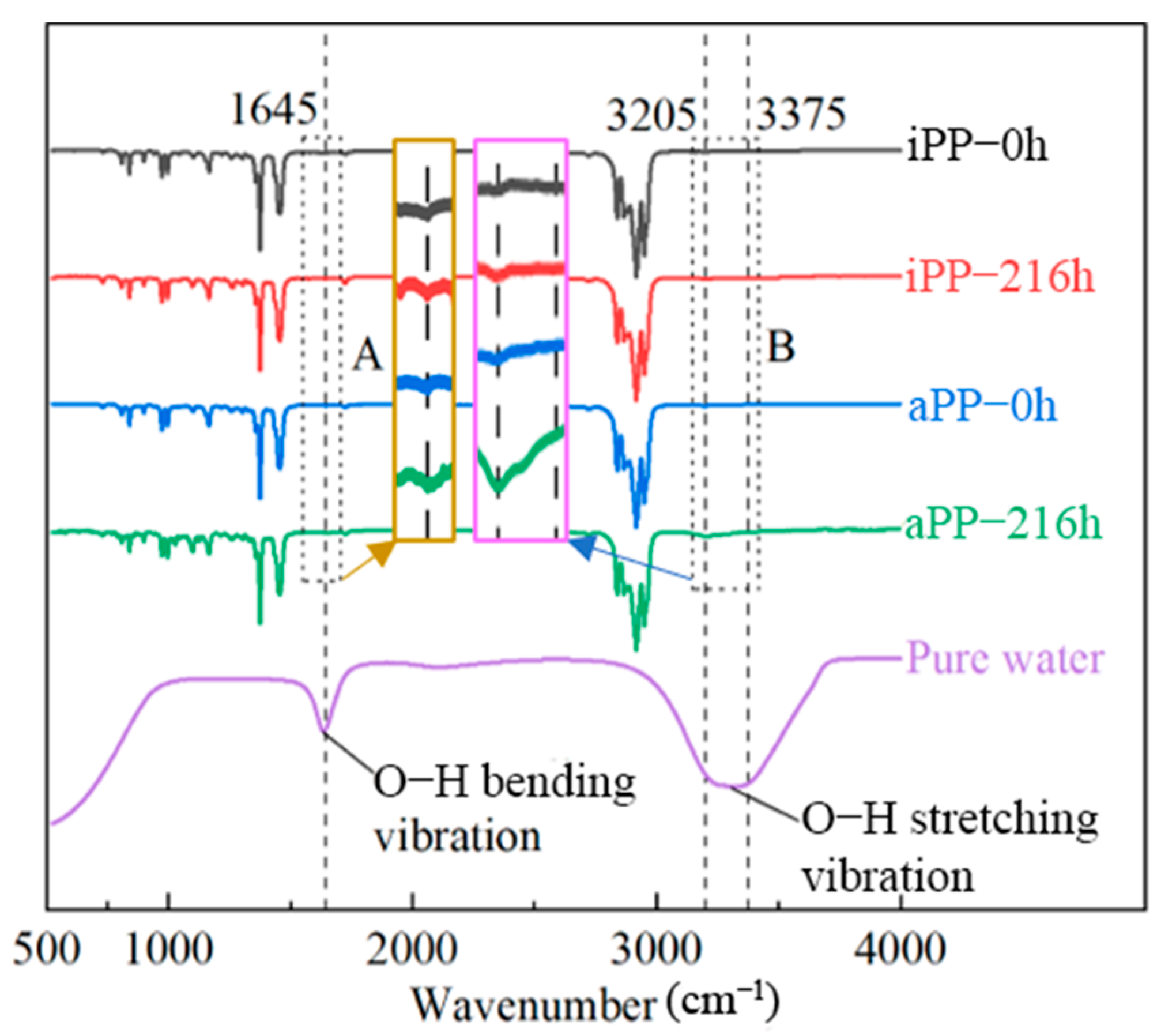
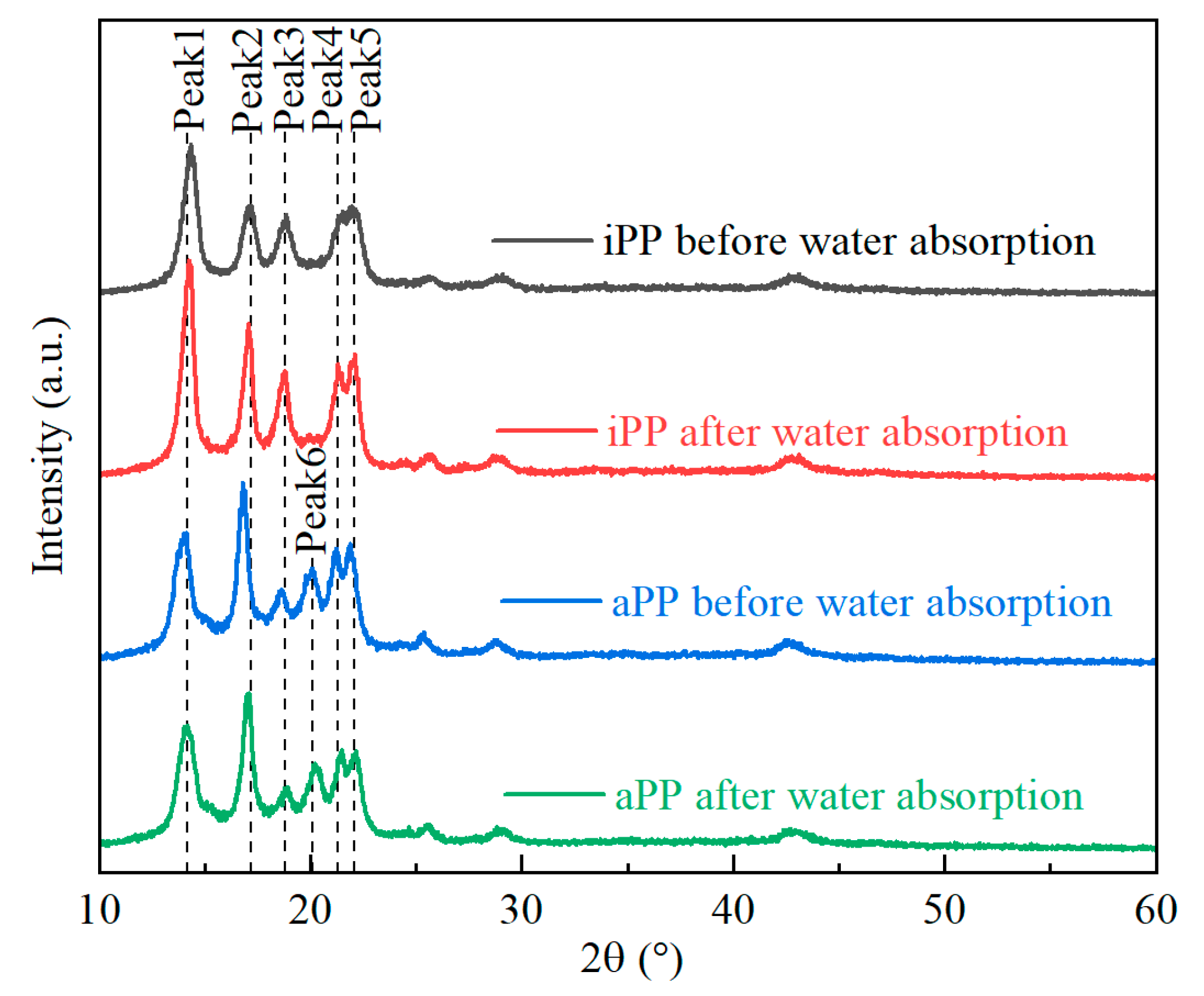
3.3. Structure Changes before and after Water Absorption of PPs

From the FTIR spectra given in Figure 3, comparing differences in chemical groups between PPs in their dry and water-saturated states, it can be observed that for the pure water, the peak at 1645 cm−1 and the broad peak ranging from 3205 cm−1 to 3375 cm−1 correspond to the stretching vibration peak and bending vibration peak of the O–H bond, respectively. For aPP, the peak at 3205 cm−1 in the water-saturated state increased significantly, indicating that the content of water in the bulk of iPP increases after water immersion. In the water-saturated state, the transmittance difference between iPP and aPP at 3205 cm−1 before and after water absorption was 2.68% and 3.98%. Therefore, the peak that appeared at 3205 cm−1 for iPP was not as significant for aPP. From the equivalent water absorption rate and the reduction in absorption intensity, it could be inferred that the O–H bond stretching vibration is suppressed in iPP, and further investigation is needed to determine how this occurs.
Further analysis of the XRD patterns for the two PPs before and after water absorption in Figure 4 reveals that no new peaks appeared, nor did the original peaks disappear after water absorption for PPs, which shows that the water intrusion process has little influence on crystal forms of PP and water itself has minimal impact on the crystal forms of the PP.
In order to study differences in the crystalline structure between the two PPs before and after water absorption, the interplanar spacing of samples is calculated based on the Bragg’s equation.
2d·sinθ = n·λ,
where d is interplanar spacing in Å; θ is the incident angle in °; n is the series of reflection; λ is the wavelength of the incident X-rays, 1.54056 Å. The calculated results are shown in Table 2. Before water absorption, interplanar spacing d of a single α-crystal for iPP are smaller, while interplanar spacing d of the α-γ mixed crystal for aPP is larger. After water absorption, as presented in Table 2, the peaks of the iPP shift to the direction of angle increment after water absorption, while the peaks of the aPP shift to the direction of angle reduction. Changes in the angle θ reveal the changes in interplanar spacing d, and the phenomenon above indicates that after water absorption, the interplanar spacing of iPP increases, while the interplanar spacing of aPP decreases.

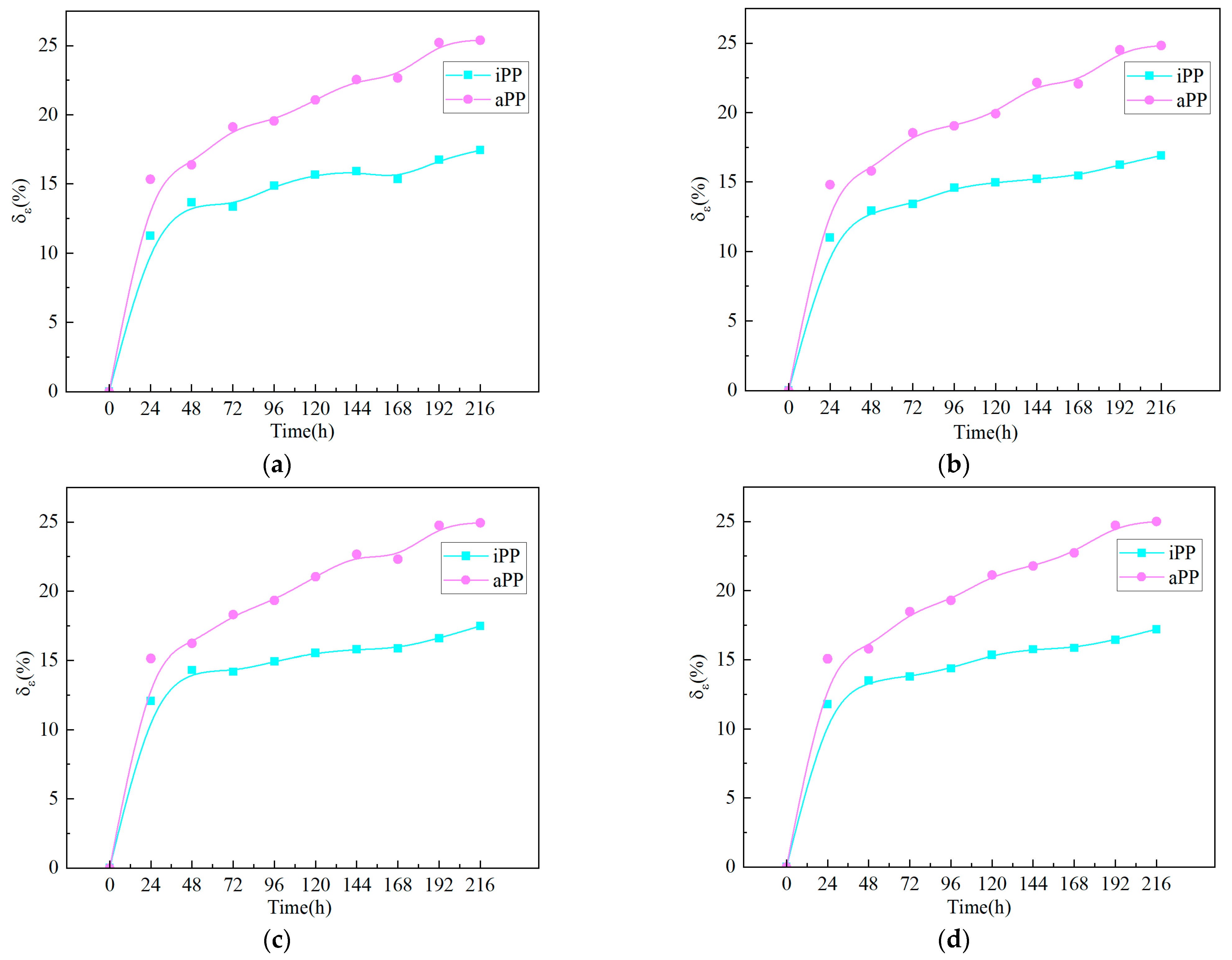
3.4. Variation of Electrical Properties with Water Immersion Time for PPs

Figure 5 depicts the changes in the volume resistivity of PPs with different water immersion times. It could be noted that with the increase in water immersion time, the volume resistivity of both two PPs exhibits a decreasing trend. This trend is attributed to the water absorption of PPs, resulting in an increase in the amount of adsorbed water in their bulk as the immersion time is prolonged. Consequently, the conductivity of PPs increases, leading to a decrease in internal insulation resistance. Notably, the decrease in volume resistivity is more pronounced for iPP compared to aPP. Under dry conditions, the volume resistivity of iPP is higher than that of aPP, but after 216 h of water immersion, the volume resistivity of iPP becomes lower than that of aPP. Taken together with the trends in weight gain of the PPs at different immersion time obtained in Figure 2, it can be concluded that the volume resistivity of iPP is more susceptible to water absorption.
To eliminate systematic errors within the measuring instruments, the relative permittivity growth rate δε is adopted to characterize the variation of the relative permittivity with the increase in immersion time under 1 Hz, 50 Hz, 1 kHz, and 10 kHz. The relative permittivity growth rate δε is defined as
δε = (εrt − εr0)/εrt × 100%,
where εrt represents the relative permittivity of samples at the immersion time t, and εr0 is the relative permittivity of samples at dry condition.
Figure 6 depicts the growth rates of relative permittivity for two PPs at different immersion times at frequencies of 1 Hz, 50 Hz, 1 kHz, and 10 kHz. It can be observed that frequency has little effect on the relative permittivity of two PPs at the same immersion time.
As the immersion time increases, the relative permittivity of both PPs also increases. The growth rate of relative permittivity for both samples at the four frequencies is relatively fast in the early stages of immersion. However, as the immersion time continues to increase, the growth rate gradually slows down. After an immersion time of 216 h, the relative permittivity of iPP increased by approximately 17%, while the relative permittivity of aPP increased by approximately 25%. This is because water is polar dielectric with the relative permittivity of ~78 at 25 °C, which is much greater than the relative permittivity of PPs. Therefore, based on the relationship between the water absorption rates of the two PPs shown in Figure 2, as the immersion time increases, the water content in the two PPs increases gradually, leading to the obvious increment in their relative permittivities.
A comparative analysis of the relative permittivity changes in the two PPs at four different frequencies reveals that the change in relative permittivity is greater for aPP than for iPP. However, when the immersion time reaches 216 h, the relative permittivity of aPP is more affected by water absorption than that of iPP.
Further, the correlation between the relative permittivity and water absorption rates of the two PPs at different immersion times under a frequency of 50 Hz is illustrated in Figure 7, in which linear fitting is used and the confidence level reaches 95%. The R-square of iPP is about 0.940, and the R-square of aPP is approximately 0.963, which shows that the linear correlation between water absorption and variation of relative permittivity for both PPs is relatively good and there is a strong correlation between the increase in relative permittivity of the two PPs and the increase in water content. However, it should be noted that the slope of iPP is relatively lower than that of aPP, which indicates the effect of water intrusion for the relative permittivity of iPP is more sensitive than that of aPP.

4. Discussion

PP is a semi-crystalline polymer containing micro pores or free volume area at molecular scale. As shown in Figure 1b, the radii of the spherical free volume for iPP and aPP are 0.617 nm and 0.598 nm, respectively, and it has been reported that the diameter of the water molecule is about 0.2 nm [34]. So even though PP is hydrophobic, water can still spread into the intermolecular space of PP as a result of the concentration difference. Thus, it is possible to observe the saturated water absorption state of PP, and its possible mechanisms are shown in Figure 8.
Water molecules have polarity. Upon the application of the DC electric field, absorbed water in PPs could take part in the polarization process of the whole bulk, which could increase the polarization current. Meanwhile, water may accelerate the ionic conductivity process, enhancing the long-range charge transference.
The relative permittivity growth rate of aPP is greater than that of iPP, which may be attributed to several factors. First of all, the change in crystal plane spacing between the two PP samples caused by water diffusion affects polarization behavior. As illustrated by Table 2, the crystal plane spacing of iPP increases after immersion, while the crystal plane spacing of aPP decreases. Secondly, differences in crystallinity may also affect the polarization behavior of semi-crystalline polymers. The higher the crystallinity, and the more orderly the arrangement of the molecular chains, the stronger the intermolecular forces, and the more difficult polarization is. This leads to the diffusion of water molecules into the interior of the iPP sample, and the growth rate of its dielectric constant is slower compared to aPP. Therefore, the growth rate of the relative permittivity of the aPP sample is higher.

5. Conclusions

Water absorption of insulating materials in a power cable system can degrade the insulation performance, posing a severe threat to the safe operation of the power grid. This investigation explores the impact of water absorption for iPP and aPP. The conclusions are as follows.
(1)
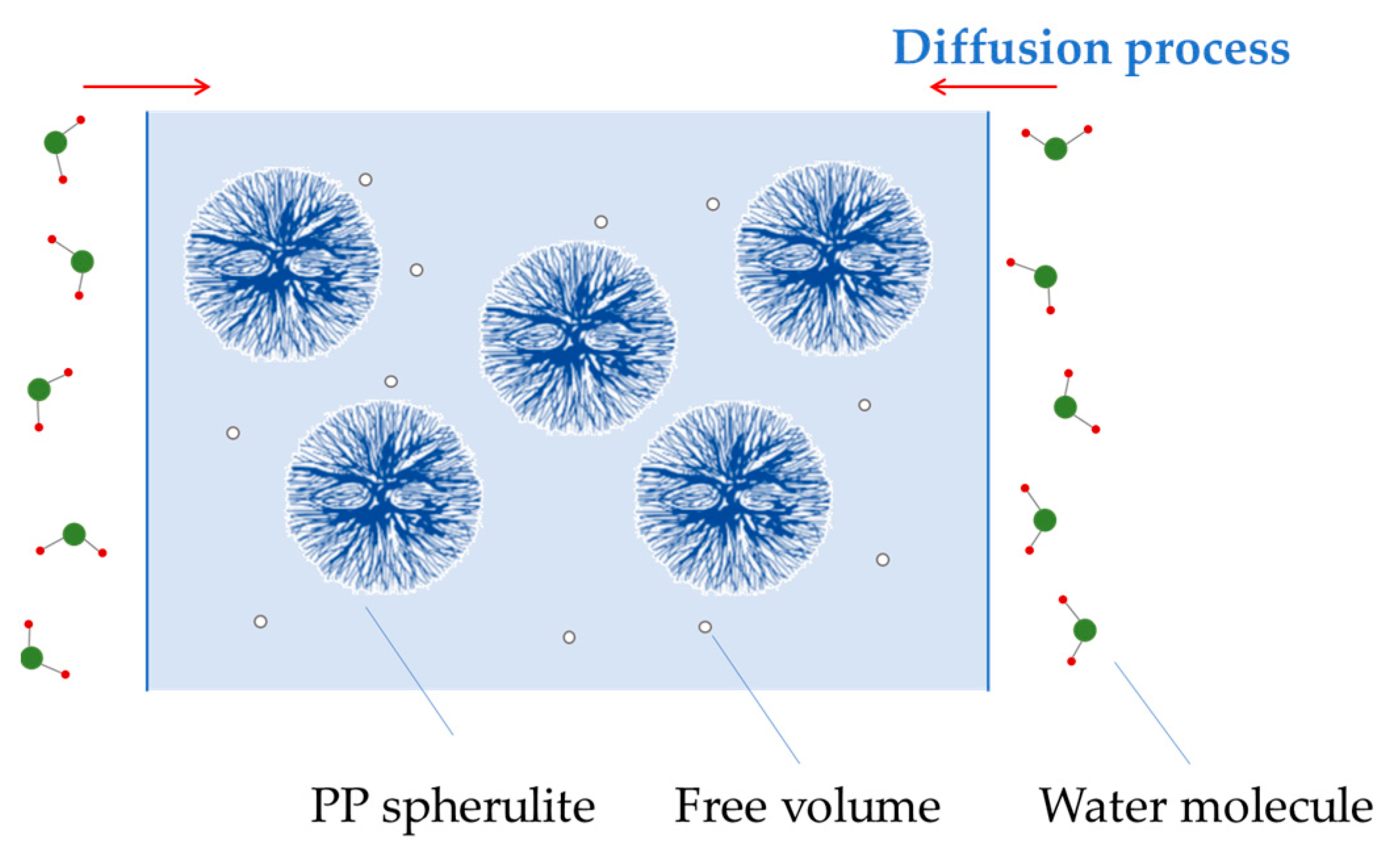
The water absorption rates of both iPP and aPP increase with extended water immersion, eventually reaching a saturation level of approximately 0.28% after around 216 h. Although the crystal form of PPs remains unchanged in a water environment, water intrusion causes the interplanar spacing of iPP to increase, while the interplanar spacing of aPP decreases slightly.
(2)
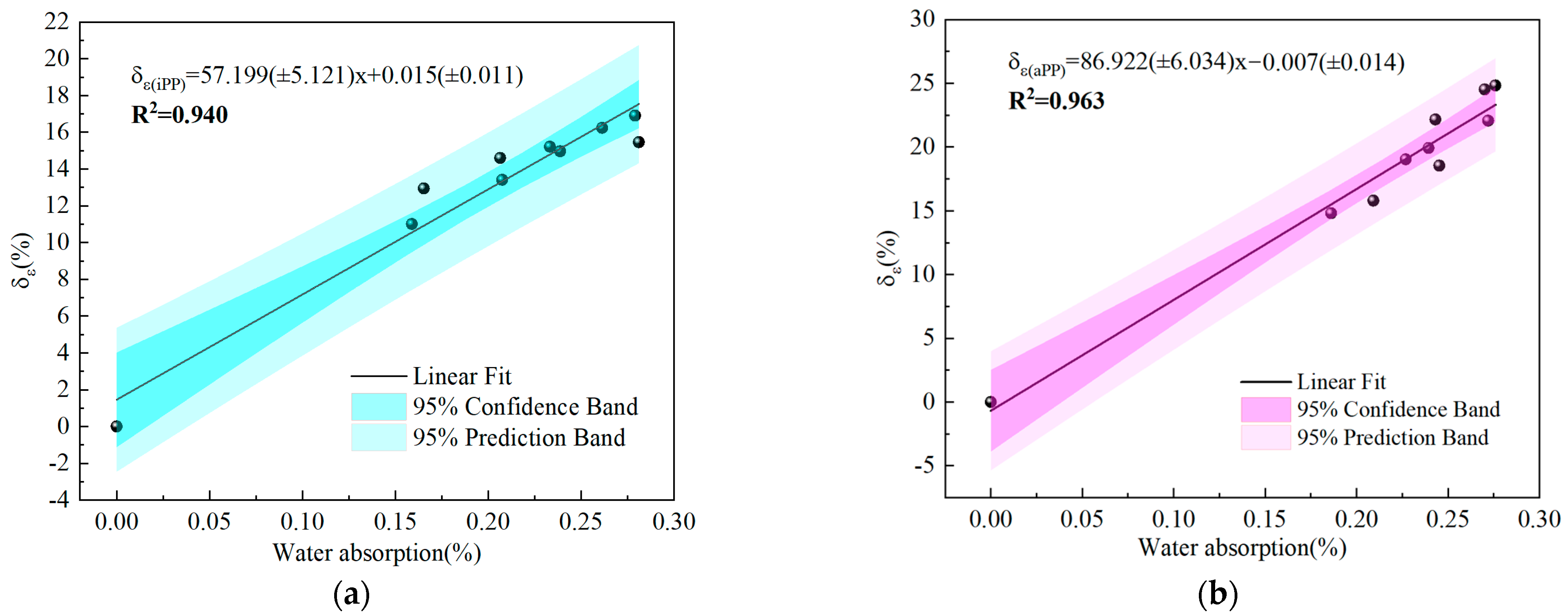
The volume resistivity of both PPs decreases as the water absorption rate increases, with iPP showing greater sensitivity to water intrusion. The increase in the water absorption rate leads to an increase in the relative permittivity of PP materials. In response to the saturated water absorption, the relative permittivity of iPP increased by approximately 17%, whereas that of aPP rose by about 25%, indicating that the relative permittivity of aPP is more affected by water.
There may be some limitations in this study, and future research should consider the role of the electric field and the temperature in water diffusion process in PPs. These findings may help us to understand the degradation of polymer applications in complex conditions.

Author Contributions

Conceptualization, R.X. and L.C.; methodology, R.X. and Q.J.; software, R.X.; validation, C.L., J.H. and Y.Z.; formal analysis, G.H.; investigation, R.X. and L.C.; resources, C.L. and J.H.; data curation, R.X. and L.C.; writing—original draft preparation, R.X., C.L., J.H. and Y.Z.; writing—review and editing, L.C., G.H., Y.G. and C.T.; visualization, R.X. and L.C.; supervision, L.C.; project administration, L.C. and C.T.; funding acquisition, L.C. All authors have read and agreed to the published version of the manuscript.

Funding

This research was funded by National Natural Science Foundation of China, grant number 52107030, and Natural Science Foundation of Chongqing, China, grant number sl202100000470.

Data Availability Statement

The original contributions presented in the study are included in the article, further inquiries can be directed to the corresponding author.

Acknowledgments

The authors would like to express their appreciation for the help of Libing Qian from Hubei University of Science and Technology, who assisted with the free volume characterization.

Conflicts of Interest

Authors Chuping Li, Jiaxun He and Ya Zhang were employed by the company Chongqing Taishan Cable Co., Ltd. The remaining authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

References

  1. Al-Khalidi, H.; Kalam, A. The impact of underground cables on power transmission and distribution networks. In Proceedings of the 2006 IEEE International Power and Energy Conference, Putra Jaya, Malaysia, 28–29 November 2006; pp. 576–580. [Google Scholar] [CrossRef]
  2. Tan, K.M.; Babu, T.S.; Ramachandaramurthy, V.K.; Kasinathan, P.; Solanki, S.G.; Raveendran, S.K. Empowering smart grid: A comprehensive review of energy storage technology and application with renewable energy integration. J. Energy Storage 2004, 39, 102591. [Google Scholar] [CrossRef]
  3. Koohi-Fayegh, S.; Rosen, M.A. A review of energy storage types, applications and recent developments. J. Energy Storage 2020, 27, 101047. [Google Scholar] [CrossRef]
  4. Wang, S.H.; Chen, P.X.; Li, H.; Li, J.Y.; Chen, Z.Z. Improved DC performance of crosslinked polyethylene insulation depending on a higher purity. IEEE Trans. Dielectr. Electr. Insul. 2017, 24, 1809–1817. [Google Scholar] [CrossRef]
  5. Florkowski, M. Influence of insulating material properties on partial discharges at DC voltage. Energies 2020, 13, 4305. [Google Scholar] [CrossRef]
  6. Green, C.D.; Vaughan, A.S.; Stevens, G.C.; Pye, A.; Sutton, S.J.; Geussens, T.; Fairhurst, M.J. Thermoplastic cable insulation comprising a blend of isotactic polypropylene and a propylene-ethylene copolymer. IEEE Trans. Dielectr. Electr. Insul. 2015, 22, 639–648. [Google Scholar] [CrossRef]
  7. Zhu, L.W.; Du, B.X.; Li, Z.L.; Su, J.G.; Jiang, J.P.; Kong, X.X. Electrical tree characteristics in polypropylene under impulse superimposed DC voltage in LN2. IEEE Trans. Appl. Supercond. 2019, 29, 7701403. [Google Scholar] [CrossRef]
  8. Zha, J.W.; Wu, Y.H.; Wang, S.J.; Wu, D.H.; Yan, H.D.; Dang, Z.M. Improvement of space charge suppression of polypropylene for potential application in HVDC cables. IEEE Trans. Dielectr. Electr. Insul. 2016, 23, 2337–2343. [Google Scholar] [CrossRef]
  9. Huang, X.Y.; Fan, Y.Y.; Zhang, J.; Jiang, P.K. Polypropylene based thermoplastic polymers for potential recyclable HVDC cable insulation applications. IEEE Trans. Dielectr. Electr. Insul. 2017, 24, 1446–1456. [Google Scholar] [CrossRef]
  10. Zhang, G.; Nam, C.; Chung, T.C.M.; Petersson, L.; Hillborg, H. Polypropylene copolymer containing cross-linkable antioxidant moieties with long-term stability under elevated temperature conditions. Macromolecules 2017, 50, 7041–7051. [Google Scholar] [CrossRef]
  11. Kurahashi, K.; Matsuda, Y.; Miyashita, Y.; Demura, T.; Ueda, A.; Yoshino, K. The application of novel polypropylene to the insulation of electric power cable. Electr. Eng. Jpn. 2006, 155, 1–8. [Google Scholar] [CrossRef]
  12. Huang, X.Y.; Zhang, J.; Jiang, P.K.; Tanaka, T. Material progress toward recyclable insulation of power cables. Part 1: Polyethylene based thermoplastic materials: Dedicated to the 80th birthday of professor Toshikatsu Tanaka. IEEE Electr. Insul. Mag. 2019, 35, 7–19. [Google Scholar] [CrossRef]
  13. Cheng, L.; Chi, X.H.; Yan, C.Y.; Xie, D.R.; Liu, X.W.; Wen, Y.N.; Liu, W.F.; Li, S.T. Polypropylene nanocomposite for power equipment: A review. IET Nanodielectrics 2018, 1, 92–103. [Google Scholar] [CrossRef]
  14. Li, Z.; Du, B. Polymeric insulation for high-voltage DC extruded cables: Challenges and development directions. IEEE Electr. Insul. Mag. 2018, 34, 30–43. [Google Scholar] [CrossRef]
  15. Chang, W.Z.; Du, F.; Bi, J.G.; Yuan, S.; Yang, Y. Assessment of creeping discharge initiated by metal particles on the silicone rubber/XLPE interface in cable joints. J. Electr. Eng. 2019, 70, 370–378. [Google Scholar] [CrossRef]
  16. Tao, W.; Song, S.; Yue, Z.; Wei, H.; Ming, H. Study on the electric-field characteristics of water tree region on the dry or wet condition in XLPE cables. In Proceedings of the 2016 IEEE International Conference on High Voltage Engineering and Application (ICHVE), Chengdu, China, 19–22 September 2016; pp. 1–4. [Google Scholar] [CrossRef]
  17. Zhao, X.F.; Pu, L.; Xu, L.; Zhang, X.; Guo, B.H.; Zhao, A.X.; Deng, J.B.; Zhang, G.J.; Hui, N.; Fan, M.H. Research on the defect development of cable accessories under AC voltage. In Proceedings of the 2017 1st International Conference on Electrical Materials and Power Equipment (ICEMPE), Xi’an, China, 14–17 May 2017; pp. 457–462. [Google Scholar] [CrossRef]
  18. Li, G.C.; Liang, X.J.; Zhang, J.H.; Li, X.J.; Wei, Y.H.; Hao, C.C.; Lei, Q.Q.; Li, S.T. Insulation properties and interface defect simulation of distribution network cable accessories under moisture condition. IEEE Trans. Dielectr. Electr. Insul. 2022, 29, 403–411. [Google Scholar] [CrossRef]
  19. Li, J.; Zhao, X.; Yin, G.; Li, S.; Zhao, J.; Ouyang, B. The effect of accelerated water tree ageing on the properties of XLPE cable insulation. IEEE Trans. Dielectr. Electr. Insul. 2011, 18, 1562–1569. [Google Scholar] [CrossRef]
  20. Pompili, M.; Calcara, L.; D’Orazio, L.; Ricci, D.; Derviškadić, A.; He, H. Joints defectiveness of MV underground cable and the effects on the distribution system. Electr. Power Syst. Res. 2021, 192, 107004. [Google Scholar] [CrossRef]
  21. Cao, W.K.; Li, Z.; Sheng, G.H.; Jiang, X. Insulating property of polypropylene nanocomposites filled with nano-MgO of different concentration. IEEE Trans. Dielectr. Electr. Insul. 2017, 24, 1430–1437. [Google Scholar] [CrossRef]
  22. Hu, S.X.; Zhou, Y.; Yuan, C.; Wang, W.; Hu, J.; Li, Q.; He, J. Surface-modification effect of MgO nanoparticles on the electrical properties of polypropylene nanocomposite. High Volt. 2020, 5, 249–255. [Google Scholar] [CrossRef]
  23. Xie, D.R.; Min, D.M.; Huang, Y.; Li, S.T.; Nazir, M.T.; Phung, B.T. Classified effects of nanofillers on DC breakdown and partial discharge resistance of polypropylene/alumina nanocomposites. IEEE Trans. Dielectr. Electr. Insul. 2019, 26, 698–705. [Google Scholar] [CrossRef]
  24. IEC 60212-2014; Standard Conditions for Use Prior to and During the Testing of Solid Electrical Insulating Materials. International Electrotechnical Commission (IEC): London, UK, 2014.
  25. Kouini, B. Water absorption and hygrothermal aging behaviors of polyamide66/maleated polypropylene/nanoclay nanocomposites. J. Macromol. Sci. Part A-Pure Appl. Chem. 2020, 57, 512–518. [Google Scholar] [CrossRef]
  26. Chen, X.L.; Yu, J.; Guo, S.Y. Structure and properties of polypropylene composites filled with magnesium hydroxide. J. Appl. Polym. Sci. 2006, 102, 4943–4951. [Google Scholar] [CrossRef]
  27. Xu, Y.; Sun, Z.; Chen, X.L.; Chen, M.; Hu, S.C.; Zhang, Z.B. Mechanical properties and crystallization behavior of polycarbonate/polypropylene blends. J. Macromol. Sci. Part B-Phys. 2012, 52, 716–725. [Google Scholar] [CrossRef]
  28. Sharma, S.K.; Pujari, P.K. Role of free volume characteristics of polymer matrix in bulk physical properties of polymer nanocomposites: A review of positron annihilation lifetime studies. Prog. Polym. Sci. 2017, 75, 31–47. [Google Scholar] [CrossRef]
  29. Kim, H.; Kobayashi, S.; AbdurRahim, M.A.; Zhang, M.J.; Khusainova, A.; Hillmyer, M.A.; Abdala, A.A.; Macosko, C.W. Graphene/polyethylene nanocomposites: Effect of polyethylene functionalization and blending methods. Polymer 2011, 52, 1837–1846. [Google Scholar] [CrossRef]
  30. He, D.; Peng, Z.; Gong, W.; Luo, Y.; Zhao, P.; Kong, L. Mechanism of a green graphene oxide reduction with reusable potassium carbonate. RSC Adv. 2015, 5, 11966–11972. [Google Scholar] [CrossRef]
  31. Zhang, Y.C.; Li, J.X.; Shen, L.G.; Lin, H.J.; Shan, Y.D. The observation of PP/EVA blends in which isotactic PP was preradiated with different radiation absorbed doses. J. Appl. Polym. Sci. 2017, 134, 45057. [Google Scholar] [CrossRef]
  32. Shen, Y.; Wu, P.Y. Two-Dimensional ATR−FTIR spectroscopic investigation on water diffusion in polypropylene film:  Water bending vibration. J. Phys. Chem. B 2003, 18, 4424–4426. [Google Scholar] [CrossRef]
  33. Veluri, S.; Sowinski, P.; Svyntkivska, M.; Bartczak, Z.; Makowski, T.; Piorkowska, E. Structure and mechanical properties of iPP-based nanocomposites crystallized under high pressure. Nanomaterials 2024, 14, 629. [Google Scholar] [CrossRef]
  34. Guo, J.; Meng, X.; Chen, J.; Peng, J.; Sheng, J.; Li, X.; Xu, L.; Shi, J.; Wang, E.; Jiang, Y. Real-space imaging of interfacial water with submolecular resolution. Nat. Mater. 2014, 13, 184–189. [Google Scholar] [CrossRef]
Energies 17 04576 g001
Figure 1. Comparison in structure of iPP and aPP under dry conditions. (a) FTIR spectra of PPs; (b) free volume characteristics of PPs; (c) XRD images of PPs; (d) melting curves of PPs.
Figure 1. Comparison in structure of iPP and aPP under dry conditions. (a) FTIR spectra of PPs; (b) free volume characteristics of PPs; (c) XRD images of PPs; (d) melting curves of PPs.
Energies 17 04576 g001
Energies 17 04576 g002
Figure 2. Water absorption rate of PPs vs. immersion time.
Figure 2. Water absorption rate of PPs vs. immersion time.
Energies 17 04576 g002
Energies 17 04576 g003
Figure 3. FITR spectra of PPs before and after water absorption, using pure water as a reference; A and B are enlarged partial views of the four curves at the dashed box.
Figure 3. FITR spectra of PPs before and after water absorption, using pure water as a reference; A and B are enlarged partial views of the four curves at the dashed box.
Energies 17 04576 g003
Energies 17 04576 g004
Figure 4. X-ray diffraction images of PPs before and after water absorption.
Figure 4. X-ray diffraction images of PPs before and after water absorption.
Energies 17 04576 g004
Energies 17 04576 g005
Figure 5. The volume resistivity of PPs at different water absorption time.
Figure 5. The volume resistivity of PPs at different water absorption time.
Energies 17 04576 g005
Energies 17 04576 g006
Figure 6. The variation in the relative permittivity growth rate for PPs with immersion time at various frequencies, including (a) 1 Hz, (b) 50 Hz, (c) 1 kHz, and (d) 10 kHz.
Figure 6. The variation in the relative permittivity growth rate for PPs with immersion time at various frequencies, including (a) 1 Hz, (b) 50 Hz, (c) 1 kHz, and (d) 10 kHz.
Energies 17 04576 g006
Energies 17 04576 g007
Figure 7. Relationship between the relative permittivity and water absorption rate of PPs. (a) iPP and (b) aPP.
Figure 7. Relationship between the relative permittivity and water absorption rate of PPs. (a) iPP and (b) aPP.
Energies 17 04576 g007
Energies 17 04576 g008
Figure 8. A possible water diffusion model in both iPP and aPP.
Figure 8. A possible water diffusion model in both iPP and aPP.
Energies 17 04576 g008
Table 1. Details of PPs used in this investigation.
Table 1. Details of PPs used in this investigation.
SamplesIsotacticity/%Melt Index/(g/10 min)
(230 °C/2.16 kg)		Density/(g/cm3)
(23 °C)		Tensile Strength
/MPa
iPP98%3.0~0.9132.8
aPP\2.5~0.9028.0
Table 2. Differences in peak location and corresponding d of PPs before and after water absorption.
Table 2. Differences in peak location and corresponding d of PPs before and after water absorption.
PeaksiPPaPP
Before Water AbsorptionAfter Water AbsorptionBefore Water AbsorptionAfter Water Absorption
2θ/°d/Å2θ/°d/Å2θ/°d/Å2θ/°d/Å
Peak 114.36.2014.26.2514.06.3414.26.25
Peak 217.25.1617.15.1916.85.2917.15.19
Peak 318.94.7018.84.7318.64.7818.94.70
Peak 421.44.1621.34.1821.24.2021.54.14
Peak 522.14.0322.14.0321.94.0722.24.01
Peak 6////20.14.4220.24.40
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Xi, R.; Jiang, Q.; Cao, L.; Li, C.; He, J.; Zhang, Y.; He, G.; Gui, Y.; Tang, C. Effects of Water Absorption on the Insulating Properties of Polypropylene. Energies 2024, 17, 4576. https://doi.org/10.3390/en17184576

AMA Style

Xi R, Jiang Q, Cao L, Li C, He J, Zhang Y, He G, Gui Y, Tang C. Effects of Water Absorption on the Insulating Properties of Polypropylene. Energies. 2024; 17(18):4576. https://doi.org/10.3390/en17184576

Chicago/Turabian Style

Xi, Rui, Qiyang Jiang, Liang Cao, Chuping Li, Jiaxun He, Ya Zhang, Gaohui He, Yingang Gui, and Chao Tang. 2024. "Effects of Water Absorption on the Insulating Properties of Polypropylene" Energies 17, no. 18: 4576. https://doi.org/10.3390/en17184576

APA Style

Xi, R., Jiang, Q., Cao, L., Li, C., He, J., Zhang, Y., He, G., Gui, Y., & Tang, C. (2024). Effects of Water Absorption on the Insulating Properties of Polypropylene. Energies, 17(18), 4576. https://doi.org/10.3390/en17184576

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Back to TopTop