Life Estimation of HVDC Cables Subjected to Fast and Slow Polarity Reversals
Abstract
1. Introduction
2. Theoretical Framework Development
2.1. Voltage Polarity Reversals (VPRs)
2.2. Temperature Calculations
2.3. Electric Field Calculations
2.4. Life Estimation
3. Case Study
4. Results
4.1. Electric Field Distribution before, during and after VPRs
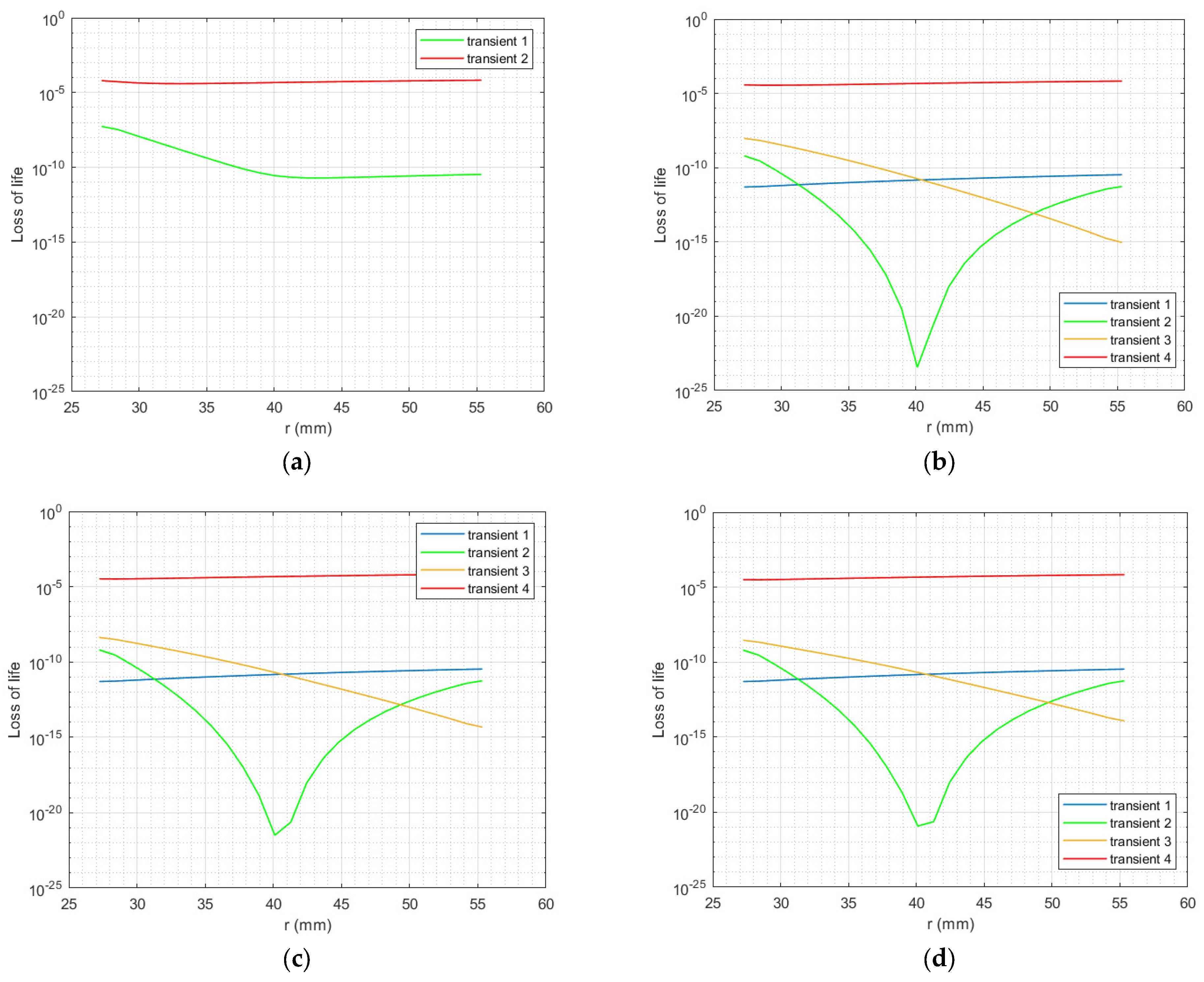
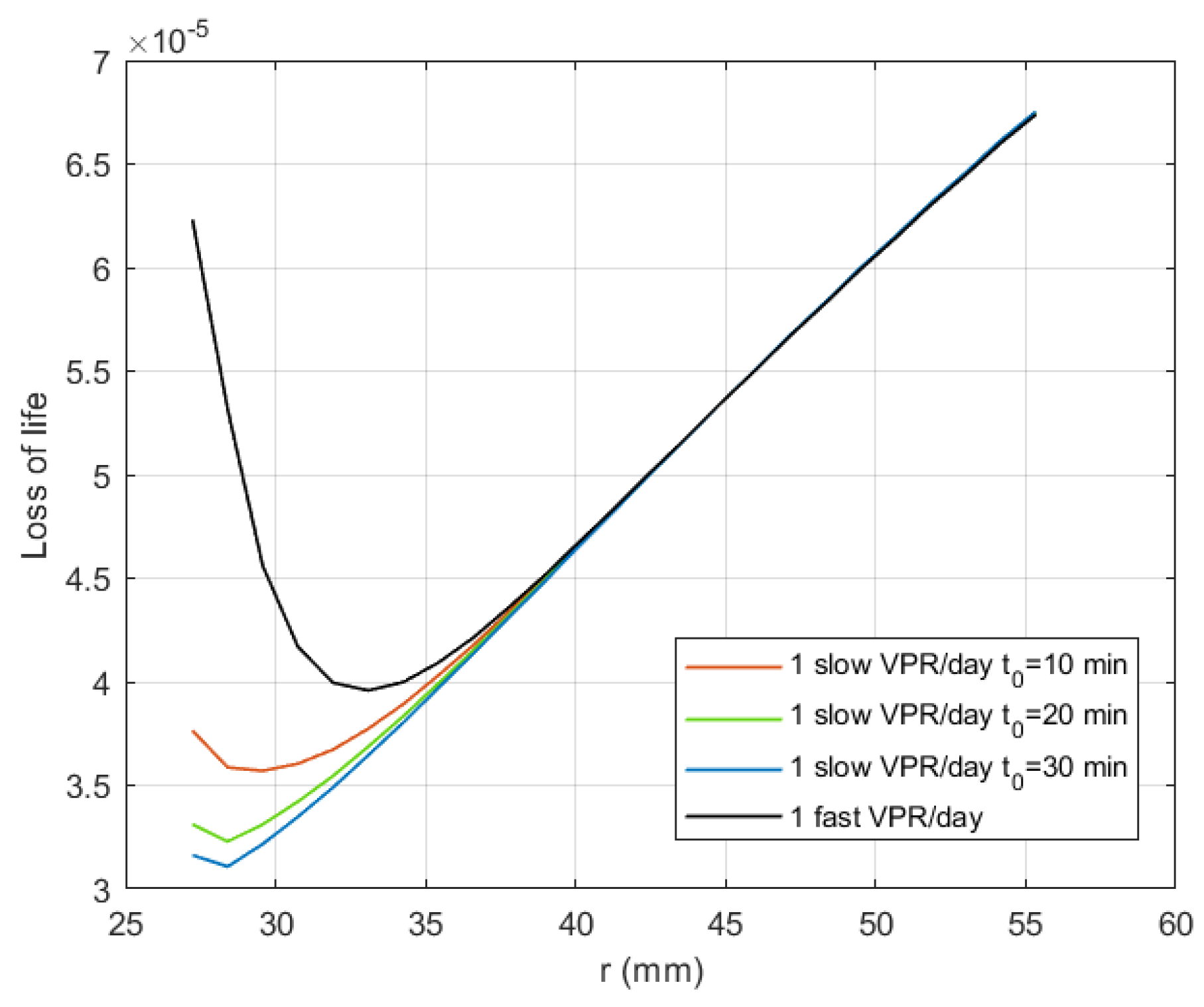
4.2. Loss of Life before, during and after VPRs
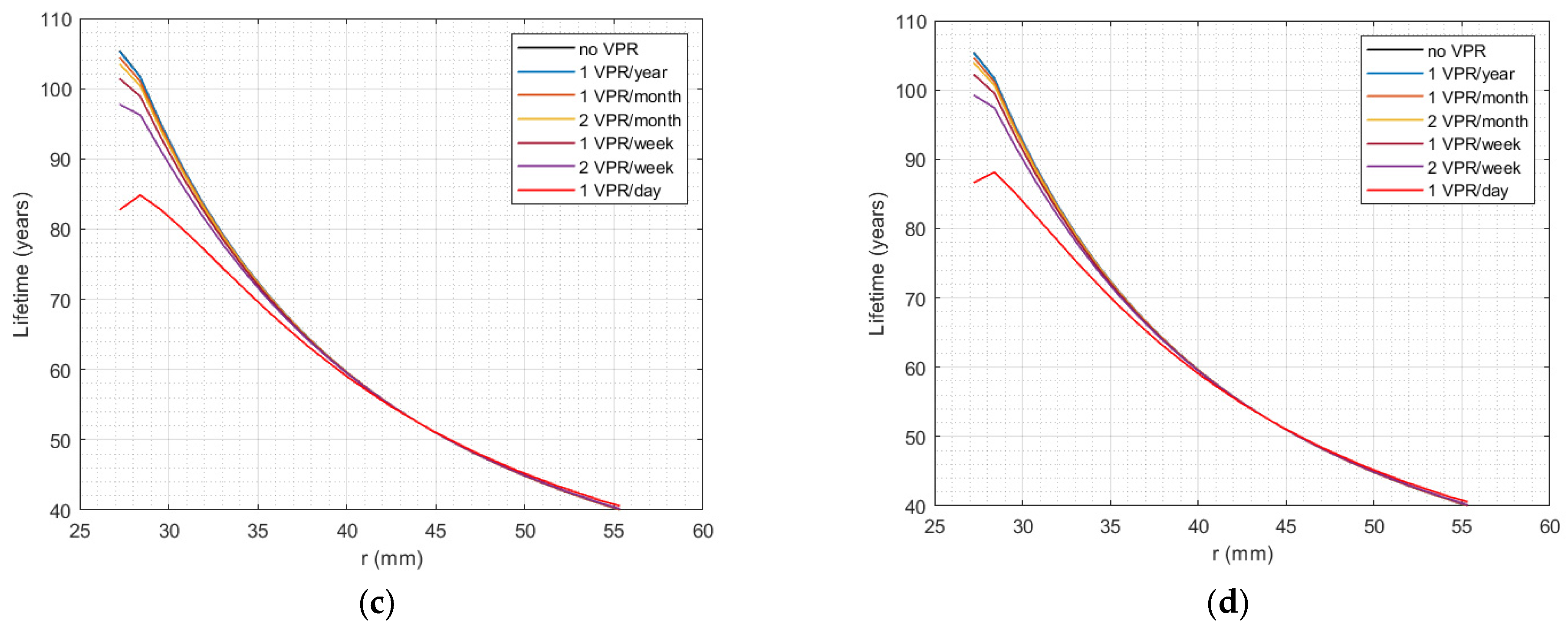
4.3. Total Life of Cable
5. Discussion
6. Conclusions
- (1)
- A noticeable effect of fast VPRs on the local life in the inner insulation.
- (2)
- The slow VPRs have less loss of life due to the electrical stress relieved as a result of space charge relaxation inside the insulation thickness.
- (3)
- The longer the zero-voltage period in slow VPRs, the less the electrical stress, and thus, the longer the inner insulation’s local life inside the insulation.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Hussein, I.I.; Ismael, I.; Essallah, S.; Khedher, A. The Impact of HVDC on the Current and Future Energy Markets. Int. J. Electr. Electron. Eng. Telecommun. 2022, 11, 426–434. [Google Scholar] [CrossRef]
- Diban, B. Models for Reliability Estimation of HVDC Cable Systems. Ph.D. Thesis, University of Bologna, Bologna, Italy, 2023. Available online: https://amsdottorato.unibo.it/10525/3/Diban_Bassel_thesis_PhD.pdf (accessed on 16 April 2024).
- 50Hertz Transmission GmbH; Amprion GmbH; Tennet TSO GmbH; TransentBw GmbH. Grid Development Plan. (GDP) 2030, 2nd Draft; Technical Report; German TSOs: Berlin, Germany, 2019. [Google Scholar]
- Jeroense, M. Fully Qualified 640 Kv Underground Extruded DC Cable System, Paper B1-309; CIGRÉ: Paris, France, 2018. [Google Scholar]
- Diban, B.; Mazzanti, G.; Seri, P. Life-based Geometric Design of HVDC Cables. Part 2: Effect of Electrical and Thermal Transients. IEEE Trans. Dielectr. Electr. Insul. 2022, 30, 97–105. [Google Scholar] [CrossRef]
- Rizzo, G.; Romano, P.; Imburgia, A.; Albertini, M.; Bononi, S.F.; Siripurapu, S.; Ala, G. The effect of Transient Over Voltages on the Partial Discharges activity in HVDC joints. In Proceedings of the 2021 AEIT HVDC International Conference (AEIT HVDC), Genoa, Italy, 27–28 May 2021; pp. 1–6. [Google Scholar] [CrossRef]
- Mazzanti, G.; Marzinotto, M.; Battaglia, A. A first step towards predicting the life of HVDC cables subjected to load cycles and voltage polarity reversal. In Proceedings of the 2015 IEEE Conference on Electrical Insulation and Dielectric Phenomena (CEIDP), Ann Arbor, MI, USA, 18–21 October 2015; pp. 783–786. [Google Scholar] [CrossRef]
- Battaglia, A.; Marzinotto, M.; Mazzanti, G. A Deeper Insight in Predicting the Effect of Voltage Polarity Reversal on HVDC Cables. In Proceedings of the 2019 IEEE Conference on Electrical Insulation and Dielectric Phenomena (CEIDP), Richland, WA, USA, 20–23 October 2019. [Google Scholar] [CrossRef]
- Naderiallaf, H.; Seri, P.; Montanari, G.C. Effect of Voltage Slew Rate on Partial Discharge Phenomenology During Voltage Transient in HVDC Insulation: The Case of Polymeric Cables. IEEE Trans. Dielectr. Electr. Insul. 2022, 29, 215–222. [Google Scholar] [CrossRef]
- Cambareri, P.; de Falco, C.; Rienzo, L.D.; Seri, P.; Montanari, G.C. Electric Field Calculation During Voltage Transients in HVDC Cables: Contribution of Polarization Processes. IEEE Trans. Power Deliv. 2022, 37, 5425–5432. [Google Scholar] [CrossRef]
- Mazzanti, G.; Diban, B. The Effects of Transient Overvoltages on the Reliability of HVDC Extruded Cables. Part 2: Superimposed Switching Impulses. IEEE Trans. Power Deliv. 2021, 36, 3795–3804. [Google Scholar] [CrossRef]
- Marzinotto, M.; Mazzanti, G.; Vercellotti, U.; Jahn, H. On the way to compare the polarity reversal withstand capability of HVDC Mass-Impregnated and extruded cable systems. In Proceedings of the 9th International Conference on Insulated Power Cables, Versailles, France, 21–25 June 2015; pp. 1–4. [Google Scholar]
- Albertini, M.; Cotugno, S.; Pietribiasi, D.; Remy, C. HPTE Extruded Cables Polarity Reversals Performance in LCC HVDC Systems. In Proceedings of the 2020 AEIT International Annual Conference (AEIT), Catania, Italy, 23–25 September 2020. [Google Scholar] [CrossRef]
- Dao, N.L.; Lewin, P.L.; Swingler, S.G. Lightning impulse ageing of HV cable insulation. In Proceedings of the 16th International Symposium on High Voltage Engineering, Johannesburg, South Africa, 24–28 August 2009; pp. 562–565. [Google Scholar]
- Hartlein, R.A.; Harper, V.S.; Ng, H.W. Effects of Voltage Impulses on Solid Dielectric Cable Life. IEEE Power Eng. Rev. 1989, 9, 39–40. [Google Scholar] [CrossRef]
- Ildstad, E.; Mauseth, F.; Olsen, E.T. Breakdown Voltage of Polymeric HVDC Insulation at DC Stress and Superimposed Lightning Impulse Voltages. In Proceedings of the 27th Nordic Insulation Symposium on Materials, Components and Diagnostics NordIS-22, Trondheim, Norway, 13–15 June 2022. [Google Scholar]
- He, D.; Tao, Z.; Fansong, M.; Li, Q.; Wang, W.; Liu, H.; Teyssedre, G. Space Charge Behaviours in Cable Insulation under a DC-Superimposed Pulsed Electric Field. High Voltage. 2020. Available online: https://hal.science/hal-03002410/document (accessed on 16 April 2024).
- Diban, B.; Mazzanti, G. The Effect of Insulation Characteristics on Thermal Instability in HVDC Extruded Cables. Energies 2021, 14, 550. [Google Scholar] [CrossRef]
- Mazzanti, G. The combination of electro-thermal stress, load cycling and thermal transients and its effects on the life of high voltage ac cables. IEEE Trans. Dielectr. Electr. Insul. 2009, 16, 1168–1179. [Google Scholar] [CrossRef]
- Miner, M.A. Cumulative damage in fatigue. J. Appl. Mech. 1945, 12, A159–A163. [Google Scholar] [CrossRef]
- IEC 60853-2:1989; Calculation of the Cyclic and Emergency Current Rating of Cables, Part 2: Cyclic Rating of Cables Greater Than 18/30 (36) kV and Emergency Ratings for Cables of All Voltages. International Electrotechnical Commission: Geneva, Switzerland, 1989.
- Ashraf, E.; Kabeel, A.E.; Elmashad, Y.; Ward, S.A.; Shaban, W.M. Predicting solar distiller productivity using an AI Approach: Modified genetic algorithm with Multi-Layer Perceptron. Solar Energy 2023, 263, 111964. [Google Scholar] [CrossRef]
- Shaalan, E.; Samy, M.; Ghania, M.; Ward, S.A. Analysis of electric field inside HV substations using charge simulation method in three dimensional. In Proceedings of the 2010 Annual Report Conference on Electrical Insulation and Dielectic Phenomena, West Lafayette, IN, USA, 17–20 October 2010; IEEE: Piscataway, NJ, USA, 2010. [Google Scholar]
- Li, G.; An, T.; Liang, J.; Liu, W.; Joseph, T.; Lu, J. Power reversal strategies for hybrid LCC/MMC HVDC systems. CSEE J. Power Energy Syst. 2020, 6, 203–221. [Google Scholar] [CrossRef]
- Hampton, R.N. Some of the considerations for materials operating under high-voltage direct-current stresses. IEEE Electr. Insul. Mag. 2008, 24, 5–13. [Google Scholar] [CrossRef]
- Recommendations to Improve HVDC Cable Systems Reliability. Available online: https://europacable.eu/wp-content/uploads/2021/01/Joint-paper-HVDC-Cable-Reliability-ENTSO-E-Europacable_FINAL_13.06.2019_.pdf (accessed on 16 April 2024).

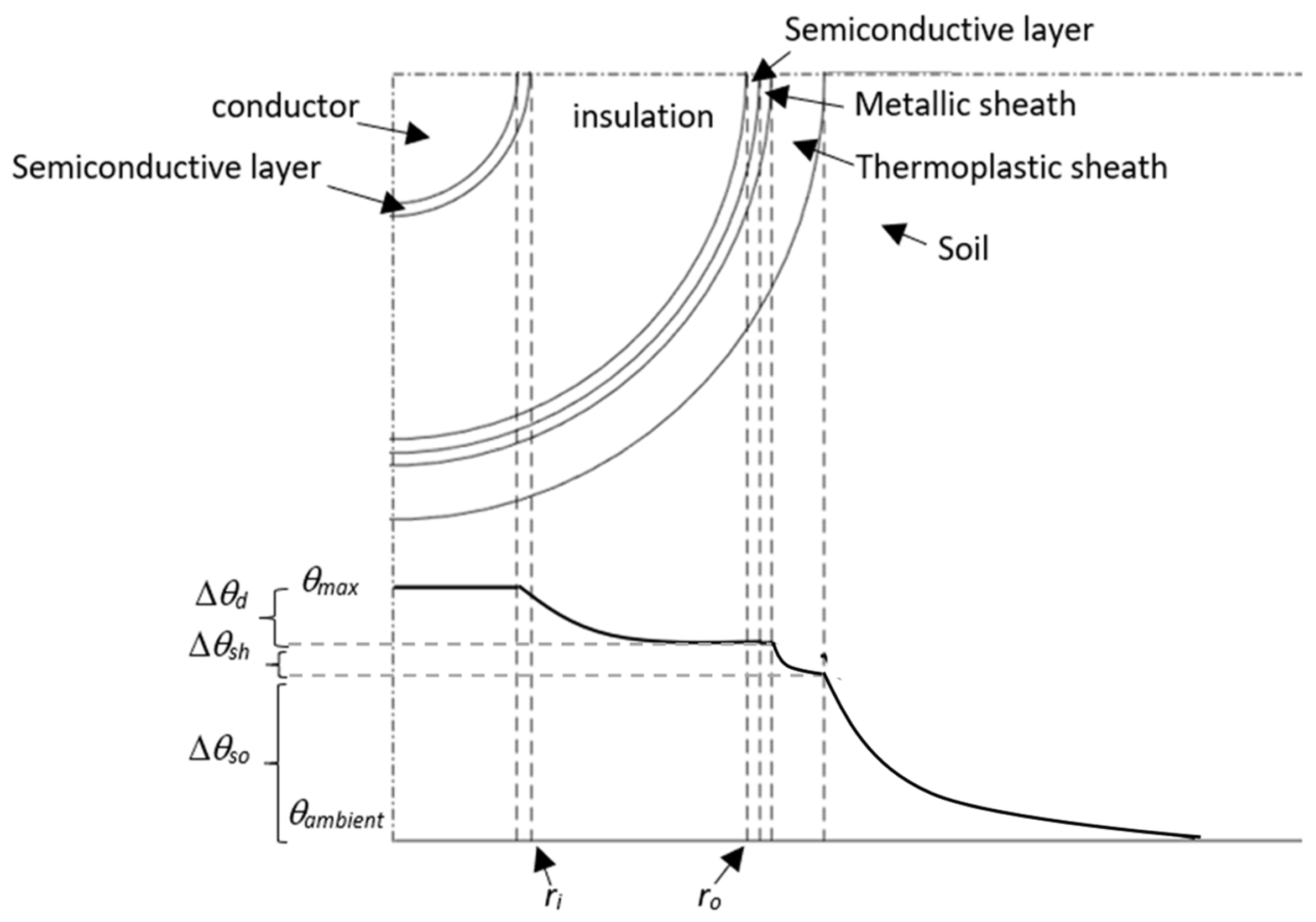
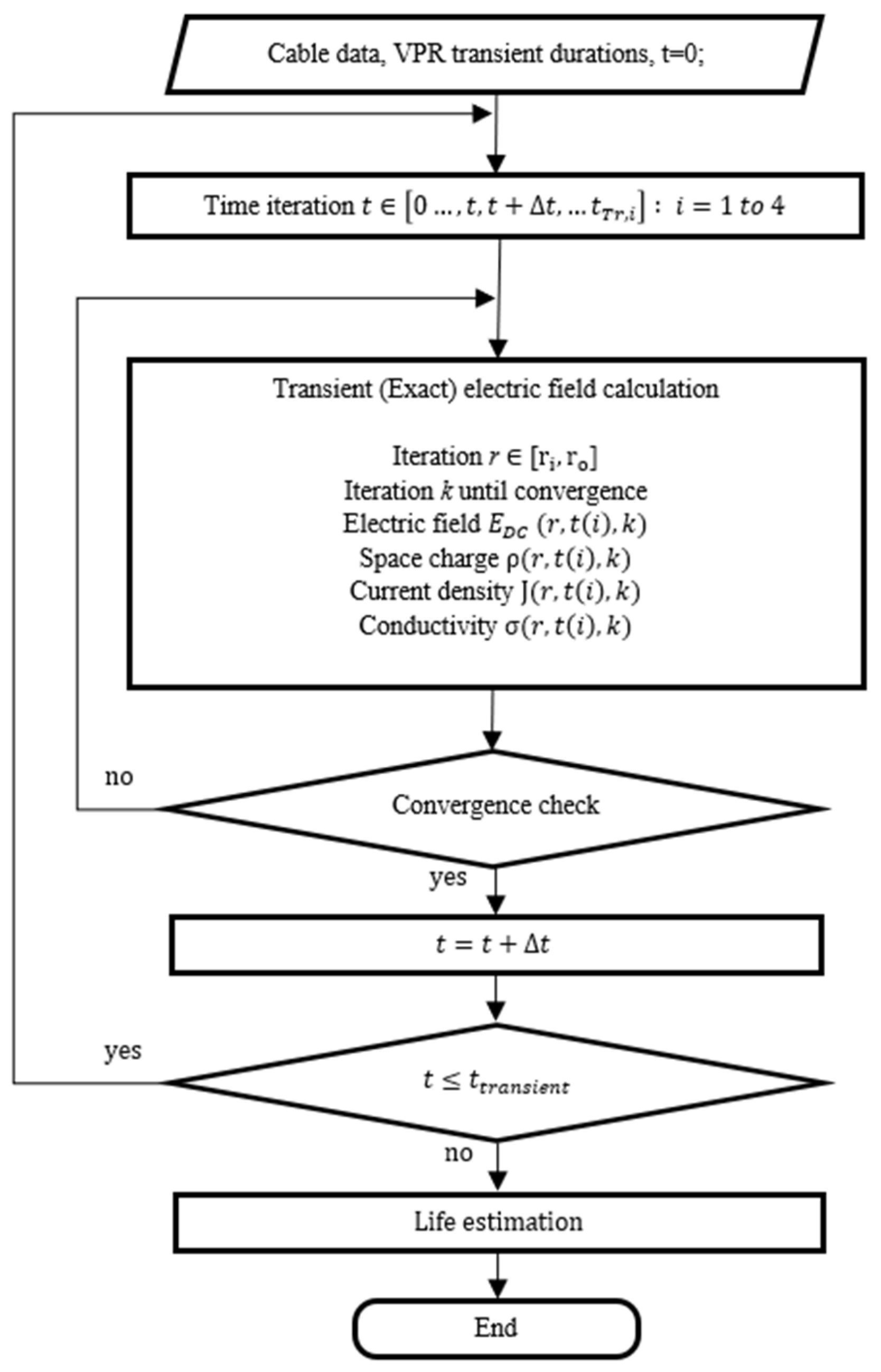
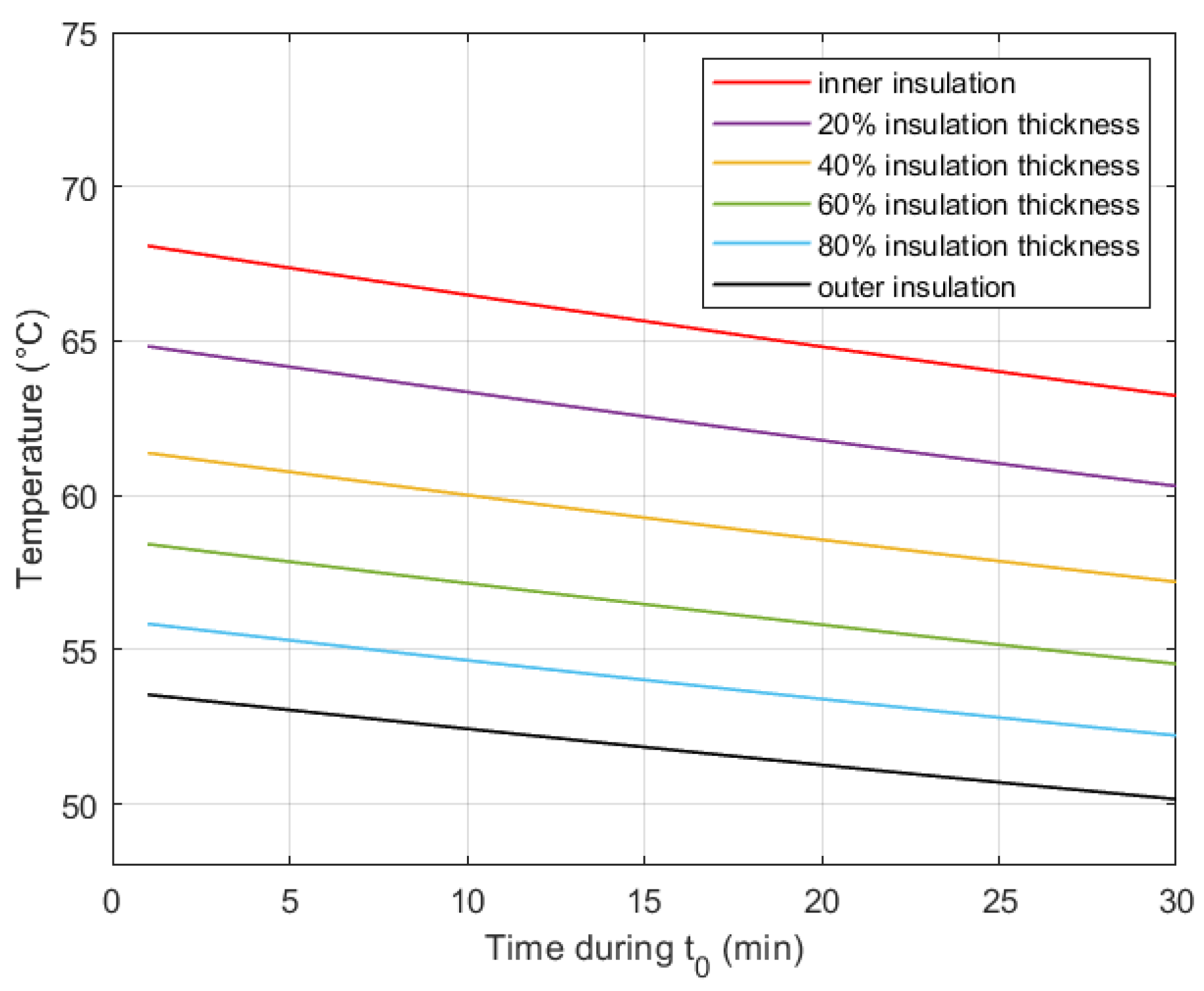
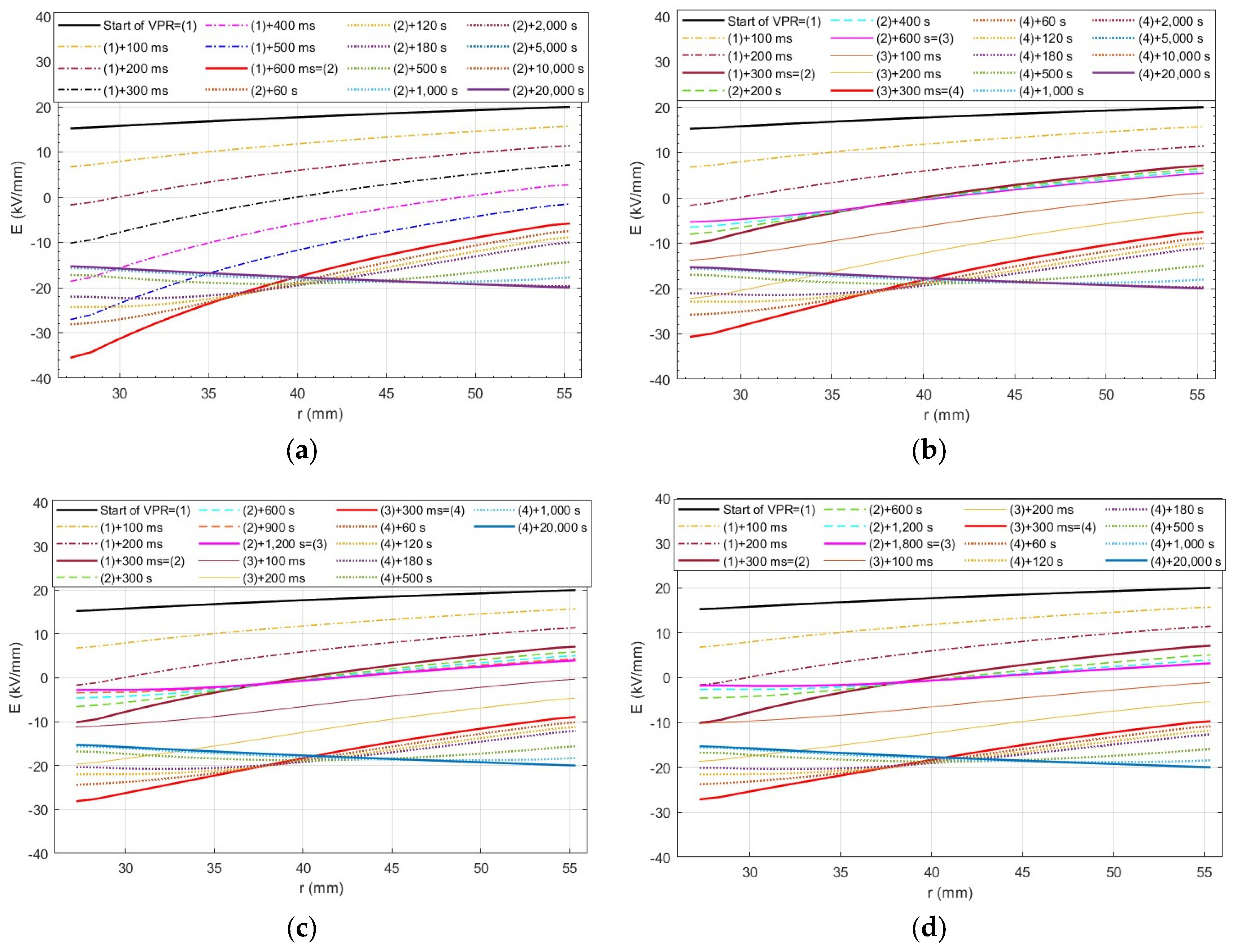

| Parameter | Value |
|---|---|
| Rated power (bipolar scheme) (MW) | 1920 |
| Rated voltage (kV) | 500 |
| Conductor material | Cu |
| Insulation material | DC-XLPE |
| Relative permittivity | 2.3 |
| Rated conductor temperature (°C) | 70 |
| Ambient temperature a (°C) | 20 |
| Conductor cross-section (mm2) | 2000 |
| Inner semiconductor thickness (mm) | 2 |
| Inner insulation radius ri (mm) | 27.2 |
| Insulation thickness (mm) | 28.1 |
| Outer insulation radius ro (mm) | 55.3 |
| Outer semiconductor thickness (mm) | 1 |
| Metallic shield thickness (mm) | 1 |
| Thermoplastic sheath thickness (mm) | 4.5 |
| Thermal resistivity of dielectric [K·m/W] | 3.5 |
| Thermal resistivity of sheath [K·m/W] | 3.5 |
| Thermal resistivity of soil [K·m/W] | 1.3 |
| Burial depth bb (m) | 1.3 |
| Design life LD (years) | 40 |
| ttot (year) | 1 |
| Temperature coefficient of conductivity a (1/°C) [25] | 0.084 |
| Field coefficient of conductivity b (mm/kV) [25] | 0.0645 |
| Parameter | Value |
|---|---|
| Transient type | Fast and slow VPR |
| Frequency of VPR events [26] | 0 VPR/year (fast) |
| 1 VPR/year (fast) | |
| 1 VPR/month (fast) | |
| 2 VPRs/month (slow) | |
| 1 VPR/week (slow) | |
| 2 VPRs/week (slow) | |
| 1 VPR/day (slow) | |
| t0 | 0 (fast VPR) |
| 10 min | |
| 20 min | |
| 30 min |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Diban, B.; Mazzanti, G.; Marzinotto, M.; Battaglia, A. Life Estimation of HVDC Cables Subjected to Fast and Slow Polarity Reversals. Energies 2024, 17, 3182. https://doi.org/10.3390/en17133182
Diban B, Mazzanti G, Marzinotto M, Battaglia A. Life Estimation of HVDC Cables Subjected to Fast and Slow Polarity Reversals. Energies. 2024; 17(13):3182. https://doi.org/10.3390/en17133182
Chicago/Turabian StyleDiban, Bassel, Giovanni Mazzanti, Massimo Marzinotto, and Antonio Battaglia. 2024. "Life Estimation of HVDC Cables Subjected to Fast and Slow Polarity Reversals" Energies 17, no. 13: 3182. https://doi.org/10.3390/en17133182
APA StyleDiban, B., Mazzanti, G., Marzinotto, M., & Battaglia, A. (2024). Life Estimation of HVDC Cables Subjected to Fast and Slow Polarity Reversals. Energies, 17(13), 3182. https://doi.org/10.3390/en17133182










