Sinusoidal Rotor Core Shape for Low Torque Ripple in Hollow-Cup Machines
Abstract
1. Introduction
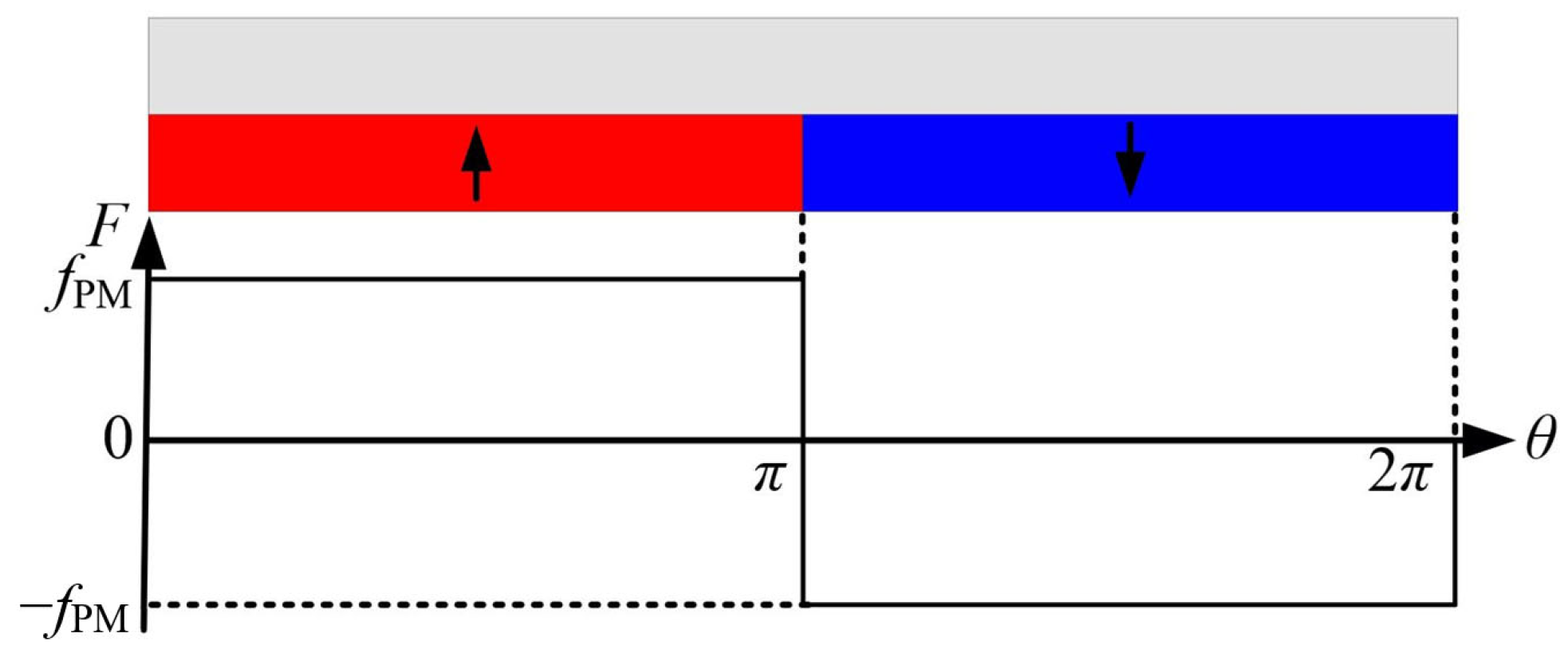
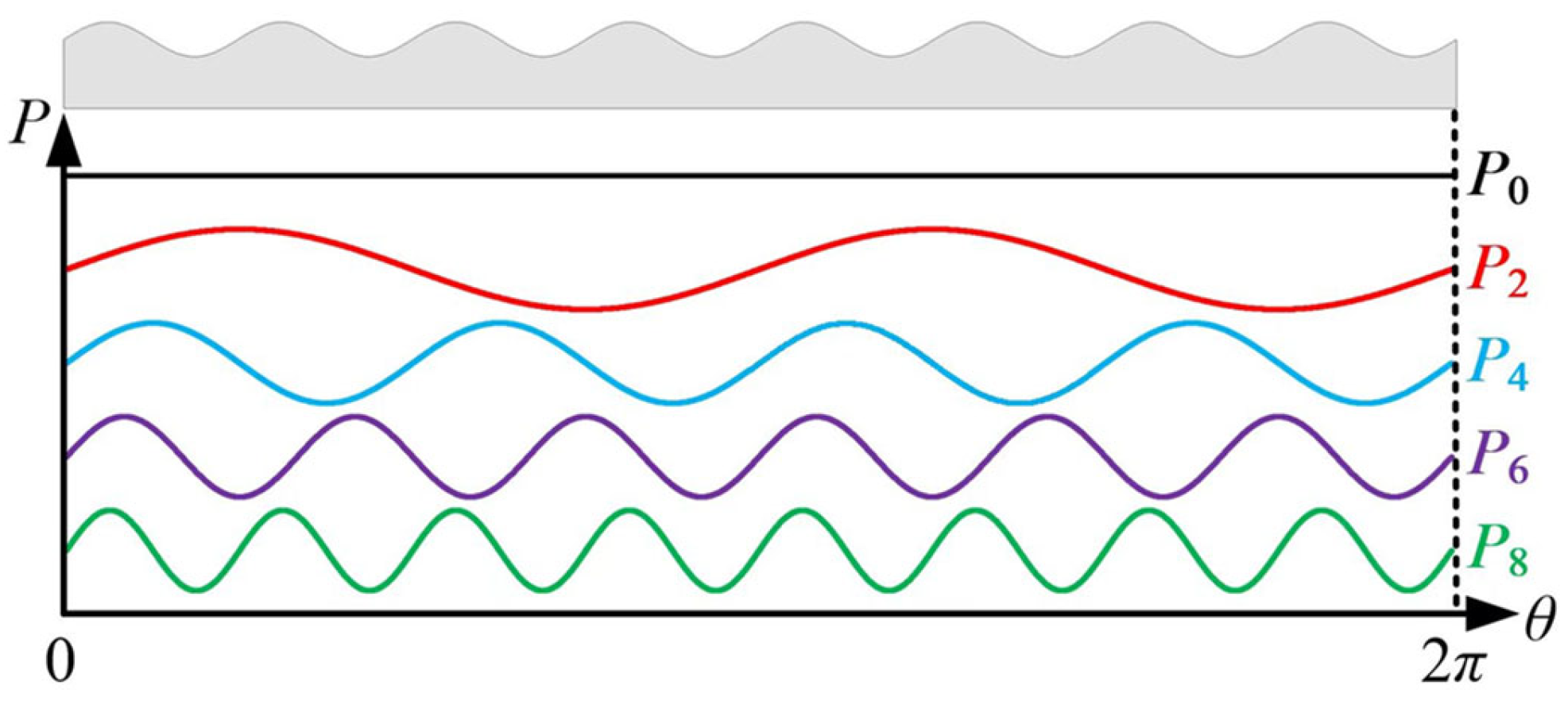
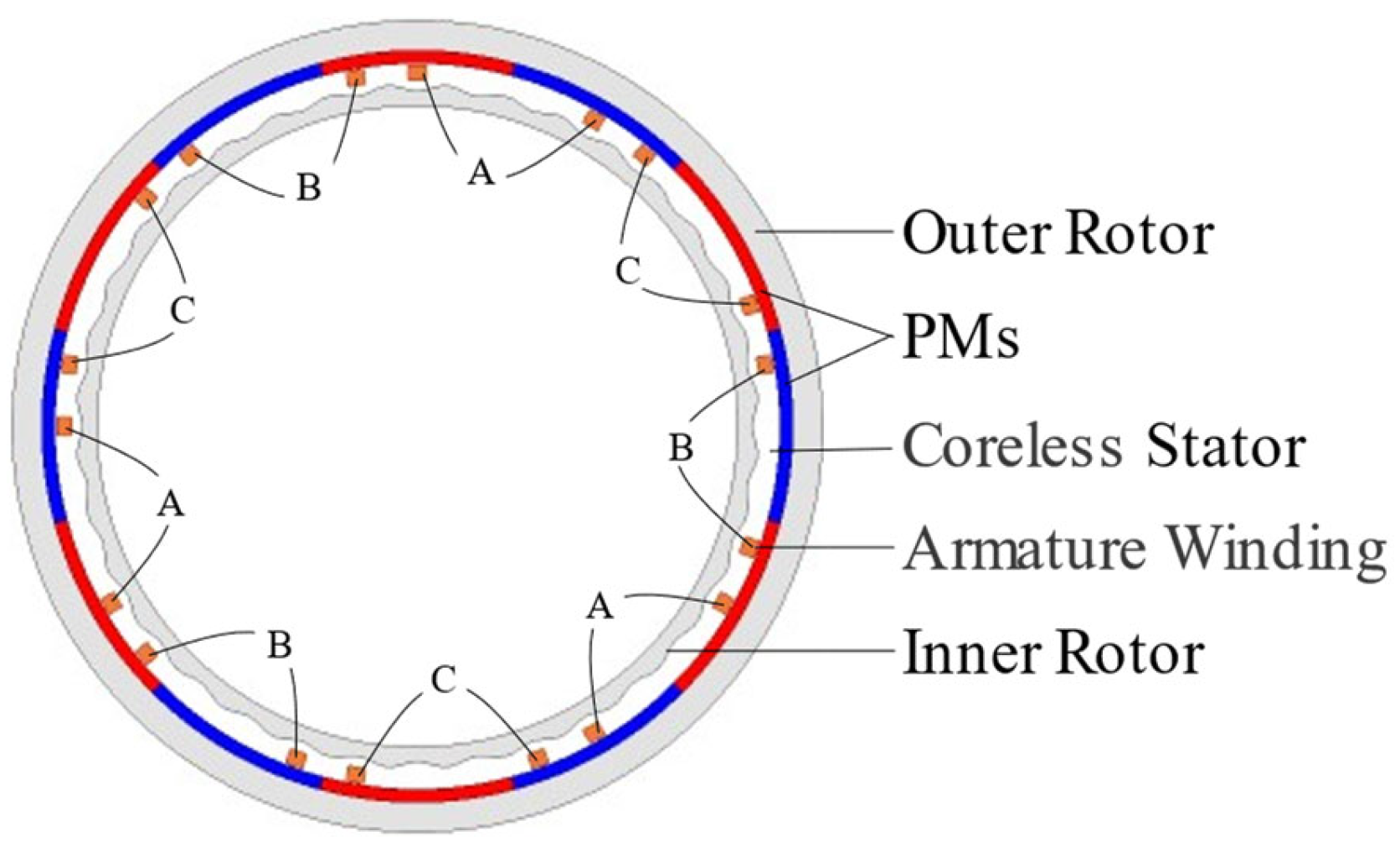
2. Topology and Principle of Proposed Inner Rotor Shape
3. Influence of Injected Harmonics on Machine Performance
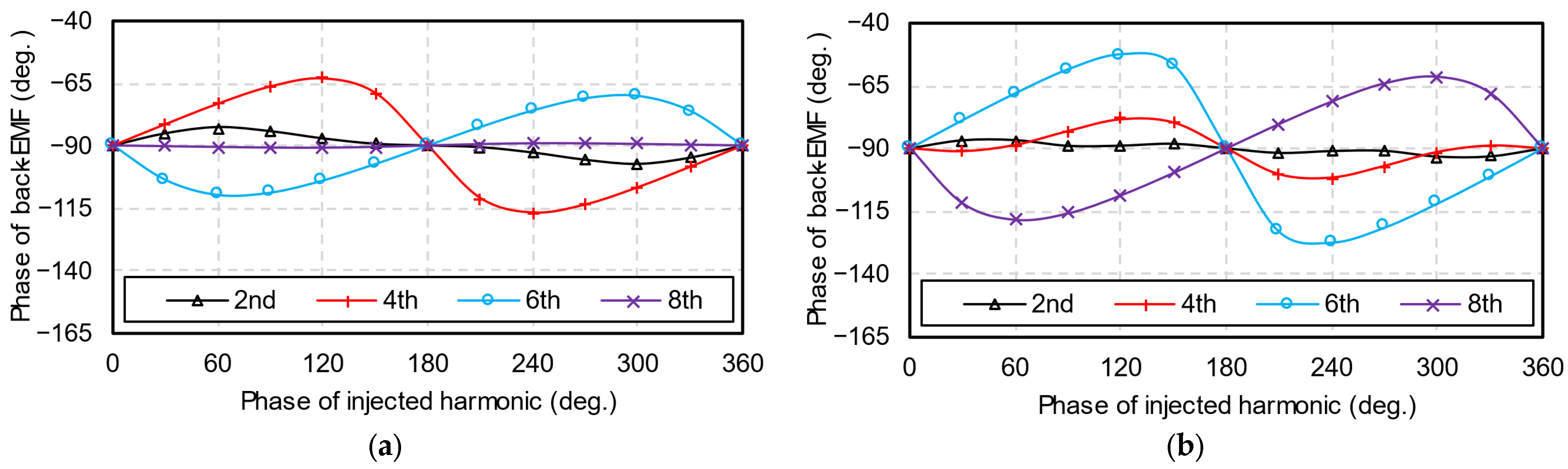
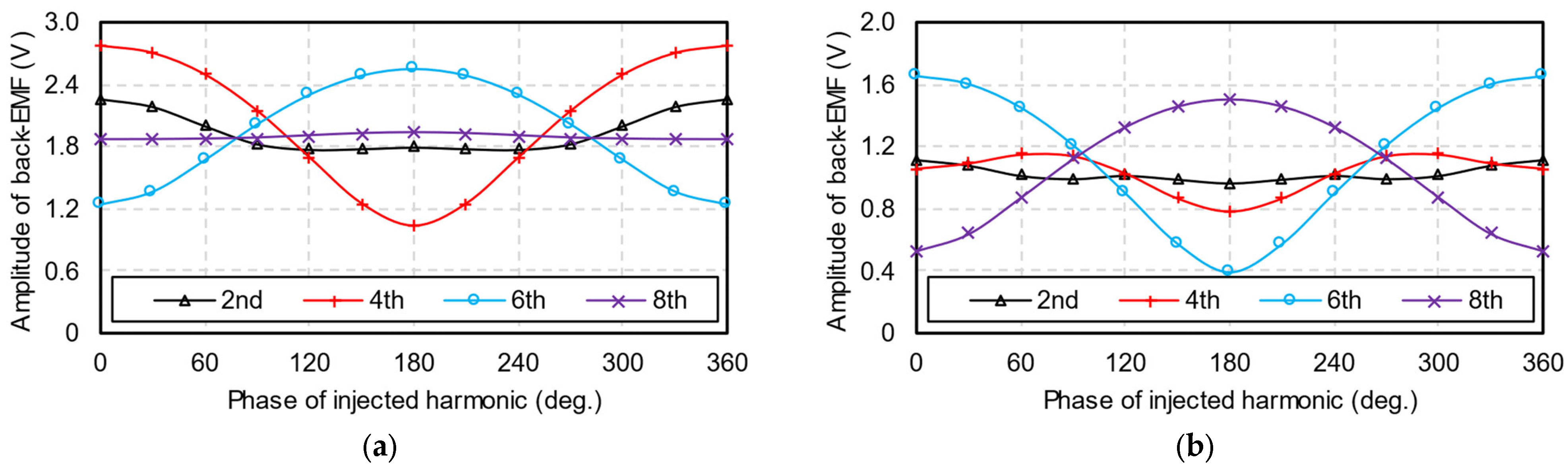
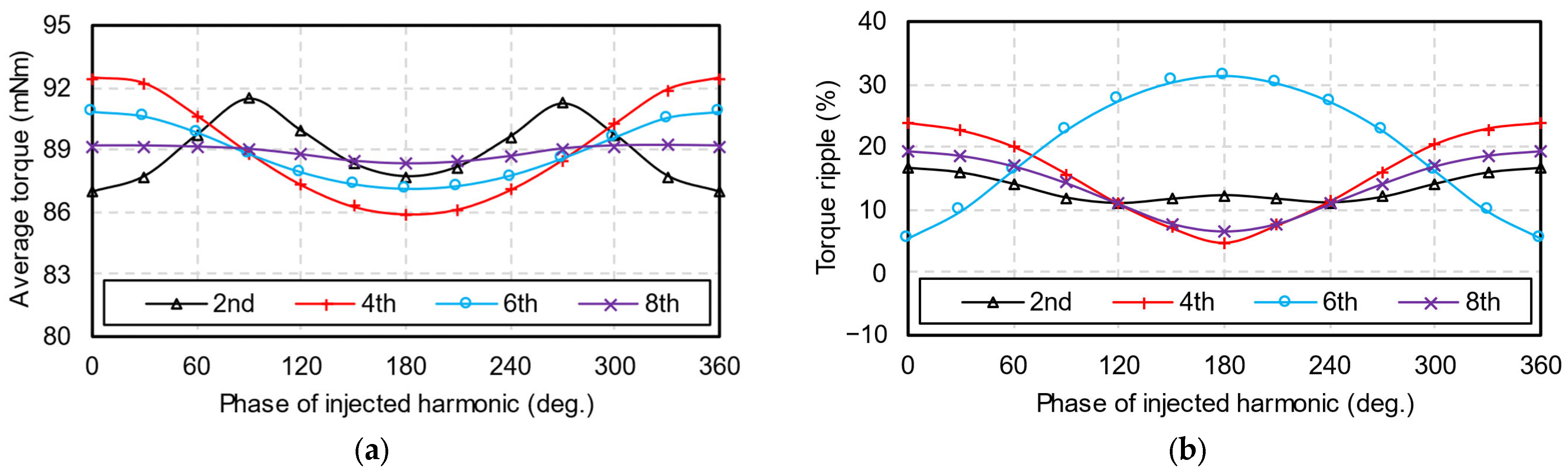
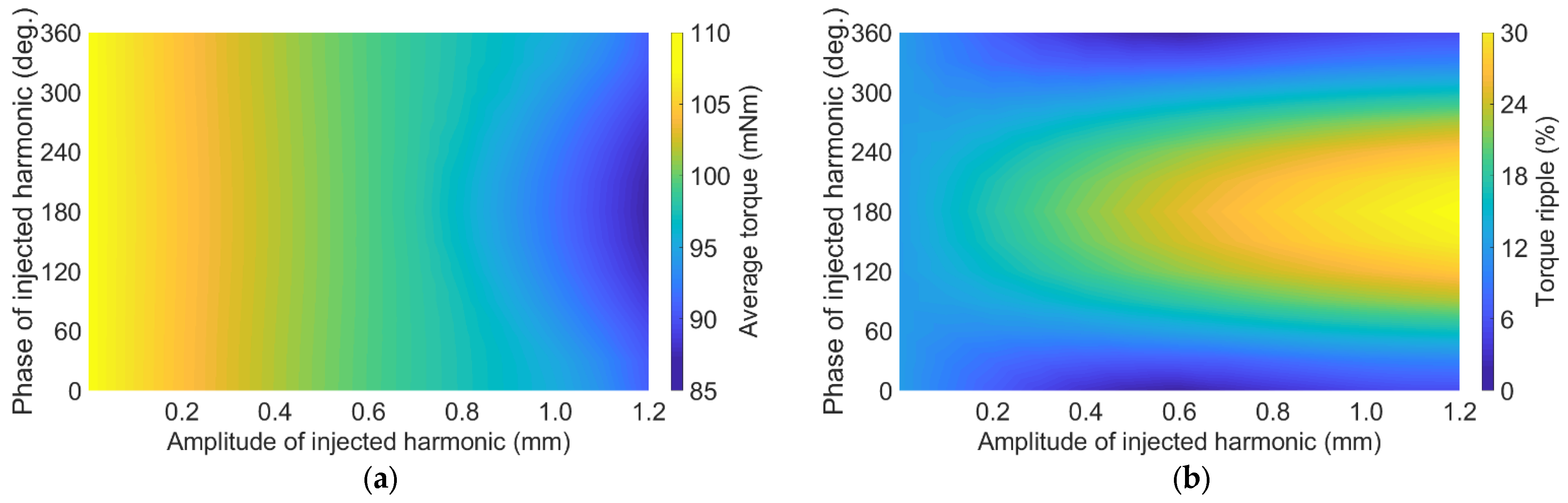
3.1. Phases of Injected Harmonics
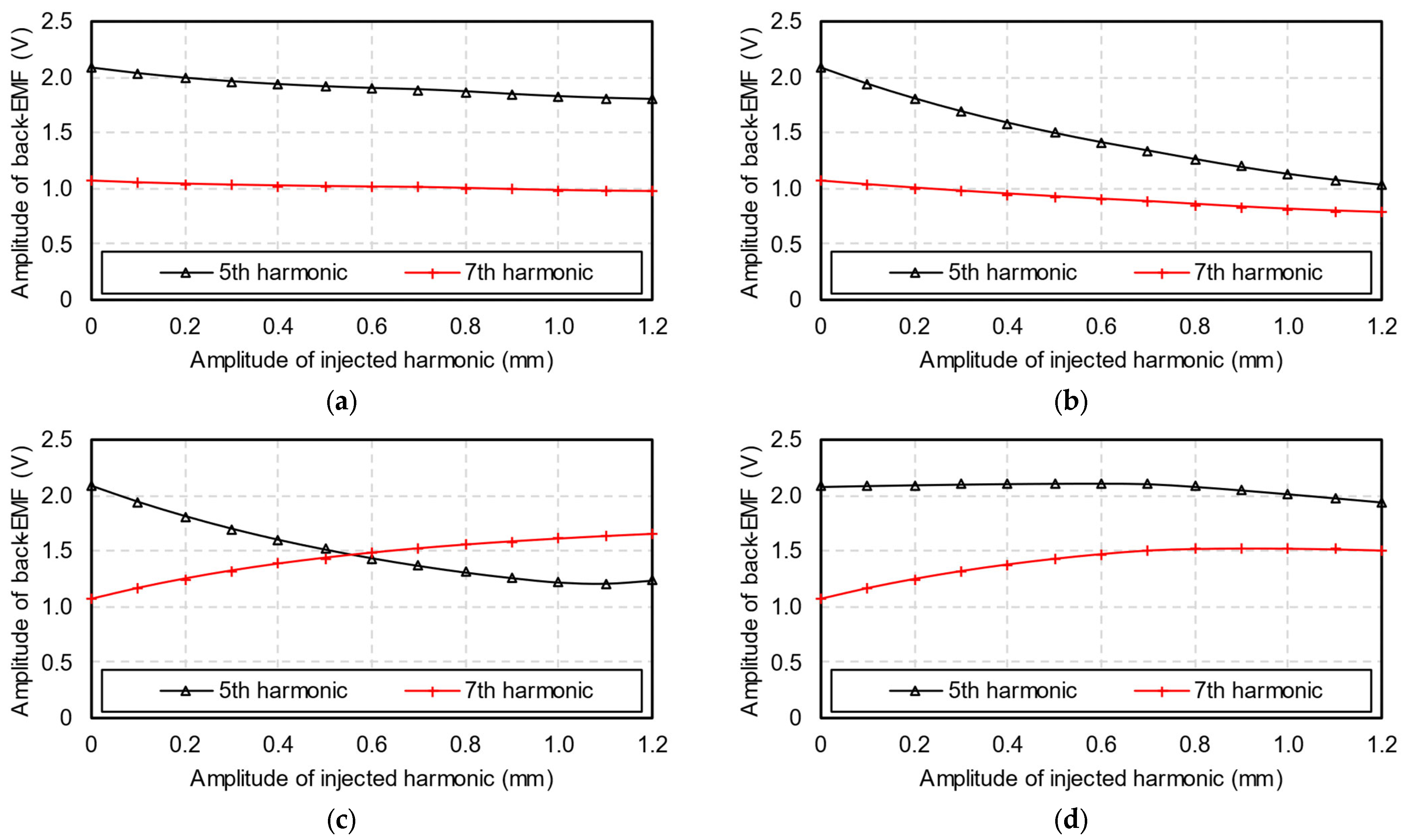
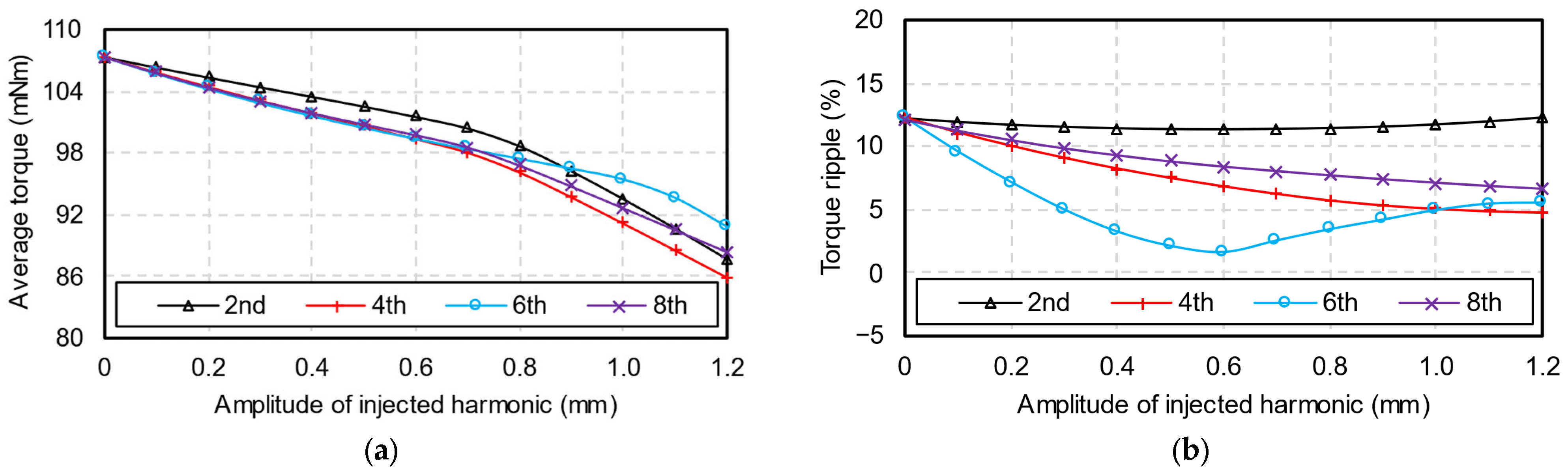
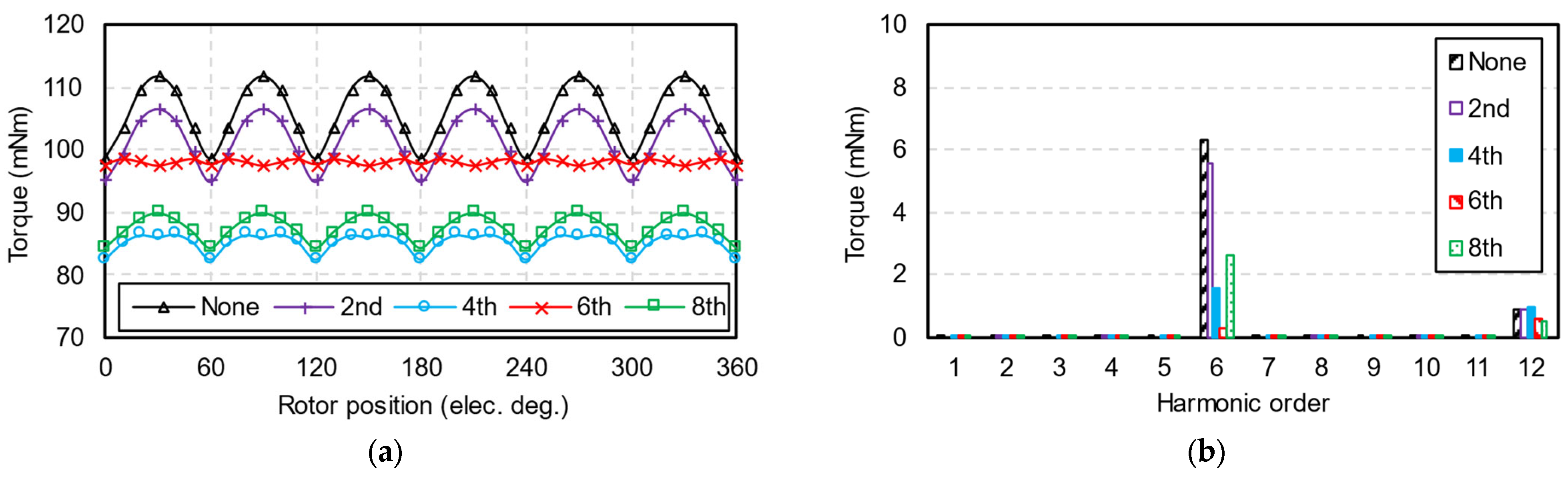
3.2. Amplitudes of Injected Harmonics
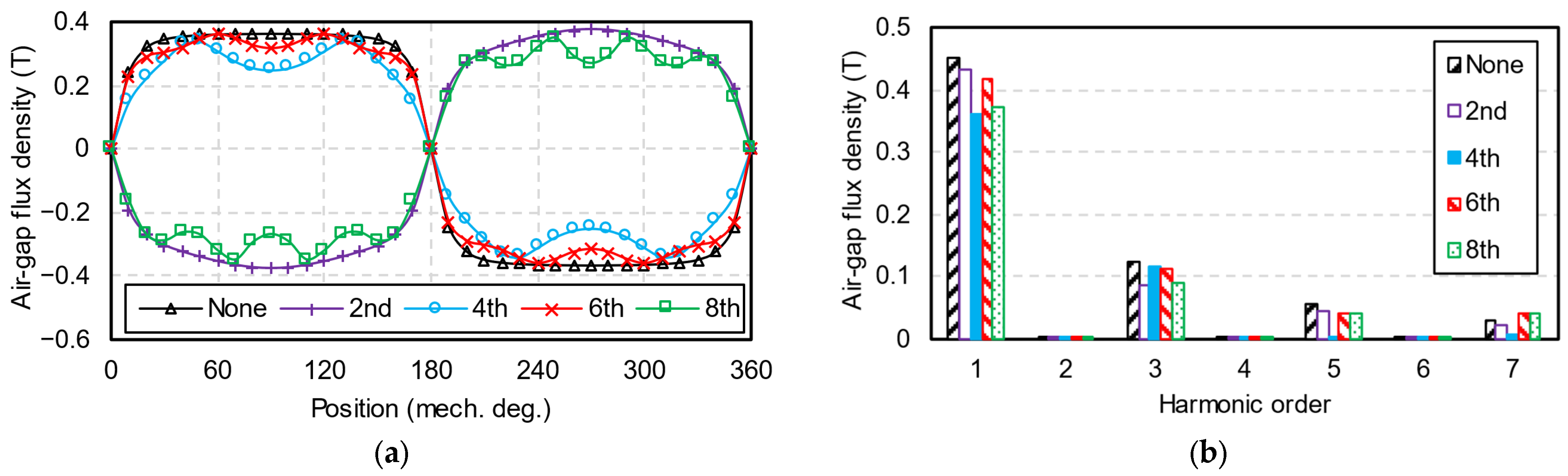
3.3. Best Injected Harmonic
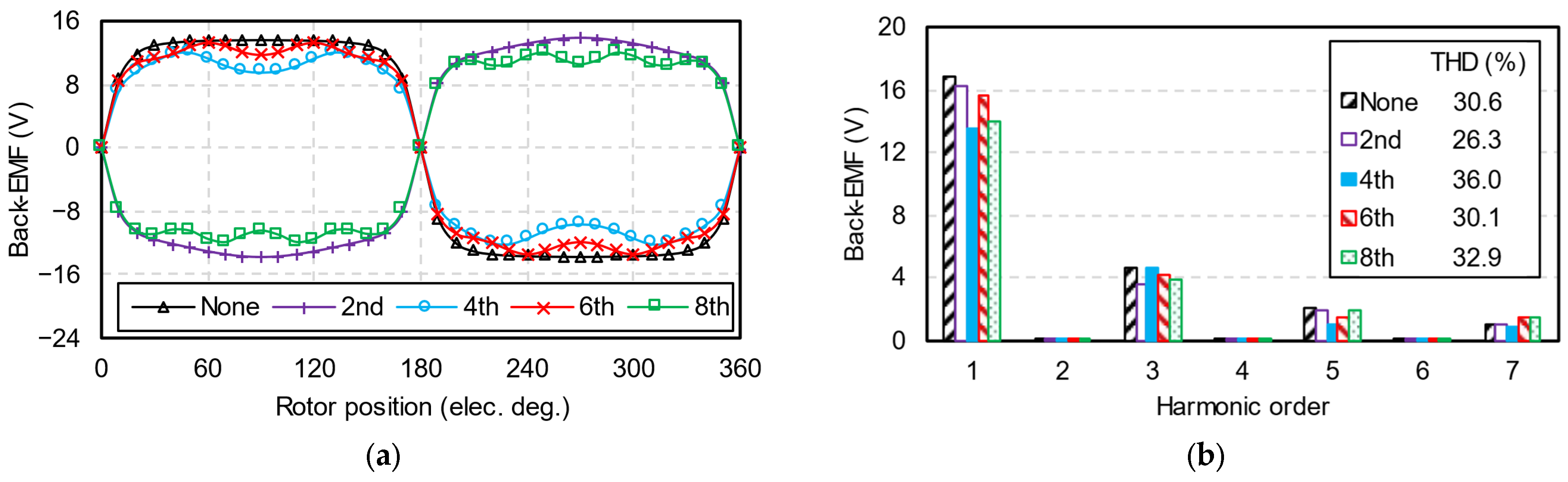
4. Electromagnetic Performance Comparison
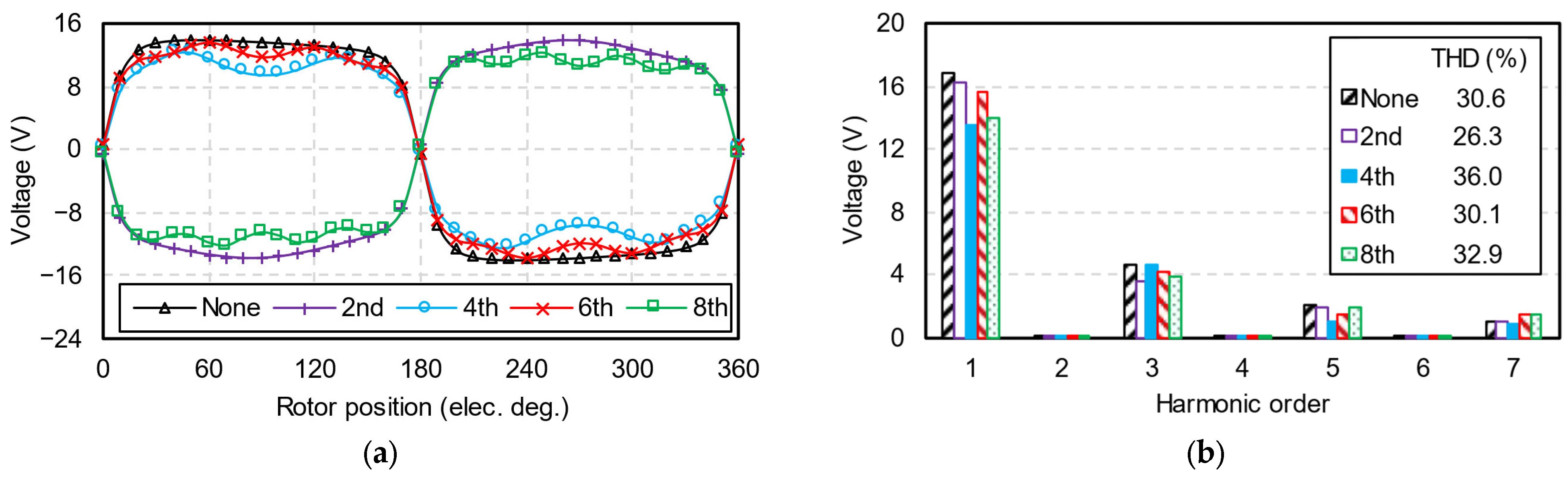
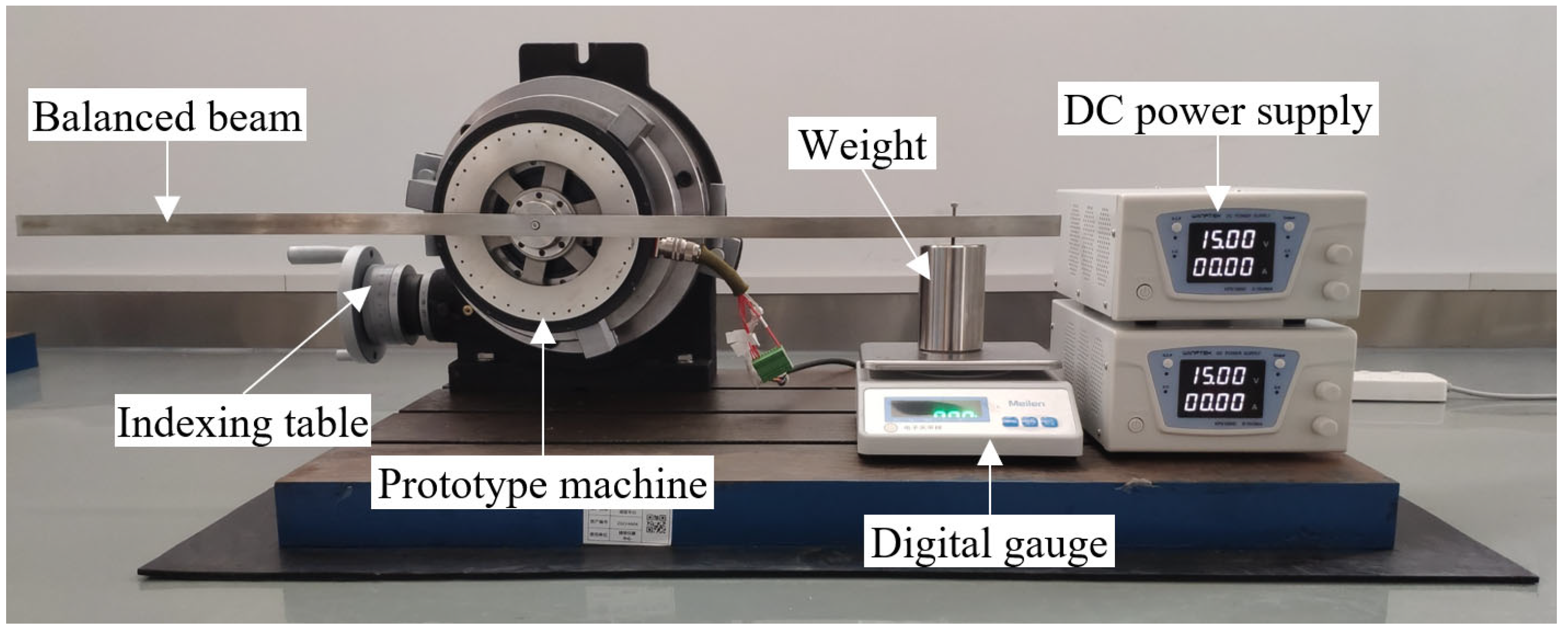
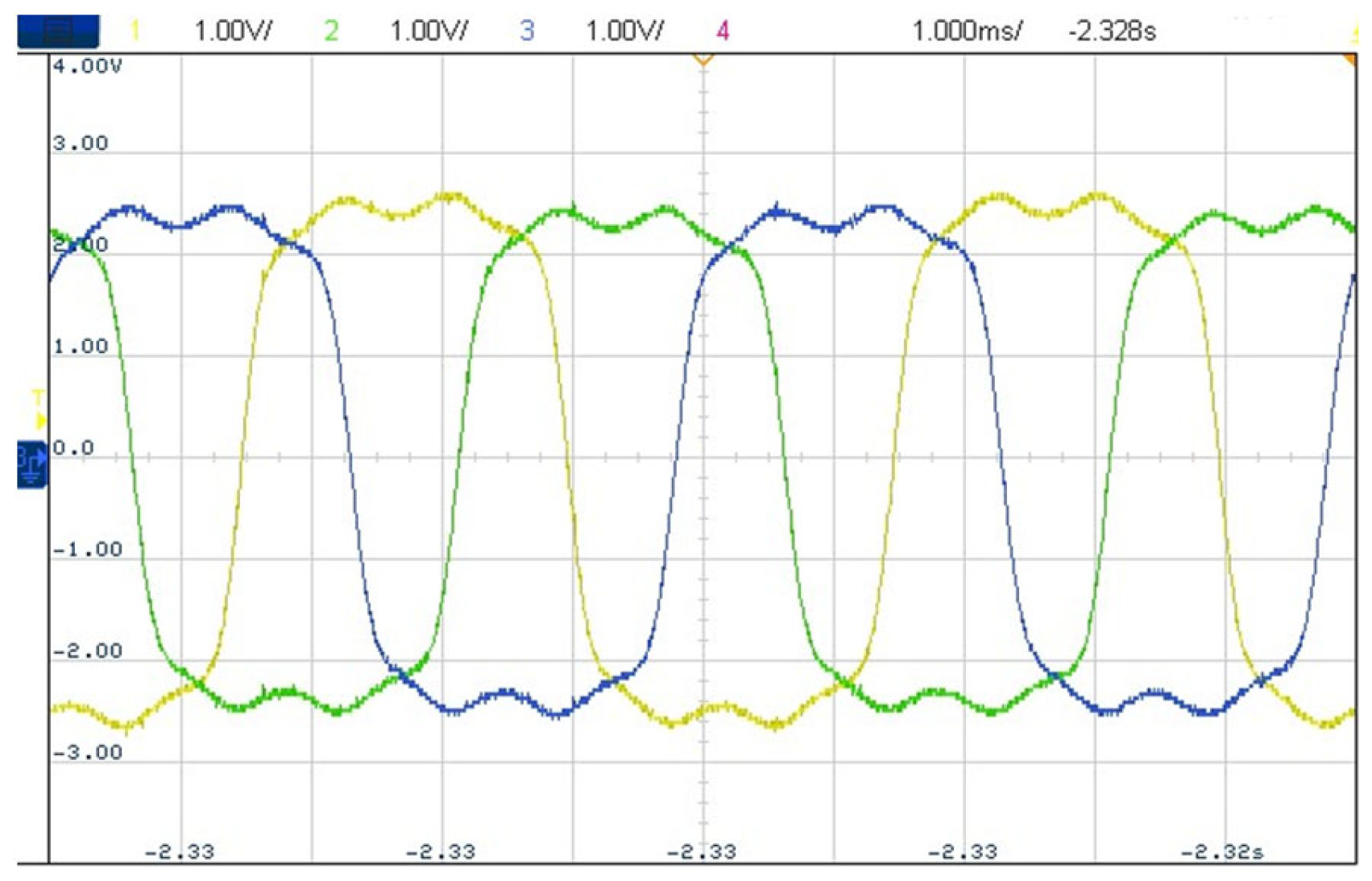
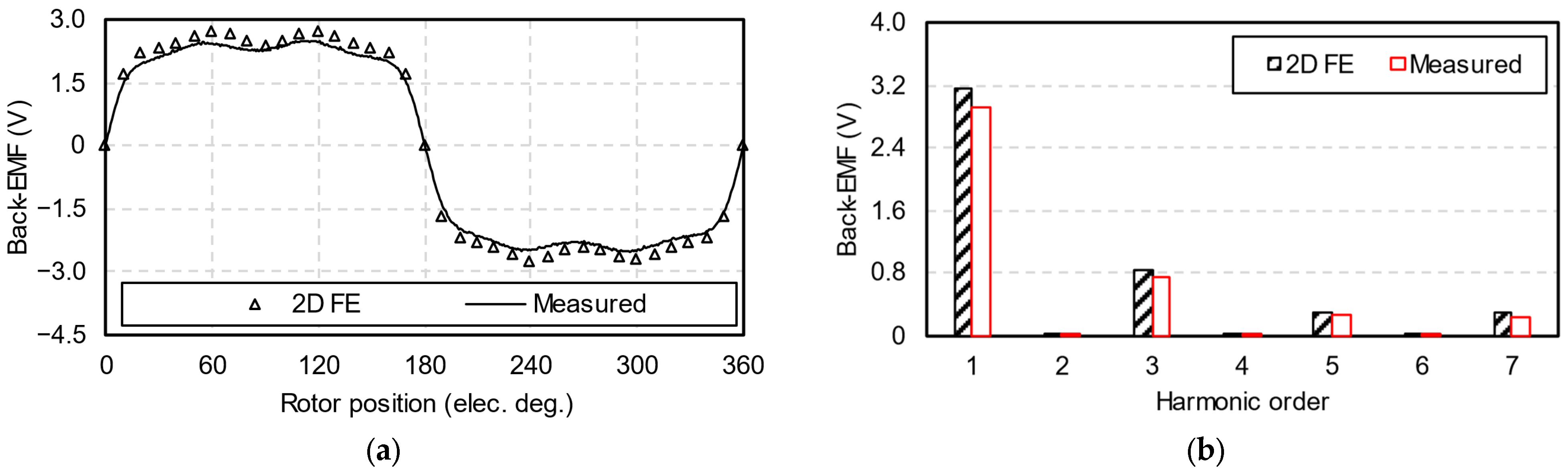
5. Experimental Validation
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Peng, C.; Fang, J.C.; Cui, P.L. Dynamics modeling and measurement of the microvibrations for a magnetically suspended flywheel. IEEE Trans. Instrum. Meas. 2015, 64, 3239–3252. [Google Scholar] [CrossRef]
- Liu, Q.; Wang, K.; Ren, Y.; Chen, X.C.; Ma, L.M.; Zhao, Y. Optimization design of launch locking protective device (LLPD) based on carbon fiber bracket for magnetically suspended flywheel (MSFW). Acta Astronaut. 2019, 154, 9–17. [Google Scholar]
- Tang, D.Y.; Gong, M.T.; Tian, L.M.; Yu, J.S.; Zhang, J.Y.; Zhang, Q. Health indicator construction of high-speed rotating bearings in aerospace CMG based on physics-inspired machine-learning approach. IEEE Trans. Instrum. Meas. 2022, 71, 3524411. [Google Scholar] [CrossRef]
- Sun, J.J.; Xing, G.; Zhou, X.X.; Sun, H.X. Static magnetic field analysis of hollow-cup motor model and bow-shaped permanent magnet design. Chin. J. Aeronaut. 2022, 35, 306–313. [Google Scholar] [CrossRef]
- Sun, J.J.; Ren, J.Y.; Sun, H.X. A novel design of an inner rotor for optimizing the air-gap magnetic field of hollow-cup motors. Machines 2022, 10, 314. [Google Scholar] [CrossRef]
- Zhu, X.F.; Hua, W.; Wu, Z.Z.; Huang, W.T.; Zhang, H.L.; Cheng, M. Analytical approach for cogging torque reduction in flux-switching permanent magnet machines based on magnetomotive force-permeance model. IEEE Trans. Ind. Electron. 2018, 65, 1965–1979. [Google Scholar] [CrossRef]
- Gao, Y.T.; Qu, R.H.; Li, D.W.; Li, J. Torque performance analysis of three-phase flux reversal machines. IEEE Trans. Ind. Appl. 2017, 53, 2110–2119. [Google Scholar] [CrossRef]
- Zhang, L.; Wu, L.J.; Wen, H.; Niu, F.; Lu, Q.F. Improved primary/secondary pole number combinations for dual-armature linear switched flux permanent magnet machines. IEEE Trans. Transport. Electrif. 2021, 7, 2589–2599. [Google Scholar] [CrossRef]
- Qi, J.; Zhu, Z.Q.; Yan, L.C.; Jewell, G.W.; Gan, C.W.; Ren, Y.; Brockway, S.; Hilton, C. Suppression of torque ripple for consequent pole PM machine by asymmetric pole shaping method. IEEE Trans. Ind. Appl. 2022, 58, 3545–3557. [Google Scholar] [CrossRef]
- Zhang, X.T.; Zhang, C.M.; Yu, J.K.; Du, P.C.; Li, L.Y. Analytical model of magnetic field of a permanent magnet synchronous motor with a trapezoidal Halbach permanent magnet array. IEEE Trans. Magn. 2019, 55, 8105205. [Google Scholar] [CrossRef]
- Zhang, L.F.; Wang, K.; Sun, H.Y.; Zhu, S.S. Multiphase PM machines with Halbach array considering third harmonic flux density. IEEE Trans. Ind. Electron. 2019, 66, 9184–9193. [Google Scholar] [CrossRef]
- Liu, K.; Yin, M.; Hua, W.; Ma, Z.Q.; Lin, M.Y.; Kong, Y. Design and analysis of Halbach ironless flywheel BLDC motor/generators. IEEE Trans. Magn. 2018, 54, 8109305. [Google Scholar] [CrossRef]
- Wang, Z.Y.; Yang, J.T.; Dai, S.R.; Feng, Y.J.; Huang, S.D. Novel dual-rotor single-stator coreless permanent magnet machine with dual-flywheel. IEEE Trans. Magn. 2022, 58, 8106506. [Google Scholar] [CrossRef]
- Xia, C.L.; Guo, L.Y.; Wang, H.M. Modeling and analyzing of magnetic field of segmented Halbach array permanent magnet machine considering gap between segments. IEEE Trans. Magn. 2014, 50, 8106009. [Google Scholar] [CrossRef]
- Li, Y.; Xing, J.W.; Wang, T.B.; Lu, Y.P. Programmable design of magnet shape for permanent-magnet synchronous motors with sinusoidal back EMF waveforms. IEEE Trans. Magn. 2008, 44, 2163–2167. [Google Scholar]
- Zhou, Y.; Li, H.S.; Meng, G.W.; Zhou, S.; Cao, Q. Analytical calculation of magnetic field and cogging torque in surface-mounted permanent-magnet machines accounting for any eccentric rotor shape. IEEE Trans. Ind. Electron. 2015, 62, 3438–3447. [Google Scholar] [CrossRef]
- Chen, Z.F.; Xia, C.L.; Geng, Q.; Yan, Y. Modeling and analyzing of surface-mounted permanent-magnet synchronous machines with optimized magnetic pole shape. IEEE Trans. Magn. 2014, 50, 8102804. [Google Scholar] [CrossRef]
- Zeng, Y.; Cheng, M.; Liu, G.H.; Zhao, W.X. Effects of magnet shape on torque capability of surface-mounted permanent magnet machine for servo applications. IEEE Trans. Ind. Electron. 2020, 67, 2977–2990. [Google Scholar] [CrossRef]
- Du, Z.S.; Lipo, T.A. High torque density and low torque ripple shaped-magnet machines using sinusoidal plus third harmonic shaped magnets. IEEE Trans. Ind. Appl. 2019, 55, 2601–2610. [Google Scholar] [CrossRef]
- Park, J.C.; Kim, J.H.; Park, S.H.; Kim, K.O.; Sung, M.H.; Lim, M.S. Design optimization using asymmetric rotor in IPMSM for torque ripple reduction considering forward and reverse directions. IEEE Trans. Magn. 2023, 59, 8205805. [Google Scholar] [CrossRef]
- Shimizu, Y.; Morimoto, S.; Sanada, M.; Inoue, Y. Investigation of rotor topologies for reducing torque ripple in double-layer IPMSMs for automotive applications. IEEE Trans. Ind. Electron. 2023, 70, 8276–8285. [Google Scholar] [CrossRef]
- Xu, Y.M.; Xu, Z.Y.; Cao, H.P.; Liu, W.H. Torque ripple suppression of synchronous reluctance motors for electric vehicles based on rotor improvement design. IEEE Trans. Transport. Electrific. 2023, 9, 4328–4338. [Google Scholar] [CrossRef]
- Mohammadi, S.E.M.; Chen, P.Y.; Moallem, M.; Fahimi, B.; Kiani, M. An alternate rotor geometry for switched reluctance machine with reduced torque ripple. IEEE Trans. Energy Convers. 2023, 38, 939–947. [Google Scholar] [CrossRef]
- Wu, Z.Z.; Zhu, Z.Q. Analysis of air-gap field modulation and magnetic gearing effects in switched flux permanent magnet machines. IEEE Trans. Magn. 2015, 51, 8105012. [Google Scholar] [CrossRef]
- Zhu, Z.Q. A simple method for measuring cogging torque in permanent magnet machines. In Proceedings of the 2009 IEEE Power & Energy Society General Meeting, Calgary, AB, Canada, 26–30 July 2009. [Google Scholar]






| Harmonic Order of Air-Gap MMF | 1 | 3 | 5 | 7 | |
|---|---|---|---|---|---|
| Harmonic Order of Air-Gap Permeance | |||||
| 0 | 1 | 3 | 5 | 7 | |
| 1 | 0, 2 | 2, 4 | 4, 6 | 6, 8 | |
| 2 | 1, 3 | 1, 5 | 3, 7 | 5, 9 | |
| 3 | 2, 4 | 0, 6 | 2, 8 | 4, 10 | |
| 4 | 3, 5 | 1, 7 | 1, 9 | 3, 11 | |
| 5 | 4, 6 | 2, 8 | 0, 10 | 2, 12 | |
| 6 | 5, 7 | 3, 9 | 1, 11 | 1, 13 | |
| 7 | 6, 8 | 4, 10 | 2, 12 | 0, 14 | |
| 8 | 7, 9 | 5, 11 | 3, 13 | 1, 15 | |
| Parameter | Unit | Value |
|---|---|---|
| Number of poles | - | 12 |
| Number of slots | - | 9 |
| Rated speed | rpm | 10,000 |
| Rated phase current | Arms | 3.1 |
| Active axial length | mm | 6 |
| Outer radius of outer rotor | mm | 81 |
| Thickness of outer rotor core | mm | 6 |
| Thickness of PM | mm | 2.5 |
| Pole-arc to pole-pitch ratio of PM | - | 1 |
| Maximum thickness of inner rotor core | mm | 4.2 |
| Item | Unit | Injected Harmonic Order | |||
|---|---|---|---|---|---|
| 2nd | 4th | 6th | 8th | ||
| Amplitude | mm | 0.6 | 1.2 | 0.6 | 1.2 |
| Phase | ° | 180 | 180 | 0 | 180 |
| Item | Unit | Injected Harmonic Order | ||||
|---|---|---|---|---|---|---|
| None | 2nd | 4th | 6th | 8th | ||
| Average torque | mNm | 107 | 101 | 86 | 99 | 88 |
| Peak-to-peak torque | mNm | 13.1 | 11.5 | 4.1 | 1.6 | 5.8 |
| Torque ripple | % | 12.2 | 11.4 | 4.8 | 1.6 | 6.6 |
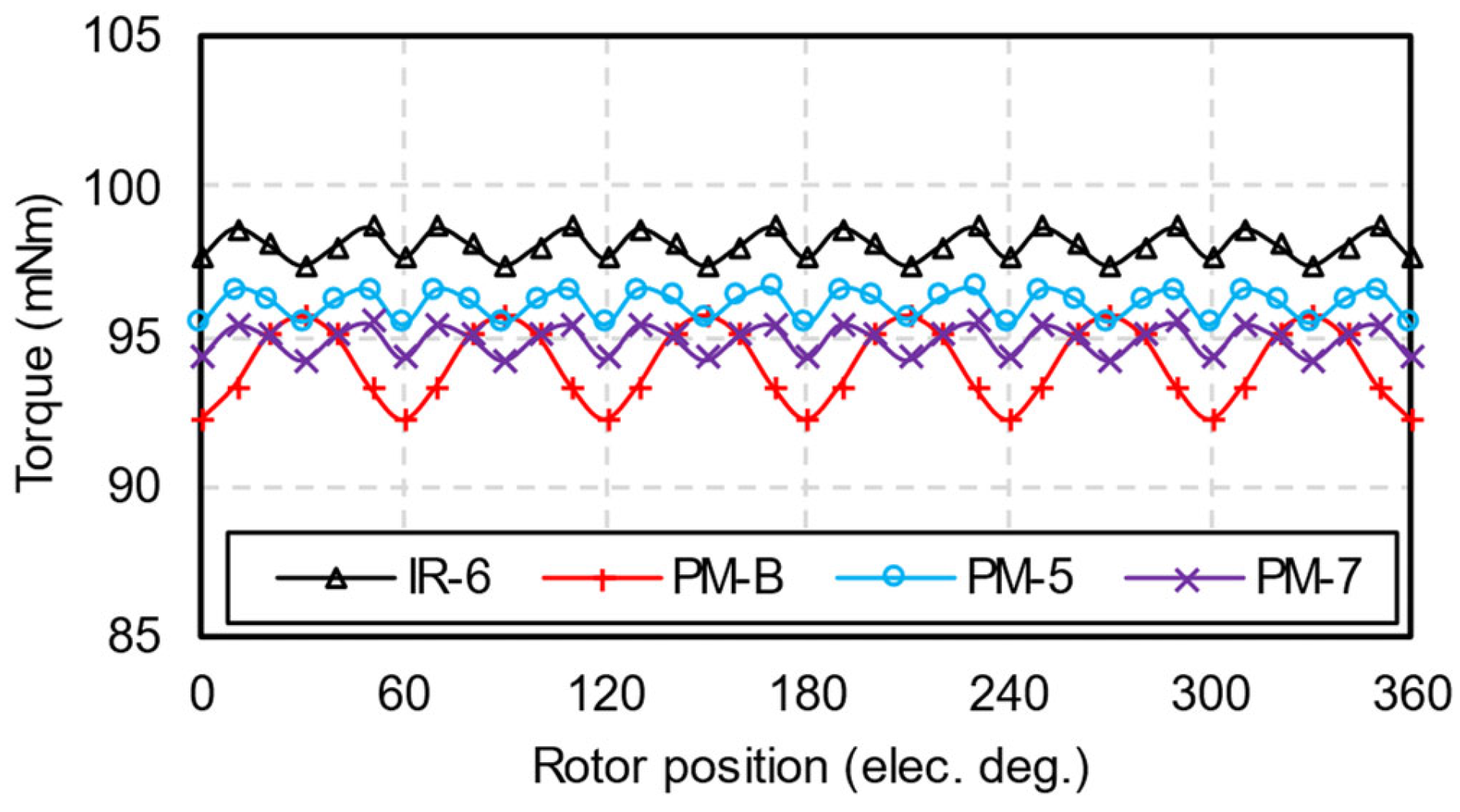
| Method | Average Torque (mNm) | Peak-to-Peak Torque (mNm) | Torque Ripple (%) |
|---|---|---|---|
| IR-6 | 99 | 1.6 | 1.6 |
| PM-B | 94 | 3.6 | 3.8 |
| PM-5 | 96 | 1.3 | 1.4 |
| PM-7 | 95 | 1.4 | 1.5 |
| SS | 97 | 1.5 | 1.5 |
| SP | 97 | 2.2 | 2.3 |
| PMW | 97 | 3.5 | 3.6 |
| IG | 96 | 11.4 | 11.9 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, L.; Cui, Z.; Song, P.; Wang, L.; Liu, X. Sinusoidal Rotor Core Shape for Low Torque Ripple in Hollow-Cup Machines. Energies 2024, 17, 3168. https://doi.org/10.3390/en17133168
Zhang L, Cui Z, Song P, Wang L, Liu X. Sinusoidal Rotor Core Shape for Low Torque Ripple in Hollow-Cup Machines. Energies. 2024; 17(13):3168. https://doi.org/10.3390/en17133168
Chicago/Turabian StyleZhang, Liu, Zhanpeng Cui, Pengfei Song, Liming Wang, and Xikai Liu. 2024. "Sinusoidal Rotor Core Shape for Low Torque Ripple in Hollow-Cup Machines" Energies 17, no. 13: 3168. https://doi.org/10.3390/en17133168
APA StyleZhang, L., Cui, Z., Song, P., Wang, L., & Liu, X. (2024). Sinusoidal Rotor Core Shape for Low Torque Ripple in Hollow-Cup Machines. Energies, 17(13), 3168. https://doi.org/10.3390/en17133168







