Study on the Influences of an Outer-Coreless-Rotor Permanent Magnet Synchronous Machine Using Halbach Magnet Array
Abstract
1. Introduction
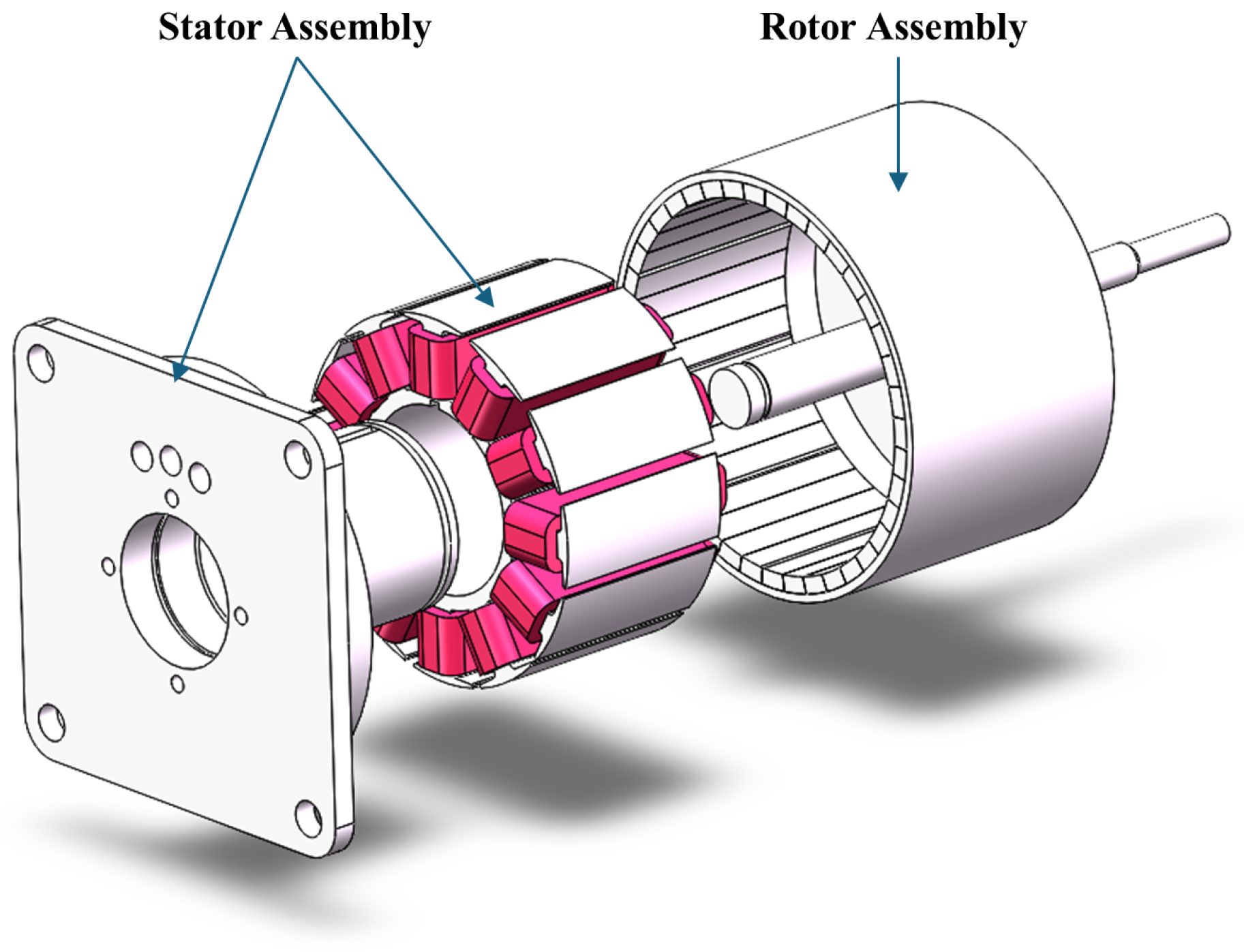
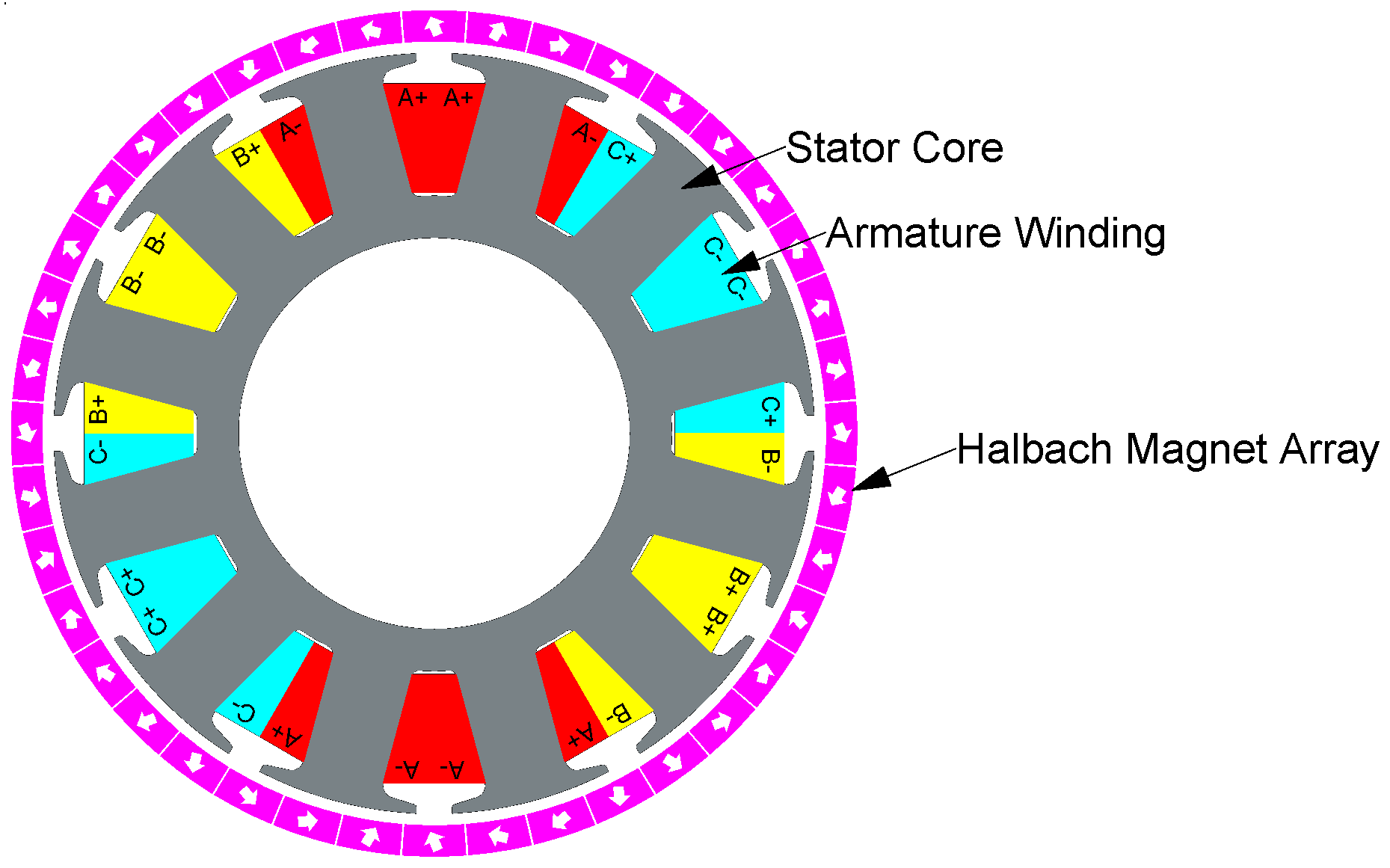
2. Topology and Analytical Model
3. Influences of Halbach Array Parameters on Open-Circuit Phase Back-EMF
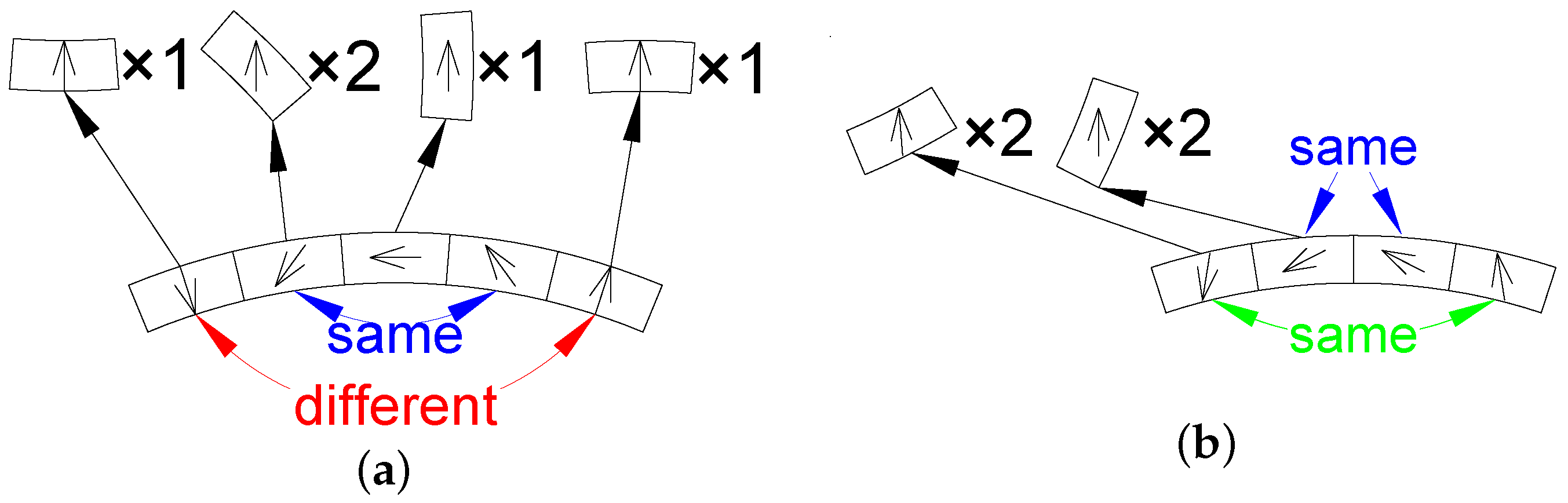
3.1. Influence of Dividing Number per Pole
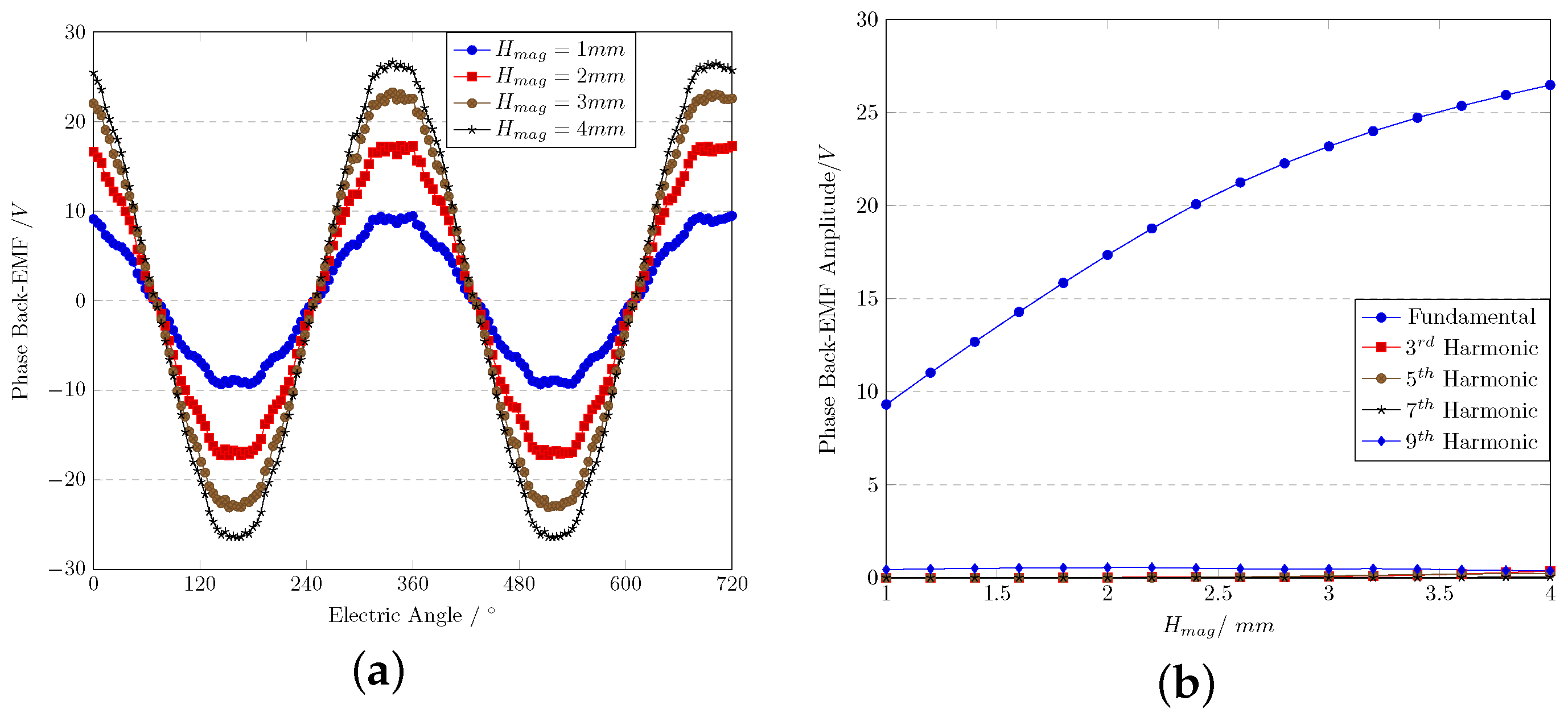
3.2. Influence of Magnet Thickness
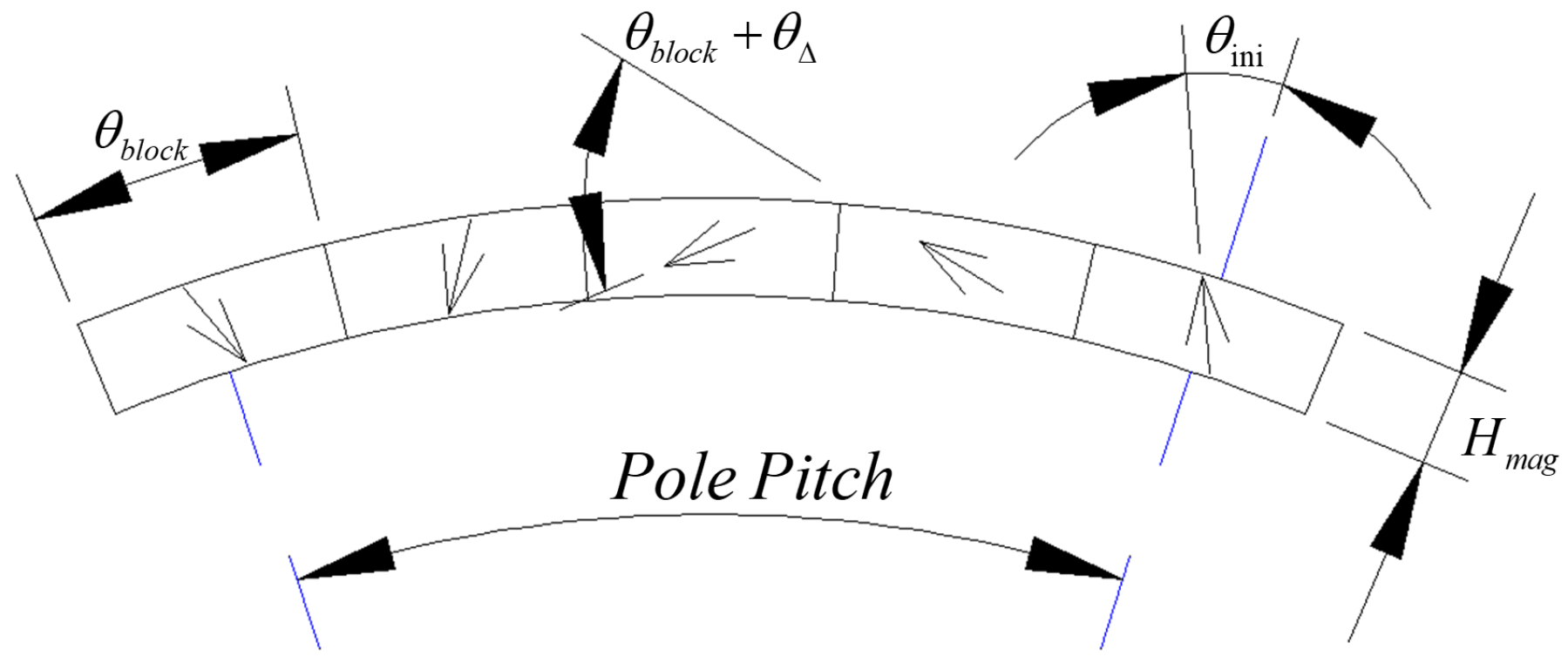
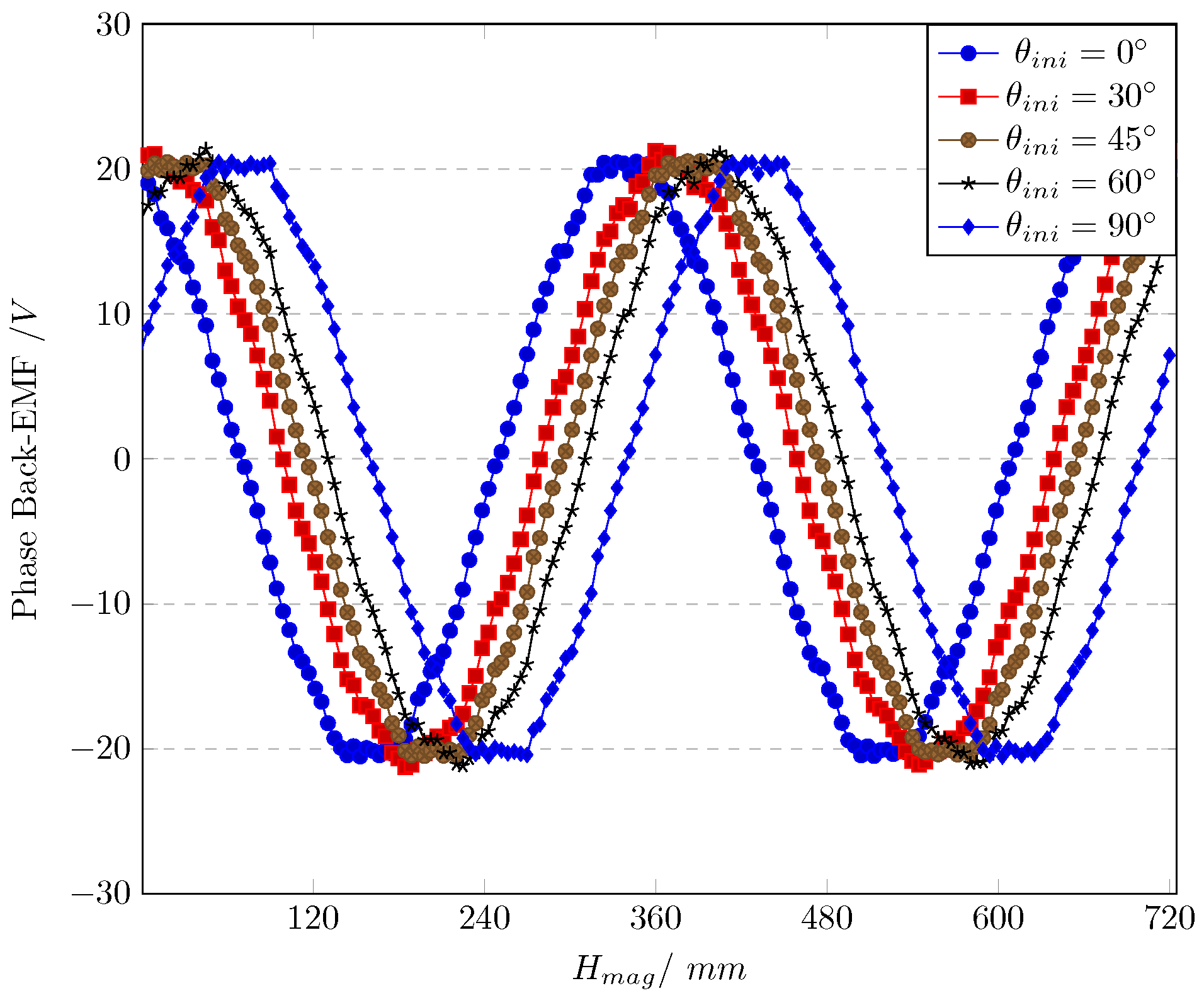
3.3. Influence of Initial Angle and Manufacturing
4. Machine Performance
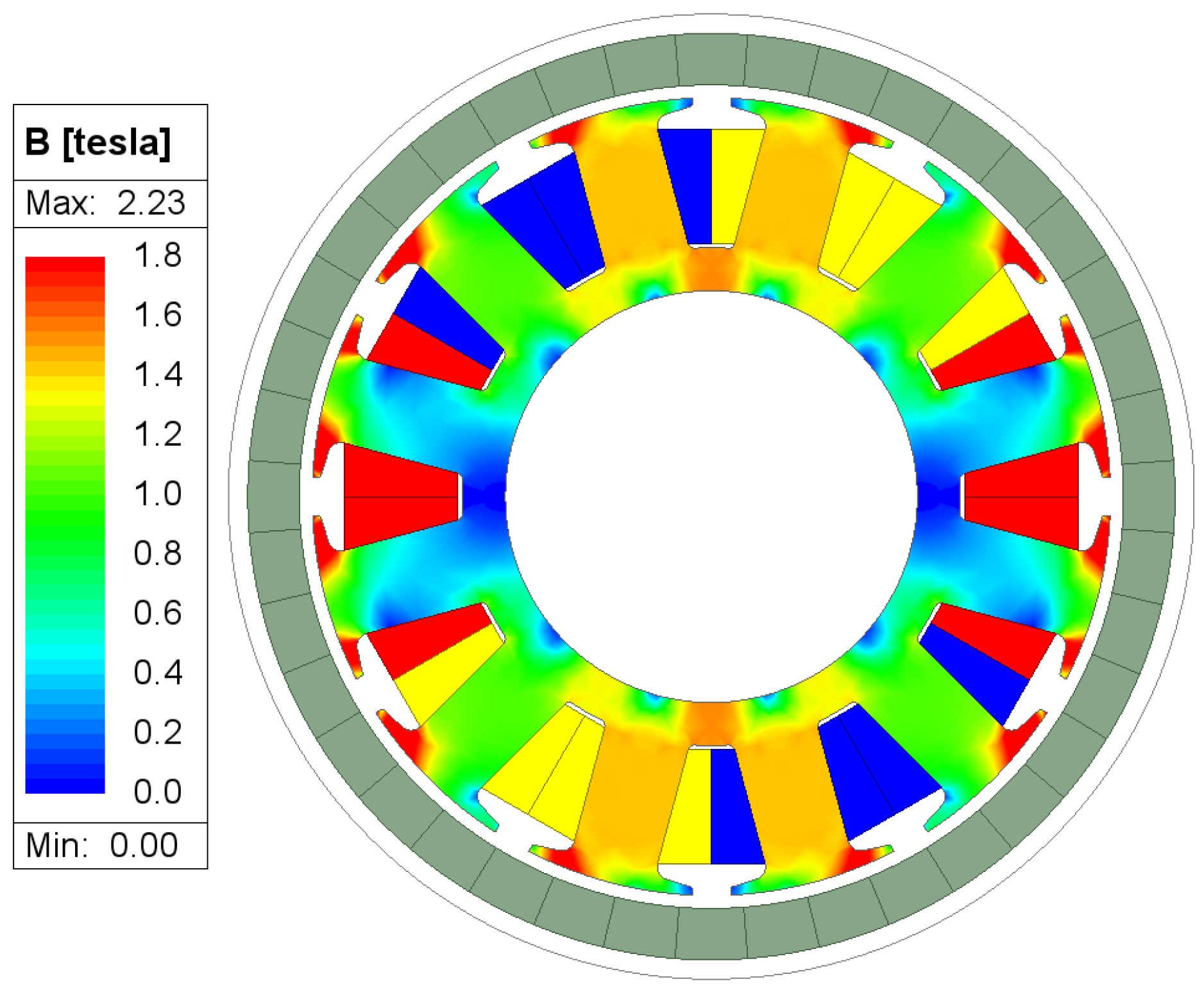
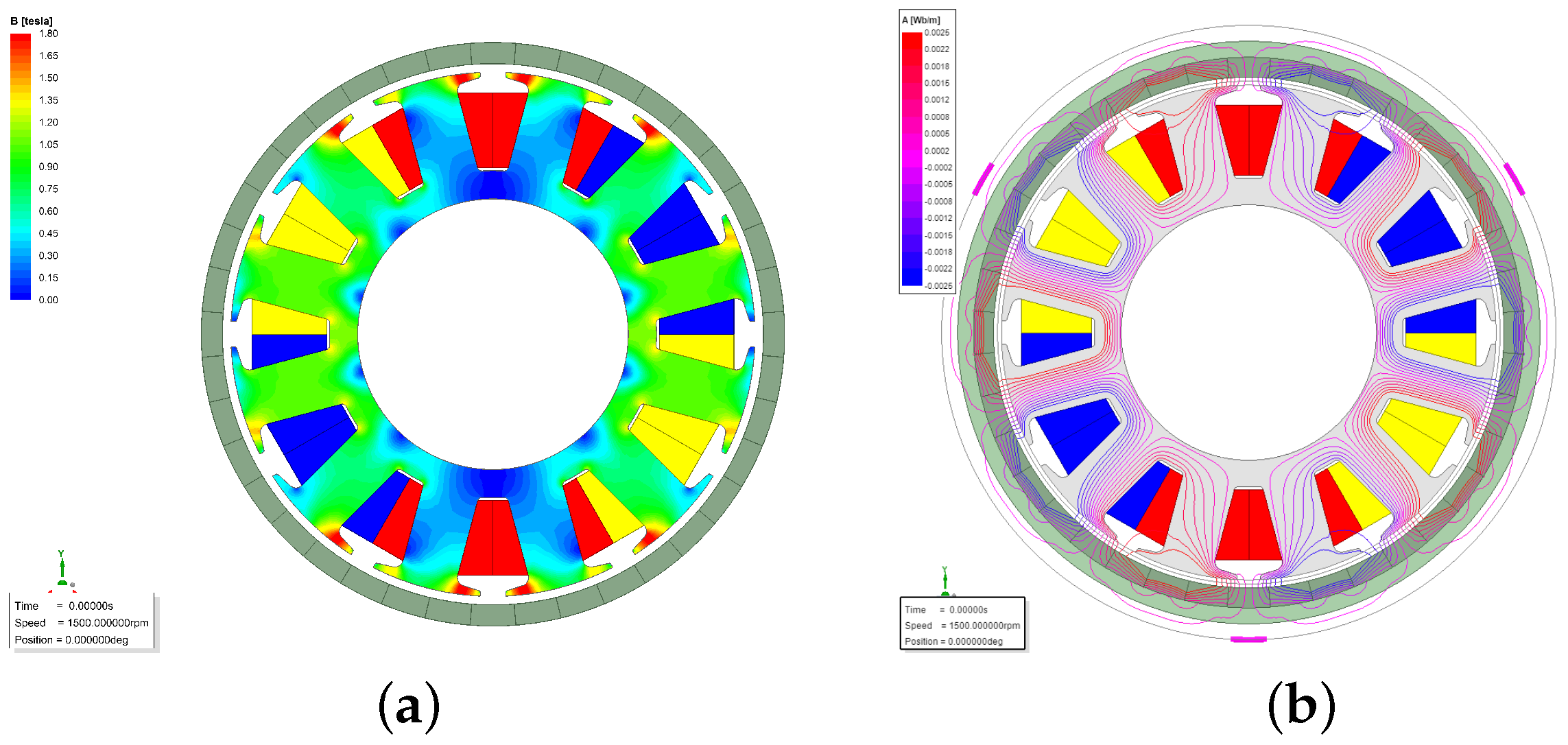
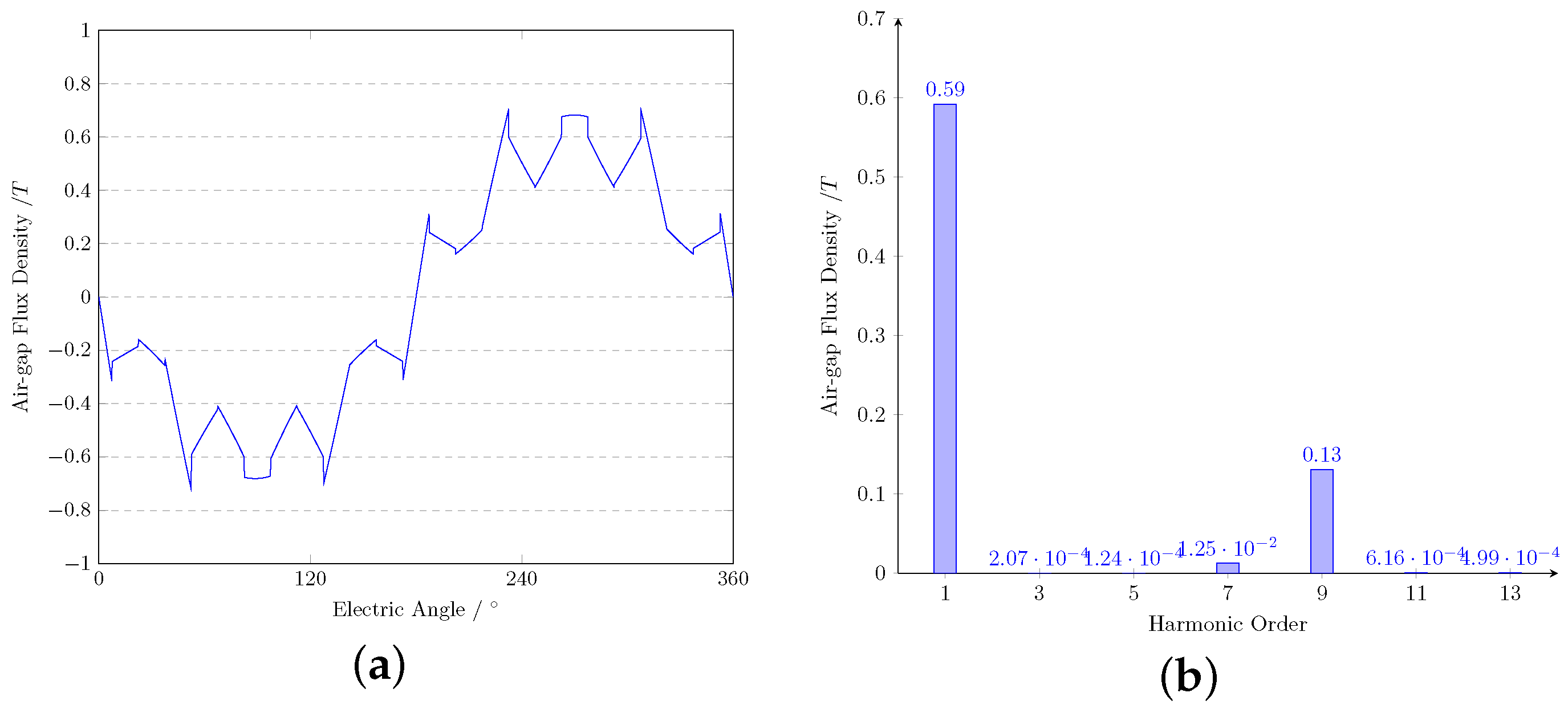
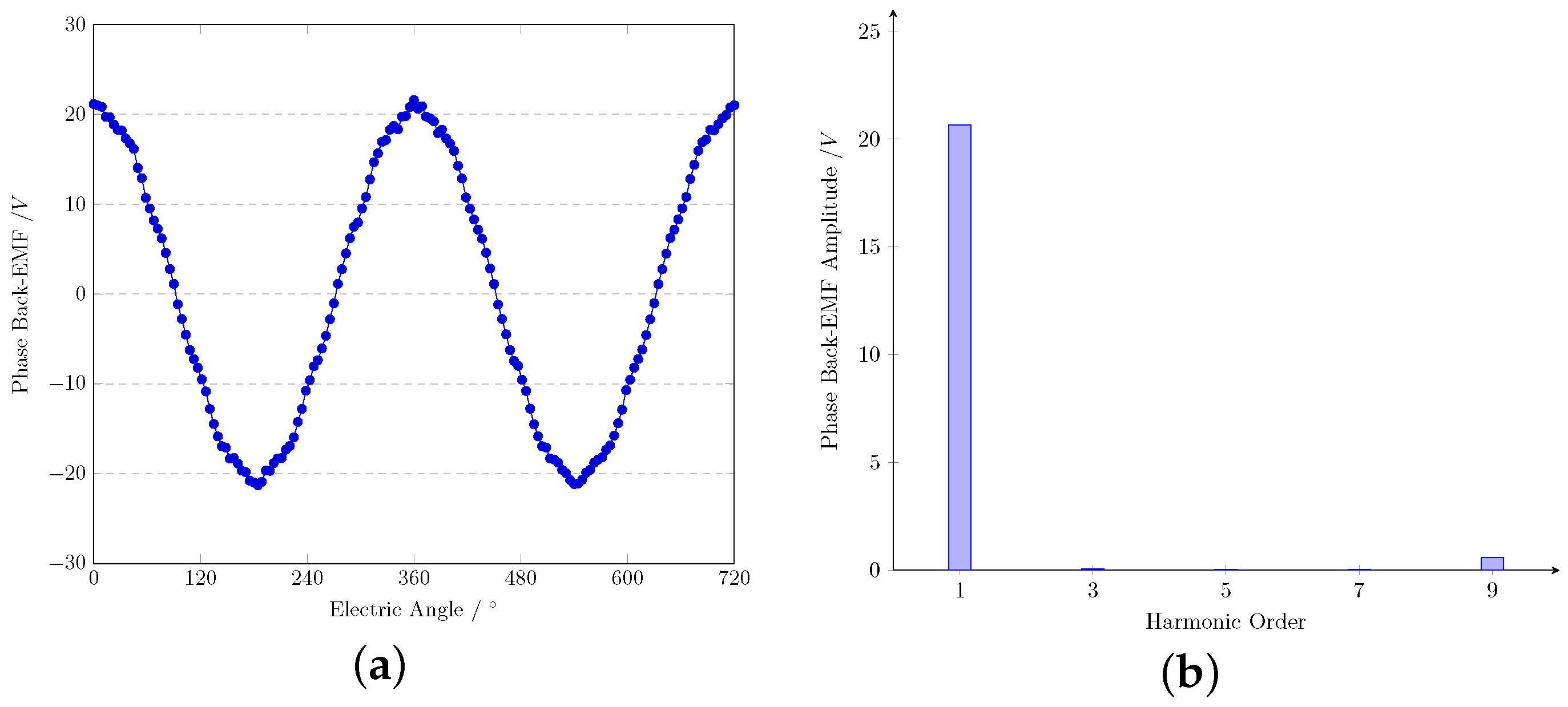
4.1. Open-Circuit Performance
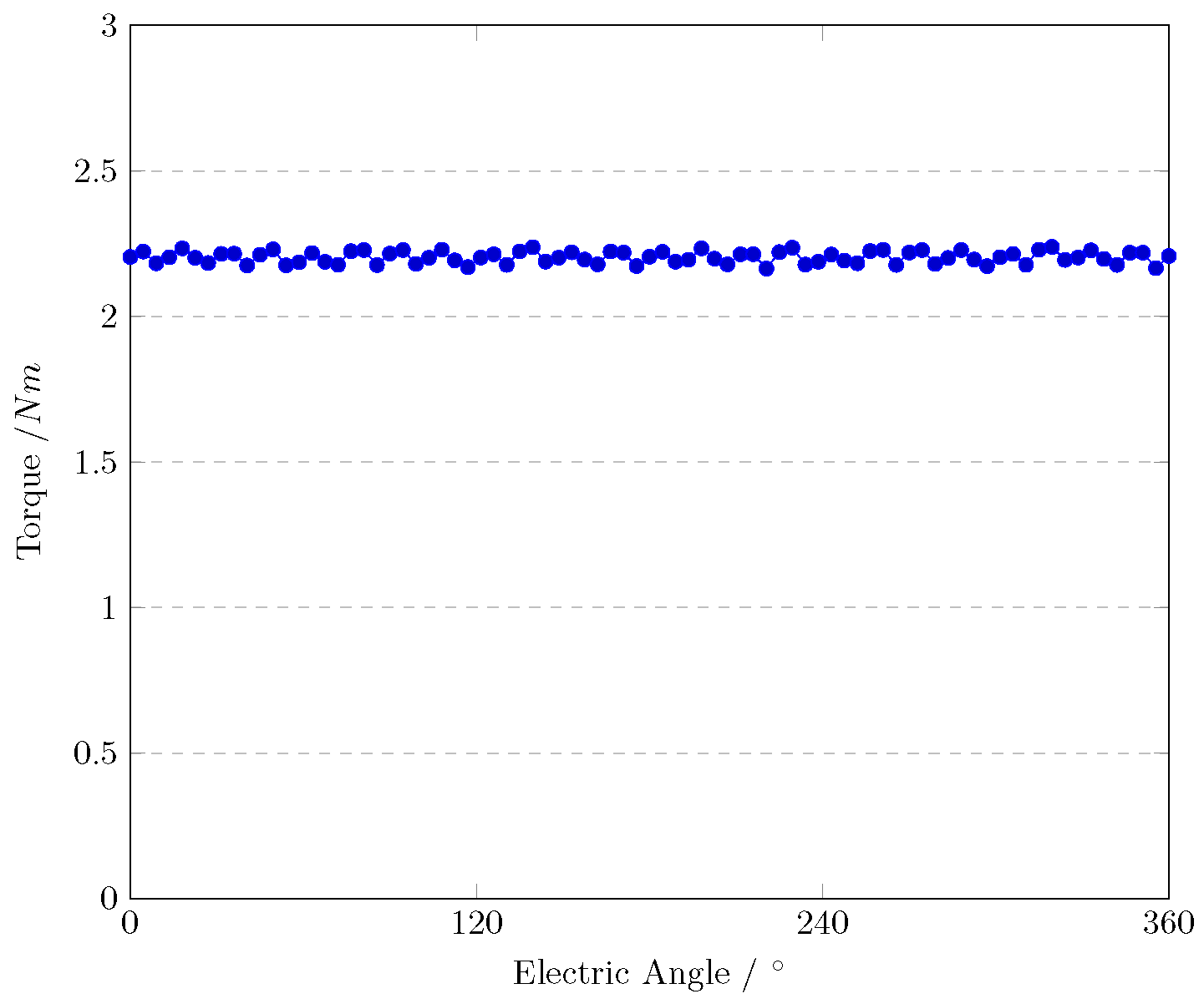
4.2. Torque Performances
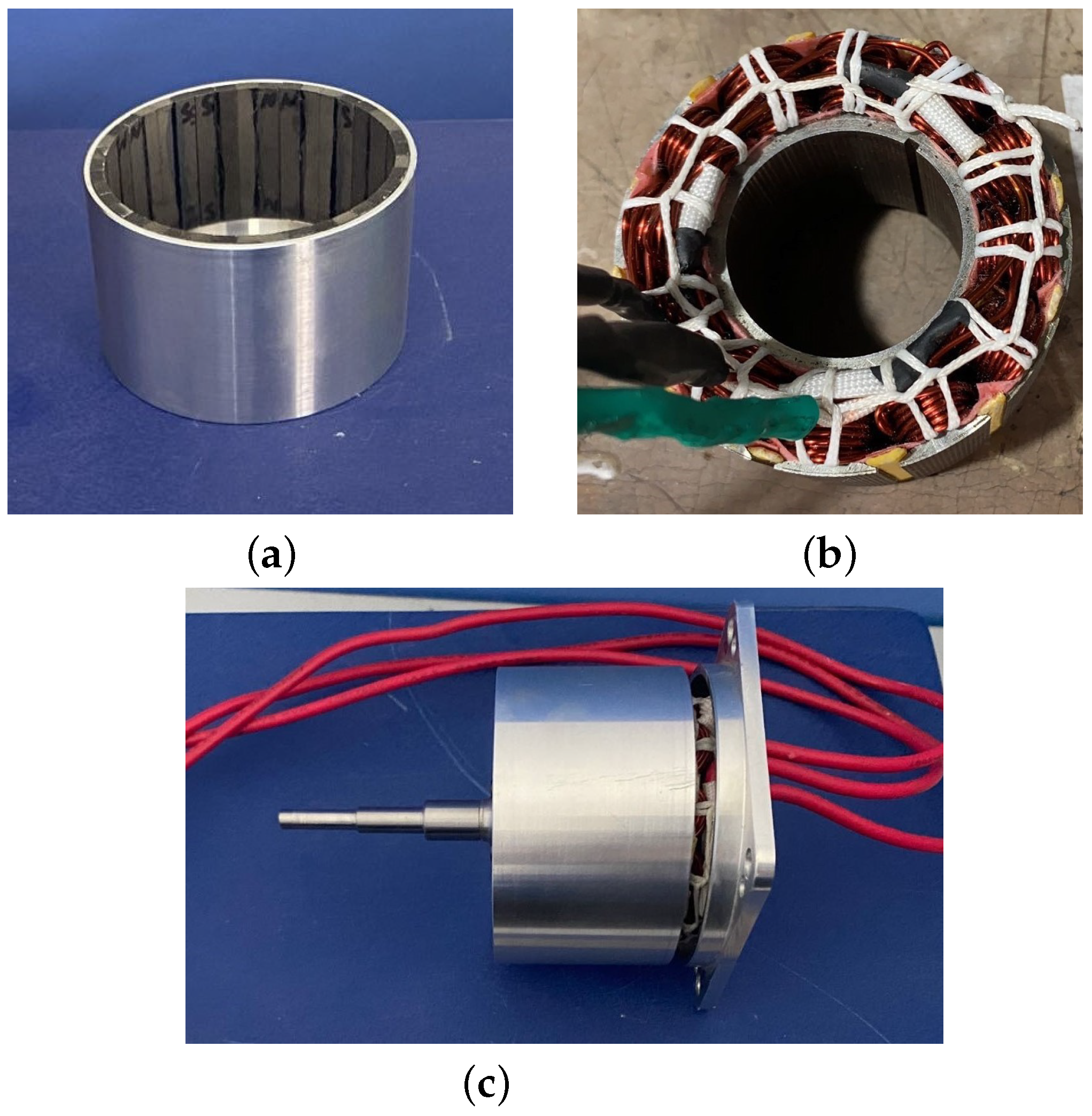
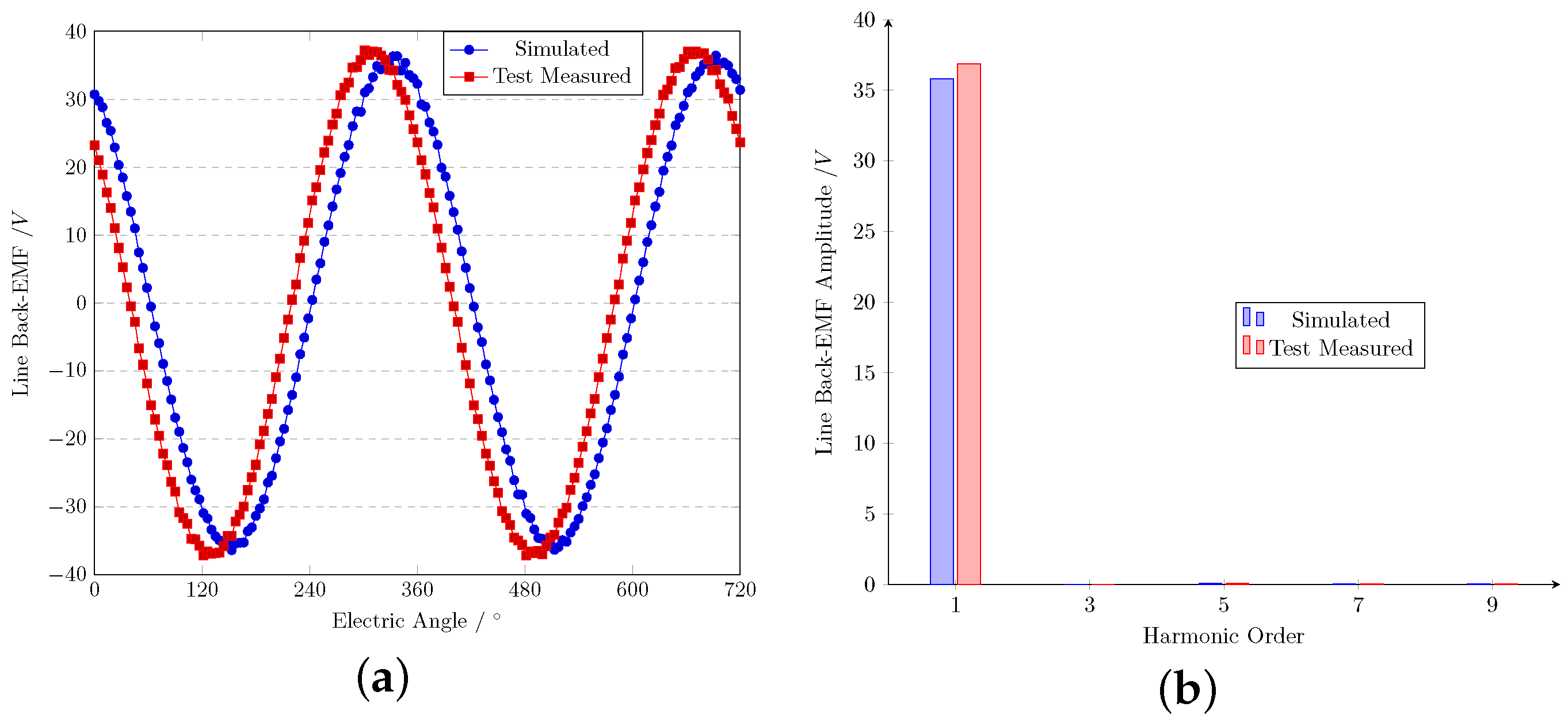
4.3. Experimental Validation
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhu, Z.Q.; Xiao, Y. Novel Magnetic-Field-Shifting Techniques in Asymmetric Rotor Pole Interior PM Machines With Enhanced Torque Density. IEEE Trans. Magn. 2022, 58, 8100610. [Google Scholar] [CrossRef]
- Du, Z.S.; Lipo, T.A. Cost-Effective High Torque Density Bi-Magnet Machines Utilizing Rare Earth and Ferrite Permanent Magnets. IEEE Trans. Energy Convers. 2020, 35, 1577–1584. [Google Scholar] [CrossRef]
- Zhang, Z.; Ma, R.; Wang, L.; Zhang, J. Novel PMSM Control for Anti-Lock Braking Considering Transmission Properties of the Electric Vehicle. IEEE Trans. Veh. Technol. 2018, 67, 10378–10386. [Google Scholar] [CrossRef]
- Chen, H.; Demerdash, N.A.O.; EL-Refaie, A.M.; Guo, Y.; Hua, W.; Lee, C.H.T. Investigation of a 3D-Magnetic Flux PMSM With High Torque Density for Electric Vehicles. IEEE Trans. Energy Convers. 2022, 37, 1442–1454. [Google Scholar] [CrossRef]
- Sant, A.V.; Khadkikar, V.; Xiao, W.; Zeineldin, H.H. Four-Axis Vector-Controlled Dual-Rotor PMSM for Plug-in Electric Vehicles. IEEE Trans. Ind. Electron. 2015, 62, 3202–3212. [Google Scholar] [CrossRef]
- Wang, Y.; Fang, S.; Hu, J. Active Disturbance Rejection Control Based on Deep Reinforcement Learning of PMSM for More Electric Aircraft. IEEE Trans. Power Electron. 2023, 38, 406–416. [Google Scholar] [CrossRef]
- Zhao, T.; Wu, S.; Cui, S. Multiphase PMSM With Asymmetric Windings for More Electric Aircraft. IEEE Trans. Transp. Electrif. 2020, 6, 1592–1602. [Google Scholar] [CrossRef]
- Song, Z.; Liu, C.; Feng, K.; Zhao, H.; Yu, J. Field Prediction and Validation of a Slotless Segmented-Halbach Permanent Magnet Synchronous Machine for More Electric Aircraft. IEEE Trans. Transp. Electrif. 2020, 6, 1577–1591. [Google Scholar] [CrossRef]
- Tymoori, V.; Kamper, M.J.; Wang, R.J.; Garner, G. Comparison of Sensorless Control Methods of PMSM Ship Propulsion Drives. In Proceedings of the 2023 International Conference on Electrical, Computer and Energy Technologies (ICECET), Cape Town, South Africa, 16–17 November 2023; pp. 1–7. [Google Scholar] [CrossRef]
- Yin, C.; He, Z. Vibration Reduction of Marine Permanent Magnet Propulsion Motor without Position Sensor. In Proceedings of the 2022 IEEE 10th Joint International Information Technology and Artificial Intelligence Conference (ITAIC), Chongqing, China, 17–19 June 2022; Volume 10, pp. 317–322. [Google Scholar] [CrossRef]
- Sonandkar, S.; Selvaraj, R.; Chelliah, T.R. Fault Tolerant Capability of Battery Assisted Quasi-Z-Source Inverter Fed Five Phase PMSM Drive for Marine Propulsion Applications. In Proceedings of the 2020 IEEE International Conference on Power Electronics, Drives and Energy Systems (PEDES), Jaipur, India, 16–19 December 2020; pp. 1–6. [Google Scholar] [CrossRef]
- Halbach, K. Design of Permanent Multipole Magnets with Oriented Rare Earth Cobalt Material. Nucl. Instrum. Methods 1980, 169, 1–10. [Google Scholar] [CrossRef]
- Shastri, S.; Singh, B. Rapid Flux Concentration Factor Determination for Halbach Array Based PM Rotors Using Composite FE Based Method. IEEE J. Multiscale Multiphysics Comput. Tech. 2023, 8, 241–251. [Google Scholar] [CrossRef]
- Wang, J.; Geng, W.; Li, Q.; Li, L.; Zhang, Z. A New Flux-Concentrating Rotor of Permanent Magnet Motor for Electric Vehicle Application. IEEE Trans. Ind. Electron. 2022, 69, 10882–10892. [Google Scholar] [CrossRef]
- Praveen, R.P.; Ravichandran, M.H.; Sadasivan Achari, V.T.; Jagathy Raj, V.P.; Madhu, G.; Bindu, G.R. A Novel Slotless Halbach-Array Permanent-Magnet Brushless DC Motor for Spacecraft Applications. IEEE Trans. Ind. Electron. 2012, 59, 3553–3560. [Google Scholar] [CrossRef]
- Vats, P.; Singh, B. Design and Analysis of Air Core Outer Rotor Halbach Array Compulsator. IEEE Trans. Plasma Sci. 2020, 48, 3663–3669. [Google Scholar] [CrossRef]
- Honjo, Y.; Caremel, C.; Kawahara, Y.; Sasatani, T. Suppressing Leakage Magnetic Field in Wireless Power Transfer Using Halbach Array-Based Resonators. IEEE Antennas Wirel. Propag. Lett. 2024, 23, 94–98. [Google Scholar] [CrossRef]
- Jing, L.; Huang, Z.; Chen, J.; Qu, R. An Asymmetric Pole Coaxial Magnetic Gear With Unequal Halbach Arrays and Spoke Structure. IEEE Trans. Appl. Supercond. 2020, 30, 5200305. [Google Scholar] [CrossRef]
- Brēķis, A.; Buligins, L.; Bucenieks, I.; Goldšteins, L.; Kravalis, K.; Lācis, A.; Mikanovskis, O.; Jēkabsons, N. Electromagnetic Pump with Rotating Permanent Magnets Operation at Low Inlet Pressures. Fusion Eng. Des. 2023, 194, 113919. [Google Scholar] [CrossRef]
- Yao, L.; Su, D.D.; Li, X.B.; Zhang, H.N.; Li, F.C. A Novel Halbach Array Permanent Magnet Flowmeter for Liquid Metal Flow Measurement. Part I: Design Proposal and Performance Analysis. Int. J. Heat Mass Transf. 2024, 228, 125692. [Google Scholar] [CrossRef]
- Yu, P.; Wang, Y.; Xu, Y.; Wu, Z.; Zhao, Y.; Peng, B.; Wang, F.; Tang, Y.; Yang, X. Theoretical Foundation for Designing Multilayer Halbach Array Magnets for Benchtop NMR and MRI. J. Magn. Reson. 2022, 344, 107322. [Google Scholar] [CrossRef] [PubMed]
- Shen, Y.; Lu, Q.; Li, Y. Design Criterion and Analysis of Hybrid-Excited Vernier Reluctance Linear Machine With Slot Halbach PM Arrays. IEEE Trans. Ind. Electron. 2023, 70, 5074–5084. [Google Scholar] [CrossRef]
- Lu, Y.; Kai, Y.; Songjun, S.; Yixiao, L.; Fei, X.; Cheng, L. Study on the Influence of a Combined-Halbach Array for the Axial Flux Permanent Magnet Electrical Machine With Yokeless and Segmented Armature. IEEE Trans. Magn. 2024, 60, 8101005. [Google Scholar] [CrossRef]
- Yang, K.; Zhao, F.; Yu, J.; Zhang, C.; Wang, Y. Nonlinear Analytical Model of a Double-Stator Flux Reversal Halbach Array Permanent Magnet Machine. IEEE Trans. Energy Convers. 2024, 39, 493–503. [Google Scholar] [CrossRef]
- Wang, J.; Yan, L.; Su, H.; Gao, X.; Dong, Z.; Chen, I.M. Modeling and Analysis of Short-Circuit Current for a Novel Dual Three-Phase Electric Machine With Mixed Iron-Pole Halbach Magnet Pattern. IEEE Trans. Instrum. Meas. 2024, 73, 3509411. [Google Scholar] [CrossRef]
- Sun, H.; Cheng, S.S. A Cylindrical Halbach Array Magnetic Actuation System for Longitudinal Robot Actuation. IEEE Robot. Autom. Lett. 2024, 9, 5847–5854. [Google Scholar] [CrossRef]
- Li, H.; Cui, L.; Ma, Z.; Li, B. Multi-Objective Optimization of the Halbach Array Permanent Magnet Spherical Motor Based on Support Vector Machine. Energies 2020, 13, 5704. [Google Scholar] [CrossRef]
- Zhang, S.; Zhang, W.; Wang, R.; Zhang, X.; Zhang, X. Optimization Design of Halbach Permanent Magnet Motor Based on Multi-Objective Sensitivity. CES Trans. Electr. Mach. Syst. 2020, 4, 20–26. [Google Scholar] [CrossRef]
- Koo, B.; Kim, J.; Nam, K. Halbach Array PM Machine Design for High Speed Dynamo Motor. IEEE Trans. Magn. 2021, 57, 8202105. [Google Scholar] [CrossRef]
- Yang, K.; Zhao, F.; Wang, Y.; Bao, Z. Consequent-Pole Flux Reversal Permanent Magnet Machine With Halbach Array Magnets in Rotor Slot. IEEE Trans. Magn. 2021, 57, 8100905. [Google Scholar] [CrossRef]
- Ma, Z.; Mohapatra, J.; Wei, K.; Liu, J.P.; Sun, S. Magnetic Nanoparticles: Synthesis, Anisotropy, and Applications. Chem. Rev. 2023, 123, 3904–3943. [Google Scholar] [CrossRef] [PubMed]


| Parameters | Value |
|---|---|
| Pole Pairs | 5 |
| Number of Slots | 12 |
| Rotor Inner diameter [mm] | 63.2 |
| Stator Outer diameter [mm] | 62 |
| Airgap length [mm] | 0.6 |
| Axial length [mm] | 33 |
| Tooth width [mm] | 6.7 |
| Stator Yoke thickness [mm] | 3.35 |
| Stator Inner diameter [mm] | 32 |
| Rotor Housing thickness [mm] | 1.5 |
| Number of turns per coil | 30 |
| Harmonic Order | ||||
|---|---|---|---|---|
| Fundamental, V | 18.59 | 20.53 | 20.67 | 21.28 |
| 3rd, mV | 170.9 | 74.87 | 50.23 | 25.86 |
| 5th, mV | 392.7 | 45.36 | 39.91 | 54.32 |
| 7th, mV | 33.29 | 204.9 | 32.77 | 31.53 |
| 9th, mV | 463.8 | 124.5 | 592.3 | 53.93 |
| 11th, mV | 249.2 | 53.59 | 24.43 | 29.89 |
| 13th, mV | 267.3 | 263.1 | 47.23 | 33.01 |
| THD | 3.85% | 1.80% | 2.89% | 0.46% |
| Harmonic Order | = 2.0 mm | = 2.5 mm | = 3.0 mm | = 4.0 mm |
|---|---|---|---|---|
| Fundamental, V | 17.34 | 20.67 | 23.17 | 26.47 |
| 3rd, mV | 23.52 | 53.69 | 79.97 | 372.7 |
| 5th, mV | 8.204 | 43.74 | 113.1 | 234.4 |
| 7th, mV | 19.04 | 18.68 | 14.51 | 55.02 |
| 9th, mV | 553.9 | 500.6 | 480.6 | 384.3 |
| 11th, mV | 8.269 | 61.29 | 193.1 | 133.5 |
| 13th, mV | 12.81 | 38.59 | 71.23 | 147.2 |
| THD | 3.2% | 2.5% | 2.3% | 2.3% |
| Harmonic Order | ||||
|---|---|---|---|---|
| Fundamental, V | 20.67 | 20.67 | 20.67 | 20.68 |
| 3rd, mV | 53.69 | 47.44 | 49.23 | 48.93 |
| 5th, mV | 43.74 | 37.02 | 45.55 | 49.13 |
| 7th, mV | 18.68 | 28.81 | 15.45 | 27.45 |
| 9th, mV | 500.6 | 574.4 | 507.27 | 581.13 |
| 11th, mV | 61.29 | 25.41 | 58.83 | 36.70 |
| 13th, mV | 38.59 | 39.11 | 42.26 | 48.72 |
| THD | 2.5% | 2.8% | 2.5% | 2.8% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, C.; Wu, H.; Shi, Y.; Wang, N.; Song, L. Study on the Influences of an Outer-Coreless-Rotor Permanent Magnet Synchronous Machine Using Halbach Magnet Array. Energies 2024, 17, 3108. https://doi.org/10.3390/en17133108
Xu C, Wu H, Shi Y, Wang N, Song L. Study on the Influences of an Outer-Coreless-Rotor Permanent Magnet Synchronous Machine Using Halbach Magnet Array. Energies. 2024; 17(13):3108. https://doi.org/10.3390/en17133108
Chicago/Turabian StyleXu, Cong, Hao Wu, Yuchao Shi, Ning Wang, and Liwei Song. 2024. "Study on the Influences of an Outer-Coreless-Rotor Permanent Magnet Synchronous Machine Using Halbach Magnet Array" Energies 17, no. 13: 3108. https://doi.org/10.3390/en17133108
APA StyleXu, C., Wu, H., Shi, Y., Wang, N., & Song, L. (2024). Study on the Influences of an Outer-Coreless-Rotor Permanent Magnet Synchronous Machine Using Halbach Magnet Array. Energies, 17(13), 3108. https://doi.org/10.3390/en17133108






