Economic and Exergy Analysis of TiO2 + SiO2 Ethylene-Glycol-Based Hybrid Nanofluid in Plate Heat Exchange System of Solar Installation
Abstract
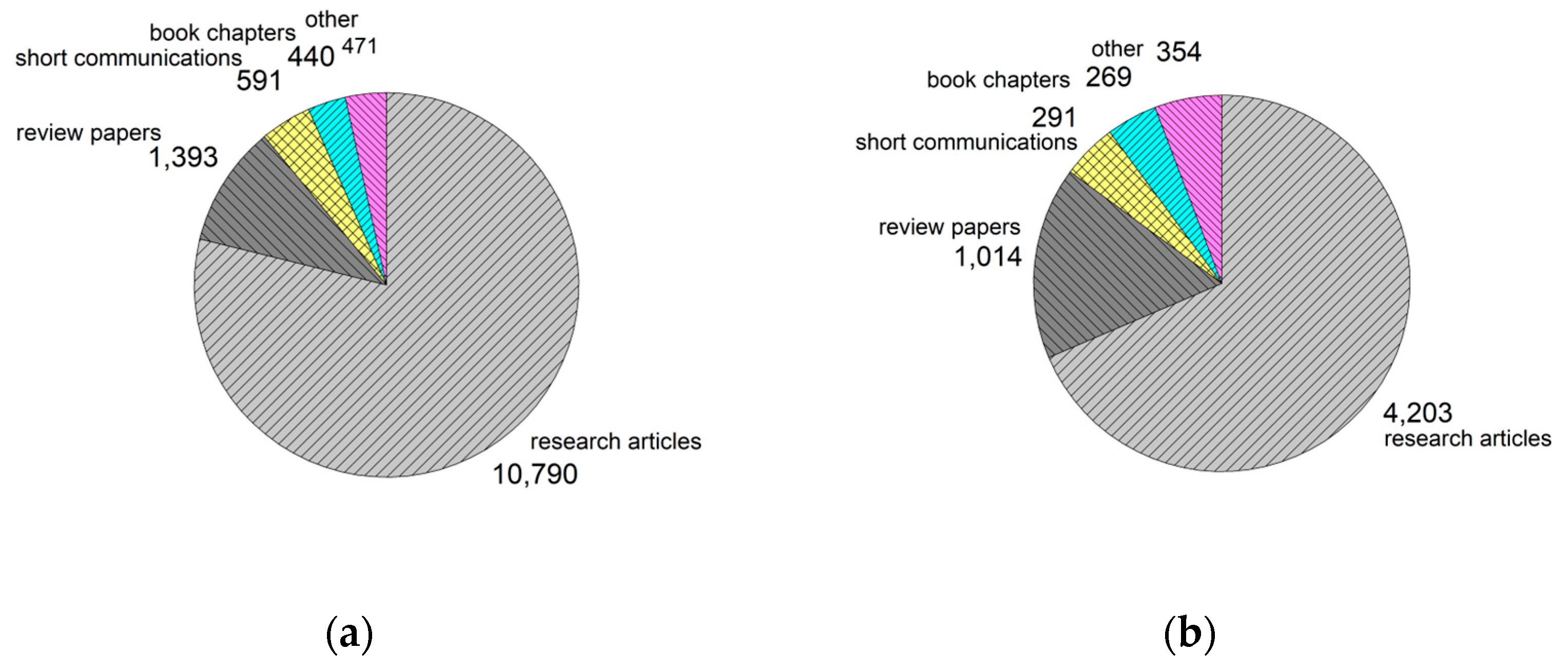
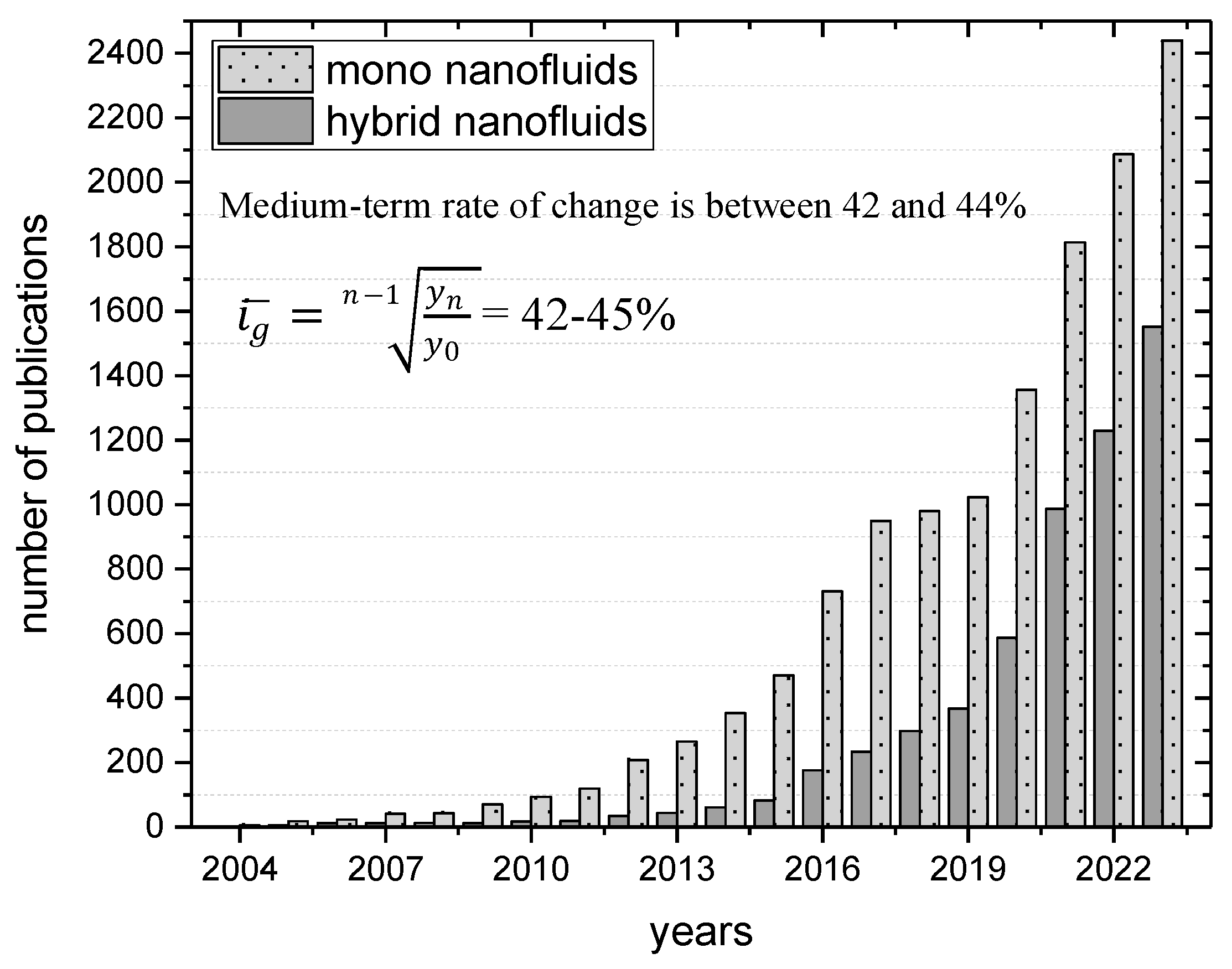
1. Introduction
2. Materials and Methods
2.1. Hybrid Nanofluids Preparation and Stability
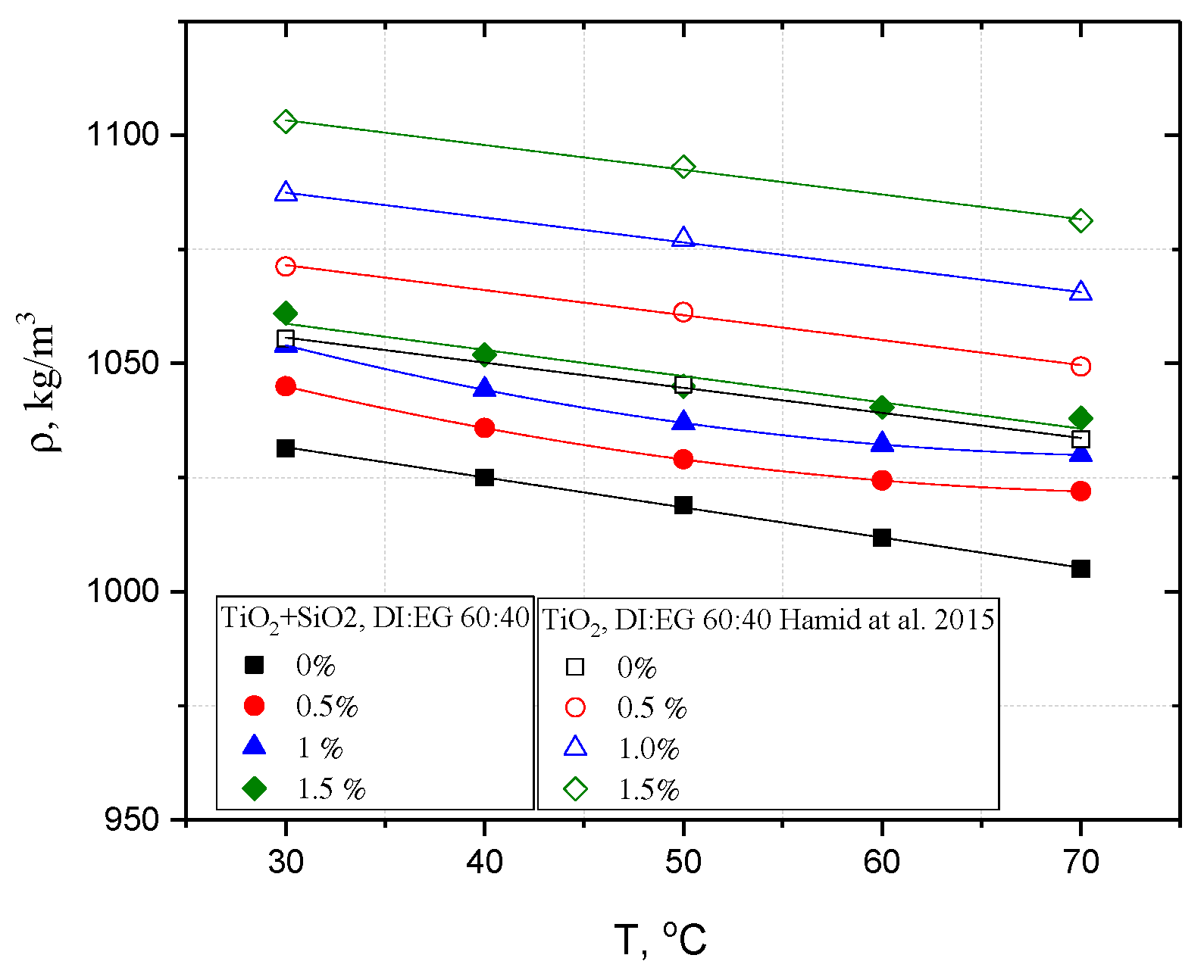
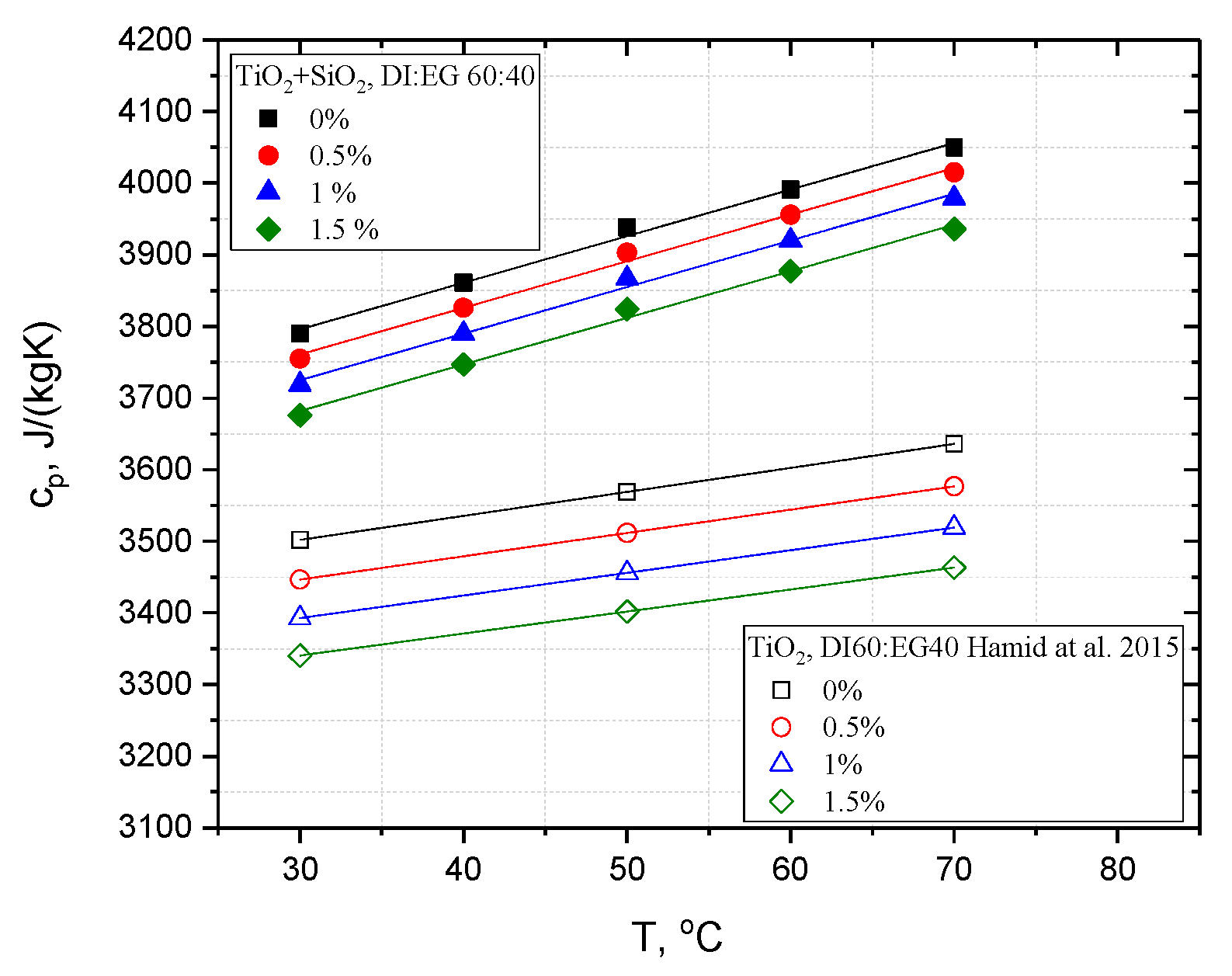
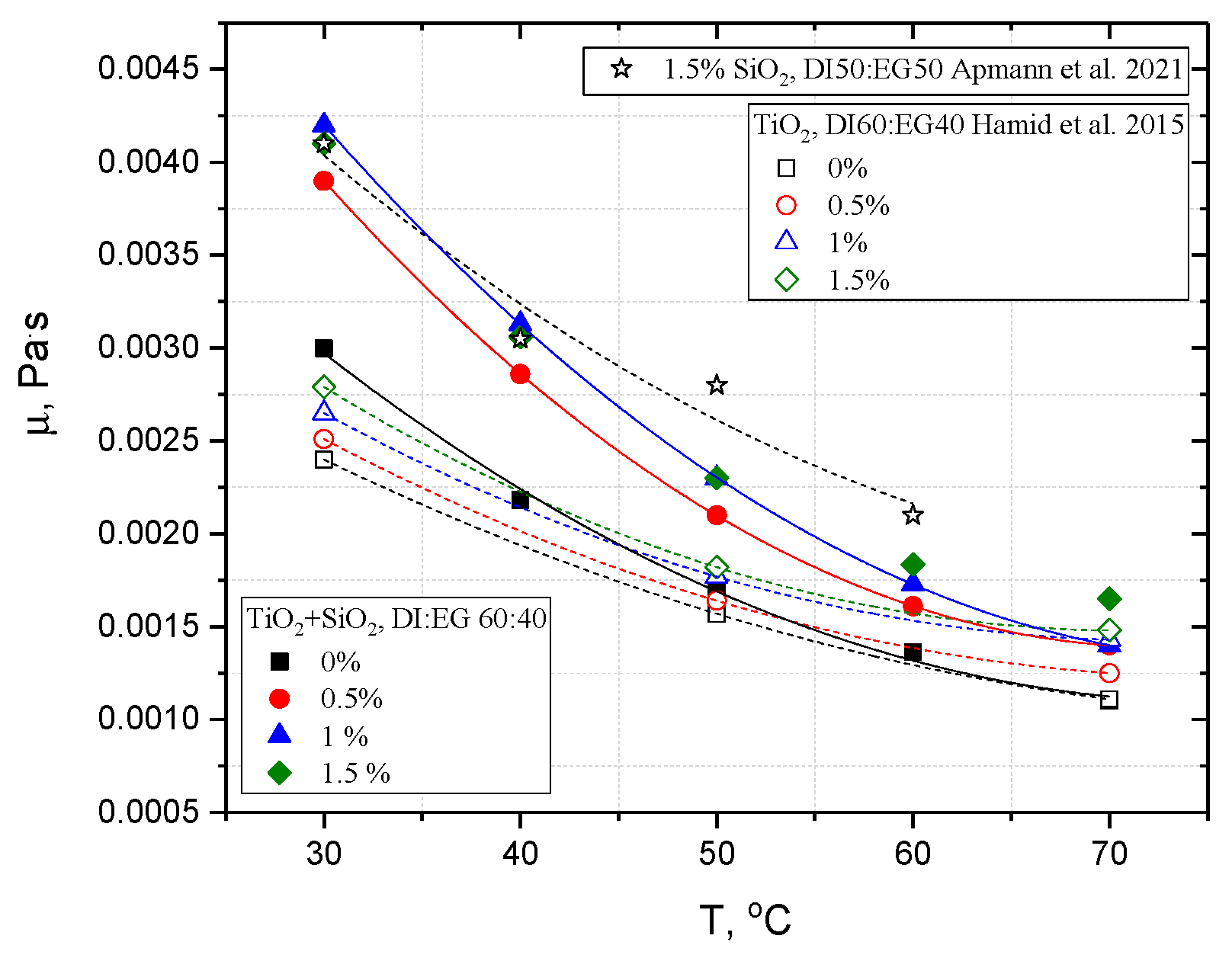
2.2. Hybrid Nanofluids Properties
2.3. Numerical Modeling
- the active area of a single collector is 3.0 m2,
- the required unit flow rate of solar fluid is .
2.4. Heat Transfer Study
2.5. Entropy and Exergy Study of PHE
3. Results and Discussion
4. Economic Analysis
5. Conclusions
- -
- the thermal conductivity coefficient, k, increased significantly with the temperature and concentration of the nanoparticles of approximately 6%;
- -
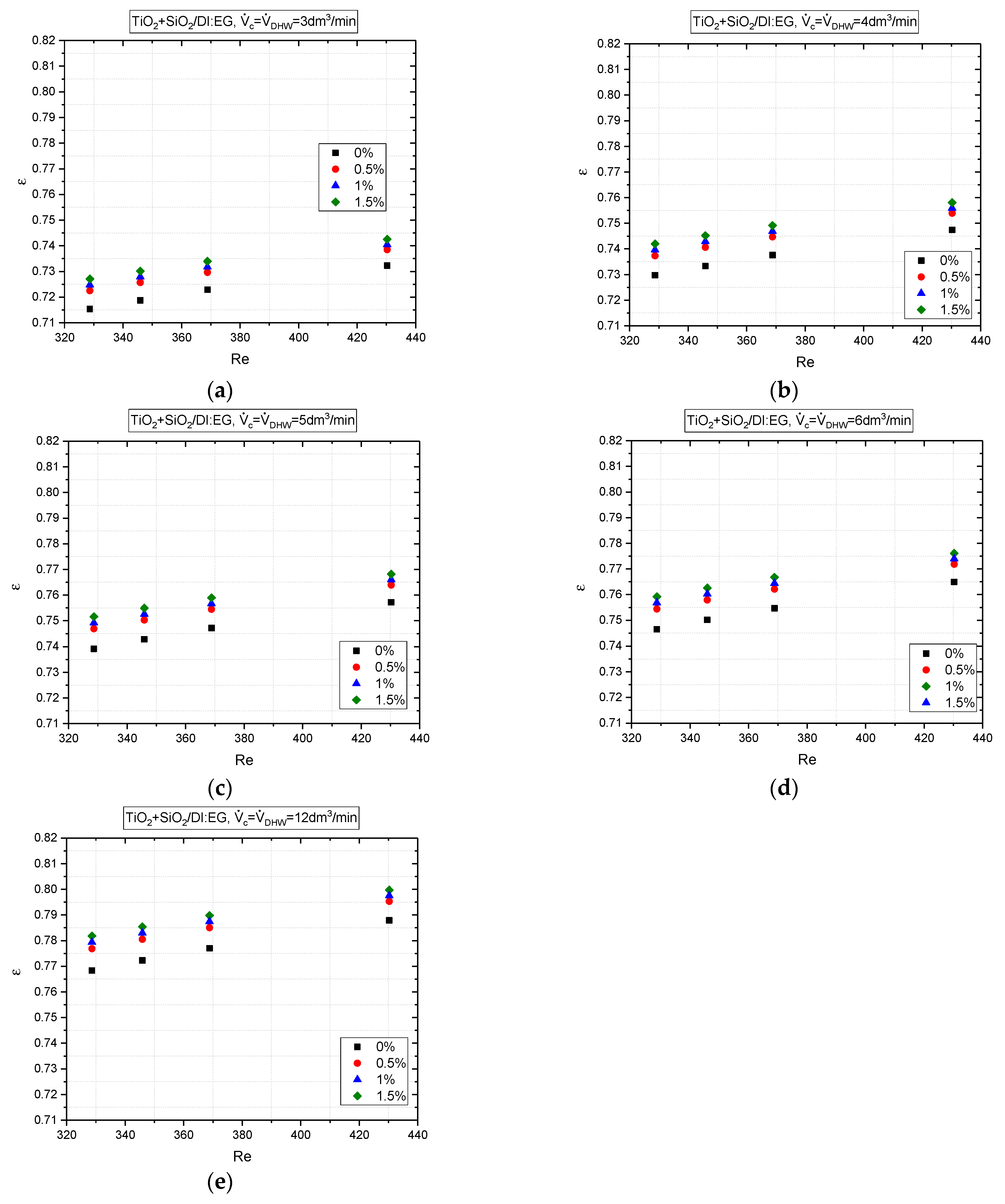
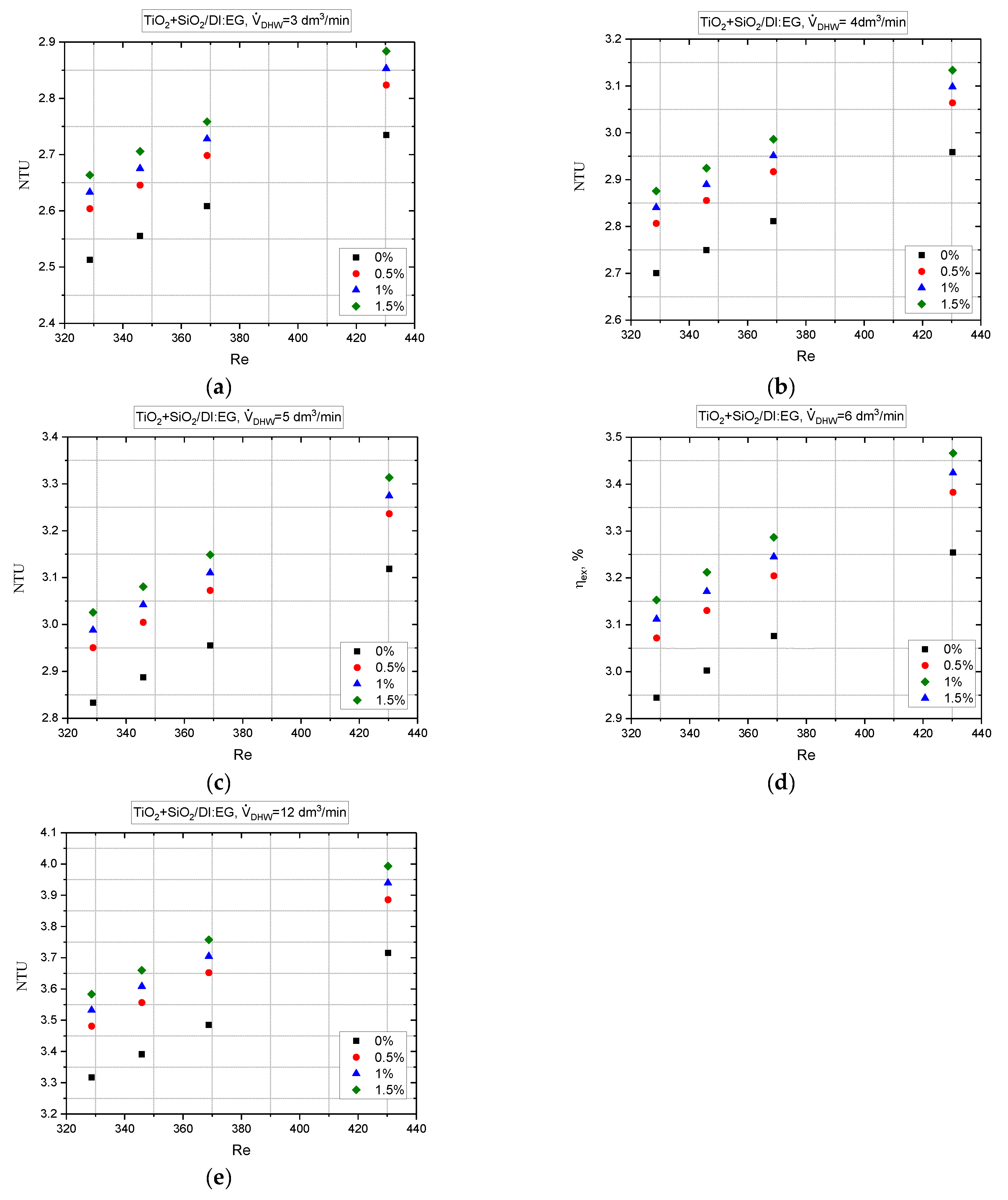
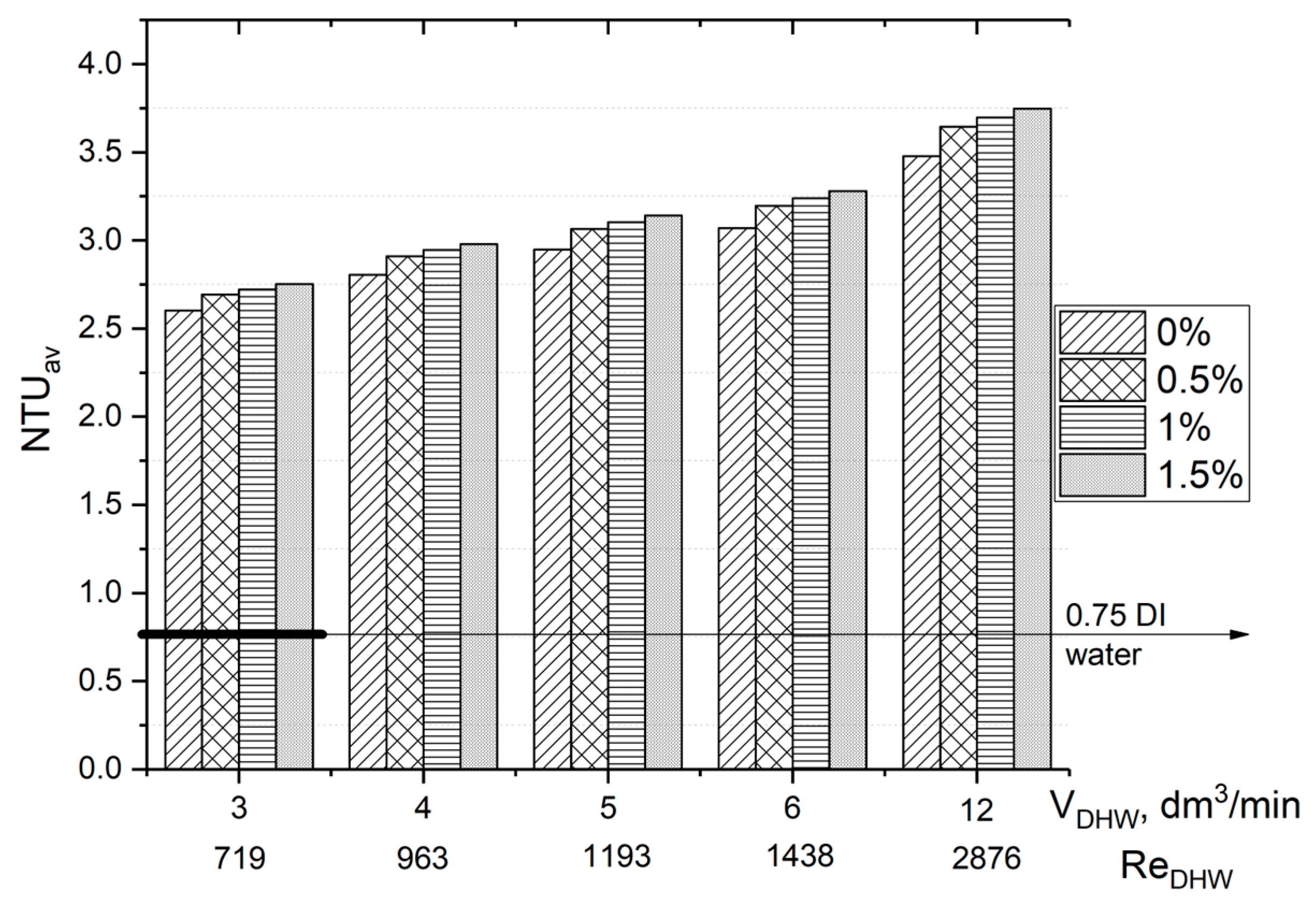
- a change in NTUav was observed in the range from 2.6 (for the base liquid) to 3.75 (for the 1.5% nanofluid). The higher the flow in the DHW installation, the proportionally higher the NTUav; it varied from 25% to 27% within a given number of ReDHW;
- -
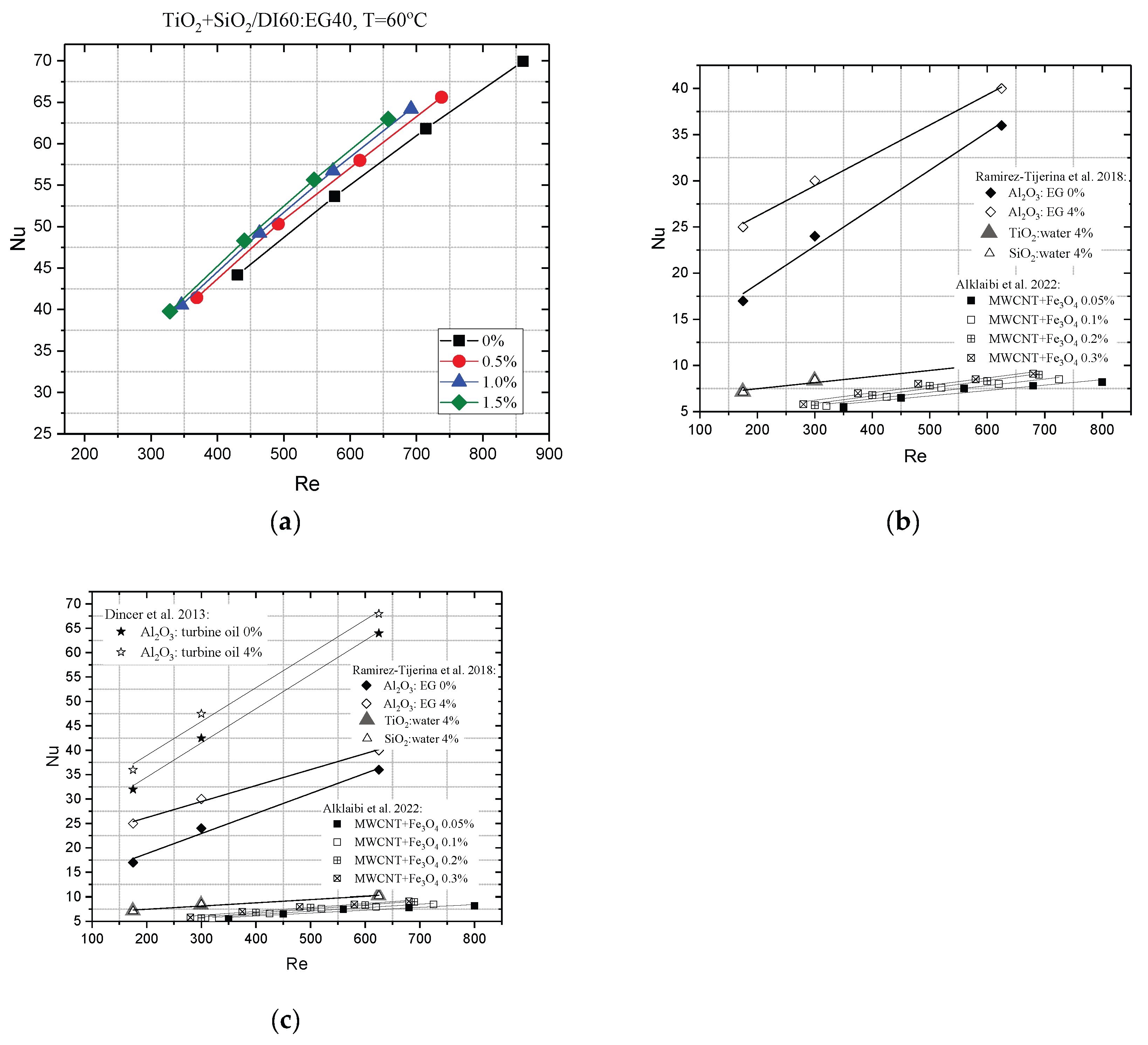
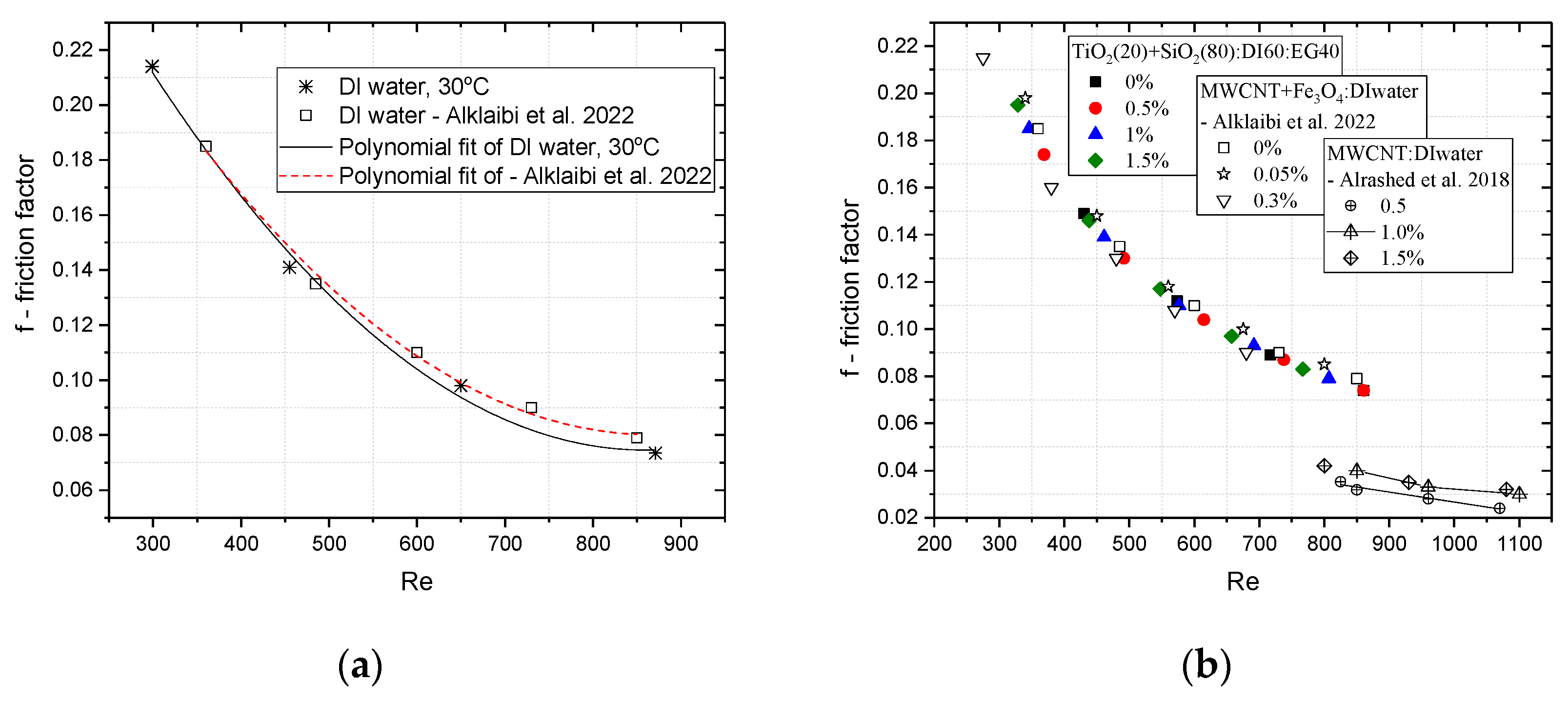
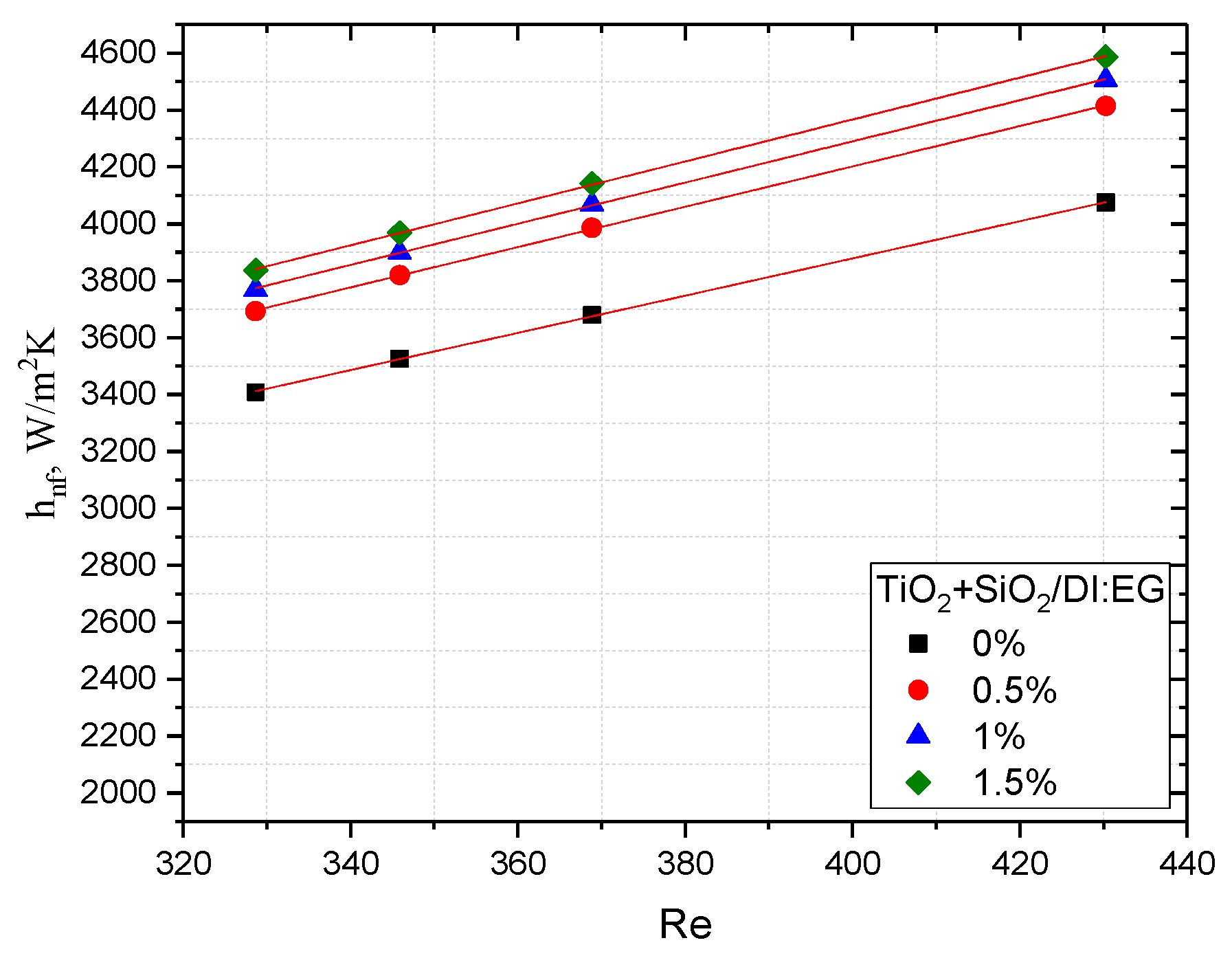
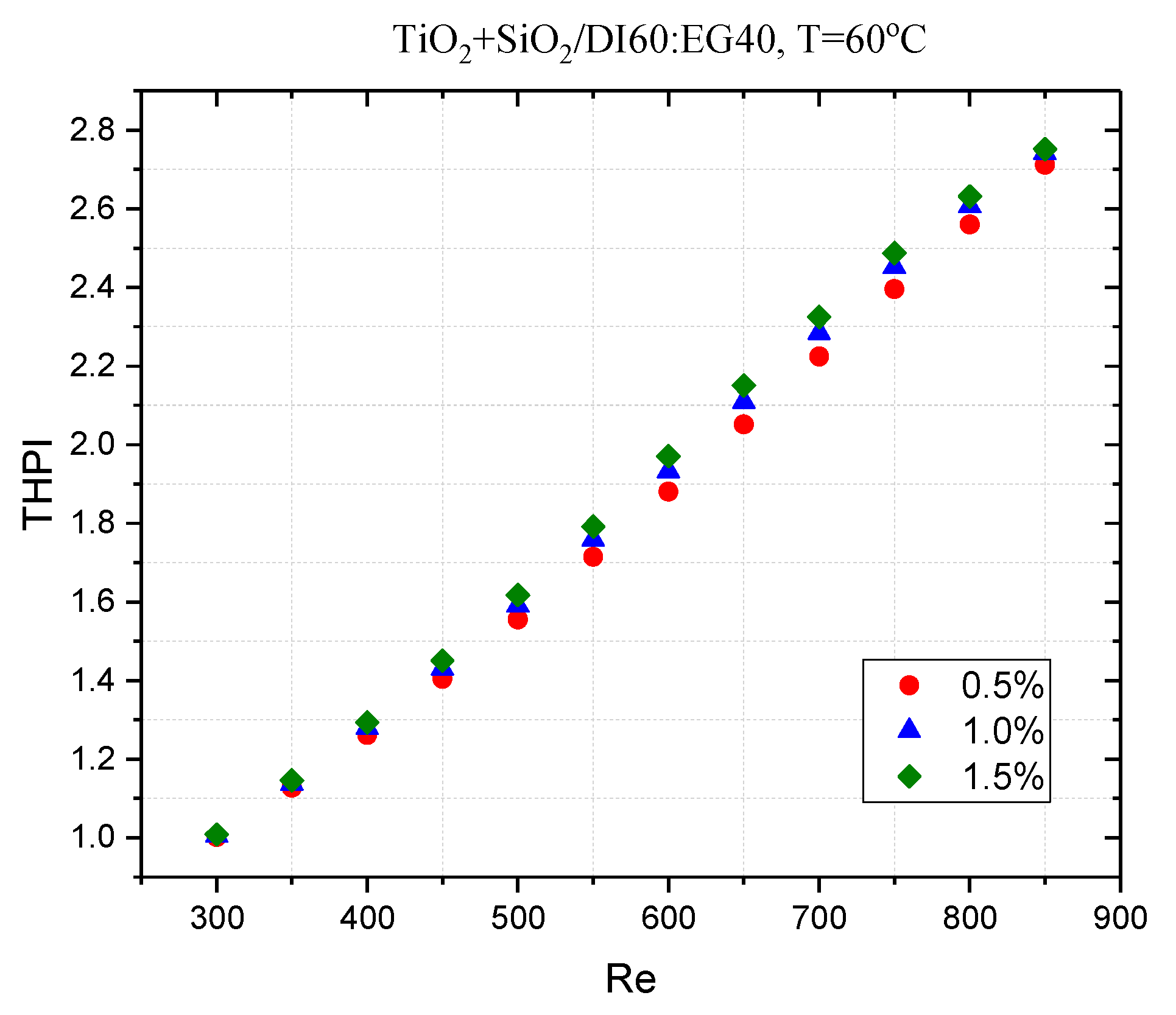
- ethylene-glycol-based nanofluids increased the Nu number from 10 to 32% depending on the suspension concentration; at Re = 657, the Nu of 1.5% TiO2:SiO2/DI:EG hybrid nanofluid in the PHE was 5% higher than that of the base fluid; but the friction factor, f, increased with the concentration and did not significantly exceed the base fluid friction factor—0 to 4% growth;
- -
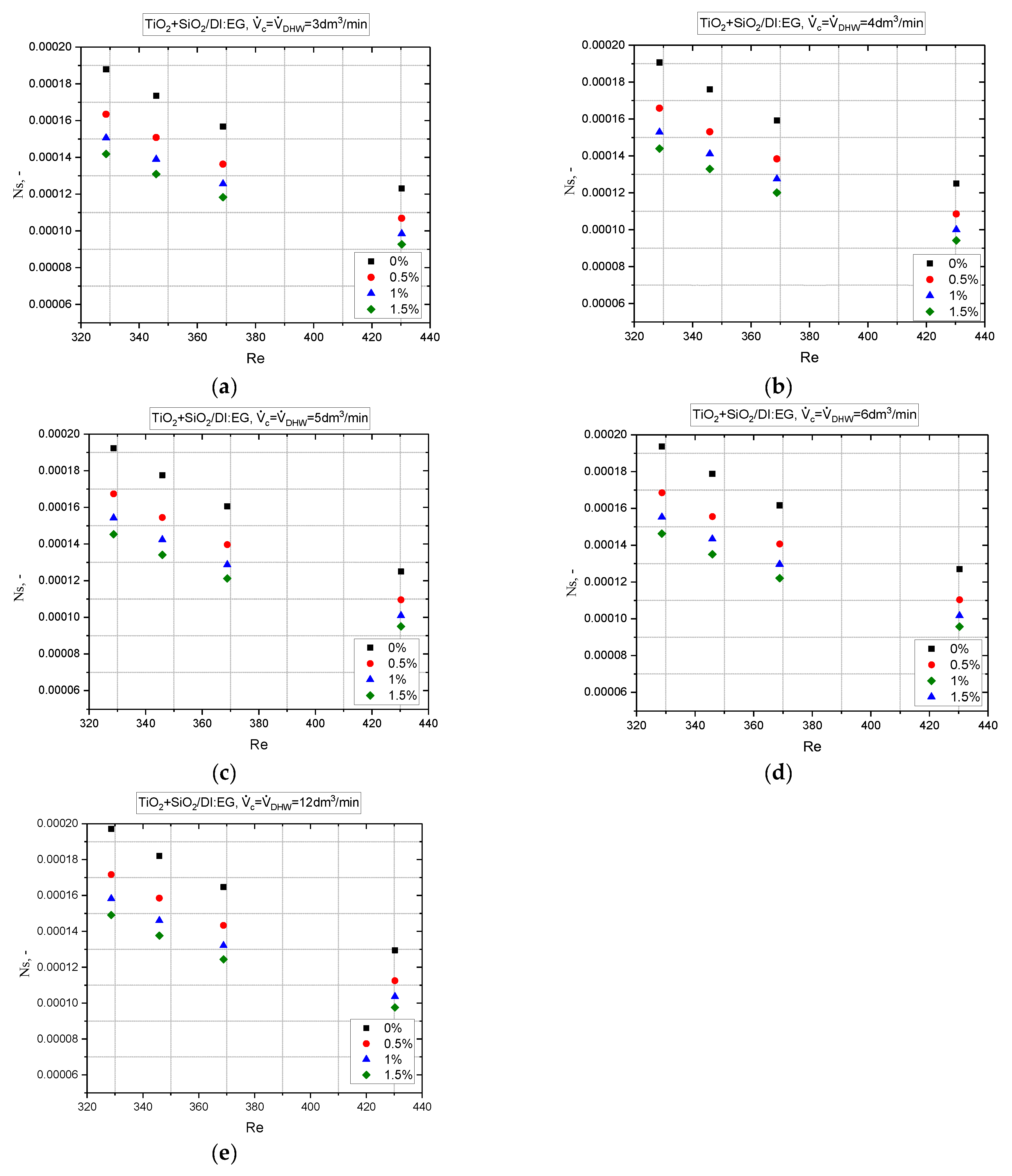
- the entropy generation number, Ns, was lowered to ~15% using a 1.5% suspension compared to the base fluid;
- -
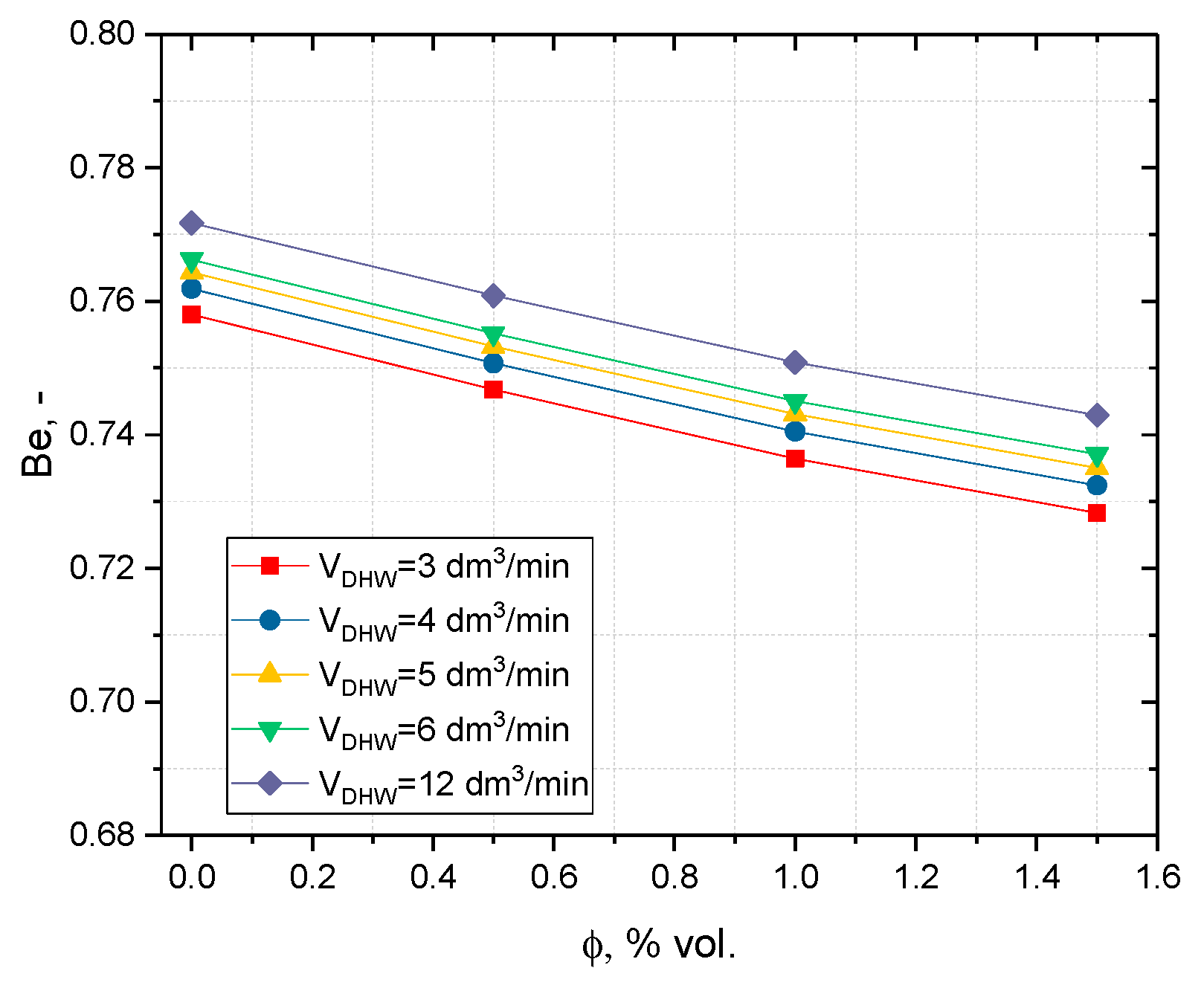
- the Bejan number, Be, was inversely proportional to the concentration of the nanofluid and increased with the DHW flow by approximately 25% on average, for each of the analyzed concentrations; it also decreased with the increase in the flow of nanofluids through the PHE on the primary side of the analyzed solar installation;
- -
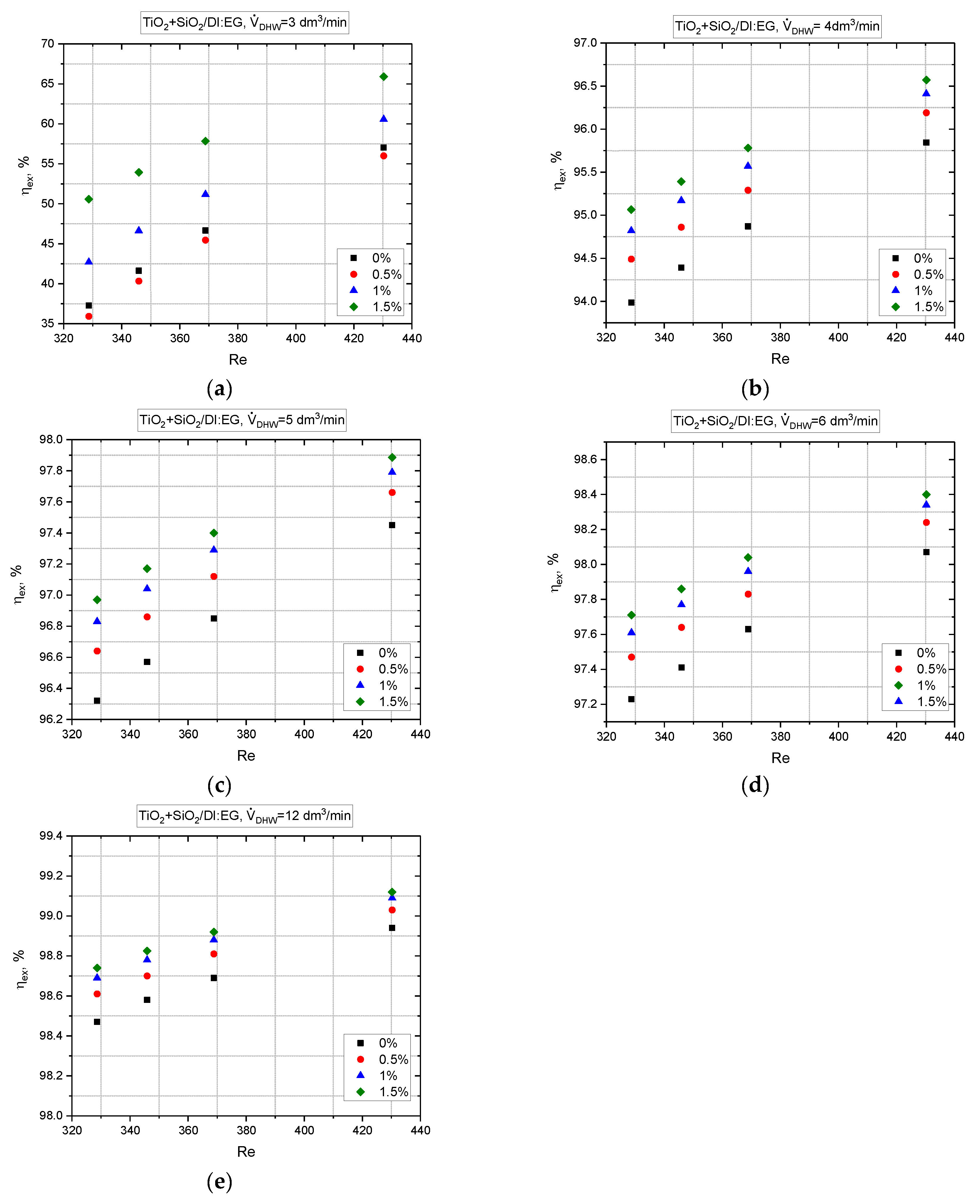
- The TiO2:SiO2/DI:EG hybrid nanofluid was a 13 to 26% more effective working fluid than the traditional solar fluid; at Re = 329, the exergy efficiency was = 37.29%, with a nanoparticle concentration of 0% and (1.5% vol.) = 50.56%; with Re = 430, (0%) = 57.03% and (1.5%) = 65.9%;
- -
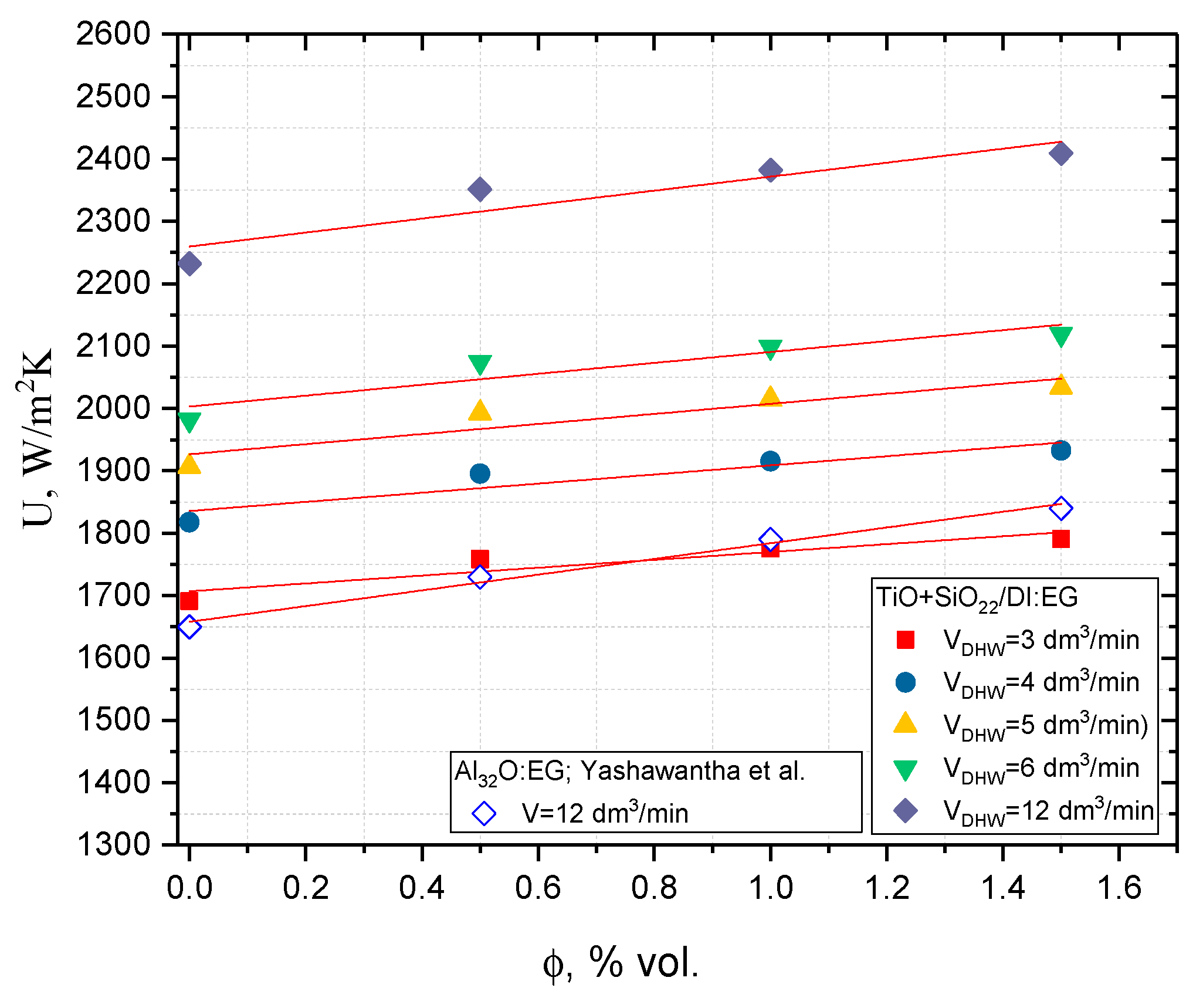
- the overall heat transfer coefficient, U, increased by 100 W/m2K for VDHW = 3 dm3/min and by 177 W/m2K for VDHW = 12 dm3/min, which corresponded to approx. a 7% intensification of the heat transfer;
- -
- based on the heat transfer enhancement ratios, it is concluded that the use of 1.5% TiO2:SiO2/DI:EG hybrid nanofluid in a flat solar collector system can contribute to approximately a 35% intensification of heat transfer compared to the base fluid;
- -
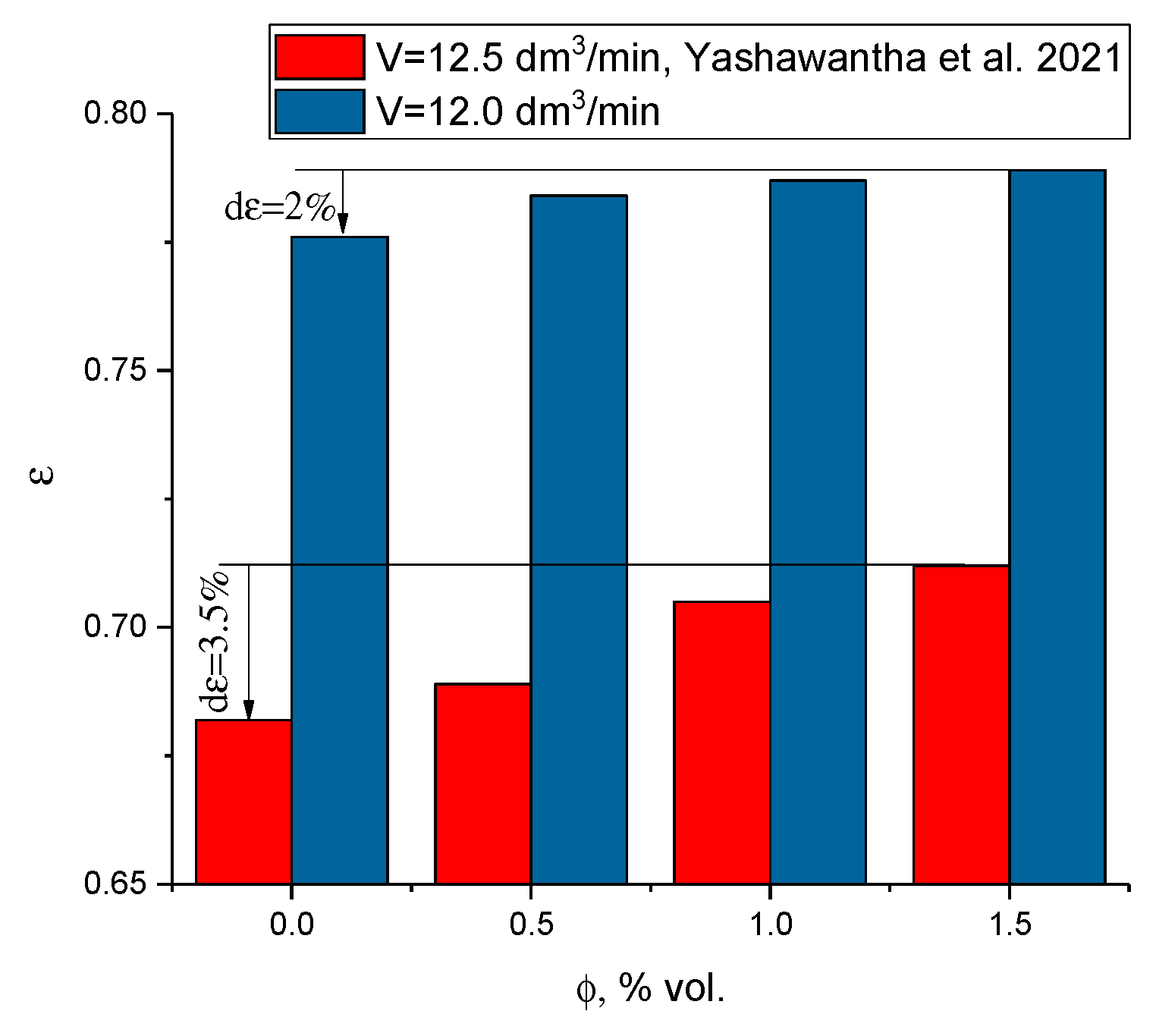
- the effectiveness, ε, of the PHE increased with the nanofluid concentration and the flow in the DHW system, and was 3.5% higher for the 1.5% nanofluid in comparison with the base fluid for each DHW flow;
- -
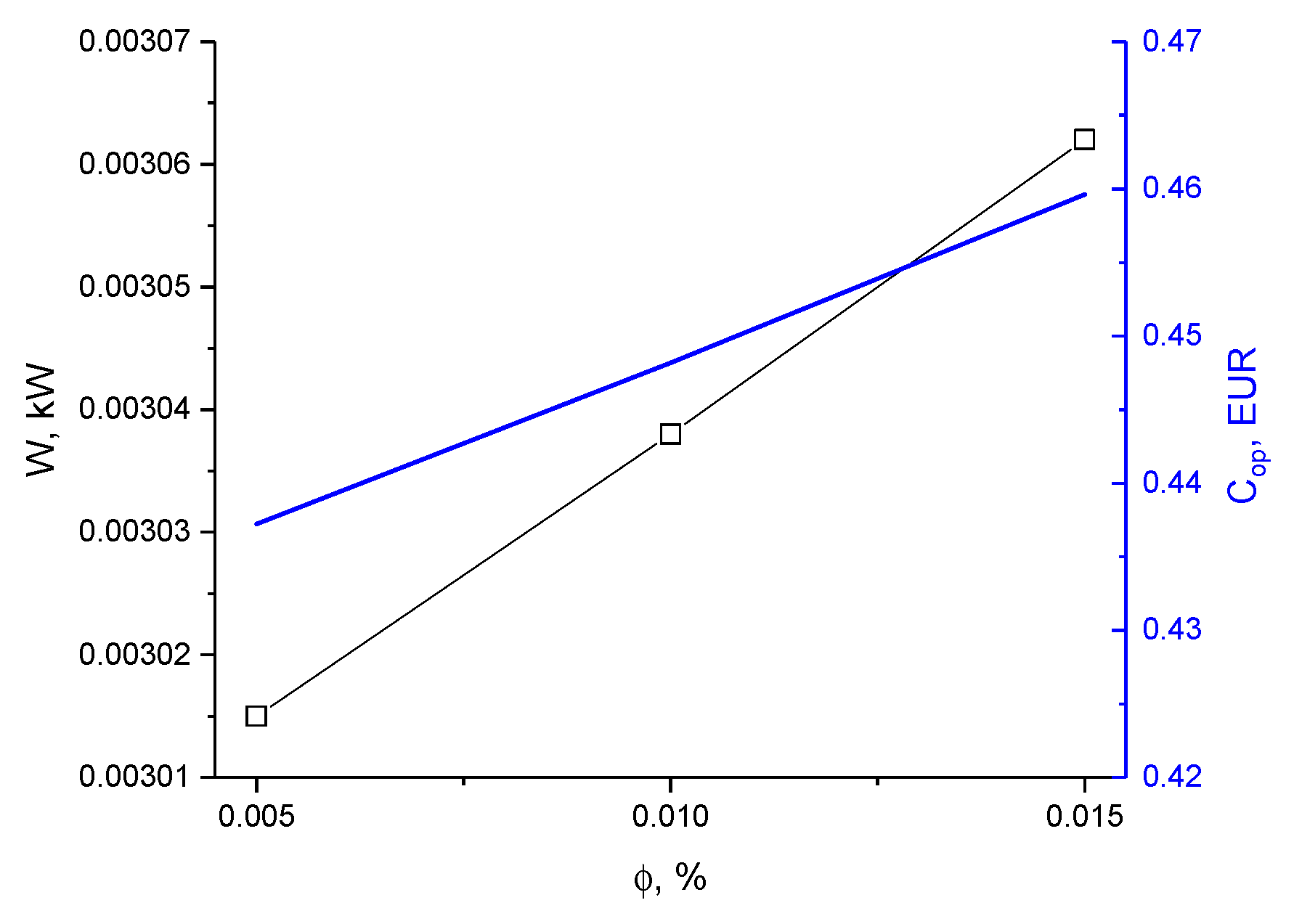
- using nanofluids, in some cases, will pay off after approximately 7 years.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Węglarz, K.; Taler, D.; Taler, J. New non-iterative method for computation of tubular cross-flow heat exchangers. Energy 2022, 260, 124955. [Google Scholar] [CrossRef]
- Maleki, N.M.; Pourahmad, S.; Khoshkhoo, R.H.; Ameri, M. Performance improvement of a double tube heat exchanger using novel electromagnetic vibration (EMV) method in the presence of Al2O3-water and CuO-water nanofluid; An experimental study. Energy 2023, 281, 128193. [Google Scholar] [CrossRef]
- Desisa, T.R. Experimental and numerical investigation of heat transfer characteristics in solar flat plate collector using nanofluids. Int. J. Thermofluids 2023, 18, 100325. [Google Scholar] [CrossRef]
- Gupta, S.K.; Verma, H.; Yadav, N. A review on recent development of nanofluid utilization in shell & tube heat exchanger for saving of energy. Mater. Today Proc. 2022, 54 Pt 3, 579–589. [Google Scholar] [CrossRef]
- Mukherjee, S.; Wciślik, S.; Mishra, P.C.; Chaudhuri, P. Nanofluids: Critical issues, economics and sustainability perspectives. Particuology 2024, 87, 147–172. [Google Scholar] [CrossRef]
- Available online: https://www.sciencedirect.com/search?qs=nanofluids%2C%20plate%20heat%20exchanger&lastSelectedFacet=years (accessed on 5 September 2023).
- Heulings, S.M. Review of commercial pasteurization, Bulletin No. 147. Am. J. Public Health 1925, 15, 723–725. [Google Scholar] [CrossRef][Green Version]
- Mota, F.A.S.; Carvalho, E.P.; Ravagnani, M.A.S.S. Modeling and Design of Plate Heat Exchanger. In Heat Transfer Studies and Applications; InTech: London, UK, 2015. [Google Scholar] [CrossRef]
- Shah, R.K.; Sekulic, D.P. Fundamentals of Heat Exchanger Design; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2003; p. 24. [Google Scholar]
- Iranmanesh, S.; Silakhori, M.; Naghavi, M.S.; Ang, B.C.; Ong, H.C.; Esmaeilzadeh, A. Using Graphene Nanoplatelets Nanofluid in a Closed-Loop Evacuated Tube Solar Collector—Energy and Exergy Analysis. J. Compos. Sci. 2021, 5, 277. [Google Scholar] [CrossRef]
- Lazarevikj, M.; Filkoski, R.V.; Markov, Z. CFD analysis of heat transfer and fluid flow in plate heat exchanger with nanofluid. In Proceedings of the 3rd South East European Conference on Sustainable Development of Energy, Water and Environment Systems—SDEWES SEE 2018, Novi Sad, Serbia, 30 June–4 July 2018. [Google Scholar]
- Wciślik, S. The Influence of Nusselt Correlation on Exergy Efficiency of a Plate Heat Exchanger Operating with TiO2:SiO2/EG:DI Hybrid Nanofluid. Inventions 2024, 9, 11. [Google Scholar] [CrossRef]
- Haghighi, E.B.; Saleemi, M.; Nikkam, N.; Khodabandeh, R.; Toprak, M.S.; Muhammed, M.; Palm, B. Accurate basis of comparison for convective heat transfer in nanofluids. Int. Commun. Heat Mass Transf. 2014, 52, 1–7. [Google Scholar] [CrossRef]
- Yashawantha, K.M.; Gurjar, G.; Vinod, A.V. Low Temperature Heat Transfer in Plate Heat Exchanger Using Ethylene Glycol–Water Based Al2O3 Nanofluid. Int. J. Thermophys. 2021, 42, 90. [Google Scholar] [CrossRef]
- Moghaieb, H.S.; Amendola, V.; Khalil, S.; Chakrabarti, S.; Maguire, P.; Mariotti, D. Nanofluids for Direct-Absorption Solar Collectors—DASCs: A Review on Recent Progress and Future Perspectives. Nanomaterials 2023, 13, 1232. [Google Scholar] [CrossRef] [PubMed]
- Nkurikiyimfura, I.; Wang, Y.; Safari, B.; Nshingabigwi, E. Electrical and thermal performances of photovoltaic/thermal systems with magnetic nanofluids: A review. Particuology 2021, 54, 181–200. [Google Scholar] [CrossRef]
- Choudhary, S.; Sachdeva, A.; Kumar, P. Investigation of the stability of MgO nanofluid and its effect on the thermal performance of flat plate solar collector. Renew. Energy 2020, 147, 1801–1814. [Google Scholar] [CrossRef]
- Ahmadlouydarab, M.; Ebadolahzadeh, M.; Ali, H.M. Effects of utilizing nanofluid as working fluid in a lab-scale designed FPSC to improve thermal absorption and efficiency. Phys. A Stat. Mech. Appl. 2020, 540, 123109. [Google Scholar] [CrossRef]
- Saffarian, M.R.; Moravej, M.; Doranehgard, M.H. Heat transfer enhancement in a flat plate solar collector with different flow path shapes using nanofluid. Renew. Energy 2020, 146, 2316–2329. [Google Scholar] [CrossRef]
- Mehdizadeh-Fard, M.; Pourfayaz, F.; Maleki, A. Exergy analysis of multiple heat exchanger networks: An approach based on the irreversibility distribution ratio. Energy Rep. 2021, 7, 174–193. [Google Scholar] [CrossRef]
- Tong, Y.; Lee, H.; Kang, W.; Cho, H. Energy and exergy comparison of a flat-plate solar collector using water, Al2O3 nanofluid, and CuO nanofluid. Appl. Therm. Eng. 2019, 159, 113959. [Google Scholar] [CrossRef]
- Ehyaei, M.A.; Ahmadi, A.; Assad, M.E.H.; Hachicha, A.A.; Said, Z. Energy, exergy and economic analyses for the selection of working fluid and metal oxide nanofluids in a parabolic trough collector. Sol. Energy 2019, 187, 175–184. [Google Scholar] [CrossRef]
- Mostafizur, R.M.; Rasul, M.G.; Nabi, M.N.; Haque, R.; Jahirul, M.I. Thermodynamic Analysis of a Flat Plate Solar Collector with Different Hybrid Nanofluids as Working Medium—A Thermal Modelling Approach. Nanomaterials 2023, 13, 1320. [Google Scholar] [CrossRef] [PubMed]
- Zhai, C.; Wu, W. Energetic, exergetic, economic, and environmental analysis of microchannel membrane-based absorption refrigeration system driven by various energy sources. Energy 2022, 239, 122193. [Google Scholar] [CrossRef]
- Orzechowski, T.; Stokowiec, K. Quasi-stationary phase change heat transfer on a fin. EPJ Web. Conf. 2016, 114, 02086. [Google Scholar] [CrossRef]
- De Oliveira, L.R.; Ribeiro, S.R.F.L.; Reis, M.H.M.; Cardoso, V.L.; Filho, E.P.B. Experimental study on the thermal conductivity and viscosity of ethylene glycol-based nanofluid containing diamond-silver hybrid material. Diam. Relat. Mater. 2019, 96, 216–230. [Google Scholar] [CrossRef]
- Akhgar, A.; Toghraie, D.; Sina, N.; Afrand, M. Developing dissimilar artificial neural networks (ANNs) to prediction the thermal conductivity of MWCNT-TiO2/Water-ethylene glycol hybrid nano fluid. Powder Technol. 2019, 355, 602–610. [Google Scholar] [CrossRef]
- Wohld, J.; Beck, J.; Inman, K.; Palmer, M.; Cummings, M.; Fulmer, R.; Vafaei, S. Hybrid Nanofluid Thermal Conductivity and Optimization: Original Approach and Background. Nanomaterials 2022, 12, 2847. [Google Scholar] [CrossRef] [PubMed]
- Hamid, K.A.; Azmi, W.H.; Nabil, M.F.; Mamat, R. Heat transfer augmentation of mixture ratio TiO2 to SiO2 in hybrid nanofluid. J. Mech. Eng. 2017, 4, 51–63. [Google Scholar]
- Javadi, F.S.; Sadeghipour, S.; Saidur, R.; BoroumandJazi, G.; Rahmati, B.; Elias, M.M.; Sohel, M.R. The effects of nanofluid on thermophysical properties and heat transfer characteristics of a plate heat exchanger. Int. Commun. Heat Mass Transf. 2013, 44, 58–63. [Google Scholar] [CrossRef]
- Okonkwo, E.C.; Wole-Osho, I.; Almanassra, I.W.; Abdullatif, Y.M.; Al-Ansari, T. An updated review of nanofluids in various heat transfer devices. J. Therm. Anal. Calorim. 2021, 145, 2817–2872. [Google Scholar] [CrossRef]
- Wciślik, S. A simple economic and heat transfer analysis of the nanoparticles use. Chem. Pap. 2017, 71, 2395–2401. [Google Scholar] [CrossRef] [PubMed]
- Mukherjee, S.; Wciślik, S.; Khadanga, V.; Mishra, P.C. Influence of nanofluids on the thermal performance and entropy generation of varied geometry microchannel heat sink. Case Stud. Therm. Eng. 2023, 49, 103241. [Google Scholar] [CrossRef]
- Wciślik, S. Efficient Stabilization of Mono and Hybrid Nanofluids. Energies 2020, 13, 3793. [Google Scholar] [CrossRef]
- Vladisavljević, G.T. Preparation of microparticles and nanoparticles using membrane-assisted dispersion, micromixing, and evaporation processes. Particuology 2024, 84, 30–44. [Google Scholar] [CrossRef]
- Dincer, I.; Rosen, M.A. Chapter 7—Exergy Analysis of Heat Pump Systems. In Exergy, 2nd ed.; Dincer, I., Rosen, M.A., Eds.; Elsevier: Amsterdam, The Netherlands, 2013; pp. 101–113. ISBN 9780080970899. [Google Scholar] [CrossRef]
- Qing, S.H.; Rashmi, W.; Khalid, M.; Gupta, T.C.S.M.; Nabipoor, M.; Hajibeigy, M.T. Thermal conductivity and electrical properties of Hybrid SiO2-graphene naphthenic mineral oil nanofluid as potential transformer oil. Mater. Res. Express. 2017, 4, 015504. [Google Scholar] [CrossRef]
- Hamid, K.A.; Azmi, W.H.; Mamat, R.; Usri, N.A.; Najafi, G. Effect of Temperature on Heat Transfer Coefficient of Titanium Dioxide in Ethylene Glycol-based Nanofluid. J. Mech. Eng. Sci. 2015, 8, 1367–1375. [Google Scholar] [CrossRef]
- Teng, T.-P.; Hung, Y.-H. Estimation and experimental study of the density and specific heat for alumina nanofluid. J. Exp. Nanosci. 2014, 9, 707–718. [Google Scholar] [CrossRef]
- Raja, R.A.; Sunil, J.; Maheswaran, R. Estimation of thermo-physical properties of nanofluids using theoretical correlations. Int. J. Appl. Eng. Res. 2018, 13, 7950–7953. [Google Scholar]
- Ahammed, N.; Asirvatham, L.G.; Wongwises, S. Entropy generation analysis of graphene–alumina hybrid nanofluid in multiport minichannel heat exchanger coupled with thermoelectric cooler. Int. J. Heat Mass Transf. 2016, 103, 1084–1097. [Google Scholar] [CrossRef]
- Hormozi, F.; ZareNezhad, B.; Allahyar, H.R. An experimental investigation on the effects of surfactants on the thermal performance of hybrid nanofluids in helical coil heat exchangers. Int. Commun. Heat Mass Transf. 2016, 78, 271–276. [Google Scholar] [CrossRef]
- Sundar, L.S.; Sharma, K.V.; Singh, M.K.; Sousa AC, M. Hybrid nanofluids preparation, thermal properties, heat transfer and friction factor—A review. Renew. Sustain. Energy Rev. 2017, 68, 185–198. [Google Scholar] [CrossRef]
- Zainon, S.N.M.; Azmi, W.H.; Hamisa, A.H. Thermo-physical Properties of TiO2-SiO2 Hybrid Nanofluids Dispersion with Water/Bio-glycol Mixture. J. Phys. Conf. Ser. 2021, 2000, 012003. [Google Scholar] [CrossRef]
- Apmann, K.; Fulmer, R.; Soto, A.; Vafaei, S. Thermal Conductivity and Viscosity: Review and Optimization of Effects of Nanoparticles. Materials 2021, 14, 1291. [Google Scholar] [CrossRef] [PubMed]
- Viessmann Technical Guide, VITOSOL Flat-Plate Collectors and Vacuum Tube Collectors Flat and Pitched Roof Installation, and Wall Mounting, 5822440 GB5, 2018. Available online: https://viessmanndirect.co.uk/files//faa19e98-f0eb-4b23-98a7-aa3201061e16/1805%20Vitosol%20Technical%20Guide%205822440VPA00013_1.PDF (accessed on 28 June 2023).
- Lavine, A.S.; Incropera, F.P. Fundamentals of Heat and Mass Transfer; John Wiley & Sons Inc.: Hoboken, NJ, USA, 2020. [Google Scholar]
- Kakaç, S.; Liu, H. Heat Exchangers Selection, Rating, and Thermal Design, 3rd ed.; CRC Press: Boca Raton, FL, USA, 2002. [Google Scholar]
- Akturk, F.; Sezer-Uzol, N.; Aradag, S.; Kakac, S. Experimental investigation and performance analysis of gasketed-plate heat exchangers. J. Therm. Sci. Technol. 2015, 35, 43–52. [Google Scholar]
- Alklaibi, A.M.; Sundar, L.S.; Mouli, K.V.V.C. Experimental investigation on the performance of hybrid Fe3O4 coated MWCNT/Water nanofluid as a coolant of a Plate heat exchanger. Int. J. Therm. Sci. 2022, 171, 107249. [Google Scholar] [CrossRef]
- Sundar, L.S.; Bhramara, P.; Kumar, N.T.R.; Singh, M.K.; Sousa, A.C.M. Experimental heat transfer, friction factor and effectiveness analysis of Fe3O4 nanofluid flow in a horizontal plain tube with return bend and wire coil inserts. Int. J. Heat Mass Transf. 2017, 109, 440–453. [Google Scholar] [CrossRef]
- Sundar, L.S.; Singh, M.K.; Sousa, A.C.M. Heat transfer and friction factor of nanodiamond-nickel hybrid nanofluids flow in a tube with longitudinal strip inserts. Int. J. Heat Mass Transf. 2018, 121, 390–401. [Google Scholar] [CrossRef]
- Bejan, A. Advanced Engineering Thermodynamics; Wiley Interscience: New York, NY, USA, 1988. [Google Scholar]
- Sundar, L.S.; Mesfin, S.; Ramana, E.V.; Said, Z.; Sousa, A.C.M. Experimental investigation of thermo-physical properties, heat transfer, pumping power, entropy generation, and exergy efficiency of nanodiamond + Fe3O4/60:40% water-ethylene glycol hybrid nanofluid flow in a tube. Therm. Sci. Eng. Prog. 2021, 21, 100799. [Google Scholar] [CrossRef]
- Kabeel, A.E.; El Maaty, T.A.; El Samadony, Y. The effect of using nano-particles on corrugated plate heat exchanger performance. Appl. Therm. Eng. 2013, 52, 221–229. [Google Scholar] [CrossRef]
- Teng, T.-P.; Hsiao, T.-C.; Chung, C.-C. Characteristics of carbon-based nanofluids and their application in a brazed plate heat exchanger under laminar flow. Appl. Therm. Eng. 2019, 146, 160–168. [Google Scholar] [CrossRef]
- Goodarzi, M.; Amiri, A.; Goodarzi, M.S.; Safaei, M.R.; Karimipour, A.; Languri, E.M.; Dahari, M. Investigation of heat transfer and pressure drop of a counter flow corrugated plate heat exchanger using MWCNT based nanofluids. Int. Commun. Heat Mass Transf. 2015, 66, 172–179. [Google Scholar] [CrossRef]
- Jiang, P.X.; Fan, M.H.; Si, G.S.; Ren, Z.P. Thermal–hydraulic performance of small scale micro-channel and porous-media heat-exchangers. Int. J. Heat Mass Transf. 2001, 44, 1039–1051. [Google Scholar] [CrossRef]
- Sheikh, R.; Fallahsohi, S.H.; Goodarzi, M.; Taheri, M.M.; Bagheri, M. Improving the efficiency of an exhaust thermoelectric generator based on changes in the baffle distribution of the heat exchanger. J. Therm. Anal. Calorim. 2021, 143, 523–533. [Google Scholar] [CrossRef]
- Ramirez-Tijerina, R.; Rivera-Solorio, C.I.; Singh, J.; Nigam, K.D.P. Numerical Study of Heat Transfer Enhancement for Laminar Nanofluids Flow. Appl. Sci. 2018, 8, 2661. [Google Scholar] [CrossRef]
- Alrashed, A.A.; Akbari, O.A.; Heydari, A.; Toghraie, D.; Zarringhalam, M.; Shabani, G.; Seifi, A.; Goodarzi, M. The numerical modeling of water/FMWCNT nanofluid flow and heat transfer in a backward-facing contracting channel. Phys. B Condens. Matter 2018, 537, 176–183. [Google Scholar] [CrossRef]
- Sundar, L.S.; Mesfin, S.; Raman, E.V.; Punnaiah, V.; Chamkha, A.J.; Sousa, A.C.M. Thermal entropy generation and exergy efficiency analyses of coiled wire inserted nanodiamond + Fe3O4/water hybrid nanofluid in a tube. J. Therm. Anal. Calorim. 2022, 147, 7917–7944. [Google Scholar] [CrossRef]
- Hsieh, S.S.; Liu, H.H.; Yeh, Y.F. Nanofluids spray heat transfer enhancement. Int. J. Heat Mass Transf. 2016, 94, 104–118. [Google Scholar] [CrossRef]
- Janaszek, A.; Nartowska, E.; Rozenvalde, A.; Zotovs, I. Comparison of the Use of Renewable Energy Sources (Res) by Poland and Latvia in the Perspective of the Environmental Objectives Set by the European Union. Civ. Environ. Eng. Rep. 2023, 33, 111–128. [Google Scholar] [CrossRef]
- Boujelbene, M.; Albouchi, F.; Ullah, Z.; Aldhabani, M.S.; Alsulami, S.H.; Hassan, A.M. Solar radiation and lower gravitational effects on wave oscillations in heat transfer along magnetic-driven porous cone in the presence of Joule heating. Case Stud. Therm. Eng. 2024, 53, 103782. [Google Scholar] [CrossRef]
- Boukholda, I.; Ullah, Z.; Mahrous, Y.M.; Alamer, A.; Amara, M.B.; Alsulami, M.D.; Faqihi, A.A.; Khedher, N.B. Analysis of thermal density and heat sink on dissipative nanofluid along magnetized sheet and applications in microelectronic cooling systems. Case Stud. Therm. Eng. 2024, 55, 104185. [Google Scholar] [CrossRef]
- Dąbek, L.; Kapjor, A.; Orman, Ł.J. Boiling heat transfer augmentation on surfaces covered with phosphor bronze meshes. MATEC Web. Conf. 2018, 168, 07001. [Google Scholar] [CrossRef][Green Version]
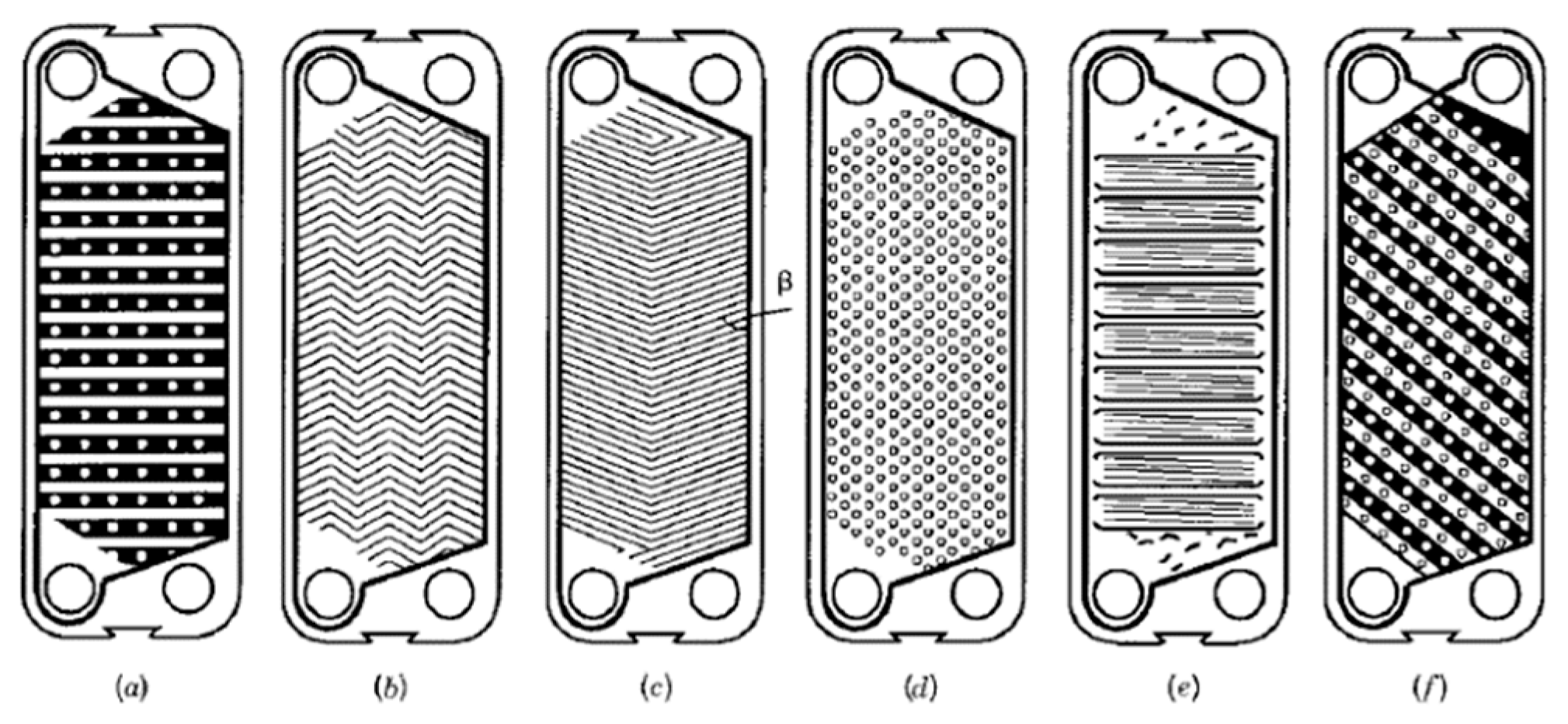

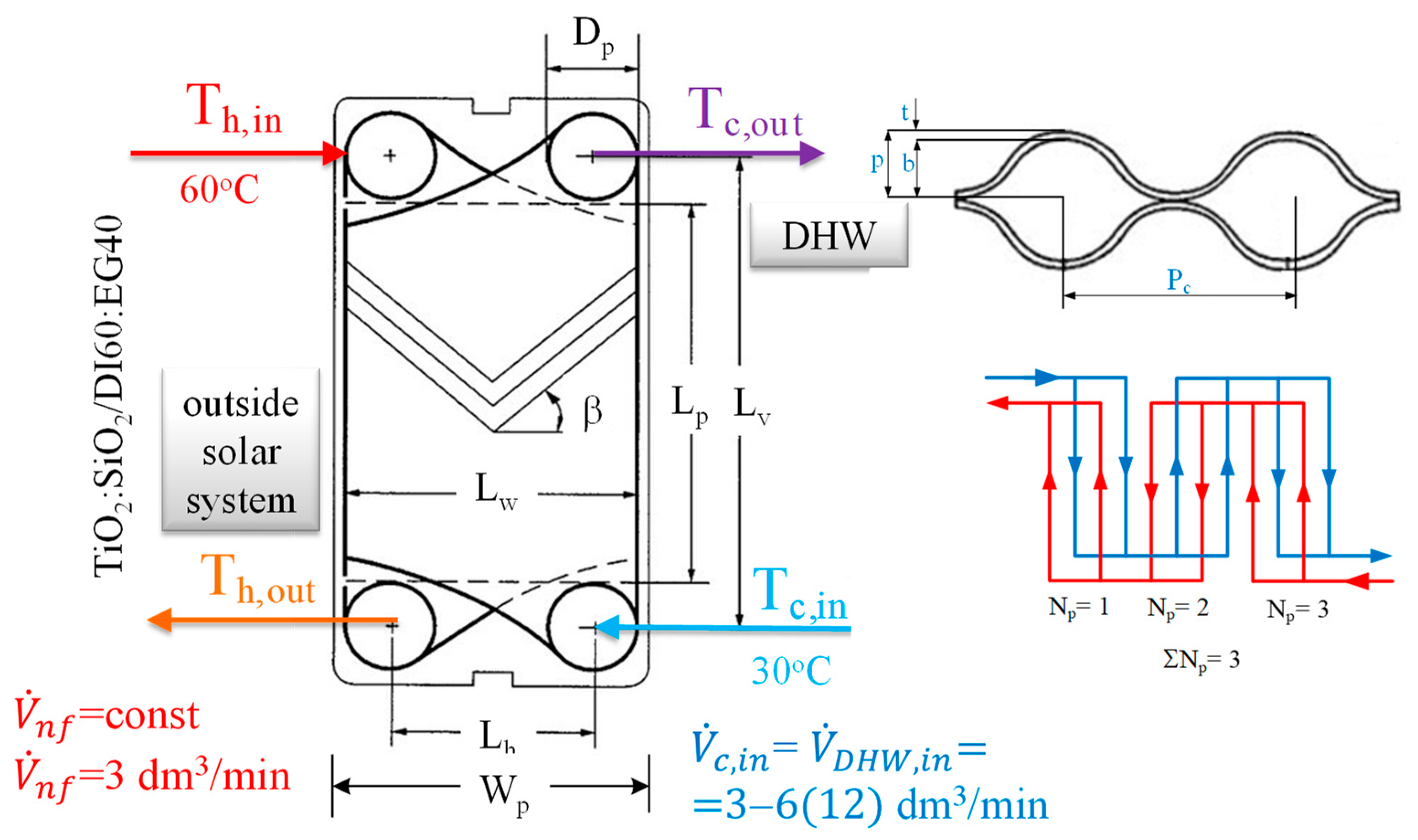





| Plate width between gaskets, m | Lw | 0.18 |
| Plate height between ports, m | Lv | 0.48 |
| Plate height between gaskets, m | Lp | 0.357 |
| Plate width between ports, m | Lh | 0.06 |
| Port diameter | Dp | 30 |
| Chevron angle, ° | β | 30 |
| Enhancement factor | ϕ | 1.15 |
| Surface area/heat transfer area, m2 | A | 0.3 |
| Corrugation pitch, mm | Pc | 14.2 |
| Mean channel spacing, mm | b | 2.8 |
| Plate pitch, mm | p | 2.8 |
| Plate thickness, mm | t | 0.45 |
| Total number of plates | 6 | |
| Pass number | 3 | |
| Thermal conductivity, W/mK | kp | 9.5 |
| No. | Working Fluid | Concentration, % vol. | Nanofluid Gross Costs, EUR | Nanoparticle Size, nm | Market Net Unit Price of Nanoparticle, EUR |
|---|---|---|---|---|---|
| of 1 dm3, EUR | |||||
| 1 | DI60:EG40 | - | 1.87 | - | - |
| 2 | TiO2:SiO2 | 0.5 | 137.25 | 4–8 10–25 | TiO2: 10 g~61 SiO2: 50 g~114 |
| 1.0 | 554.13 | ||||
| 1.5 | 1261.59 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wciślik, S.; Taler, D. Economic and Exergy Analysis of TiO2 + SiO2 Ethylene-Glycol-Based Hybrid Nanofluid in Plate Heat Exchange System of Solar Installation. Energies 2024, 17, 3107. https://doi.org/10.3390/en17133107
Wciślik S, Taler D. Economic and Exergy Analysis of TiO2 + SiO2 Ethylene-Glycol-Based Hybrid Nanofluid in Plate Heat Exchange System of Solar Installation. Energies. 2024; 17(13):3107. https://doi.org/10.3390/en17133107
Chicago/Turabian StyleWciślik, Sylwia, and Dawid Taler. 2024. "Economic and Exergy Analysis of TiO2 + SiO2 Ethylene-Glycol-Based Hybrid Nanofluid in Plate Heat Exchange System of Solar Installation" Energies 17, no. 13: 3107. https://doi.org/10.3390/en17133107
APA StyleWciślik, S., & Taler, D. (2024). Economic and Exergy Analysis of TiO2 + SiO2 Ethylene-Glycol-Based Hybrid Nanofluid in Plate Heat Exchange System of Solar Installation. Energies, 17(13), 3107. https://doi.org/10.3390/en17133107






