Assessing Open Circuit Voltage Losses in PEMFCs: A New Methodological Approach
Abstract
1. Introduction
2. Experiments
2.1. Experimental Set-Up
2.2. OCV Measurements
2.3. Stack Disassembly
3. OCV Calculation
3.1. OCV Calculation Methodology
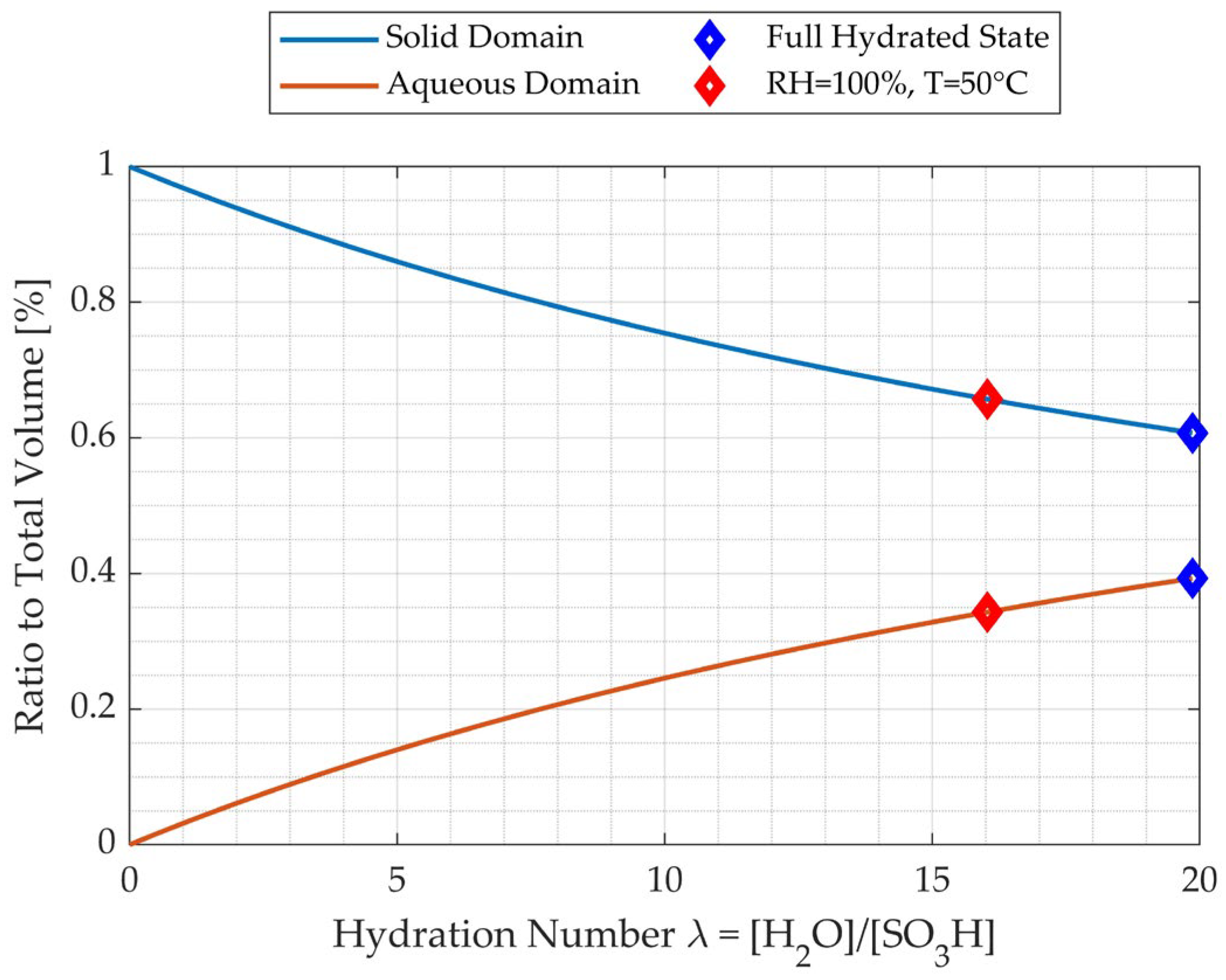
3.2. PEM Domain Division
3.3. Gas Crossover
- The hydrogen that permeates through the membrane does not react, releasing ions and electrons, thus leading to fuel inefficiency.
- The hydrogen that reaches the cathode compartment is subject to an oxidation-reduction reaction with oxygen, which causes local hotspots and degradation as well as a reduction in the oxygen available for the electrochemical reaction with a consequent decrease in the voltage of the FC.
3.3.1. Hydrogen Crossover Model
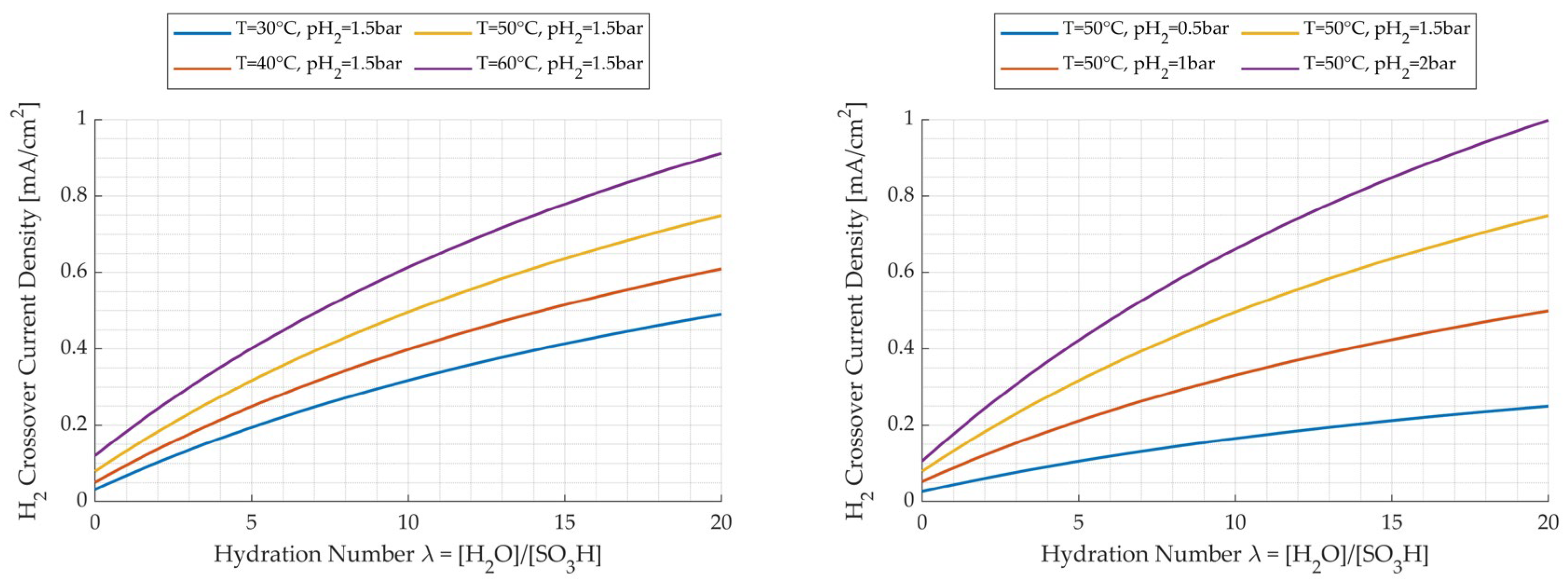
3.3.2. Influence of Pressure
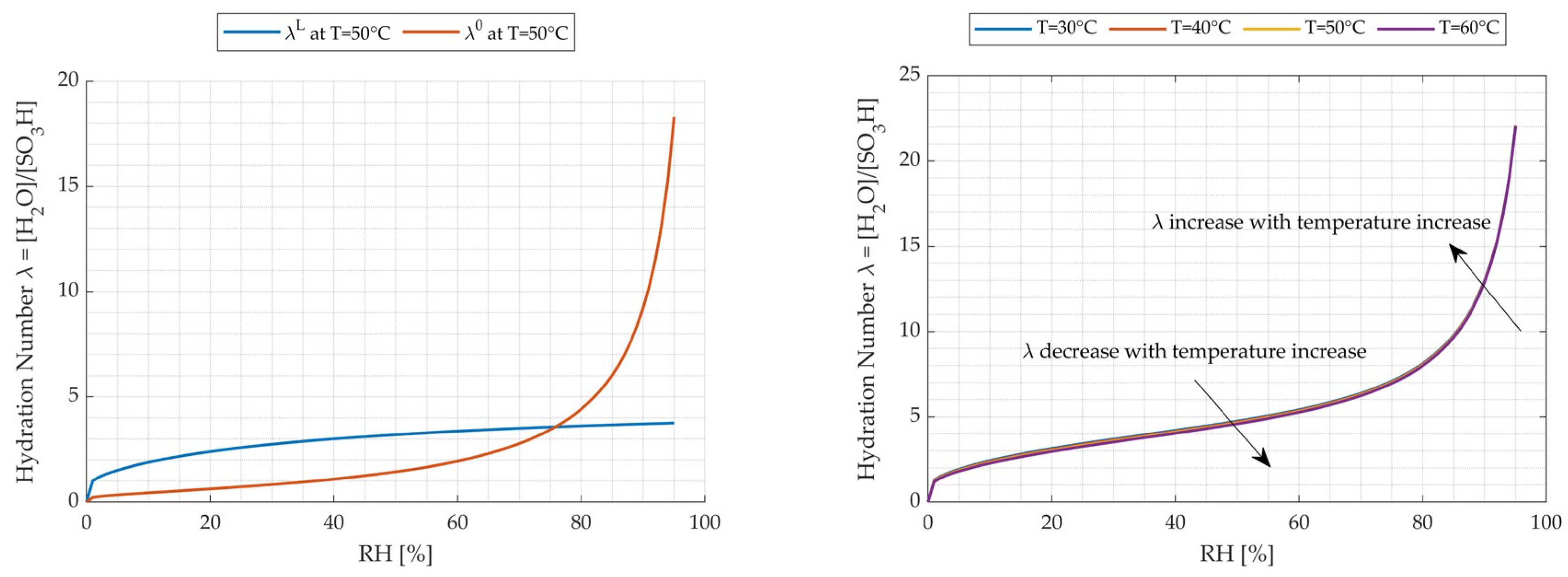
3.3.3. Influence of Relative Humidity (RH)
3.3.4. Influence of Temperature
3.3.5. Evaluation of the Overall Permeability Coefficient
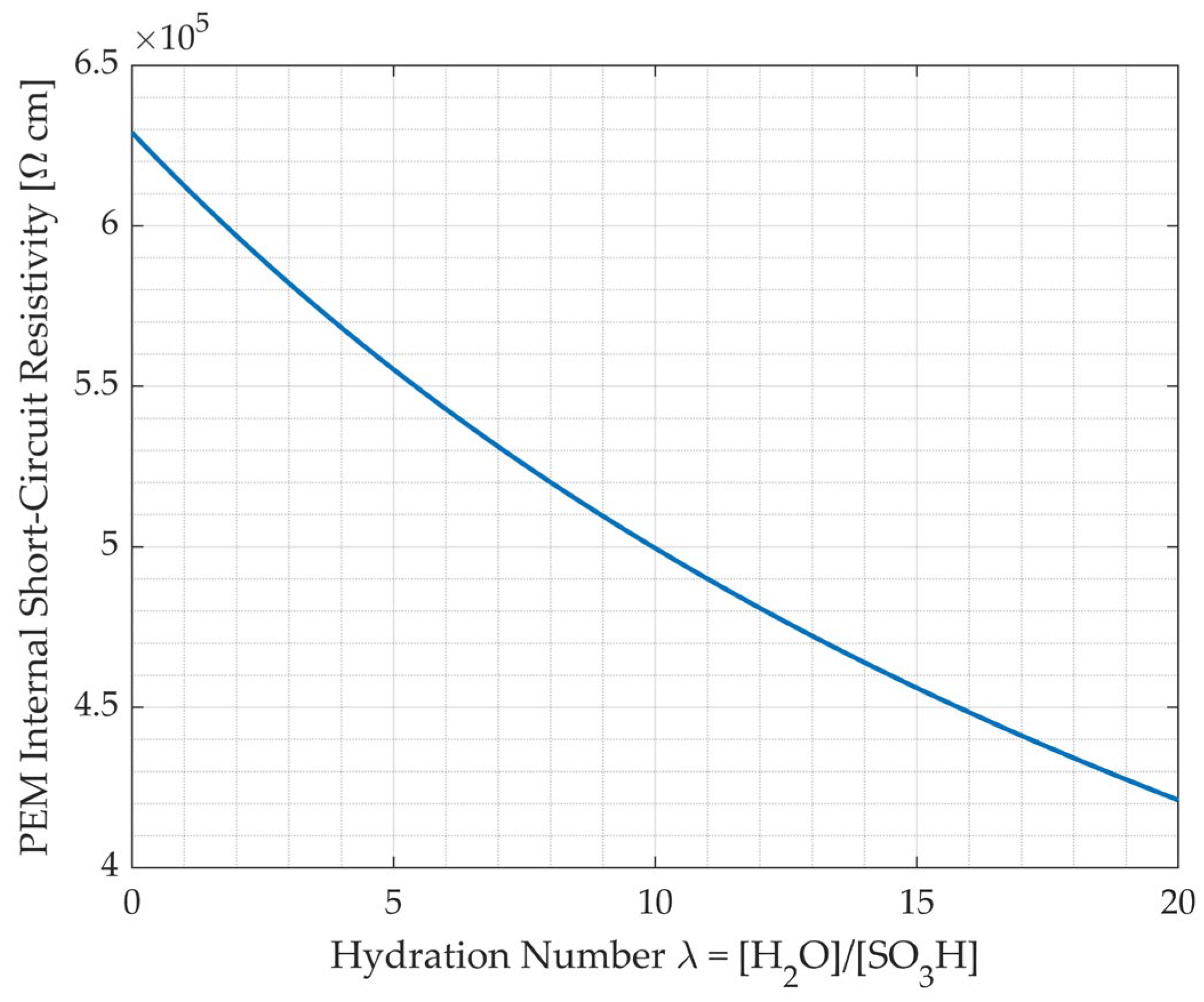
3.4. Internal Short-Circuits
Internal Short-Circuit Model
3.5. Mixed Potential Treatment
4. Results and Discussion
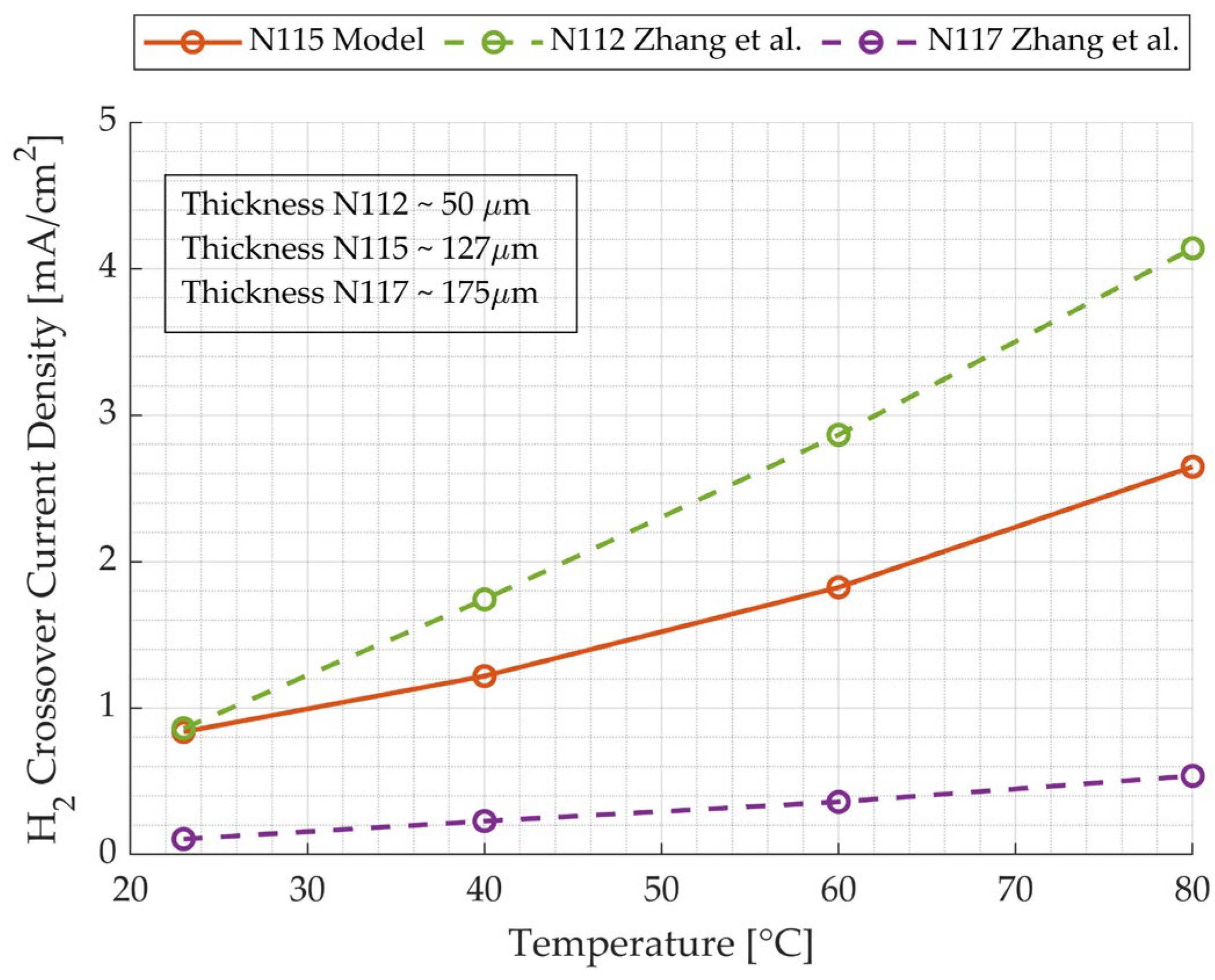
4.1. Hydrogen Crossover Model Results and Validation
4.2. Internal Short-Circuit Model Results
4.3. Hydrogen Crossover and Internal Short-Circuit Comparison
4.4. OCV Model Validation and Results
5. Conclusions
- Hydrogen crossover and internal short circuits increase as the aqueous domain increases, as the hydrogen permeability and electronic conductivity of water are higher than those of Nafion.
- The hydrogen crossover was modeled in a very accurate way, exploiting the data present in the literature, considering the dependence of the permeability of hydrogen in water and in Nafion with respect to temperature. The hydrogen crossover current density, the output of the model, was compared with values extracted from other literature studies with different membranes at different temperatures, confirming the validity of the results. Moreover, in the temperature range of 30–60 °C, the effect of pressure was more influential on hydrogen crossover losses.
- The impact of internal short circuits is notably significant when water quantities are low, whereas they are predominantly governed by the H2 crossover phenomenon under conditions of high water quantities. Nonetheless, it is noteworthy that they contribute to a comparable extent, representing approximately 20% of the open-circuit voltage (OCV) loss current.
- The OCV of the model perfectly follows the trend of the experimental one, decreasing as the temperature increases. This trend was also compared and validated with studies in the literature.
- The Nernst equation for calculating the OCV is not precise enough for modeling real PEMFC systems.
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Decarbonising Road Freight|Shell Global. Available online: https://www.shell.com/sustainability/our-climate-target/reducing-emissions-from-transport-and-industry/decarbonising-road-freight.html (accessed on 3 May 2024).
- CO2 Emissions in 2022—Analysis, IEA. Available online: https://www.iea.org/reports/co2-emissions-in-2022 (accessed on 3 May 2024).
- Global Hydrogen Review 2022—Analysis, IEA. Available online: https://www.iea.org/reports/global-hydrogen-review-2022 (accessed on 3 May 2024).
- Ball, M.; Weeda, M. The hydrogen economy—Vision or reality? Int. J. Hydrog. Energy 2015, 40, 7903–7919. [Google Scholar] [CrossRef]
- Pukrushpan, J.T.; Stefanopoulou, A.G.; Peng, H. Modeling and Control for PEM Fuel Cell Stack System. In Proceedings of the 2002 American Control Conference (IEEE Cat. No.CH37301), Anchorage, AK, USA, 8–10 May 2002; Volume 4, pp. 3117–3122. [Google Scholar] [CrossRef]
- Carello, M.; de Carvalho Pinheiro, H.; Longega, L.; Di Napoli, L. Design and Modelling of the Powertrain of a Hybrid Fuel Cell Electric Vehicle. SAE Int. J. Adv. Curr. Pract. Mobil. 2021, 3, 2878–2892. [Google Scholar] [CrossRef]
- Zhang, C.; Zhou, W.; Ehteshami, M.M.; Wang, Y.; Chan, S.H. Determination of the optimal operating temperature range for high temperature PEM fuel cell considering its performance, CO tolerance and degradation. Energy Convers. Manag. 2015, 105, 433–441. [Google Scholar] [CrossRef]
- Chen, C.-Y.; Lai, W.-H. Effects of temperature and humidity on the cell performance and resistance of a phosphoric acid doped polybenzimidazole fuel cell. J. Power Sources 2010, 195, 7152–7159. [Google Scholar] [CrossRef]
- Giner-Sanz, J.J.; Ortega, E.M.; Pérez-Herranz, V. Hydrogen crossover and internal short-circuit currents experimental characterization and modelling in a proton exchange membrane fuel cell. Int. J. Hydrog. Energy 2014, 39, 13206–13216. [Google Scholar] [CrossRef]
- Vilekar, S.A.; Datta, R. The effect of hydrogen crossover on open-circuit voltage in polymer electrolyte membrane fuel cells. J. Power Sources 2010, 195, 2241–2247. [Google Scholar] [CrossRef]
- Arato, E.; Costa, P. Transport mechanisms and voltage losses in PEMFC membranes and at electrodes: A discussion of open-circuit irreversibility. J. Power Sources 2006, 159, 861–868. [Google Scholar] [CrossRef]
- Kravos, A.; Ritzberger, D.; Hametner, C.; Jakubek, S.; Katrašnik, T. Methodology for efficient parametrisation of electrochemical PEMFC model for virtual observers: Model based optimal design of experiments supported by parameter sensitivity analysis. Int. J. Hydrog. Energy 2021, 46, 13832–13844. [Google Scholar] [CrossRef]
- Asensio, F.J.; San Martín, J.I.; Zamora, I.; Saldaña, G.; Oñederra, O. Analysis of electrochemical and thermal models and modeling techniques for polymer electrolyte membrane fuel cells. Renew. Sustain. Energy Rev. 2019, 113, 109283. [Google Scholar] [CrossRef]
- Lazar, A.L.; Konradt, S.C.; Rottengruber, H. Open-Source Dynamic Matlab/Simulink 1D Proton Exchange Membrane Fuel Cell Model. Energies 2019, 12, 3478. [Google Scholar] [CrossRef]
- Li, S.; Wei, X.; Jiang, S.; Yuan, H.; Ming, P.; Wang, X.; Dai, H. Hydrogen crossover diagnosis for fuel cell stack: An electrochemical impedance spectroscopy based method. Appl. Energy 2022, 325, 119884. [Google Scholar] [CrossRef]
- Fu, X.; Wei, J.; Ning, F.; Bai, C.; Wen, Q.; Jin, H.; Li, Y.; Zou, S.; Pan, S.; Chen, J.; et al. Highly flat and highly homogeneous carbon paper with ultra-thin thickness for high-performance proton exchange membrane fuel cell (PEMFC). J. Power Sources 2022, 520, 230832. [Google Scholar] [CrossRef]
- Nafion/PTFE Composite Membranes for a High Temperature PEM Fuel Cell Application|Industrial & Engineering Chemistry Research. Available online: https://pubs.acs.org/doi/10.1021/acs.iecr.1c01447 (accessed on 3 May 2024).
- Xu, Y.; Yang, Z.; Jiao, K.; Hao, D.; Du, Q. Development of a comprehensive transient fuel cell-battery hybrid system model and rule-based energy management strategy. Int. J. Green Energy 2023, 20, 844–858. [Google Scholar] [CrossRef]
- Guo, L.; Li, Z.; Outbib, R.; Gao, F. Function approximation reinforcement learning of energy management with the fuzzy REINFORCE for fuel cell hybrid electric vehicles. Energy AI 2023, 13, 100246. [Google Scholar] [CrossRef]
- H 500|Professional|Stack Fuel-Cell|H 500-2000|H2Planet—Re-Evolution Started—Hydrogen & Fuel-Cell Experience. Available online: https://www.h2planet.eu/it/detail/H500 (accessed on 3 May 2024).
- Shell Eco-Marathon. Available online: https://www.shellecomarathon.com/ (accessed on 3 May 2024).
- Carello, M.; De Vita, A.; Ferraris, A. Method for Increasing the Humidity in Polymer Electrolyte Membrane Fuel Cell. Fuel Cells 2016, 16, 157–164. [Google Scholar] [CrossRef]
- Ferraris, A.; Messana, A.; Airale, A.G.; Sisca, L.; de Carvalho Pinheiro, H.; Zevola, F.; Carello, M. Nafion tubing humidification system for polymer electrolyte membrane fuel cells. Energies 2019, 12, 1773. [Google Scholar] [CrossRef]
- Wang, Z.; Liu, Z.; Fan, L.; Du, Q.; Jiao, K. Application Progress of Small-Scale Proton Exchange Membrane Fuel Cell. Energy Rev. 2023, 2, 100017. [Google Scholar] [CrossRef]
- TESCOM Product Catalog Edition IV November 2018. Available online: https://www.emerson.com/cs-cz/automation/tescom (accessed on 4 June 2024).
- Hydrogen Flowmeter Red-y Compact 2 GCM, Mileage Challenge. Available online: https://mileage-challenge.com/flow-meters/32-flowmeter-red-y-compact-gcm.html (accessed on 26 May 2024).
- 2U, EA Elektro-Automatik. Available online: https://elektroautomatik.com/it/prodotti/serie-ea-10000/2u/ (accessed on 26 May 2024).
- Song, C.; Tang, Y.; Zhang, J.L.; Zhang, J.; Wang, H.; Shen, J.; McDermid, S.; Li, J.; Kozak, P. PEM fuel cell reaction kinetics in the temperature range of 23–120 °C. Electrochim. Acta 2007, 52, 2552–2561. [Google Scholar] [CrossRef]
- Arif, M.; Cheung, S.C.P.; Andrews, J. A systematic approach for matching simulated and experimental polarization curves for a PEM fuel cell. Int. J. Hydrog. Energy 2020, 45, 2206–2223. [Google Scholar] [CrossRef]
- Lee, H.; Han, C.; Park, T. Experimental investigation of charge transfer coefficient and exchange current density in standard fuel cell model for polymer electrolyte membrane fuel cells. Korean J. Chem. Eng. 2020, 37, 577–582. [Google Scholar] [CrossRef]
- Mennola, T.; Mikkola, M.; Noponen, M.; Hottinen, T.; Lund, P. Measurement of ohmic voltage losses in individual cells of a PEMFC stack. J. Power Sources 2002, 112, 261–272. [Google Scholar] [CrossRef]
- Springer, T.E.; Zawodzinski, T.A.; Gottesfeld, S. Polymer Electrolyte Fuel Cell Model. J. Electrochem. Soc. 1991, 138, 2334. [Google Scholar] [CrossRef]
- Kreuer, K.-D. The role of internal pressure for the hydration and transport properties of ionomers and polyelectrolytes. Solid State Ion. 2013, 252, 93–101. [Google Scholar] [CrossRef]
- Zhang, J.; Tang, Y.; Song, C.; Zhang, J.; Wang, H. PEM fuel cell open circuit voltage (OCV) in the temperature range of 23 °C to 120 °C. J. Power Sources 2006, 163, 532–537. [Google Scholar] [CrossRef]
- Gummalla, M.; Atrazhev, V.V.; Condit, D.; Cipollini, N.; Madden, T.; Kuzminyh, N.Y.; Weiss, D.; Burlatsky, S.F. Degradation of Polymer-Electrolyte Membranes in Fuel Cells: II. Theoretical model. J. Electrochem. Soc. 2010, 157, B1542. [Google Scholar] [CrossRef]
- Kaspar, R.B.; Wittkopf, J.A.; Woodroof, M.D.; Armstrong, M.J.; Yan, Y. Reverse-Current Decay in Hydroxide Exchange Membrane Fuel Cells. J. Electrochem. Soc. 2016, 163, F377. [Google Scholar] [CrossRef]
- Characterization of Gas Crossover and Its Implications in PEM Fuel Cells—Kocha—2006—AIChE Journal—Wiley Online Library. Available online: https://aiche.onlinelibrary.wiley.com/doi/full/10.1002/aic.10780 (accessed on 26 May 2024).
- Ye, D.; Gauthier, E.; Benziger, J.B.; Pan, M. Bulk and contact resistances of gas diffusion layers in proton exchange membrane fuel cells. J. Power Sources 2014, 256, 449–456. [Google Scholar] [CrossRef]
- Gas Permeation through Nafion. Part 1: Measurements|The Journal of Physical Chemistry C. Available online: https://pubs.acs.org/doi/10.1021/acs.jpcc.5b04155. (accessed on 3 May 2024).
- Eikerling, M.H.; Berg, P. Poroelectroelastic theory of water sorption and swelling in polymer electrolyte membranes. Soft Matter 2011, 7, 5976–5990. [Google Scholar] [CrossRef]
- Permeation of Gases and Vapours in Polymers|SpringerLink. Available online: https://link.springer.com/chapter/10.1007/978-94-009-4858-7_2. (accessed on 3 May 2024).
- Ito, H.; Maeda, T.; Nakano, A.; Takenaka, H. Properties of Nafion membranes under PEM water electrolysis conditions. Int. J. Hydrog. Energy 2011, 36, 10527–10540. [Google Scholar] [CrossRef]
- Wise, D.L.; Houghton, G. The diffusion coefficients of ten slightly soluble gases in water at 10–60 °C. Chem. Eng. Sci. 1966, 21, 999–1010. [Google Scholar] [CrossRef]
- Larminie, J.; Dicks, A. Fuel Cell Systems Explained, 2nd ed.; J. Wiley: Chichester, UK, 2003. [Google Scholar]
- Measurement and Prediction of Electrical Contact Resistance Between Gas Diffusion Layers and Bipolar Plate for Applications to PEM Fuel Cells|J. Electrochem. En. Conv. Stor|ASME Digital Collection. Available online: https://asmedigitalcollection.asme.org/electrochemical/article/1/1/2/463124/Measurement-and-Prediction-of-Electrical-Contact (accessed on 3 May 2024).
- Hristovski, K.; Dhanasekaran, B.; Tibaquirá, J.; Posner, J.; Westerhoff, P. Producing drinking water from hydrogen fuel cells. J. Water Supply Res. Technol.-AQUA 2009, 58, 327–335. [Google Scholar] [CrossRef]
- Matos, B.R. The genuine ac-to-dc proton conductivity crossover of nafion and polymer dielectric relaxations as a fuel cell polarization loss. J. Electroanal. Chem. 2020, 871, 114357. [Google Scholar] [CrossRef]
- Tawalbeh, M.; Alarab, S.; Al-Othman, A.; Javed, R.M.N. The Operating Parameters, Structural Composition, and Fuel Sustainability Aspects of PEM Fuel Cells: A Mini Review. Fuels 2022, 3, 28. [Google Scholar] [CrossRef]
- Dewangan, S.K.; Shrivastava, S.; Kadri, M.; Saruta, S.; Yadav, S.; Minj, N. Temperature effect on electrical conductivity(EC) & total dissolved solids (TDS) of water: A review. Int. J. Res. Anal. Rev. 2023, 10, 514–520. [Google Scholar]
- The Influence of Platinum Surface Oxidation on the Performance of a Polymer Electrolyte Membrane Fuel Cell—Probing Changes of Catalytically Active Surface Sites on a Polycrystalline Platinum Electrode for the Oxygen Reduction Reaction—Eckl—2022—Electrochemical Science Advances—Wiley Online Library. Available online: https://chemistry-europe.onlinelibrary.wiley.com/doi/full/10.1002/elsa.202100049 (accessed on 3 May 2024).
- Darling, R.M.; Meyers, J.P. Kinetic Model of Platinum Dissolution in PEMFCs. J. Electrochem. Soc. 2003, 150, A1523. [Google Scholar] [CrossRef]
- Schneider, P.; Sadeler, C.; Scherzer, A.-C.; Zamel, N.; Gerteisen, D. Fast and Reliable State-of-Health Model of a PEM Cathode Catalyst Layer. J. Electrochem. Soc. 2019, 166, F322. [Google Scholar] [CrossRef]
- Samris, A.; Mounir, H.; El Marjani, A. Effect of platinum dispersity, platinum loading and Pt-Oxide coverage on oxygen reduction reaction in PEMFC-Cathode. J. Electroanal. Chem. 2021, 895, 115414. [Google Scholar] [CrossRef]
- Dickinson, E.J.F.; Hinds, G. The Butler-Volmer Equation for Polymer Electrolyte Membrane Fuel Cell (PEMFC) Electrode Kinetics: A Critical Discussion. J. Electrochem. Soc. 2019, 166, F221. [Google Scholar] [CrossRef]
- Burye, T.E. Effect of PEM fuel cell exhaust water conductivity on catalyst degradation using thermal degradation resistant polymer membranes. Int. J. Hydrog. Energy 2020, 45, 11733–11748. [Google Scholar] [CrossRef]
- Martin, A.; Trinke, P.; Stähler, M.; Stähler, A.; Scheepers, F.; Bensmann, B.; Carmo, M.; Lehnert, W.; Hanke-Rauschenbach, R. The Effect of Cell Compression and Cathode Pressure on Hydrogen Crossover in PEM Water Electrolysis. J. Electrochem. Soc. 2022, 169, 014502. [Google Scholar] [CrossRef]
- Kuhnert, E.; Heidinger, M.; Sandu, D.; Hacker, V.; Bodner, M. Analysis of PEM Water Electrolyzer Failure Due to Induced Hydrogen Crossover in Catalyst-Coated PFSA Membranes. Membranes 2023, 13, 348. [Google Scholar] [CrossRef] [PubMed]
- Afshari, E.; Khodabakhsh, S.; Jahantigh, N.; Toghyani, S. Performance assessment of gas crossover phenomenon and water transport mechanism in high pressure PEM electrolyzer. Int. J. Hydrog. Energy 2021, 46, 11029–11040. [Google Scholar] [CrossRef]
- Dang, J.; Zhang, J.; Deng, X.; Yang, S.; Liu, B.; Zhu, X.; Li, Y.; Yang, F.; Ouyang, M. Hydrogen crossover measurement and durability assessment of high-pressure proton exchange membrane electrolyzer. J. Power Sources 2023, 563, 232776. [Google Scholar] [CrossRef]



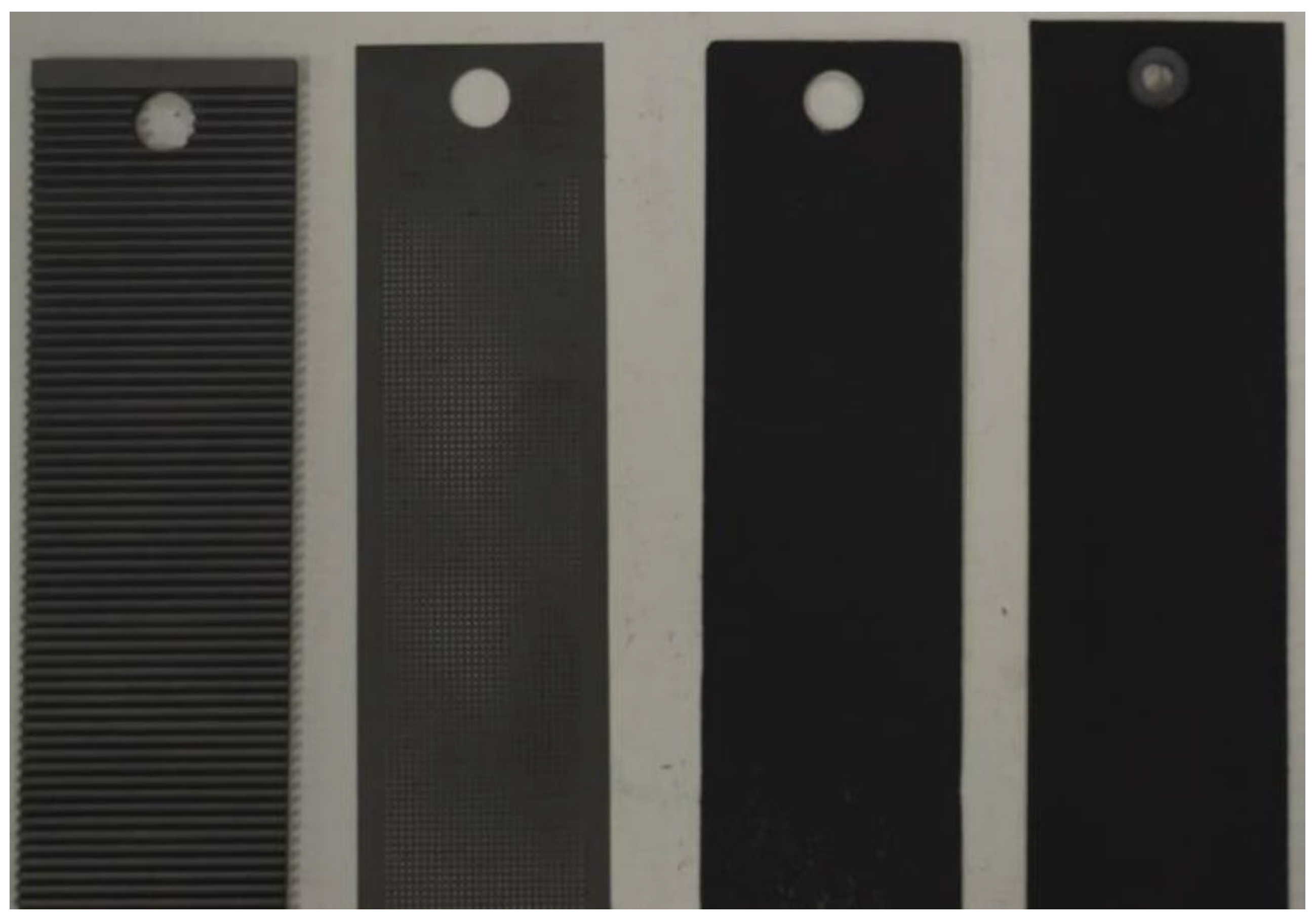




| Parameter | Value | Unit of Measurement |
|---|---|---|
| Temperature | 20–70 | [°C] |
| Air Pressure (being an open-cathode PEMFC using a fan to provide oxygen from external air, the air pressure is function of the angular velocity of the fan itself) | 0.1 | [barg] |
| H2 Pressure | 0.5 | [barg] |
| RH inlet Air (the air humidity was kept constant by the laboratory humidification system) | 50 | [%] |
| RH inlet H2 (pure hydrogen with no humidification system was used for the test) | 0 | [%] |
| Parameter | Value | Unit of Measurement |
|---|---|---|
| PEM thickness | ||
| Cathode flow field plat thickness | ||
| Anode flow field plate thickness | ||
| Active area |
| Parameter | Value | Unit of Measurement |
|---|---|---|
| H2O Molar Volume | 1.82 × 10−5 | [m3/mol] |
| Nafion 115 Density | 1900 | [kg/m3] |
| Nafion 115 Molar Volume | 5.79 × 10−4 | [m3/mol[SO3H]] |
| Nafion 115 EW | 1.10 | [kg/mol[SO3H]] |
| Parameter | Value | Unit of Measurement |
|---|---|---|
| H2O Molar Volume | 1.82 × 10−5 | [m3/mol] |
| Dry Nafion EA | 6.2 ± 0.1 | [10−20J] |
| Water EA | 2.68 | [10−20J] |
| Dry Nafion pre-exp. factor | 378 ± 18 | [10−8 mol cm−1s−1 bar−1] |
| Water pre-exp. factor | 3.17 | [10−8 mol cm−1s−1 bar−1] |
| Parameter | Value | Unit of Measurement |
|---|---|---|
| Electronic resistivity in dry Nafion 115 at 80 °C | 6.29 × 105 | [Ω cm] |
| Electronic resistivity in water at 80 °C | 1.013 × 105 | [Ω cm] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mazzeo, F.; Di Napoli, L.; Carello, M. Assessing Open Circuit Voltage Losses in PEMFCs: A New Methodological Approach. Energies 2024, 17, 2785. https://doi.org/10.3390/en17112785
Mazzeo F, Di Napoli L, Carello M. Assessing Open Circuit Voltage Losses in PEMFCs: A New Methodological Approach. Energies. 2024; 17(11):2785. https://doi.org/10.3390/en17112785
Chicago/Turabian StyleMazzeo, Francesco, Luca Di Napoli, and Massimiliana Carello. 2024. "Assessing Open Circuit Voltage Losses in PEMFCs: A New Methodological Approach" Energies 17, no. 11: 2785. https://doi.org/10.3390/en17112785
APA StyleMazzeo, F., Di Napoli, L., & Carello, M. (2024). Assessing Open Circuit Voltage Losses in PEMFCs: A New Methodological Approach. Energies, 17(11), 2785. https://doi.org/10.3390/en17112785






