Grid-Forming Control: Advancements towards 100% Inverter-Based Grids—A Review
Abstract
:1. Introduction
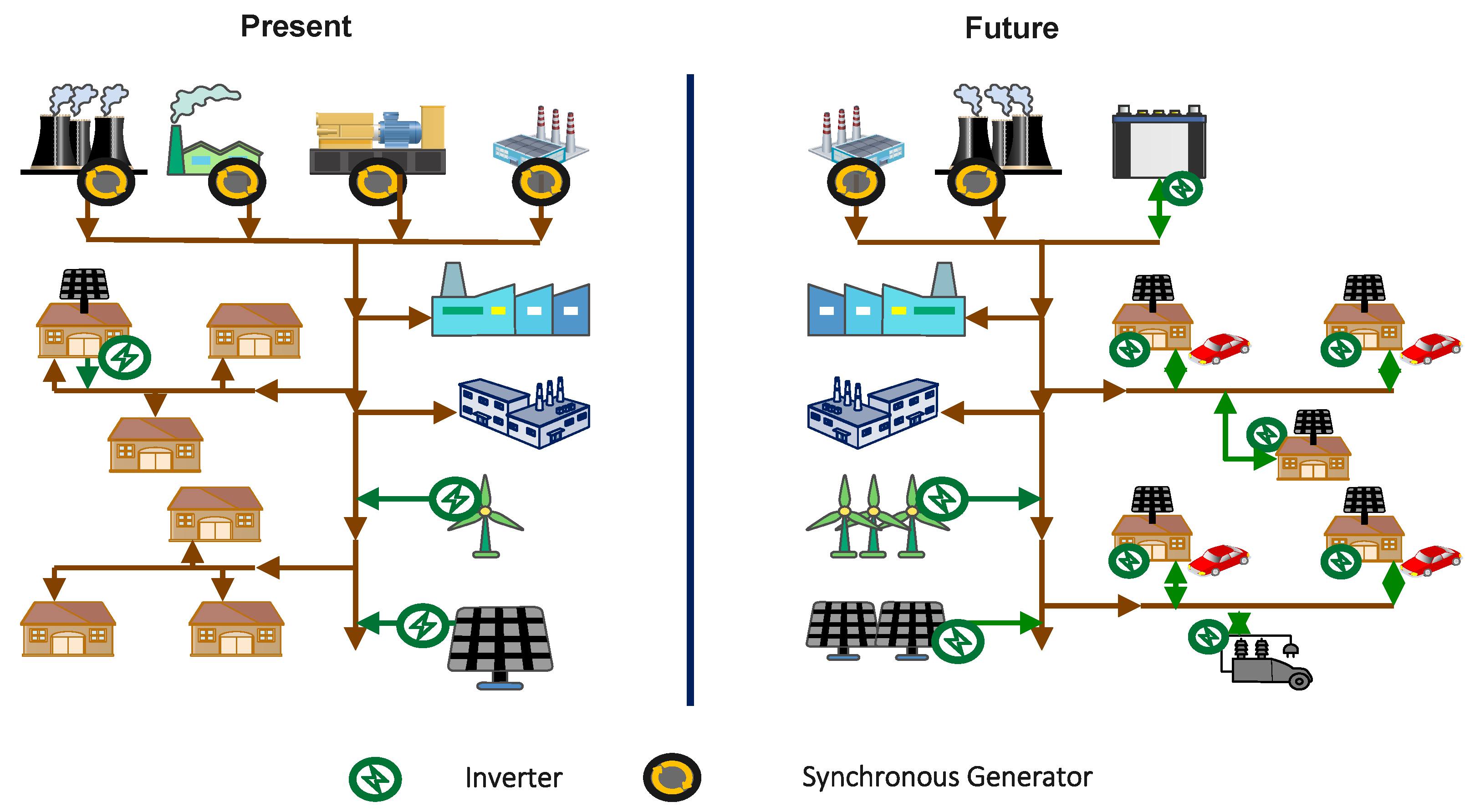
2. The Changing Dynamics of the Grid
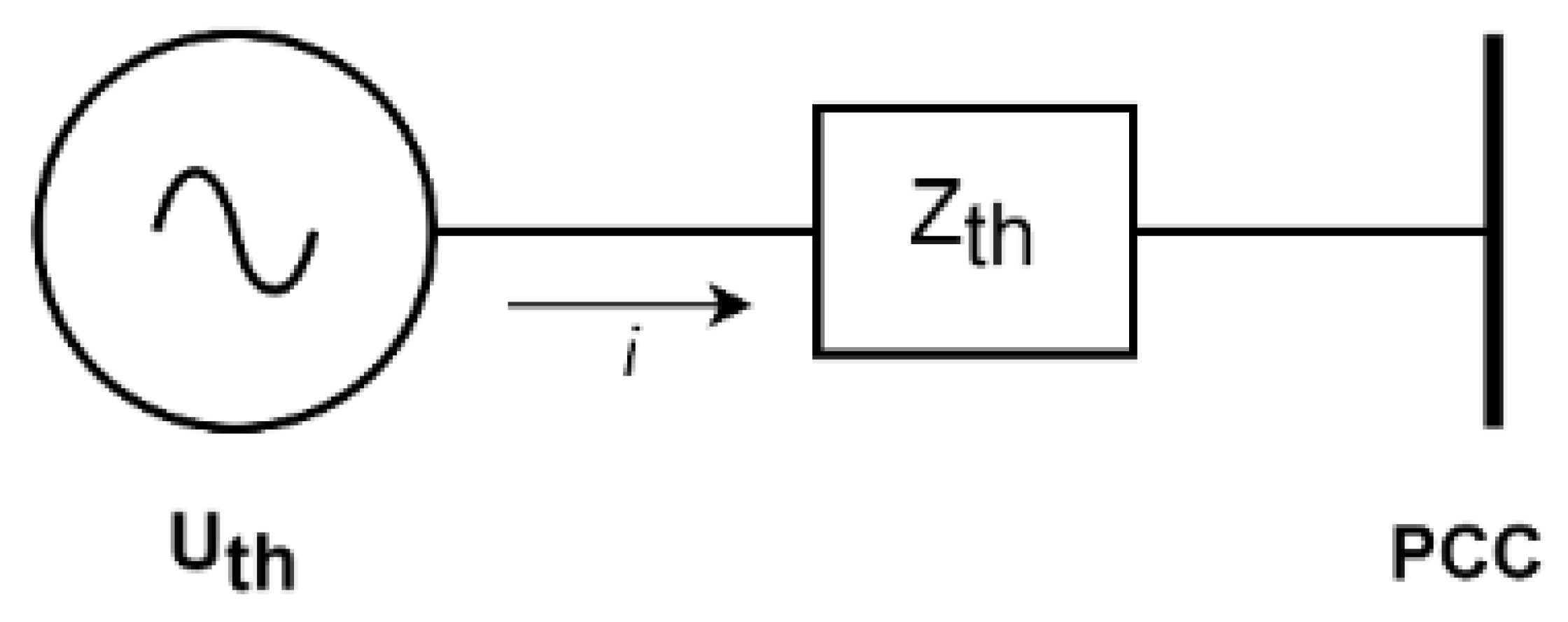
2.1. SCR and Grid Strength
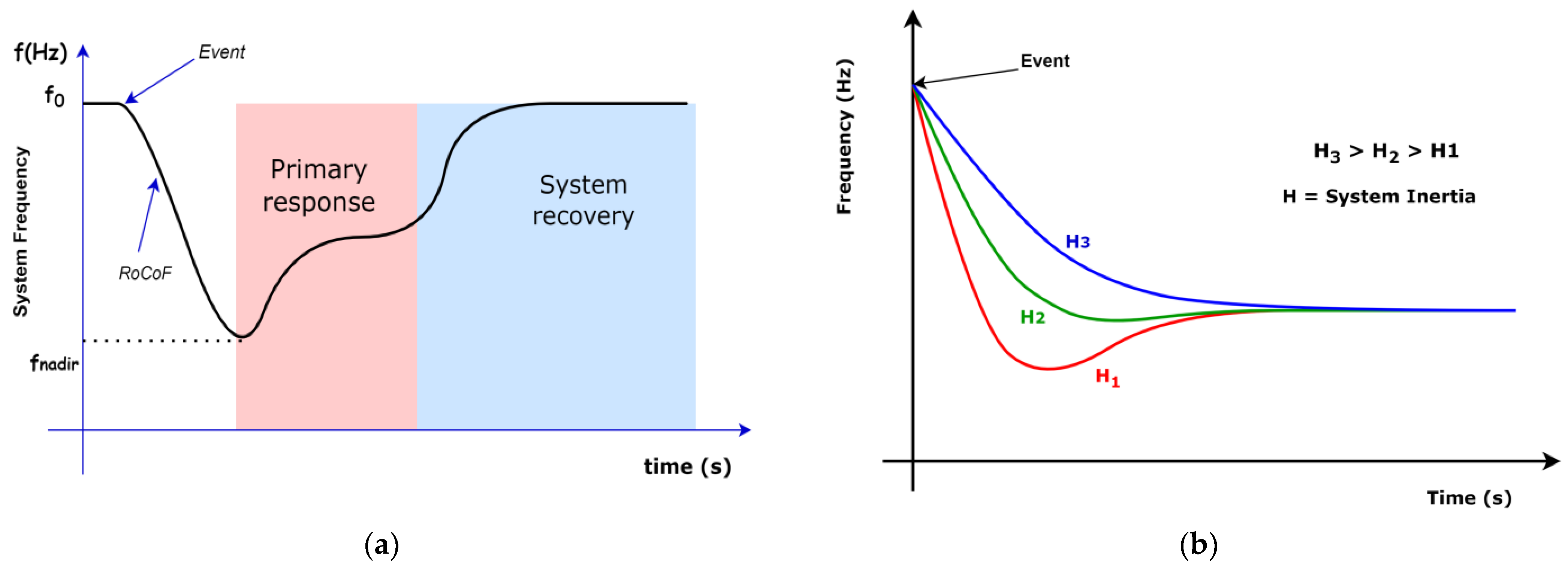
2.2. Low-Inertia Systems
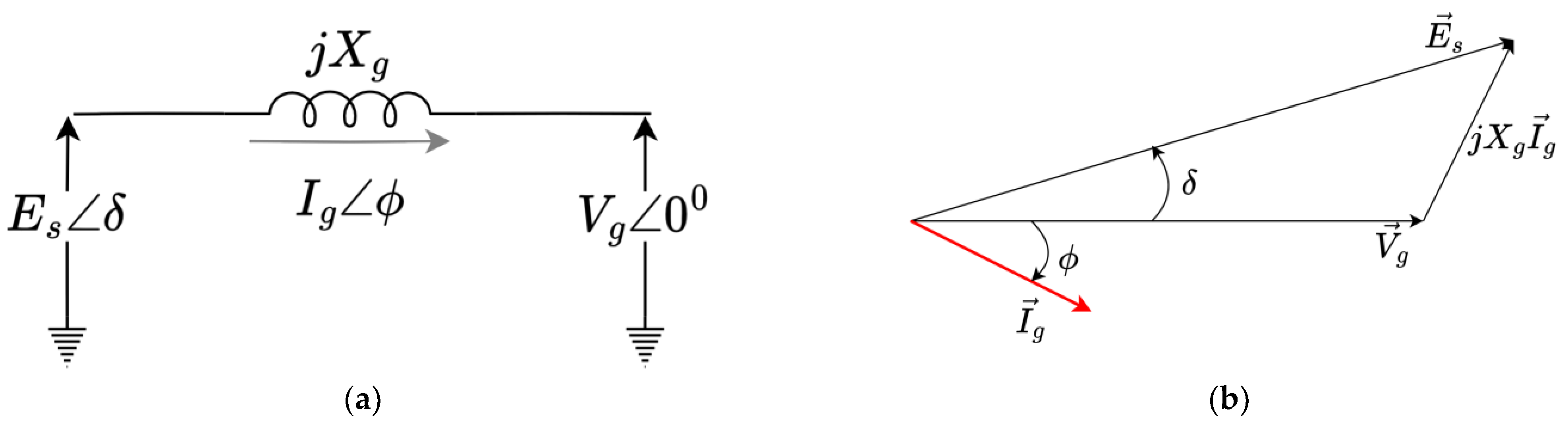
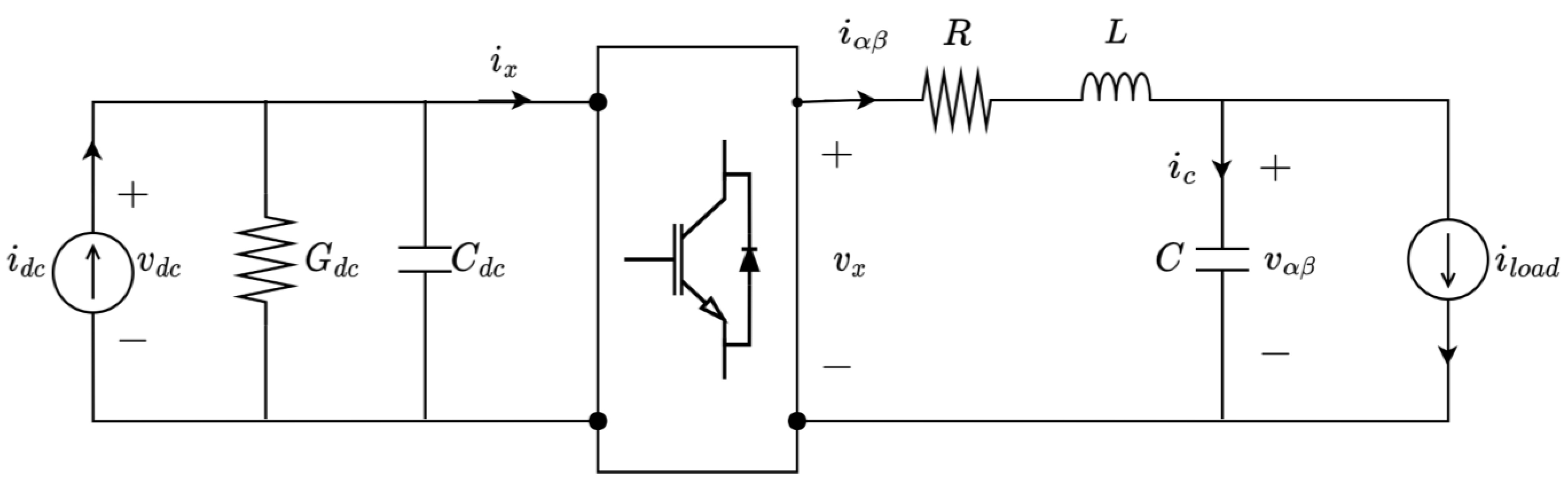
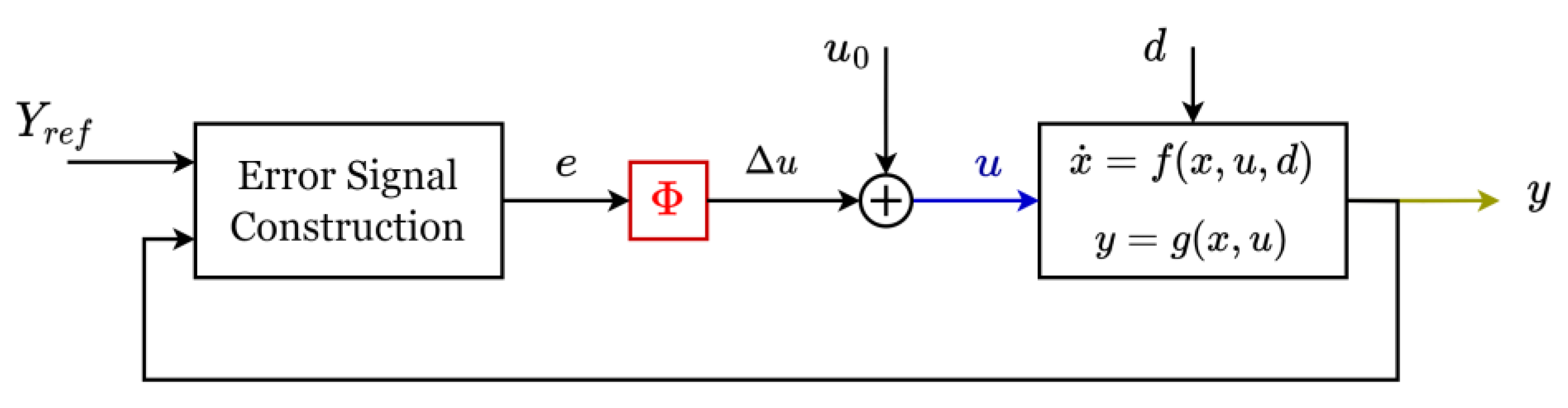
3. Grid-Connected Inverter Control Classification
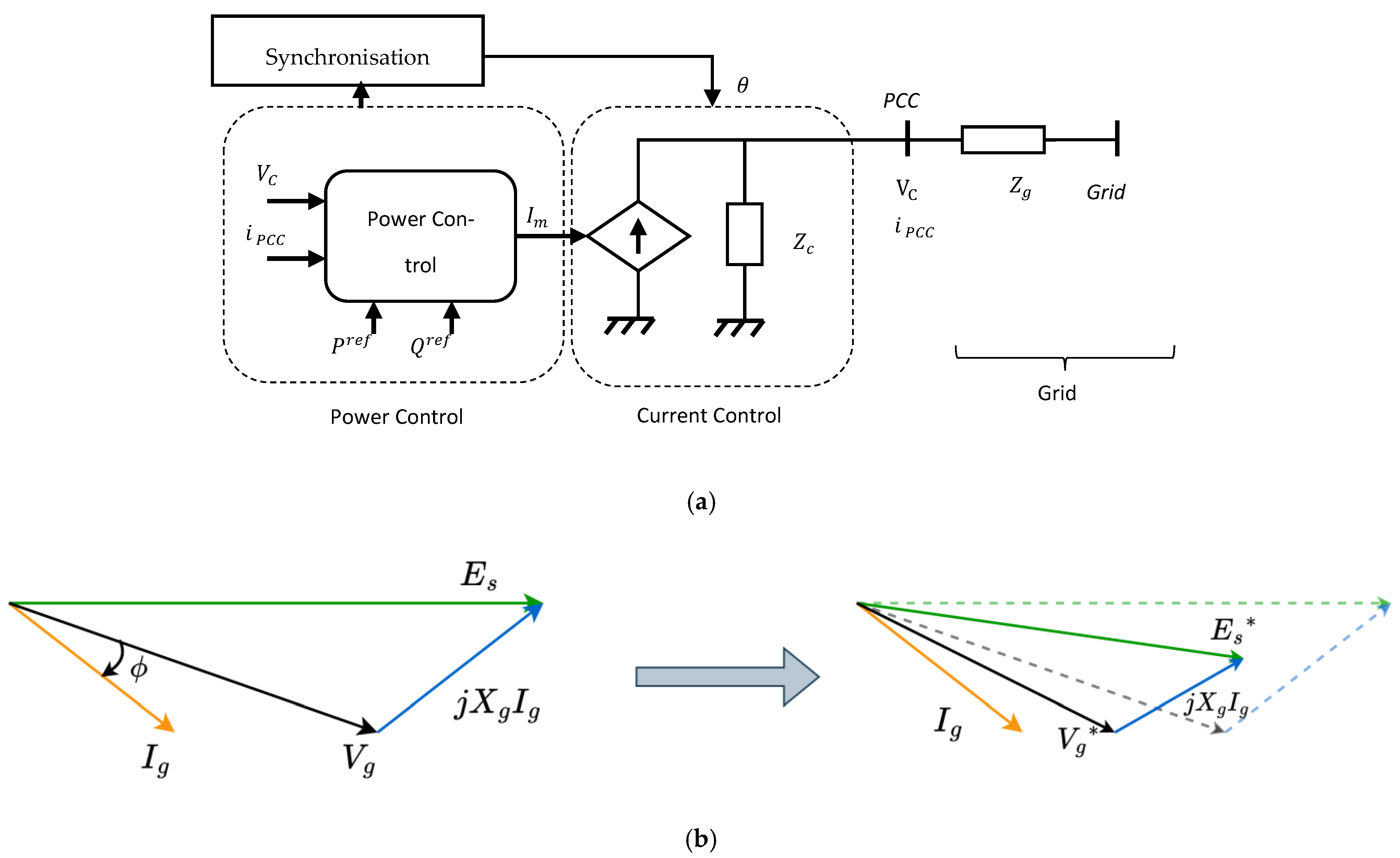
3.1. GFL Control
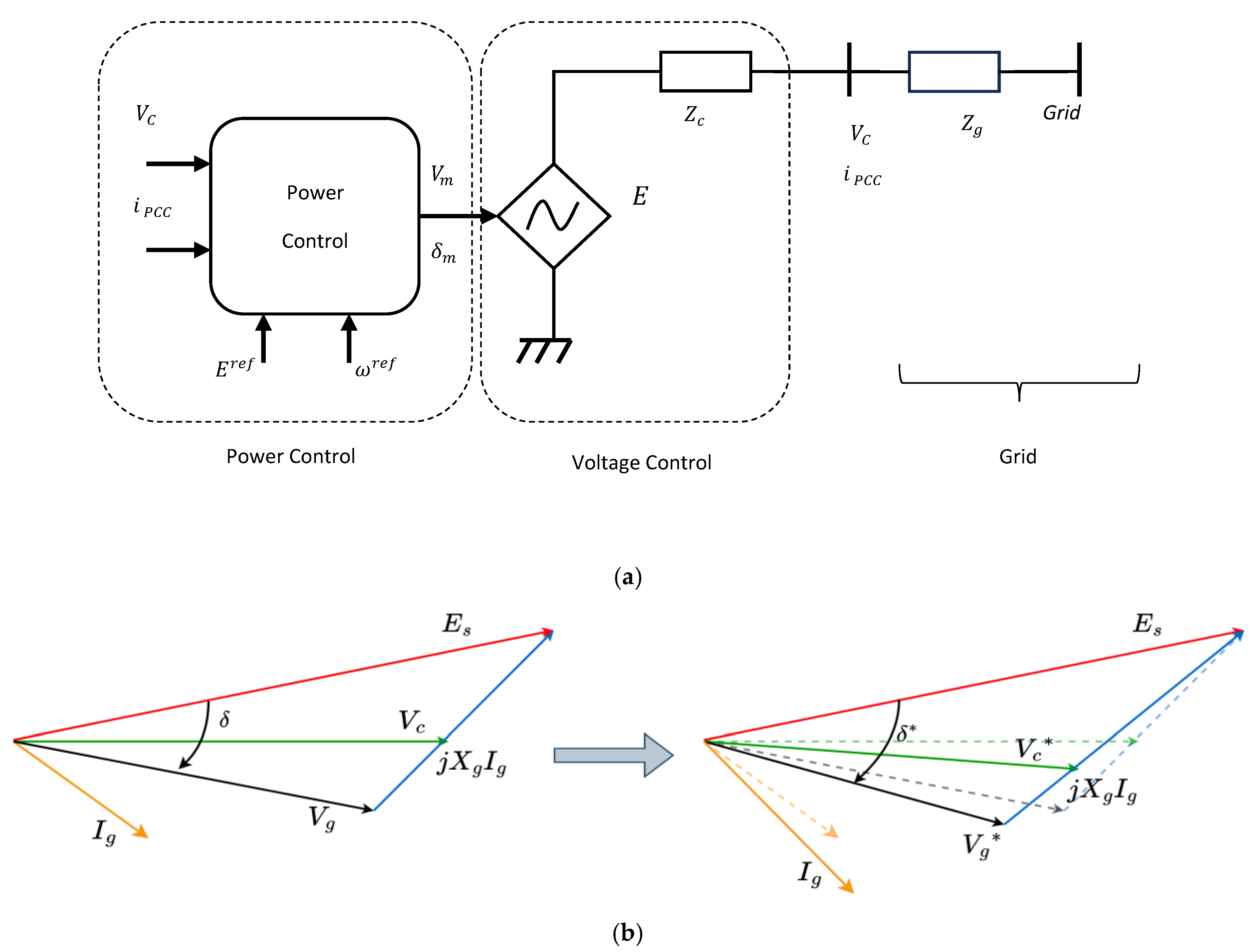
3.2. GFM Control
3.3. Comparison between Grid-Following and Grid-Forming Inverter Control
4. GFM Control Approaches
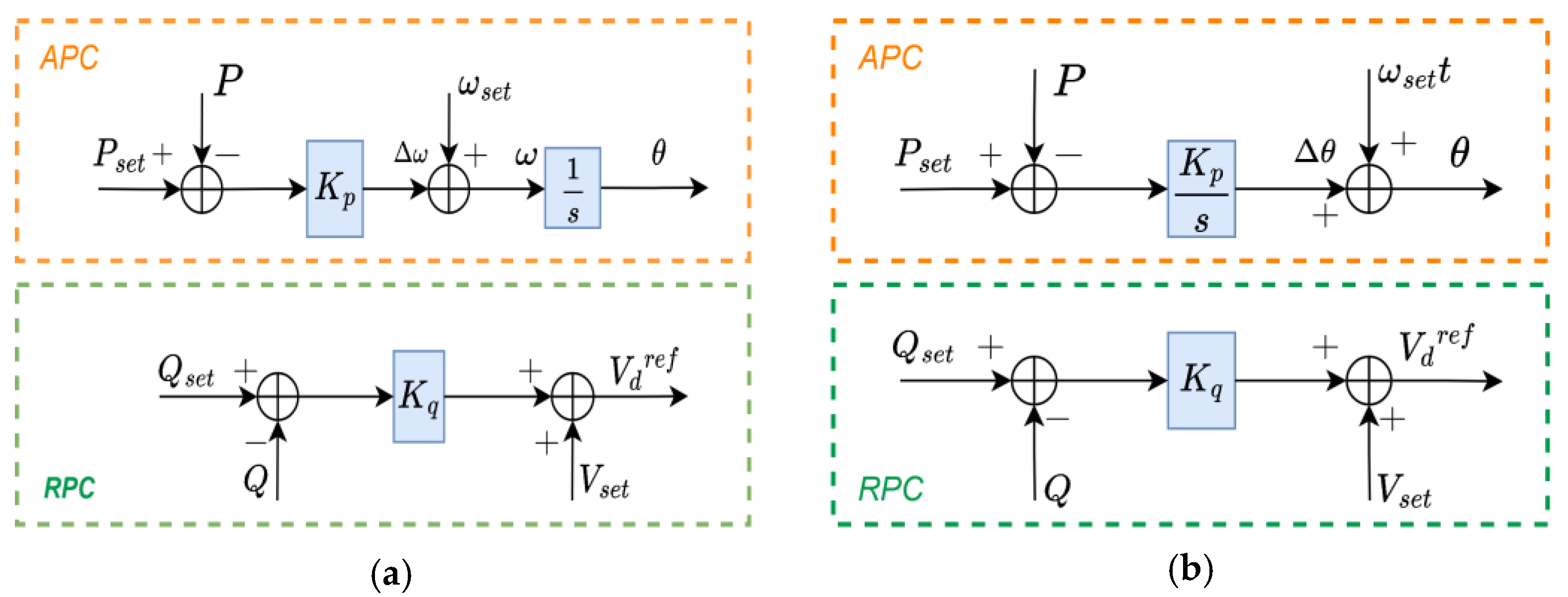
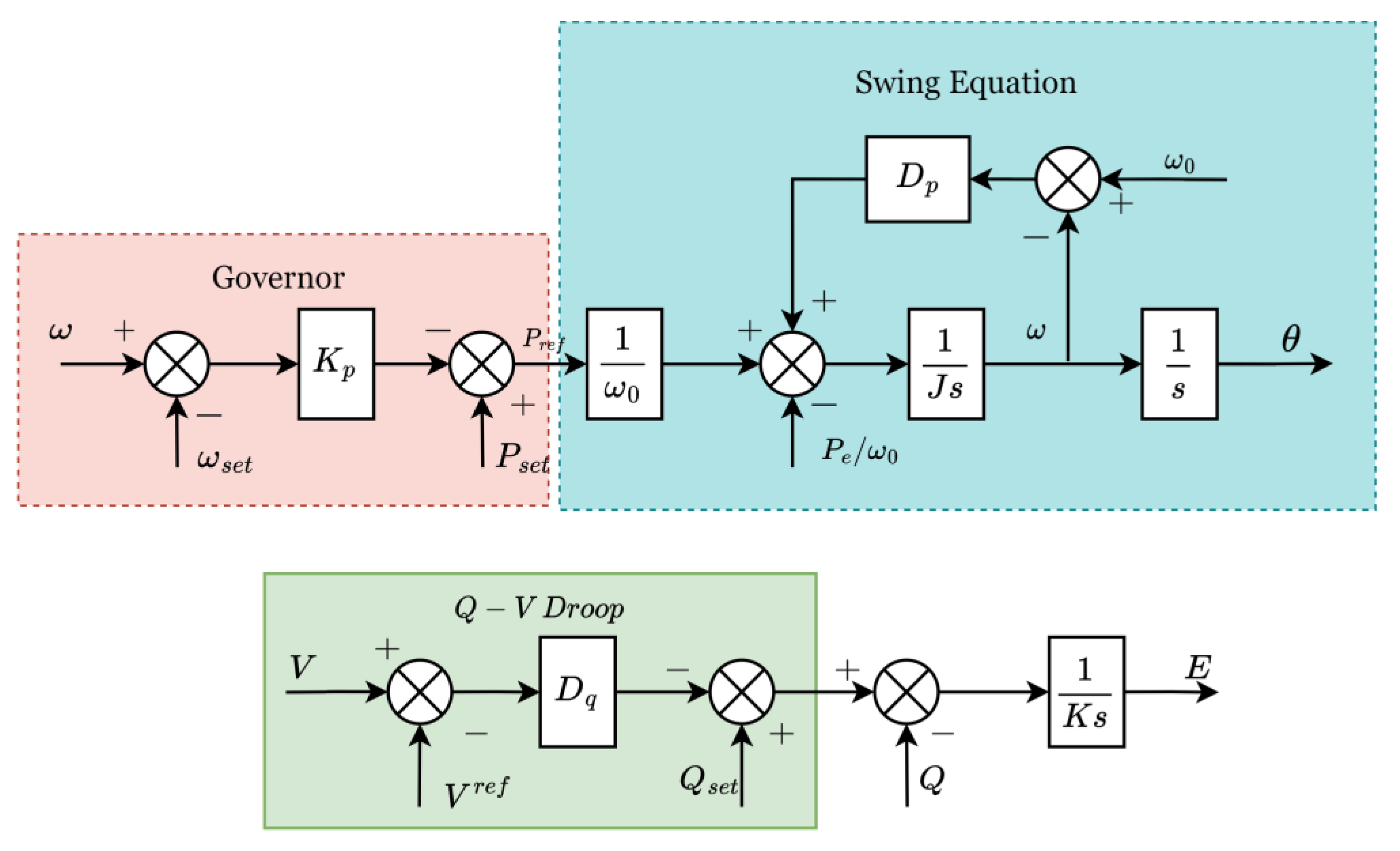
4.1. Droop Control
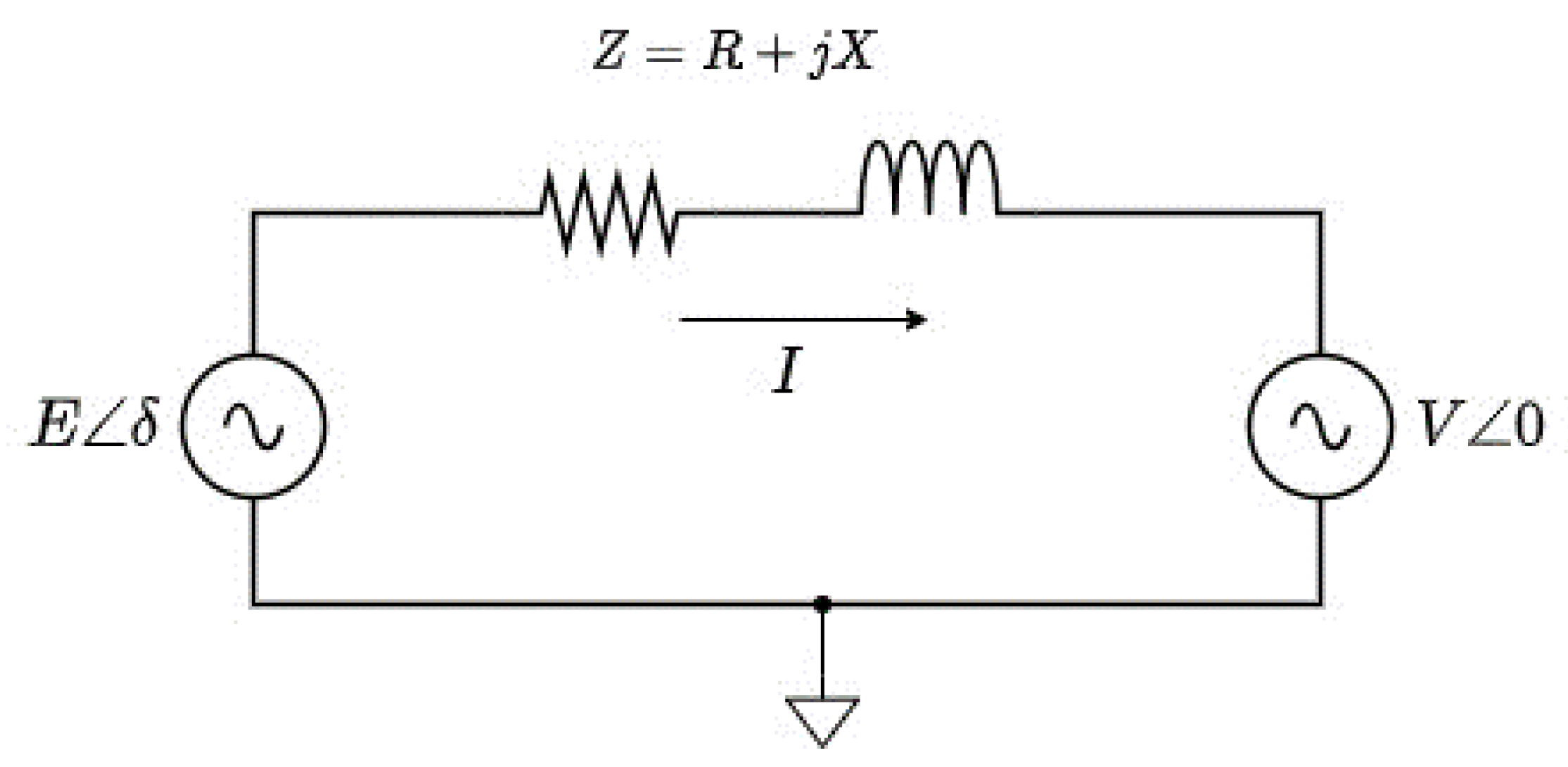
4.2. Power Synchronisation Control (PSC)
4.3. Synchronous Machine Emulation Controllers
4.3.1. Virtual Synchronous Generator (VSG) Control
4.3.2. Synchronverter
4.4. Matching Control
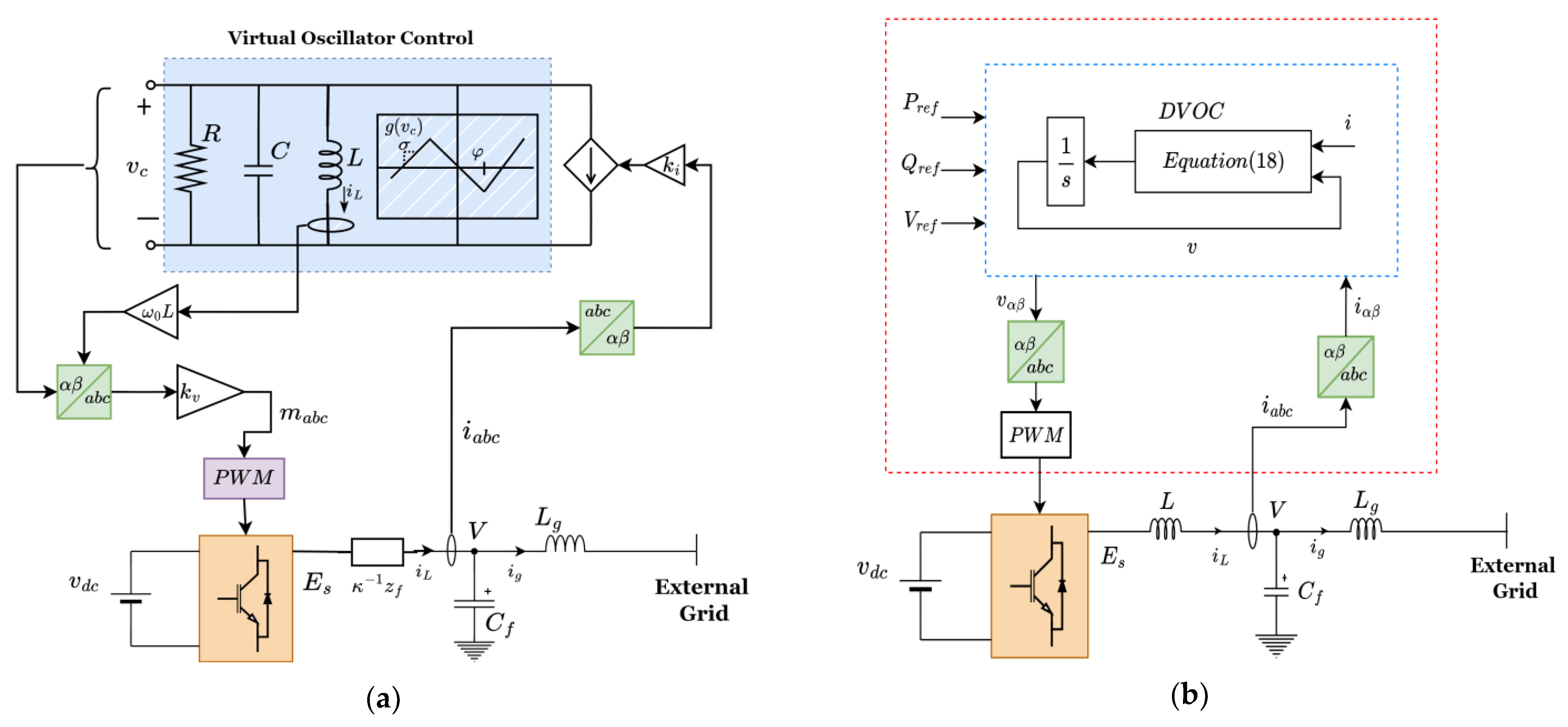
4.5. Virtual Oscillator Control (VOC)
- Parallel tank, which determines the resonant frequency that sets the system frequency.
- represents a voltage-dependent source. The value of its maximum slope, , is related to the resistance, used for damping.
- The constant is the voltage scaling factor related to the grid RMS value, and and are used to ensure that the voltage of the inverter is within the limits for safe supply to load. is related to the power rating of the GFM in proportion to other inverters within the network.
5. State-of-the-Art GFM Controller Designs
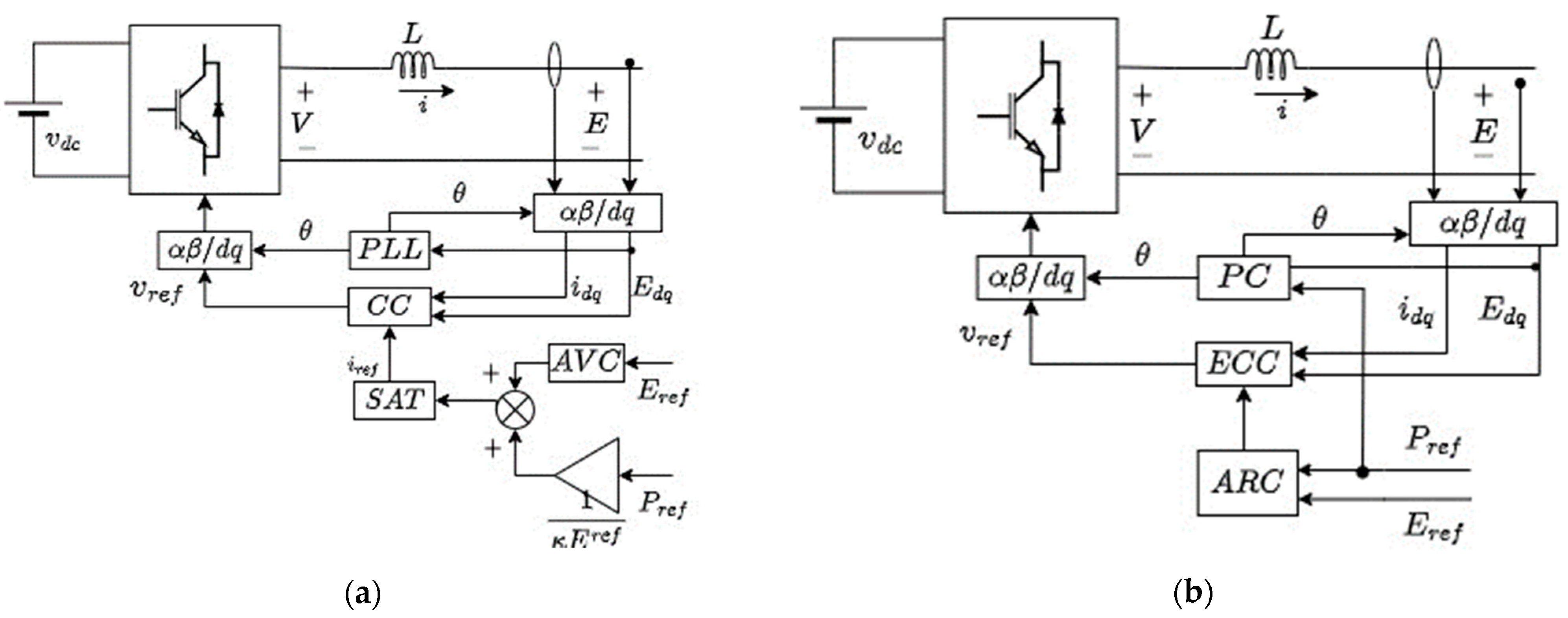
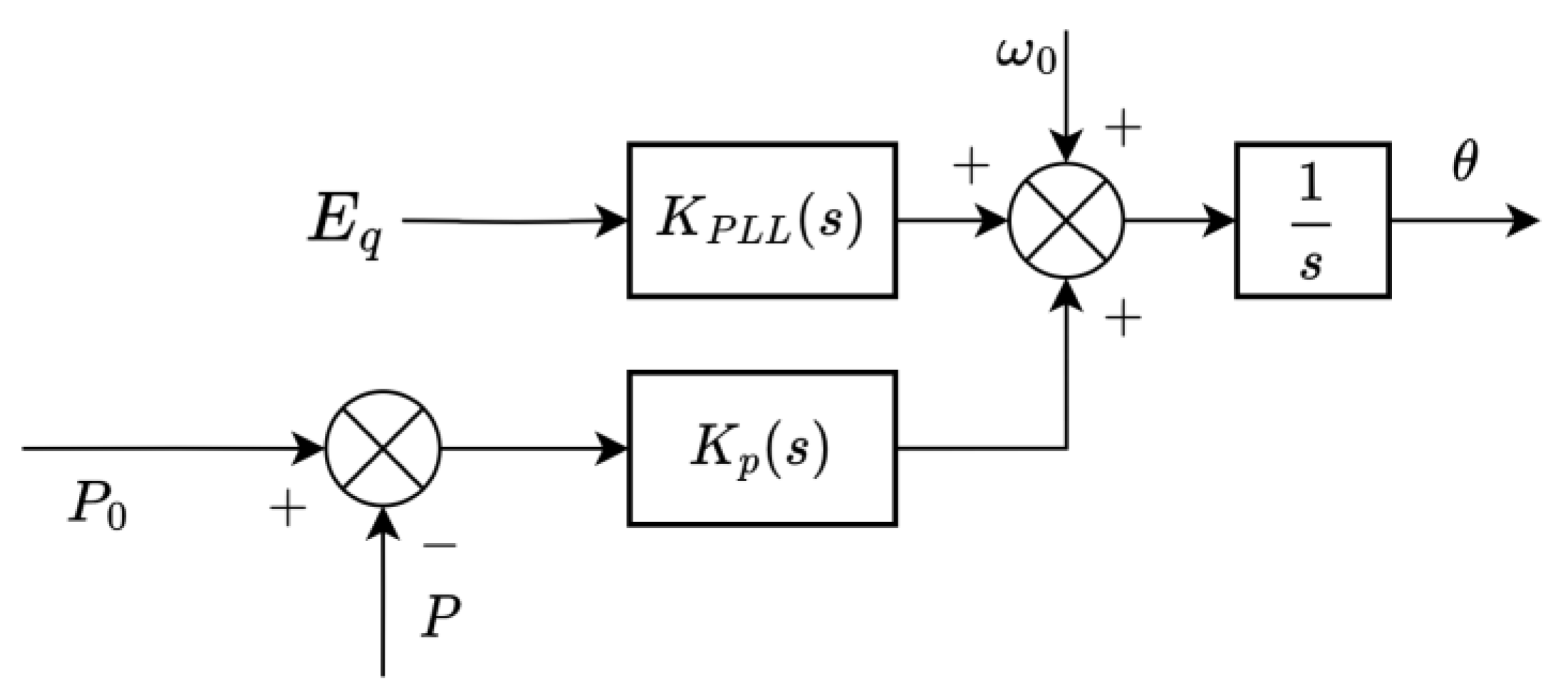
5.1. Hybrid Synchronisation Control
- PC and PLL integration
- 2.
- Modification of AVC
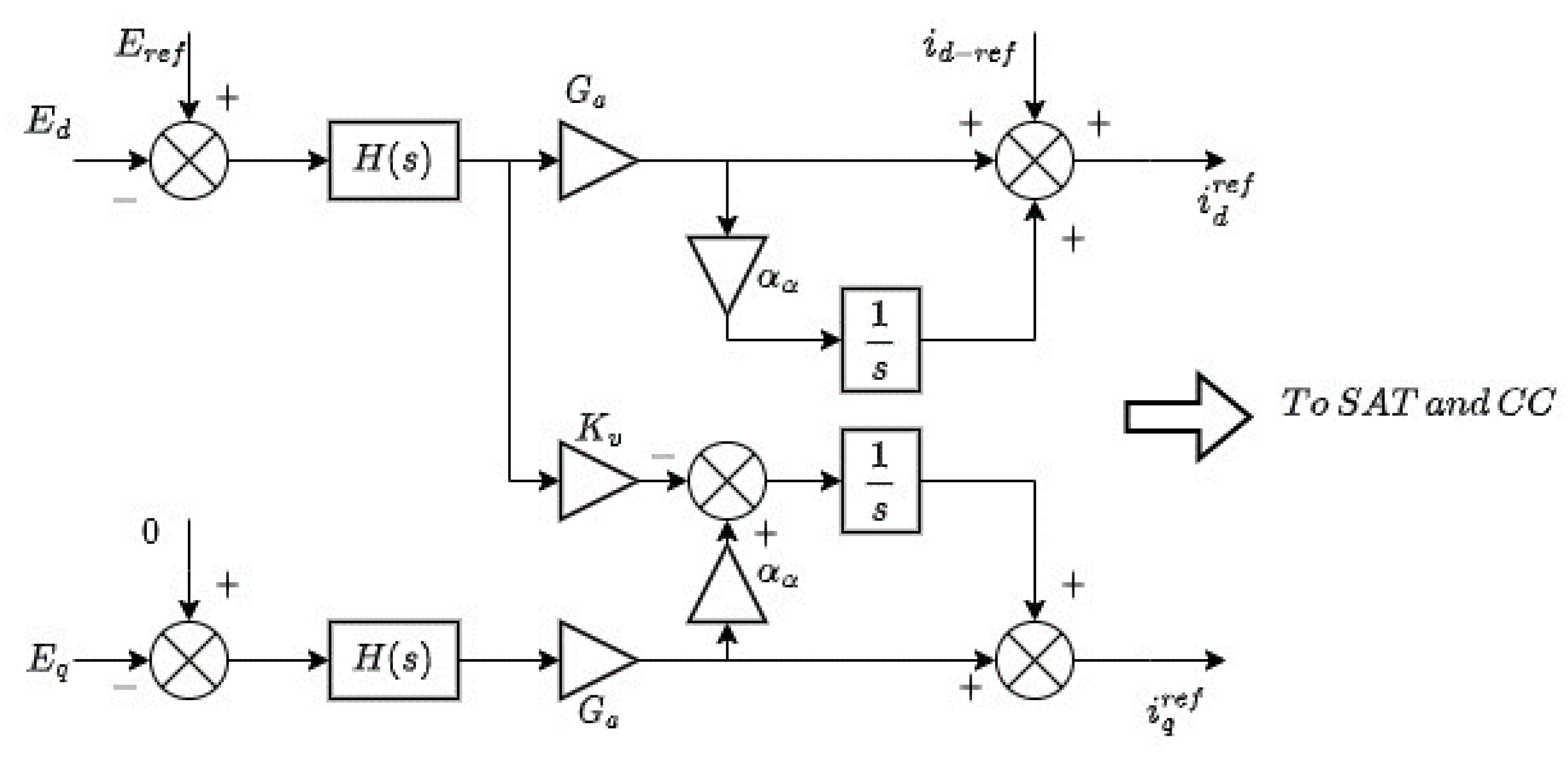
5.2. Unified Virtual Oscillator Controller (uVOC)
- GFL operation
- b.
- GFM operation
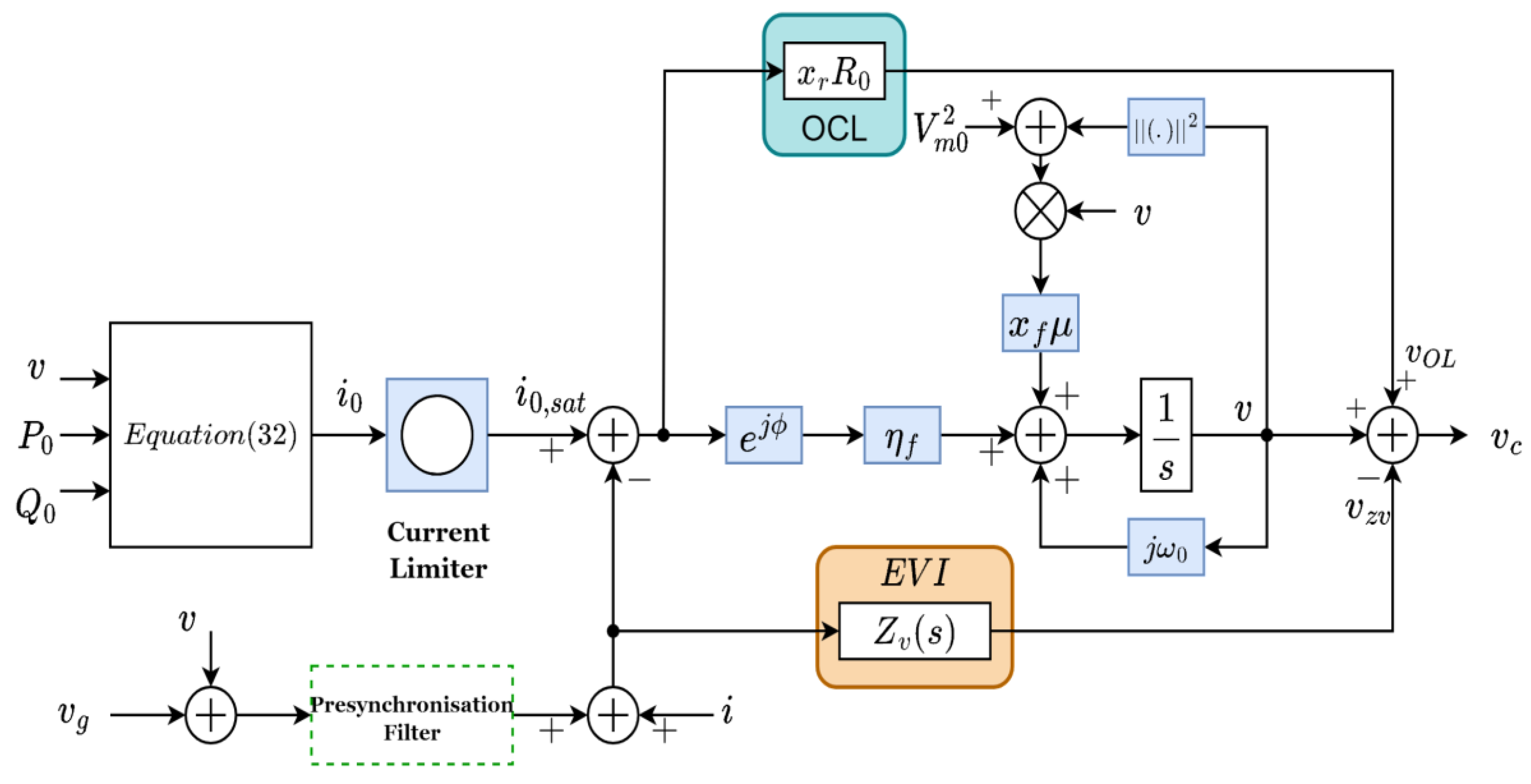
5.3. Universal Control Scheme (UniCon)
5.3.1. Rapid Phase Jump Algorithm
5.3.2. Fault Ride-through Capability
- Overcurrent protection mechanism:
- Dynamic virtual impedance:
- Fault recovery:
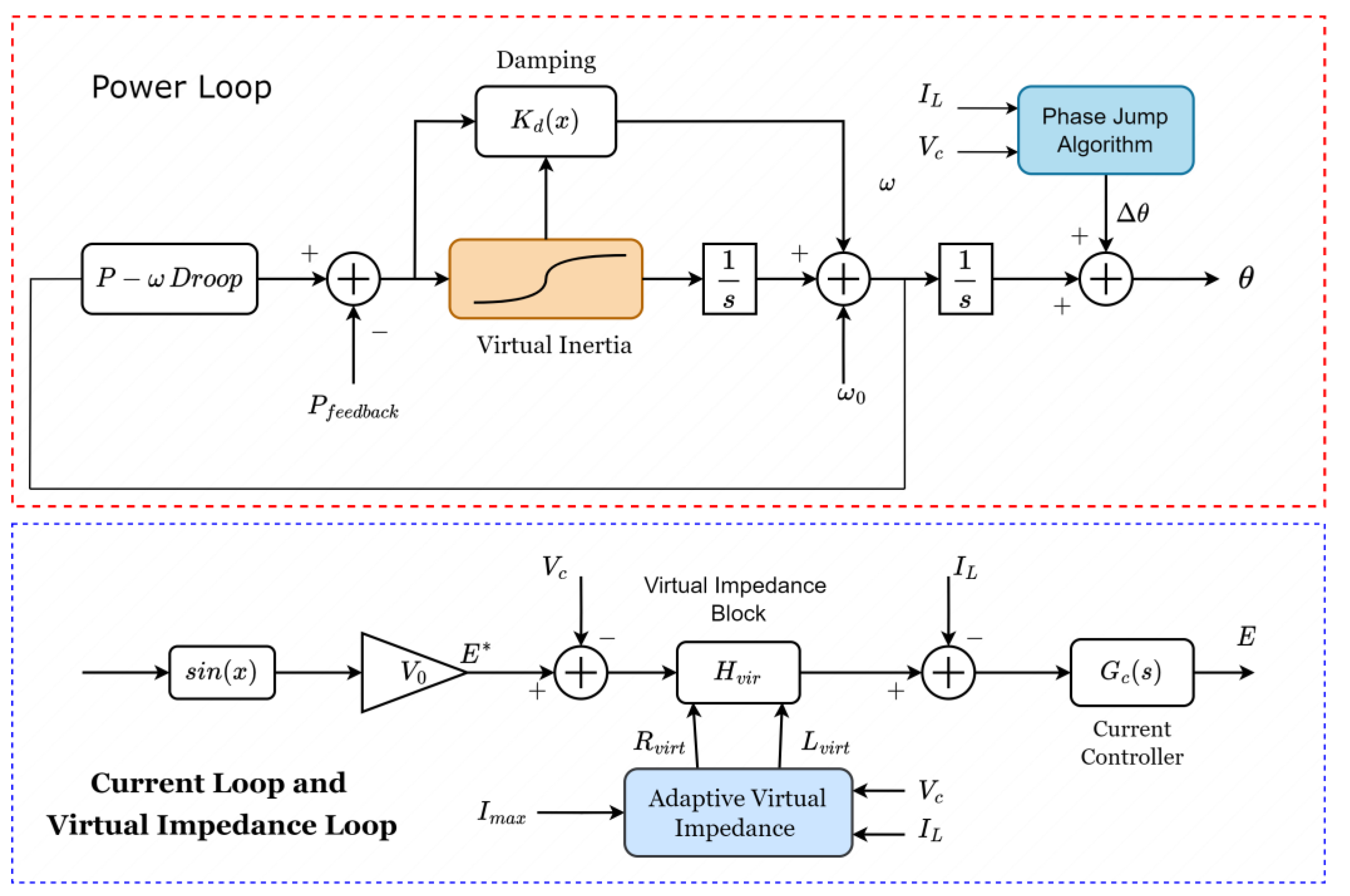
5.3.3. Variable Inertia and Damping
5.4. Multiple Input–Multiple Output (MIMO) GFM Converters
5.5. Other Methods
6. Issues Affecting Inverter Controllers and Their Mitigation Approaches
6.1. Power Coupling in Inverter Controls
6.2. Overcurrent Protection in GFMs
6.2.1. Mode-Switching
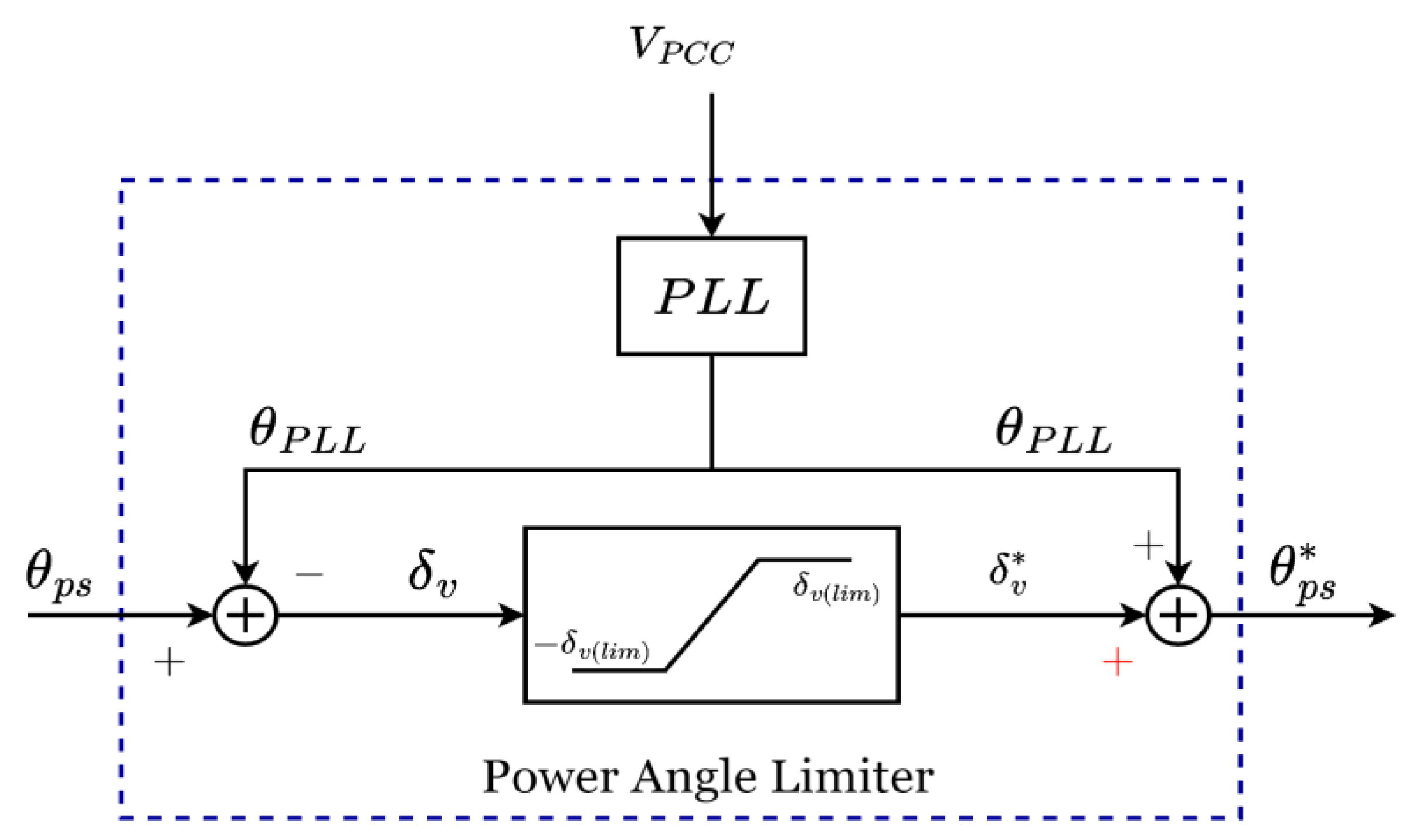
6.2.2. Power Angle Limiting
6.2.3. Virtual Impedance
6.2.4. Reference Parameter Limiting
6.2.5. Voltage-Based Frequency Feedforward
6.2.6. Current Saturation
7. Discussion
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| GFL | Grid following |
| GFM | Grid forming |
| VSC | Voltage source converter |
| IBR | Inverter-based resources |
| SCR | Short circuit ratio |
| SG | Synchronous generator |
| SM | Synchronous machine |
| PLL | Phase-locked loop |
| FLL | Frequency-locked loop |
| APC | Active power control |
| RPC | Reactive power control |
| PSC | Power synchronisation control |
| MIMO | Multiple input–multiple output |
| RoCoF | Rate of change of frequency |
| VOC | Virtual oscillator control |
| dVOC | Dispatchable virtual oscillator control |
| uVOC | Unified virtual oscillator control |
| VSG | Virtual synchronous generator |
| HSC | Hybrid synchronisation control |
| AC | Alternating current |
| DC | Direct current |
| RMS | Root mean square |
| PCC | Point of common coupling |
| FACTS | Flexible AC transmission systems |
| HVDC | High-voltage direct current |
| VDP | Van der Pol oscillator |
| VCC | Vector current control |
| PC | Power controller |
| CC | Current controller |
| AVC | Alternating bus voltage controller |
| UniCon | Universal control scheme |
References
- Cohn, J. The Grid: Biography of an American Technology. 27 September 2021. Available online: https://youtu.be/rJSR-Pt5tOI (accessed on 2 July 2023).
- Abdel-Rahim, O.; Chub, A.; Vinnikov, D.; Blinov, A. DC Integration of Residential Photovoltaic Systems: A Survey. IEEE Access 2022, 10, 66974–66991. [Google Scholar] [CrossRef]
- International Energy Agency. Net Zero by 2050—A Roadmap for the Global Energy Sector. International Energy Agency, Special Report. Available online: https://www.iea.org/reports/net-zero-by-2050 (accessed on 12 June 2023).
- Wang, H.; Abdel-Rahim, O. Five-level one-capacitor boost multilevel inverter. IET Power Electron. 2020, 13, 2245–2251. [Google Scholar] [CrossRef]
- Kroposki, B.; Johnson, B.; Zhang, Y.; Gevorgian, V.; Denholm, P.; Hodge, B.-M.; Hannegan, B. Achieving a 100% Renewable Grid: Operating Electric Power Systems with Extremely High Levels of Variable Renewable Energy. IEEE Power Energy Mag. 2017, 15, 61–73. [Google Scholar] [CrossRef]
- Simpson-Porco, J.W.; Dörfler, F.; Bullo, F. Voltage collapse in complex power grids. Nat. Commun. 2016, 7, 10790. [Google Scholar] [CrossRef] [PubMed]
- Liu, T.; Song, Y.; Zhu, L.; Hill, D.J. Stability and Control of Power Grids. Annu. Rev. Control Robot. Auton. Syst. 2022, 5, 689–716. [Google Scholar] [CrossRef]
- Ramasubramanian, D.; Baker, W.; Matevosyan, J.; Pant, S.; Achilles, S. Asking for fast terminal voltage control in grid following plants could provide benefits of grid forming behavior. IET Gener. Transm. Distrib. 2023, 17, 411–426. [Google Scholar] [CrossRef]
- Ackermann, T.; Prevost, T.; Vittal, V.; Roscoe, A.J.; Matevosyan, J.; Miller, N. Paving the Way: A Future Without Inertia Is Closer Than You Think. IEEE Power Energy Mag. 2017, 15, 61–69. [Google Scholar] [CrossRef]
- Lin, Y.; Eto, J.H.; Johnson, B.B.; Flicker, J.D.; Lasseter, R.H.; Pico, H.N.V.; Seo, G.-S.; Pierre, B.J.; Ellis, A.; Miller, J.; et al. Pathways to the Next-Generation Power System with Inverter-Based Resources: Challenges and recommendations. IEEE Electrif. Mag. 2022, 10, 10–21. [Google Scholar] [CrossRef]
- Abdel-Rahim, O.; Funato, H.; Haruna, J. A comprehensive study of three high-gain DC-DC topologies based on Cockcroft-Walton voltage multiplier for reduced power PV applications. IEEJ Trans. Electr. Electron. Eng. 2018, 13, 642–651. [Google Scholar] [CrossRef]
- Anttila, S.; Döhler, J.S.; Oliveira, J.G.; Boström, C. Grid Forming Inverters: A Review of the State of the Art of Key Elements for Microgrid Operation. Energies 2022, 15, 5517. [Google Scholar] [CrossRef]
- de Leon, F.; de Leon, F. The future belongs to dc: Edison will beat Tesla after all. IEEE Power Energy Mag. 2023, 21, 78–80. [Google Scholar] [CrossRef]
- Mahmoud, K.; Astero, P.; Peltoniemi, P.; Lehtonen, M. Promising Grid-Forming VSC Control Schemes Toward Sustainable Power Systems: Comprehensive Review and Perspectives. IEEE Access 2022, 10, 130024–130039. [Google Scholar] [CrossRef]
- Matevosyan, J.; Badrzadeh, B.; Prevost, T.; Quitmann, E.; Ramasubramanian, D.; Urdal, H.; Achilles, S.; MacDowell, J.; Huang, S.H.; Vital, V.; et al. Grid-Forming Inverters: Are They the Key for High Renewable Penetration? IEEE Power Energy Mag. 2019, 17, 89–98. [Google Scholar] [CrossRef]
- CIGRÉ. Connection of Wind Farms to Weak AC Networks; CIGRÉ: Paris, France, 2016. [Google Scholar]
- Abdel-Rahim, O.; Furiato, H. Switched inductor quadratic boosting ratio inverter with proportional resonant controller for grid-tie PV applications. In Proceedings of the IECON 2014—40th Annual Conference of the IEEE Industrial Electronics Society, Dallas, TX, USA, 29 October–1 November 2014; pp. 5606–5611. [Google Scholar]
- Gomis-Bellmunt, O.; Song, J.; Cheah-Mane, M.; Prieto-Araujo, E. Steady-state impedance mapping in grids with power electronics: What is grid strength in modern power systems? Int. J. Electr. Power Energy Syst. 2022, 136, 107635. [Google Scholar] [CrossRef]
- Mitsugi, Y.; Baba, J. Phaser-Based Transfer Function Analysis of Power Synchronization Control Instability for a Grid Forming Inverter in a Stiff Grid. IEEE Access 2023, 11, 42146–42159. [Google Scholar] [CrossRef]
- Harnefors, L.; Hinkkanen, M.; Riaz, U.; Rahman, F.M.M.; Zhang, L. Robust Analytic Design of Power-Synchronization Control. IEEE Trans. Ind. Electron. 2019, 66, 5810–5819. [Google Scholar] [CrossRef]
- IEEE Guide for Planning DC Links Terminating at AC Locations Having Low Short-Circuit Capacities; IEEE: New York, NY, USA, 1997. [CrossRef]
- Bikdeli, E.; Islam, R.; Rahman, M.; Muttaqi, K.M. State of the Art of the Techniques for Grid Forming Inverters to Solve the Challenges of Renewable Rich Power Grids. Energies 2022, 15, 1879. [Google Scholar] [CrossRef]
- Ratnam, K.S.; Palanisamy, K.; Yang, G. Future low-inertia power systems: Requirements, issues, and solutions—A review. Renew. Sustain. Energy Rev. 2020, 124, 109773. [Google Scholar] [CrossRef]
- Faragalla, A.; Abdel-Rahim, O.; Orabi, M.; Abdelhameed, E.H. Enhanced Virtual Inertia Control for Microgrids with High-Penetration Renewables Based on Whale Optimization. Energies 2022, 15, 9254. [Google Scholar] [CrossRef]
- Todorovic, I.; Isakov, I.; Reljic, D.; Jerkan, D.G.; Dujic, D. Mitigation of Voltage and Frequency Excursions in Low-Inertia Microgrids. IEEE Access 2023, 11, 9351–9367. [Google Scholar] [CrossRef]
- Ahmed, F.; Al Kez, D.; McLoone, S.; Best, R.J.; Cameron, C.; Foley, A. Dynamic grid stability in low carbon power systems with minimum inertia. Renew. Energy 2023, 210, 486–506. [Google Scholar] [CrossRef]
- Rezkalla, M.; Pertl, M.; Marinelli, M. Electric power system inertia: Requirements, challenges and solutions. Electr. Eng. 2018, 100, 2677–2693. [Google Scholar] [CrossRef]
- Milano, F.; Dörfler, F.; Hug, G.; Hill, D.J.; Verbič, G. Foundations and Challenges of Low-Inertia Systems (Invited Paper). In Proceedings of the 2018 Power Systems Computation Conference (PSCC), Dublin, Ireland, 11–15 June 2018; pp. 1–25. [Google Scholar] [CrossRef]
- Yehia, D.M.; Taha, I.B.M. Application of Superconducting Fault Current Limiter as a Virtual Inertia for DC Distribution Systems. IEEE Access 2021, 9, 135384–135391. [Google Scholar] [CrossRef]
- Ippolito, M.G.; Musca, R.; Sanseverino, E.R.; Zizzo, G. Frequency Dynamics in Fully Non-Synchronous Electrical Grids: A Case Study of an Existing Island. Energies 2022, 15, 2220. [Google Scholar] [CrossRef]
- Hu, Q.; Han, R.; Quan, X.; Wu, Z.; Tang, C.; Li, W.; Wang, W. Grid-Forming Inverter Enabled Virtual Power Plants with Inertia Support Capability. IEEE Trans. Smart Grid 2022, 13, 4134–4143. [Google Scholar] [CrossRef]
- Dalboni, M.; Soldati, A. On the Synchronization of Parallel Power Converters via Emulation of Linear Mechanical Oscillators. In IEEE/ASME Transactions on Mechatronics; IEEE: New York, NY, USA, 2023; pp. 1–12. [Google Scholar] [CrossRef]
- Sajadi, A.; Kenyon, R.W.; Hodge, B.-M. Synchronization in electric power networks with inherent heterogeneity up to 100% inverter-based renewable generation. Nat. Commun. 2022, 13, 2490. [Google Scholar] [CrossRef]
- Matter, E.M.; Mahmoud, E.S.; El-Sayed, M. Power Quality Improvement Considering Voltage Sag and Unbalance Using DVR. J. Al-Azhar Univ. Eng. Sect. 2023, 18, 83–97. [Google Scholar] [CrossRef]
- Perera, U.; Oo, A.M.T.; Zamora, R. Sub Synchronous Oscillations under High Penetration of Renewables—A Review of Existing Monitoring and Damping Methods, Challenges, and Research Prospects. Energies 2022, 15, 8477. [Google Scholar] [CrossRef]
- Markovic, U.; Stanojev, O.; Vrettos, E.; Aristidou, P.; Hug, G. Understanding Stability of Low-Inertia Systems. engrXiv 2019. [Google Scholar] [CrossRef]
- Markovic, U.; Stanojev, O.; Aristidou, P.; Vrettos, E.; Callaway, D.S.; Hug, G. Understanding Small-Signal Stability of Low-Inertia Systems. IEEE Trans. Power Syst. 2021, 36, 3997–4017. [Google Scholar] [CrossRef]
- Abdel-Rahim, O.; Orabi, M.; Ahmed, M.E. Development an efficient photovoltaic (PV) configuration for low power applications. In Proceedings of the 2010 IEEE International Conference on Power and Energy (PECon), Kuala Lumpur, Malaysia, 29 November–1 December 2010; pp. 622–627. [Google Scholar] [CrossRef]
- Abdel-Rahim, O.; Takeuchi, M.; Funato, H.; Junnosuke, H. T-type three-level neutral point clamped inverter with model predictive control for grid connected photovoltaic applications. In Proceedings of the 2016 19th International Conference on Electrical Machines and Systems (ICEMS), Chiba, Japan, 13–16 November 2016; pp. 1–5. [Google Scholar]
- Li, Y.; Gu, Y.; Green, T.C. Revisiting Grid-Forming and Grid-Following Inverters: A Duality Theory. IEEE Trans. Power Syst. 2022, 37, 4541–4554. [Google Scholar] [CrossRef]
- Gao, X.; Zhou, D.; Anvari-Moghaddam, A.; Blaabjerg, F. A Comparative Study of Grid-Following and Grid-Forming Control Schemes in Power Electronic-Based Power Systems. Power Electron. Drives 2023, 8, 1–20. [Google Scholar] [CrossRef]
- Pattabiraman, D.; Lasseter, R.H.; Jahns, T.M. Comparison of Grid Following and Grid Forming Control for a High Inverter Penetration Power System. In Proceedings of the 2018 IEEE Power & Energy Society General Meeting (PESGM), Portland, OR, USA, 5–10 August 2018; pp. 1–5. [Google Scholar] [CrossRef]
- Rosso, R.; Wang, X.; Liserre, M.; Lu, X.; Engelken, S. Grid-Forming Converters: Control Approaches, Grid-Synchronization, and Future Trends—A Review. IEEE Open J. Ind. Appl. 2021, 2, 93–109. [Google Scholar] [CrossRef]
- Li, Z. Advanced Control of Grid-Forming Inverters under Uncertain Operating Conditions in AC Microgrids. Ph.D. Thesis, The Hong Kong Polytechnic University, Hongkong, China, 2021. Available online: https://theses.lib.polyu.edu.hk/handle/200/11693 (accessed on 17 July 2023).
- Dokus, M.; Mertens, A. On the Coupling of Power-Related and Inner Inverter Control Loops of Grid-Forming Converter Systems. IEEE Access 2021, 9, 16173–16192. [Google Scholar] [CrossRef]
- Pereira, A.T.; Pinheiro, H. Inner Loop Controllers for Grid-Forming Converters. In Proceedings of the 2022 14th Seminar on Power Electronics and Control (SEPOC), Santa Maria, Brazil, 12–15 November 2022; pp. 1–6. [Google Scholar] [CrossRef]
- Buso, S.; Caldognetto, T.; Liu, Q. Analysis and Experimental Characterization of a Large-Bandwidth Triple-Loop Controller for Grid-Tied Inverters. IEEE Trans. Power Electron. 2019, 34, 1936–1949. [Google Scholar] [CrossRef]
- Gursoy, M.; Mirafzal, B. Direct vs. Indirect Control Schemes for Grid-Forming Inverters–Unveiling a Performance Comparison in a Microgrid. IEEE Access 2023, 11, 75023–75036. [Google Scholar] [CrossRef]
- Pogaku, N.; Prodanovic, M.; Green, T.C. Modeling, Analysis and Testing of Autonomous Operation of an Inverter-Based Microgrid. IEEE Trans. Power Electron. 2007, 22, 613–625. [Google Scholar] [CrossRef]
- Yazdani, A.; Iravani, R. Voltage-Sourced Converters in Power Systems: Modeling, Control, and Applications; Wiley: Hoboken, NJ, USA, 2010. [Google Scholar]
- Unruh, P.; Nuschke, M.; Strauß, P.; Welck, F. Overview on Grid-Forming Inverter Control Methods. Energies 2020, 13, 2589. [Google Scholar] [CrossRef]
- Zhang, H.; Xiang, W.; Lin, W.; Wen, J. Grid Forming Converters in Renewable Energy Sources Dominated Power Grid: Control Strategy, Stability, Application, and Challenges. J. Mod. Power Syst. Clean Energy 2021, 9, 1239–1256. [Google Scholar] [CrossRef]
- Abdel-Rahim, O.; Funato, H.; Junnosuke, H. Droop method based on model predictive control for DC microgrid. In Proceedings of the 2016 19th International Conference on Electrical Machines and Systems (ICEMS), Chiba, Japan, 13–16 November 2016; pp. 1–6. [Google Scholar]
- Majumder, R.; Ledwich, G.; Ghosh, A.; Chakrabarti, S.; Zare, F. Droop Control of Converter-Interfaced Microsources in Rural Distributed Generation. IEEE Trans. Power Deliv. 2010, 25, 2768–2778. [Google Scholar] [CrossRef]
- D’Arco, S.; Suul, J.A. Virtual Synchronous Machines—Classification of Implementations and Analysis of Equivalence to Droop Controllers for Microgrids. In Proceedings of the 2013 IEEE Powertech Grenoble Conference, Grenoble, France, 16–20 June 2013; pp. 1–7. [Google Scholar] [CrossRef]
- D’Arco, S.; Suul, J.A. Equivalence of Virtual Synchronous Machines and Frequency-Droops for Converter-Based MicroGrids. IEEE Trans. Smart Grid 2013, 5, 394–395. [Google Scholar] [CrossRef]
- Sun, Y.; Hou, X.; Yang, J.; Han, H.; Su, M.; Guerrero, J.M. New Perspectives on Droop Control in AC Microgrid. IEEE Trans. Ind. Electron. 2017, 64, 5741–5745. [Google Scholar] [CrossRef]
- Du, W.; Chen, Z.; Schneider, K.P.; Lasseter, R.H.; Nandanoori, S.P.; Tuffner, F.K.; Kundu, S. A Comparative Study of Two Widely Used Grid-Forming Droop Controls on Microgrid Small-Signal Stability. IEEE J. Emerg. Sel. Top. Power Electron. 2020, 8, 963–975. [Google Scholar] [CrossRef]
- Shi, Y.; Gu, X.; Yin, X.; Feng, S.; Zhang, S. Design of droop controller in islanded microgrids using multi-objective optimisation based on accurate small-signal model. IET Power Electron. 2022, 15, 1093–1109. [Google Scholar] [CrossRef]
- Eberlein, S.; Rudion, K. Small-signal stability modelling, sensitivity analysis and optimization of droop controlled inverters in LV microgrids. Int. J. Electr. Power Energy Syst. 2021, 125, 106404. [Google Scholar] [CrossRef]
- Eberlein, S.; Rudion, K. Optimisation, benchmark testing and comparison of droop control variants in microgrids. IET Smart Grid 2021, 4, 536–548. [Google Scholar] [CrossRef]
- Belal, E.K.; Yehia, D.M.; Azmy, A.M. Effective Power Management of DC Microgrids Using Adaptive Droop Control. In Proceedings of the 2018 Twentieth International Middle East Power Systems Conference (MEPCON), Cairo, Egypt, 18–20 December 2018; pp. 905–910. [Google Scholar] [CrossRef]
- Gurugubelli, V.; Ghosh, A.; Panda, A.K.; Rudra, S. Implementation and comparison of droop control, virtual synchronous machine, and virtual oscillator control for parallel inverters in standalone microgrid. Int. Trans. Electr. Energy Syst. 2021, 31, e12859. [Google Scholar] [CrossRef]
- Zhang, L.; Harnefors, L.; Nee, H.-P. Power-Synchronization Control of Grid-Connected Voltage-Source Converters. IEEE Trans. Power Syst. 2010, 25, 809–820. [Google Scholar] [CrossRef]
- Ahmed, H.Y.; Abdel-Rahim, O.; Ali, Z.M. New High-Gain Transformerless DC/DC Boost Converter System. Electronics 2022, 11, 734. [Google Scholar] [CrossRef]
- Zhang, L.; Harnefors, L.; Nee, H.-P. Interconnection of Two Very Weak AC Systems by VSC-HVDC Links Using Power-Synchronization Control. IEEE Trans. Power Syst. 2011, 26, 344–355. [Google Scholar] [CrossRef]
- Sepehr, A.; Gomis-Bellmunt, O.; Pouresmaeil, E. Employing Machine Learning for Enhancing Transient Stability of Power Synchronization Control During Fault Conditions in Weak Grids. IEEE Trans. Smart Grid 2022, 13, 2121–2131. [Google Scholar] [CrossRef]
- Zhang, L.; Nee, H.-P.; Harnefors, L. Analysis of Stability Limitations of a VSC-HVDC Link Using Power-Synchronization Control. IEEE Trans. Power Syst. 2011, 26, 1326–1337. [Google Scholar] [CrossRef]
- Xiong, X.; Zhou, Y.; Luo, B.; Cheng, P.; Blaabjerg, F. Analysis and Suppression Strategy of Synchronous Frequency Resonance for Grid-Connected Converters with Power-Synchronous Control Method. IEEE Trans. Power Electron. 2023, 38, 6945–6955. [Google Scholar] [CrossRef]
- Wu, H.; Wang, X. Design-Oriented Transient Stability Analysis of Grid-Connected Converters with Power Synchronization Control. IEEE Trans. Ind. Electron. 2019, 66, 6473–6482. [Google Scholar] [CrossRef]
- Yazdani, S.; Davari, M.; Ferdowsi, M.; Shamsi, P. Internal Model Power Synchronization Control of a PV-Based Voltage-Source Converter in Weak-Grid and Islanded Conditions. IEEE Trans. Sustain. Energy 2021, 12, 1360–1371. [Google Scholar] [CrossRef]
- Yazdani, S.; Ferdowsi, M.; Shamsi, P. Power Synchronization PID Control Method for Grid-Connected Voltage-Source Converters. In Proceedings of the 2020 IEEE Kansas Power and Energy Conference (KPEC), Manhattan, KS, USA, 13–14 July 2020; pp. 1–6. [Google Scholar] [CrossRef]
- Driesen, J.; Visscher, K. Virtual synchronous generators. In Proceedings of the 2008 IEEE Power and Energy Society General Meeting Conversion and Delivery of Electrical Energy in the 21st Century, Pittsburgh, PA, USA, 20–24 July 2008; pp. 1–3. [Google Scholar] [CrossRef]
- Liu, J.; Miura, Y.; Bevrani, H.; Ise, T. Enhanced Virtual Synchronous Generator Control for Parallel Inverters in Microgrids. IEEE Trans. Smart Grid 2017, 8, 2268–2277. [Google Scholar] [CrossRef]
- Li, B.; Zhou, L.; Yu, X.; Zheng, C.; Liu, J. Improved power decoupling control strategy based on virtual synchronous generator. IET Power Electron. 2017, 10, 462–470. [Google Scholar] [CrossRef]
- Shuai, Z.; Shen, C.; Liu, X.; Li, Z.; Shen, Z.J. Transient Angle Stability of Virtual Synchronous Generators Using Lyapunov’s Direct Method. IEEE Trans. Smart Grid 2019, 10, 4648–4661. [Google Scholar] [CrossRef]
- Chen, M.; Zhou, D.; Blaabjerg, F. Enhanced Transient Angle Stability Control of Grid-Forming Converter Based on Virtual Synchronous Generator. IEEE Trans. Ind. Electron. 2022, 69, 9133–9144. [Google Scholar] [CrossRef]
- Xiong, X.; Wu, C.; Hu, B.; Pan, D.; Blaabjerg, F. Transient Damping Method for Improving the Synchronization Stability of Virtual Synchronous Generators. IEEE Trans. Power Electron. 2021, 36, 7820–7831. [Google Scholar] [CrossRef]
- Sadeque, F.; Fateh, F. On Control Schemes for Grid-Forming Inverters. In Proceedings of the 2022 IEEE Kansas Power and Energy Conference (KPEC), Manhattan, KS, USA, 25–26 April 2022; pp. 1–6. [Google Scholar] [CrossRef]
- Shintai, T.; Miura, Y.; Ise, T. Oscillation Damping of a Distributed Generator Using a Virtual Synchronous Generator. IEEE Trans. Power Deliv. 2014, 29, 668–676. [Google Scholar] [CrossRef]
- Wang, L.; Zhou, H.; Hu, X.; Hou, X.; Su, C.; Sun, K. Adaptive Inertia and Damping Coordination (AIDC) Control for Grid-Forming VSG to Improve Transient Stability. Electronics 2023, 12, 2060. [Google Scholar] [CrossRef]
- Cheema, K.M.; Chaudhary, N.I.; Tahir, M.F.; Mehmood, K.; Mudassir, M.; Kamran, M.; Milyani, A.H.; Elbarbary, Z.S. Virtual synchronous generator: Modifications, stability assessment and future applications. Energy Rep. 2022, 8, 1704–1717. [Google Scholar] [CrossRef]
- Li, J.; Li, Y.; Du, Z.; Xu, Z.; Dong, Z. Damping Turning Rule of Virtual Synchronous Generator for Global Stability. IEEE Trans. Power Deliv. 2023, 38, 2650–2660. [Google Scholar] [CrossRef]
- Li, C.; Yang, Y.; Cao, Y.; Wang, L.; Blaabjerg, F.; Dragicevic, T. Frequency and Voltage Stability Analysis of Grid-Forming Virtual Synchronous Generator Attached to Weak Grid. IEEE J. Emerg. Sel. Top. Power Electron. 2022, 10, 2662–2671. [Google Scholar] [CrossRef]
- Chen, S.; Sun, Y.; Han, H.; Fu, S.; Luo, S.; Shi, G. A Modified VSG Control Scheme with Virtual Resistance to Enhance Both Small-Signal Stability and Transient Synchronization Stability. IEEE Trans. Power Electron. 2023, 38, 6005–6014. [Google Scholar] [CrossRef]
- Miranbeigi, M.; Kandula, P.; Divan, D. A New Representation based on Virtual Capacitor for Virtual Synchronous Generators. In Proceedings of the 2020 IEEE 11th International Symposium on Power Electronics for Distributed Generation Systems (PEDG), Dubrovnik, Croatia, 28 September–1 October 2020; pp. 205–210. [Google Scholar] [CrossRef]
- Suvorov, A.; Askarov, A.; Bay, Y.; Maliuta, B.; Achitaev, A.; Suslov, K. Comparative small-signal stability analysis of voltage-controlled and enhanced current-controlled virtual synchronous generators under weak and stiff grid conditions. Int. J. Electr. Power Energy Syst. 2023, 147, 108891. [Google Scholar] [CrossRef]
- Yang, Y.; Xu, J.; Li, C.; Zhang, W.; Wu, Q.; Wen, M.; Blaabjerg, F. A New Virtual Inductance Control Method for Frequency Stabilization of Grid-Forming Virtual Synchronous Generators. IEEE Trans. Ind. Electron. 2023, 70, 441–451. [Google Scholar] [CrossRef]
- Lu, S.; Zhu, Y.; Dong, L.; Na, G.; Hao, Y.; Zhang, G.; Zhang, W.; Cheng, S.; Yang, J.; Sui, Y. Small-Signal Stability Research of Grid-Connected Virtual Synchronous Generators. Energies 2022, 15, 7158. [Google Scholar] [CrossRef]
- Zhong, Q.-C.; Weiss, G. Synchronverters: Inverters that mimic synchronous generators. IEEE Trans. Ind. Electron. 2011, 58, 1259–1267. [Google Scholar] [CrossRef]
- Tayyebi, A.; Dörfler, F.; Kupzog, F.; Miletic, Z.; Hribernik, W. Grid-Forming Converters—Inevitability, Control Strategies and Challenges in Future Grids Application. In Proceedings of the CIRED 2018 Ljubljana Workshop, Ljubljana, Slovenia, 7–8 June 2018. [Google Scholar] [CrossRef]
- Rosso, R.; Engelken, S.; Liserre, M. A Generalized Formulation of Active Power Synchronization Based Control Algorithms for Grid Connected Converters. In Proceedings of the IECON 2018—44th Annual Conference of the IEEE Industrial Electronics Society, Washington, DC, USA, 21–23 October 2018; pp. 883–888. [Google Scholar] [CrossRef]
- Zhong, Q.-C.; Nguyen, P.-L.; Ma, Z.; Sheng, W. Self-Synchronized Synchronverters: Inverters Without a Dedicated Synchronization Unit. IEEE Trans. Power Electron. 2014, 29, 617–630. [Google Scholar] [CrossRef]
- Natarajan, V.; Weiss, G. Synchronverters with Better Stability Due to Virtual Inductors, Virtual Capacitors, and Anti-Windup. IEEE Trans. Ind. Electron. 2017, 64, 5994–6004. [Google Scholar] [CrossRef]
- Lorenzetti, P.; Kustanovich, Z.; Shivratri, S.; Weiss, G. The Equilibrium Points and Stability of Grid-Connected Synchronverters. IEEE Trans. Power Syst. 2022, 37, 1184–1197. [Google Scholar] [CrossRef]
- Vasudevan, K.R.; Ramachandaramurthy, V.K.; Babu, T.S.; Pouryekta, A. Synchronverter: A Comprehensive Review of Modifications, Stability Assessment, Applications and Future Perspectives. IEEE Access 2020, 8, 131565–131589. [Google Scholar] [CrossRef]
- Gomes, L.D.N.; Abrantes-Ferreira, A.J.G.; Dias, R.F.d.S.; Rolim, L.G.B. Synchronverter-Based STATCOM With Voltage Imbalance Compensation Functionality. IEEE Trans. Ind. Electron. 2022, 69, 4836–4844. [Google Scholar] [CrossRef]
- Remon, D.; Cantarellas, A.M.; Rakhshani, E.; Candela, I.; Rodriguez, P. An active power synchronization control loop for grid-connected converters. In Proceedings of the IEEE Power & Energy Society General Meeting, National Harbor, MD, USA, 27–31 July 2014; IEEE: Piscataway, NJ, USA, 2014; pp. 1–5. [Google Scholar] [CrossRef]
- Arghir, C.; Jouini, T.; Dörfler, F. Grid-forming control for power converters based on matching of synchronous machines. Automatica 2018, 95, 273–282. [Google Scholar] [CrossRef]
- Curi, S.; Gross, D.; Dorfler, F. Control of low-inertia power grids: A model reduction approach. In Proceedings of the 2017 IEEE 56th Annual Conference on Decision and Control (CDC), Melbourne, VIC, Australia, 12–15 December 2017; pp. 5708–5713. [Google Scholar] [CrossRef]
- Jouini, T.; Arghir, C.; Dörfler, F. Grid-Friendly Matching of Synchronous Machines by Tapping into the DC Storage. IFAC-Pap. Online 2016, 49, 192–197. [Google Scholar] [CrossRef]
- Arghir, C.; Dorfler, F. The Electronic Realization of Synchronous Machines: Model Matching, Angle Tracking, and Energy Shaping Techniques. IEEE Trans. Power Electron. 2020, 35, 4398–4410. [Google Scholar] [CrossRef]
- Mahamedi, B.; Fletcher, J.E. The Equivalent Models of Grid-Forming Inverters in the Sequence Domain for the Steady-State Analysis of Power Systems. IEEE Trans. Power Syst. 2020, 35, 2876–2887. [Google Scholar] [CrossRef]
- Dörfler, F.; Chertkov, M.; Bullo, F. Synchronization in complex oscillator networks and smart grids. Proc. Natl. Acad. Sci. USA 2013, 110, 2005–2010. [Google Scholar] [CrossRef]
- Torres, L.A.B.; Hespanha, J.P.; Moehlis, J. Synchronization of Identical Oscillators Coupled Through a Symmetric Network with Dynamics: A Constructive Approach with Applications to Parallel Operation of Inverters. IEEE Trans. Autom. Control 2015, 60, 3226–3241. [Google Scholar] [CrossRef]
- Johnson, B.B.; Dhople, S.V.; Hamadeh, A.O.; Krein, P.T. Synchronization of Parallel Single-Phase Inverters with Virtual Oscillator Control. IEEE Trans. Power Electron. 2014, 29, 6124–6138. [Google Scholar] [CrossRef]
- Dhople, S.V.; Johnson, B.B.; Hamadeh, A.O. Virtual Oscillator Control for voltage source inverters. In Proceedings of the 2013 51st Annual Allerton Conference on Communication, Control, and Computing (Allerton), Monticello, IL, USA, 2–4 October 2013; pp. 1359–1363. [Google Scholar] [CrossRef]
- Aghdam, S.A.; Agamy, M. Virtual oscillator-based methods for grid-forming inverter control: A review. IET Renew. Power Gener. 2022, 16, 835–855. [Google Scholar] [CrossRef]
- Sinha, M.; Dorfler, F.; Johnson, B.B.; Dhople, S.V. Virtual Oscillator Control subsumes droop control. In Proceedings of the 2015 American Control Conference (ACC), Chicago, IL, USA, 1–3 July 2015; pp. 2353–2358. [Google Scholar] [CrossRef]
- Lu, M.; Dutta, S.; Purba, V.; Dhople, S.; Johnson, B. A Grid-compatible Virtual Oscillator Controller: Analysis and Design. In Proceedings of the 2019 IEEE Energy Conversion Congress and Exposition (ECCE), Baltimore, MD, USA, 29 September–3 October 2019; pp. 2643–2649. [Google Scholar] [CrossRef]
- Johnson, B.B.; Sinha, M.; Ainsworth, N.G.; Dorfler, F.; Dhople, S.V. Synthesizing Virtual Oscillators to Control Islanded Inverters. IEEE Trans. Power Electron. 2016, 31, 6002–6015. [Google Scholar] [CrossRef]
- Sinha, M.; Dorfler, F.; Johnson, B.B.; Dhople, S.V. Uncovering Droop Control Laws Embedded Within the Nonlinear Dynamics of Van der Pol Oscillators. IEEE Trans. Control Netw. Syst. 2017, 4, 347–358. [Google Scholar] [CrossRef]
- Luo, S.; Wu, W.; Koutroulis, E.G.; Chung, H.S.-H.; Blaabjerg, F.G. A New Virtual Oscillator Control Without Third-Harmonics Injection For DC/AC Inverter. IEEE Trans. Power Electron. 2021, 36, 10879–10888. [Google Scholar] [CrossRef]
- Awal, M.A.; Yu, H.; Husain, I.; Yu, W.; Lukic, S.M. Selective Harmonic Current Rejection for Virtual Oscillator Controlled Grid-Forming Voltage Source Converters. IEEE Trans. Power Electron. 2020, 35, 8805–8818. [Google Scholar] [CrossRef]
- Raisz, D.; Thai, T.T.; Monti, A. Power Control of Virtual Oscillator Controlled Inverters in Grid-Connected Mode. IEEE Trans. Power Electron. 2019, 34, 5916–5926. [Google Scholar] [CrossRef]
- Colombino, M.; Gros, D.; Dorfler, F. Global phase and voltage synchronization for power inverters: A decentralized consensus-inspired approach. In Proceedings of the 2017 IEEE 56th Annual Conference on Decision and Control (CDC), Melbourne, VIC, Australia, 12–15 December 2017; pp. 5690–5695. [Google Scholar] [CrossRef]
- Colombino, M.; Groz, D.; Brouillon, J.-S.; Dorfler, F. Global Phase and Magnitude Synchronization of Coupled Oscillators with Application to the Control of Grid-Forming Power Inverters. IEEE Trans. Autom. Control 2019, 64, 4496–4511. [Google Scholar] [CrossRef]
- Seo, G.-S.; Colombino, M.; Subotic, I.; Johnson, B.; Gros, D.; Dorfler, F. Dispatchable Virtual Oscillator Control for Decentralized Inverter-dominated Power Systems: Analysis and Experiments. In Proceedings of the 2019 IEEE Applied Power Electronics Conference and Exposition (APEC), Anaheim, CA, USA, 17–21 March 2019; pp. 561–566. [Google Scholar] [CrossRef]
- Lu, M.; Dhople, S.V.; Johnson, B. Benchmarking Nonlinear Oscillators for Grid-Forming Inverter Control. IEEE Trans. Power Electron. 2022, 37, 10250–10266. [Google Scholar] [CrossRef]
- Ali, M.; Nurdin, H.I.; Fletcher, J.E. Dispatchable Virtual Oscillator Control for Single-Phase Islanded Inverters: Analysis and Experiments. IEEE Trans. Ind. Electron. 2021, 68, 4812–4826. [Google Scholar] [CrossRef]
- Lu, M. Virtual Oscillator Grid-Forming Inverters: State of the Art, Modeling, and Stability. IEEE Trans. Power Electron. 2022, 37, 11579–11591. [Google Scholar] [CrossRef]
- He, X.; Haberle, V.; Subotic, I.; Dorfler, F. Nonlinear Stability of Complex Droop Control in Converter-Based Power Systems. IEEE Control Syst. Lett. 2023, 7, 1327–1332. [Google Scholar] [CrossRef]
- Awal, M.A.; Yu, H.; Tu, H.; Lukic, S.M.; Husain, I. Hierarchical Control for Virtual Oscillator Based Grid-Connected and Islanded Microgrids. IEEE Trans. Power Electron. 2020, 35, 988–1001. [Google Scholar] [CrossRef]
- Lu, M.; Dutta, S.; Johnson, B. Self-Synchronizing Cascaded Inverters with Virtual Oscillator Control. IEEE Trans. Power Electron. 2022, 37, 6424–6436. [Google Scholar] [CrossRef]
- Kong, L.; Xue, Y.; Qiao, L.; Wang, F. Enhanced Synchronization Stability of Grid-Forming Inverters with Passivity-Based Virtual Oscillator Control. IEEE Trans. Power Electron. 2022, 37, 14141–14156. [Google Scholar] [CrossRef]
- Li, J.; Fletcher, J.E.; Holmes, D.; McGrath, B. Developing a machine equivalent inertial response for a Virtual Oscillator Controlled Inverter in a machine-inverter based microgrid. In Proceedings of the 2020 IEEE Energy Conversion Congress and Exposition (ECCE), Detroit, MI, USA, 11–15 October 2020; pp. 4314–4321. [Google Scholar] [CrossRef]
- Awal, M.A.; Husain, I. Unified Virtual Oscillator Control for Grid-Forming and Grid-Following Converters. IEEE J. Emerg. Sel. Top. Power Electron. 2021, 9, 4573–4586. [Google Scholar] [CrossRef]
- Ajala, O.; Lu, M.; Johnson, B.B.; Dhople, S.V.; Dominguez-Garcia, A. Model Reduction for Inverters with Current Limiting and Dispatchable Virtual Oscillator Control. IEEE Trans. Energy Convers. 2022, 37, 2250–2259. [Google Scholar] [CrossRef]
- Ghosh, R.; Tummuru, N.R.; Rajpurohit, B.S. Modified VOC Using Three Symmetrical Components for Grid-Supporting Operation During Unbalanced Grid Voltages and Grid-Forming Operation in Hybrid Single-Phase/Three-Phase Microgrid. IEEE Trans. Ind. Electron. 2023, 70, 11276–11286. [Google Scholar] [CrossRef]
- Ghosh, R.; Tummuru, N.R.; Rajpurohit, B.S. A New Virtual Oscillator-Based Grid-Forming Controller with Decoupled Control Over Individual Phases and Improved Performance of Unbalanced Fault Ride-Through. IEEE Trans. Ind. Electron. 2023, 70, 12465–12474. [Google Scholar] [CrossRef]
- Harnefors, L.; Kukkola, J.; Routimo, M.; Hinkkanen, M.; Wang, X. A Universal Controller for Grid-Connected Voltage-Source Converters. IEEE J. Emerg. Sel. Top. Power Electron. 2021, 9, 5761–5770. [Google Scholar] [CrossRef]
- Harnefors, L.; Schweizer, M.; Kukkola, J.; Routimo, M.; Hinkkanen, M.; Wang, X. Generic PLL-Based Grid-Forming Control. IEEE Trans. Power Electron. 2021, 37, 1201–1204. [Google Scholar] [CrossRef]
- Wang, X.; Taul, M.G.; Wu, H.; Liao, Y.; Blaabjerg, F.; Harnefors, L. Grid-Synchronization Stability of Converter-Based Resources—An Overview. IEEE Open J. Ind. Appl. 2020, 1, 115–134. [Google Scholar] [CrossRef]
- Zhao, F.; Wang, X.; Zhou, Z.; Sun, Y.; Harnefors, L.; Zhu, T. Robust Grid-Forming Control with Active Susceptance. IEEE Trans. Power Electron. 2023, 38, 2872–2877. [Google Scholar] [CrossRef]
- Liu, T.; Wang, X. Physical Insight into Hybrid-Synchronization-Controlled Grid-Forming Inverters Under Large Disturbances. IEEE Trans. Power Electron. 2022, 37, 11475–11480. [Google Scholar] [CrossRef]
- Pan, R.; Liu, S.; Gu, H.; Liu, D.; Zhu, L.; Chen, E. Stability analysis of hybrid synchronization controller based grid forming control. Energy Rep. 2023, 9, 1291–1298. [Google Scholar] [CrossRef]
- Gong, H.; Wang, X. Design-Oriented Analysis of Grid-Forming Control with Hybrid Synchronization. In Proceedings of the 2022 International Power Electronics Conference (IPEC-Himeji 2022-ECCE Asia), Himeji, Japan, 15–19 May 2022; pp. 440–446. [Google Scholar] [CrossRef]
- Abdel-Rahim, O.; Funato, H. Model Predictive Control based Maximum Power Point Tracking technique applied to Ultra Step-Up Boost Converter for PV applications. In Proceedings of the 2014 IEEE Innovative Smart Grid Technologies—Asia (ISGT ASIA), Kuala Lumpur, Malaysia, 20–23 May 2014; pp. 138–142. [Google Scholar] [CrossRef]
- Gong, H.; Wang, X. Interaction analysis and enhanced design of grid-forming control with hybrid synchronization and virtual admittance loops. iEnergy 2023, 2, 71–84. [Google Scholar] [CrossRef]
- Zhao, L.; Wang, X.; Gong, H.; Jin, Z. Stability Impact of Hybrid Synchronization Strategy on Virtual-Admittance-Based Grid-Forming Inverters. In Proceedings of the 2023 IEEE Applied Power Electronics Conference and Exposition (APEC), Orlando, FL, USA, 19–23 March 2023; pp. 2735–2740. [Google Scholar] [CrossRef]
- Awal, M.A.; Rachi, R.K.; Yu, H.; Husain, I.; Lukic, S. Double Synchronous Unified Virtual Oscillator Control for Asymmetrical Fault Ride-Through in Grid-Forming Voltage Source Converters. IEEE Trans. Power Electron. 2023, 38, 6759–6763. [Google Scholar] [CrossRef]
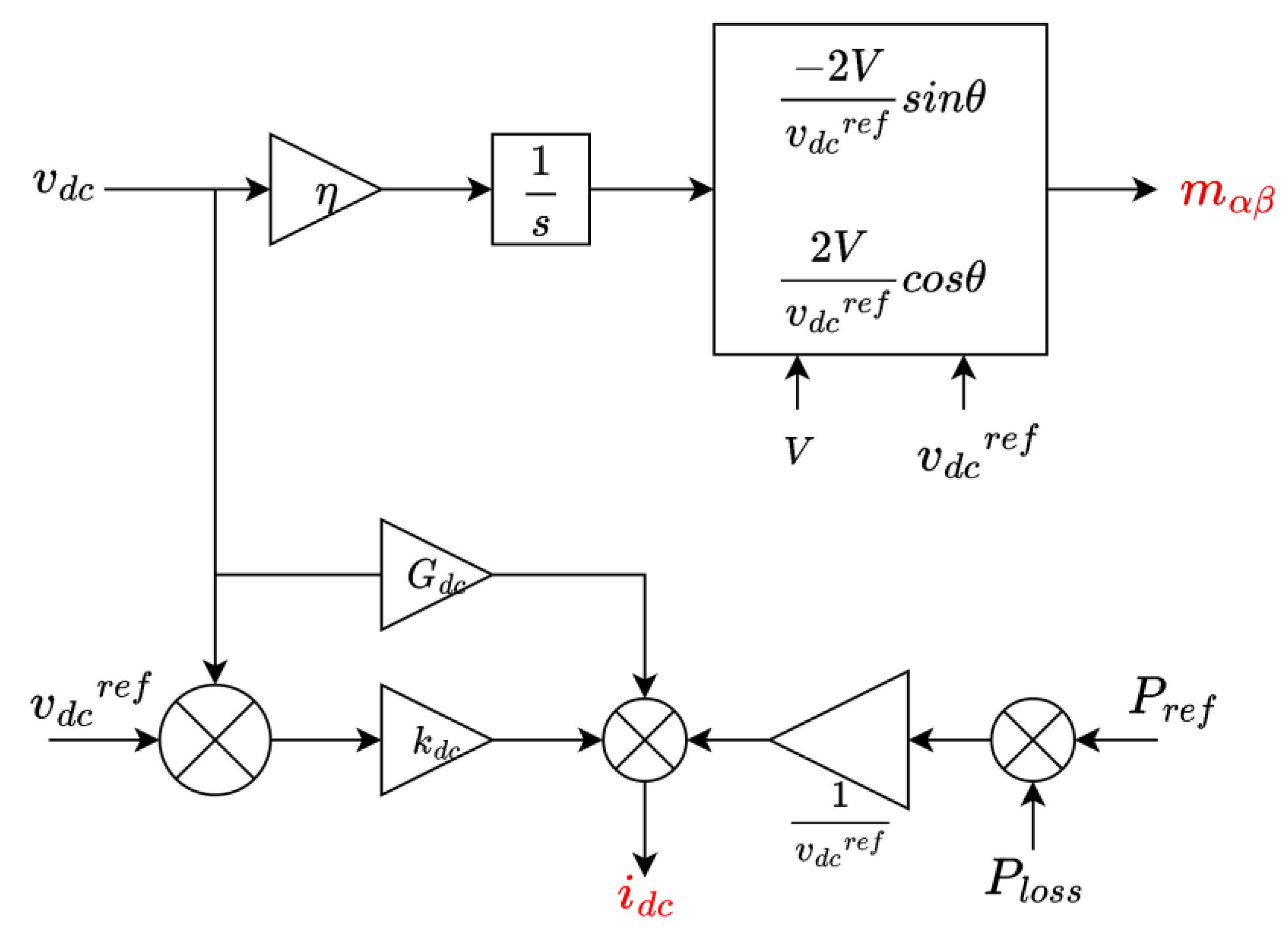
- Miranbeigi, M.; Kandula, P.; Divan, D. Unified Control (UniCon) Strategies for Grid-Connected Inverters. In Proceedings of the 2021 IEEE Energy Conversion Congress and Exposition (ECCE), Vancouver, BC, Canada, 10–14 October 2021; pp. 3000–3006. [Google Scholar] [CrossRef]
- Benzaquen, J.; Miranbeigi, M.; Kandula, P.; Divan, D. Collaborative Autonomous Grid-Connected Inverters: Flexible grid-forming inverter control for the future grid. IEEE Electrification Mag. 2022, 10, 22–29. [Google Scholar] [CrossRef]
- Rafiee, A.; Batmani, Y.; Bevrani, H.; Kato, T. Robust MIMO Controller Design for VSC-Based Microgrids: Sequential Loop Closing Concept and Quantitative Feedback Theory. IEEE Trans. Smart Grid 2022, 13, 129–138. [Google Scholar] [CrossRef]
- Alamir, N.; Abdel-Rahim, O.; Ismeil, M.; Orabi, M.; Kennel, R. Fixed Frequency Predictive MPPT for Phase-Shift Modulated LLC Resonant Micro-Inverter. In Proceedings of the 2018 20th European Conference on Power Electronics and Applications (EPE’18 ECCE Europe), Riga, Latvia, 17–21 September 2018; pp. 1–9. [Google Scholar]
- Abdel-Rahim, O.; Funato, H. A novel model predictive control for high gain switched inductor power conditioning system for photovoltaic applications. In Proceedings of the 2014 IEEE Innovative Smart Grid Technologies—Asia (ISGT ASIA), Kuala Lumpur, Malaysia, 20–23 May 2014; pp. 170–174. [Google Scholar] [CrossRef]
- Huang, L.; Xin, H.; Dorfler, F. H∞-Control of Grid-Connected Converters: Design, Objectives and Decentralized Stability Certificates. IEEE Trans. Smart Grid 2020, 11, 3805–3816. [Google Scholar] [CrossRef]
- Johnson, B.; Roberts, T.G.; Ajala, O.; Dominguez-Garcia, A.; Dhople, S.; Ramasubramanian, D.; Tuohy, A.; Divan, D.; Kroposki, B. A Generic Primary-control Model for Grid-forming Inverters: Towards Interoperable Operation & Control. In Proceedings of the Hawaii International Conference on System Sciences, Maui, HI, USA, 4–7 January 2022. [Google Scholar] [CrossRef]
- Chen, M.; Zhou, D.; Tayyebi, A.; Prieto-Araujo, E.; Dorfler, F.; Blaabjerg, F. Generalized Multivariable Grid-Forming Control Design for Power Converters. IEEE Trans. Smart Grid 2022, 13, 2873–2885. [Google Scholar] [CrossRef]
- Tayyebi, A.; Groß, D.; Anta, A.; Kupzog, F.; Dörfler, F. Interactions of Grid-Forming Power Converters and Synchronous Machines. arXiv 2019, arXiv:1902.10750. Available online: http://arxiv.org/abs/1902.10750 (accessed on 20 July 2023).
- Kroutikova, N.; Hernandez-Aramburo, C.; Green, T. State-space model of grid-connected inverters under current control mode. IET Electr. Power Appl. 2007, 1, 329–338. [Google Scholar] [CrossRef]
- Mo, O.; D’Arco, S.; Suul, J.A. Evaluation of Virtual Synchronous Machines with Dynamic or Quasi-Stationary Machine Models. IEEE Trans. Ind. Electron. 2017, 64, 5952–5962. [Google Scholar] [CrossRef]
- Chen, M.; Zhou, D.; Blaabjerg, F. Power Control of Grid-Forming Converters Based on Full-State Feedback. In Proceedings of the IECON 2022—48th Annual Conference of the IEEE Industrial Electronics Society, Brussels, Belgium, 17–20 October 2022; pp. 1–6. [Google Scholar] [CrossRef]
- Chen, M.; Zhou, D.; Tayyebi, A.; Prieto-Araujo, E.; Dörfler, F.; Blaabjerg, F. On Power Control of Grid-Forming Converters: Modeling, Controllability, and Full-State Feedback Design. IEEE Trans. Sustain. Energy 2023, 1–12. [Google Scholar] [CrossRef]
- Lin, Y.; Eto, J.H.; Johnson, B.B.; Flicker, J.D.; Lasseter, R.H.; Pico, H.N.V.; Seo, G.-S.; Pierre, B.J.; Ellis, A. Research Roadmap on Grid-Forming Inverters’, National Renewable Energy Laboratory, Technical Report NREL/TP-5D00-73476. Available online: https://www.nrel.gov/docs/fy21osti/73476.pdf (accessed on 15 September 2023).
- Musca, R.; Vasile, A.; Zizzo, G. Grid-forming converters. A critical review of pilot projects and demonstrators. Renew. Sustain. Energy Rev. 2022, 165, 112551. [Google Scholar] [CrossRef]
- Ferreira, A.J.G.A.; Oliveira, A.C.; Lima, A.M.N. A Unified Control Framework for Grid-Forming Inverters. EasyChair, 2022; preprint. [Google Scholar]
- Wu, T.; Liu, Z.; Liu, J.; Wang, S.; You, Z. A Unified Virtual Power Decoupling Method for Droop-Controlled Parallel Inverters in Microgrids. IEEE Trans. Power Electron. 2016, 31, 5587–5603. [Google Scholar] [CrossRef]
- Rodriguez, P.; Candela, I.; Citro, C.; Rocabert, J.; Luna, A. Control of grid-connected power converters based on a virtual admittance control loop. In Proceedings of the 2013 15th European Conference on Power Electronics and Applications (EPE), Lille, France, 2–6 September 2013; pp. 1–10. [Google Scholar] [CrossRef]
- Rocabert, J.; Luna, A.; Blaabjerg, F.; Rodríguez, P. Control of Power Converters in AC Microgrids. IEEE Trans. Power Electron. 2012, 27, 4734–4749. [Google Scholar] [CrossRef]
- Du, W.; Lasseter, R.H.; Khalsa, A.S. Survivability of Autonomous Microgrid During Overload Events. IEEE Trans. Smart Grid 2019, 10, 3515–3524. [Google Scholar] [CrossRef]
- Rathnayake, D.B.; Akrami, M.; Phurailatpam, C.; Me, S.P.; Hadavi, S.; Jayasinghe, G.; Zabihi, S.; Bahrani, B. Grid Forming Inverter Modeling, Control, and Applications. IEEE Access 2021, 9, 114781–114807. [Google Scholar] [CrossRef]
- Zhao, F.; Wang, X.; Zhu, T. Power Dynamic Decoupling Control of Grid-Forming Converter in Stiff Grid. IEEE Trans. Power Electron. 2022, 37, 9073–9088. [Google Scholar] [CrossRef]
- Li, M.; Wang, Y.; Hu, W.; Shu, S.; Yu, P.; Zhang, Z.; Blaabjerg, F. Unified Modeling and Analysis of Dynamic Power Coupling for Grid-forming Converters. IEEE Trans. Power Electron. 2021, 37, 2321–2337. [Google Scholar] [CrossRef]
- De Brabandere, K.; Bolsens, B.; Van den Keybus, J.; Woyte, A.; Driesen, J.; Belmans, R. A Voltage and Frequency Droop Control Method for Parallel Inverters. IEEE Trans. Power Electron. 2007, 22, 1107–1115. [Google Scholar] [CrossRef]
- Li, Y.; Li, Y.W. Power Management of Inverter Interfaced Autonomous Microgrid Based on Virtual Frequency-Voltage Frame. IEEE Trans. Smart Grid 2011, 2, 30–40. [Google Scholar] [CrossRef]
- Gong, Z.; Liu, C.; Gui, Y.; da Silva, F.F.; Bak, C.L. Power Decoupling Method for Voltage Source Inverters Using Grid Voltage Modulated Direct Power Control in Unbalanced System. IEEE Trans. Power Electron. 2023, 38, 3084–3099. [Google Scholar] [CrossRef]
- Wen, T.; Zou, X.; Zhu, D.; Guo, X.; Peng, L.; Kang, Y. Comprehensive perspective on virtual inductor for improved power decoupling of virtual synchronous generator control. IET Renew. Power Gener. 2020, 14, 485–494. [Google Scholar] [CrossRef]
- Long, B.; Long, B.; Zhu, S.; Zhu, S.; Rodriguez, J.; Rodriguez, J.; Guerrero, J.M.; Guerrero, J.M.; Chong, K.T.; Chong, K.T. Enhancement of Power Decoupling for Virtual Synchronous Generator: A Virtual Inductor and Virtual Capacitor Approach. IEEE Trans. Ind. Electron. 2023, 70, 6830–6843. [Google Scholar] [CrossRef]
- Rathnayake, D.B.; Bahrani, B. Multivariable Control Design for Grid-Forming Inverters with Decoupled Active and Reactive Power Loops. IEEE Trans. Power Electron. 2023, 38, 1635–1649. [Google Scholar] [CrossRef]
- Peng, Z.; Wang, J.; Bi, D.; Wen, Y.; Dai, Y.; Yin, X.; Shen, J. Droop Control Strategy Incorporating Coupling Compensation and Virtual Impedance for Microgrid Application. IEEE Trans. Energy Convers. 2019, 34, 277–291. [Google Scholar] [CrossRef]
- Gong, Z.; Liu, C.; Shang, L.; Lai, Q.; Terriche, Y. Power Decoupling Strategy for Voltage Modulated Direct Power Control of Voltage Source Inverters Connected to Weak Grids. IEEE Trans. Sustain. Energy 2023, 14, 152–167. [Google Scholar] [CrossRef]
- Yan, X.; Zhang, Y. Power coupling analysis of inverters based on relative gain method and decoupling control based on feedforward compensation. In Proceedings of the International Conference on Renewable Power Generation (RPG 2015), Beijing, China, 17–18 October 2015; pp. 1–5. [Google Scholar] [CrossRef]
- Oureilidis, K.O.; Demoulias, C.S. A Fault Clearing Method in Converter-Dominated Microgrids with Conventional Protection Means. IEEE Trans. Power Electron. 2016, 31, 4628–4640. [Google Scholar] [CrossRef]
- Huang, L.; Wu, C.; Zhou, D.; Blaabjerg, F. A Power Angle Limiting Method for Improving Stability of Grid-Forming Inverter Under Overcurrent Condition. In Proceedings of the 2022 IEEE Energy Conversion Congress and Exposition (ECCE), Detroit, MI, USA, 9–13 October 2022; pp. 1–6. [Google Scholar] [CrossRef]
- Huang, L.; Wu, C.; Zhou, D.; Blaabjerg, F. A Power-Angle-Based Adaptive Overcurrent Protection Scheme for Grid-Forming Inverter Under Large Grid Disturbances. IEEE Trans. Ind. Electron. 2023, 70, 5927–5936. [Google Scholar] [CrossRef]
- Liu, B.; Liu, Z.; Liu, J.; An, R.; Zheng, H.; Shi, Y. An Adaptive Virtual Impedance Control Scheme Based on Small-AC-Signal Injection for Unbalanced and Harmonic Power Sharing in Islanded Microgrids. IEEE Trans. Power Electron. 2019, 34, 12333–12355. [Google Scholar] [CrossRef]
- Zhong, C.; Zhang, Z.; Zhu, A.; Liang, B. An Adaptive Virtual Impedance Method for Grid-Connected Current Quality Improvement of a Single-Phase Virtual Synchronous Generator under Distorted Grid Voltage. Sensors 2023, 23, 6857. [Google Scholar] [CrossRef]
- Azizi, A.; Banaiemoqadam, A.; Hooshyar, A.; Patel, M. A Blind Spot in the LVRT Current Requirements of Modern Grid Codes for Inverter-Based Resources. IEEE Trans. Power Deliv. 2023, 38, 319–334. [Google Scholar] [CrossRef]
- Garnica Lopez, M.A.; de Vicuna, J.L.G.; Miret, J.; Castilla, M.; Guzmán, R. Control Strategy for Grid-Connected Three-Phase Inverters During Voltage Sags to Meet Grid Codes and to Maximize Power Delivery Capability. IEEE Trans. Power Electron. 2018, 33, 9360–9374. [Google Scholar] [CrossRef]
- Xu, J.; Xie, S.; Tang, T. Improved control strategy with grid-voltage feedforward for LCL-filter-based inverter connected to weak grid. IET Power Electron. 2014, 7, 2660–2671. [Google Scholar] [CrossRef]
- Deng, H.; Qi, Y.; Fang, J.; Debusschere, V.; Tang, Y. A Low-Voltage-Ride-Through Strategy for Grid-Forming Converters Based on Reactive Power Synchronization. In Proceedings of the 2022 IEEE Energy Conversion Congress and Exposition (ECCE), Detroit, MI, USA, 9–13 October 2022; pp. 1–6. [Google Scholar] [CrossRef]
- Huang, L.; Xin, H.; Wang, Z.; Zhang, L.; Wu, K.; Hu, J. Transient Stability Analysis and Control Design of Droop-Controlled Voltage Source Converters Considering Current Limitation. IEEE Trans. Smart Grid 2019, 10, 578–591. [Google Scholar] [CrossRef]
- Deng, H.; Qi, Y.; Fang, J.; Tang, Y.; Debusschere, V. A Robust Low-Voltage-Ride-Through Strategy for Grid-Forming Converters Based on Reactive Power Synchronization. IEEE Trans. Power Electron. 2023, 38, 346–357. [Google Scholar] [CrossRef]
- Qoria, T.; Wang, X.; Kadri, R. Grid-forming control VSC-based including current limitation and re-synchronization functions to deal with symmetrical and asymmetrical faults. Electr. Power Syst. Res. 2023, 223, 109647. [Google Scholar] [CrossRef]



| GFL | GFM | |
|---|---|---|
| Instability | Unstable in weak grids due to oscillations in PLLs. | Unstable in strong grids and series-compensated grids. |
| Basic operation | In the presence of a stiff grid or voltage. | Island and grid-connected modes. |
| Ideal performance | Operates ideally when connected to an ideal voltage source ( | Operates ideally when connected to an ideal current source ( |
| Non-ideal grid conditions | Weak grid voltage, strong current, and small admittance. | Strong grid, weak current, and small impedance. |
| Current management | Operates vector current control; able to manage current in fault mode. | Uses current-limiting controls, causing loss of characteristics. |
| Contribution to grid | Decreases the strength of a grid. | Improves the strength of a grid. |
| Black start | No black start capability. | Capability of black start with energy storage. |
| Comparison to machines | Operates like a motor with vector current control in conventional grids. | Operates like a synchronous machine of conventional grids. |
| Synchronisation | PLL or FLL. | Power synchronisation; oscillator coupling. |
| Grid characteristics | Grid voltage following; current forming. | Grid current following; voltage forming. |
| Swing characteristics | Voltage (reactive power) angle swing. | Current (active power) angle swing. |
| Increased penetration levels | Results in a decrease in system damping. | Causes an increase in damping in the system, due to faster controls. |
| Current control | Good current control, due to inherent vector current control. | Prone to overload; must implement current limitation techniques to control current. |
| GFM Control | References | Basic Operation Characteristics | Controller Parameters | Fault Ride-through Performance | Inertia Support | Transient Stability |
|---|---|---|---|---|---|---|
| Droop Control | [59,60,61] | droop Power sharing | √ | N/A | √ | |
| PSC | [71] | Angle power droop | √ | N/A | √ | |
| VSG | [73,74,75,76,77,78,79,80,81,82,83,84,85,86,87,88,89] | droop Virtual inertia damping | , , | √ | √ | × |
| Synchronverter | [90,91,92,93,94,95,96,97] | droop SG excitation emulation Virtual inertia damping | , , , | √ | √ | √ |
| Matching Control | [99,100,101,102] | DC-link voltage control droop | , | √ | √ | √ |
| VOC | VOC [104,105,106,107,108,109,110,111,112,113,114,115] | Global synchronisation | N/A | N/A | √ | |
| dVOC [116,117,118,119,120,121,122] | Global synchronisation with power control | , | N/A | √ | √ |
| Major Features | Improvements | |
|---|---|---|
| HSC [131,132,134,135,136,137] |
|
|
| uVOC [127,141] |
|
|
| UniCon [142,143] |
| GFM control for all SCR and system conditions. |
| MIMO concept [144,148,153,154] |
| Provides the ease of converter modelling and modification to achieve the desired performance. |
| Design for interoperability [148,157] |
|
|
| Method | Basic Operation | References |
|---|---|---|
| Virtual Power Method | Derives new references for power through vector rotation. | [165] |
| Virtual Frequency and Voltage | Rotates the frequency and voltage vectors to new references. | [75,166,167] |
| Virtual Impedance | Reshapes line impedances through the introduction of virtual impedance terms. | [168,169,170] |
| Coupling Compensation and Feedforward Compensation | Augments power control loops through components of real and reactive power as compensation. | [163,171,172,173] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ebinyu, E.; Abdel-Rahim, O.; Mansour, D.-E.A.; Shoyama, M.; Abdelkader, S.M. Grid-Forming Control: Advancements towards 100% Inverter-Based Grids—A Review. Energies 2023, 16, 7579. https://doi.org/10.3390/en16227579
Ebinyu E, Abdel-Rahim O, Mansour D-EA, Shoyama M, Abdelkader SM. Grid-Forming Control: Advancements towards 100% Inverter-Based Grids—A Review. Energies. 2023; 16(22):7579. https://doi.org/10.3390/en16227579
Chicago/Turabian StyleEbinyu, Emmanuel, Omar Abdel-Rahim, Diaa-Eldin A. Mansour, Masahito Shoyama, and Sobhy M. Abdelkader. 2023. "Grid-Forming Control: Advancements towards 100% Inverter-Based Grids—A Review" Energies 16, no. 22: 7579. https://doi.org/10.3390/en16227579
APA StyleEbinyu, E., Abdel-Rahim, O., Mansour, D.-E. A., Shoyama, M., & Abdelkader, S. M. (2023). Grid-Forming Control: Advancements towards 100% Inverter-Based Grids—A Review. Energies, 16(22), 7579. https://doi.org/10.3390/en16227579