Optimal Generation Dispatch in Electrical Microgrids Based on Inertia Markets as a Solution to Frequency Stability
Abstract
:1. Introduction
Literature Review
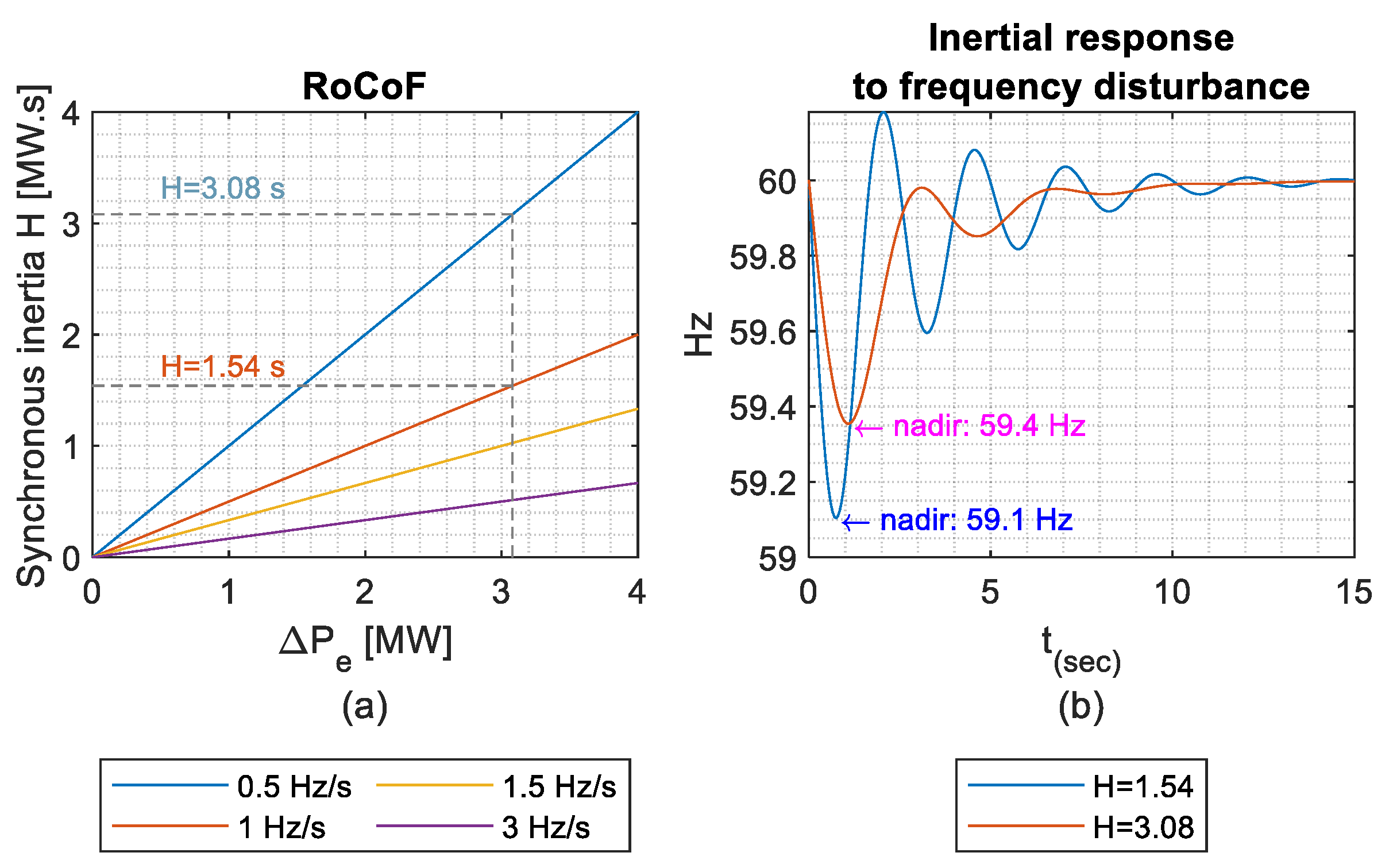
2. Frequency Response
3. Methods and Materials
3.1. Minimum Inertia Levels
3.2. Economic Dispatch Problem Formulation
- A first case arises where all renewable resources must be consumed first, and conventional generators must reduce their generation capacity to give preference to these.
- To evaluate the differences between costs, a second case is proposed where the inertia restriction is included in the economic dispatch.
- None of the renewable resources have synthetic inertia.
- We simplify the model by ignoring the randomness of renewable resources due to the focus of our proposal on reducing the participation of renewable energy in the dispatch. Therefore, an hourly dispatch period is considered over a 24-h horizon in all case studies.
- It is assumed that all renewable energy sources have resources based on 24-h power profiles.
- The system operator dispatches all conventional and renewable generation sources.
- It is considered that the way to control or limit the dispatch of renewable resources is through the diversion or derivation of the power produced for other uses such as heating or storage.
- In the case of the Slack bar and the synchronous generator, a quadratic cost function is considered because in our approach, they are the type of source that predominates in the dispatch.
- In the case of renewable resources, linear cost functions are considered.
3.3. Objective Function
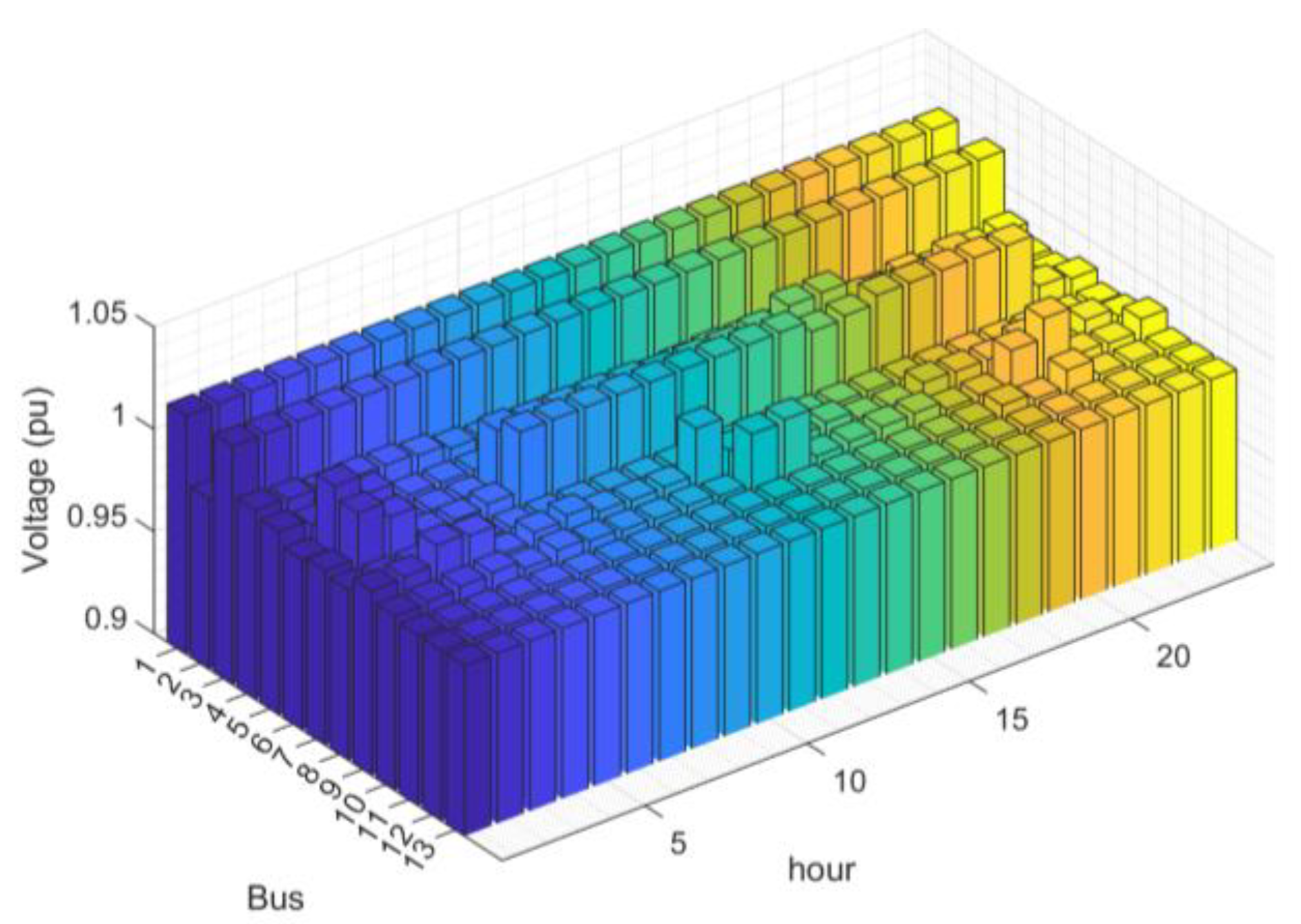
4. Simulation and Discussion
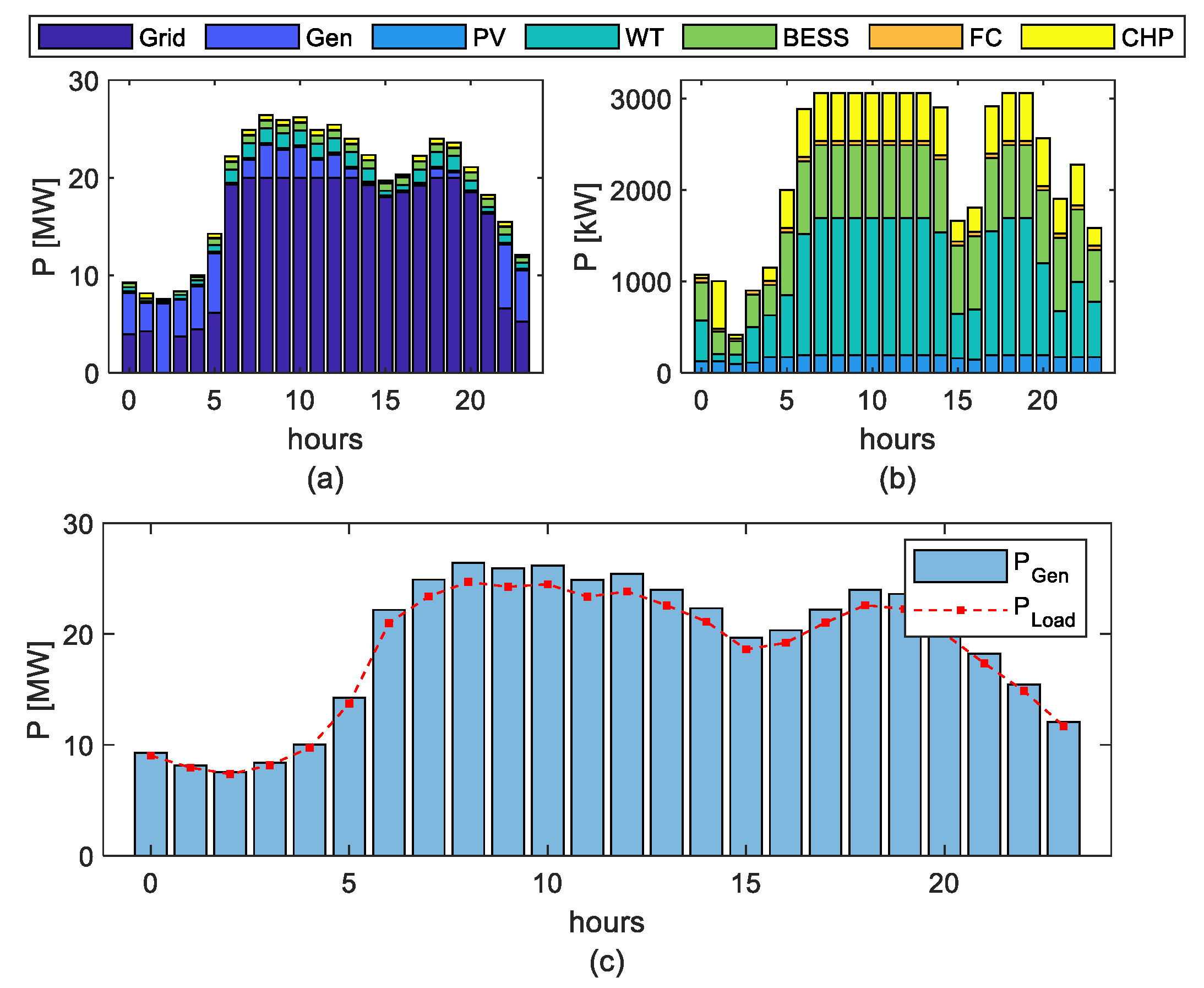
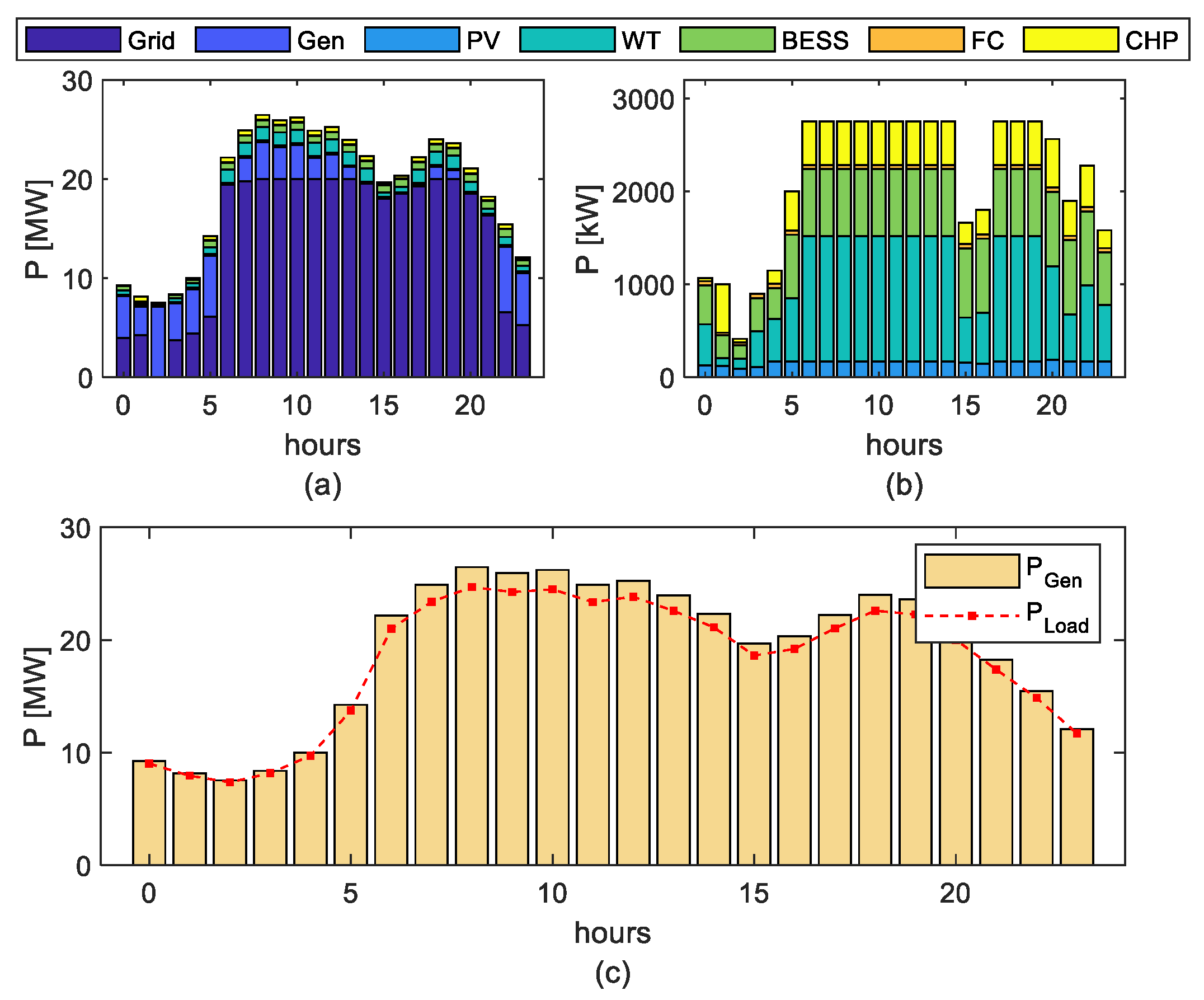
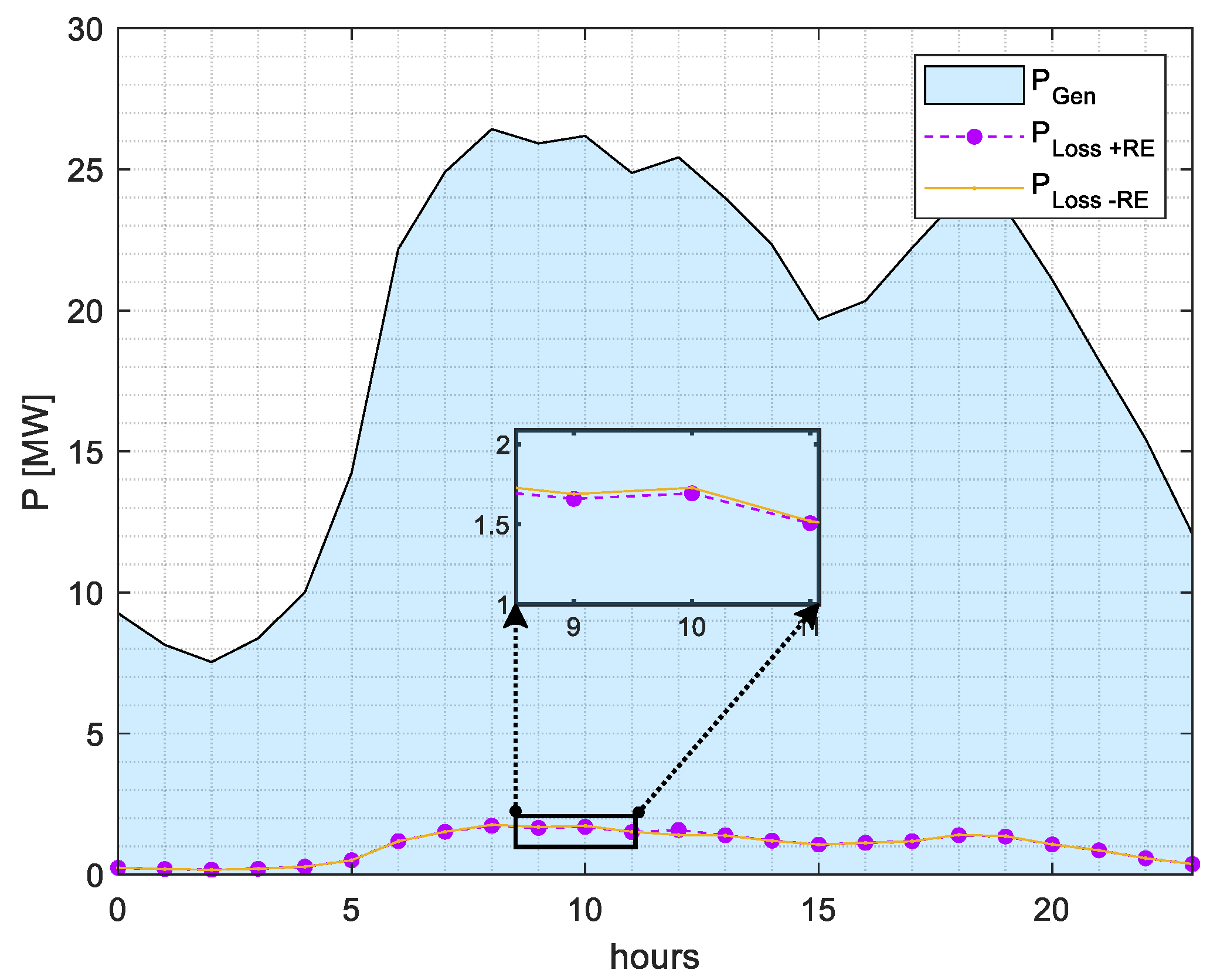
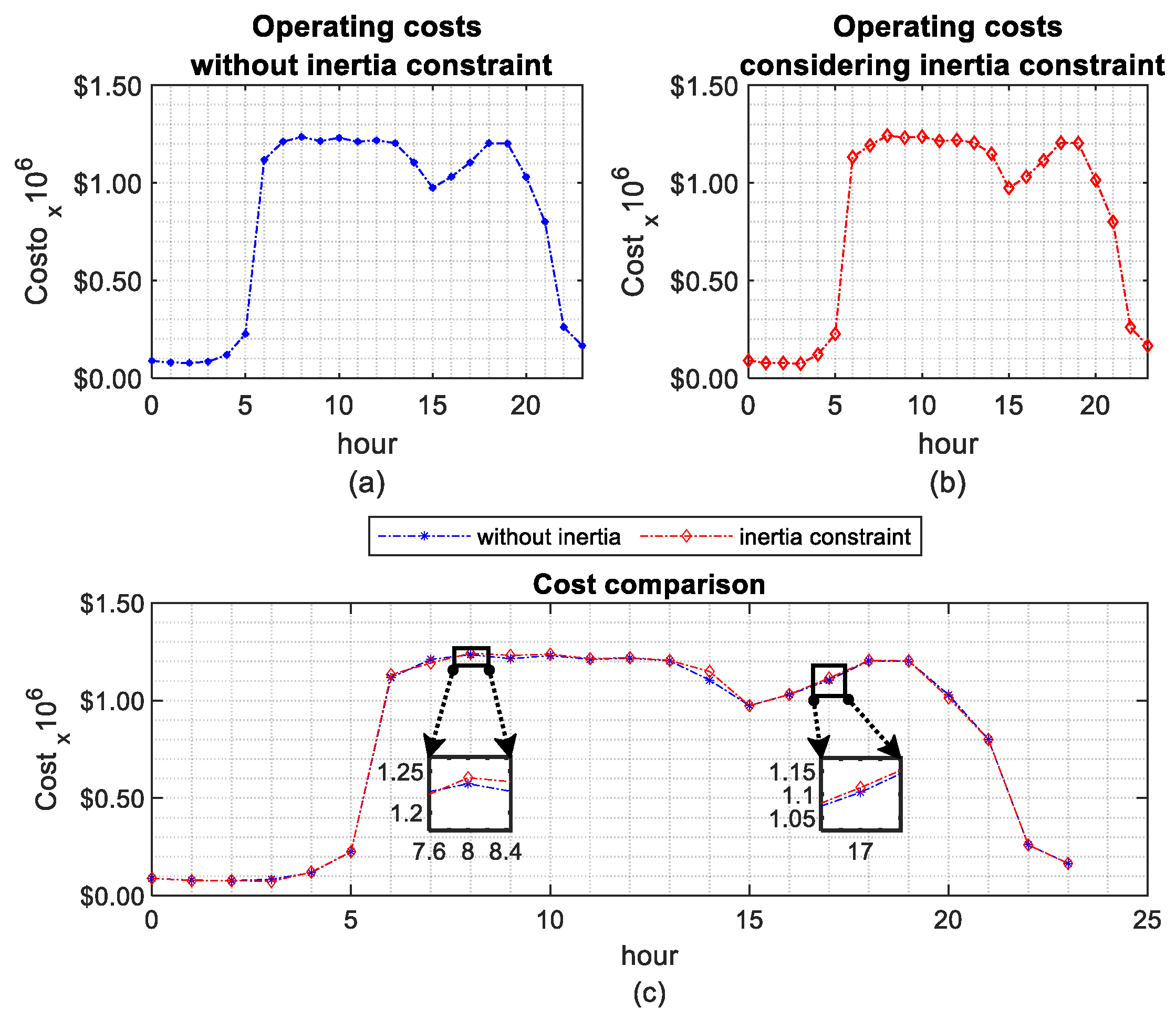
4.1. Base Case: Priority of Renewable Resources in Energy Dispatch
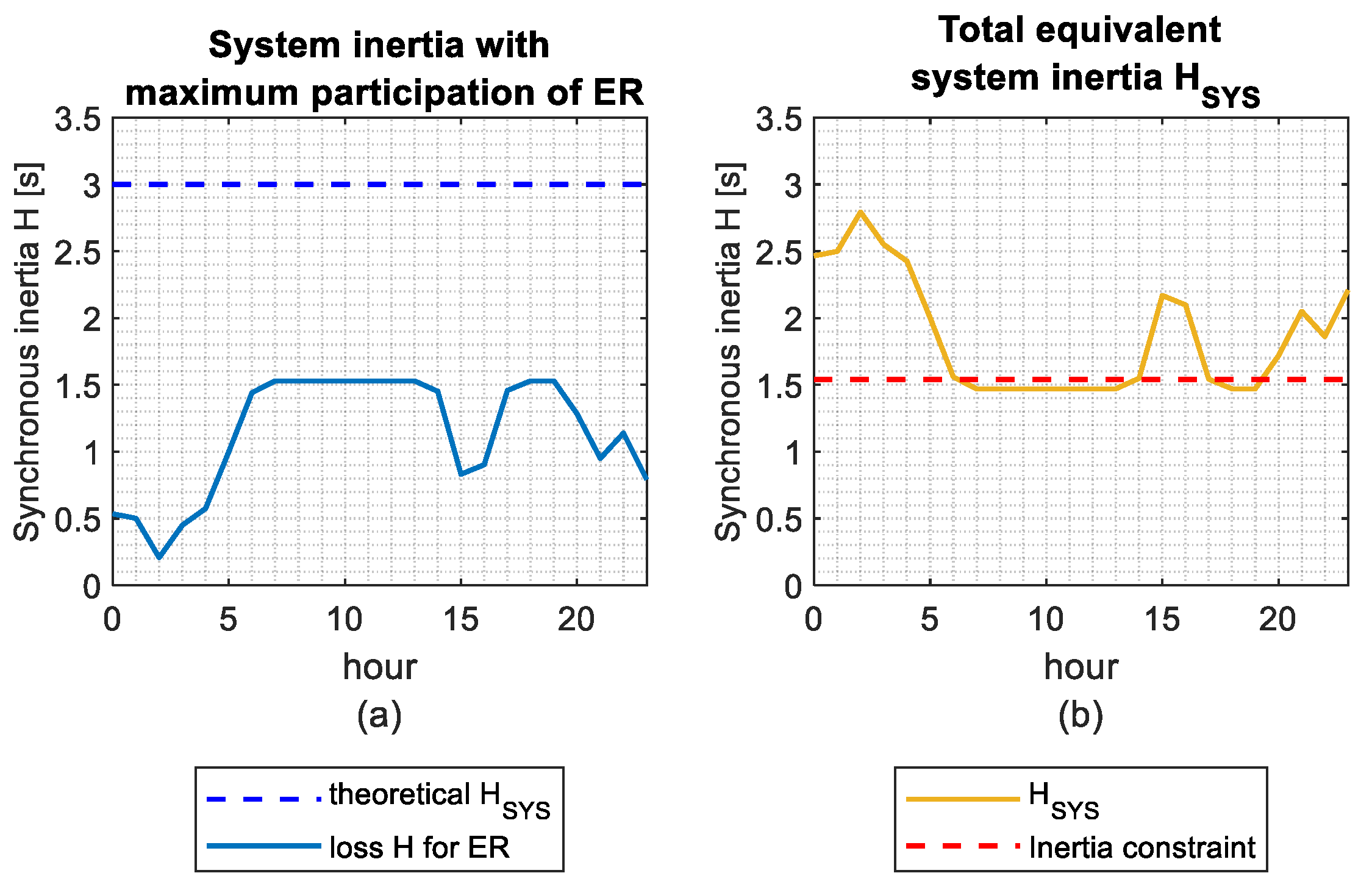
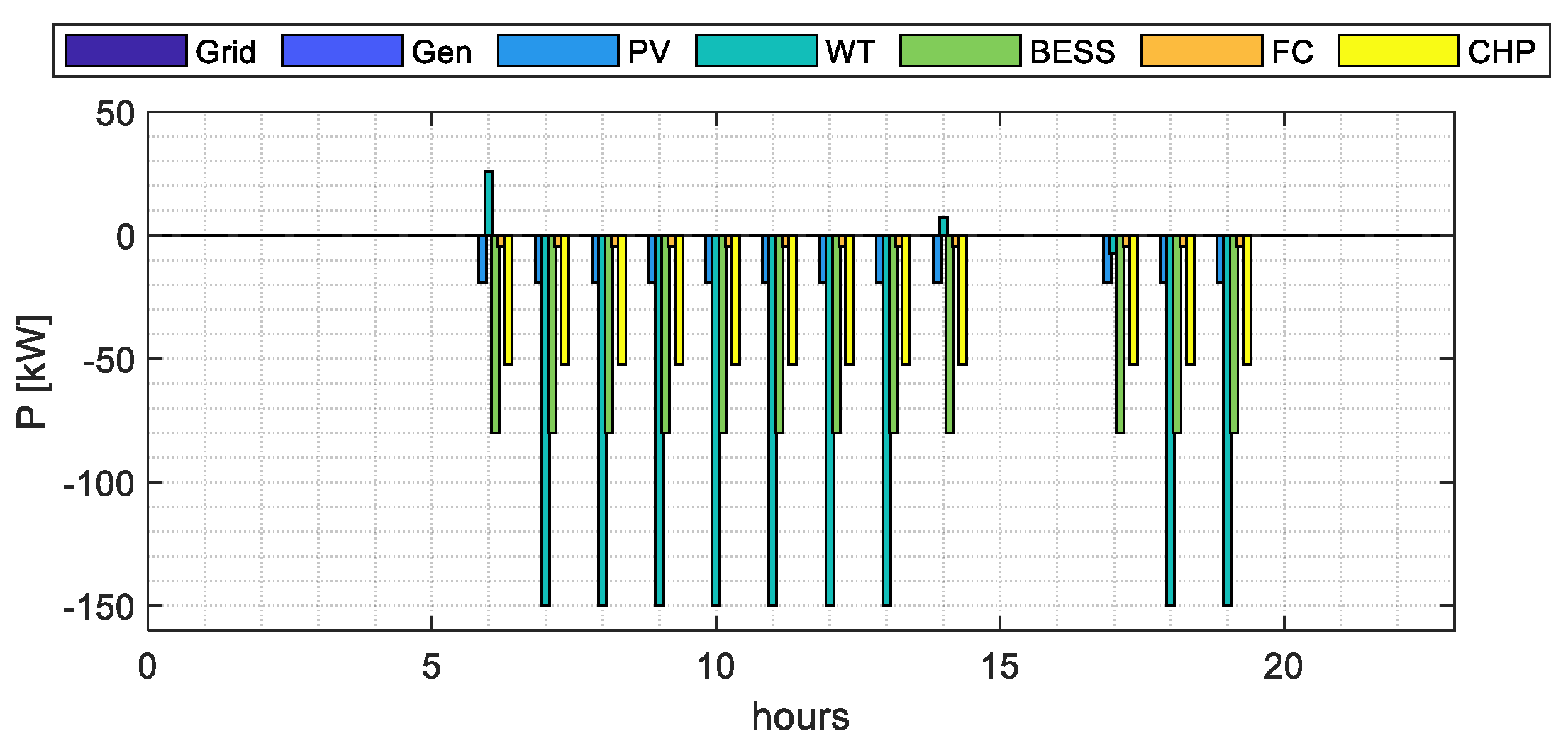
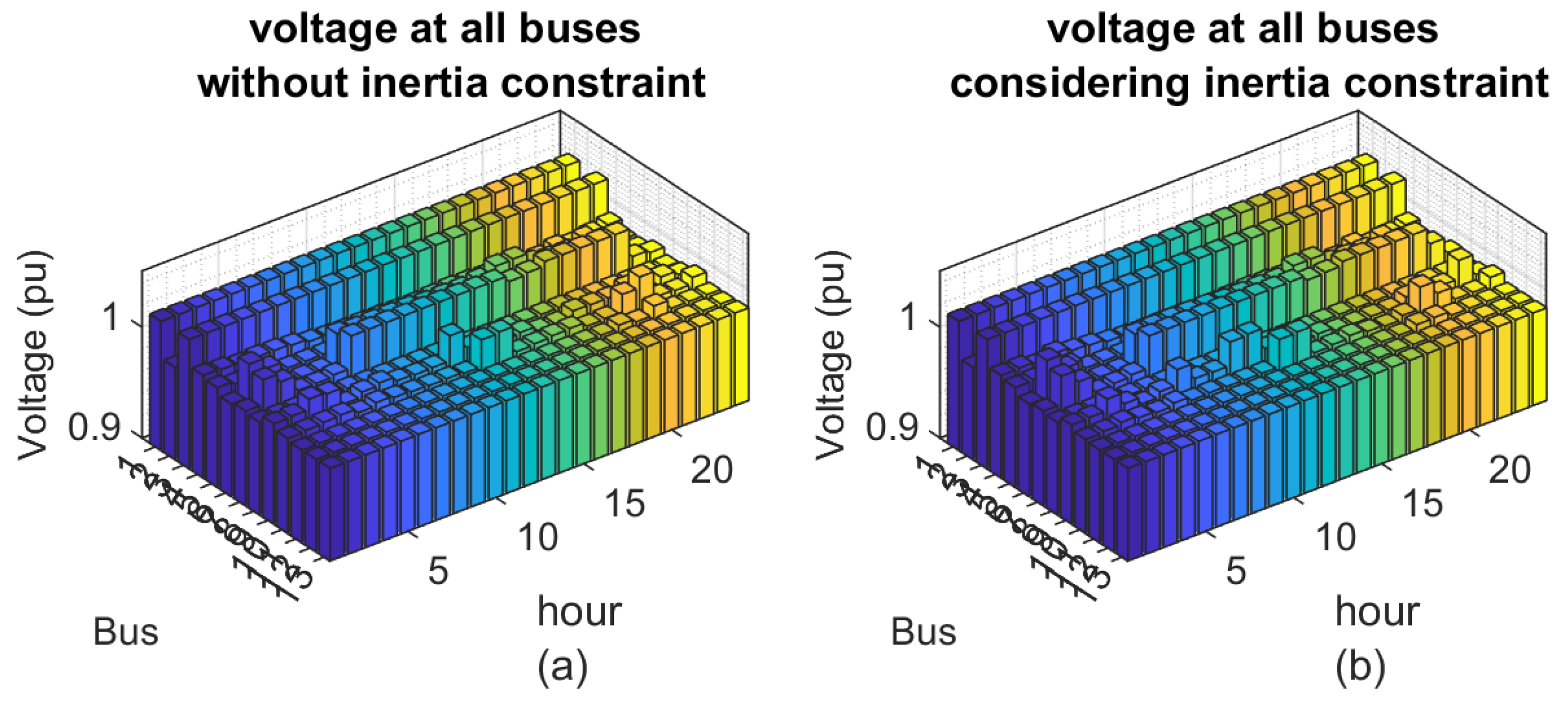
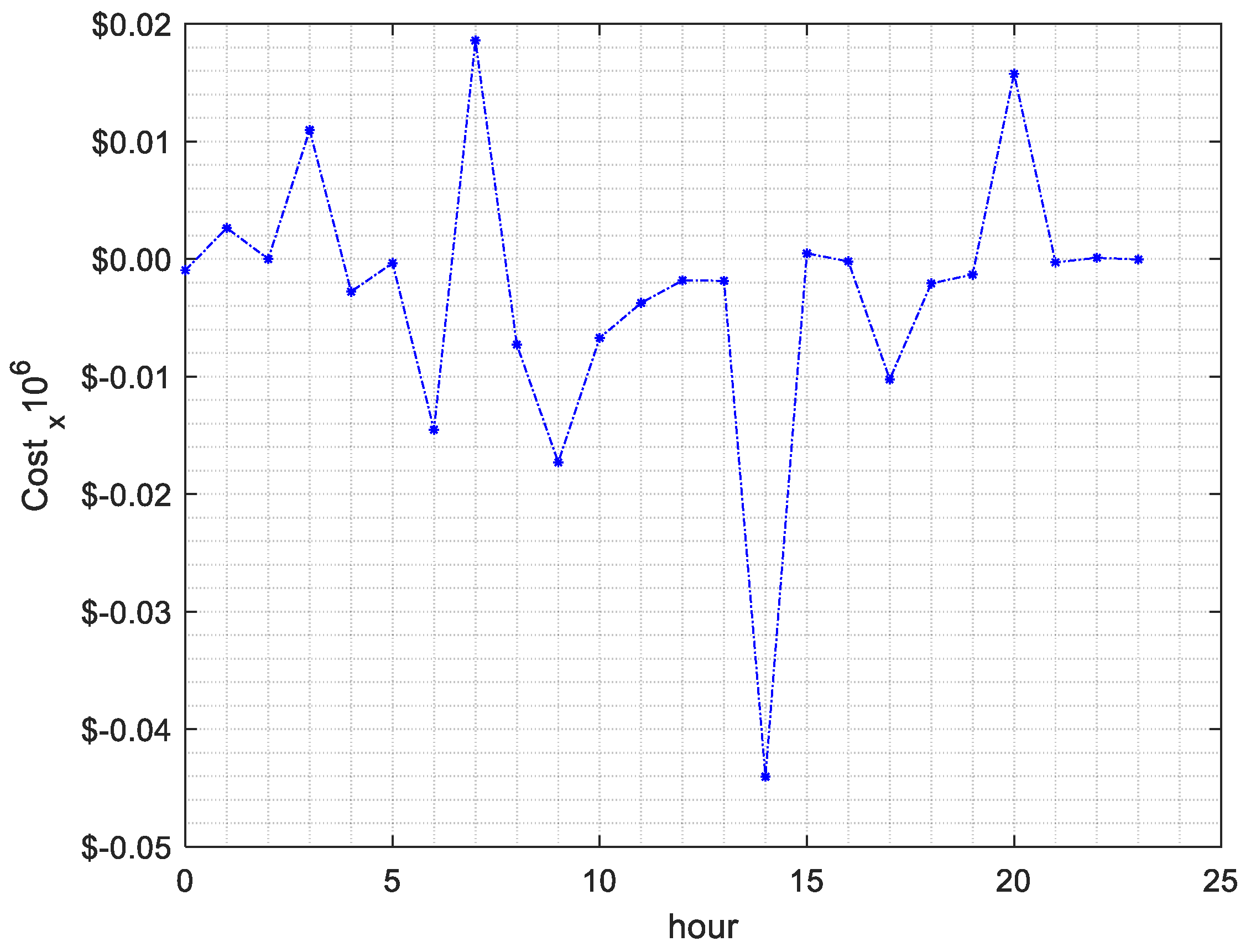
4.2. Case: Inclusion of the Inertia (H) Constraint
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| AND | Active distribution network |
| APSO | Adaptive particle swarm optimization |
| BESS | Battery energy storage system |
| CHP | Combined heat and power |
| CS | Cuckoo search |
| DER | Distributed energy resources |
| ED | Economic dispatch |
| ERCOT | Electric Reliability Council of Texas |
| FC | Fuel cell |
| MG | Microgrid |
| MILP | Mixed integer linear programming |
| NLP | Nonlinear programming |
| NORDIC | The Nordic Region |
| OPF | Optimal power flow |
| PSO | Particle swarm optimization |
| PV | Solar photovoltaics |
| RES | Renewable energy sources |
| ROCOF | Rate of change of frequency |
| UC | Unit commitment |
| UCED | Unit commitment and economic dispatch |
| UFLS | Under-frequency load shedding |
| WBS | Wind–battery hybrid power system |
| WT | Wind turbine |
| Subscripts | Definition |
| i | Synchronous generation units |
| j | Renewable Energy Sources |
| k | Sending bus |
| m | Receiving bus |
| t | Time intervals |
| Parameters | |
| Minimum inertia of the system (s) | |
| Active power demand at bus k (MW) | |
| Maximum/minimum limits of active power, synchronous generation unit i | |
| Reactive power demand at bus k (MW) | |
| Maximum/minimum limits of reactive power, synchronous generation unit i | |
| Vmax/min | Maximum/minimum voltage limits at all nodes |
| ai, bi, ci | Operating cost coefficients of unit i ($/MWh2, $MWh and $/h) |
| f0 | Nominal frequency of the system (p.u) |
| Active power connected to bus k at time t (MW) | |
| Reactive power connected to bus k at time t (MVAr) | |
| ykm | Admittance of branch connecting bus k to m |
| βj | Operating cost coefficient of RES j at time t ($/MWh) |
| Nb | Set of all buses |
| Ng | Set of buses with synchronous generators. |
| Nr | Set of buses with RESs |
| T | Number of time intervals t (24 h) |
| π | Limits of voltage angle of bus k (rad) |
| Variables | |
| CCG | Cost of synchronous generation ($) |
| CDER | Cost of generation with RES ($) |
| CT | Total operating costs ($) |
| Scheduled output active power for synchronous generation unit i at time t (MW) | |
| Scheduled output active power for RES generation unit j at time t (MW) | |
| Scheduled output reactive power for synchronous generation unit i at time t (MVAr) | |
| vk/m | Voltage magnitude at bus km at time t (V) |
| δk,t | Voltage angle of bus k at time t (rad) |
References
- Zografos, D. Power System Inertia Estimation and Frequency Response Assessment. Ph.D. Thesis, KTH, School of Electrical Engineering and Computer Science, Stockholm, Sweden, 2019. [Google Scholar]
- Hartmann, B.; Vokony, I.; Táczi, I. Effects of decreasing synchronous inertia on power system dynamics—Overview of recent experiences and marketisation of services. Int. Trans. Electr. Energy Syst. 2019, 29, e12128. [Google Scholar] [CrossRef]
- Agrawal, U.; O’Brien, J.; Somani, A.; Mosier, T.; Dagle, J. A Study of the Impact of Reduced Inertia in Power Systems. In Proceedings of the 53rd Hawaii International Conference on System Sciences, Grand Wailea, HI, USA, 7–10 January 2020; Volume 3, pp. 3010–3017. [Google Scholar]
- Denholm, P.; Mai, T.; Kenyon, R.W.; Kroposki, B.; O’Malley, M. Inertia and the Power Grid: A Guide Without the Spin; Technical Report 1659820; National Renewable Energy Lab: Golden, CO, USA, 2020. [Google Scholar]
- Fernández-Guillamón, A.; Gómez-Lázaro, E.; Muljadi, E.; Molina-García, Á. Power systems with high renewable energy sources: A review of inertia and frequency control strategies over time. Renew. Sustain. Energy Rev. 2019, 115, 109369. [Google Scholar] [CrossRef]
- Zhao, J.; Lyu, X.; Fu, Y.; Hu, X.; Li, F. Coordinated Microgrid Frequency Regulation Based on DFIG Variable Coefficient Using Virtual Inertia and Primary Frequency Control. IEEE Trans. Energy Convers. 2016, 31, 833–845. [Google Scholar] [CrossRef]
- Kerdphol, T.; Rahman, F.S.; Watanabe, M.; Mitani, T. Virtual Inertia Synthesis and Control; Part of the book series Power Systems; Springer: Berlin/Heidelberg, Germany, 2021. [Google Scholar]
- National Technical University of Athens. Renewables 2018—Global Status Report; REN21: Paris, France, 2018. [Google Scholar]
- Daly, P.; Flynn, D.; Cunniffe, N. Inertia considerations within unit commitment and economic dispatch for systems with high non-synchronous penetrations. In Proceedings of the 2015 IEEE Eindhoven PowerTech, Eindhoven, The Netherlands, 29 June–2 July 2015; Institute of Electrical and Electronics Engineers Inc.: Piscataway, NJ, USA, 2015. [Google Scholar]
- Davarinejad, E.; Reza Hesamzadeh, M.; Chavez, H. Incorporating Inertia Constraints into the Power Market; Technical Report 2017:392; Energiforsk: Stockholm, Sweden, 2017. [Google Scholar]
- Wang, T.; Meskin, M.; Zhao, Y.; Grinberg, I. Optimal Power Flow in Distribution Networks with High Penetration of Photovoltaic Units. In Proceedings of the IEEE Electrical Power and Energy Conference (EPEC), Saskatoon, SK, Canada, 22–25 October 2017. [Google Scholar]
- Ma, Y.; Zou, G.; Hou, M.; Dong, Q.; Yang, J. Optimal dispatching for active distribution network with wind-battery hybrid power system. In Proceedings of the 2017 IEEE Electrical Power and Energy Conference (EPEC), Saskatoon, SK, Canada, 22–25 October 2017; pp. 1–6. [Google Scholar]
- Xia, H.; Li, Q.; Xu, R.; Chen, T.; Wang, J.; Hassan, M.A.S.; Chen, M. Distributed Control Method for Economic Dispatch in Islanded Microgrids with Renewable Energy Sources. IEEE Access 2018, 6, 21802–21811. [Google Scholar] [CrossRef]
- Guggilam, S.S.; Zhao, C.; Dall’Anese, E.; Chen, Y.C.; Dhople, S.V. Optimizing Power-Frequency Droop Characteristics of Distributed Energy Resources. IEEE Trans. Power Syst. 2018, 33, 3076–3086. [Google Scholar] [CrossRef]
- Moghaddas-Tafreshi, S.M.; Mohseni, S.; Karami, M.E.; Kelly, S. Optimal energy management of a grid-connected multiple energy carrier micro-grid. Appl. Therm. Eng. 2019, 152, 796–806. [Google Scholar]
- Rebollal, D.; Chinchilla, M.; Santos-Martin, D.; Guerrero, J.M. Endogenous approach of a frequency-constrained unit commitment in islanded microgrid systems. Energies 2021, 14, 19. [Google Scholar]
- Lu, T.; Jiiang, D.; Jia, Y. Estimation of Generator Inertia Using Mean Value of Active Power Change After a Disturbance. In Proceedings of the 2019 IEEE Sustainable Power and Energy Conference (iSPEC), Beijing, China, 21–23 November 2019; IEEE: Piscataway, NJ, USA, 2019. [Google Scholar]
- Hellebust, H. Analysis of Frequency Stability: How Wind Power and HVDC Connections Affect the Future Power System. Master’s Thesis, Norwegian University of Science and Technology, Trondheim, Norway, 2018. [Google Scholar]
- Sølna, A.H. Strategies to Ensure Sufficient Inertia in the Norwegian Power System. Master’s Thesis, Norwegian University of Science and Technology, Trondheim, Norway, 2018. [Google Scholar]
- Seneviratne, C.; Ozansoy, C. Frequency response due to a large generator loss with the increasing penetration of wind/PV generation—A literature review. Renew. Sustain. Energy Rev. 2016, 57, 659–668. [Google Scholar] [CrossRef]
- Persson, M. Frequency Response by Wind Farms in Power Systems with High Wind Power Penetration. Ph.D. Thesis, Chalmers University of Technology, Gothenburg, Sweden, 2017; 178p. [Google Scholar]
- Fang, J.; Li, H.; Tang, Y.; Blaabjerg, F. On the Inertia of Future More-Electronics Power Systems. IEEE J. Emerg. Sel. Top. Power Electron. 2019, 7, 2130–2146. [Google Scholar] [CrossRef]
- Jorgenson, J.; Denholm, P. Modeling Primary Frequency Response for Grid Studies; Technical Report 1489895; National Renewable Energy Lab: Golden, CO, USA, 2019. [Google Scholar]
- Greve, T.; Teng, F.; Pollitt, M.G.; Strbac, G. A system operator’s utility function for the frequency response market. Appl. Energy 2018, 231, 562–569. [Google Scholar] [CrossRef]
- Chen, H.; Ngan, H.; Zhang, Y. Power System Optimization: Large-Scale Complex Systems Approaches; Wiley: Hoboken, NJ, USA, 2016; 359p. [Google Scholar]
- Wood, A.J.; Wollenberg, B.F.; Sheblé, B.G. Power Generation, Operation, and Control; Wiley: Hoboken, NJ, USA, 2014. [Google Scholar]
- Zhu, J. Optimization of Power System Operation, 2nd ed.; Mohamed, E., Hawary, E., Eds.; Wiley: Hoboken, NJ, USA, 2015. [Google Scholar]
- Gómez, A. Analisis y Operacion de Sistemas de Energia Eléctrica; Graw Hill: New York, NY, USA, 2002. [Google Scholar]
- Scarabaggio, P.; Carli, R.; Dotoli, M. Noncooperative Equilibrium-Seeking in Distributed Energy Systems under AC Power Flow Nonlinear Constraints. IEEE Trans. Control Netw. Syst. 2022, 9, 1731–1742. [Google Scholar] [CrossRef]
- Yao, M.; Molzahn, D.K.; Mathieu, J.L. An optimal power-flow approach to improve power system voltage stability using demand response. IEEE Trans. Control Netw. Syst. 2019, 6, 1015–1025. [Google Scholar] [CrossRef]
- Nayak, C.K.; Kasturi, K.; Nayak, M.R. Economical management of microgrid for optimal participation in electricity market. J. Energy Storage 2019, 21, 657–664. [Google Scholar] [CrossRef]
- Strunz, K. Benchmark Systems for Network Integration of Renewable and Distributed Energy Resources. CIGRE Task Force C6.04.02; ELECTRA. July 2014. Volume 575. Available online: https://e-cigre.org/publication/ELT_273_8-benchmark-systems-for-network-integration-of-renewable-and-distributed-energy-resources (accessed on 25 June 2022).
- Solanki, B.V.; Bhattacharya, K.; Canizares, C.A. A Sustainable Energy Management System for Isolated Microgrids. IEEE Trans. Sustain. Energy 2017, 8, 1507–1517. [Google Scholar] [CrossRef]
- Henze, V. Scale-Up of Solar and Wind Puts Existing Coal, Gas at Risk//BloombergNEF. 2020. Available online: https://about.bnef.com/blog/scale-up-of-solar-and-wind-puts-existing-coal-gas-at-risk/#_ftnref1 (accessed on 25 June 2022).





| Ref | ED | UC | OPF | Technique |
|---|---|---|---|---|
| [9] | X | X | MILP | |
| [10] | X | MILP | ||
| [11] | X | Cuckoo | ||
| [12] | X | PSO | ||
| [13] | X | Lagrange | ||
| [14] | X | X | Lagrange | |
| [15] | X | PSO | ||
| [16] | X | MILP |
| Node | Resource | Rated Power | Cost Function Coefficients | ||
|---|---|---|---|---|---|
| kW | a, $/MWh2 | b, $/MWh | c, $/h | ||
| 1 | Slack | 20,000 | 0.0030 | 0.020 | 0.1 |
| 17 | Gen | 15,000 | 0.0035 | 0.048 | 0.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cruz, L.; Téllez, A.Á.; Ortiz, L. Optimal Generation Dispatch in Electrical Microgrids Based on Inertia Markets as a Solution to Frequency Stability. Energies 2023, 16, 7500. https://doi.org/10.3390/en16227500
Cruz L, Téllez AÁ, Ortiz L. Optimal Generation Dispatch in Electrical Microgrids Based on Inertia Markets as a Solution to Frequency Stability. Energies. 2023; 16(22):7500. https://doi.org/10.3390/en16227500
Chicago/Turabian StyleCruz, Luis, Alexander Águila Téllez, and Leony Ortiz. 2023. "Optimal Generation Dispatch in Electrical Microgrids Based on Inertia Markets as a Solution to Frequency Stability" Energies 16, no. 22: 7500. https://doi.org/10.3390/en16227500
APA StyleCruz, L., Téllez, A. Á., & Ortiz, L. (2023). Optimal Generation Dispatch in Electrical Microgrids Based on Inertia Markets as a Solution to Frequency Stability. Energies, 16(22), 7500. https://doi.org/10.3390/en16227500








