1. Introduction
A piezoelectric vibration energy harvester directly harvests mechanical energy from environmental excitations and converts it into electrical energy by utilizing the positive piezoelectric effect of PZT patches. It has the advantages of a simple structure, a small size and high energy efficiency performance and is widely used in wireless sensor networks, remote monitoring devices, the low power consumption of devices and other scenarios [
1,
2,
3]. By harvesting vibrational energy in the environment, microelectronic devices can be self-powered, reducing or eliminating the need for conventional battery replacement [
4], thereby reducing device maintenance costs and increasing device reliability and lifetime. Therefore, harvesting energy directly from the environment is a perfect solution for powering microelectronic devices in the future and has great value in research and application [
5,
6].
Linear piezoelectric energy harvesters are usually limited to a narrow frequency band, making it difficult to efficiently generate electrical power at low frequencies [
7,
8]. Nonlinear piezoelectric vibration energy harvesters seem to have better harvesting performance and can generally be classified into four categories: monostable [
9], bistable [
10], tristable [
11] and multistable [
12]. Many scholars have done a lot of research on the nonlinear multi-stable state. Erturk and Inman et al. [
13,
14] experimentally verified that the nonlinear transition behavior induces high-energy interwell oscillations of the bistable energy harvester under a harmonic excitation, which greatly improves the energy harvesting performance. Singh et al. [
15] proposed an accurate model of the bistable sensor using a nonlinear extraction circuit, and the average power obtained in the experiment was more than 100 times higher than the extraction power reported in the similar literature. Cao et al. [
16,
17] studied the nonlinear dynamic characteristics of magnetically coupled broadband energy harvesters under low-frequency excitation. Experiments proved that rotating, magnetically coupled energy harvesters have available frequency bandwidths for a wide range of low-frequency excitations. Energy harvesting is dominated by high-energy chaotic attractors and inter-trap oscillations. In [
18], the influence of excitation parameters and magnet spacing on the output power of the bistable harvester was investigated in the time–frequency domain. The bistable motion not only widens the frequency bandwidth of the system but also increases the average power of the system.
However, bistable large-amplitude well-to-well oscillations require stronger excitation triggers. Zhou et al. [
19,
20] were the first to carry out theoretical modeling and experimental research on the nonlinear-broadband, bistable energy harvester. The experimental results show that compared with the bistable energy harvester, the tristable energy harvester has a shallower potential well and can produce higher energy output in a wider frequency range. Zhou and Zou [
21] used the harmonic balance method to study the asymmetric tristable energy harvester and found that the potential barrier is the key factor determining the orbital height of the high-energy interwell oscillation. Li et al. [
22] studied the influence of parameters such as the distance between the magnets at the fixed end on the nonlinear response characteristics of the tristable harvester. Wang et al. [
23] and Li et al. [
24] investigated geometric nonlinearity, gravity models and ladder-trap tristable energy harvesters, respectively, to increase the output voltage under weak random excitation. Since then, Zhou and Qin have studied the tetrastable [
25] and even pentastable [
26] systems and found that the more potential wells, the more uniform the potential energy distribution is and the lower the potential barrier between the potential wells, but the structure becomes more complicated.
The above research mainly focuses on the piezoelectric vibration energy harvester of the rectangular cross-section cantilever beam. Because the bending stress of the rectangular cantilever beam changes along the length, the maximum power output of the bending stress is the highest at the fixed end, and the minimum power output tends to zero at the free end. The variable-cross-section beam vibration energy harvester can optimize the uniform distribution of a PZT patch strain along the length of the piezoelectric beam well and improve the power generation power of the piezoelectric sheet pasted on both sides of the cantilever beam root [
27,
28].
In order to obtain the higher output performance of piezoelectric energy harvesters, scholars also studied triangular, trapezoidal, concave and arc-shaped beam structures [
8]. Baker et al. [
29] found that linearly varying the cross section of the beam along its length increased the output power density of the energy harvester by up to 30%. Shan et al. [
30] found that for the same volume of piezoelectric material, the maximum output power of the triangular piezoelectric structure is more than three times higher than that of the rectangular structure. Wang et al. [
31] studied the structure of a triangular-cross-section cantilever beam piezoelectric energy harvester and experimentally proved that the output power is higher as the width of the end becomes narrower. Ma et al. [
32] and Tan et al. [
33] studied the trapezoidal-cross-section cantilever piezoelectric energy harvester and proved that the stress distribution in the trapezoidal cantilever is more uniform than that in the rectangular cantilever, and the trapezoidal-cantilever piezoelectric energy harvester has a higher voltage output. He et al. [
27] compared trapezoidal and rectangular piezoelectric vibration energy harvesters. The piezoelectric vibration energy harvester with a concave beam shape has a uniform surface stress distribution and performs the best output.
The above studies on the cross section of the cantilever show that by optimizing the cross section of the nonlinear vibration cantilever structure, better strain distribution, lower resonance frequency and higher output power density can be obtained. Finding a more suitable cantilever-cross-section optimization function is the biggest challenge to achieving better performance.
In order to describe the dynamic vibration response of a piezoelectric energy harvester, it is necessary to obtain an accurate solution of the kinetic equation of the cantilever beam. The distributed parametric model (DPM) can give an accurate solution for the uniform-cross-section cantilever beam, and the dynamic characteristics of the energy harvester can be easily obtained [
34,
35]. However, the derivation of DPM for cantilever beams with linear changes in cross section is more complicated. Ismail [
36] uses the finite element method to establish the finite element model of a linear-gradient piezoelectric cantilever beam, which improves the solution accuracy of the concentrated parametric model (LPM). For energy harvesters with exponentially changing cross sections, Salmani [
37] studied piezoelectric energy harvesters with exponential cross sections and tip mass, proving that exponential gradients can increase the power density of piezoelectric beams, but did not study the dynamic characteristics of collectors with external magnets.
In summary, among many optimized piezoelectric energy harvesters with variable sections, although exponential-attenuation piezoelectric energy harvesters show potential advantages in theory, it is difficult to comprehensively evaluate their performance in practical engineering applications due to the lack of research on the dynamic, steady-state characteristics of exponential piezoelectric energy harvesters under the action of a multi-magnet, nonlinear magnetic force, as well as the influence of different optimization exponential attenuation coefficients and system structure parameters on their dynamic characteristics, energy conversion efficiency and numerical output power. This limits the development and application of models in real-world engineering applications. Therefore, further research in these areas will help to fully reveal the potential and application areas of these energy harvesters.
In order to study the geometric structure of a variable-cross-section cantilever beam piezoelectric energy harvester with better energy harvesting performance, a variable-exponential-cantilever-beam piezoelectric energy harvester is proposed in this paper. The distributed parameter force–magnetism–electricity-coupled dynamics model of the variable-exponential-cross-section, tristable, piezoelectric vibration energy harvester was established by the Euler–Bernoulli beam theory and Lagrange equation. The time–frequency dynamics and energy output characteristics were studied through experimental simulation; the influence of the main structural parameters of the harvester on its nonlinear dynamic response and voltage output performance was numerically investigated, and the variable exponential coefficient on the output was analyzed. The mapping relationship of power density is expected to provide a reference for the geometric structure design and optimization of variable-cross-section cantilever piezoelectric energy harvesters.
4. Analysis of System Dynamic Response
In this paper, a variable-section cantilever beam with length L = 0.07 m and initial width b
0 = 0.01 m is studied. The length of the PZT patch is L
p = 0.01 m and the initial width is also b
0 = 0.01 m. Preliminary, the exponential coefficient
p = −0.5 is seleted. For specific physical parameters,
Table A1 of
Appendix A can be referred to.
The 4th–5th order Rungekuta algorithm ODE45 is used to solve the electrolinear coupling equation of the piezoelectric vibration energy harvester represented by Equation (26) in the MATLAB simulation software, and the time-domain solution of the system under different initial conditions and different system parameters is obtained, such as vibration displacement, vibration velocity, output voltage and phase diagram. Here we uniformly default the initial condition to (0,0,0).
4.1. Potential Energy Analysis of Harvester
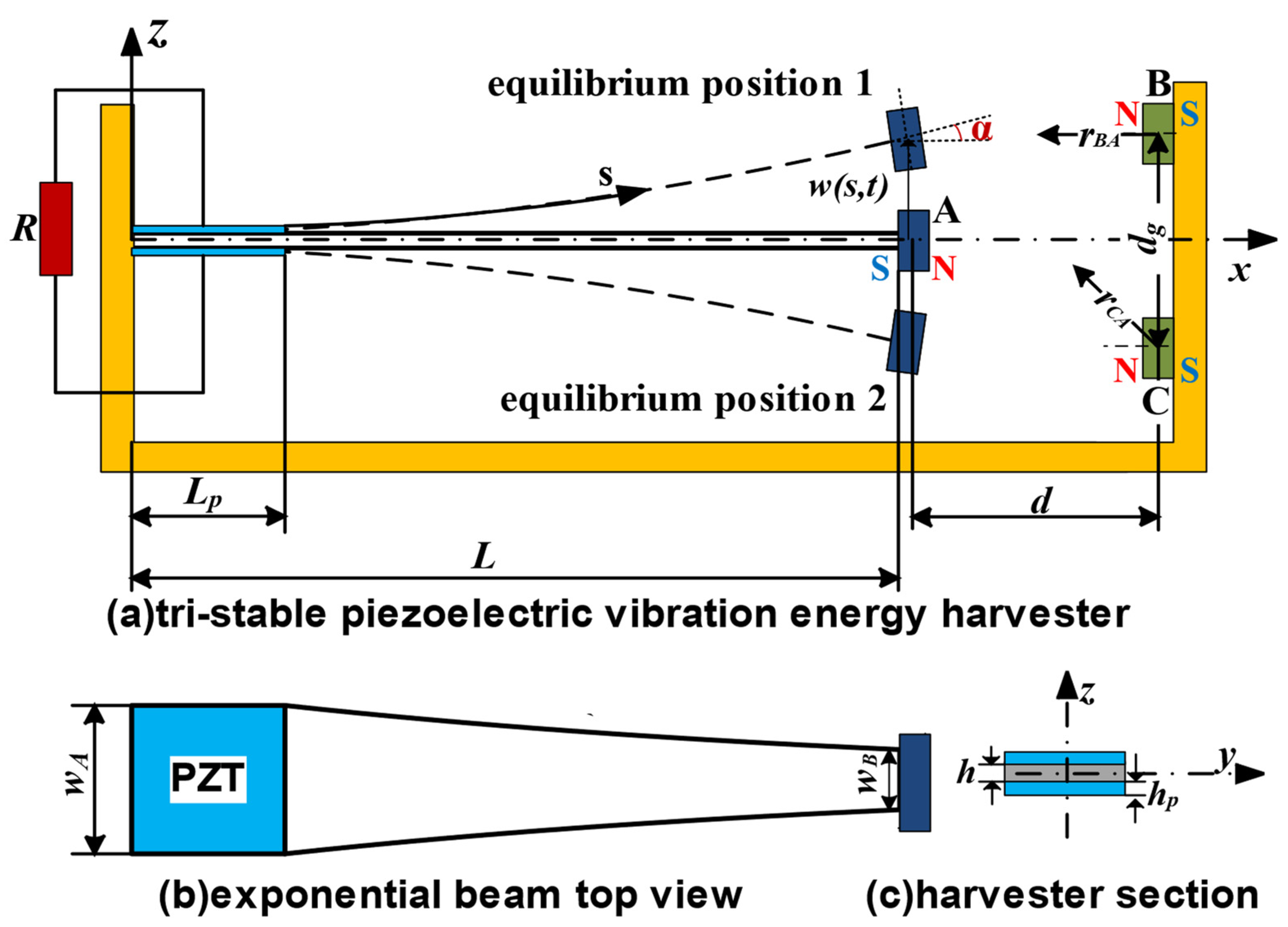
The interaction between magnets A, B and C is the main cause of the nonlinearity of the system. According to the Formulas (7)–(10), the distance dg between the fixed-end magnets and the horizontal distance d between the end magnets and the fixed-end magnets jointly determine the distribution of the magnetic field and the magnitude of the magnetic force of the tristable energy harvester system, thus affecting the potential energy of the energy harvester.
Figure 2 shows the distribution curves of the magnetic potential energy and total potential energy of the harvester when d = 17 mm and d
g is 13 mm, 17 mm and 50 mm, respectively. It can be seen from the figure that as d
g gradually increases, the harvester successively experienced a bistable state, monostable state and tristable state. When d
g = 13 mm, the magnetic potential energy at the central position is the largest, so that the total potential energy forms two potential barriers at symmetrical positions on both sides, with the largest width and the harvester presents a bistable state; as d
g increases to 17 mm, the magnetic potential energy is in the two short potential barriers formed on both sides, the magnetic potential energy at the center is concave, and the total potential energy appears at three stable equilibrium points, the potential well at the center becomes deeper and the harvester moves in a tristable state; as d
g further increases to 50 mm, the horizontal distance between the magnets is too large, the total potential energy only has a potential well in the center and the harvester degenerates into monostable state.
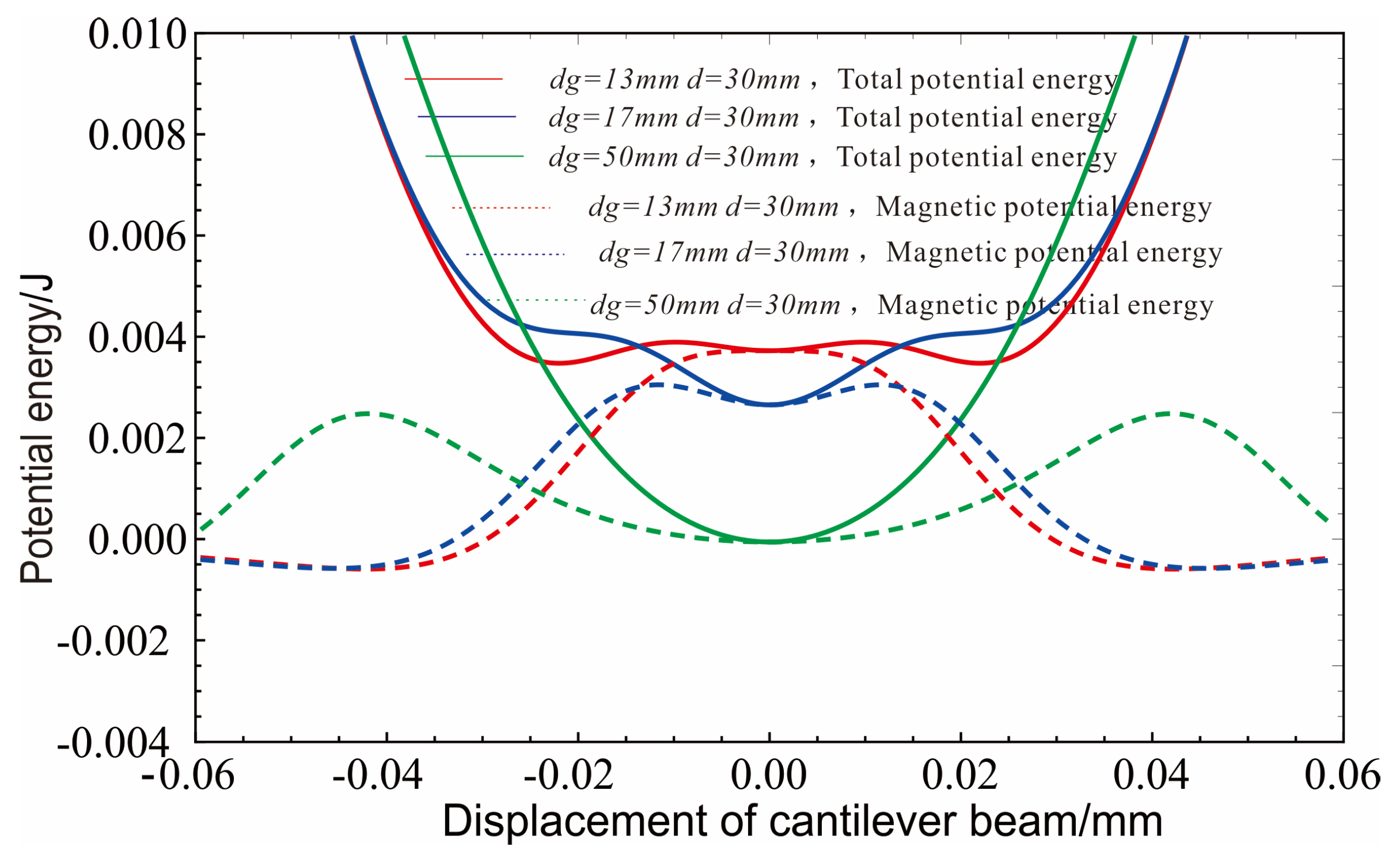
Figure 3 shows the distribution curves of the magnetic potential energy and total potential energy of the harvester when d
g = 17 mm and d is 30 mm, 35 mm and 50 mm, respectively. It can be seen from the figure that as d
g gradually decreases, the magnetic potential energy at the center gradually increases, and the total potential energy also changes with this law. When d = 50 mm, because the distance between the end magnet and the fixed-end magnet is too large, the magnetic potential energy is small, the total potential energy curve is slightly affected and there is only one equilibrium position, which is in monostable state. As d increases to 35 mm, the potential energy of the magnetic field increases significantly, and a potential well begins to appear, which is still in a monostable state; when d = 30 mm, the potential energy of the magnetic field continues to increase, two potential wells appear, the center of the total potential energy well becomes deeper and the harvester begins to appear in three equilibrium positions at this time, forming a tristable structure. The case of d = 30 mm is consistent with
Figure 2.
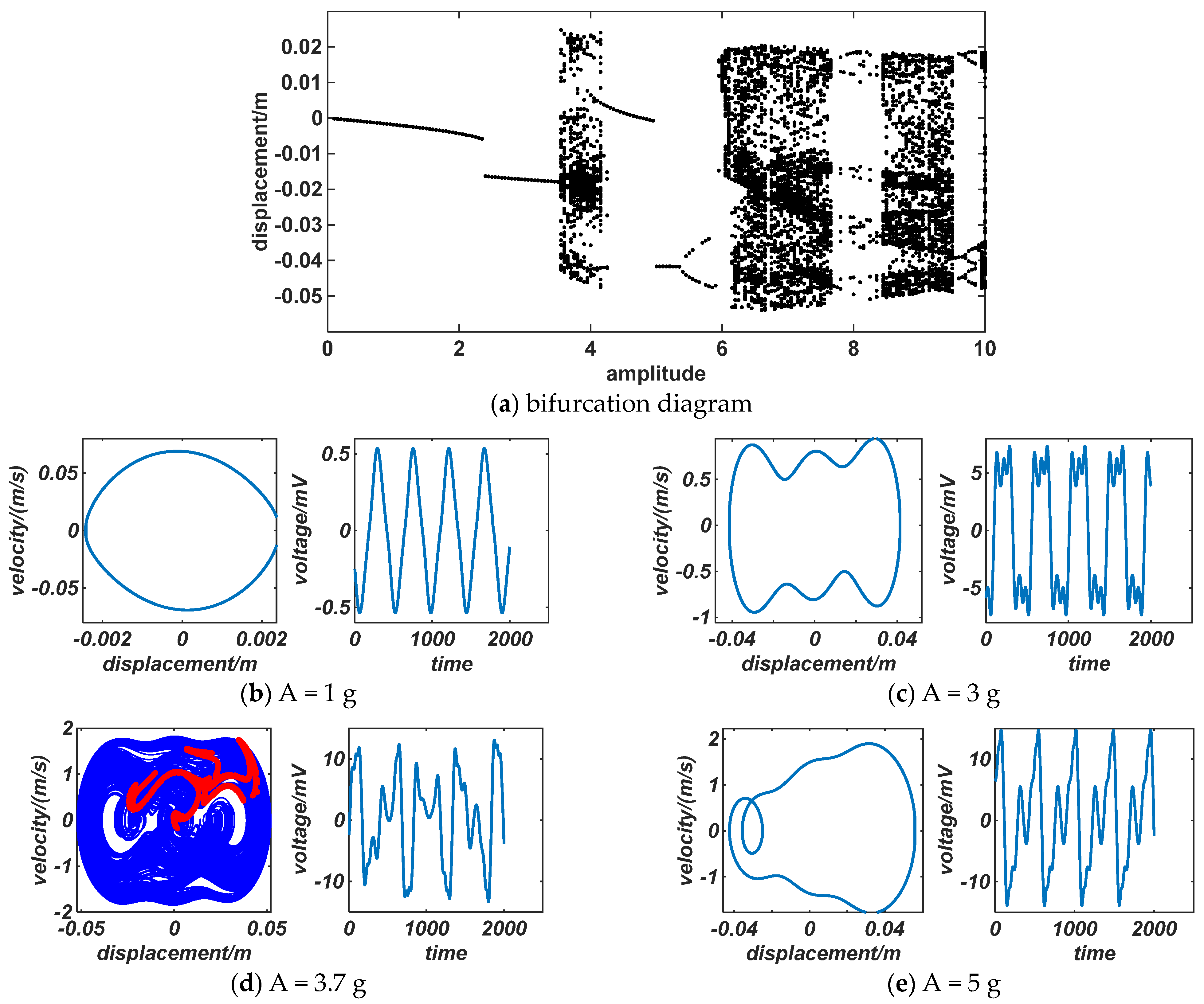
4.2. Influence of Acceleration Amplitude on Dynamic Characteristics
Under the magnet distances d = 30 mm and d
g = 17 mm, the excitation frequency f = 4 Hz is constant, and the excitation acceleration amplitude A is used as the bifurcation parameter to draw the bifurcation diagram. The tristable system exhibits a variety of nonlinear phenomena, as shown in
Figure 4a.
When the acceleration amplitude A < 2.4 g, the system cannot cross the potential barrier, makes small periodic oscillations in a single potential well and performs only monostable motion.
Figure 4b shows the displacement velocity of the system when A = 1 g, the phase diagram and the output voltage response. The output voltage of the harvester is 0.5 mV. When the acceleration amplitude A = 2.4 g, the system crosses the potential barrier, and then the vibration amplitude and output voltage of the system continue to increase, and there is a tristable motion, and the output voltage can reach 6 mV, as shown in
Figure 4c, A = 3 g. As the acceleration amplitude increases to 3.5~4.2 g, the system begins to exhibit a large-scale chaotic motion and there is a strange attractor (indicated by the red line in the
Figure 4), which represents the acyclical, disordered system state in the chaotic system. The voltage output jumps rapidly, as shown in
Figure 4d. By further increasing the excitation acceleration, the system crosses the next potential barrier until A = 5 g, the system behaves as a bistable single-cycle vibration, and the motion amplitude and output voltage amplitude are both large, as shown in
Figure 4e. As the excitation acceleration amplitude is further increased, the system response alternates between chaotic, quasi-periodic and periodic motions.
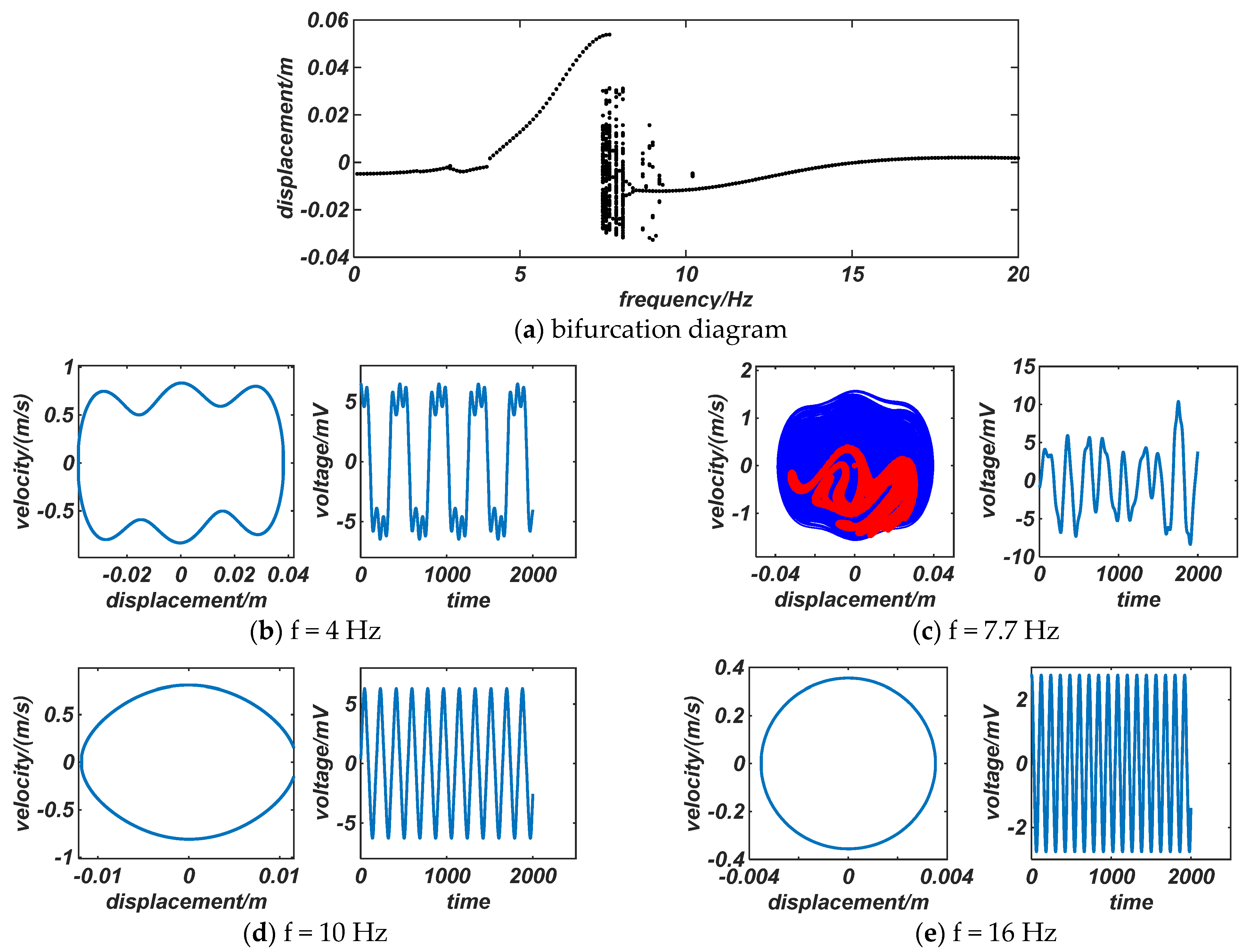
4.3. Influence of Excitation Frequency on System Response Characteristics
Keeping the acceleration excitation amplitude at 2 g, the bifurcation diagram of the system with respect to the excitation frequency, f, is drawn. Changing the excitation frequency also causes chaos, as shown in
Figure 5a.
In the low-frequency range f = 0~7.5 Hz, the harvester response shows a single-cycle motion whose amplitude gradually increases.
Figure 5b shows the motion phase diagram when f = 4 Hz, and the maximum output voltage of the acquisition is 6 mV at this time; when the frequency comes to f = 7.6~8.1 Hz, the system responds to chaotic motion, and the output voltage jumps rapidly with time.
Figure 5c shows the Poincaré diagram and output voltage of the system at f = 7.7 Hz;
Figure 5d shows that within f = 8.2~15 Hz, the system initially exhibits a small-amplitude, double-periodic motion and some chaotic motion. As the excitation frequency is increased, the system gradually stabilizes and the motion amplitude gradually decreases. When entering the high-frequency region f > 15 Hz, the system has only a very small-amplitude, monostable periodic vibration, and the output voltage is only 3 mV, as shown in
Figure 5e.
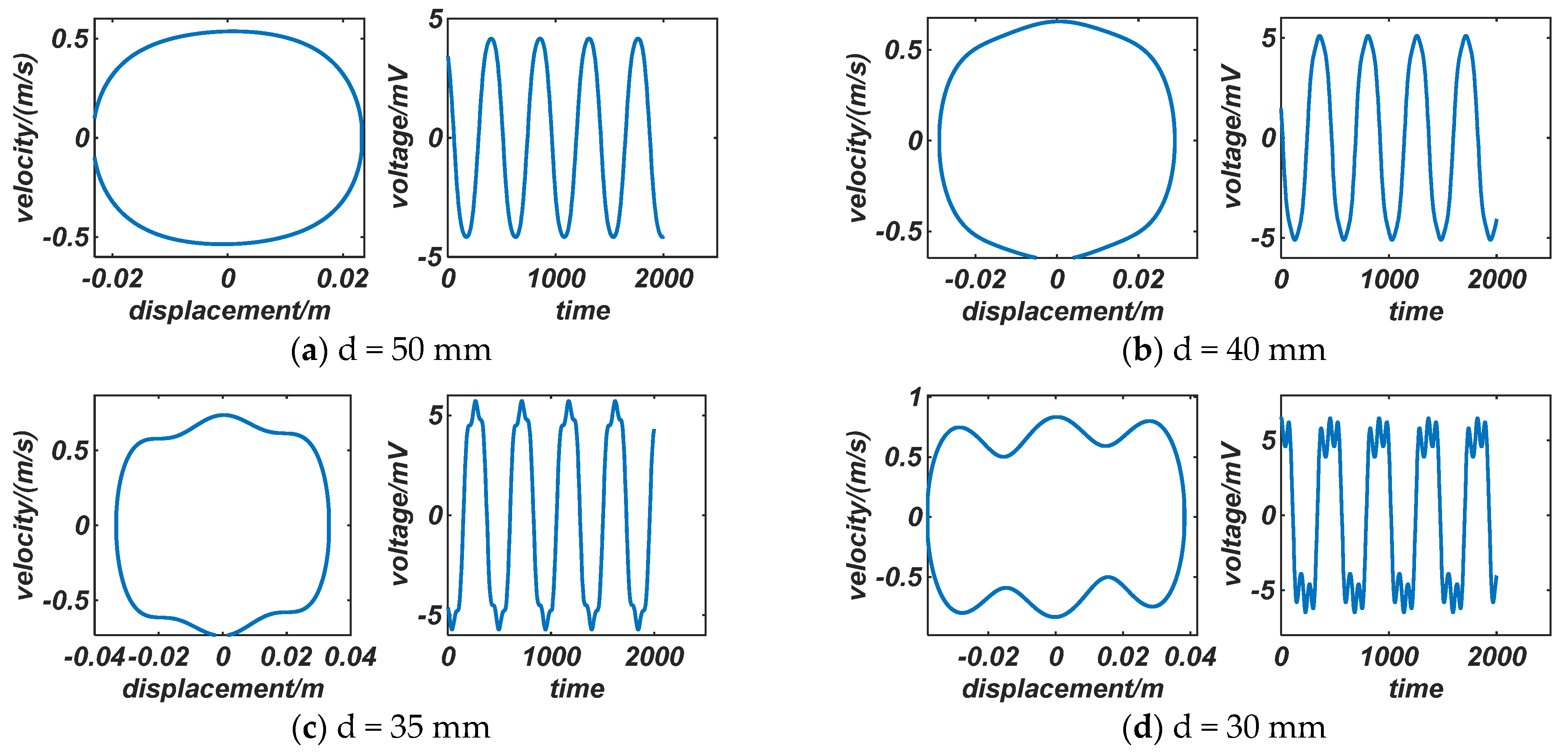
4.4. Influence of Magnet Spacing d on System Dynamics
Under the condition that the frequency f = 4 Hz, the excitation amplitude A = 2 g and the distance between the fixed magnets d
g = 17 mm remain unchanged, the distance d between the terminal magnet and the fixed magnet is 30 mm, 35 mm and 50 mm, respectively, and an energy harvester is manufactured. The phase diagram and voltage output diagram are shown in
Figure 6.
It can be seen from
Figure 6a that when d = 50 mm, due to the large horizontal distance between the end magnet and the fixed-end magnet, the nonlinear magnetic force has little effect on the harvester, and the harvester performs monostable periodic motion, and the output voltage of the harvester is 4 mV. When the distance between the end magnet and the fixed-end magnet is reduced to 40 mm, it can be seen from the phase diagram of the reaper movement that the magnetic force received by the end magnet increases, the speed of the reaper in the equilibrium position increases significantly, and the output voltage of the harvester reaches 5 mV, as shown in
Figure 6b.
When the horizontal distance between the end magnet and the fixed-end magnet decreases to d = 35 mm, the nonlinear magnetic force between the magnets increases, the constraint of the nonlinear magnetic force on the energy harvester becomes larger and the motion state of the harvester jumps from monostable to tristable. In this state, an equilibrium position is formed at the central equilibrium position and at both ends, but the potential wells between each steady state are relatively shallow, and the acquisition voltage reaches 5.6 mV, as shown in
Figure 6c.
When the horizontal distance between the end magnet and the fixed-end magnet is further reduced to d = 30 mm, the influence of the nonlinear magnetic force on the end magnet becomes more obvious, the tristable motion of the harvester becomes more obvious, and the potential well between the steady states becomes deeper, forming three stable equilibrium states, and the acquisition voltage reaches 6.2 mV, as shown in
Figure 6d.
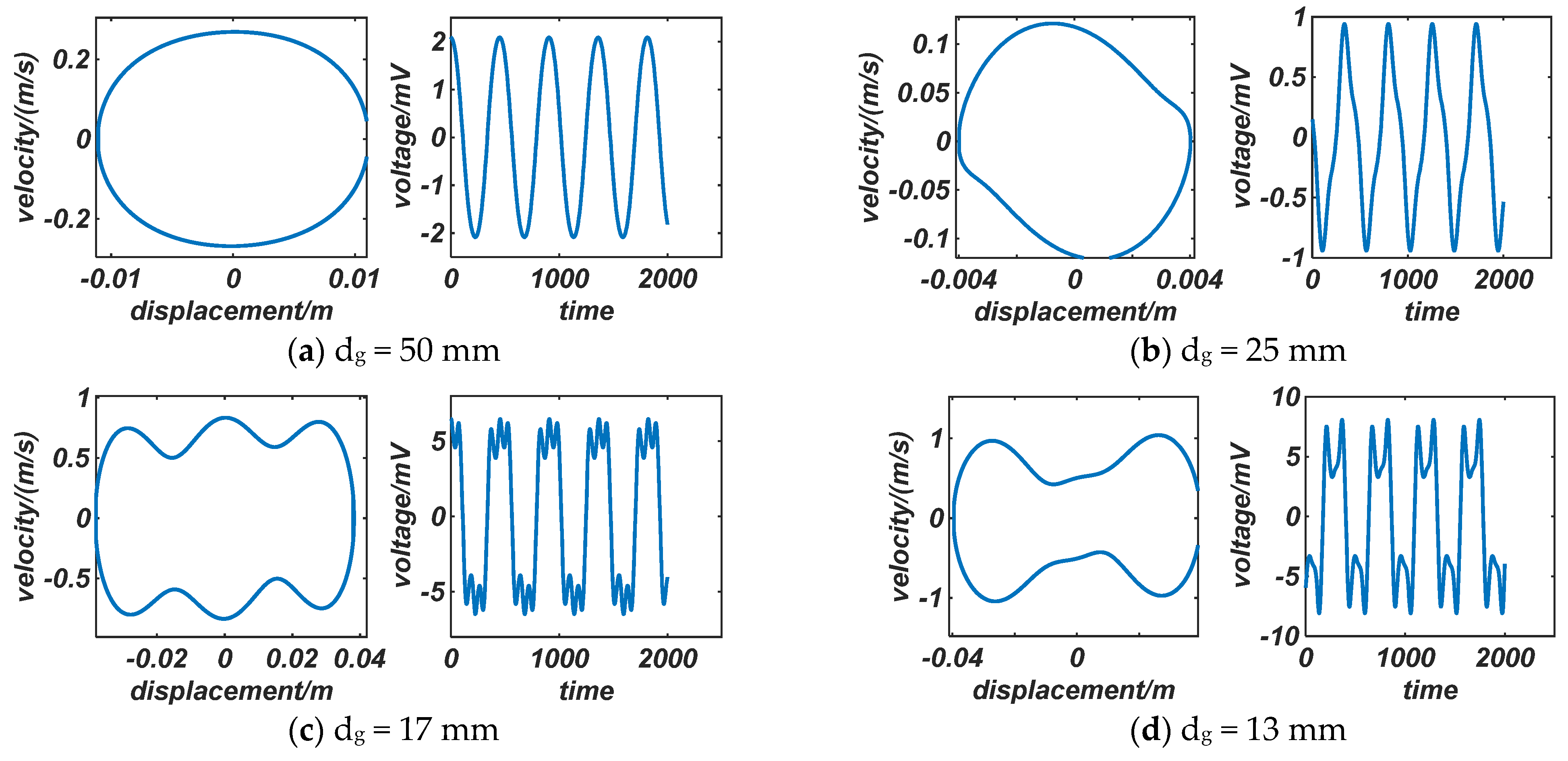
4.5. Influence of Magnet Spacing dg on System Dynamics
Through the analysis in
Section 4.4, when the horizontal distance between the magnets is d = 30 mm, the influence of the nonlinear magnetic force on the system is more obvious. In the case when the frequency f = 4 Hz, the excitation amplitude A = 2 g and d = 30 mm, the distance of the end magnet from the fixed magnet, d
g, is 13 mm, 17 mm and 50 mm, respectively, and the phase diagram and voltage output diagram of the energy harvester are shown in
Figure 7. The harvester performs monostable, tristable and bistable motion, respectively, as d
g decreases.
It can be seen from
Figure 7a that when d
g = 50 mm, because the distance between the end magnet and the fixed-end magnet is too large, the effect of the fixed-end magnet on the harvester is almost negligible, the harvester moves in a monostable state, the phase diagram is approximately circular, the system degenerates into a linear system and the output voltage of the harvester drops to 2 mV. When the distance of the fixed end magnet is reduced to 25 mm, the magnetic force of the end magnet increases, the movement of the harvester is irregular under the action of a nonlinear magnetic force and the output voltage of the harvester reaches 1 mV, which is 10 times higher than when d
g = 50 mm, as shown in
Figure 7b.
When the distance between the fixed-end magnets is reduced to d
g = 17 mm, the distance between the fixed-end magnets and the end magnets decreases, the nonlinear magnetic force increases, the constraint of the nonlinear magnetic force on the energy harvester becomes larger, the motion state of the harvester changes from the monostable state to the tristable state, the potential well between the stable points is deep, forming three stable equilibrium states and the detection voltage reaches 6 mV, as shown in
Figure 7c. When the distance between the fixed-end magnets is further reduced to d = 13 mm, the influence of the nonlinear magnetic force on the end magnets becomes more obvious, the tristable motion of the harvester degenerates into a bistable state, and the two fixed-end magnets are equivalent. For the magnet at the center of the fixed end, the essentially double magnetic force makes the potential well between the stable points deeper, and the acquisition voltage can reach 8 mV, as shown in
Figure 7d.
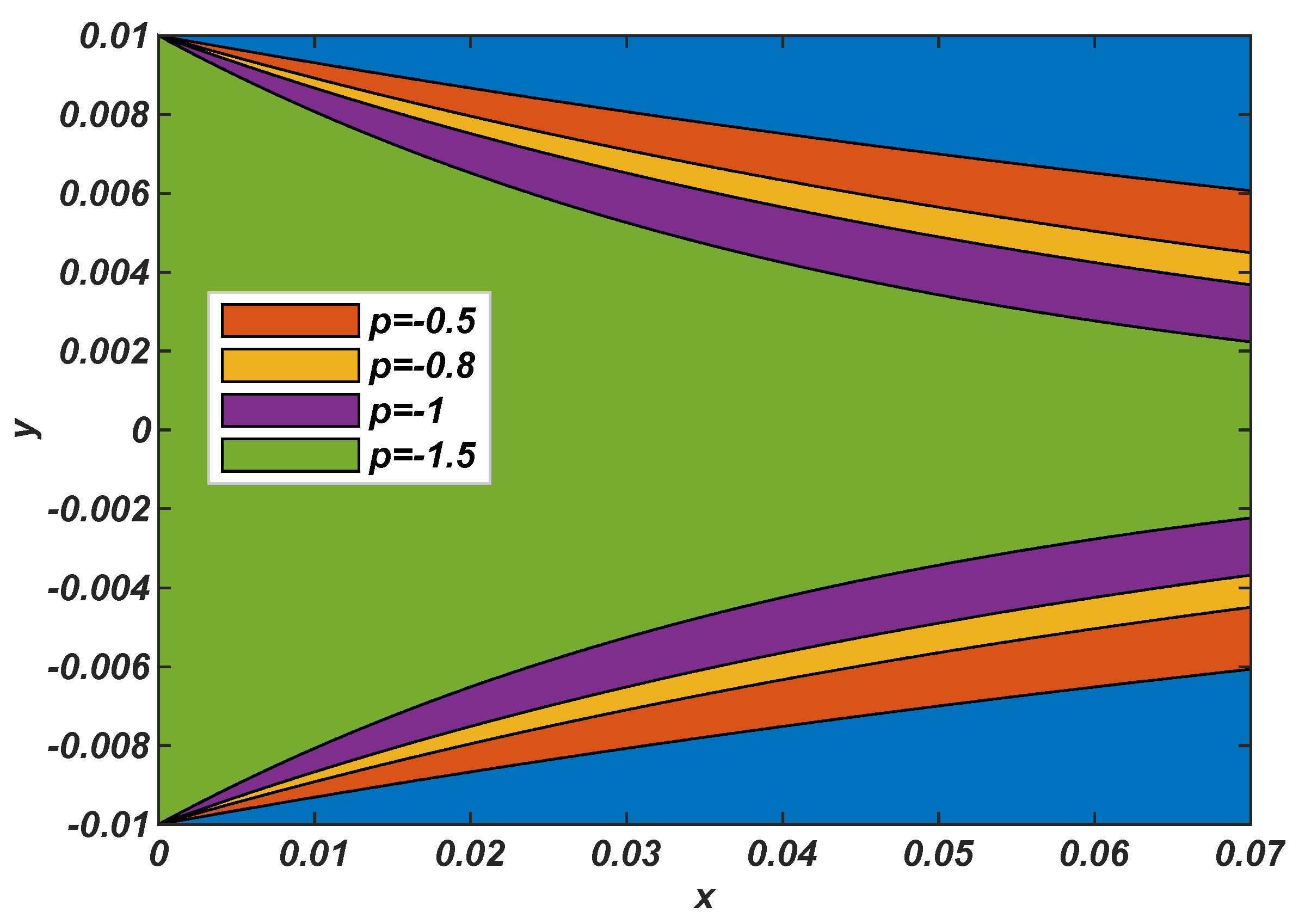
4.6. Influence of Exponential Decay Coefficient on System Dynamics
Studies have shown that narrowing the cantilever beam with a variable cross section can reduce the resonant frequency of the cantilever and improve the performance of the harvester [
30]. In this section, four exponential sections with
p = −0.5,
p = −0.8,
p = −1 and
p = −1.5 are selected, as shown in
Figure 8, and the first-order natural frequencies of the four-section cantilever beam structure are obtained as shown in
Table 1. It can be seen from the table that the natural frequency of the rectangular section beam is the largest. For the exponential section cantilever beam, as
p decreases, the natural frequency of the cantilever beam structure gradually decreases, and when the excitation frequency of the external environment reaches the natural frequency of the cantilever beam, the piezoelectric cantilever beam has the best energy-harvesting performance. Therefore, the energy-harvesting performance of the exponential cantilever is better at low frequencies.
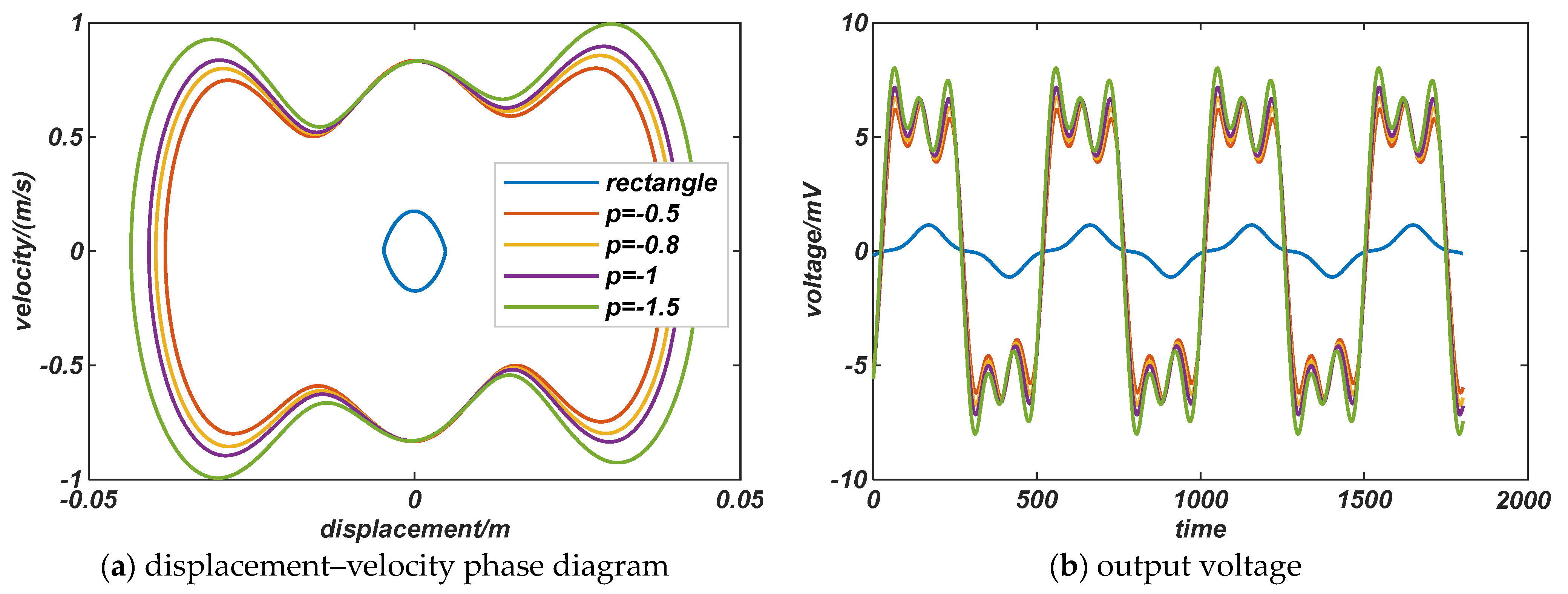
When the excitation frequency = 4 Hz, the excitation acceleration amplitude is A = 2 g, the horizontal distance between the magnets is d = 30 mm, and the distance between the magnets at the fixed end is d
g = 17 mm, the harvesters with five cross sections can be tristable. The phase diagram and voltage output diagram of the exponential decay section of the energy harvester are shown in
Figure 9.
According to
Figure 9, after the variable exponential section of the harvester reaches the tristable state; the motion amplitudes in the left and right steady states are much larger than that of the rectangular beam. As for the output voltage, the output voltages of the four kinds of exponential cantilever beams are all higher than those of the rectangular beams, and the output voltage of the exponential cantilever beams gradually increases with the decrease in the exponential decay coefficient due to the excessive concentration of the piezoelectric patches attached to the fixed end of the cantilever, and the exponentially changing section changes the strain distribution of the cantilever beam, making the stress distribution of the cantilever beam more uniform on the whole beam, and the PZT patches can better generate the piezoelectric effect and increase the output voltage.
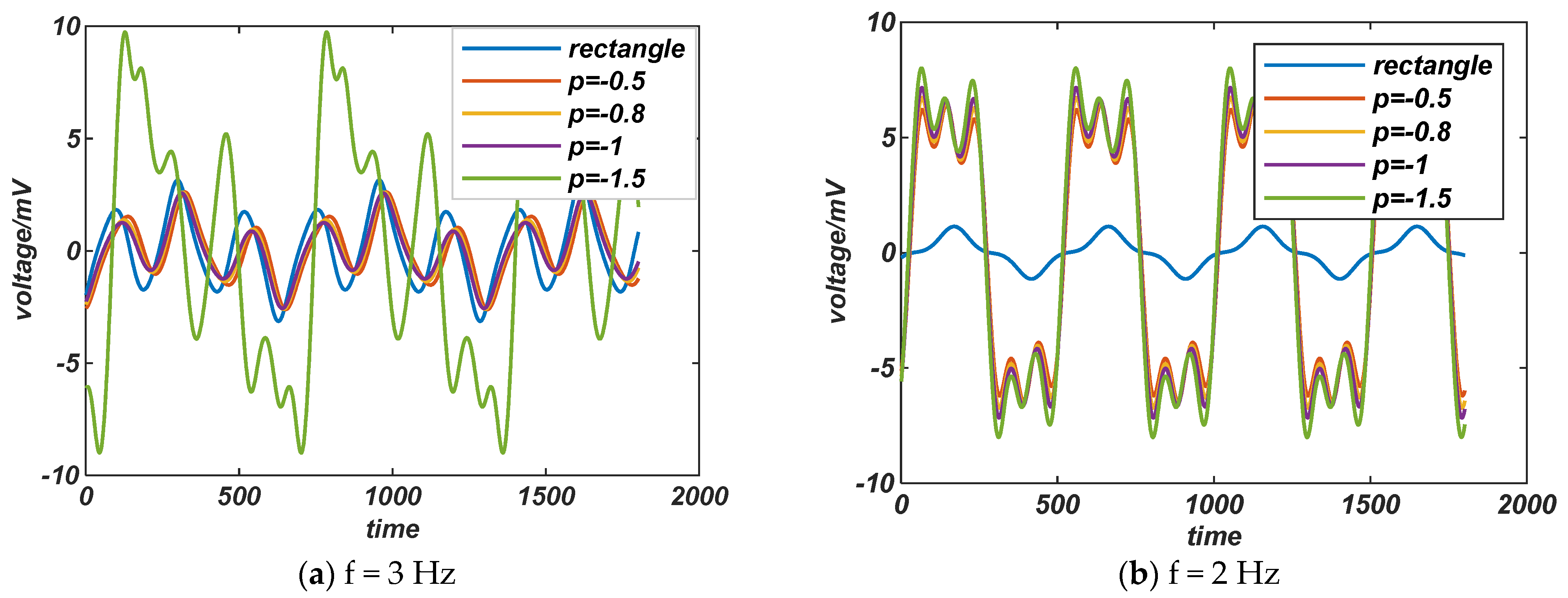
If the excitation frequency is reduced to f = 3 Hz and f = 2 Hz and other conditions are kept unchanged, the phase diagram and voltage output diagram of the energy harvester with an exponential decay cross section are shown in
Figure 10.
When f = 3 Hz, the cantilever beam with exponent coefficient p = −1.5 is first excited to tristable motion, and the output voltage remains the highest. This is related to its lowest natural frequency, and it is precisely because of its high energy harvesting efficiency at low frequencies that its use in harvesting voltage in the environment is greatly improved, and it has high research value. As the excitation frequency is reduced to f = 2 Hz, the rectangular-cross-section energy harvester moves in the well with low frequency and small amplitude, and the voltage of the energy harvester is much lower than that of the exponential-cross-section harvester. At the same time, the peak voltage of the exponential attenuation section of the harvester has the widest frequency band, which is three times that of the rectangular section of the cantilever beam.
In order to further study the performance of the exponentially changing cross section on the harvesting voltage and power of the piezoelectric cantilever, a power sweep analysis of five energy harvesters was carried out. According to the research in
Section 4.1,
Section 4.2,
Section 4.3,
Section 4.4 and
Section 4.5, the parameters d = 30 mm and d
g = 17 mm are selected, f is gradually increased from 0 Hz to 15 Hz and the average output power of each energy harvester at the current frequency is calculated at the same time.
In this paper, the time-domain voltage output of the voltage signal changing with time can be obtained through the ODE45 algorithm. The energy harvesting circuit is completely composed of a load resistor with R = 1 MΩ. The output of the energy harvester is completely consumed on the load resistor, and the instantaneous power can be obtained by multiplying the voltage and current values at each time step.
Since it is a purely resistive circuit, the time-domain output current can be expressed as:
where V(t) is a function of voltage over time and I(t) is a function of current over time.
Therefore, at each time t, the time-domain power, P(t), can be calculated as:
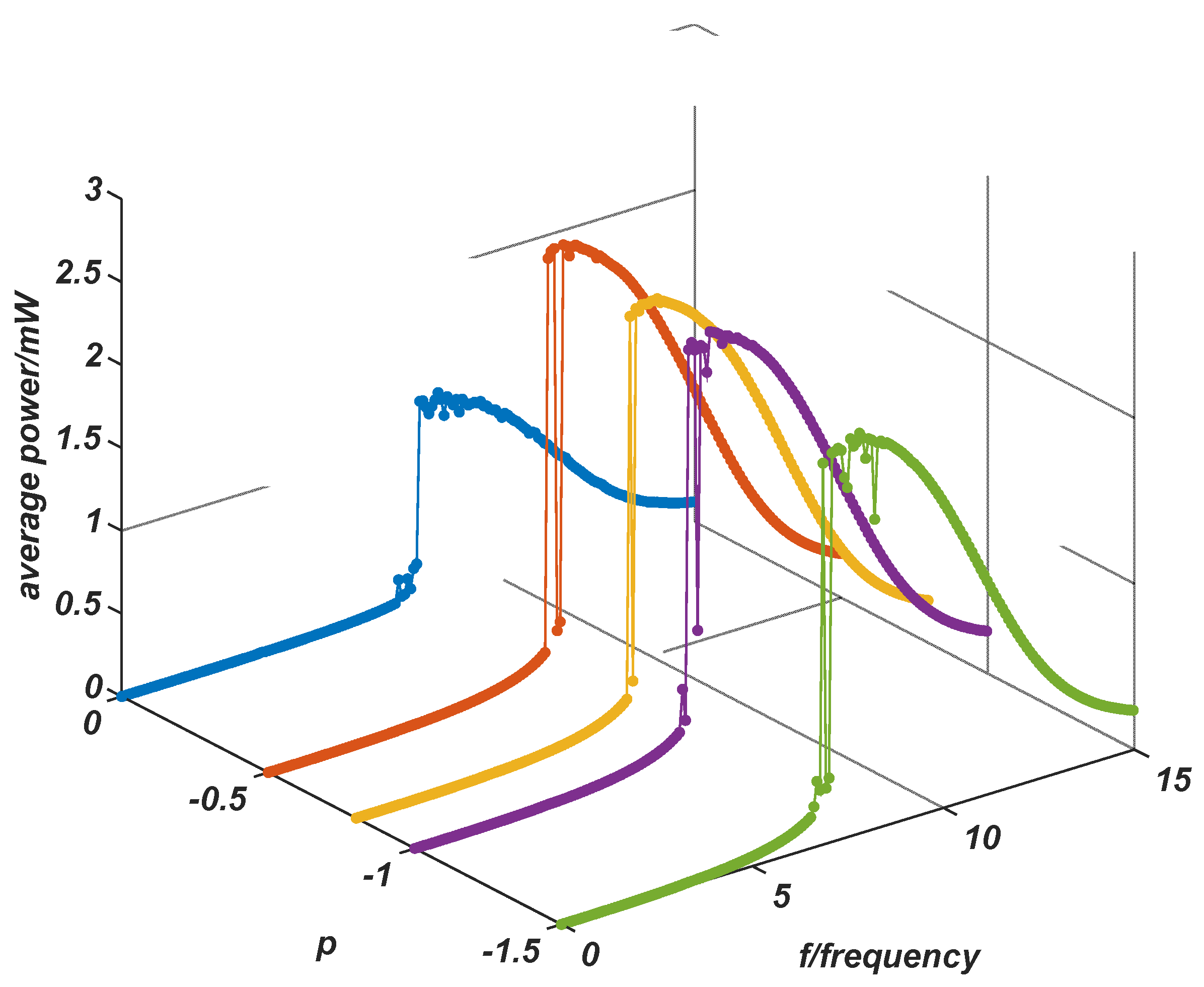
Figure 11 is the frequency–power sweep response graph of five energy harvesters.
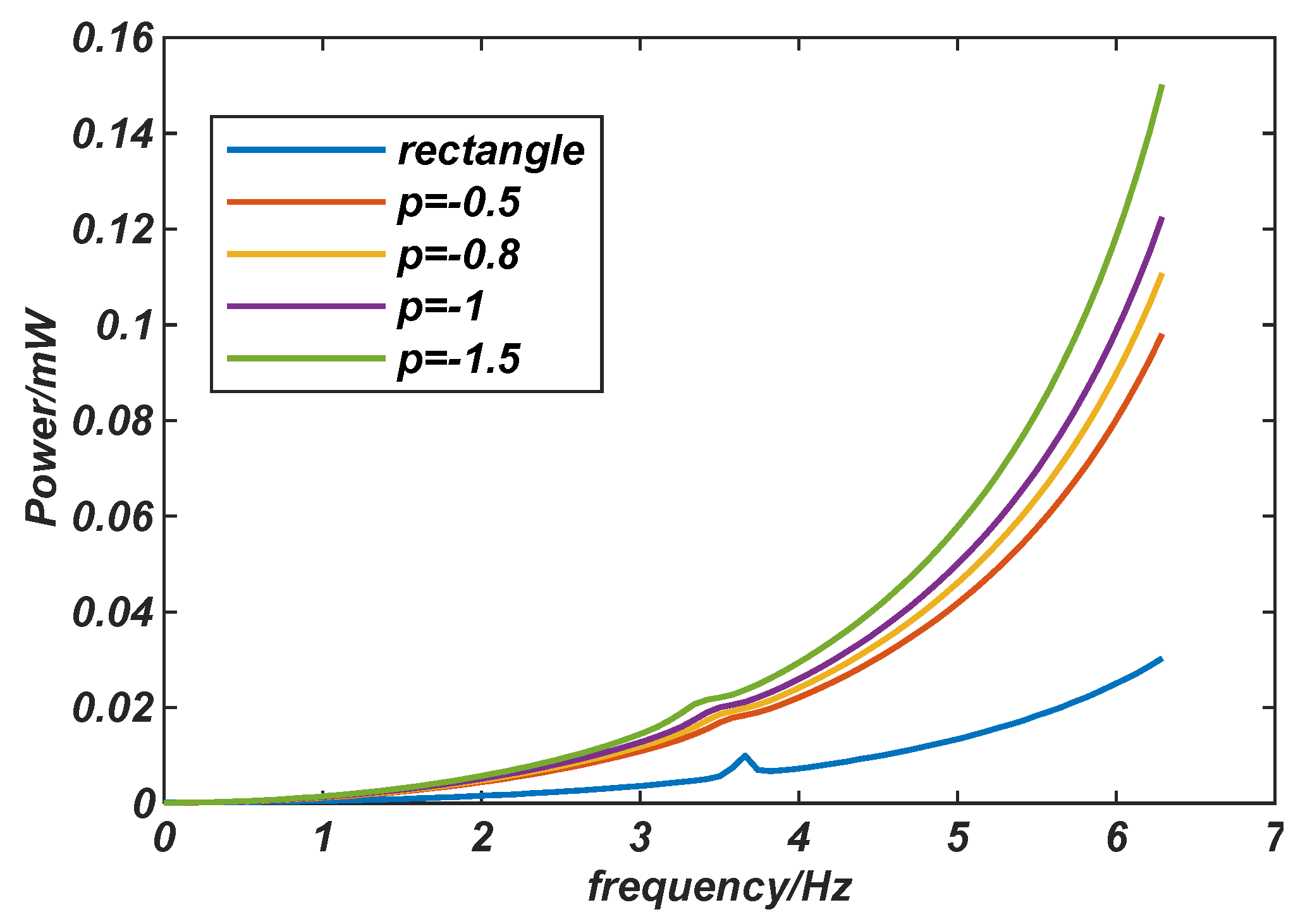
Figure 12 shows the frequency–power sweep response of five energy harvesters at low frequency.
It can be seen from
Figure 11 that the average power of the exponential cantilever is higher than that of the rectangular cantilever. In the mid-frequency band, each cantilever reaches the resonance frequency correspondingly, the power increases sharply, and the average power of the exponential cantilever is higher than that of the rectangular cantilever. In the high-frequency band, the power of the four exponential cantilever beams has the opposite relationship to the low-frequency band, which is related to its natural frequency, and the power gradually decreases as the decay coefficient becomes smaller.
It can be seen from
Figure 12 that the average power of the exponential cantilever is always higher than that of the rectangular cantilever in the low-frequency band, the average power gradually increases as the decay coefficient decreases and the power of the cantilever with the decay coefficient
p = −1.5 is always the highest.
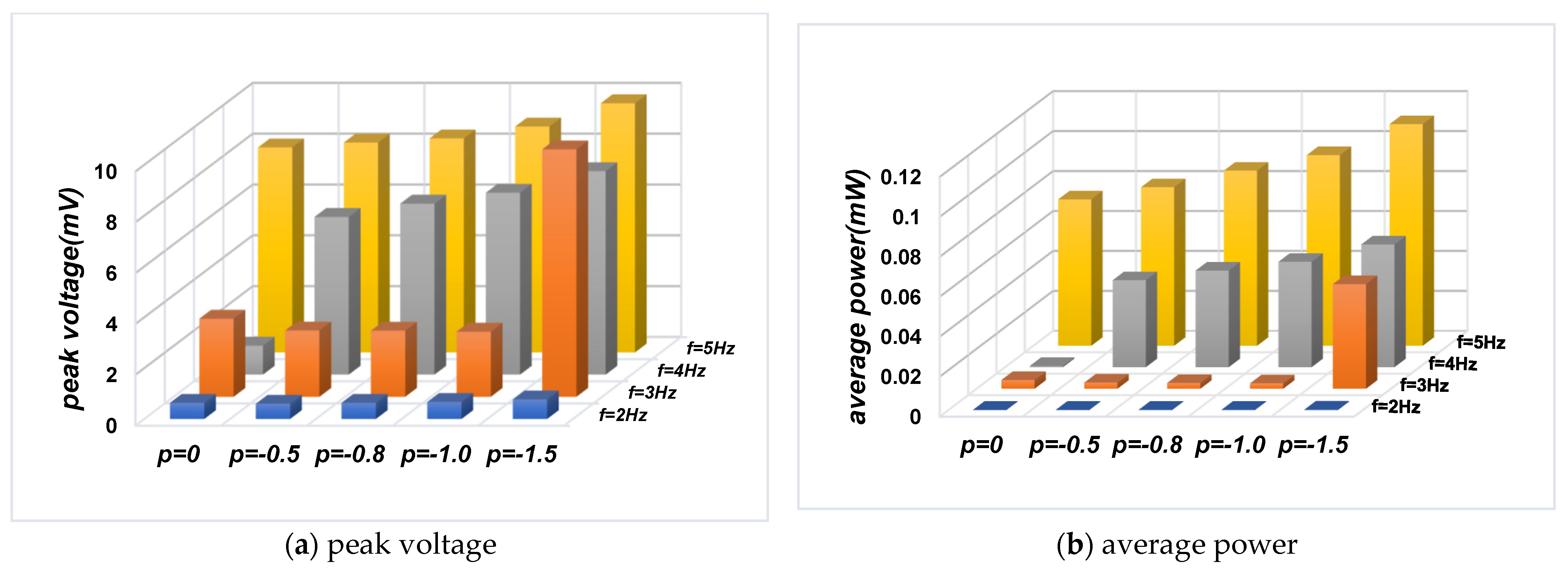
In order to better study the energy harvesting performance of exponentially attenuated cantilever beams under low-frequency excitation,
Table 2 shows the average power and peak voltage of five piezoelectric energy harvesters under several low-frequency excitations. It can be found from
Table 2 that under an f = 2, 3, 4, 5 Hz excitation, the peak voltage of the energy harvester at the exponential decay section increases by 1.51–88.24 times, and the average power increases by 1.22–7.03 times. This also verifies that the exponential decay section has better performance than the constant section of the cantilever beam.
In order to compare the performance of each energy harvester more directly, the data visualization in
Table 2 is shown in
Figure 13.
5. Discussion
This study shows that narrowing the cross section can improve the energy harvesting performance of piezoelectric energy harvesters and increase the energy output power. At the same time, this paper studies the influence of external influence and internal structure on the piezoelectric energy harvester. Research shows that for external influences, the acceleration amplitude and excitation frequency of the external excitation can change the motion amplitude of the energy harvester. By adjusting the excitation acceleration amplitude to A = 2–3.5 g and the excitation frequency to f = 4–7.5 Hz, a larger and more stable voltage output can be obtained when the harvester is in a state of large-amplitude motion.
For the internal structure of the harvester, the settings of parameters such as the horizontal magnet distance d and the fixed-end magnet distance dg directly determine the steady state of the harvester. When d = 30 mm and dg = 17 m are set, the piezoelectric vibration energy harvester is made to move in a tristable state, respectively, which can increase the frequency bandwidth and output power of the peak voltage.
The article further studies the output characteristics of the cantilever beam with different exponential decay coefficient sections and finds that the exponential decay section can optimize the stress distribution of the cantilever beam. Through the power sweep analysis, in the low-frequency band f = 0–7 Hz, the output power of the section with coefficient p = −1.5 is up to 7.03 times that of the rectangular section. In the mid-frequency band f = 7–12 Hz, the output power of each harvester can reach two times more than that of the rectangular cantilever. In the high-frequency band above f = 12 Hz, the energy harvesting power of the exponential cantilever is still higher than that of the rectangular cantilever.
Table 3 shows the energy harvesting data for other energy harvesters with different structures. Through comparison, it can be found that under low-frequency excitation (0–10 Hz), the tristable exponential section of the piezoelectric energy harvester proposed in this paper has greater advantages at the highest power density. However, this result can be influenced by a variety of factors, including the material of the cantilever and PZT and the amplitude parameter of the applied excitation. It is important to note that while increasing the applied force results in a higher voltage output, it can also lead to more microstructural cracks, which reduce the life expectancy of the energy harvester.
In practical applications, we have to make a trade-off between lifetime and power density. Even as we pursue higher power densities, we need to consider the challenge of crack generation, which can affect the long-term performance and reliability of the harvester. Therefore, when designing and optimizing the harvester, these factors need to be considered comprehensively to ensure the best performance and lifetime in specific application scenarios.
In general, the cantilever beam with an exponential decay cross section investigated in this paper has high research value in the field of low-frequency micromechanics. This paper carries a meaningful exploration on the determination of the optimal section parameters of the future piezoelectric cantilever beam under certain environmental excitations.