Impact of Manufacturing and Material Uncertainties in Performance of a Transverse Flux Machine for Aerospace

Abstract

3-dimensional (3D) flux machines accommodate many design and analysis challenges. Transverse flux machines (TFMs) are mostly useful for low speed, high torque applications but in this research a fault tolerant transverse flux alternator with segmented stator has been prototyped and several important design and manufacturing challenges have been thoroughly investigated as different scenarios. The proposed machine consists of axially separated, 4-single phase to achieve low speed power of 40 Watts at 400 revolutions/minute (rpm). In addition, the machine must achieve several high-speed specifications at 15,000 rpm. It is demonstrated that the proposed TFM could be an alternative to more conventional radial flux machines in aerospace as validated within the paper. However, the necessity of stator segmentation and the use of soft magnetic composites for the proposed 3D flux machine lead to many important design and optimization considerations. Therefore, this paper investigates the manufacturing and material uncertainties in a TFM for an aerospace application. The results indicate that transverse flux alternator might be an option as an aerospace alternator but the peak performance of the prototype machine is still far from the 3D models investigated using finite element electromagnetic simulations.

1. Introduction

Electrification in aerospace applications is becoming more important to reduce carbon emissions. Civil aerospace industry constantly makes an effort to increase the power density of electrical machines which is critical to achieve net-zero targets of many countries. Aerospace industry needs fault tolerant machines, drives and power electronics in order to satisfy the requirements of safety critical applications. Fault tolerant electrical machines are therefore crucial in different applications of civil aerospace. Permanent magnet (PM) machines are increasingly used in critical systems such as fuel pumps, actuator, landing gears, etc. [1]. In addition, fault tolerant, small volume aerospace alternators are required for engine electronics to ensure that flight–critical systems are provided with a high-reliability power generation system at all times [2].

Radial flux, multi-phase PM electrical machines are quite common in aerospace but the performance of alternative machine topologies compared to radial flux ones is still constituting a part of research in both academia and industry [2,3,4]. As an alternative, transverse flux machines (TFM) offer good fault-tolerant capability due to having high inductance which limits the fault current [5]. Their applications include vehicle traction, ship propulsion, railway and aerospace [6,7,8,9,10]. However, most of the proposed TFMs are for propulsion rather than power generation due to the fact that TFM inherently exhibits low power factor [11,12,13,14].

Low power factor could be viewed as an advantage when designing a TFM with fault tolerance capability, especially high-speed aerospace applications. The use of PM in transverse flux machines might be still a concern for safety-critical applications due to a fixed excitation of rotor field which cannot be shut down at any operating speed. In aerospace applications, phase/machine terminals shorting is a method to reduce short circuit (SC) fault current. This technique enables the shorted winding to share the magneto motive force (MMF) and so it reduces the fault current for reliability purposes [1]. Therefore, a multi-phase TFM with PM rotor along with their fault tolerance capability could be an alternative to more conventional radial flux machines in aerospace.

In the literature, there are many configurations of transverse flux machines as reported in [8,15]. In [16], the authors classify TFMs into several structures such as U-core and I-bridge TFM, C-core TFM, claw-pole TFM, quasi-U TFM and E-core TFMs, etc. TFMs with flux-concentrating PMs do not need magnetic shunts as they use of all magnets at the same time as described in [16]. Some proposed, different TFM configurations in the literature might not be a good candidate in safety-critical applications since some of the TFM configurations are comprised of many bespoke parts that might cause complications in manufacturing. For this reason, C-core TFM along with 3D flux magnetic materials could be the best option for an aerospace application due to their relatively easy to assemble feature for mass production and mechanical reliability as it is previously introduced in [4] and experience of build and testing is presented here.

In this paper, a fault tolerant PM TFM with soft magnetic composite (SMC) stator and flux-concentrating rotor poles is proposed for electrical power generation in civil aerospace as depicted in Figure 1. The proposed machine includes a lot of parts which can be seen as a disadvantage for mechanical rigidity yet elimination of laminated iron steels makes this topology attractive in terms of mass production. The performance and manufacturing challenges of the prototype machine have been presented thoroughly. This research mainly aims to investigate the manufacturing and material uncertainties in the prototype machine causing high speed loss and low speed power discrepancies in comparison to finite element analysis (FEA) of it under ideal material properties.

The paper is structured as follows: In Section 2, key machine properties are given along with optimization work. In Section 3, manufacturing and assembly of the proposed machine have been reported. In Section 4, uncertainties due to materials and manufacturing and their effects upon the machine performance are investigated along with important experimental results. Iron loss modelling for TFM SMC segments has been discussed in Section 5 and lastly Section 6 concludes the paper with a brief summary.

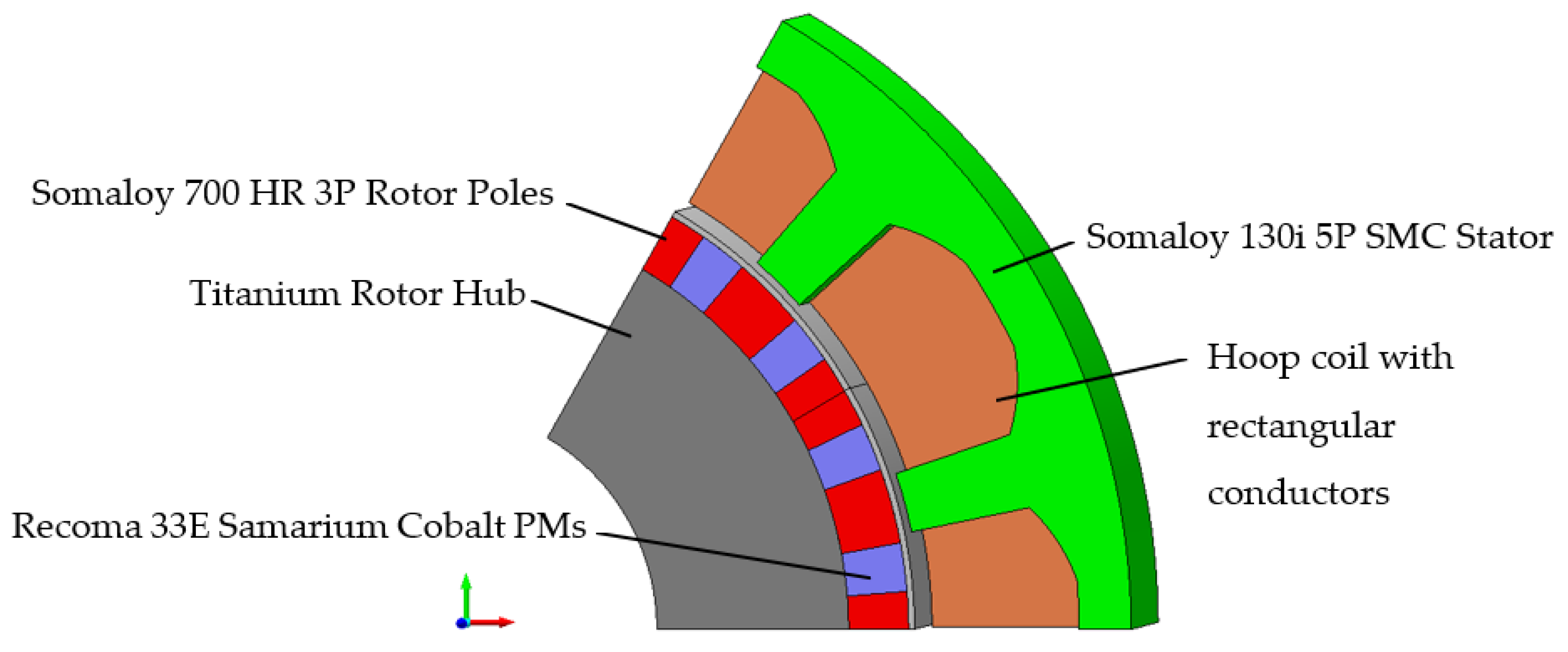
2. Proposed Machine Topology and Key Properties

In Section 2.1 the key features along with an optimization approach are given. This is important to understand the FE modelling details of the proposed TFM for aerospace.

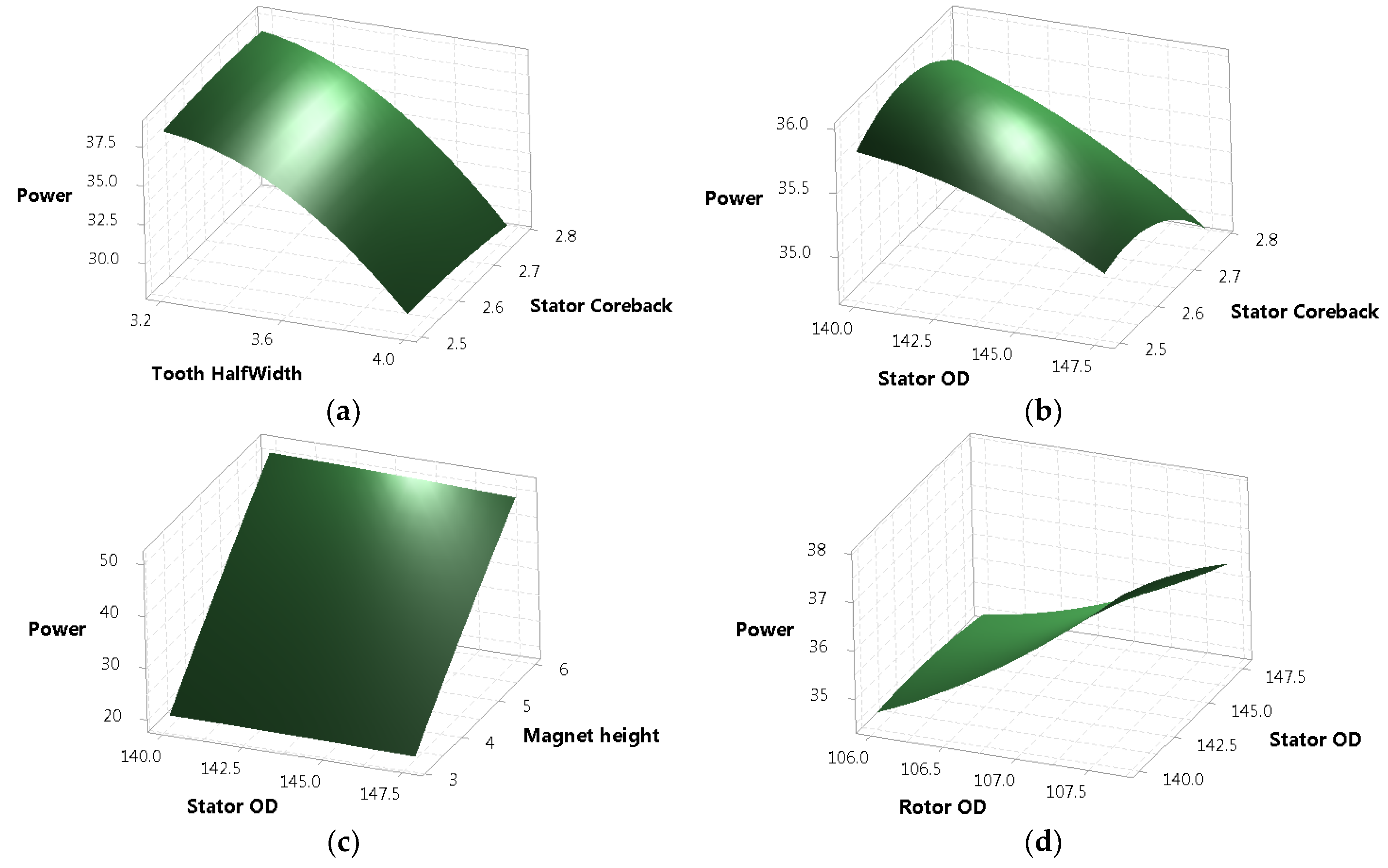
2.1. Finitie Element Analysis of TFM with GA Optimization

The proposed machine is 4-single phase, fault tolerant transverse flux alternator. Each phase is electrically 90° apart from each other.

Table 1. Key properties of the proposed transverse flux machine.

Number of phases	4-single phase (independent)
Number of pole pairs	12
Number of stator teeth	24
Back EMF at rated speed	≤265 V rms
Stator outer diameter	≤160 mm
Active stack length (4 axially positioned TFM phases)	≤100 mm
Short circuit current at 15,000 rpm—per phase	≤25 A rms
Power delivered from each sub-machine (single phase alternator) at 400 rpm	≥40 Watts
Power delivered from each sub-machine (single phase alternator) at 15,000 rpm with a DC link voltage of 55V after a passive rectifier	≥1200 Watts
Speed range	0–15,000 rpm

shows the key properties of the machine proposed in this study.

A pole pair of single phase TFM is depicted in Figure 2. The finite element (FE) model is parametric and the geometry has been optimized by using genetic algorithm (GA) with an objective function aiming to maximize the power at 400 rpm (i.e., low speed operation).

The constraints are peak open circuit voltage, peak short circuit current at rated speed and volume of the copper coil which limits the RMS current density per conductor. The GA optimization variables are a range for the dimensions of geometry and number of turns. The GA approach of the TFM in this study is previously published in [4]. Therefore, the optimization routine will not be thoroughly described here. However, response surface plots regarding the effect of important geometrical design variables on low speed power are shown in Figure 3.

It can be noted that the GA optimization is performed on a single phase TFM only rather than 4-phase model. This is because a GA optimization study would take a long time to converge a solution for a full 3D model with non-linear magnetic materials. Therefore, the 4-phase TFM further modified manually to meet the performance requirements given in Table 1.

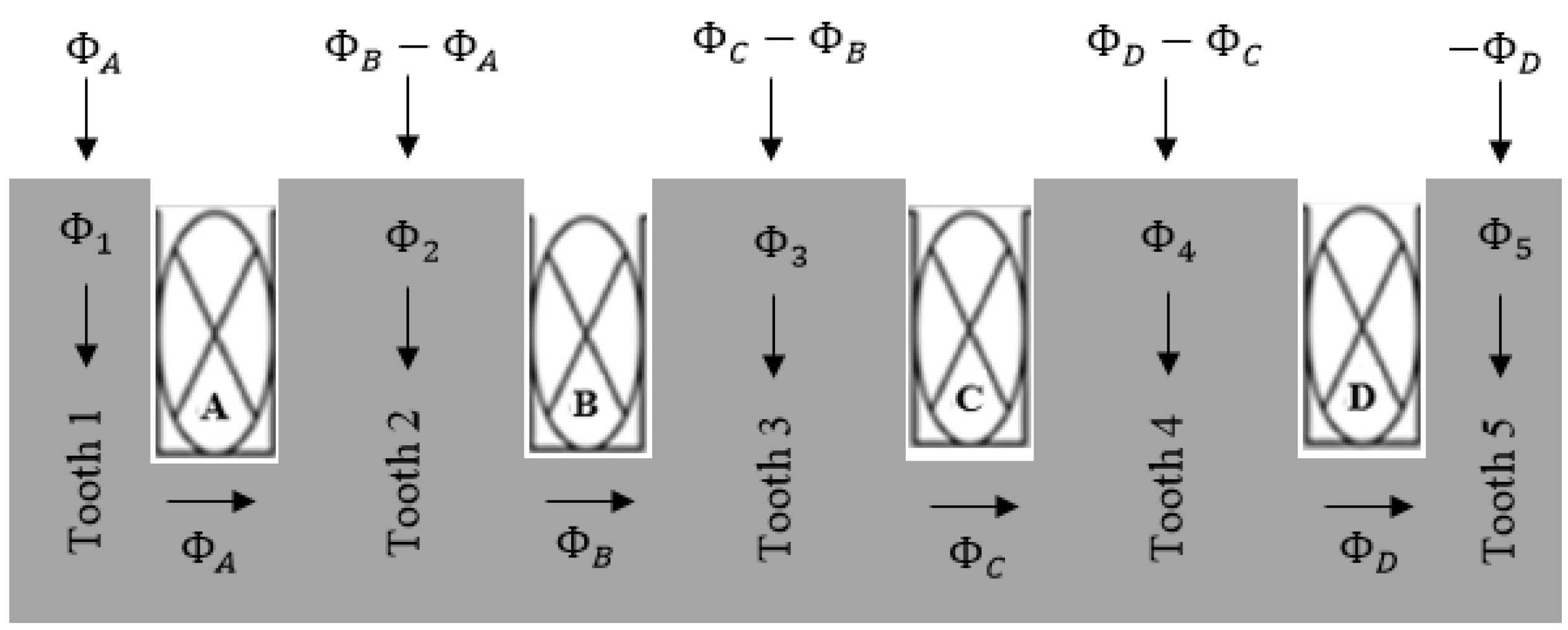
2.2. Four Single Phase Transverse Flux Machine

Axially stacked transverse flux machines with flux concentrating rotor poles can be designed under two categories as illustrated in Figure 4. In the first arrangement as shown in Figure 4a, the stator and rotor sections for each phases can be physically separated where mutual flux paths between the phases can be reduced significantly. This arrangement also supports the machine’s fault tolerance requirements. In the second arrangement as shown in Figure 4b, the stator teeth such as Tooth 1’ (i.e., opposite side of Tooth1) and Tooth 2 can be combined. In order to get a balanced four-phase machine, three central teeth in Figure 5, need to be re-dimensioned as the magnetic loading on the central teeth increases in the combined-phase machine due to shared flux paths. In a separate-phase TFM, the phases are shifted 90° electrically to reduce the coupling between the phases as much as possible. This is in fact similar to a balanced three-phase machine where the phase windings are designed 120° electrically apart from each other. However, in a combined-phase machine topology, a balanced four-phase machine can be obtained after establishing a vector diagram in which the flux, $\mathsf{\Phi }$ in each of the five teeth can be found by superposition [17,18]. This is given in Equation (1):

$${\Phi }_1={\Phi }_A$$

(1a)

$${\Phi }_2={\Phi }_B-{\Phi }_A$$

(1b)

$${\Phi }_3={\Phi }_C-{\Phi }_B$$

(1c)

$${\Phi }_4={\Phi }_D-{\Phi }_C$$

(1d)

$${\Phi }_5=-{\Phi }_D$$

(1e)

where Φ_A = |Φ| < 0°, Φ_B = |Φ| < 90°, Φ_C = |Φ| < 180°, Φ_D = |Φ| < 270°.

By setting up a vector diagram and using Equation (1), the magnitude and phase of the flux in each of the five teeth can be calculated. The results are given in

Table 2. Teeth flux linkages (magnitude and phase) for a 4-phase, combined-phase TFM.

Tooth #	Flux Linkage	Magnitude (Per-Unit)	Phase (degE)
1	${\mathsf{\Phi }}_1$	1 pu	<0°
2	${\mathsf{\Phi }}_2$	$\sqrt{2}$ pu	<135°
3	${\mathsf{\Phi }}_3$	$\sqrt{2}$ pu	<225°
4	${\mathsf{\Phi }}_4$	$\sqrt{2}$ pu	<315°
5	${\mathsf{\Phi }}_5$	1 pu	<90°

.

After obtaining magnitudes and phases of the flux linkages for the combined-phase TFM, the central teeth are re-dimensioned to maintain a similar flux flow through these teeth, as depicted in Figure 6. The mechanical shifts (°m) between the stator stacks are also given in Figure 6 that are calculated by setting up a relationship between electrical and mechanical phase angles.

In Figure 6, it is clear that combined phase gives higher cogging torque and phase back EMFs are smoother in separate phase TFM. Total harmonic distortion (THD) of phase back EMF in separate phase TFM is 7.57% (mid-phase), while the THD for the combined phase TFM is 12.1% (mid-phase). The difference between 3rd, 5th and 7th harmonics can be seen in Figure 6e. Combining the magnetic circuits in Figure 6a might not be a good option in fault tolerant applications since mutual flux linkage between adjacent phases will reduce the fault tolerance during a SC fault. Moreover, vibration and acoustic noise might be a problem in combined phase TFM which is not desired for an aerospace application. For this reason, separate phase TFM is considered and has been prototyped after some essential mechanical modifications on the final FE model. This is described in Section 2.3.

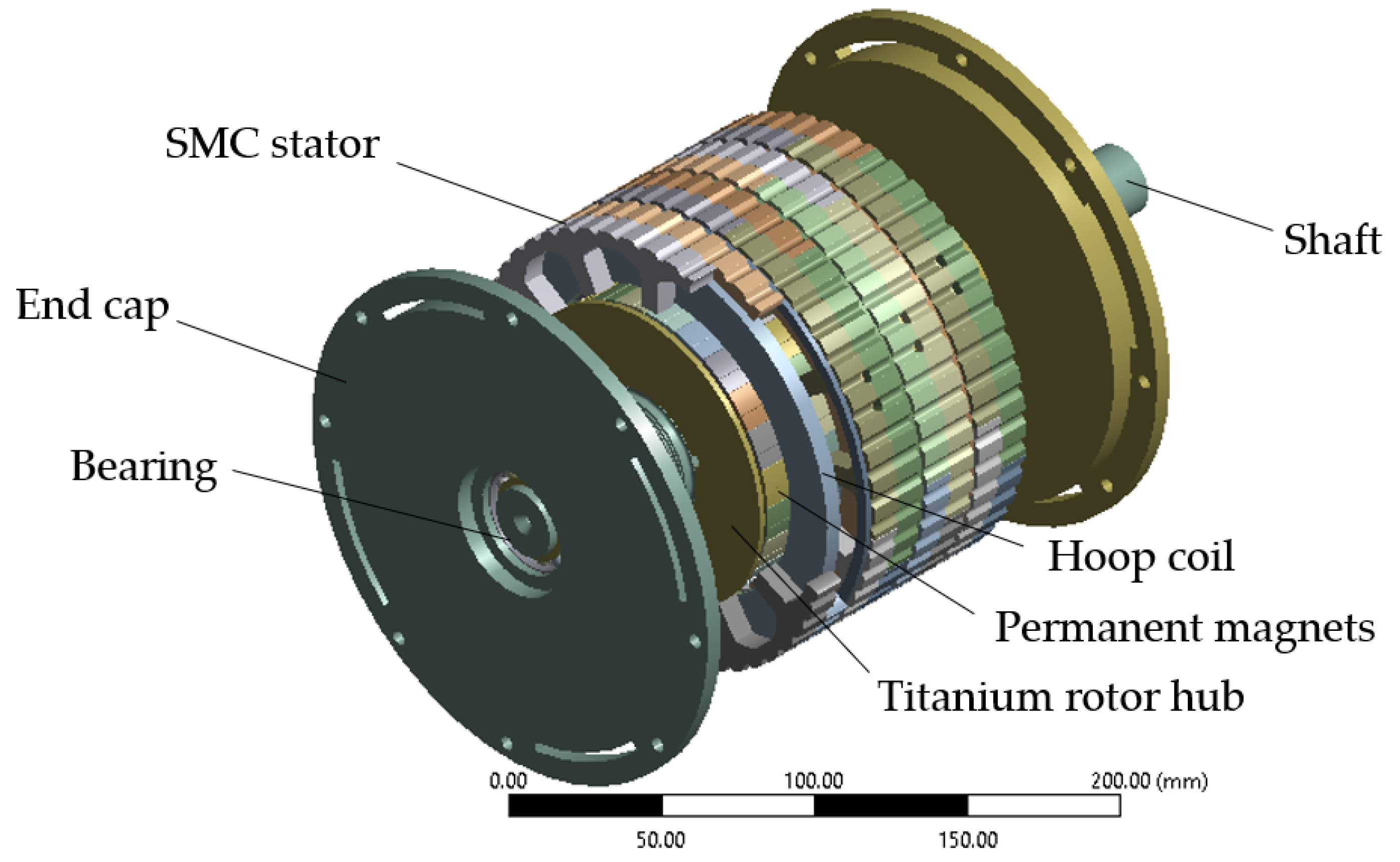
2.3. Final TFM Geometry with Essential Mechanical Considerations

After electromagnetic performance optimization, the machine geometry has been slightly altered to be able to build the TFM stator with SMC segments. Since the machine is intended to be built with SMC segments, there are several mechanical considerations need to be taken into account as compressing of SMC segments at 800 MPa requires some mechanical modifications. The authors have previously described these in [4]. The final stator consists of 32 SMC segments where 8 of them come together to form a phase of the machine. Similarly, the TFM rotor has 96 magnets and flux concentrating SMC rotor poles where 12 pole pairs form the machine’s electrical frequency reaching 3 kHz at rated speed.

In order to achieve smoother and balanced power output from the machine phases, several design techniques have been considered. The authors have noticed that if the number of turns is chosen to be the same for all axially distributed phases in 3D FE simulations, the RMS value of short circuit currents is different which is not desired for a fault tolerant machine design. Additionally, due to unbalanced synchronous reactance of the TFM phases, instantaneous power output of the machine will have ripples. Therefore, the constructed machine includes 26 turns for end (i.e., side) phases and 20 turns for middle phases to get balanced short circuit current. This was previously discussed in [4] in detail. The final 3D FE model is given in Figure 7.

3. Construction of a Fault Tolerant Transverse Flux Machine

In this section, prototyping of a fault tolerant transverse flux machine has been presented along with its mechanical challenges. Potential material-based problems have been highlighted.

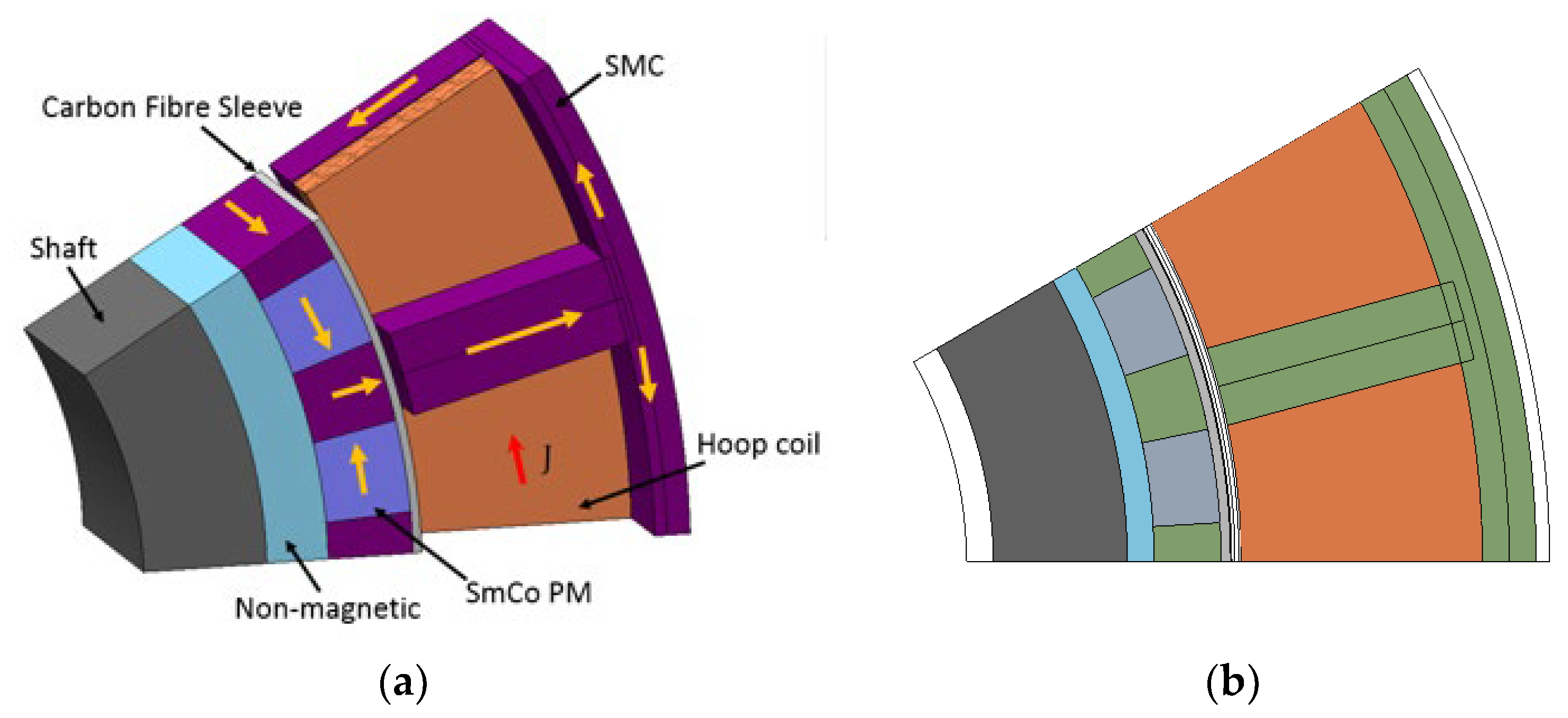
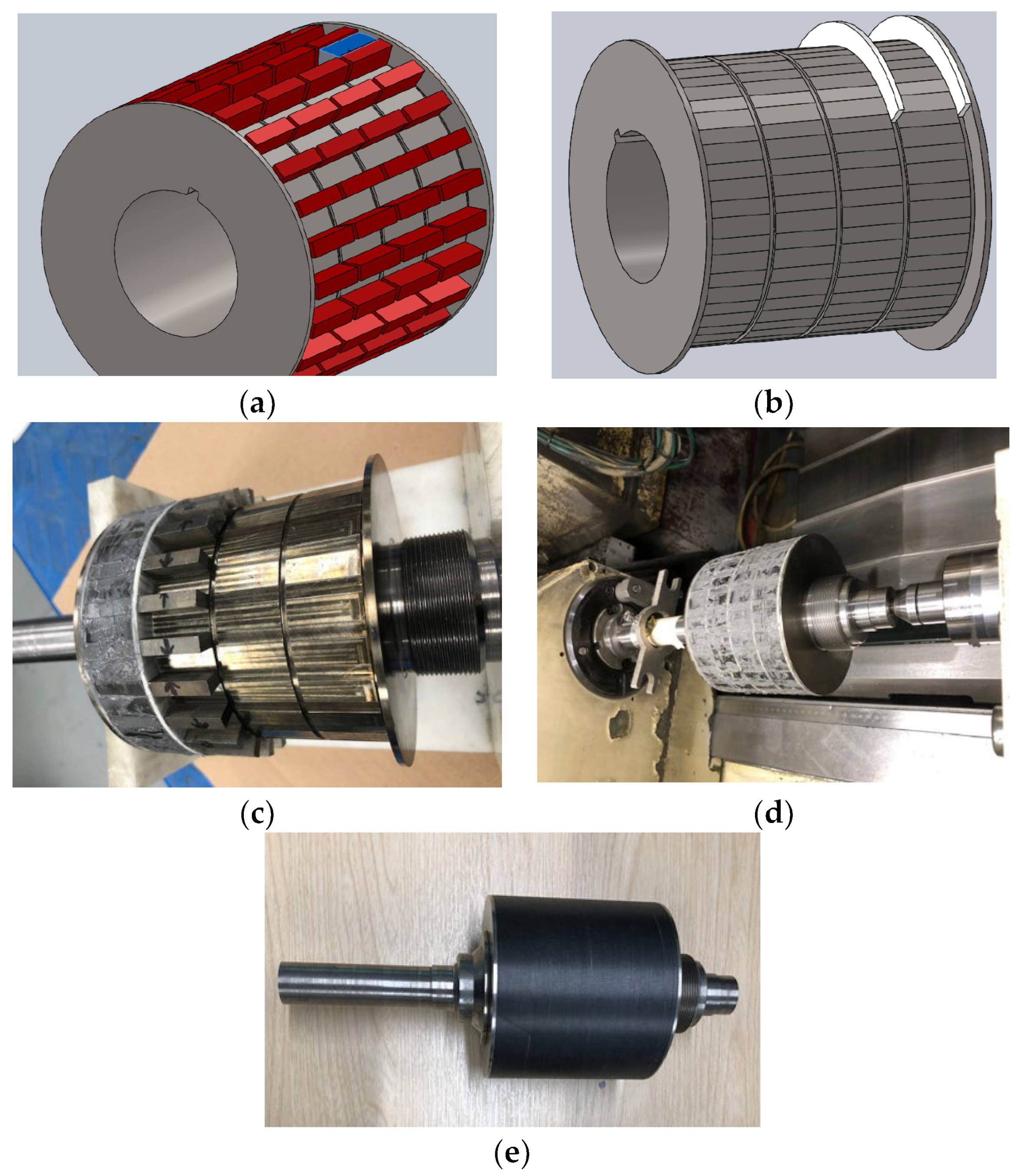
3.1. TFM Rotor Construction

The flux concentrating SMC rotor poles in Figure 8a require modification to make them suitable for pressing. To avoid any sharp edges 0.3 mm radii were added to all the corners. SMC rotor poles and permanent magnets (Samarium Cobalt, Recoma 33E) have flat faces enabling them to be precisely located on the rotor’s polygonal outer perimeter as shown in Figure 8b. We have chosen ceramic spacers with a thickness of 2 mm between the rotor sections as it is durable at high speeds in terms of thermal and mechanical aspects. The spacers are a non-magnetic material and of a material with a very high electrical resistivity to reduce induced eddy currents on the spacers and permanent magnets.

In Figure 8, the potential manufacturing related performance problems of the prototype machine are as follows:

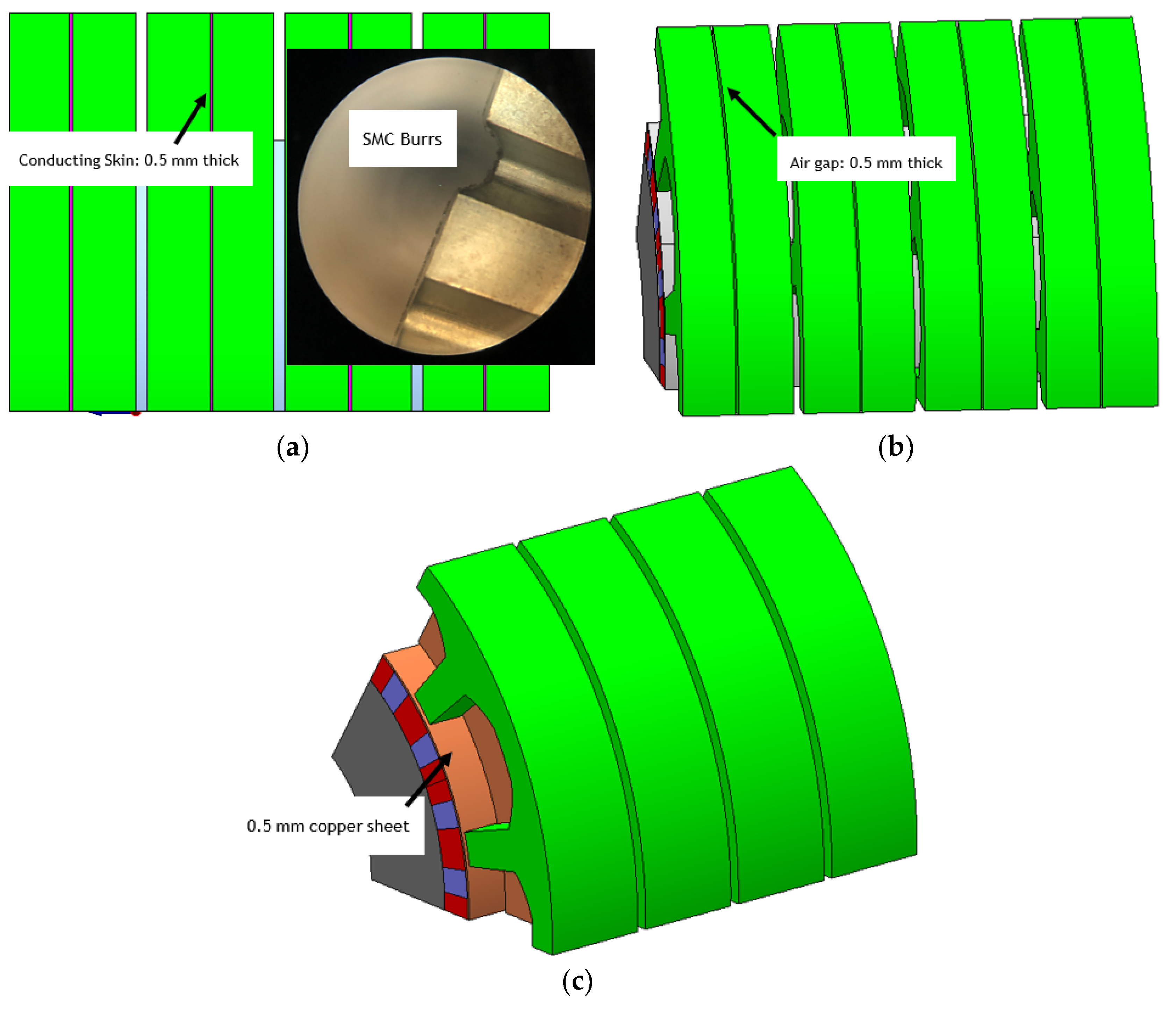
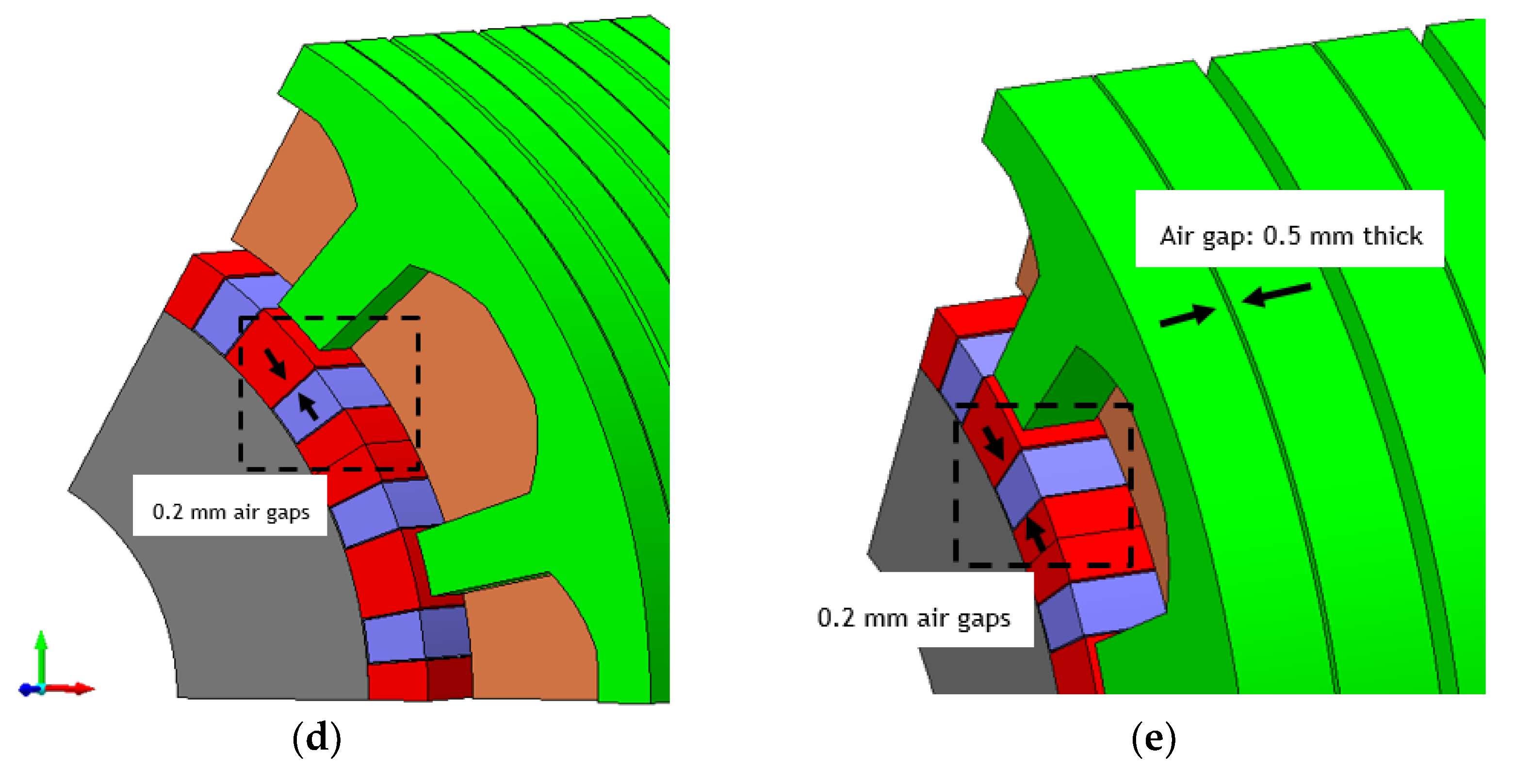
• Flux concentrating SMC rotor poles might not be perfectly in contact with Recoma 33E permanent magnets. This might introduce air regions in the magnetic circuit, between the magnets and SMC poles.
• Necessary mechanical grinding, as shown in Figure 8d, of rotor PMs and SMC poles, before the rotor is wrapped with a carbon fiber sleeve might cause a conducting skin on SMC components. This will end up with performance drop due to the fact that longer eddy current paths will be formed in the rotor.
• Although titanium material as a rotor body is a good choice due its non-magnetic properties, electrical conductivity of rotor material is a still concern as the machine at high speeds will induce eddy currents in the rotor body that will drop the phase back EMF. It should be noted that titanium rotor hub is ideally not a part of the machine’s magnetic circuit.

The potential manufacturing related problems due to rotor manufacture have been simulated in 3D FE simulations and the results are given in Section 4.2.

3.2. TFM Stator Construction

In Figure 9, the stator construction is depicted. Eight SMC segments come together to form a phase. Therefore, a perfect annular assembly for the stator is necessary. This is achieved by using a jig shown in Figure 9a. In addition, Figure 9b indicates some diameter checks after assembly whether the stator assembly diverges from an annulus to an ellipse. This is crucial since an elliptical stator phase cannot be perfectly fit with an aluminum housing. Furthermore, Figure 9c shows the alignment of gear-like outer edges of the stator phases. The features around the SMC stator segments in Figure 10a are required to provide electrical phase displacement in four-phase complete TFM. In terms of magnetics, the features on SMC stator segments are not significant and therefore 3D FE simulations do not include those notches around the stator. Figure 9d demonstrates a successful assembly of SMC stator phases with the housing. L-shape push plates have been finally placed both sides of the stator for axial alignment between rotor and stator as given in Figure 10b. The final stator phases are separated by 2 mm-thick glass fiber (Tufnol^® by Ensinger, UK) spacers. Otherwise, the separated-phase fault tolerant stator configuration cannot be accomplished for the proposed TFM.

Many components are involved in the final stator assembly and the mechanical issues might affect the final electromagnetic and thermal performance. They are listed as below:

• As shown in Figure 10a, compressing of SMC components leave burrs on the component surfaces. These need to be removed to ensure that SMC segments are magnetically in good contact with each other. The removal of burrs known as de-burring might cause conducting skin on stator segments that is certainly not desired due to the fact that eddy currents will be easily induced in conducting SMC iron particles (electrically conducting) at high speed operation.
• It has been noted that some of the stator assemblies are not perfectly annular and they are slightly elliptical (Figure 9b). The diametrical divergence from a perfect ring to an ellipse is about 1.27 mm. This is an unavoidable manufacturing problem due to stator segmentation.
• The stator segments are glued to each other that might weaken the magnetic flux in the machine’s magnetic circuit.
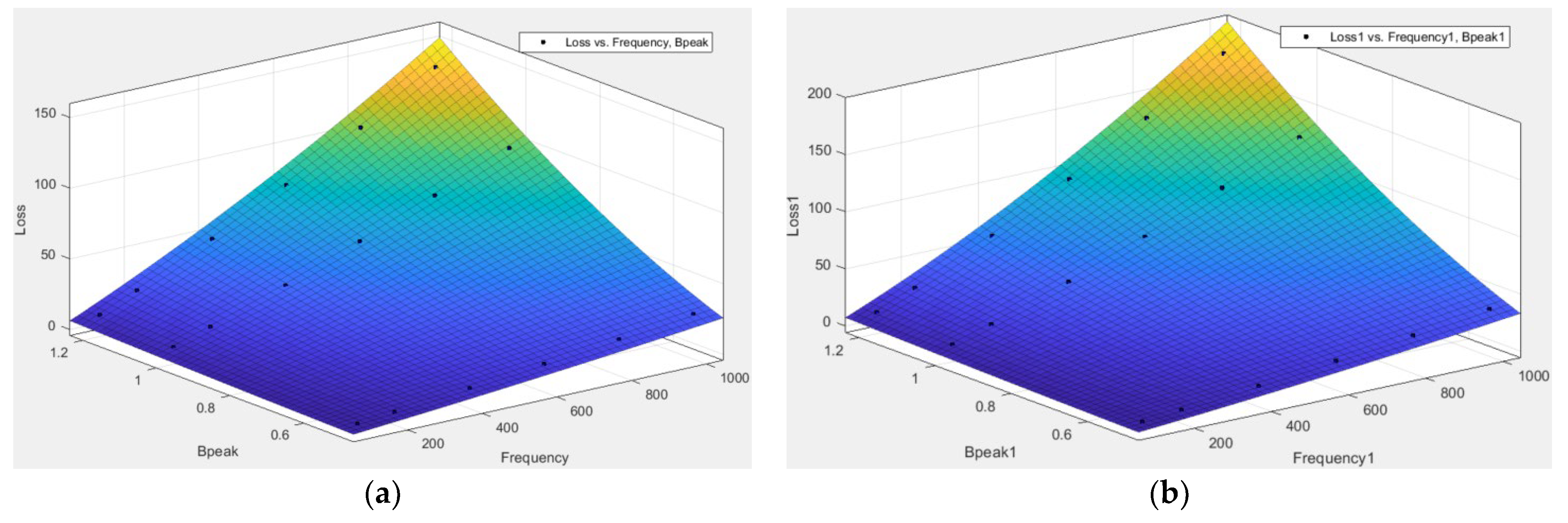
• SMC materials are unique in terms of their 3D flux carrying capability. However, they might under-perform as their iron loss characteristic is much more complicated than traditional laminated steels. This is further discussed in Section 5.

3D flux machines such as transverse flux machines therefore need more investigation in terms of their performance versus manufacturing complexity before they can be mass produced. The effects of potential manufacturing/assembly-based problems on the prototype machine performance have been demonstrated later in Section 4 with some quantitative results.

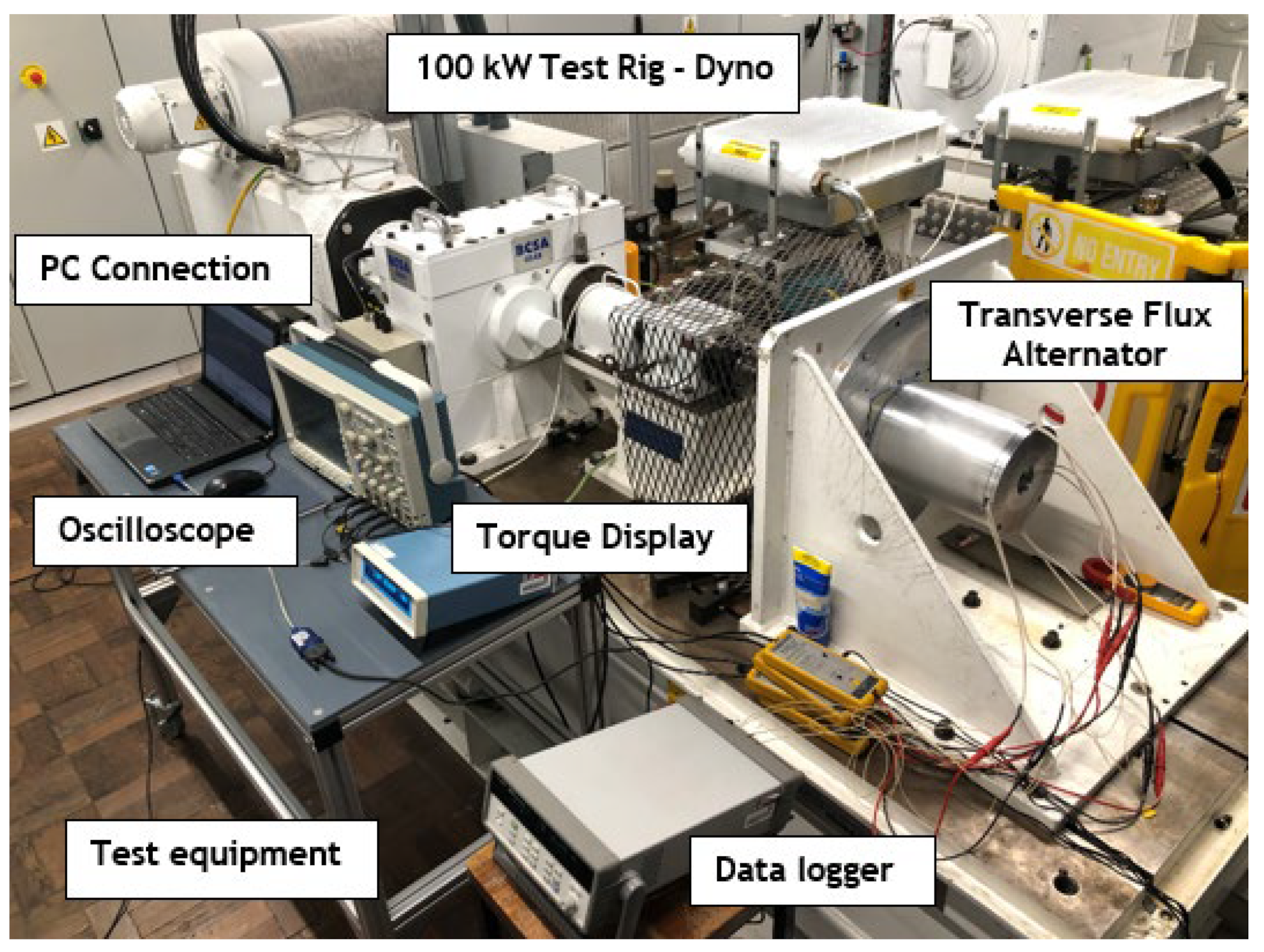
4. Experimental Work

As part of experimental work, the prototype machine’s performance results have been obtained by conducting open circuit and short circuit electrical tests by using a 100 kW drive motor rated up to 30,000 rpm. Low speed power capability was measured and also some loss results have been acquired by means of a precise torque sensor.

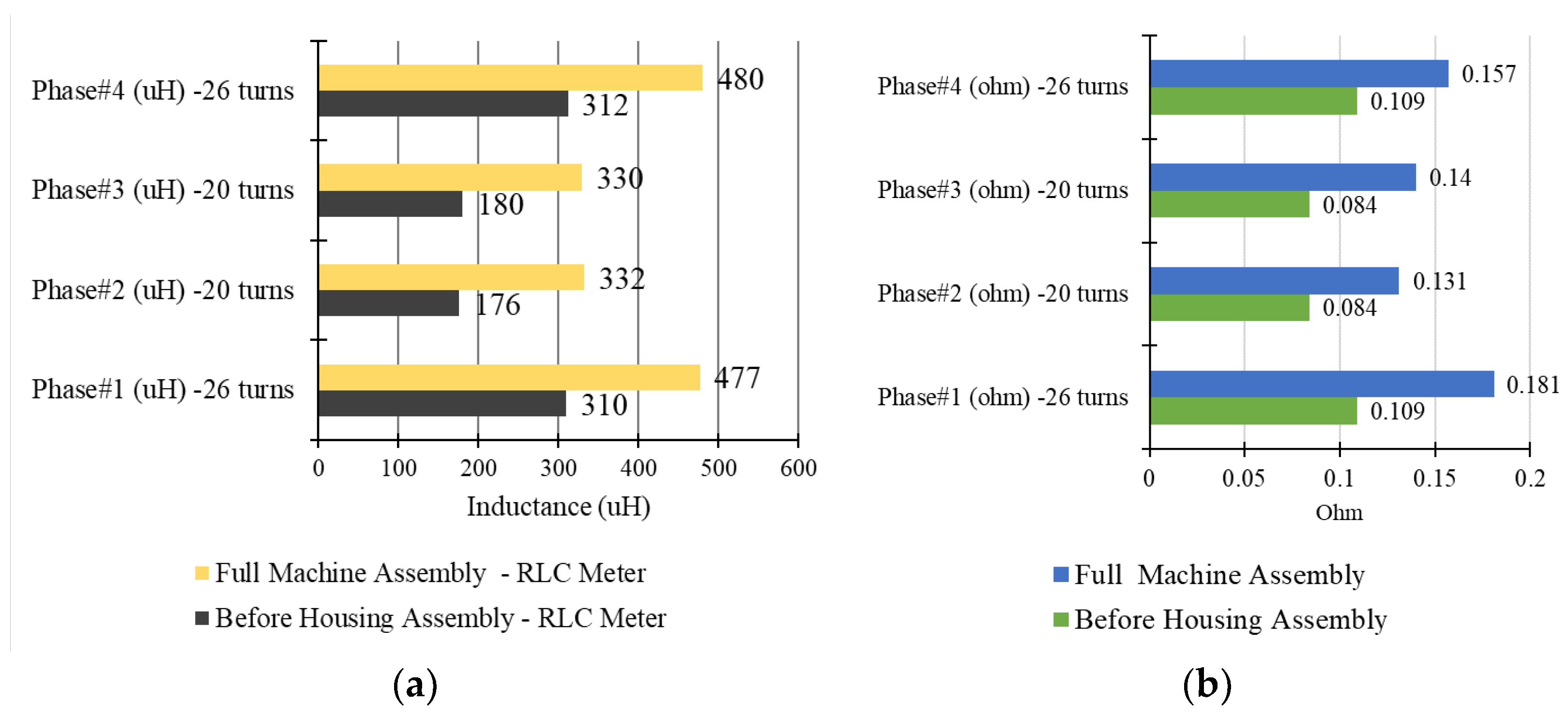
4.1. Test Setup and Open/Short Circuit Electrical Test Results

In Figure 11, the experimental test setup is shown. The prototype TFM is mounted on a test rig. The experimental work mainly aims to prove mechanical resilience, validate back EMFs and short circuit currents and confirm low speed power output of the machine. Moreover, open circuit loss results have been acquired at varying speeds.

4.1.1. Open Circuit Tests

Open circuit tests have been conducted at different speeds. Figure 12a shows how back EMFs vary with the speed of the machine. The results indicate that back EMFs are directly proportional with the speed and 26-turn coils achieve higher back EMF compared to 20-turn coils as expected. The linearity proves that 3D flux materials (Somaloy 130i 5P and Somaloy 700 HR 3P by Höganas AB, Sweden) provide smooth flux linkage for the coils. However, comparison between 3D FE simulations and measurements in Figure 12b demonstrates that the prototype machine is affected by a number of manufacture and material related uncertainties. Open circuit test results give about 23.7% lower back EMFs in average in comparison to 3D FE simulations.

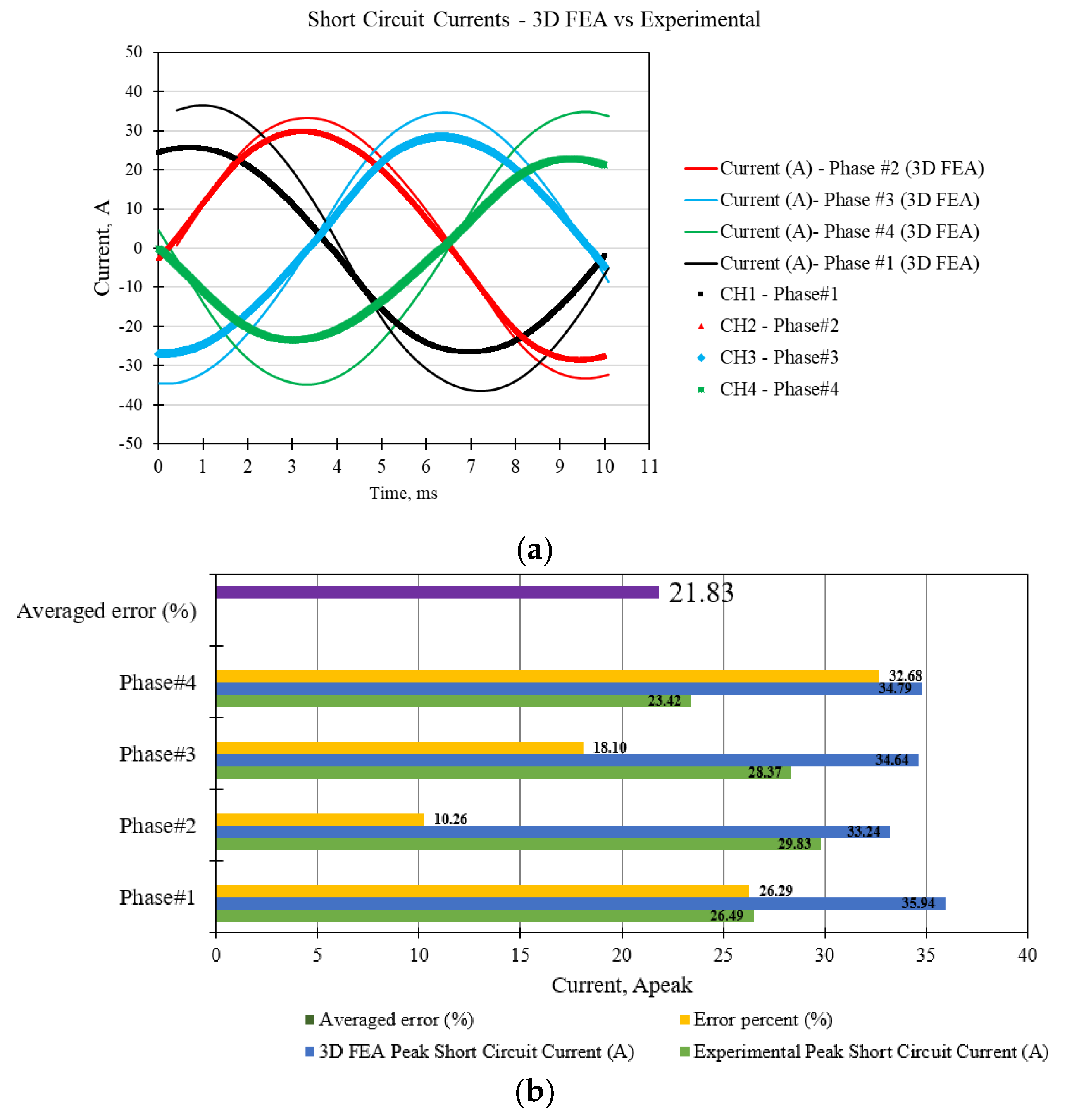
4.1.2. Short Circuit Tests

Figure 13a shows short circuit currents at 400 rpm, which is the low speed power operating point for the machine under investigation. Due to the relationship between back EMF, $V_{oc}$ and SC current, $I_{sc}$ (i.e., $X_s=V_{oc}/I_{sc}$) by using the machine’s synchronous reactance, it is expected that short circuit currents will also be affected by the drop in back EMFs. On average, short circuit currents are 21.8% lower than those in the simulations as shown in Figure 13b.

In Figure 13a, 3D FEA short circuit currents are balanced since a balancing method of different number of turns for middle and end phases has been implemented. In other words, the middle phases utilize 20 turns, whereas end (i.e., side) phases of TFM utilize 26 turns. The principal goal to use different number of turns to obtain similar RMS short circuit currents for the design. In TFMs with more than three phases as in this study, short circuit currents differ based upon the axial position of the coil. Middle phases experience higher flux linkages then side phases. Magnetic isolation between axially distributed phases is not possible. Therefore, number of turns are varied to obtain more balanced fault tolerant TFM.

However, the prototype machine experiences a very limited mutual flux from the side phases as opposed to given in Figure 14a and therefore, short circuit currents are not balanced as demonstrated in Figure 14b in the experiments. There might be several reasons for that as given below:

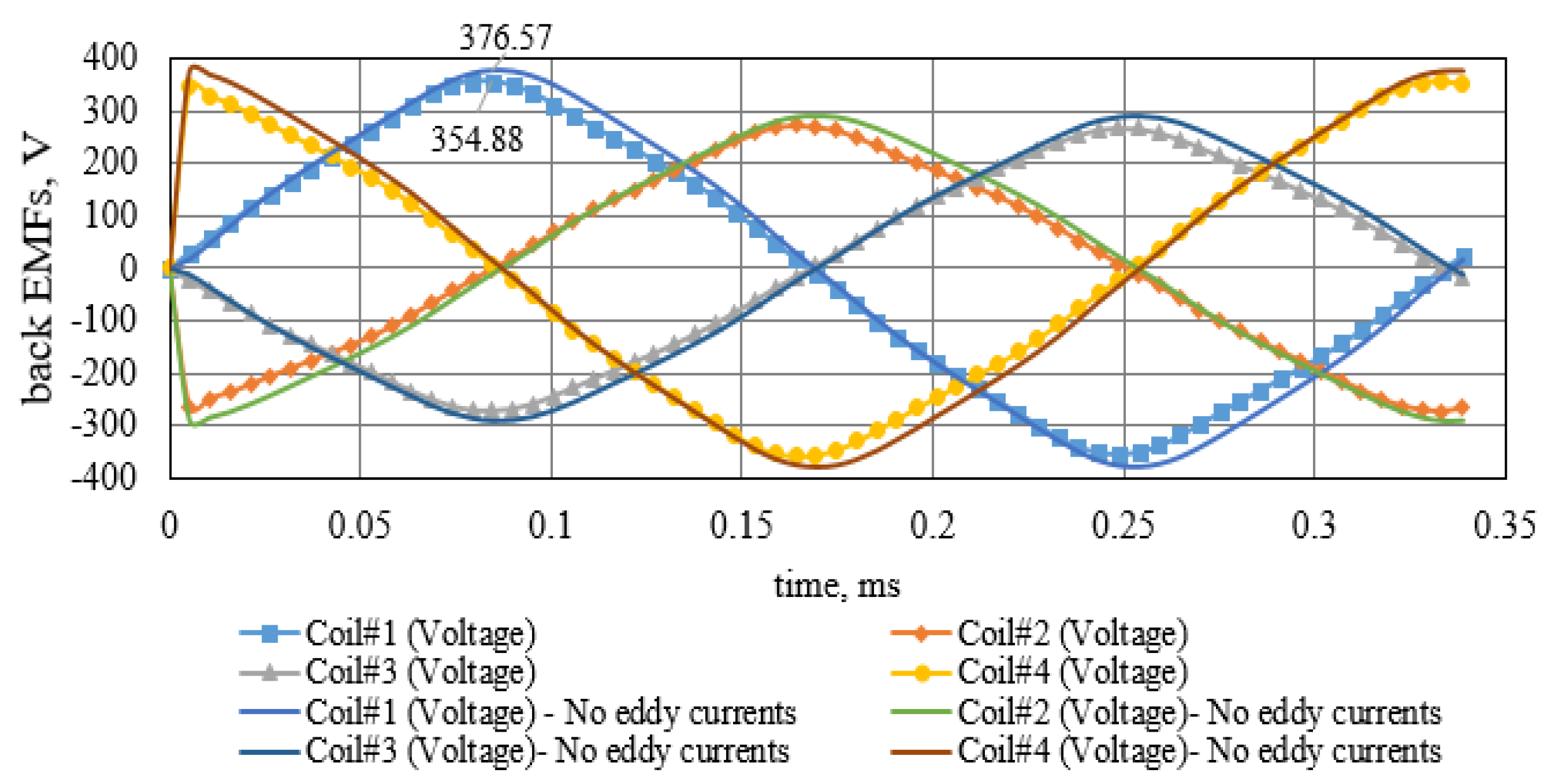
• The perfect axial alignment of TFM phases in 3D FEA might differ from the reality. In experiments, TFM phases are separated by 2–mm thick insulators as in FEA simulations. However, 3D FE simulations tend to give higher mutual flux linkage between axially separated phases.
• The magnetics of the materials might be an issue here. In 3D FE simulations, relative permeability of the SMC might offer improved flux paths. However, the real material (i.e., SMC segments) might be far from the ideal situation.

Therefore, the authors conclude that choosing the same number of turns for all 4-phases of the TFM is a more realistic approach without seeing an effect on short circuit currents. Therefore, 26-turn coils would give a better performance regarding open circuit voltages and short circuit currents of the TFM.

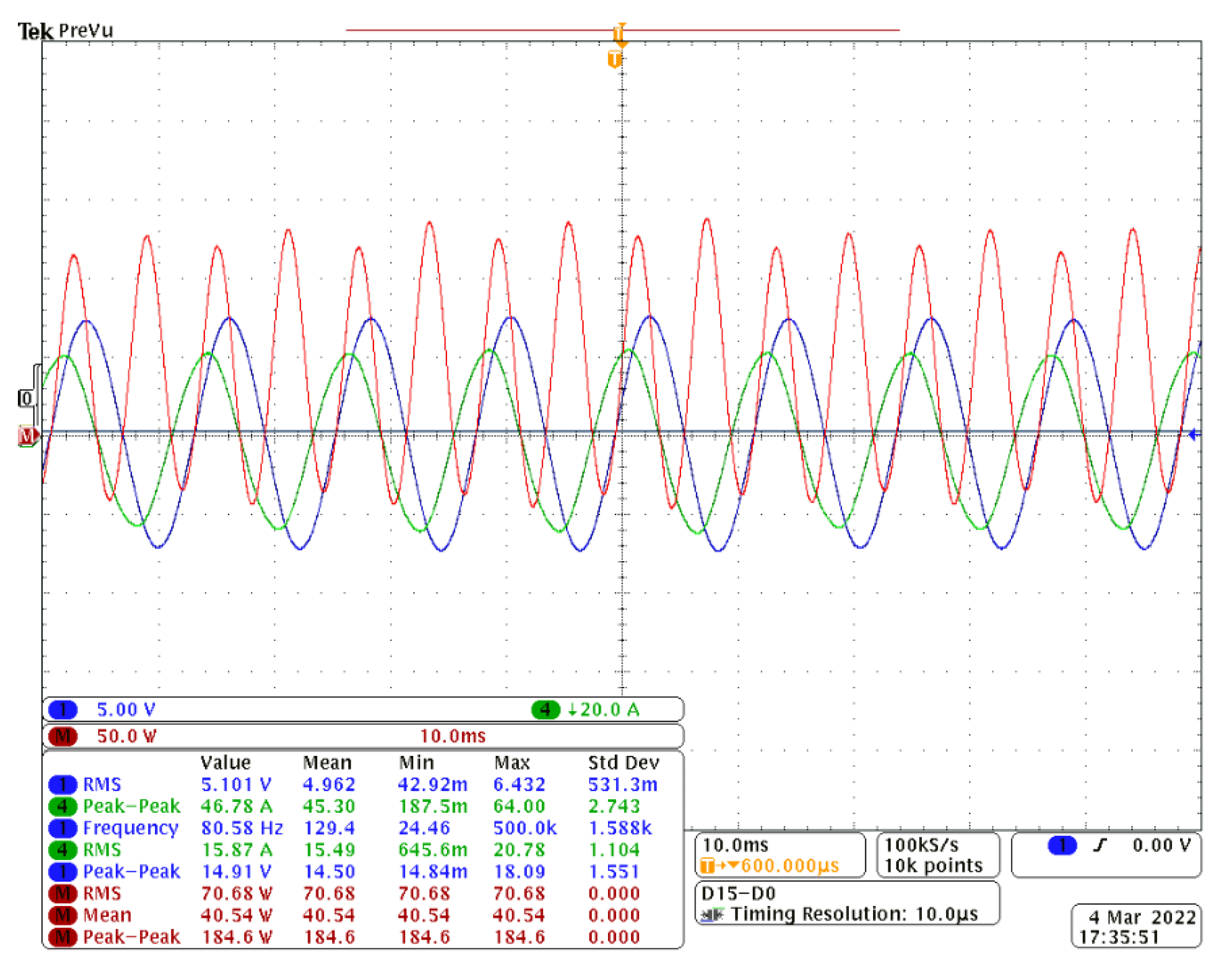
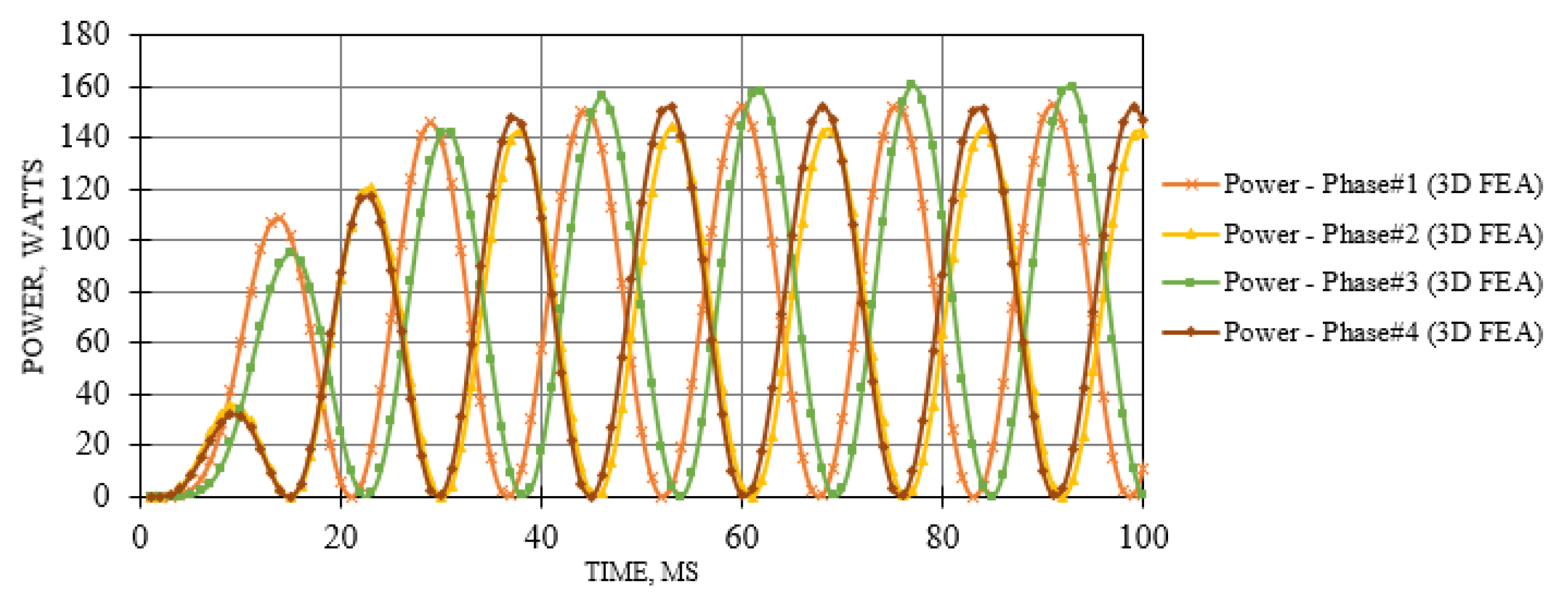
4.2. Low Speed Power

The machine phase resistance and inductance measurements are given in Figure 15. As given in Table 1, the machine must satisfy a minimum of 40 Watts power at low speed per channel. Simple equivalent circuit a PM generator is also shown in Figure 16 for a single phase (26-turns). Power factor correction has been considered at the output by matching input and output impedances using Q-factor of the circuit.

In the experimental work, variable resistors (i.e., rheostat) with bipolar shunt capacitors have been used to achieve the maximum power output. In 3D FE simulation, the machine coils are connected with loads as shown in Figure 16. Hence, the generator output power can be obtained at 400 rpm. In Section 4.1.1 and Section 4.1.2, it is demonstrated that the prototype machine under performs with regard to available back EMFs. Exotic materials such as Somaloy 130i 5P in a 3D flux machine might be the reason of low back EMFs. In addition, the manufacturing technique of this machine is unusual and this might be another reason of lower back EMFs. Therefore, manufacturing and material uncertainties have been simulated as different scenarios to match the finite element simulation results with the measurements. Thus, it can be understood how the machine is influenced by different manufacturing- and material-based uncertainties. Figure 17 shows the measured, instantaneous output power at 400 rpm.

The power measurements indicate that 26-turn TFM phases achieve 40.5 Watts average power at 400 rpm, while 20-turn mid-phases achieve about 35 Watts at the output. From the authors’ experience, there are several reasons that must be considered to understand why the prototype machine cannot achieve more than 40.5 Watts in the experimental work. They are investigated as separate scenarios as follows:

• Scenario 1: Ideal case—the TFM is considered as not a segmented machine.
• Scenario 2: Electrically conductive skin between upper and lower layers of SMC segments
• Scenario 3: Axial contact gaps (i.e., air voids) between the upper and lower layers of SMC segments
• Scenario 4: Electrically conductive skin on rotor surface due grinding
• Scenario 5: A clearance (i.e., air gap) between the SMC flux concentrating rotor poles and samarium cobalt PMs
• Scenario 6: Combination of Scenario 3 and Scenario 5 together

4.2.1. Scenario 1: Ideal Case

In this scenario, all components and assembly are assumed ideal and it is also assumed that there is no segmentation related problems in 3D FE simulations for low speed power investigation. Figure 18 shows the instantaneous power of the TFM at 400 rpm when the machine is connected to a capacitive load in the FE software. In this case, 26-turn end-phases of the TFM achieves 70.5 Watts average power, whereas 20-turn coils give about 68 Watts of power. The power results are similar for all-phases but not exactly the same due to unavoidable mutual flux linkages between the separate phases and slightly different SC currents.

4.2.2. Scenarios 2–6 with Manufacturing Related Uncertainties

Different scenarios have been investigated as shown in Figure 19 to observe the effect of manufacturing uncertainties in the prototype alternator. In Figure 19a, the compressed SMC iron powder causes burrs on the surface of the segments. They are not wanted and the authors preferred to remove the burrs manually which is also called manual de-burring as illustrated in Figure 20b. However, in Figure 20a, a machine brushed (i.e., polished) version is shown. The machine brushed version reduces the burrs completely but also degrades the insulated iron particles significantly causing a conducting layer. It should be noted that the magnetic flux flows in axial direction in the stator core back.

Electrically un-insulated iron particles might cause longer eddy current paths and so increase in eddy currents due to conducting skin decreases back EMF, because induced eddy currents in a 3D flux machine generates opposing magnetic field with respect to the source magnetic field. Figure 19a therefore assumes an artificial SMC layer with an electrical conductivity of 10 times higher than the original material. Thus, flux linkages and back EMFs are affected negatively. In Figure 16b, the SMC segments of the TFM are considered to be not in perfect contact. Therefore, the authors add 0.5 mm axial air gap between the segments. In Figure 19c, rotor grinding has been simulated with a conductive skin of 0.5 mm on the surface of the rotor. Rotor grinding is also shown in Figure 8d which is necessary for wrapping carbon fiber sleeve on the rotor. Rotor grinding might cause conducting skin. Therefore, the authors assumed a very thin layer (i.e., 0.5 mm) of aluminum and copper sheets (high electrical conductivity) on the rotor surface for the simulations. Figure 19d considers a clearance between the flux concentrating SMC rotor poles and PMs. Any air gap between the SMC poles and PMs can reduce the air-gap flux density significantly. The authors simulated 0.2 mm gaps between the rotor SMC poles and PMs. Lastly, Figure 19e assume both rotor and stator related manufacturing uncertainties in the performance of the machine, namely Scenario 3 and 5 together.

The results are summarized in Figure 21. The discrepancy between the ideal case and experimental machine is 31.7 Watts, corresponding to about 44% error. If we consider air voids due to burrs in the stator core back, the error in power output will be about 25% compared to the prototype machine’s power. Similarly, if a mechanical clearance of 0.2 mm occurs between the flux concentrating SMC poles and PMs, the power output diverges 30.7% from the ideal case. In other words, it is clear that manufacturing related uncertainties in the TFM might cause a wide range of power results at low speed. It should be also noted that manufacturing related problems might occur simultaneously which is certainly not desired. The target output power is 40 Watts which is satisfied yet the potential of the machine in terms of low speed, output power might reach 70 Watts. This is however only possible if there is no discrepancy between the ideal, simulated machine and the prototype.

4.2.3. Material Uncertainties in Rotor and Stator

The prototype TFM has Grade 5 titanium rotor hub with an electrical resistivity of 1.7 µΩ.m which is one hundred times more resistive than bulk copper. However, it is still electrically conducting material and affects the machine performance negatively regarding eddy currents. In 3D FE simulations, the non-magnetic titanium rotor hub with zero electrical conductivity and maximum electrical conductivity has been simulated to see how conductive rotor core back influences the TFM performance. The open circuit voltages comparison at 15,000 rpm is given in Figure 22.

In Figure 22, the back EMF drops about 5.7% in end (i.e., side) phases with 26-turns, while back EMFs are reduced by 6.5% for mid-phases with 20-turns. This implies that there is a disadvantage of electrically conducting rotor hub on the power output. Nonetheless, it is not possible to avoid a titanium rotor hub in this machine due to required mechanical strength. In 3D FE simulations, contribution of titanium rotor hub to overall loss at 15,000 rpm is 27 Watts due to induced eddy currents. Computational cost of 3D FE simulations with eddy current loss calculations increase by about 16 times (i.e., 94 h) in which eddy currents are disabled for 3D optimization of the proposed machine.

Another cause of relatively lower back EMFs in the prototype machine is magnetic properties of the soft magnetic composite materials. Modelling of SMC is not as straightforward as laminated steels and low power output could be because of optimistic modelling of SMC in FE simulations [19]. Especially bulk (i.e., inter-particle) eddy currents in SMC could be easily underestimated in numerical models [19]. This is explained in the following sub-section.

5. Iron Loss Modelling for SMC Segments

Iron loss modelling for SMC cores requires an accurate representation of Steinmetz equation. The authors previously proposed an empirical Steinmetz equation as reported in [19] which differs from datasheets given by Höganas AB, Sweden [20]. It is given in Equation (2).

$$P_{total}=K_h{f}^a{\widehat{B}}^{\beta }+\widetilde{K_{ep}}{f}^2{\widehat{B}}^2 (\mathrm{Watts}/\mathrm{kg})$$

(2)

$$\widetilde{K_{ep}}=K_{ep}+\frac{d^2}{1800\times \rho \times {\rho }_e}={\theta }_1\times S^{{\theta }_2}$$

(3)

where $K_{ep}$ stands for particle eddy current loss coefficient and also information of d, minimum cross-sectional dimension of the component (mm), $\rho$ (g/cm³) and ${\rho }_e$ (µΩm) are required to specify the iron loss in W/kg. Electrical resistivity must be known to accurately calculate the specific loss in SMC. Additionally, $K_h$ is hysteresis loss coefficient, $f$ is electrical frequency (Hz) and $\widehat{B}$ is peak flux density in the SMC core. As we described previously in [19], the electrical resistivity of SMC is not easy to measure and it causes error for loss computations. Therefore, in Equation (3), eddy current coefficient $\widetilde{K_{ep}}$ which includes both particle ($K_{ep})$ and bulk eddy current loss terms (i.e., second term) is written as a concave power equation with the unknowns of ${\theta }_1$, $S$ and ${\theta }_2$. These unknowns are obtained by curve fitting for $P_{total}$ as a function of frequency and peak magnetic flux density.

For Somaloy 130i 5P and Somaloy 700 HR 3P which are the materials for TFM stator segments and flux concentrating rotor poles, respectively, $\widetilde{K_{ep}}$ is first obtained by using Equation (3) and loss data with respect to frequency and peak magnetic flux density, as shown in Figure 23. Variation of cross-sectional dimensions ($d$) is considered to determine the parameters of ${\theta }_1$ and ${\theta }_2$ via curve-fitting of $\widetilde{K_{ep}}$ as a function of $d$. Then, two-term Steinmetz equations have been set as in Equation (2) for different grades of SMCs in order to use in 3D FE simulations. The final and more accurate form of Steinmetz equations (Watts/kg) for the given SMC materials are tabulated in Equations (4) and (5) below.

$$P_{loss-S130i-5P}=0.081207f^{1.0017}{\widehat{B}}^{1.75033}+5.45805\times {10}^{-6}{S}^{0.250573}{f}^2{\widehat{B}}^2$$

(4)

$$P_{loss-S700HR-3P}=0.10894f^{1.000633}{\widehat{B}}^{1.7716}+6.59201\times {10}^{-6}{S}^{0.337708}{f}^2{\widehat{B}}^2$$

(5)

The equations given in (4) and (5) are useful for iron loss modelling of SMC in 3D FE simulations. Since the term containing material conductivity is already fitted into the Steinmetz equation as proposed in Equation (3), the FE user must take the SMC material resistivity or conductivity null in the simulations as the proposed Steinmetz equations take the ohmic (i.e., Joule) losses due to material’s conductivity into account when overall iron losses are calculated. More description of the followed approach is given previously in [19].

It should be noted that Equations (4) and (5) are obtained through magnetic measurements on SMC ring samples (i.e., toroids). However, the stator segments as shown in Figure 10 are much complicated geometries compared to simple ring samples and the implementation of Equations (4) and (5) might still underestimate the eddy current losses. Furthermore, higher eddy currents implies reduction in back EMFs and the machine performance is degraded. For this reason, the design of SMC based 3D flux machines needs to take underestimated eddy currents in early stages of the design and optimization. Otherwise, the build machines might underperform. The authors noted that in open circuit loss measurements at 15,000 rpm, the prototype machine gives about 398 Watts higher loss compared to 3D FE simulations after mechanical air friction/windage and bearing losses are added to the iron loss and Joule loss results acquired via electromagnetic 3D FE simulations. Air friction and bearing losses are calculated as reported in [21]. The reason behind the discrepancy could be: uncertainty in SMC material resistivity, PM material resistivity (i.e., permanent magnet loss) and some stray losses in the prototype machine.

6. Conclusions

This paper has demonstrated a 4-phase TFM made from pressed SMC components.

In open circuit tests, the prototype machine gives lower back EMFs at 400 rpm (i.e., about 23.7%). In addition, in short circuit tests, the prototype machine gives lower short circuit currents (i.e., about 21.83% lower). There are several reasons for low back EMFs as follows:

• Magnetically low performance of the SMC stator segments.
• Physically non-homogeneous air gap length (with some deviations) around the rotor perimeter due to slightly elliptical stator bodies.
• Degradation of rotor surface via grinding that causes a conducting skin on the surface of the rotor.
• Rough contact surfaces due to burrs between stator SMC segments, coming together axially, might cause air gap in the stator yoke.
• Manual or automated de-burring of SMC stator segments after compaction might cause degraded SMC particles and that will end up with a conducting skin with a thickness of about 0.5 mm.
• Misalignment between the rotor and stator bodies.

The reasons given above might occur simultaneously in the final machine assembly and each might contribute to degradation of electromagnetic performance in the prototype machine. It should be noted that the assembly of the proposed transverse flux alternator is novel and accommodates many challenges as described in this paper. Experimentally, 26 turn end phases achieve more than 40 Watts at low speed which is the target. The machine has the potential of higher power output in generating mode but the construction of this kind of 3D flux machine is still not as straightforward as in radial flux machines and requires more investigation.