Review on the Traditional and Integrated Passives: State-of-the-Art Design and Technologies
Abstract
:1. Introduction
- Space and mass reduction over traditional drive components (discrete ones).
- Fewer manufacturing process.
- Possible reduction of EMI problems at high frequency due to the elimination of cables transferring power.
- Ability to replace direct online machines with variable frequency machines without significantly adjusting the associated plant.
- Single package installation which further reduces installation time and cost.
- Drive topologies from greater design synergy greater flexibility in the machine.
2. Filter Inductor Design
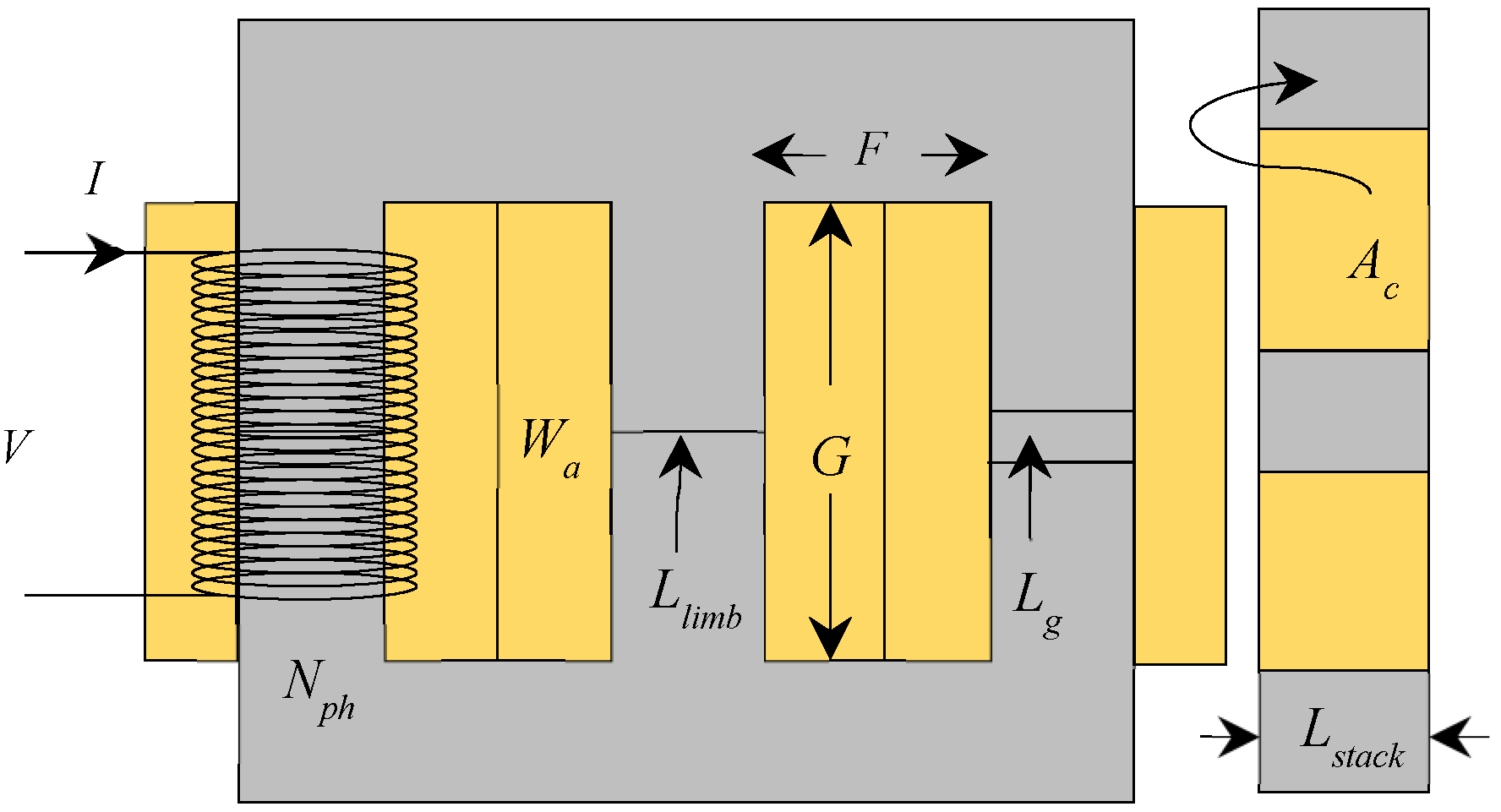
2.1. Expression for Area Product
2.2. Typical Inductor Requirments & Sizing Constraints
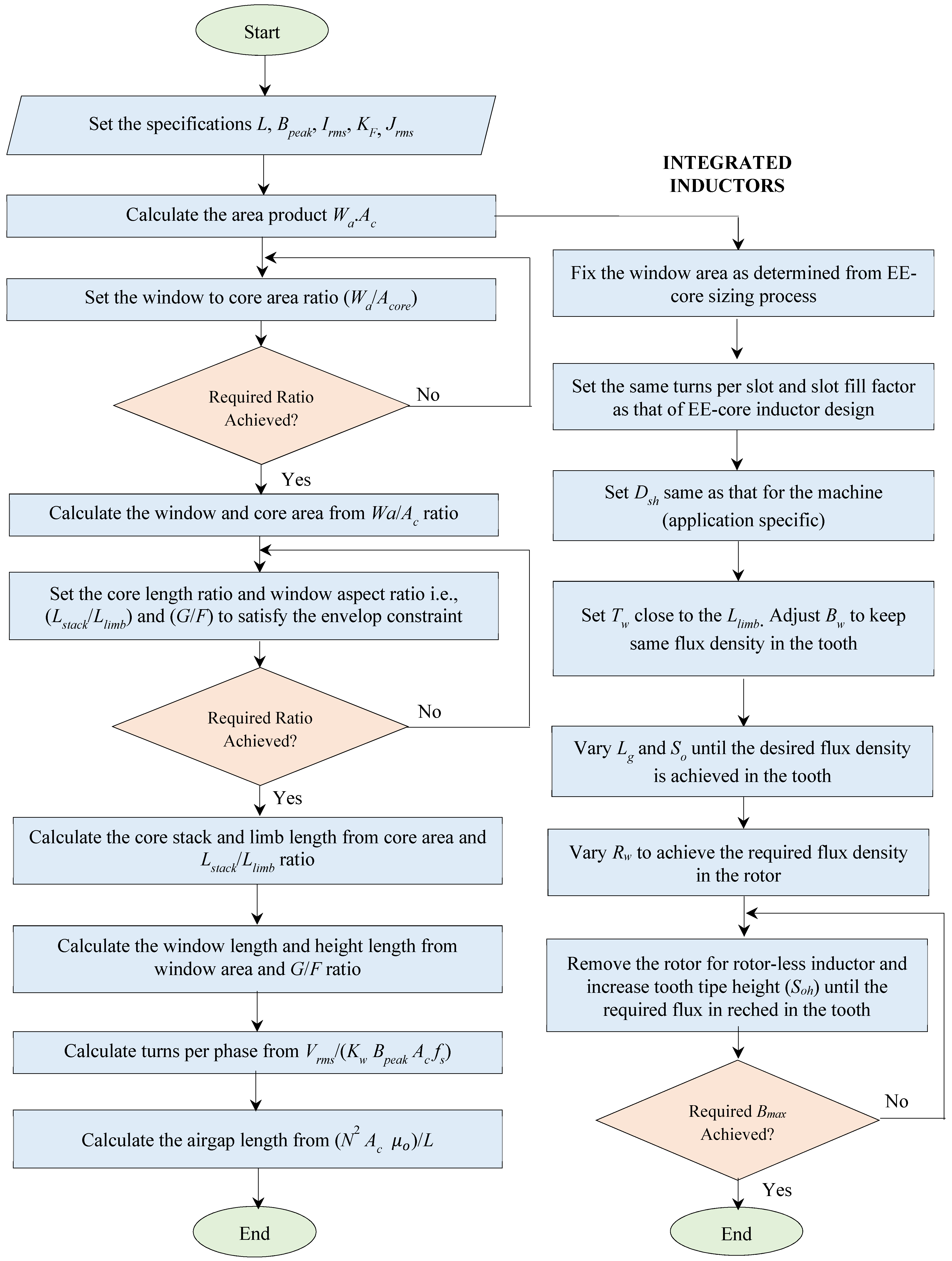
2.3. Inductor Sizing
2.3.1. EE-Core Inductor
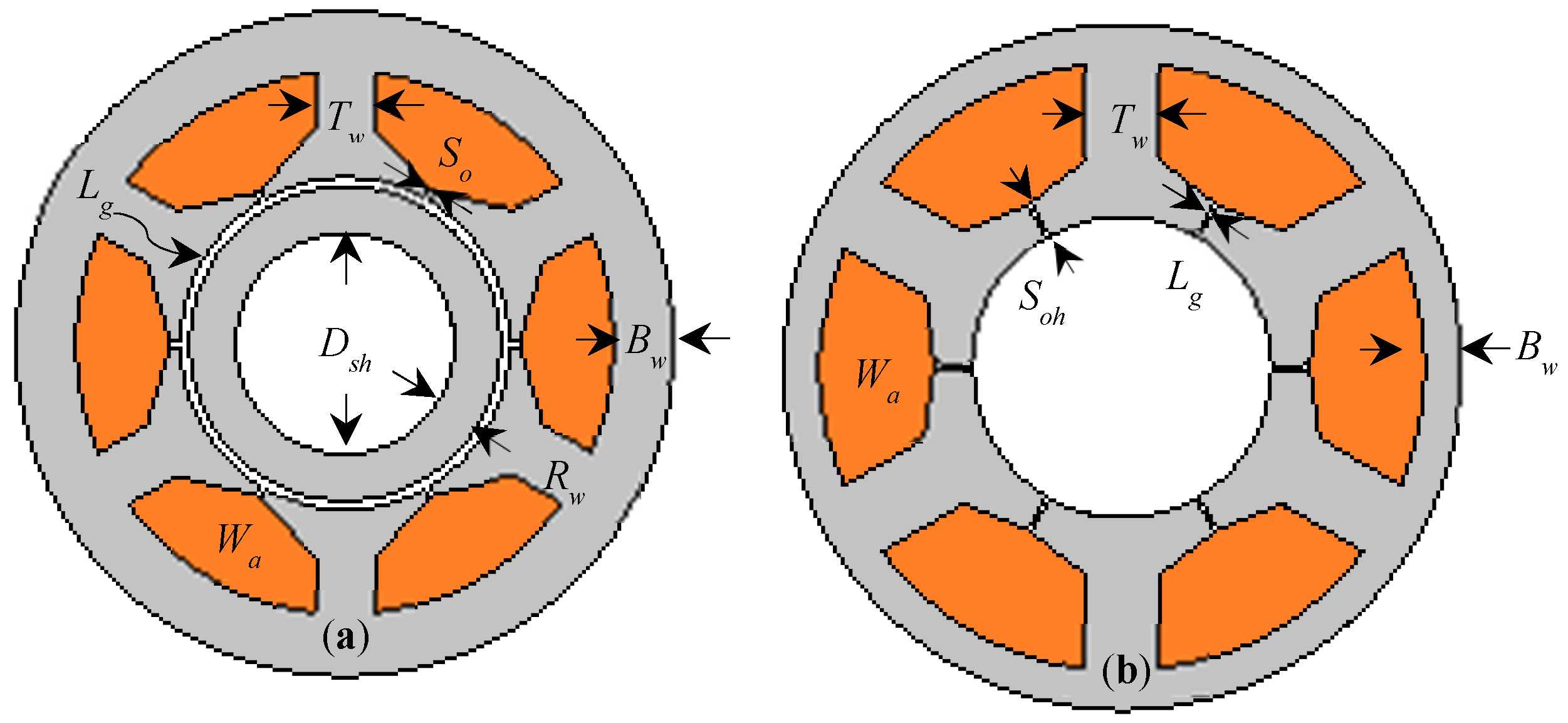
2.3.2. Integrated Inductors
2.3.3. Inductor Sizing Using Simulation-Driven Approach
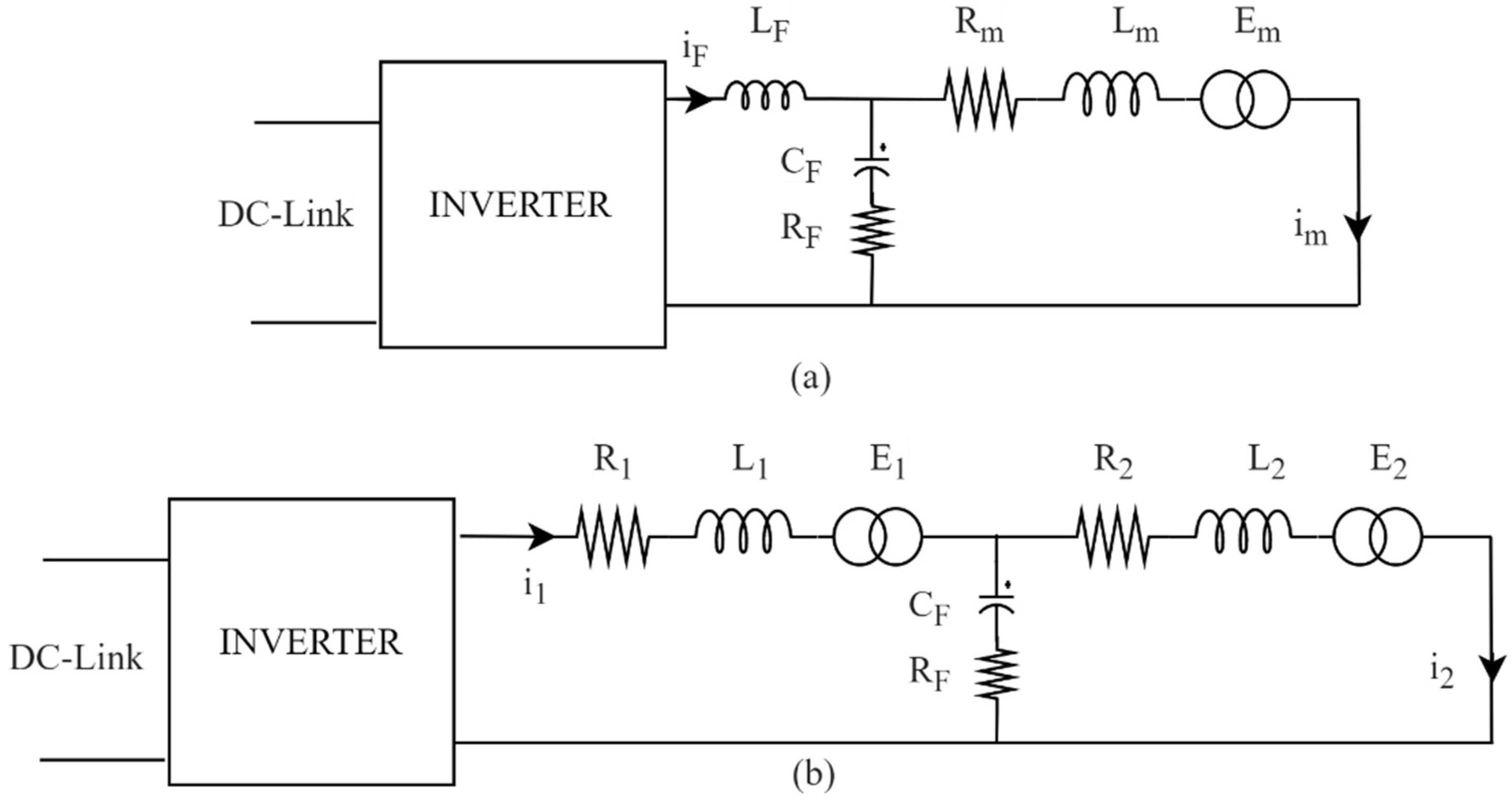
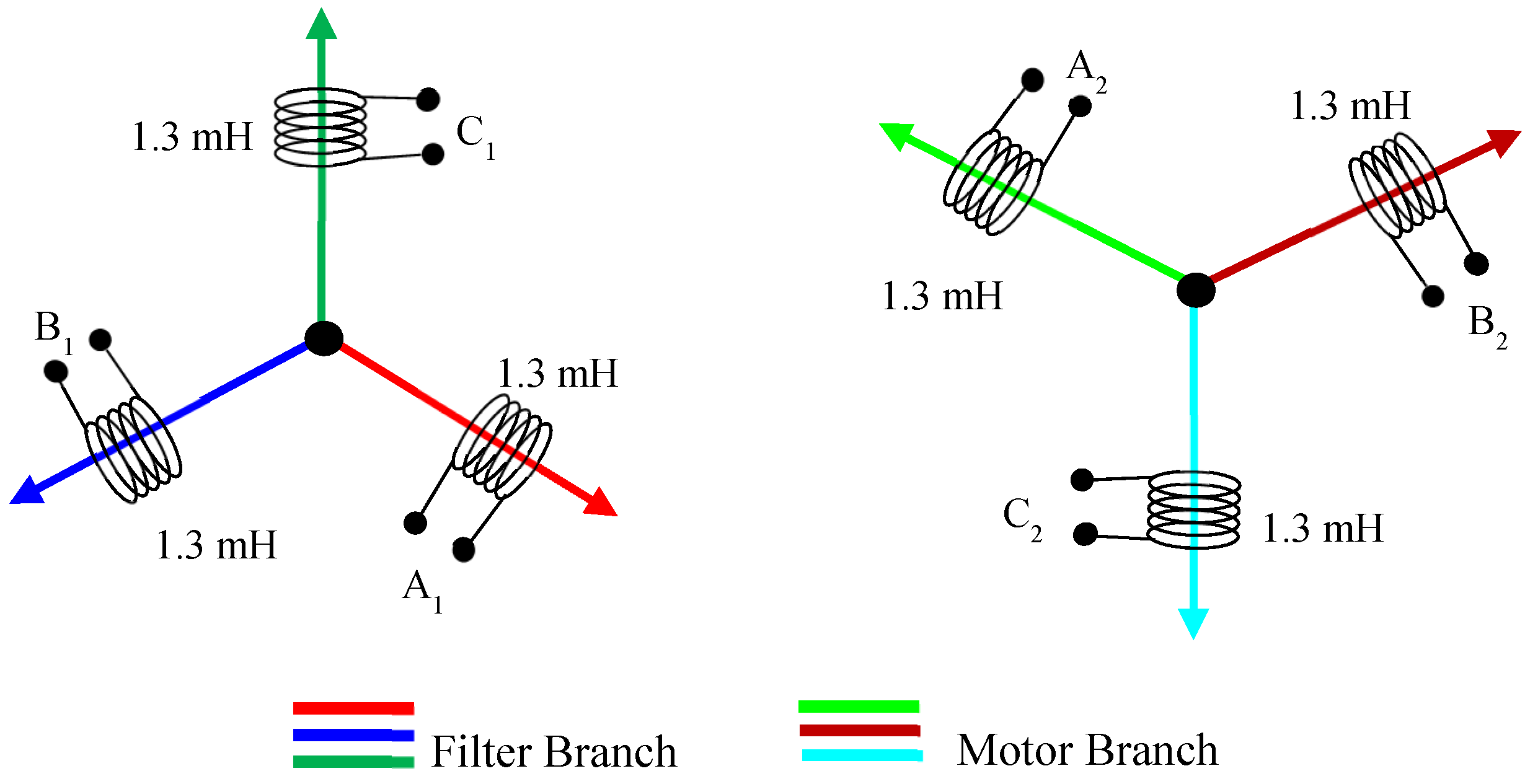
3. Structural Layout & Integration of Filter Inductors
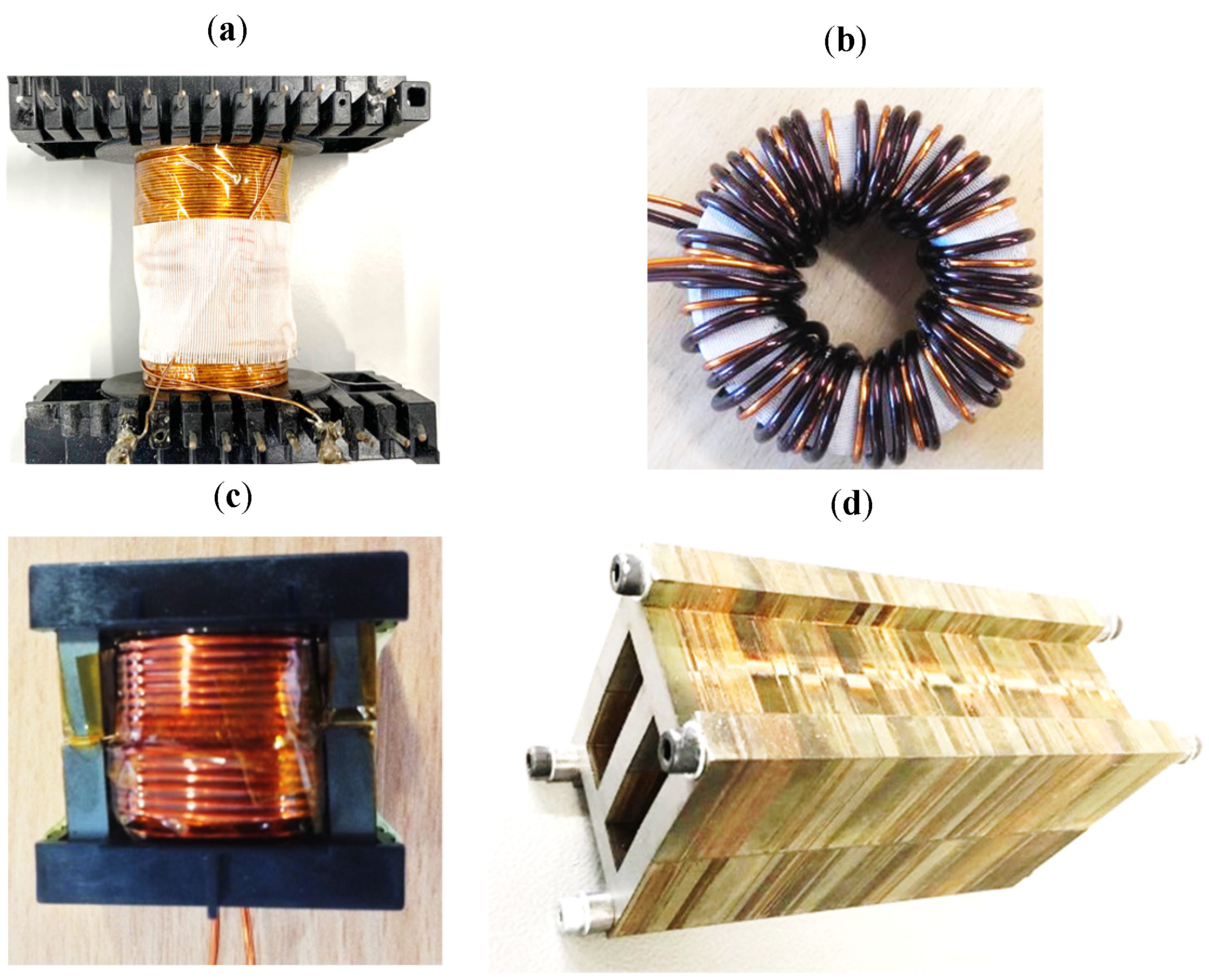
3.1. Traditional Inductors
3.2. Integrated Inductors
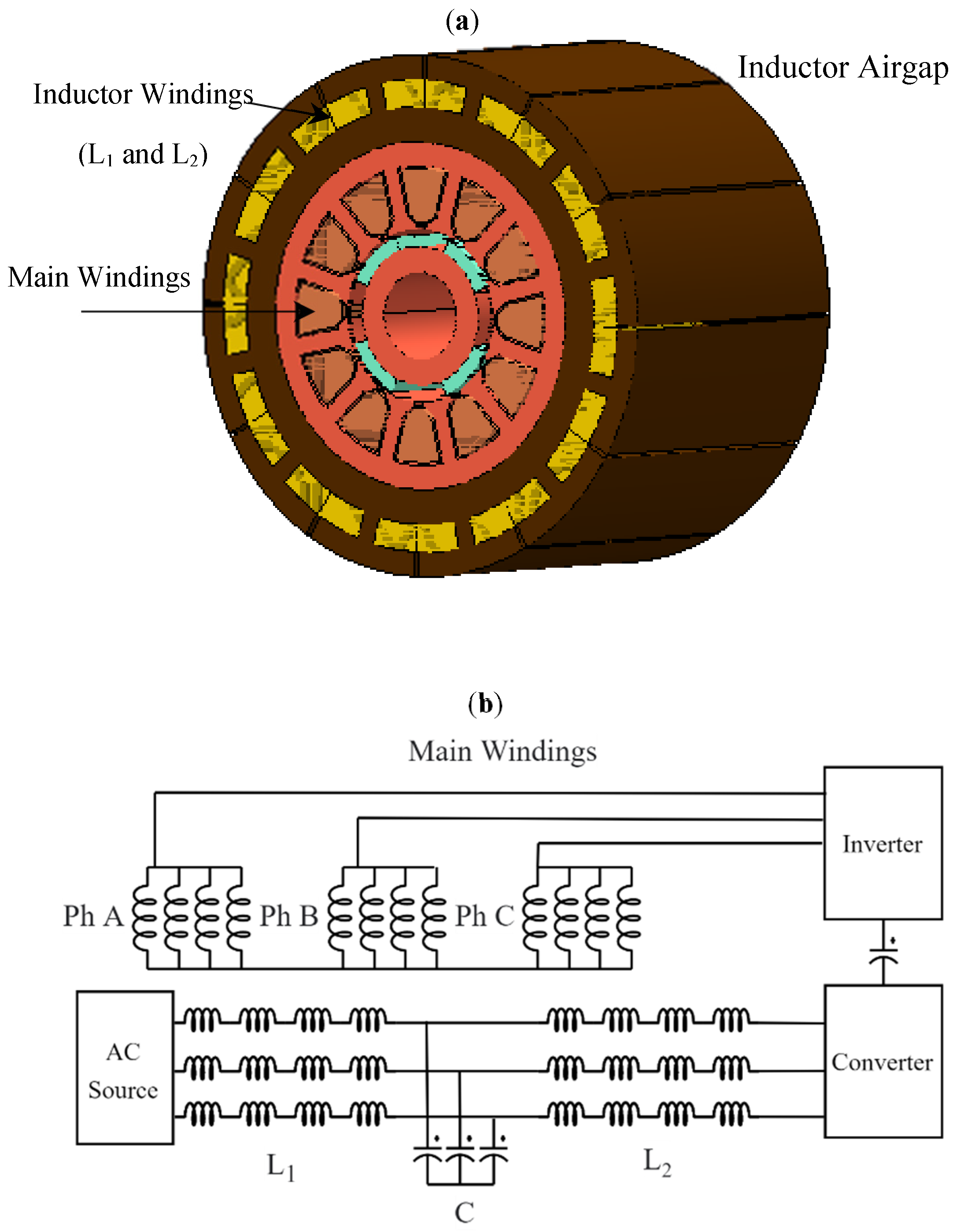
3.2.1. Integration within the Machine’s Envelope
Motor with Integrated Output Filter Inductor
Motor-Shaped Inductors
High Current Density Rotor-Less Inductor
Integrated Inductor Wound on the Stator Back Iron
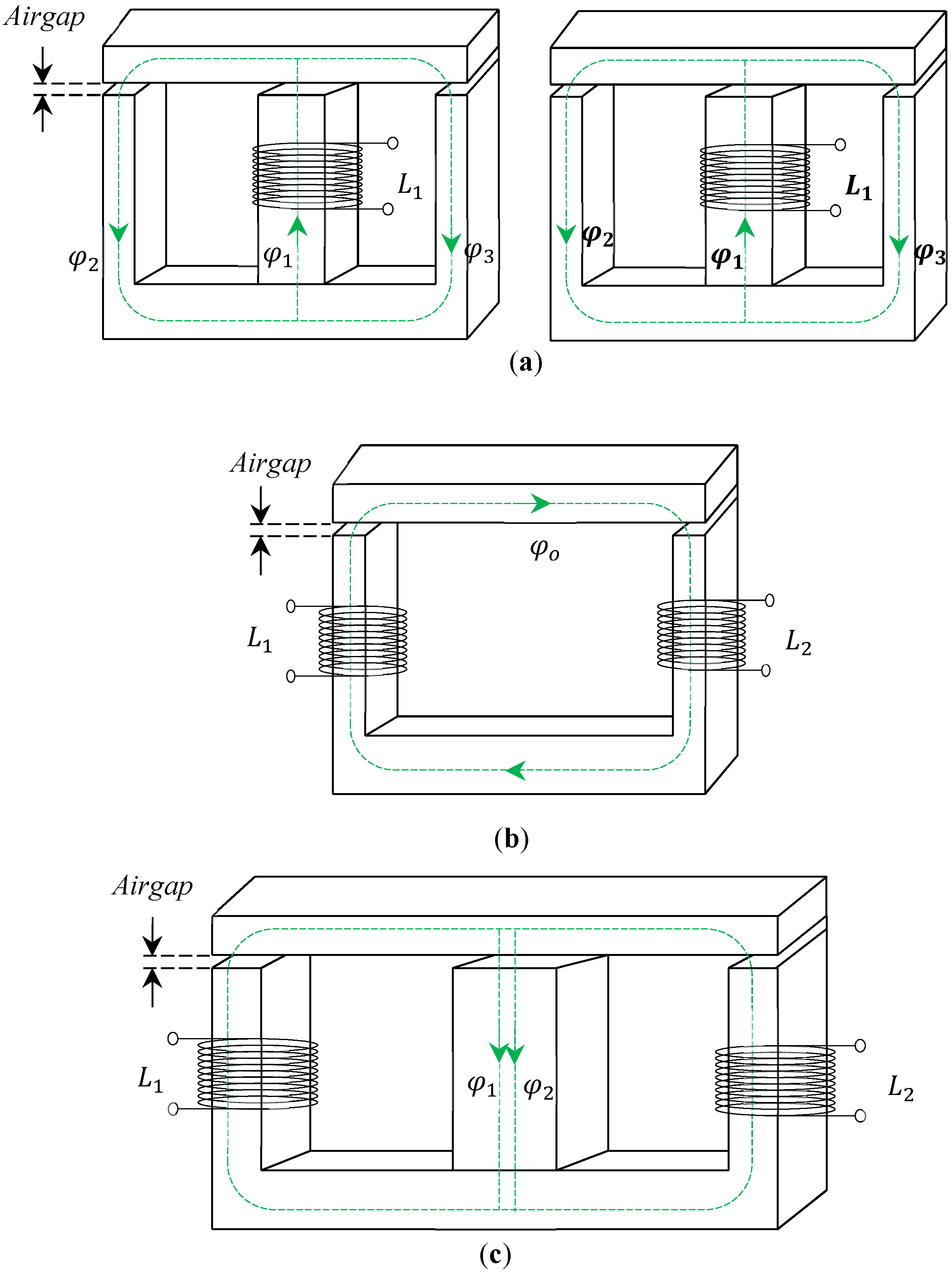
3.2.2. Integration within the Traditional Cores
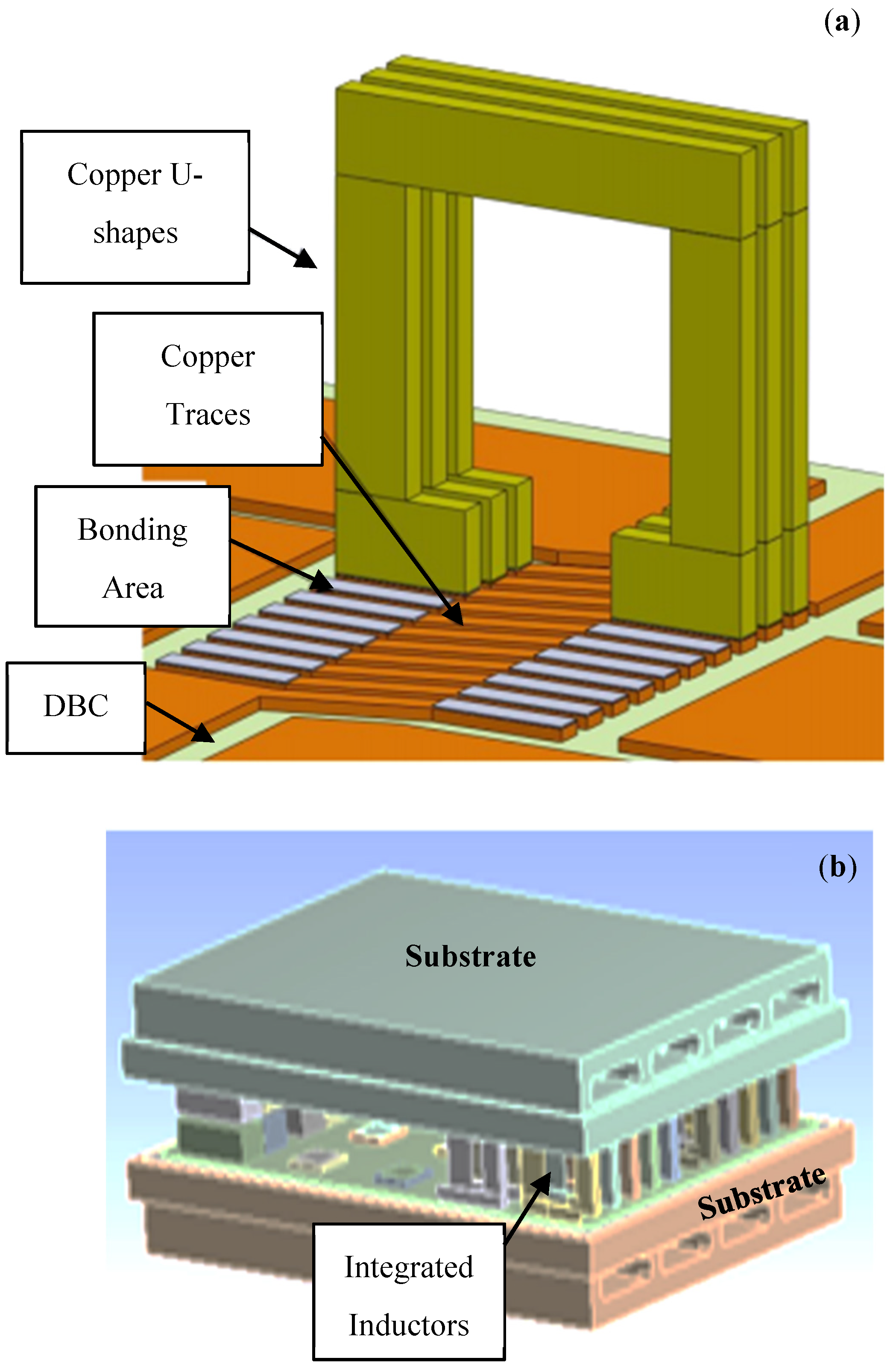
3.2.3. Integrated Inductor on Power Module Substrate
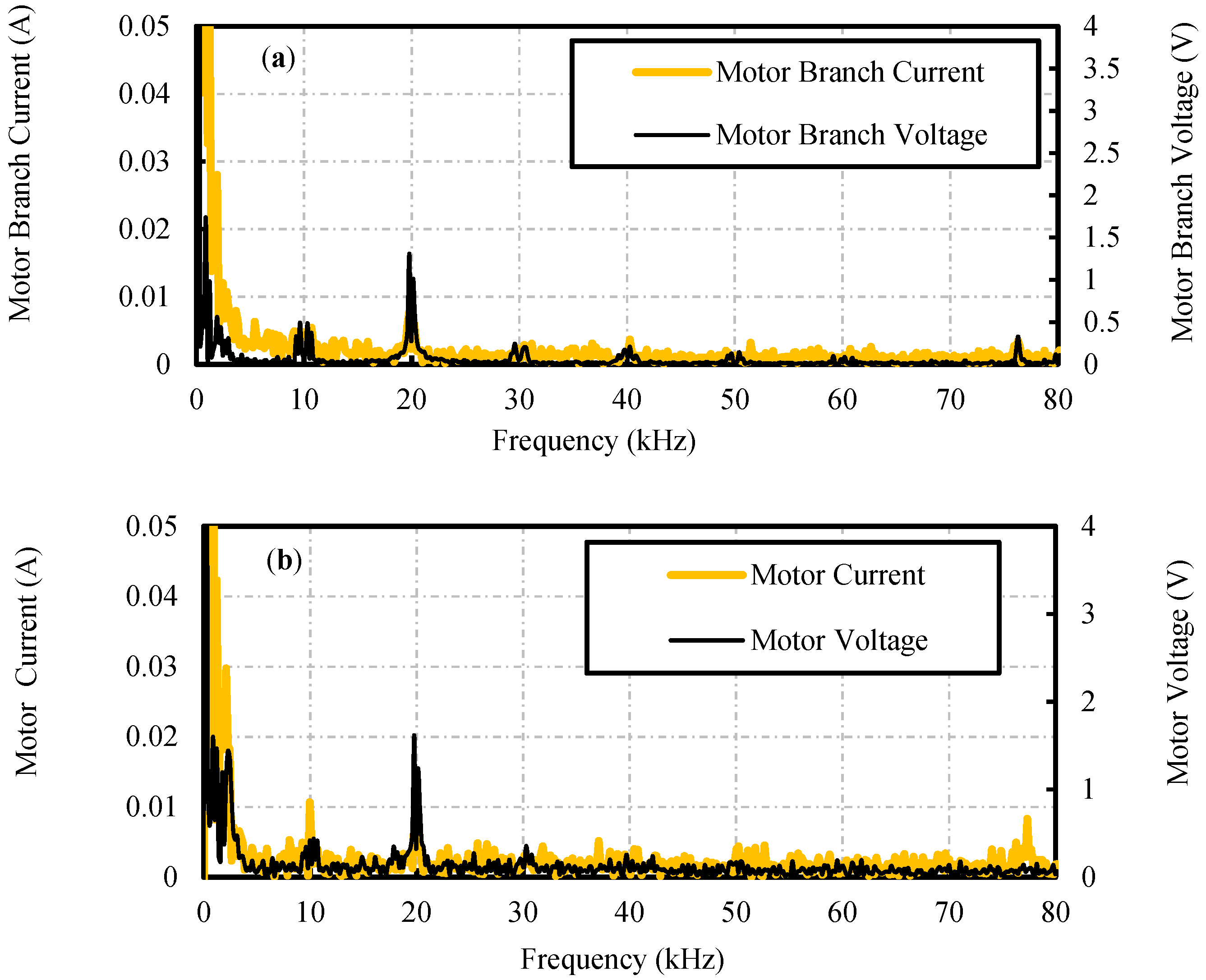
4. Integration of RC Branch and Capacitor Technologies for Integrated Filters
4.1. Integration of RC Branch
4.2. Capacitor Technologies for Integrated Filters
- Film capacitors
- Electrolytic capacitors
- Ceramic capacitors
- Electro-chemical double layer (ECDL)
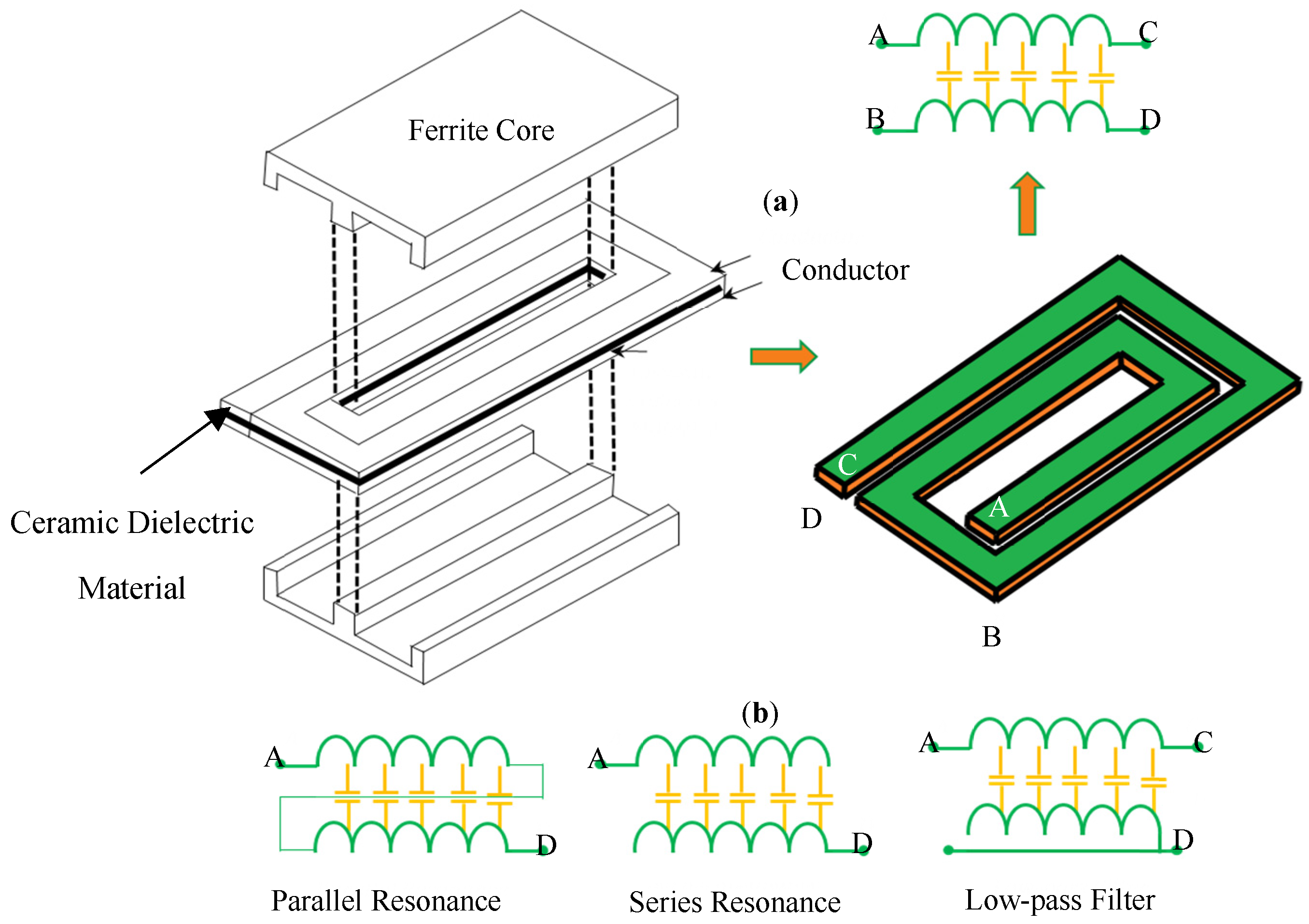
4.2.1. Film Capacitors
4.2.2. Electrolytic Capacitors
4.2.3. Ceramic Capacitors
4.2.4. Electro-Chemical Double Layer (ECDL) Capacitors
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Khowja, M.R.; Gerada, C.; Vakil, G.; Abebe, R.; Odhano, S.A.; Patel, C.; Wheeler, P. Novel Motor-Shaped Rotational Inductor for Motor Drive Applications. IEEE Trans. Ind. Electron. 2020, 67, 1844–1854. [Google Scholar] [CrossRef]
- Walker, A.; Vakil, G.; Gerada, C. Novel Core Designs to Miniaturise Passive Magnetic Components. In Proceedings of the 2018 IEEE Transportation Electrification Conference and Expo (ITEC), Long Beach, CA, USA, 13–15 June 2018; pp. 644–649. [Google Scholar]
- Khowja, M.R.; Gerada, C.; Vakil, G.; Wheeler, P.; Patel, C. Integrated Output Filter Inductor for Permanent Magnet Motor Drives. In Proceedings of the Industrial Electronics Society, IECON 2016—42nd Annual Conference of the IEEE, Florence, Italy, 23–26 October 2016. [Google Scholar]
- Khowja, M.R.; Gerada, C.; Vakil, G.; Wheeler, P.; Patel, C. Novel Integrative Options for Passive Filter Inductor in High-Speed AC Drives. In Proceedings of the Industrial Electronics Society, IECON 2016—42nd Annual Conference of the IEEE, Florence, Italy, 23–26 October 2016. [Google Scholar]
- Lambert, S.M.; Mecrow, B.C.; Abebe, R.; Vakil, G.; Johnson, C.M.G. Integrated Drives for Transport—A Review of the Enabling Electronics Technology. In Proceedings of the 2015 IEEE Vehicle Power and Propulsion Conference (VPPC), Montreal, QC, Canada, 19–22 October 2015; pp. 1–6. [Google Scholar]
- Wu, S.; Tian, C.; Zhao, W.; Zhou, J.; Zhang, X. Design and Analysis of an Integrated Modular Motor Drive for More Electric Aircraft. IEEE Trans. Transp. Electrif. 2020, 6, 1412–1420. [Google Scholar] [CrossRef]
- Mohamed, M.A.; Lambert, S.M.; Mecrow, B.C.; Atkinson, D.J.; Smith, A.C. An electrical machine with integrated drive LCL filter components. In Proceedings of the 8th IET International Conference on Power Electronics, Machines and Drives (PEMD 2016), Glasgow, UK, 19–21 April 2016; pp. 1–6. [Google Scholar]
- Popovic, J.; Ferreira, J.A.; Gerber, M.B.; Konig, A.; Doncker, R.d. Integration technologies for high power density power electronic converters for AC drives. In Proceedings of the International Symposium on Power Electronics, Electrical Drives, Automation and Motion, Taormina, Italy, 23–26 May 2006; pp. 634–639. [Google Scholar]
- Popovic-Gerber, J.; Gerber, M.; Ferreira, B. Integrated filter in electrolytic capacitor technology for implementation in high power density industrial drives. In Proceedings of the 2008 IEEE Power Electronics Specialists Conference, Rhodes, Greece, 15–19 June 2008; pp. 2968–2974. [Google Scholar]
- Abebe, R.; Vakil, G.; Calzo, G.L.; Cox, T.; Lambert, S.; Johnson, M.; Gerada, C.; Mecrow, B. Integrated motor drives: State of the art and future trends. IET Electr. Power Appl. 2016, 10, 757–771. [Google Scholar] [CrossRef] [Green Version]
- Mclyman, C.W.T. Transformer and Inductor Design Handbook, 4th ed.; Taloy Francis Group: Idyllwild, CA, USA, 2004. [Google Scholar]
- Kazimierczuk, M.K.; Sekiya, H. Design of AC resonant inductors using area product method. In Proceedings of the 2009 IEEE Energy Conversion Congress and Exposition, San Jose, CA, USA, 20–24 September 2009; pp. 994–1001. [Google Scholar]
- Nijende, H.; Frohleke, N.; Bocker, J. Optimized size design of integrated magnetic components using area product approach. In Proceedings of the 2005 European Conference on Power Electronics and Applications, Dresden, Germany, 11–14 September 2005; p. 10. [Google Scholar]
- Mclyman, C.W.T. Magnetic Core Selection for Transformers and Inductors, 2nd ed.; Taloy Francis Group: Boca Raton, CA, USA, 2017. [Google Scholar]
- Goodrick, K.J.; Seo, G.-S.; Mukherjee, S.; Roy, J.; Mallik, R.; Majmunovic, B.; Dutta, S.; Maksimović, D.; Johnson, B. LCOE Design Optimization Using Genetic Algorithm with Improved Component Models for Medium-Voltage Transformerless PV Inverters. In Proceedings of the 2020 IEEE Energy Conversion Congress and Exposition (ECCE), Detroit, MI, USA, 11–15 October 2020; pp. 2262–2267. [Google Scholar]
- Schobre, T.; Ariztegui, R.G.; Mallwitz, R. Genetic Algorithm Based Multi Objective Optimization for Inductor Design. In Proceedings of the 2020 22nd European Conference on Power Electronics and Applications (EPE’20 ECCE Europe), Lyon, France, 7–11 September 2020; pp. 1–9. [Google Scholar]
- Ho, S.L.; Chen, N.; Fu, W.N. An Optimal Design Method for the Minimization of Cogging Torques of a Permanent Magnet Motor Using FEM and Genetic Algorithm. IEEE Trans. Appl. Supercond. 2010, 20, 861–864. [Google Scholar] [CrossRef]
- Hwang, C.; Lyu, L.; Liu, C.; Li, P. Optimal Design of an SPM Motor Using Genetic Algorithms and Taguchi Method. IEEE Trans. Magn. 2008, 44, 4325–4328. [Google Scholar] [CrossRef]
- Fernando, N.; Vakil, G.; Arumugam, P.; Amankwah, E.; Gerada, C.; Bozhko, S. Impact of Soft Magnetic Material on Design of High-Speed Permanent-Magnet Machines. IEEE Trans. Ind. Electron. 2017, 64, 2415–2423. [Google Scholar] [CrossRef]
- Khowja, M.R.; Gerada, C.; Vakil, G.; Quadri, S.Q.; Wheeler, P.; Patel, C. Design of an Integrated Inductor for 45kW Aerospace Starter-Generator. In Proceedings of the 2018 IEEE Transportation Electrification Conference and Expo (ITEC), Long Beach, CA, USA, 13–15 June 2018; pp. 570–575. [Google Scholar]
- Trentin, A.; Zanchetta, P.; Clare, J.; Wheeler, P. Automated Optimal Design of Input Filters for Direct AC/AC Matrix Converters. IEEE Trans. Ind. Electron. 2012, 59, 2811–2823. [Google Scholar] [CrossRef]
- Khowja, M.R.; Vakil, G.; Gerada, C.; Yang, T.; Bozhko, S.; Wheeler, P. Trade-off Study of a High Power Density Starter-Generator for Turboprop Aircraft System. In Proceedings of the IECON 2019—45th Annual Conference of the IEEE Industrial Electronics Society, Lisbon, Portugal, 14–17 October 2019; Volume 1, pp. 1435–1440. [Google Scholar]
- Khowja, M.R.; Gerada, C.; Vakil, G.; Patel, C.; Odhano, S.A.; Walker, A.; Wheeler, P. Novel Permanent Magnet Synchronous Motor with Integrated Filter Inductor, Using Motor’s Inherent Magnetics. IEEE Trans. Ind. Electron. 2020, 68, 5638–5649. [Google Scholar] [CrossRef]
- Nee, B.M.; Chapman, P.L. Integrated Filter Elements in Electric Drives. In Proceedings of the 2007 IEEE Vehicle Power and Propulsion Conference, Arlington, TX, USA, 9–12 September 2007; pp. 148–153. [Google Scholar]
- Garvey, S.D.; Norris, W.T.; Wright, M.T. The role of integrated passive components in protecting motor windings. IEE Proc.-Electr. Power Appl. 2000, 147, 367–370. [Google Scholar] [CrossRef]
- Nakajima, Y.I.; Mizukoshi, T.; Sato, Y.; Zushi, S.; Yusuke, Z. Ntegrated Capacitor Type Stator. U.S. Patent US 8,049,383 B2, 31 October 2011. [Google Scholar]
- Rocca, A.L.; Khowja, M.R.; Vakil, G.; Gerada, C.; Wheeler, P. Thermal Design of an Integrated Inductor for 45kW Aerospace Starter-Generator. In Proceedings of the 2020 IEEE Transportation Electrification Conference and Expo (ITEC), Chicago, IL, USA, 23–26 June 2020. [Google Scholar]
- Deng, X.; Lambert, S.; Mecrow, B.; Mohamed, M.A.S. Design Consideration of a High-Speed Integrated Permanent Magnet Machine and its Drive System, In Proceedings of the 2018 XIII International Conference on Electrical Machines (ICEM). Alexandroupoli, Greece, 3–6 September 2018; pp. 1465–1470. [Google Scholar]
- Deng, X.; Lambert, S.; Mecrow, B.; Mohamed, M.A.S.; Ullah, S. Winding connection solution for an integrated synchronous motor drive. In Proceedings of the 2017 IEEE International Electric Machines and Drives Conference (IEMDC), Miami, FL, USA, 21–24 May 2017; pp. 1–7. [Google Scholar]
- Mohamed, M.A.S.; Lambert, S.M.; Mecrow, B.C.; Deng, X.; Ullah, S.; Smith, A.C. Integrating the magnetics of an LCL filter into a high speed machine with pre-compressed coils. In Proceedings of the 2017 IEEE International Electric Machines and Drives Conference (IEMDC), Miami, FL, USA, 21–24 May 2017; pp. 1–7. [Google Scholar]
- Deng, X.; Mohamed, M.A.S.; Lambert, S.; Mecrow, B.C. Development of a High-Speed, Permanent Magnet, SiC-Based Drive with Integrated Input Filters. IEEE Trans. Energy Convers. 2020, 35, 863–874. [Google Scholar] [CrossRef]
- Vrankovic, Z.; Wei, L.; Winterhalter, C.; Hong, B.Y. The influence of the DC link inductor design on the rectifier voltage stress in an adjustable speed drive during a mains voltage surge. In Proceedings of the 2009 IEEE Energy Conversion Congress and Exposition, San Jose, CA, USA, 20–24 September 2009; pp. 3653–3659. [Google Scholar]
- Luo, F.; Robutel, R.; Wang, S.; Wang, F.; Boroyevich, D. Integrated Input EMI Filter for a 2 kW DC-fed 3-phase Motor Drive. In Proceedings of the 2009 Twenty-Fourth Annual IEEE Applied Power Electronics Conference and Exposition, Washington, DC, USA, 15–19 February 2009; pp. 325–329. [Google Scholar]
- Rengang, C.; Strydom, J.T.; van Wyk, J.D. Design of planar integrated passive module for zero-voltage-switched asymmetrical half-bridge PWM converter. IEEE Trans. Ind. Appl. 2003, 39, 1648–1655. [Google Scholar] [CrossRef]
- Rengang, C.; Shuo, W.; van Wyk, J.D.; Odendaal, W.G. Integration of EMI filter for distributed power system (DPS) front-end converter. In Proceedings of the IEEE 34th Annual Conference on Power Electronics Specialist, Acapulco, Mexico, 15–19 June 2003; Volume 1, pp. 296–300. [Google Scholar]
- Zhao, L.; Strydom, J.T.; van Wyk, J.D. Wide band modeling of integrated power passive structures: The series resonator. IEEE Trans. Power Electron. 2002, 3, 1283–1288. [Google Scholar]
- Lingyin, Z.; Strydom, J.T.; van Wyk, J.D. An alternative planar multi-cell structure integrated reactive module. In Proceedings of the Conference Record of the 2001 IEEE Industry Applications Conference. 36th IAS Annual Meeting (Cat. No.01CH37248), Chicago, IL, USA, 30 September–4 October 2001; Volume 4, pp. 2217–2223. [Google Scholar]
- Strydom, J.T.; Wyk, J.D.v. Ferreira, J.A. Some limits of integrated LCT modules for resonant converters at 1 MHz. IEEE Trans. Ind. Appl. 1999, 2, 1411–1417. [Google Scholar]
- Stratta, A.; Mouawad, B.; Antonini, M.; Lillo, L.D.; Empringham, L.; Johnson, M.C. Heterogeneous Integration of Magnetic Component Windings on Ceramic Substrates. IEEE J. Emerg. Sel. Top. Power Electron. 2021, 9, 3867–3876. [Google Scholar] [CrossRef]
- Espina, J.; Ahmadi, B.; Empringham, L.; Lillo, L.D.; Johnson, M. Highly-integrated power cell for high-power wide band-gap power converters. In Proceedings of the 2017 IEEE 3rd International Future Energy Electronics Conference and ECCE Asia (IFEEC 2017–ECCE Asia), Kaohsiung, Taiwan, 3–7 June 2017; pp. 146–150. [Google Scholar]
- Mouawad, B.; Espina, J.; Li, J.; Empringham, L.; Johnson, C.M. Novel Silicon Carbide Integrated Power Module for EV application. In Proceedings of the 2018 1st Workshop on Wide Bandgap Power Devices and Applications in Asia (WiPDA Asia), Xi’an, China, 16–18 May 2018; pp. 176–180. [Google Scholar]
- Saeed, R.; Johnson, C.M.; Empringham, L.; Lillo, L.D. High current density air cored Inductors for direct power module integration. In Proceedings of the 2014 16th European Conference on Power Electronics and Applications, Lappeenranta, Finland, 26–28 August 2014; pp. 1–6. [Google Scholar]
- Marz, M.; Schletz, A.; Eckardt, B.; Egelkraut, S.; Rauh, H. Power electronics system integration for electric and hybrid vehicles. In Proceedings of the Integrated Power Electronics Systems (CIPS), 2010 6th International Conference IEEE, Nuremberg, Germanypp, 16–18 March 2010; pp. 1–10. [Google Scholar]
- Mantooth, H.A.; Mojarradi, M.M.; Johnson, R.W. Emerging capabilities in electronics technologies for extreme environments. Part I-High temperature electronics. IEEE Power Electron. Soc. Newsl. 2006, 18, 1054–7230. [Google Scholar]
- Sarjeant, W.J.; Zirnheld, J.; MacDougall, F.W. Capacitors. IEEE Trans. Plasma Sci. 1998, 26, 1368–1392. [Google Scholar] [CrossRef]
- Yaohong, C.; Fuchang, L.; Hua, L. Study on self-healing and lifetime characteristics of metallized film capacitor under high electric field. IEEE Trans. Plasma Sci. 2012, 40, 711–716. [Google Scholar]
- The New High Temperature Dielectric Film for Power Capacitors. 2019. Available online: https://www.birkelbachfilm.de/fileadmin/pdf/DTF_Teonex_HV_0912.pdf (accessed on 6 December 2021).
- Caliari, L.; Bettacchi, P.; Boni, E.; Montanari, D.; Gamberini, A.; Barbieri, L.; Bergamaschi, F. KEMET film capacitors for high temperature, high voltage and high current. In Proceedings of the Carts International Proceedings-ECA 2013, Houston, TX, USA, 25–28 March 2013. [Google Scholar]
- Both, J. Electrolytic capacitors from the postwar period to the present. IEEE Electr. Insul. Mag. 2016, 32, 8–26. [Google Scholar] [CrossRef]
- Deshpande, R.P. Capacitors: Technology and Trends; Tata McGraw-Hill Education: New York, NY, USA, 2012. [Google Scholar]
- Bramoulle, M. Electrolytic or film capacitors. In Proceedings of the Industry Applications Conference, Thirty-Third IAS Annual Meeting, St. Louis, MO, USA, 12–15 October 1998; pp. 1138–1142. [Google Scholar]
- Parler, S.G., Jr. Deriving life multipliers for electrolytic capacitors. IEEE Power Electron. Soc. Newsl. 2004, 16, 11–12. [Google Scholar]
- Maddula, S.K.; Balda, J.C. Lifetime of electrolytic capacitors in regenerative induction motor drives. In Proceedings of the Power Electronics Specialists Conference, Dresden, Germany, 16 June 2005; pp. 153–159. [Google Scholar]
- Spanik, P.; Frivaldsky, M.; Kanovsky, A. Life time of the electrolytic capacitors in power applications. In Proceedings of the ELEKTRO, Rajecke Teplice, Slovakia, 19–20 May 2014; pp. 233–238. [Google Scholar]
- Randall, M.; Skamser, D.; Kinard, T. Thin film MLCC. In Proceedings of the CARTS 2007 Symposium, Albuquerque, NM, USA, 26–29 March 2007; pp. 1–12. [Google Scholar]
- Takamizawa, H.; Utsumi, K.; Yonezawa, M.; Ohno, T. Large capacitance multilayer ceramic capacitor. IEEE Trans. Compon. Hybrids Manuf. Technol. 1981, 4, 345–349. [Google Scholar] [CrossRef]
- Pan, M.J.; Randall, C.A. A brief introduction to ceramic capacitors. Electr. Insul. Mag. 2010, 26, 44–50. [Google Scholar] [CrossRef]
- Yano Research Institute. Capacitor Market in Japan: Key Research Findings 2009; Yano Research Institute: Tokyo, Japan, 2009. [Google Scholar]
- Phillips, R.; Bultitude, J.; Gurav, A.; Park, K.; Murillo, S.; Flores, P.; Laps, K. High temperature ceramic capacitors for deep well applications. In Proceedings of the CARTS International Proceedings, Houston, TX, USA, 25–28 March 2013. [Google Scholar]
- Kim, W.; Shin, S.H.; Ryu, D.S. Reliability evaluation and failure analysis for high voltage ceramic capacitor. In Proceedings of the Advances in Electronic Materials and Packaging, Jeju Island, Korea, 19–22 November 2001; pp. 195–286. [Google Scholar]
- Ahmar, A.J.; Wiese, S. Fracture mechanics analysis of cracks in multilayer ceramic capacitors. In Proceedings of the Electronics System-Integration Technology Conference (ESTC), Helsinki, Finland, 16–18 September 2014; pp. 1–15. [Google Scholar]
- Teverovsky, A. Insulation resistance and leakage currents in low-voltage ceramic capacitors with cracks. IEEE Trans. Compon. Packag. Manuf. Technol. 2014, 4, 1169–1176. [Google Scholar] [CrossRef]
- Konrad, J.; Koini, M.; Schossmann, M.; Puff, M. New demands in DC link power capacitors. In Proceedings of the Congress on Automotive Electronic Systems, Deutschlandsberg, Austria, 3–4 December 2014. [Google Scholar]
- Wang, H.; Blaabjerg, F. Reliability of Capacitors for DC-Link Applications in Power Electronic Converters—An Overview. IEEE Trans. Ind. Appl. 2014, 50, 3569–3578. [Google Scholar] [CrossRef] [Green Version]
- Ceramic Capacitor Technology-Ceralink Opens New Dimensions in Power Electronics. Available online: https://www.tdk-electronics.tdk.com/download/1195592/d62195f9eeeeff9a067709c53988aa25/ceralink-presentation.pdf (accessed on 6 December 2021).
- Conway, B. Electrochemical Supercapacitors: Scientific Fundamentals and Technological Applications; Springer: Boston, MA, USA, 1999. [Google Scholar]
- Sharma, P.; Bhatti, T. A review on electrochemical double-layer capacitors. Energy Convers. Manag. 2010, 51, 2901–2912. [Google Scholar] [CrossRef]
- Torregrossa, D.; Toghill, K.E.; Girault, H.H.; Paolone, M. Understanding the ageing process, recovering phase and fault diagnosis of electrochemical double layer capacitors. In Proceedings of the 2014 IEEE Conference on Technologies for Sustainability (SusTech), Portland, OR, USA, 24–26 July 2014; pp. 239–244. [Google Scholar]
- Chen, H.; Cong, T.N.; Yang, W.; Tan, C.; Li, Y.; Ding, Y. Progress in electrical energy storage system: A critical review. Prog. Nat. Sci. 2009, 19, 291–312. [Google Scholar] [CrossRef]

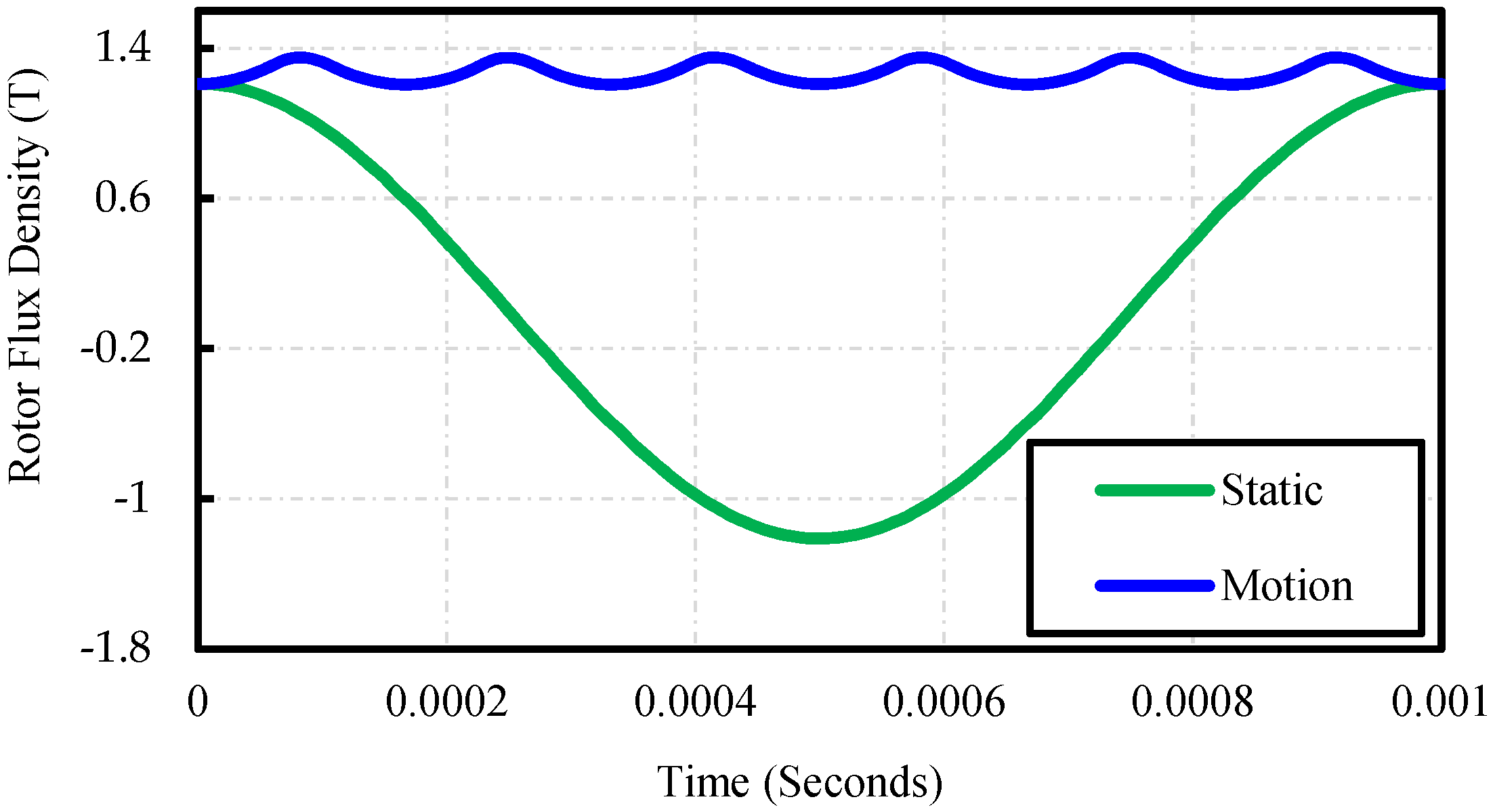
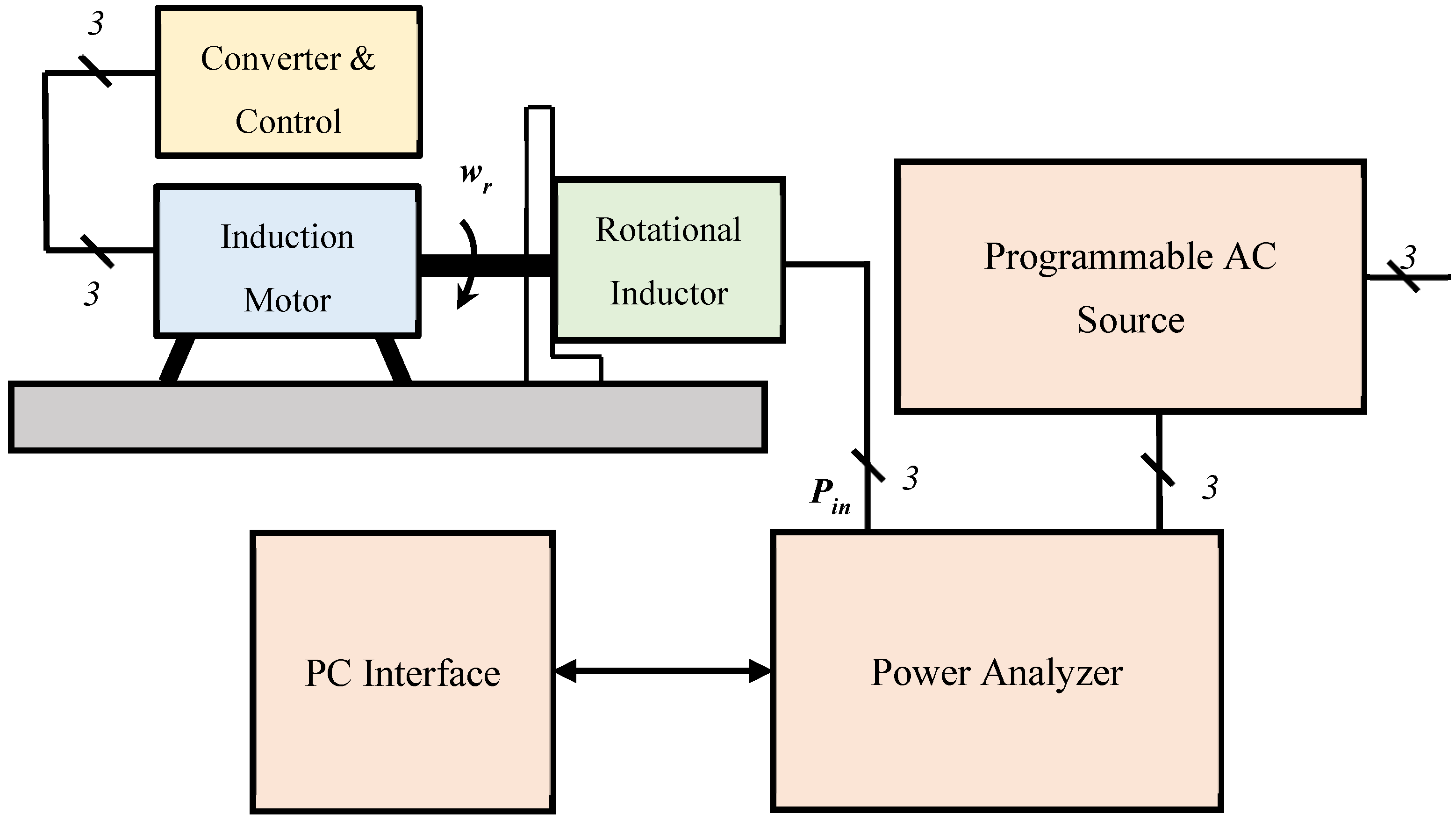

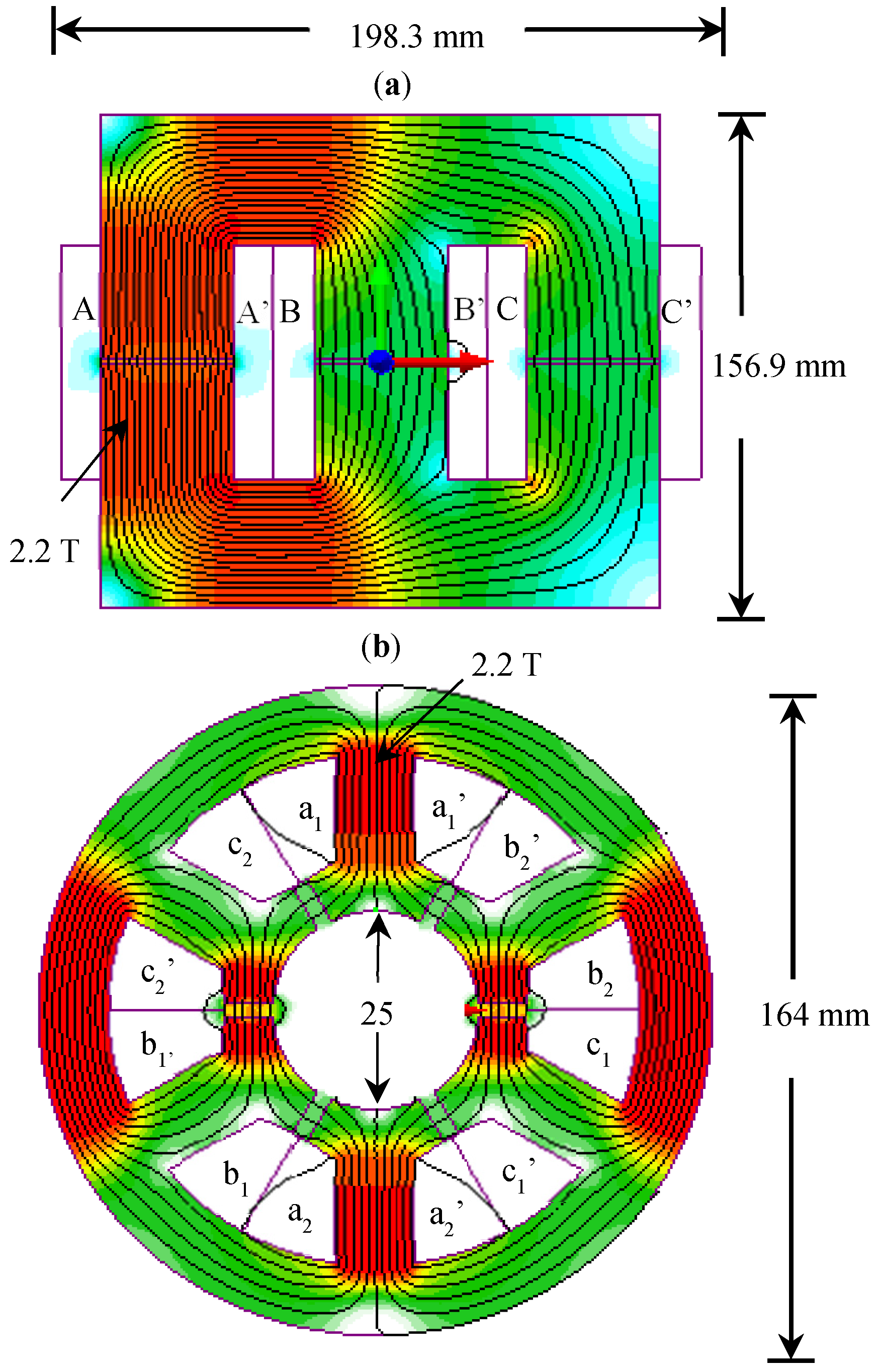

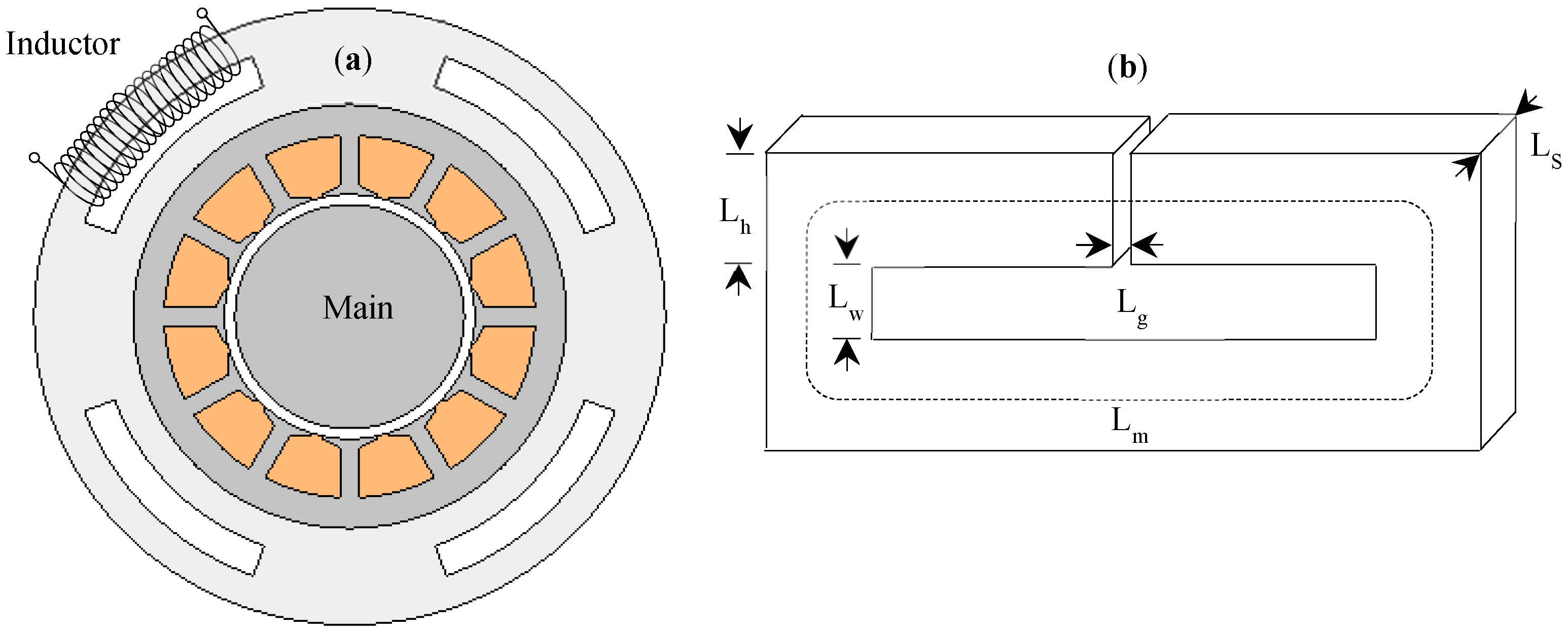


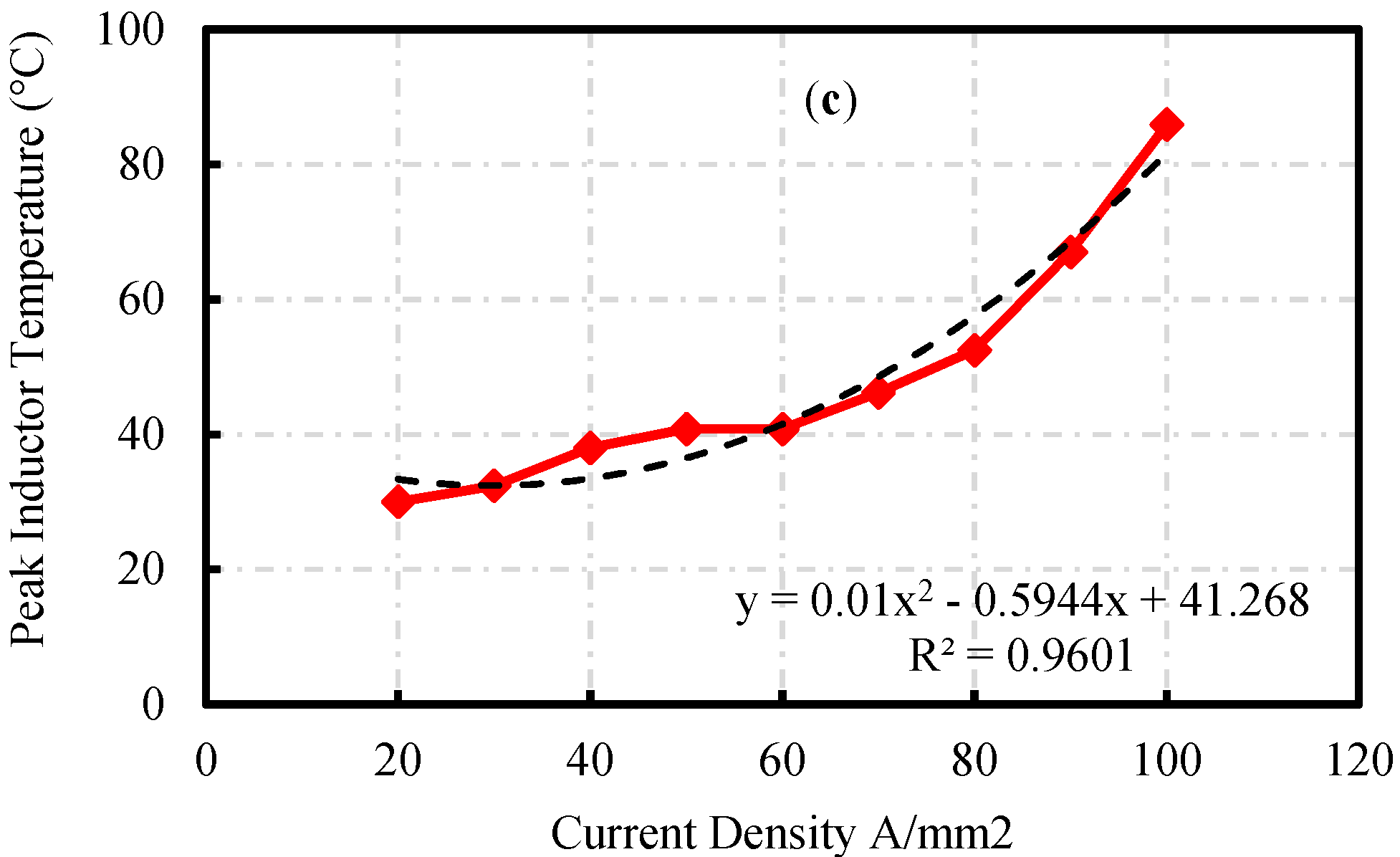
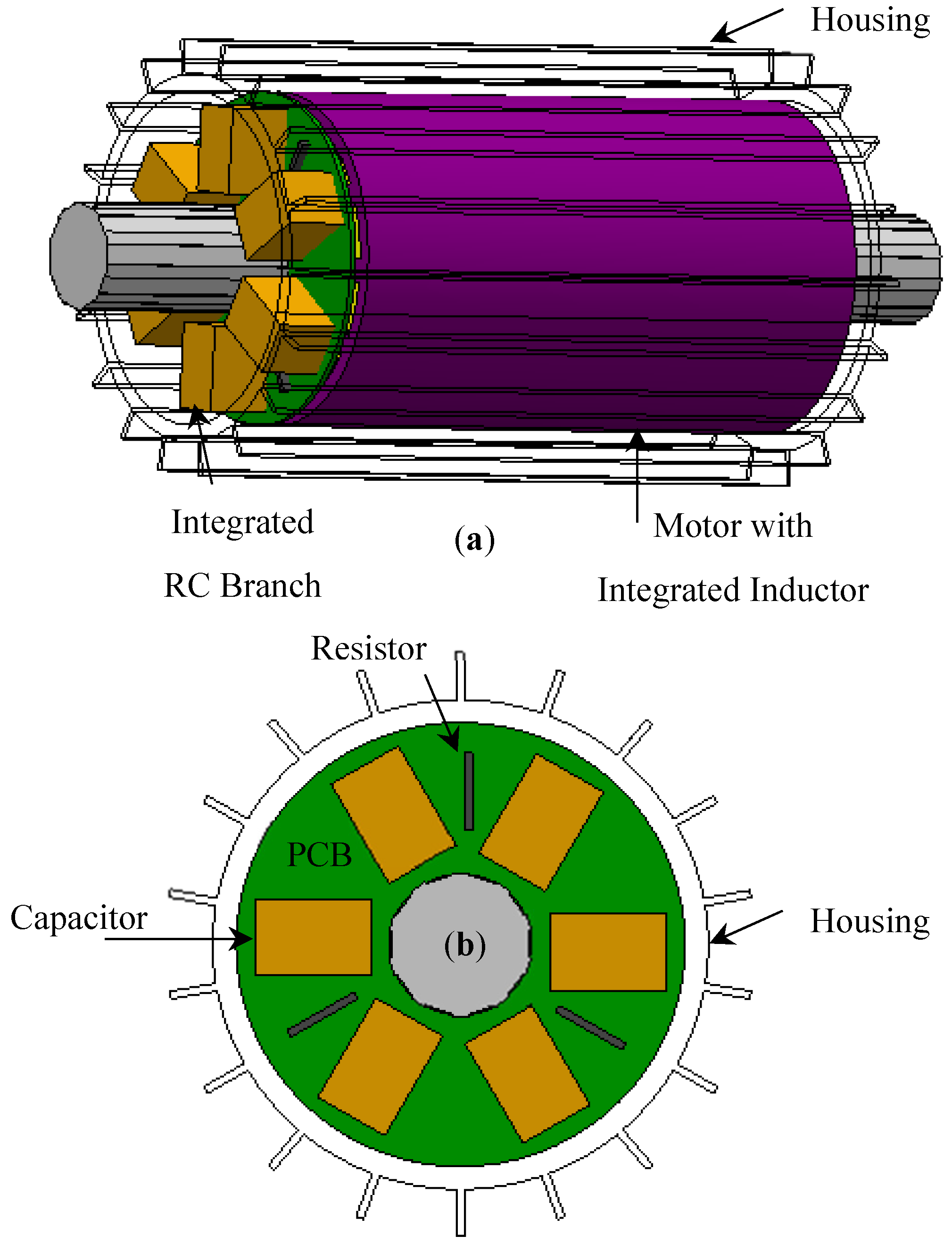
| MOTOR WITH | Motor Power Losses (W) | External Inductor Losses (W) | Total System Losses (W) | External Inductor Mass (kg) | External Inductor Volume (cm3) |
|---|---|---|---|---|---|
| Integrated Inductor | 36.0 | 0 | 36.0 | 0 | 0 |
| External Inductor | 26.1 | 40.67 | 66.77 | 1.52 | 338.7 |
| MOTOR WITH | Average Torque (Nm) | Torque Ripple (%) at Tload = 1.5 Nm | Torque Ripple (%) at Tload = 3 Nm | Torque Ripple (%) at Tload = 4.5 Nm |
|---|---|---|---|---|
| No Filter | 1.49 | 9.54 | 5.33 | 4.2 |
| Integrated Inductor | 1.485 | 9.54 | 5.33 | 4.2 |
| External Inductor | 1.49 | 0.7 | 0.574 | 0.54 |
| Supply Frequency (kHz) | Rotor Speed (rpm) | EE-Core Inductor (W/mH) | Rotational Inductor (W/mH) | Total Loss Reduction (%) |
|---|---|---|---|---|
| 0.10 | 6000 | 30 | 32 | −6.7 |
| 0.20 | 12,000 | 45 | 41 | 8.9 |
| 0.30 | 18,000 | 66 | 51 | 22.7 |
| Sizing Parameters | EE-Core Inductor | Integrated Inductor | Starter-Generator |
|---|---|---|---|
| Total Losses | 0.658 kW | 1.57 kW | 2.31 kW |
| Total Volume | 2078 cm3 | 981 cm3 | 1689 cm3 |
| Total Weight | 9.94 kg | 4.43 kg | 11.7 kg |
| Combined (SG + Inductor) Volume | 3767 cm3 | 2670 cm3 | - |
| Combined (SG + Inductor) Weight | 21.6 kg | 16.1 kg | - |
| Percent Reduction in Volume (Combined System) | - | 29.1% | - |
| Percent Reduction in Weight (Combined System) | - | 25.5% | - |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khowja, M.R.; Abebe, R.; Vakil, G.; Walker, A.; Patel, C.; Gerada, C.; Bobba, P.B.; Cascella, G.L. Review on the Traditional and Integrated Passives: State-of-the-Art Design and Technologies. Energies 2022, 15, 88. https://doi.org/10.3390/en15010088
Khowja MR, Abebe R, Vakil G, Walker A, Patel C, Gerada C, Bobba PB, Cascella GL. Review on the Traditional and Integrated Passives: State-of-the-Art Design and Technologies. Energies. 2022; 15(1):88. https://doi.org/10.3390/en15010088
Chicago/Turabian StyleKhowja, Muhammad Raza, Robert Abebe, Gaurang Vakil, Adam Walker, Chintan Patel, Chris Gerada, Phaneendra Babu Bobba, and Giuseppe Leonardo Cascella. 2022. "Review on the Traditional and Integrated Passives: State-of-the-Art Design and Technologies" Energies 15, no. 1: 88. https://doi.org/10.3390/en15010088
APA StyleKhowja, M. R., Abebe, R., Vakil, G., Walker, A., Patel, C., Gerada, C., Bobba, P. B., & Cascella, G. L. (2022). Review on the Traditional and Integrated Passives: State-of-the-Art Design and Technologies. Energies, 15(1), 88. https://doi.org/10.3390/en15010088








