Synthetic Approach to the Design, Manufacturing and Examination of Gerotor and Orbital Hydraulic Machines
Abstract
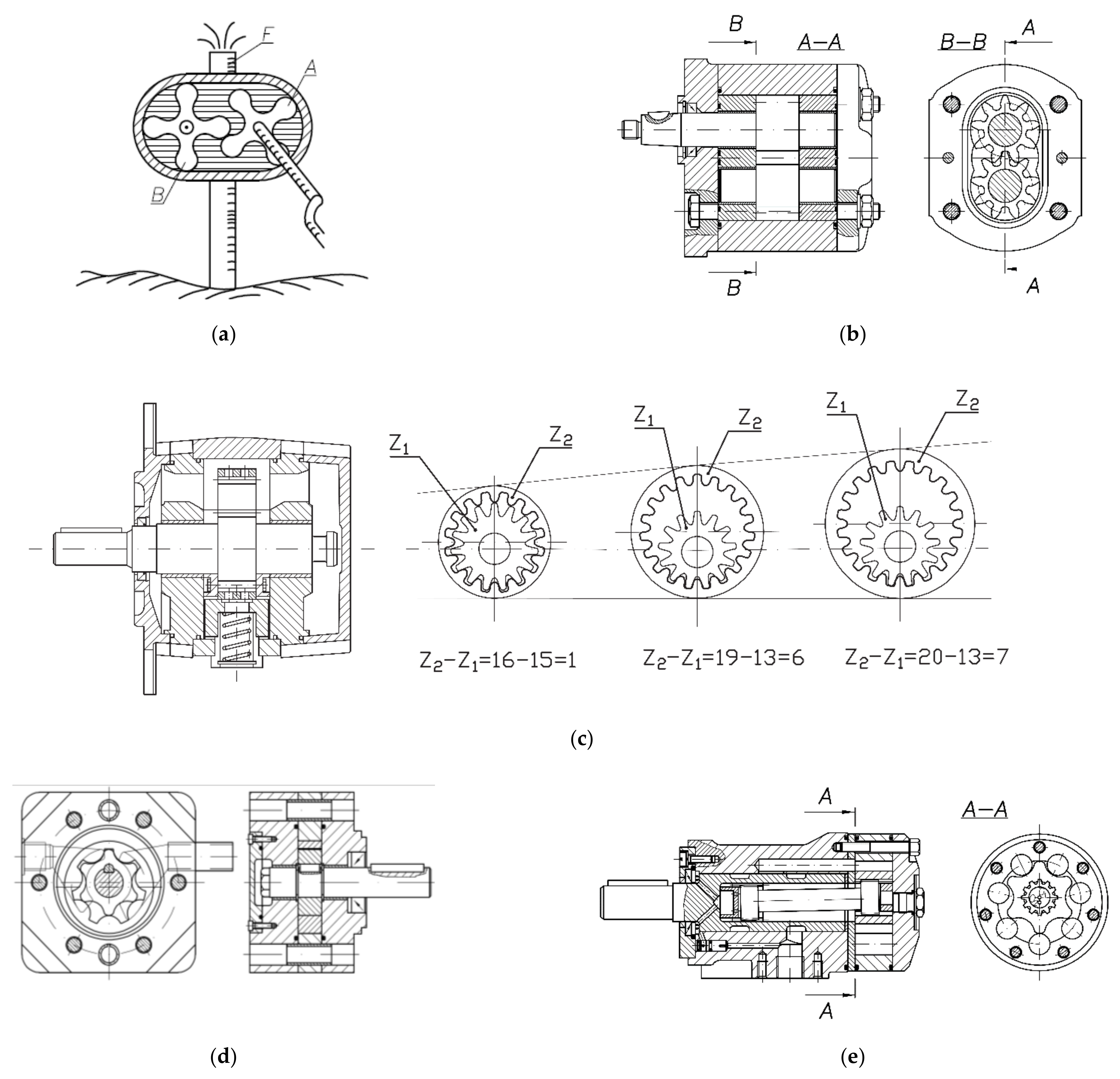
1. Introduction—From the Kepler Pump to the Gerotor and Orbital Machines. Aims of the Paper
- The analysis of epitrochoidal curves and methods of constructing outlines of gerotor wheels [15];
- The comparison of the operation principles of gerotor and orbital machines [14];
- The history of gerotor and orbital machines’ development in the USA [16];
- The determination of forces and torques in the gearing of gerotor pumps [23];
- The review of publications on gerotor technology and setting development trends in this field [29].
- Fundamentals of the geometry and kinematics of gerotor and orbital gears;
- Hydraulics of gerotor and orbital machines;
- Principles of manufacturing gerotor and orbital gear systems;
- Methods of examining gerotor and orbital gear systems and machines.
- Development of the theoretical foundations of gerotor and orbital gearing, including geometry and kinematics;
- Development of the basics of hydraulics for gerotor and orbital machines, including equations defining the delivery (capacity), delivery pulsation and design of the internal channels and clearances of those machines;
- Development of methods of producing gerotor and orbital systems;
- Development of simulation and experimental methods of examination of gerotor and orbital machines.
2. Geometry and Kinematics of Gerotor and Orbital Gears
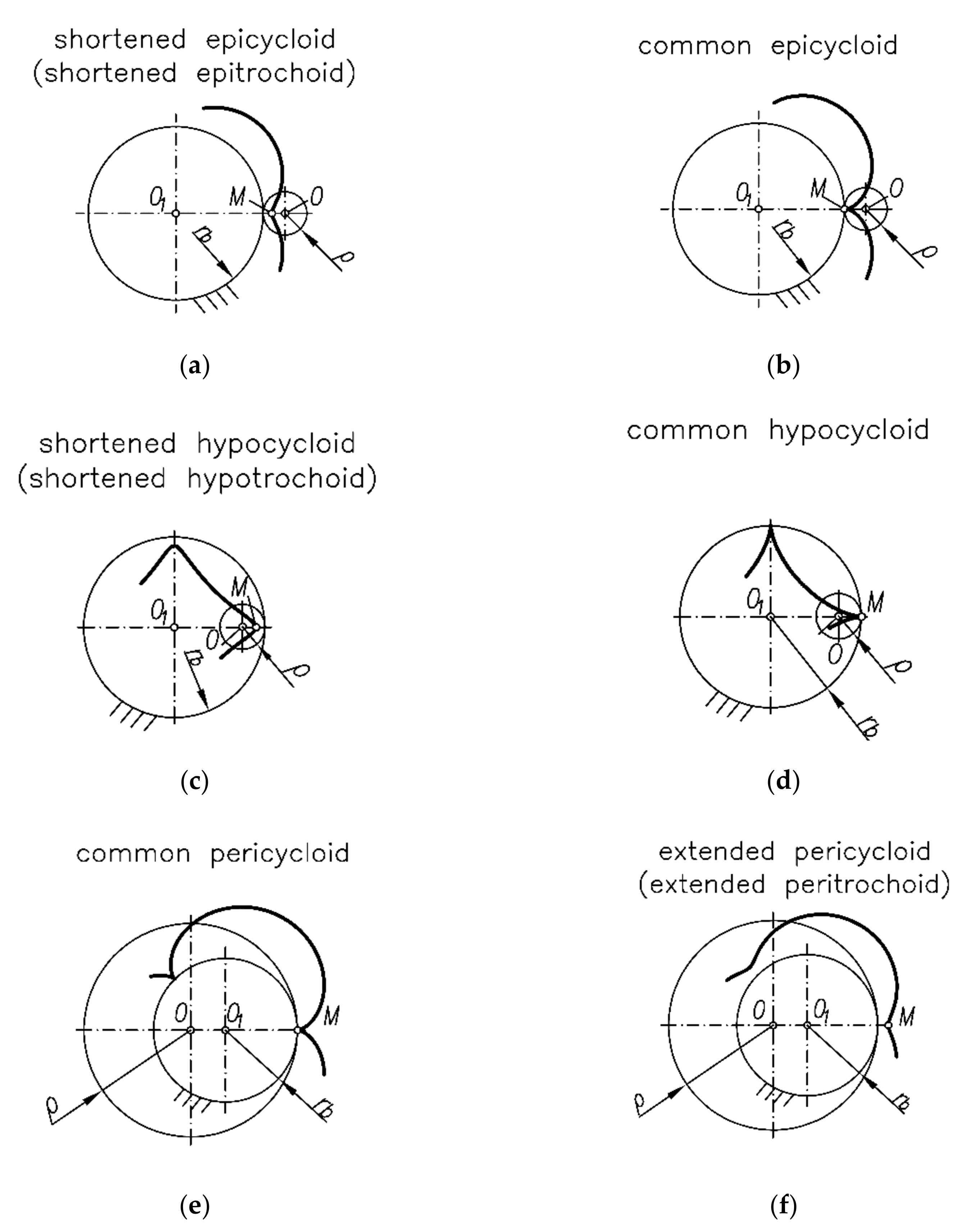
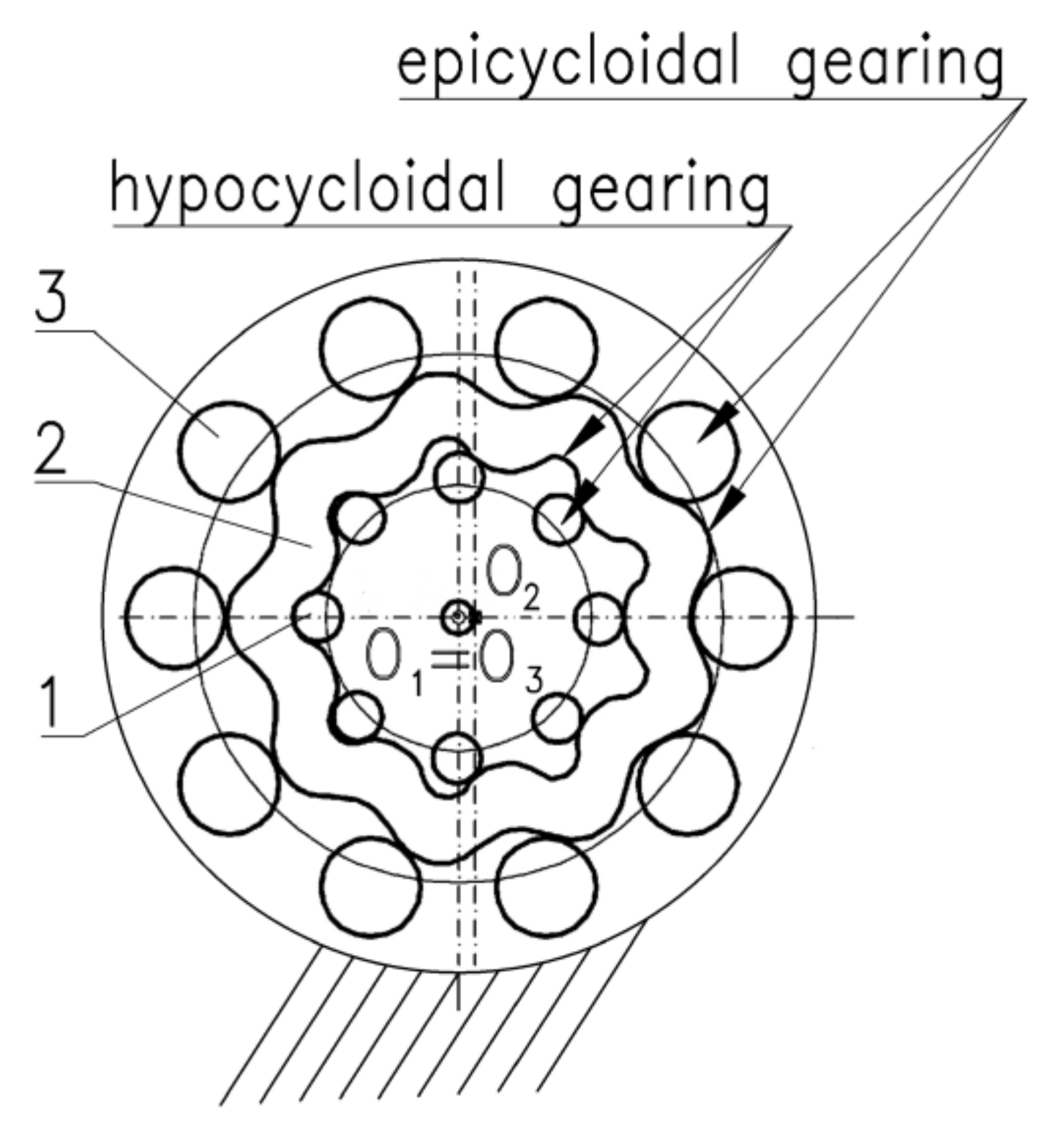
2.1. Cycloidal Curves and Cycloidal Gears
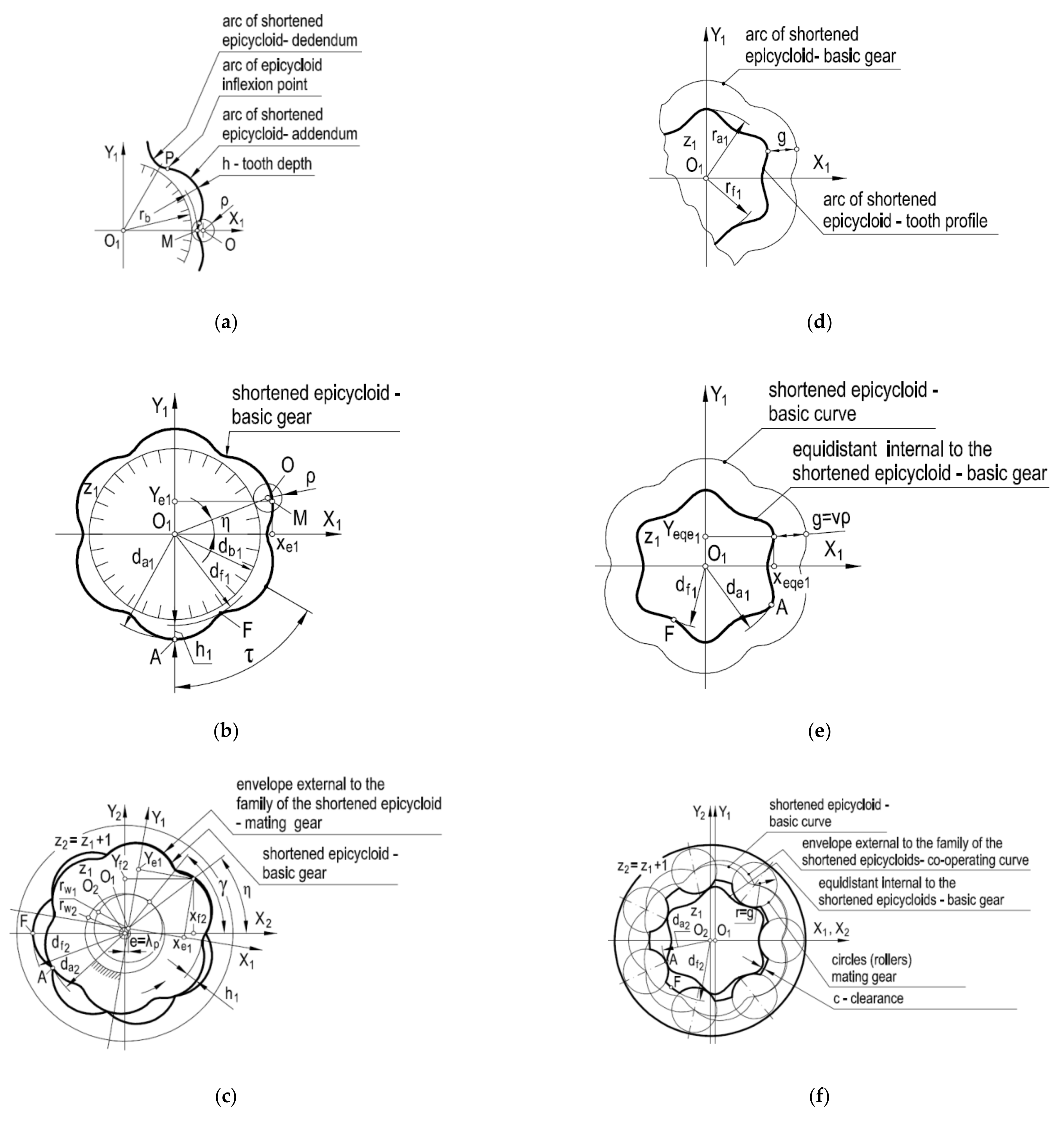
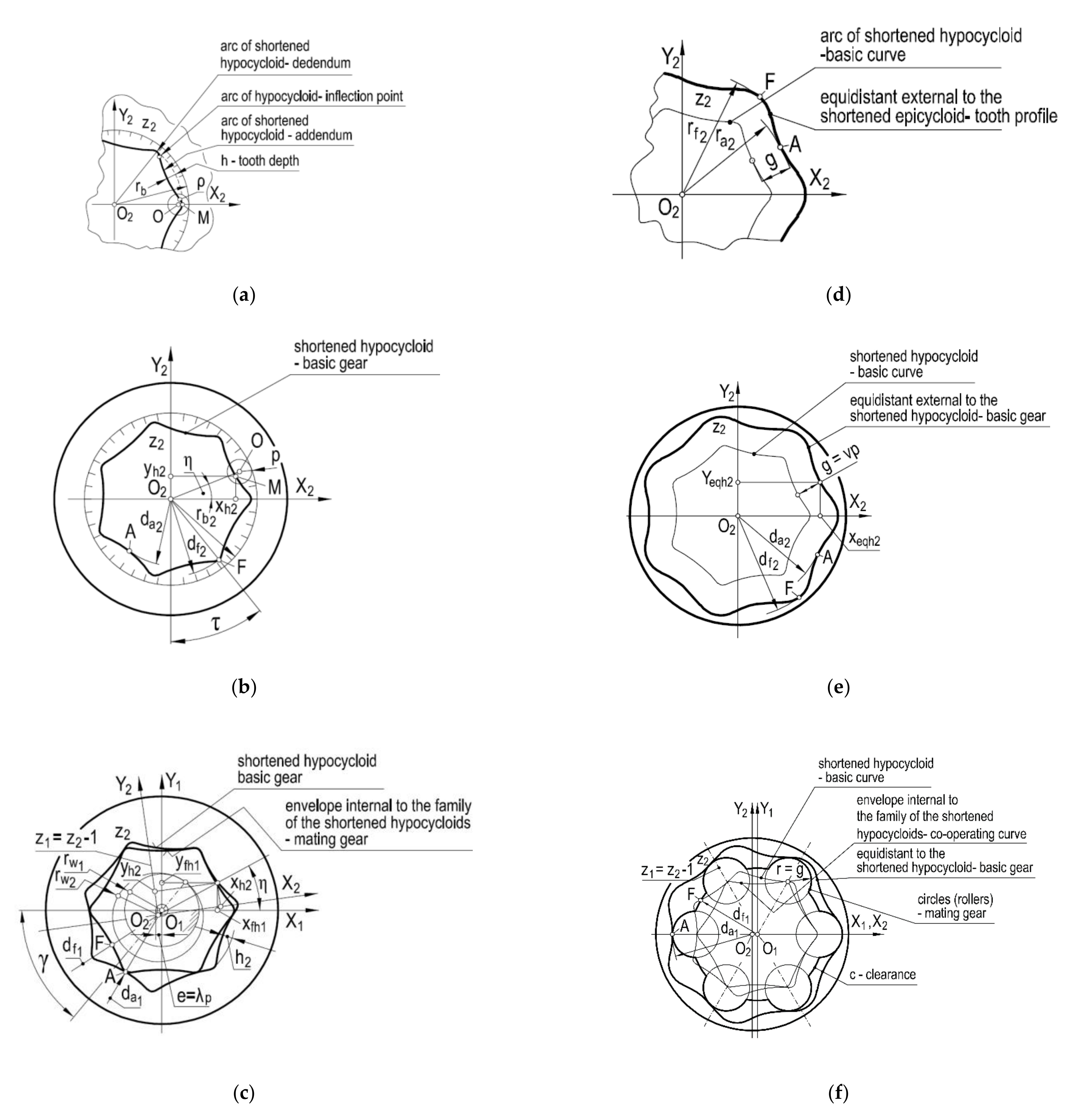
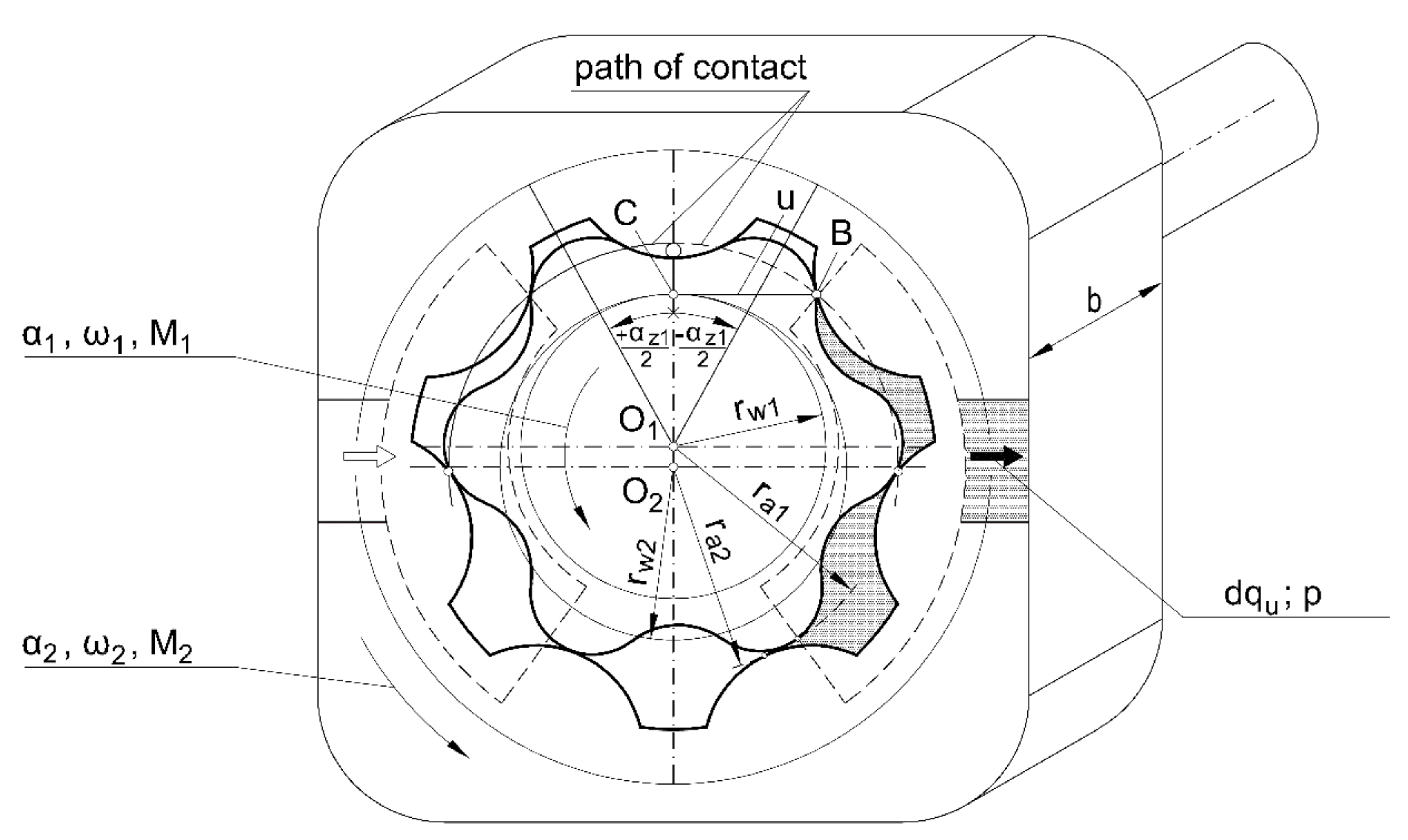
2.2. Parameters of the Cycloidal (Epicycloidal and Hypocycloidal) Teeth and Gearing
- z—the number of teeth equal to the number of arcs of the epicycloid or hypocycloid found in the entire closed cycloidal curve;
- m—a module which, according to the principle of drawing cycloidal curves, is equal to the diameter of the movable gear ρ, i.e., m = 2ρ (Figure 4a);
- η—cycloidal curve angle (Figure 4b);
- v—cycloidal range correction factor;
- b—tooth width.
2.3. Teeth, Gears and Epicycloidal Gear Systems
- Equations (7) and (8) for determining the profile of the internal gear are difficult to use directly;
- The teeth of both gears have disturbed proportions between the head and the root, and the intertooth pressure may be too high (Figure 4c);
- Manufacturing of an internal gear of a complicated profile is difficult and expensive.
- Equations which are simpler and easier to use in the design process;
- More proportionate teeth and gears;
- Manufacture which is considerably simpler and cheaper than the manufacture of the uncorrected gears, especially in the case of the internal mating gear.
2.4. Teeth, Gears and Hypocycloidal Gear Systems
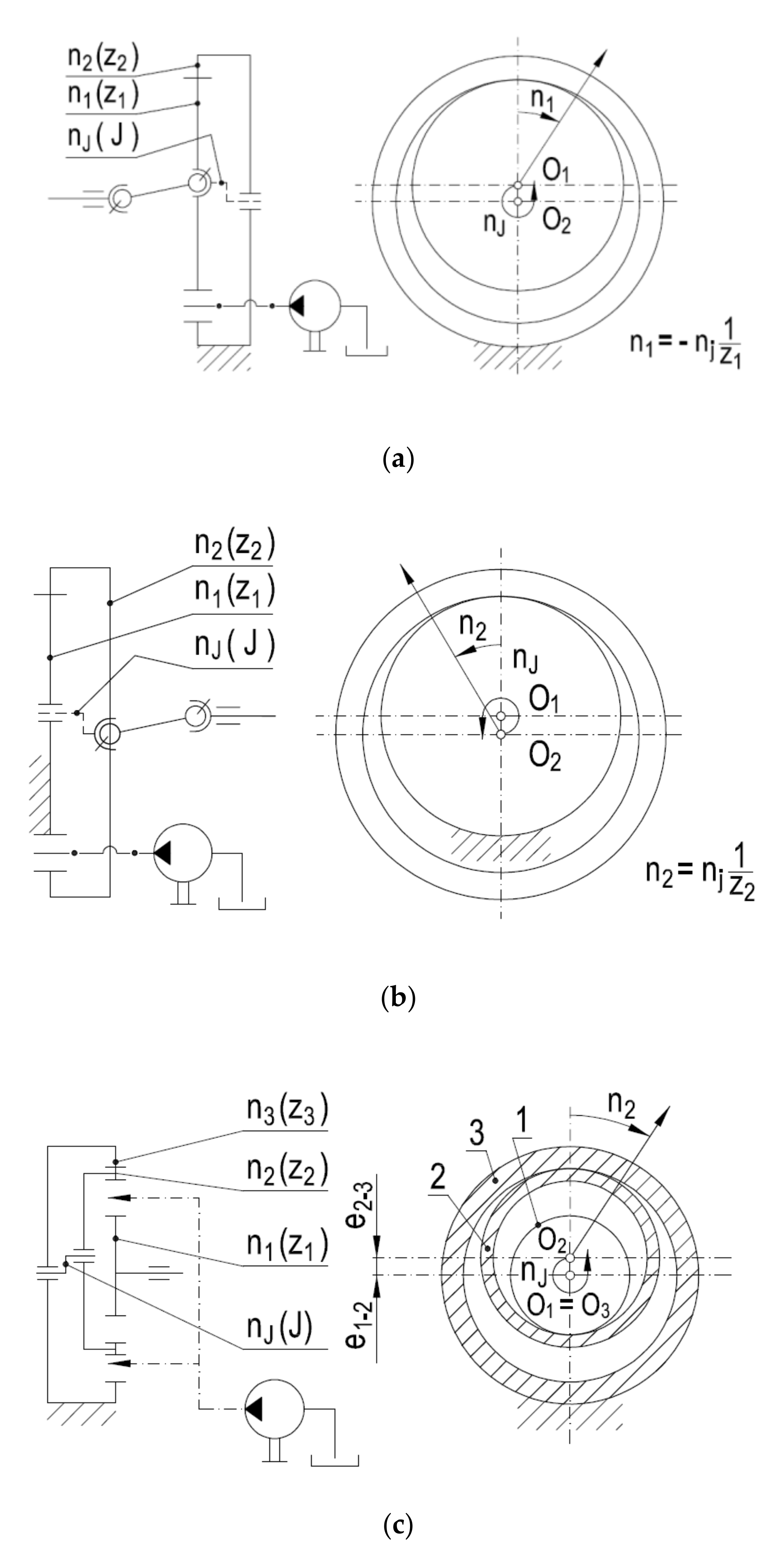
2.5. Kinematics of Gerotor and Orbital Gears
3. Hydraulics of Gerotor and Orbital Machines
3.1. Delivery (Capacity) and Delivery Pulsation (Capacity) of Gerotor and Orbital Machines
- M1, M2—torques on the active and passive gears, respectively;
- Dα1, dα2—angles of rotation of the active and passive gears, respectively;
- qu—elementary volume delivered at the gear rotation by angles dα1 and dα2;
- Δp—pressure difference on the inlet and outlet of the pump, approximately equal to the delivery pressure Δp = p.
- qorb—delivery (capacity) of the orbital machine;
- q(e, h)—delivery (capacity) of the gerotor machine calculated according to Equation (31) or (32);
- n1 = z1 or z2—number of rotations of the driving gear per one revolution of the drive shaft.
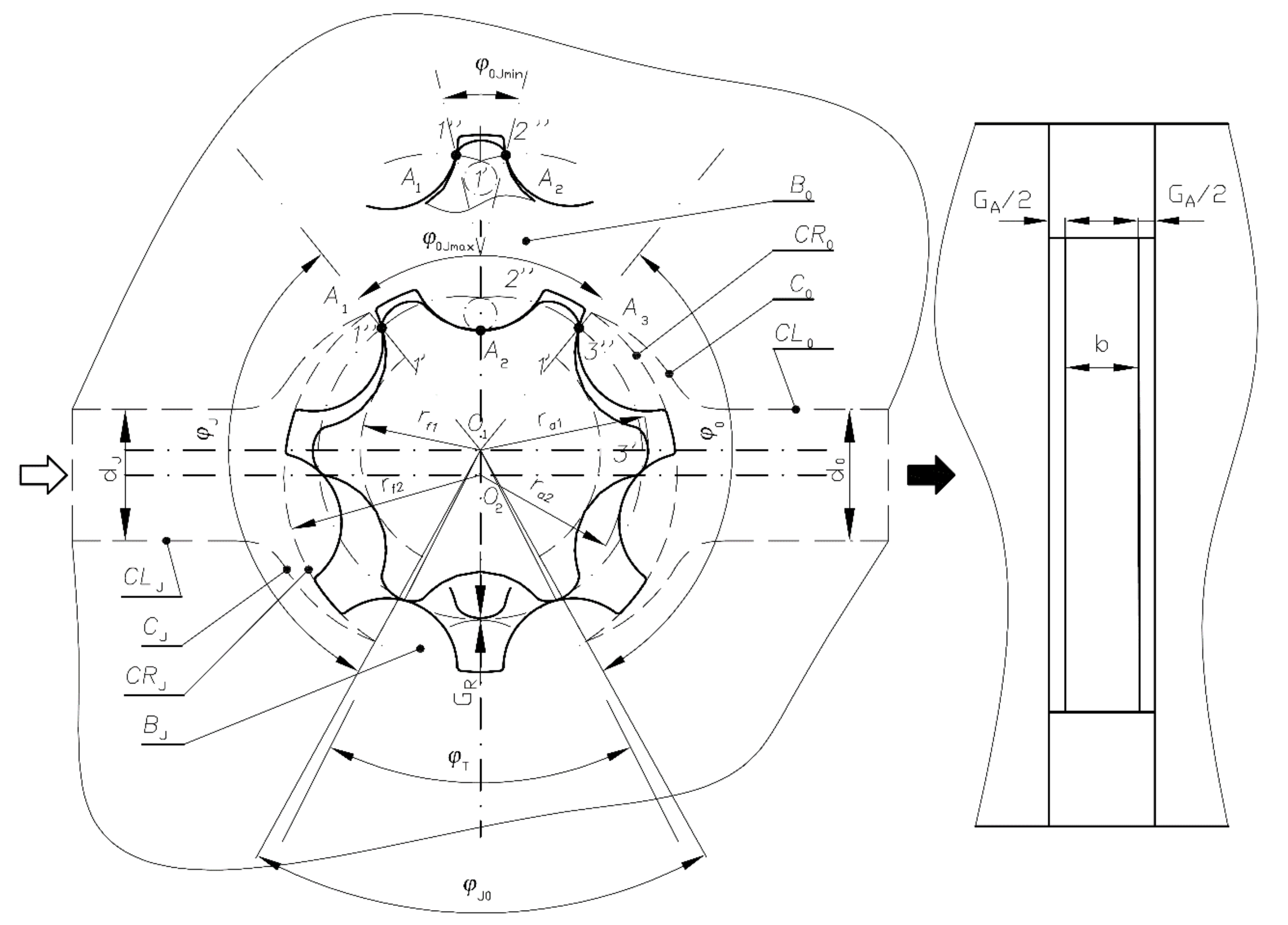
3.2. Shaping the Arrangement of Internal Channels and Clearances in Gerotor and Orbital Machines
- System of fixed channels;
- System of movable channels.
- Inlet channel CLI, inlet chamber CRI and inlet bridge BI;
- Outlet channel CLO, outlet chamber CRO and outlet bridge BO;
- Axial clearance GA;
- Radial clearance GR.
- A system of channels built on the basis of a rotary distributor, in which the channels and clearances are formed between a rotating shaft (sleeve) and a fixed housing;
- A system of channels made using a disc distributor, in which the channels and clearances are formed between the rotating and the fixed disc of the distributor.
4. Manufacturing of the Gerotor and Orbital Gear Systems
- The method in which the path of the universal tool maps the profile of the gearing;
- The method in which the shape of the cutting edge of a special tool copies the form of the gearing.
5. Examination of Gerotor and Orbital Machines
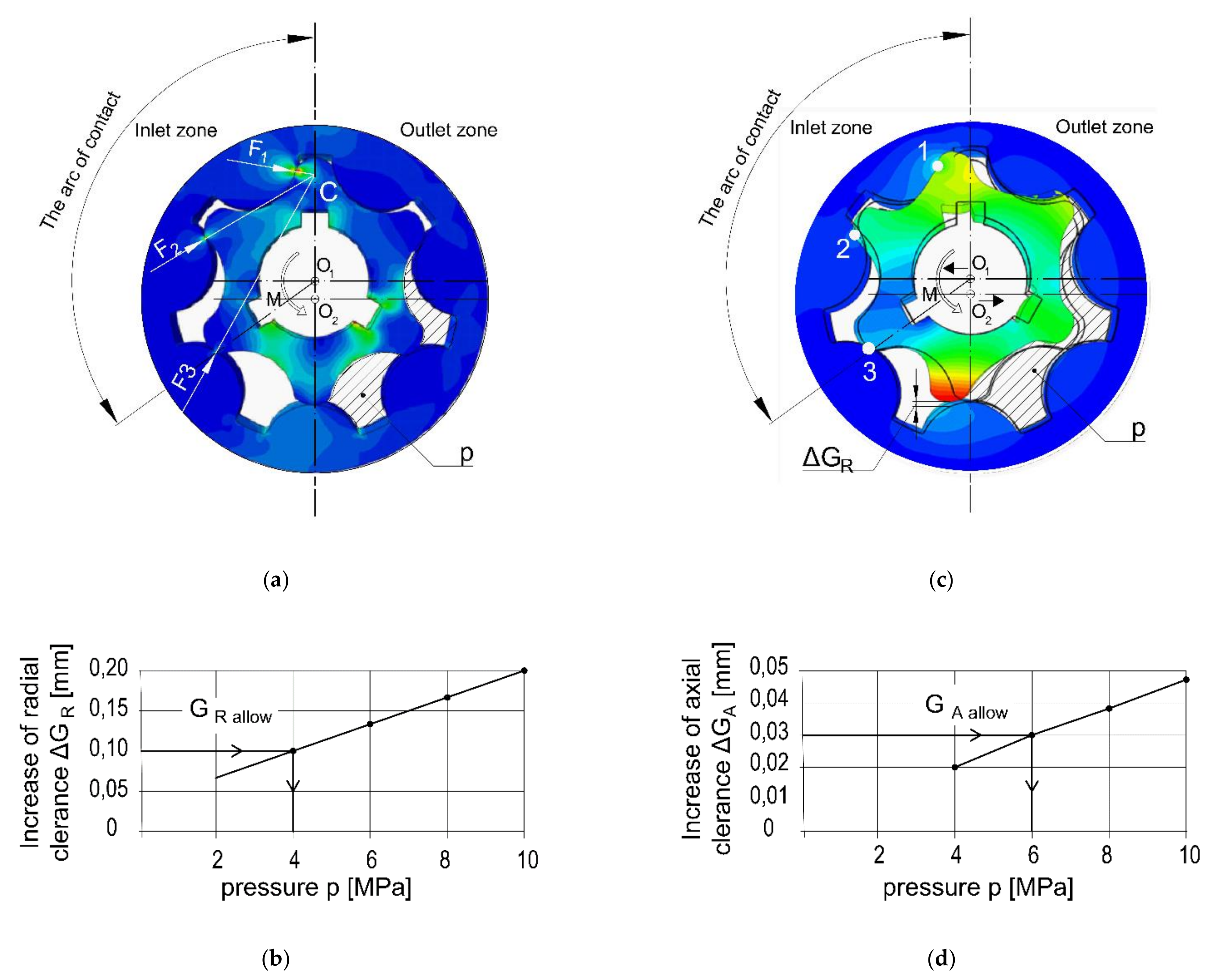
5.1. Forecasting of the Axial and Radial Clearances and Working Pressures in a Pump with Gerotor Wheels Made of POM
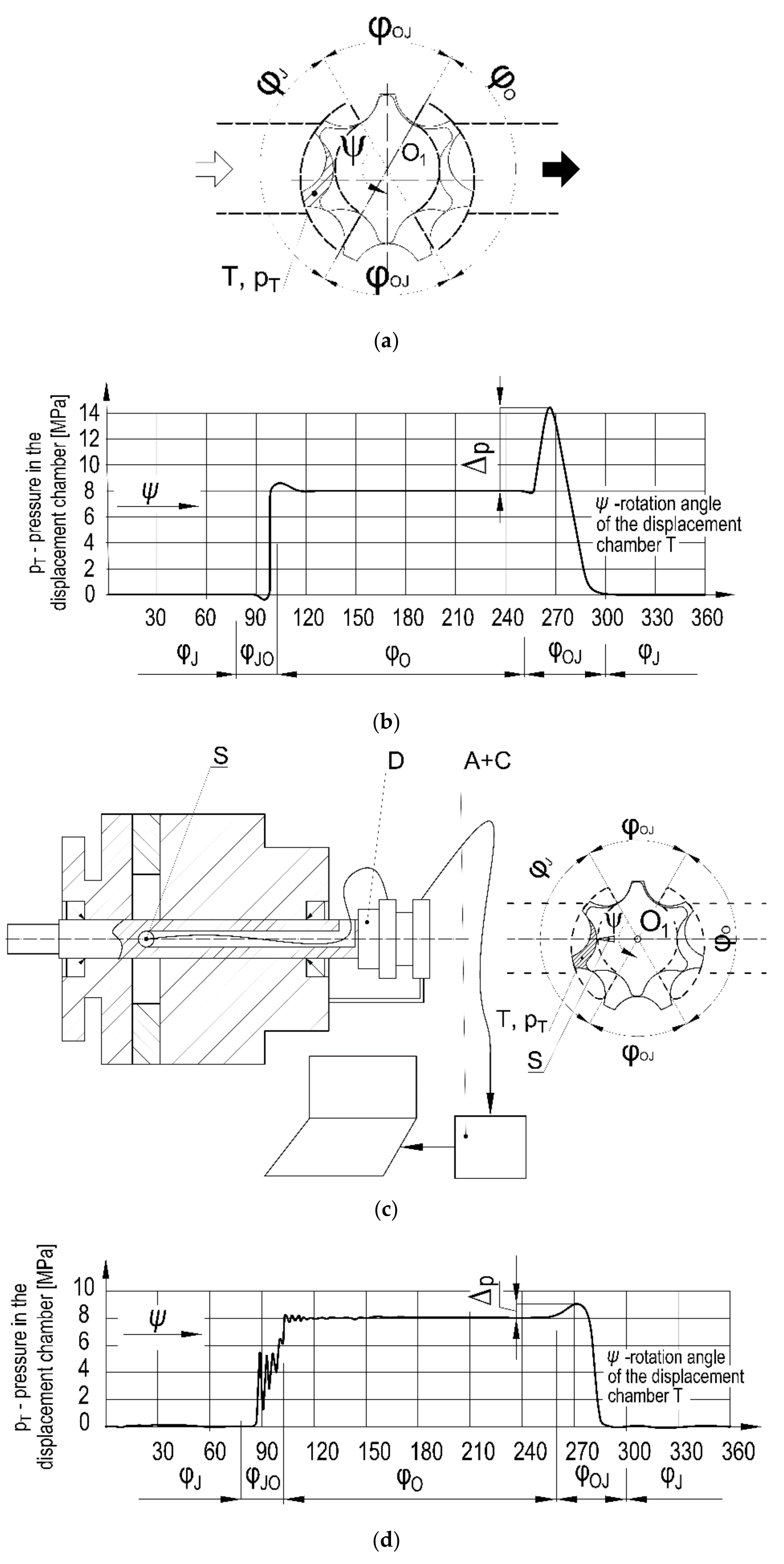
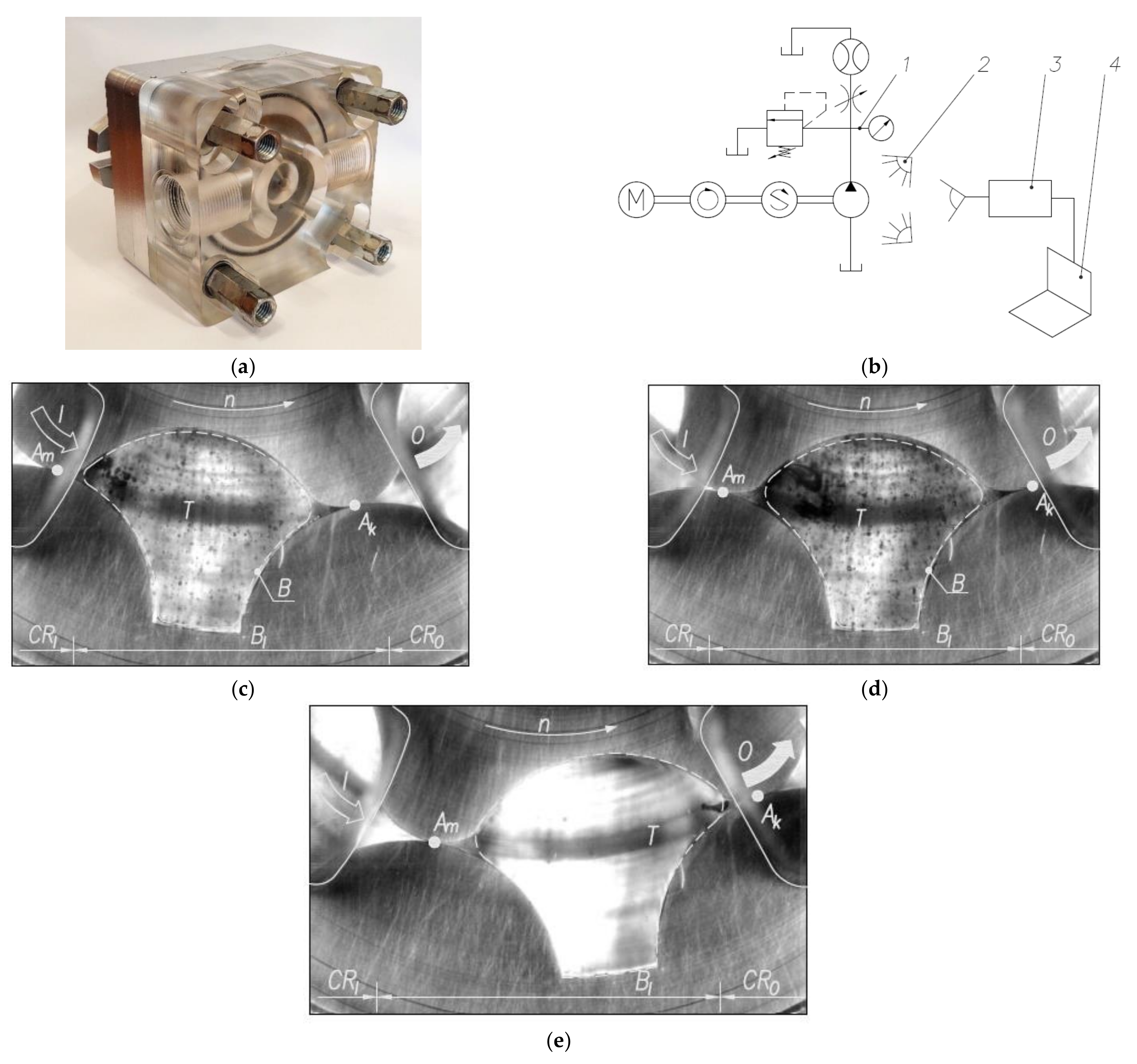
5.2. Research into the Hydraulic Processes and Phenomena in the Internal Channels and Clearances of the Gerotor Pump
- pt—pressure in the displacement chamber T;
- φ—angle determining the location of the displacement chamber T;
- VT—displacement chamber volume;
- QG—main flow rate;
- QTI—leakage flow rate on the way: displacement chamber–inlet chamber (suction chamber);
- QOT—leakage flow rate on the way: outlet chamber (discharge)–displacement chamber.
6. Conclusions
- A uniform system of parameters and basic concepts regarding toothing and cycloidal gearing (z, m, λ, v, g) which is consistently used to describe the geometry, kinematics, hydraulics and manufacture of those elements; that system is compatible with the system applied for involute gearing, which allows for comparing the cycloidal and involute gears;
- Description of the geometry and kinematics of epicycloidal and hypocycloidal gears with the use of the adopted parameters system. Additionally, epicycloidal/hypocycloidal double gearing is presented, which is an original idea of the authors;
- Description of the hydraulics of gerotor and orbital machines, and in particular,
- Determination of equations for delivery (capacity) q and irregularity of delivery (capacity) Δ using the above-mentioned system of basic parameters;
- Formulation of the principles of designing internal channels and clearances in gerotor machines and presentation of the original disc distributor in the epicycloidal/hypocycloidal orbital motor;
- Presentation of the methods of manufacturing epicycloidal and hypocycloidal gearings and of 12 examples of the systems implemented in practice, which proves the above-mentioned theoretical formulas to be correct and useful;
- Presentation of the research methods applied for the examination of the gerotor machines, combining computer simulation and experimental research into a coherent and cohesive whole, which results in the effect of research synergy. That concerned:
- Methods of modeling axial and radial clearances in gerotor assemblies using FEM and experimental tests;
- Methods of studying the hydraulic processes and phenomena in the gerotor pump using the authors’ own computer program as well as the pressure analysis and the PIV visualization analysis.
Author Contributions
Funding
Conflicts of Interest
References
- Abel, F. Johannes Kepler and His Importance to Modern Science; Inter Nationes Verlag: Bonn—Bad Godesberg, Germany, 1971. [Google Scholar]
- Beck, T. Jean Leurechon (1591 bis 1670). Z.d. VDI Bd. 1901, 45, 1498. [Google Scholar]
- Feldhaus, F.M. Die Technik der Vorzeit, der Geschichtlichen Zeit und der Naturvolker; Verlag von W. Engelmann: Leipzig/Berlin, Germany, 1914. [Google Scholar]
- Leupold, J. Teatrum Machinarum Hydrotechnicarum; Christop Zunkel Verlag: Leipzig, Germany, 1724. [Google Scholar]
- Wieczorek, K. PZ4-pompy zębate czwartej generacji. Hydraulika i Pneumatyka 2004, 1, 17–19. (In Polish) [Google Scholar]
- Borghi, M.; Zardin, B. Modelling and simulation of external gear pump and motors, Disussing the Influence of Elastohydrodynamic Lubrication in the Axial Clearance. In Proceedings of the 5th FPNI PhD Symposium, Crakov, Poland, 1–5 July 2008; pp. 323–341. [Google Scholar]
- Pippes, R.; Mayer, C. Clearance-compensated high-pressure internal pumps. In Proceedings of the 3rd International Fluid Power Conference, Aachen, Germany; 2002; pp. 203–214. [Google Scholar]
- Amann, R. Zahnradpumpen mil Evolventen Verzalmung. In Mitteilungen des Hydraulischen Instituts Technischen Hochschule Munchen, Heft 1; Verlag Oldenburg: Munchen, Germany, 1926. [Google Scholar]
- Willekens, F.A.M. Instantes Fordervolumen, geometrisches Hubvolumen und Ungleichformigkeitsgrad von Zahnradpumpen. Industrie-Anzeiger 1971, 26, 549–554. [Google Scholar]
- Gutbrod, W. Forderstrom von Aussen und Innenzahnradpumpen und seine Ungleichfórmigkeit. Olhydraulik und Pneumatik 1975, 2, 236–241. [Google Scholar]
- Gosele, R. Volumenstromschwankungen zykloidisch und aquidistant zykloidisch verzahnter Pumpen. Industrie Anzeiger 1979, 68, 25–26. [Google Scholar]
- Stryczek, J. Pompy zębate o zazębieniu ewolwentowym. Przegląd Mechaniczny 1978, 7, 5–10. (In Polish) [Google Scholar]
- Scholler, K. Die Ring-Rotor-Pumpe ein gerauscharmer Hydrogenerator. Oelhydraulik und Pneumatik 1974, 5, 396–398. [Google Scholar]
- Ohrberg, C.V. Comparaisions entre le moteur gerotor et le moteur orbit. EFL HPA 1969, 7, 11–13. [Google Scholar]
- Nag, A.; Maiti, R. Unification of epitrochoid origin profile design approaches for external lobed star member used in hydrostatic and gear units. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. 2012, 227, 299–310. [Google Scholar] [CrossRef]
- Pippenger, J.J.; Dong, J.X. History of Gerotor Hydraulics; SAE Off-Highway Engineering; SAE: Warrendale, PA, USA, 2004; Volume 2, pp. 40–45. [Google Scholar]
- Birjukow, B.N. Rotarno-Porsniewyje Gidrawlićeskie Masiny; Masinostrojenie: Moscow, Russia, 1972. (In Russian) [Google Scholar]
- Shannikov, V. Planetarnye Reduktory s Vniecentroidnym Ceroinym Zaceplieniem; Masgiz Izdatielstvo: Moscow, Russia, 1948. (In Russian) [Google Scholar]
- Bashta, T.M. Mashinostroitielnaja Gidravlika; Izdatielstvo Mashinostrojenie: Moscow, Russian, 1971. (In Russian) [Google Scholar]
- Stryczek, S. Napęd Hydrostatyczny—Elementy; Wydawnictwa Naukowo—Techniczne: Warsaw, Poland, 1990. (In Polish) [Google Scholar]
- Colbourne, J.R. The geometry of Trochoid Envelopes and Their Application in Rotary Pumps. Mech. Mach. Theory 1974, 4, 421–435. [Google Scholar] [CrossRef]
- Colburne, J.R. Gear Shape and Theoretical Flow Rate in Internal Gear Pumps. Trans. CSME 1975, 3, 215–223. [Google Scholar] [CrossRef]
- Ivanovic, L.; Deredzic, G.; Miric, N.; Cukovic, S. Analysis of forces and torques in gerotor pumps. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. 2010, 224, 2257–2269. [Google Scholar] [CrossRef]
- Castilla, R.; Gamez-Montero, P.; Raush, G.; Codina, E. Method for Fluid Flow Simulation of a Gerotor Pump using Open FOAM. J. Fluid Eng. 2017, 139, 1–9. [Google Scholar] [CrossRef]
- Pellegri, M.; Manne, V.; Vacca, A. A simulation Model of Gerotor Pumps Considering Fluid—Structure Interaction Effects: Formulation and Validation. Mech. Syst. Signal Proc. 2020, 140, 106720. [Google Scholar] [CrossRef]
- Eisenmann, S.A. Neue Trochoideninnenzahnradpumpe mit Geringer Pulsation und extrem ruhigem Lauf. Oelhydraulik und Pneumatik 1973, 4, 302–311. [Google Scholar]
- Wusthof, P.; Schneider, J. Grundlagen der Planetmotoren—Vorstellung eines neuen Planetmotors. Oelhydraulik und Pneumatik 1981, 2, 91–94. [Google Scholar]
- Manco, S.; Narvegna, N.; Rundo, M.; Margaria, M. Miniature gerotor pump prototype for automotive applications. In Proceedings of the 3rd JFK International Fluid Power Conference, Aachen, Germany, 5–6 March 2002; pp. 153–167. [Google Scholar]
- Gamez-Montero, P.; Codina, E.; Castilla, R. A Review of Gerotor Technology in Hydraulic Machines. Energies 2019, 12, 2423. [Google Scholar] [CrossRef]
- Bronsztejn, J.N.; Siemiendiajew, K.A. Matematyka, Poradnik Encyklopedyczny; PWN: Warsaw, Poland, 1970. (In Polish) [Google Scholar]
- Litvin, F.L. Tieorja Zubcatyh Zaceplieni; Izdatielstvo Nauka: Moscow, Russia, 1968. (In Russian) [Google Scholar]
- Gamez-Montero, P.; Antoniak, P.; Castilla, R.; Freire, J.; Krawczyk, J.; Stryczek, J.; Codina, E. Magnet-Sleeve-Sealed Mini Trochoidal-Gear Pump Prototype with Polymer Composite Gear. Energies 2017, 10, 1458. [Google Scholar] [CrossRef]
- Ivanovic, L.; Josifovic, D. Specific Sliding of Trochoidal Gearing Profile in the Gerotor Pumps. FME Trans. 2006, 34, 121–127. [Google Scholar]
- Gamez-Montero, P.J.; Castilla, R.; Khamashta, M.; Codina, E. Contact problems of a trochoidal gear pump. Int. J. Mech. Sci. 2006, 48, 1471–1480. [Google Scholar] [CrossRef]
- Maiti, R.; Sinha, G.L. Kinematics of active contact in modified epitrochoidal generated rotary piston machines. Mech. Mach. Theory 1988, 23, 39–45. [Google Scholar] [CrossRef]
- Nhuan, P.D. O Doborze Parametrów Konstrukcyjnych i Modelowaniu Pewnych Maszyn Hydraulicznych o Zazębieniu Cykloidalnym; Politechnika Warszawska: Warsaw, Poland, 1978. (In Polish) [Google Scholar]
- Lobastov, V.K. K tieorii Vniecentroidnogo Epicykloidalnego Zacieplenia Planietarnych Miechanizmov; Tieoria Miechanizmov i Mashin; Izdatielstvo Charkovskogo Gosudarstviennogo Univieristeta: Mashin, Russia, 1978. (In Russian) [Google Scholar]
- Stryczek, J. Cycloidal Gears in Design of Gear Pumps and Motors. Arch. Masch. Des. 1990, 1–2, 57–88. [Google Scholar]
- Stryczek, J. Principles of the Design of Cycloidal Gears in Hydraulic Machinery. Arch. Masch. Des. 1990, 3, 201–218. [Google Scholar]
- Stryczek, J. Hydraulic Machines with Cycloidal Gearing. Arch. Masch. Des. 1996, 1, 29–72. [Google Scholar]
- Stryczek, J. Projektieren der Zykloidenverzahnungen hydraulischer Verdrangermaschinen. Mech. Mach. Theory J. 1990, 6, 597–610. [Google Scholar] [CrossRef]
- Stryczek, J. Fundamentals of Designing Hydraulic Gear Machines; Polish Scientific Publishers PWN: Warsaw, Poland, 2020. [Google Scholar]
- Stryczek, J. Charakteristische Parameter hydraulischer Verdrangermaschinen mit Zykloidenverzahnungen. Mech. Mach. Theory J. 1993, 1, 97–112. [Google Scholar] [CrossRef]
- Stryczek, J. Koła Zębate Maszyn Hydraulicznych; Oficyna Wydawnicza Politechniki Wrocławskiej: Wroclaw, Poland, 2007. (In Polish) [Google Scholar]
- Bald, C. Hydrauliczna Maszyna Robocza z Obrotowym Tłokiem i Obrotowym Cylindrem. Urząd Patentowy Rzeczypospolitej Polskiej 54,006, 19 October 1992. (In Polish). [Google Scholar]
- Stryczek, J.; Bednarczyk, S.; Biernacki, K. Gerotor pump with POM gears: Design production technology, research. Arch. Civ. Mech. Eng. 2015, 15, 391–397. [Google Scholar] [CrossRef]
- Biernacki, K.; Stryczek, J. Analysis of stress and deformation in plastic gears used in gerotor pumps. J. Strain Anal. Eng. Des. 2010, 45, 465–479. [Google Scholar] [CrossRef]
- Antoniak, P. Kształtowanie Procesów Przepływowych w Rotacyjnych Pompach Wyporowych na Przykładzie Pompy Gerotorowej; Politechnika Wrocławska: Wroclaw, Poland, 2006. (In Polish) [Google Scholar]
- Antoniak, P.; Stryczek, J. Visualisation study of the flow processes and phenomena in the external gear pump. Arch. Civ. Mech. Eng. 2018, 18, 1103–1115. [Google Scholar] [CrossRef]
- Antoniak, P.; Stryczek, J. Hybrydowy Zespół Pompujący. Zgłoszenie Patentowe P428,702, 29 January 2019. (In Polish). [Google Scholar]







Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stryczek, J.; Stryczek, P. Synthetic Approach to the Design, Manufacturing and Examination of Gerotor and Orbital Hydraulic Machines. Energies 2021, 14, 624. https://doi.org/10.3390/en14030624
Stryczek J, Stryczek P. Synthetic Approach to the Design, Manufacturing and Examination of Gerotor and Orbital Hydraulic Machines. Energies. 2021; 14(3):624. https://doi.org/10.3390/en14030624
Chicago/Turabian StyleStryczek, Jarosław, and Piotr Stryczek. 2021. "Synthetic Approach to the Design, Manufacturing and Examination of Gerotor and Orbital Hydraulic Machines" Energies 14, no. 3: 624. https://doi.org/10.3390/en14030624
APA StyleStryczek, J., & Stryczek, P. (2021). Synthetic Approach to the Design, Manufacturing and Examination of Gerotor and Orbital Hydraulic Machines. Energies, 14(3), 624. https://doi.org/10.3390/en14030624




