Convective Drying of Ceramic Bricks by CFD: Transport Phenomena and Process Parameters Analysis
Abstract
1. Introduction
- (a)
- The practicality of observing the temperature and moisture gradients, inside the material as it dries;
- (b)
- The possibility of quantifying heat and mass fluxes inside the material over time and space: then, it is possible to control the drying process adequately;
- (c)
- Greater sensitivity to detect the effects of changes in temperature and moisture content inside the material, eliminating uncertainties of experimental tests;
- (d)
- The predictive capacity of the modeling allows the design and optimization of dryers and drying processes, eliminating the construction of the prototype, and reducing costs;
- (e)
- Depending on the problem, numerical simulation can does not require a reduction of scale; enabling the study to be performed on a real scale, giving more physical values to the results which are obtained.
- (a)
- Liquid diffusion due to the concentration gradient;
- (b)
- Vapor diffusion due to the gradient of moisture concentration and partial vapor pressure;
- (c)
- Liquid movement due to capillary forces;
- (d)
- Liquid or vapor flux due to differences in total pressure;
- (e)
- Flux by effusion (Knudsen): important in conditions of high vacuum, as in freeze drying, and occurs when the average free path of the vapor molecules is of the same order of magnitude as the pore diameter;
- (f)
- Flux of liquid due to gravity.
2. Methodology
2.1. Experimental Procedure
2.2. Mathematical and Computational Modeling
2.2.1. The Geometry and Mesh
2.2.2. Governing Equations
2.2.3. Initial and Boundary Conditions
- (a)
- Solid Phase
- Initial conditions:
- ○
- Prescribed temperature, Ts_in;
- ○
- Prescribed water mass, ms_in;
- Boundary conditions:
- ○
- Conservative interface flux at the interface between the solid and fluid phases.
- (b)
- Fluid Phase
- Initial conditions:
- ○
- Prescribed temperature inside the oven, Tf;
- ○
- Prescribed water mass inside the oven, mf_in;
- Boundary conditions:
- ○
- Prescribed air flow rate in the oven inlet,
- ○
- Prescribed water flow rate in the oven inlet,
- ○
- Conservative interface flux at the interface between the solid and fluid phases was used;
- ○
- Average pressure of 101,325 Pa in the oven outlet.
2.2.4. Interface between the Solid and Fluid Phases
2.2.5. Process Parameters and Studied Cases
- Solid domain (clay brick):
- Fluid domain (air as an ideal gas):
2.2.6. Statistical Treatment of Data
3. Results and Discussion
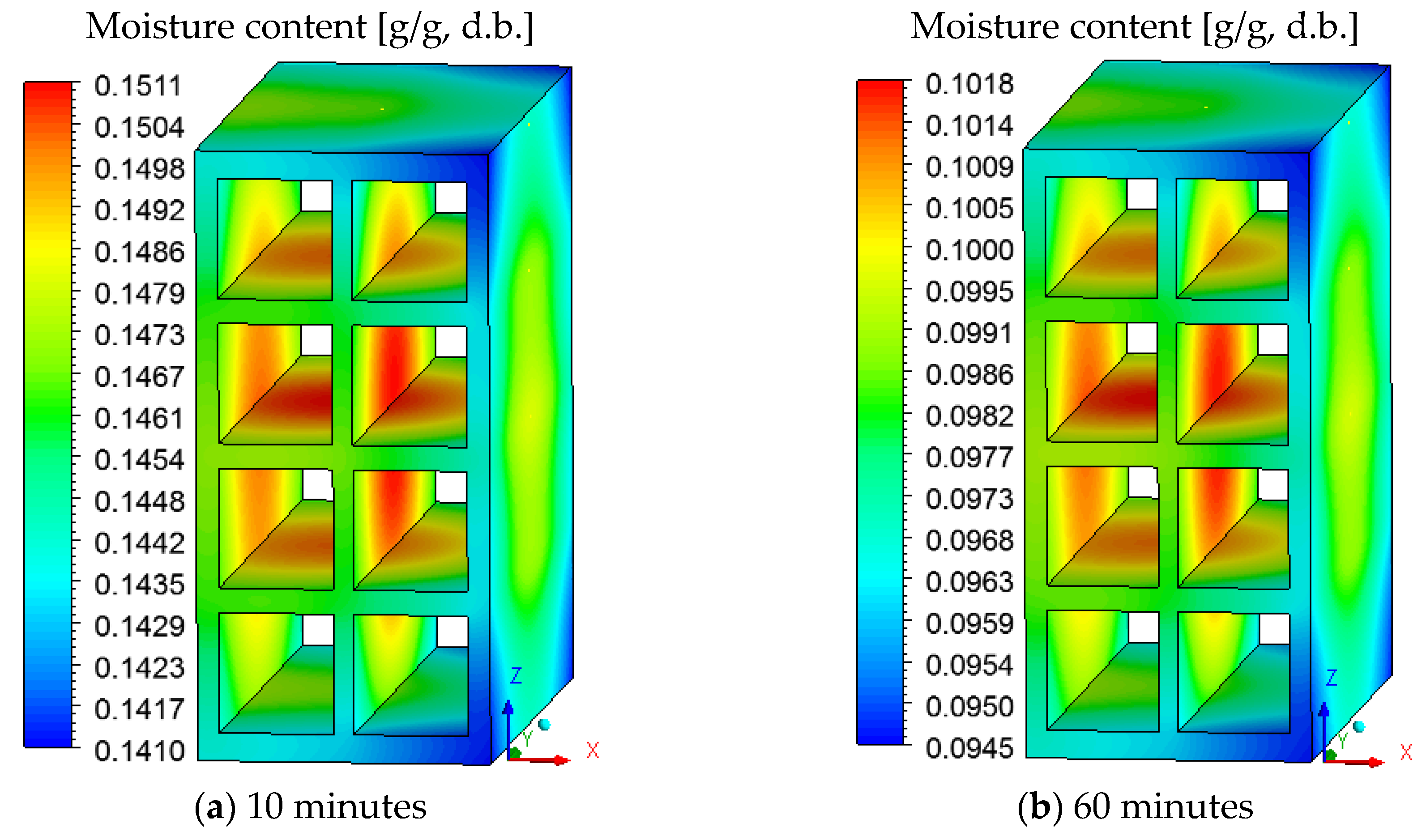
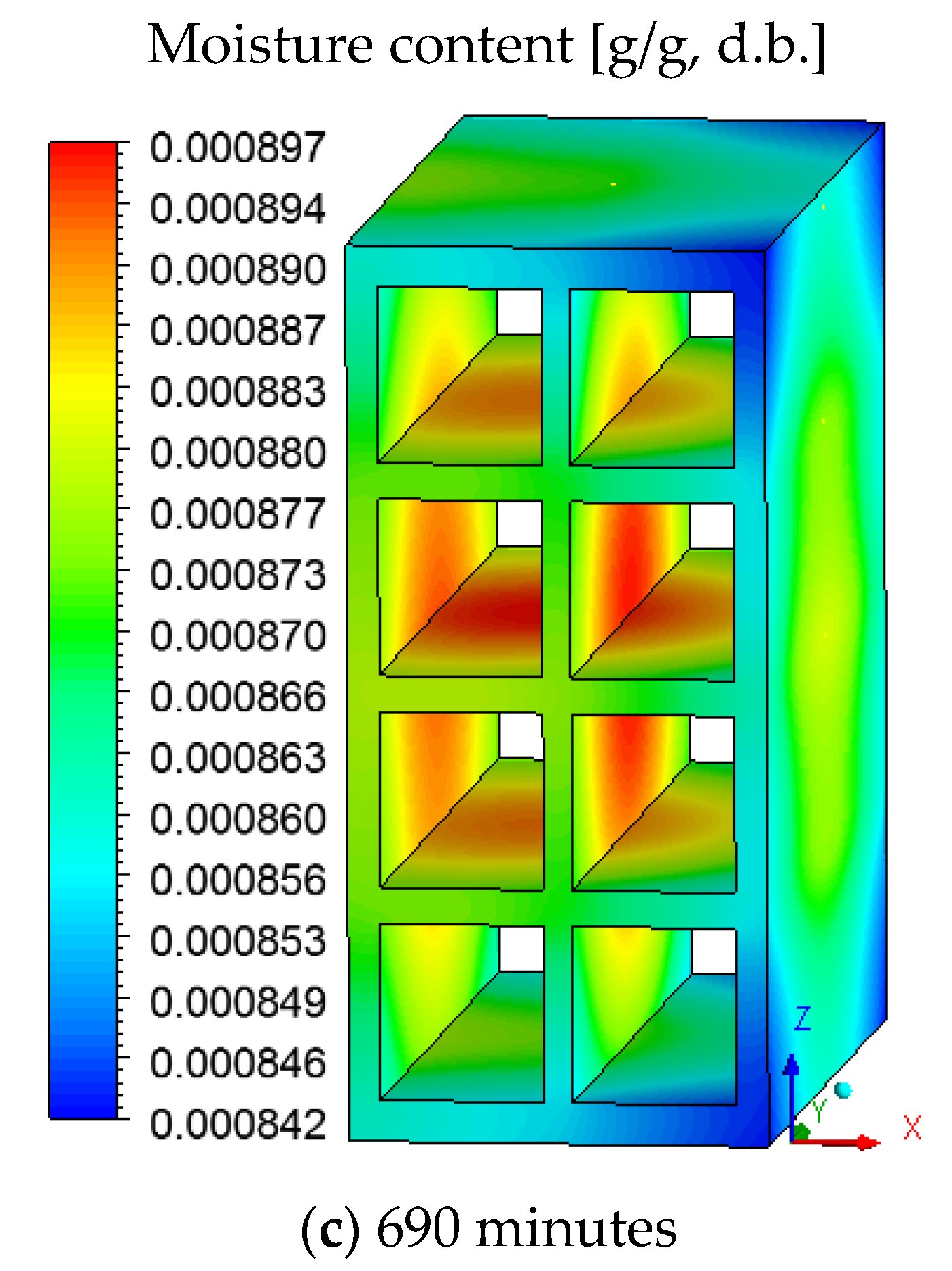
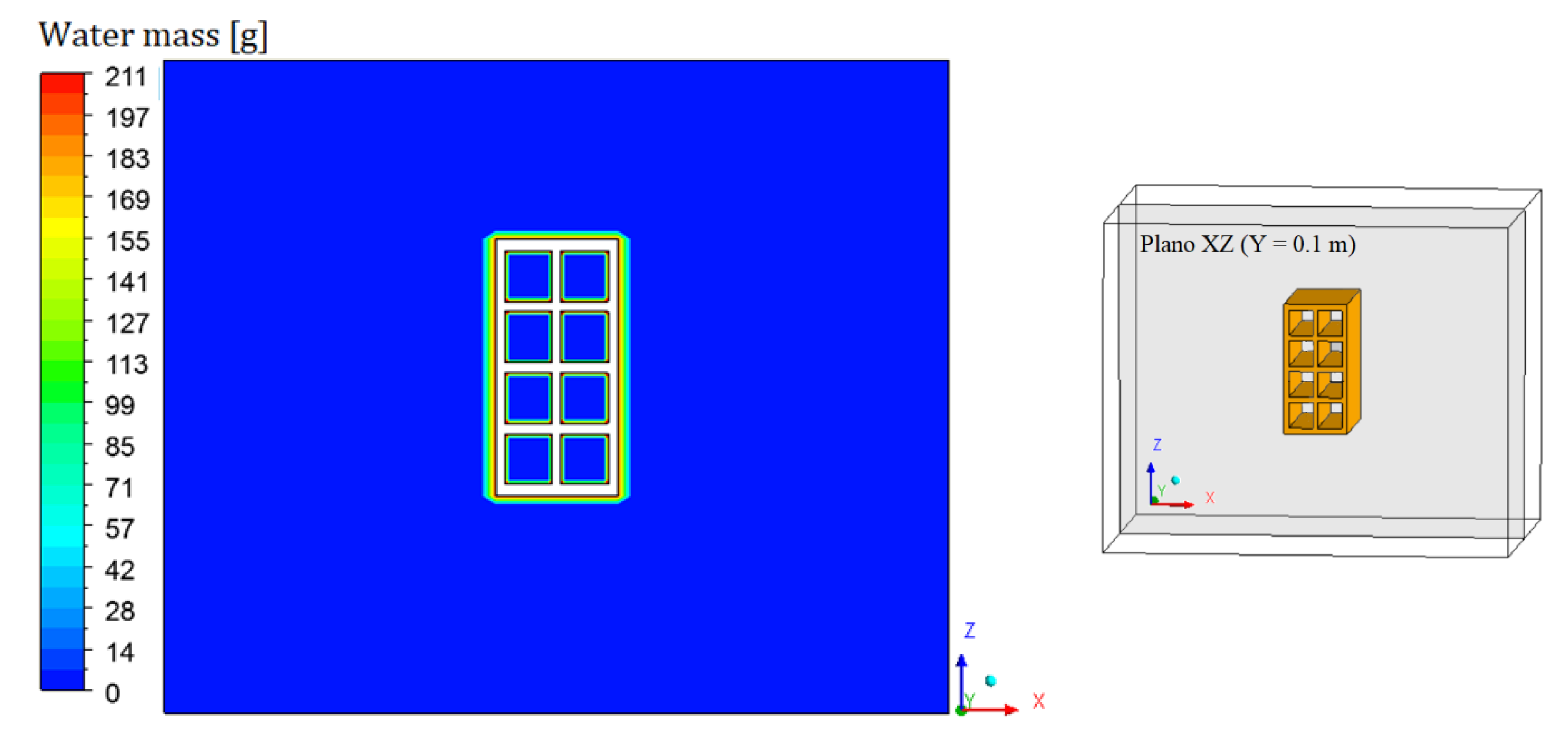
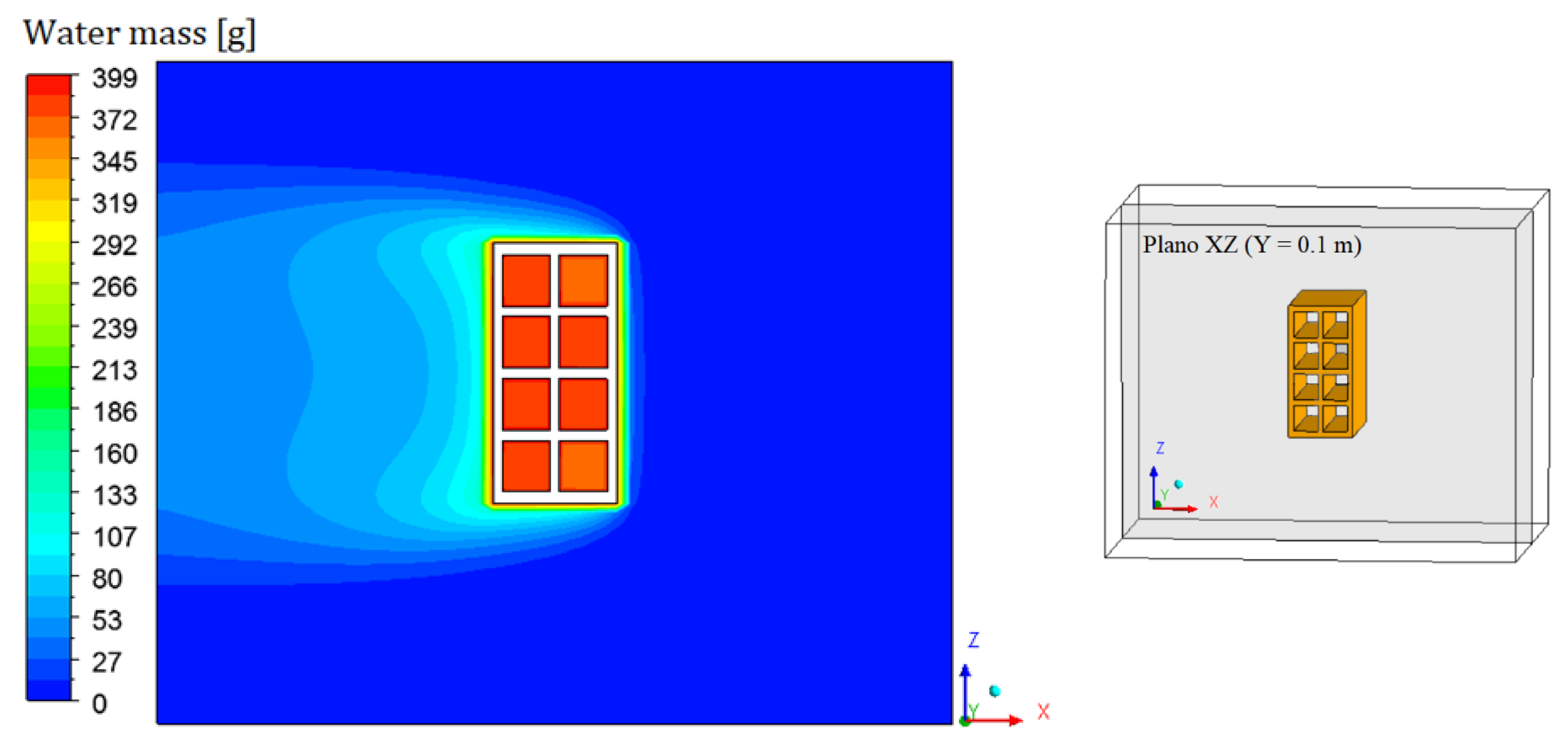
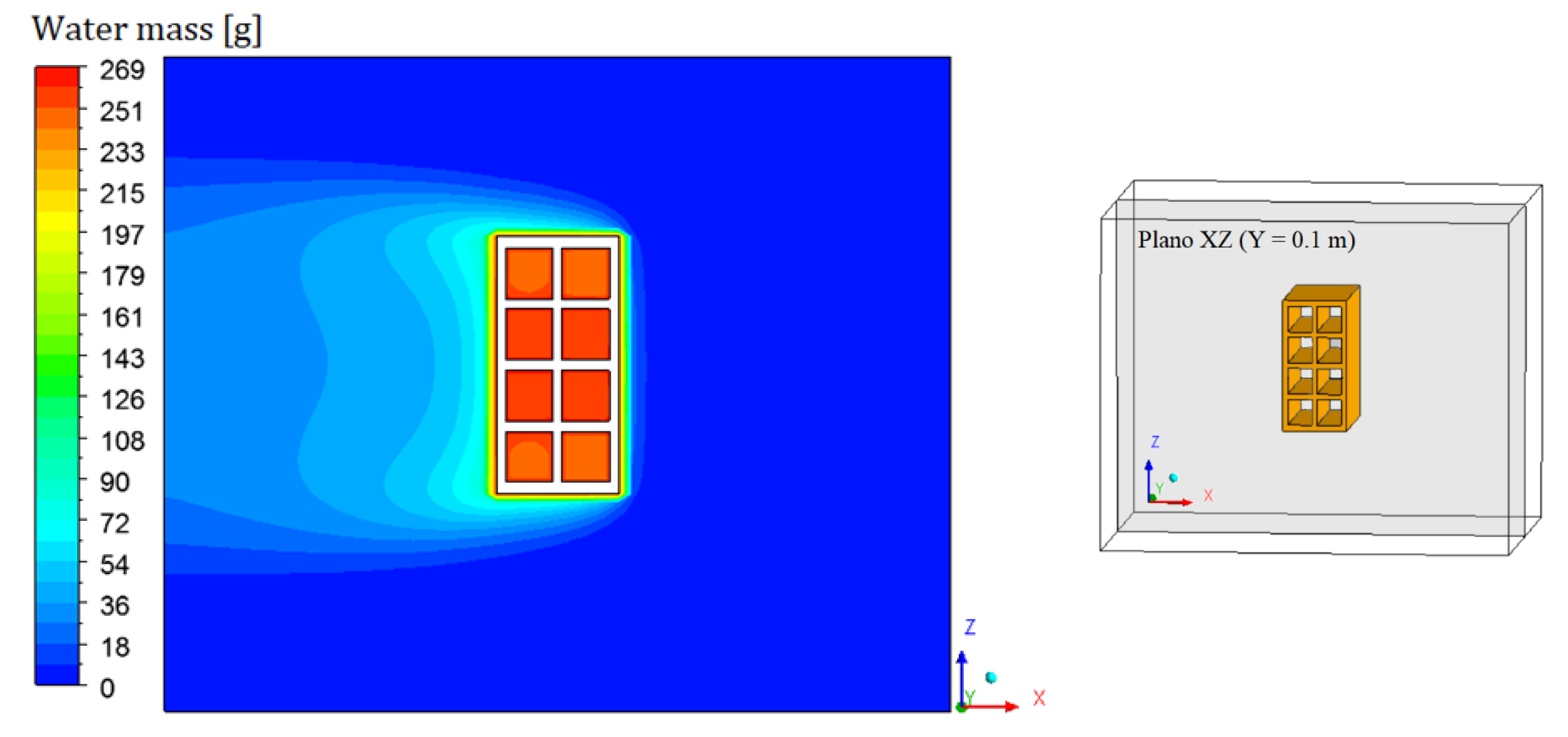
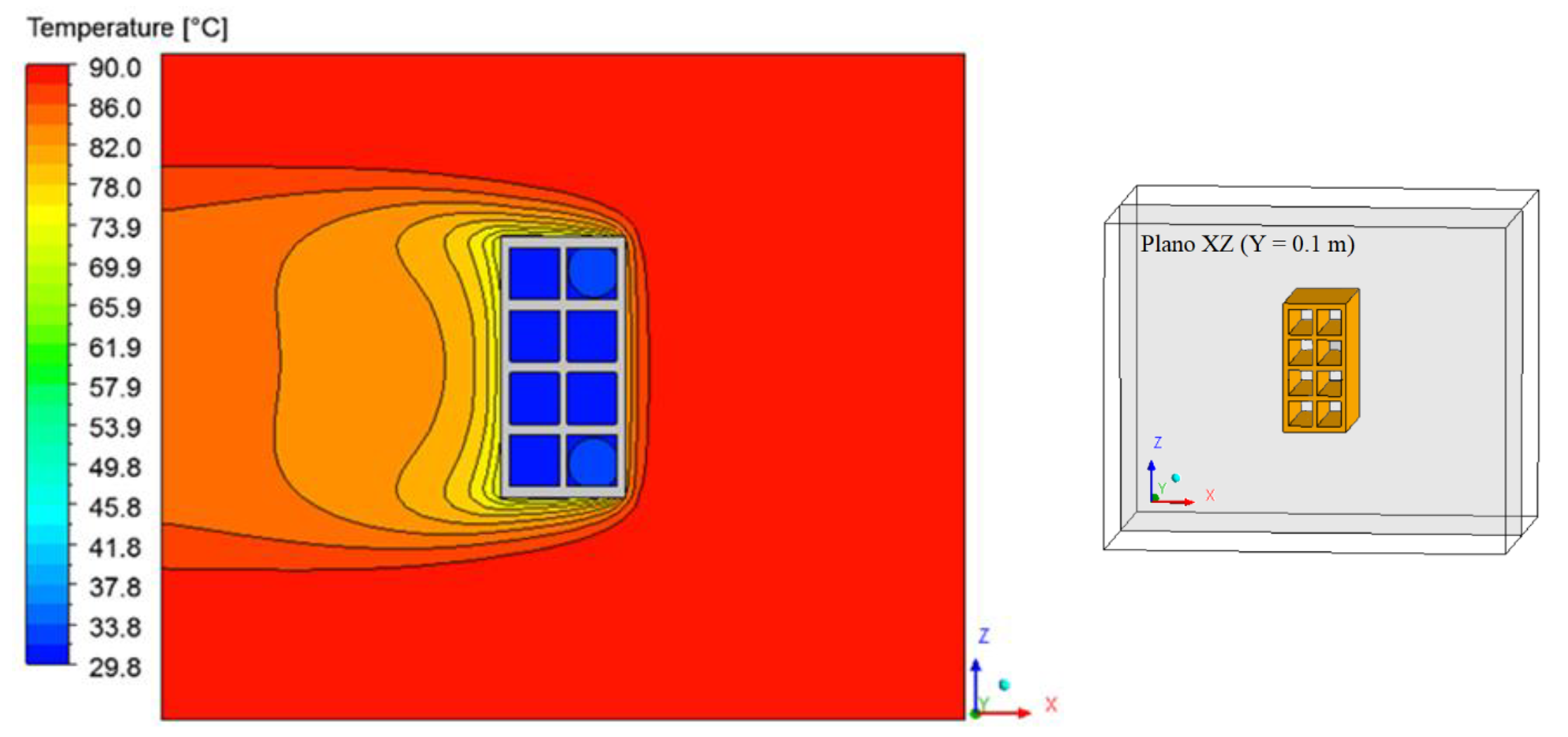
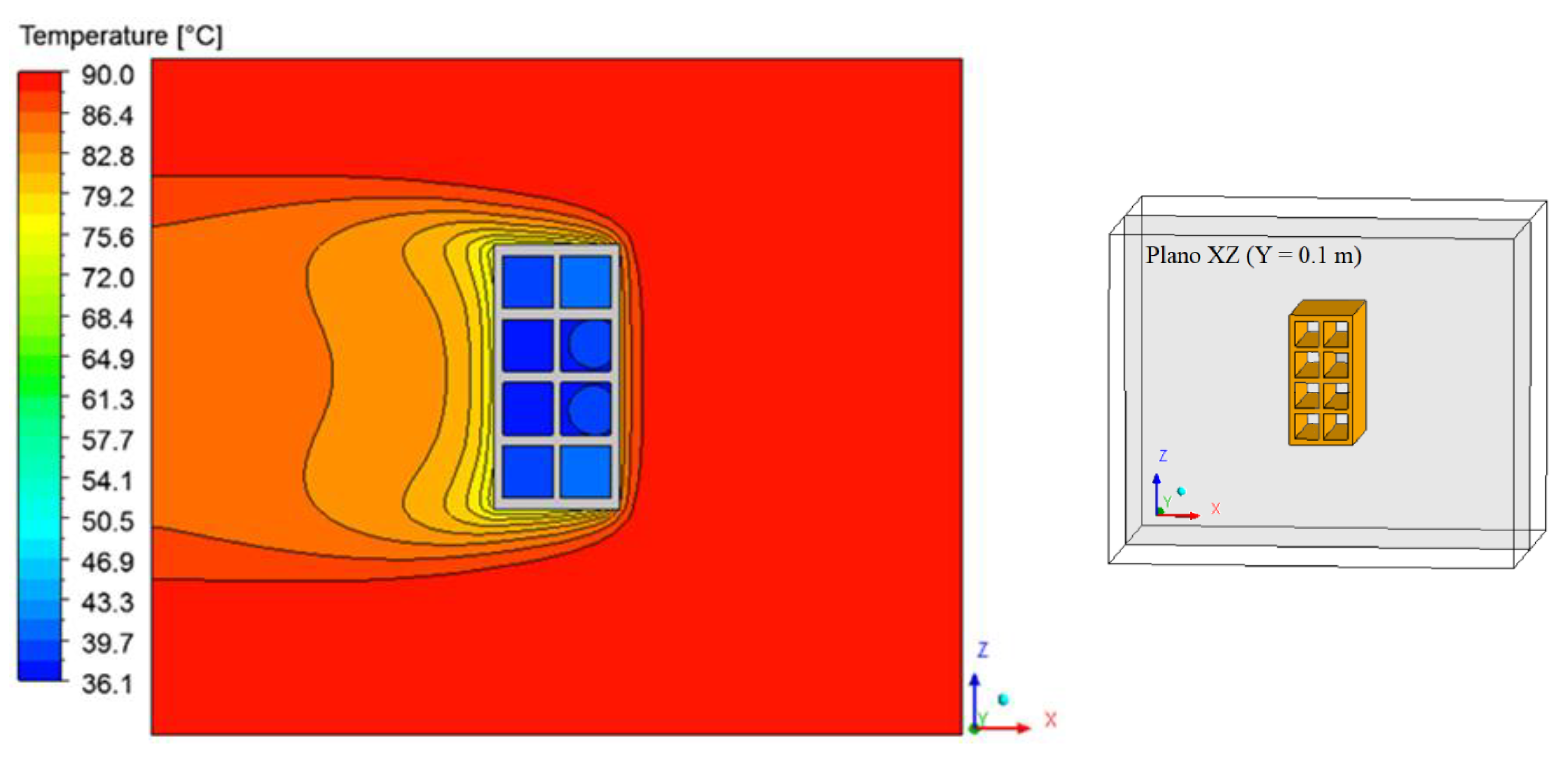
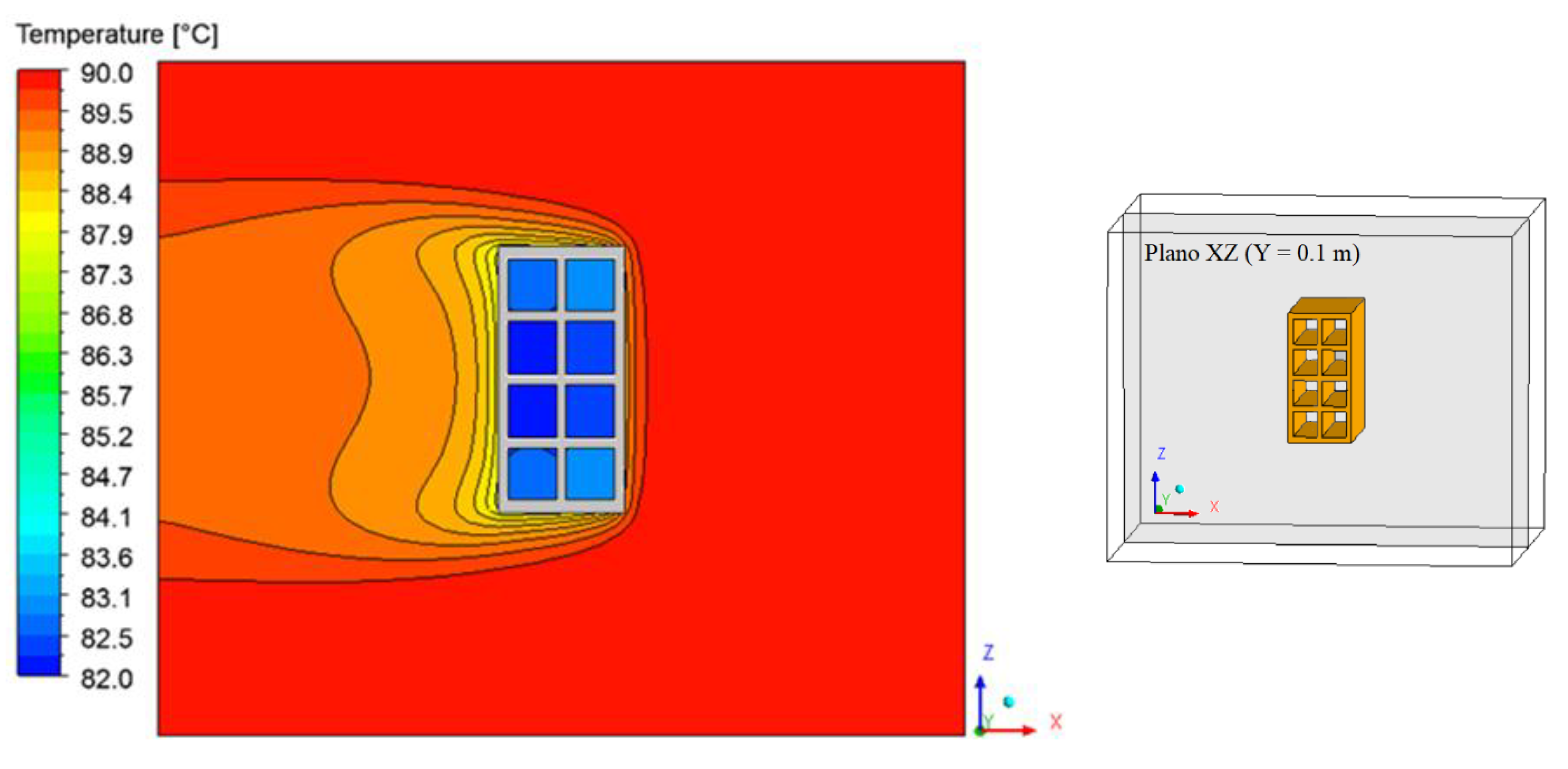
3.1. Transient Analysis of Mass Transfer
3.2. Transient Analysis of Heat Transfer
4. Conclusions
- (a)
- The proposed mathematical modeling was adequate, providing numerical results of the temperature at the vertex and water mass of the bricks along the drying process with a good agreement with the experimental data;
- (b)
- The higher the drying air temperature, the faster the heating and drying rates are;
- (c)
- The temperature and moisture content distribution on the brick surface have shown a strong dependence with the direction of air flow inside the oven and drying conditions;
- (d)
- There were variations in both moisture content and temperature on the brick surface and temperature and absolute humidity, showing that a convective boundary condition is more appropriate for describing this process;
- (e)
- The heat and mass transfer coefficients by convection and the mass diffusion coefficient increased with increasing drying air temperature;
- (f)
- The rise in mass diffusivity with the drying-air temperature is in accordance with the Arrhenius law.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Foust, A.S.; Wenzel, L.A.; Clump, C.W.; Maus, L.; Andersen, L.B. Principles of Unit Operations. Rio Jan. Guanabara Dois S. A 2015, 1. [Google Scholar]
- Batista, V.R.; Nascimento, J.J.S.; Lima, A.G.B. Drying and burning of massive and cast ceramic bricks including dimensional variations and structural damage. Rev. Eletrônica Mater. Process. 2008, 3, 46–61. (In Portuguese) [Google Scholar]
- Batista, V.R.; Nascimento, J.J.S.; Lima, A.G.B. Drying and volumetric retraction of solid and hollow ceramic bricks: A theoretical and experimental investigation. Matéria 2009, 14, 1088–1100. (In Portuguese) [Google Scholar]
- Cadé, M.A.; Nascimento, J.J.S.; Lima, A.G.B. Drying of Holed Ceramic Bricks: An Approach by Finite-Volumes. Matéria 2005, 10, 443–453. (In Portuguese) [Google Scholar]
- Nascimento, J.J.S.; Lima, A.G.B. Experimental Drying of Ceramics Bricks Including Shrinkage. Defect Diffus. Forum 2015, 365, 106–111. [Google Scholar] [CrossRef]
- Silva, J.B.; Almeida, G.S.; Lima, W.C.P.B.; Neves, G.A.; Lima, A.G.B. Heat and Mass Transfer and Volume Variations during Drying of Industrial Ceramic Bricks: An Experimental Investigation. Defect Diffus. Forum 2012, 326, 267–272. [Google Scholar] [CrossRef]
- Lopez, E.M.; Cortes, L.L. Moisture transfer analysis during drying of brick by temperature and relative humidity profiles. Eur. Sci. J. 2012, 9, 109–122. [Google Scholar]
- Pel, L.; Ketelaars, A.A.J.; Adan, O.C.G.; Van Well, A.A. Determination of moisture diffusivity in porous media using scanning neutron radiography. Int. J. Heat Mass Transf. 1993, 36, 1261–1267. [Google Scholar] [CrossRef]
- Gualtieri, A.F.; Ricchi, A.; Gualtieri, M.L.; Maretti, S.; Tamburini, M. Kinetic study of the drying process of clay bricks. J. Therm. Anal. Calorim. 2016, 123, 153–167. [Google Scholar] [CrossRef]
- Brooker, D.B.; Bakker-Arkema, F.W.; Hall, C.W. Drying and Storage of Grains and Oilseeds; AVI Book: New York, NY, USA, 1992; 450p. [Google Scholar]
- Fortes, M.; Okos, M.R. Advances in Drying; Hemisphere Publishing Corporation: Washington, WA, USA, 1980; Volume 1, pp. 119–154. [Google Scholar]
- Strumillo, C.; Kudra, T. Drying: Principles, Science and Design; Gordon and Breach Science Publishers: New York, NY, USA, 1986; 448p. [Google Scholar]
- Augier, F.; Coumans, W.J.; Hugget, A.; Kaasschieter, E.F. On the risk of cracking in clay drying. Chem. Eng. J. 2002, 86, 133–138. [Google Scholar] [CrossRef]
- Van der Zanden, A.J.J.; Schoenmakers, A.M.E.; Kerkhof, P.J.A.M. Isothermal vapour and liquid transport inside clay during drying. Dry. Technol. 1996, 14, 647–676. [Google Scholar] [CrossRef]
- Silva, W.P.; Farias, V.S.O.; Neves, G.A.; Lima, A.G.B. Modeling of water transport in roof tiles by removal of moisture at isothermal conditions. Heat Mass Transf. 2012, 48, 809–821. [Google Scholar] [CrossRef]
- Tomas, S.; Skansi, D.; Sokele, M. Convection drying of porous material. Ceram. Int. 1994, 20, 9–16. [Google Scholar] [CrossRef]
- Van der Zanden, A.J.J.; de Wit, M.H. A procedure to measure the diffusion coefficient of water in brick as a function of the water concentration. Dry. Technol. Int. J. 2012, 30, 526–534. [Google Scholar] [CrossRef]
- Telljohann, U.; Junge, K.; Specht, E. Moisture diffusion coefficients for modeling the first and second drying sections of green bricks. Dry. Technol. 2008, 26, 855–863. [Google Scholar] [CrossRef]
- Araújo, M.V.; Santos, R.S.; Silva, R.M.E.; Lima, A.G.B. Drying of Industrial Hollow Ceramic Brick: Analysis of the Moisture Content and Temperature Parameters. Defect Diffus. Forum 2017, 380, 72–78. [Google Scholar] [CrossRef]
- Araújo, M.V.; Delgado, J.M.P.Q.; Barbosa de Lima, A.G. On the Use of CFD in Thermal Analysis of Industrial Hollow Ceramic Brick. Diffus. Found. 2017, 10, 70–82. [Google Scholar] [CrossRef]
- Araújo, M.V.; Santos, R.S.; Silva, R.M.; Nascimento, J.B.S.; Santos, W.R.G.; Lima, A.G.B. Drying of Industrial Hollow Ceramic Brick: A Numerical Analysis Using CFD. Defect Diffus. Forum 2019, 391, 48–53. [Google Scholar] [CrossRef]
- Araújo, M.V.; Pereira, A.S.; Oliveira, J.L.; Brandão, V.A.A.; Brasileiro Filho, F.; De, A.; Silva, R.M.; Lima, A.G.B. Industrial Ceramic Brick Drying in Oven by CFD. Materials 2019, 12, 1612. [Google Scholar] [CrossRef]
- Silva, J.B. Simulation and Experimentation of Drying Ceramic Bricks. Ph.D. Thesis, Federal University of Campina Grande, Campina Grande, Brazil, 2009; 173p. (In Portuguese). [Google Scholar]
- Inc Ansys. NSYS CFX-Solver Theory Guide; Inc Ansys: Canonsburg, PA, USA, 2013; 356p. [Google Scholar]
- Araújo, M.V. Numerical Study of the Water and Oil Biphasic Flow in T-Connections in the Presence of Leak. Master’s Thesis, dissertation in Chemical Engineering, Federal University of Campina Grande, Campina Grande, Brazil, 2014; 87p. (In Portuguese). [Google Scholar]
- Almeida, G.S. Simulation and Experimentation of Red Ceramic Drying in Industrial Thermal Systems. Ph.D. Thesis, Federal University of Campina Grande, Campina Grande, Brazil, 2009; 189p. (In Portuguese). [Google Scholar]
- Çengel, Y.A.E.; Ghajar, A.J. Heat and Mass Transfer: Fundamentals & Applications; AMGH: Porto Alegre, Brazil, 2012. (In Portuguese) [Google Scholar]
- Corrêa, P.C.; Resende, O.; Goneli, A.L.D.; Botelho, F.M.; Nogueira, B.L. Determination of the liquid diffusion coefficient of beans. Braz. J. Agro Ind. Prod. 2006, 8, 117–126. (In Portuguese) [Google Scholar]
- Silva, J.B.; Almeida, G.S.; Lima, W.C.P.B.; Neves, G.A.; Lima, A.G.B. Heat and mass diffusion including shrinkage and hygrothermal stress during drying of holed ceramics bricks. Defect Diffus. Forum 2011, 312, 971–976. [Google Scholar] [CrossRef]
- Silva, W.P.; Silva, C.M.D.P.S.; Silva, L.D.; Farias, V.S.O. Drying of clay slabs: Experimental determination and prediction by two-dimensional diffusion models. Ceram. Int. 2013, 39, 7911–7919. [Google Scholar] [CrossRef]
- Shokouhmand, H.; Abdollahi, V.; Hosseini, S.; Vahidkhah, K. Performance optimization of a brick dryer using porous simulation approach. Dry. Technol. Int. J. 2011, 29, 360–370. [Google Scholar] [CrossRef]

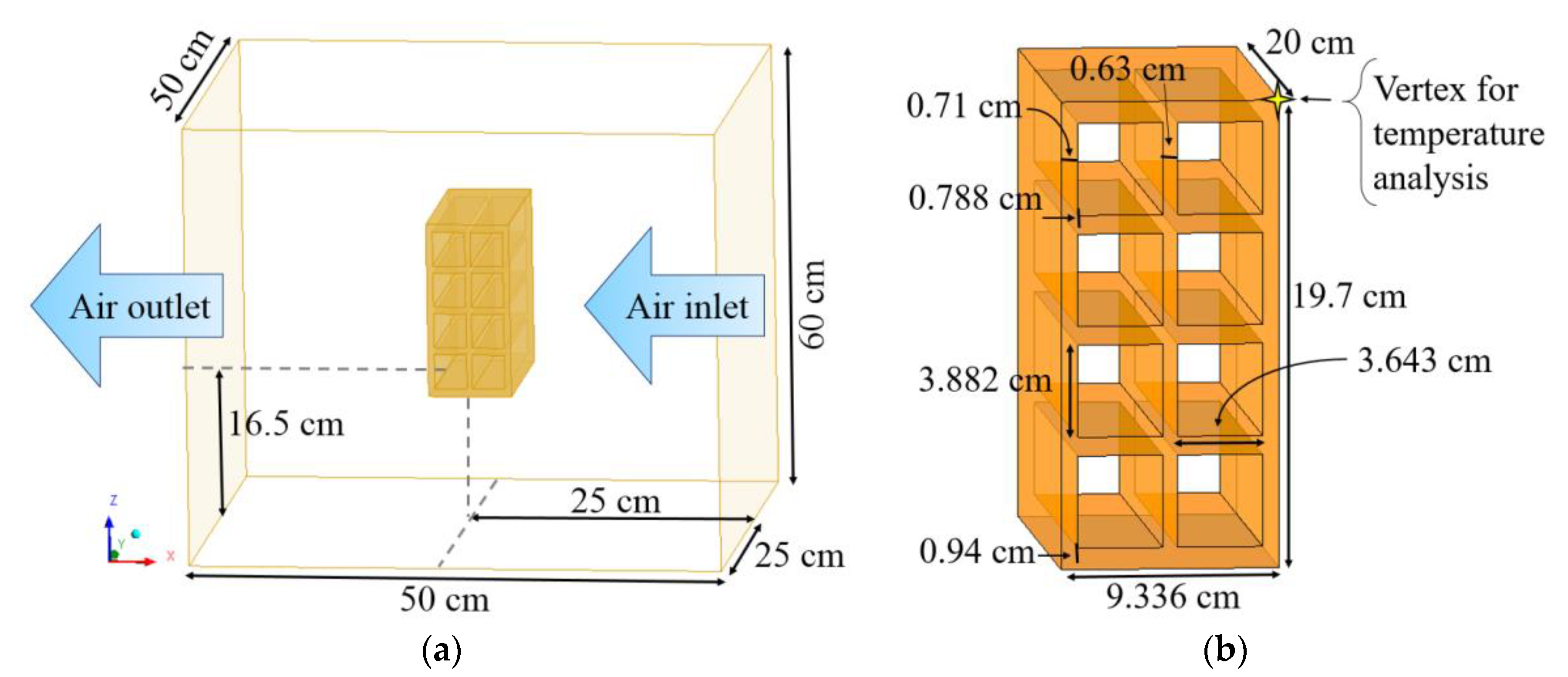


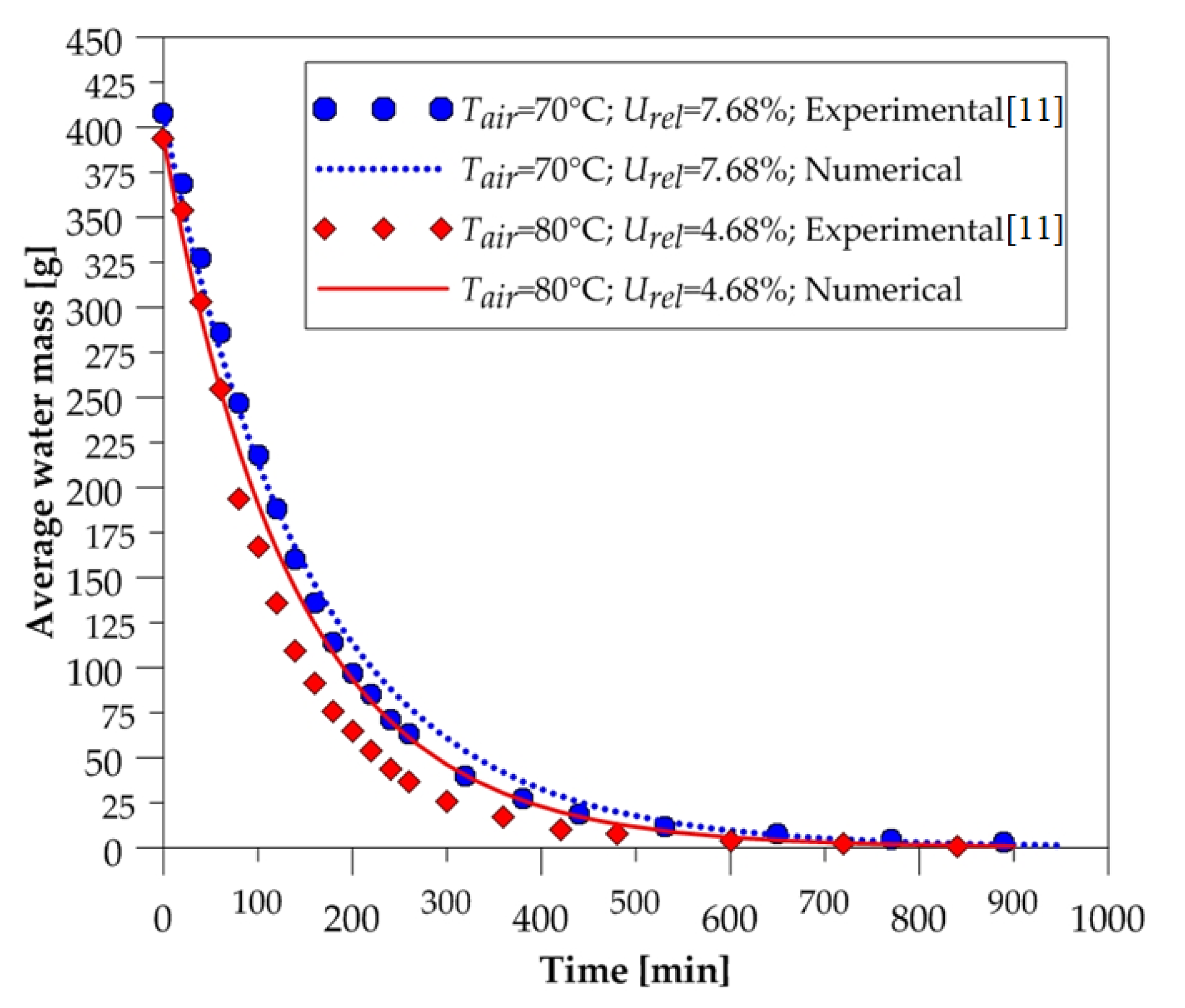
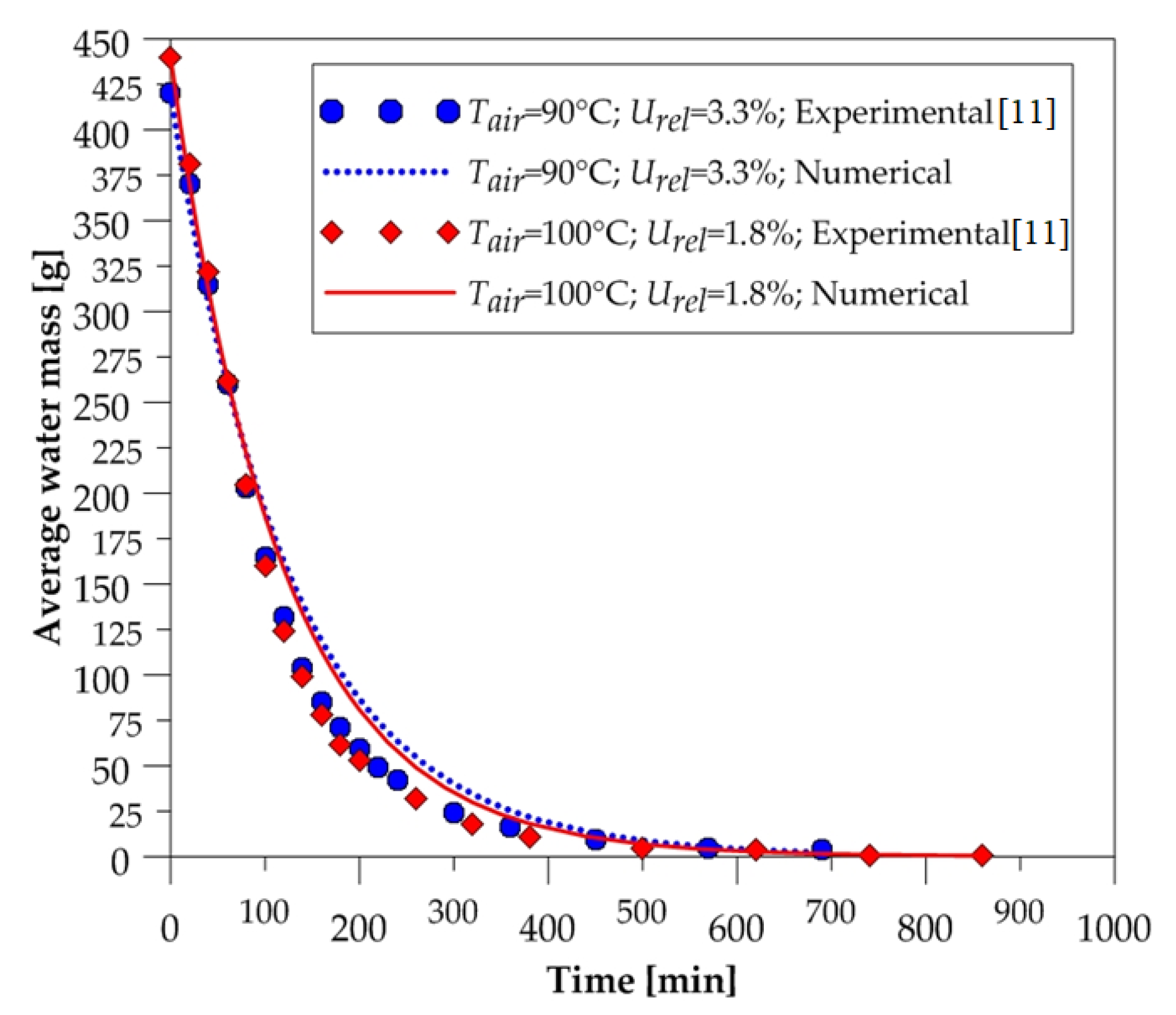
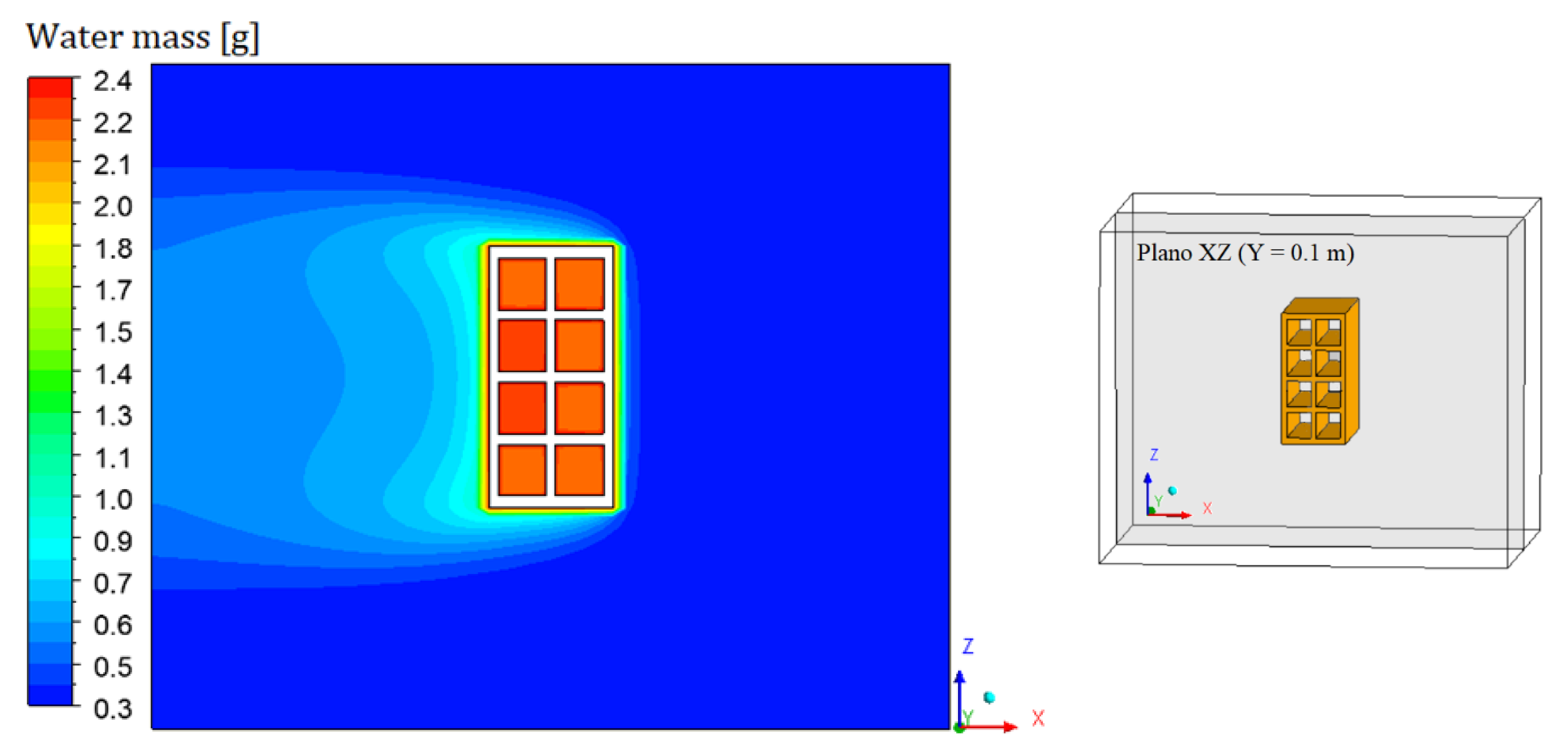
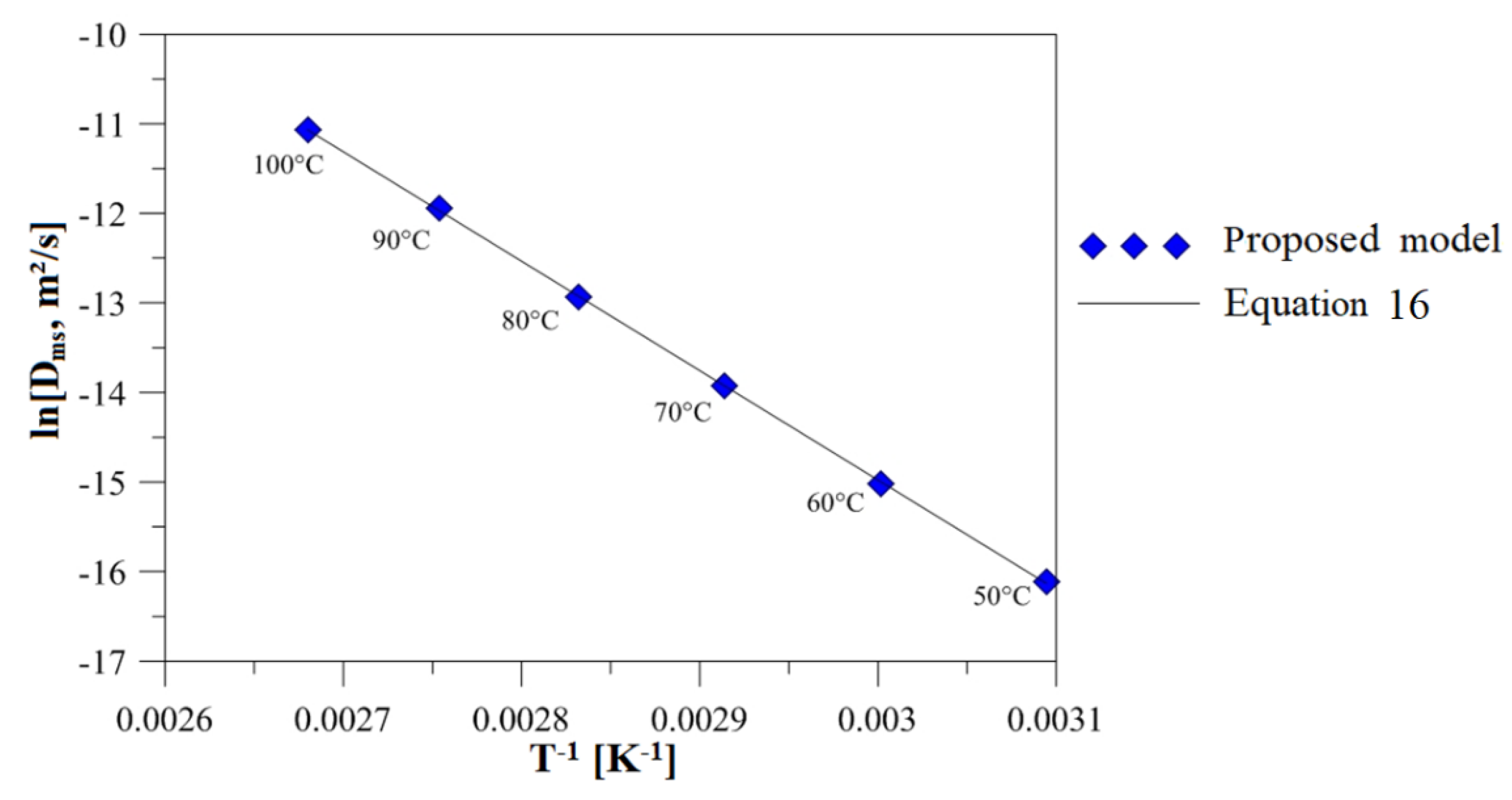
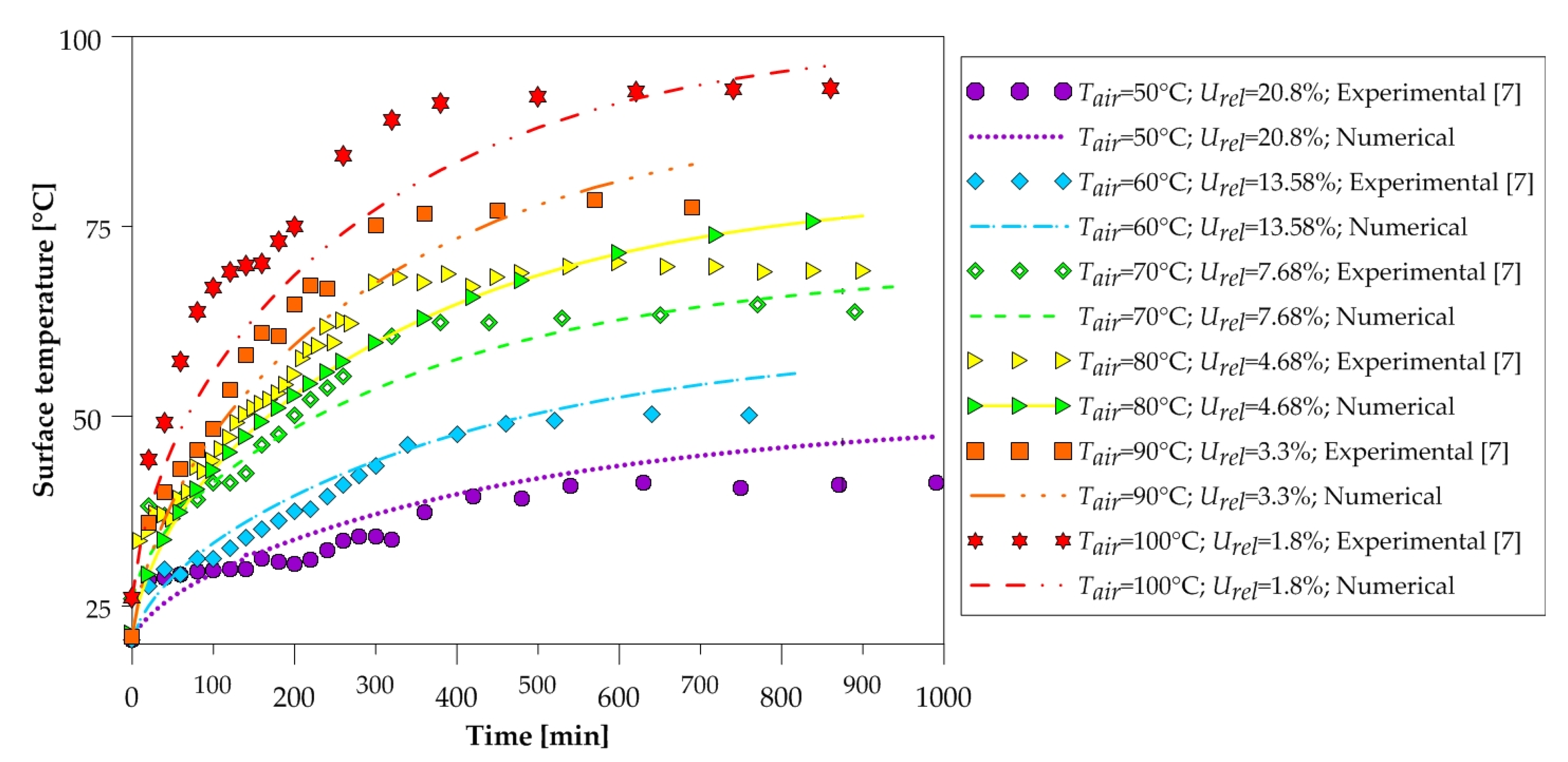
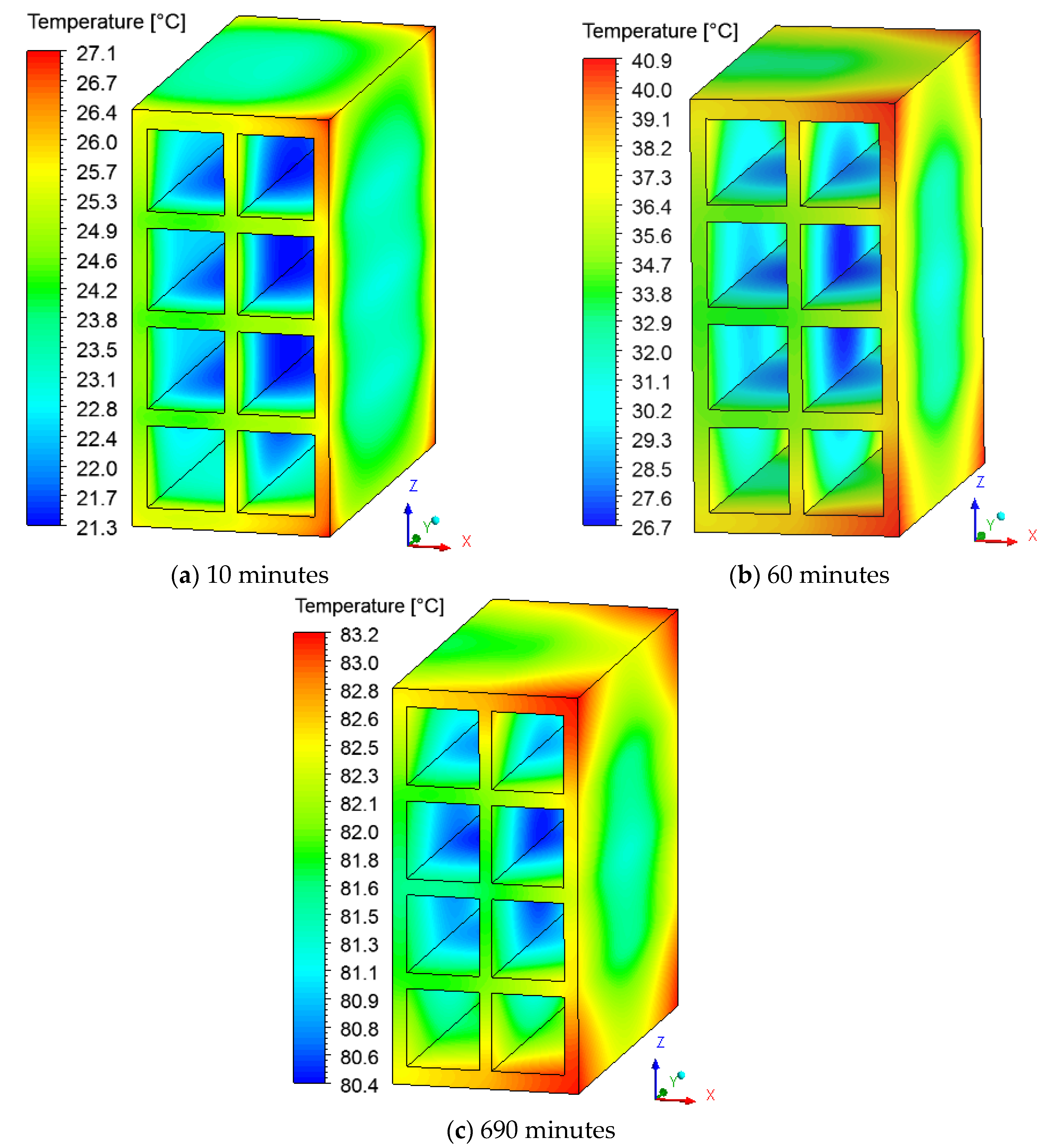
| Tf [°C] * | 50 | 60 | 70 | 80 | 90 | 100 |
|---|---|---|---|---|---|---|
| Dms [m2/s] * | 4.0 × 10−10 | 0.3 × 10−10 | 0.9 × 10−10 | 8.0 × 10−10 | 10 × 10−10 | 16 × 10−10 |
| Ts_in [°C] * | 20.6 | 20.5 | 26 | 21.4 | 21 | 26.1 |
| Urel [%] * | 20.80 | 13.58 | 7.68 | 4.68 | 3.30 | 1.80 |
| U [m/s] * | 0.05 | 0.06 | 0.07 | 0.08 | 0.09 | 0.10 |
| ms_in [g] * | 365 | 386 | 408 | 394 | 420 | 440 |
| mf_in [g] | 2.62601 | 2.68886 | 2.30198 | 2.06730 | 2.10014 | 1.60376 |
| [g/s] | 13.65458 | 15.89367 | 18.00225 | 19.99141 | 21.87102 | 23.64989 |
| [g/s] | 0.22092 | 0.27145 | 0.27112 | 0.27827 | 0.31802 | 0.26984 |
| Dmf [m2/s] | 2.728 × 10−5 | 2.8948 × 10−5 | 3.0662 × 10−5 | 3.2419 × 10−5 | 3.4218 × 10−5 | 3.6058 × 10−5 |
| ttotal [min] * | 990 | 820 | 950 | 900 | 690 | 860 |
| Temperature [°C] | Dms [m2/s] |
|---|---|
| 50 | 1 × 10−7 |
| 60 | 3 × 10−7 |
| 70 | 9 × 10−7 |
| 80 | 24 × 10−7 |
| 90 | 65 × 10−7 |
| 100 | 155 × 10−7 |
| Drying Temperature | |hm,X| [m/s] | |hm,Y| [m/s] | |hm,Z| [m/s] | |hm| [m/s] |
|---|---|---|---|---|
| 50 °C | 6.87 × 10−5 | 5.95 × 10−7 | 1.82 × 10−6 | 8.38 × 10−4 |
| 60 °C | 4.50 × 10−5 | 4.22 × 10−7 | 4.56 × 10−7 | 4.78 × 10−4 |
| 70 °C | 4.78 × 10−5 | 4.87 × 10−7 | 1.08 × 10−7 | 4.83 × 10−4 |
| 80 °C | 5.10 × 10−5 | 5.46 × 10−7 | 1.11 × 10−7 | 5.07 × 10−4 |
| 90 °C | 5.41 × 10−5 | 6.00 × 10−7 | 2.33 × 10−7 | 5.31 × 10−4 |
| 100 °C | 5.89 × 10−5 | 6.65 × 10−7 | 2.86 × 10−7 | 5.71 × 10−4 |
| Temperature [°C] | hc [W/(m2·K)] |
|---|---|
| 50 | 0.9655 |
| 60 | 1.0700 |
| 70 | 1.1603 |
| 80 | 1.2486 |
| 90 | 1.2887 |
| 100 | 1.3931 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
de Vasconcellos Araújo, M.; Raquel de Brito Correia, B.; Alves Agra Brandão, V.; Rodrigues de Oliveira, I.; Sousa Santos, R.; de Oliveira Neto, G.L.; de Lucena Silva, L.P.; Gilson Barbosa de Lima, A. Convective Drying of Ceramic Bricks by CFD: Transport Phenomena and Process Parameters Analysis. Energies 2020, 13, 2073. https://doi.org/10.3390/en13082073
de Vasconcellos Araújo M, Raquel de Brito Correia B, Alves Agra Brandão V, Rodrigues de Oliveira I, Sousa Santos R, de Oliveira Neto GL, de Lucena Silva LP, Gilson Barbosa de Lima A. Convective Drying of Ceramic Bricks by CFD: Transport Phenomena and Process Parameters Analysis. Energies. 2020; 13(8):2073. https://doi.org/10.3390/en13082073
Chicago/Turabian Stylede Vasconcellos Araújo, Morgana, Balbina Raquel de Brito Correia, Vanderson Alves Agra Brandão, Iran Rodrigues de Oliveira, Rosilda Sousa Santos, Guilherme Luiz de Oliveira Neto, Leonardo Pereira de Lucena Silva, and Antonio Gilson Barbosa de Lima. 2020. "Convective Drying of Ceramic Bricks by CFD: Transport Phenomena and Process Parameters Analysis" Energies 13, no. 8: 2073. https://doi.org/10.3390/en13082073
APA Stylede Vasconcellos Araújo, M., Raquel de Brito Correia, B., Alves Agra Brandão, V., Rodrigues de Oliveira, I., Sousa Santos, R., de Oliveira Neto, G. L., de Lucena Silva, L. P., & Gilson Barbosa de Lima, A. (2020). Convective Drying of Ceramic Bricks by CFD: Transport Phenomena and Process Parameters Analysis. Energies, 13(8), 2073. https://doi.org/10.3390/en13082073






