Modified Modelling for Heat Like Equations within Caputo Operator

Abstract

The present paper is related to the analytical solutions of some heat like equations, using a novel approach with Caputo operator. The work is carried out mainly with the use of an effective and straight procedure of the Iterative Laplace transform method. The proposed method provides the series form solution that has the desired rate of convergence towards the exact solution of the problems. It is observed that the suggested method provides closed-form solutions. The reliability of the method is confirmed with the help of some illustrative examples. The graphical representation has been made for both fractional and integer-order solutions. Numerical solutions that are in close contact with the exact solutions to the problems are investigated. Moreover, the sample implementation of the present method supports the importance of the method to solve other fractional-order problems in sciences and engineering.

1. Introduction

Heat is the source of energy that can be transferred from one device to another due to differing temperatures. A thermodynamic analysis refers to the amount of heat transferred as a scheme that undergoes a transition from one state of equilibrium to another [1,2]. Heat transfer is the science that deals with determining the rate of such energy transfers. Transformation of energy as heat is usually from the higher to the lower temperature, and the heat transfer ceases when both mediums exceed the same temperature [3,4]. Heat can be transmitted in three differing classes—radiation, conduction and convection. The problem of fractal heat conduction describes heat transport in inhomogeneous materials as fibrous materials, coal deposits, textiles and other discontinuous media where homogenization is not acceptable because this approach neglects the important physical characteristics of transport processes. 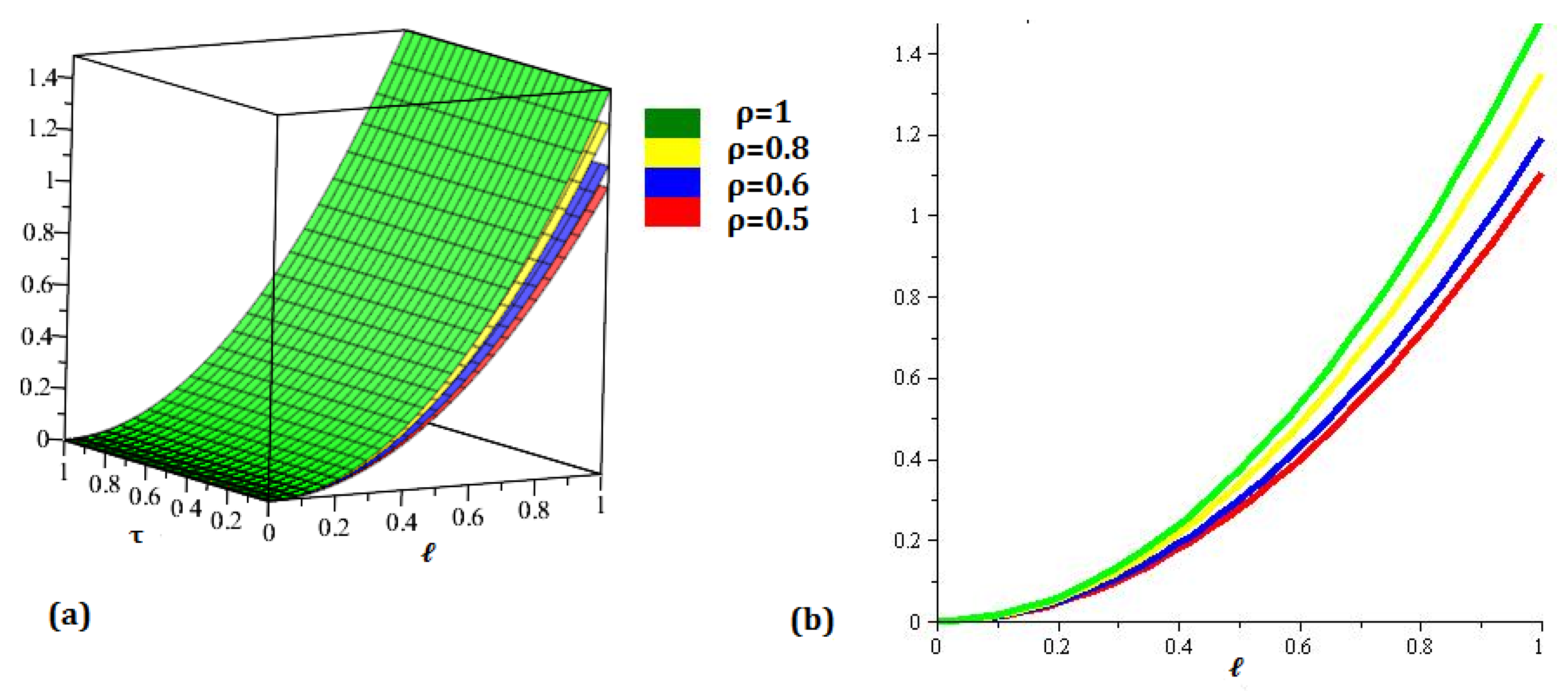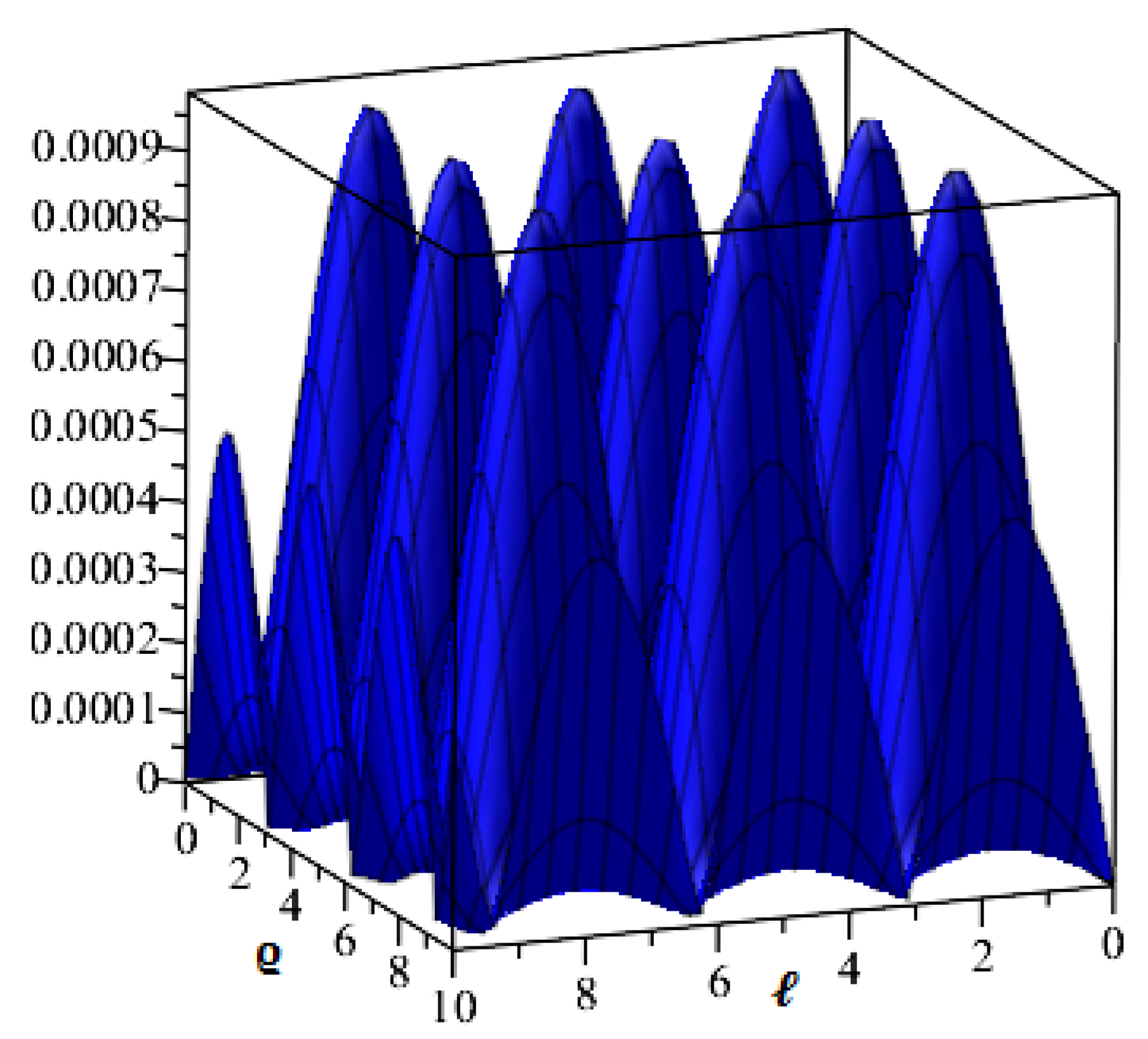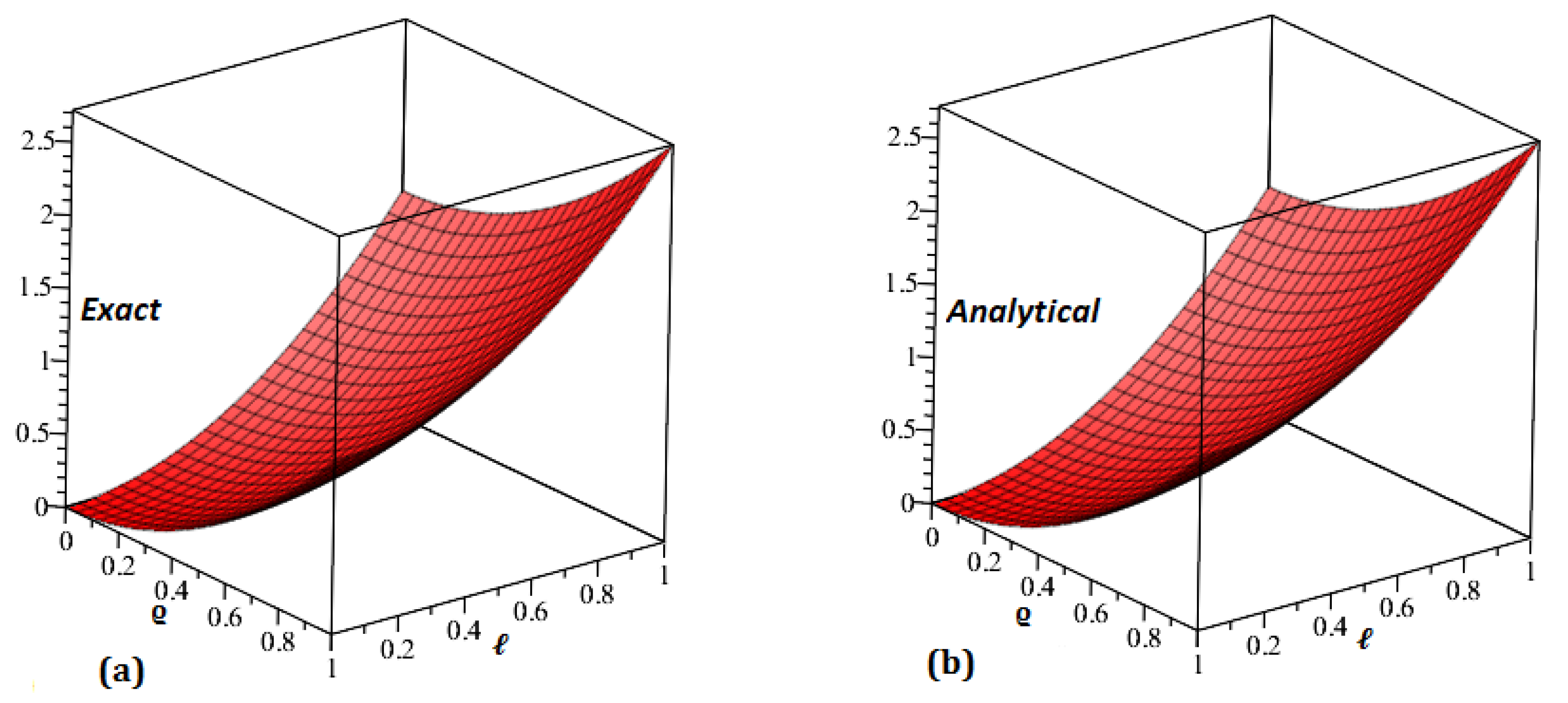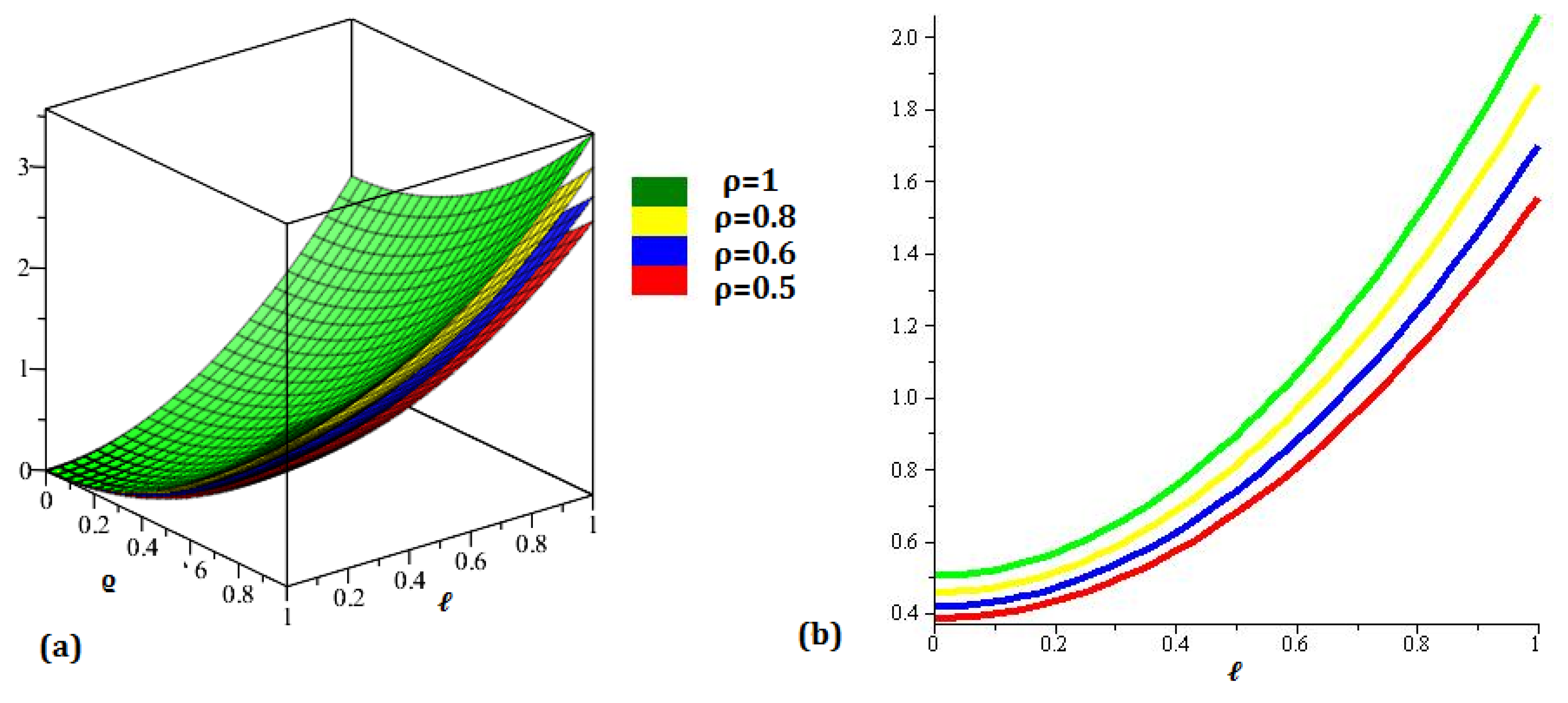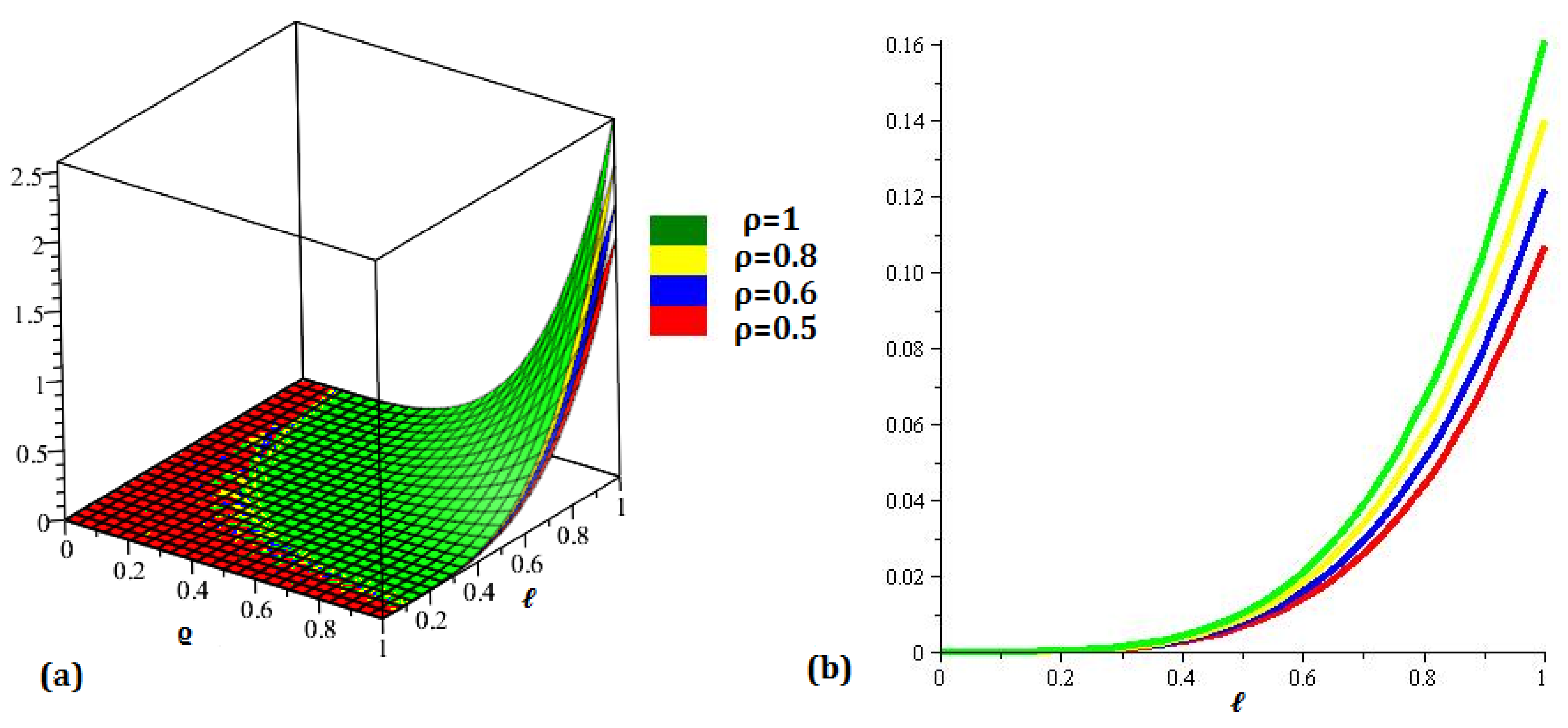Non-smoothness raises problems and avoids the implementation of classical calculus a fractional and integer-order [5,6,7].

In this article, we will consider the fractional-order heat equations of the form [8]

$$\frac{{\partial }^{\rho }\vartheta }{\partial {\tau }_1^{\rho }}=f(\ell ,\varrho ,\kappa ){\vartheta }_{\ell \ell }+g(\ell ,\varrho ,\kappa ){\vartheta }_{\varrho ,\varrho }+h(\ell ,\varrho ,\kappa ){\vartheta }_{\kappa ,\kappa },0<\rho \le 1,{\tau }_1\ge 0,$$

with initial condition

$$\vartheta (\ell ,\varrho ,\kappa ,0)=\varphi (\ell ,\varrho ,\kappa ),$$

where $\rho$ is a parameter defining a fractional derivative, ${\vartheta }_{{\tau }_1}$ is the rate of temperature change over time. Fractional heat equation solutions with variable coefficients attracted the attention of many researchers in the mathematics society, such as the homotopy perturbation method (HPM), Adomian decomposition method (ADM) [9], the optimal homotopy asymptotic method (OHAM) [10], Natural decomposition method [11], differential transform method (DTM) [12]; the fractional-order heat equation has been solved by the variational iteration transform method (VITM) [13] and Shou et al. [14] used VIM to solve different kinds of heat equations.

Nowadays, researchers pay more attention to the study of fractional-order differential problems due to their occurrence in numerous implementations and the exact explanation of the non-linear phenomenon, particularly fractional-order partial differential equations (FPDEs). FPDEs are the key mathematical methods used to model many physical processes in various branches of applied science, such as physics, engineering, or other sciences. Modeling in the form of FPDEs appears in several engineering and science applications, including microelectronics, chemistry, biology, thermodynamics, chemical kinetics and other physical processes [15,16,17,18,19,20,21,22,23,24,25,26]. Different analytical and numerical methods to solve these forms of FPDEs have been published in the literature. The numerical techniques are a finite difference method with non-uniform time steps [27,28,29,30], higher-order numerical methods [31], an implicit finite difference method [32], compact difference techniques [33], the Laplace Adomian decomposition method [34,35,36,37,38], the Homotopy analysis transform method [39] and the Sumudu transform technique (ISTM) [40]. The above-mentioned techniques have straight forward implementations for both linear and non-linear FDEs.

The iterative technique for solving numerically nonlinear functional equations [41,42] was first published by Daftardar-Gejji and Jafari in 2006. The solution of fractional-order linear and nonlinear differential equations have been solved by iterative method [43]. Jafari et al. used Laplace transformation together with iterative technique and became a well-known technique called iterative Laplace transformation technique [44], solutions of a scheme of fractional-order PDEs and Fokker–Plank equation [45]. Recently, time fractional-order Schrödinger problems [46], fractional-order Telegraph equations [47] and fractional heat and wave-like equation [48] are solved successfully by the use of iterative Laplace transform method.

This article is organized as follows, in the second section we introduce a brief history of the fractional-order derivative and its properties. In the third section, we discuss the basic ideal of iterative Laplace transformation technique to fractional-order partial differential equations. In Section 4, we implemented the iterative Laplace transform method to solve the fractional-order heat equation and presented the graphical representation of the solutions for the targeted problems. The conclusions is then presented in Section 5.

2. Definitions and Preliminaries

In this part of the paper, some important definitions related to FC and Laplace transform are briefly discussed [44]. These preliminaries are important to continue and complete the present research work.

Definition 1.

The fractional derivative in terms of Caputo operator is expressed as [44]

$$\begin{array}{cc}\hfill & D_{{\tau }_1}^{\rho }\vartheta (\ell ,{\tau }_1)=\frac{1}{\mathsf{\Gamma }(n-\rho )}{\int }_0^{{\tau }_1}{({\tau }_1-\zeta )}^{n-\rho -1}{\vartheta }^{\left(n\right)}(\ell ,\zeta )d\zeta ,n-1<\rho \le n,n\in N.\hfill \end{array}$$

(1)

Definition 2.

The fractional integral in terms of Riemann-Liouville is expressed as [44]

$$j_{{\tau }_1}^{\rho }\vartheta (\ell ,{\tau }_1)=\frac{1}{\mathsf{\Gamma }\left(\rho \right)}{\int }_0^{{\tau }_1}{({\tau }_1-\zeta )}^{\rho -1}\vartheta (\ell ,\zeta )d\zeta ,\zeta >0(n-1<\rho \le n),n\in N,$$

(2)

$j_{{\tau }_1}^{\rho }$ represents the fractional integral operator.

Definition 3.

The Laplace transform is described as [44]

$$L\left[k\left({\tau }_1\right)\right]=K\left({\tau }_1\right)={\int }_0^{\infty }{e}^{-s{\tau }_1}g\left({\tau }_1\right)d{\tau }_1.$$

(3)

Definition 4.

The Laplace transform of the fractional derivative $D_{{\tau }_1}^{\rho }\vartheta (\ell ,{\tau }_1)$ is defined as [44]

$$L\left[D_{{\tau }_1}^{\rho }\vartheta (\ell ,{\tau }_1)\right]=s^{\rho }L\left[\vartheta (\ell ,{\tau }_1)\right]-\sum _{k=0}^{n-1}{\vartheta }^{\left(k\right)}(\ell ,0)s^{\rho -k-1},n-1<\rho \le n,n\in N.$$

(4)

Definition 5.

The Mittag-Leffler function is expressed as [44]

$$E_{\rho }\left(z\right)=\sum _{q=0}^{\infty }\frac{z^q}{\mathsf{\Gamma }(\rho q+1)},(\rho \in C,Re\left(\rho \right)>0).$$

(5)

3. The Presentation of the Method

In this section, we will briefly discuss ILTM, to solve fractional-order nonlinear PDEs [44].

$$D_{{\tau }_1}^{\rho }\vartheta (\ell ,\varrho ,{\tau }_1)+R\vartheta (\ell ,\varrho ,{\tau }_1)+N\vartheta (\ell ,\varrho ,{\tau }_1)=g(\ell ,\varrho ,{\tau }_1),n-1<\rho \le n,n\in N,$$

(6)

$${\vartheta }^{\left(k\right)}(\ell ,\varrho ,0)=h_k(\ell ,\varrho ),k=0,1,2,\dots ,n-1,$$

(7)

where $D_{{\tau }_1}^{\rho }\vartheta (\ell ,\varrho ,{\tau }_1)$ is the fractional Caputo operator of order $\rho$, $n-1<\rho \le n$, denoted by Equation (3), R and N are linear and nonlinear operators. The function $g(\ell ,\varrho ,{\tau }_1)$ is the source function.

Using Laplace transform of Equation (6) we get

$$L\left[D_{{\tau }_1}^{\rho }\vartheta (\ell ,\varrho ,{\tau }_1)\right]+L[R\vartheta (\ell ,\varrho ,{\tau }_1)+N\vartheta (\ell ,\varrho ,{\tau }_1)]=L\left[g(\ell ,\varrho ,{\tau }_1)\right].$$

(8)

Applying the property of Laplace differentiation [44]

$$L\left[\vartheta (\ell ,\varrho ,{\tau }_1)\right]=\frac{1}{s^{\rho }}\sum _{k=0}^{m-1}{s}^{\rho -1-k}{\vartheta }^{\left(k\right)}(\ell ,\varrho ,0)+\frac{1}{s^{\rho }}L\left[g(\ell ,\varrho ,{\tau }_1)\right]-\frac{1}{s^{\rho }}L[R\vartheta (\ell ,\varrho ,{\tau }_1)+N\vartheta (\ell ,\varrho ,{\tau }_1)].$$

(9)

By using the inverse Laplace transform of Equation (9), we obtain

$$\vartheta (\ell ,\varrho ,{\tau }_1)=L^{-1}\left[\frac{1}{s^{\rho }}\left({\sum }_{k=0}^{m-1}{s}^{\rho -1-k}{\vartheta }^k(\ell ,\varrho ,0)+L\left[g(\ell ,\varrho ,{\tau }_1)\right]\right)\right]-L^{-1}\left[\frac{1}{s^{\rho }}L[R\vartheta (\ell ,\varrho ,{\tau }_1)+N\vartheta (\ell ,\varrho ,{\tau }_1)]\right].$$

(10)

From the iterative technique,

$$\vartheta (\ell ,\varrho ,{\tau }_1)=\sum _{i=0}^{\infty }{\vartheta }_i(\ell ,\varrho ,{\tau }_1).$$

(11)

Since R is a linear operator

$$R\left(\sum _{i=0}^{\infty }{\vartheta }_i(\ell ,\varrho ,{\tau }_1)\right)=\sum _{i=0}^{\infty }R\left[{\vartheta }_i(\ell ,\varrho ,{\tau }_1)\right],$$

(12)

and the non-linear operator N is split as [44]

$$N\left(\sum _{i=0}^{\infty }{\vartheta }_i(\ell ,\varrho ,{\tau }_1)\right)=N\left[{\vartheta }_0(\ell ,\varrho ,{\tau }_1)\right]+\sum _{i=1}^{\infty }\left\{N\left(\sum _{k=0}^i{\vartheta }_k(\ell ,\varrho ,{\tau }_1)\right)-N\left(\sum _{k=0}^{i-1}{\vartheta }_k(\ell ,\varrho ,{\tau }_1)\right)\right\}.$$

(13)

Putting Equations (11)–(13) in Equation (10), we obtain

$$\begin{array}{c}\sum _{i=0}^{\infty }{\vartheta }_i(\ell ,\varrho ,{\tau }_1)=L^{-1}\left[\frac{1}{s^{\rho }}\left(\sum _{k=0}^{m-1}{s}^{\rho -1-k}{\vartheta }^k(\ell ,\varrho ,0)+L\left[g(\ell ,\varrho ,{\tau }_1)\right]\right)\right]-L^{-1}\left[\frac{1}{s^{\rho }}L\right.\hfill \\ \left.\left[\sum _{i=0}^{\infty }R\left[{\vartheta }_i(\ell ,\varrho ,{\tau }_1)\right]+N\left[{\vartheta }_0(\ell ,\varrho ,{\tau }_1)\right]+\sum _{i=1}^{\infty }\left\{N\left(\sum _{k=0}^i{\vartheta }_k\left(\ell ,\varrho ,{\tau }_1\right)-N\left(\sum _{k=0}^{i-1}{\vartheta }_k(\ell ,\varrho ,{\tau }_1)\right)\right)\right\}\right]\right].\hfill \end{array}$$

(14)

Using Equation (14), we defined the following iterative formula [44]

$${\vartheta }_0(\ell ,\varrho ,{\tau }_1)=L^{-1}\left[\frac{1}{s^{\rho }}\left(\sum _{k=0}^{m-1}{s}^{\rho -1-k}{\vartheta }^k(\ell ,\varrho ,0)+\frac{1}{s^{\rho }}L\left(g(\ell ,\varrho ,{\tau }_1)\right)\right)\right],$$

(15)

$${\vartheta }_1(\ell ,\varrho ,{\tau }_1)=-L^{-1}\left[\frac{1}{s^{\rho }}L[R\left[{\vartheta }_0(\ell ,\varrho ,{\tau }_1)\right]+N\left[{\vartheta }_0(\ell ,\varrho ,{\tau }_1)\right]\right],$$

(16)

$$\begin{array}{c}{\vartheta }_{m+1}(\ell ,\varrho ,{\tau }_1)=-L^{-1}\left[\frac{1}{s^{\rho }}L\left[R\left({\vartheta }_m(\ell ,\varrho ,{\tau }_1)\right)-\left\{N\left(\sum _{k=0}^m{\vartheta }_k(\ell ,\varrho ,{\tau }_1)\right)-N\left(\sum _{k=0}^{m-1}{\vartheta }_k(\ell ,\varrho ,{\tau }_1)\right)\right\}\right]\right]\hfill \\ m\ge 1\hfill \\ .\hfill \end{array}$$

(17)

The approximate m-term solution of Equations (6) and (7) in form of series as [44]

$$\vartheta (\ell ,\varrho ,{\tau }_1)\cong {\vartheta }_0(\ell ,\varrho ,{\tau }_1)+{\vartheta }_1(\ell ,\varrho ,{\tau }_1)+{\vartheta }_2(\ell ,\varrho ,{\tau }_1)+\dots +{\vartheta }_m(\ell ,\varrho ,{\tau }_1),m=1,2,\dots$$

(18)

4. Implementation of the Method

In this section, ILTM and ISTM are applied to examine the solution of fractional-order heat-like equations. It has been shown that the ILTM and ISTM are an accurate and appropriate analytical technique to solve non-linear FPDEs.

Example 1.

Consider the following one-dimensional fractional-order heat equation [8,14,48]:

$$D_{{\tau }_1}^{\rho }\vartheta (\ell ,{\tau }_1)=\frac{1}{2}{\ell }^2\frac{{\partial }^2\vartheta }{\partial {\ell }^2},0<\ell <1,1<\rho \le 2,{\tau }_1>0,$$

(19)

with initial condition

$$\vartheta (\ell ,0)={\ell }^2.$$

(20)

The Sumudu transformation to Equation (19) is expressed as

$$\begin{array}{cc}\hfill & u^{-\rho }S\left[\vartheta (\ell ,{\tau }_1)\right]-\sum _{k=0}^{m-1}{\vartheta }^{\left(k\right)}(\ell ,0)u^{-\rho +k}=S\left[\left(\frac{1}{2}{\ell }^2\frac{{\partial }^2\vartheta }{\partial {\ell }^2}\right)\right],\hfill \\ \hfill & u^{-\rho }S\left[\vartheta (\ell ,{\tau }_1)\right]={\vartheta }^{\left(0\right)}(\ell ,0)\frac{u^{-\rho }}{u}+S\left[\left(\frac{1}{2}{\ell }^2\frac{{\partial }^2\vartheta }{\partial {\ell }^2}\right)\right],\hfill \end{array}$$

(21)

$$S\left[\vartheta (\ell ,{\tau }_1)\right]=\frac{\vartheta (\ell ,0)}{u}+u^{\rho }\left[\left(\frac{1}{2}{\ell }^2\frac{{\partial }^2\vartheta }{\partial {\ell }^2}\right)\right].$$

(22)

Using the inverse Sumudu transformation of Equation (22),we get

$$\vartheta (\ell ,{\tau }_1)=S^{-1}\left[\frac{\vartheta (\ell ,0)}{u}\right]+S^{-1}\left[u^{\rho }S\left(\frac{1}{2}{\ell }^2\frac{{\partial }^2\vartheta }{\partial {\ell }^2}\right)\right].$$

(23)

Using the iterative technique described in Equations (11)–(13), we obtain the following solution components of Example 1

$$\begin{array}{cc}\hfill & {\vartheta }_0(\ell ,{\tau }_1)={\ell }^2,\hfill \\ \hfill & {\vartheta }_1(\ell ,{\tau }_1)=S^{-1}\left[u^{\rho }\left\{S\left(\frac{1}{2}{\ell }^2\frac{{\partial }^2{\vartheta }_0}{\partial {\ell }^2}\right)\right\}\right],\hfill \end{array}$$

(24)

$${\vartheta }_1(\ell ,{\tau }_1)=\frac{{\tau }_1^{\rho }{\ell }^2}{\mathsf{\Gamma }(\rho +1)}.$$

(25)

$$\begin{array}{cc}\hfill & {\vartheta }_2(\ell ,{\tau }_1)=S^{-1}\left[u^{\rho }\left\{S\left(\frac{1}{2}{\ell }^2\frac{{\partial }^2{\vartheta }_1}{\partial {\ell }^2}\right)\right\}\right],\hfill \\ \hfill & {\vartheta }_2(\ell ,{\tau }_1)=\frac{{\tau }_1^{2\rho }{\ell }^2}{\mathsf{\Gamma }(2\rho +1)}.\hfill \end{array}$$

(26)

$$\begin{array}{cc}\hfill & {\vartheta }_3(\ell ,\tau )=S^{-1}\left[u^{\rho }\left\{S\left(\frac{1}{2}{\ell }^2\frac{{\partial }^2{\vartheta }_2}{\partial {\ell }^2}\right)\right\}\right],\hfill \\ \hfill & {\vartheta }_3(\ell ,{\tau }_1)=\frac{{\tau }_1^{3\rho }{\ell }^2}{\mathsf{\Gamma }(3\rho +1)}.\hfill \end{array}$$

(27)

The analytical solution of the series form is given as

$$\begin{array}{cc}\hfill & \vartheta (\ell ,{\tau }_1)={\vartheta }_0(\ell ,{\tau }_1)+{\vartheta }_1(\ell ,{\tau }_1)+{\vartheta }_2(\ell ,{\tau }_1)+{\vartheta }_3(\ell ,{\tau }_1)+,\dots ,\hfill \\ \hfill & \vartheta (\ell ,{\tau }_1)={\ell }^2+\frac{{\tau }_1^{\rho }{\ell }^2}{\mathsf{\Gamma }(\rho +1)}+\frac{{\tau }_1^{2\rho }{\ell }^2}{\mathsf{\Gamma }(2\rho +1)}+\frac{{\tau }_1^{3\rho }{\ell }^2}{\mathsf{\Gamma }(3\rho +1)}+,\dots .\hfill \end{array}$$

(28)

Therefore, the approximate solution of Equation (19) is given as

$$={\ell }^2\left(1+\frac{{\tau }_1^{\rho }}{\mathsf{\Gamma }(\rho +1)}+\frac{{\tau }_1^{2\rho }}{\mathsf{\Gamma }(2\rho +1)}+\frac{{\tau }_1^{3\rho }}{\mathsf{\Gamma }(3\rho +1)}\dots \right).$$

(29)

Now that in the case $\rho =1$

$$={\ell }^2\left(1+{\tau }_1+\frac{{\tau }_1^2}{2!}+\frac{{\tau }_1^3}{3!}\dots \right),$$

(30)

$$\vartheta (\ell ,{\tau }_1)={\ell }^2{exp}^{\left({\tau }_1\right)}.$$

(31)

This is the exact solution for this case.

Figure 1 represents the exact and ISTM solutions in graphs (a) and (b), respectively, for Example 1 at $\rho =1$. The best contact is observed between the exact and ISTM solutions of Example 1. In Figure 2, the solutions at different fractional orders of the derivatives are calculated for Example 1. In Figure 2, the fractional order solutions of Example 1 at $\gamma =1$, $0.8$, $0.6$ and $0.5$ are expressed in three and two dimensions by sub-figures (a) and (b) respectively. The graphical representation in Figure 2 confirmed the convergence phenomena of fractional order solutions towards the integer-order solution of Example 1.

Example 2.

Consider the two-dimensional fractional-order heat equation [8,14,48]:

$$D_{{\tau }_1}^{\rho }\vartheta (\ell ,\varrho ,{\tau }_1)=\frac{{\partial }^2\vartheta }{\partial {\ell }^2}+\frac{{\partial }^2\vartheta }{\partial {\varrho }^2},0<\ell ,\varrho <2\pi ,{\tau }_1>0,0<\rho \le 1,$$

(32)

with initial condition

$$\vartheta (\ell ,\varrho ,0)=sin\left(\ell \right)sin\left(\varrho \right).$$

(33)

The Laplace transformation to Equation (32) is expressed as

$$\begin{array}{cc}\hfill & s^{\rho }L\left[\vartheta (\ell ,\varrho ,{\tau }_1)\right]-\sum _{k=0}^{m-1}{\vartheta }^{\left(k\right)}(\ell ,\varrho ,0)s^{\rho -k-1}=L\left[\left(\frac{{\partial }^2\vartheta }{\partial {\ell }^2}+\frac{{\partial }^2\vartheta }{\partial {\varrho }^2}\right)\right],\hfill \\ \hfill & s^{\rho }L\left[\vartheta (\ell ,\varrho ,{\tau }_1)\right]={\vartheta }^{\left(0\right)}(\ell ,\varrho ,0)\frac{s^{\rho }}{s}+L\left[\left(\frac{{\partial }^2\vartheta }{\partial {\ell }^2}+\frac{{\partial }^2\vartheta }{\partial {\varrho }^2}\right)\right],\hfill \end{array}$$

(34)

$$L\left[\vartheta (\ell ,\varrho ,{\tau }_1)\right]=\frac{\vartheta (\ell ,\varrho ,0)}{s}+\frac{1}{s^{\rho }}\left[\left(\frac{{\partial }^2\vartheta }{\partial {\ell }^2}+\frac{{\partial }^2\vartheta }{\partial {\varrho }^2}\right)\right].$$

(35)

Using inverse Laplace transformation of Equation (35),we get

$$\vartheta (\ell ,\varrho ,{\tau }_1)=L^{-1}\left[\frac{\vartheta (\ell ,\varrho ,0)}{s}\right]+L^{-1}\left[\frac{1}{s^{\rho }}L\left(\frac{{\partial }^2\vartheta }{\partial {\ell }^2}+\frac{{\partial }^2\vartheta }{\partial {\varrho }^2}\right)\right].$$

(36)

Using iterative technique describe in Equations (11)–(13), we obtain the following solution components of Example 2

$$\begin{array}{cc}\hfill & {\vartheta }_0(\ell ,\varrho ,{\tau }_1)=sin\ell sin\varrho ,\hfill \\ \hfill & {\vartheta }_1(\ell ,\varrho ,{\tau }_1)=L^{-1}\left[\frac{1}{s^{\rho }}\left\{L\left(\frac{{\partial }^2{\vartheta }_0}{\partial {\ell }^2}+\frac{{\partial }^2{\vartheta }_0}{\partial {\varrho }^2}\right)\right\}\right],\hfill \end{array}$$

(37)

$$=-2\frac{{\tau }_1^{\rho }sin\ell sin\varrho }{\mathsf{\Gamma }(\rho +1)}.$$

(38)

$$\begin{array}{cc}\hfill & {\vartheta }_2(\ell ,\varrho ,{\tau }_1)=L^{-1}\left[\frac{1}{s^{\rho }}\left\{L\left(\frac{{\partial }^2{\vartheta }_1}{\partial {\ell }^2}+\frac{{\partial }^2{\vartheta }_1}{\partial {\varrho }^2}\right)\right\}\right],\hfill \\ \hfill & =4\frac{{\tau }_1^{2\rho }sin\ell sin\varrho }{\mathsf{\Gamma }(2\rho +1)}.\hfill \end{array}$$

(39)

$$\begin{array}{cc}\hfill & {\vartheta }_3(\ell ,\varrho ,{\tau }_1)=L^{-1}\left[\frac{1}{s^{\rho }}\left\{L\left(\frac{{\partial }^2{\vartheta }_2}{\partial {\ell }^2}+\frac{{\partial }^2{\vartheta }_2}{\partial {\varrho }^2}\right)\right\}\right],\hfill \\ \hfill & =-8\frac{{\tau }_1^{3\rho }sin\ell sin\varrho }{\mathsf{\Gamma }(3\rho +1)}.\hfill \end{array}$$

(40)

The analytical solution of series form is given as

$$\begin{array}{cc}\hfill & \vartheta (\ell ,\varrho ,{\tau }_1)={\vartheta }_0(\ell ,\varrho ,{\tau }_1)+{\vartheta }_1(\ell ,\varrho ,{\tau }_1)+{\vartheta }_2(\ell ,\varrho ,{\tau }_1)+{\vartheta }_3(\ell ,\varrho ,{\tau }_1)+,\dots ,\hfill \\ \hfill & =sin\ell sin\varrho -2\frac{{\tau }_1^{\rho }sin\ell sin\varrho }{\mathsf{\Gamma }(\rho +1)}+4\frac{{\tau }_1^{2\rho }sin\ell sin\varrho }{\mathsf{\Gamma }(2\rho +1)}-8\frac{{\tau }_1^{3\rho }sin\ell sin\varrho }{\mathsf{\Gamma }(3\rho +1)}+,\dots .\hfill \end{array}$$

(41)

Therefore the approximate solution of the equation is given as

$$=sin\ell sin\varrho \left(1-2\frac{{\tau }_1^{\rho }}{\mathsf{\Gamma }(\rho +1)}+4\frac{{\tau }_1^{2\rho }}{\mathsf{\Gamma }(2\rho +1)}-8\frac{{\tau }_1^{3\rho }}{\mathsf{\Gamma }(3\rho +1)}+\dots \right),$$

(42)

when $\rho =1$ we get,

$$=sin\ell sin\varrho \left(1-2{\tau }_1+4\frac{{\tau }_1^2}{2!}-8\frac{{\tau }_1^3}{3!}\dots \right),$$

(43)

the exact solution is

$$\vartheta (\ell ,\varrho ,{\tau }_1)=sin\left(\ell \right)sin\left(\varrho \right){exp}^{(-2{\tau }_1)}.$$

(44)

The comparison between ILTM and the exact solution has been done in Figure 3 for Example 2. The comparison has shown the closed resemblance between the actual and ILTM solutions. In Figure 4, the error analysis of ILTM has been done for Example 2 at $\rho =1$. It is observed that the error associated with ILTM is consistent throughout the collocation points.

Example 3.

Consider the following two-dimensional fractional-order heat equation [8,14]:

$$D_{{\tau }_1}^{\rho }\vartheta (\ell ,\varrho ,{\tau }_1)=\frac{{\varrho }^2}{2}\frac{{\partial }^2\vartheta }{\partial {\ell }^2}+\frac{{\ell }^2}{2}\frac{{\partial }^2\vartheta }{\partial {\varrho }^2},0<\rho \le 1,$$

(45)

with initial condition

$$\vartheta (\ell ,\varrho ,0)={\varrho }^2.$$

(46)

The Laplace transformation to Equation (45) is expressed as

$$\begin{array}{cc}\hfill & s^{\rho }L\left[\vartheta (\ell ,\varrho ,{\tau }_1)\right]-\sum _{k=0}^{m-1}{\vartheta }^{\left(k\right)}(\ell ,\varrho ,0)s^{\rho -k-1}=L\left[\left(\frac{{\varrho }^2}{2}\frac{{\partial }^2\vartheta }{\partial {\ell }^2}+\frac{{\ell }^2}{2}\frac{{\partial }^2\vartheta }{\partial {\varrho }^2}\right)\right],\hfill \\ \hfill & s^{\rho }L\left[\vartheta (\ell ,\varrho ,{\tau }_1)\right]={\vartheta }^{\left(0\right)}(\ell ,\varrho ,0)\frac{s^{\rho }}{s}+L\left[\left(\frac{{\varrho }^2}{2}\frac{{\partial }^2\vartheta }{\partial {\ell }^2}+\frac{{\ell }^2}{2}\frac{{\partial }^2\vartheta }{\partial {\varrho }^2}\right)\right],\hfill \end{array}$$

(47)

$$L\left[\vartheta (\ell ,\varrho ,{\tau }_1)\right]=\frac{\vartheta (\ell ,\varrho ,0)}{s}+\frac{1}{s^{\rho }}\left[\left(\frac{{\varrho }^2}{2}\frac{{\partial }^2\vartheta }{\partial {\ell }^2}+\frac{{\ell }^2}{2}\frac{{\partial }^2\vartheta }{\partial {\varrho }^2}\right)\right].$$

(48)

Using the inverse Laplace transformation of Equation (48), we get

$$\vartheta (\ell ,\varrho ,{\tau }_1)=L^{-1}\left[\frac{\vartheta (\ell ,\varrho ,0)}{s}\right]+L^{-1}\left[\frac{1}{s^{\rho }}L\left(\frac{{\varrho }^2}{2}\frac{{\partial }^2\vartheta }{\partial {\ell }^2}+\frac{{\ell }^2}{2}\frac{{\partial }^2\vartheta }{\partial {\varrho }^2}\right)\right].$$

(49)

Using the iterative technique described in Equations (11)–(13), we obtain the following solution components of Example 3

$$\begin{array}{cc}\hfill & {\vartheta }_0(\ell ,\varrho ,{\tau }_1)={\varrho }^2,\hfill \\ \hfill & {\vartheta }_1(\ell ,\varrho ,{\tau }_1)=L^{-1}\left[\frac{1}{s^{\rho }}\left\{L\left(\frac{{\varrho }^2}{2}\frac{{\partial }^2{\vartheta }_0}{\partial {\ell }^2}+\frac{{\ell }^2}{2}\frac{{\partial }^2{\vartheta }_0}{\partial {\varrho }^2}\right)\right\}\right],\hfill \end{array}$$

(50)

$$={\ell }^2\frac{{\tau }_1^{\rho }}{\mathsf{\Gamma }(\rho +1)}.$$

(51)

$$\begin{array}{cc}\hfill & {\vartheta }_2(\ell ,\varrho ,{\tau }_1)=L^{-1}\left[\frac{1}{s^{\rho }}\left\{L\left(\frac{{\varrho }^2}{2}\frac{{\partial }^2{\vartheta }_1}{\partial {\ell }^2}+\frac{{\ell }^2}{2}\frac{{\partial }^2{\vartheta }_1}{\partial {\varrho }^2}\right)\right\}\right],\hfill \\ \hfill & ={\varrho }^2\frac{{\tau }_1^{2\rho }}{\mathsf{\Gamma }(2\rho +1)}.\hfill \end{array}$$

(52)

$$\begin{array}{cc}\hfill & {\vartheta }_3(\ell ,\varrho ,{\tau }_1)=L^{-1}\left[\frac{1}{s^{\rho }}\left\{L\left(\frac{{\varrho }^2}{2}\frac{{\partial }^2{\vartheta }_2}{\partial {\ell }^2}+\frac{{\ell }^2}{2}\frac{{\partial }^2{\vartheta }_2}{\partial {\varrho }^2}\right)\right\}\right],\hfill \\ \hfill & ={\ell }^2\frac{{\tau }_1^{3\rho }}{\mathsf{\Gamma }(3\rho +1)}.\hfill \end{array}$$

(53)

$$\begin{array}{cc}\hfill & {\vartheta }_4(\ell ,\varrho ,{\tau }_1)=L^{-1}\left[\frac{1}{s^{\rho }}\left\{L\left(\frac{{\varrho }^2}{2}\frac{{\partial }^2{\vartheta }_3}{\partial {\ell }^2}+\frac{{\ell }^2}{2}\frac{{\partial }^2{\vartheta }_3}{\partial {\varrho }^2}\right)\right\}\right],\hfill \\ \hfill & ={\varrho }^2\frac{{\tau }_1^{4\rho }}{\mathsf{\Gamma }(4\rho +1)}.\hfill \end{array}$$

(54)

The series form of the analytical solution is given as

$$\begin{array}{cc}\hfill & \vartheta (\ell ,\varrho ,{\tau }_1)={\vartheta }_0(\ell ,\varrho ,{\tau }_1)+{\vartheta }_1(\ell ,\varrho ,{\tau }_1)+{\vartheta }_2(\ell ,\varrho ,{\tau }_1)+{\vartheta }_3(\ell ,\varrho ,{\tau }_1)+{\vartheta }_4(\ell ,\varrho ,{\tau }_1)+,\dots ,\hfill \\ \hfill & ={\varrho }^2+{\ell }^2\frac{{\tau }_1^{\rho }}{\mathsf{\Gamma }(\rho +1)}+{\varrho }^2\frac{{\tau }_1^{2\rho }}{\mathsf{\Gamma }(2\rho +1)}+{\ell }^2\frac{{\tau }_1^{3\rho }}{\mathsf{\Gamma }(3\rho +1)}+{\varrho }^2\frac{{\tau }_1^{4\rho }}{\mathsf{\Gamma }(4\rho +1)}+,\dots .\hfill \end{array}$$

(55)

Therefore the approximate solution of the equation is given as

$$={\ell }^2\left\{\left(\frac{{\tau }_1^{\rho }}{\mathsf{\Gamma }(\rho +1)}+\frac{{\tau }_1^{3\rho }}{\mathsf{\Gamma }(3\rho +1)}+\frac{{\tau }_1^{5\rho }}{\mathsf{\Gamma }(5\rho +1)}\dots \right)+\left({\varrho }^{2}1+\frac{{\tau }_1^{2\rho }}{\mathsf{\Gamma }(2\rho +1)}+\frac{{\tau }_1^{4\rho }}{\mathsf{\Gamma }(4\rho +1)}\dots \right)\right\},$$

(56)

when $\rho =1$ we get,

$$={\ell }^2\left({\tau }_1+\frac{{\tau }_1^3}{3!}+\frac{{\tau }_1^5}{5!}\dots )+{\varrho }^2(1+\frac{{\tau }_1^2}{2!}+\frac{{\tau }_1^4}{4!}\dots \right),$$

(57)

the exact solution is

$$\vartheta (\ell ,\varrho ,{\tau }_1)={\ell }^{2}sinh{\tau }_1+{\varrho }^{2}cosh{\tau }_1.$$

(58)

In Figure 5, the graphical representation of the ILTM and exact solutions of Example 3 are presented. The closed contact between the exact and ILTM solutions in graphs (a) and (b) of Figure 5 for Example 3 is observed. In Figure 6, the ILTM solutions at different fractional orders for Example 3 is shown. The analysis shows that there is a strong convergence of the fractional-order solutions towards the integer order solution of Example 3. The solution convergence can be seen in both one and two-dimensional graphs represented by (a) and (b), respectively.

Example 4.

Consider the following three-dimensional fractional-order heat equation [8,14,48]:

$$D_{{\tau }_1}^{\rho }\vartheta (\ell ,\varrho ,\kappa ,{\tau }_1)={\ell }^4{\varrho }^4{\kappa }^4+\frac{1}{36}\left({\ell }^2\frac{{\partial }^2\vartheta }{\partial {\ell }^2}+{\varrho }^2\frac{{\partial }^2\vartheta }{\partial {\varrho }^2}+{\kappa }^2\frac{{\partial }^2\vartheta }{\partial {\kappa }^2}\right),0<\ell ,\varrho ,\kappa <1,0<\rho \le 1,$$

(59)

with initial condition

$$\vartheta (\ell ,\varrho ,\kappa ,0)=0.$$

(60)

The Sumudu transformation to Equation (59) is expressed as

$$\begin{array}{c}{u}^{-\rho }S\left[\vartheta (\ell ,\varrho ,\kappa ,{\tau }_1)\right]-\sum _{k=0}^{m-1}{\vartheta }^{\left(k\right)}(\ell ,\varrho ,\kappa ,0)u^{-\rho +k}=S\left[\left(el{l}^4{\varrho }^4{\kappa }^4\right)\right]+S\left[\frac{1}{36}\left({\ell }^2\frac{{\partial }^2\vartheta }{\partial {\ell }^2}+{\varrho }^2\frac{{\partial }^2\vartheta }{\partial {\varrho }^2}+{\kappa }^2\frac{{\partial }^2\vartheta }{\partial {\kappa }^2}\right)\right],\hfill \\ u^{-\rho }S\left[\vartheta (\ell ,\varrho ,\kappa ,{\tau }_1)\right]={\vartheta }^{\left(0\right)}(\ell ,\varrho ,\kappa ,0)\frac{u^{-\rho }}{u}+S\left[\left({\ell }^4{\varrho }^4{\kappa }^4\right)\right]+S\left[\frac{1}{36}\left({\ell }^2\frac{{\partial }^2\vartheta }{\partial {\ell }^2}+{\varrho }^2\frac{{\partial }^2\vartheta }{\partial {\varrho }^2}+{\kappa }^2\frac{{\partial }^2\vartheta }{\partial {\kappa }^2}\right)\right],\hfill \end{array}$$

(61)

$$S\left[\vartheta (\ell ,\varrho ,\kappa ,{\tau }_1)\right]=\left[\frac{\vartheta (\ell ,\varrho ,\kappa ,0)}{u}+u^{\rho }S\left({\ell }^4{\varrho }^4{\kappa }^4\right)\right]+u^{\rho }S\left[\frac{1}{36}\left({\ell }^2\frac{{\partial }^2\vartheta }{\partial {\ell }^2}+{\varrho }^2\frac{{\partial }^2\vartheta }{\partial {\varrho }^2}+{\kappa }^2\frac{{\partial }^2\vartheta }{\partial {\kappa }^2}\right)\right].$$

(62)

Using the inverse Sumudu transformation of Equation (62),we get

$$\vartheta (\ell ,\varrho ,\kappa ,{\tau }_1)=S^{-1}\left[\frac{\vartheta (\ell ,\varrho ,\kappa ,0)}{u}+u^{\rho }S\left({\ell }^4{\varrho }^4{\kappa }^4\right)\right]+S^{-1}\left[u^{\rho }\left\{S\left(\frac{1}{36}\left({\ell }^2\frac{{\partial }^2\vartheta }{\partial {\ell }^2}+{\varrho }^2\frac{{\partial }^2\vartheta }{\partial {\varrho }^2}+{\kappa }^2\frac{{\partial }^2\vartheta }{\partial {\kappa }^2}\right)\right)\right\}\right].$$

(63)

Using the Iterative technique described in Equations (11)–(13), we obtain the following solution components of Example 4

$$\begin{array}{cc}\hfill & {\vartheta }_0(\ell ,\varrho ,\kappa ,{\tau }_1)={\ell }^4{\varrho }^4{\kappa }^4\frac{{\tau }_1^{\rho }}{\mathsf{\Gamma }(\rho +1)},\hfill \\ \hfill & {\vartheta }_1(\ell ,\varrho ,\kappa ,{\tau }_1)=S^{-1}\left[u^{\rho }\left\{S\left(\frac{1}{36}\left({\ell }^2\frac{{\partial }^2\vartheta }{\partial {\ell }^2}+{\varrho }^2\frac{{\partial }^2\vartheta }{\partial {\varrho }^2}+{\kappa }^2\frac{{\partial }^2\vartheta }{\partial {\kappa }^2}\right)\right)\right\}\right],\hfill \end{array}$$

(64)

$$={\ell }^4{\varrho }^4{\kappa }^4\frac{{\tau }_1^{2\rho }}{\mathsf{\Gamma }(2\rho +1)}.$$

(65)

$$\begin{array}{cc}\hfill & {\vartheta }_2(\ell ,\varrho ,\kappa ,{\tau }_1)=S^{-1}\left[u^{\rho }\left\{S\left(\frac{1}{36}\left({\ell }^2\frac{{\partial }^2\vartheta }{\partial {\ell }^2}+{\varrho }^2\frac{{\partial }^2\vartheta }{\partial {\varrho }^2}+{\kappa }^2\frac{{\partial }^2\vartheta }{\partial {\kappa }^2}\right)\right)\right\}\right],\hfill \\ \hfill & ={\ell }^4{\varrho }^4{\kappa }^4\frac{{\tau }_1^{3\rho }}{\mathsf{\Gamma }(3\rho +1)}.\hfill \end{array}$$

(66)

$${\vartheta }_3(\ell ,\varrho ,\kappa ,{\tau }_1)=S^{-1}\left[u^{\rho }\left\{S\left(\frac{1}{36}\left({\ell }^2\frac{{\partial }^2\vartheta }{\partial {\ell }^2}+{\varrho }^2\frac{{\partial }^2\vartheta }{\partial {\varrho }^2}+{\kappa }^2\frac{{\partial }^2\vartheta }{\partial {\kappa }^2}\right)\right)\right\}\right],$$

(67)

$$={\ell }^4{\varrho }^4{\kappa }^4\frac{{\tau }_1^{4\rho }}{\mathsf{\Gamma }(4\rho +1)}.$$

(68)

$${\vartheta }_4(\ell ,\varrho ,\kappa ,{\tau }_1)=S^{-1}\left[u^{\rho }\left\{S\left(\frac{1}{36}\left({\ell }^2\frac{{\partial }^2\vartheta }{\partial {\ell }^2}+{\varrho }^2\frac{{\partial }^2\vartheta }{\partial {\varrho }^2}+{\kappa }^2\frac{{\partial }^2\vartheta }{\partial {\kappa }^2}\right)\right)\right\}\right],$$

(69)

$$={\ell }^4{\varrho }^4{\kappa }^4\frac{{\tau }_1^{5\rho }}{\mathsf{\Gamma }(5\rho +1)}.$$

(70)

The analytical solution of the series form is given as

$$\begin{array}{c}\vartheta (\ell ,\varrho ,\kappa ,{\tau }_1)={\vartheta }_0(\ell ,\varrho ,\kappa ,{\tau }_1)+{\vartheta }_1(\ell ,\varrho ,\kappa ,{\tau }_1)+{\vartheta }_2(\ell ,\varrho ,\kappa ,{\tau }_1)+{\vartheta }_3(\ell ,\varrho ,\kappa ,{\tau }_1)+{\vartheta }_4(\ell ,\varrho ,\kappa ,{\tau }_1)+\dots ,\hfill \\ ={\ell }^4{\varrho }^4{\kappa }^4\frac{{\tau }_1^{\rho }}{\mathsf{\Gamma }(\rho +1)}+{\ell }^4{\varrho }^4{\kappa }^4\frac{{\tau }_1^{2\rho }}{\mathsf{\Gamma }(2\rho +1)}+{\ell }^4{\varrho }^4{\kappa }^4\frac{{\tau }_1^{3\rho }}{\mathsf{\Gamma }(3\rho +1)}+{\ell }^4{\varrho }^4{\kappa }^4\frac{{\tau }_1^{4\rho }}{\mathsf{\Gamma }(4\rho +1)}+\dots .\hfill \end{array}$$

(71)

Therefore the approximate solution of the equation is given as

$$={\ell }^4{\varrho }^4{\kappa }^4\left(\frac{{\tau }_1^{\rho }}{\mathsf{\Gamma }(\rho +1)}+\frac{{\tau }_1^{2\rho }}{\mathsf{\Gamma }(2\rho +1)}+\frac{{\tau }_1^{3\rho }}{\mathsf{\Gamma }(3\rho +1)}+\frac{{\tau }_1^{4\rho }}{\mathsf{\Gamma }(4\rho +1)}\dots \right),$$

(72)

when $\rho =1$ we get,

$$={\ell }^4{\varrho }^4{\kappa }^4\left({\tau }_1+\frac{{\tau }_1^2}{2!}+\frac{{\tau }_1^3}{3!}+\frac{{\tau }_1^4}{4!}\dots \right),$$

(73)

the exact solution is

$$\vartheta (\ell ,\varrho ,\kappa ,{\tau }_1)={\ell }^4{\varrho }^4{\kappa }^4({exp}^{{\tau }_1}-1).$$

(74)

In Figure 7, the fractional order solutions of Example 4 are given in both dimensional graphs (a) and (b), respectively. In both cases, the convergence phenomena of fractional-order solutions to the integer-order solution can be seen for Example 4. The implementation of the suggested method for various numerical examples has supported the validity of the proposed method. The present technique has the greater capacity to solve partial differential equations of fractional-order and integer-order as well.

5. Conclusions

The fractional view analysis of the heat like equations, using an efficient analytical approach, was the focus of the present research work. The approximate analytical solutions for both fractional and integer-order heat like equations are obtained in a sophisticated manner. The graphical analysis of the obtained solutions has been done successfully. The analysis has confirmed the strong agreement between the proposed and exact solutions to the problems. The present method have proved to be an effective and straightforward procedure as compared with other analytical and numerical techniques. Moreover, the suggested method required fewer calculations and therefore can be extended for the solutions of other fractional-order problems.