Thermal Gradients with Sintered Solid State Electrolytes in Lithium-Ion Batteries
Abstract
1. Introduction
1.1. Role of the Electrolyte
1.2. Heat and Thermal Conductivity
2. Experimental
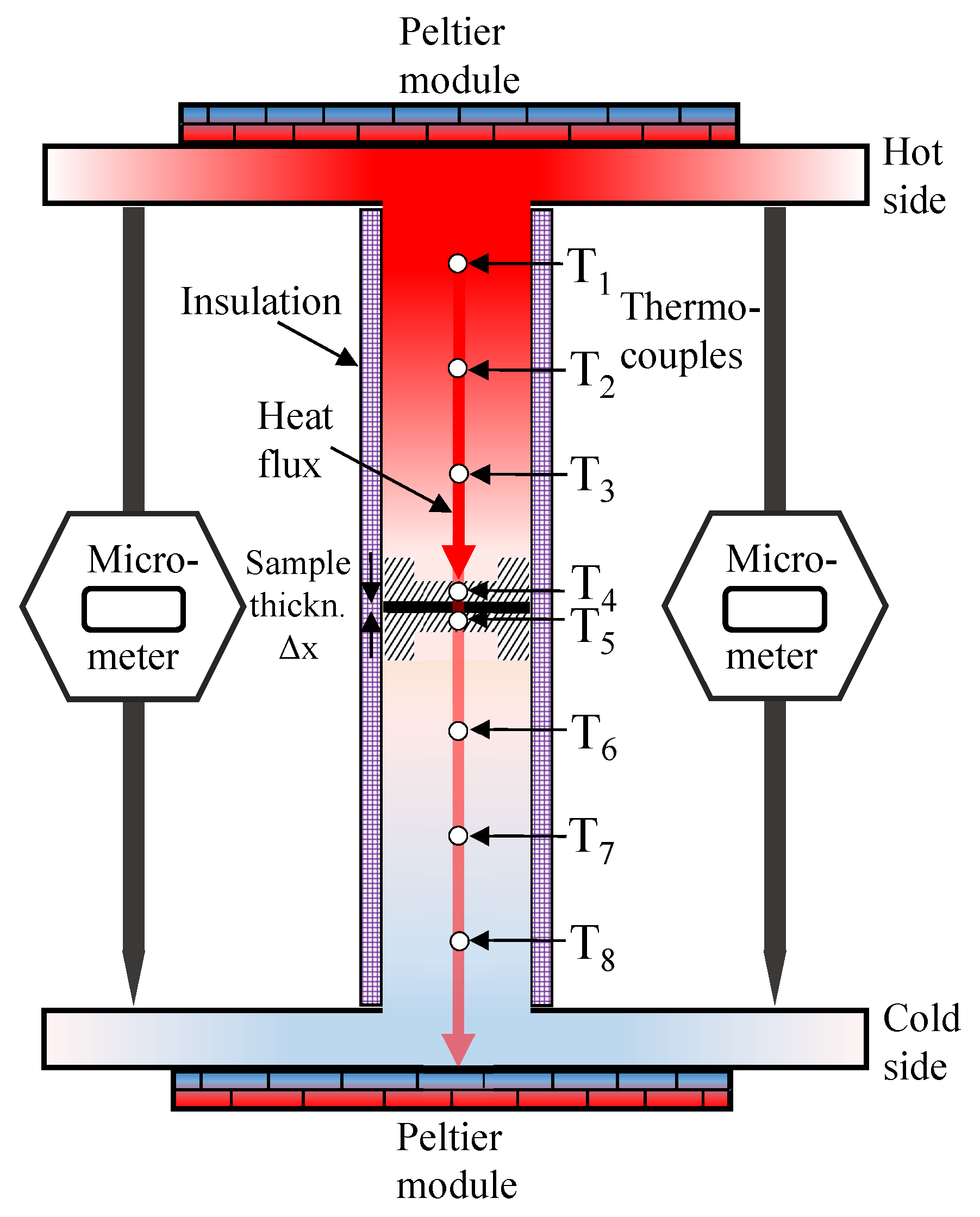
2.1. Thermal Conductivity Measurements
2.2. Sample Preparation
2.3. Modeling of the Temperature Distribution
3. Results and Discussion
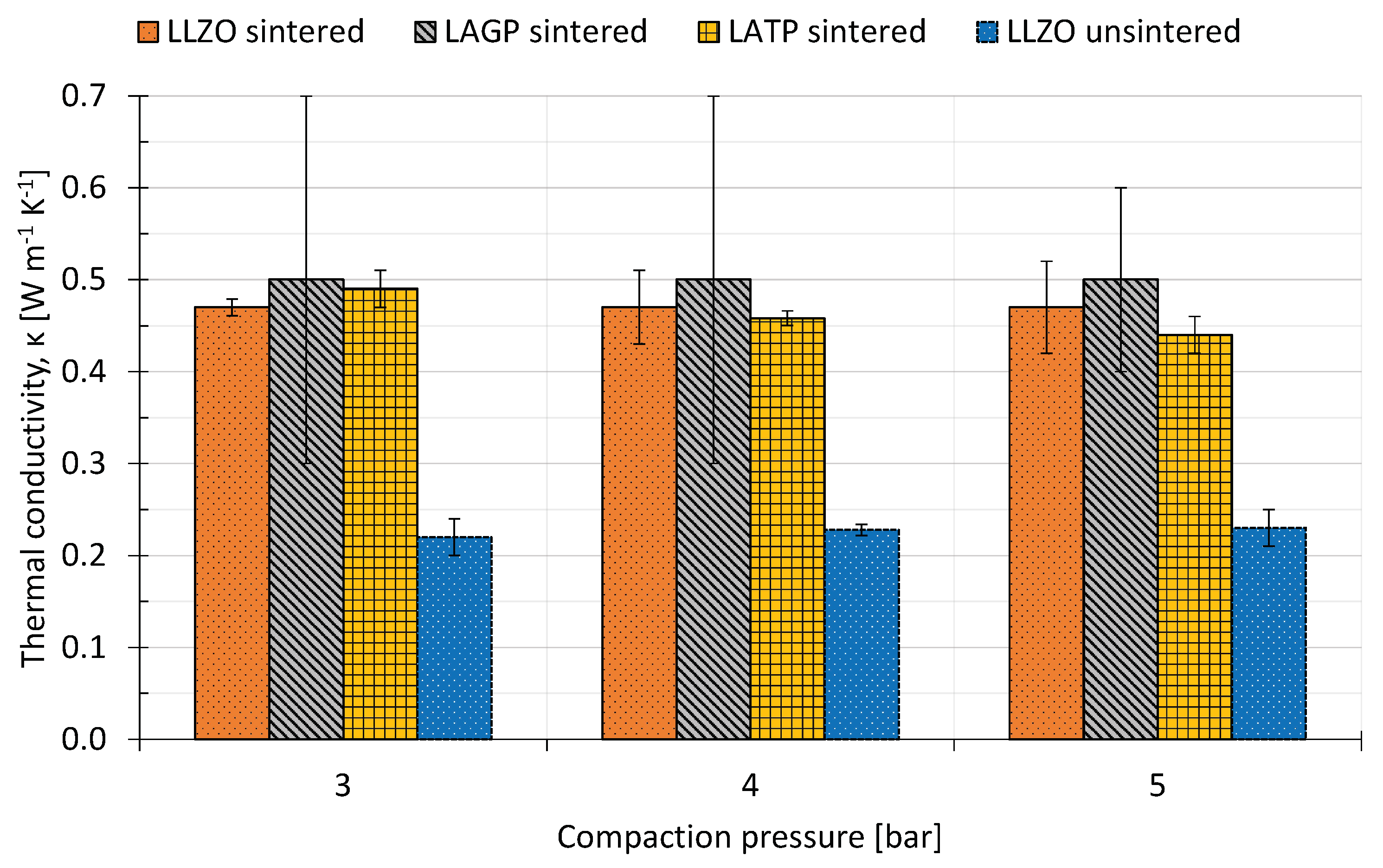
3.1. Thermal Conductivity Measurements
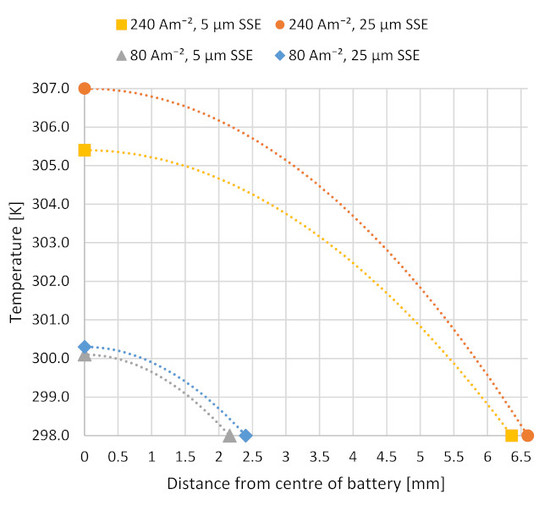
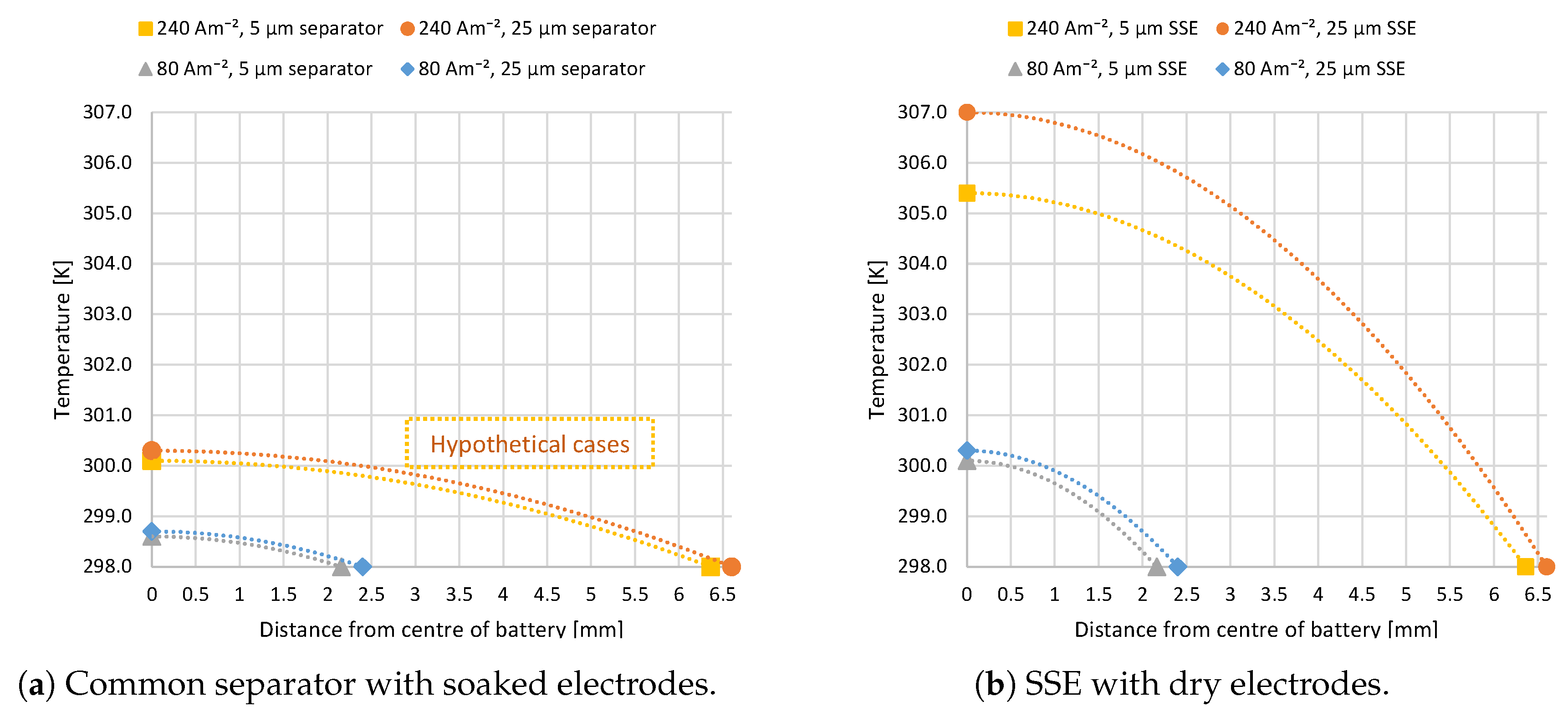
3.2. Modeling of Discharge Temperature Distribution
4. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Burheim, O.S. Engineering Energy Storage, 1st ed.; Elsevier Academic Press: London, UK, 2017; ISBN 9780128141007. [Google Scholar]
- Kasnatscheew, J.; Rodehorst, U.; Streipert, B.; Wiemers-Meyer, S.; Jakelski, R.; Wagner, R.; Laskovic, I.C.; Winter, M. Learning from Overpotentials in Lithium Ion Batteries: A Case Study on the LiNi1/3Co1/3Mn1/3O2 (NCM) Cathode. J. Electrochem. Soc. 2016, 163, A2943–A2950. [Google Scholar] [CrossRef]
- Canaday, J.; Wheat, T.; Kuriakose, A.; Ahmad, A. A polarization model for protonic solid electrolyte fuel cells. Int. J. Hydrogen Energy 1987, 12, 151–157. [Google Scholar] [CrossRef]
- Rao, V.; Kluy, N.; Ju, W.; Stimming, U. Handbook of Membrane Separations: Chemical, Pharmaceutical, Food, and Biotechnological Applications; CRC Press: Boca Raton, FL, USA, 2015; Chapter 21; pp. 567–609. [Google Scholar]
- Mikolajczak, C.; Kahn, M.; White, K.; Long, R.T. Lithium-Ion Batteries Hazard and Use Assessment; Technical Report; Fire Protection Research Foundation: Quincy, MA, USA, 2011. [Google Scholar]
- Jorne, J. The Chalkboard: C Rating of Batteries: A Misleading Concept, C Flux Rather than C Rate. Electrochem. Soc. Interface 2018, 27, 42–43. [Google Scholar]
- Aurbach, D. Nonaqueous Electrochemistry; CRC Press: New York, NY, USA, 1999; ISBN 9780367399573. [Google Scholar]
- Wu, M.S.; Liao, T.L.; Wang, Y.Y.; Wan, C.C. Assessment of the Wettability of Porous Electrodes for Lithium-Ion Batteries. J. Appl. Electrochem. 2004, 34, 797–805. [Google Scholar] [CrossRef]
- Fong, R.; von Sacken, U.; Dahn, J.R. Studies of Lithium Intercalation into Carbons Using Nonaqueous Electrochemical Cells. J. Electrochem. Soc. 1990, 137, 2009–2013. [Google Scholar] [CrossRef]
- Peled, E. The Electrochemical Behavior of Alkali and Alkaline Earth Metals in Nonaqueous Battery Systems—The Solid Electrolyte Interphase Model. J. Electrochem. Soc. 1979, 126, 2047–2051. [Google Scholar] [CrossRef]
- Winter, M. The Solid Electrolyte Interphase—The Most Important and the Least Understood Solid Electrolyte in Rechargeable Li Batteries. Z. Phys. Chem. 2009, 223, 1395–1406. [Google Scholar] [CrossRef]
- Brissot, C.; Rosso, M.; Chazalviel, J.; Lascaud, S. Dendritic growth mechanisms in lithium/polymer cells. J. Power Sources 1999, 81-82, 925–929. [Google Scholar] [CrossRef]
- Rosso, M.; Gobron, T.; Brissot, C.; Chazalviel, J.N.; Lascaud, S. Onset of dendritic growth in lithium/polymer cells. J. Power Sources 2001, 97-98, 804–806. [Google Scholar] [CrossRef]
- Nyman, A.; Zavalis, T.G.; Elger, R.; Behm, M.; Lindbergh, G. Analysis of the Polarization in a Li-Ion Battery Cell by Numerical Simulations. J. Electrochem. Soc. 2010, 157, A1236–A1246. [Google Scholar] [CrossRef]
- Maier, J. Concentration Polarization of Salt-Containing Liquid Electrolytes. Adv. Funct. Mater. 2011, 21, 1448–1455. [Google Scholar] [CrossRef]
- Janek, J.; Zeier, W. A solid future for battery development. Nat. Energy 2016, 1, 16141. [Google Scholar] [CrossRef]
- Kato, Y.; Shiotani, S.; Morita, K.; Suzuki, K.; Hirayama, M.; Kanno, R. All-Solid-State Batteries with Thick Electrode Configurations. J. Phys. Chem. Lett. 2018, 9, 607–613. [Google Scholar] [CrossRef] [PubMed]
- Sakuda, A. Favorable composite electrodes for all-solid-state batteries. J. Ceram. Soc. Jpn. 2018, 126, 675–683. [Google Scholar] [CrossRef]
- Takada, K. Progress and prospective of solid-state lithium batteries. Acta Mater. 2013, 61, 759–770. [Google Scholar] [CrossRef]
- Yan, X.; Li, Z.; Wen, Z.; Han, W. Li/Li7La3Zr2O12/LiFePO4 All-Solid-State Battery with Ultrathin Nanoscale Solid Electrolyte. J. Phys. Chem. C 2017, 121, 1431–1435. [Google Scholar] [CrossRef]
- Kato, Y.; Hori, S.; Saito, T.; Suzuki, K.; Hirayama, M.; Mitsui, A.; Yonemura, M.; Iba, H.; Kanno, R. High-power all-solid-state batteries using sulfide superionic conductors. Nat. Energy 2016, 1, 16030. [Google Scholar] [CrossRef]
- Diederichsen, K.M.; McShane, E.J.; McCloskey, B.D. Promising Routes to a High Li+ Transference Number Electrolyte for Lithium Ion Batteries. ACS Energy Lett. 2017, 2, 2563–2575. [Google Scholar] [CrossRef]
- Baek, S.W.; Lee, J.M.; Kim, T.Y.; Song, M.S.; Park, Y. Garnet related lithium ion conductor processed by spark plasma sintering for all solid state batteries. J. Power Sources 2014, 249, 197–206. [Google Scholar] [CrossRef]
- Shao, C.; Liu, H.; Yu, Z.; Zheng, Z.; Sun, N.; Diao, C. Structure and ionic conductivity of cubic Li7La3Zr2O12 solid electrolyte prepared by chemical co-precipitation method. Solid State Ionics 2016, 287, 13–16. [Google Scholar] [CrossRef]
- Cui, Y.; Mahmoud, M.M.; Rohde, M.; Ziebert, C.; Seifert, H.J. Thermal and ionic conductivity studies of lithium aluminum germanium phosphate solid-state electrolyte. Solid State Ionics 2016, 289, 125–132. [Google Scholar] [CrossRef]
- Mertens, A.; Yu, S.; Schön, N.; Gunduz, D.C.; Tempel, H.; Schierholz, R.; Hausen, F.; Kungl, H.; Granwehr, J.; Eichel, R.A. Superionic bulk conductivity in Li1.3Al0.3Ti1.7(PO4)3 solid electrolyte. Solid State Ionics 2017, 309, 180–186. [Google Scholar] [CrossRef]
- Dudley, J.; Wilkinson, D.; Thomas, G.; LeVae, R.; Woo, S.; Blom, H.; Horvath, C.; Juzkow, M.; Denis, B.; Juric, P.; et al. Conductivity of electrolytes for rechargeable lithium batteries. J. Power Sources 1991, 35, 59–82. [Google Scholar] [CrossRef]
- Valøen, L.O.; Reimers, J.N. Transport Properties of LiPF6-Based Li-Ion Battery Electrolytes. J. Electrochem. Soc. 2005, 152, A882–A891. [Google Scholar] [CrossRef]
- Logan, E.R.; Tonita, E.M.; Gering, K.L.; Li, J.; Ma, X.; Beaulieu, L.Y.; Dahn, J.R. A Study of the Physical Properties of Li-Ion Battery Electrolytes Containing Esters. J. Electrochem. Soc. 2018, 165, A21–A30. [Google Scholar] [CrossRef]
- Bernuy-Lopez, C.; Manalastas, W.; Lopez del Amo, J.M.; Aguadero, A.; Aguesse, F.; Kilner, J.A. Atmosphere Controlled Processing of Ga-Substituted Garnets for High Li-Ion Conductivity Ceramics. Chem. Mater. 2014, 26, 3610–3617. [Google Scholar] [CrossRef]
- Jin, Y.; McGinn, P.J. Al-doped Li7La3Zr2O12 synthesized by a polymerized complex method. J. Power Sources 2011, 196, 8683–8687. [Google Scholar] [CrossRef]
- Wang, D.; Zhong, G.; Dolotko, O.; Li, Y.; McDonald, M.J.; Mi, J.; Fu, R.; Yang, Y. The synergistic effects of Al and Te on the structure and Li+-mobility of garnet-type solid electrolytes. J. Mater. Chem. A Mater. Energy Sustain. 2014, 2, 20271–20279. [Google Scholar] [CrossRef]
- Allen, J.; Wolfenstine, J.; Rangasamy, E.; Sakamoto, J. Effect of substitution (Ta, Al, Ga) on the conductivity of Li7La3Zr2O12. J. Power Sources 2012, 206, 315–319. [Google Scholar] [CrossRef]
- Dhivya, L.; Murugan, R. Effect of Simultaneous Substitution of Y and Ta on the Stabilization of Cubic Phase, Microstructure, and Li+ Conductivity of Li7La3Zr2O12 Lithium Garnet. ACS Appl. Mater. Interfaces 2014, 6, 17606–17615. [Google Scholar] [CrossRef]
- Tong, X.; Thangadurai, V.; Wachsman, E.D. Highly Conductive Li Garnets by a Multielement Doping Strategy. Inorg. Chem. 2015, 54, 3600–3607. [Google Scholar] [CrossRef]
- Han, F.; Gao, T.; Zhu, Y.; Gaskell, K.J.; Wang, C. A Battery Made from a Single Material. Adv. Mater. 2015, 27, 3473–3483. [Google Scholar] [CrossRef]
- Yao, X.; Huang, B.; Yin, J.; Peng, G.; Huang, Z.; Gao, C.; Liu, D.; Xu, X. All-solid-state lithium batteries with inorganic solid electrolytes: Review of fundamental science. Chin. Phys. B 2016, 25, 018802. [Google Scholar] [CrossRef]
- Goodenough, J.B.; Singh, P. Review—Solid Electrolytes in Rechargeable Electrochemical Cells. J. Electrochem. Soc. 2015, 162, A2387–A2392. [Google Scholar] [CrossRef]
- Guo, X.; Maier, J. Comprehensive Modeling of Ion Conduction of Nanosized CaF2/BaF2 Multilayer Heterostructures. Adv. Funct. Mater. 2008, 19, 96–101. [Google Scholar] [CrossRef]
- Bandhauer, T.M.; Garimella, S.; Fuller, T.F. A Critical Review of Thermal Issues in Lithium-Ion Batteries. J. Electrochem. Soc. 2011, 158, R1–R25. [Google Scholar] [CrossRef]
- Broussely, M.; Biensan, P.; Bonhomme, F.; Blanchard, P.; Herreyre, S.; Nechev, K.; Staniewicz, R. Main aging mechanisms in Li ion batteries. J. Power Sources 2005, 146, 90–96. [Google Scholar] [CrossRef]
- Vetter, J.; Novák, P.; Wagner, M.; Veit, C.; Möller, K.C.; Besenhard, J.; Winter, M.; Wohlfahrt-Mehrens, M.; Vogler, C.; Hammouche, A. Ageing mechanisms in lithium-ion batteries. J. Power Sources 2005, 147, 269–281. [Google Scholar] [CrossRef]
- Leng, F.; Tan, C.M.; Pecht, M. Effect of Temperature on the Aging rate of Li Ion Battery Operating above Room Temperature. Sci. Rep. 2015, 5, 12967. [Google Scholar] [CrossRef]
- Waldmann, T.; Wilka, M.; Kasper, M.; Fleischhammer, M.; Wohlfahrt-Mehrens, M. Temperature dependent ageing mechanisms in Lithium-ion batteries—A Post-Mortem study. J. Power Sources 2014, 262, 129–135. [Google Scholar] [CrossRef]
- Heubner, C.; Lämmel, C.; Junker, N.; Schneider, M.; Michaelis, A. Microscopic in-operando thermography at the cross section of a single lithium ion battery stack. Electrochem. Commun. 2014, 48, 130–133. [Google Scholar] [CrossRef]
- Heubner, C.; Schneider, M.; Lämmel, C.; Langklotz, U.; Michaelis, A. In-operando temperature measurement across the interfaces of a lithium-ion battery cell. Electrochim. Acta 2013, 113, 730–734. [Google Scholar] [CrossRef]
- Zhang, G.; Cao, L.; Ge, S.; Wang, C.Y.; Shaffer, C.E.; Rahn, C.D. In Situ Measurement of Radial Temperature Distributions in Cylindrical Li-Ion Cells. J. Electrochem. Soc. 2014, 161, A1499–A1507. [Google Scholar] [CrossRef]
- Veth, C.; Dragicevic, D.; Merten, C. Thermal characterizations of a large-format lithium ion cell focused on high current discharges. J. Power Sources 2014, 267, 760–769. [Google Scholar] [CrossRef]
- Eddahech, A.; Briat, O.; Vinassa, J.M. Performance comparison of four lithium–ion battery technologies under calendar aging. Energy 2015, 84, 542–550. [Google Scholar] [CrossRef]
- Richter, F.; Kjelstrup, S.; Vie, P.J.S.; Burheim, O.S. Thermal conductivity and internal temperature profiles of Li-ion secondary batteries. J. Power Sources 2017, 359, 592–600. [Google Scholar] [CrossRef]
- Burheim, O.; Onsrud, M.; Pharoah, J.; Vullum-Bruer, F.; Vie, P. Thermal conductivity, heat sources and temperature profiles of Li-ion batteries. ECS Trans. 2014, 58, 145–171. [Google Scholar] [CrossRef]
- Broussely, M.; Herreyre, S.; Biensan, P.; Kasztejna, P.; Nechev, K.; Staniewicz, R. Aging mechanism in Li ion cells and calendar life predictions. J. Power Sources 2001, 97–98, 13–21. [Google Scholar] [CrossRef]
- Bock, R.; Shum, A.D.; Xiao, X.; Karoliussen, H.; Seland, F.; Zenyuk, I.V.; Burheim, O.S. Thermal Conductivity and Compaction of GDL-MPL Interfacial Composite Material. J. Electrochem. Soc. 2018, 165, F514–F525. [Google Scholar] [CrossRef]
- Liu, Y.; Liu, J.; Sun, Q.; Wang, D.; Adair, K.R.; Liang, J.; Zhang, C.; Zhang, L.; Lu, S.; Huang, H.; et al. Insight into the Microstructure and Ionic Conductivity of Cold Sintered NASICON Solid Electrolyte for Solid-State Batteries. ACS Appl. Mater. Interfaces 2019, 11, 27890–27896. [Google Scholar] [CrossRef]
- Huang, X.; Lu, Y.; Song, Z.; Xiu, T.; Badding, M.E.; Wen, Z. Preparation of dense Ta-LLZO/MgO composite Li-ion solid electrolyte: Sintering, microstructure, performance and the role of MgO. J. Energy Chem. 2019, 39, 8–16. [Google Scholar] [CrossRef]
- Richter, F.; Vie, P.J.; Kjelstrup, S.; Burheim, O.S. Measurements of ageing and thermal conductivity in a secondary NMC-hard carbon Li-ion battery and the impact on internal temperature profiles. Electrochim. Acta 2017, 250, 228–237. [Google Scholar] [CrossRef]
- Vishwakarma, V.; Waghela, C.; Wei, Z.; Prasher, R.; Nagpure, S.C.; Li, J.; Liu, F.; Daniel, C.; Jain, A. Heat transfer enhancement in a lithium-ion cell through improved material-level thermal transport. J. Power Sources 2015, 300, 123–131. [Google Scholar] [CrossRef]
- Burheim, O.S.; Pollet, B.G. Thermal Gradients and Thermal Conductivity in PEM Fuel Cells, Compared to Li-Ion Batteries and Super Capacitors. ECS Trans. 2018, 86, 97–109. [Google Scholar] [CrossRef]
- Viswanathan, V.V.; Choi, D.; Wang, D.; Xu, W.; Towne, S.; Williford, R.E.; Zhang, J.G.; Liu, J.; Yang, Z. Effect of entropy change of lithium intercalation in cathodes and anodes on Li-ion battery thermal management. J. Power Sources 2010, 195, 3720–3729. [Google Scholar] [CrossRef]
- Klemens, P. Heat conduction in solids by phonons. Thermochim. Acta 1993, 218, 247–255. [Google Scholar] [CrossRef]

| Solid State | Comp. Pressure | Heating Rate | Sintering Temp. | Sintering Time |
|---|---|---|---|---|
| Electrolyte | (bar) | (C min) | (C) | (min) |
| LLZO | 200 | 200 | 1100 | 60 |
| LAGP | 50 | 200 | 1100 | 60 |
| LATP | 500 | 200 | 1100 | 60 |
| Material | (through-Plane) | Ref. | |
|---|---|---|---|
| (WKm) | (m) | ||
| Liquid electrolyte soaked separator | 0.6 | 5 and 25 | [50] |
| LLZO, LAGP, LATP; sintered | 0.5 | 5 and 25 | [*] |
| Cathode, dry | 0.3 | 80 and 240 | [50] |
| Cathode, soaked | 1.0 | 80 and 240 | [50] |
| Anode, dry | 0.3 | 95 and 285 | [50] |
| Anode, soaked | 1.0 | 95 and 285 | [50] |
| Heat | Loss Terms | Current Density | Ref. | Heat Flux, T = 25 C |
|---|---|---|---|---|
| Source | (V) | j (Am) | (Wm) | |
| Entropic | 35 JmolK · T/F | 80 and 240 | [59] | 8.6–27.0 |
| Ohmic | /·j | 80 and 240 | [26,29] | 0.03–15.6 |
| Activation | −0.039 + 0.068 log(j) | 80 and 240 | [51] | 7.2–31.0 |
| Compaction | LLZO | LLZO | LAGP | LATP |
|---|---|---|---|---|
| Pressure | Unsintered | Sintered | Sintered | Sintered |
| (bar) | (WKm) | (WKm) | (WKm) | (WKm) |
| 3 | ||||
| 4 | ||||
| 5 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bock, R.; Onsrud, M.; Karoliussen, H.; Pollet, B.G.; Seland, F.; Burheim, O.S. Thermal Gradients with Sintered Solid State Electrolytes in Lithium-Ion Batteries. Energies 2020, 13, 253. https://doi.org/10.3390/en13010253
Bock R, Onsrud M, Karoliussen H, Pollet BG, Seland F, Burheim OS. Thermal Gradients with Sintered Solid State Electrolytes in Lithium-Ion Batteries. Energies. 2020; 13(1):253. https://doi.org/10.3390/en13010253
Chicago/Turabian StyleBock, Robert, Morten Onsrud, Håvard Karoliussen, Bruno G. Pollet, Frode Seland, and Odne S. Burheim. 2020. "Thermal Gradients with Sintered Solid State Electrolytes in Lithium-Ion Batteries" Energies 13, no. 1: 253. https://doi.org/10.3390/en13010253
APA StyleBock, R., Onsrud, M., Karoliussen, H., Pollet, B. G., Seland, F., & Burheim, O. S. (2020). Thermal Gradients with Sintered Solid State Electrolytes in Lithium-Ion Batteries. Energies, 13(1), 253. https://doi.org/10.3390/en13010253