Mapping of Absolute Host Concentration and Exchange Kinetics of Xenon Hyper-CEST MRI Agents
Abstract
1. Introduction
2. Results
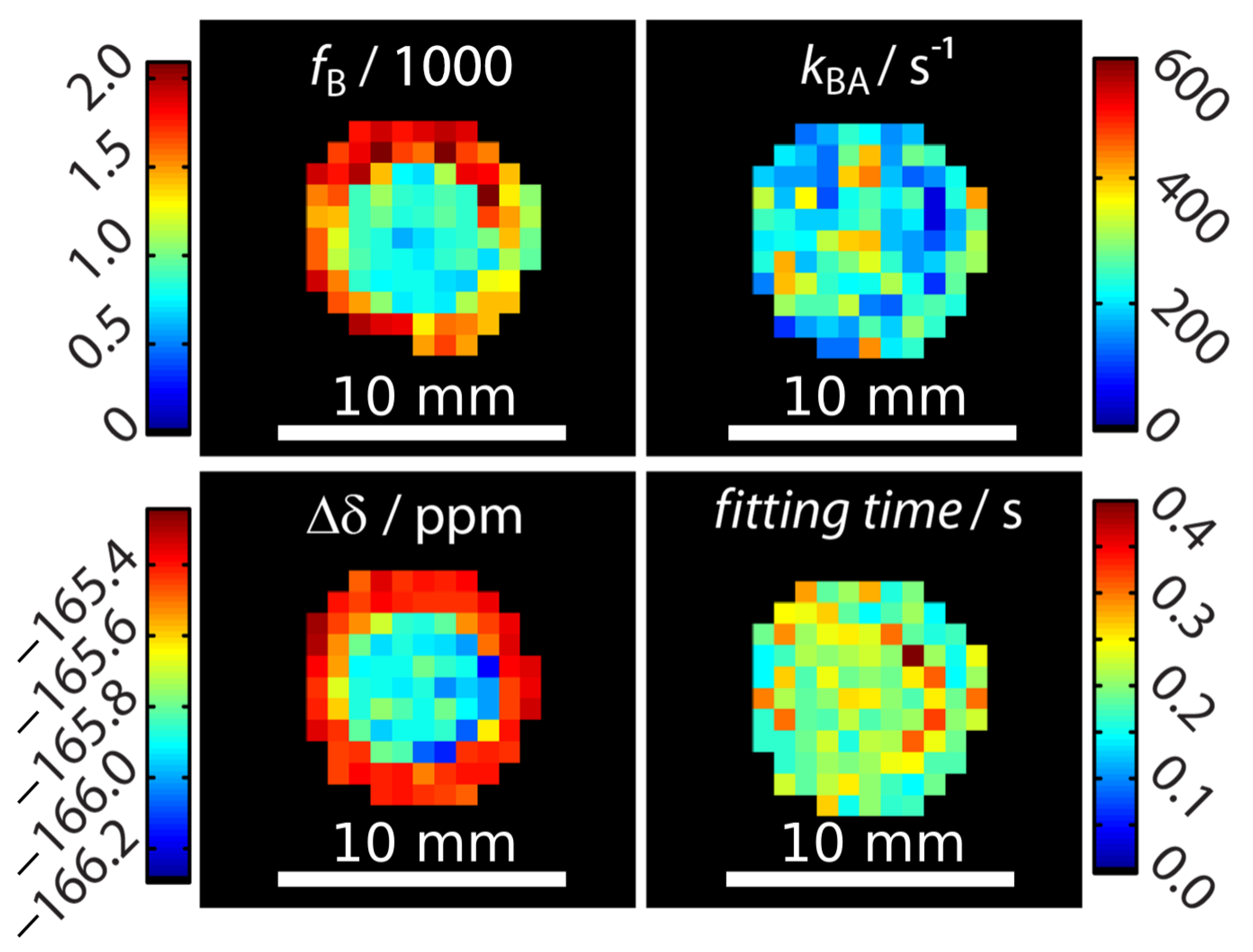
2.1. Mapping of Xenon Exchange Kinetics
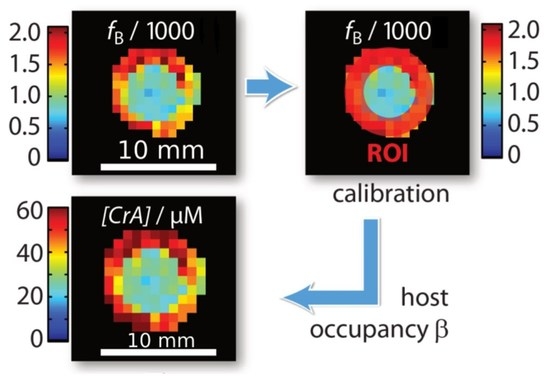
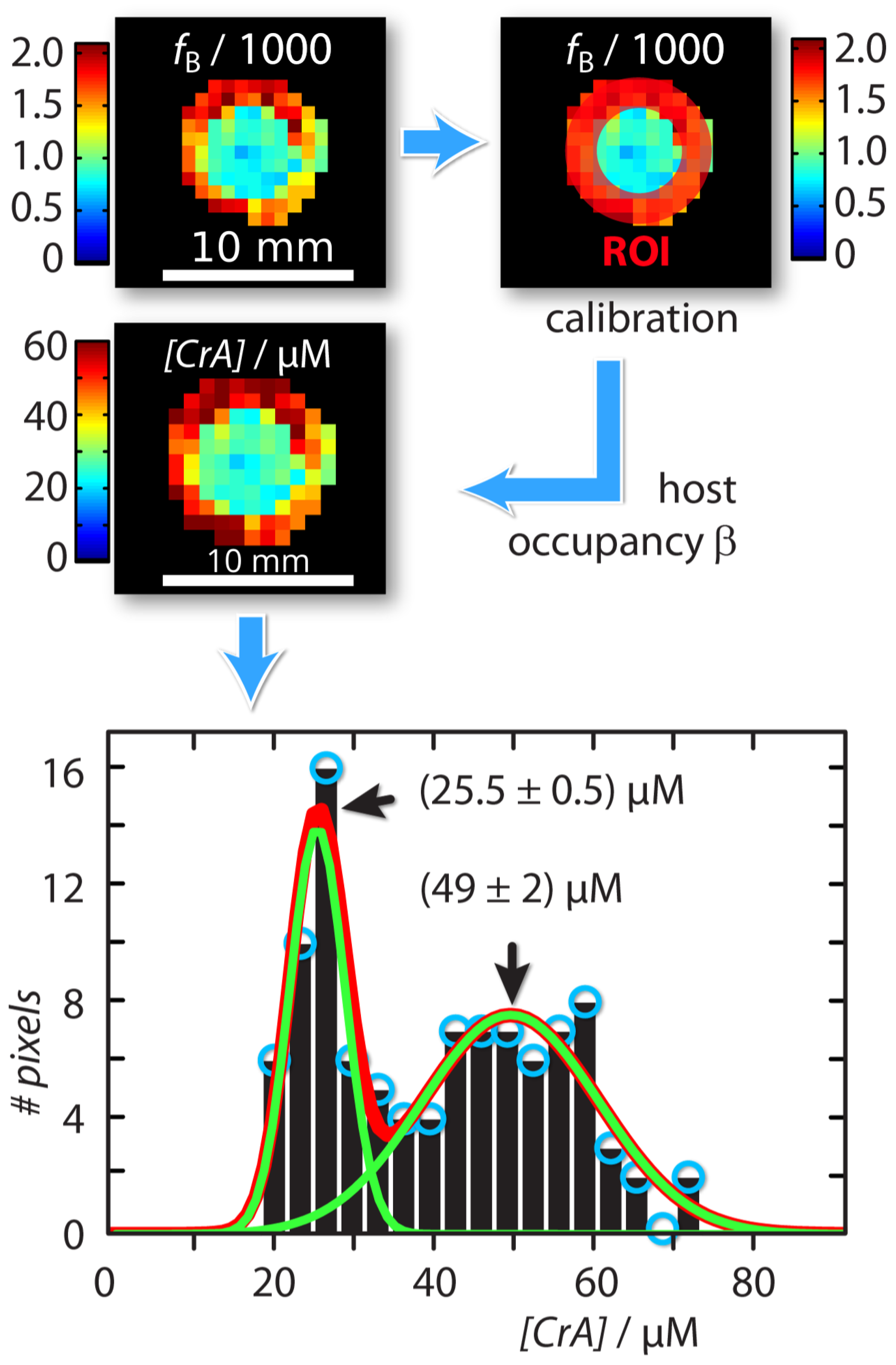
2.2. Absolute Xenon Host Concentration Mapping
2.3. Limitations
3. Discussion
4. Materials and Methods
4.1. Sample Preparation
4.2. Hyperpolarization and 129Xe Delivery
4.3. NMR Experiments
4.4. Data Fitting
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| CEST | chemical exchange saturation transfer |
| CrA-ma | cryptophane-A monoacid |
| DMSO | dimethyl sulfoxide |
| DNP | dynamic nuclear polarization |
| EPI | echo planar imaging |
| FHC | full Hyper-CEST |
| FOV | field of view |
| GBCA | Gadolinium-based contrast agent |
| Gd | Gadolinium |
| Hyper-CEST | linear dichroism |
| MRI | magnetic resonance imaging |
| NMR | nuclear magnetic resonance |
| qHyper-CEST | quantitative chemical exchange saturation transfer with hyperpolarized xenon |
| ROI | region of interest |
| Xe | Xenon |
Appendix A. EPI Image Series
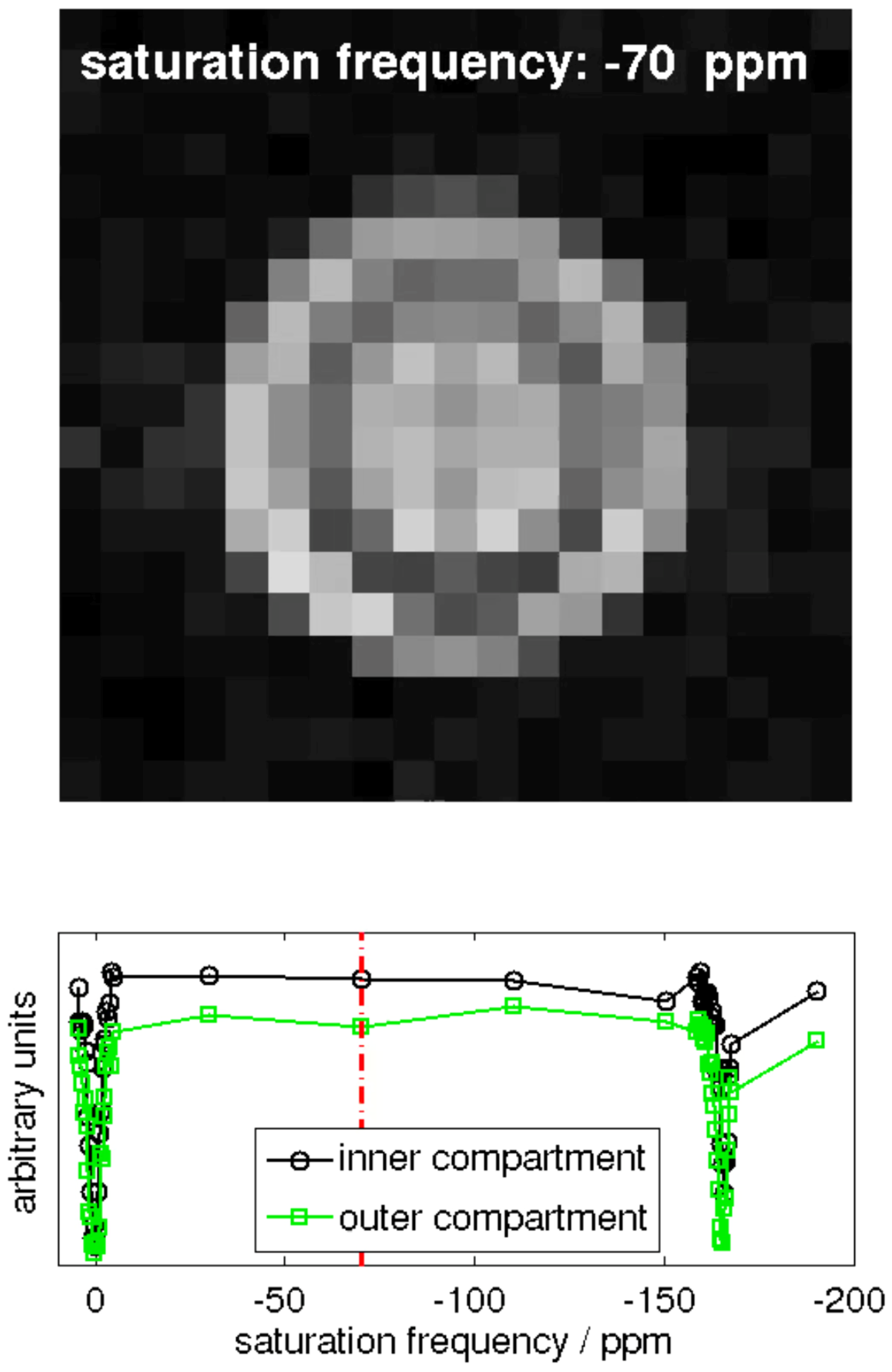
Appendix B. Details of Pixel Wise z Spectra

Appendix C. Chemical Shift Difference between Inner and Outer Compartment

References
- Angelovski, G. What We Can Really Do with Bioresponsive MRI Contrast Agents. Angew. Chem. Int. Ed. 2016, 55, 7038–7046. [Google Scholar] [CrossRef] [PubMed]
- Akakuru, O.U.; Iqbal, M.Z.; Saeed, M.; Liu, C.; Paunesku, T.; Woloschak, G.; Hosmane, N.S.; Wu, A. The Transition from Metal-Based to Metal-Free Contrast Agents for T1 Magnetic Resonance Imaging Enhancement. Bioconjug. Chem. 2019, 30, 2264–2286. [Google Scholar] [CrossRef] [PubMed]
- Wahsner, J.; Gale, E.M.; Rodríguez-Rodríguez, A.; Caravan, P. Chemistry of MRI Contrast Agents: Current Challenges and New Frontiers. Chem. Rev. 2019, 119, 957–1057. [Google Scholar] [CrossRef]
- Bar-Shir, A.; Bulte, J.W.M.; Gilad, A.A. Molecular Engineering of Nonmetallic Biosensors for CEST MRI. ACS Chem. Biol. 2015, 10, 1160–1170. [Google Scholar] [CrossRef] [PubMed]
- Jones, K.M.; Pollard, A.C.; Pagel, M.D. Clinical applications of chemical exchange saturation transfer (CEST) MRI. J. Magn. Reson. Imaging 2018, 47, 11–27. [Google Scholar] [CrossRef] [PubMed]
- Goldenberg, J.M.; Pagel, M.D. Assessments of tumor metabolism with CEST MRI. NMR Biomed. 2019, 32, e3943. [Google Scholar] [CrossRef] [PubMed]
- Schröder, L.; Lowery, T.J.; Hilty, C.; Wemmer, D.E.; Pines, A. Molecular Imaging Using a Targeted Magnetic Resonance Hyperpolarized Biosensor. Science 2006, 314, 446–449. [Google Scholar] [CrossRef] [PubMed]
- Haouaj, M.E.; Luhmer, M.; Ko, Y.H.; Kim, K.; Bartik, K. NMR study of the reversible complexation of xenon by cucurbituril. J. Chem. Soc. Perkin Trans. 2001, 2, 804–807. [Google Scholar] [CrossRef][Green Version]
- Kim, B.S.; Ko, Y.H.; Kim, Y.; Lee, H.J.; Selvapalam, N.; Lee, H.C.; Kim, K. Water soluble cucurbit[6]uril derivative as a potential Xe carrier for 129-Xe NMR-based biosensors. Chem. Commun. 2008, 2756–2758. [Google Scholar] [CrossRef]
- Adiri, T.; Marciano, D.; Cohen, Y. Potential 129-Xe-NMR biosensors based on secondary and tertiary complexes of a water-soluble pillar-[5]arene derivative. Chem. Commun. 2013, 49, 7082–7084. [Google Scholar] [CrossRef]
- Roukala, J.; Zhu, J.; Giri, C.; Rissanen, K.; Lantto, P.; Telkki, V.V.A. Encapsulation of Xenon by a Self-Assembled Fe4L6 Metallo-supramolecular Cage. J. Am. Chem. Soc. 2015. [Google Scholar] [CrossRef] [PubMed]
- Spence, M.M.; Rubin, S.M.; Dimitrov, I.E.; Ruiz, E.J.; Wemmer, D.E.; Pines, A.; Yao, S.Q.; Tian, F.; Schultz, P.G. Functionalized xenon as a biosensor. Proc. Natl. Acad. Sci. USA 2001, 98, 10654–10657. [Google Scholar] [CrossRef] [PubMed]
- Hill, P.A.; Wei, Q.; Eckenhoff, R.G.; Dmochowski, I.J. Thermodynamics of Xenon Binding to Cryptophane in Water and Human Plasma. J. Am. Chem. Soc. 2007, 129, 9262–9263. [Google Scholar] [CrossRef] [PubMed]
- Chambers, J.M.; Hill, P.A.; Aaron, J.A.; Han, Z.; Christianson, D.W.; Kuzma, N.N.; Dmochowski, I.J. Cryptophane Xenon-129 Nuclear Magnetic Resonance Biosensors Targeting Human Carbonic Anhydrase. J. Am. Chem. Soc. 2009, 131, 563–569. [Google Scholar] [CrossRef] [PubMed]
- Hill, P.A.; Wei, Q.; Troxler, T.; Dmochowski, I.J. Substituent Effects on Xenon Binding Affinity and Solution Behavior of Water-Soluble Cryptophanes. J. Am. Chem. Soc. 2009, 131, 3069–3077. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Taratula, O.; Hill, P.A.; Khan, N.S.; Carroll, P.J.; Dmochowski, I.J. Crystallographic observation of ‘induced fit’ in a cryptophane host–guest model system. Nat. Commun. 2010, 1, 148. [Google Scholar] [CrossRef]
- Kunth, M.; Döpfert, J.; Witte, C.; Rossella, F.; Schröder, L. Optimized Use of Reversible Binding for Fast and Selective NMR Localization of Caged Xenon. Angew. Chem. Int. Ed. 2012, 51, 8217–8220. [Google Scholar] [CrossRef]
- Klippel, S.; Döpfert, J.; Jayapaul, J.; Kunth, M.; Rossella, F.; Schnurr, M.; Witte, C.; Freund, C.; Schröder, L. Cell Tracking with Caged Xenon: Using Cryptophanes as MRI Reporters upon Cellular Internalization. Angew. Chem. Int. Ed. 2014, 53, 493–496. [Google Scholar] [CrossRef]
- Rose, H.M.; Witte, C.; Rossella, F.; Klippel, S.; Freund, C.; Schröder, L. Development of an antibody-based, modular biosensor for 129-Xe NMR molecular imaging of cells at nanomolar concentrations. Proc. Natl. Acad. Sci. USA 2014, 111, 11697–11702. [Google Scholar] [CrossRef]
- Witte, C.; Martos, V.; Rose, H.M.; Reinke, S.; Klippel, S.; Schröder, L.; Hackenberger, C.P.R. Live-cell MRI with Xenon Hyper-CEST Biosensors Targeted to Metabolically Labeled Cell-Surface Glycans. Angew. Chem. Int. Ed. 2015, 54, 2806–2810. [Google Scholar] [CrossRef]
- Bai, Y.; Wang, Y.; Goulian, M.; Driks, A.; Dmochowski, I.J. Bacterial spore detection and analysis using hyperpolarized 129-Xe chemical exchange saturation transfer (Hyper-CEST) NMR. Chem. Sci. 2014, 5, 3197–3203. [Google Scholar] [CrossRef] [PubMed]
- Shapiro, M.G.; Ramirez, R.M.; Sperling, L.J.; Sun, G.; Sun, J.; Pines, A.; Schaffer, D.V.; Bajaj, V.S. Genetically encoded reporters for hyperpolarized xenon magnetic resonance imaging. Nat. Chem. 2014, 6, 629–634. [Google Scholar] [CrossRef] [PubMed]
- Wolber, J.; Rowland, I.J.; Leach, M.O.; Bifone, A. Perfluorocarbon emulsions as intravenous delivery media for hyperpolarized xenon. Magn. Reson. Med. 1999, 41, 442–449. [Google Scholar] [CrossRef]
- Stevens, T.K.; Ramirez, R.M.; Pines, A. Nanoemulsion Contrast Agents with Sub-picomolar Sensitivity for Xenon NMR. J. Am. Chem. Soc. 2013, 135, 9576–9579. [Google Scholar] [CrossRef] [PubMed]
- Klippel, S.; Freund, C.; Schröder, L. Multichannel MRI Labeling of Mammalian Cells by Switchable Nanocarriers for Hyperpolarized Xenon. Nano Lett. 2014, 14, 5721–5726. [Google Scholar] [CrossRef] [PubMed]
- Schoenborn, B.P.; Watson, H.C.; Kendrew, J.C. Binding of Xenon to Sperm Whale Myoglobin. Nature 1965, 28–30. [Google Scholar] [CrossRef]
- Tilton, R.F.; Kuntz, I.D.; Petsko, G.A. Cavities in proteins: Structure of a metmyoglobin xenon complex solved to 1.9. ANG. Biochemistry 1984, 23, 2849–2857. [Google Scholar] [CrossRef]
- Duff, A.P.; Trambaiolo, D.M.; Cohen, A.E.; Ellis, P.J.; Juda, G.A.; Shepard, E.M.; Langley, D.B.; Dooley, D.M.; Freeman, H.C.; Guss, J.M. Using Xenon as a Probe for Dioxygen-binding Sites in Copper Amine Oxidases. J. Mol. Biol. 2004, 344, 599–607. [Google Scholar] [CrossRef]
- Truxal, A.E.; Cao, L.; Isaacs, L.; Wemmer, D.E.; Pines, A. Directly Functionalized Cucurbit[7]uril as Biosensor for the Selective Detection of Protein Interactions by 129Xe hyperCEST NMR. Chemistry 2019, 25, 6108–6112. [Google Scholar] [CrossRef]
- Wang, Y.; Dmochowski, I.J. Cucurbit[6]uril is an ultrasensitive 129Xe NMR contrast agent. Chem. Commun. 2015, 51, 8982–8985. [Google Scholar] [CrossRef]
- Riggle, B.A.; Wang, Y.; Dmochowski, I.J. A “Smart” 129Xe NMR Biosensor for pH-Dependent Cell Labeling. J. Am. Chem. Soc. 2015, 137, 5542–5548. [Google Scholar] [CrossRef] [PubMed]
- Roose, B.; Zemerov, S.D.; Dmochowski, I.J. Nanomolar small-molecule detection using a genetically encoded 129Xe NMR contrast agent. Chem. Sci. 2017, 8, 7631–7636. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Roose, B.W.; Palovcak, E.J.; Carnevale, V.; Dmochowski, I.J. A Genetically Encoded β-Lactamase Reporter for Ultrasensitive 129Xe NMR in Mammalian Cells. Angew. Chem. Int. Ed. 2016, 55, 8984–8987. [Google Scholar] [CrossRef] [PubMed]
- Slack, C.C.; Finbloom, J.A.; Jeong, K.; Bruns, C.J.; Wemmer, D.E.; Pines, A.; Francis, M.B. Rotaxane probes for protease detection by 129Xe hyperCEST NMR. Chem. Commun. 2017, 53, 1076–1079. [Google Scholar] [CrossRef]
- Riggle, B.A.; Greenberg, M.L.; Wang, Y.; Wissner, R.F.; Zemerov, S.D.; Petersson, E.J.; Dmochowski, I.J. A cryptophane-based “turn-on” 129Xe NMR biosensor for monitoring calmodulin. Org. Biomol. Chem. 2017, 15, 8883–8887. [Google Scholar] [CrossRef] [PubMed]
- Schnurr, M.; Sloniec-Myszk, J.; Döpfert, J.; Schröder, L.; Hennig, A. Supramolecular Assays for Mapping Enzyme Activity by Displacement-Triggered Change in Hyperpolarized 129Xe Magnetization Transfer NMR Spectroscopy. Angew. Chem. Int. Ed. 2015, 54, 13444–13447. [Google Scholar] [CrossRef]
- Döpfert, J.; Schnurr, M.; Kunth, M.; Rose, H.M.; Hennig, A.; Schröder, L. Time-resolved monitoring of enzyme activity with ultrafast Hyper-CEST spectroscopy. Magn. Reson. Chem. 2018, 56, 679–688. [Google Scholar] [CrossRef]
- Schnurr, M.; Joseph, R.; Naugolny-Keisar, A.; Kaizerman-Kane, D.; Bogdanoff, N.; Schuenke, P.; Cohen, Y.; Schröder, L. High Exchange Rate Complexes of 129Xe with Water-Soluble Pillar[5]arenes for Adjustable Magnetization Transfer MRI. ChemPhysChem 2019, 20, 246–251. [Google Scholar] [CrossRef]
- Klass, S.H.; Truxal, A.E.; Fiala, T.A.; Kelly, J.; Nguyen, D.; Finbloom, J.A.; Wemmer, D.E.; Pines, A.; Francis, M.B. Rotaxane Probes for the Detection of Hydrogen Peroxide by 129Xe HyperCEST NMR Spectroscopy. Angew. Chem. Int. Ed. 2019, 58, 9948–9953. [Google Scholar] [CrossRef]
- Huber, G.; Brotin, T.; Dubois, L.; Desvaux, H.; Dutasta, J.P.; Berthault, P. Water. JACS 2006, 128, 6239–6246. [Google Scholar] [CrossRef]
- Schnurr, M.; Witte, C.; Schröder, L. Functionalized 129-Xe as a potential biosensor for membrane fluidity. Phys. Chem. Chem. Phys. 2013, 15, 14178–14181. [Google Scholar] [CrossRef]
- McMahon, M.T.; Gilad, A.A.; Zhou, J.; Sun, P.Z.; Bulte, J.W.M.; van Zijl, P.C.M. Quantifying exchange rates in chemical exchange saturation transfer agents using the saturation time and saturation power dependencies of the magnetization transfer effect on the magnetic resonance imaging signal (QUEST and QUESP): Ph calibration for poly-L-lysine and a starburst dendrimer. Magn. Reson. Med. 2006, 55, 836–847. [Google Scholar] [CrossRef]
- Kunth, M.; Witte, C.; Schröder, L. Quantitative chemical exchange saturation transfer with hyperpolarized nuclei (qHyper-CEST): Sensing xenon-host exchange dynamics and binding affinities by NMR. J. Chem. Phys. 2014, 141, 194202. [Google Scholar] [CrossRef]
- Kunth, M.; Witte, C.; Schröder, L. Absolute Xenon-Host Concentration Determination by Quantitative 129-Xe MRI Using Hyper-CEST. In Proceedings of the World Molecular Imaging Congress, Seoul, Korea, 17–20 September 2014; p. 136. [Google Scholar]
- McMahon, M.T.; Gilad, A.A.; DeLiso, M.A.; Cromer Berman, S.M.; Bulte, J.W.; van Zijl, P.C. New “multicolor” polypeptide diamagnetic chemical exchange saturation transfer (DIACEST) contrast agents for MRI. Magn. Reson. Med. 2008, 60, 803–812. [Google Scholar] [CrossRef]
- Kunth, M.; Schröder, L. Binding site exchange kinetics revealed through efficient spin–spin dephasing of hyperpolarized 129Xe. Chem. Sci. 2020. [Google Scholar] [CrossRef]
- Kunth, M.; Witte, C.; Hennig, A.; Schröder, L. Identification, classification, and signal amplification capabilities of high-turnover gas binding hosts in ultra-sensitive NMR. Chem. Sci. 2015, 6, 6069–6075. [Google Scholar] [CrossRef] [PubMed]
- Zaiss, M.; Schnurr, M.; Bachert, P. Analytical solution for the depolarization of hyperpolarized nuclei by chemical exchange saturation transfer between free and encapsulated xenon (HyperCEST). J. Chem. Phys. 2012, 136, 144106. [Google Scholar] [CrossRef] [PubMed]
- Kunth, M.; Witte, C.; Schröder, L. Continuous-wave saturation considerations for efficient xenon depolarization. NMR Biomed. 2015, 28, 601–606. [Google Scholar] [CrossRef] [PubMed]
- Woessner, D.E.; Zhang, S.; Merritt, M.E.; Sherry, A.D. Numerical solution of the Bloch equations provides insights into the optimum design of PARACEST agents for MRI. Magn. Reson. Med. 2005, 53, 790–799. [Google Scholar] [CrossRef] [PubMed]
- Murase, K.; Tanki, N. Numerical solutions to the time-dependent Bloch equations revisited. Magn. Reson. Imaging 2011, 29, 126–131. [Google Scholar] [CrossRef]
- Schröder, L.; Meldrum, T.; Smith, M.; Lowery, T.J.; Wemmer, D.E.; Pines, A. Temperature Response of Xe-129 Depolarization Transfer and Its Application for Ultrasensitive NMR Detection. Phys. Rev. Lett. 2008, 100, 257603. [Google Scholar] [CrossRef] [PubMed]
- Schilling, F.; Schröder, L.; Palaniappan, K.K.; Zapf, S.; Wemmer, D.E.; Pines, A. MRI thermometry based on encapsulated hyperpolarized xenon. ChemPhysChem 2010, 11, 3529–3533. [Google Scholar] [CrossRef] [PubMed]
- Barskiy, D.A.; Coffey, A.M.; Nikolaou, P.; Mikhaylov, D.M.; Goodson, B.M.; Branca, R.T.; Lu, G.J.; Shapiro, M.G.; Telkki, V.V.; Zhivonitko, V.V.; et al. NMR Hyperpolarization Techniques of Gases. Chemistry 2017, 23, 725–751. [Google Scholar] [CrossRef]
- Goodson, B.M.; Kidd, B.; Hövener, J.B.; Schröder, L.; Theis, T.; Whiting, N.; Chekmenev, E.Y. Nuclear Magnetic Resonance Spectroscopy | Hyperpolarization for Sensitivity Enhancement. In Encyclopedia of Analytical Science, 3rd ed.; Worsfold, P., Poole, C., Townshend, A., Miró, M., Eds.; Academic Press: Oxford, UK, 2019; pp. 168–181. [Google Scholar] [CrossRef]
- Witte, C.; Kunth, M.; Rossella, F.; Schröder, L. Observing and preventing rubidium runaway in a direct-infusion xenon-spin hyperpolarizer optimized for high-resolution hyper-CEST (chemical exchange saturation transfer using hyperpolarized nuclei) NMR. J. Chem. Phys. 2014, 140, 084203. [Google Scholar] [CrossRef] [PubMed]
- Kunth, M.; Lu, G.J.; Witte, C.; Shapiro, M.G.; Schröder, L. Protein Nanostructures Produce Self-Adjusting Hyperpolarized Magnetic Resonance Imaging Contrast through Physical Gas Partitioning. ACS Nano 2018, 12, 10939–10948. [Google Scholar] [CrossRef]
- Zaiss, M.; Angelovski, G.; Demetriou, E.; McMahon, M.T.; Golay, X.; Scheffler, K. QUESP and QUEST revisite—Fast and accurate quantitative CEST experiments. Magn. Reson. Med. 2018, 79, 1708–1721. [Google Scholar] [CrossRef]
- Jayapaul, J.; Schröder, L. Molecular Sensing with Host Systems for Hyperpolarized 129Xe. Molecules 2020, 25, 4627. [Google Scholar] [CrossRef]
- Jayapaul, J.; Schröder, L. Probing Reversible Guest Binding with Hyperpolarized 129Xe-NMR: Characteristics and Applications for Cucurbit[N]urils. Molecules 2020, 25, 957. [Google Scholar] [CrossRef]
- Witte, C.; Kunth, M.; Döpfert, J.; Rossella, F.; Schröder, L. Hyperpolarized Xenon for NMR and MRI Applications. J. Vis. Exp. 2012, 67, e4268. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kunth, M.; Witte, C.; Schröder, L. Mapping of Absolute Host Concentration and Exchange Kinetics of Xenon Hyper-CEST MRI Agents. Pharmaceuticals 2021, 14, 79. https://doi.org/10.3390/ph14020079
Kunth M, Witte C, Schröder L. Mapping of Absolute Host Concentration and Exchange Kinetics of Xenon Hyper-CEST MRI Agents. Pharmaceuticals. 2021; 14(2):79. https://doi.org/10.3390/ph14020079
Chicago/Turabian StyleKunth, Martin, Christopher Witte, and Leif Schröder. 2021. "Mapping of Absolute Host Concentration and Exchange Kinetics of Xenon Hyper-CEST MRI Agents" Pharmaceuticals 14, no. 2: 79. https://doi.org/10.3390/ph14020079
APA StyleKunth, M., Witte, C., & Schröder, L. (2021). Mapping of Absolute Host Concentration and Exchange Kinetics of Xenon Hyper-CEST MRI Agents. Pharmaceuticals, 14(2), 79. https://doi.org/10.3390/ph14020079