A Collocation Method for Numerical Solution of Nonlinear Delay Integro-Differential Equations for Wireless Sensor Network and Internet of Things
Abstract
1. Introduction
- To develop efficient technique for the approximate solution of nonlinear FVIDEs arising in WSN and IIoT
- To design algorithm for proposed technique
- To examine the efficiency of the developed technique on some test problems and compare the results of our technique with results [1] available in the literature
2. Literature Review
3. Haar Wavelet
- To find solutions of different nonlinear FVIDEs arising in WSN and IIoT
- To check the efficiency and accuracy of the developed technique, the proposed method is applied on some test problems
4. Numerical Method for Nonlinear Delay IDEs Arising in WSN and IIoT
4.1. Nonlinear Delay Fredholm IDEs
4.2. Nonlinear Delay Volterra IDEs
4.3. Nonlinear Delay Volterra–Fredholm IDEs
5. Numerical Assessments
6. Results and Discussion
7. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Ayad, A. The numerical solution of first order delay integro-differential equations by spline functions. Int. J. Comput. Math. 2001, 77, 125–134. [Google Scholar] [CrossRef]
- Cardoso, A.A.; Vieira, F.H. Adaptive estimation of haar wavelet transform parameters applied to fuzzy prediction of network traffic. Signal Process. 2018, 151, 155–159. [Google Scholar] [CrossRef]
- Reisenhofer, R.; Bosse, S.; Kutyniok, G.; Wiegand, T. A haar wavelet-based perceptual similarity index for image quality assessment. Signal Process. Image Commun. 2018, 61, 33–43. [Google Scholar] [CrossRef]
- Adiguna, B.J.; Buchanb, A.G.; Adama, A.; Dargavillea, S.; Goffina, M.A.; Paina, C.C. A haar wavelet method for angularly discretising the boltzmann transport equation. Prog. Nucl. Energy 2018, 108, 295–309. [Google Scholar] [CrossRef]
- Kothari, K.; Mehta, U.; Vanualailai, J. A novel approach of fractional-order time delay system modeling based on haar wavelet. ISA Trans. 2018, 80, 371–380. [Google Scholar] [CrossRef]
- Belkadhi, K.; Manai, K. Dose calculation using a numerical method based on haar wavelets integration. Nucl. Instrum. Methods Phys. Res. 2016, 812, 73–80. [Google Scholar] [CrossRef]
- Dai, Q.; Cao, Q.; Chen, Y. Frequency analysis of rotating truncated conical shells using the haar wavelet method. Appl. Math. Model. 2018, 57, 603–613. [Google Scholar] [CrossRef]
- Aziz, I.; Siraj-ul-Islam; Khan, F. A new method based on Haar wavelet for the numerical solution of two-dimentional nonlinear integral equations. J. Comput. Appl. Math. 2014, 272, 70–80. [Google Scholar] [CrossRef]
- Vampa, V.; Martin, M.T.; Serrano, E. A hybrid method using wavelets for the numerical solution of boundary value problems. Appl. Math. Comput. 2010, 217, 3355–3367. [Google Scholar] [CrossRef]
- Chiavassa, G.; Guichaoua, M.; Liandrat, J. Two adaptive wavelet algorithms for non-linear parabolic partial differential equations. Comput. Fluids 2002, 31, 467–480. [Google Scholar] [CrossRef]
- Wu, J.L. A wavelet operational method for solving fractional partial differential equations numerically. Appl. Math. Comput. 2009, 214, 31–40. [Google Scholar] [CrossRef]
- Aziz, I.; Amin, R. Numerical solution of a class of delay differential and delay partial differential equations via haar wavelet. Appl. Math. Model. 2016, 40, 10286–10299. [Google Scholar] [CrossRef]
- Maleknejad, K.; Kajani, M.T. Solving linear integro-differential equation system by galerkin methods with hybrid functions. Appl. Math. Comput. 2004, 159, 603–612. [Google Scholar] [CrossRef]
- Biazar, J.; Babolian, E.; Islam, R. Solution of a system of volterra integral equations of the first kind by adomian method. Appl. Math. Comput. 2003, 139, 249–258. [Google Scholar] [CrossRef]
- Goghary, H.S.; Javadi, S.; Babolian, E. Restarted adomian method for system of nonlinear volterra integral equations. Appl. Math. Comput. 2005, 161, 745–751. [Google Scholar]
- Yusufoglu, E. A homotopy perturbation algorithm to solve a system of fredholm volterra type integral equations. Math. Comput. Model. 2008, 47, 1099–1107. [Google Scholar] [CrossRef]
- Wang, S.; He, J. Variational iteration method for solving integro-differential equations. Phys. Lett. A 2007, 367, 188–191. [Google Scholar] [CrossRef]
- Brunner, H. The numerical treatment of volterra integro-differential equations with unbounded delay. Comput. Appl. Math. 1989, 28, 5–23. [Google Scholar] [CrossRef][Green Version]
- Sadri, K.; Amini, A.; Cheng, C. A new numerical method for delay and advanced integro-differential equations. Comput. Appl. Math. 2018, 77, 381–412. [Google Scholar] [CrossRef]
- Makroglou, A. A block-by-block method for the numerical solution of Volterra delay and advanced integro-differential equations. Comput. Appl. Math. 1983, 30, 49–62. [Google Scholar]
- Bellour, A.; Bousselsal, M. A taylor collocation method for solving delay integral equations. Numer. Algorithms 2014, 65, 843–857. [Google Scholar] [CrossRef]
- Rabiei, F.; Lazim, N.; Hamid, F.; Rashidi, M.M. Numerical solution of volterra integro-differential equations using fifth order improved runge-kutta method. Int. J. Manag. Appl. Sci. 2017, 3, 2394–7926. [Google Scholar]
- Sekar, R.G.; Murugesan, K. Numerical solutions of delay volterra-integral equations using single term walsh series ppproach. Int. J. Appl. Comput. Math. 2017, 3, 2409–2421. [Google Scholar] [CrossRef]
- El-Hawary, H.M.; El-Shami, K. Numerical solution of volterra delay integro-differential equations via spline and spectral methods. Int. J. Differ. Equ. Appl. 2013, 12, 149–157. [Google Scholar]
- Ghomanjani, F.; Farahi, M.H.; Pariz, N. A new approach for numerical solution of a linear system with distributed delays, volterra delay integro-differential equations, and nonlinear volterra-fredholm integral equation by bezier curves. J. Comput. Appl. Math. 2017, 36, 1349–1365. [Google Scholar] [CrossRef]
- Sakran, M. Numerical solutions of integral and integro-differential equations using Chebyshev polynomials of the third kind. Appl. Math. Comput. 2019, 351, 66–82. [Google Scholar] [CrossRef]
- Abubakar, A.; Taiwo, O. Integral collocation approximation methods for the numerical solution of high orders linear Fredholm-Volterra integro-differential equations. Am. J. Comput. Appl. Math. 2014, 4, 111–117. [Google Scholar]
- Yuzbasi, S.; Gok, E.; Sezer, M. Muntz Legendre matrix method to solve the delay Fredholm integro-differential equations with constant coefficients. New Trends Math. Sci. 2015, 2, 159–167. [Google Scholar]
- Khirallah, M.Q.; Mahiub, M.A. Numerical method for solving delay integro-diffenential equations. Res. J. Appl. Sci. 2018, 13, 103–105. [Google Scholar]
- Zaidan, L.I. Solving linear delay volterra integro-differential equations by using galerkin’s method with bernstien polynomial. J. Bablyon Appl. Sci. 2012, 20, 1305–1313. [Google Scholar]
- Burden, R.L.; Faires, J.D. Numerical Analysis; Richard Stratton: Boston, MA, USA, 2001. [Google Scholar]
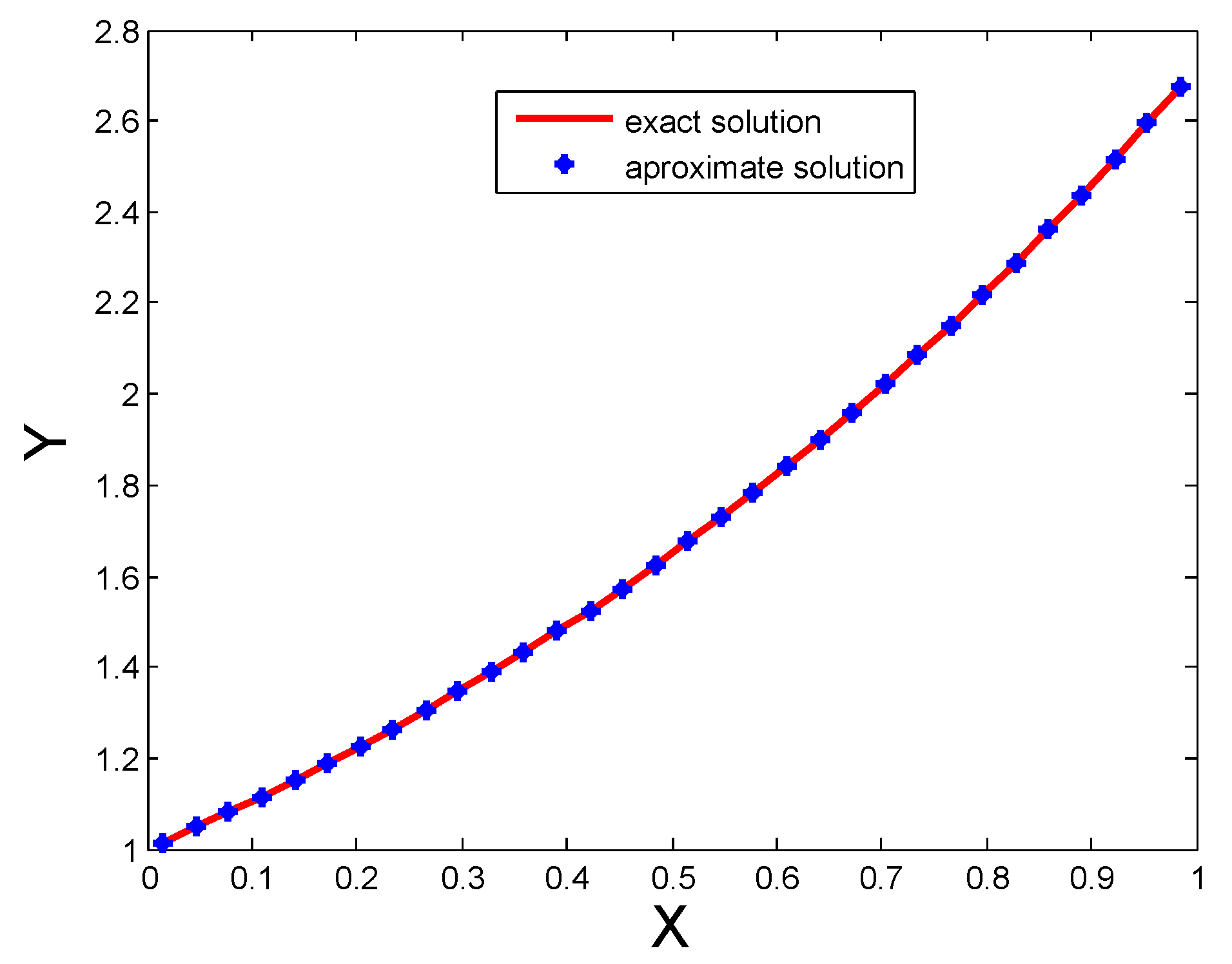
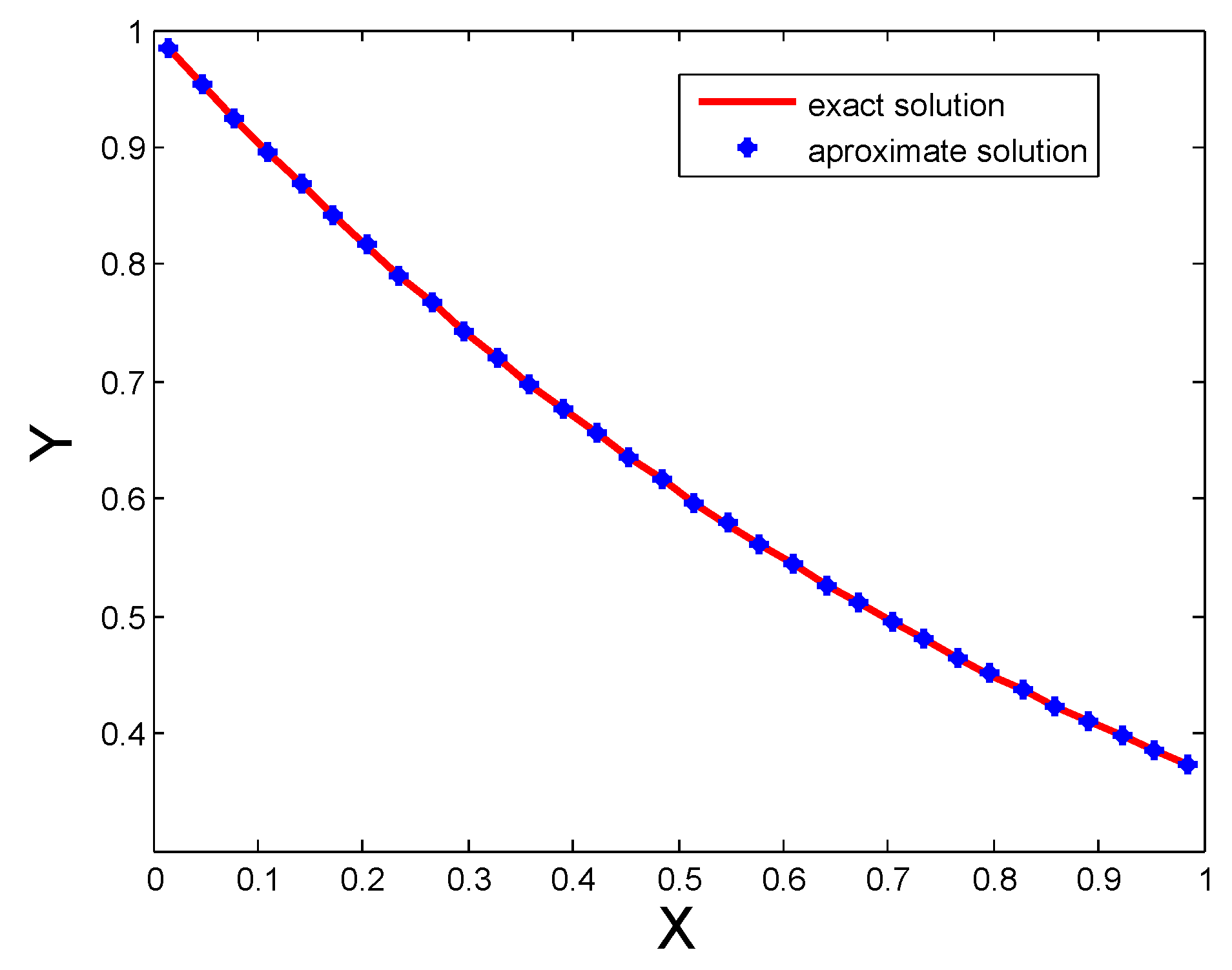

| J | Results [1] | |||
|---|---|---|---|---|
| 1 | 4 | 2.1194 | 1.5933 | 4.84 |
| 2 | 8 | 6.3911 | 4.0412 | 1.36 |
| 3 | 16 | 1.7632 | 1.0141 | 2.01 |
| 4 | 32 | 4.6373 | 2.5447 | 3.54 |
| 5 | 64 | 1.1898 | 6.4159 | 3.85 |
| 6 | 128 | 3.0129 | 1.6638 | — |
| 7 | 256 | 7.5791 | 4.9327 | — |
| J | Results [1] | |||
|---|---|---|---|---|
| 1 | 4 | 5.4262 | 4.6432 | 1.1 |
| 2 | 8 | 1.5086 | 3.1776 | 1.1 |
| 3 | 16 | 3.9748 | 2.9549 | 1.9 |
| 4 | 32 | 1.0203 | 7.3941 | 2.8 |
| 5 | 64 | 2.5847 | 1.8489 | 9.5 |
| 6 | 128 | 6.5045 | 4.6226 | — |
| 7 | 256 | 1.6315 | 1.1556 | — |
| 8 | 512 | 4.0855 | 2.8892 | — |
| 9 | 1024 | 1.0222 | 7.2230 | — |
| 10 | 2048 | 2.5566 | 1.8057 | — |
| J | |||
|---|---|---|---|
| 1 | 4 | 4.71362 | 2.89263 |
| 2 | 8 | 1.02141 | 1.00189 |
| 3 | 16 | 3.72674 | 3.60812 |
| 4 | 32 | 2.61325 | 1.46973 |
| 5 | 64 | 4.58470 | 2.25831 |
| 6 | 128 | 1.84356 | 3.96374 |
| 7 | 256 | 4.01407 | 1.32281 |
| 8 | 512 | 1.37851 | 2.13459 |
| 9 | 1024 | 3.93057 | 4.53722 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Amin, R.; Nazir, S.; García-Magariño, I. A Collocation Method for Numerical Solution of Nonlinear Delay Integro-Differential Equations for Wireless Sensor Network and Internet of Things. Sensors 2020, 20, 1962. https://doi.org/10.3390/s20071962
Amin R, Nazir S, García-Magariño I. A Collocation Method for Numerical Solution of Nonlinear Delay Integro-Differential Equations for Wireless Sensor Network and Internet of Things. Sensors. 2020; 20(7):1962. https://doi.org/10.3390/s20071962
Chicago/Turabian StyleAmin, Rohul, Shah Nazir, and Iván García-Magariño. 2020. "A Collocation Method for Numerical Solution of Nonlinear Delay Integro-Differential Equations for Wireless Sensor Network and Internet of Things" Sensors 20, no. 7: 1962. https://doi.org/10.3390/s20071962
APA StyleAmin, R., Nazir, S., & García-Magariño, I. (2020). A Collocation Method for Numerical Solution of Nonlinear Delay Integro-Differential Equations for Wireless Sensor Network and Internet of Things. Sensors, 20(7), 1962. https://doi.org/10.3390/s20071962






