Spacecraft Angular Rates Estimation with Gyrowheel Based on Extended High Gain Observer
Abstract
:1. Introduction
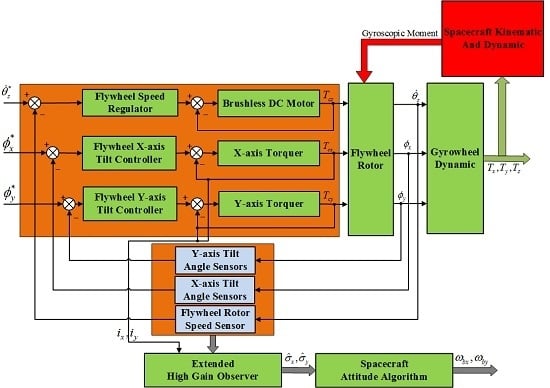
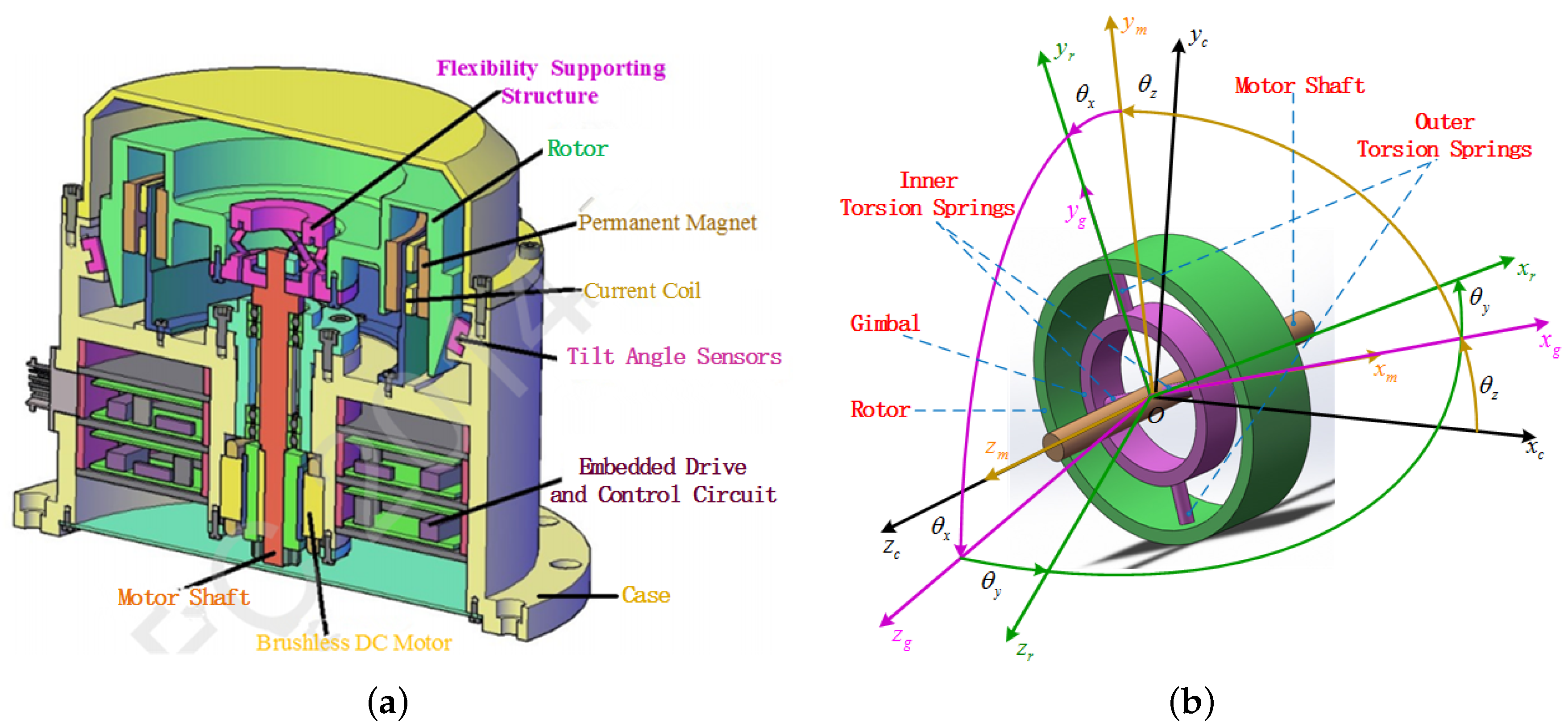
2. Descriptions of Gyrowheel
Gyrowheel Physical Structure
3. Gyrowheel Mathematical Modeling
3.1. Gyrowheel Coordinates and Frames
3.2. Gyrowheel Kinematic Equations
3.3. Gyrowheel Dynamic Equations
3.4. Relationship between the Unmeasurable Variables and the Measurable Variables
3.5. Description of Angular Rates Estimation Problem Using Gyrowheel State Equation
- (1)
- The errors caused by the linearization of nonlinear equations of GW in large tilt angles can be avoided.
- (2)
- The problem of dynamic drift error compensation existing both traditional mechanic gyroscopes [14] and GW can be solved by the above derivation and the following application of full dynamical model in angular rates estimation.
- (3)
- The initial iteration error accumulation of the calculated angular rates of the spacecraft, caused by the angular acceleration () in the term , is eliminated by the real-time estimation of the term.
- (4)
- The amplification of measurement noise by the multi-difference of measured tilt angles with measurement noise can be weakened by appropriately choosing the design parameters of HGO [15].
4. Gyrowheel High Gain Observer for Angular Rates Estimation
4.1. Gyrowheel High Gain Observer Design
4.2. EHGO Error Convergence Proof
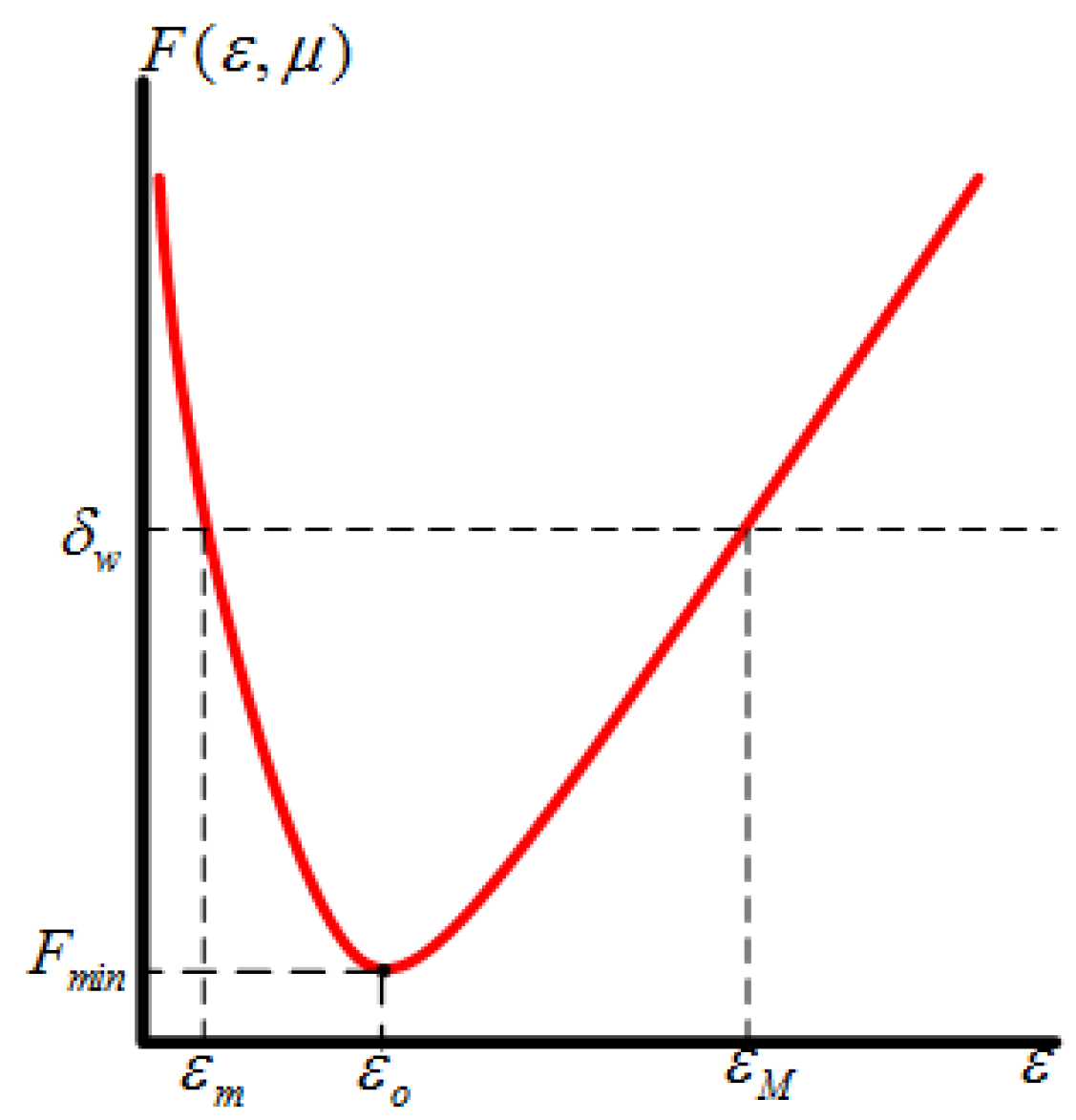
4.3. Influence Analysis of Measurement Noise
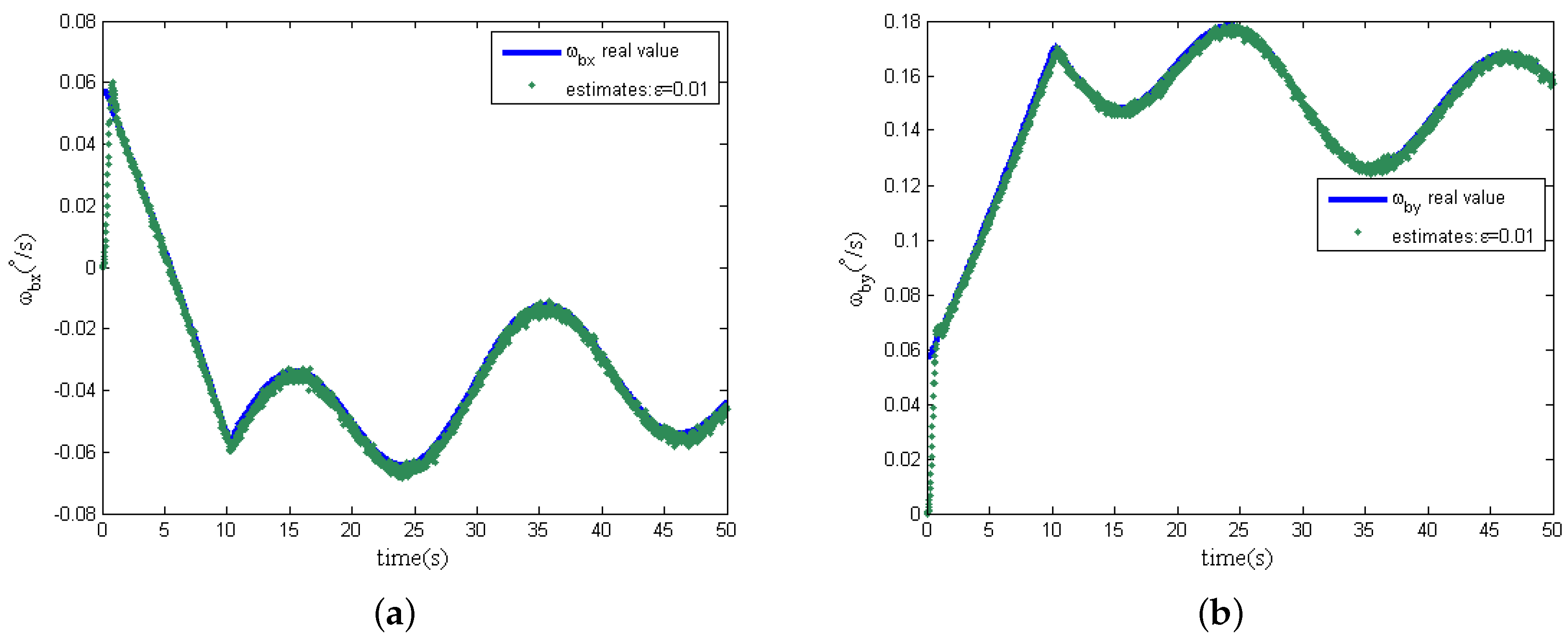
5. Simulation
6. Conclusions
- (1)
- A complete dynamical model of GW is built with chosen generalized coordinates () by Lagrange’s Method, and since the generalized coordinates () and its derivatives in the GW dynamical model are unmeasurable, the relationships between the unmeasurable generalized coordinates and the measurable variables () by sensors are derived to construct the nonlinear state equation expressed by measurable variables for the spacecraft rate estimations with the GW.
- (2)
- The affine nonlinear state equation of GW and measurement equation are built based on the contribution (1). Combining the affine nonlinear state equation with measurement equation and extending the relevant terms of spacecraft angular rates as states, a high gain observer is designed to estimate the relevant terms of the spacecraft angular rates. Through solving the known differential equation, the spacecraft angular rates can be calculated.
- (3)
- The stability of the designed EHGO in contribution (2) is proved by Lyapunov’s stability theory, and the effects of the design parameter ε and measurement noise on the estimation accuracy are also analyzed.
Acknowledgments
Author Contributions
Conflicts of Interest
Abbreviations
| GW7 | Gyrowheel |
| ACS | Attitude control system |
| HGO | High gain observer |
| EHGO | Extended high gain observer |
| CMG | Control moment gyroscope |
| MSDGCMG | Magnetically suspended double-gimbal control moment gyroscope |
| AMBs | Active magnetic bearings |
| DOF | Degrees of freedom |
| SINS | Strapdown inertial navigation system |
| DTG | Dynamically tuned gyroscope |
| VSDGCMG | Variable speed control moment gyroscope |
Appendix A
References
- Richie, D.J.; Lappas, V.J.; Palmer, P.L. Sizing/optimization of a small satellite energy storage and attitude control system. J. Spacecr. Rocket. 2007, 44, 940–952. [Google Scholar] [CrossRef]
- Richie, D.J.; Lappas, V.J.; Prassinos, G. A practical small satellite variable-speed control moment gyroscope for combined energy storage and attitude control. Acta Astronaut. 2009, 65, 1745–1764. [Google Scholar] [CrossRef]
- Chobotov, V.A. Spacecraft Attitude Dynamics and Control; Krieger Publishing Company: Tallahassee, FL, USA, 1991. [Google Scholar]
- Fang, J.; Zheng, S.; Han, B. Attitude sensing and dynamic decoupling based on active magnetic bearing of MSDGCMG. Instrum. Meas. IEEE Trans. 2012, 61, 338–348. [Google Scholar] [CrossRef]
- Cui, P.; Zhang, H.; Yan, N. Performance Testing of a Magnetically Suspended Double Gimbal Control Moment Gyro Based on the Single Axis Air Bearing Table. Sensors 2012, 12, 9129–9145. [Google Scholar] [CrossRef] [PubMed]
- Liu, B.; Fang, J.; Liu, G. Design of a magnetically suspended gyrowheel and analysis of key technologies. Acta Aeronaut. Astronaut. Sin. 2011, 32, 1478–1487. [Google Scholar]
- Senez, M.; Pokrupa, N.; Taylor, B. The GyroWheel testing and flight qualification program. Adv. Astronaut. Sci. 2002, 111, 283–295. [Google Scholar]
- Tyc, G.; Whitehead, W.; Pradhan, S. GyroWheelTM—An Innovative New Actuator∖Sensor for 3-axis Spacecraft Attitude Control. In Proceedings of the 13th Annual AIAA/USU Conference on Small Satellites, Logan, UT, USA, 23–26 August 1999.
- Xu, X.; Chen, S. Field balancing and harmonic vibration suppression in rigid AMB-rotor systems with rotor imbalances and sensor runout. Sensors 2015, 15, 21876–21897. [Google Scholar] [CrossRef] [PubMed]
- Fang, J.; Zheng, S.; Han, B. AMB vibration control for structural resonance of double-gimbal control moment gyro with high-speed magnetically suspended rotor. IEEE/ASME Trans. Mech. 2013, 18, 32–43. [Google Scholar] [CrossRef]
- Zheng, S.; Han, B. Investigations of an integrated angular velocity measurement and attitude control system for spacecraft using magnetically suspended double-gimbal CMGs. Adv. Space Res. 2013, 51, 2216–2228. [Google Scholar] [CrossRef]
- Ower, J.C. Analysis and Control System Design of an Innovative Tuned-Rotor Instrument; Carleton University: Ottawa, ON, Canada, 2000. [Google Scholar]
- Hall, J.M. Calibration of an Innovative Rate Sensing/momentum Management Instrument for De-tuned Operation and Temperature Effects; Carleton University: Ottawa, ON, Canada, 2008. [Google Scholar]
- Titterton, D.H.; Weston, J.L. Strapdown Inertial Navigation Technology, 2nd ed.; Peter Peregrinus Ltd. on behalf of the Institute of Electrical Engineers: London, UK, 2004. [Google Scholar]
- Oh, S.; Khalil, H.K. Nonlinear output-feedback tracking using high-gain observer and variable structure control. Automatica 1997, 33, 1845–1856. [Google Scholar] [CrossRef]
- Ahrens, J.H.; Khalil, H.K. High-gain observers in the presence of measurement noise: A switched-gain approach. Automatica 2009, 45, 936–943. [Google Scholar] [CrossRef]
- Khalil, H.K.; Praly, L. High-gain observers in nonlinear feedback control. Int. J. Robust Nonlinear Control 2014, 24, 993–1015. [Google Scholar] [CrossRef]
- Ma, K.; Khalil, H.K.; Yao, Y. Guidance law implementation with performance recovery using an extended high-gain observer. Aerosp. Sci. Technol. 2013, 24, 177–186. [Google Scholar] [CrossRef]
- Cain, J.S. Investigation of the Crossed Flexure Pivot and the Dynamics of the Momentum Management System Spacecraft Control Component and the Dynamically Tuned Gyroscope; Carleton University: Ottawa, ON, Canada, 1999. [Google Scholar]
- Dabroom, A.M.; Khalil, H.K. Output feedback sampled-data control of nonlinear systems using high-gain observers. IEEE Trans. Autom. Control 2001, 46, 1712–1725. [Google Scholar] [CrossRef]
- Lefferts, E.J.; Markley, F.L.; Shuster, M.D. Kalman filtering for spacecraft attitude estimation. J. Guid. Control Dyn. 1982, 5, 417–429. [Google Scholar] [CrossRef]
- Song, T.L. Observability of target tracking with bearings-only measurements. IEEE Trans. Aerosp. Electron. Syst. 1996, 32, 1468–1472. [Google Scholar] [CrossRef]
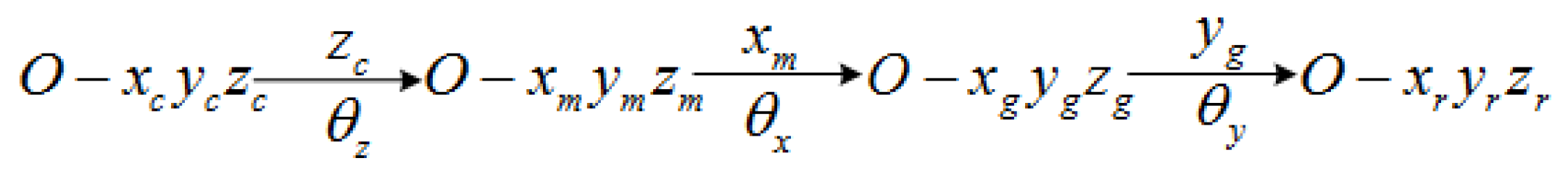




| Parameter Name | Value |
|---|---|
| Rotor transverse-axis inertia | kg · m2 |
| Rotor spin-axis inertia | kg · m2 |
| Gimbal transverse-axis inertia | kg · m2 |
| Gimbal spin-axis inertia | kg · m2 |
| Torsion Spring Stiffness | |
| Torsion Spring damping | 0 Nm/(rad/s) |
| Spacecraft Inertia | kg · m2 |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, X.; Yao, Y.; Ma, K.; Zhao, H.; He, F. Spacecraft Angular Rates Estimation with Gyrowheel Based on Extended High Gain Observer. Sensors 2016, 16, 537. https://doi.org/10.3390/s16040537
Liu X, Yao Y, Ma K, Zhao H, He F. Spacecraft Angular Rates Estimation with Gyrowheel Based on Extended High Gain Observer. Sensors. 2016; 16(4):537. https://doi.org/10.3390/s16040537
Chicago/Turabian StyleLiu, Xiaokun, Yu Yao, Kemao Ma, Hui Zhao, and Fenghua He. 2016. "Spacecraft Angular Rates Estimation with Gyrowheel Based on Extended High Gain Observer" Sensors 16, no. 4: 537. https://doi.org/10.3390/s16040537
APA StyleLiu, X., Yao, Y., Ma, K., Zhao, H., & He, F. (2016). Spacecraft Angular Rates Estimation with Gyrowheel Based on Extended High Gain Observer. Sensors, 16(4), 537. https://doi.org/10.3390/s16040537