Design and Vibration Sensitivity Analysis of a MEMS Tuning Fork Gyroscope with an Anchored Diamond Coupling Mechanism
Abstract
:1. Introduction
2. Architecture Design
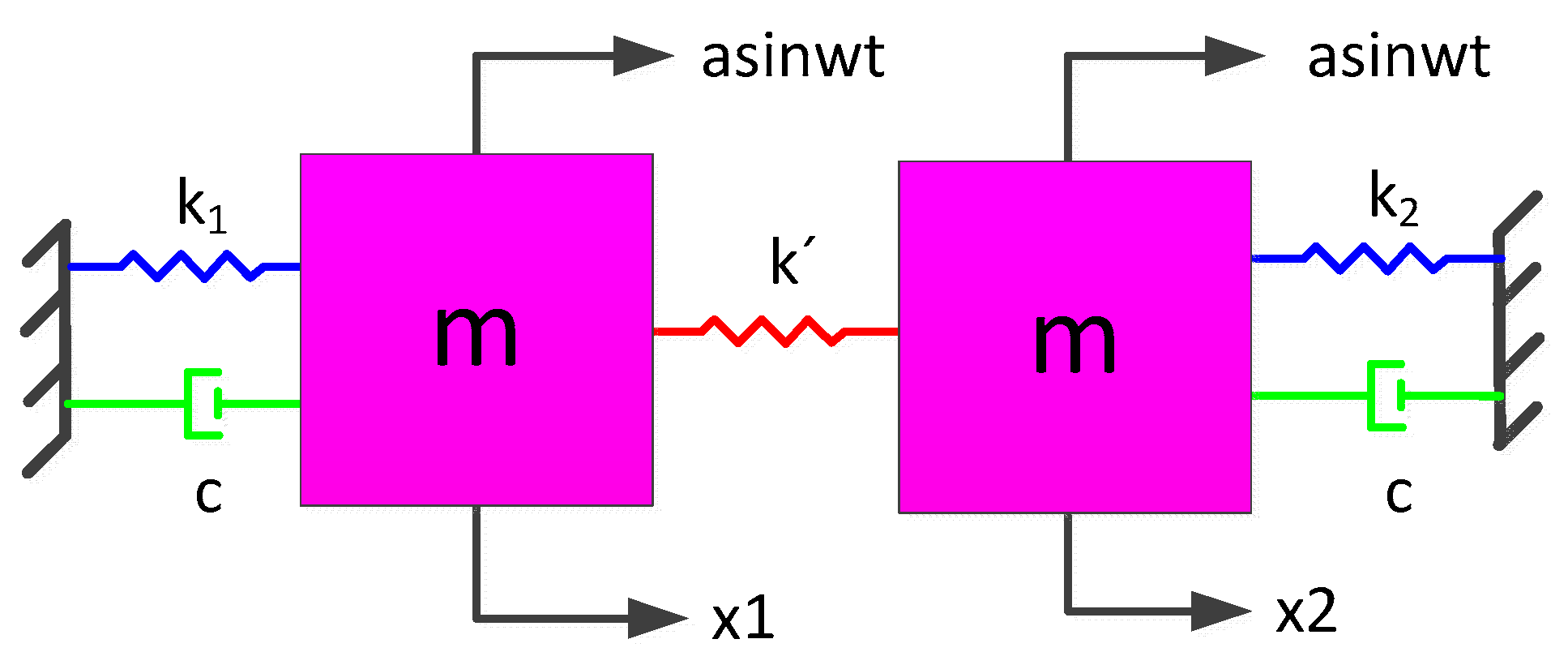
3. Theoretical Analysis
4. FEM Simulations
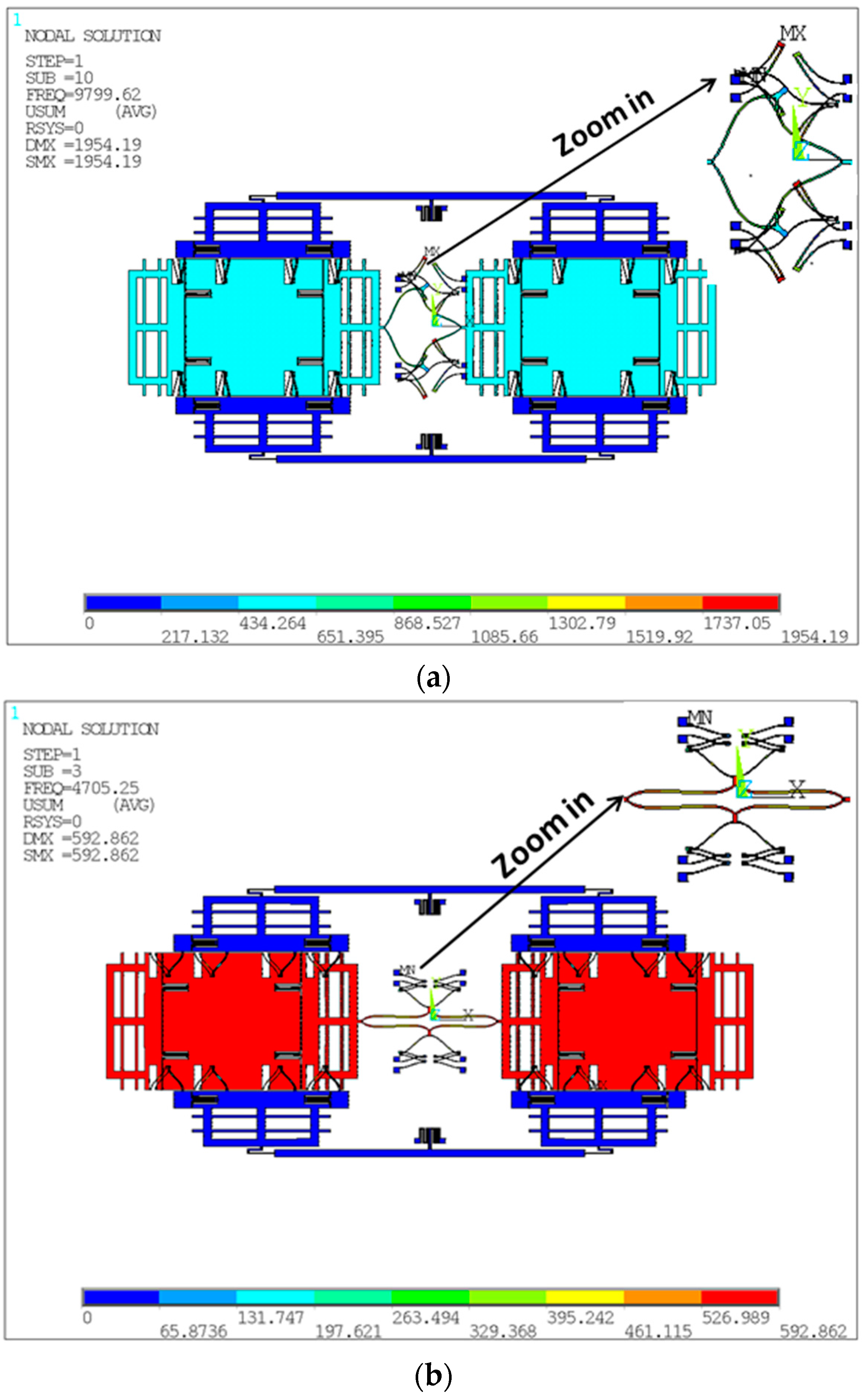
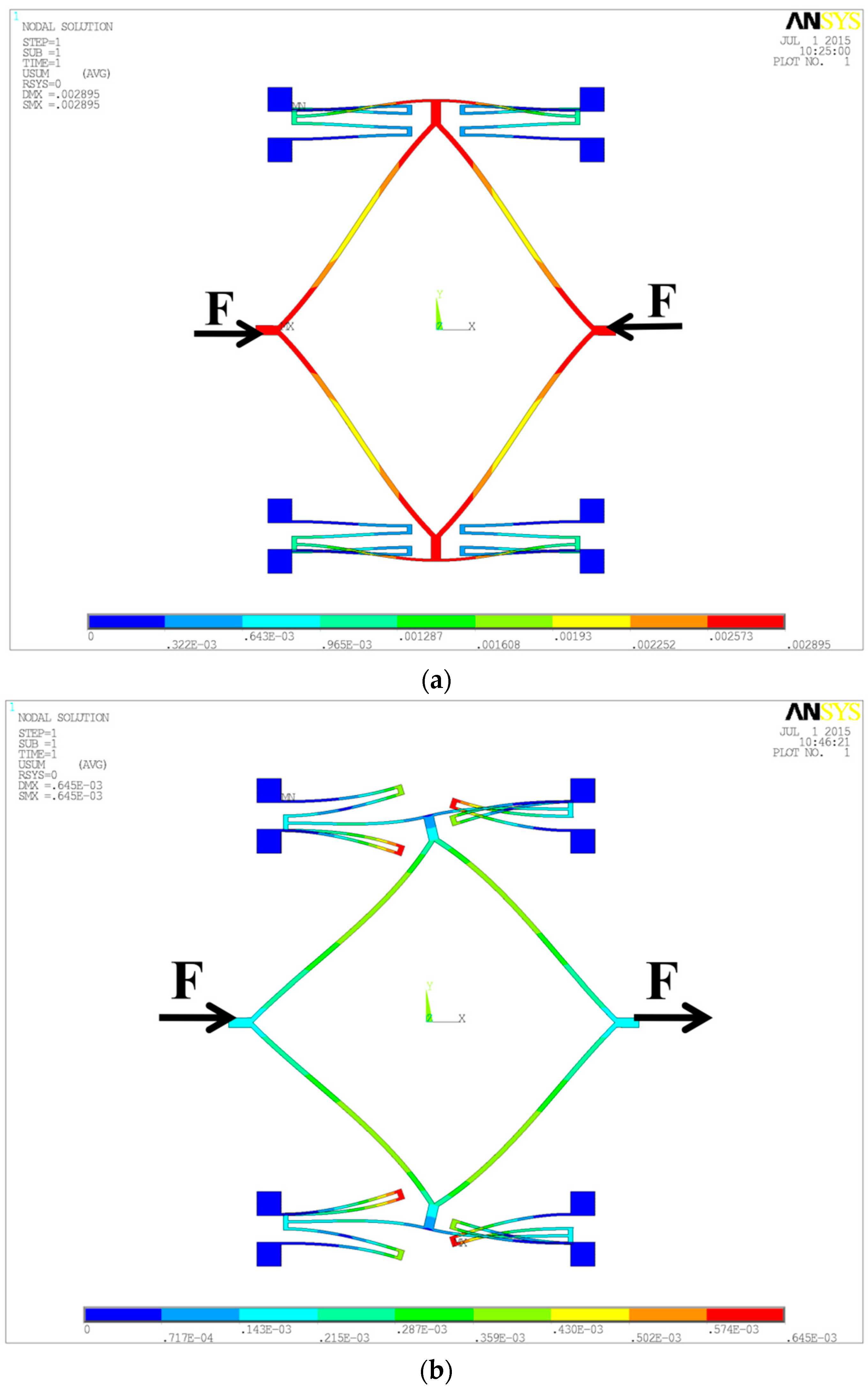
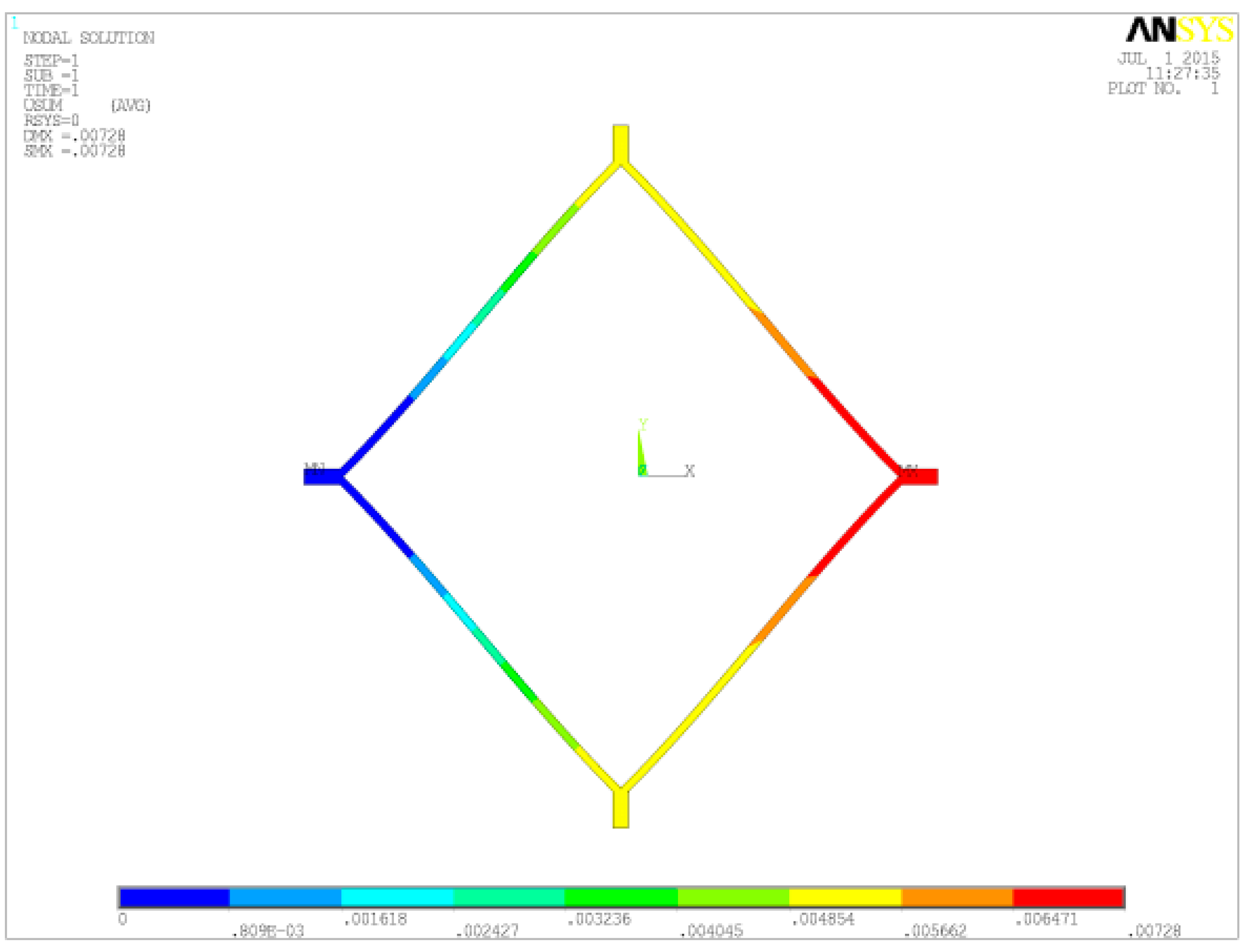
4.1. SDR Analysis
4.2. Simulations
4.3. Numerical and Theoretical Comparisons
5. Discussion
6. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Shkel, A.M. Type I and type II micromachined vibratory gyroscopes. In Proceedings of the IEEE PLANS, Position Location and Navigation Symposium, San Diego, CA, USA, 25–27 April 2006; pp. 586–593.
- Sharma, A.; Zaman, M.F.; Ayazi, F. A sub-0.2°/hr bias drift micromechanical silicon gyroscope with automatic CMOS mode-matching. IEEE J. Solid State Circuits 2009, 44, 1593–1608. [Google Scholar] [CrossRef]
- Zotov, S.A.; Trusov, A.A.; Shkel, A.M. High-range angular rate sensor based on mechanical frequency modulation. J. Microelectromech. Syst. 2012, 21, 398–405. [Google Scholar] [CrossRef]
- Yoon, S.W.; Lee, S.; Najafi, K. Vibration-induced errors in MEMS tuning fork gyroscopes. Sens. Actuators A Phys. 2012, 180, 32–44. [Google Scholar] [CrossRef]
- Wang, W.; Lv, X.Y.; Sun, F. Design of a novel MEMS gyroscope array. Sensors 2013, 13, 1651–1663. [Google Scholar] [CrossRef] [PubMed]
- Xia, D.Z.; Yu, C.; Kong, L. The development of micromachiend gyroscope structure and circuitry technology. Sensors 2014, 14, 1394–1473. [Google Scholar] [CrossRef] [PubMed]
- Xie, H.K.; Fedder, G.K. Fabrication, characterization, and analysis of a DRIE CMOS-MEMS gyroscope. IEEE Sens. J. 2003, 3, 622–631. [Google Scholar]
- Zaman, M.F.; Sharma, A.; Amini, B.; Ayazi, F.A. Towards inertial grade vibratory microgyros: A high-Q in-plane silicon-on-insulator tuning fork device. In Proceedings of the Solid-State Sensor, Actuator and Microsystems Workshop, Hilton Head Island, SC, USA, 6–10 June 2004; pp. 384–385.
- Azgin, K.; Temiz, Y.; Akin, T. An SOI-MEMS tuning fork gyroscope with linearly coupled drive mechanism. In Proceedings of the IEEE 20th International Conference on Micro Electro Mechanical Systems, Kobe, Japan, 21–25 January 2007; pp. 607–610.
- Ding, X.K.; Li, H.S.; Ni, Y.F.; Sang, P.C. Analysis of frequency response and scale-factor of tuning fork micro-gyroscope operating at atmospheric pressure. Sensors 2015, 15, 2453–2472. [Google Scholar] [CrossRef] [PubMed]
- Trusov, A.A.; Schofield, A.R.; Shkel, A.M. Micromachined rate gyroscope architecture with ultra-high quality factor and improved mode ordering. Sens. Actuators A Phys. 2011, 165, 26–34. [Google Scholar] [CrossRef]
- Singh, T.P.; Sugano, K.; Tsuchiya, T.; Tabata, O. Experimental verification of frequency decoupling effect on acceleration sensitivity in tuning fork gyroscopes using in-plane coupled resonators. Microsyst. Technol. 2013, 20, 403–411. [Google Scholar]
- Guan, Y.W.; Gao, S.Q.; Liu, H.P.; Niu, S.H. Acceleration sensitivity of tuning fork gyroscopes: Theoretical model, simulation and experimental verification. Microsyst. Technol. 2015, 21, 1313–1323. [Google Scholar] [CrossRef]
- Simon, B.R.; Trusov, A.A.; Shkel, A.M. Anti-phase mode isolation in tuning-fork MEMS using a lever coupling design. In Proceedings of the 2012 IEEE Sensors, Taipei, Taiwan, 28–31 October 2012; pp. 42–45.
- Zotov, S.A.; Simon, B.R.; Prikhodko, I.P.; Trusov, A.A.; Shkel, A.M. Quality factor maximization through dynamic balancing of tuning fork resonator. IEEE Sens. J. 2014, 14, 2706–2714. [Google Scholar] [CrossRef]
- Guan, Y.W.; Gao, S.Q.; Jin, L.; Cao, L.M. Design and vibration sensitivity of a MEMS tuning fork gyroscope with anchored coupling mechanism. Microsyst. Technol. 2016, 22, 247–254. [Google Scholar] [CrossRef]


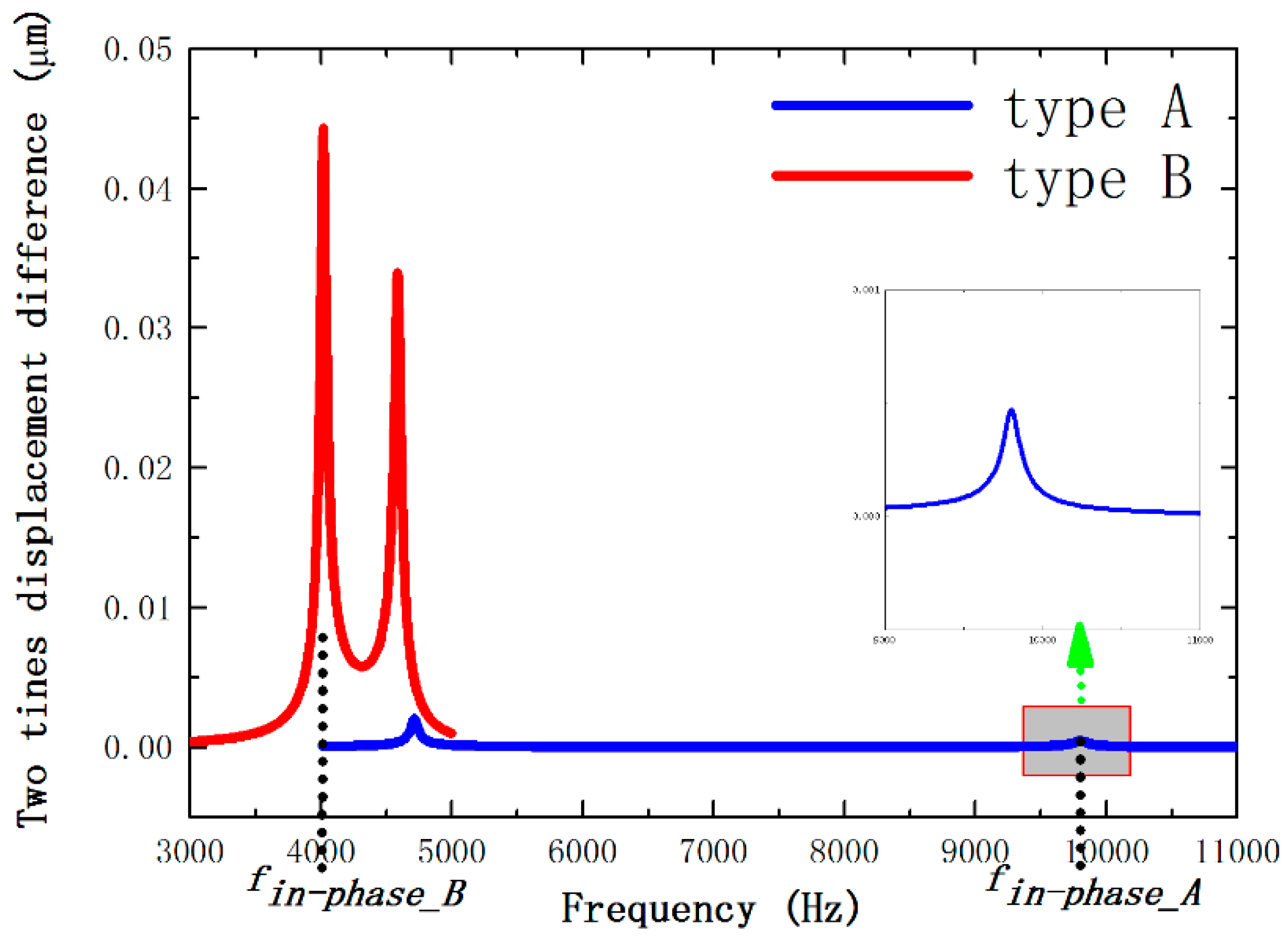
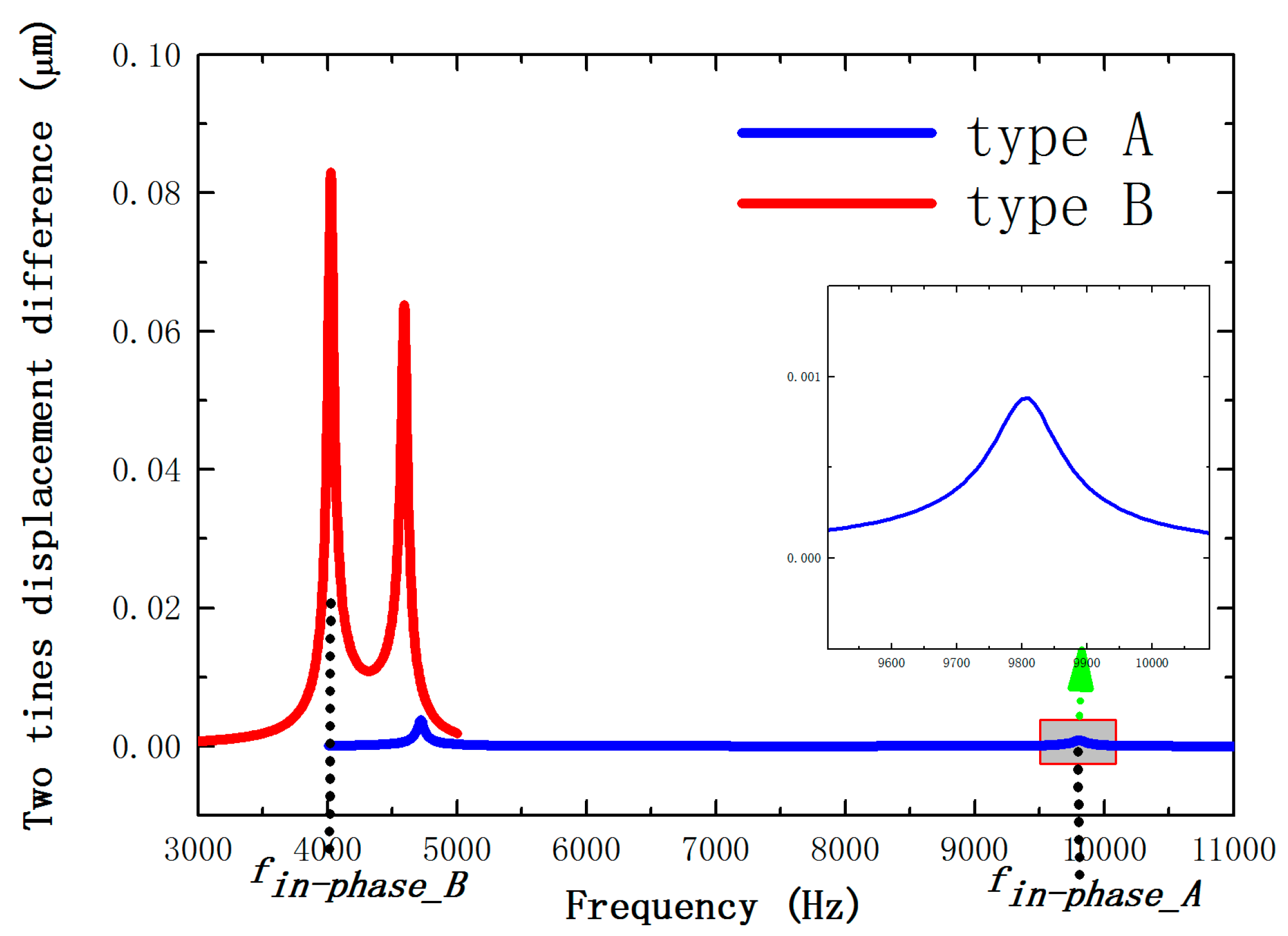
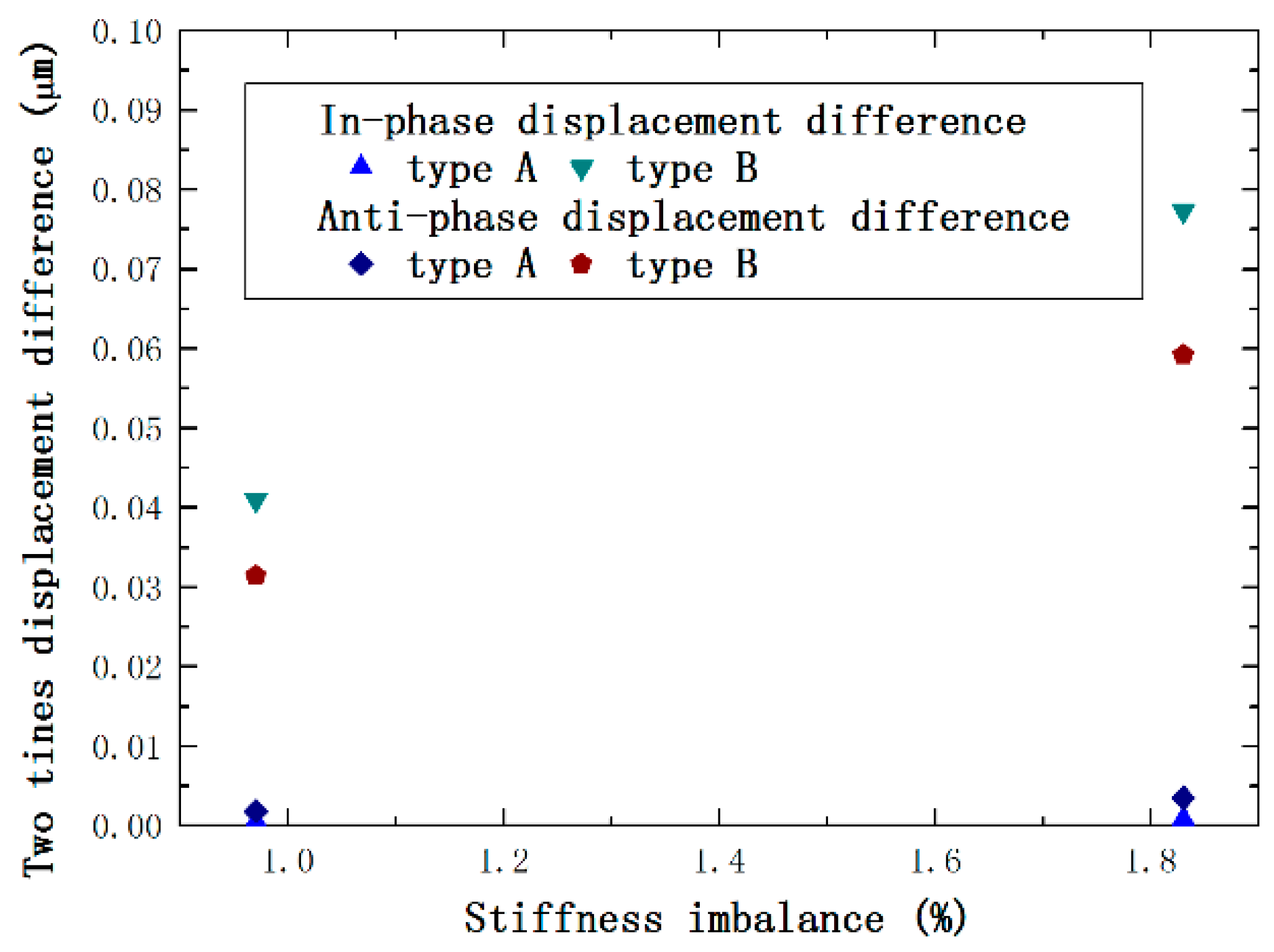
| Parameters | Value | Parameters | Value |
|---|---|---|---|
| Sense-mode mass | 1.3738 × 10−6 Kg | Structural thickness | 60 µm |
| Structure type | Type A/Type B | Sense-mode Q | 100 |
| Stiffness imbalance | 0.97%/1.83% | Common acceleration | 9.8 m/s2 |
| Springs stiffness k | 748.5 N/m | Stiffness difference ratio ηa/ηb | 11.80/0.73 |
| ε (%) | In-Phase Modal Frequency (Hz) | Anti-Phase Modal Frequency (Hz) | ||
|---|---|---|---|---|
| Type A | Type B | Type A | Type B | |
| 0 | 9799.6 | 4007.1 | 4705.3 | 4578.1 |
| 0.97 | 9803.0 | 4015.7 | 4712.7 | 4585.9 |
| 1.83 | 9806.0 | 4023.2 | 4719.3 | 4592.9 |
| Type | Type A | Type B | |||||
|---|---|---|---|---|---|---|---|
| ε | Theoretical Value | Simulation Value | Error Rate | Theoretical Value | Simulation Value | Error Rate | |
| In-phase displacement difference (µm) | 0.97% | 0.000425 | 0.000456 | 7.33% | 0.0410 | 0.0444 | 8.20% |
| 1.83% | 0.000802 | 0.000853 | 6.40% | 0.0773 | 0.0832 | 7.62% | |
| Anti-phase displacement difference (µm) | 0.97% | 0.00184 | 0.00199 | 7.89% | 0.0314 | 0.0340 | 8.17% |
| 1.83% | 0.00347 | 0.00372 | 7.08% | 0.0592 | 0.0636 | 7.35% | |
| Type | Type C | Type D | |||||
|---|---|---|---|---|---|---|---|
| ε | Theoretical Value | Simulation Value | Error Rate | Theoretical Value | Simulation Value | Error Rate | |
| In-phase displacement difference (µm) | 0.97% | 0.00627 | 0.0068 | 6.14% | 0.0525 | 0.0561 | 6.42% |
| 1.83% | 0.0118 | 0.0125 | 5.60% | 0.0986 | 0.105 | 6.10% | |
| Anti-phase displacement difference (µm) | 0.97% | 0.0106 | 0.0114 | 7.02% | 0.0423 | 0.0453 | 6.62% |
| 1.83% | 0.0200 | 0.0212 | 5.66% | 0.0795 | 0.0844 | 5.81% | |
| ε (%) | Type A | Type B | Reduced Rate | |
|---|---|---|---|---|
| In-phase displacement difference (µm) | 0.97 | 0.000425 | 0.0410 | 99.0% |
| 1.83 | 0.000802 | 0.0773 | ||
| Anti-phase displacement difference (µm) | 0.97 | 0.00184 | 0.0314 | 94.1% |
| 1.83 | 0.00347 | 0.0592 | ||
| ε (%) | Type A | Type C | Reduced Rate | |
|---|---|---|---|---|
| In-phase displacement difference (µm) | 0.97 | 0.000425 | 0.00627 | 93.2% |
| 1.83 | 0.000802 | 0.0118 | ||
| Anti-phase displacement difference (µm) | 0.97 | 0.00184 | 0.0106 | 82.6% |
| 1.83 | 0.00347 | 0.0200 | ||
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guan, Y.; Gao, S.; Liu, H.; Jin, L.; Niu, S. Design and Vibration Sensitivity Analysis of a MEMS Tuning Fork Gyroscope with an Anchored Diamond Coupling Mechanism. Sensors 2016, 16, 468. https://doi.org/10.3390/s16040468
Guan Y, Gao S, Liu H, Jin L, Niu S. Design and Vibration Sensitivity Analysis of a MEMS Tuning Fork Gyroscope with an Anchored Diamond Coupling Mechanism. Sensors. 2016; 16(4):468. https://doi.org/10.3390/s16040468
Chicago/Turabian StyleGuan, Yanwei, Shiqiao Gao, Haipeng Liu, Lei Jin, and Shaohua Niu. 2016. "Design and Vibration Sensitivity Analysis of a MEMS Tuning Fork Gyroscope with an Anchored Diamond Coupling Mechanism" Sensors 16, no. 4: 468. https://doi.org/10.3390/s16040468
APA StyleGuan, Y., Gao, S., Liu, H., Jin, L., & Niu, S. (2016). Design and Vibration Sensitivity Analysis of a MEMS Tuning Fork Gyroscope with an Anchored Diamond Coupling Mechanism. Sensors, 16(4), 468. https://doi.org/10.3390/s16040468







