Water Dynamics in Highly Concentrated Protein Systems—Insight from Nuclear Magnetic Resonance Relaxometry
Abstract
1. Introduction
Theory
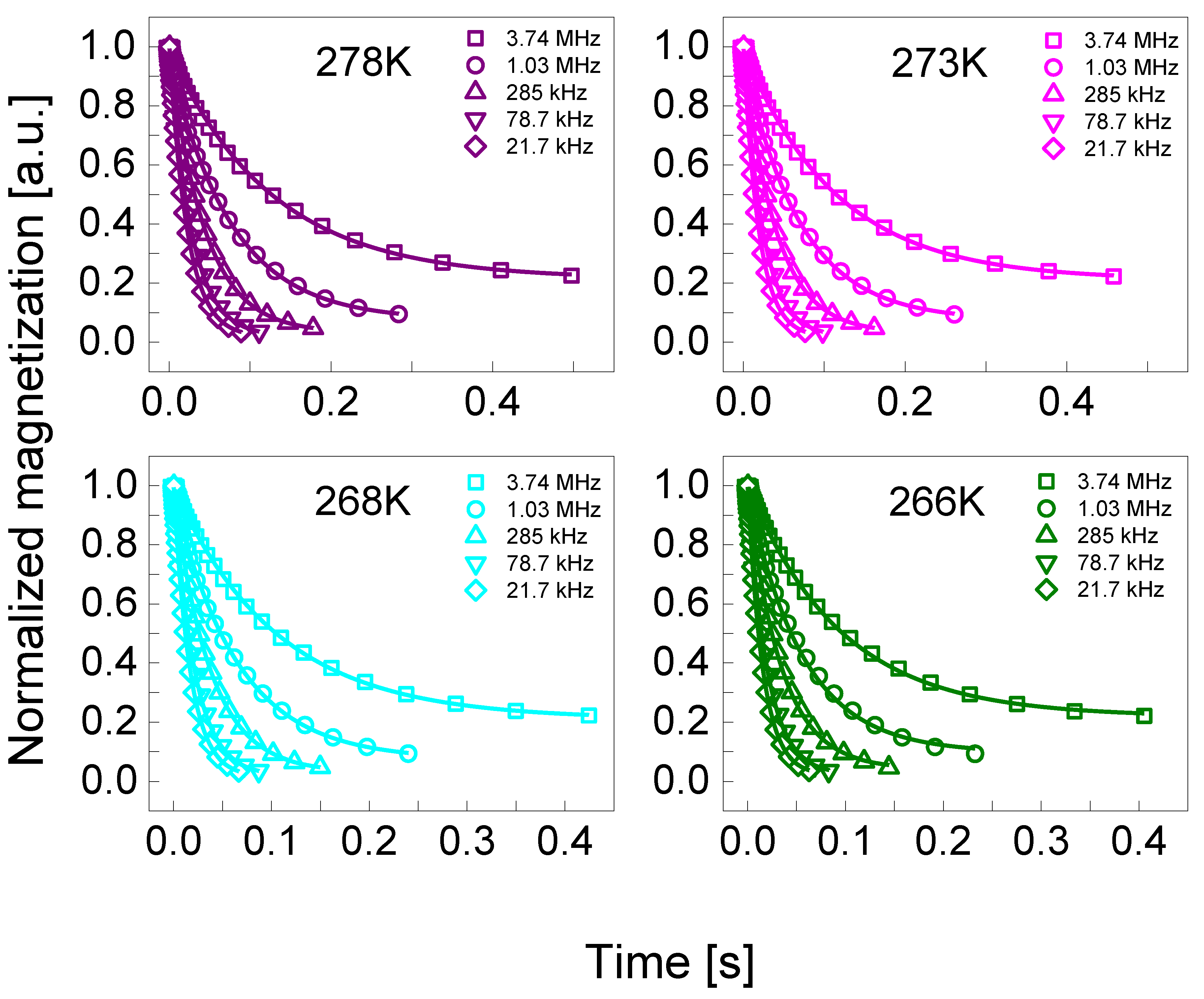
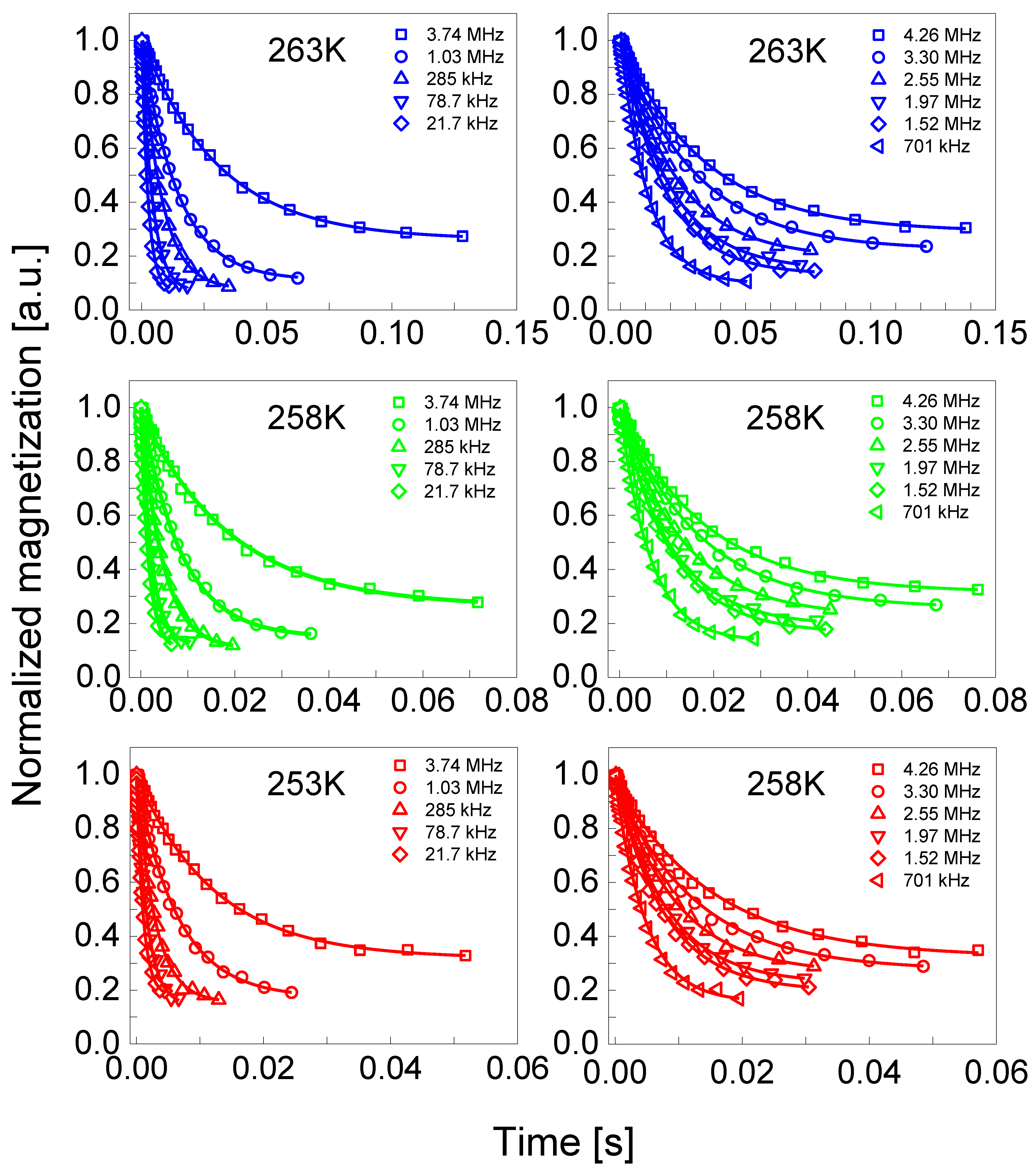
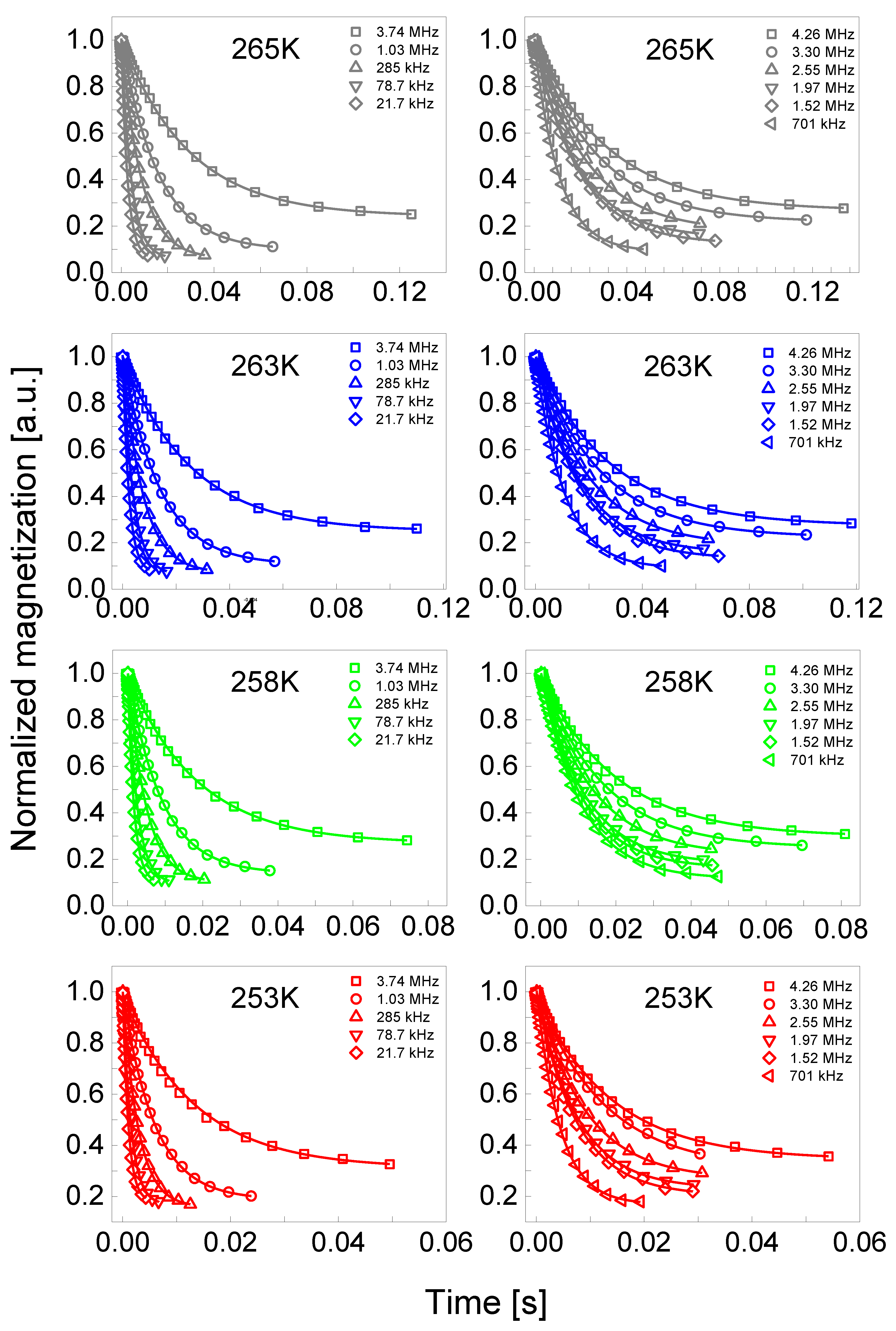
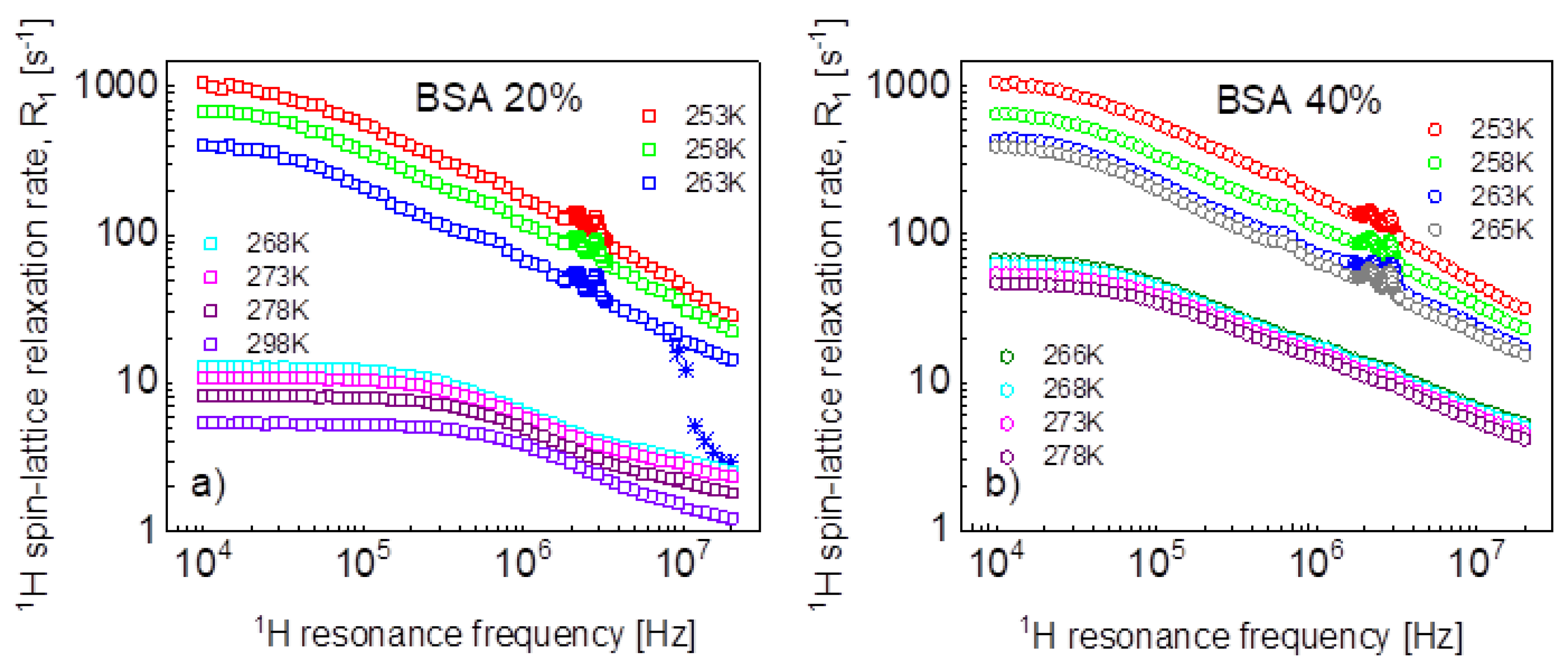
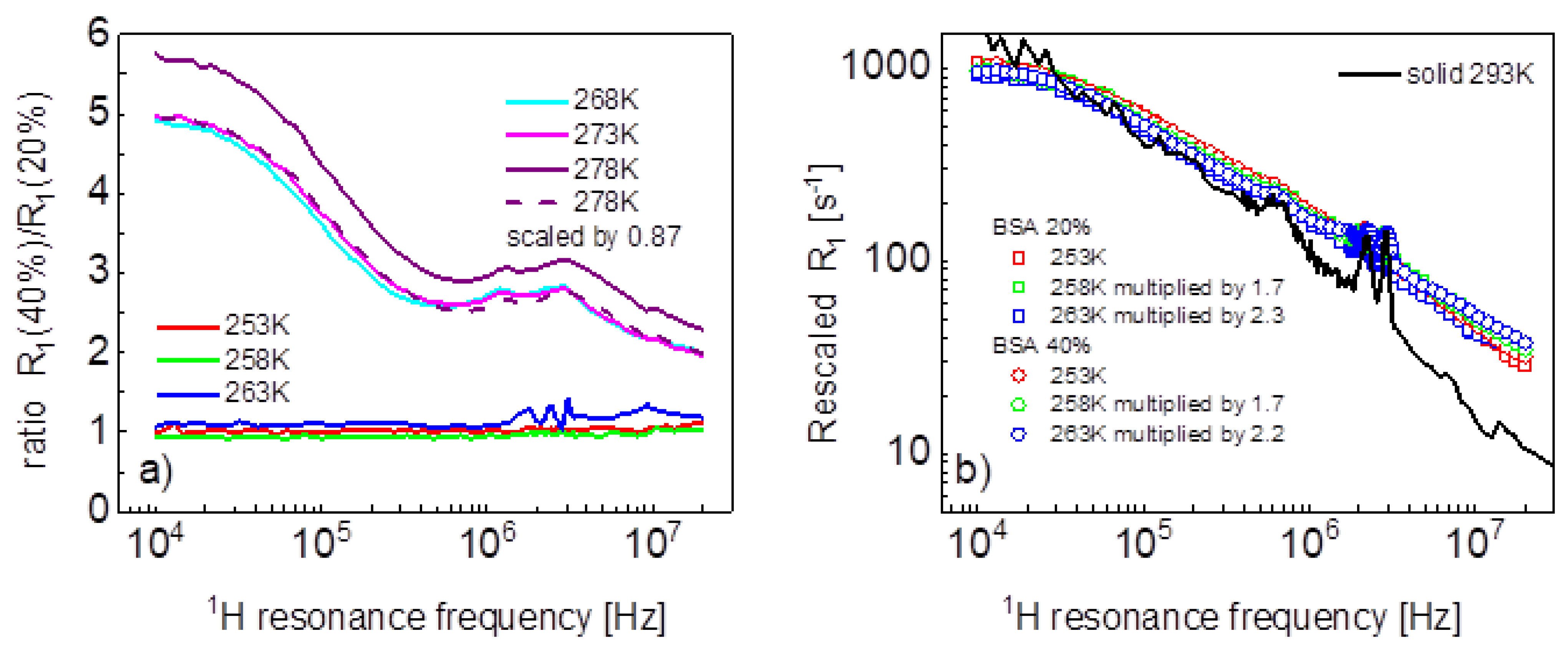
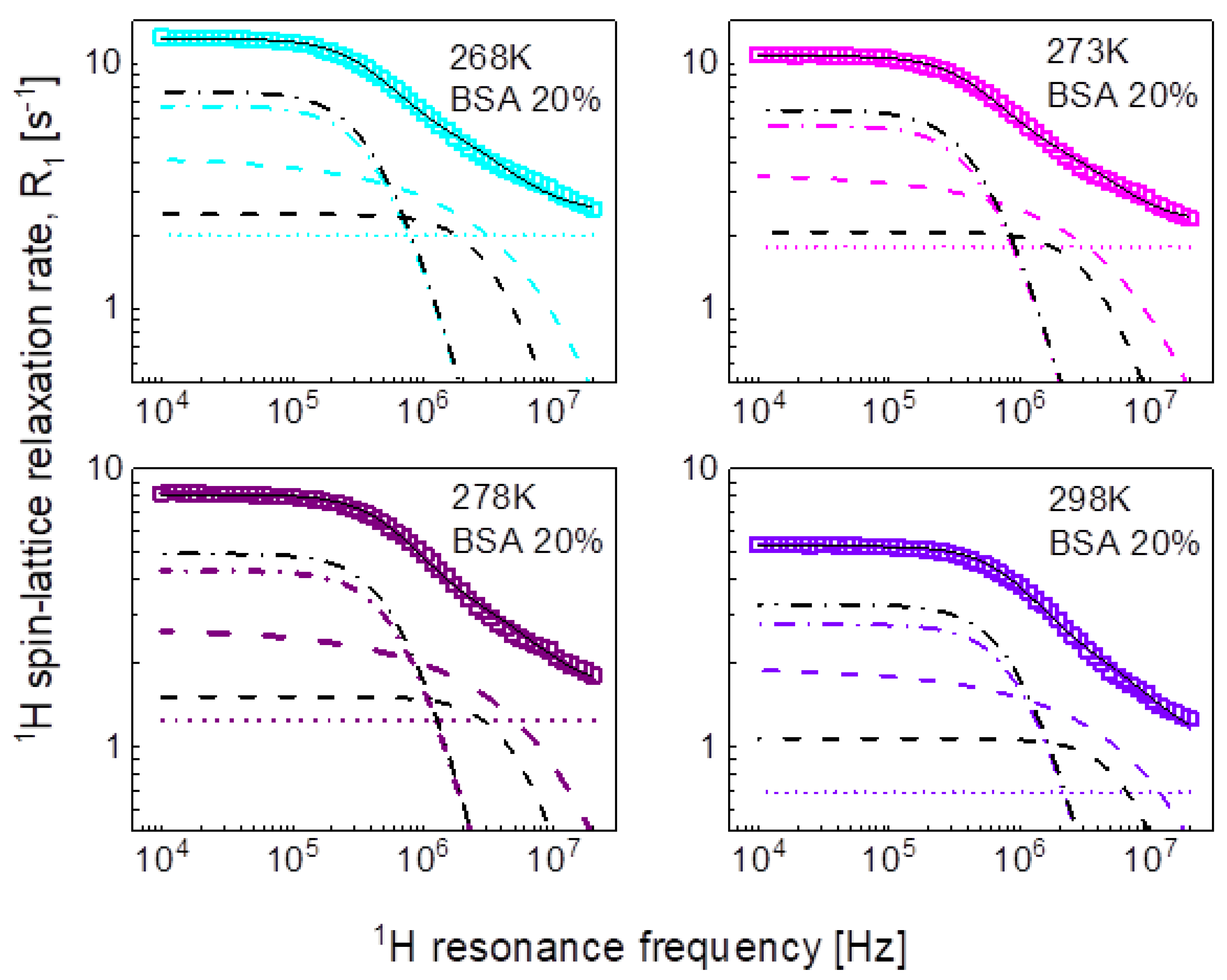
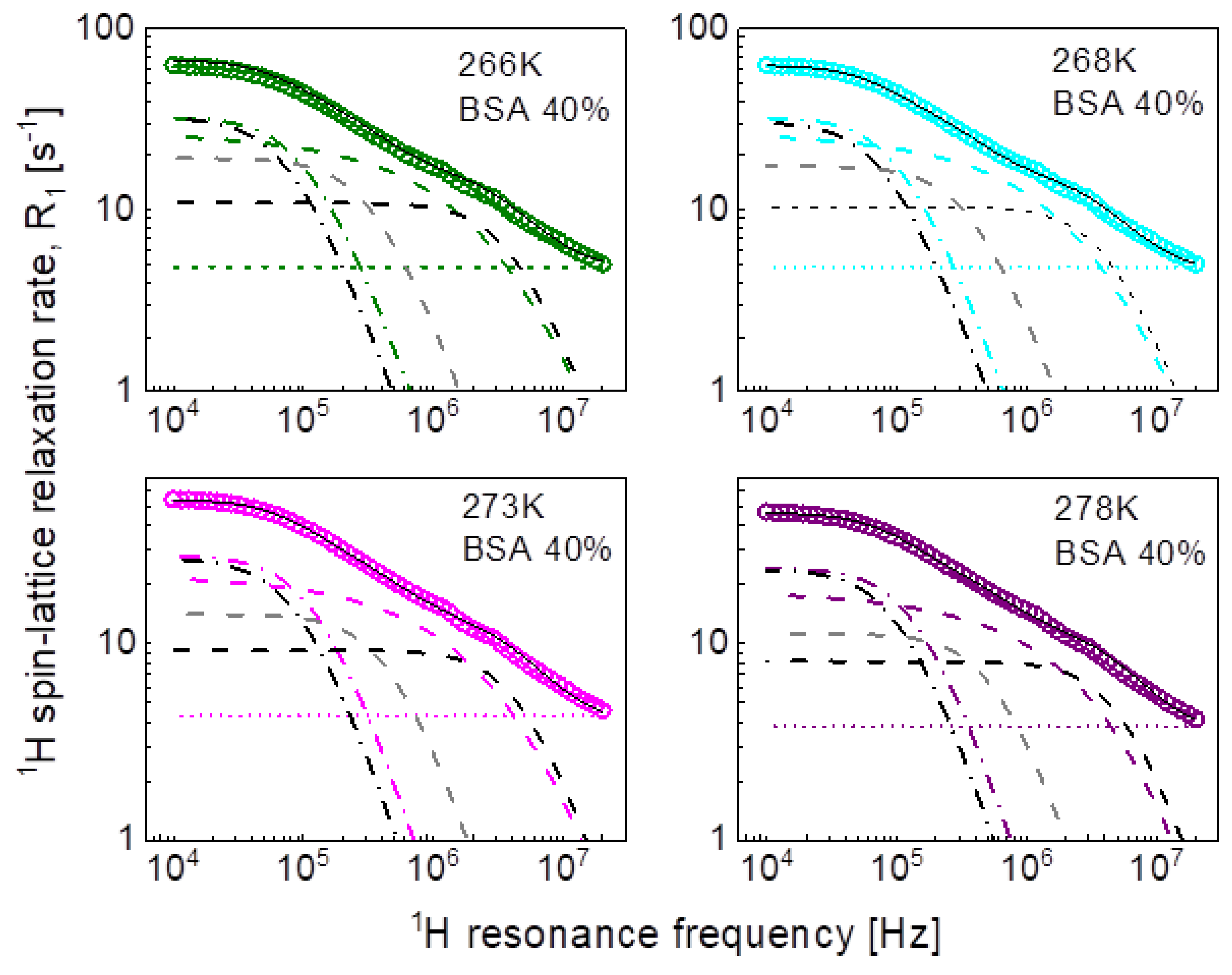
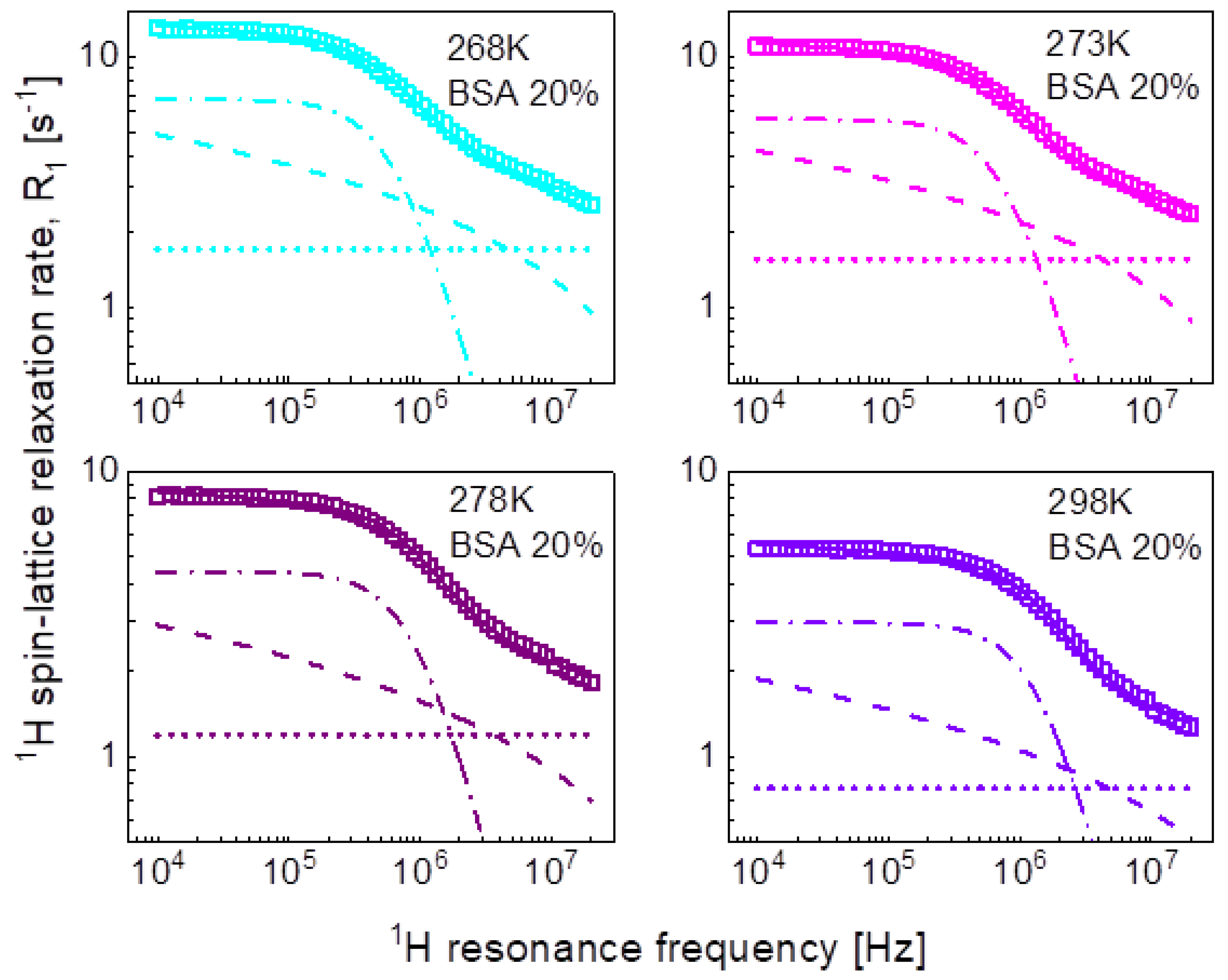
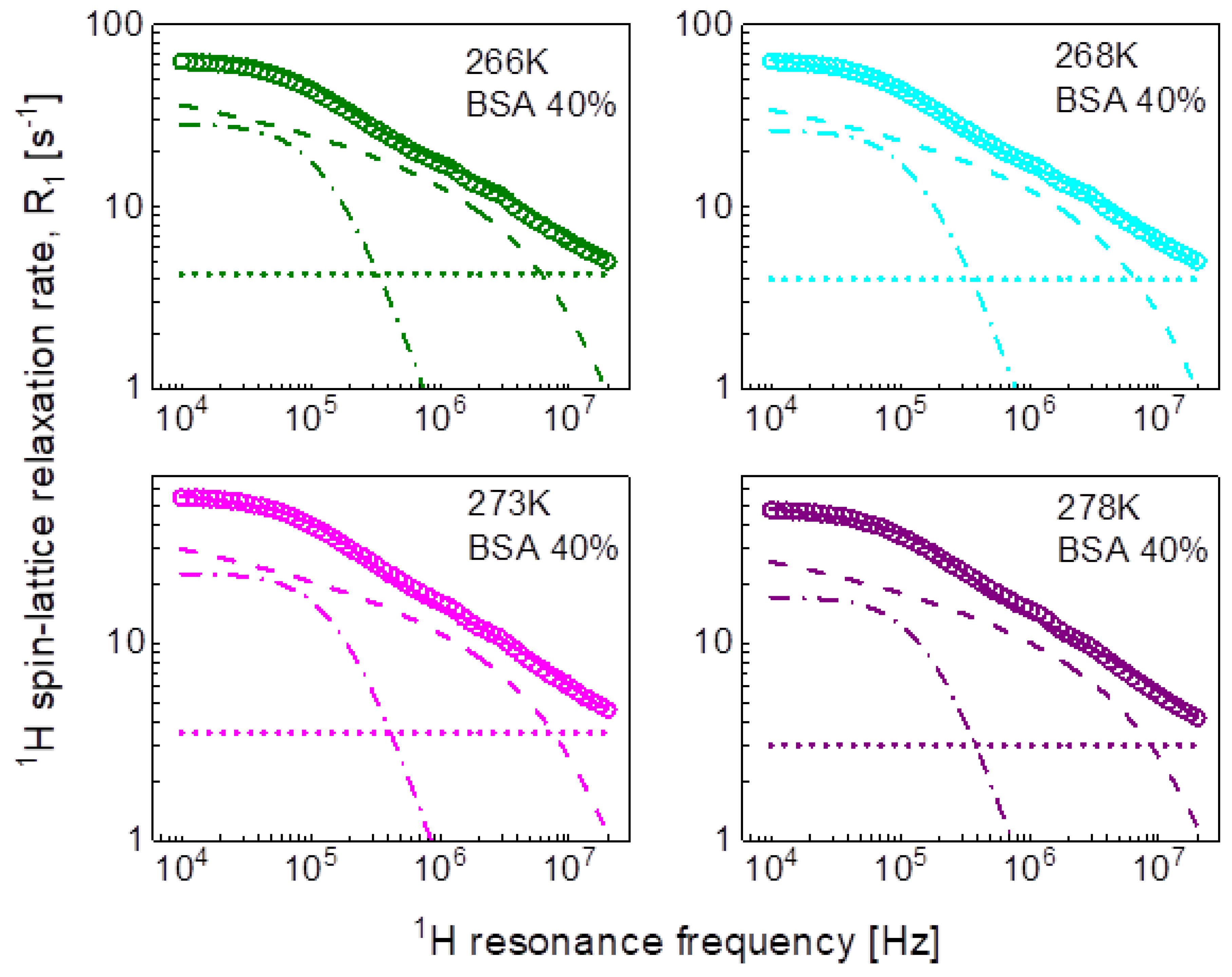
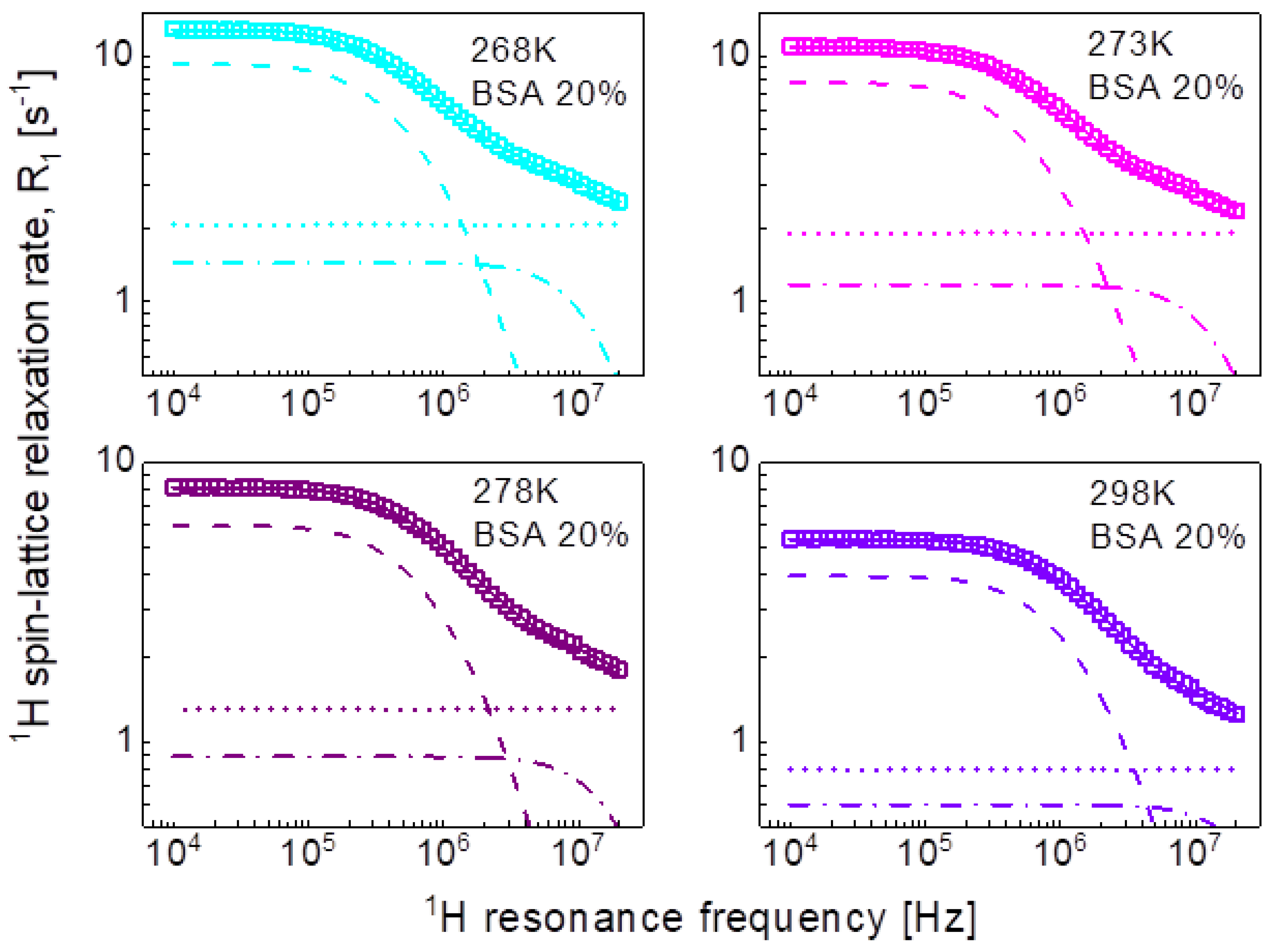
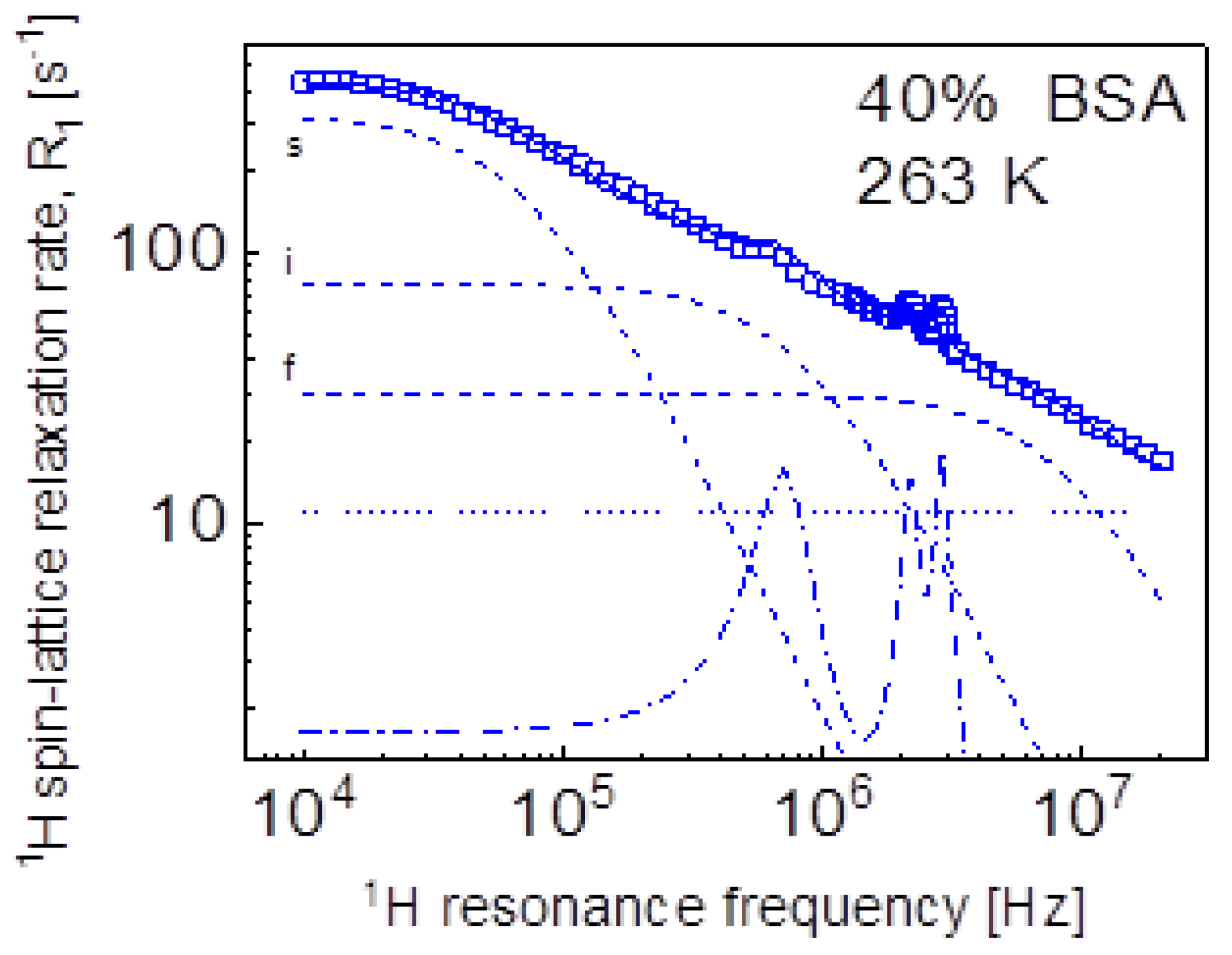
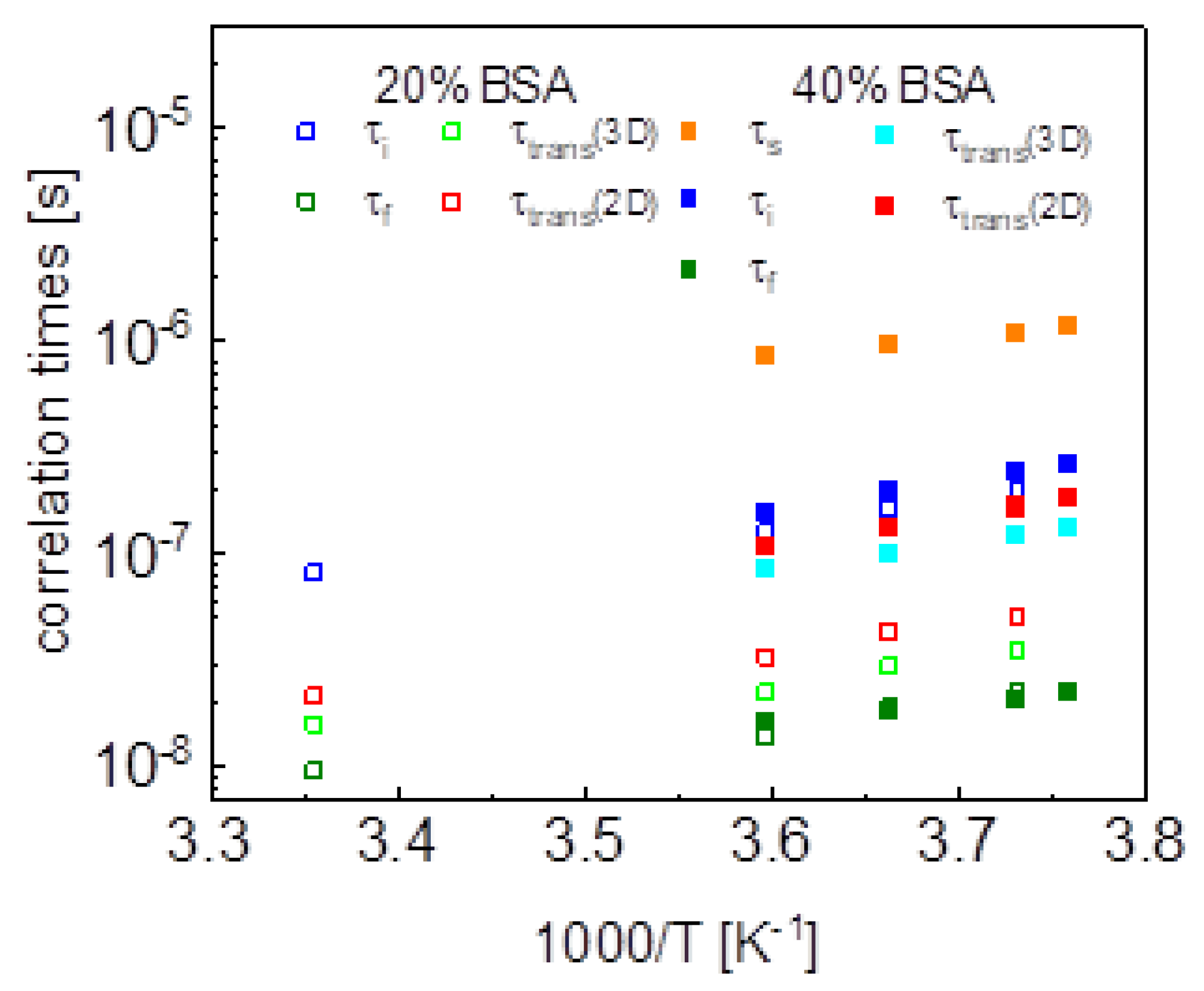
2. Results
3. Discussion
4. Materials and Methods
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A

References
- Slichter, C.P. Principles of Magnetic Resonance, 3rd ed.; Springer: Berlin, Germany, 1990. [Google Scholar]
- Kruk, D. Understanding Spin Dynamics; CRC Press–Pan Stanford Publishing: Boca Raton, FL, USA, 2015. [Google Scholar]
- Kowalewski, J.; Maler, L. Nuclear Spin Relaxation in Liquids: Theory, Experiments, and Applications, 2nd ed.; CRC Press–Taylor & Francis Group: Boca Raton, FL, USA, 2019. [Google Scholar]
- Korb, J. Multiscale nuclear magnetic relaxation dispersion of complex liquids in bulk and confinement. Prog. Nucl. Magn. Reson. Spectrosc. 2018, 104, 12–55. [Google Scholar] [CrossRef] [PubMed]
- Meier, R.; Kruk, D.; Gmeiner, J.E.; Rössler, E.A. Intermolecular relaxation in glycerol as revealed by field cycling 1H NMR relaxometry dilution experiments. J. Chem. Phys. 2012, 136, 034508. [Google Scholar] [CrossRef] [PubMed]
- Meier, R.; Kruk, D.; Bourdick, A.; Schneider, E.; Rössler, E.A. Inter- and intramolecular relaxation in molecular liquids by field cycling 1H NMR relaxometry. Appl. Magn. Reson. 2012, 44, 153–168. [Google Scholar] [CrossRef]
- Kimmich, R.; Fatkullin, N. Polymer chain dynamics and NMR. Adv. Polym. Sci. 2004, 170, 1–113. [Google Scholar]
- Goddard, Y.A.; Korb, J.-P.; Bryant, R.G. Water molecule contributions to proton spin–lattice relaxation in rotationally immobilized proteins. J. Magn. Reson. 2009, 199, 68–74. [Google Scholar] [CrossRef]
- Korb, J.-P.; Bryant, R.G. Magnetic field dependence of proton spin–lattice relaxation of confined proteins. C. R. Phys. 2004, 5, 349–357. [Google Scholar] [CrossRef]
- Goddard, Y.; Korb, J.-P.; Bryant, R.G. The magnetic field and temperature dependences of proton spin–lattice relaxation in proteins. J. Chem. Phys. 2007, 126, 175105. [Google Scholar] [CrossRef]
- Bertini, I.; Fragai, M.; Luchinat, C.; Parigi, G. 1H NMRD profiles of diamagnetic proteins: A model-freeanalysis. Magn. Reson. Chem. 2000, 38, 543–550. [Google Scholar] [CrossRef]
- Ravera, E.; Parigi, G.; Mainz, A.; Religa, T.L.; Reif, B.; Luchinat, C. Experimental determination of microsecond reorientation correlation times in protein solutions. J. Phys. Chem. B 2013, 117, 3548–3553. [Google Scholar] [CrossRef]
- Bryant, R.G. Dynamics of water in and around proteins characterized by 1H-spin-lattice relaxometry. C. R. Phys. 2010, 11, 128–135. [Google Scholar] [CrossRef]
- Diakova, G.; Goddard, Y.A.; Korb, J.-P.; Bryant, R.G. Water and backbone dynamics in a hydrated protein. Biophys. J. 2010, 98, 138–146. [Google Scholar] [CrossRef] [PubMed]
- Grebenkov, D.S.; Goddard, Y.A.; Diakova, G.; Korb, J.-P.; Bryant, R.G. Dimensionality of diffusive exploration at the protein interface in solution. J. Phys. Chem. B 2009, 113, 13347–13356. [Google Scholar] [CrossRef] [PubMed]
- Kruk, D.; Masiewicz, E.; Borkowska, A.M.; Rochowski, P.; Fries, P.H.; Broche, L.M.; Lurie, D.J. Dynamics of solid proteins by means of Nuclear Magnetic Resonance Relaxometry. Biomolecules 2019, 9, 652. [Google Scholar] [CrossRef] [PubMed]
- Kruk, D.; Masiewicz, E.; Wojciechowski, M.; Florek-Wojciechowska, M.; Broche, L.M.; Lurie, D.J. Slow dynamics of solid proteins—Nuclear magnetic resonance relaxometry versus dielectric spectroscopy. J. Magn. Reson. 2020, 314, 106721. [Google Scholar] [CrossRef] [PubMed]
- Parigi, G.; Ravera, E.; Fragai, M.; Luchinat, C. Unveiling protein dynamics in solution with field-cycling NMR relaxometry. Prog. Nucl. Magn. Reson. Spectrosc. 2021, 124–125, 85–98. [Google Scholar] [CrossRef] [PubMed]
- Luchinat, C.; Parigi, G.; Ravera, E. Water and Protein Dynamics in Sedimented Systems: A Relaxometric Investigation. ChemPhysChem 2013, 14, 3156–3161. [Google Scholar] [CrossRef] [PubMed]
- Jank, T.; Korb, J.-P.; Luksic, M.; Vlachy, V.; Bryant, R.G.; Meriguet, G.; Malikova, N.; Rollet, A.-L. Multiscale Water Dynamics on Protein Surfaces: Protein-Specific Response to Surface Ions. J. Phys. Chem. B 2021, 125, 8673–8681. [Google Scholar] [CrossRef]
- Lurie, D.J.; Aime, S.; Baroni, S.; Booth, N.A.; Broche, L.M.; Choi, C.H.; Davies, G.R.; Ismail, S.; Ó hÓgáin, D.; Pine, K.J. Fast field-cycling magnetic resonance imaging. C. R. Phys. 2010, 11, 136–148. [Google Scholar] [CrossRef]
- Masiewicz, E.; Ashcroft, G.P.; Boddie, D.; Dundas, S.R.; Kruk, D.; Broche, L.M. Towards applying NMR relaxometry as a diagnostic tool for bone and soft tissue sarcomas: A pilot study. Sci. Rep. 2020, 10, 14207. [Google Scholar] [CrossRef]
- Kruk, D.; Meier, R.; Rachocki, A.; Korpała, A.; Singh, R.K.; Rössler, E.A. Determining diffusion coefficients of ionic liquids by means of field cycling nuclear magnetic resonance relaxometry. J. Chem. Phys. 2014, 140, 244509. [Google Scholar] [CrossRef]
- Ordikhani, A.; Stapf, S.; Mattea, C. Nuclear magnetic relaxation and diffusion study of the ionic liquids 1-ethyl- and 1-butyl-3-methylimidazolium bis(trifluoromethylsulfonyl)imide confined in porous glass. Magn. Reson. Chem. 2019, 57, 818–828. [Google Scholar] [CrossRef] [PubMed]
- Kruk, D.; Wojciechowski, M.; Verma, Y.L.; Chaurasia, S.K.; Singh, R.K. Dynamical properties of EMIM-SCN confined in a SiO2 matrix by means of 1H NMR relaxometry. Phys. Chem. Chem. Phys. 2017, 19, 32605–32616. [Google Scholar] [CrossRef] [PubMed]
- Kruk, D.; Wojciechowski, M.; Brym, S.; Singh, R.K. Dynamics of ionic liquids in bulk and in confinement by means of 1H NMR relaxometry–BMIM-OcSO4 in an SiO2 matrix as an example. Phys. Chem. Chem. Phys. 2016, 18, 23184–23194. [Google Scholar] [CrossRef] [PubMed]
- Seyedlar, A.O.; Stapf, S.; Mattea, C. Dynamics of the ionic liquid 1-butyl-3-methylimidazolium bis(trifluoromethylsulphonyl)imide studied by nuclear magnetic resonance dispersion and diffusion. Phys. Chem. Chem. Phys. 2014, 17, 1653–1659. [Google Scholar] [CrossRef]
- Wencka, M.; Apih, T.; Korošec, R.C.; Jenczyk, J.; Jarek, M.; Szutkowski, K.; Jurga, S.; Dolinšek, J. Molecular dynamics of 1-ethyl-3- methylimidazolium triflate ionic liquid studied by 1H and 19F nuclear magnetic resonances. Phys. Chem. Chem. Phys. 2017, 19, 15368–15376. [Google Scholar] [CrossRef]
- Kruk, D.; Wojciechowski, M.; Florek-Wojciechowska, M.; Singh, R.K. Dynamics of ionic liquids in confinement by means of NMR relaxometry—EMIM-FSI in a silica matrix as an example. Materials 2020, 13, 4351. [Google Scholar] [CrossRef]
- Pilar, K.; Rua, A.; Suarez, S.N.; Mallia, C.; Lai, S.; Jayakody, J.R.P.; Hatcher, J.L.; Wishart, J.F.; Greenbaum, S. Investigation of dynamics in BMIM TFSA ionic liquid through variable temperature and pressure NMR relaxometry and diffusometry. J. Electrochem. Soc. 2017, 164, H5189–H5196. [Google Scholar] [CrossRef]
- Hwang, L.; Freed, J.H. Dynamic effects of pair correlation functions on spin relaxation by translational diffusion in liquids. J. Chem. Phys. 1975, 63, 4017–4025. [Google Scholar] [CrossRef]
- Fries, P.H.; Belorizky, E. Time correlation functions of isotropic intermolecular site-site interactions in liquids: Effects of the site eccentricity and of the molecular distribution. J. Phys. 1989, 50, 3347–3363. [Google Scholar] [CrossRef]
- Kruk, D.; Meier, R.; Rössler, E.A. Nuclear magnetic resonance relaxometry as a method of measuring translational diffusion coefficients in liquids. Phys. Rev. E. Stat. Nonlin. Soft Matter Phys. 2012, 85, 020201. [Google Scholar] [CrossRef]
- Fries, P.H. Dipolar nuclear spin relaxation in liquids and plane fluids undergoing chemical reactions. Mol. Phys. 2006, 48, 503–526. [Google Scholar] [CrossRef]
- Kruk, D.; Rochowski, P.; Masiewicz, E.; Wilczynski, S.; Wojciechowski, M.; Broche, L.M.; Lurie, D.J. Mechanism of water dynamics in hyaluronic dermal fillers revealed by nuclear magnetic resonance relaxometry. ChemPhysChem 2019, 20, 2816–2822. [Google Scholar] [CrossRef] [PubMed]
- Winter, F.; Kimmich, R. 14N1H and 2H1H cross-relaxation in hydrated proteins. Biophys. J. 1985, 48, 331–335. [Google Scholar] [CrossRef] [PubMed]
- Westlund, P.-O. Quadrupole-enhanced proton spin relaxation for a slow reorienting spin pair: (I)–(S). A stochastic Liouville approach. Mol. Phys. 2009, 107, 2141–2148. [Google Scholar] [CrossRef]
- Westlund, P.-O. The quadrupole enhanced 1H spin–lattice relaxation of the amide proton in slow tumbling proteins. Phys. Chem. Chem. Phys. 2010, 12, 3136–3140. [Google Scholar] [CrossRef] [PubMed]
- Sunde, E.P.; Halle, B. Mechanism of 1H–14N cross-relaxation in immobilized proteins. J. Magn. Reson. 2010, 203, 257–273. [Google Scholar] [CrossRef]
- Kruk, D.; Privalov, A.; Medycki, W.; Uniszkiewicz, C.; Masierak, W.; Jakubas, R. NMR studies of solid-state dynamics. Annu. Rep. NMR Spectrosc. 2012, 76, 67–138. [Google Scholar]
- Kruk, D.; Umut, E.; Masiewicz, E.; Fischer, R.; Scharfetter, H. Multi-quantum quadrupole relaxation enhancement effects in 209Bi compounds. J. Chem. Phys. 2019, 150, 184309. [Google Scholar] [CrossRef]
- Kruk, D.; Umut, E.; Masiewicz, E.; Sampl, C.; Fischer, R.; Spirk, S.; Gösweiner, C.; Scharfetter, H. 209Bi quadrupole relaxation enhancement in solids as a step towards new contrast mechanisms in magnetic resonance imaging. Phys. Chem. Chem. Phys. 2018, 20, 12710–12718. [Google Scholar] [CrossRef]
- Fries, P.H.; Belorizky, E. Simple expressions of the nuclear relaxation rate enhancement due to quadrupole nuclei in slowly tumbling molecules. J. Chem. Phys. 2015, 143, 044202. [Google Scholar] [CrossRef]
- Kowalewski, J.; Egorov, A.; Kruk, D.; Laaksonen, A.; Nikkhou Aski, S.; Parigi, G.; Westlund, P.-O. Extensive NMRD studies of Ni(II) salt solutions in water and water–glycerol mixtures. J. Magn. Reson. 2008, 195, 103–111. [Google Scholar] [CrossRef] [PubMed]
- Ader, C.; Schneider, R.; Seidel, K.; Etzkorn, M.; Becker, S.; Baldus, M. Structural Rearrangements of Membrane Proteins Probed by Water-Edited Solid-State NMR Spectroscopy. J. Am. Chem. Soc. 2009, 131, 170–176. [Google Scholar] [CrossRef] [PubMed]
- Wang, T.; Hyunil, J.; DeGrado, W.F.; Hong, M. Water distribution, dynamics, and interactions with Alzheimer’s β-amyloid fibrils investigated by solid-state NMR. J. Am. Chem. Soc. 2017, 139, 6242–6252. [Google Scholar] [CrossRef] [PubMed]
- Hediger, S.; Emsley, L.; Fischer, M. Solid-state NMR characterization of hydration effects on polymer mobility in onion cell-wall material. Carbohydr. Res. 1999, 322, 102–112. [Google Scholar] [CrossRef]
- White, P.B.; Wang, T.; Park, Y.B.; Cosgrove, D.; Hong, M. Water–Polysaccharide Interactions in the Primary Cell Wall of Arabidopsis thaliana from Polarization Transfer Solid-State NMR. J. Am. Chem. Soc. 2014, 136, 10399–10409. [Google Scholar] [CrossRef]

| 20%wt of BSA | ||||
| Temp. [K] | [s] | [s] | [s−1] | |
| 268 | 1.97 × 10−7 | 2.28 × 10−8 | 2.52 | |
| 273 | 1.65 × 10−7 | 1.91 × 10−8 | 2.25 | |
| 278 | 1.27 × 10−7 | 1.39 × 10−8 | 1.62 | |
| 298 | 8.33 × 10−8 | 9.62 × 10−9 | 0.98 | |
| 40%wt of BSA | ||||
| Temp. [K] | [s] | [s] | [s] | [s−1] |
| 266 | 1.16 × 10−6 | 2.66 × 10−7 | 2.24 × 10−8 | 4.68 |
| 268 | 1.10 × 10−6 | 2.41 × 10−7 | 2.09 × 10−8 | 4.50 |
| 273 | 9.67 × 10−7 | 1.95 × 10−7 | 1.84 × 10−8 | 3.91 |
| 278 | 8.53 × 10−7 | 1.56 × 10−7 | 1.59 × 10−8 | 3.40 |
| Temp. [K] | [s] | [m2/s] | [s−1] | [s] |
|---|---|---|---|---|
| 20%wt of BSA | ||||
| 268 | 1.85 × 10−7 | 2.08 × 10−12 | 1.98 | 3.50 × 10−8 |
| 273 | 1.54 × 10−7 | 2.44 × 10−12 | 1.79 | 2.98 × 10−8 |
| 278 | 1.18 × 10−7 | 3.28 × 10−12 | 1.25 | 2.22 × 10−8 |
| 298 | 7.60 × 10−8 | 4.56 × 10−12 | 0.69 | 1.60 × 10−8 |
| 40%wt of BSA | ||||
| 266 | 9.08 × 10−7 | 5.56 × 10−13 | 5.00 | 1.31 × 10−7 |
| 268 | 8.47 × 10−7 | 6.10 × 10−13 | 4.84 | 1.20 × 10−7 |
| 273 | 7.32 × 10−7 | 7.23 × 10−13 | 4.31 | 1.01 × 10−7 |
| 278 | 6.35 × 10−7 | 8.68 × 10−13 | 3.82 | 8.40 × 10−8 |
| Temp. [K] | [s] | [s] | [s−1] | [m2/s] |
|---|---|---|---|---|
| 20%wt of BSA | ||||
| 268 | 1.37 × 10−7 | 7.26 × 10−10 | 1.70 | 5.02 × 10−11 |
| 273 | 1.14 × 10−7 | 6.15 × 10−10 | 1.55 | 5.93 × 10−11 |
| 278 | 9.05 × 10−8 | 4.05 × 10−10 | 1.19 | 9.00 × 10−11 |
| 298 | 5.99 × 10−8 | 2.50 × 10−10 | 0.77 | 1.46 × 10−10 |
| 40%wt of BSA | ||||
| 266 | 6.92 × 10−7 | 7.14 × 10−9 | 4.32 | 5.11 × 10−12 |
| 268 | 6.39 × 10−7 | 6.64 × 10−9 | 4.01 | 5.49 × 10−12 |
| 273 | 5.42 × 10−7 | 5.67 × 10−9 | 3.50 | 6.43 × 10−12 |
| 278 | 4.63 × 10−7 | 4.79 × 10−9 | 3.03 | 7.61 × 10−12 |
| Temp. [K] | [s] | [s] | [s] | [s−1] | [m2/s] |
|---|---|---|---|---|---|
| 20%wt of BSA | |||||
| 268 | 6.70 × 10−9 | 5.17 × 10−8 | 4.81 × 10−7 | 2.06 | 7.05 × 10−13 |
| 273 | 5.46 × 10−9 | 4.33 × 10−8 | 4.07 × 10−7 | 1.90 | 8.42 × 10−13 |
| 278 | 4.13 × 10−9 | 3.28 × 10−8 | 3.12 × 10−7 | 1.31 | 1.11 × 10−12 |
| 298 | 2.78 × 10−9 | 2.14 × 10−8 | 2.09 × 10−7 | 0.80 | 1.70 × 10−12 |
| 40%wt of BSA | |||||
| 266 | 2.38 × 10−8 | 1.83 × 10−7 | 2.71 × 10−6 | 5.06 | 1.99 × 10−13 |
| 268 | 2.24 × 10−8 | 1.65 × 10−7 | 2.66 × 10−6 | 4.70 | 2.21 × 10−13 |
| 273 | 1.91 × 10−8 | 1.33 × 10−7 | 2.60 × 10−6 | 4.20 | 2.74 × 10−13 |
| 278 | 1.66 × 10−8 | 1.07 × 10−7 | 2.60 × 10−6 | 3.51 | 3.41 × 10−13 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kruk, D.; Kasparek, A.; Masiewicz, E.; Kolodziejski, K.; Cybulski, R.; Nowak, B. Water Dynamics in Highly Concentrated Protein Systems—Insight from Nuclear Magnetic Resonance Relaxometry. Int. J. Mol. Sci. 2023, 24, 4093. https://doi.org/10.3390/ijms24044093
Kruk D, Kasparek A, Masiewicz E, Kolodziejski K, Cybulski R, Nowak B. Water Dynamics in Highly Concentrated Protein Systems—Insight from Nuclear Magnetic Resonance Relaxometry. International Journal of Molecular Sciences. 2023; 24(4):4093. https://doi.org/10.3390/ijms24044093
Chicago/Turabian StyleKruk, Danuta, Adam Kasparek, Elzbieta Masiewicz, Karol Kolodziejski, Radoslaw Cybulski, and Bartosz Nowak. 2023. "Water Dynamics in Highly Concentrated Protein Systems—Insight from Nuclear Magnetic Resonance Relaxometry" International Journal of Molecular Sciences 24, no. 4: 4093. https://doi.org/10.3390/ijms24044093
APA StyleKruk, D., Kasparek, A., Masiewicz, E., Kolodziejski, K., Cybulski, R., & Nowak, B. (2023). Water Dynamics in Highly Concentrated Protein Systems—Insight from Nuclear Magnetic Resonance Relaxometry. International Journal of Molecular Sciences, 24(4), 4093. https://doi.org/10.3390/ijms24044093






