Structures and Dynamics of Complex Guest Molecules in Confinement, Revealed by Solid-State NMR, Molecular Dynamics, and Calorimetry
Abstract
1. Introduction
2. Materials and Methods
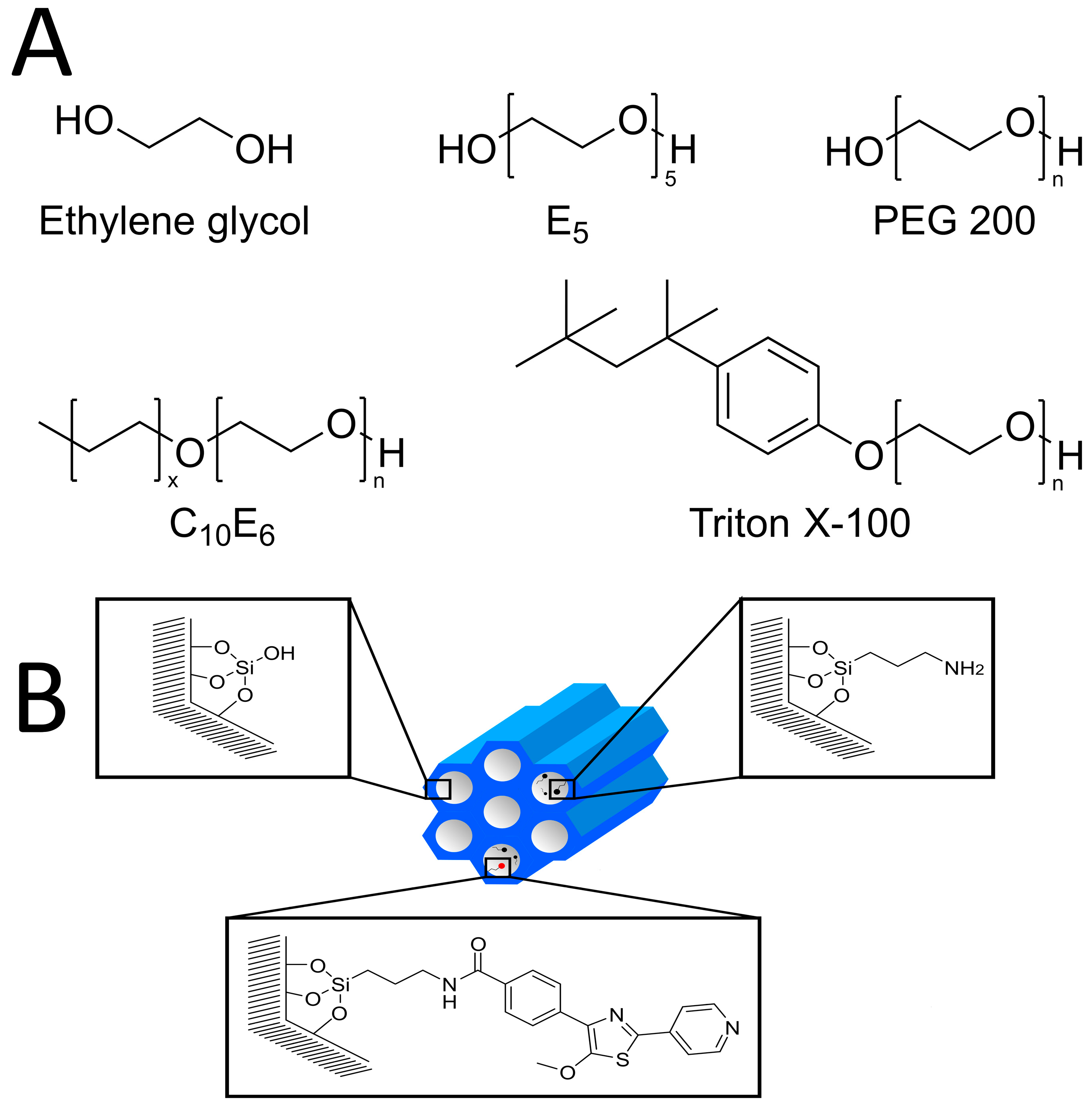
2.1. Host-Materials
2.2. Probe Molecules
2.2.1. 1-Octanol
2.2.2. Ethylene Glycol, Pentaethylene Glycol, and Polyethylene Glycol (PEG)
2.3. Simulation Methods
2.4. Differential Scanning Calorymetry (DSC)
3. Exemplary Studies
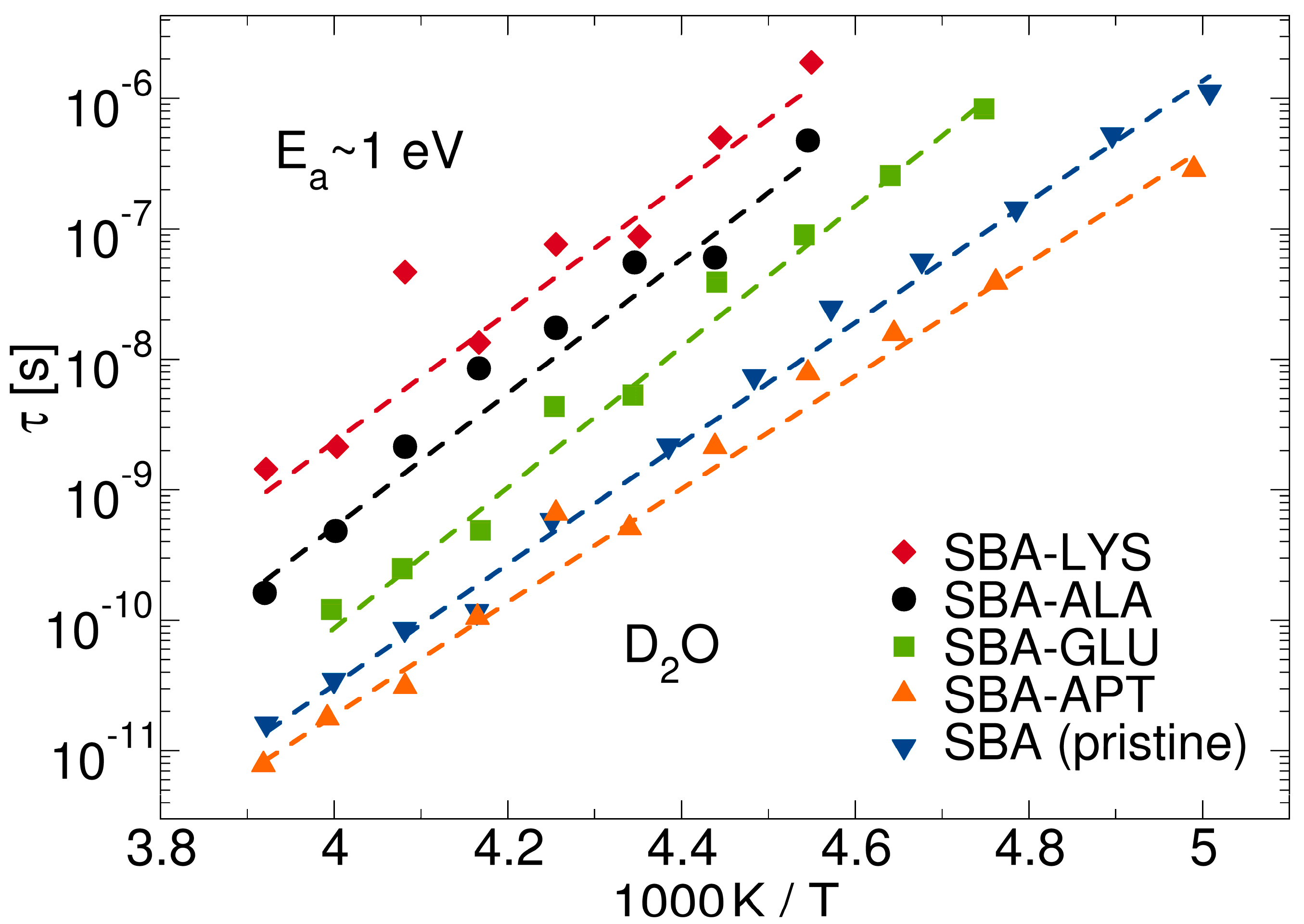
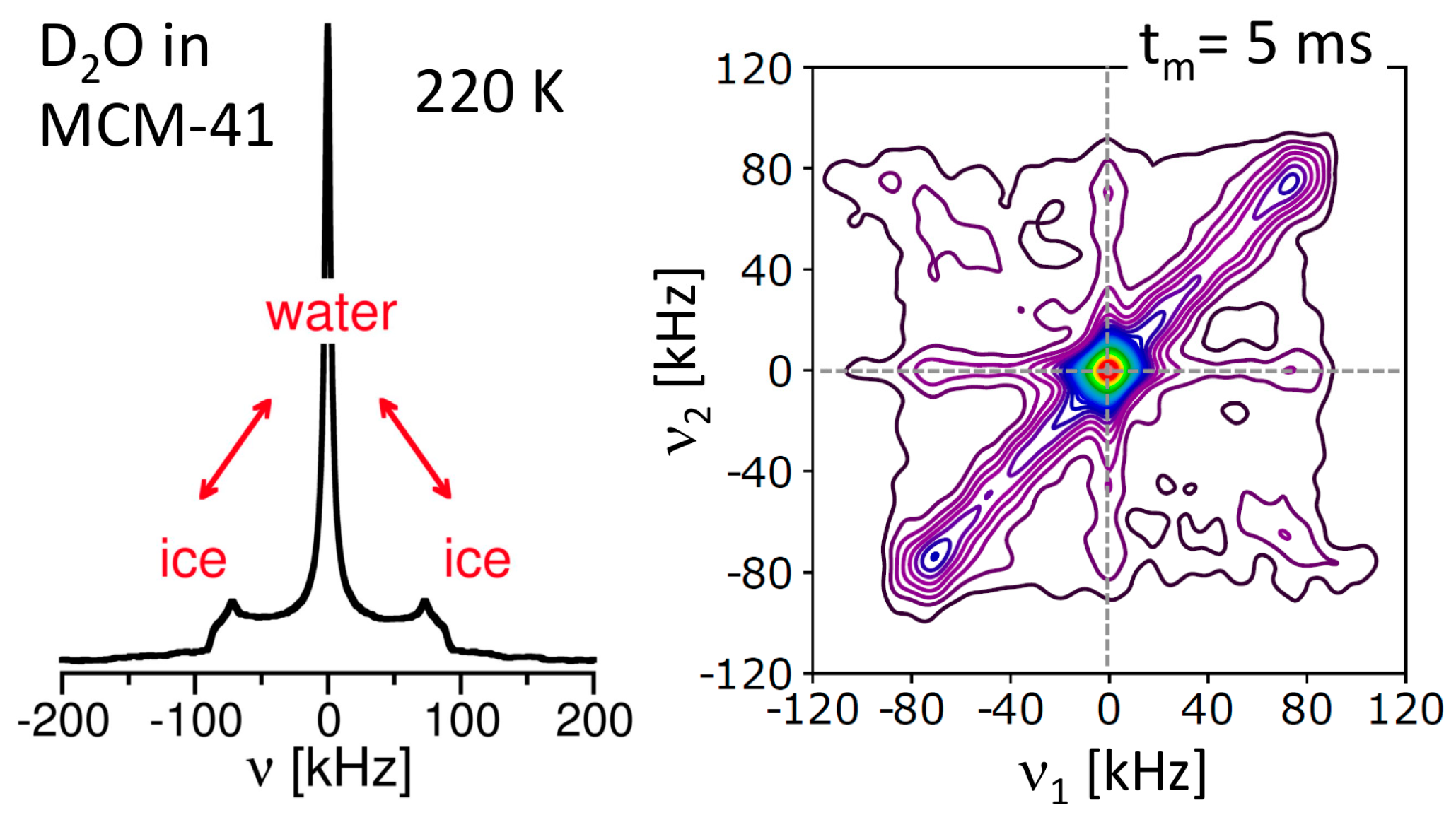
3.1. Water and Ion Dynamics
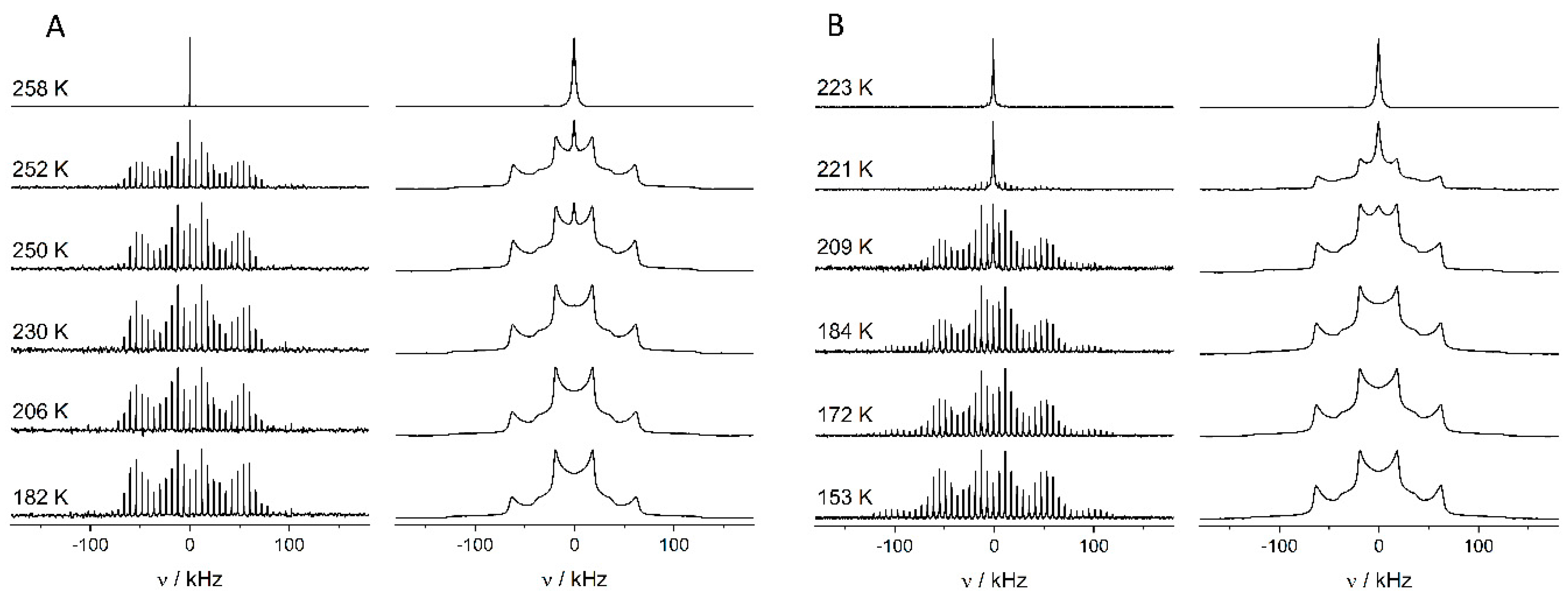
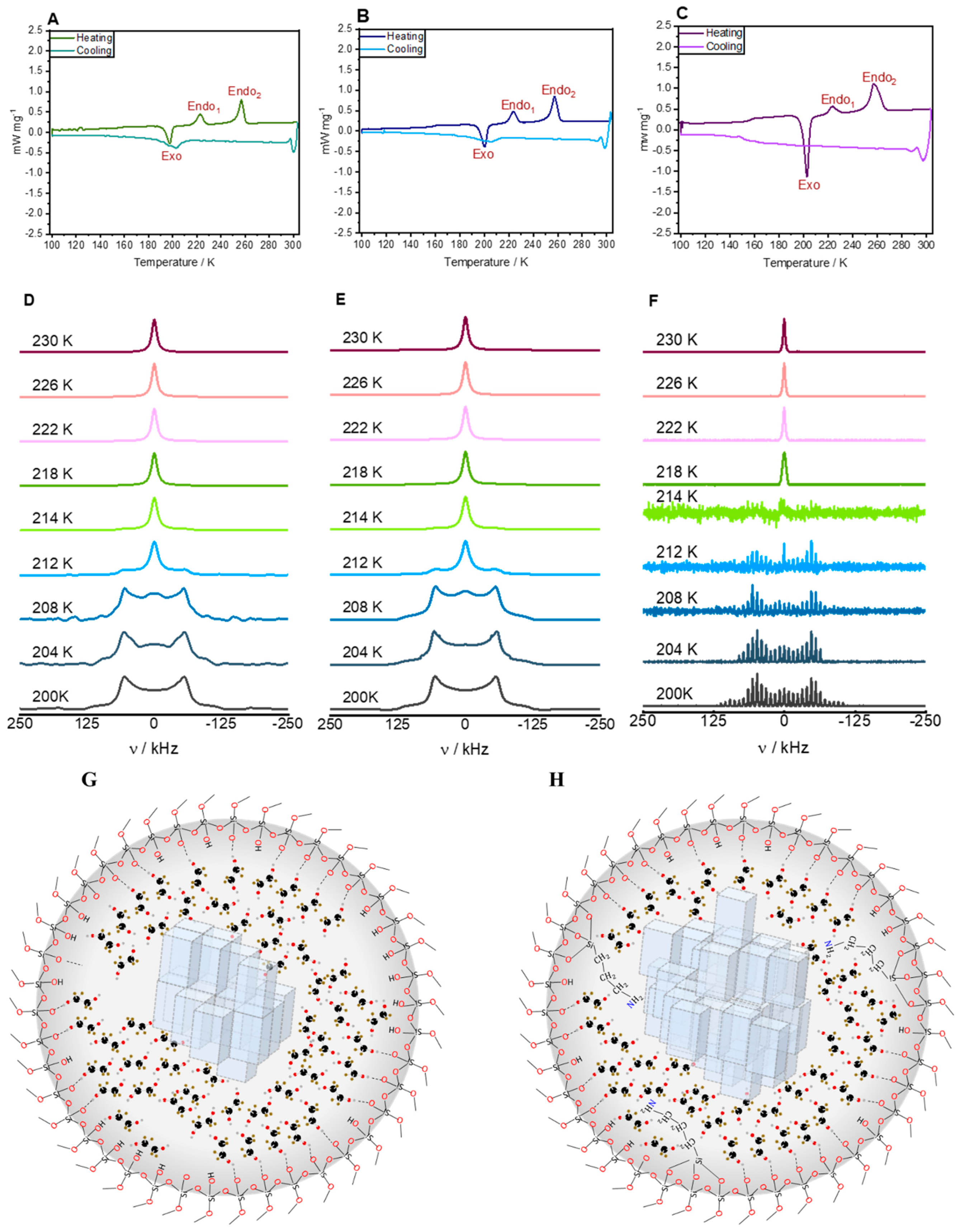
3.2. Octanol
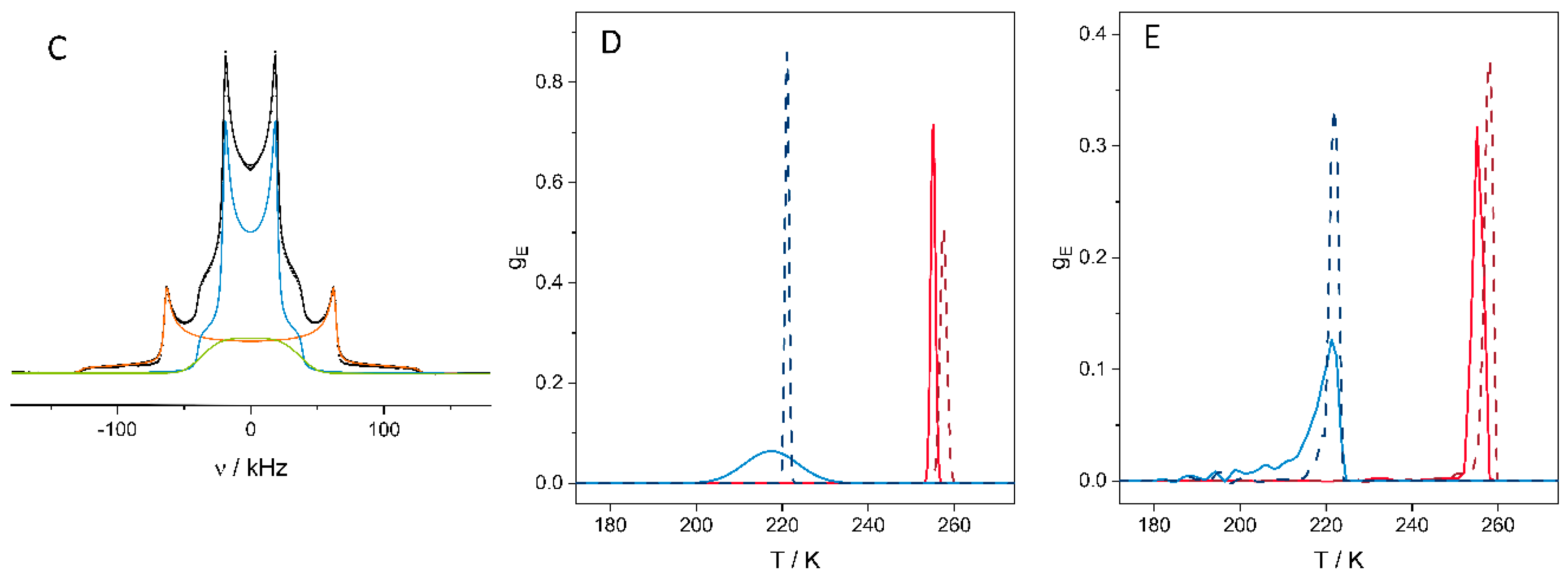
3.3. Ethylene Glycol
3.4. Polyethylene Glycol and Related Surfactants
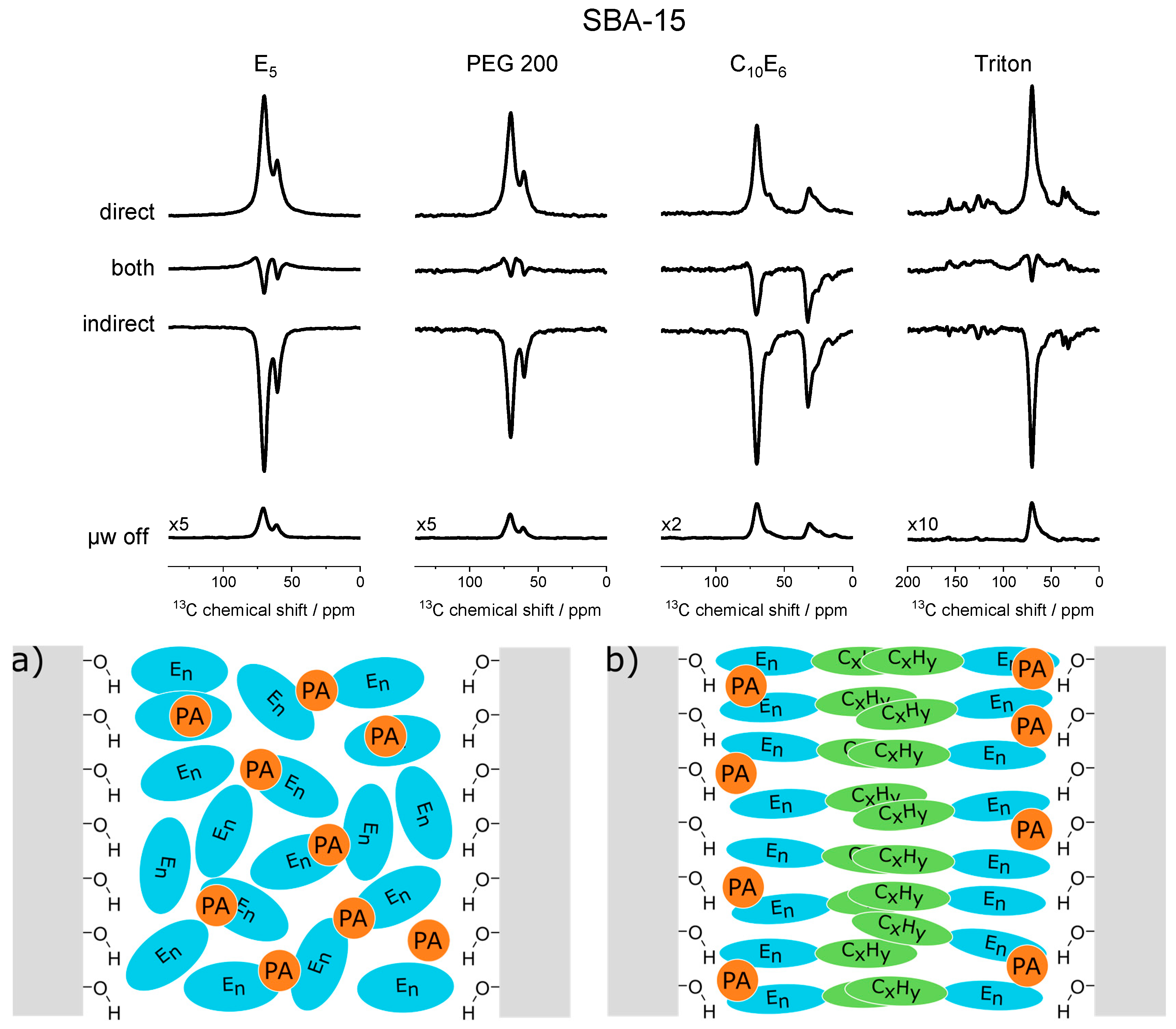
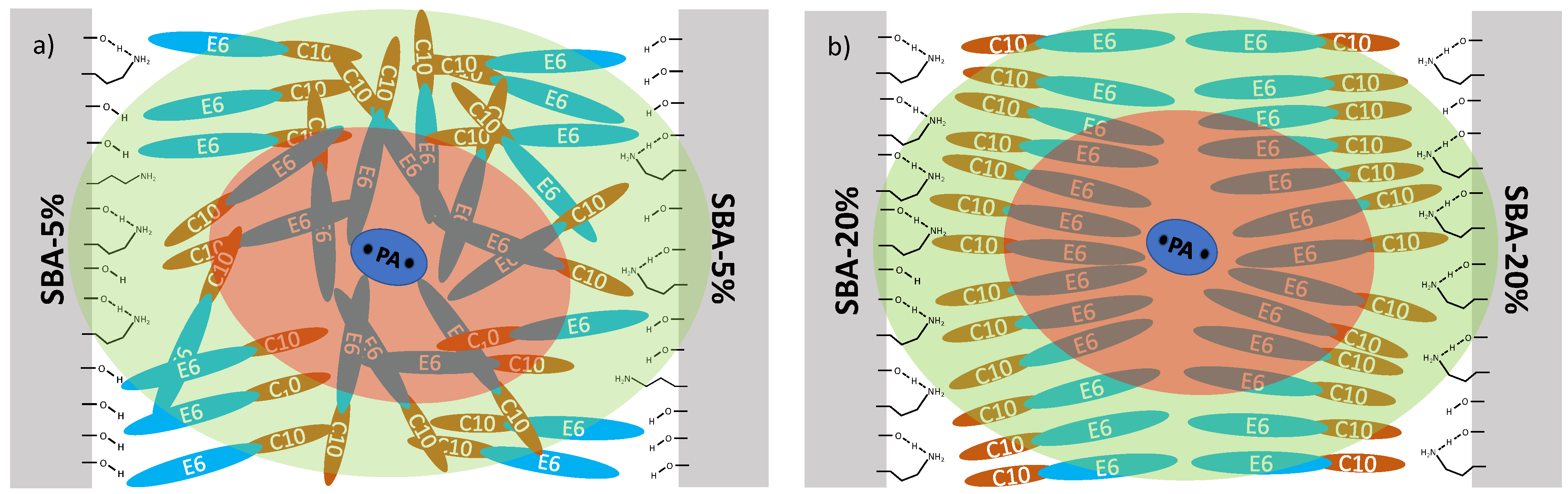
3.4.1. Experimental Studies
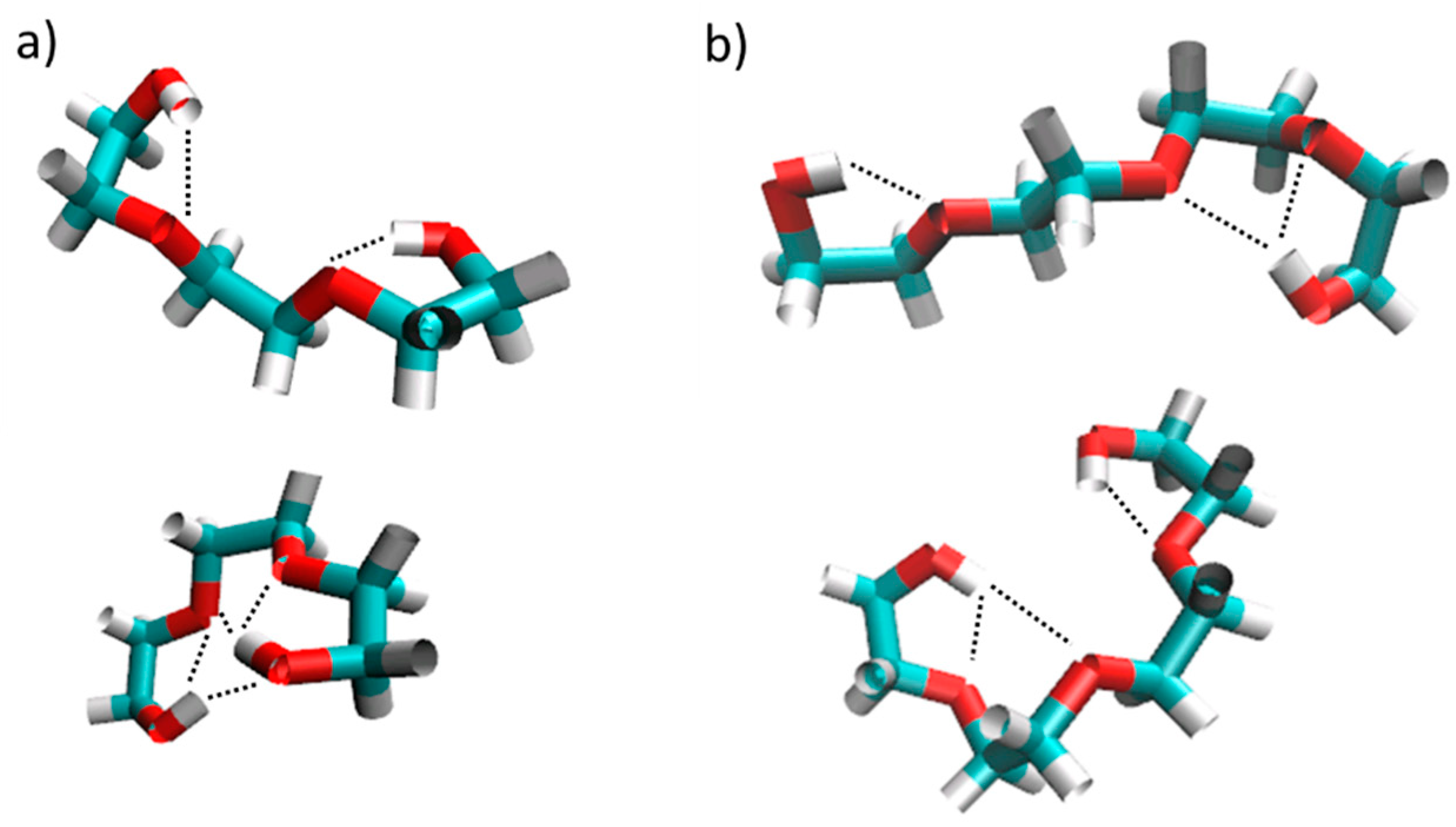
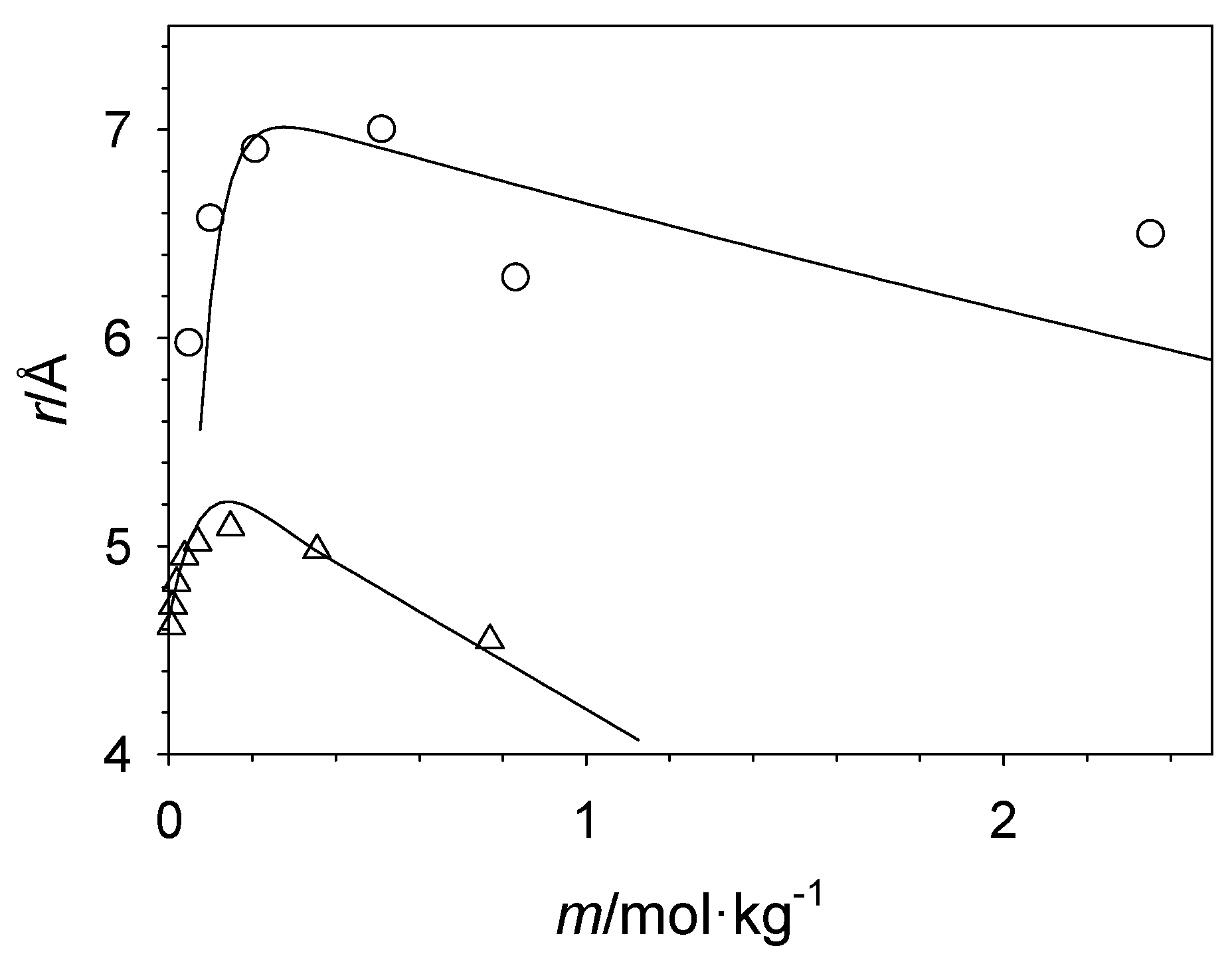
3.4.2. Molecular Dynamics Simulations of PEG
4. Summary and Outlook
Funding
Conflicts of Interest
References
- Le Page, M.; Beau, R.; Duchene, J. Porous Silica Particles Containing a Crystallized Phase and Method. U.S. Patent 3,493,341, 3 February 1970. [Google Scholar]
- Chiola, V.; Ritsko, J.E.; Vanderpool, C.D. Process for Producing Low-Bulk DENSITY Silica. U.S. Patent 3,556,725, 19 January 1971. [Google Scholar]
- Beck, J.S.; Vartuli, J.C.; Roth, W.J.; Leonowicz, M.E.; Kresge, C.T.; Schmitt, K.D.; Chu, C.T.; Olson, D.H.; Sheppard, E.W. A new family of mesoporous molecular sieves prepared with liquid crystal templates. J. Am. Chem. Soc. 1992, 114, 10834–10843. [Google Scholar] [CrossRef]
- Zhao, D.Y.; Huo, Q.S.; Feng, J.L.; Chmelka, B.F.; Stucky, G.D. Nonionic triblock and star diblock copolymer and oligomeric surfactant syntheses of highly ordered, hydrothermally stable, mesoporous silica structures. J. Am. Chem. Soc. 1998, 120, 6024–6036. [Google Scholar] [CrossRef]
- Zhao, D.Y.; Feng, J.L.; Huo, Q.S.; Melosh, N.; Fredrickson, G.H.; Chmelka, B.F.; Stucky, G.D. Triblock copolymer syntheses of mesoporous silica with periodic 50 to 300 angstrom pores. Science 1998, 279, 548–552. [Google Scholar] [CrossRef] [PubMed]
- Vinu, A.; Miyahara, M.; Ariga, K. Assemblies of biomaterials in mesoporous media. J. Nanosci. Nanotechnol. 2006, 6, 1510–1532. [Google Scholar] [CrossRef] [PubMed]
- Vinu, A.; Hossain, K.Z.; Ariga, K. Recent advances in functionalization of mesoporous silica. J. Nanosci. Nanotechnol. 2005, 5, 347–371. [Google Scholar] [CrossRef] [PubMed]
- Yokoi, T.; Yoshitake, H.; Tatsumi, T. Synthesis of amino-functionalized MCM-41 via direct co-condensation and post-synthesis grafting methods using mono-, di- and tri-amino-organoalkoxysilanes. J. Mater. Chem. 2004, 14, 951–957. [Google Scholar] [CrossRef]
- Wang, X.; Lin, K.S.K.; Chan, J.C.C.; Cheng, S. Direct Synthesis and Catalytic Applications of Ordered Large Pore Aminopropyl-Functionalized SBA-15 Mesoporous Materials. J. Phys. Chem. B 2005, 109, 1763–1769. [Google Scholar] [CrossRef] [PubMed]
- Saravanan, M.; Sudalai, S.; Dharaneesh, A.B.; Prahaaladhan, V.; Srinivasan, G.; Arumugam, A. An extensive review on mesoporous silica from inexpensive resources: Properties, synthesis, and application toward modern technologies. J. Sol-Gel Sci. Technol. 2023, 105, 1–29. [Google Scholar] [CrossRef]
- Matlahov, I.; Geiger, Y.; Goobes, G. Trapping RNase A on MCM41 pores: Effects on structure stability, product inhibition and overall enzymatic activity. Phys. Chem. Chem. Phys. 2014, 16, 9031–9038. [Google Scholar] [CrossRef] [PubMed]
- Shimon, D.; Chen, C.-H.; Lee, J.J.; Didas, S.A.; Sievers, C.; Jones, C.W.; Hayes, S.E. 15N Solid State NMR Spectroscopic Study of Surface Amine Groups for Carbon Capture: 3-Aminopropylsilyl Grafted to SBA-15 Mesoporous Silica. Environ. Sci. Technol. 2018, 52, 1488–1495. [Google Scholar] [CrossRef]
- Ravera, E.; Martelli, T.; Geiger, Y.; Fragai, M.; Goobes, G.; Luchinat, C. Biosilica and bioinspired silica studied by solid-state NMR. Coordin. Chem. Rev. 2016, 327–328, 110–122. [Google Scholar] [CrossRef]
- Treacy, M.M.J.; Higgins, J.B.; von Ballmoos, R. Collection of Simulated XRD Powder Patterns for Zeolites; Elsevier: London, UK, 1996. [Google Scholar]
- Marler, B.; Oberhagemann, U.; Vortmann, S.; Gies, H. Influence of the sorbate type on the XRD peak intensities of loaded MCM-41. Microporous Mater. 1996, 6, 375–383. [Google Scholar] [CrossRef]
- Yao, M.H.; Baird, R.J.; Kunz, F.W.; Hoost, T.E. An XRD and TEM investigation of the structure of alumina-supported ceria–zirconia. J. Catal. 1997, 166, 67–74. [Google Scholar] [CrossRef]
- Hoffmann, I.; Malayil Kalathil, F.; Lopian, T.; Touraud, D.; Czakkel, O.; Plazanet, M.; Alba-Simionesco, C. Unexpected molecular dynamics of ethanol in hydrogen-bonded binary mixtures, ethanol-octanol and ethanol-water. EPJ Web Conf. 2022, 272, 1003. [Google Scholar] [CrossRef]
- Höhne, G.; Hemminger, W.F.; Flammersheim, H.-J. Differential Scanning Calorimetry; Springer: Berlin/Heidelberg, Germany, 2003; ISBN 354000467X. [Google Scholar]
- Brunauer, S.; Emmett, P.H.; Teller, E. Adsorption of Gases in Multimolecular Layers. J. Am. Chem. Soc. 1938, 60, 309–319. [Google Scholar] [CrossRef]
- Barrett, E.P.; Joyner, L.G.; Halenda, P.P. The Determination of Pore Volume and Area Distributions in Porous Substances. I. Computations from Nitrogen Isotherms. J. Am. Chem. Soc. 1951, 73, 373–380. [Google Scholar] [CrossRef]
- Shenderovich, I.G.; Mauder, D.; Akcakayiran, D.; Buntkowsky, G.; Limbach, H.-H.; Findenegg, G.H. NMR provides checklist of generic properties for atomic-scale models of periodic mesoporous silicas. J. Phys. Chem. B 2007, 111, 12088–12096. [Google Scholar] [CrossRef]
- Freude, D.; Kärger, J. NMR Techniques. In Handbook of Porous Solids; Schüth, F., Sing, K.S.W., Weitkamp, J., Eds.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2002. [Google Scholar] [CrossRef]
- Kärger, J. Transport Phenomena in Nanoporous Materials. ChemPhysChem 2015, 16, 24–51. [Google Scholar] [CrossRef]
- Shenderovich, I.G. For Whom a Puddle Is the Sea? Adsorption of Organic Guests on Hydrated MCM-41 Silica. Langmuir 2020, 36, 11383–11392. [Google Scholar] [CrossRef]
- Lesnichin, S.B.; Kamdem, N.; Mauder, D.; Denisov, G.S.; Shenderovich, I.G. Studies of adsorption of 2,2′-bipyridyl on the surface of highly regulated silica matrix of the MCM-41 type by means of N-15 NMR spectroscopy. Russ. J. Gen. Chem. 2010, 80, 2027–2031. [Google Scholar] [CrossRef]
- Overhauser, A.W. Polarization of Nuclei in Metals. Phys. Rev. 1953, 92, 411–415. [Google Scholar] [CrossRef]
- Wind, R.A.; Duijvestijn, M.J.; Vanderlugt, C.; Manenschijn, A.; Vriend, J. Applications of dynamic nuclear-polarization in C-13 NMR in solids. Prog. NMR Spec. 1985, 17, 33–67. [Google Scholar] [CrossRef]
- Maly, T.; Debelouchina, G.T.; Bajaj, V.S.; Hu, K.N.; Joo, C.G.; Mak-Jurkauskas, M.L.; Sirigiri, J.R.; van der Wel, P.C.A.; Herzfeld, J.; Temkin, R.J.; et al. Dynamic nuclear polarization at high magnetic fields. J. Chem. Phys. 2008, 128, 52211. [Google Scholar] [CrossRef] [PubMed]
- Thankamony, A.S.L.; Wittmann, J.J.; Kaushik, M.; Corzilius, B. Dynamic nuclear polarization for sensitivity enhancement in modern solid-state NMR. Prog. NMR Spec. 2017, 102–103, 120–195. [Google Scholar] [CrossRef]
- Lesage, A.; Lelli, M.; Gajan, D.; Caporini, M.A.; Vitzthum, V.; Mieville, P.; Alauzun, J.; Roussey, A.; Thieuleux, C.; Mehdi, A.; et al. Surface Enhanced NMR Spectroscopy by Dynamic Nuclear Polarization. J. Am. Chem. Soc. 2010, 132, 15459–15461. [Google Scholar] [CrossRef] [PubMed]
- de Oliveira, M.; Herr, K.; Brodrecht, M.; Haro-Mares, N.B.; Wissel, T.; Klimavicius, V.; Breitzke, H.; Gutmann, T.; Buntkowsky, G. Solvent-free dynamic nuclear polarization enhancements in organically modified mesoporous silica. Phys. Chem. Chem. Phys. 2021, 23, 12559–12568. [Google Scholar] [CrossRef] [PubMed]
- Kobayashi, T.; Singappuli-Arachchige, D.; Wang, Z.R.; Slowing, I.I.; Pruski, M. Spatial distribution of organic functional groups supported on mesoporous silica nanoparticles: A study by conventional and DNP-enhanced Si-29 solid-state NMR. Phys. Chem. Chem. Phys. 2017, 19, 1781–1789. [Google Scholar] [CrossRef] [PubMed]
- Kobayashi, T.; Singappuli-Arachchige, D.; Slowing, I.I.; Pruski, M. Spatial distribution of organic functional groups supported on mesoporous silica nanoparticles (2): A study by H-1 triple-quantum fast-MAS solid-state NMR. Phys. Chem. Chem. Phys. 2018, 20, 22203–22209. [Google Scholar] [CrossRef] [PubMed]
- Sebastiani, D. Ab-Initio Calculations of NMR Parameters in Condensed Phases. Mod. Phys. Lett. B 2003, 17, 1301–1319. [Google Scholar] [CrossRef]
- Xu, Y.; Watermann, T.; Limbach, H.-H.; Gutmann, T.; Sebastiani, D.; Buntkowsky, G. Water and small organic molecules as probes for geometric confinement in well-ordered mesoporous carbon materials. Phys. Chem. Chem. Phys. 2014, 16, 9327–9336. [Google Scholar] [CrossRef] [PubMed]
- Buntkowsky, G.; Vogel, M.; Winter, R. Properties of Hydrogen-Bonded Liquids at Interfaces. Z. Phys. Chem. 2018, 232, 937–972. [Google Scholar] [CrossRef]
- Buch, V. (Ed.) Water in Confining Geometries; Springer: Berlin/Heidelberg, Germany, 2003; ISBN 3540004114. [Google Scholar]
- Björneholm, O.; Hansen, M.H.; Hodgson, A.; Liu, L.-M.; Limmer, D.T.; Michaelides, A.; Pedevilla, P.; Rossmeisl, J.; Shen, H.; Tocci, G.; et al. Water at Interfaces. Chem. Rev. 2016, 116, 7698–7726. [Google Scholar] [CrossRef] [PubMed]
- Huber, P. Soft matter in hard confinement: Phase transition thermodynamics, structure, texture, diffusion and flow in nanoporous media. J. Phys. Condens. Matter 2015, 27, 103102. [Google Scholar] [CrossRef] [PubMed]
- Swenson, J.; Cerveny, S. Dynamics of deeply supercooled interfacial water. J. Phys. Condens. Matter 2015, 27, 33102. [Google Scholar] [CrossRef] [PubMed]
- Amann-Winkel, K.; Bellissent-Funel, M.-C.; Bove, L.E.; Loerting, T.; Nilsson, A.; Paciaroni, A.; Schlesinger, D.; Skinner, L. X-ray and Neutron Scattering of Water. Chem. Rev. 2016, 116, 7570–7589. [Google Scholar] [CrossRef] [PubMed]
- Kimmich, R. NMR; Springer: Berlin/Heidelberg, Germany, 1997; ISBN 978-3-642-64465-8. [Google Scholar]
- Laage, D.; Elsaesser, T.; Hynes, J.T. Water Dynamics in the Hydration Shells of Biomolecules. Chem. Rev. 2017, 117, 10694–10725. [Google Scholar] [CrossRef] [PubMed]
- Horstmann, R.; Hecht, L.; Kloth, S.; Vogel, M. Structural and Dynamical Properties of Liquids in Confinements: A Review of Molecular Dynamics Simulation Studies. Langmuir 2022, 38, 6506–6522. [Google Scholar] [CrossRef] [PubMed]
- Vogel, M. NMR studies on simple liquids in confinement. Eur. Phys. J. 2010, 189, 47–64. [Google Scholar] [CrossRef]
- Demuth, D.; Sattig, M.; Steinrücken, E.; Weigler, M.; Vogel, M. 2H NMR Studies on the Dynamics of Pure and Mixed Hydrogen-Bonded Liquids in Confinement. Z. Phys. Chem. 2018, 232, 1059–1087. [Google Scholar] [CrossRef]
- Buntkowsky, G.; Vogel, M. Small Molecules, Non-Covalent Interactions, and Confinement. Molecules 2020, 25, 3311. [Google Scholar] [CrossRef] [PubMed]
- Buntkowsky, G.; Döller, S.; Haro-Mares, N.; Gutmann, T.; Hoffmann, M. Solid-state NMR studies of non-ionic surfactants confined in mesoporous silica. Z. Phys. Chem. 2022, 236, 939–960. [Google Scholar] [CrossRef]
- Buntkowsky, G.; Breitzke, H.; Adamczyk, A.; Roelofs, F.; Emmler, T.; Gedat, E.; Grünberg, B.; Xu, Y.; Limbach, H.H.; Shenderovich, I.; et al. Structural and Dynamical Properties of Guest Molecules confined in mesoporous Silica Materials revealed by NMR. Phys. Chem. Chem. Phys. 2007, 9, 4843–4853. [Google Scholar] [CrossRef] [PubMed]
- Gedat, E.; Schreiber, A.; Albrecht, J.; Shenderovich, I.; Findenegg, G.; Limbach, H.-H.; Buntkowsky, G. 2H- Solid State NMR Study of Benzene-d6 confined in mesoporous Silica SBA-15. J. Phys. Chem. B 2002, 106, 1977. [Google Scholar] [CrossRef]
- Aksnes, D.W.; Gjerdåker, L.; Kimtys, L.; Førland, K. Dynamic 1 H and 2 H NMR investigations of acetonitrile confined in porous silica. Phys. Chem. Chem. Phys. 2003, 5, 2680–2685. [Google Scholar] [CrossRef]
- Sen, S.; Risbud, S.H.; Bartl, M.H. Thermodynamic and Kinetic Transitions of Liquids in Nanoconfinement. Acc. Chem. Res. 2020, 53, 2869–2878. [Google Scholar] [CrossRef] [PubMed]
- Steinrücken, E.; Weigler, M.; Schiller, V.; Vogel, M. Dynamical Susceptibilities of Confined Water from Room Temperature to the Glass Transition. J. Phys. Chem. Lett. 2023, 14, 4104–4112. [Google Scholar] [CrossRef] [PubMed]
- Becher, M.; Lichtinger, A.; Minikejew, R.; Vogel, M.; Rössler, E.A. NMR Relaxometry Accessing the Relaxation Spectrum in Molecular Glass Formers. Int. J. Mol. Sci. 2022, 23, 5118. [Google Scholar] [CrossRef]
- Vogel, M. Origins of apparent fragile-to-strong transitions of protein hydration waters. Phys. Rev. Lett. 2008, 101, 225701. [Google Scholar] [CrossRef] [PubMed]
- Sattig, M.; Reutter, S.; Fujara, F.; Werner, M.; Buntkowsky, G.; Vogel, M. NMR studies on the temperature-dependent dynamics of confined water. Phys. Chem. Chem. Phys. 2014, 16, 19229–19240. [Google Scholar] [CrossRef]
- Weigler, M.; Brodrecht, M.; Buntkowsky, G.; Vogel, M. Reorientation of Deeply Cooled Water in Mesoporous Silica: NMR Studies of the Pore-Size Dependence. J. Phys. Chem. B 2019, 123, 2123–2134. [Google Scholar] [CrossRef] [PubMed]
- Weigler, M.; Winter, E.; Kresse, B.; Brodrecht, M.; Buntkowsky, G.; Vogel, M. Static field gradient NMR studies of water diffusion in mesoporous silica. Phys. Chem. Chem. Phys. 2020, 22, 13989–13998. [Google Scholar] [CrossRef] [PubMed]
- Winterstein, S.F.; Privalov, A.F.; Greve, C.; Siegel, R.; Pötzschner, B.; Bettermann, M.; Adolph, L.; Timm, J.; Marschall, R.; Rössler, E.A.; et al. Ultrafast Proton Conduction in an Aqueous Electrolyte Confined in Adamantane-like Micropores of a Sulfonated, Aromatic Framework. J. Am. Chem. Soc. 2023, 145, 27563–27575. [Google Scholar] [CrossRef] [PubMed]
- Paquet, E.; Viktor, H.L. Computational Methods for Ab Initio Molecular Dynamics. Adv. Chem. 2018, 2018, 9839641. [Google Scholar] [CrossRef]
- Jämbeck, J.P.M.; Lyubartsev, A.P. Update to the general amber force field for small solutes with an emphasis on free energies of hydration. J. Phys. Chem. B 2014, 118, 3793–3804. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Wolf, R.M.; Caldwell, J.W.; Kollman, P.A.; Case, D.A. Development and testing of a general amber force field. J. Comput. Chem. 2004, 25, 1157–1174. [Google Scholar] [CrossRef] [PubMed]
- Jorgensen, W.L.; Maxwell, D.S.; Tirado-Rives, J. Development and Testing of the OPLS All-Atom Force Field on Conformational Energetics and Properties of Organic Liquids. J. Am. Chem. Soc. 1996, 118, 11225–11236. [Google Scholar] [CrossRef]
- Vanommeslaeghe, K.; Hatcher, E.; Acharya, C.; Kundu, S.; Zhong, S.; Shim, J.; Darian, E.; Guvench, O.; Lopes, P.; Vorobyov, I.; et al. CHARMM general force field: A force field for drug-like molecules compatible with the CHARMM all-atom additive biological force fields. J. Comput. Chem. 2010, 31, 671–690. [Google Scholar] [CrossRef]
- Horta, B.A.C.; Merz, P.T.; Fuchs, P.F.J.; Dolenc, J.; Riniker, S.; Hünenberger, P.H. A GROMOS-Compatible Force Field for Small Organic Molecules in the Condensed Phase: The 2016H66 Parameter Set. J. Chem. Theory Comput. 2016, 12, 3825–3850. [Google Scholar] [CrossRef] [PubMed]
- Grunewald, F.; Rossi, G.; de Vries, A.H.; Marrink, S.J.; Monticelli, L. Transferable MARTINI Model of Poly(ethylene Oxide). J. Phys. Chem. B 2018, 122, 7436–7449. [Google Scholar] [CrossRef]
- Prada-Gracia, D.; Shevchuk, R.; Rao, F. The quest for self-consistency in hydrogen bond definitions. J. Chem. Phys. 2013, 139, 84501. [Google Scholar] [CrossRef] [PubMed]
- Lemkul, J. From Proteins to Perturbed Hamiltonians: A Suite of Tutorials for the GROMACS-2018 Molecular Simulation Package [Article v1.0]. Living J. Comput. Mol. Sci. 2019, 1, 5068. [Google Scholar] [CrossRef]
- Braun, E.; Gilmer, J.; Mayes, H.B.; Mobley, D.L.; Monroe, J.I.; Prasad, S.; Zuckerman, D.M. Best Practices for Foundations in Molecular Simulations Article v1.0. Living J. Comput. Mol. Sci. 2019, 1, 5957. [Google Scholar] [CrossRef] [PubMed]
- Medick, P.; Blochowicz, T.; Vogel, M.; Roessler, E. Comparing the dynamical heterogeneities in binary glass formers and in a glass former embedded in a zeolite—A (2) HNMR study. J. Non-Cryst. Solids 2002, 307, 565–572. [Google Scholar] [CrossRef]
- Dosseh, G.; Xia, Y.; Alba-Simionesco, C. Cyclohexane and Benzene Confined in MCM-41 and SBA-15: Confinement Effects on Freezing and Melting. J. Phys. Chem. B 2003, 107, 6445. [Google Scholar] [CrossRef]
- Lusceac, S.A.; Koplin, C.; Medick, P.; Vogel, M.; Brodie-Linder, N.; LeQuellec, C.; Alba-Simionesco, C.; Roessler, E.A. Type a versus type B glass formers: NMR relaxation in bulk and confining geometry. J. Phys. Chem. B 2004, 108, 16601–16605. [Google Scholar] [CrossRef]
- Alba-Simionesco, C.; Coasne, B.; Dosseh, G.; Dudziak, G.; Gubbins, K.E.; Radhakrishnan, R.; Sliwinska-Bartkowiak, M. Effects of confinement on freezing and melting. J. Phys. Condens. Matter 2006, 18, R15. [Google Scholar] [CrossRef]
- Kiwilsza, A.; Pajzderska, A.; Gonzalez, M.A.; Mielcarek, J.; Wąsicki, J. QENS and NMR Study of Water Dynamics in SBA-15 with a Low Water Content. J. Phys. Chem. C 2015, 119, 16578–16586. [Google Scholar] [CrossRef]
- Krzyżak, A.T.; Habina, I. Low field 1H NMR characterization of mesoporous silica MCM-41 and SBA-15 filled with different amount of water. Microporous Mesoporous Mater. 2016, 231, 230–239. [Google Scholar] [CrossRef]
- Shenderovich, I.G.; Buntkowsky, G.; Schreiber, A.; Gedat, E.; Sharif, S.; Albrecht, J.; Golubev, N.S.; Findenegg, G.H.; Limbach, H.H. Pyridine-N-15—A mobile NMR sensor for surface acidity and surface defects of mesoporous silica. J. Phys. Chem. B 2003, 107, 11924–11939. [Google Scholar] [CrossRef]
- Vyalikh, A.; Emmler, T.; Gedat, E.; Shenderovich, I.; Findenegg, G.H.; Limbach, H.H.; Buntkowsky, G. Evidence of microphase separation in controlled pore glasses. Solid State Nucl. Mag. 2005, 28, 117–124. [Google Scholar] [CrossRef] [PubMed]
- Brodrecht, M.; Breitzke, H.; Gutmann, T.; Buntkowsky, G. Biofunctionalization of Nano Channels by Direct In-Pore Solid-Phase Peptide Synthesis. Chem. Eur. J. 2018, 24, 17814–17822. [Google Scholar] [CrossRef] [PubMed]
- Brodrecht, M.; Kumari, B.; Thankamony, A.S.S.L.; Breitzke, H.; Gutmann, T.; Buntkowsky, G. Structural Insights into Peptides Bound to the Surface of Silica Nanopores. Chem. Eur. J. 2019, 25, 5214–5221. [Google Scholar] [CrossRef] [PubMed]
- Brodrecht, M.; Kumari, B.; Breitzke, H.; Gutmann, T.; Buntkowsky, G. Chemically Modified Silica Materials as Model Systems for the Characterization of Water-Surface Interactions. Z. Phys. Chem. 2018, 232, 1127–1146. [Google Scholar] [CrossRef]
- Grünberg, A.; Yeping, X.; Breitzke, H.; Buntkowsky, G. Solid-state NMR characterization of Wilkinson’s catalyst immobilized in mesoporous SBA-3 silica. Chemistry 2010, 16, 6993–6998. [Google Scholar] [CrossRef]
- Gutmann, T.; Grunberg, A.; Rothermel, N.; Werner, M.; Srour, M.; Abdulhussain, S.; Tan, S.L.; Xu, Y.P.; Breitzke, H.; Buntkowsky, G. Solid-state NMR concepts for the investigation of supported transition metal catalysts and nanoparticles. Solid State Nucl. Mag. 2013, 55–56, 1–11. [Google Scholar] [CrossRef] [PubMed]
- Grün, M.; Unger, K.K.; Matsumoto, A.; Tsutsumi, K. Novel pathways for the preparation of mesoporous MCM-41 materials: Control of porosity and morphology. Microporous Mesoporous Mater. 1999, 27, 207–216. [Google Scholar] [CrossRef]
- Werner, M.; Rothermel, N.; Breitzke, H.; Gutmann, T.; Buntkowsky, G. Recent Advances in Solid State NMR of Small Molecules in Confinement. Isr. J. Chem. 2014, 54, 60–73. [Google Scholar] [CrossRef]
- Grünberg, B.; Emmler, T.; Gedat, E.; Shenderovich, I.; Findenegg, G.H.; Limbach, H.H.; Buntkowsky, G. Hydrogen bonding of water confined in mesoporous silica MCM-41 and SBA-15 studied by H-1 solid-state NMR. Chem. Eur. J. 2004, 10, 5689–5696. [Google Scholar] [CrossRef] [PubMed]
- Richert, R. Dynamics of Nanoconfined Supercooled Liquids. Annu. Rev. Phys. Chem. 2011, 62, 65–84. [Google Scholar] [CrossRef]
- Brodrecht, M.; Klotz, E.; Lederle, C.; Breitzke, H.; Stühn, B.; Vogel, M.; Buntkowsky, G. A combined Solid-State NMR, Dielectric Spectroscopy and Calorimetric Study of Water in lowly hydrated MCM-41 Samples. Z. Phys. Chem. 2018, 232, 1003–1016. [Google Scholar] [CrossRef]
- Hoffmann, M.M.; Bothe, S.; Gutmann, T.; Hartmann, F.-F.; Reggelin, M.; Buntkowsky, G. Directly vs Indirectly Enhanced 13 C in Dynamic Nuclear Polarization Magic Angle Spinning NMR Experiments of Nonionic Surfactant Systems. J. Phys. Chem. C 2017, 121, 2418–2427. [Google Scholar] [CrossRef]
- Hermens, J.L.; de Bruijn, J.H.; Brooke, D.N. The octanol–water partition coefficient: Strengths and limitations. Environ. Toxicol. Chem. 2013, 32, 732–733. [Google Scholar] [CrossRef] [PubMed]
- Leo, A.; Hansch, C.; Elkins, D. Partition coefficients and their uses. Chem. Rev. 1971, 71, 525–616. [Google Scholar] [CrossRef]
- Kumari, B.; John, D.; Hoffmann, P.; Spende, A.; Toimil-Molares, M.E.; Trautmann, C.; Hess, C.; Ruff, P.; Stark, R.; Schulze, M.; et al. Surface enhanced DNP assisted solid-state NMR of functionalized SiO2 coated Polycarbonate Membranes. Z. Phys. Chem. 2018, 232, 1173–1186. [Google Scholar] [CrossRef]
- Kumari, B.; Brodrecht, M.; Breitzke, H.; Werner, M.; Grunberg, B.; Limbach, H.H.; Forg, S.; Sanjon, E.P.; Drossel, B.; Gutmann, T.; et al. Mixtures of Alcohols and Water confined in Mesoporous Silica: A Combined Solid-State NMR and Molecular Dynamics Simulation Study. J. Phys. Chem. C 2018, 122, 19540–19550. [Google Scholar] [CrossRef]
- Kumari, B.; Brodrecht, M.; Gutmann, T.; Breitzke, H.; Buntkowsky, G. Efficient Referencing of FSLG CPMAS HETCOR Spectra Using 2D H-1-H-1 MAS FSLG. Appl. Magn. Reson. 2019, 50, 1399–1407. [Google Scholar] [CrossRef]
- Kärger, J.; Pfeifer, H. NMR self-diffusion studies in zeolite science and technology. Zeolites 1987, 7, 90–107. [Google Scholar] [CrossRef]
- Kärger, J.; Freude, D. In situ studies of catalytic reactions in zeolites by means of PFG and MAS NMR techniques. Stud. Surf. Sci. Catal. 1997, 105, 551–558. [Google Scholar]
- Kärger, J.; Freude, D. Mass transfer in micro- and mesoporous materials. Chem. Eng. Technol. 2002, 25, 769–778. [Google Scholar] [CrossRef]
- Kaerger, J.; Valiullin, R. Mass transfer in mesoporous materials: The benefit of microscopic diffusion measurement. Chem. Soc. Rev. 2013, 42, 4172–4197. [Google Scholar] [CrossRef] [PubMed]
- Findenegg, G.H.; Jaehnert, S.; Akcakayiran, D.; Schreiber, A. Freezing and Melting of Water Confined in Silica Nanopores. ChemPhysChem 2008, 9, 2651–2659. [Google Scholar] [CrossRef] [PubMed]
- Geppi, M.; Borsacchi, S.; Mollica, G.; Veracini, C.A. Applications of Solid-State NMR to the Study of Organic/Inorganic Multicomponent Materials. Appl. Spectrosc. Rev. 2009, 44, 1–89. [Google Scholar] [CrossRef]
- Yang, Y.; Beele, B.; Bluemel, J. Easily immobilized di- and tetraphosphine linkers: Rigid scaffolds that prevent interactions of metal complexes with oxide supports. J. Am. Chem. Soc. 2008, 130, 3771–3773. [Google Scholar] [CrossRef] [PubMed]
- Bluemel, J. Linkers and catalysts immobilized on oxide supports: New insights by solid-state NMR spectroscopy. Coordin. Chem. Rev. 2008, 252, 2410–2423. [Google Scholar] [CrossRef]
- Vafaeezadeh, M.; Hashemi, M.M. Polyethylene glycol (PEG) as a green solvent for carbon–carbon bond formation reactions. J. Mol. Liq. 2015, 207, 73–79. [Google Scholar] [CrossRef]
- Sayari, A.; Hamoudi, S. Periodic mesoporous silica-based organic-inorganic nanocomposite materials. Chem. Mater. 2001, 13, 3151–3168. [Google Scholar] [CrossRef]
- Linssen, T.; Cassiers, K.; Cool, P.; Vansant, E.F. Mesoporous templated silicates: An overview of their synthesis, catalytic activation and evaluation of the stability. Adv. Colloid Interface Sci. 2003, 103, 121–147. [Google Scholar] [CrossRef]
- Hoffmann, M.M. Polyethylene glycol as a green chemical solvent. Curr. Opin. Colloid Interface Sci. 2022, 57, 101537. [Google Scholar] [CrossRef]
- Campos, J.F.; Berteina-Raboin, S. Greener Synthesis of Nitrogen-Containing Heterocycles in Water, PEG, and Bio-Based Solvents. Catalysts 2020, 10, 429. [Google Scholar] [CrossRef]
- Kardooni, R.; Kiasat, A.R. Polyethylene Glycol as a Green and Biocompatible Reaction Media for the Catalyst Free Synthesis of Organic Compounds. Curr. Org. Chem. 2020, 24, 1275–1314. [Google Scholar] [CrossRef]
- Soni, J.; Sahiba, N.; Sethiya, A.; Agarwal, S. Polyethylene glycol: A promising approach for sustainable organic synthesis. J. Mol. Liq. 2020, 315, 113766. [Google Scholar] [CrossRef]
- Stanley, H.E.; Buldyrev, S.V.; Franzese, G.; Kumar, P.; Mallamace, F.; Mazza, M.G.; Stokely, K.; Xu, L. Liquid polymorphism: Water in nanoconfined and biological environments. J. Phys. Condens. Matter 2010, 22, 284101. [Google Scholar] [CrossRef]
- Geske, J.; Harrach, M.; Heckmann, L.; Horstmann, R.; Klameth, F.; Müller, N.; Pafong, E.; Wohlfromm, T.; Drossel, B.; Vogel, M. Molecular Dynamics Simulations of Water, Silica, and Aqueous Mixtures in Bulk and Confinement. Z. Phys. Chem. 2018, 232, 1187–1225. [Google Scholar] [CrossRef]
- Baschnagel, J.; Meyer, H.; Varnik, F.; Metzger, S.; Aichele, M.; Müller, M.; Binder, K. Computer Simulations of Polymers Close to Solid Interfaces: Some Selected Topics. Interface Sci. 2003, 11, 159–173. [Google Scholar] [CrossRef]
- Berendsen, H.; van der Spoel, D.; van Drunen, R. GROMACS: A message-passing parallel molecular dynamics implementation. Comput. Phys. Commun. 1995, 91, 43–56. [Google Scholar] [CrossRef]
- van der Spoel, D.; Lindahl, E.; Hess, B.; Groenhof, G.; Mark, A.E.; Berendsen, H.J.C. GROMACS: Fast, flexible, and free. J. Comput. Chem. 2005, 26, 1701–1718. [Google Scholar] [CrossRef]
- Páll, S.; Hess, B. A flexible algorithm for calculating pair interactions on SIMD architectures. Comput. Phys. Commun. 2013, 184, 2641–2650. [Google Scholar] [CrossRef]
- Essmann, U.; Perera, L.; Berkowitz, M.L.; Darden, T.; Lee, H.; Pedersen, L.G. A smooth particle mesh Ewald method. J. Chem. Phys. 1995, 103, 8577–8593. [Google Scholar] [CrossRef]
- Bussi, G.; Donadio, D.; Parrinello, M. Canonical sampling through velocity rescaling. J. Chem. Phys. 2007, 126, 14101. [Google Scholar] [CrossRef]
- Parrinello, M.; Rahman, A. Polymorphic transitions in single crystals: A new molecular dynamics method. J. Appl. Phys. 1981, 52, 7182–7190. [Google Scholar] [CrossRef]
- Nosé, S.; Klein, M.L. Constant pressure molecular dynamics for molecular systems. Mol. Phys. 1983, 50, 1055–1076. [Google Scholar] [CrossRef]
- Hoffmann, M.M.; Too, M.D.; Paddock, N.A.; Horstmann, R.; Kloth, S.; Vogel, M.; Buntkowsky, G. On the Behavior of the Ethylene Glycol Components of Polydisperse Polyethylene Glycol PEG200. J. Phys. Chem. B 2023, 127, 1178–1196. [Google Scholar] [CrossRef] [PubMed]
- Haro Mares, N.B.; Brodrecht, M.; Wissel, T.; Döller, S.C.; Rösler, L.; Breitzke, H.; Hoffmann, M.M.; Gutmann, T.; Buntkowsky, G. Influence of APTES-Decorated Mesoporous Silica on the Dynamics of Ethylene Glycol Molecules─Insights from Variable Temperature 2 H Solid-State NMR. J. Phys. Chem. C 2023, 127, 19735–19746. [Google Scholar] [CrossRef]
- Vyalikh, A.; Emmler, T.; Gruenberg, B.; Xu, Y.; Shenderovich, I.; Findenegg, G.H.; Limbach, H.H.; Buntkowsky, G. Hydrogen bonding of water confined in controlled-pore glass 10-75 studied by H-1-solid state NMR. Z. Phys. Chem. 2007, 221, 155–168. [Google Scholar] [CrossRef]
- Weigler, M.; Brodrecht, M.; Breitzke, H.; Dietrich, F.; Sattig, M.; Buntkowsky, G.; Vogel, M. 2H NMR studies on water dynamics in functionalized mesoporous silica. Z. Phys. Chem. 2018, 232, 1041–1058. [Google Scholar] [CrossRef]
- Yao, Y.; Fella, V.; Huang, W.; Zhang, K.A.I.; Landfester, K.; Butt, H.J.; Vogel, M.; Floudas, G. Crystallization and Dynamics of Water Confined in Model Mesoporous Silica Particles: Two Ice Nuclei and Two Fractions of Water. Langmuir 2019, 35, 5890–5901. [Google Scholar] [CrossRef]
- Steinrücken, E.; Wissel, T.; Brodrecht, M.; Breitzke, H.; Regentin, J.; Buntkowsky, G.; Vogel, M. 2H NMR study on temperature-dependent water dynamics in amino-acid functionalized silica nanopores. J. Chem. Phys. 2021, 154, 114702. [Google Scholar] [CrossRef] [PubMed]
- Cerveny, S.; Mallamace, F.; Swenson, J.; Vogel, M.; Xu, L. Confined Water as Model of Supercooled Water. Chem. Rev. 2016, 116, 7608–7625. [Google Scholar] [CrossRef]
- Sattig, M.; Vogel, M. Dynamic Crossovers and Stepwise Solidification of Confined Water: A (2)H NMR Study. J. Phys. Chem. Lett. 2014, 5, 174–178. [Google Scholar] [CrossRef]
- Poole, P.H.; Sciortino, F.; Essmann, U.; Stanley, H.E. Phase behaviour of metastable water. Nature 1992, 360, 324–328. [Google Scholar] [CrossRef]
- Schneider, S.; Vogel, M. NMR studies on the coupling of ion and water dynamics on various time and length scales in glass-forming LiCl aqueous solutions. J. Chem. Phys. 2018, 149, 104501. [Google Scholar] [CrossRef] [PubMed]
- Schneider, S.; Säckel, C.; Brodrecht, M.; Breitzke, H.; Buntkowsky, G.; Vogel, M. NMR studies on the influence of silica confinements on local and diffusive dynamics in LiCl aqueous solutions approaching their glass transitions. J. Chem. Phys. 2020, 153, 244501. [Google Scholar] [CrossRef]
- Schneider, S.; Brodrecht, M.; Breitzke, H.; Wissel, T.; Buntkowsky, G.; Varol, H.S.; Brilmayer, R.; Andrieu-Brunsen, A.; Vogel, M. Local and diffusive dynamics of LiCl aqueous solutions in pristine and modified silica nanopores. J. Chem. Phys. 2022, 157, 34503. [Google Scholar] [CrossRef] [PubMed]
- Schiller, V.; Vogel, M. Ice-Water Equilibrium in Nanoscale Confinement. Phys. Rev. Lett. 2024, 132, 16201. [Google Scholar] [CrossRef] [PubMed]
- Valiullin, R.; Furó, I. The morphology of coexisting liquid and frozen phases in porous materials as revealed by exchange of nuclear spin magnetization followed by H1 nuclear magnetic resonance. J. Chem. Phys. 2002, 117, 2307–2316. [Google Scholar] [CrossRef]
- Kuhs, W.F.; Sippel, C.; Falenty, A.; Hansen, T.C. Extent and relevance of stacking disorder in “ice I(c)”. Proc. Natl. Acad. Sci. USA 2012, 109, 21259–21264. [Google Scholar] [CrossRef]
- Malkin, T.L.; Murray, B.J.; Salzmann, C.G.; Molinero, V.; Pickering, S.J.; Whale, T.F. Stacking disorder in ice I. Phys. Chem. Chem. Phys. 2015, 17, 60–76. [Google Scholar] [CrossRef] [PubMed]
- Döller, S.C.; Brodrecht, M.; Haro Mares, N.B.; Breitzke, H.; Gutmann, T.; Hoffmann, M.; Buntkowsky, G. Deuterium NMR Studies of the Solid–Liquid Phase Transition of Octanol- d17 Confined in SBA-15. J. Phys. Chem. C 2021, 125, 25155–25164. [Google Scholar] [CrossRef]
- Vyalikh, A.; Emmler, T.; Shenderovich, I.; Zeng, Y.; Findenegg, G.H.; Buntkowsky, G. H-2-solid state NMR and DSC study of isobutyric acid in mesoporous silica materials. Phys. Chem. Chem. Phys. 2007, 9, 2249–2257. [Google Scholar] [CrossRef]
- Harrach, M.F.; Drossel, B.; Winschel, W.; Gutmann, T.; Buntkowsky, G. Mixtures of Isobutyric Acid and Water Confined in Cylindrical Silica Nanopores Revisited: A Combined Solid-State NMR and Molecular Dynamics Simulation Study. J. Phys. Chem. C 2015, 119, 28961–28969. [Google Scholar] [CrossRef]
- Masierak, W.; Emmler, T.; Gedat, E.; Schreiber, A.; Findenegg, G.H.; Buntkowsky, G. Microcrystallization of benzene-d6 in mesoporous silica revealed by 2H-solid state NMR. J. Phys. Chem. B 2004, 108, 18890–18896. [Google Scholar] [CrossRef]
- Gruenberg, B.; Gruenberg, A.; Limbach, H.-H.; Buntkowsky, G. Melting of Naphthalene Confined in Mesoporous Silica MCM-41. Appl. Magn. Reson. 2013, 44, 189–201. [Google Scholar] [CrossRef]
- Amadeu, N.d.S.; Gruenberg, B.; Frydel, J.; Werner, M.; Limbach, H.-H.; Breitzke, H.; Buntkowsky, G. Melting of Low Molecular Weight Compounds in Confinement Observed by H-2-Solid State NMR: Biphenyl, a Case Study. Z. Phys. Chem. 2012, 226, 1169–1185. [Google Scholar] [CrossRef]
- Kissinger, H.E. Variation of Peak Temperature with Heating Rate in Differential Thermal Analysis. J. Res. Nat. Bur. Stand. 1956, 57, 217. [Google Scholar] [CrossRef]
- Jackson, C.L.; McKenna, G.B. The melting behavior of organic materials confined in porous solids. J. Chem. Phys. 1990, 93, 9002–9011. [Google Scholar] [CrossRef]
- Millett, F.S.; Dailey, B.P. NMR Determination of Some Deuterium Quadrupole Coupling Constants in Nematic Solutions. J. Chem. Phys. 1972, 56, 3249–3256. [Google Scholar] [CrossRef]
- Polson, J.M.; Fyfe, J.D.D.; Jeffrey, K.R. The reorientation of t -butyl groups in butylated hydroxytoluene: A deuterium nuclear magnetic resonance spectral and relaxation time study. J. Chem. Phys. 1991, 94, 3381–3388. [Google Scholar] [CrossRef]
- Beshah, K.; Olejniczak, E.T.; Griffin, R.G. Deuterium NMR study of methyl group dynamics in L -alanine. J. Chem. Phys. 1987, 86, 4730–4736. [Google Scholar] [CrossRef]
- Spiess, H.; Sillescu, H. Solid echoes in the slow-motion region. J. Magn. Reson. 1981, 42, 381–389. [Google Scholar] [CrossRef]
- Rössler, E.; Taupitz, M.; Börner, K.; Schulz, M.; Vieth, H.-M. A simple method analyzing 2H nuclear magnetic resonance line shapes to determine the activation energy distribution of mobile guest molecules in disordered systems. J. Chem. Phys. 1990, 92, 5847–5855. [Google Scholar] [CrossRef]
- Döller, S.C.; Gutmann, T.; Hoffmann, M.; Buntkowsky, G. A case study on the influence of hydrophilicity on the signal enhancement by dynamic nuclear polarization. Solid State Nucl. Mag. 2022, 122, 101829. [Google Scholar] [CrossRef] [PubMed]
- Reuhl, M.; Weigler, M.; Brodrecht, M.; Buntkowsky, G.; Vogel, M. Nuclear Magnetic Resonance and Broadband Dielectric Spectroscopy Studies on the Dynamics of Ethylene Glycol in Mesoporous Silica. J. Phys. Chem. C 2020, 124, 20998–21012. [Google Scholar] [CrossRef]
- Doadrio, A.L.; Sánchez-Montero, J.M.; Doadrio, J.C.; Salinas, A.J.; Vallet-Regí, M. A molecular model to explain the controlled release from SBA-15 functionalized with APTES. Microporous Mesoporous Mater. 2014, 195, 43–49. [Google Scholar] [CrossRef]
- Reuhl, M.; Vogel, M. Temperature-dependent dynamics at protein-solvent interfaces. J. Chem. Phys. 2022, 157, 74705. [Google Scholar] [CrossRef] [PubMed]
- Döller, S.C.; Brodrecht, M.; Gutmann, T.; Hoffmann, M.; Buntkowsky, G. Direct and Indirect DNP NMR Uncovers the Interplay of Surfactants with Their Mesoporous Host Material. J. Phys. Chem. C 2023, 127, 12125–12134. [Google Scholar] [CrossRef]
- Hoffmann, M.M.; Bothe, S.; Brodrecht, M.; Klimavicius, V.; Haro-Mares, N.B.; Gutmann, T.; Buntkowsky, G. Direct and Indirect Dynamic Nuclear Polarization Transfer Observed in Mesoporous Materials Impregnated with Nonionic Surfactant Solutions of Polar Polarizing Agents. J. Phys. Chem. C 2020, 124, 5145–5156. [Google Scholar] [CrossRef]
- Selvam, P.; Bhatia, S.K.; Sonwane, C.G. Recent advances in processing and characterization of periodic mesoporous MCM-41 silicate molecular sieves. Ind. Eng. Chem. Res. 2001, 40, 3237–3261. [Google Scholar] [CrossRef]
- Hoffmann, M.M.; Horowitz, R.H.; Gutmann, T.; Buntkowsky, G. Densities, Viscosities, and Self-Diffusion Coefficients of Ethylene Glycol Oligomers. J. Chem. Eng. Data 2021, 66, 2480–2500. [Google Scholar] [CrossRef]
- Hoffmann, M.M.; Kealy, J.D.; Gutmann, T.; Buntkowsky, G. Densities, Viscosities, and Self-Diffusion Coefficients of Several Polyethylene Glycols. J. Chem. Eng. Data 2022, 67, 88–103. [Google Scholar] [CrossRef]
- Hoffmann, M.M.; Randall, N.P.; Apak, M.H.; Paddock, N.A.; Gutmann, T.; Buntkowsky, G. Solute–Solvent Interactions of 2,2,6,6-Tetramethylpiperidinyloxyl and 5-Tert-Butylisophthalic Acid in Polyethylene Glycol as Observed by Measurements of Density, Viscosity, and Self-Diffusion Coefficient. J. Solut. Chem. 2023, 52, 685–707. [Google Scholar] [CrossRef]
- Forsyth, C.; Taras, T.; Johnson, A.; Zagari, J.; Collado, C.; Hoffmann, M.M.; Reed, C.R. Microwave Assisted Surfactant-Thermal Synthesis of Metal-Organic Framework Materials. Appl. Sci. 2020, 10, 4563. [Google Scholar] [CrossRef]
- Beejapur, H.A.; Zhang, Q.; Hu, K.; Zhu, L.; Wang, J.; Ye, Z. TEMPO in Chemical Transformations: From Homogeneous to Heterogeneous. ACS Catal. 2019, 9, 2777–2830. [Google Scholar] [CrossRef]
- Prakash, N.; Rajeev, R.; John, A.; Vijayan, A.; George, L.; Varghese, A. 2,2,6,6-Tetramethylpiperidinyloxyl (TEMPO) Radical Mediated Electro-Oxidation Reactions: A Review. ChemistrySelect 2021, 6, 7691–7710. [Google Scholar] [CrossRef]
- Morey, M.S.; Davidson, A.; Stucky, G.D. Silica-based, cubic mesostructures: Synthesis, characterization and relevance for catalysis. J. Porous Mater. 1998, 5, 195–204. [Google Scholar] [CrossRef]
- Chen, H.-T.; Huh, S.; Wiench, J.W.; Pruski, M.; Lin, V.S.-Y. Dialkylaminopyridine-functionalized mesoporous silica nanosphere as an efficient and highly stable heterogeneous nucleophilic catalyst. J. Am. Chem. Soc. 2005, 127, 13305–13311. [Google Scholar] [CrossRef] [PubMed]
- Grünberg, A.; Breitzke, H.; Buntkowsky, G. Solid state NMR of immobilized catalysts and nanocatalysts. Spectrosc. Prop. Inorg. Organomet. Compd. 2012, 43, 289–323. [Google Scholar]
- Mellaerts, R.; Jammaer, J.A.G.; van Speybroeck, M.; Chen, H.; van Humbeeck, J.; Augustijns, P.; van den Mooter, G.; Martens, J.A. Physical state of poorly water soluble therapeutic molecules loaded into SBA-15 ordered mesoporous silica carriers: A case study with itraconazole and ibuprofen. Langmuir 2008, 24, 8651–8659. [Google Scholar] [CrossRef]
- Ukmar, T.; Cendak, T.; Mazaj, M.; Kaucic, V.; Mali, G. Structural and Dynamical Properties of Indomethacin Molecules Embedded within the Mesopores of SBA-15: A Solid-State NMR View. J. Phys. Chem. C 2012, 116, 2662–2671. [Google Scholar] [CrossRef]
- Vallet-Regi, M.; Ramila, A.; Del Real, R.P.; Pérez-Pariente, J. A new property of MCM-41: Drug delivery system. Chem. Mater. 2001, 13, 308–311. [Google Scholar] [CrossRef]
- Hall, S.R.; Walsh, D.; Green, D.; Oreffo, R.; Mann, S. A novel route to highly porous bioactive silica gels. J. Mater. Chem. 2003, 13, 186–190. [Google Scholar] [CrossRef]
- Bothe, S.; Nowag, J.; Klimavičius, V.; Hoffmann, M.; Troitskaya, T.I.; Amosov, E.V.; Tormyshev, V.M.; Kirilyuk, I.; Taratayko, A.; Kuzhelev, A.; et al. Novel Biradicals for Direct Excitation Highfield Dynamic Nuclear Polarization. J. Phys. Chem. C 2018, 122, 11422–11432. [Google Scholar] [CrossRef]
- Hoffmann, M.M.; Bothe, S.; Gutmann, T.; Buntkowsky, G. Combining Freezing Point Depression and Self-Diffusion Data for Characterizing Aggregation. J. Phys. Chem. B 2018, 122, 4913–4921. [Google Scholar] [CrossRef] [PubMed]
- Hoffmann, M.M.; Too, M.D.; Vogel, M.; Gutmann, T.; Buntkowsky, G. Breakdown of the Stokes-Einstein Equation for Solutions of Water in Oil Reverse Micelles. J. Phys. Chem. B 2020, 124, 9115–9125. [Google Scholar] [CrossRef] [PubMed]
- Scharf, N.T.; Stark, A.; Hoffmann, M.M. Ion pairing and dynamics of the ionic liquid 1-hexyl-3-methylimidazolium bis(irifluoromethylsulfonyl)amide (C6mimNTf2) in the low dielectric solvent chloroform. J. Phys. Chem. B 2012, 116, 11488–11497. [Google Scholar] [CrossRef] [PubMed]
- Cade, E.A.; Petenuci, J.; Hoffmann, M.M. Aggregation Behavior of Several Ionic Liquids in Molecular Solvents of Low Polarity--Indication of a Bimodal Distribution. ChemPhysChem 2016, 17, 520–529. [Google Scholar] [CrossRef] [PubMed]
- Mazurek, A.H.; Szeleszczuk, Ł.; Pisklak, D.M. A Review on Combination of Ab Initio Molecular Dynamics and NMR Parameters Calculations. Int. J. Mol. Sci. 2021, 22, 4378. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Haro Mares, N.B.; Döller, S.C.; Wissel, T.; Hoffmann, M.; Vogel, M.; Buntkowsky, G. Structures and Dynamics of Complex Guest Molecules in Confinement, Revealed by Solid-State NMR, Molecular Dynamics, and Calorimetry. Molecules 2024, 29, 1669. https://doi.org/10.3390/molecules29071669
Haro Mares NB, Döller SC, Wissel T, Hoffmann M, Vogel M, Buntkowsky G. Structures and Dynamics of Complex Guest Molecules in Confinement, Revealed by Solid-State NMR, Molecular Dynamics, and Calorimetry. Molecules. 2024; 29(7):1669. https://doi.org/10.3390/molecules29071669
Chicago/Turabian StyleHaro Mares, Nadia B., Sonja C. Döller, Till Wissel, Markus Hoffmann, Michael Vogel, and Gerd Buntkowsky. 2024. "Structures and Dynamics of Complex Guest Molecules in Confinement, Revealed by Solid-State NMR, Molecular Dynamics, and Calorimetry" Molecules 29, no. 7: 1669. https://doi.org/10.3390/molecules29071669
APA StyleHaro Mares, N. B., Döller, S. C., Wissel, T., Hoffmann, M., Vogel, M., & Buntkowsky, G. (2024). Structures and Dynamics of Complex Guest Molecules in Confinement, Revealed by Solid-State NMR, Molecular Dynamics, and Calorimetry. Molecules, 29(7), 1669. https://doi.org/10.3390/molecules29071669








