From Molecular Simulations to Experiments: The Recent Development of Room Temperature Ionic Liquid-Based Electrolytes in Electric Double-Layer Capacitors
Abstract
1. Introduction
2. Supposition from Molecular Simulations and Verification from Experiments
3. Studies on the Mechanism of the Charging and Discharging Process
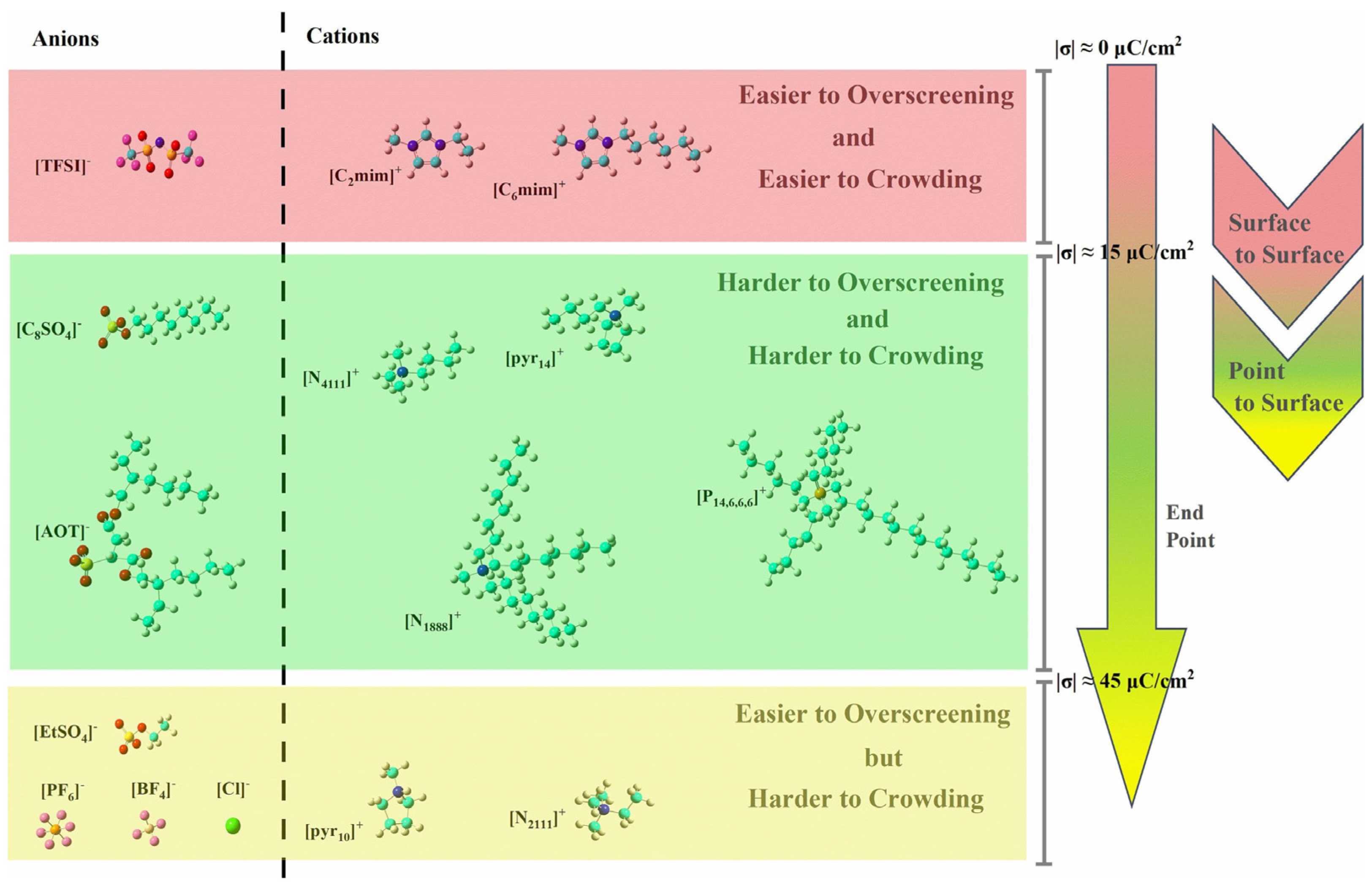
3.1. Studies on the Mechanism of the Charging Process
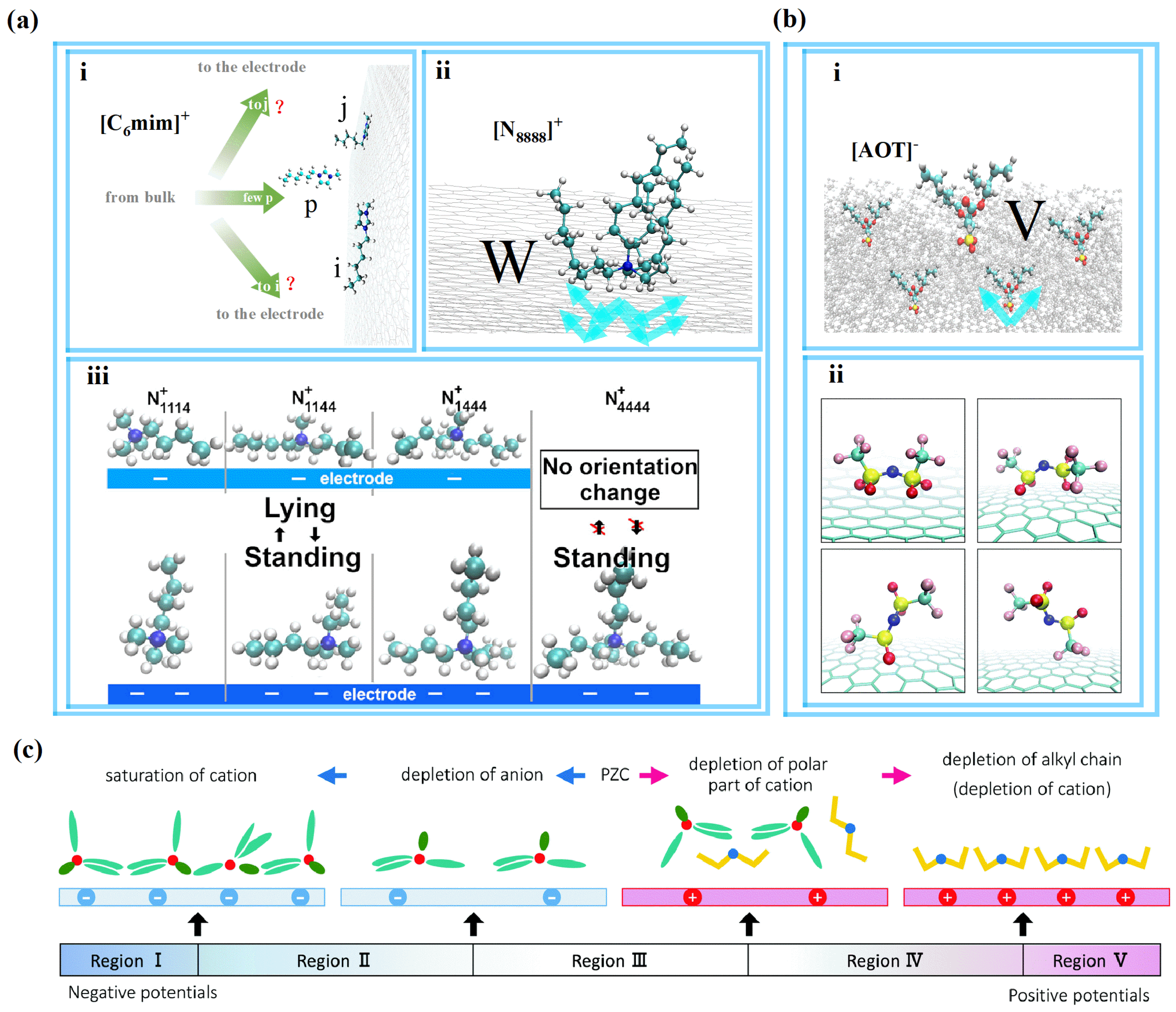
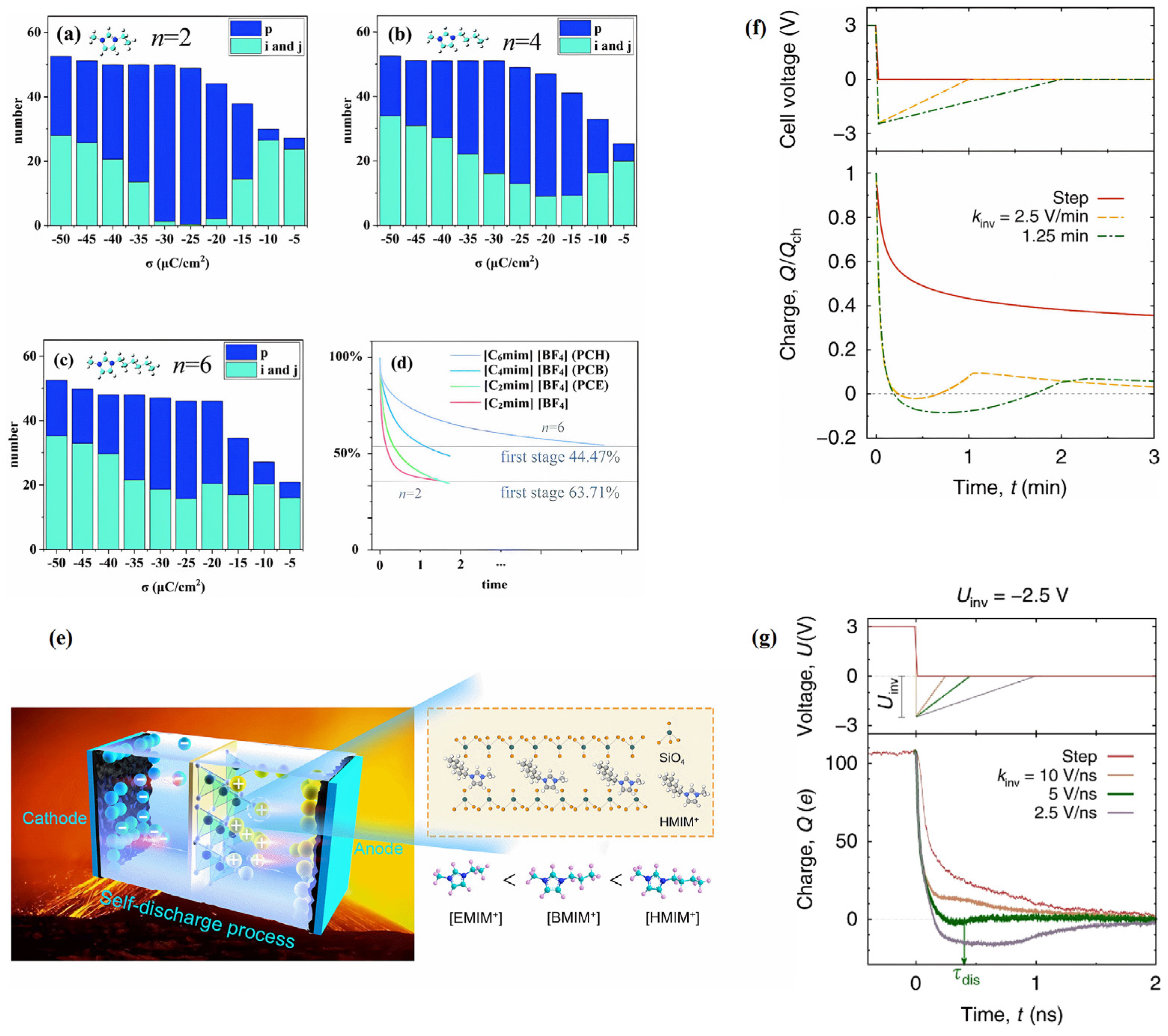
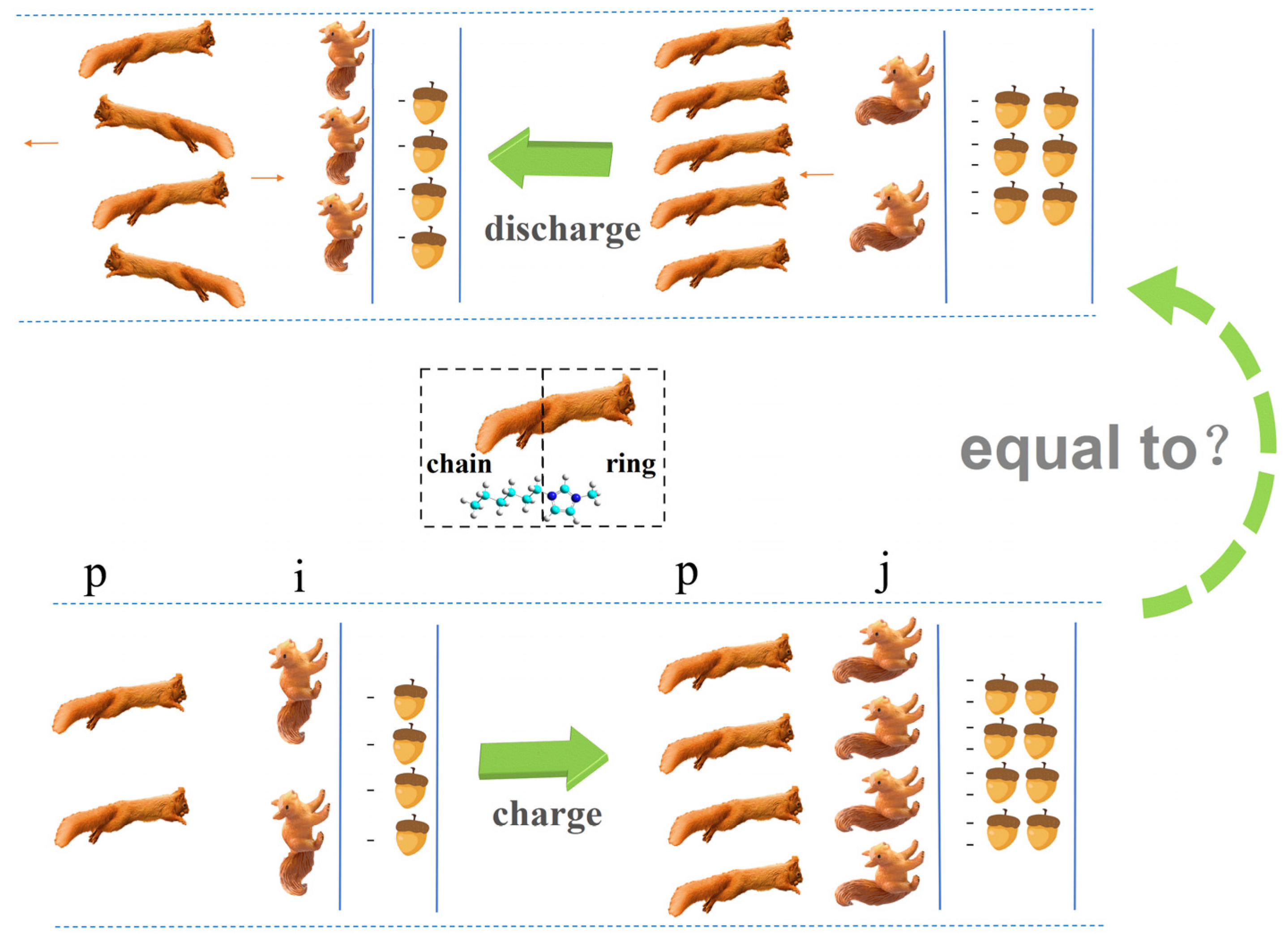
3.2. Studies on the Mechanism of the Discharging Process
3.3. Whole Process of Discharging and Charging
4. Complex RTIL-Based Electrolyte Systems
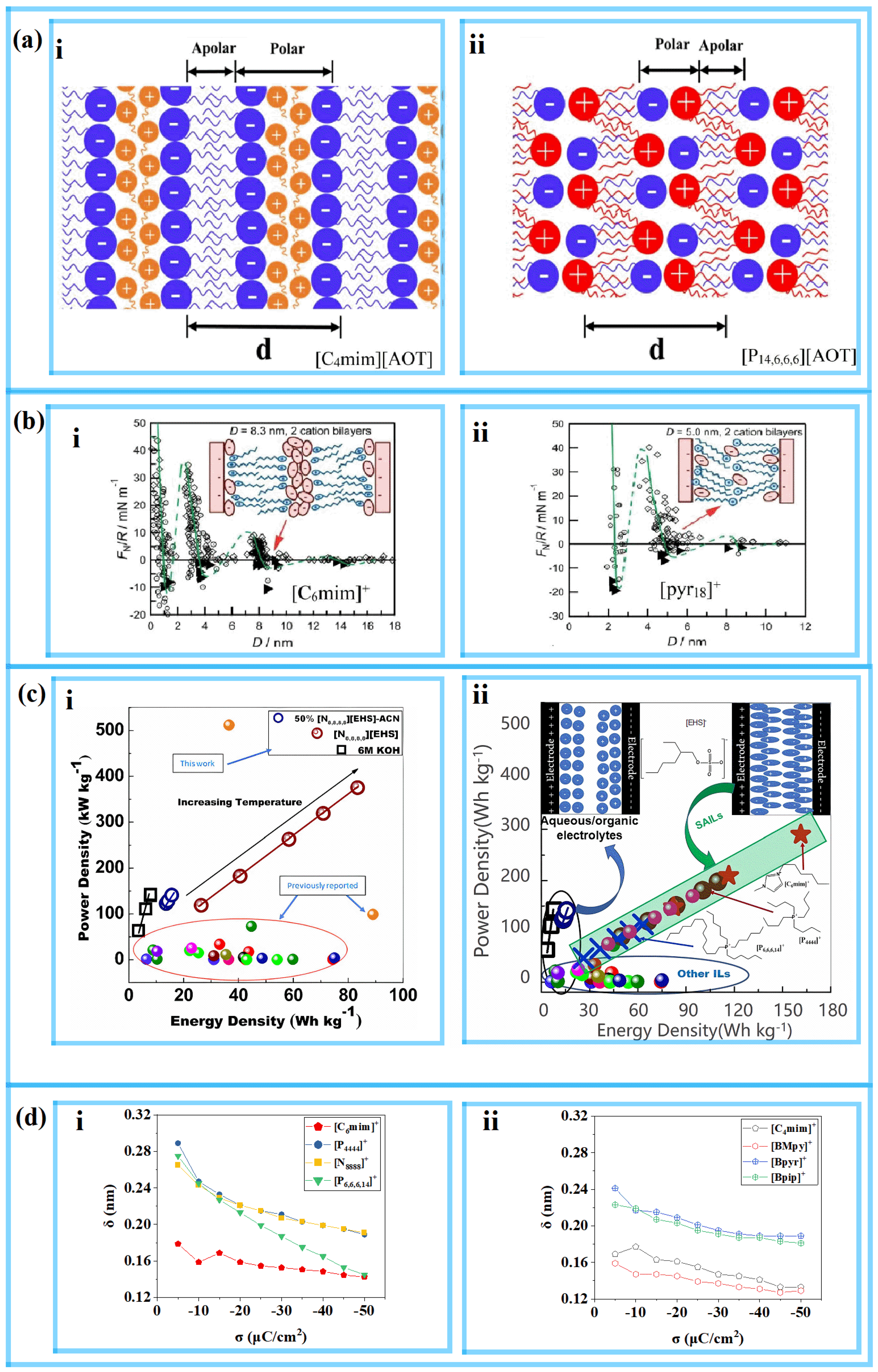
4.1. RTIL–Solvent Mixtures

4.2. RTIL Mixtures
4.3. Water in RTIL Electrolytes
5. The Influence of Novel Electrodes
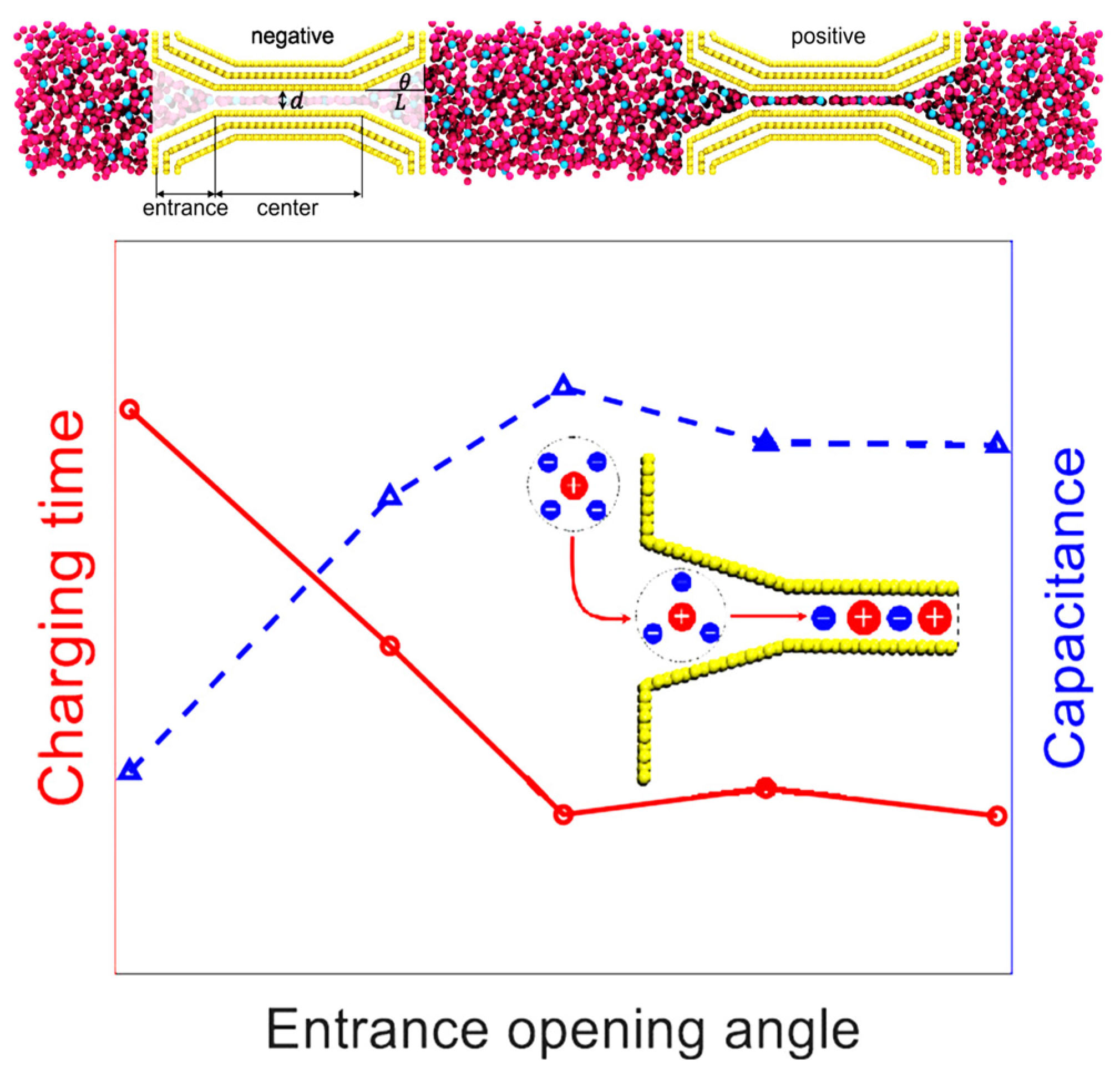
5.1. Investigations of Porous Materials
5.2. Investigations of Two-Dimensional and Other Low-Dimensional Nanomaterials
5.3. Controllable External Potential Conditions
6. Conclusions and Outlook
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gao, Y.; Cui, M.; Qu, S.; Zhao, H.; Shen, Z.; Tan, F.; Dong, Y.; Qin, C.; Wang, Z.; Zhang, W.; et al. Efficient Organic Solar Cells Enabled by Simple Non-Fused Electron Donors with Low Synthetic Complexity. Small 2022, 18, 2104623. [Google Scholar] [CrossRef] [PubMed]
- Gao, Y.; Shen, Z.; Tan, F.; Yue, G.; Liu, R.; Wang, Z.; Qu, S.; Wang, Z.; Zhang, W. Novel benzo[1,2-b:4,5-b′]difuran-based copolymer enables efficient polymer solar cells with small energy loss and high VOC. Nano Energy 2020, 76, 104964. [Google Scholar] [CrossRef]
- Huang, J.; He, S.; Zhang, W.; Saparbaev, A.; Wang, Y.; Gao, Y.; Shang, L.; Dong, G.; Nurumbetova, L.; Yue, G.; et al. Efficient and Stable All-Inorganic CsPbIBr2 Perovskite Solar Cells Enabled by Dynamic Vacuum-Assisted Low-Temperature Engineering. Sol. RRL 2022, 6, 2100839. [Google Scholar] [CrossRef]
- Yao, M.; Liu, A.; Xing, C.; Li, B.; Pan, S.; Zhang, J.; Su, P.; Zhang, H. Asymmetric supercapacitor comprising a core-shell TiNb2O7@MoS2/C anode and a high voltage ionogel electrolyte. Chem. Eng. J. 2020, 394, 124883. [Google Scholar] [CrossRef]
- Yao, M.; Ruan, Q.; Yu, T.; Zhang, H.; Zhang, S. Solid polymer electrolyte with in-situ generated fast Li+ conducting network enable high voltage and dendrite-free lithium metal battery. Energy Storage Mater. 2022, 44, 93–103. [Google Scholar] [CrossRef]
- Yao, M.; Yu, T.; Ruan, Q.; Chen, Q.; Zhang, H.; Zhang, S. High-Voltage and Wide-Temperature Lithium Metal Batteries Enabled by Ultrathin MOF-Derived Solid Polymer Electrolytes with Modulated Ion Transport. ACS Appl. Mater. Interfaces 2021, 13, 47163–47173. [Google Scholar] [CrossRef] [PubMed]
- Yao, M.; Zhang, H.; Xing, C.; Li, Q.; Tang, Y.; Zhang, F.; Yang, K.; Zhang, S. Rational design of biomimetic ant-nest solid polymer electrolyte for high-voltage Li-metal battery with robust mechanical and electrochemical performance. Energy Storage Mater. 2021, 41, 51–60. [Google Scholar] [CrossRef]
- Wang, P.; Du, X.; Wang, X.; Zhang, K.; Sun, J.; Chen, Z.; Xia, Y. Integrated fiber electrodes based on marine polysaccharide for ultrahigh-energy-density flexible supercapacitors. J. Power Sources 2021, 506, 230130. [Google Scholar] [CrossRef]
- Wang, P.; Liu, K.; Wang, X.; Meng, Z.; Xin, Z.; Cui, C.; Quan, F.; Zhang, K.; Xia, Y. Interface engineering of calligraphic ink mediated conformal polymer fibers for advanced flexible supercapacitors. J. Mater. Chem. A 2022, 10, 15776–15784. [Google Scholar] [CrossRef]
- Wang, P.; Meng, Z.; Wang, X.; Zhao, Z.; Wang, Y.; Quan, F.; Tian, W.; Yang, C.; Zhang, K.; Xia, Y. Double-core–shell polysaccharide polymer networks for highly flexible, safe, and durable supercapacitors. J. Mater. Chem. A 2022, 10, 8948–8957. [Google Scholar] [CrossRef]
- Li, C.Y.; Chen, M.; Liu, S.; Lu, X.; Meng, J.; Yan, J.; Abruna, H.D.; Feng, G.; Lian, T. Unconventional interfacial water structure of highly concentrated aqueous electrolytes at negative electrode polarizations. Nat. Commun. 2022, 13, 5330. [Google Scholar] [CrossRef]
- Wan, K.M.; Wang, Y.; Liu, C.Y.; Wei, C.L.; Lv, S.Y.; Tang, X.; Fang, T.M.; Zhao, J.; Wei, G.; Qi, P.F.; et al. Facile synthesis of hierarchical Ti3C2@FeOOH nanocomposites for antimony contaminated wastewater treatment: Performance, mechanisms, reutilization, and sustainability. Chem. Eng. J. 2022, 450, 138038. [Google Scholar] [CrossRef]
- Wei, C.; Fang, T.; Tang, X.; Jiang, K.; Liu, X. Ti2CT2 MXene as Anodes for Metal Ion Batteries: From Monolayer to Bilayer to Pillar Structure. Langmuir 2022, 38, 11732–11742. [Google Scholar] [CrossRef]
- Wu, M.X.; Wei, C.; Wang, X.H.; Xia, Q.Q.; Wang, H.; Liu, X. Construction and Sensing Amplification of Raspberry-Shaped MOF@MOF. Inorg. Chem. 2022, 61, 4705–4713. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Wei, C.; Wu, M.-X.; Wang, Y.; Jiang, H.; Zhou, G.; Tang, X.; Liu, X. A high-performance COF-based aqueous zinc-bromine battery. Chem. Eng. J. 2023, 451, 138915. [Google Scholar] [CrossRef]
- Kondrat, S.; Feng, G.; Bresme, F.; Urbakh, M.; Kornyshev, A.A. Theory and Simulations of Ionic Liquids in Nanoconfinement. Chem. Rev. 2023, 123, 6668–6715. [Google Scholar] [CrossRef]
- Piatti, E.; Guglielmero, L.; Tofani, G.; Mezzetta, A.; Guazzelli, L.; D’Andrea, F.; Roddaro, S.; Pomelli, C.S. Ionic liquids for electrochemical applications: Correlation between molecular structure and electrochemical stability window. J. Mol. Liq. 2022, 364, 120001. [Google Scholar] [CrossRef]
- Liu, R.-Z.; Shen, Z.-Z.; Wen, R.; Wan, L.-J. Recent advances in the application of scanning probe microscopy in interfacial electroanalytical chemistry. J. Electroanal. Chem. 2023, 938, 117443. [Google Scholar] [CrossRef]
- Cheng, H.; Sun, Q.; Li, L.; Zou, Y.; Wang, Y.; Cai, T.; Zhao, F.; Liu, G.; Ma, Z.; Wahyudi, W.; et al. Emerging Era of Electrolyte Solvation Structure and Interfacial Model in Batteries. ACS Energy Lett. 2022, 7, 490–513. [Google Scholar] [CrossRef]
- Kilic, M.S.; Bazant, M.Z.; Ajdari, A. Steric effects in the dynamics of electrolytes at large applied voltages. I. Double-layer charging. Phys. Rev. E 2007, 75, 021502. [Google Scholar] [CrossRef]
- Kornyshev, A.A. Double-layer in ionic liquids: Paradigm change? J. Phys. Chem. B 2007, 111, 5545–5557. [Google Scholar] [CrossRef] [PubMed]
- Zhou, T.; Gui, C.; Sun, L.; Hu, Y.; Lyu, H.; Wang, Z.; Song, Z.; Yu, G. Energy Applications of Ionic Liquids: Recent Developments and Future Prospects. Chem. Rev. 2023, 123, 12170–12253. [Google Scholar] [CrossRef]
- Jónsson, E. Ionic liquids as electrolytes for energy storage applications—A modelling perspective. Energy Storage Mater. 2020, 25, 827–835. [Google Scholar] [CrossRef]
- Xu, K.; Shao, H.; Lin, Z.; Merlet, C.; Feng, G.; Zhu, J.; Simon, P. Computational Insights into Charge Storage Mechanisms of Supercapacitors. Energy Environ. Mater. 2020, 3, 235–246. [Google Scholar] [CrossRef]
- Shahzad, S.; Shah, A.; Kowsari, E.; Iftikhar, F.J.; Nawab, A.; Piro, B.; Akhter, M.S.; Rana, U.A.; Zou, Y. Ionic Liquids as Environmentally Benign Electrolytes for High-Performance Supercapacitors. Glob. Chall. 2018, 3, 1800023. [Google Scholar] [CrossRef]
- Feng, J.; Wang, Y.; Xu, Y.; Sun, Y.; Tang, Y.; Yan, X. Ion regulation of ionic liquid electrolytes for supercapacitors. Energy Environ. Sci. 2021, 14, 2859–2882. [Google Scholar] [CrossRef]
- Zheng, Y.; Wang, D.; Kaushik, S.; Zhang, S.; Wada, T.; Hwang, J.; Matsumoto, K.; Hagiwara, R. Ionic Liquid Electrolytes for Next-generation Electrochemical Energy Devices. EnergyChem 2022, 4, 100075. [Google Scholar] [CrossRef]
- Tang, X.; Lv, S.; Jiang, K.; Zhou, G.; Liu, X. Recent development of ionic liquid-based electrolytes in lithium-ion batteries. J. Power Sources 2022, 542, 231792. [Google Scholar] [CrossRef]
- Sun, L.; Zhuo, K.; Chen, Y.; Du, Q.; Zhang, S.; Wang, J. Ionic Liquid-Based Redox Active Electrolytes for Supercapacitors. Adv. Funct. Mater. 2022, 32, 2203611. [Google Scholar] [CrossRef]
- Booth, M.J.; Haymet, A.D.J. Molten salts near a charged surface: Integral equation approximation for a model of KCl. Mol. Phys. 2001, 99, 1817–1824. [Google Scholar] [CrossRef]
- Lockett, V.; Sedev, R.; Ralston, J.; Horne, M.; Rodopoulos, T. Differential capacitance of the electrical double layer in imidazolium-based ionic liquids: Influence of potential, cation size, and temperature. J. Phys. Chem. C 2008, 112, 7486–7495. [Google Scholar] [CrossRef]
- Fedorov, M.V.; Kornyshev, A.A. Ionic liquid near a charged wall: Structure and capacitance of electrical double layer. J. Phys. Chem. B 2008, 112, 11868–11872. [Google Scholar] [CrossRef] [PubMed]
- Georgi, N.; Kornyshev, A.A.; Fedorov, M.V. The anatomy of the double layer and capacitance in ionic liquids with anisotropic ions Electrostriction vs lattice saturation. J. Electroanal. Chem. 2010, 649, 261–267. [Google Scholar] [CrossRef]
- Fedorov, M.V.; Georgi, N.; Kornyshev, A.A. Double layer in ionic liquids: The nature of the camel shape of capacitance. Electrochem. Commun. 2010, 12, 296–299. [Google Scholar] [CrossRef]
- Bazant, M.Z.; Storey, B.D.; Kornyshev, A.A. Double layer in ionic liquids: Overscreening versus crowding. Phys. Rev. Lett. 2011, 106, 046102. [Google Scholar] [CrossRef] [PubMed]
- Belotti, M.; Lyu, X.; Xu, L.; Halat, P.; Darwish, N.; Silvester, D.S.; Goh, C.; Izgorodina, E.I.; Coote, M.L.; Ciampi, S. Experimental Evidence of Long-Lived Electric Fields of Ionic Liquid Bilayers. J. Am. Chem. Soc. 2021, 143, 17431–17440. [Google Scholar] [CrossRef]
- Bonagiri, L.K.S.; Panse, K.S.; Zhou, S.; Wu, H.; Aluru, N.R.; Zhang, Y. Real-Space Charge Density Profiling of Electrode-Electrolyte Interfaces with Angstrom Depth Resolution. ACS Nano 2022, 16, 19594–19604. [Google Scholar] [CrossRef] [PubMed]
- Chu, M.; Miller, M.; Dutta, P. Crowding and Anomalous Capacitance at an Electrode-Ionic Liquid Interface Observed Using Operando X-ray Scattering. ACS Cent. Sci. 2016, 2, 175–180. [Google Scholar] [CrossRef]
- Uysal, A.; Zhou, H.; Feng, G.; Lee, S.S.; Li, S.; Fenter, P.; Cummings, P.T.; Fulvio, P.F.; Dai, S.; McDonough, J.K.; et al. Structural Origins of Potential Dependent Hysteresis at the Electrified Graphene/Ionic Liquid Interface. J. Phys. Chem. C 2014, 118, 569–574. [Google Scholar] [CrossRef]
- De Souza, J.P.; Goodwin, Z.A.H.; McEldrew, M.; Kornyshev, A.A.; Bazant, M.Z. Interfacial Layering in the Electric Double Layer of Ionic Liquids. Phys. Rev. Lett. 2020, 125, 116001. [Google Scholar] [CrossRef]
- Zhou, S.; Panse, K.S.; Motevaselian, M.H.; Aluru, N.R.; Zhang, Y. Three-Dimensional Molecular Mapping of Ionic Liquids at Electrified Interfaces. ACS Nano 2020, 14, 17515–17523. [Google Scholar] [CrossRef] [PubMed]
- Mao, X.; Brown, P.; Cervinka, C.; Hazell, G.; Li, H.; Ren, Y.; Chen, D.; Atkin, R.; Eastoe, J.; Grillo, I.; et al. Self-assembled nanostructures in ionic liquids facilitate charge storage at electrified interfaces. Nat. Mater. 2019, 18, 1350–1357. [Google Scholar] [CrossRef]
- Zhang, K.; Zhou, G.; Fang, T.; Jiang, K.; Liu, X. Structural Reorganization of Ionic Liquid Electrolyte by a Rapid Charge/Discharge Circle. J. Phys. Chem. Lett. 2021, 12, 2273–2278. [Google Scholar] [CrossRef] [PubMed]
- Katakura, S.; Nishi, N.; Kobayashi, K.; Amano, K.; Sakka, T. Surface Structure of Quaternary Ammonium-Based Ionic Liquids Studied Using Molecular Dynamics Simulation: Effect of Switching the Length of Alkyl Chains. J. Phys. Chem. C 2019, 123, 7246–7258. [Google Scholar] [CrossRef]
- Katakura, S.; Nishi, N.; Kobayashi, K.; Amano, K.; Sakka, T. Effect of Switching the Length of Alkyl Chains on Electric Double Layer Structure and Differential Capacitance at the Electrode Interface of Quaternary Ammonium-Based Ionic Liquids Studied Using Molecular Dynamics Simulation. J. Phys. Chem. C 2020, 124, 7873–7883. [Google Scholar] [CrossRef]
- Katakura, S.; Nishi, N.; Kobayashi, K.; Amano, K.I.; Sakka, T. An electric double layer structure and differential capacitance at the electrode interface of tributylmethylammonium bis(trifluoromethanesulfonyl)amide studied using a molecular dynamics simulation. Phys. Chem. Chem. Phys. 2020, 22, 5198–5210. [Google Scholar] [CrossRef]
- Jain, P.; Antzutkin, O.N. Nonhalogenated Surface-Active Ionic Liquid as an Electrolyte for Supercapacitors. ACS Appl. Energy Mater. 2021, 4, 7775–7785. [Google Scholar] [CrossRef]
- Zhang, Y.; Marlow, J.B.; Millar, W.; Aman, Z.M.; Silvester, D.S.; Warr, G.G.; Atkin, R.; Li, H. Nanostructure, electrochemistry and potential-dependent lubricity of the catanionic surface-active ionic liquid [P6,6,6,14] [AOT]. J. Colloid. Interface Sci. 2022, 608, 2120–2130. [Google Scholar] [CrossRef]
- Smith, A.M.; Lovelock, K.R.; Gosvami, N.N.; Licence, P.; Dolan, A.; Welton, T.; Perkin, S. Monolayer to Bilayer Structural Transition in Confined Pyrrolidinium-Based Ionic Liquids. J. Phys. Chem. Lett. 2013, 4, 378–382. [Google Scholar] [CrossRef]
- Smith, A.M.; Lovelock, K.R.; Perkin, S. Monolayer and bilayer structures in ionic liquids and their mixtures confined to nano-films. Faraday Discuss. 2013, 167, 279–292. [Google Scholar] [CrossRef]
- Jain, P.; Antzutkin, O.N. 2-Ethylhexylsulfate Anion-based Surface-Active Ionic Liquids (SAILs) as temperature persistent electrolytes for supercapacitors. J. Ion. Liq. 2022, 2, 100034. [Google Scholar] [CrossRef]
- Vatamanu, J.; Borodin, O.; Bedrov, D.; Smith, G.D. Molecular Dynamics Simulation Study of the Interfacial Structure and Differential Capacitance of Alkylimidazolium Bis(trifluoromethanesulfonyl)imide [Cnmim][TFSI] Ionic Liquids at Graphite Electrodes. J. Phys. Chem. C 2012, 116, 7940–7951. [Google Scholar] [CrossRef]
- Zhang, K.; Zhou, G.; Fang, T.; Tang, X.; Liu, X. Surface-active ionic liquids near the electrode surface: Development and influence on molecular dynamics simulations. Appl. Surf. Sci. 2023, 614, 156200. [Google Scholar] [CrossRef]
- Zhang, S.; Nishi, N.; Katakura, S.; Sakka, T. Evaluation of static differential capacitance at the [C4mim(+)][TFSA(-)]/electrode interface using molecular dynamics simulation combined with electrochemical surface plasmon resonance measurements. Phys. Chem. Chem. Phys. 2021, 23, 13905–13917. [Google Scholar] [CrossRef] [PubMed]
- Zhang, K.; Zhou, G.; Fang, T.; Ding, Z.; Liu, X. The ionic liquid-based electrolytes during their charging process: Movable endpoints of overscreening effect near the electrode interface. J. Colloid. Interface Sci. 2023, 650, 648–658. [Google Scholar] [CrossRef] [PubMed]
- Breitsprecher, K.; Holm, C.; Kondrat, S. Charge Me Slowly, I Am in a Hurry: Optimizing Charge-Discharge Cycles in Nanoporous Supercapacitors. ACS Nano 2018, 12, 9733–9741. [Google Scholar] [CrossRef]
- Zhang, K.; Zhou, G.; Fang, T.; Tang, X.; Liu, X. Different shapes based on ionic liquid leading to a two-stage discharge process. J. Mater. Chem. A 2022, 10, 7684–7693. [Google Scholar] [CrossRef]
- Zhao, H.; Zhang, H.; Wang, Z.; Jiang, X.; Xie, Y.; Xu, Z.; Wang, Y.; Yang, W. Chain-Elongated Ionic Liquid Electrolytes for Low Self-Discharge All-Solid-State Supercapacitors at High Temperature. ChemSusChem 2021, 14, 3895–3903. [Google Scholar] [CrossRef]
- Breitsprecher, K.; Janssen, M.; Srimuk, P.; Mehdi, B.L.; Presser, V.; Holm, C.; Kondrat, S. How to speed up ion transport in nanopores. Nat. Commun. 2020, 11, 6085. [Google Scholar] [CrossRef]
- Gu, C.Y.; Yin, L.; Li, S.; Zhang, B.H.; Liu, X.H.; Yan, T.Y. Differential capacitance of ionic liquid and mixture with organic solvent. Electrochim. Acta 2021, 367, 137517. [Google Scholar]
- Cruz, C.; Ciach, A.; Lomba, E.; Kondrat, S. Electrical Double Layers Close to Ionic Liquid-Solvent Demixing. J. Phys. Chem. C 2019, 123, 1596–1601. [Google Scholar] [CrossRef]
- Sampaio, A.M.; Siqueira, L.J.A. Ether-Functionalized Sulfonium Ionic Liquid and Its Binary Mixtures with Acetonitrile as Electrolyte for Electrochemical Double Layer Capacitors: A Molecular Dynamics Study. J. Phys. Chem. B 2020, 124, 6679–6689. [Google Scholar] [CrossRef] [PubMed]
- Bozym, D.J.; Uralcan, B.; Limmer, D.T.; Pope, M.A.; Szamreta, N.J.; Debenedetti, P.G.; Aksay, I.A. Anomalous Capacitance Maximum of the Glassy Carbon-Ionic Liquid Interface through Dilution with Organic Solvents. J. Phys. Chem. Lett. 2015, 6, 2644–2648. [Google Scholar] [CrossRef] [PubMed]
- Douglas, T.; Yoo, S.; Dutta, P. Ionic Liquid Solutions Show Anomalous Crowding Behavior at an Electrode Surface. Langmuir 2022, 38, 6322–6329. [Google Scholar] [CrossRef]
- Tian, J.R.; Cui, C.J.; Xie, Q.; Qian, W.Z.; Xue, C.; Miao, Y.H.; Jin, Y.; Zhang, G.; Guo, B.H. EMIMBF4-GBL binary electrolyte working at −70 °C and 3.7 V for a high performance graphene-based capacitor. J. Mater. Chem. A 2018, 6, 3593–3601. [Google Scholar] [CrossRef]
- Uralcan, B.; Aksay, I.A.; Debenedetti, P.G.; Limmer, D.T. Concentration Fluctuations and Capacitive Response in Dense Ionic Solutions. J. Phys. Chem. Lett. 2016, 7, 2333–2338. [Google Scholar] [CrossRef] [PubMed]
- Lian, C.; Liu, K.; Van Aken, K.L.; Gogotsi, Y.; Wesolowski, D.J.; Liu, H.L.; Jiang, D.E.; Wu, J.Z. Enhancing the Capacitive Performance of Electric Double-Layer Capacitors with Ionic Liquid Mixtures. ACS Energy Lett. 2016, 1, 21–26. [Google Scholar] [CrossRef]
- Yambou, E.P.; Gorska, B.; Beguin, F. Electrical Double-Layer Capacitors Based on a Ternary Ionic Liquid Electrolyte Operating at Low Temperature with Realistic Gravimetric and Volumetric Energy Outputs. ChemSusChem 2021, 14, 1196–1208. [Google Scholar] [CrossRef]
- Lian, C.; Liu, H.L.; Wu, J.Z. Ionic Liquid Mixture Expands the Potential Window and Capacitance of a Supercapacitor in Tandem. J. Phys. Chem. C 2018, 122, 18304–18310. [Google Scholar] [CrossRef]
- Wang, X.; Mehandzhiyski, A.Y.; Arstad, B.; Van Aken, K.L.; Mathis, T.S.; Gallegos, A.; Tian, Z.; Ren, D.; Sheridan, E.; Grimes, B.A.; et al. Selective Charging Behavior in an Ionic Mixture Electrolyte-Supercapacitor System for Higher Energy and Power. J. Am. Chem. Soc. 2017, 139, 18681–18687. [Google Scholar] [CrossRef]
- Qiao, R. Water at ionic liquids-solid interfaces. Curr. Opin. Electrochem. 2019, 13, 11–17. [Google Scholar] [CrossRef]
- Zhang, H.H.; Zhu, M.Y.; Zhao, W.; Li, S.; Feng, G. Molecular dynamics study of room temperature ionic liquids with water at mica surface. Green Energy Environ. 2018, 3, 120–128. [Google Scholar] [CrossRef]
- Bi, S.; Wang, R.; Liu, S.; Yan, J.; Mao, B.; Kornyshev, A.A.; Feng, G. Minimizing the electrosorption of water from humid ionic liquids on electrodes. Nat. Commun. 2018, 9, 5222. [Google Scholar] [CrossRef] [PubMed]
- Chen, M.; Wu, J.; Ye, T.; Ye, J.; Zhao, C.; Bi, S.; Yan, J.; Mao, B.; Feng, G. Adding salt to expand voltage window of humid ionic liquids. Nat. Commun. 2020, 11, 5809. [Google Scholar] [CrossRef] [PubMed]
- Mo, T.M.; Zeng, L.; Wang, Z.X.; Kondrat, S.; Feng, G. Symmetrizing cathode-anode response to speed up charging of nanoporous supercapacitors. Green Energy Environ. 2022, 7, 95–104. [Google Scholar] [CrossRef]
- Mo, T.; Peng, J.; Dai, W.; Chen, M.; Presser, V.; Feng, G. Horn-like Pore Entrance Boosts Charging Dynamics and Charge Storage of Nanoporous Supercapacitors. ACS Nano 2023, 17, 14974–14980. [Google Scholar] [CrossRef] [PubMed]
- Bi, S.; Banda, H.; Chen, M.; Niu, L.; Chen, M.; Wu, T.; Wang, J.; Wang, R.; Feng, J.; Chen, T.; et al. Molecular understanding of charge storage and charging dynamics in supercapacitors with MOF electrodes and ionic liquid electrolytes. Nat. Mater. 2020, 19, 552–558. [Google Scholar] [CrossRef]
- Mo, T.; Bi, S.; Zhang, Y.; Presser, V.; Wang, X.; Gogotsi, Y.; Feng, G. Ion Structure Transition Enhances Charging Dynamics in Subnanometer Pores. ACS Nano 2020, 14, 2395–2403. [Google Scholar] [CrossRef]
- Dong, M.; Zhang, K.; Wan, X.; Wang, S.; Fan, S.; Ye, Z.; Wang, Y.; Yan, Y.; Peng, X. Stable Two-dimensional Nanoconfined Ionic Liquids with Highly Efficient Ionic Conductivity. Small 2022, 18, 2108026. [Google Scholar] [CrossRef]
- Pereira, G.F.L.; Fileti, E.E.; Siqueira, L.J.A. Performance of supercapacitors containing graphene oxide and ionic liquids by molecular dynamics simulations. Carbon 2023, 208, 102–110. [Google Scholar] [CrossRef]
- Liang, K.; Matsumoto, R.A.; Zhao, W.; Osti, N.C.; Popov, I.; Thapaliya, B.P.; Fleischmann, S.; Misra, S.; Prenger, K.; Tyagi, M.; et al. Engineering the Interlayer Spacing by Pre-Intercalation for High Performance Supercapacitor MXene Electrodes in Room Temperature Ionic Liquid. Adv. Funct. Mater. 2021, 31, 2104007. [Google Scholar] [CrossRef]
- Xu, K.; Merlet, C.; Lin, Z.F.; Shao, H.; Taberna, P.L.; Miao, L.; Jiang, J.J.; Zhu, J.X.; Simon, P. Effects of functional groups and anion size on the charging mechanisms in layered electrode materials. Energy Storage Mater. 2020, 33, 460–469. [Google Scholar] [CrossRef]
- Li, D.D.; Li, E.C.; Ji, X.Y.; Yang, Y.R.; Wang, X.D.; Feng, G. Molecular Insights into Curvature Effects on the Capacitance of Electrical Double Layers in Tricationic Ionic Liquids with Carbon Nanotube Electrodes. Langmuir 2023, 39, 588–596. [Google Scholar] [CrossRef] [PubMed]
- Xu, K.; Lin, Z.; Merlet, C.; Taberna, P.-L.; Miao, L.; Jiang, J.; Simon, P. Tracking Ionic Rearrangements and Interpreting Dynamic Volumetric Changes in Two-Dimensional Metal Carbide Supercapacitors: A Molecular Dynamics Simulation Study. ChemSusChem 2018, 11, 1892–1899. [Google Scholar] [CrossRef] [PubMed]
- Li, D.D.; Li, E.C.; Yang, Y.R.; Wang, X.D.; Feng, G. Structure and Capacitance of Electrical Double Layers in Tricationic Ionic Liquids with Organic Solvents. J. Phys. Chem. B 2021, 125, 12753–12762. [Google Scholar] [CrossRef] [PubMed]
- Li, D.D.; Yang, Y.R.; Wang, X.D.; Feng, G. Electrical Double Layer of Linear Tricationic Ionic Liquids at Graphite Electrode. J. Phys. Chem. C 2020, 124, 15723–15729. [Google Scholar] [CrossRef]
- Scalfi, L.; Salanne, M.; Rotenberg, B. Molecular Simulation of Electrode-Solution Interfaces. Annu. Rev. Phys. Chem. 2021, 72, 189–212. [Google Scholar] [CrossRef]
- Bedrov, D.; Piquemal, J.-P.; Borodin, O.; MacKerell, A.D., Jr.; Roux, B.; Schröder, C. Molecular Dynamics Simulations of Ionic Liquids and Electrolytes Using Polarizable Force Fields. Chem. Rev. 2019, 119, 7940–7995. [Google Scholar] [CrossRef]
- Merlet, C.; Pean, C.; Rotenberg, B.; Madden, P.A.; Simon, P.; Salanne, M. Simulating Supercapacitors: Can We Model Electrodes As Constant Charge Surfaces? J. Phys. Chem. Lett. 2013, 4, 264–268. [Google Scholar] [CrossRef]
- Li, H.; Zhang, X.Y.; Peng, J.; He, J.P.; Huang, Z.W.; Wang, J. Cooperative CC-CV Charging of Supercapacitors Using Multicharger Systems. IEEE Trans. Ind. Electron. 2020, 67, 10497–10508. [Google Scholar] [CrossRef]
- Noori, A.; El-Kady, M.F.; Rahmanifar, M.S.; Kaner, R.B.; Mousavi, M.F. Towards establishing standard performance metrics for batteries, supercapacitors and beyond. Chem. Soc. Rev. 2019, 48, 1272–1341. [Google Scholar] [CrossRef] [PubMed]
- Zeng, L.; Wu, T.Z.; Ye, T.; Mo, T.M.; Qiao, R.; Feng, G. Modeling galvanostatic charge-discharge of nanoporous supercapacitors. Nat. Comput. Sci. 2021, 1, 725–731. [Google Scholar] [CrossRef] [PubMed]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, K.; Wei, C.; Zheng, M.; Huang, J.; Zhou, G. From Molecular Simulations to Experiments: The Recent Development of Room Temperature Ionic Liquid-Based Electrolytes in Electric Double-Layer Capacitors. Molecules 2024, 29, 1246. https://doi.org/10.3390/molecules29061246
Zhang K, Wei C, Zheng M, Huang J, Zhou G. From Molecular Simulations to Experiments: The Recent Development of Room Temperature Ionic Liquid-Based Electrolytes in Electric Double-Layer Capacitors. Molecules. 2024; 29(6):1246. https://doi.org/10.3390/molecules29061246
Chicago/Turabian StyleZhang, Kun, Chunlei Wei, Menglian Zheng, Jingyun Huang, and Guohui Zhou. 2024. "From Molecular Simulations to Experiments: The Recent Development of Room Temperature Ionic Liquid-Based Electrolytes in Electric Double-Layer Capacitors" Molecules 29, no. 6: 1246. https://doi.org/10.3390/molecules29061246
APA StyleZhang, K., Wei, C., Zheng, M., Huang, J., & Zhou, G. (2024). From Molecular Simulations to Experiments: The Recent Development of Room Temperature Ionic Liquid-Based Electrolytes in Electric Double-Layer Capacitors. Molecules, 29(6), 1246. https://doi.org/10.3390/molecules29061246







