Analyzing (3-Aminopropyl)triethoxysilane-Functionalized Porous Silica for Aqueous Uranium Removal: A Study on the Adsorption Behavior
Abstract
1. Introduction
2. Results and Discussion
2.1. Surface Characteristics of Absorbents
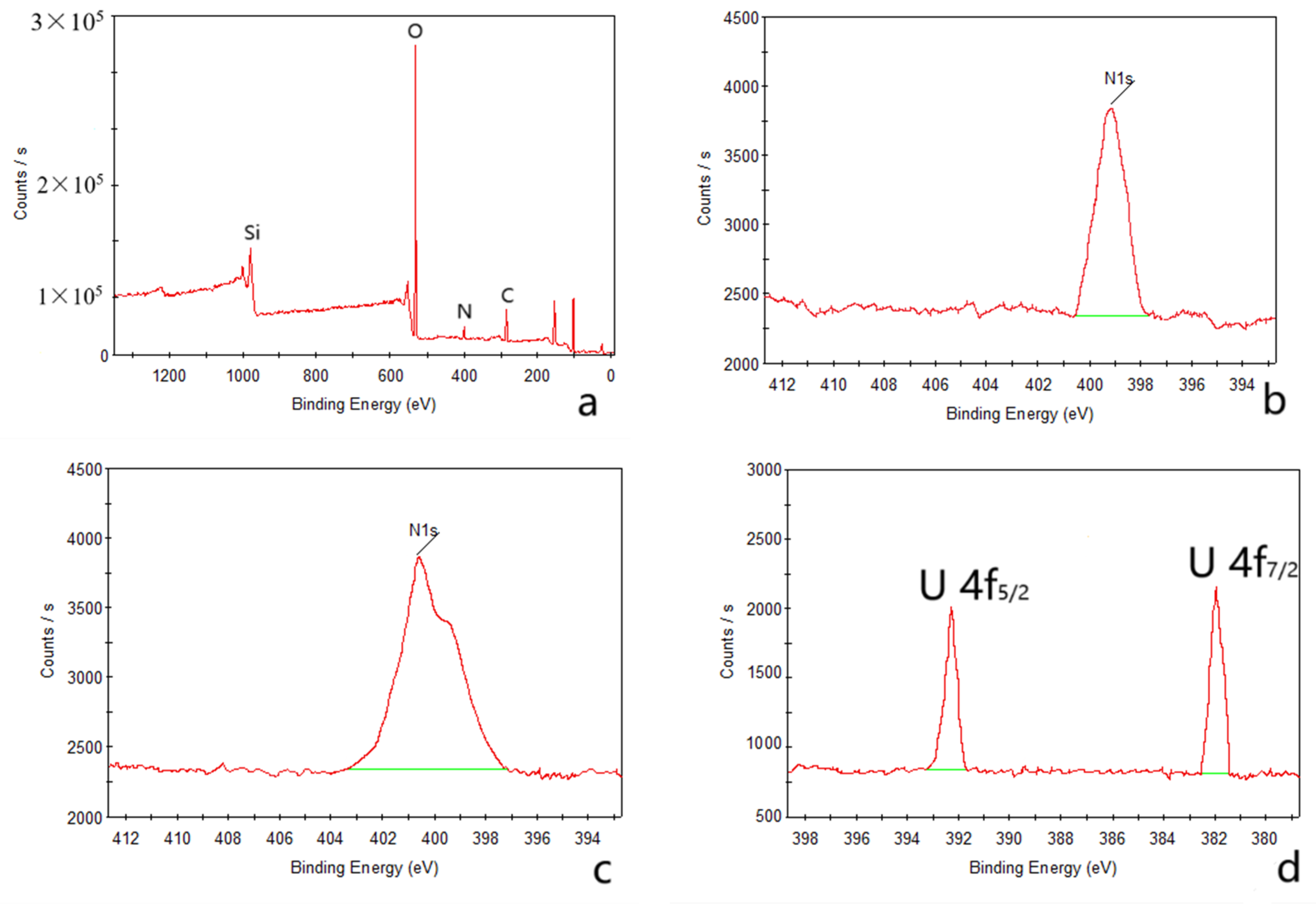
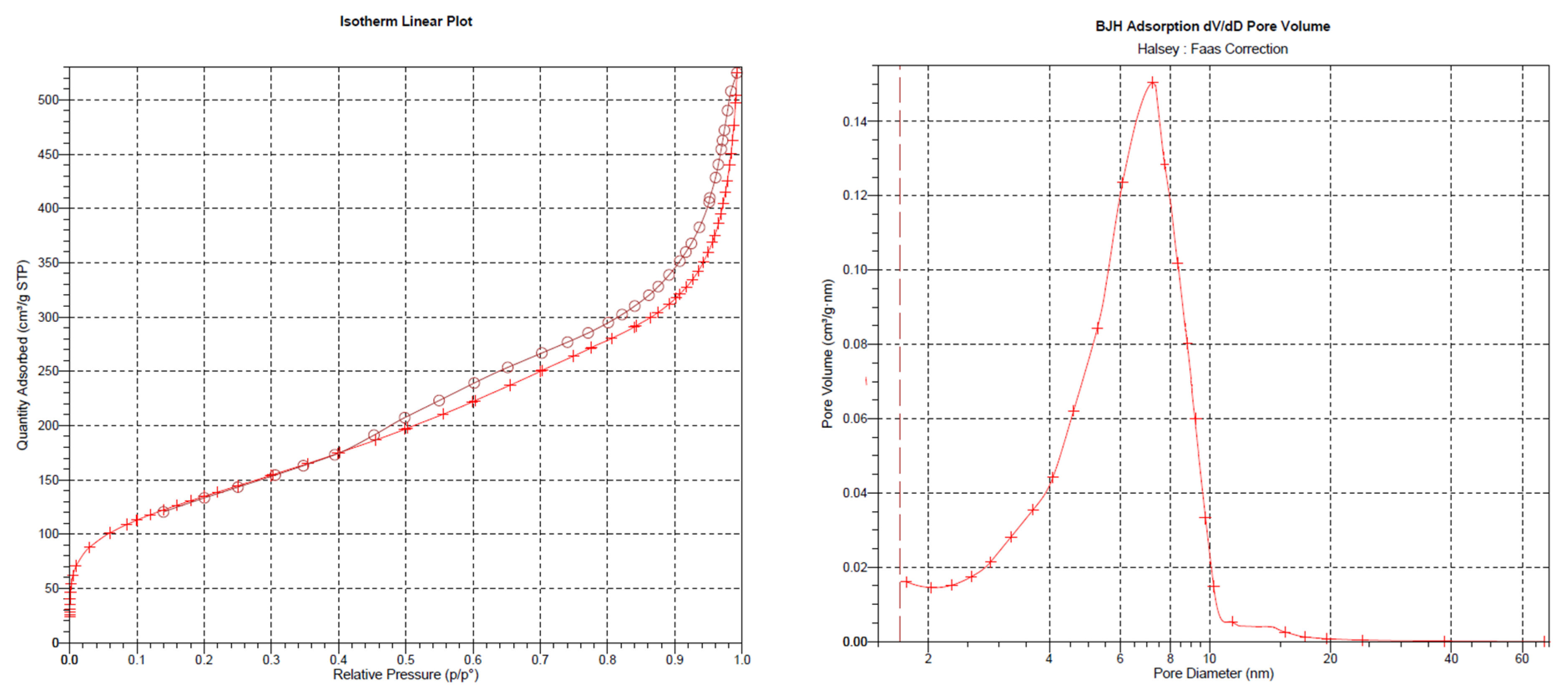
2.1.1. Physical Surface Characterization
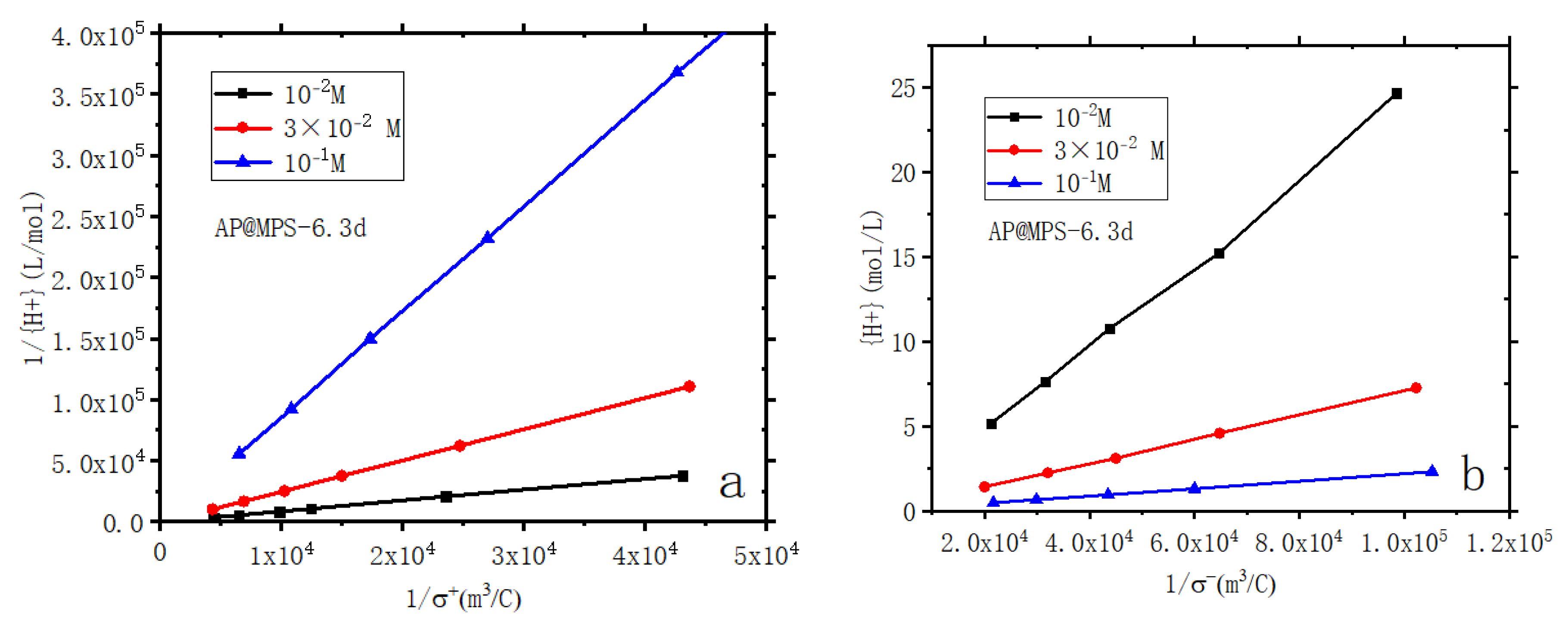
2.1.2. Surface Electrical Properties
2.2. Studied Adsorption Parameters: Equilibrium Time and Total Pore Volume
2.2.1. Equilibrium Time
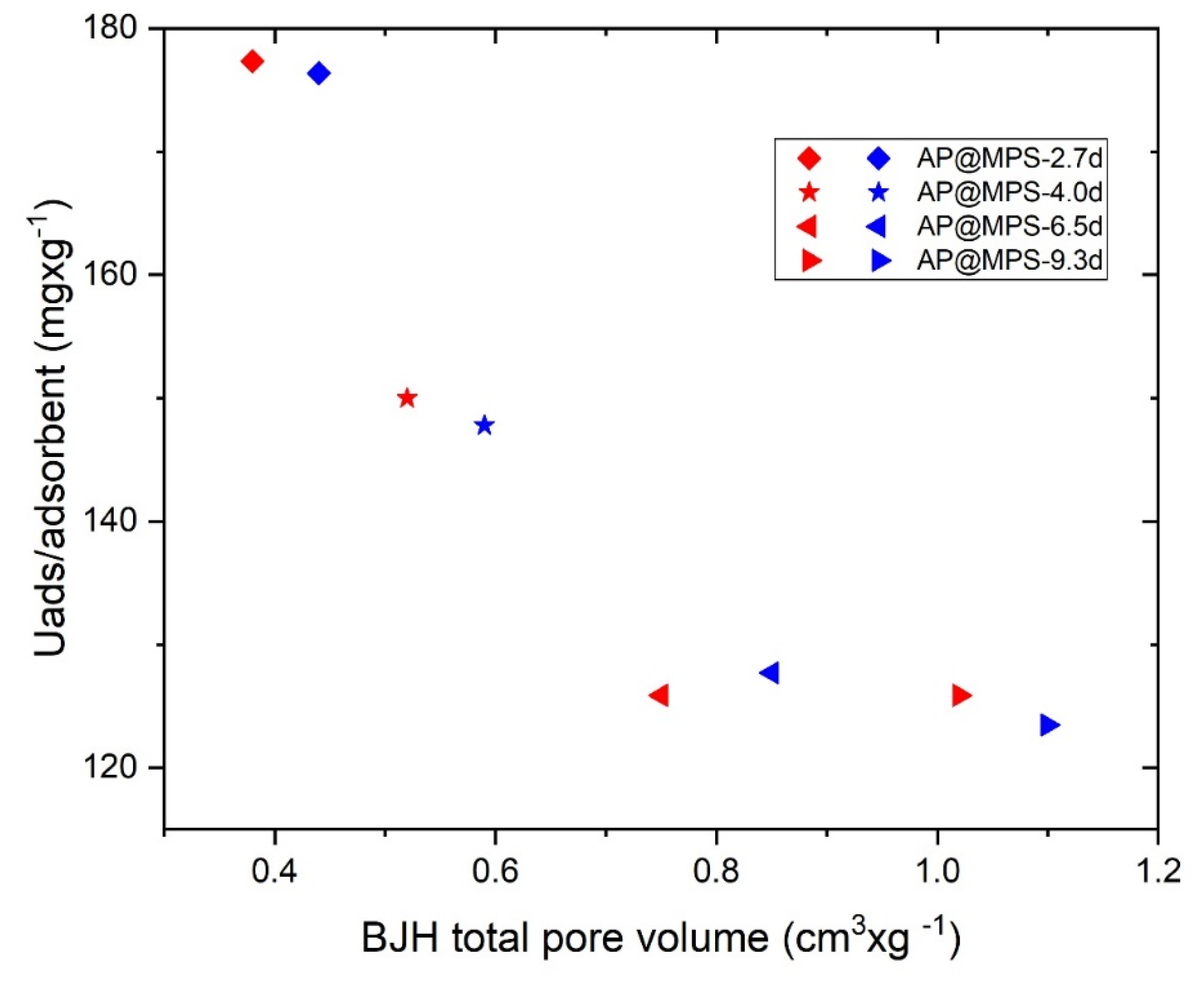
2.2.2. Total Pore Volume vs. U(VI) Capacity
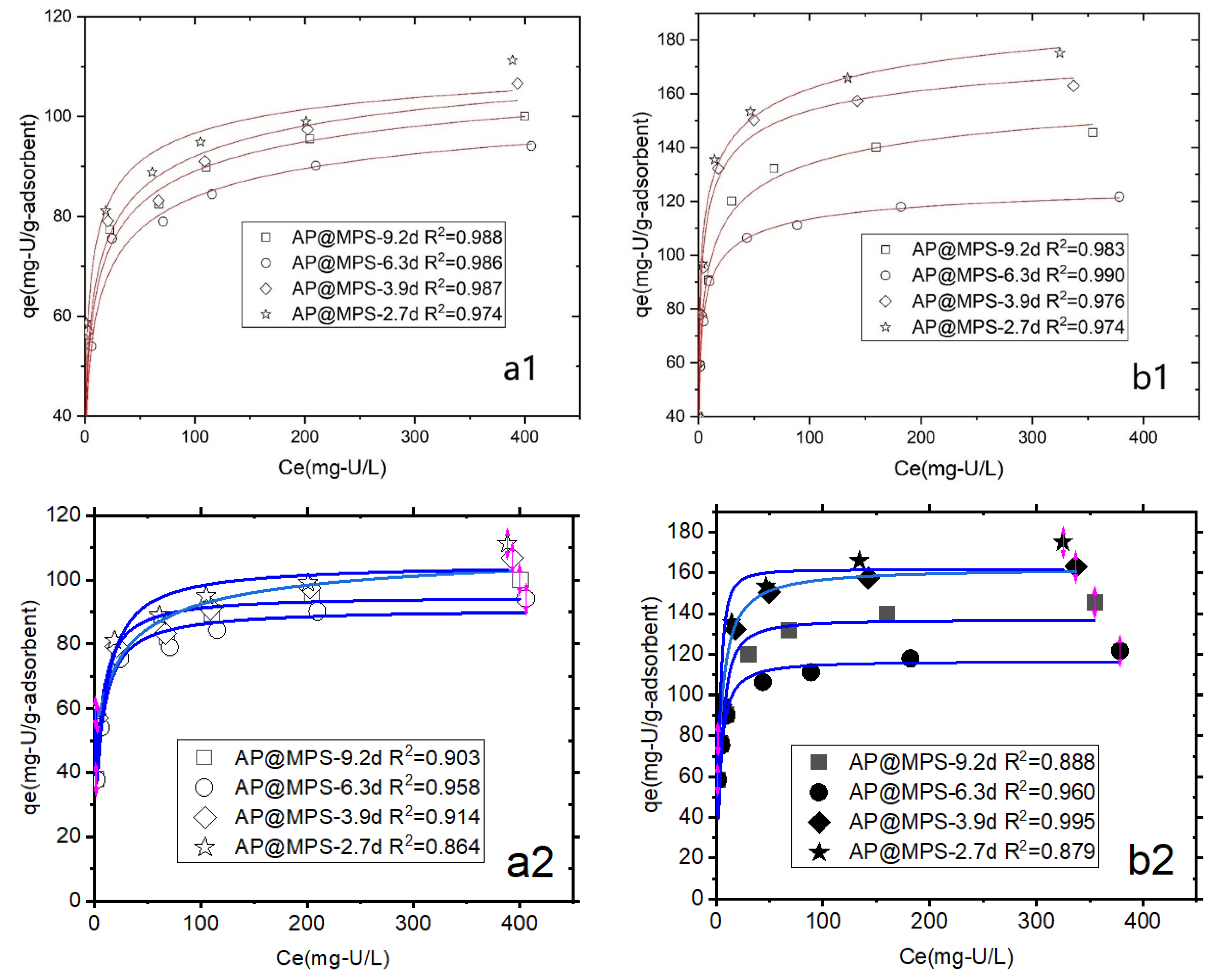
2.3. Adsorption Modeling, Average Pore Size, and Functional Groups
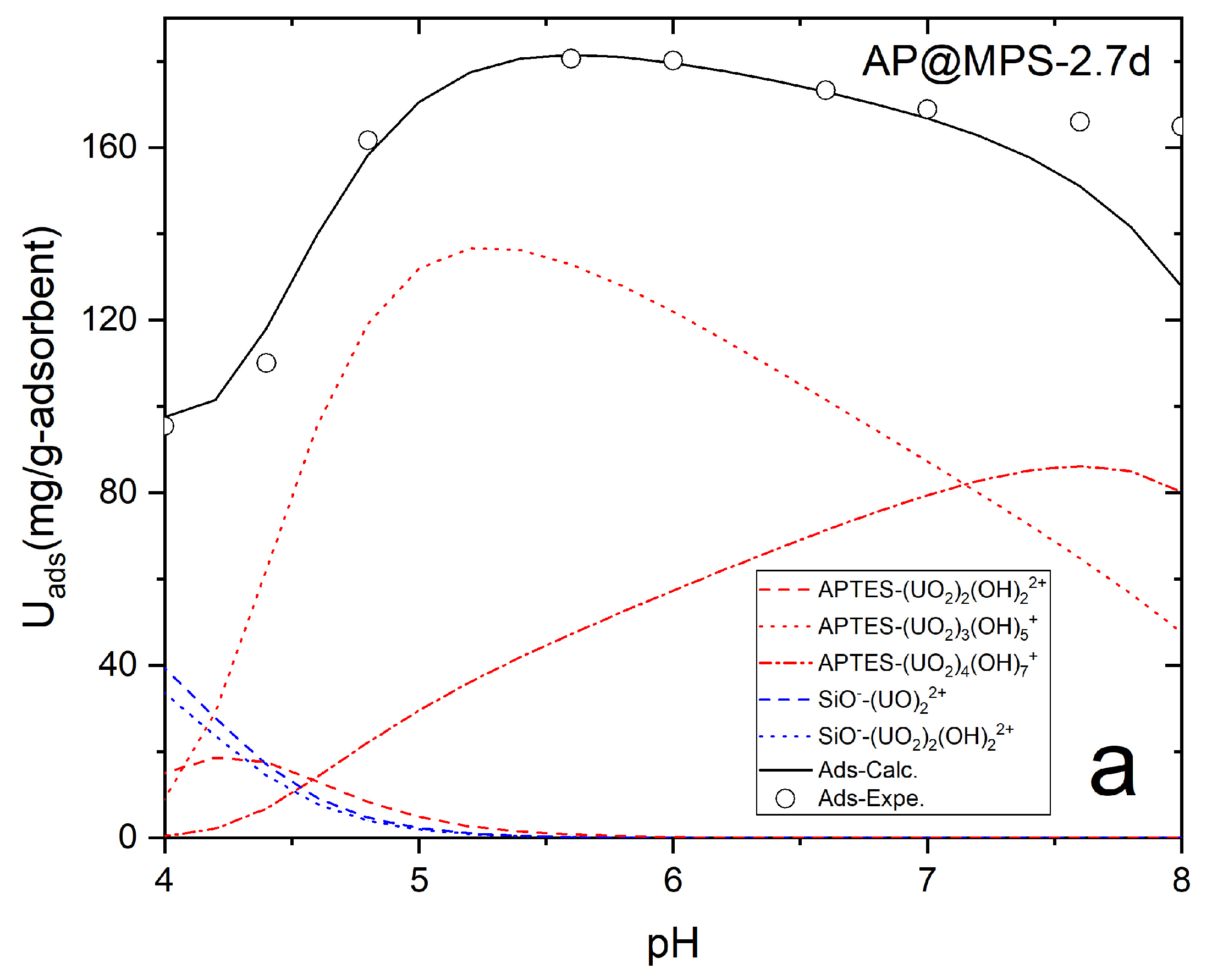
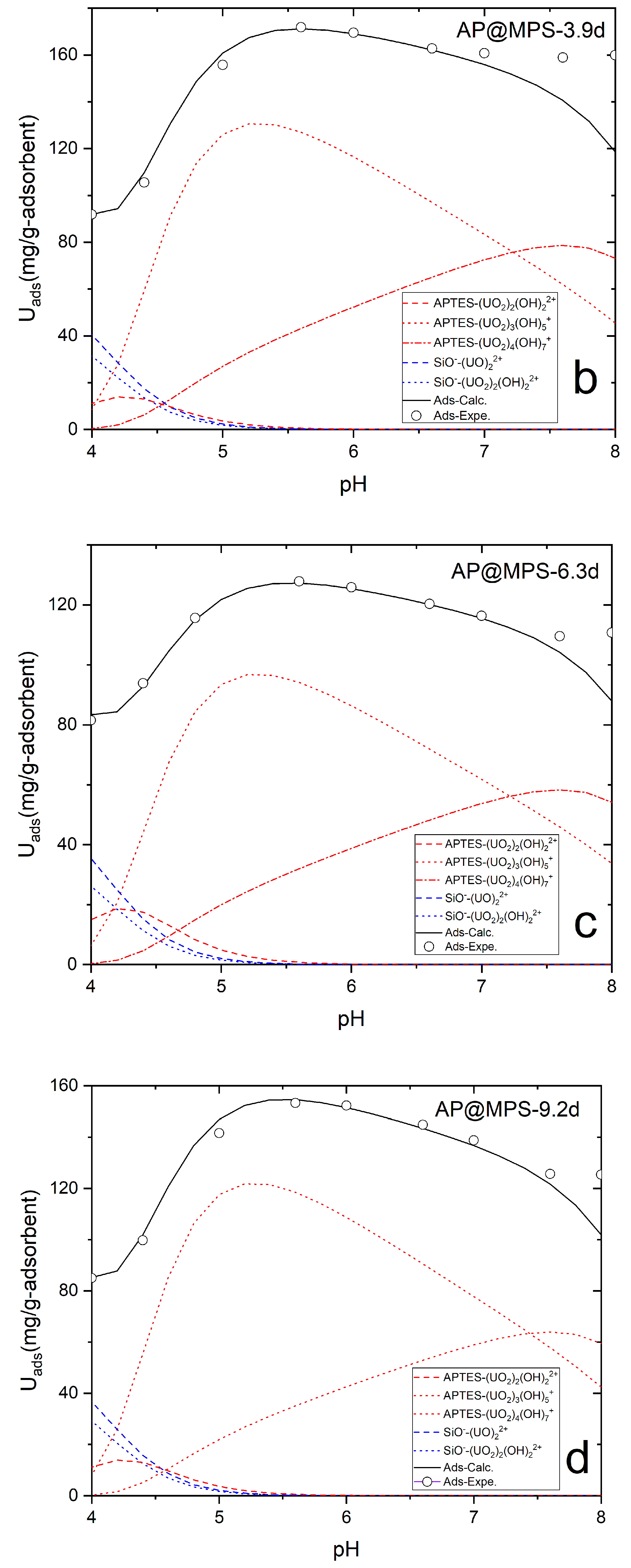
2.4. PMF and SCFM
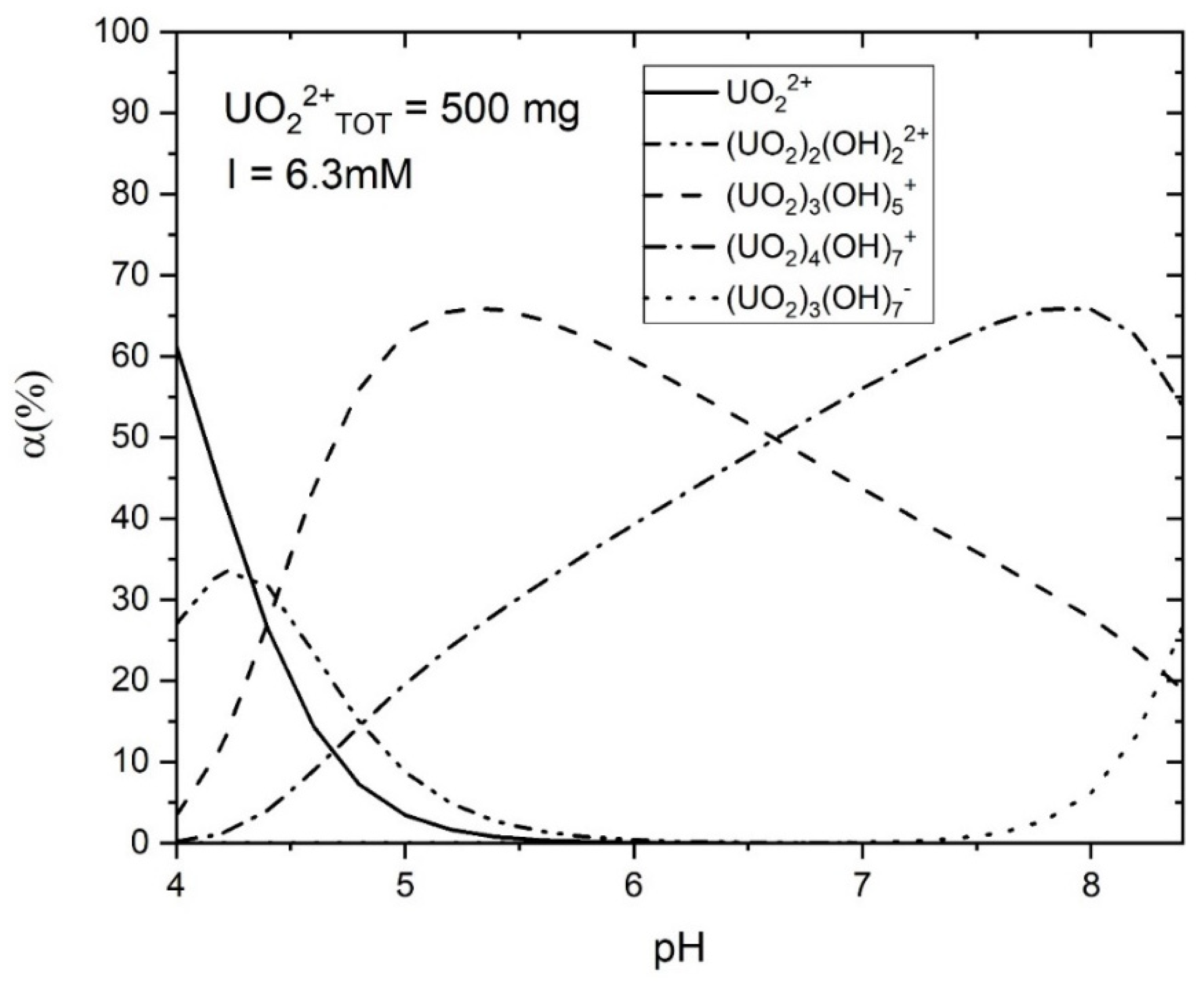
2.5. The Mechanism of AP@MPS Adsorbing U(VI)
3. Materials and Methods
3.1. Chemicals
3.2. Synthesis of MPS and AP@MPS
3.2.1. MPS Synthesis
3.2.2. AP@MPS Synthesis
3.3. Surface Properties
3.3.1. Images and Element Compositions of Samples
3.3.2. Brunauer−Emmett−Teller (BET) and Barrett−Joyner−Halenda (BJH) Analysis
3.3.3. The electrical Properties of Samples
3.4. Experimental Conditions
3.4.1. U(VI) Solution Preparation
3.4.2. Analysis of [U(VI)]
3.4.3. Duration of Reaching Equilibrium
3.4.4. The Total Pore Volume of the Adsorbents vs. the Volume of U(VI) Adsorbed
3.4.5. The Average Pore Size of the Adsorbents vs. the Mass of U(VI) Adsorbed
3.4.6. Surface Electrical Properties
3.5. Adsorption Experiments
Langmuir and Freundlich Thermodynamic Isotherms
3.6. The Speciation Diagram of [U(VI)]
3.7. The PMF Analysis
4. Comparison and Discussion
4.1. The Beta Study
4.2. The Parallel Study
4.3. Discussion
4.3.1. Insights from the Beta Study
4.3.2. Insights from the Parallel Study
4.3.3. Findings from the Current Study
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Yang, S.; Qian, J.; Kuang, L.; Hua, D. Ion-imprinted mesoporous silica for selective removal of uranium from highly acidic and radioactive effluent. ACS Appl. Mater. Interfaces 2017, 9, 29337–29344. [Google Scholar] [CrossRef]
- Khan, A.; Xing, J.; Elseman, A.M.; Gu, P.; Gul, K.; Ai, Y.; Jehan, R.; Alsaedi, A.; Hayat, T.; Wang, X. A novel magnetite nanorod-decorated Si-Schiff base complex for efficient immobilization of U (VI) and Pb (II) from water solutions. Dalton Trans. 2018, 47, 11327–11336. [Google Scholar] [CrossRef]
- Gunathilake, C.; Górka, J.; Dai, S.; Jaroniec, M. Amidoxime-modified mesoporous silica for uranium adsorption under seawater conditions. J. Mater. Chem. A 2015, 3, 11650–11659. [Google Scholar] [CrossRef]
- Le Nedelec, T.; Charlot, A.; Calard, F.; Cuer, F.; Leydier, A.; Grandjean, A. Uranium adsorption from sulfuric acid media using silica materials functionalized with amide and phosphorous ligands. New J. Chem. 2018, 42, 14300–14307. [Google Scholar] [CrossRef]
- Huang, Y.; Zheng, H.; Li, H.; Zhao, C.; Zhao, R.; Li, S. Highly selective uranium adsorption on 2-phosphonobutane-1,2,4-tricarboxylic acid-decorated chitosan-coated magnetic silica nanoparticles. Chem. Eng. J. 2020, 388, 124349. [Google Scholar] [CrossRef]
- Piechowicz, M.; Abney, C.W.; Thacker, N.C.; Gilhula, J.C.; Wang, Y.; Veroneau, S.S.; Hu, A.; Lin, W. Successful coupling of a bis-amidoxime uranophile with a hydrophilic backbone for selective uranium sequestration. ACS Appl. Mater. Interfaces 2017, 9, 27894–27904. [Google Scholar] [CrossRef] [PubMed]
- Zhu, J.; Liu, Q.; Li, Z.; Liu, J.; Zhang, H.; Li, R.; Wang, J. Efficient extraction of uranium from aqueous solution using an amino-functionalized magnetic titanate nanotubes. J. Hazard. Mater. 2018, 353, 9–17. [Google Scholar] [CrossRef]
- Huynh, J.; Palacio, R.; Safizadeh, F.; Lefèvre, G.; Descostes, M.; Eloy, L.; Guignard, N.; Rousseau, J.; Royer, S.; Tertre, E.; et al. Adsorption of uranium over NH2-functionalized ordered silica in aqueous solutions. ACS Appl. Mater. Interfaces 2017, 9, 15672–15684. [Google Scholar] [CrossRef]
- Najafi, M.; Yousefi, Y.; Rafati, A. Synthesis, characterization and adsorption studies of several heavy metal ions on amino-functionalized silica nano hollow sphere and silica gel. Sep. Purif. Technol. 2012, 85, 193–205. [Google Scholar] [CrossRef]
- Liu, Y.; Yuan, L.; Yuan, Y.; Lan, J.; Li, Z.; Feng, Y.; Zhao, Y.; Chai, Z.; Shi, W. A high efficient sorption of U(VI) from aqueous solution using amino-functionalized SBA-15. J. Radioanal. Nucl. Chem. 2011, 292, 803–810. [Google Scholar] [CrossRef]
- Wei, K.; Wang, Q.; Huang, L.; Sun, L. Amino-functionalized urea–formaldehyde framework mesoporous silica for u(vi) adsorption in wastewater treatment. Ind. Eng. Chem. Res. 2016, 55, 12420–12429. [Google Scholar] [CrossRef]
- Vukovic, S.; Watson, L.A.; Kang, S.O.; Custelcean, R.; Hay, B.P. How amidoximate binds the uranyl cation. Inorg. Chem. 2012, 51, 3855–3859. [Google Scholar] [CrossRef] [PubMed]
- Li, L.; Ma, W.; Shen, S.; Huang, H.; Bai, Y.; Liu, H. A combined experimental and theoretical study on the extraction of uranium by amino-derived metal–organic frameworks through post-synthetic strategy. ACS Appl. Mater. Interfaces 2016, 8, 31032–31041. [Google Scholar] [CrossRef] [PubMed]
- Abney, C.W.; Mayes, R.T.; Piechowicz, M.; Lin, Z.; Bryantsev, V.S.; Veith, G.M.; Dai, S.; Lin, W. XAFS investigation of polyamidoxime-bound uranyl contests the paradigm from small molecule studies. Energy Environ. Sci. 2016, 9, 448–453. [Google Scholar] [CrossRef]
- Comarmond, M.J.; Payne, T.E.; Harrison, J.J.; Thiruvoth, S.; Wong, H.K.; Aughterson, R.D.; Lumpkin, G.R.; Müller, K.; Foerstendorf, H. Uranium sorption on various forms of titanium dioxide–influence of surface area, surface charge, and impurities. Environ. Sci. Technol. 2011, 45, 5536–5542. [Google Scholar] [CrossRef]
- Stumm, W. Chemistry of the Solid-Water Interface: Processes at the Mineral-Water and Particle-Water Interface in Natural Systems; John Wiley & Son Inc.: Hoboken, NJ, USA, 1992. [Google Scholar]
- Hao, Z.; Guo, B.; Liu, H.; Gan, L.; Xu, Z.; Chen, L. Synthesis of mesoporous silica using urea–formaldehyde resin as an active template. Microporous Mesoporous Mater. 2006, 95, 350–359. [Google Scholar] [CrossRef]
- Wei, K.; Wang, Q.; Huang, C.-P. The distribution of adsorption energy of U(VI) onto AEPTES-functionalized porous silica with multiple average pore sizes. Chem. Eng. J. 2023, 451, 138716. [Google Scholar] [CrossRef]
- Qiu, H.; Lv, L.; Pan, B.-C.; Zhang, Q.-J.; Zhang, W.-M.; Zhang, Q.-X. Critical review in adsorption kinetic models. J. Zhejiang Univ. A 2009, 10, 716–724. [Google Scholar] [CrossRef]
- Gupta, S.S.; Bhattacharyya, K.G. Kinetics of adsorption of metal ions on inorganic materials: A review. Adv. Colloid Interface Sci. 2011, 162, 39–58. [Google Scholar] [CrossRef]
- James, R.O.; Healy, T.W. Adsorption of hydrolyzable metal ions at the oxide—Water interface. I. Co(II) adsorption on SiO2 and TiO2 as model systems. J. Colloid Interface Sci. 1972, 40, 42–52. [Google Scholar] [CrossRef]
- Park, S.; Huang, C. The adsorption characteristics of some heavy metal ions onto hydrous CdS(s) surface. J. Colloid Interface Sci. 1989, 128, 245–257. [Google Scholar] [CrossRef]
- Weng, C.-H.; Huang, C.P.; Allen, H.E.; Sanders, P.F. Cr(VI) adsorption onto hydrous concrete particles from groundwater. J. Environ. Eng. 2001, 127, 1124–1131. [Google Scholar] [CrossRef]
- Kerisit, S.; Liu, C. Structure, kinetics, and thermodynamics of the aqueous uranyl (VI) cation. J. Phys. Chem. A 2013, 117, 6421–6432. [Google Scholar] [CrossRef]
- Antonchenko, V.Y.; Kryachko, E.S. Interaction of uranyl ion with few molecules of water: Thought (computational) scenarios with hydrogen bonding motif. Theor. Chem. Acc. 2008, 120, 421–428. [Google Scholar] [CrossRef]
- Wang, Q.; Wei, K.; Zhang, Q.; Huang, L.; Hu, E.; Xu, N. Study of SiO2 colloids polymer for uranium(VI) absorption in water solution. J. Nanhua University. Sci. Technol. 2016, 30, 11–15. [Google Scholar]
- Muegge, I.; Martin, Y.C. A general and fast scoring function for protein− ligand interactions: A simplified potential approach. J. Med. Chem. 1999, 42, 791–804. [Google Scholar] [CrossRef]
- Muegge, I.; Martin, Y.C.; Hajduk, P.J.; Fesik, S.W. Evaluation of PMF scoring in docking weak ligands to the FK506 binding protein. J. Med. Chem. 1999, 42, 2498–2503. [Google Scholar] [CrossRef]
- Kovalenko, A.; Hirata, F. Potential of mean force between two molecular ions in a polar molecular solvent: A study by the three-dimensional reference interaction site model. J. Phys. Chem. B 1999, 103, 7942–7957. [Google Scholar] [CrossRef]
- Muegge, I. PMF scoring revisited. J. Med. Chem. 2006, 49, 5895–5902. [Google Scholar] [CrossRef]
- Trzesniak, D.; Kunz, A.E.; van Gunsteren, W.F. A comparison of methods to compute the potential of mean force. Chemphyschem 2006, 8, 162–169. [Google Scholar] [CrossRef] [PubMed]
- Nalaparaju, A.; Jiang, J. Ion exchange in metal-organic framework for water purification: Insight from molecular simulation. J. Phys. Chem. C 2012, 116, 6925–6931. [Google Scholar] [CrossRef]
- Torrie, G.M.; Valleau, J.P. Nonphysical sampling distributions in Monte Carlo free-energy estimation: Umbrella sampling. J. Comput. Phys. 1977, 23, 187–199. [Google Scholar] [CrossRef]
- Kumar, S.; Rosenberg, J.M.; Bouzida, D.; Swendsen, R.H.; Kollman, P.A. The weighted histogram analysis method for free-energy calculations on biomolecules. I. The method. J. Comput. Chem. 1992, 13, 1011–1021. [Google Scholar] [CrossRef]
- Tian, Y.; Liu, L.; Ma, F.; Zhu, X.; Dong, H.; Zhang, C.; Zhao, F. Synthesis of phosphorylated hyper-cross-linked polymers and their efficient uranium adsorption in water. J. Hazard. Mater. 2021, 419, 126538. [Google Scholar] [CrossRef] [PubMed]
- Sang, K.; Wang, Y.; Wang, Y.; Liu, L.; Mei, D.; Zhang, C.; Zhang, S.; Ma, F.; Dong, H. Hypercrosslinked phenylalaninol for efficient uranium adsorption from water. Sep. Purif. Technol. 2023, 305, 122292. [Google Scholar] [CrossRef]
- Zhao, M.; Cui, Z.; Pan, D.; Fan, F.; Tang, J.; Hu, Y.; Xu, Y.; Zhang, P.; Li, P.; Kong, X.Y.; et al. An efficient uranium adsorption magnetic platform based on amidoxime-functionalized flower-like Fe3O4@TiO2 core–shell microspheres. ACS Appl. Mater. Interfaces 2021, 13, 17931–17939. [Google Scholar] [CrossRef]
- Zhu, M.; Liu, L.; Feng, J.; Dong, H.; Zhang, C.; Ma, F.; Wang, Q. Efficient uranium adsorption by amidoximized porous polyacrylonitrile with hierarchical pore structure prepared by freeze-extraction. J. Mol. Liq. 2021, 328, 115304. [Google Scholar] [CrossRef]
- Chen, Z.; Liang, Y.; Jia, D.; Chen, W.; Cui, Z.; Wang, X. Layered silicate RUB-15 for efficient removal of UO22+ and heavy metal ions by ion exchange. Environ. Sci. Nano 2017, 4, 1851–1858. [Google Scholar] [CrossRef]
- Liu, R.; Zhang, W.; Chen, Y.; Wang, Y. Uranium (VI) adsorption by copper and copper/iron bimetallic central MOFs. Colloids Surf. A Physicochem. Eng. Asp. 2020, 587, 124334. [Google Scholar] [CrossRef]








| TEOS | Tetraethylorthosilicate |
|---|---|
| APTES | (3-aminopropyl)trimethoxysilane |
| AP@MPS | (3-aminopropyl)trimethoxysilane-functionalized porous silica |
| AP@MPS-2.7d | AP@MPS with average pore size of 2.7 nm and BET area of 380 m2·g −1 |
| AP@MPS-3.9d | AP@MPS with average pore size of 3.9 nm and BET area of 340 m2·g −1 |
| AP@MPS-6.3d | AP@MPS with average pore size of 6.3 nm and BET area of 170 m2·g −1 |
| AP@MPS-9.2d | AP@MPS with average pore size of 9.2 nm and BET area of 240 m2·g −1 |
| SCFM | Surface Complex Formation Model |
| PMF | Potential of Mean Force |
| WHAM | Weighted Histogram Analysis Method |
| Adsorbent | BET Surface Area (m2·g −1) | BJH Average Pore Diameter (nm) |
|---|---|---|
| AP@MPS-9.3d | 170 | 9.2 |
| AP@MPS-6.3d | 240 | 6.3 |
| AP@MPS-3.9d | 340 | 3.9 |
| AP@MPS-2.7d | 380 | 2.7 |
| Adsorbent | Surface Area m2·g−1 | Pore Diameter nm | {X−} mM·L− | {X+} mM·L−1 | |
|---|---|---|---|---|---|
| AP@MPS | X+: Uads Max | ||||
| AP@MPS-9.2d | 240 | 9.2 | 0.81 | 1.25 | 1:0.368 |
| AP@MPS-6.3d | 170 | 6.3 | 1.01 | 1.13 | 1:0.370 |
| AP@MPS-3.9d | 340 | 3.9 | 0.98 | 1.26 | 1:0.436 |
| AP@MPS-2.7d | 380 | 2.7 | 1.04 | 1.23 | 1:0.546 |
| Equation | ΔG0ads | ΔG0chem | ΔG0lat | ΔG0coul | ΔG0solv |
|---|---|---|---|---|---|
| APTES+(UO2)3(OH)5+ | −16.32 ± 0.1 | −2.35 ± 0.1 | 0 | −14.21 ± 0.1 | 0.24 ± 0.1 |
| APTES+(UO2)4(OH)7+ | −15.58 ± 0.1 | −1.61 ± 0.1 | 0 | −14.21 ± 0.1 | 0.24 ± 0.1 |
| APTES+(UO2)2(OH)22+ | −12.10 ± 0.1 | 16.09 ± 0.1 | 0 | −28.43 ± 0.1 | 0.24 ± 0.1 |
| SiO− + UO22+ | −45.53 ± 0.2 | −17.34 ± 0.2 | 0 | −28.43 ± 0.2 | 0.24 ± 0.2 |
| SiO− + (UO2)2(OH)22+ | −44.96 ± 0.2 | −16.77 ± 0.2 | 0 | −28.43 ± 0.2 | 0.24 ± 0.2 |
| Adsorbent | Amino Groups | Silica Groups | |||
|---|---|---|---|---|---|
| pKs(UO2)3(OH)5+ | pKs(UO2)4(OH)7+ | pKs(UO2)2(OH)22+ | pKs(UO2)2(OH)22+ | pKsUO22+ | |
| AP@MPS-9.2d | −2.86 | −2.73 | −2.12 | −7.98 | −7.88 |
| AP@MPS-6.3d | −2.76 | −2.69 | −2.25 | −8.01 | −7.88 |
| AP@MPS-3.9d | −2.89 | −2.82 | −2.12 | −8.02 | −7.91 |
| AP@MPS-2.7d | −2.91 | −2.86 | −2.25 | −8.02 | −7.95 |
| Sample | I (M) | σN (C·m−2) | −σSi (C·m−2) | {XN+} (mM·L−1) | {XSi−} (mM·L−1) | pKintN | pKintsi | pHPZC |
|---|---|---|---|---|---|---|---|---|
| AP@MPS-2.7d | 10−1 | 0.315 | 0.259 | 1.24 | 1.02 | 6.69 | 4.95 | 5.82 |
| 3 × 10−2 | 0.297 | 0.269 | 1.17 | 1.06 | ||||
| 10−2 | 0.317 | 0.264 | 1.25 | 1.04 | ||||
| AP@MPS-3.9d | 10−1 | 0.352 | 0.278 | 1.24 | 0.98 | 6.70 | 4.96 | 5.83 |
| 3 × 10−2 | 0.355 | 0.278 | 1.25 | 0.98 | ||||
| 10−2 | 0.358 | 0.275 | 1.26 | 0.97 | ||||
| AP@MPS-6.3d | 10−1 | 0.631 | 0.580 | 1.11 | 1.02 | 6.63 | 4.97 | 5.80 |
| 3 × 10−2 | 0.652 | 0.571 | 1.15 | 1.01 | ||||
| 10−2 | 0.633 | 0.591 | 1.12 | 1.04 | ||||
| AP@MPS-9.2d | 10−1 | 0.511 | 0.326 | 1.27 | 0.81 | 6.66 | 4.94 | 5.80 |
| 3 × 10−2 | 0.503 | 0.322 | 1.25 | 0.80 | ||||
| 10−2 | 0.490 | 0.323 | 1.22 | 0.83 |
| No. | Material | Experiment Conditions | Performance | Ref. |
|---|---|---|---|---|
| 1 | AEPTES-functionalized porous silica | pH0 = 6.5; [U]0 = 600 mg × L−1; [X] = 1 g × L−1; T = 25 °C | Γm = 551.97 mg-U/g; R = 92% @ 0.5 h | [18] |
| 2 | Phosphorylated hyper-cross-linked polymers | pH0 = 7; [U]0 = 60 mg × L−1; [X] = 0.2 g × L−1; T = 25 °C | Γm = 297.14 mg-U/g; R = 85% @ 0.08 h | [35] |
| 3 | Hypercrosslinked phenylalaninol | pH0 = 7; [U]0 = 100 mg × L−1; | Γm = 369.5 mg-U/g; R = 56.3% @ 2 h | [36] |
| 4 | Amidoxime-functionalized Fe3O4@TiO2 microspheres | pH0 = 6; [U]0 = 23.8 mg × L−1; [X] = 0.2 g × L−1; T = 25 °C | Γm = 313.6 mg-U/g; @ 12 h | [37] |
| 5 | Amidoximized porous polyacrylonitrile | pH0 = 6; [U]0 = 117 mg × L−1; [X] = 0.1 g × L−1; T = 25 °C | Γm = 1058 mg-U/g; R = 90.50% @ 5 h | [38] |
| 6 | Layered silicate RUB-15 | pH0 = 6; [U]0 = 20 mg × L−1; [X] = 0.5 g × L−1; T = 25 °C | Γm = 152 mg-U/g; R = 74% @ 12 h | [39] |
| 7 | Amide and phosphorous functionalized silica | pH0 = 1.7; [U]0 = 120 mg × L−1; [X] = 5 g × L−1 | Γm = 95 mg-U × gads−1; R = 40% @ 24 h | [4] |
| 8 | Ion-imprinted mesoporous silica | [U]0 = 1.68 mM; pH0 = 0; [X] = 1 g × L−1; T = 25 °C | Γm = 80 mg-U × gads−1; R = 20% @ 0.67 h | [1] |
| 9 | Amino-functionalized magnetic titanate nanotubes | pH0 = 6; [U]0 = 200 mg × L−1; [X] = 0.4 g × L−1; T = 25 °C | Γm = 509.89 mg-U × gads−1; R = 83% @ 0.83 h | [7] |
| 10 | Copper bimetallic central MOFs | pH0 = 3; [U]0 = 30 mg × L−1; T = 25 °C | Γm = 617.814 mg-U × gads−1@ 12 h | [40] |
| 11 | Copper/iron bimetallic central MOFs | pH0 = 7; [U]0 = 60 mg × L−1; T = 25 °C | Γm = 354.724 mg-U × gads−1@ 12 h | [40] |
| 12 | APTES-functionalized porous silica | pH0 = 6.5; [U]0 = 600 mg × L−1; [X] = 1 g × L−1; T = 25 °C | Γm = 381.44 mg-U/g; R = 63.6 % @ 0.5 h | present work |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wei, K.; Huang, C.-P. Analyzing (3-Aminopropyl)triethoxysilane-Functionalized Porous Silica for Aqueous Uranium Removal: A Study on the Adsorption Behavior. Molecules 2024, 29, 803. https://doi.org/10.3390/molecules29040803
Wei K, Huang C-P. Analyzing (3-Aminopropyl)triethoxysilane-Functionalized Porous Silica for Aqueous Uranium Removal: A Study on the Adsorption Behavior. Molecules. 2024; 29(4):803. https://doi.org/10.3390/molecules29040803
Chicago/Turabian StyleWei, Kegang, and Chin-Pao Huang. 2024. "Analyzing (3-Aminopropyl)triethoxysilane-Functionalized Porous Silica for Aqueous Uranium Removal: A Study on the Adsorption Behavior" Molecules 29, no. 4: 803. https://doi.org/10.3390/molecules29040803
APA StyleWei, K., & Huang, C.-P. (2024). Analyzing (3-Aminopropyl)triethoxysilane-Functionalized Porous Silica for Aqueous Uranium Removal: A Study on the Adsorption Behavior. Molecules, 29(4), 803. https://doi.org/10.3390/molecules29040803







