Structure of Triangular Numbers Modulo m
Abstract
:1. Introduction
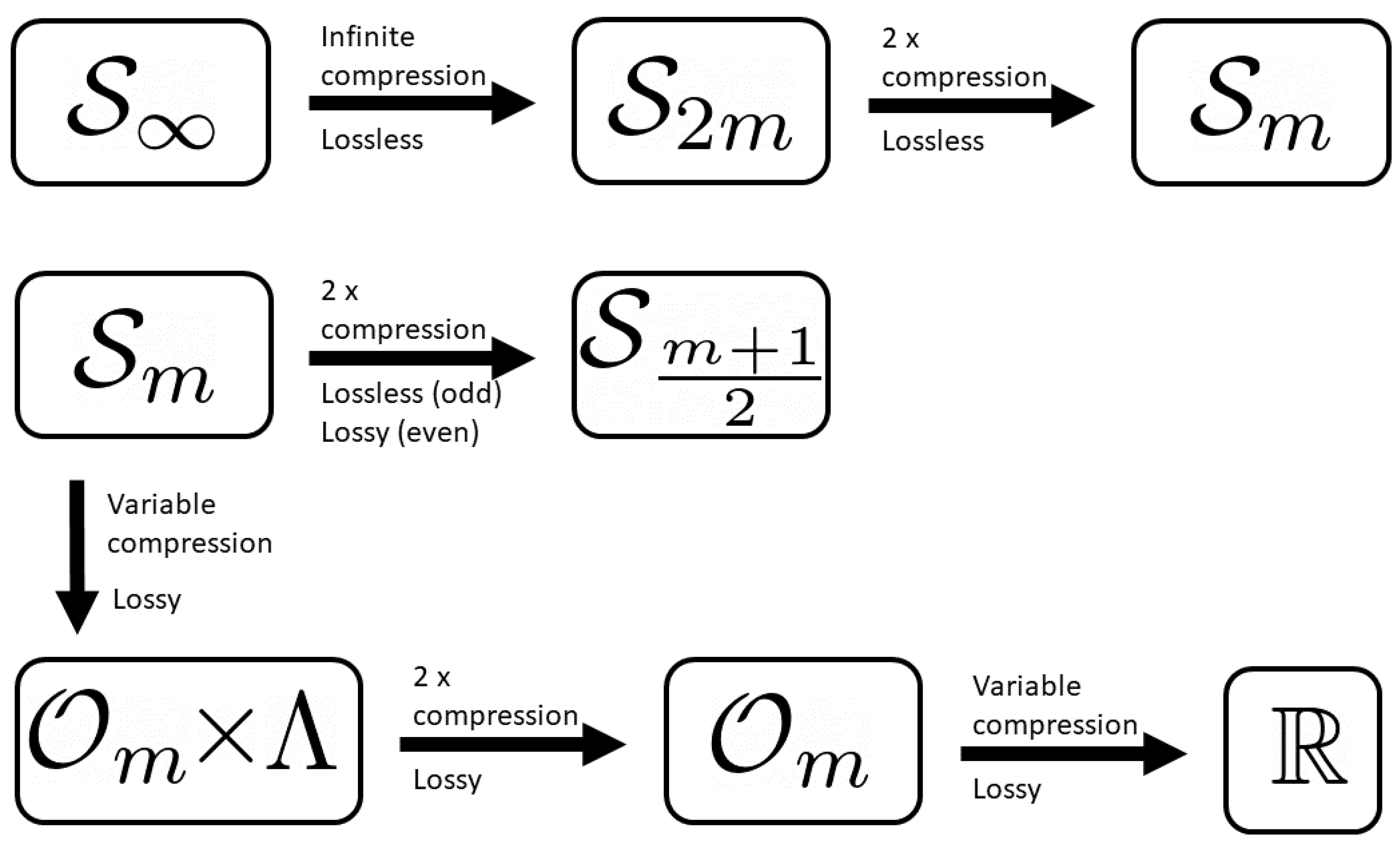
2. Information and Data Compression
2.1. Sets and Sequences
2.2. Information Content of Sets
3. Main Structure Theorems
3.1. Main Cycle Structure Theorem
3.2. Symmetry Theorems
4. Specific Structure Theorems
4.1. Theorems Related to
4.2. Theorems Involving Cases When m Is Prime
4.3. Theorems Involving Cases When m Is a Prime Squared
5. Property Theorems
6. Saturation Theorems
7. Special Sets
7.1. Monoids and Reduced Monoids
7.2. Perfect Squares and Nonsquares
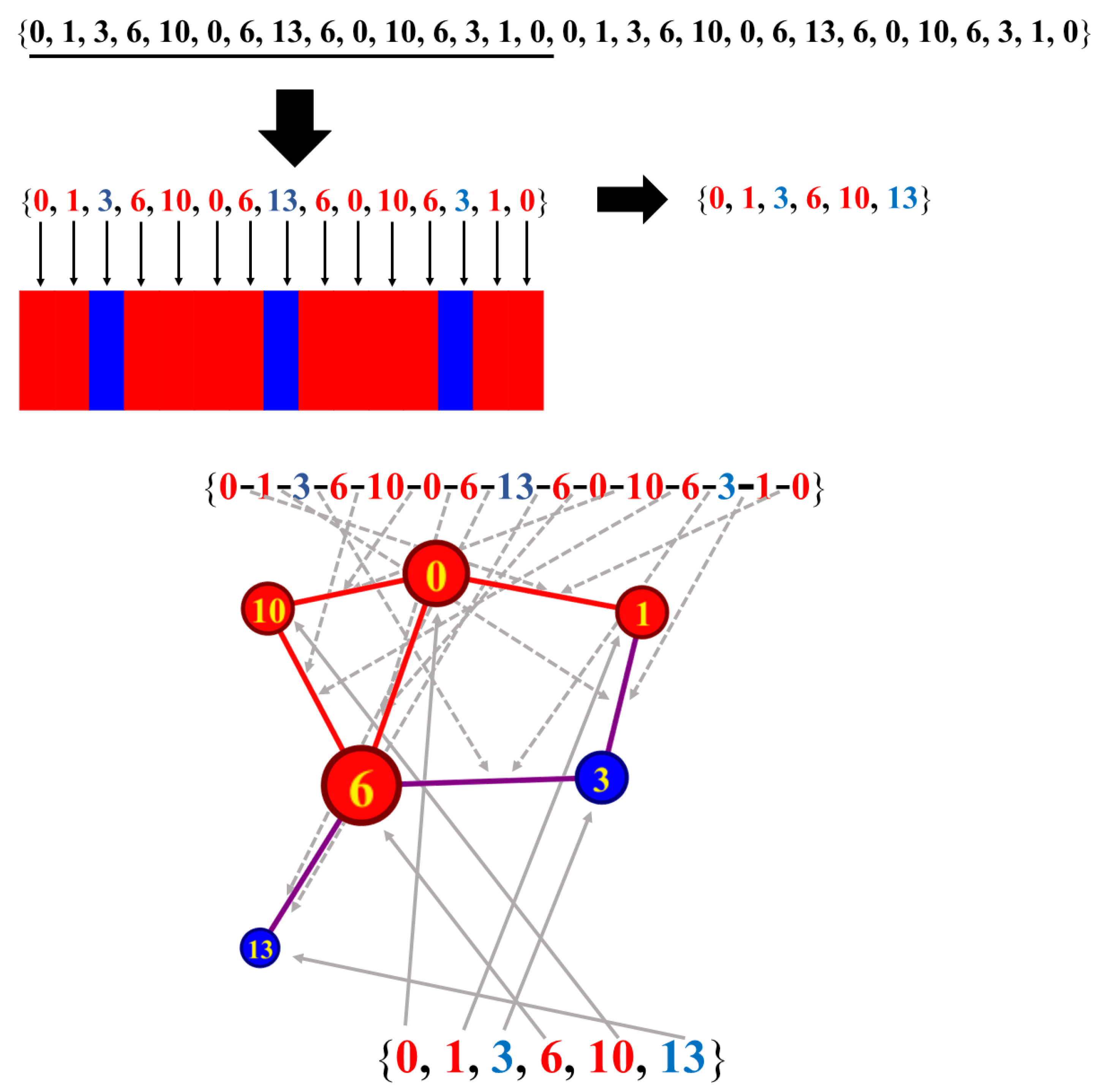
8. Graph Representation
8.1. Construction of the Graph Representation
8.2. Structure of the Graph Representation
8.3. Perfect Squares and Nonsquares in the Graph Representation
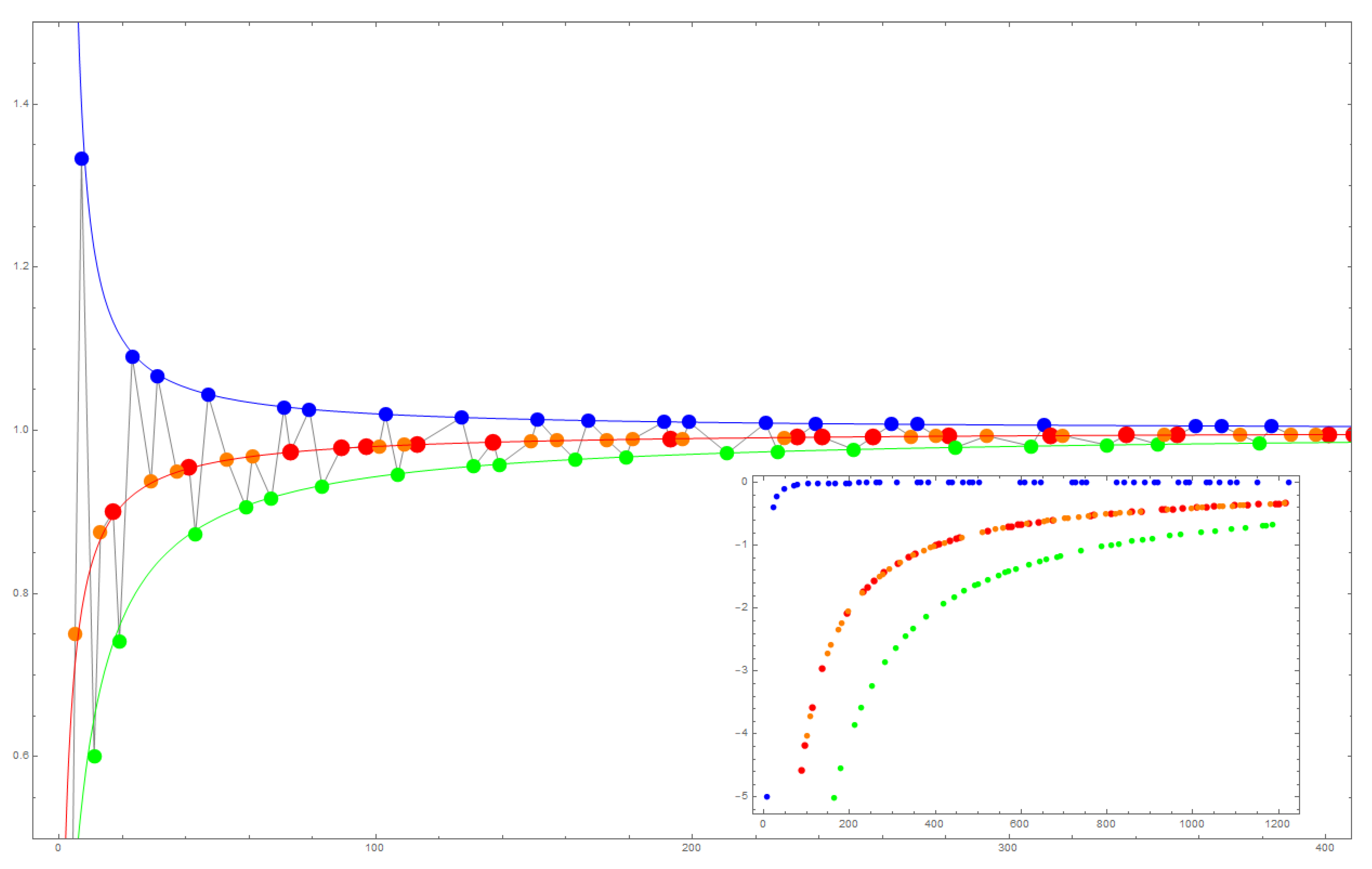
9. Self-Similarity
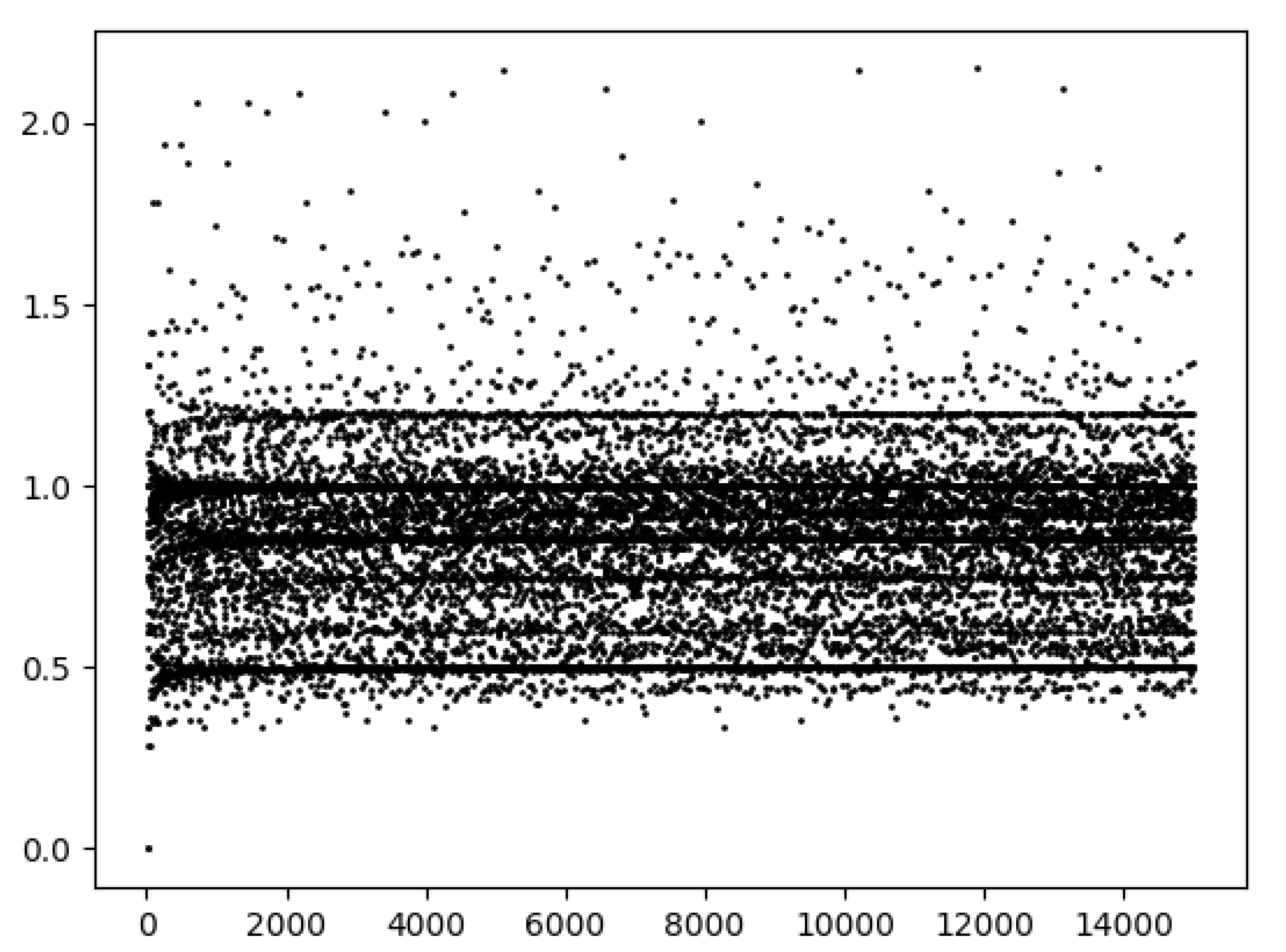
10. Scaling Properties
10.1. Sprays
10.2. Sprays, Renormalization, and Fractal Character
11. Application to Harmonic Analysis
12. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Deza, E.; Deza, M.-M. Figurate Numbers; World Scientific: Hackensack, NJ, USA, 2012. [Google Scholar]
- Hoggatt, V.E., Jr.; Bicknell, M. Triangular numbers. Fibonacci Q. 1974, 12, 221–230. [Google Scholar]
- Ono, K.; Robbins, S.; Wahl, P.T. On the representation of integers as sums of triangular numbers. Aequationes Math. 1995, 50, 73–94. [Google Scholar] [CrossRef]
- Schroeder, M.R. Number Theory in Science and Communication; Springer: Berlin/Heidelberg, Germany, 1984. [Google Scholar]
- Sun, Z.-W. On sums of primes and triangular numbers. J. Comb. Number Theory 2009, 1, 65–76. [Google Scholar]
- Wall, D.D. Fibonacci Series Modulo m. Am. Math. Mon. 1960, 67, 525–532. [Google Scholar] [CrossRef]
- Özkan, E.; Aydin, H.; Dikici, R. 3-Step Fibonacci series modulo m. Appl. Math. Comput. 2003, 143, 165–172. [Google Scholar] [CrossRef]
- Aydin, H.; Dikici, R.; Smith, G.C. Wall and Vinson Revisited. In Applications of Fibonacci Numbers; Bergum, G.E., Philippou, A.N., Horadam, A.F., Eds.; Springer: Dordrecht, The Netherlands, 1993. [Google Scholar]
- Dikici, R.; Özkan, E. An application of Fibonacci sequences in groups. Appl. Math. Comput. 2003, 136, 323–331. [Google Scholar] [CrossRef]
- Slone, N.J.A. Online Encyclopedia on Integer Sequences. Available online: https://oeis.org/ (accessed on 28 May 2022).
- Jusdon, T.W. Absract Algebra; PWS Publishing Company: Boston, MA, USA, 1994. [Google Scholar]
- Fraleigh, J.B. A First Course in Abstract Algebra, 4th ed.; Addison-Wesley: Reading, MA, USA, 1989. [Google Scholar]
- Andrews, G.E. Number Theory; Dover Publications: New York, NY, USA, 1971. [Google Scholar]
- Papoulis, A. Probability, Random Variables, and Stochastic Processes; McGraw-Hill: New York, NY, USA, 1984. [Google Scholar]
- Newman, M.E.J. The structure and function of complex networks. SIAM Rev. 2003, 45, 167–256. [Google Scholar] [CrossRef] [Green Version]
- Aigner, M. A Course in Enumeration; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Coutsias, E.A.; Kazarinoff, N.D. Disorder, renormalizability, theta functions and Cornu spirals. Phys. D Nonlinear Phenom. 1987, 26, 295–310. [Google Scholar] [CrossRef]
- Vogt, T.; Ulness, D.J. Cornu spirals and the triangular lacunary trigonometric system. Fractal Fract. 2019, 3, 40. [Google Scholar] [CrossRef] [Green Version]
- Abramowitz, M.; Stegun, I.A. Handbook of Mathematical Functions; Dover Publications: New York, NY, USA, 1972. [Google Scholar]
- Hardy, G.H. Divergent Series; AMS Chelsea Publishing: New York, NY, USA, 1991. [Google Scholar]
- Lehmer, D.H. Incomplete Gauss sums. Mathematika 1976, 23, 125–135. [Google Scholar] [CrossRef]
- Paris, R.B. An asymptotic approximation for incomplete Gauss sums. J. Comput. Appl. Math. 2008, 212, 16–30. [Google Scholar] [CrossRef] [Green Version]
- Berry, M.V.; Goldberg, J. Renormalization of curlicues. Nonlinearity 1988, 1, 1–26. [Google Scholar] [CrossRef]
- Matsumoto, M.; Nishimura, T. Mersenne twister: A 623-dimensionally equidistributed uniform pseudo-random number generator. ACM Trans. Model. Comput. Simul. 1998, 8, 3–30. [Google Scholar] [CrossRef] [Green Version]
- Jäntschi, L. Detecting Extreme Values with Order Statistics in Samples from Continuous Distributions. Mathematics 2020, 8, 216. [Google Scholar] [CrossRef] [Green Version]




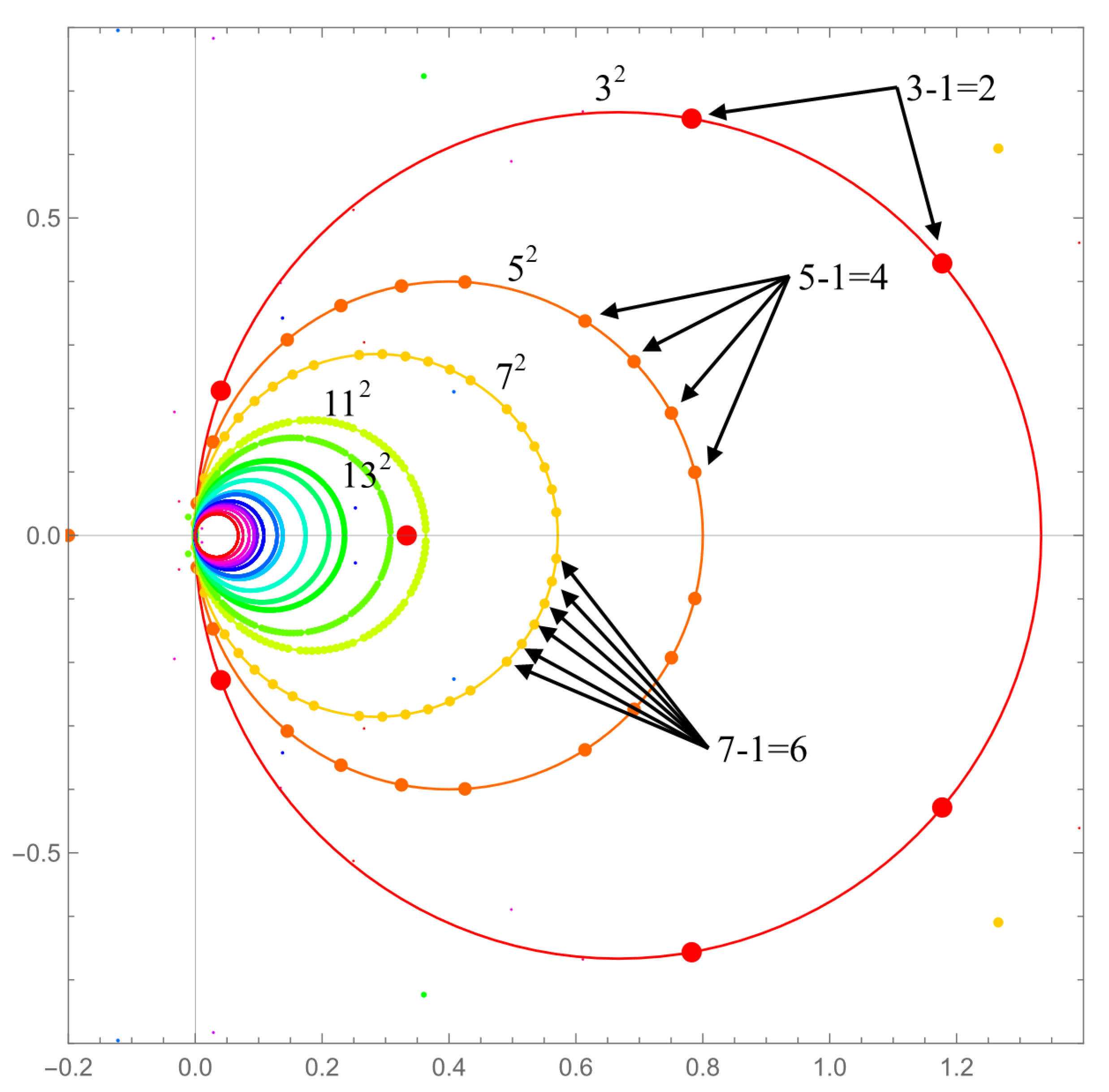
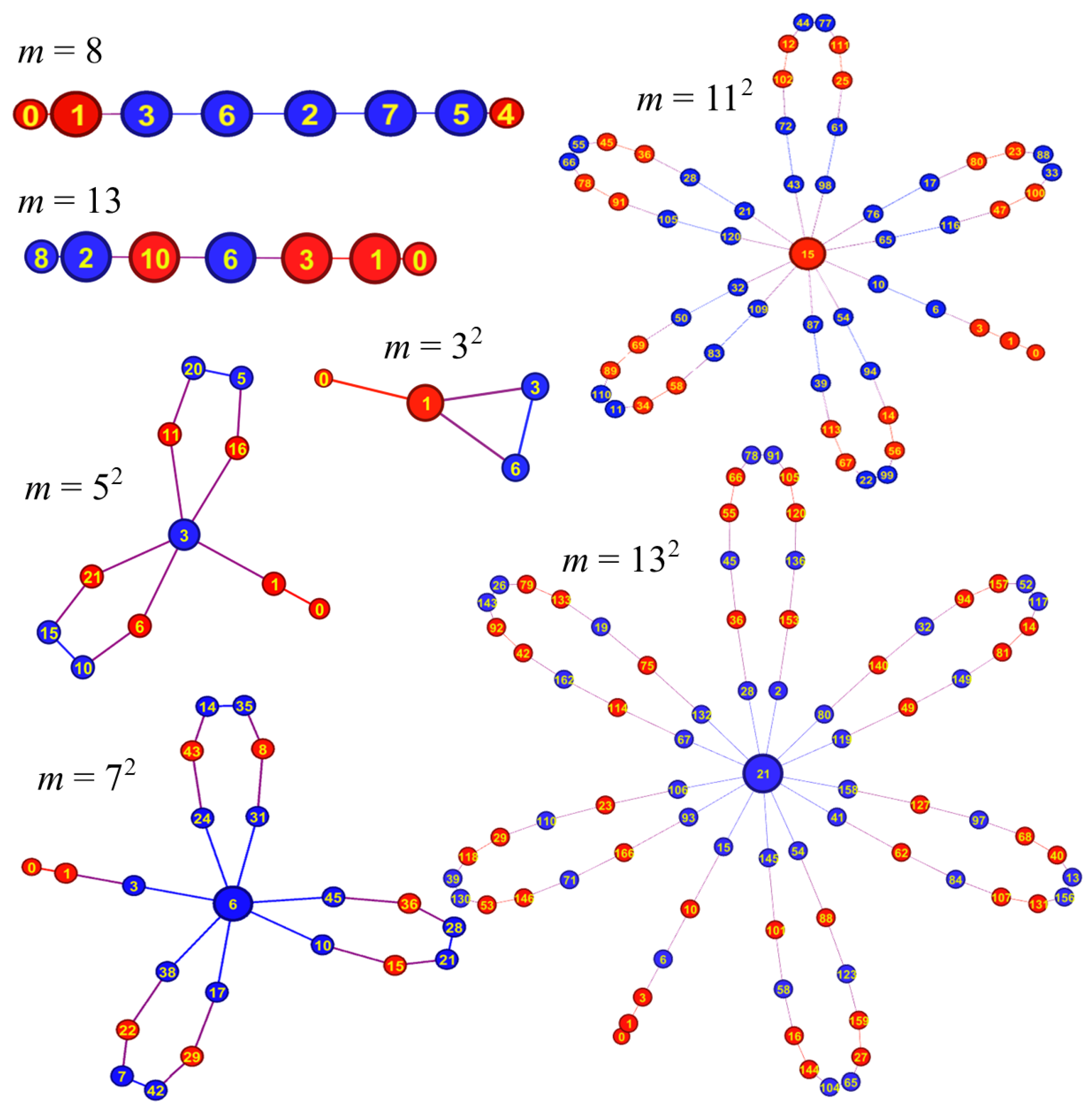
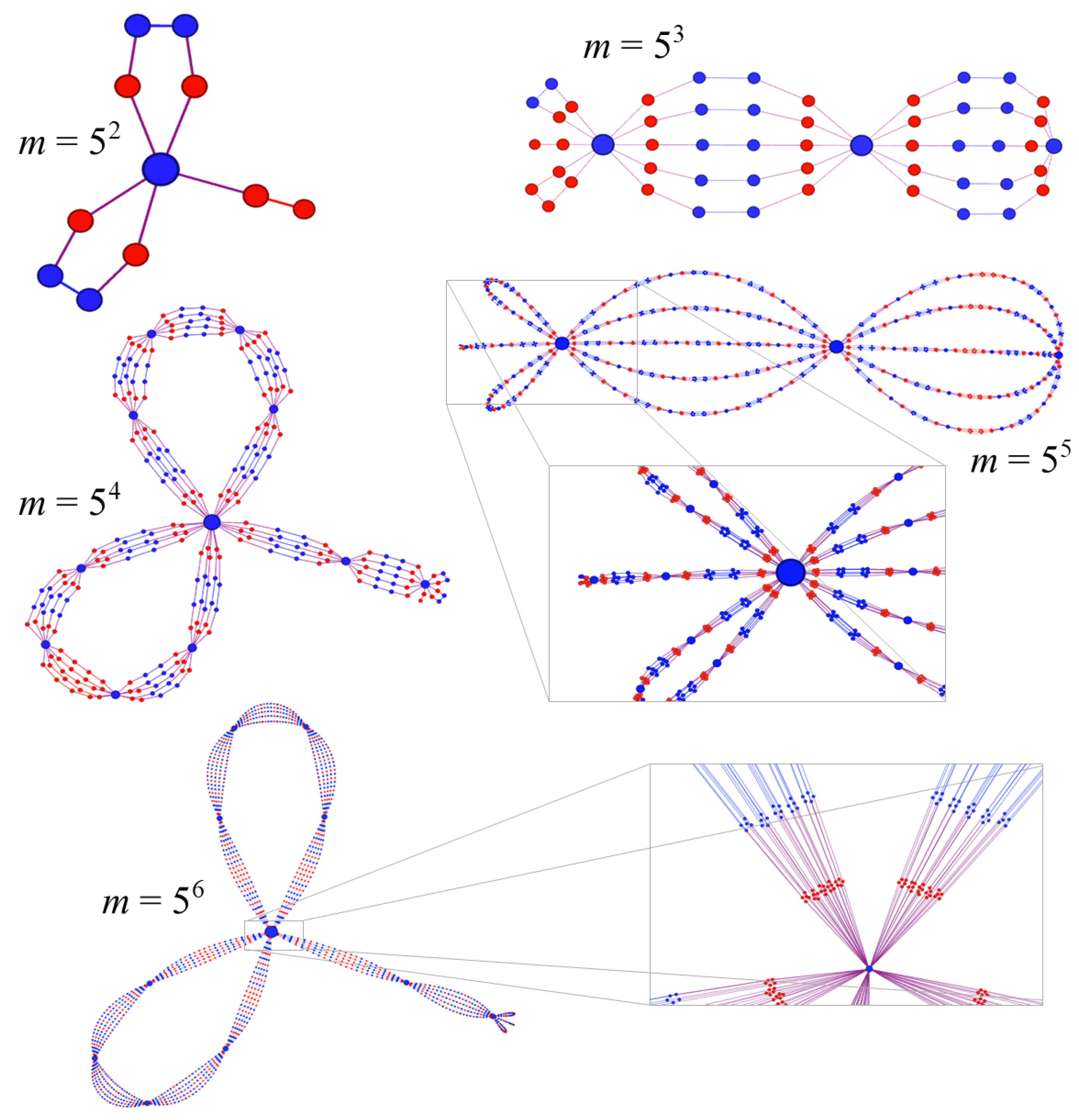
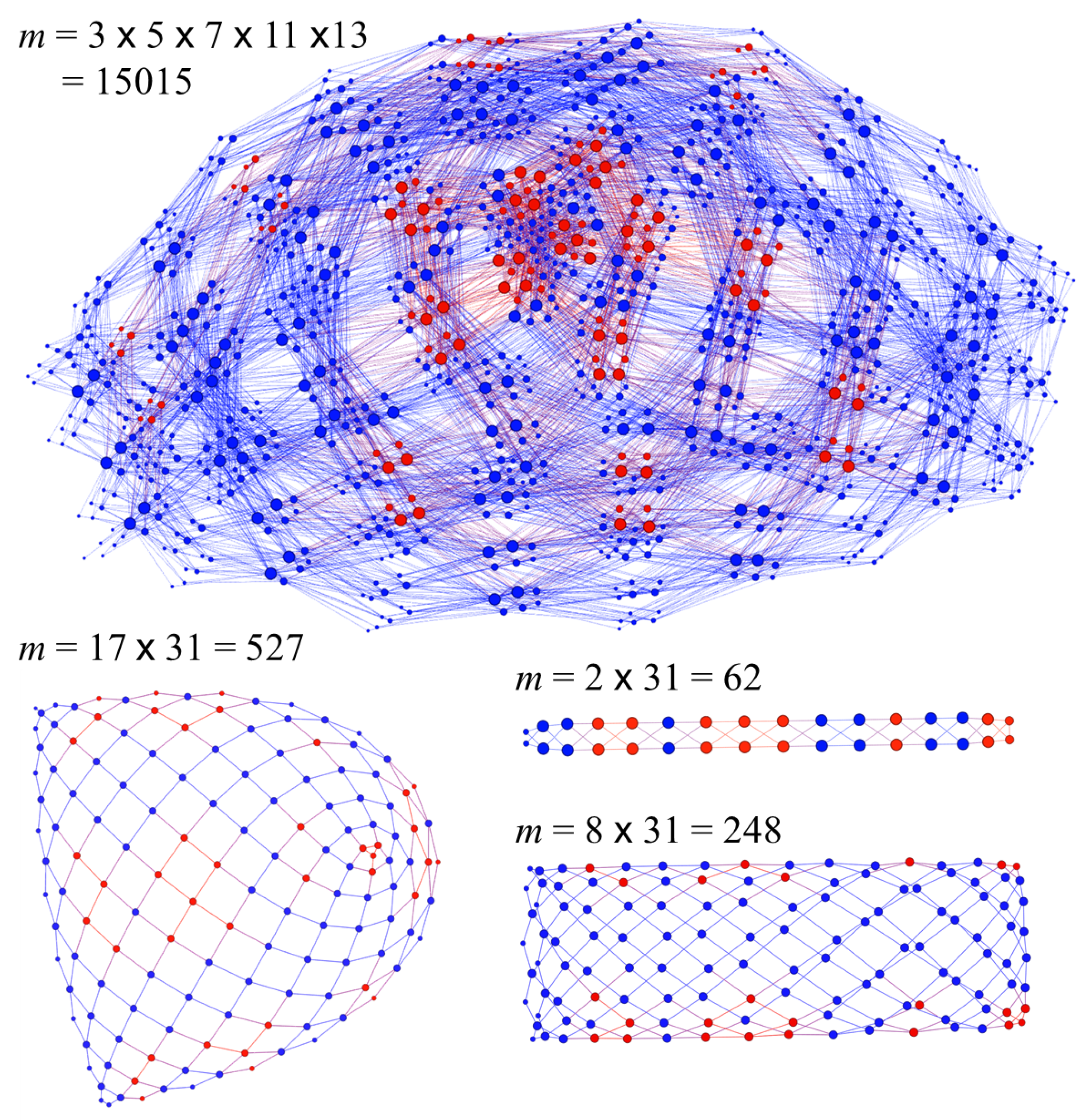

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ulness, D.J. Structure of Triangular Numbers Modulo m. AppliedMath 2022, 2, 326-358. https://doi.org/10.3390/appliedmath2030020
Ulness DJ. Structure of Triangular Numbers Modulo m. AppliedMath. 2022; 2(3):326-358. https://doi.org/10.3390/appliedmath2030020
Chicago/Turabian StyleUlness, Darin J. 2022. "Structure of Triangular Numbers Modulo m" AppliedMath 2, no. 3: 326-358. https://doi.org/10.3390/appliedmath2030020
APA StyleUlness, D. J. (2022). Structure of Triangular Numbers Modulo m. AppliedMath, 2(3), 326-358. https://doi.org/10.3390/appliedmath2030020





