Abstract
New definitions for fractional integro-differential operators are presented and referred to as delayed fractional operators. It is shown that delayed fractional derivatives give rise to the notion of functional order differentiation. Functional differentiation can be used to establish dualities and asymptotic mixtures between unrelated theories, something that conventional fractional or integer operators cannot do. In this paper, dualities and asymptotic mixtures are established between arbitrary functions, probability densities, the Gibbs–Shannon entropy and Hellinger distance, as well as higher-dimensional particle geometries in quantum mechanics.
1. Introduction
One of the goals of mathematics, science and engineering is to try and find dualities between two or more different theories or approaches that provide the same solutions even though they appear to be independent mathematical entities. In this paper, it will be shown that a modification of the differential and integral operators of fractional calculus can be used to establish dualities and asymptotic solutions between two (or more) unrelated mathematical functions or theories. What is referred to as functional-order differentiation is used to show that two probability densities, such as the one-parameter Exponential and the two-parameter Pareto, can form mathematical dualities, since either one can provide exact solutions of the other. Asymptotic solutions, which arise from dualities between these densities, are, in fact, mixtures of the two. Functional differentiation is then used to establish a Gibbs–Shannon entropy duality with the proper distance metric due to Hellinger. The duality means that both provide the same results for arbitrary probability densities, although one measures entropy and the other measures metric distance, which is symmetric and obeys the triangle inequality. Once again, asymptotic solutions between the two approaches are obtained, which show that an optimal minimum separation between densities is provided by a mixture model consisting of the Gibbs–Shannon entropy and Hellinger’s distance equations. Finally, functional differentiation is used to study the relationship between hyper-geometries and the duality between functional order and fractal dimension as applied to quantum mechanics. The solution to such problems requires a modification of the operators that appear in fractional calculus, since the latter and the conventional integer operators cannot achieve these results.
Fractional calculus goes back as far as 1695, when l’Hôspital pondered about the meaning of a derivative to half order in a letter to Leibniz. The word ‘fractional’ is a historical misnomer, given that it refers to ‘general’ or ‘generalised’ calculus. Hence, fractional calculus generalises conventional integer calculus such that the latter is a special case of the former. Throughout the centuries, fractional calculus has been developed by mathematicians such as Lacroix, Fourier, Liouville, Riemann, Grunwald, Letnikov, Abel, Riesz, Weyl, Caputo, and many others. As a result, various formulations appear in the literature at present []. Fractional calculus has become an intense area of research, starting from the second half of the last century, as many physical phenomena were better described by this. The continuous development of fractional calculus has led to a wider range of applications, not only in mathematics but also in the study and analysis of physical and engineering problems [,,,,,,,,,,,,,,,,,,,,,]. The parameter at the core of fractional differentiation or fractional integration is the fractional order . The fractional order is a constant that, unlike its integer counterpart, can also take real or complex values, i.e., and .
The natural outcome of having a constant value for the fractional order is to consider extending it to cases where it is a function of one or more variables. While this is somewhat obvious, it has not gained much traction, even after it was officially proposed in 1993 by Samko and Ross []. The primary issue has been the difficulty in obtaining closed-form solutions when the fractional order is also a function of the integration variable appearing in the fractional derivative and integral formulations, respectively. In other words, if the fractional order is a function of the integration variable x, i.e., , then the integration can become intractable, which is why choosing the correct variable dependence for the fractional order is very important. If it is a requirement that the fractional orders contain the same variable as integration, then it can be series-expanded and a sufficient number of terms can be retained for accuracy and to aid integration. In most cases, the fractional order is a function of other parameters that appear in the integrands of the fractional derivative and integral operators. The case where these parameters strictly represent space or time has been extensively studied in []. Due to the difficulty in obtaining closed-form solutions, another approach consists of using a modified version of the Grunwald–Letnikov series method with a parameter-dependent fractional order [,,]. The other alternative is to numerically calculate such fractional derivatives and integrals [,].
Differentiation or integration operators that contain a fractional order that is a function of arbitrary variables has come to be known as variable-order (VO) fractional differentiation and integration in the literature. Variable-order fractional calculus is used to solve many physical problems, with impressive results, even better than those obtained via the standard case, where the fractional order is constant. Some of these applications include linear-to-non-linear responses [] and a transition from sub-diffusive to super-diffusive flows []. Other problems that are solved via the use of VO fractional calculus appear in mechanics [], viscoelasticity [], the modelling of anomalous transport processes [], control theory [], signal filters [] and wave propagation []. A recent review of the latest developments in the area of variable-order fractional calculus can be found in [] and the references therein. In addition, the reader can also refer to [].
The ability to establish dualities and mixed solutions between independent functions or even physical theories cannot be performed via conventional integer-order or fractional-order operators. In this paper, a slight variation in the variable-order fractional operators will be considered, which facilitates these mathematical dualities and/or mixed states. Instead of including the variable order as part of the integration, the fractional operators will contain a delayed variable order. What this means is that the fractional operators will be used, as in the standard approach, where the fractional order is a constant and the latter will be mapped to a variable order . Not only does this avoid integration complexities but it will be shown that it can be used to solve a number of interesting problems, some of which will be presented in the paper. Given that the fractional operator is ‘delayed’ and not part of integration, as is the case with the variable-order definition, it will be referred to as functional differentiation (or integration), since the fractional order is a function of parameters that are not directly dependent on the initial integrations.
The paper is organised as follows. Section 2 derives the fractional derivatives of some functions that will be required later in the paper when discussing functional differentiation. In Section 3, functional differentiation is presented. This is then used to find dualities and asymptotic solutions between arbitrary functions, two probability densities, the Gibbs–Shannon entropy and Hellinger distance and hypergeometries, as applied to quantum mechanical particles. Conclusions are presented in Section 4.
2. Derivative of Functions to Fractional-Order
Conventional differentiation and integration operators have been generalised using their corresponding fractional forms. The order of either differentiation or integration is not discrete but continuous, so that the order can be either real or complex, i.e., or . There are a number of representations in the literature for these generalised operators, with those due to Riemann–Liouville, Caputo and Grunwald–Letnikov being some of the most familiar. In what follows, the Riemann–Liouville definitions will be used, but other formulations can be used instead. The fractional derivative of functions due to Riemann and Liouville is defined by the operator , acting on some function . The lower terminal is due to Liouville, and when it is , this is due to Riemann. Thus, the fractional derivative operator acting on a function takes the following form:
where n only takes on integer values: . The fractional order is bounded by , where is the floor function and is the ceiling function. The fractional integration of functions can be obtained via the Riemann–Liouville derivative operator and is defined as follows:
The fractional derivative (1) and fractional integral (2) can be re-written so that, instead of the fractional order only being a constant, it can also be a continuous function :
and
It is typical in the literature to refer to these two formulations (3) and (4) as the variable-order fractional derivative and fractional integral, respectively. The fractional order becomes a function of the variables t and x but, as can be surmised, performing the integrations with respect to these is very complicated, which is why the form for the fractional order is usually only a function of t, i.e., . What will be referred to as differentiation or integration to functional order is essentially the same as variational order differentiation and integration, but the functional order, which can be a function of arbitrary variables , will be a ‘delayed’ variational order. This means that normal fractional derivatives and integrals are obtained, as given by (1) and (2), and then the fractional order is mapped to the functional order ; hence, this is a ‘delayed’ order rather than immediately being part of the differentiation or integration, as given by the traditional variable-order expressions (3) and (4). As a result, there is less restriction, which allows for a considerable amount of flexibility when solving problems, as will be seen later in the paper.
Since the fractional derivative of monomials and exponential functions will be required in Section 3 as a first step in the process of differentiating functions to functional order; next, their fractional derivatives will be obtained, starting with the case of a monomial for some power .
2.1. Fractional Derivative of a Monomial
Using (1), let the integer order be and the lower integration limit be . Then, the fractional derivative of a monomial becomes
Subtitute the transformation in (5) to obtain the fractional derivative of as follows:
The fractional derivative of a monomial is obtained as (6) using the Riemann–Liouville method, which is equivalent to Lacroix’s approach of generalising multiple integer derivatives of monomials to fractional form.
2.2. Fractional Derivative of Exponentials
Consider the fractional derivative of for some . Proceeding similarly to the monomial case in the previous section:
Using transformations and simplifying gives the following result:
From this, one can observe that
From the fact that , we can see that the fractional derivative of becomes
where . Similarly, the fractional derivative of is determined, which takes the form:
Once again, via transformations and simplifications, the final result becomes:
Equation (12) has a dominant term:
This means that the fractional derivative has the final form,
where . Here, is the gamma-function and is the exponential–integral function . Observe that when , corresponding to conventional integer differentiation, all terms in the above expressions collapse to the conventional integer derivatives. This is because, where the denominators contain the gamma-function, this becomes whenever Following the same mathematical process as above, it can be shown that the fractional derivative of is given as:
and
Using combinations of (15) and (16), it can be shown that the fractional derivatives of and are given by
and
respectively. Thus, the fractional derivatives are generalised forms that contain the conventional integer derivatives as integer limits. At this point, it is worth addressing the approximations in (10), (14)–(16). The results contain terms that are very small and negligible, so that the exponential terms dominate in each case. The other terms are so small that they can be disregarded and only appear if the Riemann form for the operator is used, where the lower terminal in (7) is taken as zero. The correct approach is to use the Liouville operator, i.e., when the lower terminal is . Then, the negligible terms cancel out to exactly zero and the exponentials are the terms that survive. In the strict sense, the latter approach has to be used, but the Riemann version was used to ensure consistency with all other presented cases. In the end, however, both approaches are correct.
3. Derivative of Functions to Functional-Order
The Riemann–Liouville formulation will be defined slightly differently to (1):
where is the delayed-fractional order, which implies that, after the integral has been evaluated, the fractional order is mapped to . Notation-wise, the fractional order derivative operator (19) will be written as ,
A similar scheme applies to the fractional integral operator (2), which can be written as . For the rest of the paper, only the derivative as defined by (20) will be examined, for reasons of brevity, but the integral version of the integration of functions follows a similar argument. The proposition is that the fractional order can take a number of forms, such as:
In the conventional use of the fractional order, it is always taken as a constant , with values that are real or complex, or . The second definition is a function that is independent of the integration variable x. The third definition is a function of integration variable x; however, when using this definition in (21), it should be understood that this is not part of the integration. Only after the fractional derivative is obtained can be used, as indicated by (19) or (20), i.e., this is a delayed order. The first case where has always been dealt with in the literature. The second case has recently been discussed in []. The case of will be examined here. On this basis, the definition for differentiation to functional order is straightforward: the fractional derivative of a function is obtained and the fractional order is replaced with an arbitrary continuous function . Hence, the following notation is used:
This will represent the differentiation of a function to functional order. This simple definition has some very powerful mathematical properties, which allow solutions to be obtained to a number of problems. In addition, differentiation to functional order allows for dualities to be found between distinct functions. In other words, two independent functions and can be made equivalent, in the sense that
Aside from functional orders that satisfy the relation (23), it should be noted that (23) can also be used to obtain asymptotic solutions which can be thought of as ‘transitional’ functions or limits that are mixtures of the two functions and . Put another way, if the variable in the functional order is strictly time, , then this has memory properties, encompassing past present and future states. If the functional order contains spatial dimensions, e.g., , then it has spatial correlation properties, a kind of spacial dimensional fading effect. In both cases, these are equivalent to or represented by the asymptotic solutions.
If the constant parameter is added to the mathematical form of the functional order , it can be used as the mechanism that picks out these asymptotic solutions. When is set in the functional order, then , and when , then , and the solutions provide the exact form of . This requires solving the expression (23) for , which then makes it possible, through variations to , to obtain and or any combination of them. Amongst other things, this establishes a duality between the two independent functions. In addition, the functional order is an arbitrary continuous function with validity throughout the x domain. If the entire domain is not required, then a more localised derivative order (approaching a constant or integer value) can be obtained by taking the series of to any desired number of terms. In the following sections, differentiation to functional order will be demonstrated and, via the use of (23), used to solve some interesting problems.
3.1. Applications of Functional Order Differentiation
In this section, how functional differentiation can form dualities and asymptotic mixtures between various independent functions can be shown. Relating such functions is only possible via functional differentiation (integration). This is not possible with conventional fractional or integral differentiation. Consider the case of a monomial , as discussed in Section 2.1. Its derivative to functional order is given as follows:
for some functional orders, . Equation (24) can posses a number of interesting properties depending on the mathematical form of the functional order . Suppose that the monomial is quadratic with , and let a second function be . It is clear from Figure 1 that and do not intersect for . As they do not have any common solutions, they are independent functions. If the functional order is taken to be equal to the function , for example, i.e., , then it is possible to relate the two independent functions so that they intersect. There are four solutions that are related by the derivative of to functional order, as can be seen in Figure 1. This is achieved via (24) so that the derivative to functional order becomes:
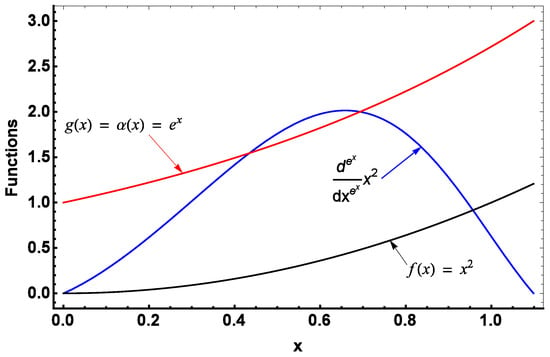
Figure 1.
Plots showing dualities and asymptotic behaviour between two independent functions and . For , these functions never have any common intersections. However, differentiating one of the functions, namely, , to functional order allows for the previously independent functions to be connected via four intersection points. The other function, , is used as the functional order, i.e., .
Note that when , (25) reduces to the conventional integer derivative while the original function corresponds to the case in (24). Hence, the function to the right of (25) now encompasses solutions that belong to the two independent functions and .
Next, allow two alternative functions and to be considered. The solution that makes both of these functions equal and provides their asymptotic ‘transitions’ will be derived. Firstly, from (23),
Equation (27) holds if the functional order is given by:
with . The constant , but here this can be set to zero: . Thus, the derivative of to functional order , as given by (28) with , provides the exact same result as function . This is shown in Figure 2, which also shows the function , as well as asymptotic solutions corresponding to , and . When , , so original function is recovered. As the value of increases from zero to , the asymptotic solutions first resemble the function , and then they approach and resemble until the two are exact when . Note that the asymptotic solutions approach the functions and from ‘above’, since the values for are all less than . However, they can also approach these functions asymptotically from ‘below’ if their values were .

Figure 2.
Plots showing dualities and asymptotic behaviour between the functions and . The function transforms to the function through differentiation to functional order, where corresponds to the case where they are equal. Asymptotic solutions that approach are given by , while shows asymptotic variations that tend towards both functions simultaneously (a kind of half-way point). When , the asymptotic solution tends towards the function . When , , so the function is recovered.
Another example of the use of differentiation to functional order is considered. From Section 2, use can be made of the functional derivative of an exponential function:
Expanding the right-hand side of (29) to the first order and setting the result to the original exponential function gives the following:
The functional order that allows for expression (30) to be exact is obtained as:
Multiplying both sides by a and setting gives:
Taking the n-th power of both sides and setting gives,
Equation (36) is an equivalent way of writing the binomial expansion, i.e., it is an identity or there is a duality between the binomial expansion and the expression on the right of (34). This duality may also allow for certain integrals to be performed more easily if, for example, integrals take the form:
or some variation of (36). Here, a and b are functions or a combination of functions and constants, such that . As an example, consider the relationship (37) and its use to solve:
by setting and and where and is the exponential integral function. Thus, the complicated integral on the left is equivalent to a simple integral on the right, via the manipulation of (37), which, in turn, can be determined in closed form. As another example, it can be shown that the integral on the left:
is equal to the closed form on the right, where is the error-function, and and . Similar expressions can be derived for other mathematical relations using the same approach as shown above. This highlights the power of using functional-order differentiation (integration) to form dualities between arbitrary and uniquely different functions. In what is to follow, functional order differentiation will be used to derive dualities and general relations in some physics and mathematics problems via the functional order.
3.2. Duality and Asymptotic Mixtures of Probability Densities
It is well-known that many physical, mathematical and engineering systems can be modelled via probability distributions. Using differentiation to functional order, a direct connection between different probability densities can be achieved when this is otherwise not possible. Consider the one-parameter Exponential-density with parameter :
where the inverse of the parameter is equal to the mean. In addition, consider the two-parameter Pareto-density, which has been extensively used in many research areas to model various processes. The ability to model some very important phenomena using the Pareto distribution has prompted its generalisation to a fractional form. Among other things, the fractional Pareto distribution has been successfully used to model the statistical properties of radar clutter returns in [], for example. The conventional Pareto density is given as follows:
The parameter regulates the shape of the distribution and is the support parameter. It is obvious that the two densities will have different distributions when compared to each other and cannot be equal, except for some cases where there is trivial overlap, typically at one or two intersections. No other solutions that make them both equal are possible. However, by using the differentiation to functional order approach, it will be shown that not only can they be made equal, but one can asymptotically approach the other. Let,
The left-hand side of (42) for the Exponential-density involves the fractional differentiation of a negative exponential term, which was performed previously; see (7). Then, replacing with provides the following form for (42),
Observe that the conventional Exponential-density is recovered in (43) when . Furthermore, (43) holds if, and only if, there is a functional order that allows for this equivalence. In fact, this is true when
The substitution of (44) into the left of (43) collapses to the right side, i.e., the Pareto density. The functional-order (44) can be rewritten as follows:
If in (45), this gives (44) and, as a result, (43) holds. Varying above and below unity provides the asymptotic solutions to the Exponential density that, in the limit, , tend towards and exactly match the Pareto density.
Figure 3 shows plots of the Pareto density for parameters , and the Exponential density parameter for varying values of , providing the exact curve that is produced by the Pareto density when , and asymptotic solutions, as shown for and . Hence, via differentiation to functional order, a one-parameter density (Exponential model) produces the same mathematical properties as a two-parameter density (Pareto model). In this case, the Exponential density was not only generalised, but can also produce specific solutions corresponding to a different density, namely the Pareto, while also producing the asymptotic solutions that approach the latter in the limit . Any value that is greater than , such as the one considered in Figure 3, namely, , will provide solutions that asymptotically approach the Pareto density from the right. As can be seen, solutions with values approach the Pareto density from the left and, as , solutions asymptotically approach the Exponential density, and equal it when (not shown in Figure 3 for reasons of brevity). The asymptotic solutions, as given by different values of , except for and , are, in fact, mixed-probability densities consisting of a mixture of both the Exponential and Pareto densities, respectively.
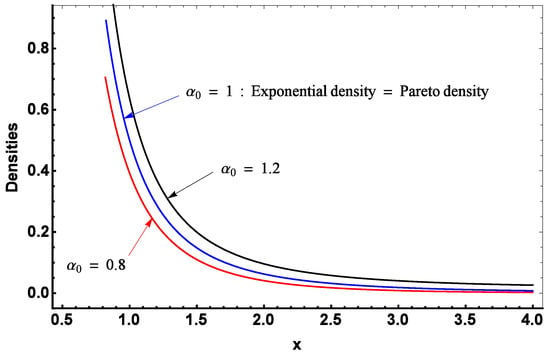
Figure 3.
Plots of the Pareto density and the Exponential density. The latter is exact with the Pareto when , while the asymptotic solutions of the Exponential density that approach the Pareto density are also shown to correspond to and , respectively. Note that if , then the curve would be equivalent to the traditional Exponential density (not shown).
3.3. Duality and Asymptotic Mixtures between the Gibbs–Shannon Entropy and the Hellinger Distance
As in the previous examples, consider another important application of differentiation to functional order that is of interest to physics, mathematics and engineering, including information geometry and information theory. Using the previously discussed differentiation method to functional-order, it will be shown that there is a duality between the Gibbs–Shannon entropy for a continuous probability density and a metric distance between the same probability density and another density with parameter space and , respectively, with and M providing the total number of parameters for each density. It has been shown that the fractional entropy for probability densities is given by []:
where is the domain of integration of the density and the modulus is used because the fractional-order is not only real but can also be imaginary, and . It is worth pointing out that the analogue of (46) for the discrete case of probability masses was derived by Ubriaco []. The dissimilarity or divergence of the two probability densities can be determined using a metric distance, referred to as the Hellinger distance:
where (47) is the square of the Hellinger distance. It should be obvious that both (46) and (47) will not provide the same result, as one is a measure of entropy and the other is a measure of the distance between the densities. Using differentiation to functional-order, it will be shown that there is a duality between the two formulations and both can give the same results. This duality will be established even though they each measure different properties between density/s: one being entropy and the other distance or divergence. In practice, this duality does not exist because the Gibbs–Shannon entropy is not a true distance metric as it is not symmetric and only measures entropy. On the other hand, the Hellinger formulation is symmetric and does obey the triangle inequality but measures distance and not entropy. Let the two densities be given as the Exponentials:
where their respective parameter spaces consist of only one parameter, namely, and . Equation (46) is already in fractional form, so there is no need to obtain the fractional derivative. If is substituted, this gives:
for the fractional entropy. A duality between the entropy and the Hellinger distance can be claimed for some functional-order , such that:
In fact this is true and (50) holds if
when . It can be shown that when (51) is substituted into the entropy on the left of (50), it collapses to the Hellinger expression on the right, hence establishing a duality between them. This duality was achieved via the application of functional-order differentiation to conventional entropy.
Numerical solutions can be obtained for the parameter values and , for example. The conventional entropy then gives:
On the other hand, the square of the Hellinger distance between the densities and cannot be the same as the entropy for the density , as expected. The square of the Hellinger distance becomes:
Using the fractional entropy and the functional-order , as given by (51), with :
gives the value:
in agreement with the Hellinger distance (53). Hence, a duality is established between them. Notice that if the square root of the right side is taken in (53), then the same can be taken for (55); they are equal either way. Asymptotic solutions between the Gibbs–Shannon and Hellinger formulations can also be obtained using (51) for varying , which will be referred to as asymptotic divergences. When , the fractional entropy is equivalent to the Hellinger distance or divergence; that is, when ,
as established above. Setting in (51) provides the conventional entropy result, i.e.,
Figure 4 shows numerical values for the asymptotic divergences between the conventional entropy and the square of the Hellinger distance as a function of . The numerical asymptotic divergence values that are bounded by the conventional entropy and Hellinger distance were plotted for in steps of . The first value on the left, corresponding to , gives the exact solution as obtained from the Hellinger distance. As varies and becomes equal to the last value on the right, i.e., when it is , the solution is exactly the same as that given by the conventional entropy. For values that lie between them, asymptotic divergence solutions are obtained, which are due to a ‘mixture’ of the two, depending on how close is to one or the other. As a result, a minimum asymptotic divergence value exists between them when . This indicates, among other things, where the conventional entropy and Hellinger distance have similar mathematical characteristics, while also minimising their respective probability densities. Finally, returning to the fact that (49) and (51) establish a duality between the conventional entropy and the Hellinger distance when , it stands to reason that there can be other valid solutions, depending on the mathematical form of . For example, if the functional order is a constant value instead of a function, i.e., if , for example, then it is possible to obtain the same result for the fractional entropy as when the functional order was a continuous function (51). Substituting this into (55) gives:
as before. This means that the entropy can also obtain the same result as the Hellinger formulation when the functional order is equal to a complex constant and not just a function. By differentiating the entropy to functional order, it was possible to find a duality between the conventional entropy and the Hellinger distance, as well as their asymptotic divergence solutions (mixture), not withstanding the fact that the two are completely different mathematical formulations.
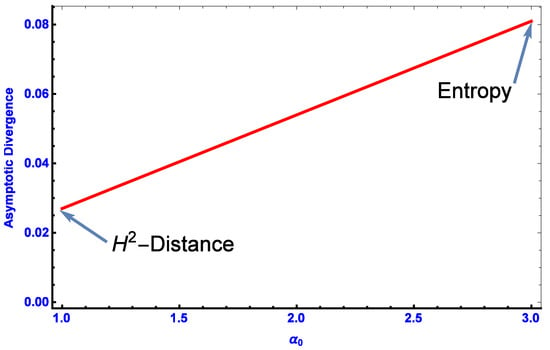
Figure 4.
Numerical asymptotic divergences between the conventional entropy and the square of the Hellinger distance as a function of the parameter .
3.4. Dualities between Higher-Dimensional Geometries and the Functional Order in Quantum Mechanics
Higher-dimensional spheres or hyperspheres have been of tremendous interest in the study of the effective properties of materials, since they exhibit interesting electromagnetic behaviour when they contain these hyperspheres as inclusions. The scattering effects of hyperspheres have been studied, and so has their use in metamaterial structures for example []. Hyperspheres, and other hypergeometries such as hypercubes, are used in the study of error rate and data correction algorithms or in packing geometries. Functional-order differentiation will be used to examine the dualities between the functional order and the dimension of hyperspheres and hypercubes. These hypergeometries and, in particular, the geometry of hyperspheres, can represent quantum mechanical particles and their classical and relativistic properties. On this basis, consider the functional derivative of the hypervolume of a hypersphere, which can be calculated from:
where is the hypervolume of a hypersphere. The modulus is included because, in principle, and . From this, the functional variation in the hypervolume of a d-dimensional sphere (hypersphere) is given by:
where d is the dimension, is the functional order and R is the radius of the hypersphere.
The functional change in the hypervolume of a hypercube is obtained as follows:
where L is the side length of the hypercube. The radius of a hypersphere R is related to the length L of a hypercube by the following:
where . The functional order, which provides equal hypervolumes between hyperspheres and hypercubes is given by:
Hence, for the case represented in Figure 5, the functional order can be determined from (63) and is . The hypervolume of the hypersphere starts from above 80 on the left in magenta and the hypervolume of the hypercube starts below 40 on the left in blue. The volume of a regular cube is constant as represented by the horizontal orange line. For the given parameters, the functional order makes the hypervolumes/volume equal. The functional order given by (63) not only provides the duality or connection between the hyperspherical and hypercubic geometries, but also does the same for the relativistic energies of particles with such geometries. The relativistic energy of a hyperspherical particle is given by the following:
where is the density of the mass, c is the speed of light and u is the speed of the hypersphere, respectively. Similarly, the energy of a hypercube particle is given by the following:
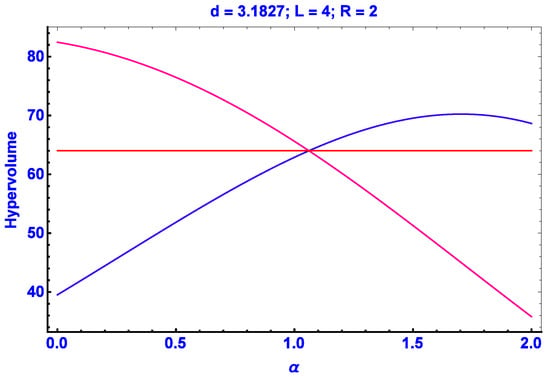
Figure 5.
The hypervolumes of a hypersphere and a hypercube are equal to the 3-dimensional volume of a cube for the chosen values of d, , L and R.
The rest energy of a hypersphere or hypercube particle is given by (64) and (65) when , respectively. For non-relativistic speeds , the energies reduce to (HyperSphere) and (HyperCube), respectively:
and
where is the rest energy (64) and is the rest energy (65), i.e., when . If a hypersphere and a hypercube particle have the same density and the same velocity, the ratio of their energies is given by the following:
Figure 6 shows the relationship between the rest energy, functional order and fractal dimension of hypersherical and hypercube particles as a comparison. Referring to Figure 7 and Figure 8, consider the case where an electron is assumed to be a hypersphere (functional order ). When the electron is assumed to have a spherical geometry, , the corresponding energy agrees with the theory. When the same electron is assumed to have the energy of a typical open superstring of Planck length and energy, the dimension is fractal at . Superstrings are considered to be approximately dimensional from the macroscopic perspective but, in reality, they possess an internal geometry that arises from their vibration in Calabi–Yau manifolds. The figures show that the fractal dimension of the superstring (electron), namely, , ‘drops’ out from extrapolating the dimension as the energies increase and match the expected values from the theory; see Figure 7 for the particle at rest and Figure 8 when it is moving at . Revisiting the hypersphere volume (60), a hyper-particle of radius and even dimensions provides a hypervolume variation,
where and . Summing (69) to infinity, i.e., summing all even dimensional hypervolumes to infinite dimensions, gives the closed form:
where is the regularised generalised hypergeometric function. This means that the sum of all relativistic energies for even dimensional hyperparticles of radius one is given by the following:
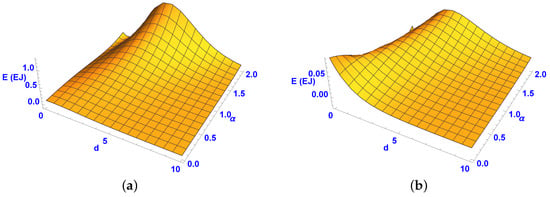
Figure 6.
The rest energies of hypersphere (a) and hypercube (b) geometries as a function of functional order and fractal dimension. Note that the energy is given in units of EJ or exa-joules () Joules.
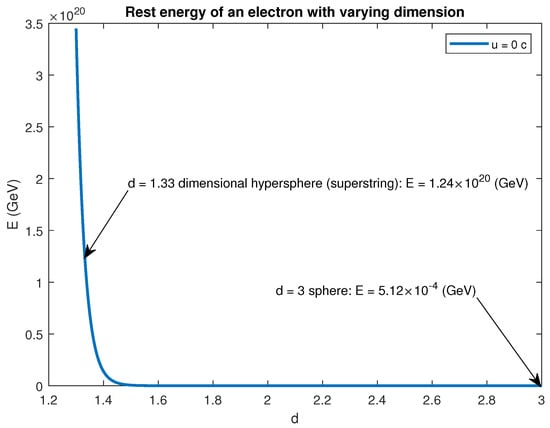
Figure 7.
Energy vs. dimension for an electron at rest.
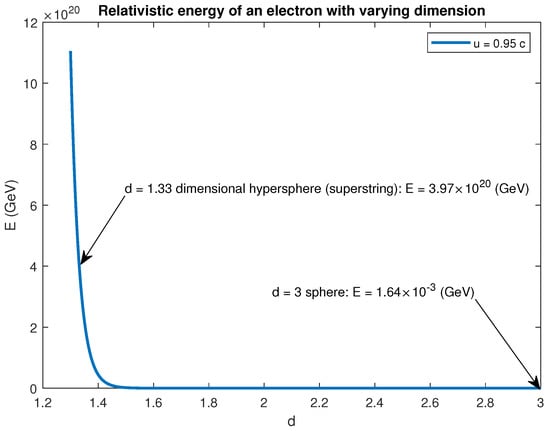
Figure 8.
Energy vs. dimension for an electron at .
If the velocity of the hyperparticles is , then:
An interesting result is obtained for the sum of the rest energies of all the even-dimensional hyperparticles. If the hypervolume is considered, i.e., when in (71) or (72), the total energy becomes:
This can easily be seen if one considers the sum in (70)
In a similar way, for and , the energies become:
and
respectively, etc. These results are very interesting and raise a number of further questions that need to be answered, such as determining their physical interpretation.
4. Conclusions
Variable-order fractional calculus is an extension of the standard constant order to a function containing arbitrary parameters. Unfortunately, these parameters can make the calculations intractable because they convolute the integrals that are present in the operators. The idea of a delayed variable order or differentiation/integration to functional order is an alternative that has been used to solve some problems, which avoid these issues. This allows for the easy derivation of asymptotic solutions between different functions and can establish dualities between them that are not otherwise possible. Such dualities and their asymptotic solutions beg the question as to whether there are further mathematical properties or ‘connections’ that exist between seemingly independent mathematical expressions or theories. If the variable in the functional order is time , then the solutions have memory properties: past, present and future. If the functional order contains a space variable , then it has spatial correlation properties, a kind of spacial dimensional fading effect. These memory and spatial correlation properties are equivalent to the asymptotic solutions between two or more functions. Aside from the direct dualities, asymptotic solutions can be obtained between two or more functions that are essentially nothing more than mixtures of these functions. It is hoped that the use of functional-order calculus to solve problems, as presented in this paper, will encourage more researchers to use this approach in their work.
Funding
This research received no external funding.
Conflicts of Interest
The author declares no conflict of interest.
References
- De Oliveira, E.C.; Machado, J.A.T. A review of definitions for fractional derivatives and integral. Math. Probl. Eng. 2014, 2014, 238459. [Google Scholar] [CrossRef] [Green Version]
- Alexopoulos, A.; Weinberg, G.V. Fractional-order formulation of power-law and Exponential distributions. Phys. Lett. A 2014, 378, 2478–2481. [Google Scholar] [CrossRef]
- Alexopoulos, A.; Weinberg, G.V. Fractional-order Pareto distributions with application to X-band maritime radar clutter. IET Radar Sonar Navig. 2015, 9, 817–826. [Google Scholar] [CrossRef]
- Alexopoulos, A. Fractional divergence of probability densities. Fractal Fract. 2017, 1, 8. [Google Scholar] [CrossRef] [Green Version]
- Alexopoulos, A. One-parameter Weibull-type distribution, its relative entropy with respect to Weibull and a fractional two-parameter Exponential distribution. Stats 2019, 2, 34–54. [Google Scholar] [CrossRef] [Green Version]
- Alexopoulos, A. The fractional Kullback-Leibler divergence. J. Phys. A Math. Theor. 2021, 54, 75001. [Google Scholar] [CrossRef]
- Hilfer, R. (Ed.) Applications of Fractional Calculus in Physics; World Scientific Publishing: Singapore, 2000. [Google Scholar]
- Podlubny, I. Fractional Differential Equations. In Mathematics in Science and Engineering; Academic Press: Cambridge, MA, USA, 1999; Volume 198. [Google Scholar]
- Kulish, V.V.; Lage, J.L. Application of fractional calculus to fluid mechanics. Fluids Eng. 2002, 124, 803. [Google Scholar] [CrossRef]
- Douglas, J.F. Some applications of fractional calculus to polymer science. In Advances in Chemical Physics; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 1997; Volume 102. [Google Scholar]
- Fellah, Z.E.A.; Depollier, C. Application of fractional calculus to the sound waves propagation in rigid porous materials: Validation via ultrasonic measuremen. Acta Acustica 2002, 88, 34–39. [Google Scholar]
- Assaleh, K.; Ahmad, W.M. Modeling of speech signals using fractional calculus. In Proceedings of the International Symposium on Signal Processing and Its Applications (ISSPA), Sharjah, United Arab Emirates, 12–15 February 2007; pp. 1–4. [Google Scholar]
- Mathieu, B.; Melchior, P.; Oustaloup, A.; Ceyral, C. Fractional differentiation for edge detection. Signal Process. 2003, 83, 2285–2480. [Google Scholar] [CrossRef]
- Soczkiewicz, E. Application of fractional calculus in the theory of viscoelasticity. Mol. Quantum Acoust. 2002, 23, 397–404. [Google Scholar]
- Machado, J.A.T.; Jesus, I.S.; Cunha, J.B.; Tar, J.K. Fractional dynamics and control of distributed parameter systems. Intell. Syst. Serv. Mank. 2006, 2, 295–305. [Google Scholar]
- Lepik, U. Solving PDEs with the aid of two-dimensional Haar wavelets. Comput. Math. Appl. 2011, 61, 1873–1879. [Google Scholar] [CrossRef] [Green Version]
- Guariglia, E. Fractional calculus, zeta functions and Shannon entropy. Open Math. 2021, 19, 87–100. [Google Scholar] [CrossRef]
- Khader, M.M.; Adel, M. Chebyshev Wavelet Procedure for Solving FLDEs. Acta Appl. Math. 2018, 158, 1–10. [Google Scholar] [CrossRef]
- Guariglia, E.; Silvestrov, S. Fractional-Wavelet Analysis of Positive definite Distributions and Wavelets on D’(C). In Engineering Mathematics II; Silvestrov, S., Rančić, M., Eds.; Springer: Berlin/Heidelberg, Germany, 2016; pp. 337–353. [Google Scholar]
- Nikan, O.; Avazzadeh, Z.; Tenreiro Machado, J.A. Numerical approach for modeling fractional heat conduction in porous medium with the generalized Cattaneo model. Appl. Math. Model. 2021, 100, 107–124. [Google Scholar] [CrossRef]
- Nikan, O.; Avazzadeh, Z. Numerical simulation of fractional evolution model arising in viscoelastic mechanics. Appl. Numer. Math. 2021, 169, 303–320. [Google Scholar] [CrossRef]
- Nikan, O.; Avazzadeh, Z.; Tenreiro Machado, J.A. Numerical study of the nonlinear anomalous reaction–subdiffusion process arising in the electroanalytical chemistry. J. Comput. Sci. 2021, 53, 101394. [Google Scholar] [CrossRef]
- Kalimbetov, B.; Abylkasymova, E.; Beissenova, G. On the asymptotic solutions of singulary perturbed differential systems of fractional order. J. Math. Comput. Sci. 2021, 24, 165–172. [Google Scholar] [CrossRef]
- Samko, S.G.; Ross, B. Integration and differentiation to a variable fractional order. Integral Transform. Spec. Funct. 1993, 1, 277–300. [Google Scholar] [CrossRef]
- Lorenzo, C.F.; Hartley, T.T. Variable order and distributed order fractional operators. Nonlinear Dyn. 2002, 29, 57–98. [Google Scholar] [CrossRef]
- Valério, D.; Sá da Costa, J. Variable-order fractional derivatives and their numerical approximations. Signal Process. 2011, 91, 470–483. [Google Scholar] [CrossRef]
- Valério, D.; Sá da Costa, J. Variable order fractional controllers. Asian J. Control 2013, 15, 648–657. [Google Scholar] [CrossRef]
- Moghaddam, B.P.; Machado, J.A.T. Extended algorithms for approximating variable order fractional derivatives with applications. J. Sci. Comput. 2017, 71, 1351–1374. [Google Scholar] [CrossRef]
- Chen, Y.; Wei, Y.; Liu, D.; Yu, H. Numerical solution for a class of nonlinear variable order fractional differential equations with Legendre wavelets. Appl. Math. Lett. 2015, 46, 83–88. [Google Scholar] [CrossRef]
- Shen, S.; Liu, F.; Chen, J.; Turner, I.; Anh, V. Numerical techniques for the variable order time fractional diffusion equation. Appl. Math. Comput. 2011, 218, 10861–10870. [Google Scholar] [CrossRef] [Green Version]
- Meng, R.; Yin, D.; Zhou, C.; Wu, H. Fractional description of time-dependent mechanical property evolution in materials with strain softening behavior. Appl. Math. Model. 2016, 40, 398–406. [Google Scholar] [CrossRef]
- Chechkin, A.V.; Gorenflo, R.; Sokolov, I.M. Fractional diffusion in inhomogeneous media. J. Phys. A Math. Gen. 2005, 38, L679. [Google Scholar] [CrossRef]
- Atanackovic, T.M.; Pilipovic, S. Hamilton’s principle with variable order fractional derivatives. Fract. Calc. Appl. Anal. 2011, 14, 94–109. [Google Scholar] [CrossRef] [Green Version]
- Bagley, R.; Torvik, P. A theoretical basis for the application of fractional calculus to viscoelasticity. J. Rheol. 1983, 27, 201–210. [Google Scholar] [CrossRef]
- Benson, D.A.; Wheatcraft, S.W.; Meerschaert, M.M. Application of a fractional advection dispersion equation. Water Resour. Res. 2000, 36, 1403–1412. [Google Scholar] [CrossRef] [Green Version]
- Orosco, J.; Coimbra, C.F.M. On the control and stability of variable-order mechanical systems. Nonlinear Dyn. 2016, 86, 695–710. [Google Scholar] [CrossRef]
- Tseng, C.C. Design of variable and adaptive fractional order FIR differentiators. Signal Process. 2006, 86, 2554–2566. [Google Scholar] [CrossRef]
- Zhao, X.; Karniadakis, G.E. Second-order approximations for variable order fractional derivatives: Algorithms and applications. J. Comput. Phys. 2015, 293, 184–200. [Google Scholar] [CrossRef]
- Patnaik, S.; Hollkamp, J.P.; Semperlotti, F. Applications of variable-order fractional operators: A review. Proc. R. Soc. A 2020, 476, 20190498. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sun, H.; Chang, A.; Zhang, Y.; Chen, W. A review on variable-order fractional differential equations: Mathematical foundations, physical models, numerical methods and applications. Fract. Calc. Appl. Anal. 2019, 22, 27–59. [Google Scholar] [CrossRef] [Green Version]
- Ubriaco, M.R. Entropies based on fractional calculus. Phys. Lett. A 2009, 373, 2516–2519. [Google Scholar] [CrossRef] [Green Version]
- Alexopoulos, A.; Yau, K.S.B. Scattering and cloaking of binary hyper-particles in metamaterials. Opt. Express 2010, 18, 19626–19644. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).