Impact of Imperfect Artefacts and the Modus Operandi on Uncertainty Quantification Using Virtual Instruments
Abstract
:1. Introduction
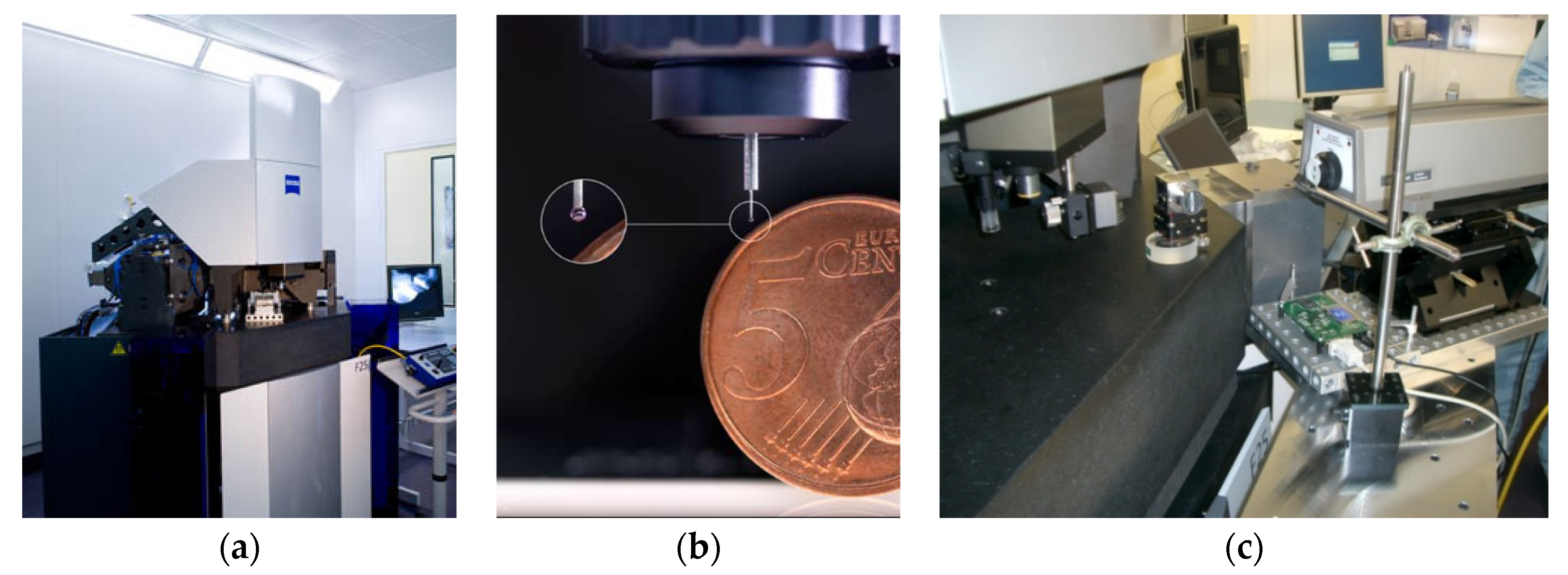
2. Materials and Methods
2.1. Numerical Simulation Model
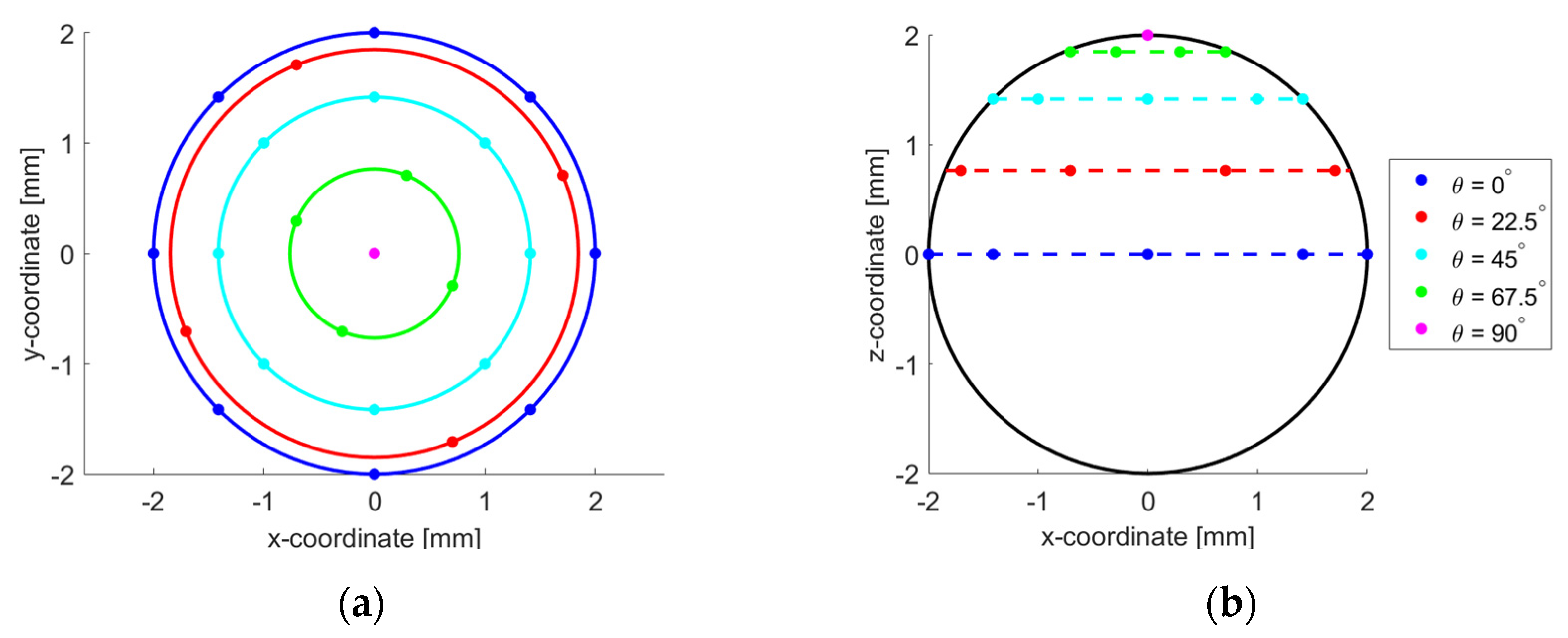
2.2. Dataset and Research Design
- Real measurement data of a measured sphere;
- Simulated data of the nominal measurement points;
- Simulated data of an imperfect sphere with a similar PV value—version 1;
- Simulated data of an imperfect sphere with a similar PV value—version 2.
3. Results
3.1. Uncertainty Calculation
3.2. Numerical Example Related to a Sphere with Form Deviation in a VCMM
3.3. Improved Method for Predicting Uncertainties with a VI
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- BIPM; IEC; IFCC; ILAC; ISO; IUPAC; IUPAP; OIML. Evaluation of Measurement Data—Guide to the Expression of Uncertainty in Measurement, JCGM 100:2008, GUM 1995 with Minor Corrections. 2008; Available online: https://www.bipm.org/documents/20126/2071204/JCGM_100_2008_E.pdf/cb0ef43f-baa5-11cf-3f85-4dcd86f77bd6 (accessed on 17 March 2022).
- Trapet, E.; Waldele, F. The Virtual CMM Concept. In Advanced Mathematical Tools in Metrology; World Scientific: Singapore, 1996; Volume 2, pp. 238–247. [Google Scholar]
- Balsamo, A.; Di Ciommo, M.; Mugno, R.; Rebaglia, B.; Ricci, E.; Grella, R. Evaluation of CMM uncertainty through Monte Carlo simulations. CIRP Ann. 1999, 48, 425–428. [Google Scholar] [CrossRef]
- Han Haitjema, B.W.; van Dorp, M.; Morel, P.; Schellekens, H.J. Uncertainty estimation by the concept of virtual instruments. In Proceedings of the SPIE 4401, Recent Developments in Traceable Dimensional Measurements, Munich, Germany, 22 October 2001. [Google Scholar] [CrossRef]
- Aggogeri, F.; Barbato, G.; Barini, E.M.; Genta, G.; Levi, R. Measurement uncertainty assessment of coordinate measuring machines by simulation and planned experimentation. CIRP J. Manuf. Sci. Technol. 2011, 4, 51–56. [Google Scholar] [CrossRef]
- Gąska, A.; Harmatys, W.; Gąska, P.; Gruza, M.; Gromczak, K.; Ostrowska, K. Virtual CMM-based model for uncertainty estimation of coordinate measurements performed in industrial conditions. Measurement 2017, 98, 361–371. [Google Scholar] [CrossRef]
- Heißelmann, D.; Franke, M.; Rost, K.; Wendt, K.; Kistner, T.; Schwehn, C. Determination of measurement uncertainty by Monte Carlo simulation. In Advanced Mathematical and Computational Tools in Metrology and Testing XI; World Scientific: Singapore, 2019; pp. 192–202. [Google Scholar]
- Vlaeyen, M.; Haitjema, H.; Dewulf, W. Digital Twin of an Optical Measurement System. Sensors 2021, 21, 6638. [Google Scholar] [CrossRef] [PubMed]
- ISO/TS 15530-4:2008; Geometrical Product Specifications (GPS). Coordinate Measuring Machines (CMM): Part 4: Evaluating Task-Specific Measurement Uncertainty Using Simulation. ISO: Geneva, Switzerland, 2008.
- Flack, D. NPL Good Practice Guide No. 130, Co-Ordinate Measuring Machine Task-Specific Measurement Uncertainties; National Physical Laboratory: Teddington, UK, 2013. [Google Scholar]
- EMPIR Project 20IND04 ATMOC. Traceable Metrology of Soft X-ray to IR Optical Constants and Nanofilms for Advanced Manufacturing, 2021–2024. Available online: https://www.euramet.org/research-innovation/search-research-projects/details/project/traceable-metrology-of-soft-x-ray-to-ir-optical-constants-and-nanofilms-for-advanced-manufacturing (accessed on 17 March 2022).
- Wübbeler, G.; Marschall, M.; Kniel, K.; Heißelmann, D.; Härtig, F.; Elster, C. GUM-Compliant Uncertainty Evaluation Using Virtual Experiments. Metrology 2022, 2, 114–127. [Google Scholar] [CrossRef]
- BIPM; IEC; IFCC; ILAC; ISO; IUPAC; IUPAP; OIML. Evaluation of Measurement Data—Guide to the Expression of Uncertainty in Measurement—Supplement 1 to the “Guide to the Expression of Uncertainty in Measurement”—Propagation of Distributions Using a Monte Carlo Method, JCGM 101:2008; 2008; Available online: https://www.bipm.org/documents/20126/2071204/JCGM_101_2008_E.pdf/325dcaad-c15a-407c-1105-8b7f322d651c (accessed on 17 March 2022).
- EMRP Project IND10. Optical and Tactile Metrology for Absolute form Characterisation, 2011–2014. Available online: https://www.ptb.de/emrp/ind10-home.html (accessed on 17 March 2022).
- EMRP Project IND59. Multi-Sensor Metrology for Microparts in Innovative Industrial Products, 2013–2016. Available online: https://www.ptb.de/emrp/microparts-project.html (accessed on 17 March 2022).
- Matlab Software. The Mathworks. Available online: https://www.mathworks.com (accessed on 17 March 2022).
- ISO 10360-2:2009; Geometrical Product Specifications (GPS)—Acceptance and Reverification Tests for Coordinate Measuring Machines (CMM)—Part 2: CMMs Used for Measuring Linear Dimensions. ISO: Geneva, Switzerland, 2009.
- Giaquinto, N.; Fabbiano, L. Examples of S1 coverage intervals with very good and very bad long-run success rate. Metrologia 2016, 53, S65. [Google Scholar] [CrossRef]
- Wübbeler, G.; Elster, C. On the transferability of the GUM-S1 type A uncertainty. Metrologia 2020, 57, 015005. [Google Scholar] [CrossRef]
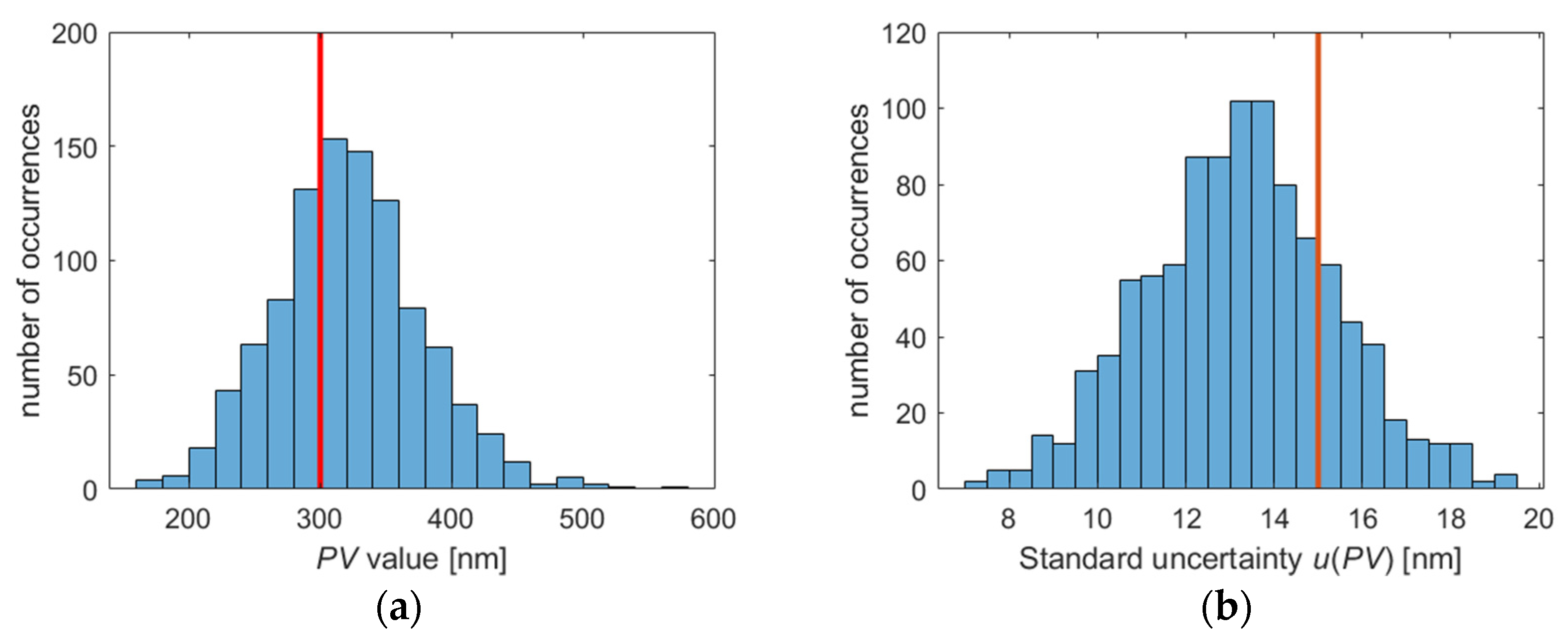
| Input Dataset | Uncertainty Sources | PV (nm) | u(PV) (nm) |
|---|---|---|---|
| Measured sphere | none | 310 | 0 |
| Measured sphere | all | 313 | 15 |
| Measured sphere | random | 310 | 6 |
| Measured sphere | systematic | 313 | 14 |
| Simulated perfect sphere | none | 0 | 0 |
| Simulated perfect sphere | all | 47 | 9 |
| Simulated perfect sphere | random | 16 | 2 |
| Simulated perfect sphere | systematic | 44 | 9 |
| Simulated imperfect sphere 1 | none | 310 | 0 |
| Simulated imperfect sphere 1 | all | 310 | 19 |
| Simulated imperfect sphere 1 | random | 310 | 6 |
| Simulated imperfect sphere 1 | systematic | 310 | 13 |
| Simulated imperfect sphere 2 | none | 310 | 0 |
| Simulated imperfect sphere 2 | all | 311 | 16 |
| Simulated imperfect sphere 2 | random | 311 | 10 |
| Simulated imperfect sphere 2 | systematic | 311 | 15 |
| Modus Operandi of VCMM | u(PV) (nm) |
|---|---|
| Uncertainty for real measurement data | 15 |
| Predicted uncertainty based on nominal data | 10 |
| Predicted uncertainty based on a single imperfect artefact (lowest value) | 7 |
| Predicted uncertainty based on simulating a large number of imperfect artefacts (proposed approach) | 19 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kok, G.; Wübbeler, G.; Elster, C. Impact of Imperfect Artefacts and the Modus Operandi on Uncertainty Quantification Using Virtual Instruments. Metrology 2022, 2, 311-319. https://doi.org/10.3390/metrology2020019
Kok G, Wübbeler G, Elster C. Impact of Imperfect Artefacts and the Modus Operandi on Uncertainty Quantification Using Virtual Instruments. Metrology. 2022; 2(2):311-319. https://doi.org/10.3390/metrology2020019
Chicago/Turabian StyleKok, Gertjan, Gerd Wübbeler, and Clemens Elster. 2022. "Impact of Imperfect Artefacts and the Modus Operandi on Uncertainty Quantification Using Virtual Instruments" Metrology 2, no. 2: 311-319. https://doi.org/10.3390/metrology2020019
APA StyleKok, G., Wübbeler, G., & Elster, C. (2022). Impact of Imperfect Artefacts and the Modus Operandi on Uncertainty Quantification Using Virtual Instruments. Metrology, 2(2), 311-319. https://doi.org/10.3390/metrology2020019






