Tensile Behavior of [0/90]7 Hemp/Elium Biocomposites after Water Aging: In-Situ Micro-CT Testing and Numerical Analysis
Abstract
1. Introduction
2. Materials and Methods
2.1. Tested Material
2.2. Aging Conditions
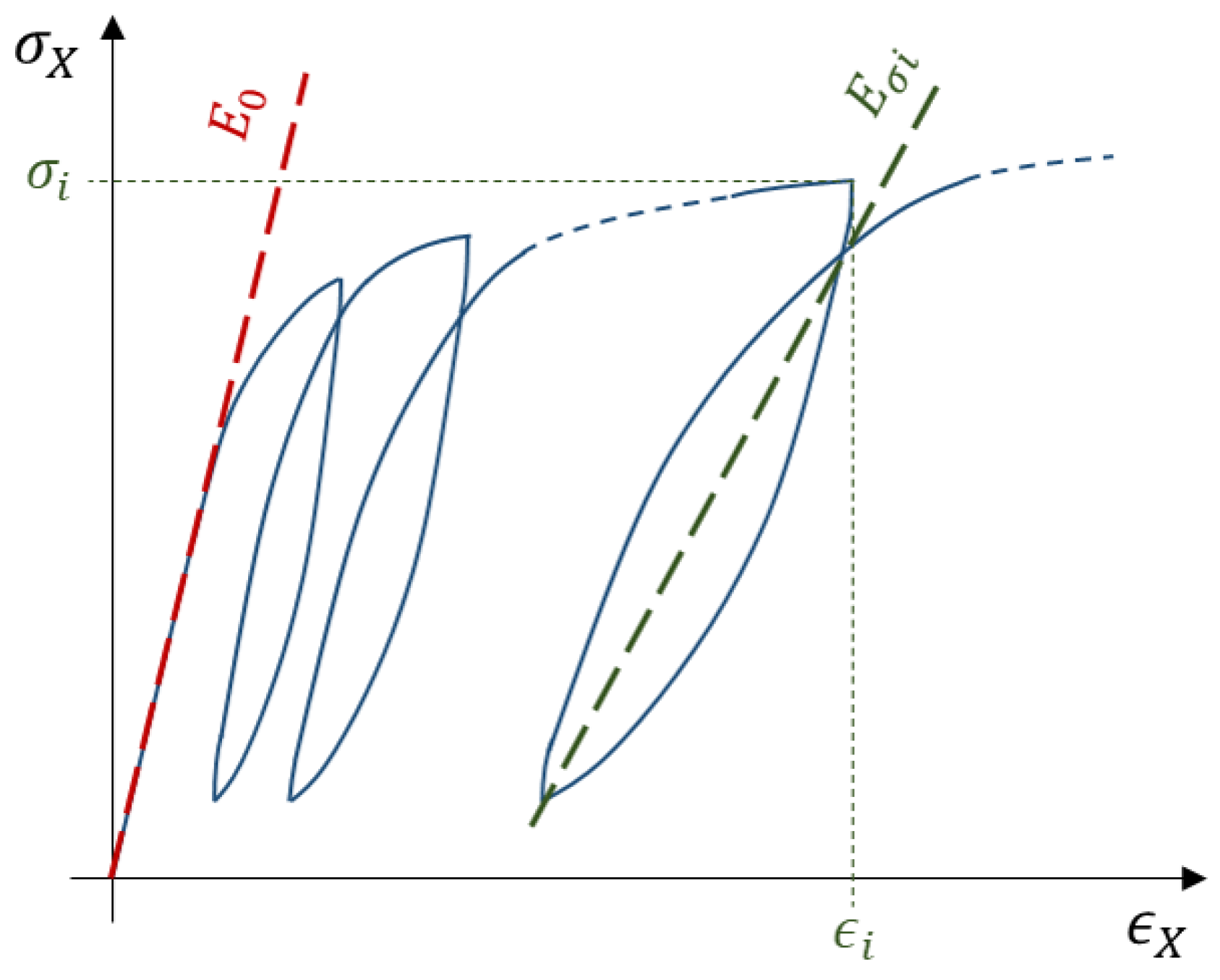
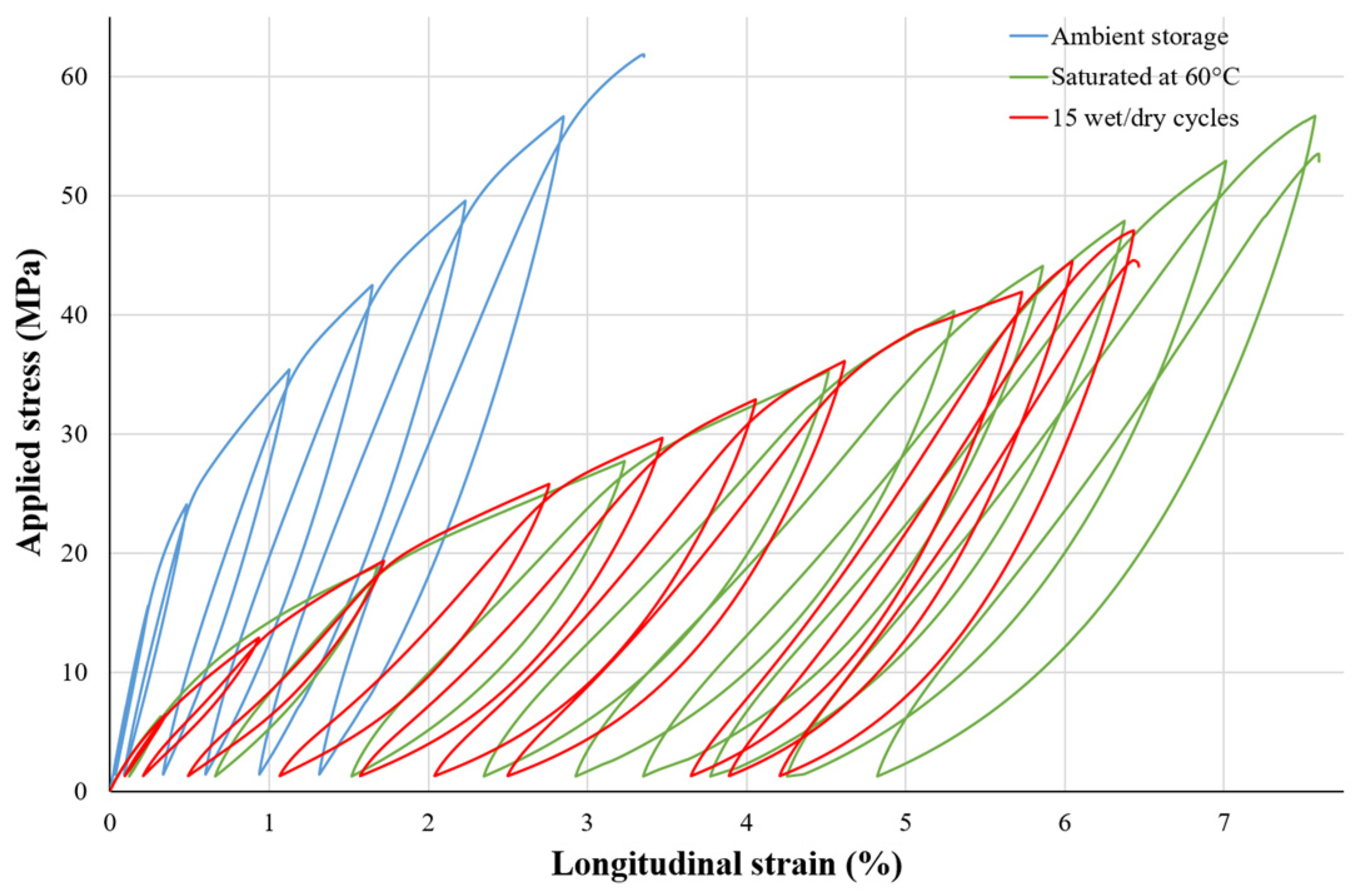
2.3. Ex-Situ Repeated Progressive Tensile Loading
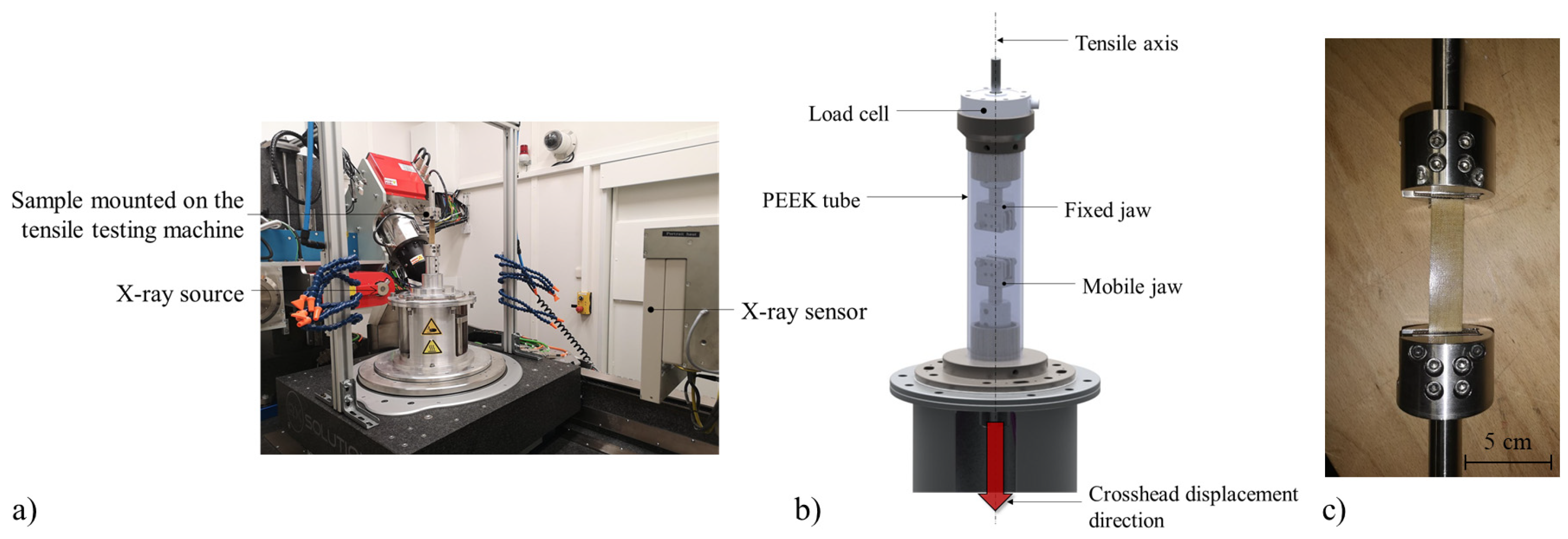
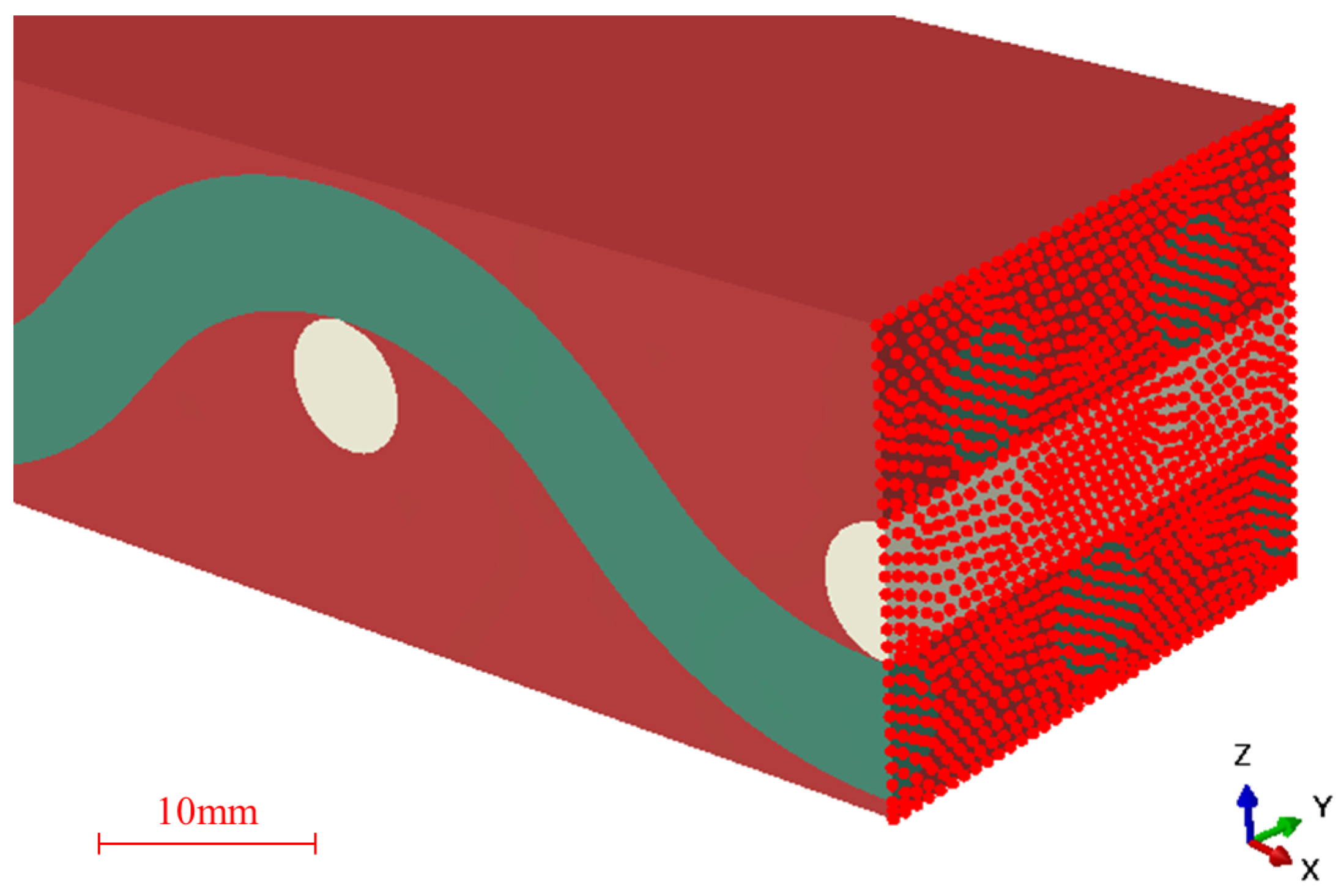
2.4. In-Situ Micro-CT Tensile Test
2.5. Image Processing
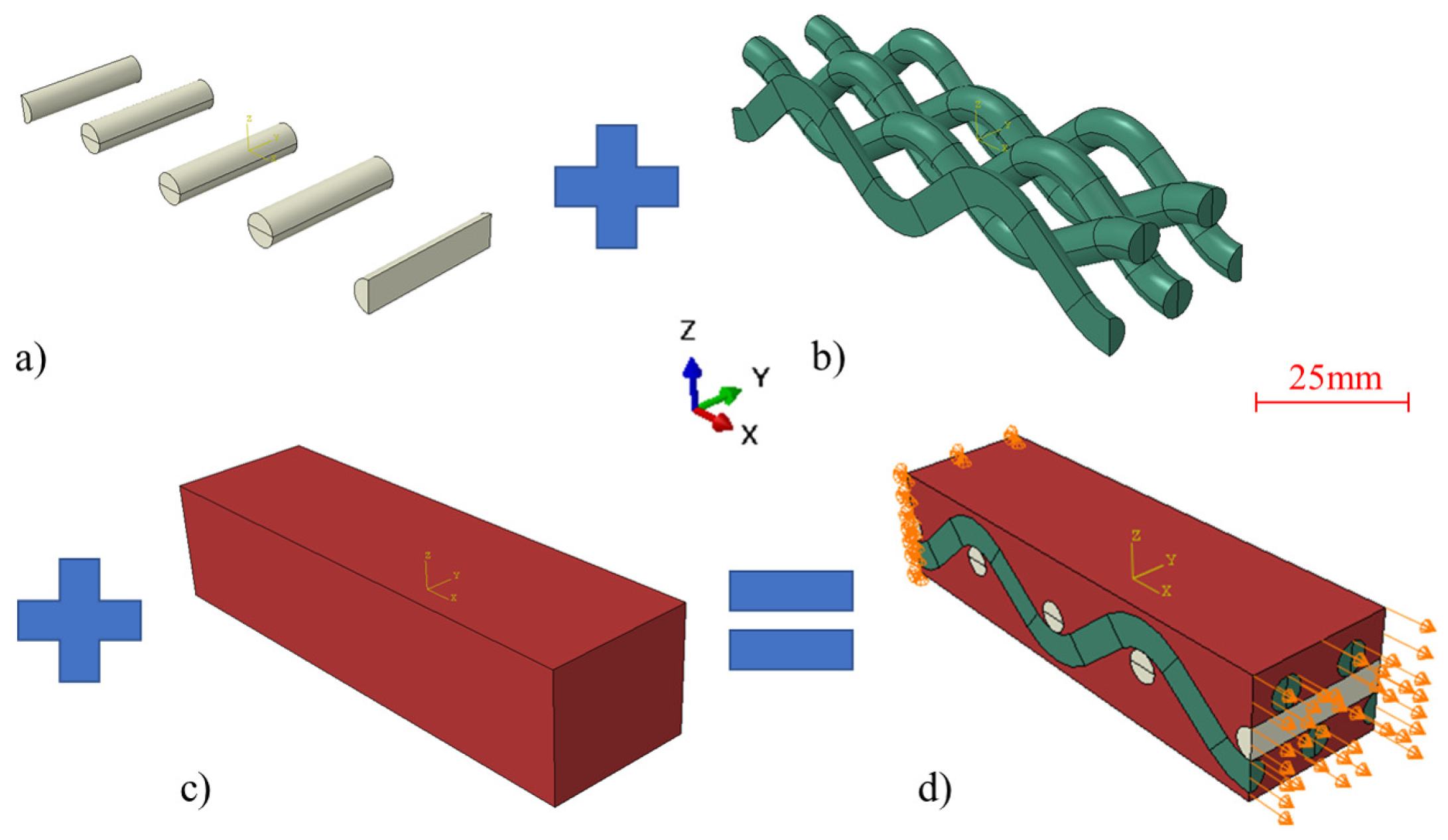
2.6. Finite Element Calculation
3. Results and Discussion
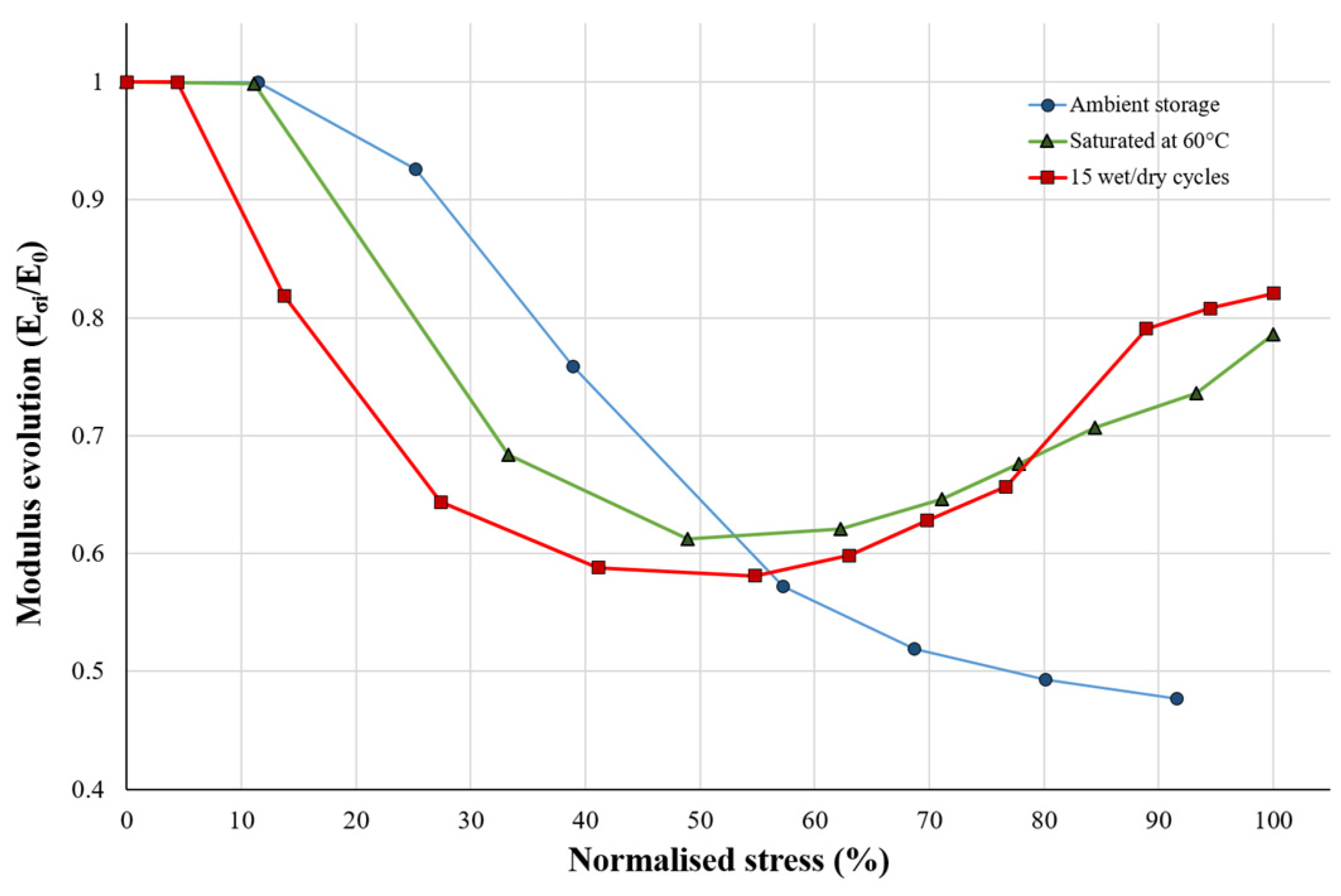
3.1. Modulus Evolution
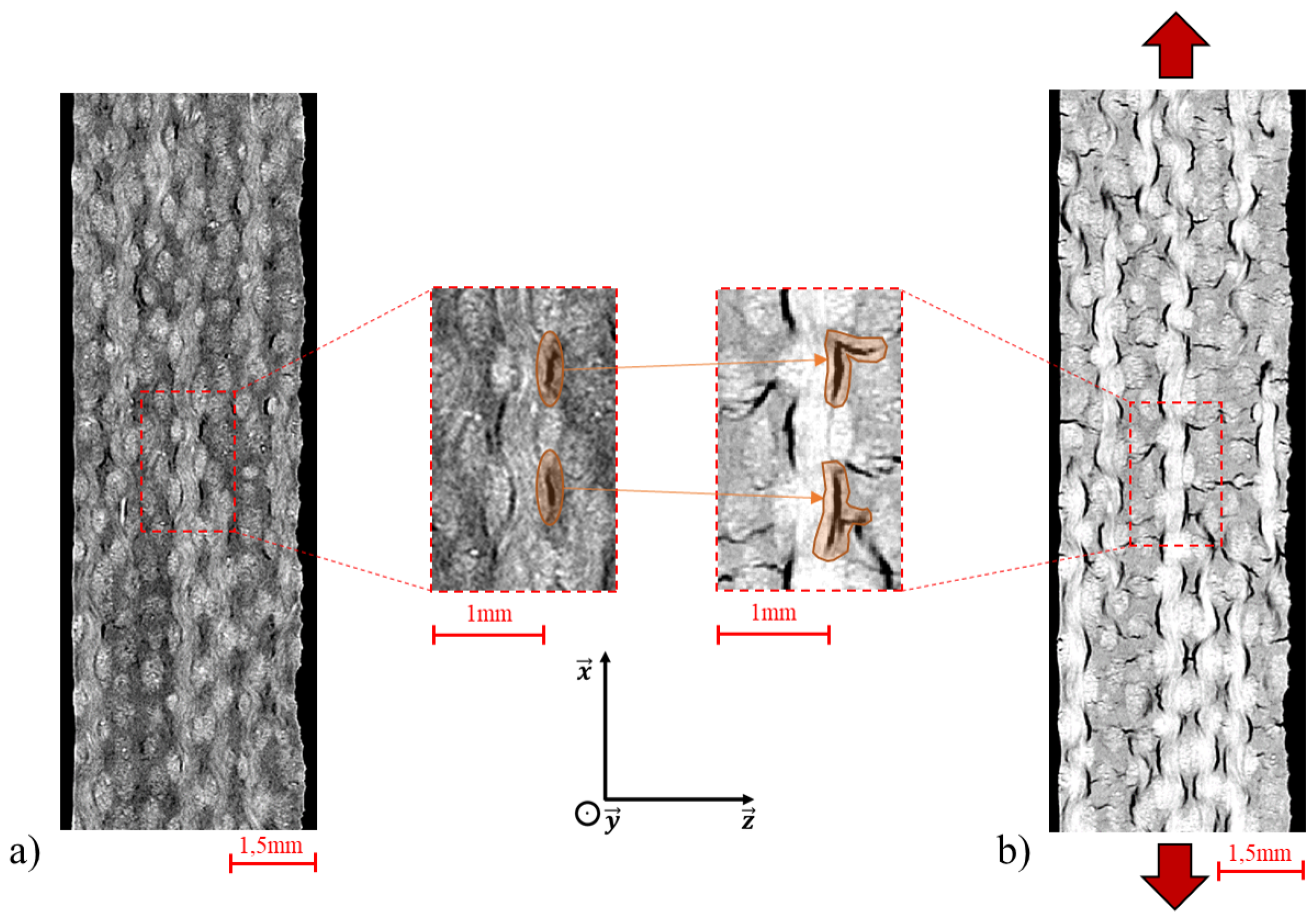
3.2. In-Situ Tensile Test
- before loading: the sample was mounted on the in-situ tensile testing machine and the displacement of the crosshead was held at zero,
- loaded: the sample was loaded and when the applied stress reached 80% of the ultimate stress (37.6 MPa in accordance with Table 2), the displacement of the crosshead was blocked,
- unloaded: the sample was unloaded and when the applied stress was close to zero, the displacement of the crosshead was blocked.
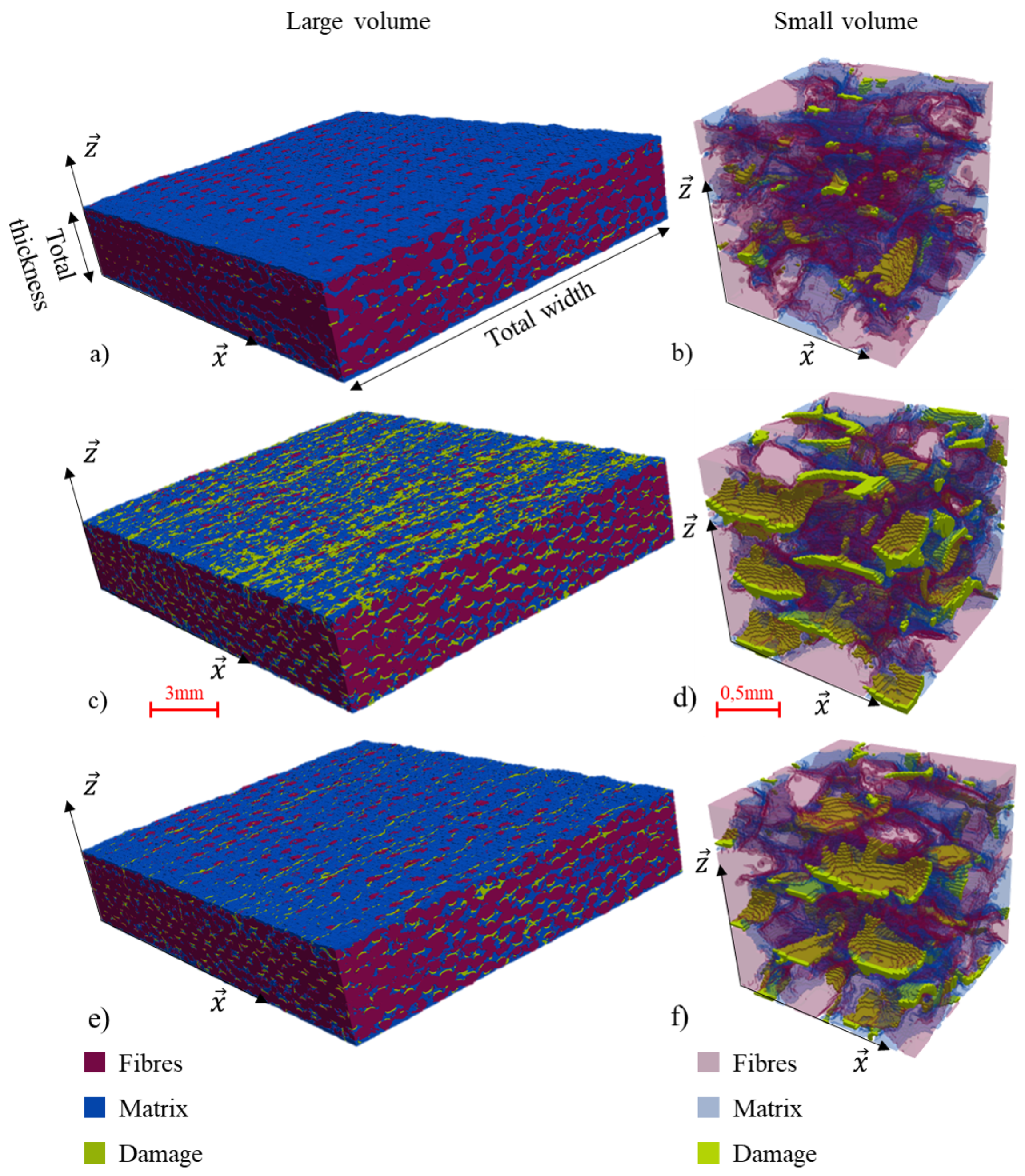
3.3. Damage Quantification
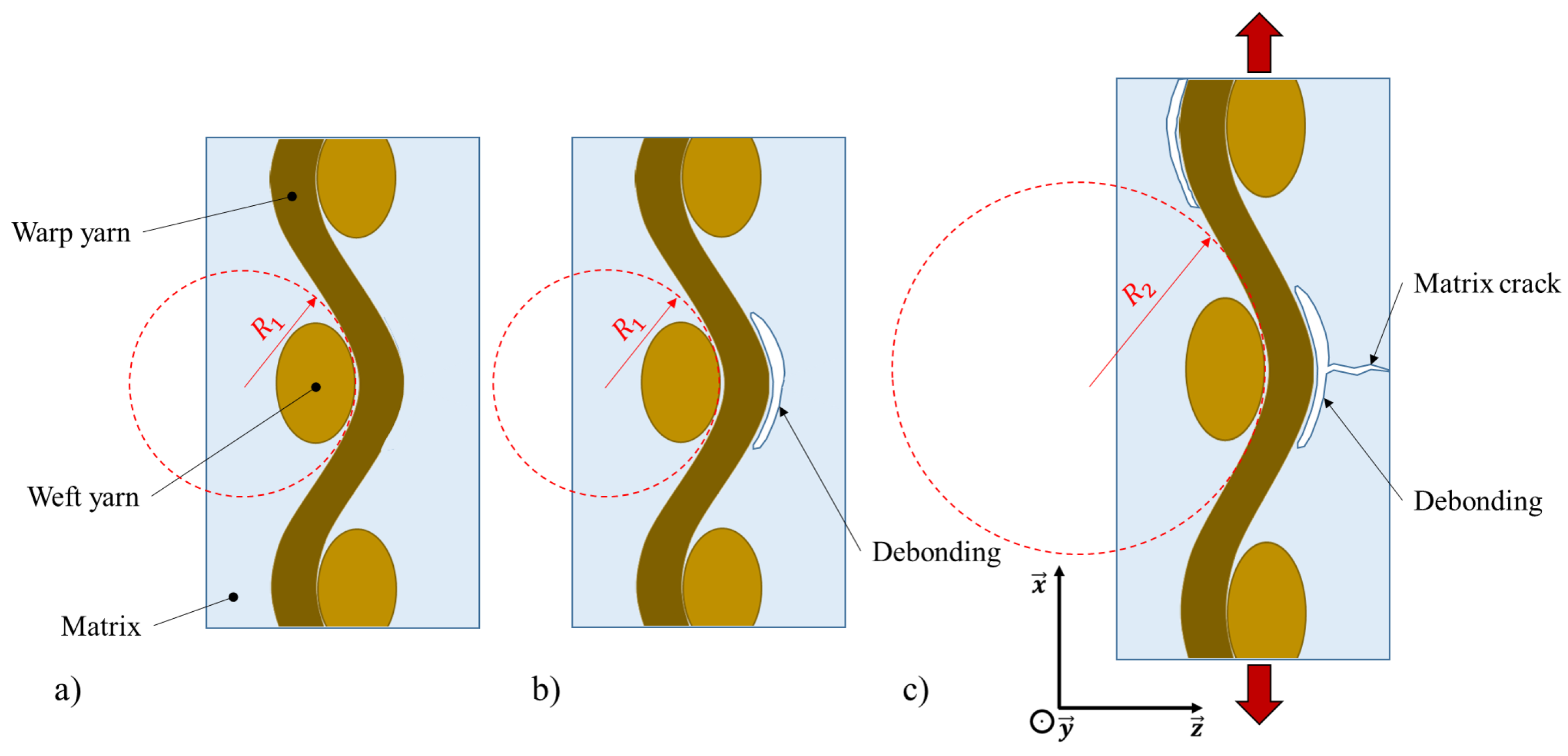
3.4. Evolution of the Curvature Radius of the Yarns
3.4.1. Micro-CT Analysis
3.4.2. Finite Element Calculations
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Vijayan, R.; Krishnamoorthy, A. Review On Natural Fiber Reinforced Composites. Mater. Today Proc. 2019, 16, 897–906. [Google Scholar] [CrossRef]
- Li, M.; Pu, Y.; Thomas, V.M.; Yoo, C.G.; Ozcan, S.; Deng, Y.; Nelson, K.; Ragauskas, A.J. Recent advancements of plant-based natural fiber–reinforced composites and their applications. Compos. Part B Eng. 2020, 200, 108254. [Google Scholar] [CrossRef]
- Zwawi, M. A Review on Natural Fiber Bio-Composites, Surface Modifications and Applications. Molecules 2021, 26, 404. [Google Scholar] [CrossRef] [PubMed]
- Faruk, O.; Bledzki, A.K.; Fink, H.-P.; Sain, M. Biocomposites reinforced with natural fibers: 2000–2010. Prog. Polym. Sci. 2012, 37, 1552–1596. [Google Scholar] [CrossRef]
- Koronis, G.; Silva, A.; Fontul, M. Green composites: A review of adequate materials for automotive applications. Compos. Part B Eng. 2013, 44, 120–127. [Google Scholar] [CrossRef]
- La Mantia, F.P.; Morreale, M. Green composites: A brief review. Compos. Part A Appl. Sci. Manuf. 2011, 42, 579–588. [Google Scholar] [CrossRef]
- Madsen, B.; Hoffmeyer, P.; Thomsen, A.B.; Lilholt, H. Hemp yarn reinforced composites–I. Yarn characteristics. Compos. Part A Appl. Sci. Manuf. 2007, 38, 2194–2203. [Google Scholar] [CrossRef]
- Manaia, J.P.; Manaia, A.T.; Rodriges, L. Industrial Hemp Fibers: An Overview. Fibers 2019, 7, 106. [Google Scholar] [CrossRef]
- Horne, M.R.L. Bast fibres: Hemp cultivation and production. In Handbook of Natural Fibres; Elsevier: Amsterdam, The Netherlands, 2012; pp. 114–145. [Google Scholar] [CrossRef]
- Lotfi, A.; Li, H.; Dao, D.V.; Prusty, G. Natural fiber–reinforced composites: A review on material, manufacturing, and machinability. J. Thermoplast. Compos. Mater. 2021, 34, 238–284. [Google Scholar] [CrossRef]
- Bensadoun, F.; Vallons, K.A.M.; Lessard, L.B.; Verpoest, I.; Van Vuure, A.W. Fatigue behaviour assessment of flax–epoxy composites. Compos. Part A Appl. Sci. Manuf. 2016, 82, 253–266. [Google Scholar] [CrossRef]
- Borrello, M.; Mele, M.; Campana, G.; Secchi, M. Manufacturing and characterization of hemp-reinforced epoxy composites. Polym. Compos. 2020, 41, 2316–2329. [Google Scholar] [CrossRef]
- de Vasconcellos, D.S.; Touchard, F.; Chocinski-Arnault, L. Tension–tension fatigue behaviour of woven hemp fibre reinforced epoxy composite: A multi-instrumented damage analysis. Int. J. Fatigue 2014, 59, 159–169. [Google Scholar] [CrossRef]
- El Sawi, I.; Fawaz, Z.; Zitoune, R.; Bougherara, H. An investigation of the damage mechanisms and fatigue life diagrams of flax fiber-reinforced polymer laminates. J. Mater. Sci. 2014, 49, 2338–2346. [Google Scholar] [CrossRef]
- Krishnasamy, P.; Rajamurugan, G.; Aravindraj, S.; Sudhagar, P.E. Vibration and Wear Characteristics of Aloevera/Flax/Hemp Woven Fiber Epoxy Composite Reinforced with Wire Mesh and BaSO4. J. Nat. Fibers 2020, 19, 2885–2901. [Google Scholar] [CrossRef]
- Neves, A.C.C.; Rohen, L.A.; Mantovani, D.P.; Carvalho, J.P.R.G.; Vieira, C.M.F.; Lopes, F.P.D.; Simonassi, N.T.; da Luz, F.S.; Monteiro, S.N. Comparative mechanical properties between biocomposites of Epoxy and polyester matrices reinforced by hemp fiber. J. Mater. Res. Technol. 2020, 9, 1296–1304. [Google Scholar] [CrossRef]
- Newman, R.H. Auto-accelerative water damage in an epoxy composite reinforced with plain-weave flax fabric. Compos. Part A Appl. Sci. Manuf. 2009, 40, 1615–1620. [Google Scholar] [CrossRef]
- Shah, D.U. Damage in biocomposites: Stiffness evolution of aligned plant fibre composites during monotonic and cyclic fatigue loading. Compos. Part A Appl. Sci. Manuf. 2016, 83, 160–168. [Google Scholar] [CrossRef]
- Allagui, S.; El Mahi, A.; Rebiere, J.-L.; Beyaoui, M.; Bouguecha, A.; Haddar, M. Effect of Recycling Cycles on the Mechanical and Damping Properties of Flax Fibre Reinforced Elium Composite: Experimental and Numerical Studies. J. Renew. Mater. 2021, 9, 695–721. [Google Scholar] [CrossRef]
- Khalili, P.; Blinzler, B.; Kádár, R.; Blomqvist, P.; Sandinge, A.; Bisschop, R.; Liu, X. Ramie fabric Elium® composites with flame retardant coating: Flammability, smoke, viscoelastic and mechanical properties. Compos. Part A Appl. Sci. Manuf. 2020, 137, 105986. [Google Scholar] [CrossRef]
- Perrier, A.; Touchard, F.; Chocinski-Arnault, L.; Mellier, D. Quantitative analysis by micro-CT of damage during tensile test in a woven hemp/epoxy composite after water ageing. Compos. Part A Appl. Sci. Manuf. 2017, 102, 18–27. [Google Scholar] [CrossRef]
- Kesentini, Z.; El Mahi, A.; Rebiere, J.L.; El Guerjouma, R.; Beyaoui, M.; Haddar, M. Static and Fatigue Tensile Behavior and Damage Mechanisms Analysis in Aged Flax Fiber/PLA Composite. Int. J. Appl. Mech. 2022, 14, 2250080. [Google Scholar] [CrossRef]
- Al-Maharma, A.; Al-Huniti, N. Critical Review of the Parameters Affecting the Effectiveness of Moisture Absorption Treatments Used for Natural Composites. J. Compos. Sci. 2019, 3, 27. [Google Scholar] [CrossRef]
- Chilali, A.; Zouari, W.; Assarar, M.; Kebir, H.; Ayad, R. Effect of water ageing on the load-unload cyclic behaviour of flax fibre-reinforced thermoplastic and thermosetting composites. Compos. Struct. 2018, 183, 309–319. [Google Scholar] [CrossRef]
- Maslinda, A.B.; Abdul Majid, M.S.; Ridzuan, M.J.M.; Afendi, M.; Gibson, A.G. Effect of water absorption on the mechanical properties of hybrid interwoven cellulosic-cellulosic fibre reinforced epoxy composites. Compos. Struct. 2017, 167, 227–237. [Google Scholar] [CrossRef]
- Muñoz, E.; García-Manrique, J.A. Water Absorption Behaviour and Its Effect on the Mechanical Properties of Flax Fibre Reinforced Bioepoxy Composites. Int. J. Polym. Sci. 2015, 2015, 390275. [Google Scholar] [CrossRef]
- Sala, B.; Gabrion, X.; Trivaudey, F.; Guicheret-Retel, V.; Placet, V. Influence of the stress level and hygrothermal conditions on the creep/recovery behaviour of high-grade flax and hemp fibre reinforced GreenPoxy matrix composites. Compos. Part A Appl. Sci. Manuf. 2021, 141, 106204. [Google Scholar] [CrossRef]
- Drouhet, Q.; Touchard, F.; Chocinski-Arnault, L. Influence of Hygrothermal Aging on Mechanical Properties and Damage Mechanisms of Hemp-Reinforced Biocomposites. J. Nat. Fibers 2022, 19, 15404–15421. [Google Scholar] [CrossRef]
- Malloum, A.; Mahi, A.E.; Idriss, M. The effects of water ageing on the tensile static and fatigue behaviors of greenpoxy–flax fiber composites. J. Compos. Mater. 2019, 53, 2927–2939. [Google Scholar] [CrossRef]
- Mak, K.; Fam, A. The effect of wet-dry cycles on tensile properties of unidirectional flax fiber reinforced polymers. Compos. Part B Eng. 2020, 183, 107645. [Google Scholar] [CrossRef]
- Cadu, T.; Van Schoors, L.; Sicot, O.; Moscardelli, S.; Divet, L.; Fontaine, S. Cyclic hygrothermal ageing of flax fibers’ bundles and unidirectional flax/epoxy composite. Are bio-based reinforced composites so sensitive? Ind. Crops Prod. 2019, 141, 111730. [Google Scholar] [CrossRef]
- Ludovic, F. Etude du Vieillissement Hygrothermique des Composites Renforcés de Fibres Naturelles: Approche Experimentale et Modélisation. Ph.D. Thesis, Université de Lorraine, Nancy, France, 2018. [Google Scholar]
- Lemaitre, J. A Continuous Damage Mechanics Model for Ductile Fracture. J. Eng. Mater. Technol. 1985, 107, 83–89. [Google Scholar] [CrossRef]
- Richard, F.; Poilâne, C.; Yang, H.; Gehring, F.; Renner, E. A viscoelastoplastic stiffening model for plant fibre unidirectional reinforced composite behaviour under monotonic and cyclic tensile loading. Compos. Sci. Technol. 2018, 167, 396–403. [Google Scholar] [CrossRef]
- Madra, A.; Adrien, J.; Breitkopf, P.; Maire, E.; Trochu, F. A clustering method for analysis of morphology of short natural fibers in composites based on X-ray microtomography. Compos. Part A Appl. Sci. Manuf. 2017, 102, 184–195. [Google Scholar] [CrossRef]
- Xiong, X.; Shen, S.Z.; Hua, L.; Liu, J.Z.; Li, X.; Wan, X.; Miao, M. Finite element models of natural fibers and their composites: A review. J. Reinf. Plast. Compos. 2018, 37, 617–635. [Google Scholar] [CrossRef]
- Daoud, H.; Rebière, J.-L.; Makni, A.; Taktak, M.; El Mahi, A.; Haddar, M. Numerical and Experimental Characterization of the Dynamic Properties of Flax Fiber Reinforced Composites. Int. J. Appl. Mech. 2016, 8, 1650068. [Google Scholar] [CrossRef]
- Xu, J.; Lomov, S.V.; Verpoest, I.; Daggumati, S.; VAN Paepegem, W.; Degrieck, J.; Olave, M. A progressive damage model of textile composites on meso-scale using finite element method: Static damage analysis. J. Compos. Mater. 2014, 48, 3091–3109. [Google Scholar] [CrossRef]
- Cheung, H.; Ho, M.; Lau, K.; Cardona, F.; Hui, D. Natural fibre-reinforced composites for bioengineering and environmental engineering applications. Compos. Part B Eng. 2009, 40, 655–663. [Google Scholar] [CrossRef]
- Perrier, A.; Ecault, R.; Touchard, F.; Urriza, M.V.; Baillargeat, J.; Chocinski-Arnault, L.; Boustie, M. Towards the development of laser shock test for mechanical characterisation of fibre/matrix interface in eco-composites. Polym. Test. 2015, 44, 125–134. [Google Scholar] [CrossRef]
- Kazemi, M.E.; Shanmugam, L.; Lu, D.; Wang, X.; Wang, B.; Yang, J. Mechanical properties and failure modes of hybrid fiber reinforced polymer composites with a novel liquid thermoplastic resin, Elium®. Compos. Part A Appl. Sci. Manuf. 2019, 125, 105523. [Google Scholar] [CrossRef]
- Orenes Balaciart, S.; Pannier, Y.; Gigliotti, M.; Mellier, D.; Guigon, C. Effect of Temperature on Damage Onset in Three-Dimensional (3D) Woven Organic Matrix Composites for Aero-Engines Application. In Proceedings of the European Conference on Composites Materials (ECCM20), Lausanne, Suisse, 26–30 June 2022. [Google Scholar]
- ImageJ Docs. Trainable Weka Segmentation. Trainable Weka Segmentation n.d. Available online: https://imagej.net/plugins/tws/ (accessed on 24 May 2022).
- Perrier, A.; Touchard, F.; Chocinski-Arnault, L.; Mellier, D. Influence of water on damage and mechanical behaviour of single hemp yarn composites. Polym. Test. 2017, 57, 17–25. [Google Scholar] [CrossRef]
- Placet, V.; Cissé, O.; Lamine Boubakar, M. Nonlinear tensile behaviour of elementary hemp fibres. Part I: Investigation of the possible origins using repeated progressive loading with in situ microscopic observations. Compos. Part A Appl. Sci. Manuf. 2014, 56, 319–327. [Google Scholar] [CrossRef]

| Material | Density (g·cm−3) | Young’s Modulus (MPa) | Ultimate Stress (MPa) | References | |
|---|---|---|---|---|---|
| Longitudinal | Transverse | ||||
| Hemp yarn | 1.48 | 23,000 | 1264 | 601 | [39,40] |
| Elium 188 | 1.01 | 3300 | 76 | [41] | |
| Mechanical Properties | Ambient Storage | Saturated at 60 °C | 15 Wet/Dry Cycles |
|---|---|---|---|
| Initial Young’s modulus (MPa) | 7323 ± 368 | 2428 ± 125 | 2392 ± 179 |
| Ultimate stress (MPa) | 61.8 ± 5.0 | 56.7 ± 2.8 | 47.1 ± 2.4 |
| Maximum strain (%) | 3.4 ± 1.0 | 7.6 ± 0.9 | 6.5 ± 0.4 |
| X-Axis | Y-Axis | |||
|---|---|---|---|---|
| In-Situ | Ex-Situ | In-Situ | Ex-Situ | |
| Loaded state: total strain (%) | 5.38 ± 0.07 | 4.98 ± 0.1 | −1.10 ± 0.13 | −1.11 ± 0.10 |
| Unloaded state: residual strain (%) | 3.70 ± 0.21 | 2.40 ± 0.25 | −0.51 ± 0.05 | −0.50 ± 0.05 |
| Damage Quantification (%) | ||
|---|---|---|
| Large Volume (1620 mm3 = 5 × 108 Voxels) | Small Volume (3.38 mm3 = 1 × 106 Voxels) | |
| Before loading | 2.0 | 2.4 |
| Loaded at 37.6 MPa | 8.9 | 8.5 |
| Unloaded | 6.0 | 6.7 |
| Model Geometry with R1 | Model Geometry with R2 | Deviation (%) | |
|---|---|---|---|
| Curvature radius (mm) | 0.5 | 1.0 | 100 |
| Young’s modulus (MPa) | 5137 | 5577 | 8.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Drouhet, Q.; Touchard, F.; Chocinski-Arnault, L. Tensile Behavior of [0/90]7 Hemp/Elium Biocomposites after Water Aging: In-Situ Micro-CT Testing and Numerical Analysis. Micro 2023, 3, 496-509. https://doi.org/10.3390/micro3020033
Drouhet Q, Touchard F, Chocinski-Arnault L. Tensile Behavior of [0/90]7 Hemp/Elium Biocomposites after Water Aging: In-Situ Micro-CT Testing and Numerical Analysis. Micro. 2023; 3(2):496-509. https://doi.org/10.3390/micro3020033
Chicago/Turabian StyleDrouhet, Quentin, Fabienne Touchard, and Laurence Chocinski-Arnault. 2023. "Tensile Behavior of [0/90]7 Hemp/Elium Biocomposites after Water Aging: In-Situ Micro-CT Testing and Numerical Analysis" Micro 3, no. 2: 496-509. https://doi.org/10.3390/micro3020033
APA StyleDrouhet, Q., Touchard, F., & Chocinski-Arnault, L. (2023). Tensile Behavior of [0/90]7 Hemp/Elium Biocomposites after Water Aging: In-Situ Micro-CT Testing and Numerical Analysis. Micro, 3(2), 496-509. https://doi.org/10.3390/micro3020033






