Thermodynamic Assessment and Solubility of Ni in LBE Coolants
Abstract
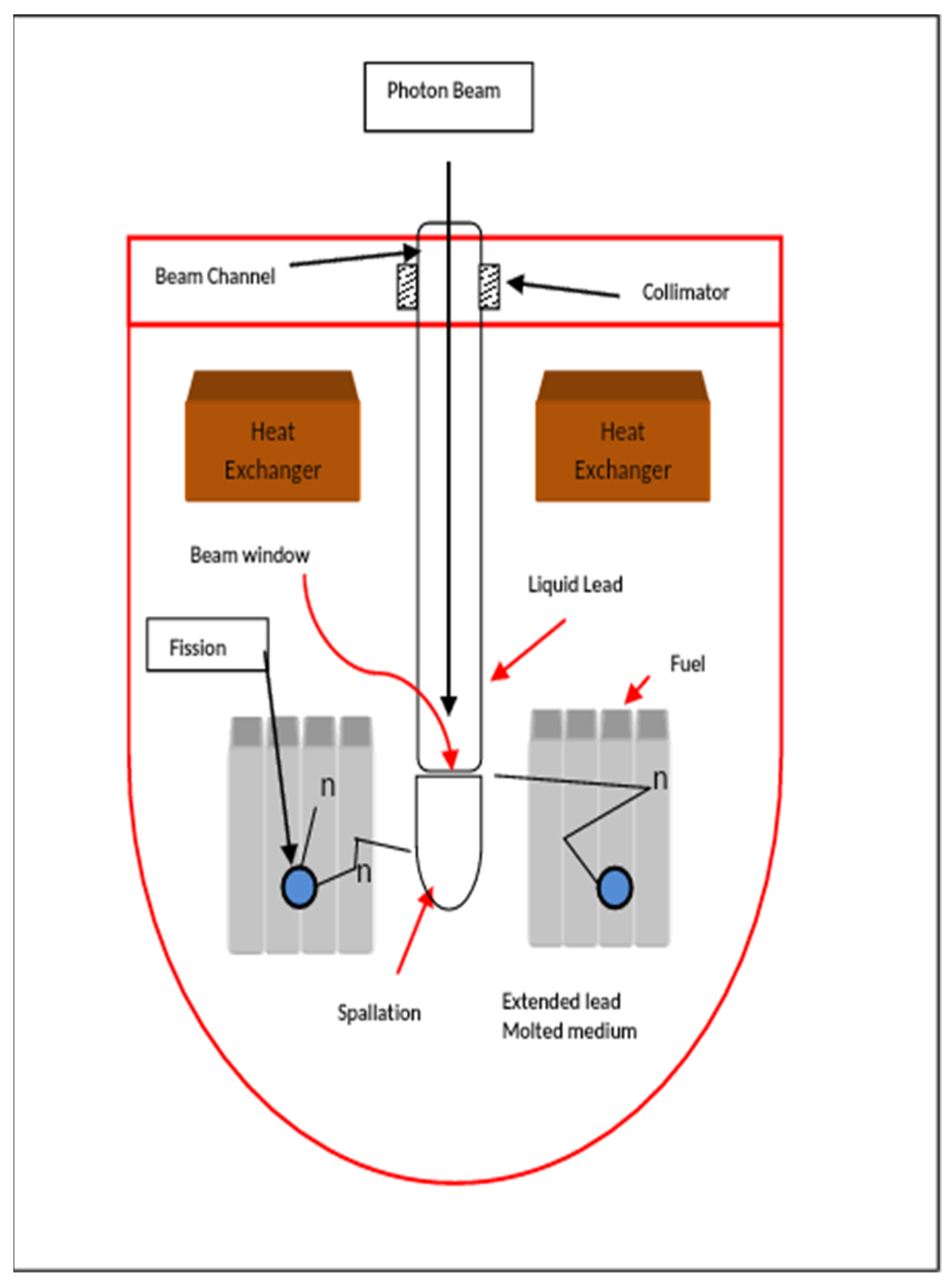
1. Introduction
2. Thermodynamic Modeling
2.1. Thermodynamic Assessment of Bi-Pb System
2.2. Thermodynamic Assessment of Bi-Ni System
3. Interaction of Pb/Bi with Ni
- ➣
- Therefore, to understand different phases formed by the interaction of Pb/Bi and Ni, the Bi-Ni-Pb ternary was computed. As discussed in Section 2.1 and Section 2.2, respectively, Bi-Pb and Bi-Ni binaries of this ternary were re-optimized using the experimental data acquired during this work. The third binary required for computing the Bi-Ni-Pb ternary is Ni-Pb. This binary system does not have any intermetallic compound and its thermodynamic assessment can be reliably obtained from a thermodynamic description of unary elements and the binary phase diagram data available in the literature. Therefore, no new experimental data was acquired for this system. The phase description for Ni-Pb system was taken from Ghosh et al. [49]. The modeling by Ghosh et al. considers numerous sets of phase diagram data and thermodynamic information from the literature [44,46,50,51,52,53]. For the sake of clarity, the Ni-Pb system is shown in the Figure 4. The Ni-Pb binary has a liquid phase miscibility gap, does not have any intermetallic compound and the FCC-Ni phase dissolves a small amount of Pb. In the absence of experimental thermodynamic or phase diagram data for the Bi-Ni-Pb ternary, computation of this system was based on the following assumptions:
- ➣
- As crystal structures of all the intermetallic compounds, Bi3Ni, BiNi and BiPb3, are quite different, they were assumed to be present as pure binary compounds in the ternary system.
- ➣
- The liquid phase and end member solubilities were modeled by using only binary interaction parameters
- ➣
- In absence of any experimental data to indicate the presence of stable ternary compounds, no new ternary phase was considered.
- ➣
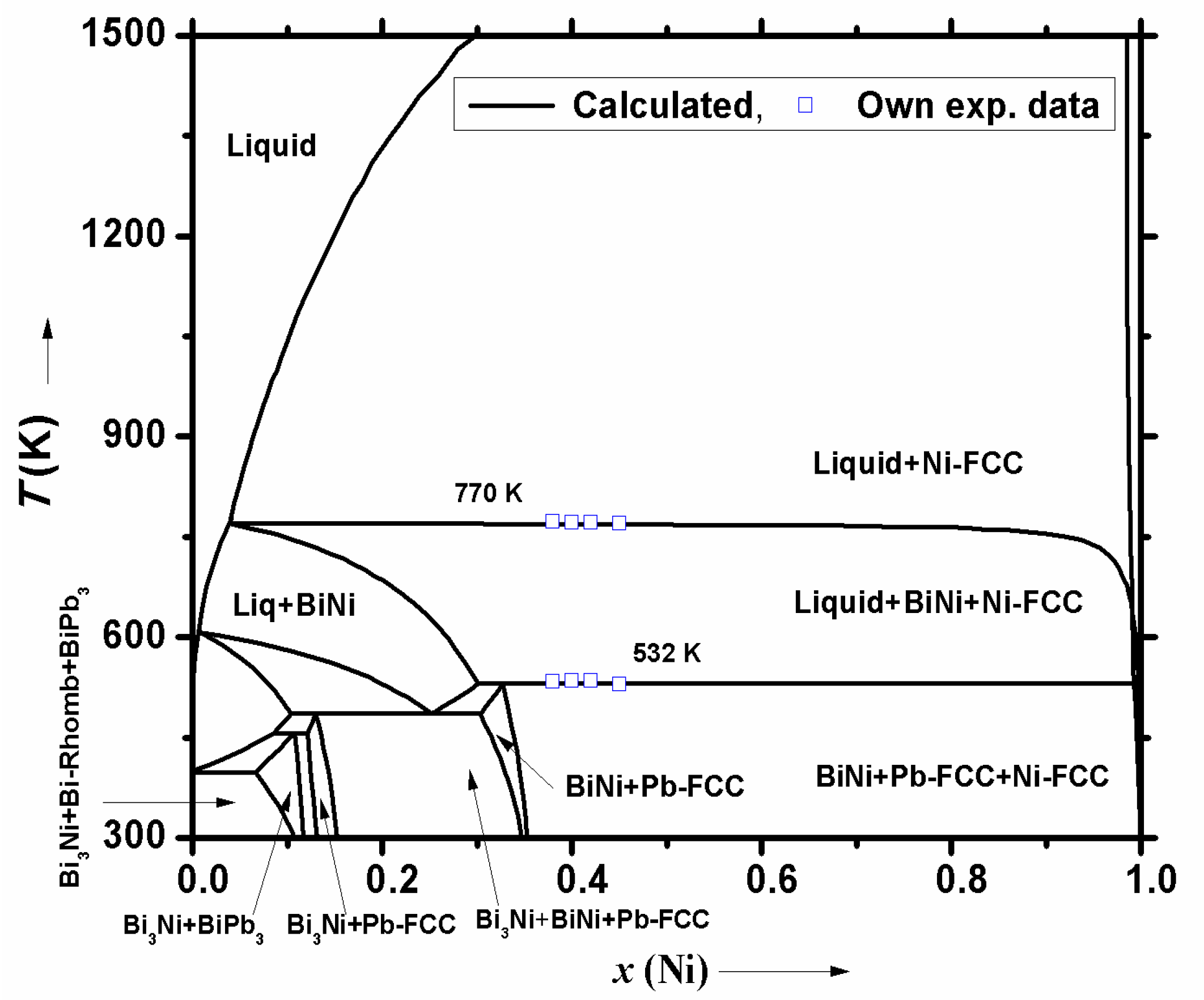
- To understand Ni interaction with LBE at different temperatures, a pseudo binary diagram along the isopleth LBE-Ni was computed (Figure 5). As there was no available experimental data in the literature on this ternary system, it was decided to study transition temperatures of this pseudo-binary system, using DTA. For this purpose, alloys with different compositions of Ni in LBE (0.38 < x(Ni) < 0.46) were prepared. The alloys were prepared by melting desired ratios of lead, bismuth and a fine powder of nickel metal. The liquid mixture was slowly cooled in a furnace to ambient temperature. DTA analysis of these alloys was carried out using an indigenously fabricated DTA instrument. The temperatures of phase transitions were obtained from the extrapolated peak onset temperature during heating of alloys at 5K/min. The present DTA results of selected compositions are plotted in Figure 5 of pseudo-binary phase diagram of LBE-Ni system. Our experimental data shows reasonable agreement with the computed pseudo binary diagram. It proves that the above listed assumptions used for the computation of the ternary system were acceptable. Hence, inferences based on the ternary database, generated using binary interactions, should be reliable.
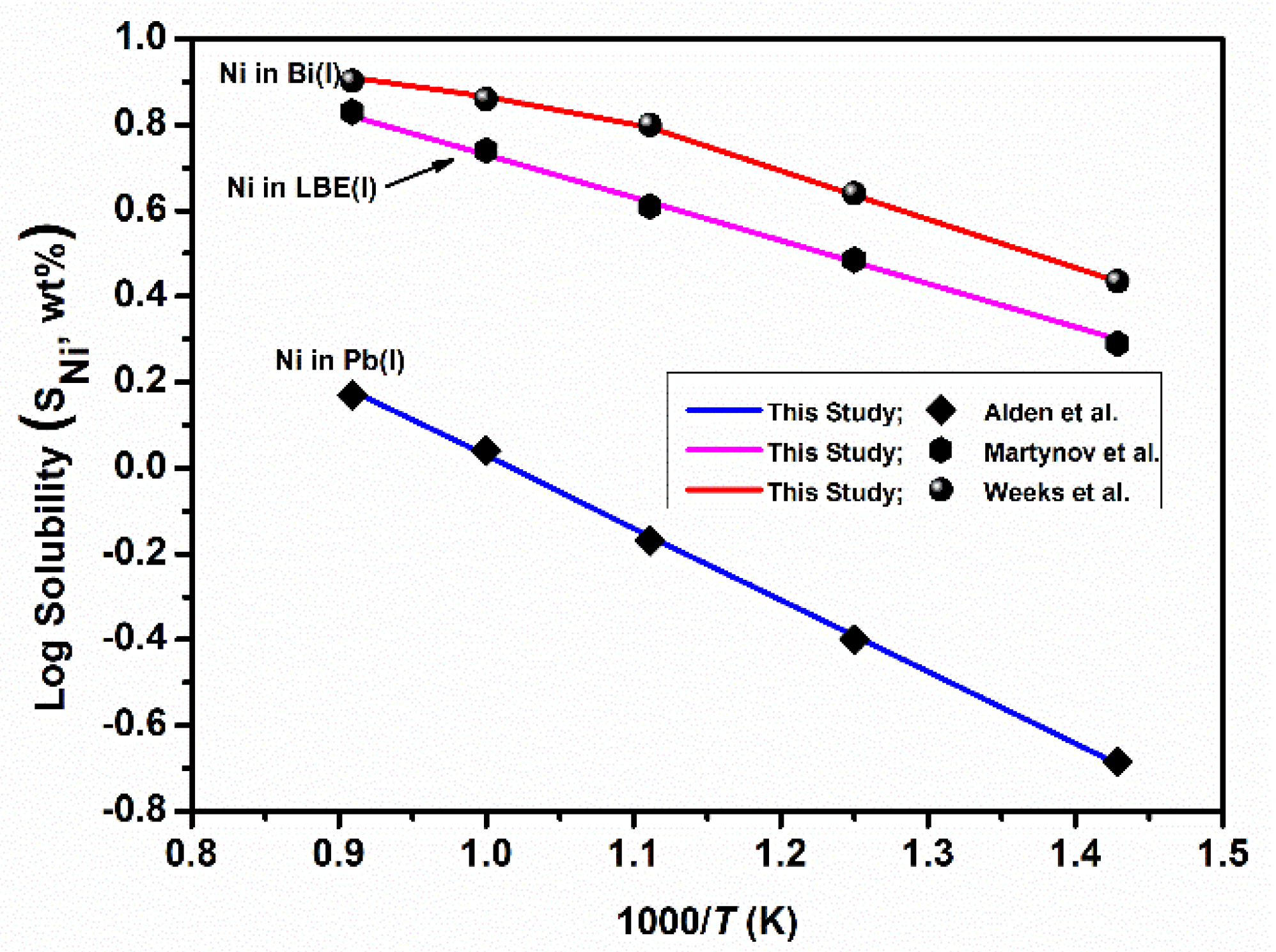
4. Solubility of Nickel in LBE/Bi/Pb
5. Mechanisms of Liquid Metal/Alloy Corrosion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kelly, J.E. Generation IV International Forum: A decade of progress through international cooperation. Prog. Nucl. Energy 2014, 77, 240–246. [Google Scholar] [CrossRef]
- Dulera, I.V.; Sinha, R.K. High temperature reactor. J. Nucl. Mater. 2008, 383, 183–188. [Google Scholar] [CrossRef]
- Rubbia, C.; Aleixandre, J.; Andriamonje, S. A European Roadmap for Developing Accelerator Driven Systems (ADS) for Nuclear Incineration; The European Technical Working Group: Lungotevere Thaon di Revel, Italy, 2001; ISBN 88-8286-008-6. [Google Scholar]
- Arkhipov, V. Future Nuclear Energy Systems: Generating Electricity, Burning Wastes; International Atomic Energy Agency: Vienna, Austria, 2007. [Google Scholar]
- OECD-NEA. Accelerator-Driven Systems (ADS) and Fast Reactors (FR) in Advanced Nuclear Fuel Cycles—A Comparative Study; NEA-3109; Nuclear Energy Agency, Organization for Economic Cooperation and Development: Paris, France, 2002. [Google Scholar]
- Bauer, G.S.; Dai, Y.; Maloy, S.Y.; Mansur, L.K.; Ullmaier, H. Summary of the Fourth International Workshop on Spallation Materials Technology (IWSMT-4). J. Nucl. Mater. 2001, 296, 321–325. [Google Scholar] [CrossRef]
- Gabriel, T.A. The National Spallation Neutron Source Target Station. In Proceedings of the Topical Meeting on Nuclear Applications of Accelerator Technology, Albuquerque, NM, USA, 16–20 November 1997. [Google Scholar]
- Gromov, B.F. Heavy Liquid-Metal Coolants in the Nuclear Technologies. Collection of the Conference Reports in Two Volumes; SSC RF-IPPE: Obninsk, Russia, 1999; Volume 1. [Google Scholar]
- Zhang, J.; Li, N. Review of Studies on Fundamental Issues in LBE Corrosion; LA-UR-04-0869; Los Alamos National Laboratory: Los Alamos, NM, USA, 2004. [Google Scholar]
- Li, N. Active control of oxygen in molten lead–bismuth eutectic systems to prevent steel corrosion and coolant contamination. J. Nucl. Mater. 2002, 300, 73–81. [Google Scholar] [CrossRef]
- Bardes, B.P. (Ed.) Properties and Selection: Non-Ferrous Alloys and Pure Metals, Metals Handbook; American Society for Metals: Materials Park, OH, USA, 1979; Volume 2. [Google Scholar]
- Zhang, J. A review of steel corrosion by liquid lead and lead–bismuth. Corros. Sci. 2009, 51, 1207–1227. [Google Scholar] [CrossRef]
- Cathcart, J.V.; Manly, W.D. The Mass-Transfer Properties of Various Metals and Alloys in Liquid Lead; Oak Ridge National Laboratory Technical Report, ORNL–2009; Oak Ridge National Laboratory: Oak Ridge, TN, USA, 1956. [Google Scholar]
- Mueller, G.; Heinzel, A.; Schumacher, G.; Weisenburger, A. Control of oxygen concentration in liquid lead and lead–bismuth. J. Nucl. Mater. 2003, 321, 256–262. [Google Scholar] [CrossRef]
- Benamati, G.; Gessi, A.; Zhang, P.Z. Corrosion experiments in flowing L B E at 450°. J. Nucl. Mater. 2006, 356, 198–202. [Google Scholar] [CrossRef]
- Ballinger, R.G.; Lim, J.; Li, N. The development and production of functionally graded composite cladding and structural materials for lead-bismuth service. Trans. Am. Nucl. Soc. 2006, 94, 761. [Google Scholar]
- Romano, A.J.; Klamut, C.J.; Gurinski, D.H. The Investigation of Container Materials for bi and pb Alloys. Part I. Thermal Convection Loops; Brookhaven National Laboratory Technical Report, BNL 811(T-313); Brookhaven National Laboratory: Upton, NY, USA, 1963. [Google Scholar]
- Schroer, C.; Wedemeyer, O.; Novotny, J.; Skrypnik, A.; Konys, J. Selective leaching of nickel and chromium from Type 316 austenitic steel in oxygen containing lead–bismuth eutectic (LBE). Corros. Sci. 2014, 84, 113–124. [Google Scholar] [CrossRef]
- Jing, L. Stress Corrosion Behavior of T91 and 316L Steels in Liquid Lead-Bismuth Eutectic. Ph.D. Thesis, University of Science and Technology of China, Hefei, China, 2015. [Google Scholar]
- Gao, Y.; Takahashi, M.; Nomura, M. Experimental study on diffusion of Ni in Lead bismuth eutectic (LBE). Energy Procedia 2015, 71, 313–319. [Google Scholar] [CrossRef]
- Gorynin, I.V.; Karzov, G.P.; Markov, V.G.; Lavrukhin, V.S.; Yakovlev, V.Y. Structural materials for power plants with heavy liquid metals as coolants. In Heavy Liquid Metal Coolants in Nuclear Technology (HLMC-98); SSC RF-IPPE: Obninsk, Russia, 1998; Volume 1, pp. 120–132. [Google Scholar]
- Yachmenev, G.S.; Rusanov, A.E.; Gromov, B.F.; Belomyttsev, Y.S.; Skvortsov, N.S.; Demishonkov, A.P. Problems of structural materials corrosion in Lead-Bismuth coolant. In Heavy Liquid Metal Coolants in Nuclear Technology (HLMC-98); SSC RF-IPPE: Obninsk, Russia, 1998; Volume 1, pp. 133–140. [Google Scholar]
- Asher, C.; Davies, D.; Beetham, S.A. Compatibility of structural materials with molten lead. Corros. Sci. 1977, 17, 545–557. [Google Scholar] [CrossRef]
- Muller, G.; Schumacher, G.; Zimmermann, F. Investigation on oxygen controlled liquid lead corrosion of surface treated steels. J. Nucl. Mater. 2000, 278, 85–95. [Google Scholar] [CrossRef]
- Glasbrenner, H.; Konys, J.; Mueller, G.; Rusanov, A. Corrosion investigations of steels in flowing lead at 400 °C and 550 °C. J. Nucl. Mater. 2001, 296, 237–242. [Google Scholar] [CrossRef]
- Cathcart, J.V.; Manly, W.D. The mass transfer properties of various metals and alloys in liquid lead. Corrosion 1956, 12, 43–47. [Google Scholar] [CrossRef]
- Fazio, C.; Sobolev, V.P.; Aerts, A.; Gavrilov, S.; Lambrinou, K.; Schuurmans, P.; Gessi, A.; Agostini, P.; Ciampichetti, A.; Martinelli, L.; et al. Handbook on Lead-Bismuth Eutectic Alloy and Lead Properties, Materials Compatibility, Thermal-Hydraulics and Technologies; Organisation for Economic Co-Operation and Development: Paris, France, 2007; ISBN 978-92-64-99002-9. [Google Scholar]
- Dinsdale, A.T. SGTE data for pure elements. Calphad 1991, 15, 317–425. [Google Scholar] [CrossRef]
- TCC. Thermo-Calc Software User’s Guide, Version R; TCC: Stockholm, Sweden, 2006. [Google Scholar]
- Redlich, O.; Kister, A.T. Algebraic representation of thermodynamic properties and the classification of solutions. Ind. Eng. Chem. 1948, 40, 345–348. [Google Scholar] [CrossRef]
- Boa, D.; Ansara, I. Thermodynamic assessment of the ternary system Bi-In-Pb. Thermochimi. Acta 1998, 314, 79–83. [Google Scholar] [CrossRef]
- Yoon, S.W.; Lee, H.M. A thermodynamic study of phase equilibria in the Sn-Bi-Pb solder system. Calphad 1998, 22, 167–178. [Google Scholar] [CrossRef]
- Wojtaszek, Z. Research on intermediate phases in Bi-Pb system. Zeszyty Nauk. Uni. Jagiell. Ser. Nauk. Mat. Przyrod. Mat. Fiz. Chem. 1956, 6, 151–161. [Google Scholar]
- Predel, B.; Schwenmann, W. Analysis of the thermodynamic properties of solid Pb-Bi alloy. Z. Metallkde. 1967, 58, 553–557. [Google Scholar]
- Fon, H.H.; Hanemann, H. Information on lead-Bismuth and lead-antimony-bismuth systerns. Z. Metallkd. 1940, 32, 112–117. [Google Scholar]
- Hayasi, M. An X-Ray determination of solid-solubility of Bi in Pb. Nippon Kinzoku Gakkai-Shi 1939, 3, 123–125. [Google Scholar]
- Agarwal, R.; Samui, P.; Jat, R.A.; Singh, Z.; Sen, B.K. Calorimetric investigation of Pb–Bi system. J. Alloys Compd. 2010, 490, 150–154. [Google Scholar] [CrossRef]
- Agarwal, R.; Samui, P. Enthalpy increment and heat capacity of Pb3Bi. J. Alloys Compd. 2010, 508, 333–337. [Google Scholar] [CrossRef]
- Nosek, M.V.; Yan-Sho-Syan, G.V.; Semibratora, N.M. The lead-bismuth phase diagram. Trudy Inst. Khim. Akad. Nauk. Kaz, USSR 1967, 15, 150–157. [Google Scholar]
- Takase, T. Equilibrium diagram of Pb-Bi system. Nippon Kinzoku Gakkai-Shi 1937, 1, 143–150. [Google Scholar]
- Samui, P.; Agarwal, R.; Padhi, A.; Kulkarni, S.G. Thermodynamic investigations of Bi-Ni system Part-I. J. Chem. Therm. 2013, 57, 470–476. [Google Scholar] [CrossRef]
- Agarwal, R.; Samui, P.; Kulkarni, S.G. Thermodynamic investigations of Bi-Ni system Part-II. J. Chem. Therm. 2013, 57, 476–484. [Google Scholar] [CrossRef]
- Vassilev, G.P.; Liu, X.J.; Ishida, K. Experimental studies and thermodynamic optimization of the Ni-Bi system. J. Phase Equil. Diffusion 2005, 26, 161–168. [Google Scholar] [CrossRef]
- Voss, G. Die Legierungen: Nickel-Zinn, Nickel-Blei, Nickel-Thallium, Nickel-Wismut, Nickel-Chrom, Nickel-Magnesium, Nickel-Zink und Nickel-Cadmium. Z. Anorg. Chem. 1908, 57, 52–58. (In German) [Google Scholar] [CrossRef]
- Seo, S.K.; Cho, M.G.; Lee, H.M. Thermodynamic assessment of the Ni-Bi binary system and phase equilibria of the Sn-Bi-Ni ternary system. J. El. Mat. 2007, 36, 1536–1544. [Google Scholar] [CrossRef]
- Portevin, M.A. The alloys of nickel and bismuth. Rev. Metall. 1908, 5, 110–120. [Google Scholar] [CrossRef]
- Predel, B.; Ruge, H. Bildungsenthalpien und bindungsverhältnisse in einigen intermetallischen verbindungen vom NiAs-Typ. Thermochim. Acta 1972, 3, 411–418. [Google Scholar] [CrossRef]
- Vassilev, G.P.; Lilova, K.I. Notes on some supposed transitions of the phase NiBi. Cryst. Res. Technol. 2009, 44, 25–30. [Google Scholar] [CrossRef]
- Ghosh, G. Thermodynamic modeling of the nickel-lead-tin system. Metall. Mater. Trans. A 1999, 30, 1481–1494. [Google Scholar] [CrossRef]
- Alden, T.; Stevenson, D.A.; Wulff, J. Solubility of Nickel and Chromium in Molten Lead. J. Trans. Metall. Soc AIME 1958, 212, 15–17. [Google Scholar]
- Fleischer, B.; Elliot, J.F. The solubility of iron-nickel alloys in liquid lead:700 °C to 1100 °C, In proceeding of the physical chemistry of metallic solutions and intermetallics compounds. Natl. Phys. Lab. 1959, 1, 2–12. [Google Scholar]
- Miller, K.O.; Elliot, J.F. Phase relationships in the systems Fe-Pb-Ni, Fe-Ni-C(sat) and Fe-Pb-Ni-C,1300 to 1550 °C. Trans. Metall. Soc. AIME 1960, 218, 900–910. [Google Scholar]
- Cavanaugh, C.R.; Elliot, J.F. The activity of nickel in liquid Pb-Ni alloys (700–1000 °C). Trans. Metall. Soc. AIME 1964, 230, 633–638. [Google Scholar]
- International Atomic Energy Agency. Comparative Assessment of Thermophysical and Thermohydraulic Characteristics of Lead, Lead-Bismuth and Sodium Coolants for Fast Reactors; IAEA-TECDOC-1289; IAEA: Vienna, Austria, 2002. [Google Scholar]
- Martynov, P.N.; Ivanov, K.D. Properties of Lead–Bismuth Coolant and Perspectives of Non-Electric Applications of Lead-Bismuth Reactor; IAEA-TECDOC-1056; IAEA: Vienna, Austria, 1997; pp. 177–184. [Google Scholar]
- Weeks, J.R. Liquid Metal Compatibility of Structural Materials with Liquid Lead bismuth and Mercury. In Proceedings of the 1997 TMS Annual Meeting, Orlando, FL, USA, 9–13 February 1997. [Google Scholar]
- Zhang, J.; Li, N. Review of the studies on fundamental issues in LBE corrosion. J. Nucl. Mater. 2008, 373, 351–377. [Google Scholar] [CrossRef]
- Gossé, S. Thermodynamic assessment of solubility and activity of iron, chromium, and nickel in lead bismuth eutectic. J. Nucl. Mater. 2014, 449, 122–131. [Google Scholar] [CrossRef]
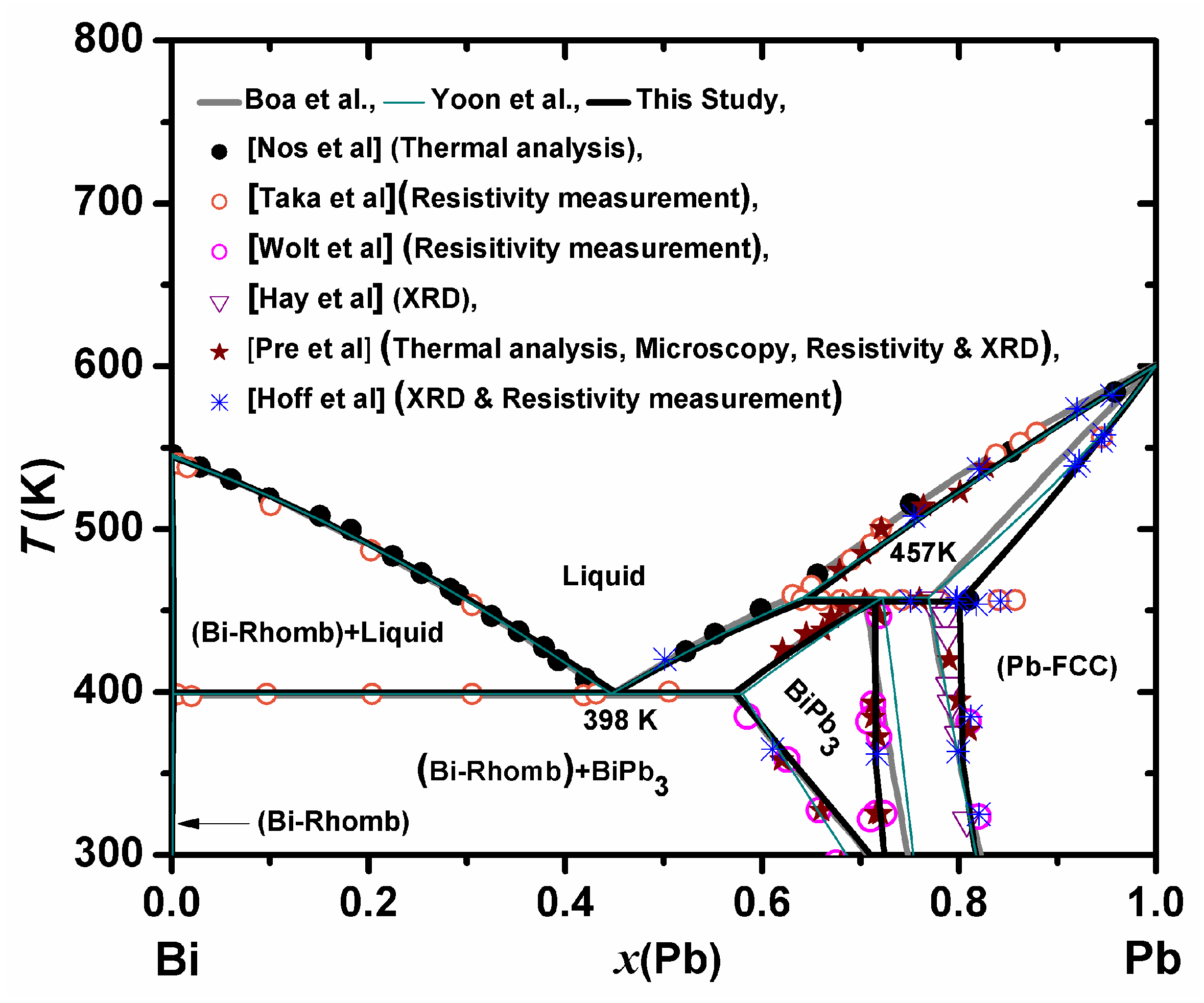
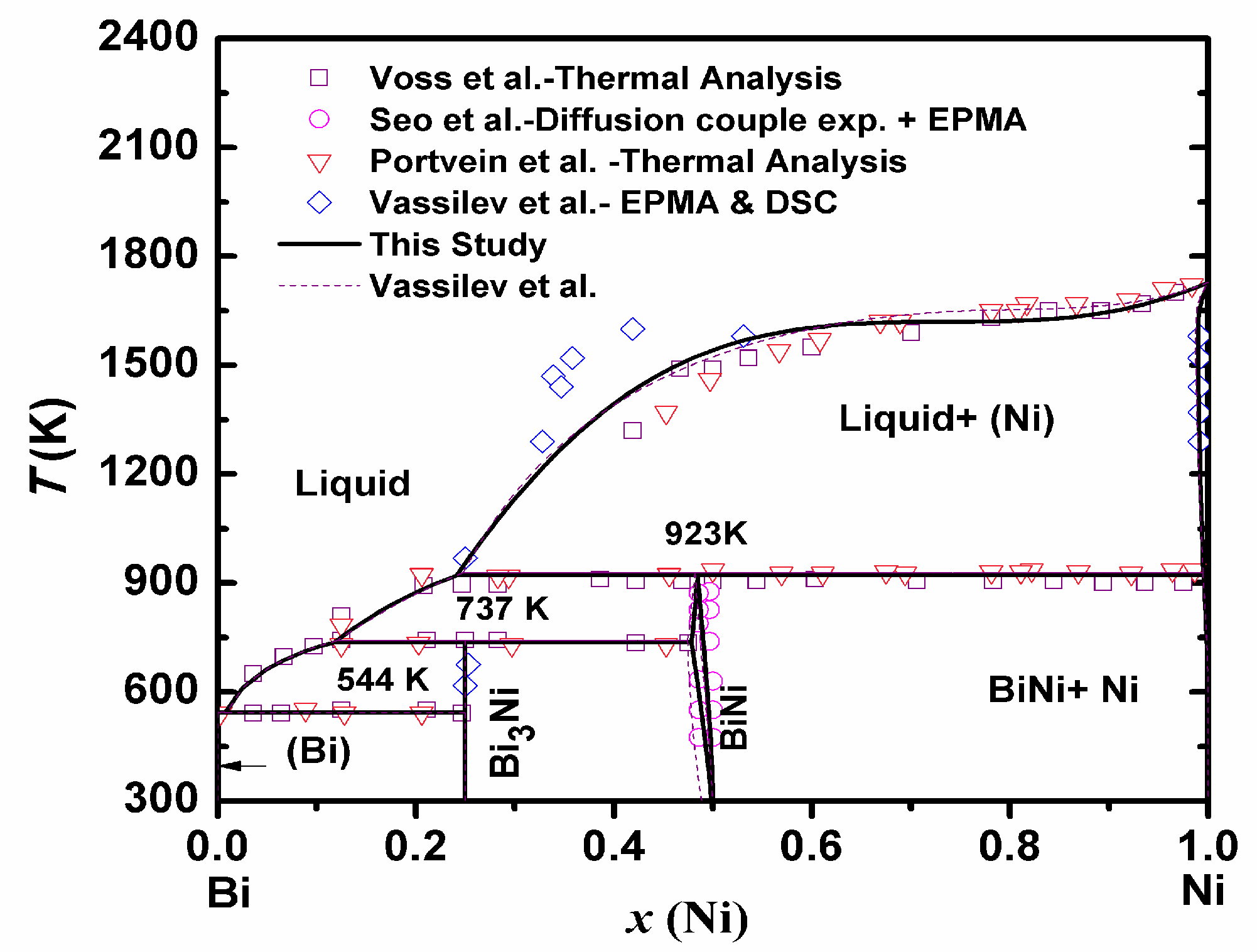
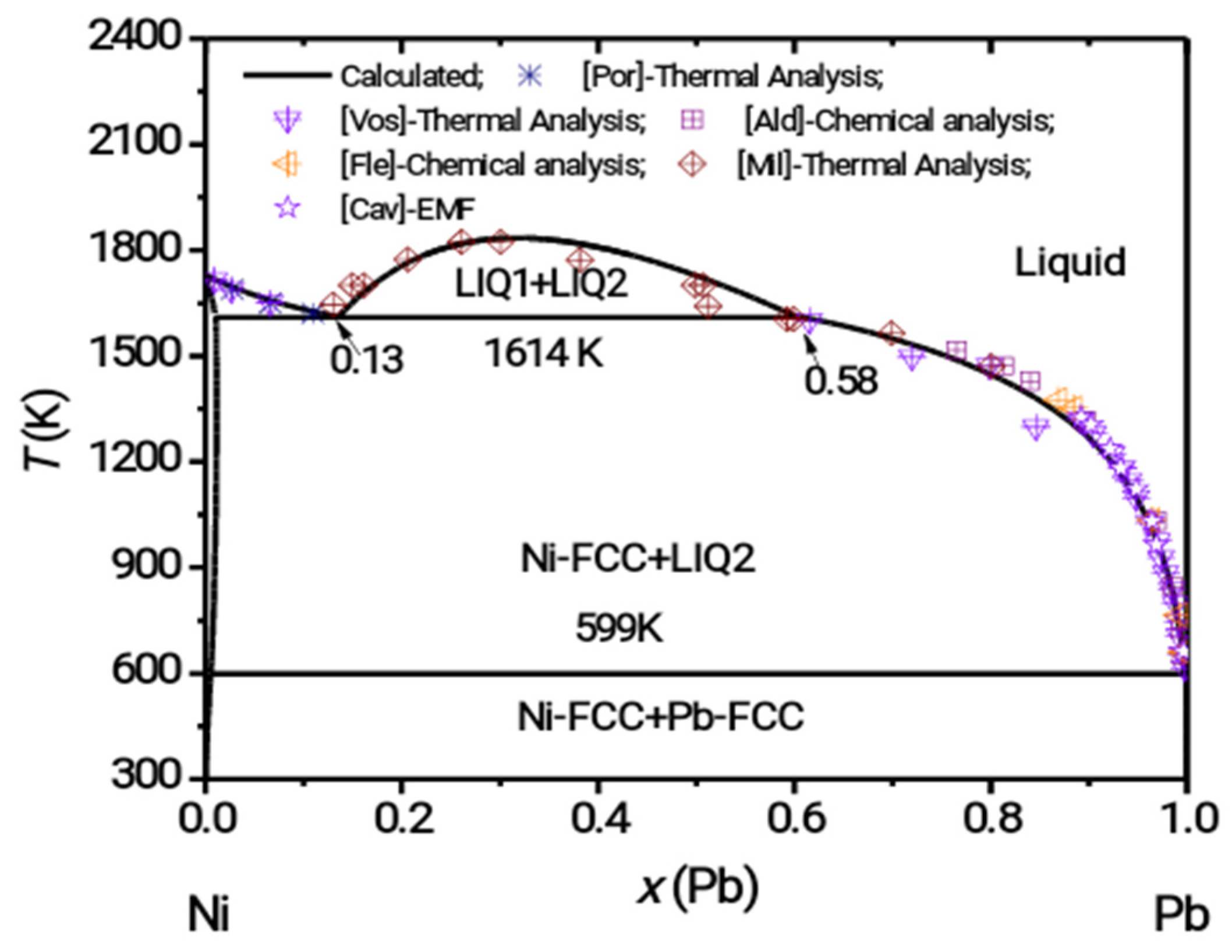
| Reaction | Type | T/K | Composition, x(Pb) | References | ||
|---|---|---|---|---|---|---|
| L + Pb-FCC = BiPb3 | Peritectic | 457.5 | 0.62 | 0.78 | 0.719 | [31] |
| 457 | 0.64 | 0.769 | 0.72 | [32] | ||
| 457 | 0.63 | 0.819 | 0.719 | This Study | ||
| L = BiPb3 + (Bi-Rhomb) | Eutectic | 398.5 | 0.446 | 0.58 | 0.005 | [31] |
| 398.5 | 0.446 | 0.58 | 0.005 | [32] | ||
| 399 | 0.446 | 0.58 | 0.005 | This Study | ||
| Phase and Model | Thermodynamic Parameters |
|---|---|
| LIQUID [Bi,Ni,Pb]1 | = −19992.628 + 99.11350 × T − 10.0574 × T × ln(T) = 1.2944 − 191.9533 × T + 25.5048 × T × ln(T) = 12521.6048 − 1.5602 × T = −5050.202 + 1.85 × T = −1050.01 + 1.18 × T = +31532.257 − 2.42 × T = +10459.949 − 2.49 × T = −10927.18 + 7.952 × T = −3732.467 − 0.109 × T |
| BiPb3 [Bi, Pb]1 | = −3450.04 + 9.781 × T − 2.5001 × T × ln(T) − 496987.08/T = −1.801 × T |
| Pb-FCC [Bi, Pb]1 | = −3550.05 + 1.11 × T |
| Bi-Rhomb [Bi, Pb]1 | = 3461.56 |
| Bi3Ni [Bi]3 [Ni]1 | = −2450.002 + 9.1195 × T − 1.9 × T × ln(T) + 0.75GBi-rhombo + 0.25GNi-FCC |
| BiNi[Bi]0.3334 [Ni]0.3333[Bi,Va]0.3333 | = 0.667GBi-rhombo + 0.333GNi-FCC = −4250 + 13.37 × T − 1.8 × T × ln(T) + 0.333GBi-rhombo + 0.333GNi-FCC = −1647 + 1.434 × T |
| Ni-FCC [Bi,Ni,Pb]1 | = −20000 + 12.5 × T = +29980 + 0.59 × T = −20000 + 25 × T |
| Reaction | Type | T/K | Composition, x(Ni) | References | ||
|---|---|---|---|---|---|---|
| BiNi = (Ni-FCC) + Liq | Peritectic | 920.7 | 0.759 | 0.515 | 0.00485 | [43] |
| 919.0 | 0.762 | 0.510 | 0.022 | [48] | ||
| 921.0 | 0.76 | 0.517 | 0.005 | This work | ||
| Bi3Ni = BiNi + Liq | Peritectic | 737.2 | 0.881 | 0.75 | 0.52 | [43] |
| 738.0 | 0.877 | 0.75 | 0.515 | [48] | ||
| 737.5 | 0.878 | 0.75 | 0.52 | This work | ||
| Liq = Bi3Ni + (Bi-Rhomb) | Eutectic | 542.8 | 0.75 | 0.993 | 1.0 | [43] |
| 543.0 | 0.75 | 0.993 | 1.0 | [48] | ||
| 543 | 0.75 | 0.991 | 1.0 | This work | ||
| Temp. | Fe Solubility | Cr Solubility | Ni Solubility | ||||||
|---|---|---|---|---|---|---|---|---|---|
| T(K) | Pb(l) | LBE(l) | Bi(l) | Pb(l) | LBE(l) | Bi(l) | Pb(l) | LBE(l) | Bi(l) |
| 600 | −8.03 | −6.76 | −5.78 | −8.86 | −5.36 | −5.07 | −1.08 | −0.6 | −0.24 |
| 700 | −6.78 | −5.71 | −4.84 | −7.27 | −4.64 | −4.20 | −0.69 | 0.30 | 0.41 |
| 800 | −5.85 | −4.93 | −4.14 | −6.09 | −4.09 | −3.57 | −0.39 | 0.48 | 0.69 |
| 900 | −5.13 | −4.32 | −3.59 | −5.16 | −3.67 | −3.06 | −0.16 | 0.62 | 0.90 |
| 1000 | −4.54 | −3.83 | −3.16 | −4.42 | −3.33 | −2.66 | 0.03 | 0.73 | 0.87 |
| 1100 | −4.07 | −3.43 | −2.80 | −3.81 | −3.05 | −2.33 | 0.18 | 0.82 | 0.91 |
| 1200 | −3.67 | −3.09 | −2.50 | −3.32 | −2.82 | −2.06 | 0.31 | 0.90 | 0.95 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Samui, P.; Agarwal, R. Thermodynamic Assessment and Solubility of Ni in LBE Coolants. Thermo 2022, 2, 371-382. https://doi.org/10.3390/thermo2040025
Samui P, Agarwal R. Thermodynamic Assessment and Solubility of Ni in LBE Coolants. Thermo. 2022; 2(4):371-382. https://doi.org/10.3390/thermo2040025
Chicago/Turabian StyleSamui, Pradeep, and Renu Agarwal. 2022. "Thermodynamic Assessment and Solubility of Ni in LBE Coolants" Thermo 2, no. 4: 371-382. https://doi.org/10.3390/thermo2040025
APA StyleSamui, P., & Agarwal, R. (2022). Thermodynamic Assessment and Solubility of Ni in LBE Coolants. Thermo, 2(4), 371-382. https://doi.org/10.3390/thermo2040025





