Effects of Initial Small-Scale Material Nonlinearity on the Pre-Yield and Pre-Buckling Response of an Externally Pressurized Ring
Abstract
1. Introduction
2. Three-Dimensional Kinematic Relations for a Shell
3. Equations of Motion and the Method of Virtual Work
4. Constitutive Relations for an Orthotropic Lamina

5. Isoparametric Finite Element Discretization
- (a)
- Geometric Symmetry:
- (b)
- Loading Symmetry:
6. Results and Discussion
Example 1. Pre-Buckling and Pre-Yield Response of Relatively Thin Perfect Isotropic Rings with Small-Scale Material Nonlinearity Subjected to External Pressures
7. Summary and Conclusions
- (i)
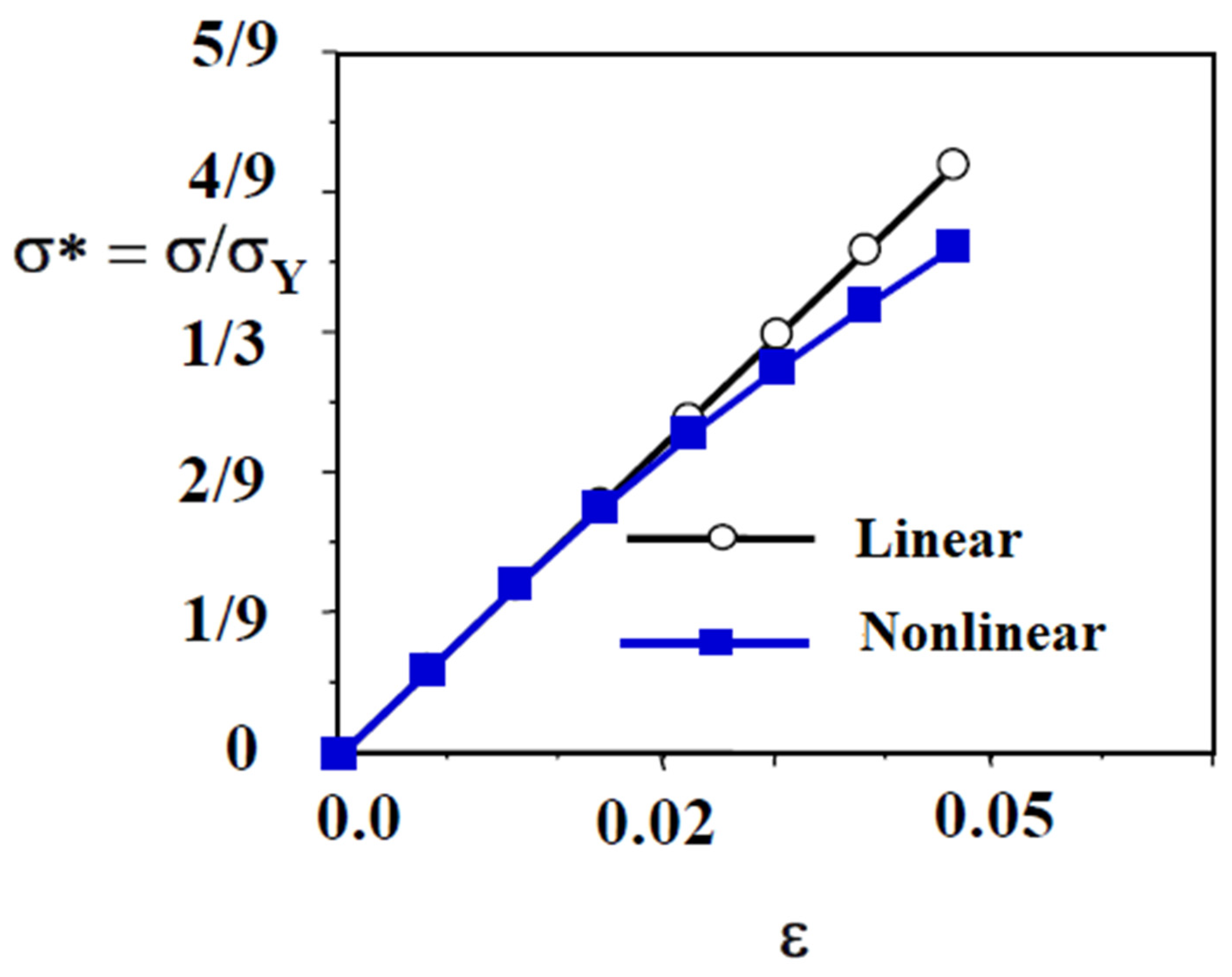
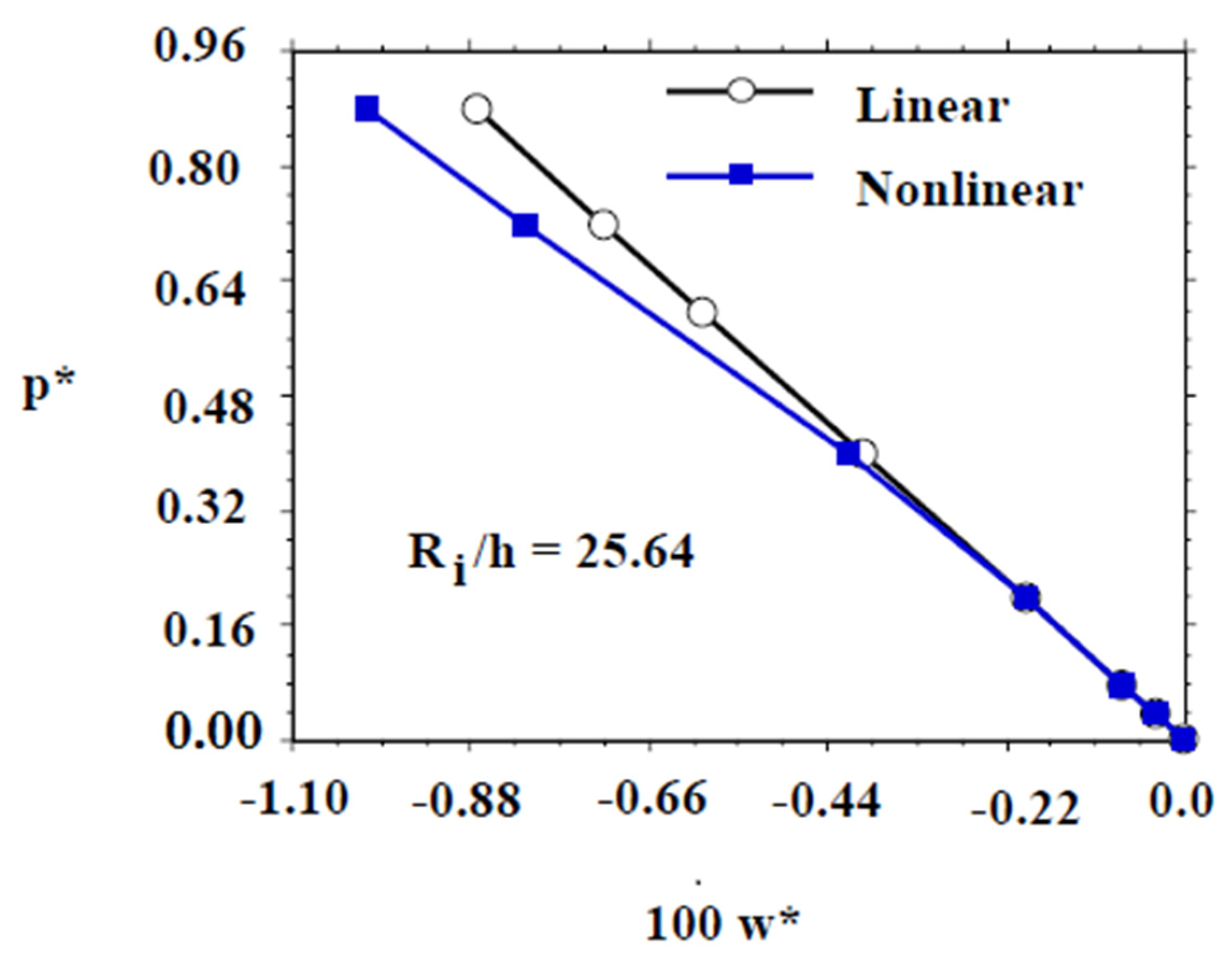
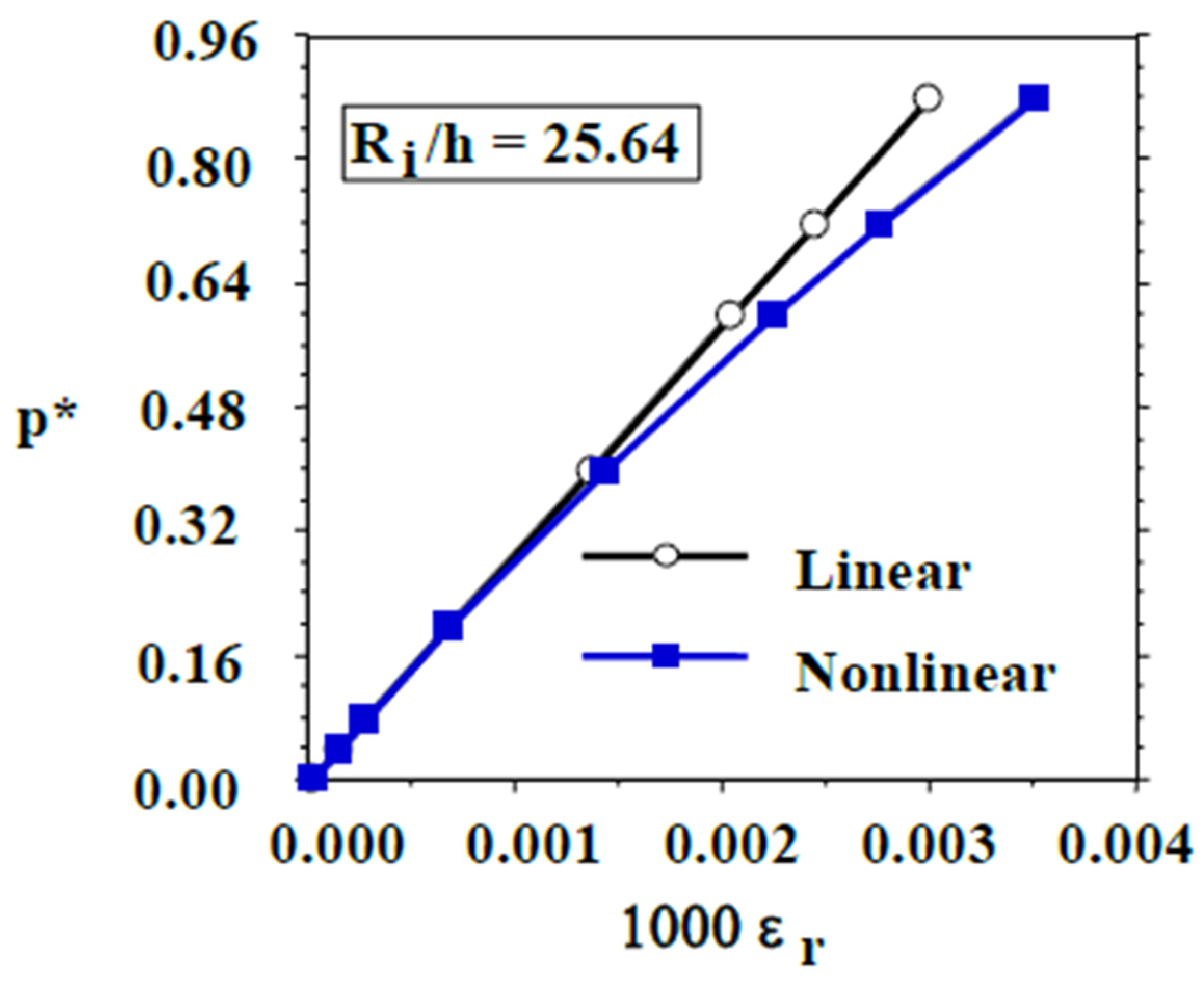
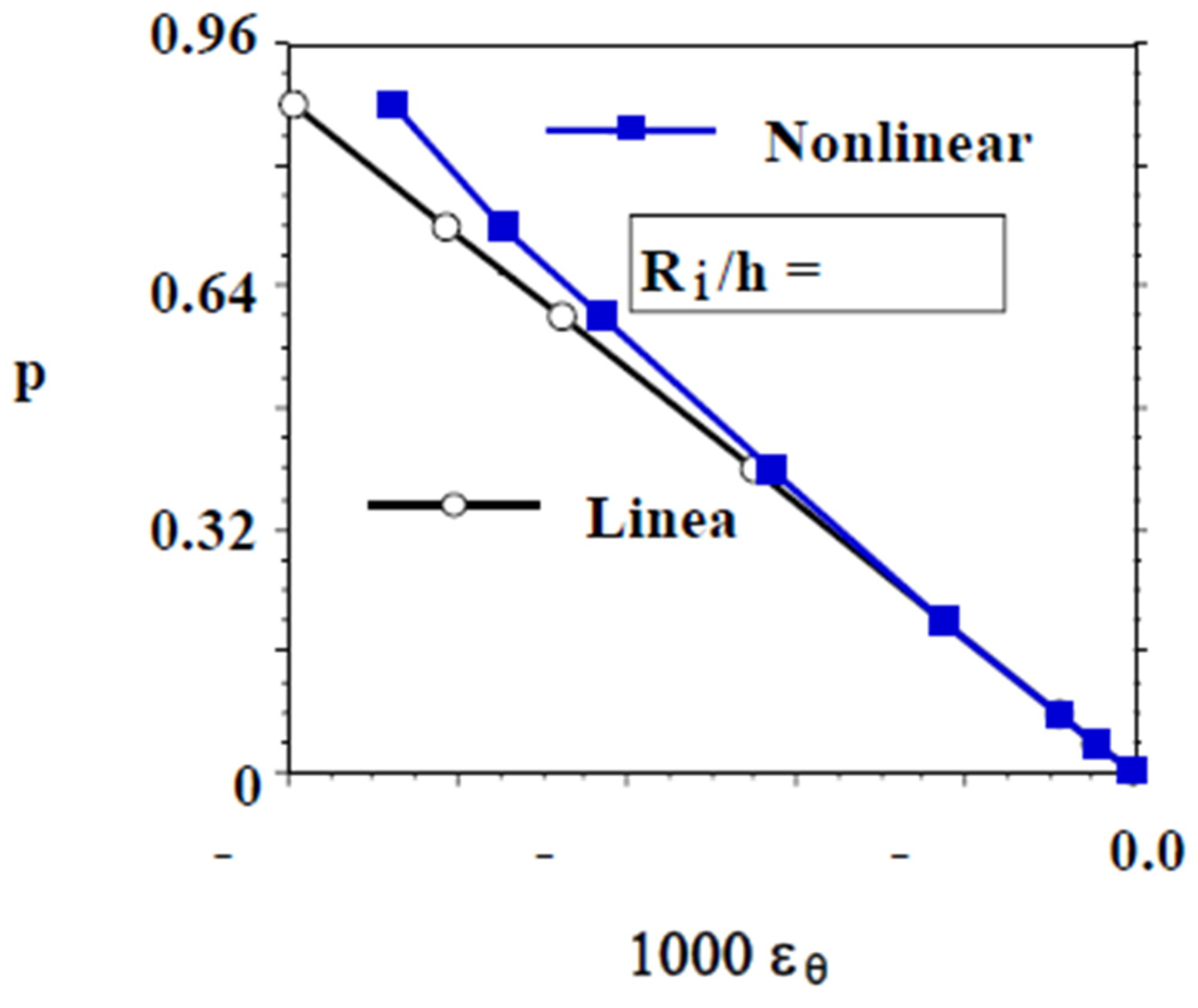
- Initial small-scale material nonlinearity has a pronounced effect on the pre-yield stress and pre buckling compressive response a perfect metallic relatively thin (Ri/h = 25.64) very long cylindrical shell (plane strain ring) under investigation.
- (ii)
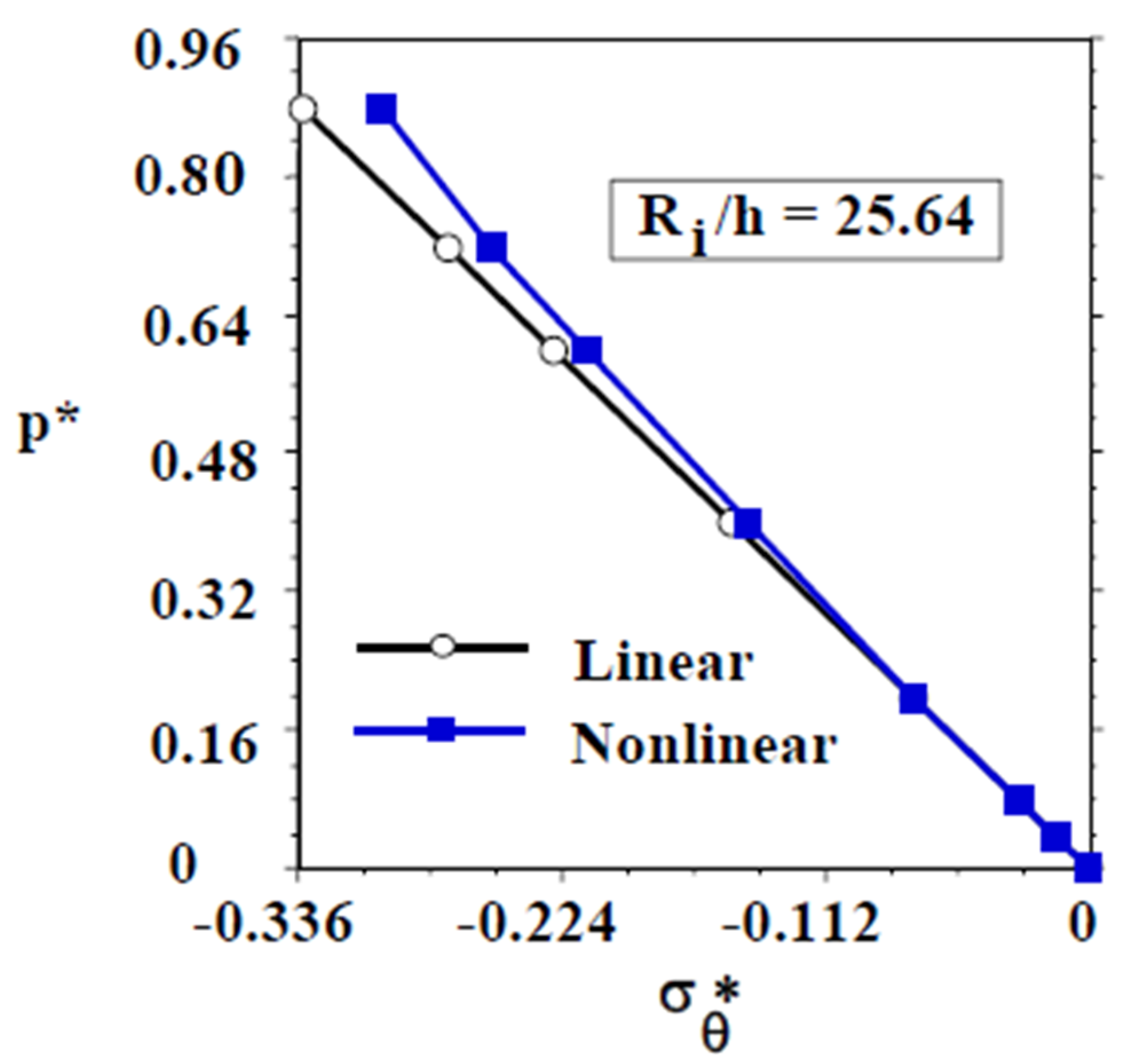
- Numerical results suggest that the pressure-deflection curve for a perfect relatively thin (Ri/h = 25.64) stainless steel 316 ring with initial small-scale material nonlinearity deviates from the corresponding linear elastic response by as much as 15% (approx.) as the buckling pressure is approached (88% of ).
- (iii)
- The pressure vs. transverse and circumferential strain curves for the perfect relatively thin (Ri/h = 25.64) stainless steel 316 ring with initial small-scale material nonlinearity deviate from their linear elastic response counterparts by as much as 16.67% and 13.64%, respectively, as the buckling pressure is approached (88% of ).
- (iv)
- The transverse normal strain values are about two orders of magnitude smaller than their circumferential strain counterparts, because of the relative thinness of the ring.
- (v)
- The pressure vs. hoop stress curve for the perfect relatively thin (Ri/h = 25.64) stainless steel 316 ring with initial small-scale material nonlinearity deviates from its linear elastic response counterpart by as much as 10.89% (approx.) as the buckling pressure is approached (88% of ).
- (vi)
- These enhanced responses for metallic rings due to initial small-scale nonlinearity are significant enough to not miss attentions from designers and operators of submersibles alike.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| Linear differential operator matrix relating the linear incremental strain components to incremental displacement components | |
| b, t | Subscript or superscript indicating the bottom and the top surface, respectively |
| Incremental elastic stiffness (material property) tensor | |
| Differential loading surface area evaluated at the first iteration of each load step when hydrostatic pressure is applied | |
| Infinitesimal control volume | |
| Linear incremental component of the 6 × 1 strain vector | |
| {fL} | Applied load vector |
| {fL} | Applied load vector at the time t + ∆t |
| {fN} | Nonlinear internal force vector |
| {fN}(i) | Nonlinear internal force vector at the ith iteration of the time step between t and t + ∆t |
| Coefficient of the first fundamental differential quadratic form of a parallel surface in the jth direction, j = 1 (x), 2 (θ), 3 (z) | |
| Coefficient of the first fundamental differential quadratic form of the bottom surface in the θ direction | |
| h | Thickness of the shell/ring |
| [KL] | Linear global stiffness matrix |
| m, n | Ratio of reference yield stress to the corresponding modulus, and strain hardening parameter, respectively, in the Ramberg-Osgood representation |
| N | Total number of elements |
| n(t) | Unit normal vector for the top surface with respect to the fixed coordinate system |
| pr, p | Applied general and uniform, respectively, hydrostatic pressure |
| pcr | Classical buckling pressure of a long cylindrical shell (plane strain ring) |
| , | Incremental elastic stiffness (material property) matrix, and its components, respectively |
| Ri | Inner radius of a long perfect cylindrical shell (plane strain ring) |
| External virtual work done on a body | |
| r | Radial coordinate of a point in an undeformed perfect ring |
| Loading surface area evaluated at the first iteration of each load step when hydrostatic pressure is applied | |
| Incremental stress component | |
| Second Piola-Kirchhoff stress tensor at time t + Δt | |
| 9 × 9 stress matrix evaluated at time t | |
| 6 × 1 stress vector evaluated at time t | |
| t | Time as an index |
| ,, | Incremental nodal displacement components at the jth node on the bottom surface in x1 (or x), x2 (or θ), and z directions, respectively |
| ,, | Incremental nodal displacement components at the jth node on the top surface in x1 (or x), x2 (or θ), and z directions, respectively |
| x, θ, z | Coordinates of a point |
| Total Green-Lagrangian strain tensor evaluated at time t + ∆t | |
| , | Force and energy convergence criteria, respectively |
| [Φ] | Quadratic global interpolation function matrix |
| Quadratic element interpolation function in terms of r’ and s’ |
Appendix A. Definition of Certain Matrix Operators
References
- Casey, S. The Abyss: The Titan Submersible Disaster Was Years in the Making, New Details Reveal. Vanit, 17 August 2023. Available online: https://www.vanityfair.com/news/2023/08/titan-submersible-implosion-warnings (accessed on 29 January 2024).
- Couch, W.P.; Ward, G.D.; Blumenberg, W.F. Investigation of Filament-Reinforced Plastic Deep Submergence Pressure Hulls July 1966 to March 1969; Department of the Navy, Naval Ship Research & Development Center: Washington, DC, USA, 1969. [Google Scholar]
- Garala, H.J. Structural Evaluation of 8-Inch Diameter Graphite-Epoxy Composite Cylinders Subjected to External Hydrostatic Compressive Loading; DTRC Report 89/016; David Taylor Research Center: Bethesda, MD, USA, 1989. [Google Scholar]
- Garala, H.J.; Chaudhuri, R.A. Structural evaluation of advanced composite thick-section cylinders under bi-axial compressive loading. In Mechanics of Thick Composites; Rajapakse, Y., Ed.; ASME AMD: New York, NY, USA, 1993; Volume 162, pp. 227–236. [Google Scholar]
- Hahn, H.T.; Sohi, M.M. Buckling of a fiber bundle embedded in epoxy. Compos. Sci. Technol. 1986, 27, 25–41. [Google Scholar] [CrossRef]
- Abdallah, M.G.; Gascoigne, H.E.; Cairnes, D.S.; Patton, K.B. Measurement of deformation in thick composite rings subjected to external pressure. In Proceedings of the SEM’s Spring Conference on Experimental Mechanics and Manufacturer’s Exhibit, Albuquerque, NM, USA, 4–6 June 1990. [Google Scholar]
- Chaudhuri, R.A. Prediction of the compressive strength of thick-section advanced composite laminates. J. Compos. Mater. 1991, 25, 1244–1276. [Google Scholar] [CrossRef]
- Chaudhuri, R.A.; Garala, H.J. Analytical/experimental evaluation of hybrid commingled carbon/glass/epoxy thick-section composites under compression. J. Compos. Mater. 1995, 29, 1695–1718. [Google Scholar] [CrossRef]
- Hunt, G.W.; Lucena Neto, E. Localized buckling in long axially-loaded cylindrical shells. J. Mech. Phys. Solids 1991, 39, 881–894. [Google Scholar] [CrossRef]
- Chaudhuri, R.A. A nonlinear zigzag theory for finite element analysis of highly shear-deformable laminated anisotropic shells. Compos. Struct. 2008, 85, 350–359. [Google Scholar] [CrossRef]
- Chaudhuri, R.A.; Kim, D. On propagation of shear crippling (kinkband) instability in a long imperfect laminated composite cylindrical shell under external pressure. Int. J. Solids Struct. 1997, 34, 3455–3486. [Google Scholar] [CrossRef]
- Chaudhuri, R.A.; Kim, D. Localization and shear-crippling (kinkband) instability in a thick imperfect laminated composite ring under hydrostatic pressure. Int. J. Solids Struct. 2003, 40, 7063–7092. [Google Scholar] [CrossRef]
- Kim, D.; Chaudhuri, R.A. Effect of lamination sequence on the localization and shear crippling instability in thick imperfect cross-ply rings under external pressure. Compos. Struct. 2007, 80, 504–513. [Google Scholar] [CrossRef]
- Kim, D.; Chaudhuri, R.A. Influence of localized imperfection on the instability of isotropic/cross-ply cylindrical shells/rings under external pressure. Compos. Struct. 2005, 67, 57–70. [Google Scholar] [CrossRef]
- Chaudhuri, R.A. Effects of thickness and fibre misalignment on compression fracture in cross-ply (very) long cylindrical shells under external pressure. Proc. R. Soc. A Lond. 2015, 471, 20150147. [Google Scholar] [CrossRef]
- Chaudhuri, R.A. Effects of fiber misalignment and transverse shear modulus on localization and shear crippling instability in thick imperfect cross-ply rings under external pressure. Compos. Struct. 2008, 82, 587–599. [Google Scholar] [CrossRef]
- Chaudhuri, R.A.; Kim, D. Influence of localized imperfection and surface-parallel shear modulus nonlinearity on the instability of a thin cross-ply cylindrical shell under external pressure. Compos. Struct. 2008, 82, 235–244. [Google Scholar] [CrossRef]
- Chaudhuri, R.A.; Kim, D. Sensitivity of the post-localization response of a thick cross-ply imperfect ring to transverse Young’s modulus nonlinearity. Compos. Struct. 2008, 84, 44–55. [Google Scholar] [CrossRef]
- Chaudhuri, R.A.; Kim, D. Effects of thickness and transverse shear modulus nonlinearity on the post-“yield” and post-localization response of an externally pressurized imperfect cross-ply ring. Compos. Struct. 2009, 88, 83–96. [Google Scholar] [CrossRef]
- Chaudhuri, R.A. Stress intensity factor and energy release rate of externally pressurized thick cross-ply (very) long cylindrical shells with low-hardening transverse shear modulus nonlinearity. Eng. Fract. Mech. 2016, 151, 138–160. [Google Scholar] [CrossRef]
- Chaudhuri, R.A. Localization, delocalization and compression fracture in externally pressurized thick cross-ply (very) long cylindrical shells with material non-linearity: A multi-scale and multi-physics analysis. Int. J. Non-Linear Mech. 2016, 84, 68–81. [Google Scholar] [CrossRef]
- Chaudhuri, R.A. Comparison of stress singularities of kinked carbon and glass fibres weakening compressed unidirectional composites: A three-dimensional trimaterial junction stress singularity analysis. Philos. Mag. 2014, 94L, 625–667. [Google Scholar] [CrossRef]
- Chaudhuri, R.A. A micro-kink theory for determination of shear modulus of a unidirectional composite lamina. Compos. Struct. 2010, 92, 395–400. [Google Scholar] [CrossRef]
- Chaudhuri, R.A.; Xie, M.S.; Garala, H.J. Stress singularity due to kink band weakening a unidirectional composite under compression. J. Compos. Mater. 1996, 30, 672–691. [Google Scholar] [CrossRef]
- Moran, P.M.; Shih, C.F. Kink band propagation and broadening in ductile matrix fiber composites, experiments and analysis. Int. J. Solids Struct. 1998, 35, 1709–1722. [Google Scholar] [CrossRef]
- Gutkin, R.; Pinho, S.T.; Robinson, P.; Curtis, P.T. On the transition from shear-driven fibre compressive failure to fibre kinking in notched CFRP laminates under longitudinal compression. Compos. Sci. Tech. 2010, 70, 1223–1231. [Google Scholar] [CrossRef]
- Timoshenko, S.P.; Gere, J.M. Theory of Elastic Stability, 2nd ed.; McGraw-Hill: New York, NY, USA, 1961. [Google Scholar]
- Simitses, G.J. Buckling and post-buckling of imperfect cylindrical shells: A review. Appl. Mech. Rev. 1986, 39, 1517–1524. [Google Scholar] [CrossRef]
- Jones, R.M.; Morgan, H.S. Buckling and vibration of cross-ply laminated circular cylindrical shells. AIAA J. 1975, 13, 664–671. [Google Scholar] [CrossRef]
- Bradford, M.A.; Uy, B.; Pi, Y.L. In-plane elastic stability of arches under a central load. ASCE J. Eng. Mech. 2002, 128, 710–719. [Google Scholar] [CrossRef]
- Fu, L.; Waas, A.M. Initial postbuckling behavior of thick rings under uniform external hydrostatic pressure. ASME J. Appl. Mech. 1995, 62, 338–345. [Google Scholar] [CrossRef]
- Wu, B.; Yu, Y.; Li, Z. Analytical approximations to large post-buckling deformation of elastic rings under uniform hydrostatic pressure. Int. J. Mech. Sci. 2007, 49, 661–668. [Google Scholar] [CrossRef]
- Djondjorov, P.A.; Vassilev, V.M.; Mladenov, I.M. Analytic description and explicit parametrization of the equilibrium shapes of elastic rings and tubes under uniform hydrostatic pressure. Int. J. Mech. Sci. 2011, 53, 355–364. [Google Scholar] [CrossRef]
- Kim, D.; Chaudhuri, R.A. Effect of thickness on buckling of perfect cross-ply rings under external pressure. Compos. Struct. 2007, 81, 525–532. [Google Scholar] [CrossRef]
- Kim, D.; Chaudhuri, R.A. Postbuckling of moderately thick imperfect rings under external pressure. ASCE J. Eng. Mech. 2006, 132, 1273–1276. [Google Scholar] [CrossRef]
- Kerdegarbakhsh, M.; Kiani, Y.; Esfahani, S.E.; Eslami, M.R. Postbuckling of FGM rings. Int. J. Solids Struct. 2014, 85, 187–195. [Google Scholar] [CrossRef]
- Asemi, K.; Kiani, Y. Postbuckling up to collapse of polar orthotropic linearly elastic rings subjected to external pressure. Int. J. Struct. Stab. Dyn. 2016, 16, 1450091. [Google Scholar] [CrossRef]
- Kim, D.; Chaudhuri, R.A. Postbuckling behavior of symmetrically laminated thin shallow circular arches. Compos. Struct. 2009, 87, 101–108. [Google Scholar] [CrossRef]
- Chaudhuri, R.A. A nonlinear resonance (eigenvalue) approach for computation of elastic collapse pressures of harmonically imperfect relatively thin rings. Thin-Walled Struct. 2018, 127, 344–353. [Google Scholar] [CrossRef]
- Chaudhuri, R.A.; Kim, D.; Pavliga, J.R. A nonlinear resonance (eigenvalue) approach for computing elastic collapse pressure of a moderately thick cross-ply imperfect ring. Compos. Struct. 2008, 82, 117–126. [Google Scholar] [CrossRef]
- Chaudhuri, R.A.; Kim, D.; Pavliga, J.R. Effects of thickness and modal imperfection amplitude on elastic collapse pressure of a cross-ply imperfect ring. Compos. Struct. 2008, 86, 370–384. [Google Scholar] [CrossRef]
- Hsia, R.L.; Chaudhuri, R.A. Geometrically nonlinear analysis of cylindrical shells using surface-parallel quadratic elements. Comput. Struct. 1996, 61, 1143–1154. [Google Scholar] [CrossRef]
- Kim, D.; Chaudhuri, R.A. Full and von Karman geometrically nonlinear analyses of laminated cylindrical panels. AIAA J. 1995, 33, 2173–2181. [Google Scholar] [CrossRef]
- Chaudhuri, R.A.; Hsia, R.L. Effect of thickness on large-deflection behavior of shells. AIAA J. 1999, 37, 403–405. [Google Scholar] [CrossRef]
- Chaudhuri, R.A.; Hsia, R.L. Effect of thickness on the large elastic deformation behavior of laminated shells. Compos. Struct. 1999, 44, 117–128. [Google Scholar] [CrossRef]
- Chaudhuri, R.A. Localization, delocalization, and compression fracture in moderately thick transversely isotropic bilinear rings under external pressure. ASME J. Eng. Mater. Technol. 2006, 128, 603–610. [Google Scholar] [CrossRef]
- Kim, D.; Chaudhuri, R.A. Localized buckling of a bilinear elastic ring under external pressure. ASCE J. Eng. Mech. 2005, 131, 221–224. [Google Scholar] [CrossRef]
- Chaudhuri, S.N.; Chaudhuri, R.A.; Benner, R.E.; Penugonda, M. Raman spectroscopy for characterization of interfacial debonds between carbon fibers and polymer matrices. Compos. Struct. 2006, 76, 375–387. [Google Scholar] [CrossRef]
- Bathe, K.J. Finite Element Procedures in Engineering Analysis; Prentice-Hall: Englewood Cliffs, NJ, USA, 1982. [Google Scholar]
- Chaudhuri, R.A. A degenerate triangular shell element with constant cross-sectional warping. Comput. Struct. 1988, 28, 315–325. [Google Scholar] [CrossRef]
- Seide, P.; Chaudhuri, R.A. Triangular finite element for analysis of thick laminated shells. Int. J. Numer. Methods Eng. 1987, 24, 1563–1579. [Google Scholar] [CrossRef]
- Chaudhuri, R.A. A new three-dimensional shell theory in general (non-lines-of-curvature) coordinates for analysis of curved panels weakened by through/part-through holes. Compos. Struct. 2009, 89, 321–332. [Google Scholar] [CrossRef]
- Chaudhuri, R.A.; Seide, P. An approximate method for prediction of transverse shear stresses in a laminated shell. Int. J. Solids Struct. 1987, 23, 1145–1161. [Google Scholar] [CrossRef]
- Chaudhuri, R.A. On the prediction of interlaminar shear stresses in a thick laminated general shell. Int. J. Solids Struct. 1990, 26, 499–510. [Google Scholar] [CrossRef]
- Ramberg, W.; Osgood, W.R. Description of Stress Strain Curves by Three Parameters; NACA-TN 902; National Advisory Committee for Aeronautics: Washington, DC, USA, 1934. [Google Scholar]
- Chaudhuri, R.A.; Abu-Arja, K.R. Plastic deformation of a boron/aluminum tube under multi-axial loadings. Comput. Struct. 1986, 24, 915–921. [Google Scholar] [CrossRef]
- Bathe, K.J.; Cimento, A.P. Some practical procedures for the solution of nonlinear finite element equations. J. Comput. Meth. Appl. Mech. Eng. 1980, 22, 59–85. [Google Scholar] [CrossRef]
- Amazigo, J.C.; Frazer, W.B. Buckling under external pressure of cylindrical shells with dimple shaped initial imperfections. Int. J. Solids Struct. 1971, 7, 883–900. [Google Scholar] [CrossRef]
- Arrayago, I.; Real, E.; Gardner, L. Description of stress–strain curves for stainless steel alloys. Mater. Design 2015, 87, 540–552. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chaudhuri, R.A.; Kim, D. Effects of Initial Small-Scale Material Nonlinearity on the Pre-Yield and Pre-Buckling Response of an Externally Pressurized Ring. Eng 2024, 5, 733-749. https://doi.org/10.3390/eng5020040
Chaudhuri RA, Kim D. Effects of Initial Small-Scale Material Nonlinearity on the Pre-Yield and Pre-Buckling Response of an Externally Pressurized Ring. Eng. 2024; 5(2):733-749. https://doi.org/10.3390/eng5020040
Chicago/Turabian StyleChaudhuri, Reaz A., and Deokjoo Kim. 2024. "Effects of Initial Small-Scale Material Nonlinearity on the Pre-Yield and Pre-Buckling Response of an Externally Pressurized Ring" Eng 5, no. 2: 733-749. https://doi.org/10.3390/eng5020040
APA StyleChaudhuri, R. A., & Kim, D. (2024). Effects of Initial Small-Scale Material Nonlinearity on the Pre-Yield and Pre-Buckling Response of an Externally Pressurized Ring. Eng, 5(2), 733-749. https://doi.org/10.3390/eng5020040




