Rheological Behavior of Cement Paste: A Phenomenological State of the Art
Abstract
1. Introduction
2. Testing Procedures
2.1. Flow Tests
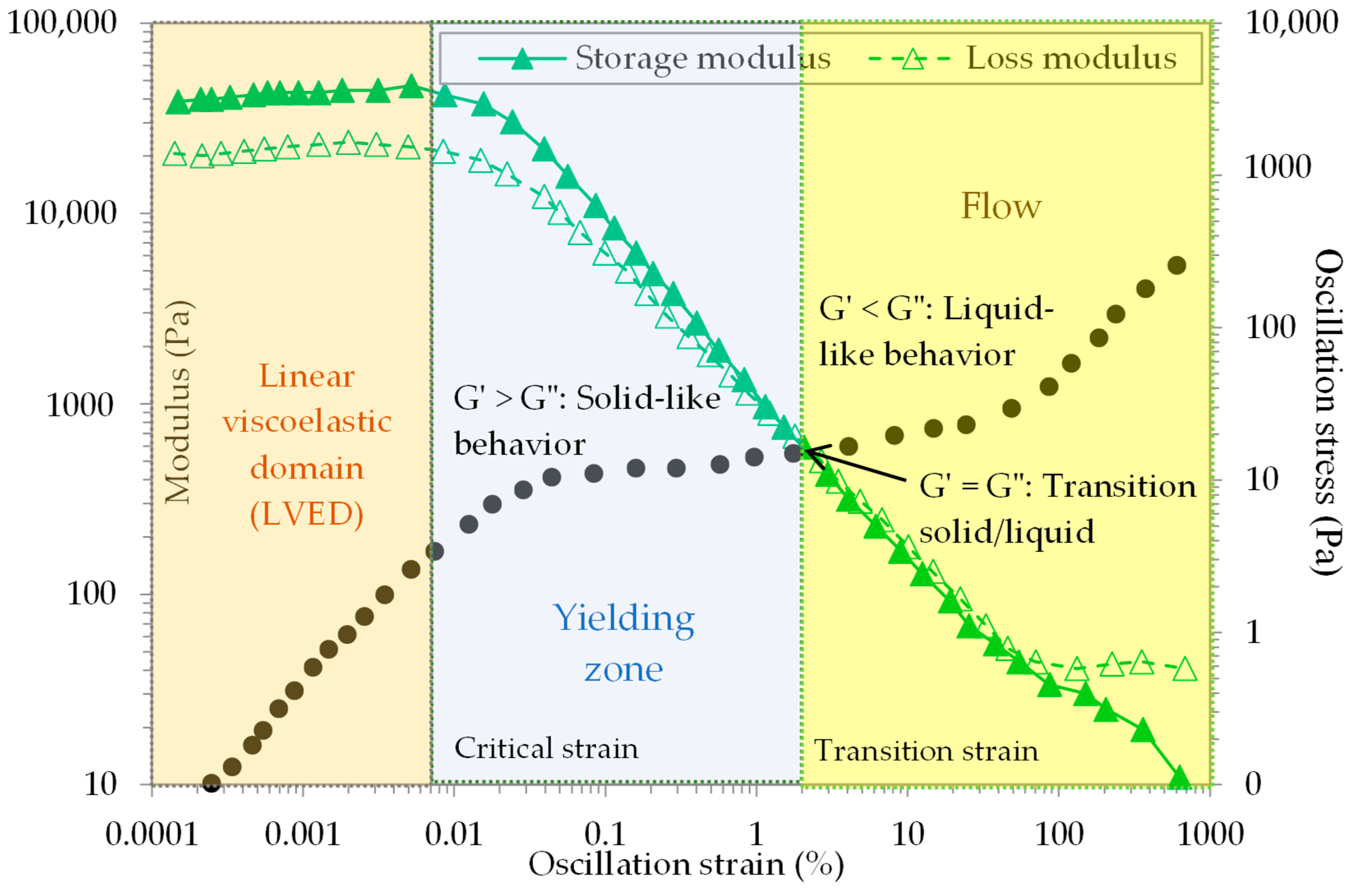
2.2. Oscillation Metods
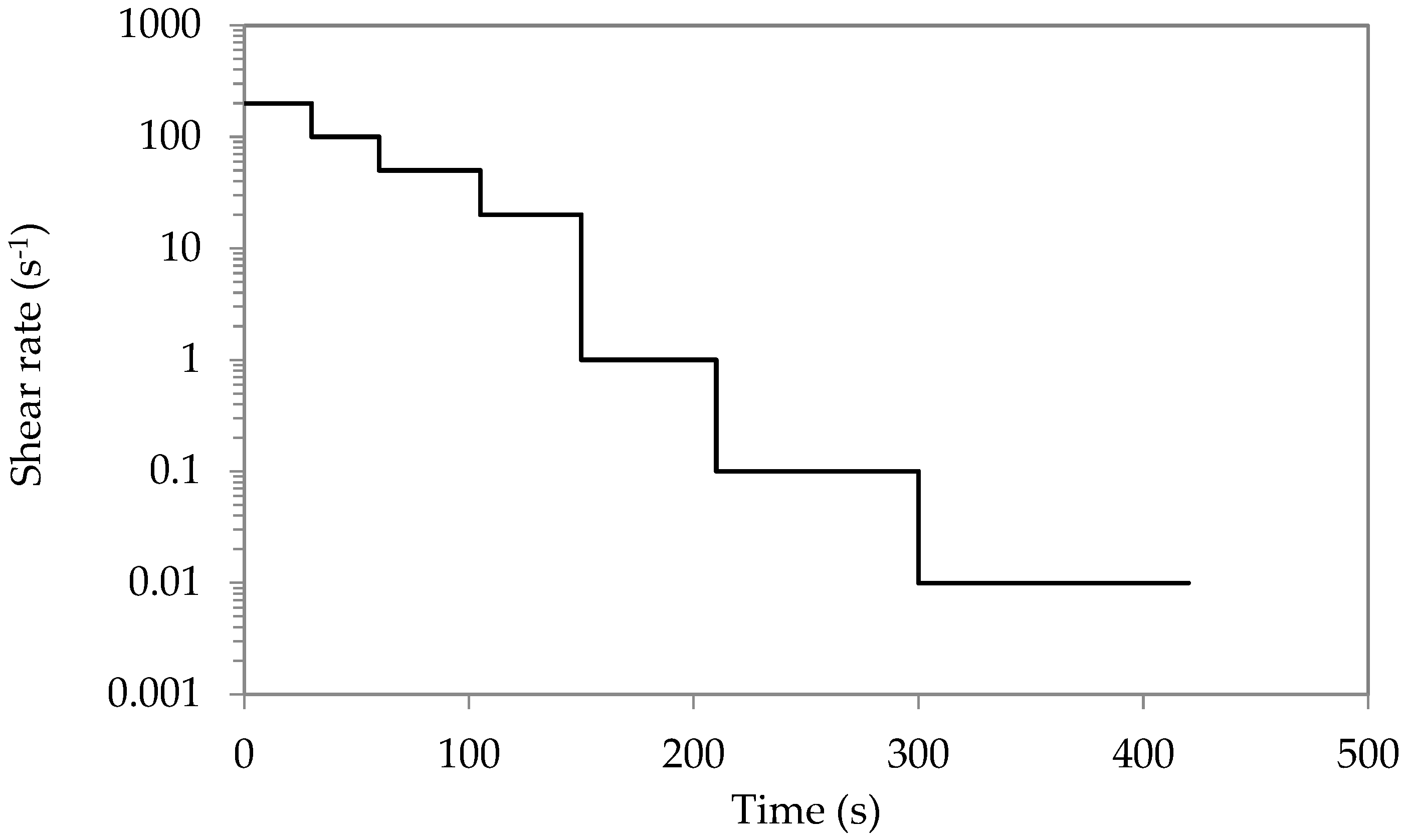
2.3. Transient Mode
3. Rheological Behavior of Cement Paste
3.1. Flow Behavior
3.2. Linear Visco-Elastic Domain
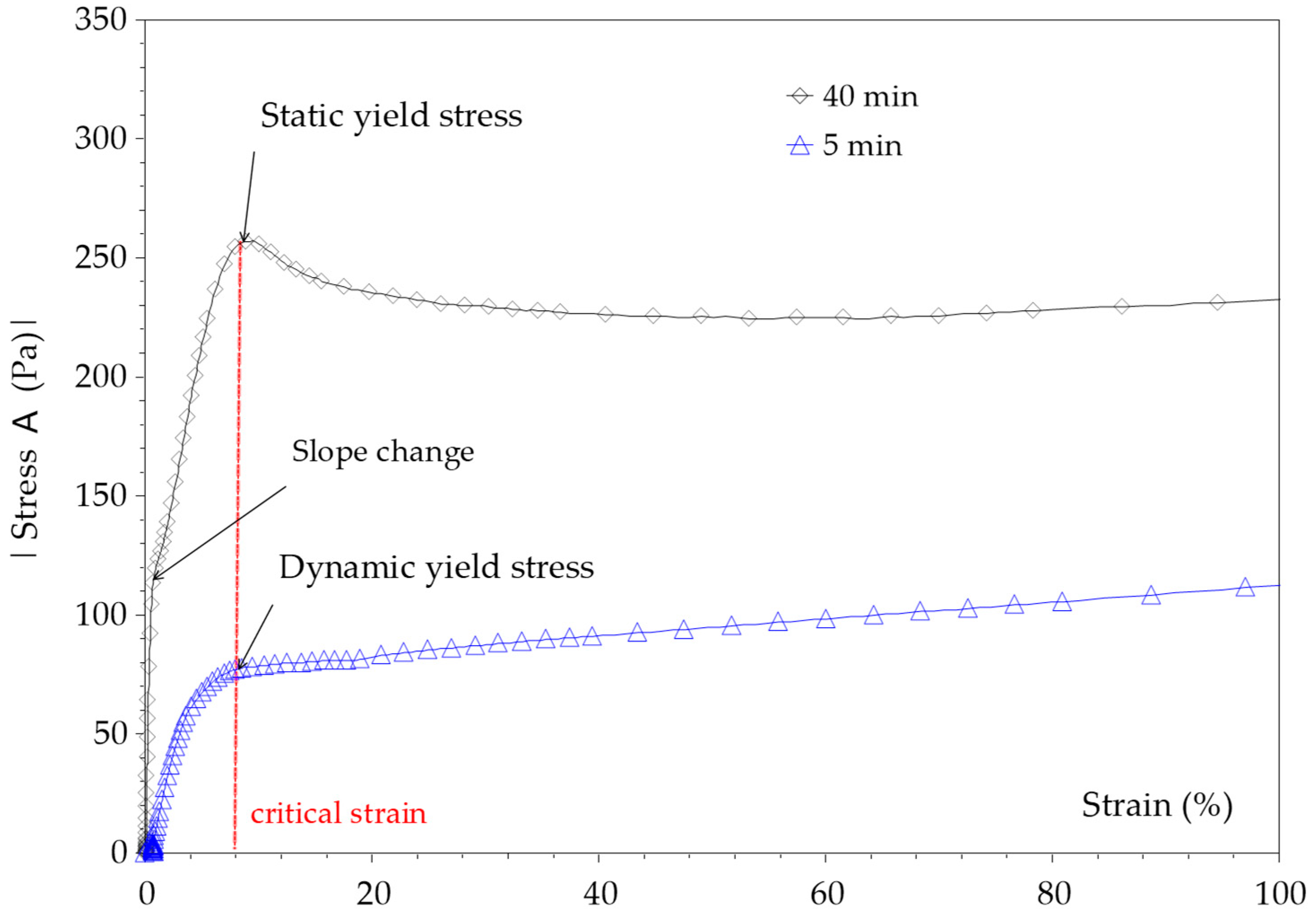
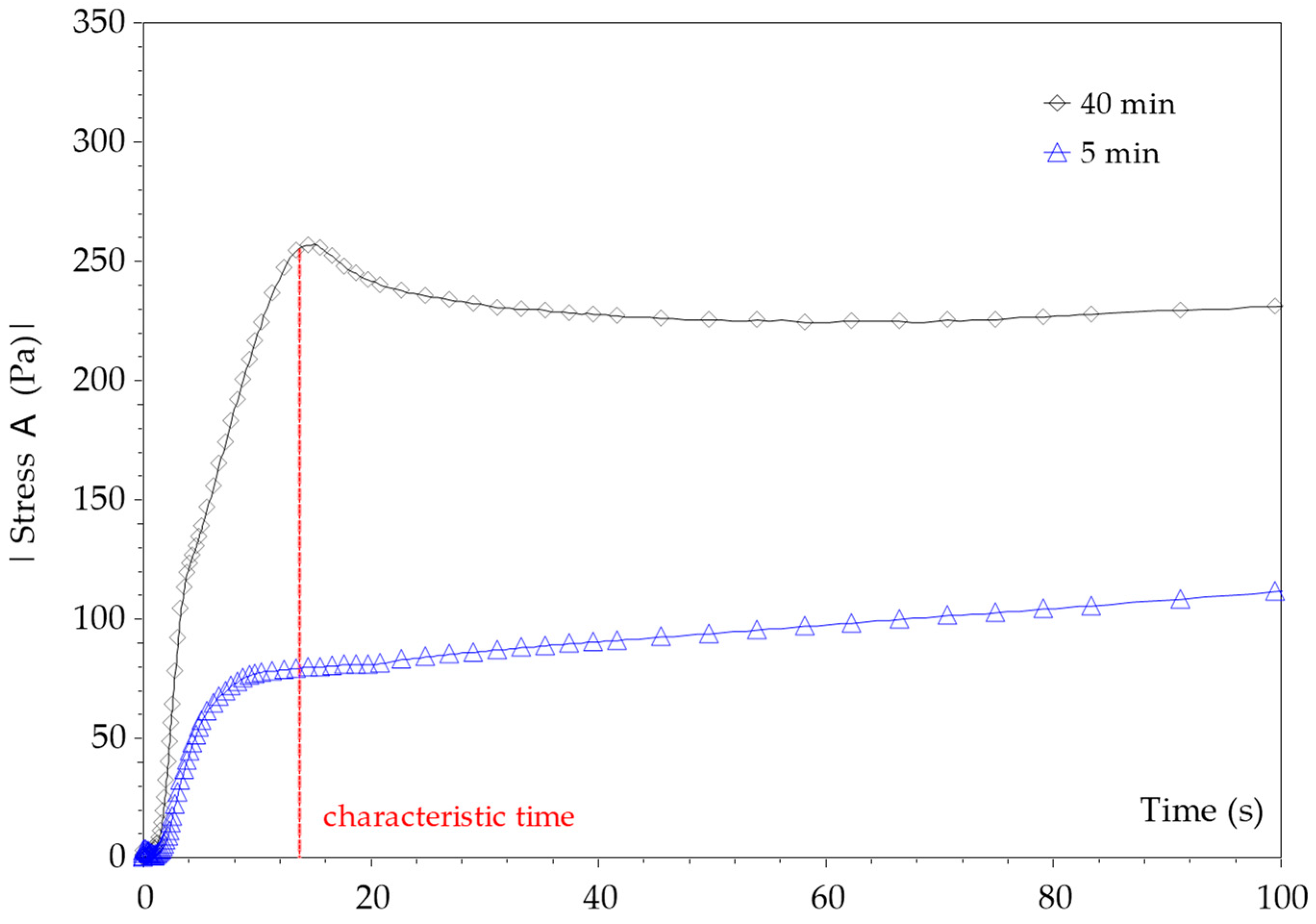
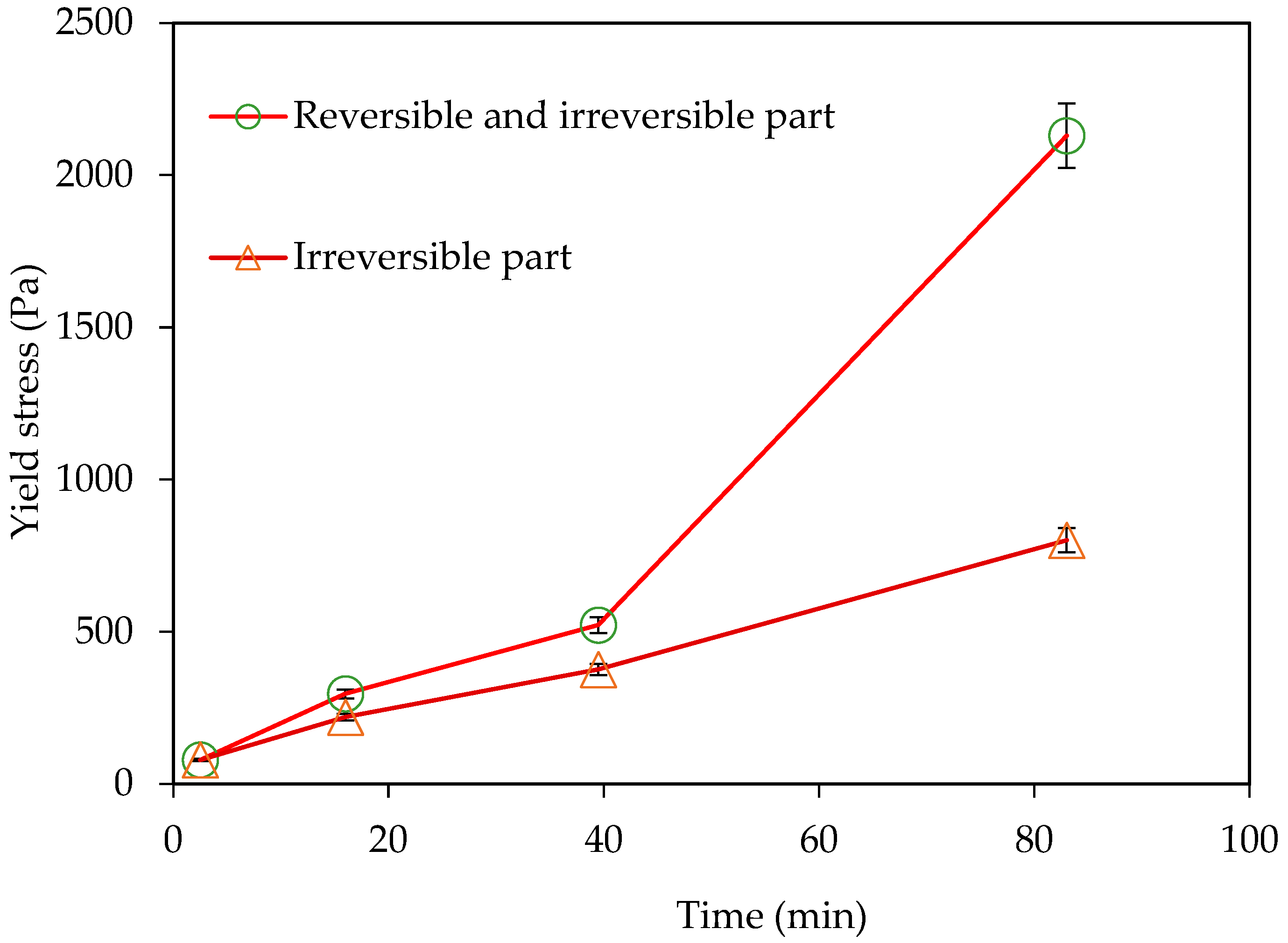
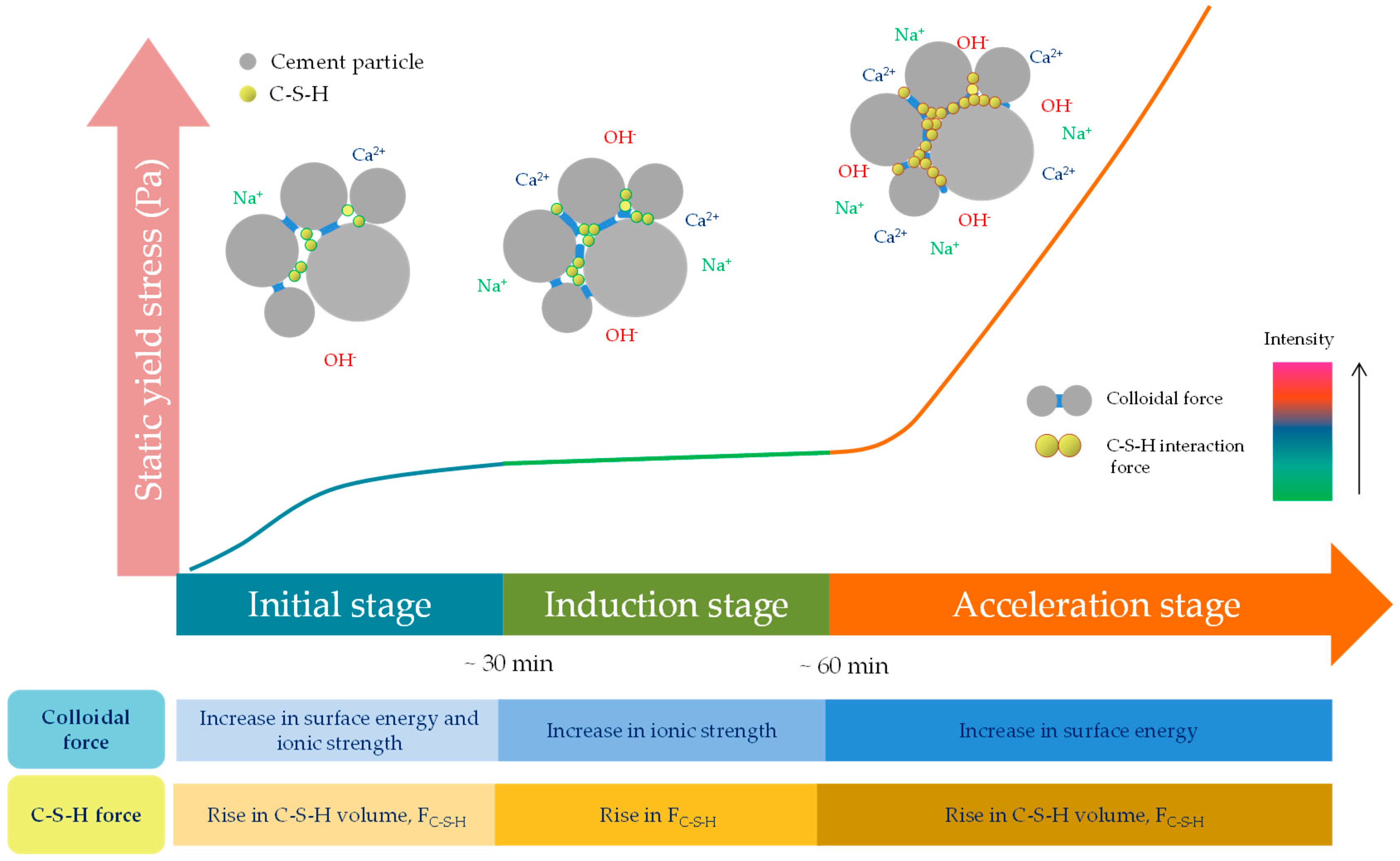
3.3. Structural Build-Up
4. Conclusions
Funding
Conflicts of Interest
References
- Nicolas, R.; Richard, B.; Nicolas, D.; Irina, I.; Temitope, K.J.; Dirk, L.; Viktor, M.; Romain, M.; Arnaud, P.; Ursula, P.; et al. Assessing the fresh properties of printable cement-based materials: High potential tests for quality control. Cem. Concr. Res. 2022, 158, 106836. [Google Scholar] [CrossRef]
- Biricik, Ö.; Mardani, A. Parameters affecting thixotropic behavior of self compacting concrete and 3D printable concrete; a state-of-the-art review. Constr. Build. Mater. 2022, 339, 127688. [Google Scholar] [CrossRef]
- Roussel, N. Rheological requirements for printable concretes. Cem. Concr. Res. 2018, 112, 76–85. [Google Scholar] [CrossRef]
- Li, R.; Lei, L.; Plank, J. Influence of PCE superplasticizers on the fresh properties of low carbon cements containing calcined clays: A comparative study of calcined clays from three different sources. Cem. Concr. Compos. 2023, 139, 105072. [Google Scholar] [CrossRef]
- Ayati, B.; Newport, D.; Wong, H.; Cheeseman, C. Low-carbon cements: Potential for low-grade calcined clays to form supplementary cementitious materials. Clean. Mater. 2022, 5, 100099. [Google Scholar] [CrossRef]
- Gaimster, R.; Dixon, N. Self-compacting concrete. In Advanced Concrete Technology; Japan Concrete Institute: Tokyo, Japan, 2003; pp. 1–23. [Google Scholar]
- Brouwers, H.J.H.; Radix, H.J. Self-compacting concrete: Theoretical and experimental study. Cem. Concr. Res. 2005, 35, 2116–2136. [Google Scholar] [CrossRef]
- Wu, H.; Hu, R.; Yang, D.; Ma, Z. Micro-macro characterizations of mortar containing construction waste fines as replacement of cement and sand: A comparative study. Constr. Build. Mater. 2023, 383, 131328. [Google Scholar] [CrossRef]
- Duan, Z.; Deng, Q.; Liang, C.; Ma, Z.; Wu, H. Upcycling of recycled plastic fiber for sustainable cementitious composites: A critical review and new perspective. Cem. Concr. Compos. 2023, 142, 105192. [Google Scholar] [CrossRef]
- Roussel, N.; Bessaies-Bey, H.; Kawashima, S.; Marchon, D.; Vasilic, K.; Wolfs, R. Recent advances on yield stress and elasticity of fresh cement-based materials. Cem. Concr. Res. 2019, 124, 105798. [Google Scholar] [CrossRef]
- Roussel, N.; Lemaître, A.; Flatt, R.J.; Coussot, P. Steady state flow of cement suspensions: A micromechanical state of the art. Cem. Concr. Res. 2010, 40, 77–84. [Google Scholar] [CrossRef]
- Hsu, K.C.; Chiu, J.J.; Da Chen, S.; Tseng, Y.C. Effect of addition time of a superplasticizer on cement adsorption and on concrete workability. Cem. Concr. Compos. 1999, 21, 425–430. [Google Scholar] [CrossRef]
- Chiocchio, G.; Paolini, A.E. Optimum time for adding superplasticizer to Portland cement pastes. Cem. Concr. Res. 1985, 15, 901–908. [Google Scholar] [CrossRef]
- Houst, Y.F.; Flatt, R.J.; Bowen, P.; Hofmann, H.; Mäder, U.; Widmer, J.; Sulser, U.; Bürge, T.A. Influence of Superplasticizer Adsorption on the Rheology of Cement Paste. In Proceedings of the International RILEM Conference on “The Role of Admixtures in High Performance Concrete”, Monterrey, Mexico, 21–26 March 1999; pp. 387–402. [Google Scholar]
- Cyr, M.; Legrand, C.; Mouret, M. Study of the shear thickening effect of superplasticizers on the rheological behaviour of cement pastes containing or not mineral additives. Cem. Concr. Res. 2000, 30, 1477–1483. [Google Scholar] [CrossRef]
- Khayat, K.H.; Yahia, A.; Sayed, M. Effect of supplementary cementitious materials on rheological properties, bleeding, and strength of structural grout. ACI Mater. J. 2008, 105, 585–593. [Google Scholar]
- Jiao, D.; De Schutter, G. Insights into the viscoelastic properties of cement paste based on SAOS technique. Constr. Build. Mater. 2022, 357, 129320. [Google Scholar] [CrossRef]
- Legrand, C. Contribution à l’étude de la rhéologie du béton frais. Matér. Constr. 1972, 5, 379–393. [Google Scholar] [CrossRef]
- Yuan, Q.; Zhou, D.; Khayat, K.H.; Feys, D.; Shi, C. On the measurement of evolution of structural build-up of cement paste with time by static yield stress test vs. small amplitude oscillatory shear test. Cem. Concr. Res. 2017, 99, 183–189. [Google Scholar] [CrossRef]
- El Bitouri, Y.; Azéma, N. On the “Thixotropic” Behavior of Fresh Cement Pastes. Eng 2022, 3, 677–692. [Google Scholar] [CrossRef]
- Mostafa, A.M.; Yahia, A. New approach to assess build-up of cement-based suspensions. Cem. Concr. Res. 2016, 85, 174–182. [Google Scholar] [CrossRef]
- Zhang, K.; Mezhov, A.; Schmidt, W. Chemical and thixotropic contribution to the structural build-up of cementitious materials. Constr. Build. Mater. 2022, 345, 128307. [Google Scholar] [CrossRef]
- Roussel, N.; Ovarlez, G.; Garrault, S.; Brumaud, C. The origins of thixotropy of fresh cement pastes. Cem. Concr. Res. 2012, 42, 148–157. [Google Scholar] [CrossRef]
- Ewoldt, R.H.; Johnston, M.T.; Caretta, L.M. Experimental Challenges of Shear Rheology: How to Avoid Bad Data BT—Complex Fluids in Biological Systems: Experiment, Theory, and Computation; Spagnolie, S.E., Ed.; Springer: New York, NY, USA, 2015; pp. 207–241. ISBN 978-1-4939-2065-5. [Google Scholar]
- Ukrainczyk, N.; Thiedeitz, M.; Kränkel, T.; Koenders, E.; Gehlen, C. Modeling SAOS Yield Stress of Cement Suspensions: Microstructure-Based Computational Approach. Materials 2020, 13, 2769. [Google Scholar] [CrossRef] [PubMed]
- Conte, T.; Chaouche, M. Rheological behavior of cement pastes under Large Amplitude Oscillatory Shear. Cem. Concr. Res. 2016, 89, 332–344. [Google Scholar] [CrossRef]
- Schultz, M.A.; Struble, L.J. Use of oscillatory shear to study flow behavior of fresh cement paste. Cem. Concr. Res. 1993, 23, 273–282. [Google Scholar] [CrossRef]
- Kawashima, S.; Chaouche, M.; Corr, D.J.; Shah, S.P. Rate of thixotropic rebuilding of cement pastes modified with highly purified attapulgite clays. Cem. Concr. Res. 2013, 53, 112–118. [Google Scholar] [CrossRef]
- Nachbaur, L.; Mutin, J.C.; Nonat, A.; Choplin, L. Dynamic mode rheology of cement and tricalcium silicate pastes from mixing to setting. Cem. Concr. Res. 2001, 31, 183–192. [Google Scholar] [CrossRef]
- Kolawole, J.T.; Combrinck, R.; Boshoff, W.P. Rheo-viscoelastic behaviour of fresh cement-based materials: Cement paste, mortar and concrete. Constr. Build. Mater. 2020, 248, 118667. [Google Scholar] [CrossRef]
- Thiedeitz, M.; Kränkel, T.; Gehlen, C. Viscoelastoplastic classification of cementitious suspensions: Transient and non-linear flow analysis in rotational and oscillatory shear flows. Rheol. Acta 2022, 61, 549–570. [Google Scholar] [CrossRef]
- Lei, W.-G.; Struble, L.J. Microstructure and Flow Behavior of Fresh Cement Paste. J. Am. Ceram. Soc. 1997, 80, 2021–2028. [Google Scholar] [CrossRef]
- Mahaut, F.; Mokéddem, S.; Chateau, X.; Roussel, N.; Ovarlez, G. Effect of coarse particle volume fraction on the yield stress and thixotropy of cementitious materials. Cem. Concr. Res. 2008, 38, 1276–1285. [Google Scholar] [CrossRef]
- Perrot, A.; Lecompte, T.; Khelifi, H.; Brumaud, C.; Hot, J.; Roussel, N. Yield stress and bleeding of fresh cement pastes. Cem. Concr. Res. 2012, 42, 937–944. [Google Scholar] [CrossRef]
- Roussel, N. Steady and transient flow behaviour of fresh cement pastes. Cem. Concr. Res. 2005, 35, 1656–1664. [Google Scholar] [CrossRef]
- El Bitouri, Y.; Azéma, N. Contribution of turbidimetry on the characterization of cement pastes bleeding. Adv. Cem. Res. 2023, 35, 180–190. [Google Scholar] [CrossRef]
- Aït-Kadi, A.; Marchal, P.; Choplin, L.; Chrissemant, A.S.; Bousmina, M. Quantitative analysis of mixer-type rheometers using the couette analogy. Can. J. Chem. Eng. 2002, 80, 1166–1174. [Google Scholar] [CrossRef]
- Fourmentin, M.; Ovarlez, G.; Faure, P.; Peter, U.; Lesueur, D.; Daviller, D.; Coussot, P. Rheology of lime paste—A comparison with cement paste. Rheol. Acta 2015, 54, 647–656. [Google Scholar] [CrossRef]
- Papo, A.; Piani, L. Flow Behavior of Fresh Portland Cement Pastes. Part. Sci. Technol. 2004, 22, 201–212. [Google Scholar] [CrossRef]
- Ivanov, Y.P.; Roshavelov, T.T. Flow behaviour of modified cement pastes. Cem. Concr. Res. 1993, 23, 803–810. [Google Scholar] [CrossRef]
- Colombo, A.; Geiker, M.R.; Justnes, H.; Lauten, R.A.; De Weerdt, K. On the effect of calcium lignosulfonate on the rheology and setting time of cement paste. Cem. Concr. Res. 2017, 100, 435–444. [Google Scholar] [CrossRef]
- Plank, J.; Sakai, E.; Miao, C.W.; Yu, C.; Hong, J.X. Chemical admixtures—Chemistry, applications and their impact on concrete microstructure and durability. Cem. Concr. Res. 2015, 78, 81–99. [Google Scholar] [CrossRef]
- Barneoud-Chapelier, A.; Le Saout, G.; Azéma, N.; El Bitouri, Y. Effect of polycarboxylate superplasticizer on hydration and properties of belite ye’elimite ferrite cement paste. Constr. Build. Mater. 2022, 322, 126483. [Google Scholar] [CrossRef]
- Otsubo, Y.; Miyai, S.; Umeya, K. Time-dependent flow of cement pastes. Cem. Concr. Res. 1980, 10, 631–638. [Google Scholar] [CrossRef]
- Emoto, T.; Bier, T.A. Rheological behavior as influenced by plasticizers and hydration kinetics. Cem. Concr. Res. 2007, 37, 647–654. [Google Scholar] [CrossRef]
- Krieger, I.M.; Dougherty, T.J. A Mechanism for Non-Newtonian Flow in Suspensions of Rigid Spheres. Trans. Soc. Rheol. 1959, 3, 137–152. [Google Scholar] [CrossRef]
- Mahaut, F.; Chateau, X.; Coussot, P.; Ovarlez, G. Yield stress and elastic modulus of suspensions of noncolloidal particles in yield stress fluids. J. Rheol. 2008, 52, 287–313. [Google Scholar] [CrossRef]
- Flatt, R.J.; Bowen, P. Yodel: A yield stress model for suspensions. J. Am. Ceram. Soc. 2006, 89, 1244–1256. [Google Scholar] [CrossRef]
- Odler, I.; Duckstein, U.; Becker, T. On the combined effect of water solubles lignosulfonates and carbonates on portland cement and clinker pastes 1. Physical properties. Cem. Concr. Res. 1978, 8, 469–479. [Google Scholar] [CrossRef]
- Roy, D.M.; Asaga, K. Rheological properties of cement mixes: III. The effects of mixing procedures on viscometric properties of mixes containing superplasticizers. Cem. Concr. Res. 1979, 9, 731–739. [Google Scholar] [CrossRef]
- Brady, J.F.; Bossis, G. The rheology of concentrated suspensions of spheres in simple shear flow by numerical simulation. J. Fluid Mech. 1985, 155, 105–129. [Google Scholar] [CrossRef]
- Hoffman, R.L. Explanations for the cause of shear thickening in concentrated colloidal suspensions. J. Rheol. 1998, 42, 111–123. [Google Scholar] [CrossRef]
- Ovarlez, G.; Bertrand, F.; Rodts, S. Local determination of the constitutive law of a dense suspension of noncolloidal particles through magnetic resonance imaging. J. Rheol. 2006, 50, 259–292. [Google Scholar] [CrossRef]
- Barnes, H.A. Shear-Thickening (“Dilatancy”) in Suspensions of Nonaggregating Solid Particles Dispersed in Newtonian Liquids. J. Rheol. 1989, 33, 329–366. [Google Scholar] [CrossRef]
- Jiao, D.; Lesage, K.; Yardimci, M.Y.; El Cheikh, K.; Shi, C.; De Schutter, G. Rheological behavior of cement paste with nano-Fe3O4 under magnetic field: Magneto-rheological responses and conceptual calculations. Cem. Concr. Compos. 2021, 120, 104035. [Google Scholar] [CrossRef]
- Jiao, D.; Shi, C.; De Schutter, G. Magneto-responsive structural build-up of highly flowable cementitious paste in the presence of PCE superplasticizer. Constr. Build. Mater. 2022, 327, 126925. [Google Scholar] [CrossRef]
- Mikanovic, N.; Jolicoeur, C. Influence of superplasticizers on the rheology and stability of limestone and cement pastes. Cem. Concr. Res. 2008, 38, 907–919. [Google Scholar] [CrossRef]
- Tramaux, A.; Azéma, N.; El Bitouri, Y.; David, G.; Negrell, C.; Poulesquen, A.; Haas, J.; Remond, S. Synthesis of phosphonated comb-like copolymers and evaluation of their dispersion efficiency on CaCO3 suspensions part II: Effect of macromolecular structure and ionic strength. Powder Technol. 2018, 334, 163–172. [Google Scholar] [CrossRef]
- Liberto, T.; Le Merrer, M.; Barentin, C.; Bellotto, M.; Colombani, J. Elasticity and yielding of calcite paste: Scaling laws in a dense colloidal suspension. Soft Matter 2017, 13, C6SM02607A. [Google Scholar] [CrossRef] [PubMed]
- Sujata, K.; Jennings, H.M. Formation of a Protective Layer During the Hydration of Cement. J. Am. Ceram. Soc. 1992, 75, 1669–1673. [Google Scholar] [CrossRef]
- Gauffinet, S.; Finot, É.; Lesniewska, E.; Nonat, A. Direct observation of the growth of calcium silicate hydrate on alite and silica surfaces by atomic force microscopy; [Observation directe de la croissance d’hydrosilicate de calcium sur des surfaces d’alite et de silice par microscopie a force atomique]. C. R. l’Acad. Sci. Ser. IIa Sci. Terre Planet. 1998, 327, 231–236. [Google Scholar]
- Zingg, A.; Holzer, L.; Kaech, A.; Winnefeld, F.; Pakusch, J.; Becker, S.; Gauckler, L. The microstructure of dispersed and non-dispersed fresh cement pastes—New insight by cryo-microscopy. Cem. Concr. Res. 2008, 38, 522–529. [Google Scholar] [CrossRef]
- Barnes, H.A. Thixotropy—A review. J. Nonnewton. Fluid Mech. 1997, 70, 1–33. [Google Scholar] [CrossRef]
- Lapasin, R.; Longo, V.; Rajgelj, S. Thixotropic behaviour of cement pastes. Cem. Concr. Res. 1979, 9, 309–318. [Google Scholar] [CrossRef]
- Roussel, N. A thixotropy model for fresh fluid concretes: Theory, validation and applications. Cem. Concr. Res. 2006, 36, 1797–1806. [Google Scholar] [CrossRef]
- Petkova, V.; Samichkov, V. Some influences on the thixotropy of composite slag Portland cement suspensions with secondary industrial waste. Constr. Build. Mater. 2007, 21, 1520–1527. [Google Scholar] [CrossRef]
- Billberg, P. Form Pressure Generated by Self-Compacting Concrete: Influence of Thixotropy and Structural Behaviour at Rest. Ph.D. Thesis, KTH Royal Institute of Technology, Stockholm, Sweden, 2006. [Google Scholar]
- Zhang, Z.; Jia, Z.; Shi, J.; Jiang, Y.; Banthia, N.; Zhang, Y. Clarifying and quantifying the driving force for the evolution of static yield stress of cement pastes. Cem. Concr. Res. 2023, 167, 107129. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
El Bitouri, Y. Rheological Behavior of Cement Paste: A Phenomenological State of the Art. Eng 2023, 4, 1891-1904. https://doi.org/10.3390/eng4030107
El Bitouri Y. Rheological Behavior of Cement Paste: A Phenomenological State of the Art. Eng. 2023; 4(3):1891-1904. https://doi.org/10.3390/eng4030107
Chicago/Turabian StyleEl Bitouri, Youssef. 2023. "Rheological Behavior of Cement Paste: A Phenomenological State of the Art" Eng 4, no. 3: 1891-1904. https://doi.org/10.3390/eng4030107
APA StyleEl Bitouri, Y. (2023). Rheological Behavior of Cement Paste: A Phenomenological State of the Art. Eng, 4(3), 1891-1904. https://doi.org/10.3390/eng4030107





